Multi-Objective Optimization Design of Cycloid-Pin Gears Based on RV Reducer Precision Transmission Performance

Abstract

This paper aims to realize multi-objective optimization of cycloid-pin gears to improve the positioning accuracy and load-carrying capacity of the rotary vector (RV) reducer, via the consideration of backlash, transmission error, and torsional stiffness. Initially, the analytical models of the RV transmission backlash and transmission error are developed by using both purely geometrical and equivalent model methods individually. Based on the generalized Hooke’s law, a torsion angle model is established to characterize the torsional stiffness of the system, utilizing methods such as Hertzian contact theory and bearing stiffness models. Subsequently, employing the Monte Carlo method, extremum method, and quality loss function, mapping objective functions for dimensional accuracy (tolerance) and transmission performance (backlash, transmission error, and torsional stiffness) are constructed. The geometry dimensions, dimensional accuracy, and modification of the cycloid-pin gear are considered as design variables to create a multi-objective optimization model. The improved Parallel Adaptive Genetic Algorithm using Deferential Evolution (PAGA-DE) is used for multi-objective solutions. Through example calculations, the impact of cycloid-pin gear parameters on transmission performance before and after optimization is determined. The reliability of backlash after optimization within 1.5′ reaches 99.99%, showing an increase of 8.24%. The reliability of transmission error within 1′ reaches 98.52%, demonstrating an increase of 1.35%. The torsional angle is reduced by 8.9% before optimization. The results indicate that the proposed multi-objective optimization design method for cycloid-pin gears can achieve the goal of improving the transmission performance of the RV reducer.

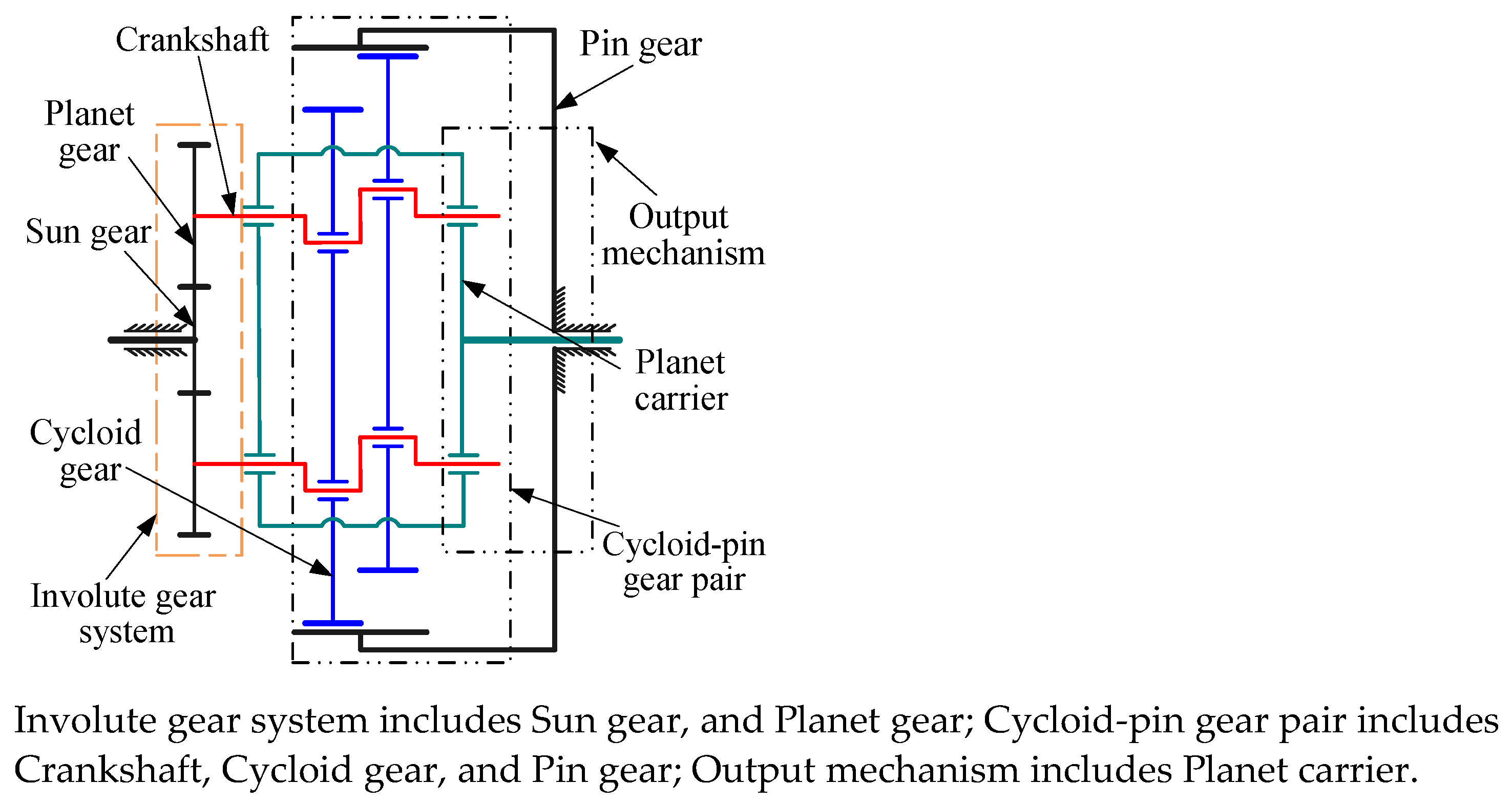
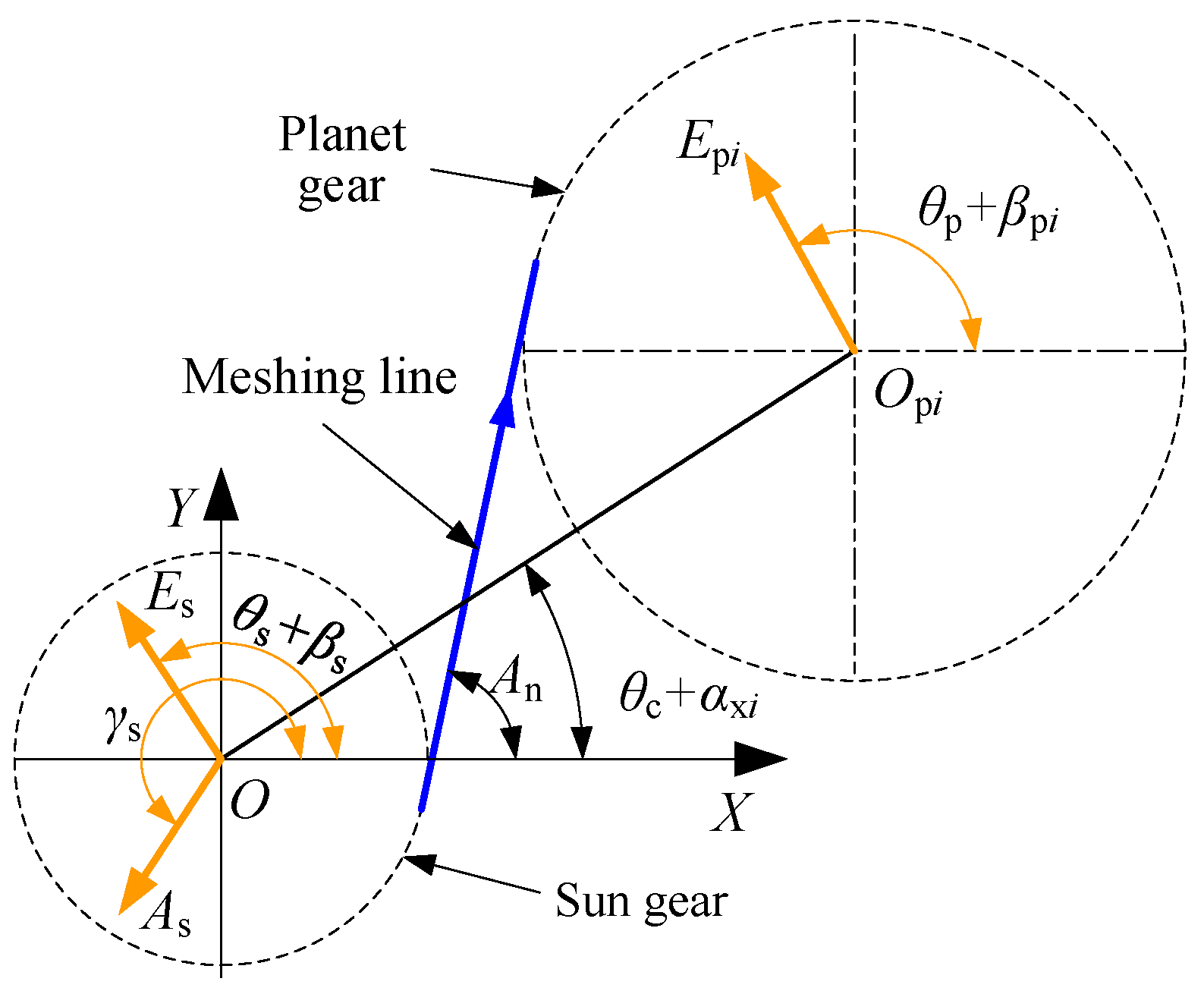
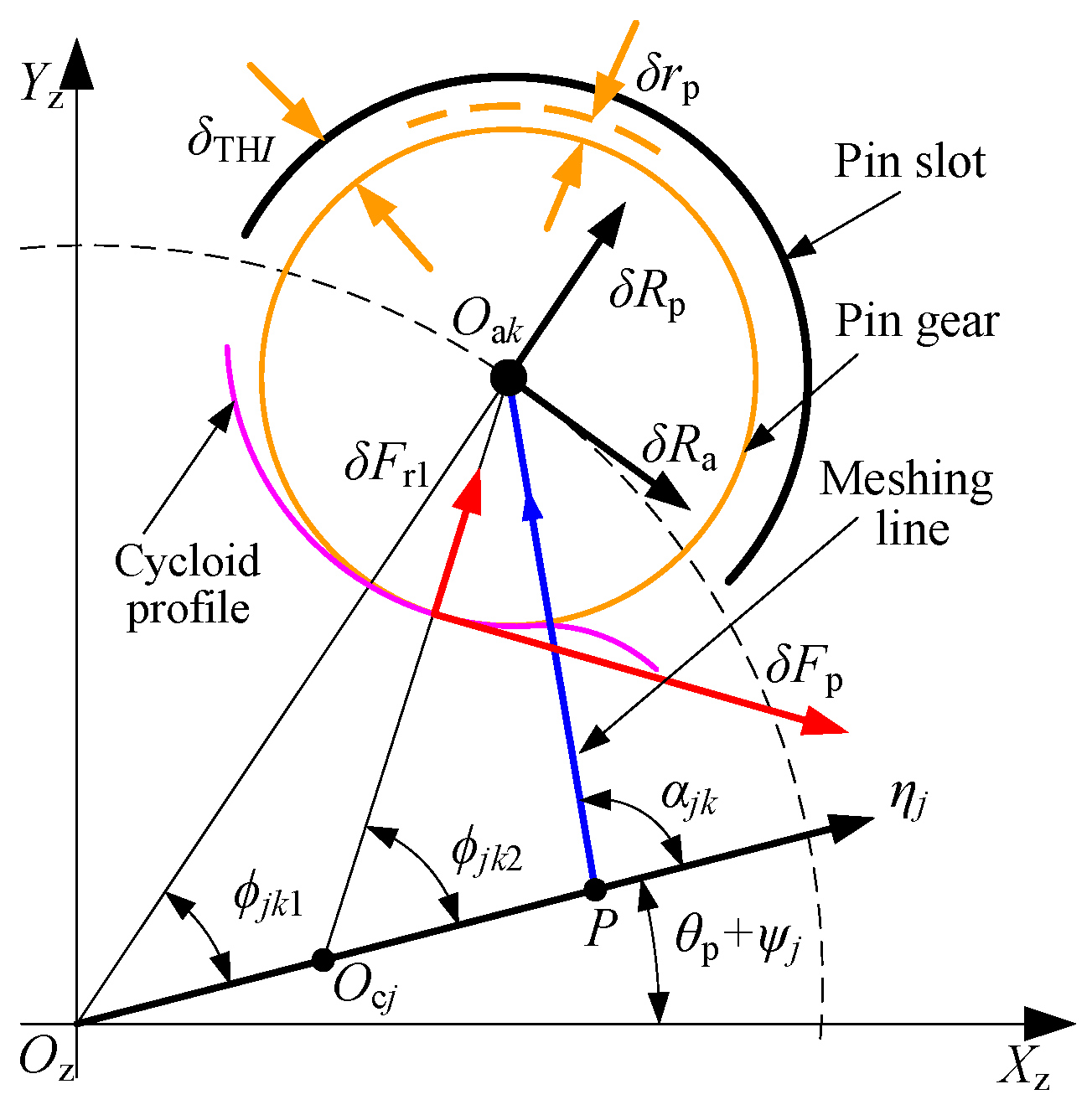
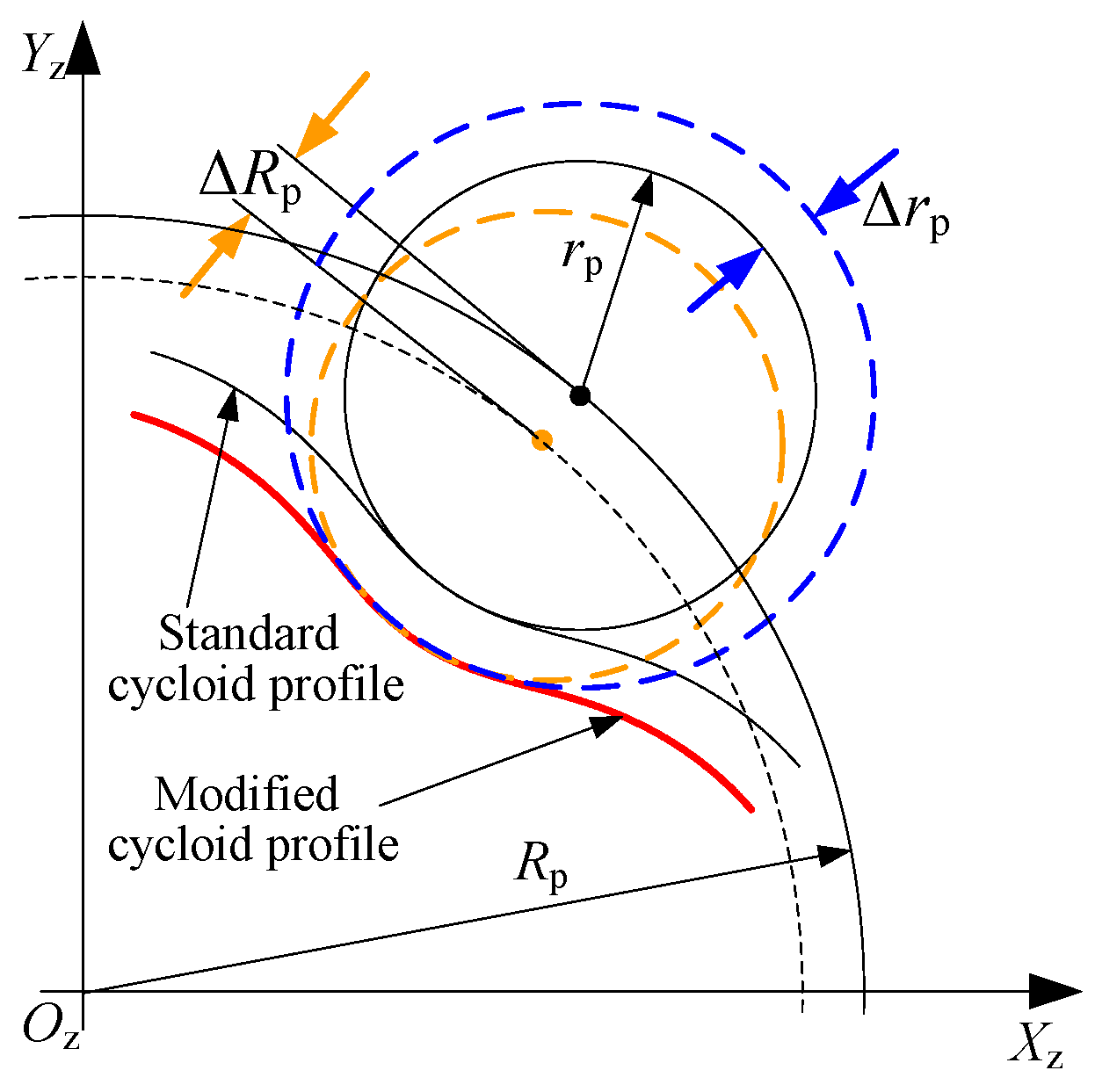
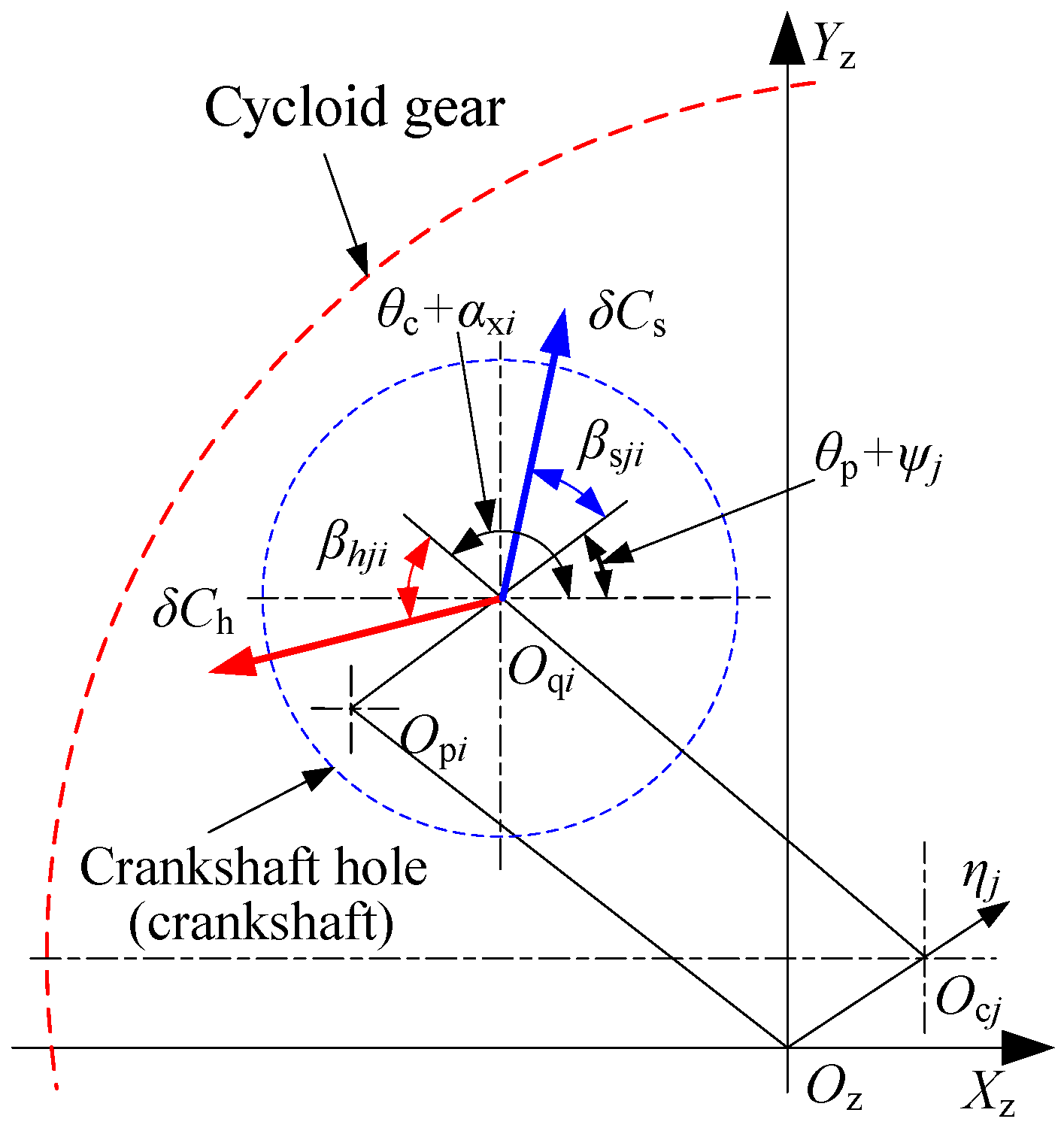
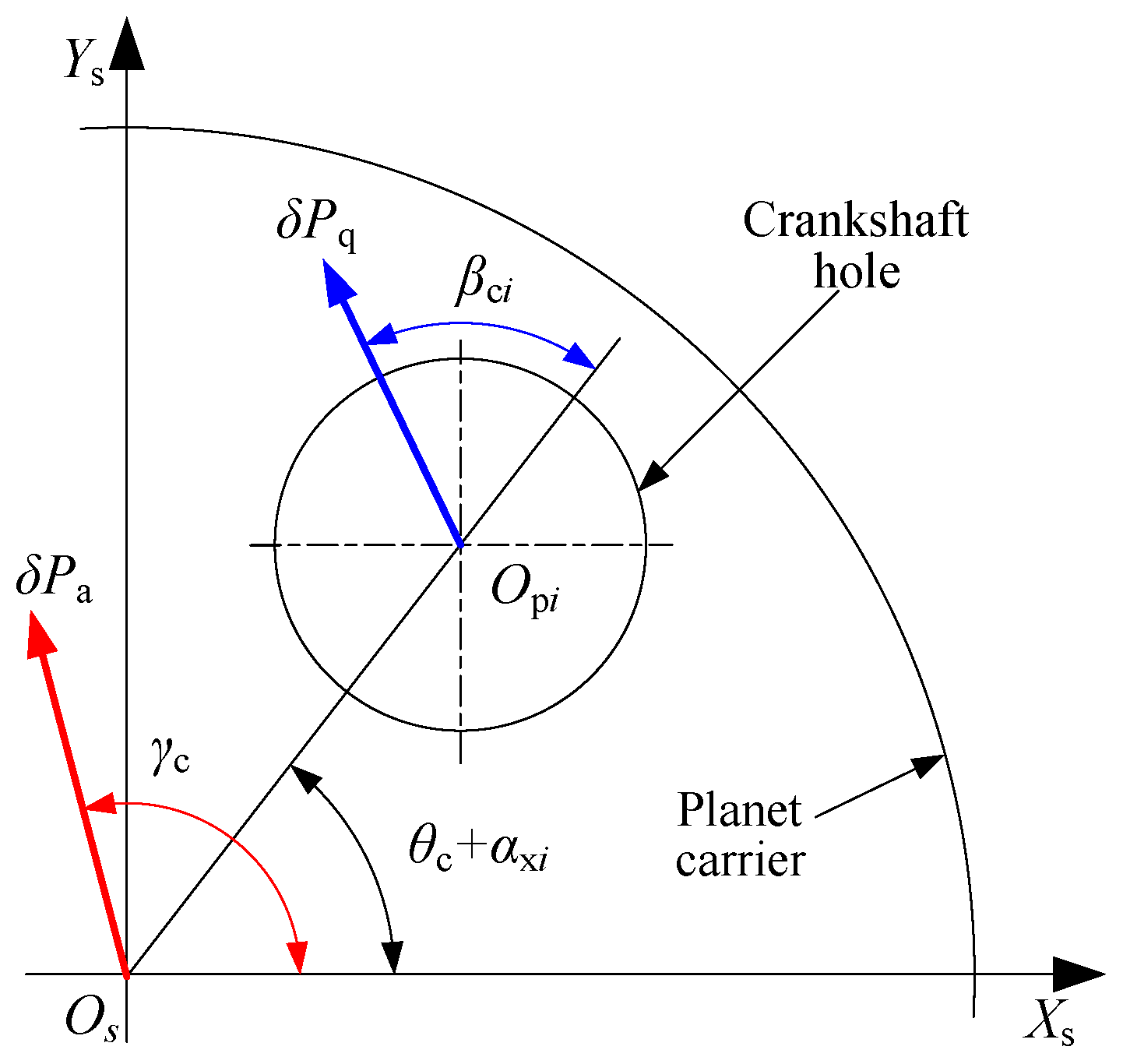
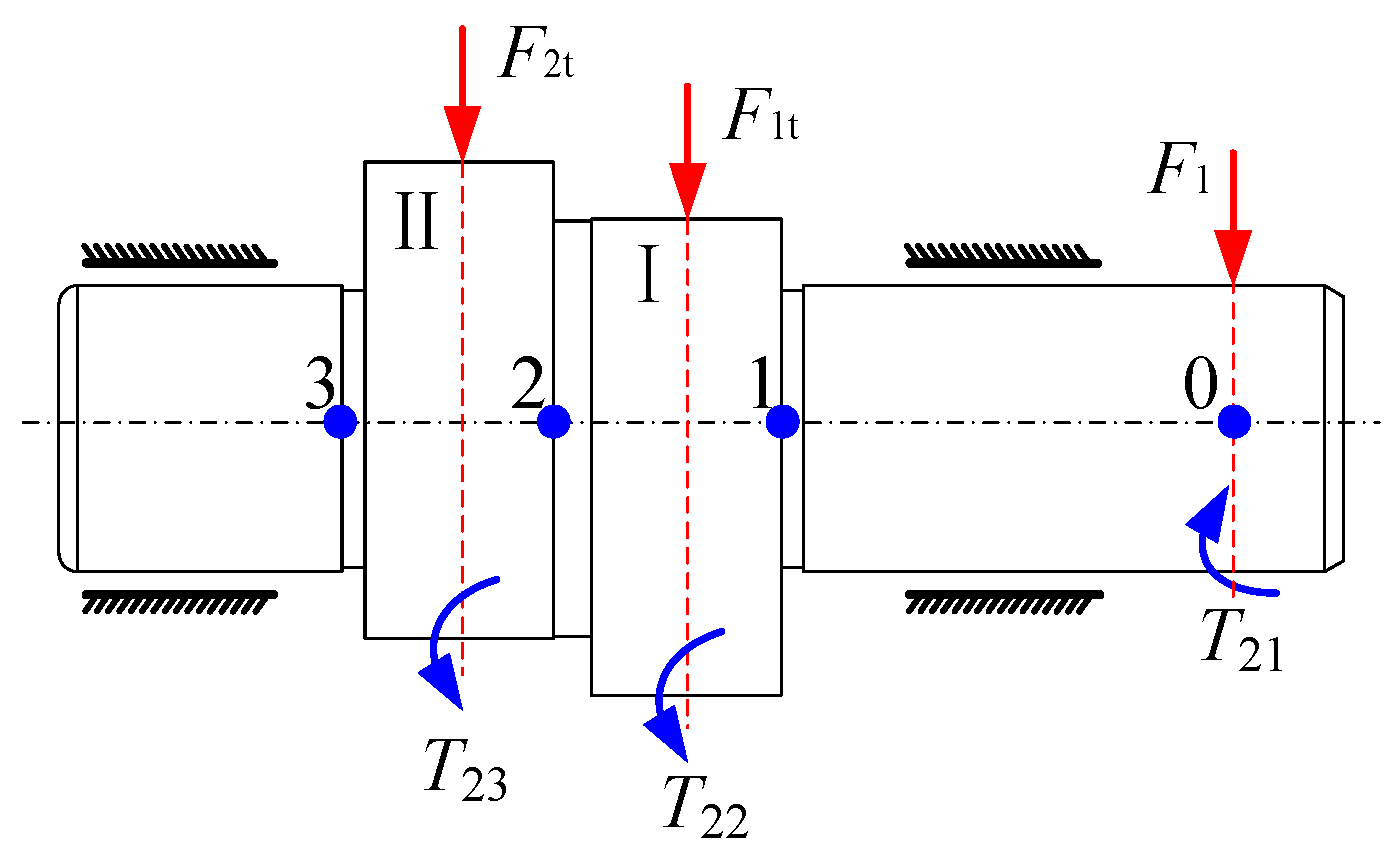
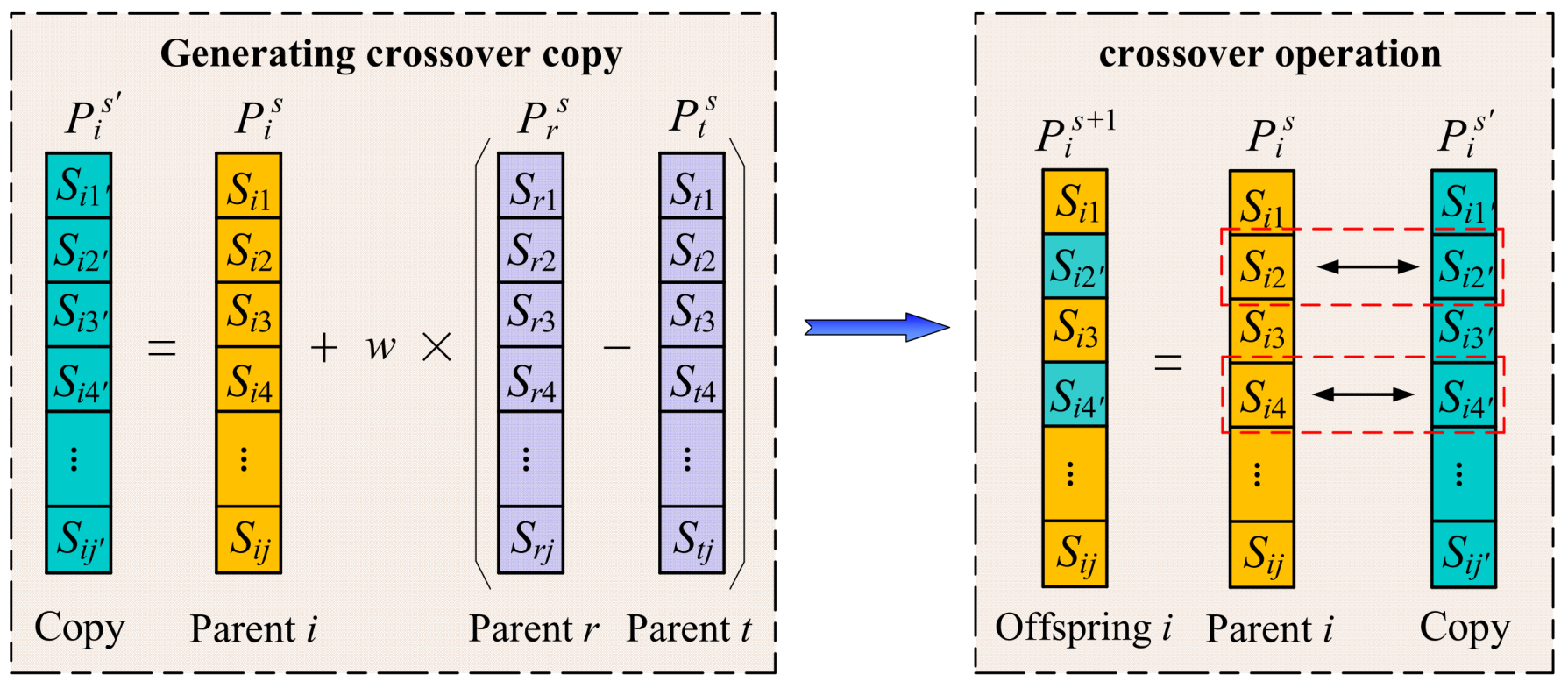
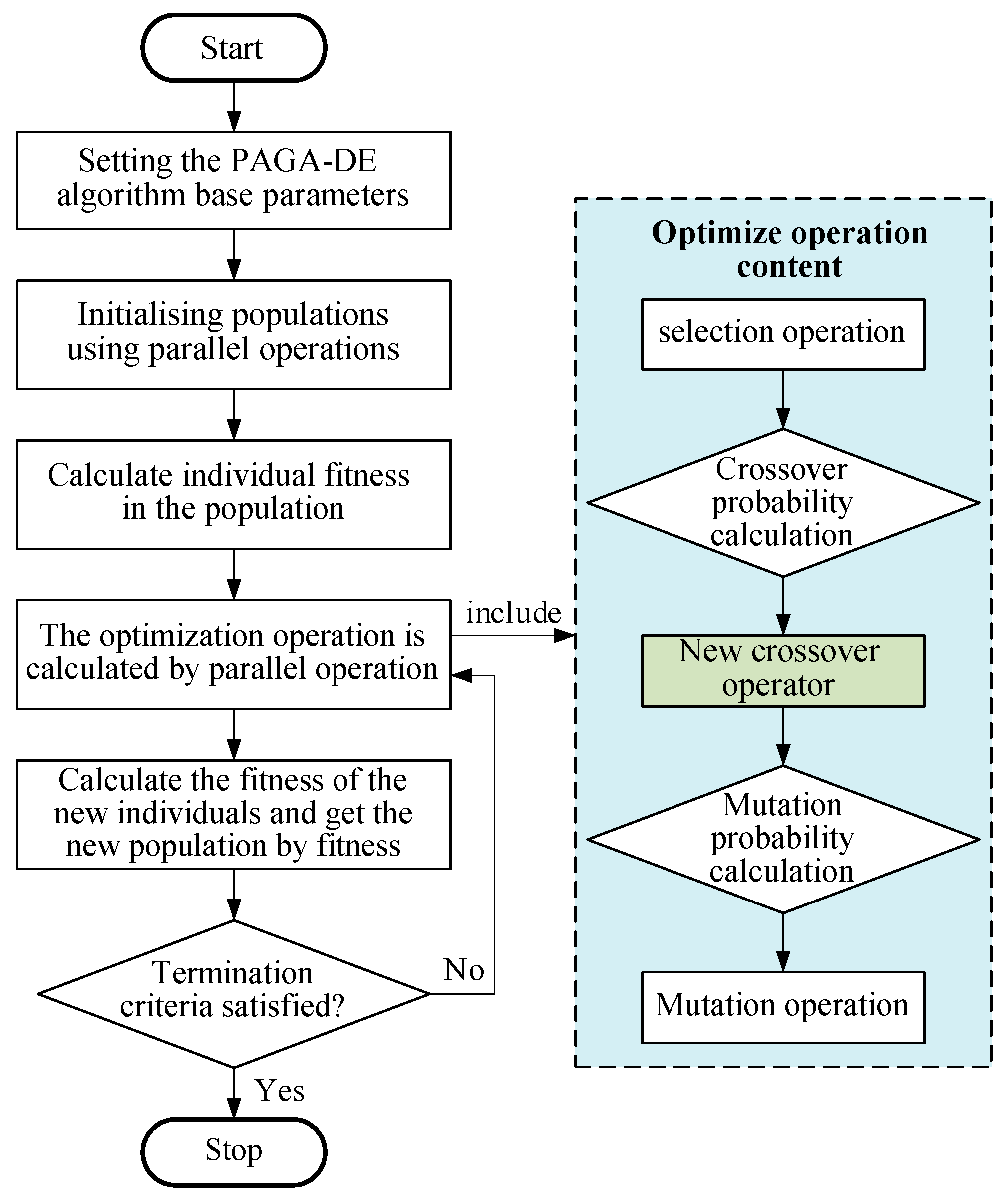
1. Introduction

The rotary vector (RV) reducer is widely used in high-end precision equipment such as robots, photovoltaic manufacturing and power generation, and semiconductor production, due to its advantages of high energy utilization rate, strong load-bearing capacity, high transmission accuracy, and a wide range of transmission ratios. The cycloid-pin gear is the core transmission component of RV reducers. Its parameter design directly affects the RV reducer ratio, transmission accuracy, load-carrying capacity, and other major transmission properties [1,2,3]. The design theory and research of cycloid-pin gears are mostly based on traditional mechanical analysis methods. In engineering practice, a tendency towards conservative strength design is often adopted [4,5]. The dimensional accuracy of each component mostly adopts the same tolerance level, which makes the reliability of the RV reducer low, resulting in a waste of human resources. As a result, the design and optimization of the cycloid-pin gear in RV reducers have been a continuous focus of research for scholars both domestically and internationally, aiming to enhance transmission accuracy and carrying capacity and reduce mass loss.

At present, research on the design of cycloid-pin gear primarily focuses on aspects such as error impact analysis, tolerance optimization and selection, profile modification design, meshing contact analysis and structural dimension parameter analysis. Errors are a critical factor influencing the accuracy of gear transmission, while tolerances determine the machining process requirements for gears [6]. Thus, error impact analysis, tolerance optimization, and selection are focal points of the active design research for cycloid-pin wheel transmission at the microscopic scale. On the basis of analyzing various error factors affecting backlash, Li et al. [7] employed orthogonal experiments and robust design to effectively control the backlash in RV transmissions. Han et al. [8], utilizing the “Sobol” method, established the global sensitivity analysis model of RV reducers to investigate the effects of manufacturing errors, assembly errors, and bearing clearance on transmission accuracy. Li et al. [9], based on the tooth contact analysis, examined the impact of manufacturing errors on the precision of RV transmission. Ahn et al. [10] presented an impact quantitatively analysis of pin radius errors and friction between the cycloid gear and pin on the meshing force in the cycloid-pin gear pair using the FE method. In terms of tolerance optimization and selection, Sun et al. [11] analyzed the sensitivity of various errors in RV reducers and, through Monte Carlo simulations, determined suitable tolerance levels for error parameters. Zhao et al. [12] constructed the distribution model of various errors and tolerances of RV reducers on the basis of the analysis of the backlash model. Chu et al. [13] developed a tolerance selection and assembly method for RV reducers based on a genetic algorithm, aiming to achieve the required backlash precision. Li et al. [14] started with the processing cost of tolerances to achieve tolerance design for the parameters inside the cycloid-pin gear. At the microscopic level, profile modification design is also a pivotal study direction in the design of the cycloid-pin gear. Wan et al. [15] quantitatively analyzed the variations in precision in cycloid-pin gear transmission due to different combinations of profile modifications. Liu et al. [16], starting from the meshing force of the cycloid-pin gear pair, optimized the design of the cycloid gear profile modification. Sun et al. [17] proposed a novel parabolic profile modification method and used a particle swarm algorithm with the minimum transmission error as the optimization objective to obtain the optimum trimming coefficients.

Meshing contact analysis is particularly crucial for the study of gear design [18,19,20]. Experts have conducted the following research in the field of cycloid-pin gears. Blagojevic et al. [21] conducted a stress analysis of single-stage cycloid gears under working conditions with only one pair of meshing teeth using finite element analysis and experimentally used the strain gauge method for experimental validation. Li et al. [22] established a theoretical contact analysis model of cycloid pin gears considering manufacturing errors and analyzed the effects of tooth shape error and pitch error of cycloid gears on meshing characteristics. Qiao et al. [23] performed transient dynamic analysis on RV reducers to investigate the stress distribution of cycloid-pin gears during the meshing process. Li et al. [24] applied the minimum energy principle to propose a mathematical model for calculating the number of simultaneously meshing teeth in the process of cycloid-pin gear transmission, which was validated by simulation and measurement experiments. Li et al. [25] considered the impact of ring pin position deviation, established an analysis model for the load distribution in the misaligned cycloid-pin gear pair, and analyzed the effects of pin tooth radial position error and phase angle on the meshing characteristics of cycloid-pin gear pair. In addition, scholars conducted qualitative and quantitative analyses of structural dimension parameters at the macro scale. Bednarczyk et al. [26] investigated the effect of the eccentricity of the cycloid gear on meshing force and power loss in gear transmission through optical elastic experiments. A generalized dynamics model of a cycloid-pin gear of an RV reducer considering bearings was developed by Xu et al. [27]. It is used to investigate the effects of geometrical parameters in the cycloid-pin gear pair on the dynamic contact response and internal load transfer characteristics. Zhang et al. [28] analyzed the impact of design parameters of the cycloid-pin gear (eccentricity, radius of pin gear distribution circle, pin gear radius, and width of the cycloid gear, etc.) on the load-bearing capacity from three aspects: load distribution coefficient, torsional stiffness, and contact stress. Li et al. [29] established a calculation model for the meshing stiffness of the cycloid-pin gear pair related to profile modification and eccentricity errors. They separately explained the effects of profile modification and eccentricity on torsional stiffness, load-bearing transmission error, and contact stress.

The above scholars have analyzed the influence of the design parameters of the cycloid-pin gear more comprehensively. However, the design parameters of components in the cycloid-pin gear transmission system have a relationship of interdependence and mutual influence, and the transmission performance is coupled with each other. Therefore, the design of the cycloid-pin gear of RV reducers should not only comprehensively analyze its failure mode, structural form, and design guidelines, but also make the comprehensive performance of the transmission system to meet the design requirements.

Meanwhile, considering multiple transmission performance indicators for parameter design is a typical multi-objective optimization problem. In this regard, experts have conducted the following research on cycloid-pin gear transmission. Wang et al. [30], with the optimization objectives of achieving the highest transmission efficiency and the smallest volume, considered constraints such as tooth profile, strength, and lifespan. They conducted an optimization design of parameters including the cycloid gear, pin gear, and pin for the cycloid-pin gear transmission. Wang et al. [31] established a multi-objective optimization model with the objectives of the volume of the cycloid-pin gear, the bearing load on the turning arm bearing, and the bending stress on the pin. Zhang et al. [32] optimized the geometric dimensions and modification of RV reducers with the objectives of volume, efficiency, and anti-adhesive capability. Wu et al. [33] proposed an optimization method for the design of the cycloid-pin gear in RV transmissions with transmission error, load of the turning arm bearing, and volume as optimization objectives. The method considers constraints such as tooth profile interference, contact strength, and bearing life. Song et al. [34] proposed a cycloid gear profile design method that considers a composite modification function and transmission error as optimization objectives, determining the magnitude of the profile modification. Furthermore, in the multi-objective optimization of other gear transmission systems, Paridhi et al. [35], considering constraints such as tooth surface contact and bending strength, minimized volume as the optimization objective. They conducted an optimization design for the profile displacement coefficient, number of teeth, face width, and module of helical gears. Daoudi et al. [36], considering constraints such as assembly, bending strength, and tooth surface contact strength, started from the mass, center distance, and efficiency of the epicyclical gear train system. They optimized and improved parameters such as the number of teeth, tooth width, tooth thickness, and shaft diameter. Yao [37] established an optimization model with the objectives of center distance, load factor, and meshing efficiency for spur gear systems. Using the NSGA-II algorithm, they achieved optimization and improvement of design parameters such as module, number of teeth, and transmission ratio. The scholars mentioned above, in the multi-objective optimization of gear transmission, mostly focus on efficiency, volume, and mechanical load-bearing performance, regulating design parameters at the macro scale. Simultaneously, research on related backlash primarily concentrates on error parameter design [7,8] and tolerance allocation issues [11,12]. There is a lack of multi-scale parameter design methods for cycloid-pin gear drives involving comprehensive analysis of backlash and other transmission performance. Moreover, most of the mentioned optimization designs use traditional intelligent optimization algorithms. For different multi-objective optimization models, the computational efficiency and optimization accuracy of the algorithm are quite different.

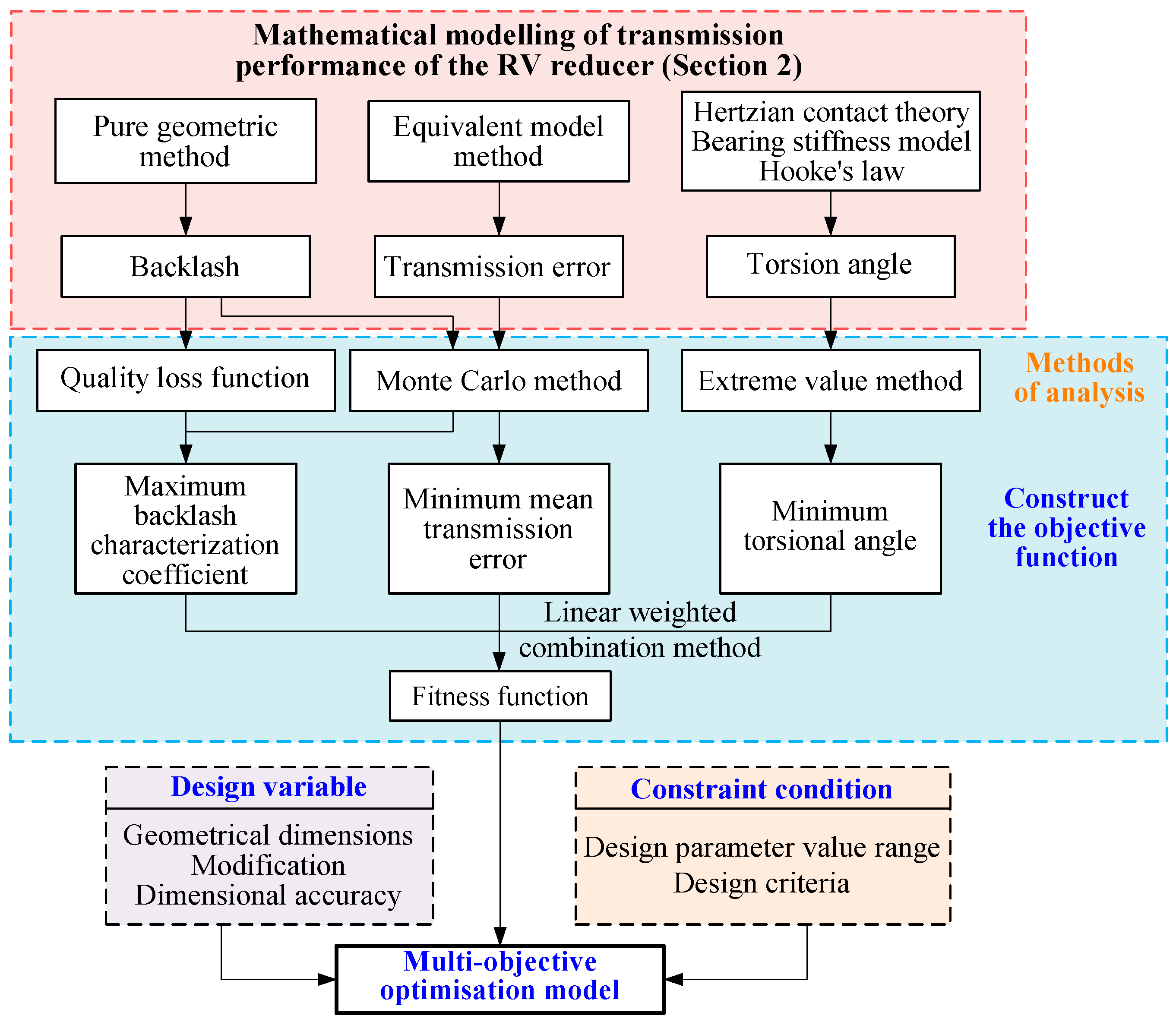
To address the above issues, this paper proposes a multi-objective optimization design method for cycloid-pin gear pairs, comprehensively considering backlash, transmission error, and torsional stiffness in RV reducers. This method takes the geometric dimensions, dimensional accuracy (tolerance), and modification of cycloid-pin gear pairs as design variables, enabling active regulation of parameters of both macro and micro dual scales on the transmission performance of RV reducers. Additionally, an improved Parallel Adaptive Genetic Algorithm using Deferential Evolution (PAGA-DE) is introduced, enhancing computational efficiency and convergence accuracy during the optimization model-solving process. The research presented in this paper essentially achieves error control in the transmission system and provides guidance for the design of geometric dimensions. The established analytical model offers theoretical support for research and optimization in the field of reducers, while also presenting a novel improvement in the algorithmic domain.

2. Transmission Performance Analysis Model of RV Reducers

In this paper, the standard RV reducer with three crankshafts is the object of study, and its transmission principle is illustrated in Figure 1. The establishment of a reasonable and effective mathematical model for transmission performance, including backlash, transmission error, and torsional stiffness, is the theoretical basis for the analysis and optimization of precision reducer transmission performance. These models are also crucial for achieving the optimal design of the cycloid gears in RV reducers.

2.1. Mathematical Model of Backlash in RV Reducers

The root cause of the formation of backlash is gaps between gear meshing pairs (i.e., side clearance) caused by various errors. Errors are generally divided into three main categories: manufacturing errors, assembly errors, and other errors (temperature, force deformation, etc.). Manufacturing errors and assembly errors are important reasons for the generation of geometric return difference. According to the transmission principle, the backlash of the RV reducer primarily comprises three parts: the first-stage involute gear system backlash ${\phi }_1$, the second-stage cycloid-pin gear backlash ${\phi }_2$, and the backlash in the output mechanism ${\phi }_3$. The total backlash ${\phi }_{\Sigma }$ of the RV transmission system is obtained by superimposing these three components [11].

$${\phi }_{\Sigma }={\phi }_1+{\phi }_2+{\phi }_3$$

(1)

2.1.1. Backlash of the Involute Gear System

The primary error parameters considered in calculating the backlash of involute gear systems comprise the average length error $E_{\mathrm{w}}$ of the base tangent, center distance error $\Delta F_{\alpha }$, and radial runout error $\Delta F_{\mathrm{r}}$ of the gear [38]. $E_{\mathrm{w}}$, $\Delta F_{\alpha }$, and $\Delta F_{\mathrm{r}}$ induce circumferential clearances as illustrated below:

$$\left\{\begin{array}{l}{j}_{\mathrm{E}1}=E_{\mathrm{w}}/\cos \alpha \\ j_{\mathrm{E}2}=2\Delta F_{\alpha }{K}_{\alpha }\tan \alpha \\ j_{\mathrm{E}3}=\Delta F_{\mathrm{r}}{K}_{\alpha }\tan \alpha \end{array}\right.,$$

(2)

where $j_{\mathrm{E}1}$, $j_{\mathrm{E}2}$ and $j_{\mathrm{E}3}$ represent the circumferential clearances induced by errors $E_{\mathrm{w}}$, $\Delta F_{\alpha }$ and $\Delta F_{\mathrm{r}}$, respectively, $\alpha$ is the pressure angle of involute gears, $K_{\alpha }$ is the conversion factor, $K_{\alpha }=\sin {\alpha }^{\prime }/\sin \alpha$, and ${\alpha }^{\prime }$ is the angle of engagement for involute gears.

Converting clearance $J_{\mathrm{E}i}$ into an angle at the output shaft, we can obtain the backlash ${\phi }_1$ of the involute gear system as follows:

$${\phi }_1=\frac{180\times 60\left(J_{\mathrm{E}1}+J_{\mathrm{E}2}+J_{\mathrm{E}3}\right)}{i_{\mathrm{z}}\pi r_1},$$

(3)

where $i_{\mathrm{z}}$ is the overall transmission ratio, and $r_1$ is the radius of the sun gear pitch circle.

2.1.2. Backlash of the Cycloid-Pin Gear Pair

By employing the pure geometric method [39], modification of equidistance $\Delta r_{\mathrm{p}}$, moving distance modification $\Delta R_{\mathrm{p}}$, and various errors in the cycloid-pin gear pair are converted into clearances along the meshing, which errors contain the radius of the circle error of the pin gear $\delta R_{\mathrm{p}}$, the radius of the pin gear error $\delta r_{\mathrm{p}}$, the radial run-out error of the cycloid gear $\delta F_{\mathrm{r}1}$, the circular position error of the pin gear hole $\delta t$, the pitch cumulative error of the cycloid gear $\delta F_{\mathrm{p}}$, the radial-moving modification error $\delta \Delta r_{\mathrm{p}}$, the equidistant modification error $\delta \Delta R_{\mathrm{p}}$, and the eccentric error of crankshaft $\delta a$. Subsequently, based on the principles of RV transmission and the relationship of motion transmission, these clearances are further transformed into angular displacements at the output shaft, thereby obtaining the backlash of the cycloid-pin gear pair.

$${\phi }_2=\frac{180\times 60}{\pi }\left(\frac{{\displaystyle \sum \Delta j_{2i}}}{a{z}_{\mathrm{c}}}+\Delta {\phi }_a\right),$$

(4)

where ${\displaystyle \sum \Delta j_{2i}}$ is the clearance on the meshing line that errors translate into, $\Delta {\phi }_a$ is the backlash caused by eccentric error of crankshaft $\delta a$, and they can be calculated by

$$\Delta j_{2i}={\mathit{C}}_{\mathrm{O}}{\mathit{E}}_{\mathrm{R}},$$

(5)

where ${\mathit{C}}_{\mathrm{O}}$ is the coefficient matrix, ${\mathit{E}}_{\mathrm{R}}$ is the error matrix,

$$\left\{\begin{array}{l}{\mathit{C}}_{\mathrm{O}}=\mathrm{diag}\left(2,-2\sqrt{1-K_1^2},2\sqrt{1-K_1^2},-2,1/2,2K_1,-K_1,2,-2\sqrt{1-K_1^2}\right)\\ {\mathit{E}}_{\mathrm{R}}={\left(\Delta r_{\mathrm{p}},\Delta R_{\mathrm{p}},\delta R_{\mathrm{p}},\delta r_{\mathrm{p}},\delta F_{\mathrm{r}1},\delta t,\delta F_{\mathrm{p}},\delta \Delta r_{\mathrm{p}},\delta \Delta R_{\mathrm{p}}\right)}^{\mathrm{T}}\end{array}\right.,$$

where $K_1$ is the short width coefficient, $a$ is eccentricity, $z_{\mathrm{c}}$ is the number of teeth of the cycloid gear.

$$\Delta {\phi }_a=-2k_{\mathrm{n}}\delta a,$$

(6)

where $k_{\mathrm{n}}$ is the conversion coefficient of eccentricity error, and it is expressed as:

$$k_{\mathrm{n}}=\frac{\Delta r_{\mathrm{p}}}{a^2{z}_{\mathrm{c}}}-\left(\frac{z_{\mathrm{c}}}{a{R}_{\mathrm{p}}^2\sqrt{1-K_1^2}}+\frac{\sqrt{1-K_1^2}}{a^2{z}_{\mathrm{c}}}\right)\Delta R_{\mathrm{p}},$$

where $R_{\mathrm{p}}$ is the radius of pin position.

2.1.3. Backlash of the Output Mechanism

The backlash ${\phi }_3$ generated by the output mechanism is mainly caused by the radial clearance of the turning arm bearing $\Delta u$, which is calculated as follows:

$${\phi }_3=\frac{180\times 60}{\pi a_0}\Delta u,$$

(7)

where $a_0$ is the center distance of involute planet gear transmission.

2.2. Mathematical Model of Transmission Errors in RV Reducers

This paper adopts the equivalent model method [40] to model and analyze transmission errors in RV reducers. The modeling principle is based on the meshing line analysis method, considering the influence of the phase angles of errors. It projects errors onto the meshing line, transforming them into equivalent meshing errors, and then calculates transmission errors. The overall transmission error calculation for RV reducers includes three parts: first-stage involute gear system transmission error ${\beta }_1$, second-stage cycloid-pin gear pair transmission error ${\beta }_2$, and output mechanism transmission error ${\beta }_3$ [33].

$${\beta }_{\Sigma }={\beta }_1+{\beta }_2+{\beta }_3$$

(8)

2.2.1. Transmission Error of the Involute Gear System

The errors primarily studied in the involute gear system include the manufacturing eccentricity error of the sun gear $E_{\mathrm{s}}$, the manufacturing eccentricity error of the planet gear $E_{\mathrm{p}i}$, and the installation eccentricity error of the sun gear $A_{\mathrm{s}}$. Their relative positions in the gear system are illustrated in Figure 2. In the figure, the center O of the sun wheel is taken as the origin of the coordinate system, a fixed coordinate system $XOY$ is established, and the direction of the meshing line away from the sun gear teeth is set as a positive direction. Using pure geometry, errors $E_{\mathrm{s}}$, $E_{\mathrm{p}i}$ and $A_{\mathrm{s}}$ are projected onto the meshing line, obtaining equivalent meshing errors $e_{\mathrm{s}}$, $e_{\mathrm{p}i}$ and ${\alpha }_{\mathrm{s}}$ along the meshing line, respectively.

$$\left\{\begin{array}{l}{e}_{\mathrm{s}}=E_{\mathrm{s}}\cos \left({\theta }_{\mathrm{s}}+{\beta }_{\mathrm{s}}-A_{\mathrm{n}}\right)\\ e_{\mathrm{p}i}=E_{\mathrm{p}i}\cos \left({\theta }_{\mathrm{p}}+{\beta }_{\mathrm{p}i}-A_{\mathrm{n}}\right)\\ {\alpha }_{\mathrm{s}}=A_{\mathrm{s}}\cos {\gamma }_{\mathrm{s}}\cos A_{\mathrm{n}}+A_{\mathrm{s}}\sin {\gamma }_{\mathrm{s}}\sin A_{\mathrm{n}}\end{array}\right.,$$

(9)

where ${\theta }_{\mathrm{s}}$ is the rotation angle of the sun gear, ${\beta }_{\mathrm{s}}$ is the phase angle of the manufacturing eccentricity error of the sun gear, $A_{\mathrm{n}}$ is the angle between the meshing line and the X-axis, $A_{\mathrm{n}}={\theta }_{\mathrm{c}}-{\alpha }^{\prime }+{\alpha }_{\mathrm{x}i}+0.5\pi$, ${\theta }_{\mathrm{c}}$ is the angle of the planet carrier, ${\theta }_{\mathrm{c}}={\theta }_{\mathrm{s}}/i_{\mathrm{z}}$, ${\alpha }_{\mathrm{x}i}$ is the relative position of the center of the planet gear in coordinate system $XOY$, ${\alpha }_{\mathrm{x}i}=120\left(i-1\right)$ (i represents the i-th planet gear, $i=1, 2, 3$), ${\theta }_{\mathrm{p}}$ is the rotation angle of the planet gear, ${\theta }_{\mathrm{p}}={\theta }_{\mathrm{s}}\left[1/i_{\mathrm{z}}+\left(1-1/i_{\mathrm{z}}\right)z_1/z_2\right]$, $z_1$ is the number of teeth of the sun gear, $z_2$ is the number of teeth of the planet gear, ${\beta }_{\mathrm{p}i}$ is the phase angle of the manufacturing eccentricity error of the planet gear, and ${\gamma }_{\mathrm{s}}$ is the phase angle of the installation eccentricity error of the sun gear.

The equivalent meshing errors calculated from Equation (9) are linearly superimposed and transformed into the angular error of the output shaft, representing the transmission error of the involute gear system ${\beta }_1$:

$${\beta }_1=\frac{180\times 3600\left(e_{\mathrm{s}}+e_{\mathrm{p}i}+{\alpha }_{\mathrm{s}}\right)}{r_1{i}_{\mathrm{z}}\pi }$$

(10)

2.2.2. Transmission Error of the Cycloid-Pin Gear Pair

The impact of various errors in the cycloid-pin gear pair is directly reflected at the output, exerting a significant influence on the overall transmission error. Therefore, starting from the installation fit and transmission motion relationship between the cycloid gear, pin, and crankshaft, an analysis is conducted on the influence of various error parameters on the system of transmission error [41,42].

Figure 3 depicts the relative positions of errors at a specific moment during the transmission process between the cycloid gear and the pin. In the figure, a Cartesian coordinate system, $X_{\mathrm{z}}{O}_{\mathrm{z}}{Y}_{\mathrm{z}}$, whose origin is the center of the pin gear $O_{\mathrm{z}}$, is employed to represent the reference coordinate system. $O_{\mathrm{c}j}$ is the center of the cycloid gear, $O_{\mathrm{a}k}$ is the center of the pin, and P is the node where the cycloid gear engages with the pin gear. Based on the meshing line analysis method, the radius of the circle error of the pin gear $\delta R_{\mathrm{p}}$, the pitch error of the pin gear $\delta R_{\mathrm{a}}$, the radial run-out error of the cycloid gear $\delta F_{\mathrm{r}1}$, the pitch cumulative error of the cycloid gear $\delta F_{\mathrm{p}}$, the radius of the pin gear error $\delta r_{\mathrm{p}}$ and the clearance error of pin and pin slot ${\delta }_{\mathrm{H}}$ are converted to the equivalent meshing errors $e_{\mathrm{R}}$, $e_{\mathrm{a}}$, $e_{\mathrm{Fr}}$, $e_{\mathrm{Fp}}$, $e_r$ and $e_{\mathrm{H}}$ on the meshing line as:

$$\left\{\begin{array}{l}{e}_{\mathrm{R}}=\delta R_{\mathrm{p}}\cos \left({\varphi }_{jk1}-{\alpha }_{jk}\right)\\ e_{\mathrm{a}}=\delta R_{\mathrm{a}}\sin \left({\alpha }_{jk}-{\varphi }_{jk1}\right)\\ e_{\mathrm{Fr}}=\delta F_{\mathrm{r}1}\cos \left({\varphi }_{jk2}-{\alpha }_{jk}\right)\\ e_{\mathrm{Fp}}=\delta F_{\mathrm{p}}\sin \left({\alpha }_{jk}-{\varphi }_{jk2}\right)\\ e_r=-\delta r_{\mathrm{p}}\\ e_{\mathrm{H}}={\delta }_{\mathrm{H}}\end{array}\right.,$$

(11)

where ${\varphi }_{kj1}$ is the angle between the line segment $O_{\mathrm{z}}{O}_{\mathrm{a}k}$ and the positive direction of ${\eta }_j$ axis, ${\varphi }_{kj2}$ is the angle between the line segment $O_{\mathrm{c}j}{O}_{\mathrm{a}k}$ and the positive direction of ${\eta }_j$ axis, and ${\alpha }_{jk}$ is the angle between the meshing line and the positive direction of the ${\eta }_j$ axis.

In addition, the change of cycloid gear tooth profile caused by modification is shown in Figure 4. Meanwhile, the coordinate system $X_{\mathrm{z}}{O}_{\mathrm{z}}{Y}_{\mathrm{z}}$ in the figure is the same as the reference coordinate system in Figure 3. Therefore, the equivalent meshing errors $e_{\mathrm{rp}}$ and $e_{\mathrm{Rp}}$ of the modification of equidistance $\Delta r_{\mathrm{p}}$ and the moving distance modification $\Delta R_{\mathrm{p}}$ are expressed as:

$$\left\{\begin{array}{l}{e}_{\mathrm{rp}}=\Delta r_{\mathrm{p}}\\ e_{\mathrm{Rp}}=-\Delta R_{\mathrm{p}}\left(1-K_1\cos {\varphi }_{jk1}\right){\left(1+K_1^2-2K_1\cos {\varphi }_{jk1}\right)}^{-1/2}\end{array}\right.$$

(12)

About the installation fit between the cycloid gear and the crankshaft, the existing errors are depicted in Figure 5. The coordinate system $X_{\mathrm{z}}{O}_{\mathrm{z}}{Y}_{\mathrm{z}}$ represents the pin gear coordinate system, and $O_{\mathrm{q}i}$ is the center of the crankshaft hole on the cycloid gear (also the center of the eccentric axis on the crankshaft). Combining with Figure 3, similarly, the expression for transforming the position error of the crankshaft hole on the cycloid gear $\delta C_{\mathrm{h}}$ and the eccentricity error of the crankshaft $\delta C_{\mathrm{s}}$ into equivalent meshing errors $e_{\mathrm{Ch}}$ and $e_{\mathrm{Cs}}$ along the meshing line is:

$$\left\{\begin{array}{l}{e}_{\mathrm{Ch}}=\delta C_{\mathrm{h}}\cos \left({\theta }_{\mathrm{c}}+{\alpha }_{\mathrm{x}i}+{\beta }_{\mathrm{h}ji}\right)\cos \left({\alpha }_{jk}+{\theta }_{\mathrm{p}}+{\psi }_j\right)+\delta C_{\mathrm{h}}\sin \left({\theta }_{\mathrm{c}}+{\alpha }_{\mathrm{x}i}+{\beta }_{\mathrm{h}ji}\right)\sin \left({\alpha }_{jk}+{\theta }_{\mathrm{p}}+{\psi }_j\right)\\ e_{\mathrm{Cs}}=\delta C_{\mathrm{s}}\cos \left({\theta }_{\mathrm{p}}+{\psi }_j+{\beta }_{\mathrm{s}ji}\right)\cos \left({\alpha }_{jk}+{\theta }_{\mathrm{p}}+{\psi }_j\right)+\delta C_{\mathrm{s}}\sin \left({\theta }_{\mathrm{p}}+{\psi }_j+{\beta }_{\mathrm{s}ji}\right)\sin \left({\alpha }_{jk}+{\theta }_{\mathrm{p}}+{\psi }_j\right)\end{array}\right.,$$

(13)

where ${\beta }_{\mathrm{h}ji}$ and ${\beta }_{\mathrm{s}ji}$ are the phase angle of the errors $\delta C_{\mathrm{h}}$ and $\delta C_{\mathrm{s}}$, respectively, and ${\psi }_j$ is the relative position of the center of the cycloid gear, ${\psi }_j=\left(j-1\right)\pi$ (j represents the j-th cycloid gear, $j=1, 2$).

The equivalent meshing errors obtained from Equations (11)–(13) are linearly superimposed and converted into angular errors on the output shaft, thus obtaining the transmission error of the cycloid-pin gear pair ${\beta }_2$.

$${\beta }_2=\frac{180\times 3600}{\pi }\left[\frac{\left(e_{\mathrm{R}}+e_{\mathrm{a}}+e_{\mathrm{Fr}}+e_{\mathrm{Fp}}+e_{\mathrm{r}}+e_{\mathrm{H}}+e_{\mathrm{rp}}+e_{\mathrm{Rp}}+e_{\mathrm{Ch}}\right)}{r_{\mathrm{c}}^{\prime }}+\frac{e_{\mathrm{Cs}}}{r_{\mathrm{c}}^{\prime }{i}_{\mathrm{h}}^{\prime }}\right],$$

(14)

where $r_{\mathrm{c}}^{\prime }$ is the pitch circle radius of the cycloid gear, $r_{\mathrm{c}}^{\prime }=a{z}_{\mathrm{c}}$, $i_{\mathrm{h}}^{\prime }$ is the transmission ratio from the crankshaft to the output shaft, $i_{\mathrm{h}}^{\prime }=\mathrm{abs}\left(i_{\mathrm{h}}\right)=\mathrm{abs}\left(-z_{\mathrm{c}}\right)=z_{\mathrm{c}}$.

2.2.3. Transmission Error of the Output Mechanism

In the installation fit and transmission motion between the planet carrier and the crankshaft, there is mainly the eccentricity error of the crankshaft hole on the planet carrier $\delta P_{\mathrm{q}}$ and the installation eccentricity error of the planet carrier $\delta P_{\mathrm{a}}$, as shown in Figure 6. With the center of the planet carrier $O_s$ as the coordinate origin, a reference coordinate system $X_s{O}_s{Y}_s$ is established, which coincides with the coordinate system $X_{\mathrm{z}}{O}_{\mathrm{z}}{Y}_{\mathrm{z}}$ in Figure 3. Based on the principle of equivalent meshing error transformation, the equivalent meshing errors $e_{\mathrm{pq}}$ and $e_{\mathrm{pa}}$ of errors $\delta P_{\mathrm{q}}$ and $\delta P_{\mathrm{a}}$, on the meshing line can be calculated by

$$\left\{\begin{array}{l}{e}_{\mathrm{pq}}=\delta P_{\mathrm{q}}\cos \left({\theta }_{\mathrm{c}}+{\alpha }_{\mathrm{x}i}+{\beta }_{\mathrm{c}i}\right)\cos \left({\alpha }_{jk}+{\theta }_{\mathrm{p}}+{\psi }_j\right)+\delta P_{\mathrm{q}}\sin ({\theta }_{\mathrm{c}}+{\alpha }_{\mathrm{x}i}+{\beta }_{\mathrm{c}i})\sin ({\alpha }_{jk}+{\theta }_{\mathrm{p}}+{\psi }_j)\\ e_{\mathrm{pa}}=\delta P_{\mathrm{a}}\cos {\gamma }_{\mathrm{c}}\cos \left({\alpha }_{jk}+{\theta }_{\mathrm{p}}+{\psi }_j\right)+A_{\mathrm{c}}\sin {\gamma }_{\mathrm{c}}\sin \left({\alpha }_{jk}+{\theta }_{\mathrm{p}}+{\psi }_j\right)\end{array}\right.,$$

(15)

where ${\beta }_{\mathrm{c}i}$ and ${\gamma }_{\mathrm{c}}$ are the phase angle of errors $\delta P_{\mathrm{q}}$ and $\delta P_{\mathrm{a}}$, respectively.

As above, according to Equation (15), the transmission error ${\beta }_3$ of the output mechanism is given by

$${\beta }_3=\frac{180\times 3600\left(e_{\mathrm{pq}}+e_{\mathrm{pa}}\right)}{\pi r_{\mathrm{c}}^{\prime }}$$

(16)

2.3. Mathematical Model of Torsion Angle in RV Reducers

According to generalized Hooke’s law, under the assumption of constant torque, the torsional angle is inversely proportional to the torsional stiffness under a certain torque. Therefore, a mathematical model of torsional angle is established to characterize the torsional stiffness. According to the RV transmission principle, the overall torsion angle ${\theta }_{\Sigma }$ can be divided into involute gear train torsion angle ${\theta }_1$, cycloid-pin gear pair torsion angle ${\theta }_2$, turning arm bearing torsion angle ${\theta }_3$, crankshaft torsion angle ${\theta }_4$ and planet carrier torsion angle ${\theta }_5$.

$${\theta }_{\Sigma }={\theta }_1+{\theta }_2+{\theta }_3+{\theta }_4+{\theta }_5$$

(17)

Among them, the torsional angle generated by the elastic deformation of the planet carrier can refer to the mathematical model in reference [43], which will not be explained in detail in this paper.

2.3.1. Torsion Angle of the Involute Gear System

From the ISO 6336 [44], the meshing stiffness of involute gears $k_{\mathrm{X}}$ is calculated as follows:

$$k_{\mathrm{X}}=\left(0.75\epsilon +0.25\right)C_{\mathrm{M}}{C}_{\mathrm{R}}{C}_{\mathrm{B}}B\frac{1}{q},$$

(18)

where $\epsilon$ is the contact degree of gear meshing, $C_{\mathrm{M}}$ is the theoretical correction coefficient, $C_{\mathrm{M}}=0.8$, $C_{\mathrm{R}}$ is the structure coefficient of the wheel blank, $C_{\mathrm{R}}=1$, $C_{\mathrm{B}}$ is the basic tooth profile coefficient, $C_{\mathrm{B}}=1$, $B$ is the tooth width of involute gear, and $q$ is the gear flexibility. Its calculation formula is

$$\begin{array}{l}q=0.04723+\frac{0.15551}{z_{\mathrm{n}1}}+\frac{0.25791}{z_{\mathrm{n}2}}-0.00635x_1-0.11654\frac{x_1}{z_{\mathrm{n}1}}-\\ 0.00193x_2-0.24188\frac{x_2}{z_{\mathrm{n}2}}+0.00529x_1^2+0.00182x_2^2\end{array},$$

(19)

where $z_{\mathrm{n}1}$ is the equivalent number of teeth of the solar wheel, $z_{\mathrm{n}2}$ is the equivalent number of teeth of the planet gear (when the gear is straight teeth, $z_{\mathrm{n}1}=z_1$, $z_{\mathrm{n}2}=z_2$), $x_1$ is the displacement coefficient of the solar wheel, and $x_2$ is the displacement coefficient of the planet gear.

Based on Hooke’s law and combined with Equation (18), the linear deformation $\Delta {\delta }_{\mathrm{p}}$ of involute gear transmission is represented as

$$\Delta {\delta }_{\mathrm{p}}=\frac{F_{\mathrm{t}}/\cos {\alpha }^{\prime }}{k_{\mathrm{X}}},$$

(20)

where $F_{\mathrm{t}}$ is the tangential component of involute gear.

The linear deformation $\Delta {\delta }_{\mathrm{p}}$ is converted to the sun gear angle, which is reduced by the transmission ratio $i_{\mathrm{z}}$ to obtain the torsion angle ${\theta }_1$ of the involute gear system in the output shaft.

$${\theta }_1=\frac{180\times 3600\Delta {\delta }_{\mathrm{p}}\cos {\alpha }^{\prime }}{\pi r_1{i}_{\mathrm{z}}}=\frac{180\times 3600F_{\mathrm{t}}}{\pi k_{\mathrm{X}}{r}_1{i}_{\mathrm{z}}}$$

(21)

2.3.2. Torsion Angle of the Cycloid-Pin Gear Pair

There is only one pair of teeth engaged in theory when the cycloid gear is engaged with the pin gear after modification. The other pairs of teeth have different sizes of initial meshing clearance ${\Delta }_1({\phi }_k)$ [45]. At the same time, $\delta R_{\mathrm{p}}$ and $\delta r_{\mathrm{p}}$ also produce a small gap ${\Delta }_2({\phi }_k)$, which affects the size of the clearance ${\Delta }_1({\phi }_k)$. Therefore, the initial meshing clearance $\Delta ({\phi }_k)$ becomes

$$\left\{\begin{array}{l}\Delta ({\phi }_k)={\Delta }_1({\phi }_k)-{\Delta }_2({\phi }_k)\\ {\Delta }_1({\phi }_k)=\Delta r_{\mathrm{p}}\left(1-S^{-1/2}\sin {\phi }_k\right)-\Delta R_{\mathrm{p}}\left(1-K_1^{\prime }\cos {\phi }_k-\sqrt{1-{K_1^{\prime }}^2}\sin {\phi }_k\right)S^{-1/2}\\ {\Delta }_2({\phi }_k)=\delta r_{\mathrm{p}}\left(1-S^{-1/2}\sin {\phi }_k\right)+\delta R_{\mathrm{p}}\left(1-K_1^{\prime }\cos {\phi }_k-\sqrt{1-{K_1^{\prime }}^2}\sin {\phi }_k\right)S^{-1/2}\end{array}\right.,$$

(22)

where $S=1+{K_1^{\prime }}^2-2K_1^{\prime }\cos {\phi }_k$, $(k=1, 2, \cdots , z_{\mathrm{p}}/2)$, $K_1^{\prime }=a{z}_{\mathrm{p}}/\left(R_{\mathrm{p}}+\Delta R_{\mathrm{p}}\right)$.

Under the action of the load torque $T_{\mathrm{c}}$, the cycloid gear contacts deformation produced with the pin, causing the cycloid gear to rotate by a small angle ${\beta }_{\mathrm{c}}$. According to the deformation coordination principle, the normal deformation $\Delta {\delta }_k$ of each tooth on the cycloid gear is given by

$$\Delta {\delta }_k=l_k{\beta }_{\mathrm{c}}=\frac{l_k\Delta {\delta }_{\max }}{r_{\mathrm{c}}^{\prime }},$$

(23)

where $l_k$ is the distance from the common normal of the engagement point of the k-th pin tooth or the normal of the point to be engaged to the center of the cycloid gear, $l_k=r_{\mathrm{c}}^{\prime }{S}^{-1/2}\sin {\phi }_k$, $\Delta {\delta }_{\max }$ is the maximum contact deformation.

Based on the Hertzian contact theory [25], the contact deformation $w$ of the cycloid gear with the pin is

$$w=\frac{2F}{\pi b_1}\left[\frac{1-{\mu }_1^2}{E_1}\left(\frac{1}{3}+\ln \frac{4R_1}{L}\right)+\frac{1-{\mu }_2^2}{E_2}\left(\frac{1}{3}+\ln \frac{4R_2}{L}\right)\right],$$

(24)

where $F$ is the force exerted on the two cylinders, $b_1$ is the contact length of the two cylinders, ${\mu }_1$ and ${\mu }_2$ are the Poisson’s ratios of the two cylinders, respectively, $E_1$ and $E_2$ are the modulus of elasticity of the two cylinders, respectively, $R_1$ and $R_2$ are the radii of the two cylinders, in addition to this

$$L=1.60\sqrt{\frac{F}{b}{K}_D\left(\frac{1-{\mu }_1^2}{E_1}+\frac{1-{\mu }_2^2}{E_2}\right)},$$

(25)

where when the cylinder is convex contact with the cylinder, $K_D=2R_1{R}_2/\left(R_1+R_2\right)$, and when the cylindrical and cylindrical holes are convex and concave contact, $K_D=2R_1{R}_2/\left(R_1-R_2\right)$.

If $F=F_{\max }$, combined with Equation (24), the maximum deformation $\Delta {\delta }_{\max }$ can be obtained.

$$\Delta {\delta }_{\max }=w_{\max }$$

(26)

If the normal displacement $\Delta {\delta }_k$ of a tooth pair is greater than the meshing clearance $\Delta ({\phi }_k)$, the pair of teeth is correspondingly in load-bearing mesh. The meshing force $F_k$ of the meshing tooth pair of the cycloid-pin gear is

$$F_k=\frac{\Delta {\delta }_k-\Delta \left({\phi }_k\right)}{\Delta {\delta }_{\max }}{F}_{\max }$$

(27)

According to the torque equilibrium condition, we obtain $T_{\mathrm{c}}={\displaystyle \sum _{k=g}^G{F}_k}{l}_k$ (g is the starting tooth number, $G$ is the end meshing tooth number). Then, combining with Equation (27), the maximum meshing force $F_{\max }$ can be deduced as

$$F_{\max }=\frac{T_{\mathrm{c}}}{{\displaystyle \sum _{i=b}^e\left(\frac{l_i}{r_c^{\prime }}-\frac{\Delta \left({\phi }_i\right)}{\Delta {\delta }_{\max }}\right)}{l}_i}$$

(28)

In light of the deformation coordination condition Equation (23) and torque balance Equation (28), the mechanical analysis model of a cycloid-pin gear is founded. The calculation flow is as follows:

Step 1, the maximum meshing force is proposed as $F_{\max 0}$, that is substituted into Equation (24) to calculate the maximum deformation $\Delta {\delta }_{\max 0}$. $\Delta {\delta }_{\max 0}$ is substituted into Equation (28) to obtain $F_{\max 1}$.

Step 2, the difference judgment, if $\left|F_{\max 1}-F_{\max 0}\right|>0.1F_{\max 1}$, assign the $F_{\max 1}$ value to $F_{\max 0}$, and then repeat the cycle step 1.

Step 3, when $\left|F_{\max 1}-F_{\max 0}\right|<0.1F_{\max 1}$ is stopped, the maximum meshing force is $F_{\max }=\left(F_{\max 0}+F_{\max 1}\right)/2$, and the meshing interval $\left[g, G\right]$ and the maximum deformation $\Delta {\delta }_{\max }$ can be obtained from Equations (22)–(26).

From the maximum deformation $\Delta {\delta }_{\max }$, the cycloid gear angle $\Delta {\theta }_{\mathrm{b}}$ caused by contact deformation is

$$\Delta {\theta }_{\mathrm{b}}=\frac{\Delta {\delta }_{\max }}{R_{\mathrm{p}1}},$$

(29)

where $R_{\mathrm{p}1}$ is the pitch circle radius of the pin gear, $R_{\mathrm{p}1}=a{z}_{\mathrm{p}}$.

By converting the cycloid gear angle $\Delta {\theta }_{\mathrm{b}}$ to the output shaft, the cycloid-pin gear pair torsion angle ${\theta }_2$ can be obtained.

$${\theta }_2=\frac{180\times 3600}{\pi }\Delta {\theta }_{\mathrm{b}}{i}_{\mathrm{b}},$$

(30)

where $i_{\mathrm{b}}$ is the transmission ratio of the output shaft relative to the pinwheel when the involute sun gear is fixed, $i_{\mathrm{b}}=-\left(1-i_{\mathrm{z}}\right)/i_{\mathrm{z}}$.

In order to analyze the torsional stiffness of cycloid-pin gear pair, the equivalent torsional stiffness mathematical model was established based on the above mechanical analysis model. Using Hertz theory, considering the distribution radius error $\delta R_{\mathrm{p}}$ and the radius error $\delta r_{\mathrm{p}}$, the meshing stiffness $k_{\mathrm{b}}$ of cycloid gear and pinwheel single pair teeth [46] is

$$k_{\mathrm{b}}=\left\{\begin{array}{ll}\frac{\pi bE{R}_{\mathrm{p}}^{\prime }{S}^{3/2}}{4\left(1-{\mu }^2\right)\left(R_{\mathrm{p}}^{\prime }{S}^{3/2}+2T^{\prime }{r}_{\mathrm{p}}^{\prime }\right)},& \left({\rho }_2>0\right)\\ \frac{\pi bE}{4\left(1-{\mu }^2\right)},& \left({\rho }_2\le 0\right)\end{array}\right.,$$

(31)

where $b$ is the width of cycloid gear teeth, $\mu$ is the Poisson ratio, $\mu =0.3$, $E$ is the elasticity modulus, $E=2.06\times {10}^{11}\mathrm{Pa}$, $R_{\mathrm{p}}^{\prime }=R_{\mathrm{p}}+\delta R_{\mathrm{p}}$, $S=1+{K_1^{\prime }}^2-2K_1^{\prime }\cos {\phi }_k$, ${\phi }_k$ is the angle of the k-th pin tooth to the swivel arm $O_{\mathrm{c}}{O}_{\mathrm{z}}$, $r_{\mathrm{p}}^{\prime }=r_{\mathrm{p}}+\delta r_{\mathrm{p}}$, ${\rho }_2$ is the actual radius of curvature of the cycloid gear, $T^{\prime }=K_1^{\prime }\left(z_{\mathrm{p}}+1\right)\cos {\phi }_i-\left(1+z_{\mathrm{p}}{K_1^{\prime }}^2\right)$.

Based on Equation (31), the equivalent torsional stiffness $k_k$ of a single pair of teeth can be calculated as

$$k_k=k_{\mathrm{b}}{l}_k^2$$

(32)

The torsional stiffness $K$ of the cycloid-pin gear pair is obtained by linear superposition of equivalent torsional stiffness $k_k$ of the contact tooth pair at different phase angles.

$$K={\displaystyle \sum _{k=g}^G{k}_k}$$

(33)

2.3.3. Torsion Angle of the Turning Arm Bearing

Take a rotor bearing in a cycloid gear as an example. Its force is shown in Figure 7. In the figure, $X_{\mathrm{z}}{O}_{\mathrm{z}}{Y}_{\mathrm{z}}$ represents the global coordinate system established on the pinwheel, and $X_{\mathrm{q}}{O}_{\mathrm{q}}{Y}_{\mathrm{q}}$ is the local coordinate system of the turning arm bearing.

The meshing force of the contact tooth pair of cycloid-pin gear pair moves along the meshing line to node P. The combined force is $F_{\mathrm{p}}$ and is decomposed into the component forces $P_{\mathrm{X}}$ and $P_{\mathrm{Y}}$ along the $X_{\mathrm{z}}$ axis and $Y_{\mathrm{z}}$ axis [47].

$$\left\{\begin{array}{l}{P}_{\mathrm{X}}={\displaystyle \sum P_x}=\frac{M_a}{2r_{\mathrm{c}}^{\prime }}=\frac{M_a{z}_{\mathrm{p}}}{2K_1^{\prime }{R}_{\mathrm{p}}^{\prime }{z}_{\mathrm{c}}}\\ P_{\mathrm{Y}}=K_{\mathrm{y}}{P}_{\mathrm{X}}\\ K_{\mathrm{y}}=\frac{2}{\pi }\left(\frac{1}{K_1^{\prime }}+\frac{{K_1^{\prime }}^2-1}{2{K_1^{\prime }}^2}\ln \frac{1+K_1^{\prime }}{1-K_1^{\prime }}\right)\end{array}\right.,$$

(34)

where $M_a$ is the load borne by the RV transmission, with its maximum value being 2.5 times the rated torque $T$.

The force generated by the crankshaft on the cycloid gear can be divided into three parts: $F_{i\mathrm{t}}$, $F_{i1}$ and $F_{i2}$. The equation can be obtained from the moment equilibrium condition, as shown below

$$\left\{\begin{array}{l}{P}_{\mathrm{X}}{r}_{\mathrm{c}}^{\prime }-{\displaystyle \sum _{i=1}^{N_{\mathrm{q}}}{F}_{i\mathrm{t}}{a}_0=0}\\ P_{\mathrm{X}}-{\displaystyle \sum _{i=1}^{N_{\mathrm{q}}}{F}_{i1}=0}\\ P_{\mathrm{Y}}-{\displaystyle \sum _{i=1}^{N_{\mathrm{q}}}{F}_{i2}=0}\end{array}\right.,$$

(35)

where $N_{\mathrm{q}}$ is the number of crankshafts

The component forces $F_{i\mathrm{t}}$, $F_{i1}$ and $F_{i2}$ are projected onto the $X_{\mathrm{q}}$ and $Y_{\mathrm{z}}$ axes of the local coordinate system to obtain the component forces $F_{\mathrm{x}}$ and $F_{\mathrm{y}}$. Thereby, the radial force $F_{\mathrm{s}}$ on the turning arm bearing is formulated as

$$F_{\mathrm{s}}=\sqrt{F_{\mathrm{x}}^2+F_{\mathrm{y}}^2},$$

(36)

where $F_{\mathrm{x}}=F_{i\mathrm{t}}+F_{i1}\cos \phi +F_{i2}\sin \phi$, $F_{\mathrm{y}}=F_{i1}\sin \phi +F_{i2}\cos \phi$, and $\phi$ is the angle between the $Y_{\mathrm{q}}$ axis and $Y_{\mathrm{z}}$ axis.

Using the stiffness model of cylindrical roller bearing [48], the stiffness of turning arm bearing $k_{\mathrm{s}}$ is expressed as

$$k_{\mathrm{s}}=0.34\times {10}^4{F_{\mathrm{s}}}^{0.1}{N_{\mathrm{g}}}^{0.9}{L}^{0.8}\cos {\left({\theta }_j\right)}^{1.9},$$

(37)

where $N_{\mathrm{g}}$ is the number of rollers, $L$ is the effective length of the roller, and ${\theta }_j$ is the contact angle of the rolling element.

According to the stiffness $k_{\mathrm{s}}$ of the turning arm bearing, the relative displacement $\Delta {\delta }_{\mathrm{s}}$ of the inner and outer rings of the turning arm bearing can be calculated

$$\Delta {\delta }_{\mathrm{s}}=\frac{F_{\mathrm{s}}}{k_{\mathrm{s}}}$$

(38)

The relative displacement $\Delta {\delta }_{\mathrm{s}}$ is converted into the angle of the output shaft, that is, the torsion angle ${\theta }_3$ of the turning arm bearing.

$${\theta }_3=\frac{180\times 3600z_{\mathrm{c}}\Delta {\delta }_{\mathrm{s}}{i}_{\mathrm{b}}}{\pi z_{\mathrm{p}}{a}_0}$$

(39)

2.3.4. Torsion Angle of the Crankshaft

In transmission, the crankshaft is not only subject to the circumferential force distributed by the cycloid gear in the bearing hole of the boom, but also to the torque action, as shown in Figure 8. Therefore, the torsion angle of the crankshaft is caused by both circumferential bending deformation and torsional deformation of the crankshaft, which will be analyzed separately in the following.

(1) In the figure, $F_{1\mathrm{t}}$ and $F_{2\mathrm{t}}$ are separately the circumferential forces of the two cycloid gears on the crank shaft under the action of torque, $F_{1\mathrm{t}}=F_{2\mathrm{t}}=T/\left(6a_0\right)$. $F_1$ is the force caused by the input torque of the planet gear, $F_1=T_{\mathrm{in}}/\left(3r_1\right)$, and $T_{\mathrm{in}}$ is the input torque. Section I and section II represent the assumed action sections of two cycloid gears on the crankshaft. According to the beam deflection theory of material mechanics, the deflection of the crankshaft subjected to $F_{1\mathrm{t}}$, $F_{2\mathrm{t}}$ and $F_1$ in section I and section II is calculated, respectively. However, due to the different deflections of the two cross-sections, the average value $f_{\mathrm{m}}$ should be used to calculate the output shaft angle $\Delta {\theta }_{\mathrm{q}1}$ caused by the bending deformation of the crankshaft.

$$\Delta {\theta }_{\mathrm{q}1}=\frac{f_{\mathrm{m}}}{a_0}=\frac{f_{\mathrm{I}}+f_{\mathrm{II}}}{2a_0},$$

(40)

where $f_{\mathrm{I}}$ is the deflection at section I, and $f_{\mathrm{II}}$ is the deflection at Section II.

(2) According to the elastic deformation theory of material mechanics, the crankshaft will have a relative angle $\Delta {\phi }_s$ between Section 0 and Section 3 under the action of torque.

$$\Delta {\phi }_{\mathrm{s}}={\displaystyle \sum _{t=1}^3\frac{T_{2t}{l}_{2t}}{G{J}_{pt}}},$$

(41)

where $T_{2t}$ is the torque of each section of the crankshaft, $T_{23}=T_{22}=T_{21}/2$, $T_{21}=T{z}_2/\left(3i_{\mathrm{z}}{z}_1\right)$, $l_{2t}$ is the length of each shaft of the crankshaft, $G$ is the shear modulus, $G=8\times {10}^4\mathrm{MPa}$, $J_{\mathrm{p}t}$ is the polar moment of inertia, $J_{\mathrm{p}t}=\pi {d_t}^4/32$, and $d_t$ is the diameter of each shaft of the crankshaft.

The angle of torsion $\Delta {\phi }_{\mathrm{s}}$, generated by the crankshaft under the action of the torque, translates into the angle of rotation $\Delta {\theta }_{\mathrm{s}2}$ of the output shaft as

$$\Delta {\theta }_{\mathrm{s}2}=\frac{\Delta {\phi }_{\mathrm{s}}{z}_2}{z_1{i}_{\mathrm{z}}}$$

(42)

The total torsion angle ${\theta }_4$ of the crankshaft is obtained by superimposing the torsion angle obtained by Equations (40) and (42).

$${\theta }_4=\Delta {\theta }_{\mathrm{s}1}+\Delta {\theta }_{\mathrm{s}2}$$

(43)

3. Multi-Objective Optimization Model and Optimization Algorithm

3.1. Establishment of Multi-Objective Optimization Model

Figure 9 shows the flow chart for building a multi-objective optimization model for RV reducers. By analyzing the transmission performance of RV reducers in Section 1, the functional relationship between basic parameters (geometrical dimensions, error parameters and modification) and transmission performance has been achieved. Then, the Monte Carlo method, extreme value method, and quality loss function are employed to further analyze the mapping relationship between dimensional accuracy (error parameter tolerance) and backlash, transmission error, and torsional angle (torsional stiffness). Finally, three objective functions are acquired, representing the maximum backlash characterization coefficient, the minimum mean transmission error, and minimum torsional angle. Simultaneously, design variables and reasonable constraints are selected.

3.1.1. Objective Function

(1) Given that the errors are mutually independent and follow certain probability distributions within the tolerances, this paper employs the Monte Carlo method to find the mapping relationship between dimensional accuracy and transmission accuracy. The Monte Carlo experimental simulation $N_{\mathrm{S}}$ is set to 20,000 times. Experimental simulations are conducted based on the backlash mathematical model established in Section 2.1. Using the experimental sample data, the reliability coefficient is calculated by dividing the number $s_1\left(X\right)$ of backlashes within 1.5’ by the total number $S_1\left(X\right)$ of simulations.

$$S_1\left(X\right)=s_1\left(X\right)/N_{\mathrm{S}}$$

(44)

The quality loss function $L\left(T\right)$ is introduced to take into account both precision requirement and quality cost [49].

$$\left\{\begin{array}{l}L\left(T_i\right)=k{T_i}^2/4\\ C\left(T_i\right)={\displaystyle \sum L\left(T_i\right)}\end{array}\right.,$$

(45)

where k is the loss coefficient, $C\left(T_i\right)$ is the total quality loss, and $T_i$ is the tolerance value of each error.

By combining the reliability coefficient with the quality loss function, the backlash characterization coefficient is established as the objective function $f_1\left(X\right)$, and the formula is as follows:

$$\max f_1\left(X\right)=S_1\left(X\right)\left[C\left(T\right)/C\left(T_{\max }\right)\right],$$

(46)

where $C\left(T_{\max }\right)$ is the maximum quality loss.

(2) The quality cost function of tolerance has been included in the establishment of the backlash objective function, so only the Monte Carlo method is used to establish the transmission error objective function. According to the experimental simulation sample data, the mean transmission error is calculated as the objective function $f_2\left(X\right)$, and the formula is as follows:

$$\left\{\begin{array}{l}{S_2}^i\left(X\right)=\max \left({\beta }_1+{\beta }_2+{\beta }_3\right)-\min \left({\beta }_1+{\beta }_2+{\beta }_3\right)\\ \min f_2\left(X\right)=\left({\displaystyle \sum _i^{N_{\mathrm{s}}}{S_2}^i\left(X\right)}\right)/N_{\mathrm{s}}\end{array}\right.,$$

(47)

where $S_{2i}\left(X\right)$ is the transmission error obtained by the simulation of the i experiment.

(3) The extreme value method is used to analyze the error in the mathematical model of torsional angle (torsional stiffness). The principle is to take the maximum value of the error within the tolerance and substitute it into the model. In the case of constant load torque, the torsional angle is inversely proportional to the torsional stiffness. To enhance the load-bearing performance of reducers, the minimum torsional angle objective function $f_3\left(X\right)$ is established, expressed as:

$$\min f_3\left(X\right)={\theta }_1+{\theta }_2+{\theta }_3+{\theta }_4+{\theta }_5$$

(48)

(4) In order to make the backlash characterization coefficient, the mean value of transmission error and the torsion angle objective function reach an optimal equilibrium state under the interaction. Based on this, the linear weighted combination method is used to construct the fitness function $F\left(X\right)$.

$$\max F\left(X\right)=w_1\frac{f_1\left(X\right)}{D_{\mathrm{n}1}}+w_2\frac{D_{\mathrm{n}2}}{f_2\left(X\right)}+w_3\frac{D_{\mathrm{n}3}}{f_3\left(X\right)},$$

(49)

where $w_i$ is the weighting factor, the corresponding weighting factor of each objective function in this paper is $w_1=0.33, w_2=0.33, w_3=0.34$, and $D_{\mathrm{n}i}$ is the dimensionless coefficient. This paper takes the optimal value of each objective function under single-objective optimization ($i=1, 2, 3$).

3.1.2. Design Variable

As a core component of RV reducers, the design parameters of the cycloid-pin gear pair play a crucial role in the overall transmission performance of the RV system. According to the established mathematical model of backlash, transmission error, and torsion angle, the geometrical dimensions, modification, and dimensional accuracy of cycloid-pin gear pair are selected as the design variables, which are expressed as:

$$X=\left( R_{\mathrm{p}}, r_{\mathrm{p}}, a, b, \Delta r_{\mathrm{p}}, \Delta R_{\mathrm{p}}, T_{\mathsf{\delta }\mathrm{Rp}}, T_{\mathsf{\delta }\mathrm{rp}}, T_{\mathsf{\delta }\mathrm{Fr}}, T_{\mathsf{\delta }\mathrm{Fp}}\right),$$

where $R_{\mathrm{p}}$ is the radius of pin position, $r_{\mathrm{p}}$ is the radius of pins, $a$ is the eccentricity, $b$ is the width of the cycloid gear, $\Delta r_{\mathrm{p}}$ is the modification of equidistance, $\Delta R_{\mathrm{p}}$ is the moving distance modification, $T_{\mathsf{\delta }\mathrm{Rp}}$ is the tolerance of the pin distribution radius, $T_{\mathsf{\delta }\mathrm{rp}}$ is the tolerance of the pin radius, $T_{\mathsf{\delta }\mathrm{Fr}}$ is the tolerance of the run-out error of the cycloid gear, and $T_{\mathsf{\delta }\mathrm{Fp}}$ is the tolerance of the pitch cumulative error of the cycloid gear.

3.1.3. Constraint Condition

The constraint conditions are primarily determined from two aspects: the design parameters and design criteria of the cycloid-pin gear pairs [23,30,33].

Among the design parameters, the short width coefficient $K_1$ affects the tooth shape of cycloid gears, thereby affecting the load-bearing force of cycloid-pin gear pairs. The pin-diameter coefficient $K_2$ plays an important role in meeting the strength requirements of the pin gear housing with the pin and avoiding mutual collision between the pin. The tolerance accuracy $T_{\mathsf{\delta }i}$ is the focus of the study of gear transmission accuracy. The width of the cycloid gear $b$ is determined by the diameter of pinwheel central circle D_P. Thus, their constraint ranges are shown in

Table 1. Constraints on the structural parameters of the cycloid-pin gear pair.

Serial Number	Parameter	Range of Values
$g_1\left(\mathrm{X}\right)$	Number of cycloid gear teeth $z_{\mathrm{c}}$	≤11	13~23	25~59	61~87
	Short width coefficient $K_1$	0.42~0.55	0.48~0.74	0.65~0.9	0.75~0.9
$g_2\left(\mathrm{X}\right)$	Number of pins $z_{\mathrm{p}}$	12~14	24~36	36~60	60~88
	Pin-diameter coefficient $K_2$	2.8~2	2~1.25	1.6~1	1.5~0.99
$g_3\left(\mathrm{X}\right)$	Diameter of pinwheel central circle $D_{\mathrm{p}}$	95~105	106~120	140~160	165~185
$g_4\left(\mathrm{X}\right)$	Tolerance accuracy $T_{\mathsf{\delta }i}$	IT4~IT6
$g_5\left(\mathrm{X}\right)$	Width of cycloid gear $b$	$0.05D_{\mathrm{p}}\sim 0.1D_{\mathrm{p}}$

.

Where the range of values of $K_1$ is related to the number of cycloid gear teeth $z_{\mathrm{c}}$, $K_1=a{z}_{\mathrm{c}}/R_{\mathrm{p}}$, the range of values of $K_2$ is determined by the number of pins $z_{\mathrm{p}}$, $K_2=R_{\mathrm{p}}\sin \left(180/z_{\mathrm{p}}\right)/r_{\mathrm{p}}$, and $D_{\mathrm{p}}$ ranges from 140 to 160.

In the design of the cycloid-pin gear, to avoid the cycloid gear tooth profile from producing the top cut or sharp angle, it is necessary to make the radius of pins less than the minimum radius of curvature of the cycloid gear cam portion. At the same time, it is also necessary to ensure that meets the requirements of the strength, so add the constraint of contact stress between the pin gear and the cycloid gear. Because of the gear processing, manufacturing errors are inevitable. To facilitate the assembly and disassembly, lubrication and deformation, and other conditions, the cycloid gear and pin need to ensure a certain meshing clearance. Reducer service life is an important issue that cannot be ignored, and the service life of the RV reducer generally depends on the life of the bearings. The transmission efficiency of the RV reducer is also an important performance indicator, so it is necessary to ensure transmission efficiency while improving the transmission accuracy and load-bearing performance. Summarize the detailed expression of the above, shown in

Table 2. Constraints on the design criteria for the cycloid-pin gear pair.

Serial Number	Constraint Condition	Calculation Formula for the Range of Values
$g_6\left(\mathrm{X}\right)$	Avoiding top cutting or sharp angle	$\left\{\begin{array}{ll}{r}_{\mathrm{p}}-{\left(1+K_1\right)}^2{R}_{\mathrm{p}}/\left(z_{\mathrm{p}}{K}_1+1\right)<0,& \left(\left(z_{\mathrm{p}}-2\right)/\left(2z_{\mathrm{p}}-1\right)\ge K_1\right)\\ r_{\mathrm{p}}-R_{\mathrm{p}}\sqrt{27\left(1-{K_1}^2\right)\left(z_{\mathrm{p}}-1\right){\left(z_{\mathrm{p}}+1\right)}^{-3}}<0,& \left(1>K_1>\left(z_{\mathrm{p}}-2\right)/\left(2z_{\mathrm{p}}-1\right)\right)\end{array}\right.$
$g_7\left(\mathrm{X}\right)$	Contact stress between the pin gear and the cycloid gear	$0.418\sqrt{\frac{E}{b}{\left(\frac{F_i}{{\rho }_{\mathrm{e}}}\right)}_{\max }}-\left[{\sigma }_{\mathrm{H}}\right]\le 0$
$g_8\left(\mathrm{X}\right)$	Meshing clearance	$\frac{2\left(\Delta R_{\mathrm{p}}-\delta R_{\mathrm{p}}\right)}{a{z}_{\mathrm{c}}}\sqrt{1-K_1^2}-\frac{2\left(\Delta r_{\mathrm{p}}-\delta r_{\mathrm{p}}\right)}{a{z}_{\mathrm{c}}}\le 0$
$g_9\left(\mathrm{X}\right)$	Bearing life	$5000-\frac{{10}^6}{60n_{\mathrm{p}}}{\left(\frac{C}{P}\right)}^{\frac{10}{3}}\le 0$
$g_{10}\left(\mathrm{X}\right)$	Transmission efficiency	$0.8-{\eta }_{12}{\eta }_{\mathrm{S}}\left(1-{\eta }_{\mathrm{M}}\right)\le 0$

.

In the table, $F_i$ is the engagement force at the contact point of the cycloid-pinwheel, ${\rho }_{\mathrm{e}}$ is the equivalent radius of curvature, ${\rho }_{\mathrm{e}}=\left|{\rho }_i{r}_{\mathrm{rp}}/\left({\rho }_i-r_{\mathrm{rp}}\right)\right|$, ${\rho }_i$ is the radius of curvature of the cycloid gear at the engagement point, $\left[{\sigma }_{\mathrm{H}}\right]$ is the allowable contact stress, $\left[{\sigma }_{\mathrm{H}}\right]=1500 \mathrm{MPa}$, $n_{\mathrm{p}}$ is the rotating speed of the boom bearing, $n_{\mathrm{p}}=n_1\left[1+1/\left(z_{\mathrm{p}}-1\right)\right]$, C is the rated dynamic load of the boom bearing, P is the actual dynamic load on the boom bearing, ${\eta }_{\mathrm{S}}$ is the total bearing efficiency, ${\eta }_{\mathrm{S}}=0.99$, ${\eta }_{\mathrm{M}}$ is the hydraulic loss efficiency, ${\eta }_{\mathrm{M}}=\left(0.01~0.02\right){\eta }_{12}$, ${\eta }_{12}$ is the meshing efficiency of RV transmission, and it is expressed as

$${\eta }_{12}=\frac{1-i_1{i}_2\left({\eta }_1{\eta }_2\right)}{i_{\mathrm{z}}}, {\eta }_1=1-2.3f_{\mathrm{z}}\left(\frac{1}{z_1}+\frac{1}{z_2}\right), {\eta }_2=\frac{1-(R_{\mathrm{p}}-r_{\mathrm{p}})\frac{4f_{\mathrm{z}}}{K_1{z}_{\mathrm{c}}{R}_{\mathrm{p}}\pi }}{1+(R_{\mathrm{p}}-r_{\mathrm{p}})\frac{4f_{\mathrm{z}}}{K_1{R}_{\mathrm{p}}\pi }},$$

where $f_{\mathrm{z}}$ is the friction coefficient of tooth meshing, $i_1=-z_2/z_1$, $i_2=z_{\mathrm{p}}/\left(z_{\mathrm{p}}-z_{\mathrm{c}}\right)$.

In summary, the multi-objective optimization model established by combining the objective function and constraints can be expressed as

$$\begin{array}{l}\max F\left(X\right)=\left[\max f_1\left(X\right), \min f_2\left(X\right), \min f_3\left(X\right)\right]\\ \mathrm{s}.\mathrm{t}. \left\{\begin{array}{l}{g_i}^{\min }\left(X\right)\le g_i\left(X\right)\le g_{i\max }\left(X\right), \left(i=1, 2, \cdots , 5\right)\\ g_i\left(X\right)\le 0, \left(i=6, 7, \cdots , 10\right)\end{array}\right.\end{array}$$

(50)

3.2. PAGA-DE Algorithm

Traditional Genetic Algorithm (GA) [50,51] is commonly used to solve objective optimization models, but it has drawbacks such as a tendency to fall into local optima, poor convergence accuracy, and low computational efficiency for solving complex models. To overcome these shortcomings, an improved Parallel Adaptive Genetic Algorithm using Deferential Evolution (PAGA-DE) is proposed. The principle of this algorithm is to integrate the mutation operator from the Differential Evolution (DE) algorithm [52,53] into the crossover operator of the Adaptive Genetic Algorithm (AGA) [54,55], redesign the crossover operator, and utilize parallel operation [56] throughout the algorithm. The improved crossover operator is illustrated in Figure 10. First, it identifies the crossover individual ${P_i}^s$ in the current iteration population. Second, it randomly selects two individuals ${P_r}^s$ and ${P_t}^s$ from the current population and calculates the crossover copy ${P_i^s}^{\prime }$. Finally, it performs a crossover calculation between ${P_i}^s$ and ${P_i^s}^{\prime }$ to obtain the individual for the next iteration ${P_i}^{s+1}$, where s is the number of current iterations, s + 1 is the number of the next iteration, i is the serial number of cross individuals in the current iteration population, r and t are individual sequence numbers in the current iteration population, and $r\ne t\ne i$.

In addition, the variable w in the figure represents the scaling factor. In the DE algorithm, w is a constant, which is not conducive to the iterative optimization of the algorithm. In the early stages of algorithm optimization, to avoid the population falling into a local optimum, a relatively large scaling factor needs to be used. In the later stages of algorithm optimization, it is necessary to reduce the scaling factor to increase local exploration intensity. Therefore, in the crossover operator of the PAGA-DE algorithm, starting from the cosine law, the scaling factor w is designed as a dynamically changing function with the iteration count. It is represented as

$$w=\left\{\begin{array}{ll}\frac{1+{\left\{\cos \left[\left(Iter-1\right)\pi /\left(Ite{r}_{\max }-1\right)\right]\right\}}^k}{2}, & \left(i\le \frac{i_{\max }}{2}\right)\\ \frac{1-{\left|\cos \left[\left(Iter-1\right)\pi /\left(Ite{r}_{\max }-1\right)\right]\right|}^k}{2}, & \left(i>\frac{i_{\max }}{2}\right)\end{array}\right.,$$

(51)

where $Iter$ indicates the current iteration times, $Ite{r}_{\max }$ indicates the maximum number of iterations, and $k$ is the decreasing exponent, $0<k\le 1$.

The solution steps of PAGA-DE algorithm are shown in Figure 11.

4. Results and Discussion

To validate the proposed multi-objective optimization design method for the cycloid-pin gear, an analysis is conducted based on the optimization results from three aspects: backlash, transmission error, and torsional stiffness. Therefore, the design variables before and after optimization are separately incorporated into the mathematical models for backlash and transmission error in Section 2.1 and Section 2.2, and Monte Carlo simulation is employed for the analysis. Since the design variables are parameters within the cycloid-pin gear, the torsional stiffness is analyzed from two aspects: the equivalent torsional stiffness model of the cycloid-pin gear in Section 2.3.2 and the stiffness of the turning arm bearing in Section 2.3.3. It is noted that, according to Equation (37), the stiffness of the output arm bearing is primarily related to the radial force it experiences. Therefore, the analysis of the bearing force is incorporated into the results.

The RV-80E reducer is taken as an optimization example.

Table 3. Setting of basic parameters.

	Parameter	Numerical Value
Operating conditions and partial basic parameters of the RV reducer	Input speed n1	1215 r/min
	Input power P	1.64 kW
	Load torque T	784 N∙m
	$\mathrm{Transmission} \mathrm{ratio} i_{\mathrm{z}}$	81
	$\mathrm{Number} \mathrm{of} \mathrm{teeth} \mathrm{of} \mathrm{the} \mathrm{sun} \mathrm{gear} z_1$	21
	$\mathrm{Number} \mathrm{of} \mathrm{teeth} \mathrm{of} \mathrm{the} \mathrm{planet} \mathrm{gear} z_2$	42
	Module m	1.5
	$\mathrm{Number} \mathrm{of} \mathrm{cycloid} \mathrm{gear} \mathrm{teeth} z_{\mathrm{c}}$	39
	$\mathrm{Number} \mathrm{of} \mathrm{pins} z_{\mathrm{p}}$	40
PAGA-DE algorithm	Population size M	50
	$\mathrm{Maximum} \mathrm{crossover} \mathrm{probability} P_{\mathrm{c}1}$	1
	$\mathrm{Minimum} \mathrm{crossover} \mathrm{probability} P_{\mathrm{c}2}$	0.7
	$\mathrm{Maximum} \mathrm{mutation} \mathrm{probability} P_{\mathrm{m}1}$	0.1
	$\mathrm{Minimum} \mathrm{mutation} \mathrm{probability} P_{\mathrm{m}2}$	0.05

presents the operating conditions and partial basic parameters of the reducer, while also including the configuration of the PAGA-DE algorithm.

Table 4. The value of the error parameter in the backlash (Unit: μm).

Error Parameter	Upward Deviation	Lower Deviation	Tolerance
$E_{\mathrm{w}}$	−25	−45	20
$\Delta F_{\alpha }$	15	−15	30
$\Delta F_{\mathrm{r}}$	14	0	14
$\delta t$	10	−10	20
$\delta a$	3	0	3
$\delta \Delta r_{\mathrm{p}}$	1	−1	2
$\delta \Delta R_{\mathrm{p}}$	1.2	−1.2	2.4
$\Delta u$	4	1	3

and

Table 5. The value of the error parameter in the transmission error (Unit: μm).

Error Parameter	Upward Deviation	Lower Deviation	Tolerance
$E_{\mathrm{s}}$	10	0	10
$E_{\mathrm{p}}$	12	0	12
$A_{\mathrm{s}}$	10	0	10
$\delta R_{\mathrm{a}}$	5	−5	10
${\delta }_{\mathrm{H}}$	5	−5	10
$\delta C_{\mathrm{h}}$	5	0	5
$\delta C_{\mathrm{s}}$	3	0	3
$\delta P_{\mathrm{q}}$	5	0	5
$\delta P_{\mathrm{a}}$	5	0	5

are the error parameters that do not participate in optimization in the backlash and transmission error, respectively.

4.1. Comparison of Optimization Algorithms

The PAGA-DE and GA algorithms are applied to solve the multi-objective model in this study. In order to verify that the PAGA-DE algorithm has higher computational efficiency and convergence than the GA algorithm, this paper will be divided into two parts: simulated optimization solution and complete optimization solution.

Initially, the maximum number of iterations $Ite{r}_{\max }$ is set to 20 in the simulation optimization solution, and 3 calculations are performed, obtaining the runtimes as shown in

Table 6. Run time comparison.

Optimization Algorithm	Serial Number	Time (s)
GA	1	14,701
	2	13,813
	3	13,308
PAGA-DE	1	4787
	2	4740
	3	4739

. The computer hardware is an AMD Ryzen 9 5900X 12-Core Processor and 128 GB RAM. The average runtime for the PAGA-DE algorithm is 4755 s, while for the GA algorithm, it is 13,941 s. It is evident that, compared to the GA algorithm, the PAGA-DE algorithm improves optimization efficiency by more than 65.89%.

Additionally, in the complete optimization solution, by setting the maximum number of iterations $Ite{r}_{\max }$ to 100 for optimization, convergence curves for different algorithms are obtained, as shown in Figure 12. In the figure, at the end of the first iteration, the fitness value of the PAGA-DE algorithm is significantly higher than that of the GA algorithm. Moreover, the convergence curve of the PAGA-DE algorithm is approaching stability by the 15th iteration cycle, while the GA algorithm achieves a stable convergence curve only around the 69th iteration cycle. The results indicate a significant improvement in both convergence and computational efficiency for the improved PAGA-DE algorithm. The optimized data is be solved using the PAGA-DE algorithm, filled in

Table 7. Before and after optimization data.

(a) Geometric dimensions and modifications
Parameter	Before optimization			After optimization
$R_{\mathrm{p}}$ (mm)	75			77.4 (77.37)
$r_{\mathrm{p}}$ (mm)	3.5			3.8 (3.81)
$a$ (mm)	1.5			1.6 (1.59)
$b$ (mm)	10			14.7 (14.71)
$\Delta r_{\mathrm{p}}$ (μm)	−15			−7 (−7.18)
$\Delta R_{\mathrm{p}}$ (μm)	−30			−13 (−12.73)
(b) Dimensional accuracy (Unit: μm)
Error parameter	Before optimization			After optimization
	Upward deviation	Lower deviation	Tolerance	Upward deviation	Lower deviation	Tolerance
$\delta R_{\mathrm{p}}$($T_{\mathsf{\delta }\mathrm{Rp}}$)	2	−8	10	3	−9	12 (12)
$\delta r_{\mathrm{p}}$($T_{\mathsf{\delta }\mathrm{rp}}$)	−1.5	−4.5	3	−1	−5	4 (4.15)
$\delta F_{\mathrm{r}1}$($T_{\mathsf{\delta }\mathrm{Fr}}$)	10	0	10	7	0	7 (6.77)
$\delta F_{\mathrm{p}}$($T_{\mathsf{\delta }\mathrm{Fp}}$)	10	0	10	8	0	8 (7.95)
(c) Objective function
Objective function	Before optimization			After optimization
Backlash characterization coefficient	0.86			0.91
Mean value of transmission error (″)	36.03			34.17
Torsion angle (″)	105.2			95.84

, and rounded. In the optimized data, the unrounded design variables are placed in ( ), as shown in Table 7(a,b).

4.2. Analysis of Backlash before and after Optimization

According to the design variables before and after optimization, the Monte Carlo method was used to calculate the backlash of RV reducers. The results of the backlash before and after optimization are entered in

Table 8. Number and percentage of the backlash with 1.5′ before and after optimization.

	Number of the Backlash within 1.5′	Proportion
Before optimization	18,318	91.75%
After optimization	19,998	99.99%

and fitted to the curve as shown in Figure 13. As can be seen from the curve in the figure, the backlash has a normal distribution trend. Before optimization, the range of backlash is between 0.53′ and 2.06′, with a mean of 1.23′. The number of simulation experiments with deviations within 1.5′ was 18,318, accounting for 91.75%. After optimization, the range of backlash is between 0.05′ and 1.61′, with a mean of 0.81′. The number of simulation experiments with deviations within 1.5′ is 19,998, accounting for 99.99%. Based on the comparison of backlash before and after optimization, it is concluded that the maximum value of backlash after optimization has decreased by 21.84%, and the reliability of backlash accuracy has improved by 8.24%.

4.3. Analysis of Transmission Error before and after Optimization

As above, the Monte Carlo method is used to calculate the transmission error of RV reducers. Before and after the optimization, the sample data from the Monte Carlo experiments is entered in

Table 9. Number and percentage of transmission error with 1.5′ before and after optimization.

	Number of Transmission Error within 60″	Proportion
Before optimization	19,433	97.17%
After optimization	19,704	98.52%

and fitted to the curve as shown in Figure 14. The distribution curve of the transmission error value tends to be Rayleigh-distributed. Before optimization, the maximum value of transmission error can be 93.43″, the mean value is 36.03″, and there are 19,433 times within 60″, accounting for 97.17%. After optimization, the maximum value of transmission error can be 88.68″, the mean value is 34.17″, and there are 19,704 times within 60″, which is 98.52% of the time. Based on the comparison of the numerical results of the transmission error before and after the optimization, the maximum value of the transmission error is reduced by 5.08% after the optimization, the mean value is reduced by 5.16%, and the number of times it is within 1′ is improved by 1.35% compared to that before the optimization.

4.4. Analysis of Torsional Stiffness before and after Optimization

As can be seen from Table 7, before and after optimization data, the torsion angle at the output end of RV machine before optimization is 105.2″, and the torsion angle after optimization is 95.84″, which is reduced by 8.9%. This reflects that the torsional rigidity of the RV is improved after optimization, and the transmission accuracy of RV reducers is improved when the load is driven.

Based on the equivalent torsional stiffness model of the cycloid pinwheel in Section 2.3.2, the changes in the equivalent torsional stiffness of the cycloid pinwheel pair before and after optimization are obtained (Figure 15), and the equivalent torsional stiffness of each pair of teeth is filled in

Table 10. The equivalent torsional stiffness before and after optimization.

	The Equivalent Torsional Stiffness × 109 (N∙mm/rad)
	1	2	3	4	5	6	7	Summation
Before optimization	0.426	1.69	6.08	5.98	5.69	5.29	4.8	29.96
After optimization	0.773	3.46	10.17	9.89	8.37	8.66	7.85	50.15

. From the data in Figure 15 and Table 10, it can be seen that the phase angle interval of the meshing tooth pair before optimization is 18°~72°, with 7 pairs of teeth participating in engagement. The equivalent torsional stiffness for each pair of engaging teeth ranges from 4.26 × 10⁸~6.1 × 10⁹ N∙mm/rad, and the total equivalent torsional stiffness is 3 × 10¹⁰ N∙mm/rad. The phase angles of the optimized meshing pairs of teeth ranged from 18° to 72°, with seven pairs of teeth engaged. The equivalent torsional stiffness of each pair of meshing teeth ranged from 7.7 × 10⁸~1.02 × 10¹⁰ N∙mm/rad, with a total equivalent torsional stiffness of 5.01 × 10¹⁰ N∙mm/rad. The number of meshed tooth pairs remains the same after optimization, but the total equivalent torsional stiffness is increased by 67.39% compared to the pre-optimization. Consequently, the load-bearing capacity of the cycloid-pin gear mechanism is improved.

To further analyze the change in torsional stiffness, based on the calculation model for the torsional angle of the arm-bearing in Section 2.3.3, the variation in radial force on the turning arm bearing with the crankshaft angle before and after optimization is obtained (Figure 16). The maximum radial force on the turning arm bearing before optimization is 5173.1 N, and after optimization, it is reduced to 5039 N, a decrease of 2.59%. The reduction in the maximum force on the bearing indicates a decrease in the contact stress between the cylindrical rollers of the turning arm bearing and the inner and outer rings. This situation is beneficial for improving the service life of the turning arm bearing.

5. Conclusions

In this work, a multi-objective optimization model used in the manufacturing and processing parameters of the cycloid-pin gear in RV reducers has been proposed. The model is optimized for backlash, transmission error, and torsional stiffness.

(1)

With the backlash, transmission error, and torsion angle as the optimization objectives, the geometric parameters, dimensional accuracy, and modification amount of cycloid-pin gear pairs as the design variables, the multi-objective optimization model is established from the constraints of geometric parameters and the requirements of design criteria.

(2)

Based on the AGA algorithm and DE algorithm, an improved PAGA-DE algorithm is proposed. By comparing with the GA algorithm, it is concluded that the PAGA-DE algorithm has improved its solving efficiency and optimization ability, which proves that the computational efficiency and convergence accuracy of the PAGA-DE algorithm to solve the optimization model basically achieve the expected effect.

(3)

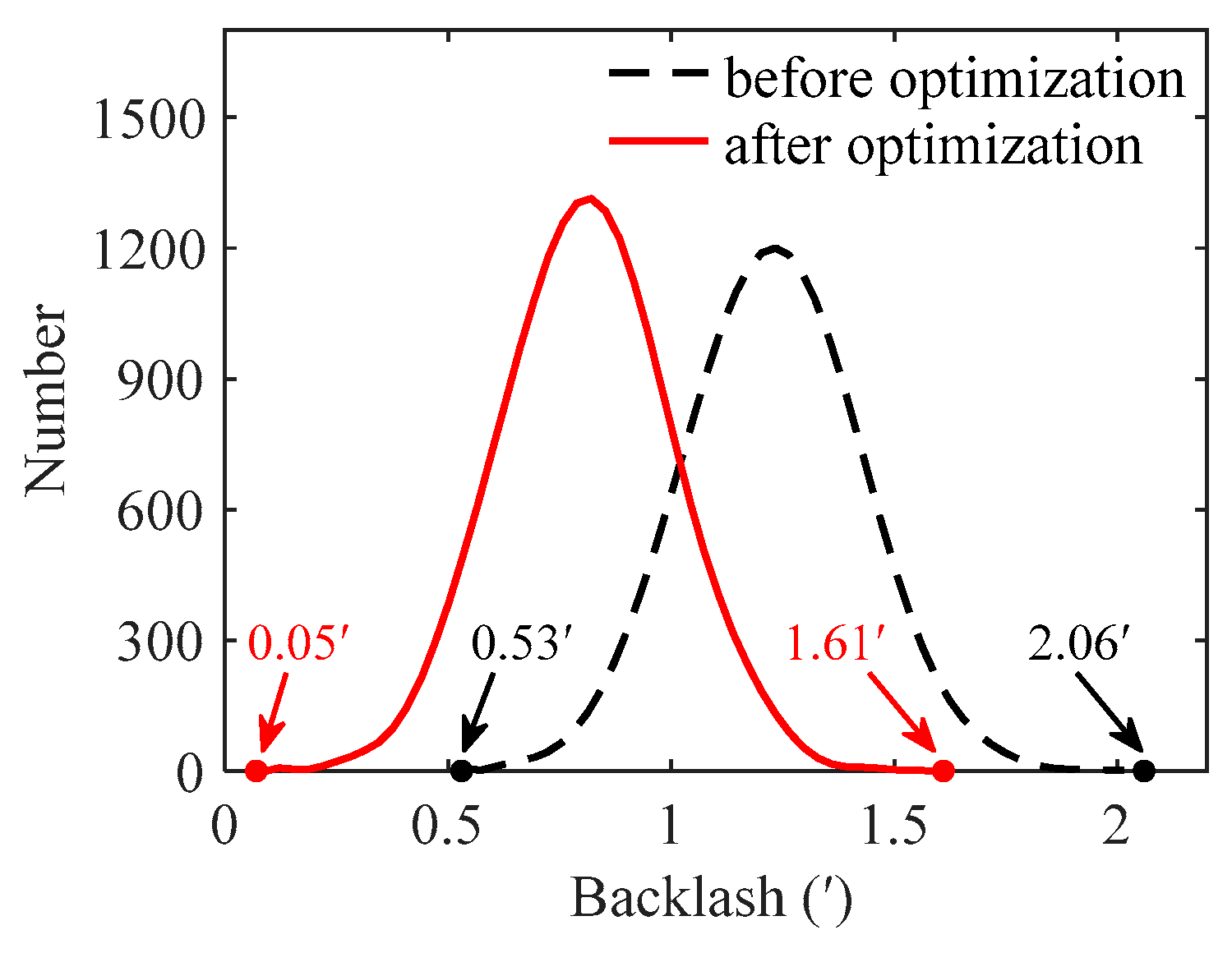
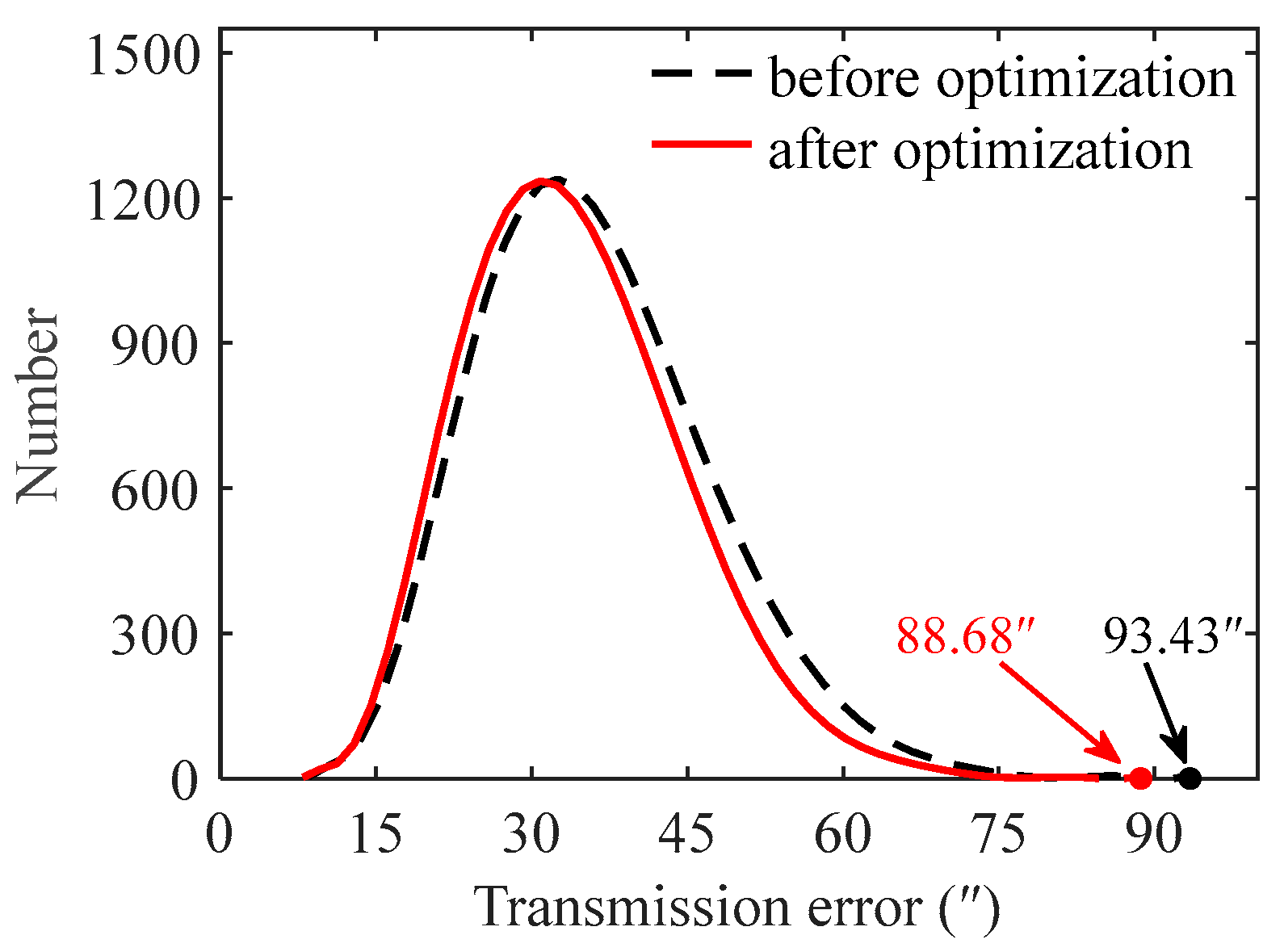
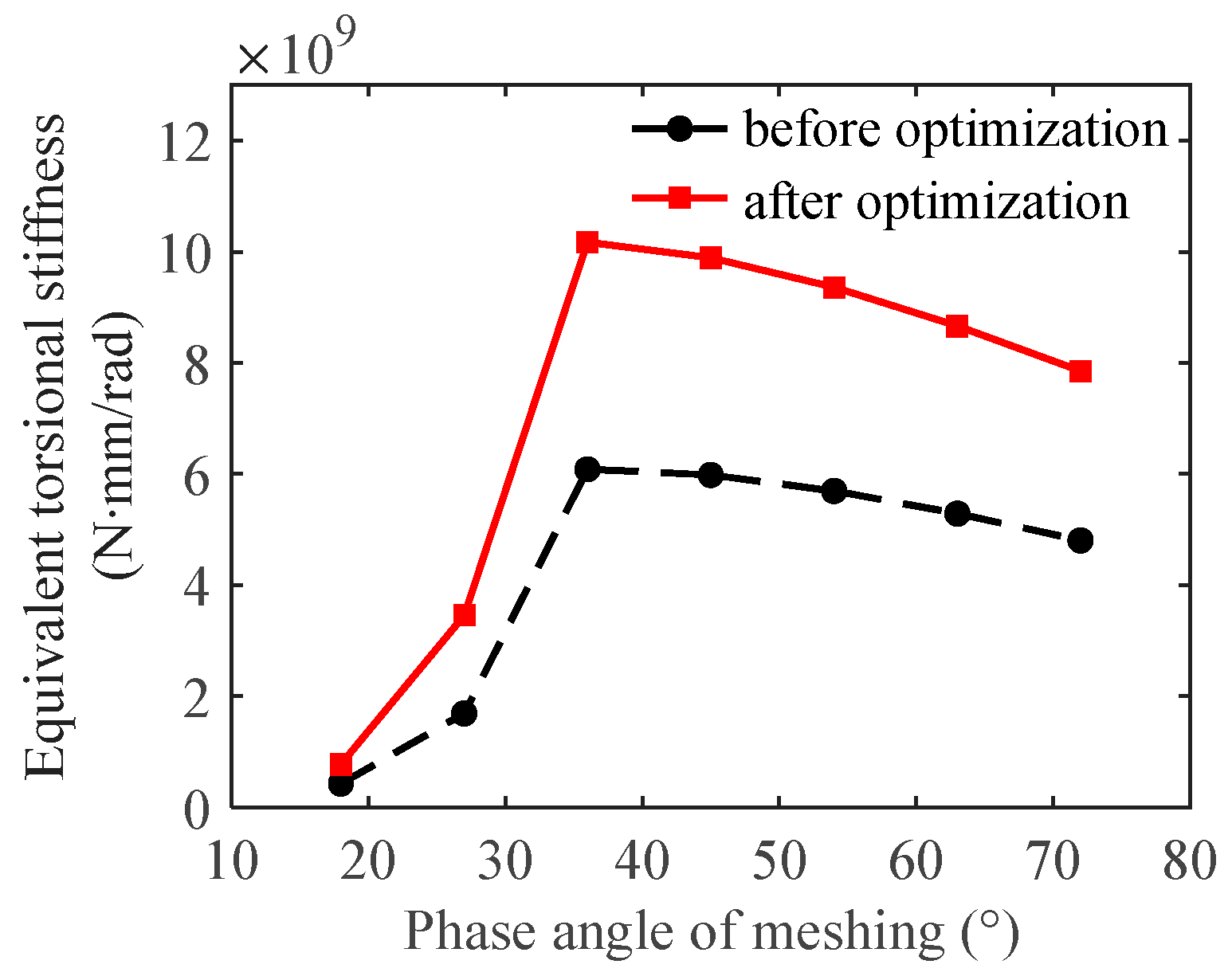
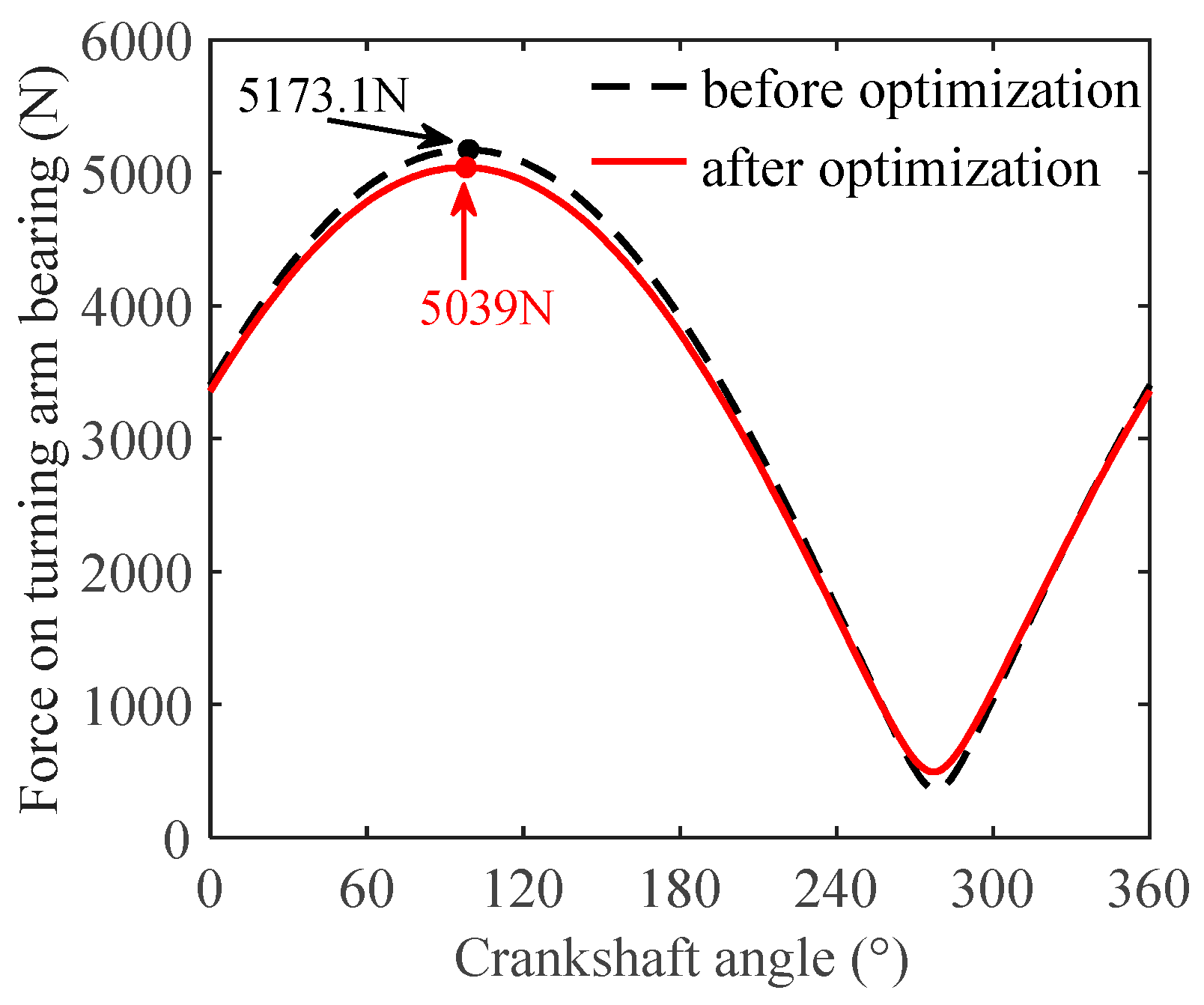
After optimization, the proportion of backlash within 1.5′ is 99.99%, and the reliability of return difference is increased by 8.24%. Transmission error within 60″ accounted for 98.52%, an increase of 1.35%. The torsion angle of the whole machine is reduced to 95.84″, which is reduced by 8.9% compared with before optimization. The driving performance and service life of RV reducers are improved. The design guidance of the macro and micro angle of the transmission system is realized. It lays a theoretical foundation for the engineering practice of precision transmission. By comparing the data of the three major performance indexes before and after optimization, the established optimization model achieves the improvement of the transmission performance of the reducer after solving, which is in line with the expected goal.

This paper has essentially achieved error control in the transmission system and provided design guidance for geometric dimensions. Moreover, the established optimization model and proposed improvement algorithm offer theoretical support for research in the optimization of reducers, providing a solid foundation for further studies.

First of all, according to the mathematical model of manufacturing and processing parameters and transmission performance established in this paper, the parameters of the cycloid-pin gear pair, the involute gear system, and the planetary carrier can be further optimized. This will enable a more comprehensive optimization design, providing more precise data support.

Secondly, on the basis of the research in this paper, more optimization objectives can be considered to establish a more comprehensive optimization model. For instance, transmission efficiency, originally treated as a constraint, can be transformed into an optimization objective. This transformation enables optimization design considering complex operating conditions such as transmission accuracy, load-bearing performance, and energy utilization efficiency. The use of a quality loss function in this paper to characterize the processing cost of dimensional accuracy may not precisely calculate actual processing costs. Therefore, introducing a processing cost function on the basis of this paper can optimize design with considerations for both cost and performance requirements.

Certainly, as the number of objective functions and design variables increases, further research is needed on how to more effectively solve the optimization model. The improved PAGA-DE algorithm in this paper makes a contribution to such research.