Age Estimation of Transmission Line Using Statistical Health Index and Failure Probability Curve-Fitting Method
Abstract
1. Introduction
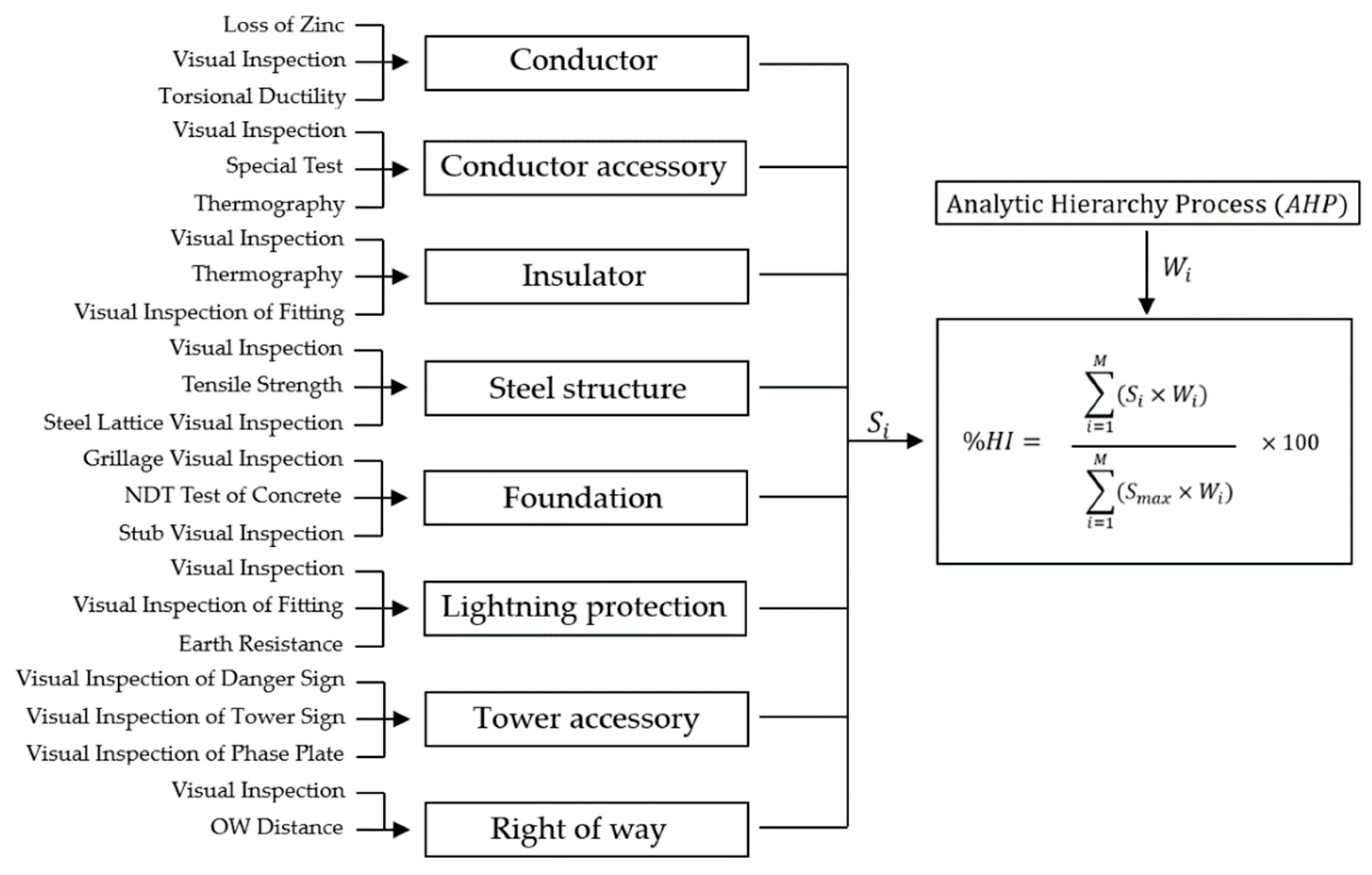
2. Health Index of Transmission Line
2.1. Condition Assessment Criteria of Transmission System
2.2. Determination Weights Using Analytic Hierarchy Process
2.3. Health Index Determination Using Scoring and Weighting Methods
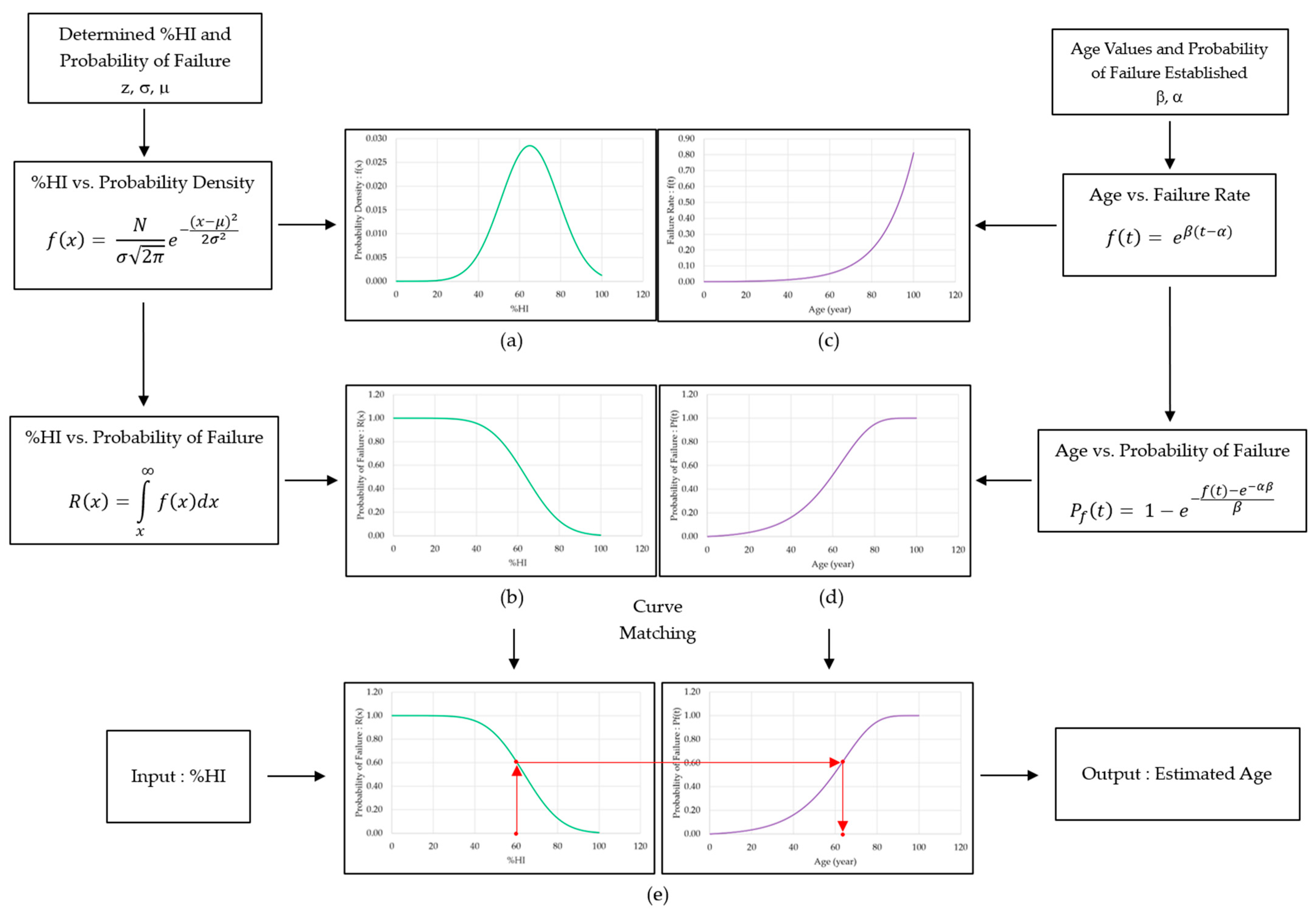
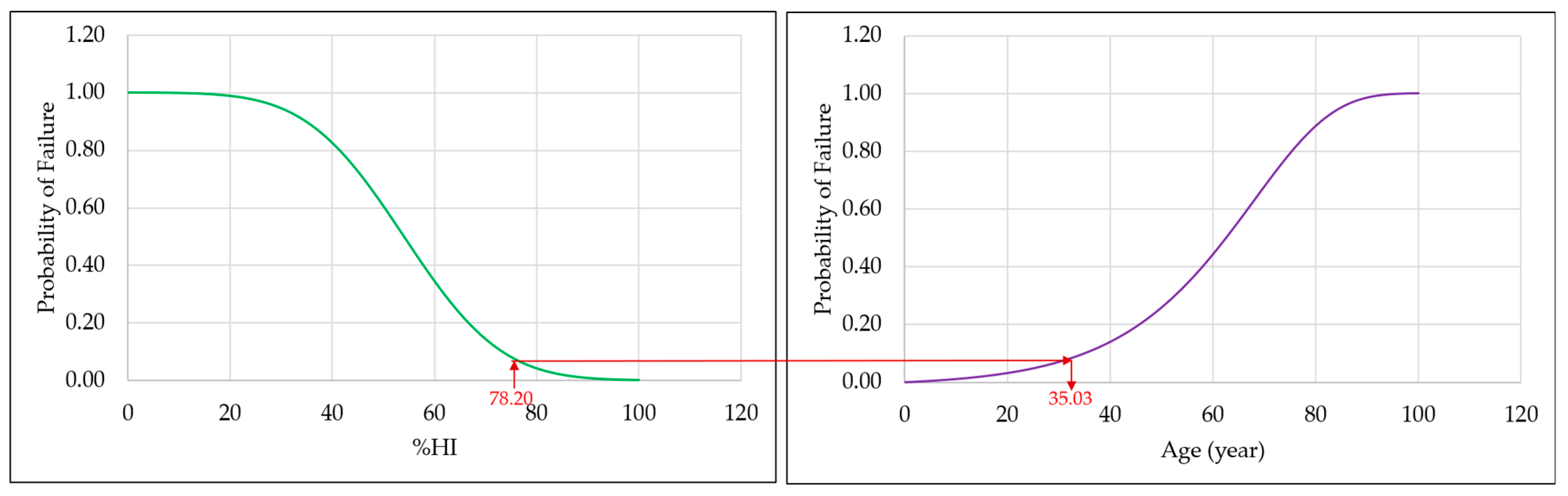
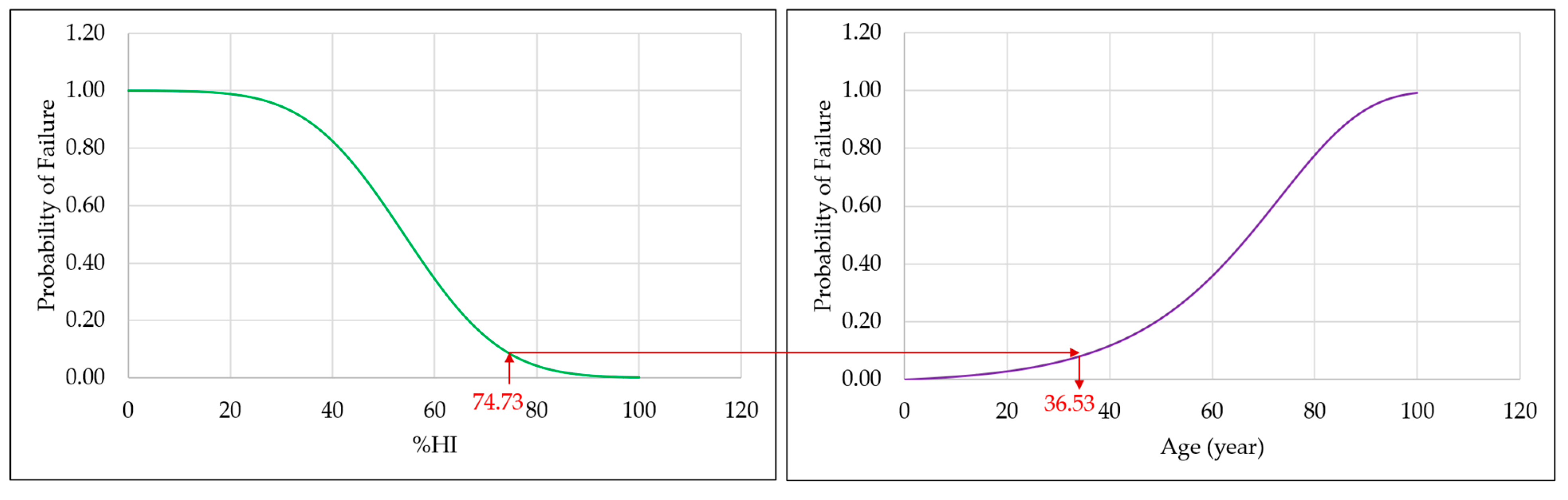
3. Age Estimation through Relative %HI to Probability of Failure Model
3.1. Health Index Curve Using Normal Distribution
3.2. Life Curve Using Gompertz–Makeham Mortality Model Based Failure Function
3.3. Single Population Mean Using Student’s t-Distribution
4. Results and Discussion
4.1. Health Index Curve Analysis Using Normal Distribution
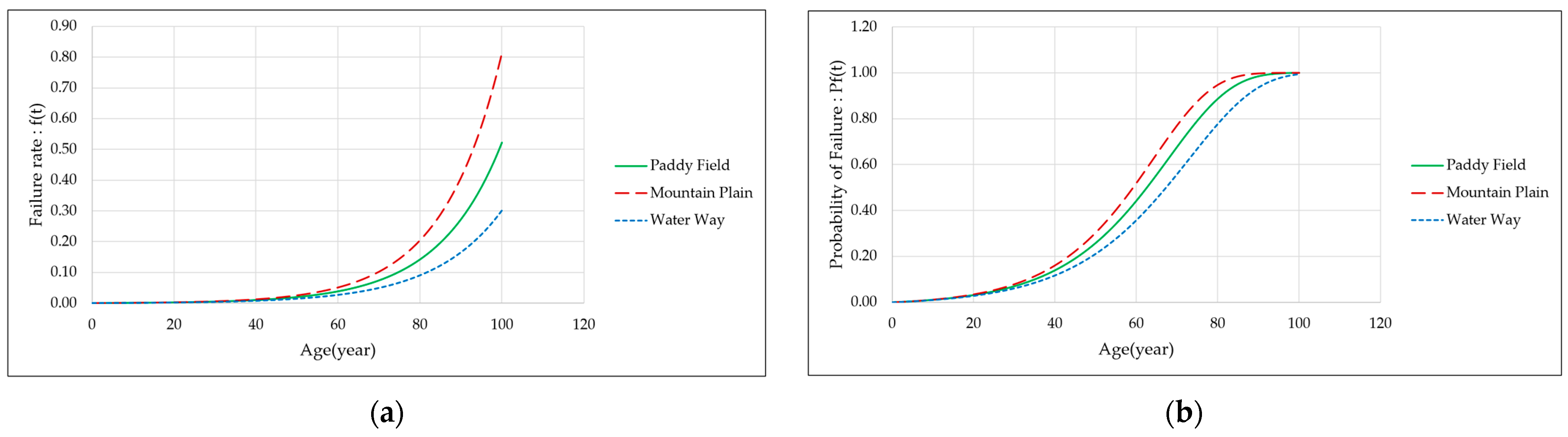
4.2. Life Curve Analysis Using Gompertz–Makeham Mortality Model
4.3. Age Analysis Using Relative Health Index to Probability of Failure Model
- (1)
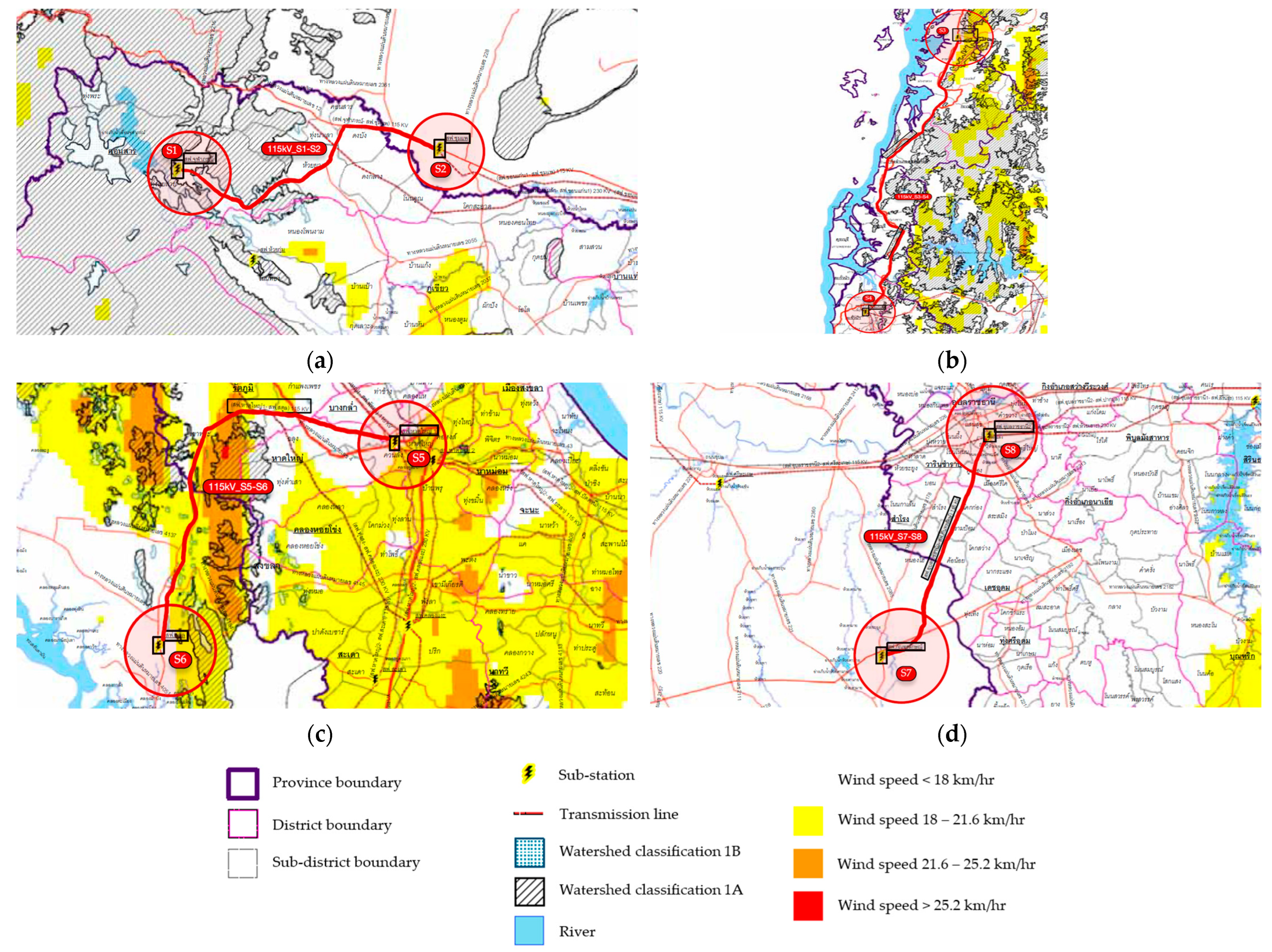
- The 115 kV S1–S2 transmission line (northeastern Thailand): This transmission line is located in a region with limestone mountains, forests, and fertile plains, as presented in Figure 10a. The results indicate that the estimated age was higher than the actual age, resulting in a high age difference, particularly in the mountainous area.
- (2)
- The 115 kV S3–S4 transmission line (southern Thailand): This transmission line is located in the southern part of Thailand, where the landscape includes a mix of coastal areas and limestone mountains, as depicted in Figure 10b. In the southern part of Thailand, where the landscape includes a mix of coastal areas and limestone mountains, the results also show a higher estimated age than the actual age, particularly in the mountainous area.
- (3)
- The 115 kV S5–S6 transmission line (southern Thailand): This transmission line is situated in a region with lower-lying landscapes, including plains and coastal areas, as illustrated in Figure 10c. The region experiences significant rainfall during the wet season, which can lead to flooding in low-lying areas and along water bodies.
- (4)
- The 115 kV S7–S8 transmission line (northeastern Thailand): In this area, the transmission line runs through paddy fields, which are characterized by standing water or flooded conditions, as shown in Figure 10d. The results show a higher estimated age than the actual age, particularly in the paddy fields. This was likely due to the challenging environmental conditions in flooded fields, which can affect the condition of transmission components.
4.4. Percentage Confidence Interval
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Suwanasri, C.; Saribut, S.; Suwanasri, T.; Phadungthin, R. Risk Analysis Using Failure Modes, Effects, and Criticality Analysis for Transmission Network Assets. Energies 2021, 14, 977. [Google Scholar] [CrossRef]
- Luejai, W.; Suwanasri, T.; Suwanasri, C. D-distance Risk Factor for Transmission Line Maintenance Management and Cost Analysis. Sustainability 2021, 13, 8208. [Google Scholar] [CrossRef]
- Van Der Wal, A.; Voncken, R.M.A.M. Extending the Service Life of Aged Overhead Line Towers; CIGRE: Paris, France, 2008. [Google Scholar]
- Grand, P. The Life Extension Policy of Overhead Lines; CIGRE: Paris, France, 2010. [Google Scholar]
- Naranpanawe, L.; Ma, H.; Saha, T.K.; Lee, C.; Ghosal, A. A Practical Health Index for Overhead Conductors: Experience from Australian Distribution Networks. IEEE Access 2020, 8, 218863–218873. [Google Scholar] [CrossRef]
- Liu, Y.; Xv, J.; Yuan, H.; Lv, J.; Ma, Z. Health Assessment and Prediction of Overhead Line Based on Health Index. IEEE Trans. Ind. Electron. 2019, 66, 5546–5557. [Google Scholar] [CrossRef]
- Foros, J.; Istad, M. Health Index, Risk and Remaining Lifetime Estimation of Power Transformers. IEEE Trans. Power Deliv. 2020, 35, 2612–2620. [Google Scholar] [CrossRef]
- Hashim, R.; Usman, F.; Baharuddin, I.N.Z. Determining health index of transmission line asset using condition-based method. Resources 2019, 8, 80. [Google Scholar] [CrossRef]
- CIGRE. Asset Management Decision Making Using Different Risk Assessment Methodologies; WG C1.25; Technical Brochure No. 541; CIGRE: Paris, France, 2013. [Google Scholar]
- Khalyasmaa, A.I.; Uteuliyev, B.A.; Tselebrovskii, Y.V. Methodology for analysing the technical state and residual life of overhead transmission lines. IEEE Trans. Power Deliv. 2021, 36, 2730–2739. [Google Scholar] [CrossRef]
- Vilanova, A.; Kim, B.-Y.; Kim, C.K.; Kim, H.-G. Linear-Gompertz Model-Based Regression of Photovoltaic Power Generation by Satellite Imagery-Based Solar Irradiance. Energies 2020, 13, 781. [Google Scholar] [CrossRef]
- Zhao, R.; Shi, X.; Wang, Q.; Su, X.; Song, X. Bayesian inference for ammunition demand based on Gompertz distribution. J. Syst. Eng. Electron. 2020, 31, 567–577. [Google Scholar]
- Makeham, W.M. On the law of mortality and the construction of annuity tables. J. Inst. Actuar. Asur. Mag. 1860, 8, 301–310. [Google Scholar] [CrossRef]
- Pham, H. Modeling U.S. mortality and risk-cost optimization on life expectancy. IEEE Trans. Reliab. 2011, 60, 125–133. [Google Scholar] [CrossRef]
- CIGRE. International Survey of Component Costs of Overhead Transmission Lines; Cigre Electra No. 137; CIGRE: Paris, France, 1991. [Google Scholar]
- Brennan, G.F. Refurbishment of Existing Overhead Transmission Lines; CIGRE: Paris, France, 2004. [Google Scholar]
- Gharakheili, M.A.; Fotuhi-Firuzabad, M.; Dehghanian, P. A new multiattribute decision making support tool for identifying critical components in power transmission systems. IEEE Syst. J. 2018, 12, 316–327. [Google Scholar] [CrossRef]
- Saaty, T.L.; Vargas, L.G. Models, Methods, Concept and Applications of the Analytic Hierarchy Process, 2nd ed.; Springer: New York, NY, USA, 2012. [Google Scholar]
- Tanaka, H.; Tsukao, S.; Yamashita, D.; Niimura, T.; Yokoyama, R. Multiple criteria assessment of substation conditions by pair-wise comparison of analytic hierarchy process. IEEE Trans. Power Deliv. 2010, 25, 3017–3023. [Google Scholar] [CrossRef]
- Yongyee, I.; Suwanasri, C.; Suwanasri, T.; Luejai, W. Condition assessment in transmission line asset for maintenance management. In Proceedings of the 2018 International Electrical Engineering Congress (iEECON), Krabi, Thailand, 7–9 March 2018. [Google Scholar]
- The Planning, Design and Construction of Overhead Power Lines; Eskom Power Series; Eskom: Johannesburg, South Africa, 2005; Volume 1.
- The Fundamentals and Practice of Overhead Line Maintenance; Eskom Power Series; Eskom: Johannesburg, South Africa, 2005; Volume 2.
- CIGRE: WG B2.03: Guide for the Assessment of Old Cap & Pin and Long-Rod Transmission Line Insulators Made of Porcelain or Glass: What to Check and When to Replace? Cigre Technical Brochure; Reference: 306; CIGRE: Paris, France, 2006.
- Garcia-Alamo, C.J.; Palacios, J. Structural Analysis for Transmission Lattice Steel Tower in the 400 kV Transmission Lines EL Tablazo—Cuatricenteneario No.1 and 2; CIGRE: Paris, France, 2010. [Google Scholar]
- Chen, C.; Jia, Z.; Ye, W.; Guan, Z.; Li, Y. Condition assessment strategies of composite insulator based on statistic methods. IEEE Trans. Dielectr. Electr. Insul. 2016, 23, 3231–3241. [Google Scholar] [CrossRef]
- Luejai, W.; Suwanasri, T.; Suwanasri, C. Condition assessment of overhead transmission line using weighting and scoring method and IT application. J. Electr. Eng. Technol. 2021, 16, 2981–2993. [Google Scholar] [CrossRef]
- Jahromi, A.; Piercy, R.; Cress, S.; Service, J.; Fan, W. An approach to power transformer asset management using health index. IEEE Electr. Insul. Mag. 2009, 25, 20–34. [Google Scholar] [CrossRef]
- Tsimberg, Y.; Lotho, K.; Dimnik, C.; Wrathall, N.; Mogilevsky, A. Determining transmission line conductor condition and remaining life. In Proceedings of the 2014 IEEE PES T&D Conference and Exposition, Chicago, IL, USA, 14–17 April 2014. [Google Scholar]
- Li, X.; Ma, X.; Xiao, F.; Wang, F.; Zhang, S. Application of Gated Recurrent Unit (GRU) Neural Network for Smart Batch Production Prediction. Energies 2020, 13, 6121. [Google Scholar] [CrossRef]
- Yu, X.; Zhang, W.; Zang, H.; Yang, H. Wind Power Interval Forecasting Based on Confidence Interval Optimization. Energies 2018, 11, 3336. [Google Scholar] [CrossRef]
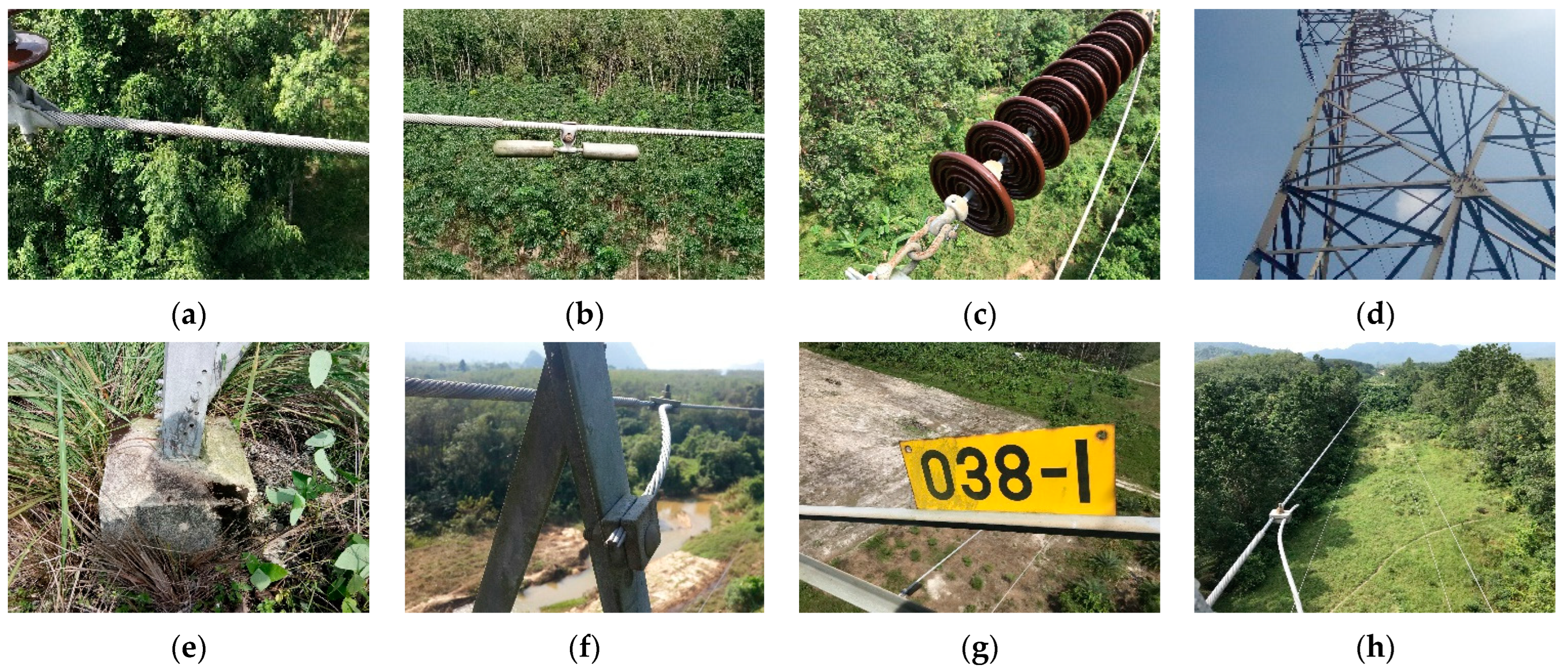



| Group | Sub-Components | Testing Method |
|---|---|---|
| Conductor | Conductor | Visual inspection, loss of zinc, tensile strength, torsional ductility |
| Conductor Accessory | Damper | Visual inspection |
| Spacer | Visual inspection, special test | |
| Dead end | Resistance, thermography | |
| Joint | Resistance | |
| PG clamp | Thermography | |
| Insulator | Insulator | Visual inspection, visual inspection of fitting, thermography |
| Steel Structure | Tower | Monopole visual inspection, concrete pole visual inspection, steel lattice visual inspection |
| Anchor and guy | Visual inspection, tensile strength | |
| Foundation | Foundation | Grillage visual inspection, concrete visual inspection, NDT test of concrete, stub visual inspection |
| Lightning Protection | OHGW/OPGW | Visual inspection, loss of zinc, tensile strength, visual inspection of fitting |
| Marker ball | Visual inspection | |
| Grounding system | Earth resistance, visual inspection | |
| Tower Accessory | Danger sign | Visual inspection |
| Phase plate | Visual inspection | |
| Right of Way | Right of way | Visual inspection, OW distance |
| Zone | %HI | Risk | Probability of Failure | z | μ | σ |
|---|---|---|---|---|---|---|
| Paddy field | 45 | Very high | 80% | 0.85 | 57.57 | 14.79 |
| 54 | High | 60% | 0.26 | |||
| 62 | Medium | 40% | −1.75 | |||
| 70 | Low | 20% | −0.84 | |||
| Mountain plain | 52 | Very high | 80% | 0.85 | 63.57 | 13.61 |
| 60 | High | 60% | 0.26 | |||
| 67 | Medium | 40% | −1.75 | |||
| 75 | Low | 20% | −0.84 | |||
| Water way | 41 | Very high | 80% | 0.85 | 53.57 | 14.79 |
| 50 | High | 60% | 0.26 | |||
| 58 | Medium | 40% | −1.75 | |||
| 66 | Low | 20% | −0.84 |
| %HI | Paddy Field | Mountain Plain | Water Way | |||
|---|---|---|---|---|---|---|
| f(x) | R(x) | f(x) | R(x) | f(x) | R(x) | |
| 0 | 0.000012 | 0.999987 | 0.000001 | 0.999999 | 0.000032 | 0.999964 |
| 5 | 0.000041 | 0.999836 | 0.000003 | 0.999989 | 0.000103 | 0.999577 |
| 10 | 0.000128 | 0.999352 | 0.000013 | 0.999944 | 0.000295 | 0.998441 |
| 15 | 0.000360 | 0.997961 | 0.000048 | 0.999768 | 0.000760 | 0.995449 |
| 20 | 0.000906 | 0.994378 | 0.000163 | 0.999161 | 0.001748 | 0.988393 |
| 25 | 0.002038 | 0.986110 | 0.000481 | 0.997321 | 0.003599 | 0.973482 |
| 30 | 0.004104 | 0.969020 | 0.001252 | 0.992401 | 0.006632 | 0.945258 |
| 35 | 0.007395 | 0.937375 | 0.002869 | 0.980801 | 0.010934 | 0.897402 |
| 40 | 0.011924 | 0.884886 | 0.005786 | 0.956697 | 0.016131 | 0.824710 |
| 45 | 0.017205 | 0.806892 | 0.010271 | 0.912542 | 0.021297 | 0.725796 |
| 50 | 0.022215 | 0.703072 | 0.016051 | 0.841244 | 0.025159 | 0.605223 |
| 55 | 0.025667 | 0.579273 | 0.022080 | 0.739755 | 0.026596 | 0.473557 |
| 60 | 0.026537 | 0.447027 | 0.026735 | 0.612407 | 0.025159 | 0.344756 |
| 65 | 0.024551 | 0.320473 | 0.028496 | 0.471542 | 0.021297 | 0.231884 |
| 70 | 0.020326 | 0.211983 | 0.026735 | 0.334186 | 0.016131 | 0.143274 |
| 75 | 0.015058 | 0.128667 | 0.022080 | 0.216117 | 0.010934 | 0.080958 |
| 80 | 0.009982 | 0.071349 | 0.016051 | 0.126652 | 0.006632 | 0.041699 |
| 85 | 0.005921 | 0.036025 | 0.010271 | 0.066892 | 0.003599 | 0.019542 |
| 90 | 0.003143 | 0.016523 | 0.005786 | 0.031703 | 0.001748 | 0.008341 |
| 95 | 0.001493 | 0.006878 | 0.002869 | 0.013439 | 0.000760 | 0.003268 |
| 100 | 0.000635 | 0.002605 | 0.001252 | 0.005082 | 0.000295 | 0.001210 |
| Zone | Age (Years) | Risk | Probability of Failure | β | α |
|---|---|---|---|---|---|
| Paddy field | 75 | Very high | 80% | 0.065 | 110 |
| 67 | High | 60% | |||
| 58 | Medium | 40% | |||
| 46 | Low | 20% | |||
| Mountain plain | 71 | Very high | 80% | 0.069 | 103 |
| 63 | High | 60% | |||
| 55 | Medium | 40% | |||
| 44 | Low | 20% | |||
| Water way | 81 | Very high | 80% | 0.060 | 120 |
| 72 | High | 60% | |||
| 62 | Medium | 40% | |||
| 49 | Low | 20% |
| Age (Years) | Paddy Field | Mountain Plain | Water Way | |||
|---|---|---|---|---|---|---|
| f(t) | Pf(t) | f(t) | Pf(t) | f(t) | Pf(t) | |
| 0 | 0.000785 | 0.000000 | 0.000819 | 0.000000 | 0.000747 | 0.000000 |
| 5 | 0.001086 | 0.004626 | 0.001157 | 0.004880 | 0.001008 | 0.004344 |
| 10 | 0.001503 | 0.010994 | 0.001634 | 0.011731 | 0.001360 | 0.010178 |
| 15 | 0.002081 | 0.019740 | 0.002307 | 0.021323 | 0.001836 | 0.017998 |
| 20 | 0.002880 | 0.031717 | 0.003257 | 0.034709 | 0.002479 | 0.028457 |
| 25 | 0.003986 | 0.048053 | 0.004599 | 0.053299 | 0.003346 | 0.042398 |
| 30 | 0.005517 | 0.070209 | 0.006493 | 0.078940 | 0.004517 | 0.060900 |
| 35 | 0.007635 | 0.100025 | 0.009168 | 0.113966 | 0.006097 | 0.085309 |
| 40 | 0.010567 | 0.139720 | 0.012946 | 0.161166 | 0.008230 | 0.117255 |
| 45 | 0.014625 | 0.191788 | 0.018279 | 0.223562 | 0.011109 | 0.158616 |
| 50 | 0.020242 | 0.258692 | 0.025810 | 0.303844 | 0.014996 | 0.211390 |
| 55 | 0.028015 | 0.342251 | 0.036443 | 0.403268 | 0.020242 | 0.277416 |
| 60 | 0.038774 | 0.442588 | 0.051457 | 0.519960 | 0.027324 | 0.357862 |
| 65 | 0.053665 | 0.556712 | 0.072657 | 0.646945 | 0.036883 | 0.452436 |
| 70 | 0.074274 | 0.677159 | 0.102592 | 0.771212 | 0.049787 | 0.558396 |
| 75 | 0.102797 | 0.791833 | 0.144858 | 0.876006 | 0.067206 | 0.669665 |
| 80 | 0.142274 | 0.886591 | 0.204538 | 0.947788 | 0.090718 | 0.776763 |
| 85 | 0.196912 | 0.951069 | 0.288806 | 0.984605 | 0.122456 | 0.868467 |
| 90 | 0.272532 | 0.984713 | 0.407791 | 0.997255 | 0.165299 | 0.935593 |
| 95 | 0.377192 | 0.996945 | 0.575797 | 0.999760 | 0.223130 | 0.975434 |
| 100 | 0.522046 | 0.999671 | 0.813020 | 0.999992 | 0.301194 | 0.993312 |
| HVTL Tower’s Name | Zone | %HI | Actual Age | Estimated Age | Age Difference | HVTL Tower’s Name | Zone | %HI | Actual Age | Estimated Age | Age Difference |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 115 kV S1–S2#10 | Mountain plain | 71.80 | 40 | 50.52 | +10.52 | 115 kV S5–S6#20 | Mountain plain | 69.17 | 35 | 54.04 | +19.04 |
| 115 kV S1–S2#20 | Mountain plain | 77.64 | 40 | 41.92 | +1.92 | 115 kV S5–S6#40 | Mountain plain | 68.10 | 35 | 55.40 | +20.40 |
| 115 kV S1–S2#30 | Paddy field | 74.42 | 40 | 41.31 | +1.31 | 115 kV S5–S6#60 | Water way | 76.77 | 35 | 32.73 | −2.27 |
| 115 kV S1–S2#40 | Paddy field | 71.88 | 40 | 45.30 | +5.30 | 115 kV S5–S6#80 | Mountain plain | 68.42 | 35 | 55.00 | +2.00 |
| 115 kV S1–S2#50 | Mountain plain | 70.45 | 40 | 52.35 | +12.35 | 115 kV S5–S6#100 | Water way | 76.16 | 35 | 33.89 | −1.11 |
| 115 kV S1–S2#60 | Mountain plain | 74.20 | 40 | 47.12 | +7.12 | 115 kV S5–S6#120 | Mountain plain | 70.74 | 35 | 51.98 | +16.98 |
| 115 kV S1–S2#70 | Paddy field | 78.94 | 40 | 33.76 | −6.24 | 115 kV S5–S6#140 | Mountain plain | 82.21 | 35 | 34.42 | −0.58 |
| 115 kV S1–S2#80 | Paddy field | 74.82 | 40 | 40.64 | +0.64 | 115 kV S5–S6#160 | Mountain plain | 73.53 | 35 | 48.10 | +13.10 |
| 115 kV S1–S2#90 | Paddy field | 76.78 | 40 | 37.42 | −2.58 | 115 kV S5–S6#180 | Mountain plain | 78.03 | 35 | 41.32 | +6.32 |
| 115 kV S1–S2#100 | Paddy field | 77.04 | 40 | 37.00 | −3.00 | 115 kV S5–S6#200 | Mountain plain | 82.64 | 35 | 33.70 | −1.30 |
| 115 kV S3–S4#38 | Mountain plain | 71.16 | 48 | 51.40 | +3.40 | 115 kV S7–S8#18 | Paddy field | 76.32 | 22 | 38.20 | +16.20 |
| 115 kV S3–S4#76 | Water way | 74.50 | 48 | 36.96 | −11.04 | 115 kV S7–S8#36 | Paddy field | 96.59 | 22 | 6.35 | −15.65 |
| 115 kV S3–S4#114 | Mountain plain | 62.99 | 48 | 61.49 | +13.49 | 115 kV S7–S8#41 | Water way | 87.30 | 22 | 13.72 | −8.28 |
| 115 kV S3–S4#152 | Water way | 75.31 | 48 | 35.47 | −12.53 | 115 kV S7–S8#72 | Paddy field | 70.52 | 22 | 47.34 | +25.34 |
| 115 kV S3–S4#190 | Mountain plain | 77.26 | 48 | 42.53 | −5.47 | 115 kV S7–S8#90 | Paddy field | 81.40 | 22 | 29.49 | +7.49 |
| 115 kV S3–S4#228 | Mountain plain | 75.61 | 48 | 45.05 | −2.95 | 115 kV S7–S8#104 | Mountain plain | 96.59 | 22 | 10.18 | −11.82 |
| 115 kV S3–S4#266 | Water way | 74.16 | 48 | 37.58 | −10.42 | 115 kV S7–S8#126 | Paddy field | 88.17 | 22 | 17.77 | −4.23 |
| 115 kV S3–S4#304 | Mountain plain | 65.41 | 48 | 58.69 | +10.69 | 115 kV S7–S8#143 | Water way | 90.71 | 22 | 8.90 | −13.10 |
| 115 kV S3–S4#342 | Mountain plain | 75.61 | 48 | 45.05 | −2.95 | 115 kV S7–S8#162 | Paddy field | 88.17 | 22 | 17.77 | −4.23 |
| 115 kV S3–S4#380 | Mountain plain | 79.60 | 48 | 38.79 | −9.21 | 115 kV S7–S8#180 | Mountain plain | 88.17 | 22 | 23.95 | +1.95 |
| Details | HVTL’s Name | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| 115 kV S1–S2 | 115 kV S3–S4 | 115 kV S5–S6 | 115 kV S7–S8 | ||||||
| Paddy Field | Mountain Plain | Mountain Plain | Water Way | Mountain Plain | Water Way | Paddy Field | Mountain Plain | Water Way | |
| n | 88 | 47 | 360 | 20 | 141 | 77 | 153 | 13 | 17 |
| s | 5.40 | 5.20 | 8.85 | 13.77 | 10.14 | 11.63 | 14.11 | 9.09 | 9.78 |
| t(α/2) | 1.987 | 2.015 | 1.984 | 2.093 | 1.984 | 1.992 | 1.984 | 2.160 | 2.110 |
| EBM | 1.14 | 1.53 | 0.93 | 6.45 | 1.69 | 2.64 | 2.26 | 5.45 | 5.00 |
| 37.78 | 45.72 | 51.92 | 41.68 | 46.84 | 37.20 | 18.07 | 20.67 | 10.29 | |
| 95% confidence interval | 36.63–38.92 | 44.19–47.25 | 50.99–52.85 | 35.23–48.12 | 45.14–48.53 | 34.56–39.84 | 15.80–20.33 | 15.23–26.12 | 5.29–15.30 |
| Details | HVTL’s Name | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| 115 kV S1–S2 | 115 kV S3–S4 | 115 kV S5–S6 | 115 kV S7–S8 | ||||||
| Paddy Field | Mountain Plain | Mountain Plain | Water Way | Mountain Plain | Water Way | Paddy Field | Mountain Plain | Water Way | |
| No. of towers (towers) | 88 | 47 | 360 | 20 | 141 | 77 | 153 | 13 | 17 |
| Actual age (years) | 40 | 40 | 48 | 48 | 35 | 35 | 22 | 22 | 22 |
| Average age (years) | 37.78 | 45.72 | 51.92 | 41.68 | 46.84 | 37.20 | 18.07 | 20.67 | 10.29 |
| Standard deviation | 5.40 | 5.20 | 8.85 | 13.77 | 10.14 | 11.63 | 14.11 | 9.09 | 9.78 |
| Median | 36.93 | 45.99 | 52.87 | 41.60 | 47.03 | 37.34 | 15.13 | 19.53 | 8.90 |
| Mode | 33.76 | 41.92 | 45.05 | - | 50.48 | 24.78 | 3.59 | 19.48 | 1.86 |
| 95% confidence interval | 36.63–38.92 | 44.19–47.25 | 50.99–52.85 | 35.23–48.12 | 45.14–48.53 | 34.56–39.84 | 15.80–20.33 | 15.23–26.12 | 5.29–15.30 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Suwanasri, C.; Yongyee, I.; Suwanasri, T. Age Estimation of Transmission Line Using Statistical Health Index and Failure Probability Curve-Fitting Method. Energies 2024, 17, 637. https://doi.org/10.3390/en17030637
Suwanasri C, Yongyee I, Suwanasri T. Age Estimation of Transmission Line Using Statistical Health Index and Failure Probability Curve-Fitting Method. Energies. 2024; 17(3):637. https://doi.org/10.3390/en17030637
Chicago/Turabian StyleSuwanasri, Cattareeya, Ittiphong Yongyee, and Thanapong Suwanasri. 2024. "Age Estimation of Transmission Line Using Statistical Health Index and Failure Probability Curve-Fitting Method" Energies 17, no. 3: 637. https://doi.org/10.3390/en17030637
APA StyleSuwanasri, C., Yongyee, I., & Suwanasri, T. (2024). Age Estimation of Transmission Line Using Statistical Health Index and Failure Probability Curve-Fitting Method. Energies, 17(3), 637. https://doi.org/10.3390/en17030637








