Power Loss Modelling and Performance Comparison of Three-Level GaN-Based Inverters Used for Electric Traction
Abstract
1. Introduction
- –
- Reduced dv/dt stress on the electric load;
- –
- Reduced common mode voltages;
- –
- Enhanced freedom to control the output waveform;
- –
- Mitigation of Total Harmonic Distortion;
- –
- Lower stress on the power switches;
- –
- Improved fault-tolerance capability.
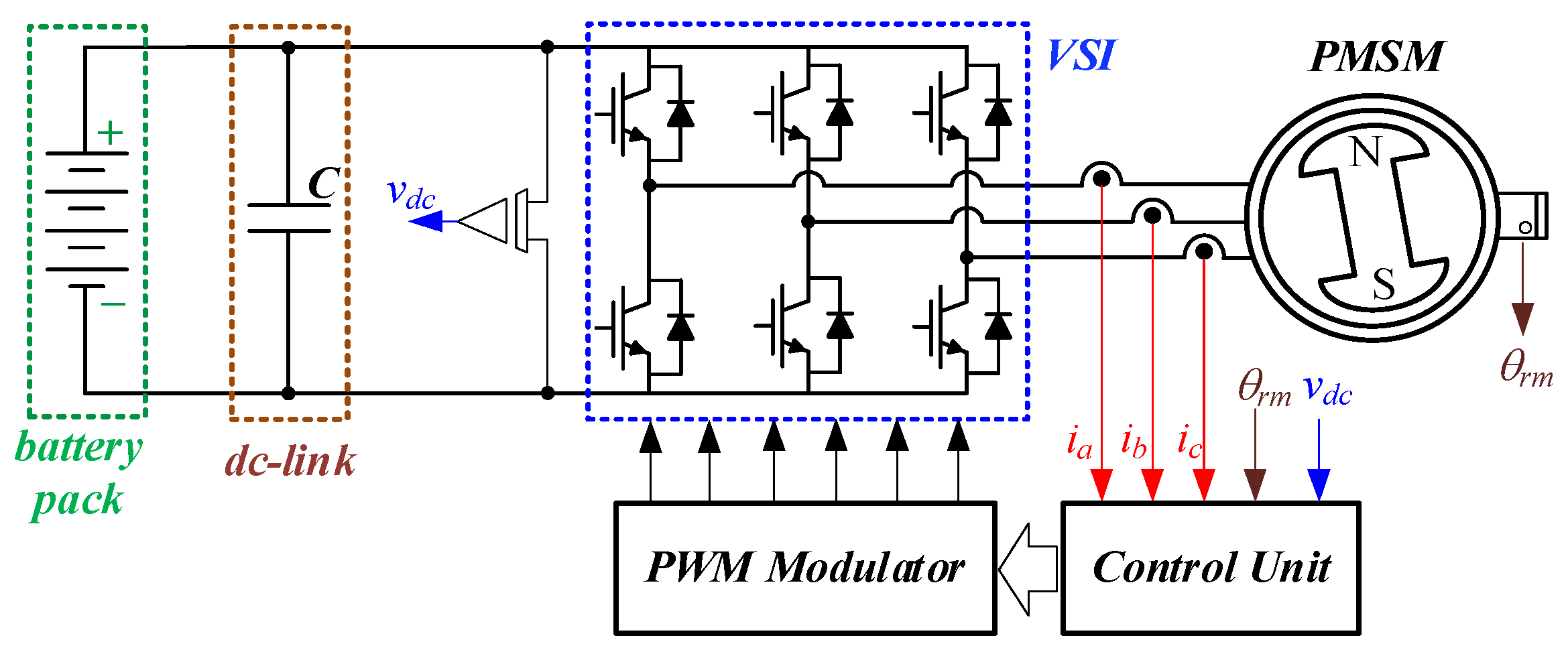
2. Electric Traction Drive Description
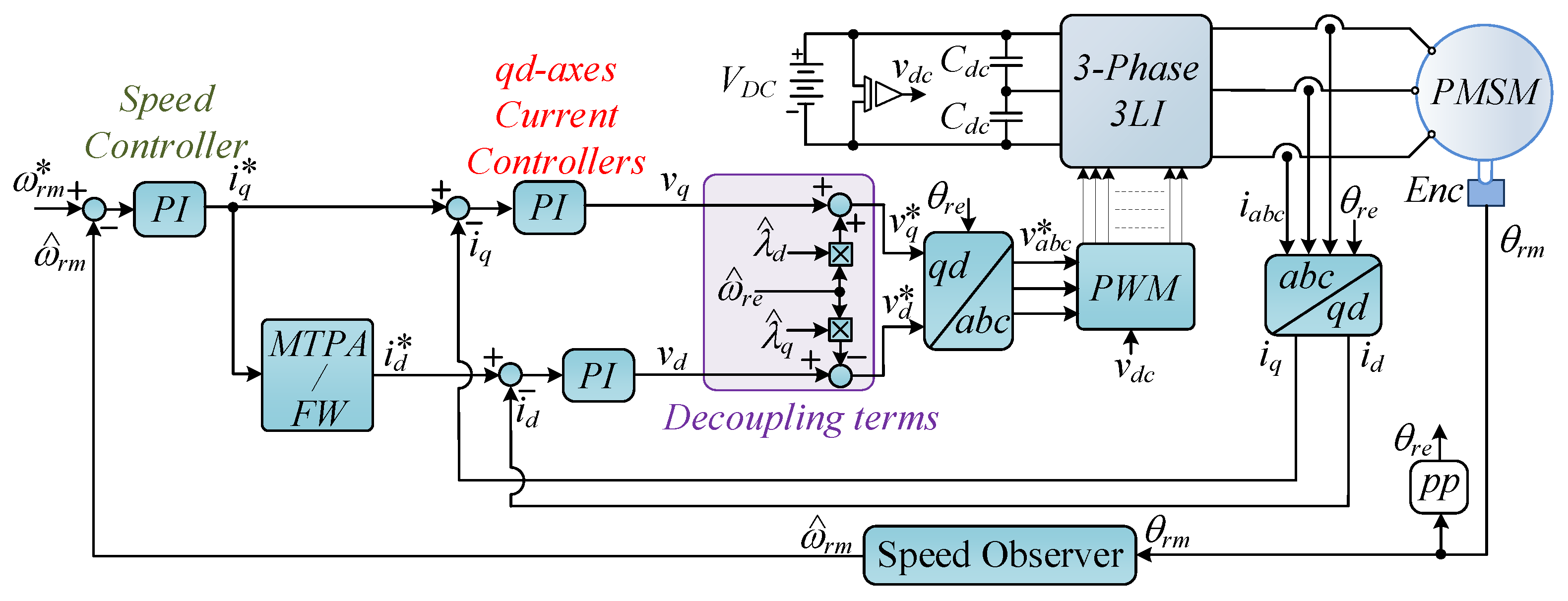
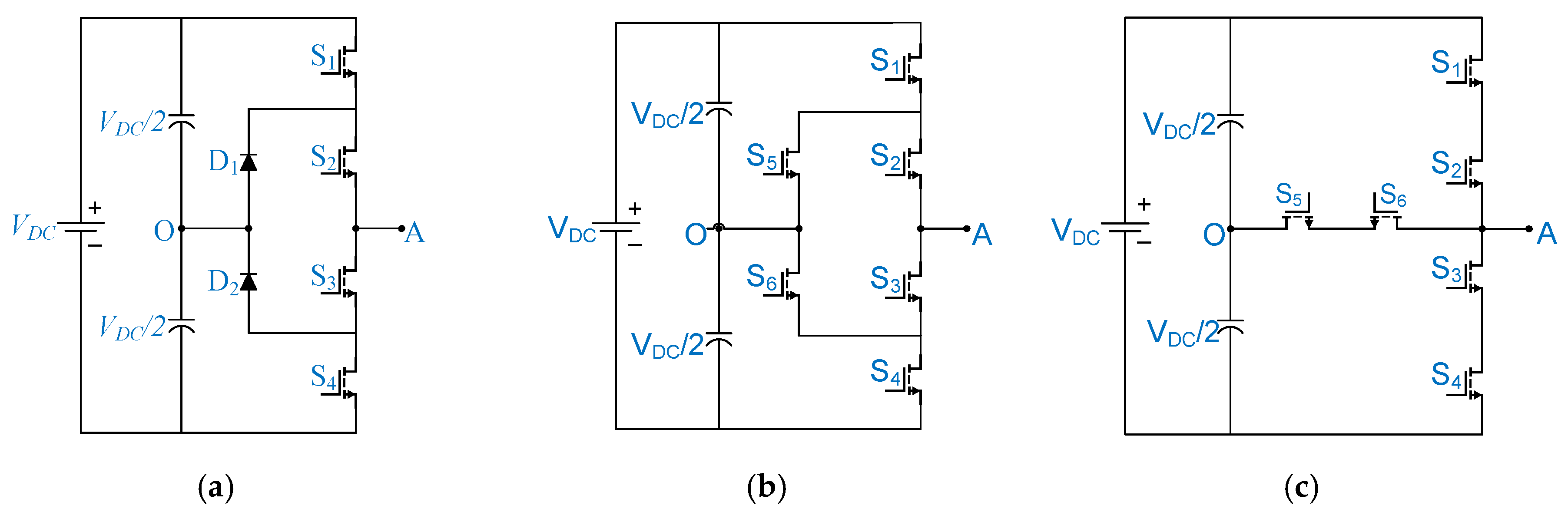
3. Methodology for the Estimation of Power Loss Distribution in GaN-Based 3LI
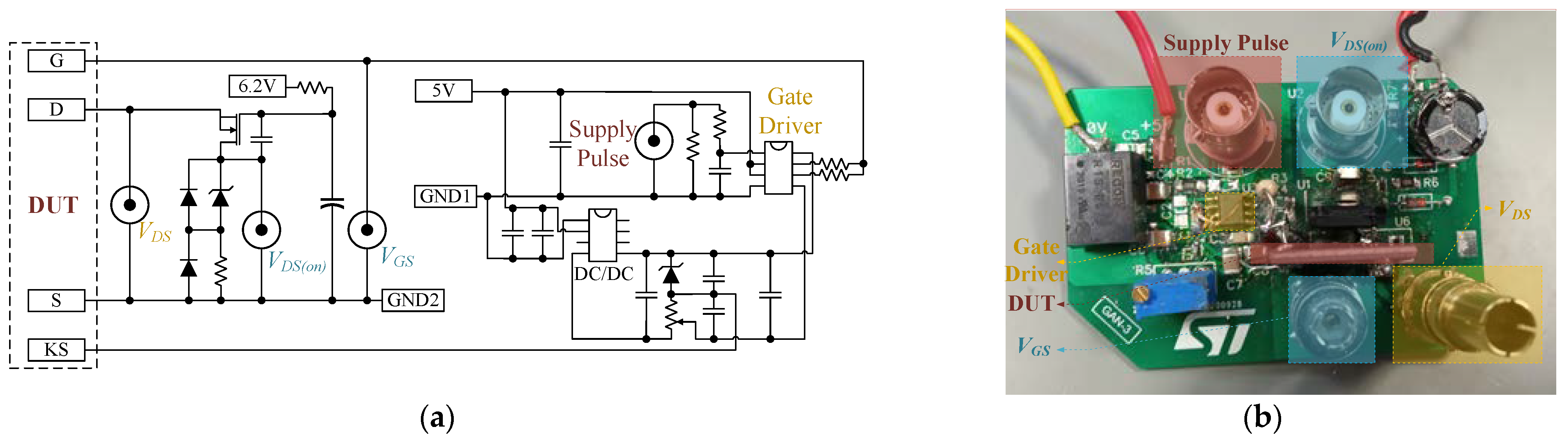
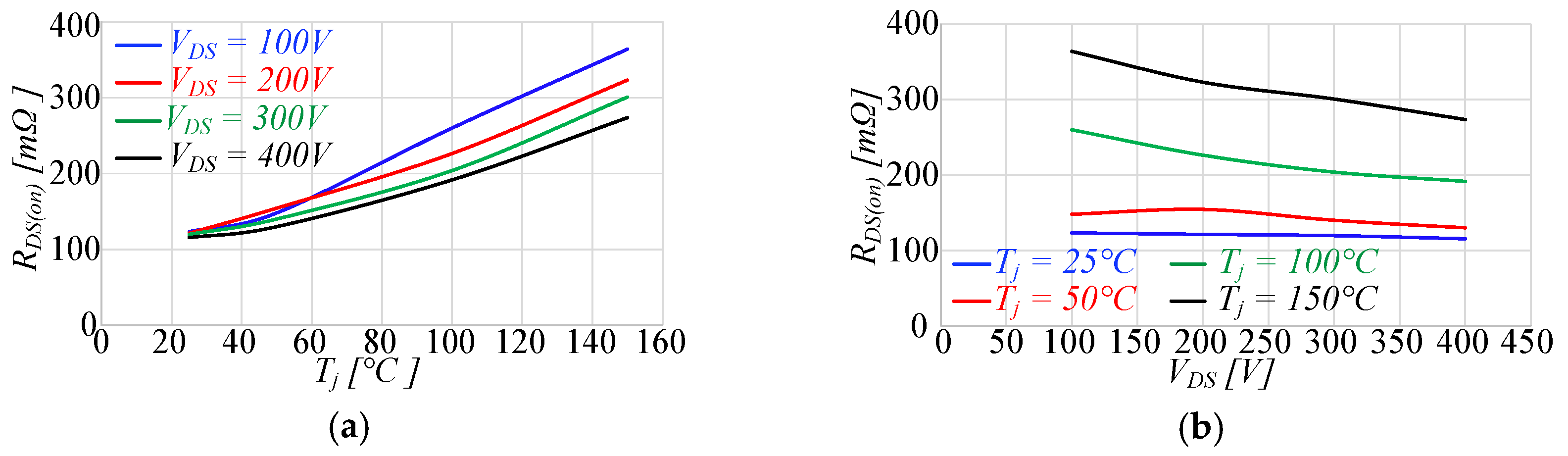
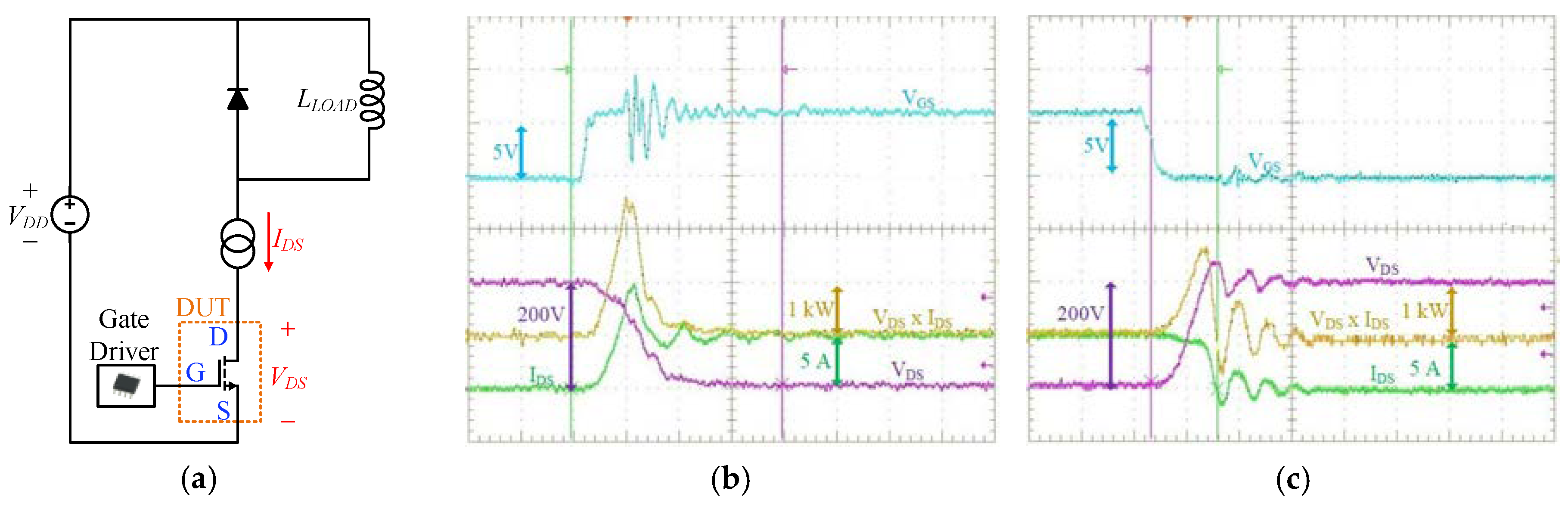
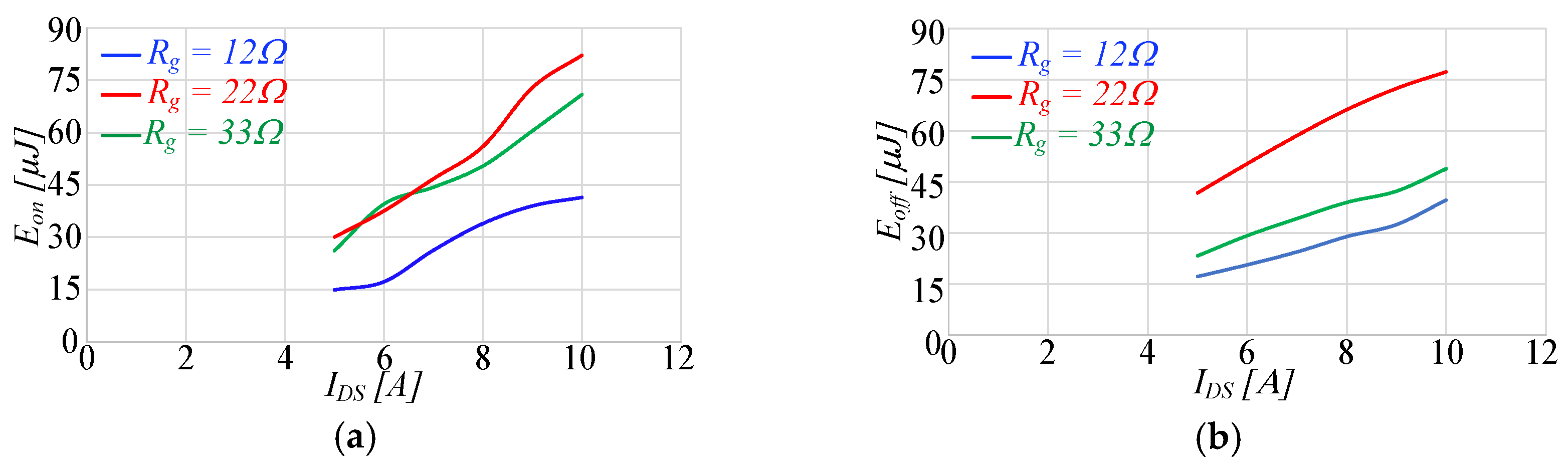
3.1. Characterization of GaN MOSFETs
3.1.1. Static Characterization
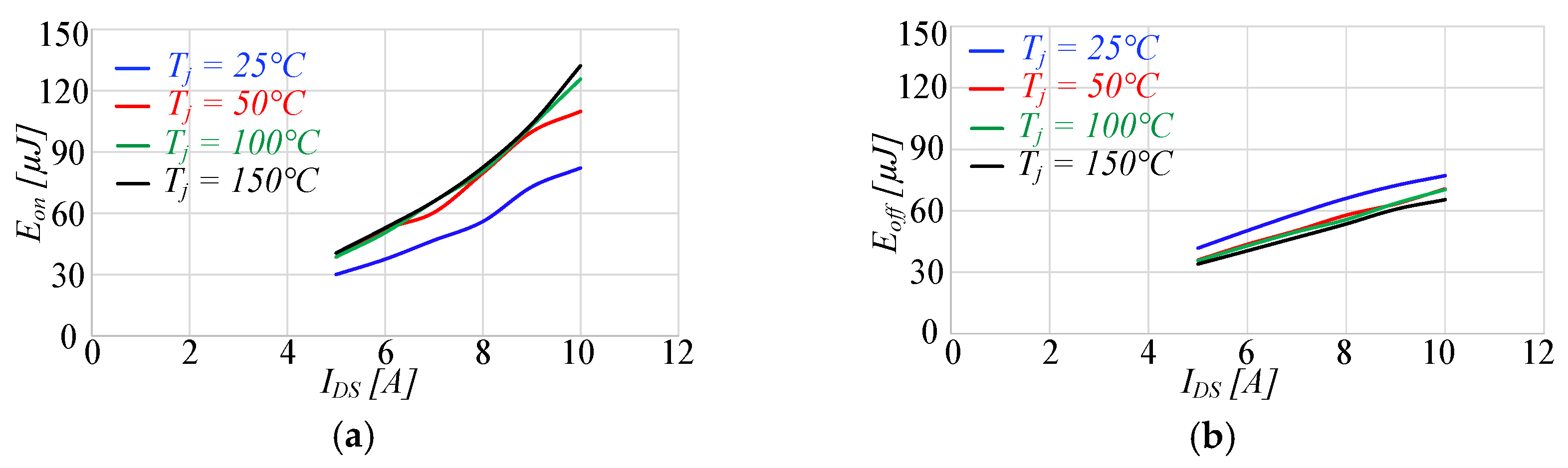
3.1.2. Dynamic Characterization
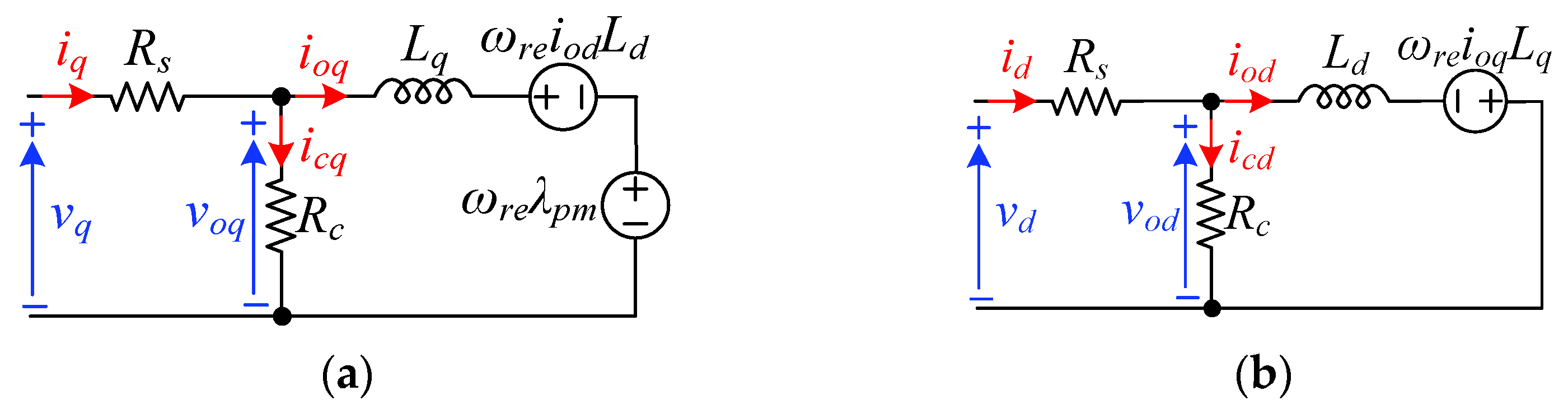
3.2. Analytical Power Loss Modelling of Three-Level Inverters
- (1)
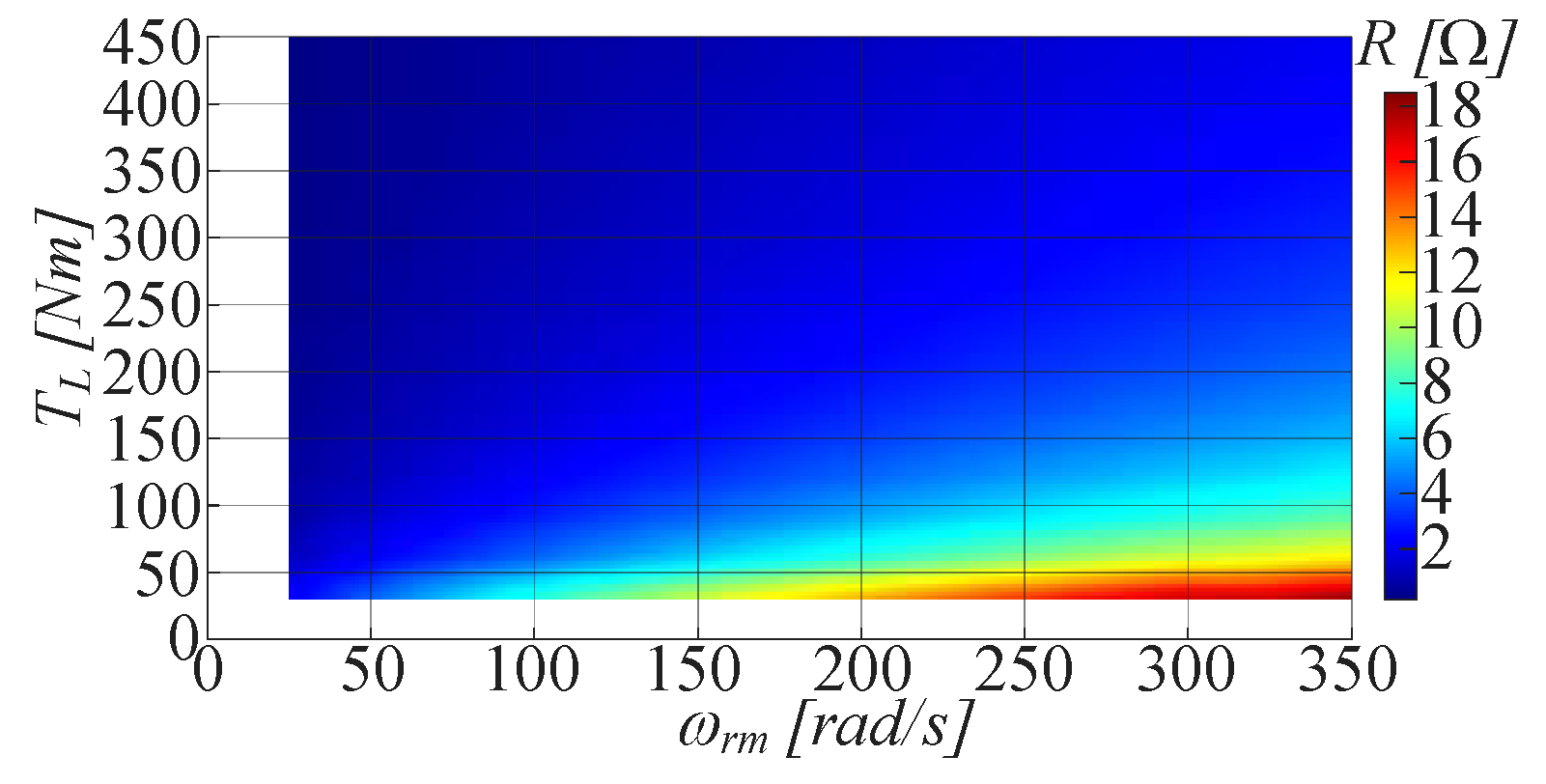
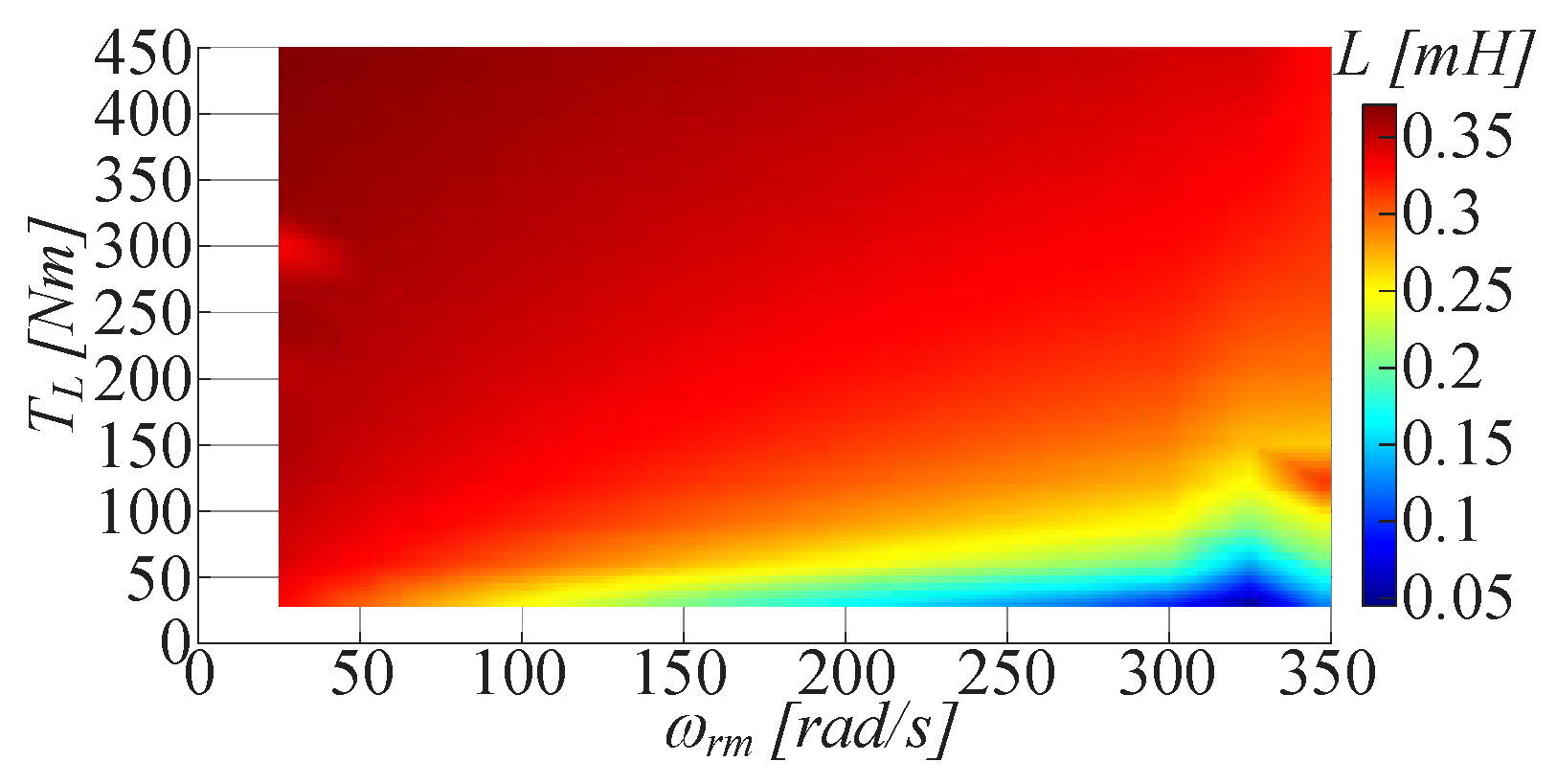
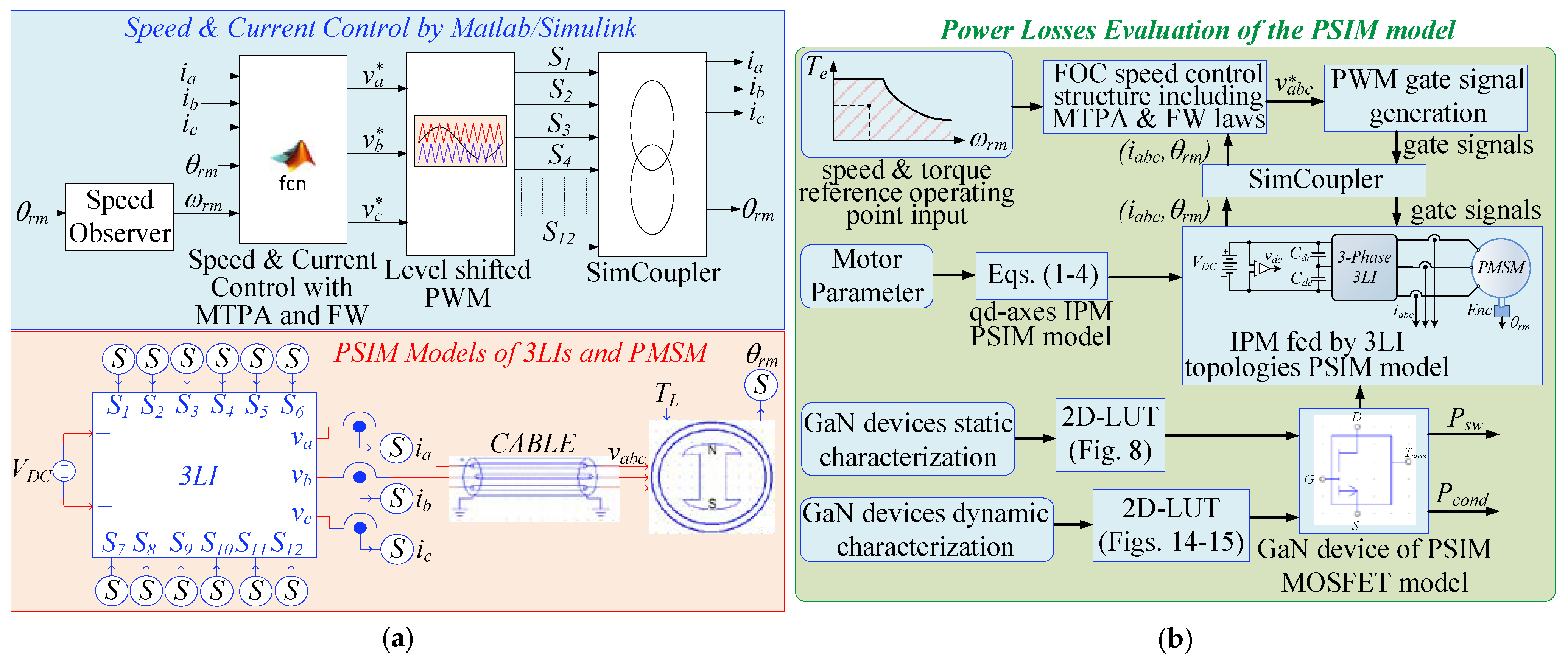
- Initially, starting from the traction drive specifications, the IPM motor is modelled according to (1)–(4) and fed with sinusoidal voltage sets, corresponding to the several operating conditions (TL, ωrm). From the motor voltages and currents the R and L maps are carried out.
- (2)
- Then, according to the considered 3LIs topologies and modulation strategies, the command signals are applied to ideal switches composing the inverter which supplies the motor modelled as in (1)–(4).
- (3)
- For each working condition, the conduction and switching losses are computed according to (6)–(8).
- (4)
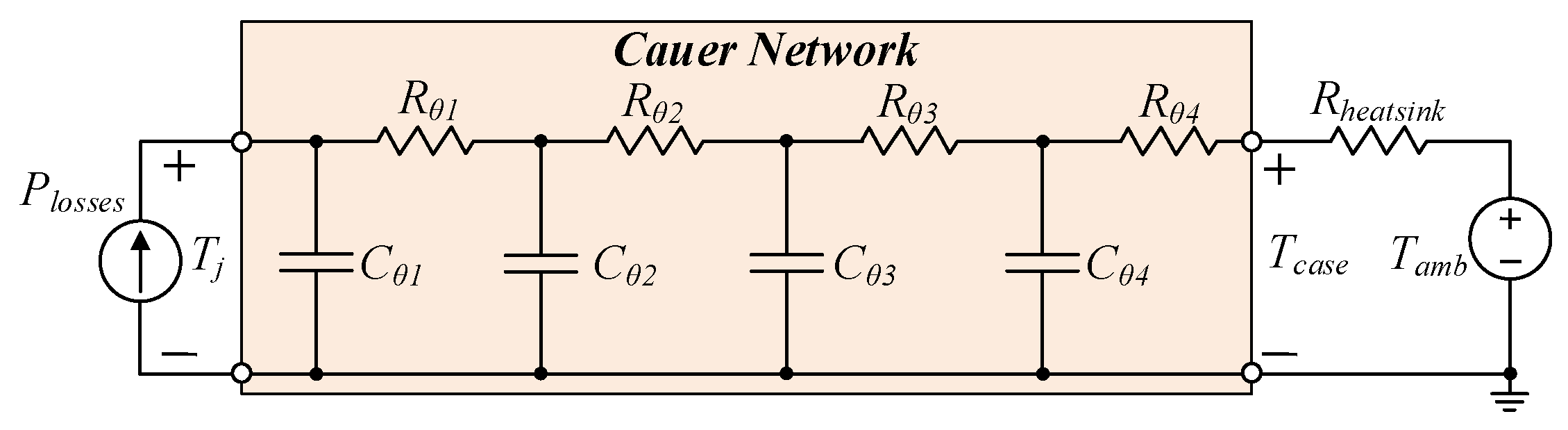
- Cauer network is exploited to update the inputs data of the LUTs, and thus the parameters determined in the GaN HEMT characterization.
4. GaN-Based Traction Drive Modelling in PSIM
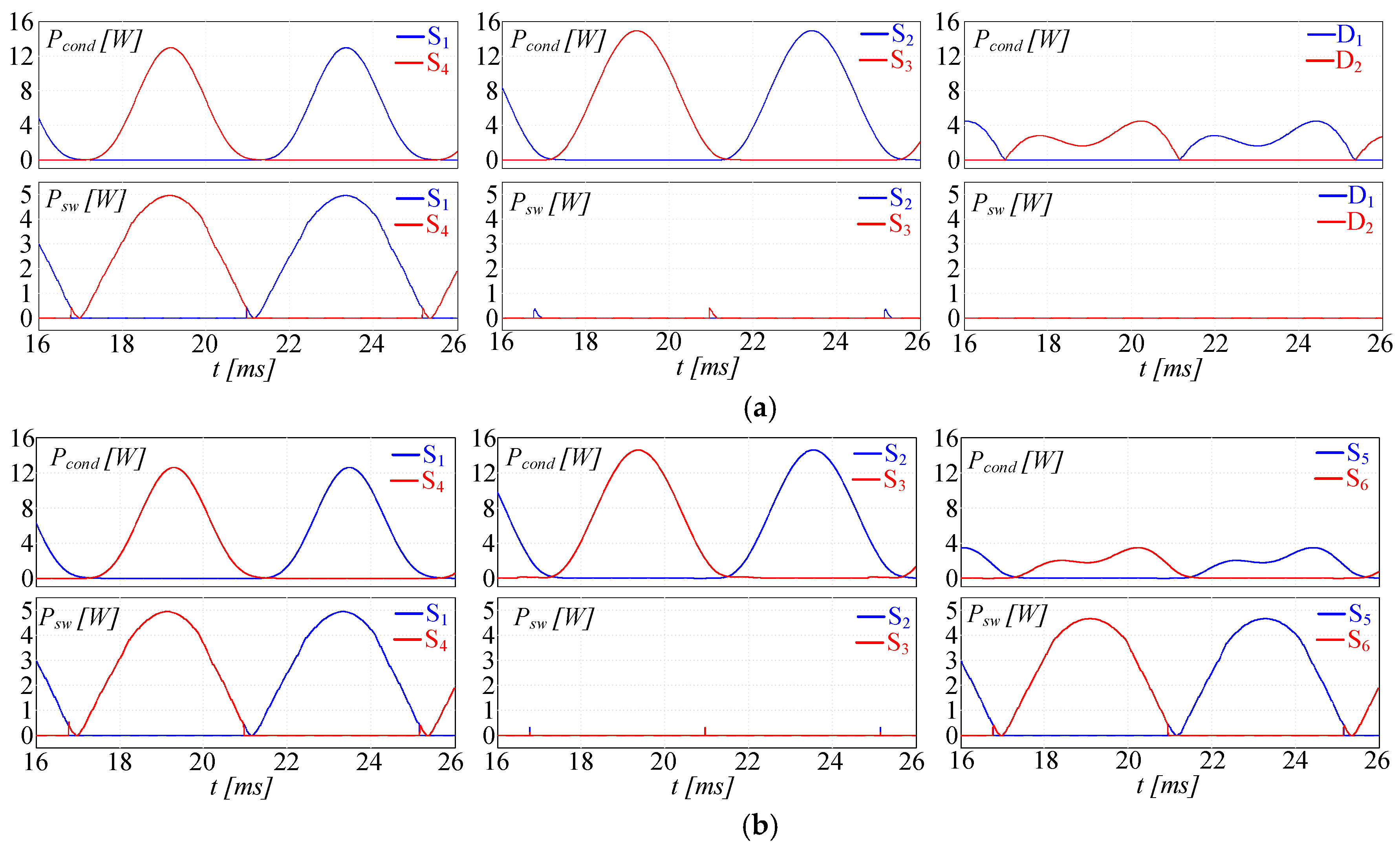
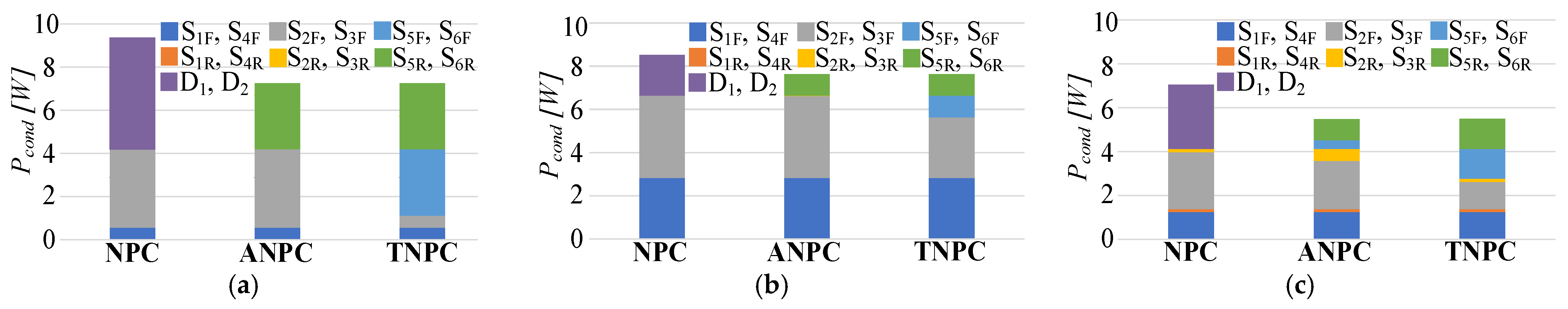
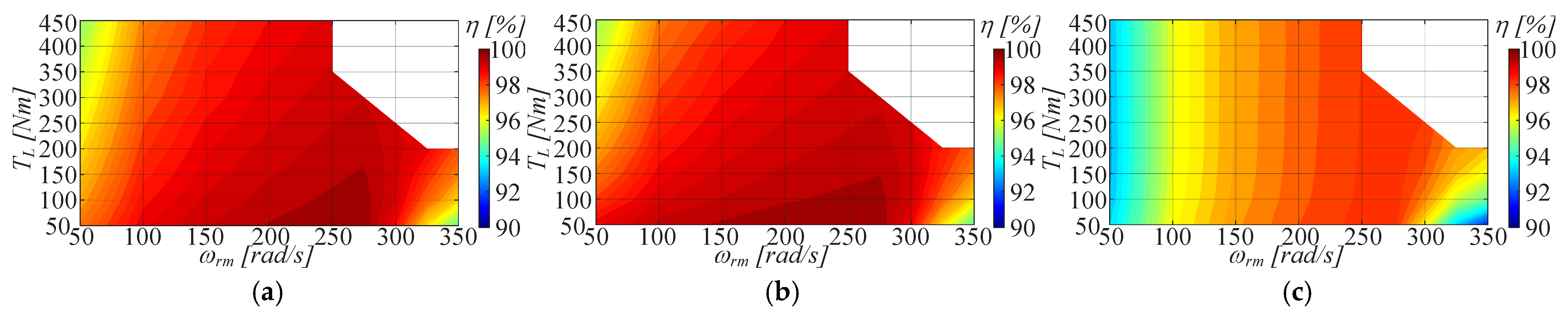
5. Analysis of Power Losses in the Case Study
6. Conclusions
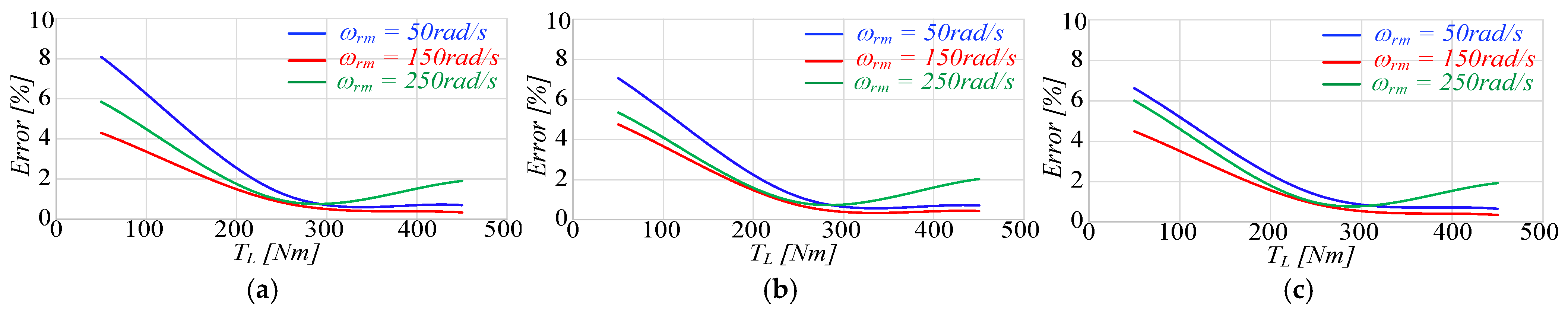
- The proposed analytical power loss modeling, combined with an equivalent representation of the electrical machine yields accurate results across a wide range of traction drive operating conditions. This is evident from the comparison shown in Figure 27.
- Moreover, this modelling proved to offer a viable solution capable of significantly reducing simulation times and computational load compared to a traditional approach, as clearly confirmed with the results summarized in Table 9.
- Additionally, this methodology holds potential for extension to encompass various other topologies and modulation strategies, representing a promising area for future research activity.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Sathiyan, S.P.; Pratap, C.B.; Stonier, A.A.; Peter, G.; Sherine, A.; Praghash, K.; Ganji, V. Comprehensive Assessment of Electric Vehicle Development, Deployment, and Policy Initiatives to Reduce GHG Emissions: Opportunities and Challenges. IEEE Access 2022, 10, 53614–53639. [Google Scholar] [CrossRef]
- Barbosa, W.; Prado, T.; Batista, C.; Câmara, J.C.; Cerqueira, R.; Coelho, R.; Guarieiro, L. Electric Vehicles: Bibliometric Analysis of the Current State of the Art and Perspectives. Energies 2022, 15, 395. [Google Scholar] [CrossRef]
- Ali, I.; Naushad, M. A Study to Investigate What Tempts Consumers to Adopt Electric Vehicles. World Electr. Veh. J. 2022, 13, 26. [Google Scholar] [CrossRef]
- Tu, H.; Feng, H.; Srdic, S.; Lukic, S. Extreme Fast Charging of Electric Vehicles: A Technology Overview. IEEE Trans. Transp. Electrif. 2019, 5, 861–878. [Google Scholar] [CrossRef]
- Jahns, T.M.; Dai, H. The past, present, and future of power electronics integration technology in motor drives. CPSS Trans. Power Electron. Appl. 2017, 2, 197–216. [Google Scholar] [CrossRef]
- Goli, C.S.; Manjrekar, M.; Essakiappan, S.; Sahu, P.; Shah, N. Landscaping and Review of Traction Motors for Electric Vehicle Applications. In Proceedings of the 2021 IEEE Transportation Electrification Conference & Expo (ITEC), Chicago, IL, USA, 21–25 June 2021; pp. 162–168. [Google Scholar]
- Goli, C.S.; Essakiappan, S.; Sahu, P.; Manjrekar, M.; Shah, N. Review of Recent Trends in Design of Traction Inverters for Electric Vehicle Applications. In Proceedings of the 2021 IEEE 12th International Symposium on Power Electronics for Distributed Generation Systems (PEDG), Chicago, IL, USA, 28 June–1 July 2021; pp. 1–6. [Google Scholar]
- Aghabali, I.; Bauman, J.; Kollmeyer, P.J.; Wang, Y.; Bilgin, B.; Emadi, A. 800-V Electric Vehicle Powertrains: Review and Analysis of Benefits, Challenges, and Future Trends. IEEE Trans. Transp. Electrif. 2021, 7, 927–948. [Google Scholar] [CrossRef]
- Allca-Pekarovic, A.; Kollmeyer, P.J.; Mahvelatishamsabadi, P.; Mirfakhrai, T.; Naghshtabrizi, P.; Emadi, A. Comparison of IGBT and SiC Inverter Loss for 400V and 800V DC Bus Electric Vehicle Drivetrains. In Proceedings of the 2020 IEEE Energy Conversion Congress and Exposition (ECCE), Detroit, MI, USA, 11–15 October 2020; pp. 6338–6344. [Google Scholar]
- Reimers, J.; Dorn-Gomba, L.; Mak, C.; Emadi, A. Automotive Traction Inverters: Current Status and Future Trends. IEEE Trans. Veh. Technol. 2019, 68, 3337–3350. [Google Scholar] [CrossRef]
- Tang, Z.; Akin, B. Suppression of Dead-Time Distortion Through Revised Repetitive Controller in PMSM Drives. IEEE Trans. Energy Convers. 2017, 32, 918–930. [Google Scholar] [CrossRef]
- Dai, S.; Wang, J.; Sun, Z.; Chong, E. Deadbeat Predictive Current Control for High-Speed Permanent Magnet Synchronous Machine Drives With Low Switching-To-Fundamental Frequency Ratios. IEEE Trans. Ind. Electron. 2022, 69, 4510–4521. [Google Scholar] [CrossRef]
- Oliveira, A.C.; Jacobina, C.B.; Lima, A.M.N. Improved Dead-Time Compensation for Sinusoidal PWM Inverters Operating at High Switching Frequencies. IEEE Trans. Ind. Electron. 2007, 54, 2295–2304. [Google Scholar] [CrossRef]
- Lidow, A.; De Rooij, M.; Strydom, J.; Reusch, D.; Glaser, J. GaN Transistors for Efficient Power Conversion; John Wiley & Sons: Hoboken, NJ, USA, 2019. [Google Scholar]
- Millán, J.; Godignon, P.; Perpiñà, X.; Pérez-Tomás, A.; Rebollo, J. A Survey of Wide Bandgap Power Semiconductor Devices. IEEE Trans. Power Electron. 2014, 29, 2155–2163. [Google Scholar] [CrossRef]
- Morya, A.; Moosavi, M.; Gardner, M.C.; Toliyat, H.A. Applications of Wide Bandgap (WBG) devices in AC electric drives: A technology status review. In Proceedings of the 2017 IEEE International Electric Machines and Drives Conference (IEMDC), Miami, FL, USA, 21–24 May 2017; pp. 1–8. [Google Scholar]
- Yang, Y.; Dorn-Gomba, L.; Rodriguez, R.; Mak, C.; Emadi, A. Automotive Power Module Packaging: Current Status and Future Trends. IEEE Access 2020, 8, 160126–160144. [Google Scholar] [CrossRef]
- Foti, S.; Testa, A.; Scimone, T.; De Caro, S.; Tornello, L.D.; Scarcella, G.; Scelba, G. A novel Hybrid N-Level T-Type Inverter Topology. In Proceedings of the 2019 IEEE Energy Conversion Congress and Exposition (ECCE), Baltimore, MD, USA, 29 September–3 October 2019; pp. 5507–5513. [Google Scholar]
- Satpathy, S.; Bhattacharya, S.; Veliadis, V. Comprehensive Loss Analysis of Two-level and Three-Level Inverter for Electric Vehicle Using Drive Cycle Models. In Proceedings of the IECON 2020 The 46th Annual Conference of the IEEE Industrial Electronics Society, Singapore, 18–21 October 2020; pp. 2017–2024. [Google Scholar]
- Satpathy, S.; Das, P.P.; Bhattacharya, S.; Veliadis, V. Design Considerations of a GaN-based Three-Level Traction Inverter for Electric Vehicles. In Proceedings of the 2022 IEEE 9th Workshop on Wide Bandgap Power Devices & Applications (WiPDA), Redondo Beach, CA, USA, 7–9 November 2022; pp. 192–197. [Google Scholar]
- Barater, D.; Concari, C.; Buticchi, G.; Gurpinar, E.; De, D.; Castellazzi, A. Performance Evaluation of a Three-Level ANPC Photovoltaic Grid-Connected Inverter With 650-V SiC Devices and Optimized PWM. IEEE Trans. Ind. Appl. 2016, 52, 2475–2485. [Google Scholar] [CrossRef]
- Valente, M.; Iannuzzo, F.; Yang, Y.; Gurpinar, E. Performance Analysis of a Single-phase GaN-based 3L-ANPC Inverter for Photovoltaic Applications. In Proceedings of the 2018 IEEE 4th Southern Power Electronics Conference (SPEC), Singapore, 10–13 December 2018; pp. 1–8. [Google Scholar]
- Haitham, A.R.; Holtz, J.; Rodriguez, J.; Baoming, G. Medium volt-age multilevel converters-state of the art, challenges, and requirements in industrial applications. IEEE Trans. Ind. Electron. 2010, 57, 2581–2596. [Google Scholar]
- Liu, Y.; Bazzi, A.M. A comprehensive analytical power loss model of an induction motor drive system with loss minimization control. In Proceedings of the 2015 IEEE International Electric Machines & Drives Conference (IEMDC), Coeur d’Alene, ID, USA, 10–13 May 2015; pp. 1638–1643. [Google Scholar]
- Mapelli, F.L.; Tarsitano, D.; Mauri, M. Plug-In Hybrid Electric Vehicle: Modeling, Prototype Realization, and Inverter Losses Reduction Analysis. IEEE Trans. Ind. Electron. 2010, 57, 598–607. [Google Scholar] [CrossRef]
- Williamson, S.S.; Emadi, A.; Rajashekara, K. Comprehensive Efficiency Modeling of Electric Traction Motor Drives for Hybrid Electric Vehicle Propulsion Applications. IEEE Trans. Veh. Technol. 2007, 56, 1561–1572. [Google Scholar] [CrossRef]
- Aarniovuori, L.; Laurila, L.I.E.; Niemela, M.; Pyrhonen, J.J. Measurements and Simulations of DTC Voltage Source Converter and Induction Motor Losses. IEEE Trans. Ind. Electron. 2012, 59, 2277–2287. [Google Scholar] [CrossRef]
- Nemeth, T.; Bubert, A.; Becker, J.N.; De Doncker, R.W.; Sauer, D.U. A Simulation Platform for Optimization of Electric Vehicles With Modular Drivetrain Topologies. IEEE Trans. Transp. Electrif. 2018, 4, 888–900. [Google Scholar] [CrossRef]
- Stabile, A.; Estima, J.O.; Boccaletti, C.; Cardoso, A.J.M. Converter Power Loss Analysis in a Fault-Tolerant Permanent-Magnet Synchronous Motor Drive. IEEE Trans. Ind. Electron. 2015, 62, 1984–1996. [Google Scholar] [CrossRef]
- Zhang, D.; Cittanti, D.; Sun, P.; Huber, J.; Bojoi, R.I.; Kolar, J.W. Detailed Modeling and In-Situ Calorimetric Verification of Three-Phase Sparse NPC Converter Power Semiconductor Losses. IEEE J. Emerg. Sel. Top. Power Electron. 2023, 11, 3409–3423. [Google Scholar] [CrossRef]
- Choe, Y.Y.; Oh, S.Y.; Ham, S.H.; Jang, I.S.; Cho, S.Y.; Lee, J.; Ko, K.C. Comparison of concentrated and distributed winding in an IPMSM for vehicle traction. Energy Procedia 2012, 14, 1368–1373. [Google Scholar] [CrossRef]
- Hassan, W.; Wang, B. Efficiency optimization of PMSM based drive system. In Proceedings of the 7th International Power Electronics and Motion Control Conference, Harbin, China, 2–5 June 2012; Volume 2, pp. 1027–1033. [Google Scholar]
- Scelba, G.; Tornello, L.D.; Scarcella, G.; Cacciato, M.; Testa, A.; Foti, S.; Pulvirenti, M. Combined Rotor Position Estimation and Temperature Monitoring in Sensorless Synchronous Motor Drives. In Proceedings of the 2018 IEEE 9th International Symposium on Sensorless Control for Electrical Drives (SLED), Helsinki, Finland, 13–14 September 2018; pp. 30–35. [Google Scholar]
- Qu, Z.; Tuovinen, T.; Hinkkanen, M. Minimizing losses of a synchronous reluctance motor drive taking into account core losses and magnetic saturation. In Proceedings of the 2014 16th European Conference on Power Electronics and Applications, Lappeenranta, Finland, 26–28 August 2014; pp. 1–10. [Google Scholar]
- Cavallaro, C.; Di Tommaso, A.O.; Miceli, R.; Raciti, A.; Galluzzo, G.R.; Trapanese, M. Efficiency enhancement of permanent-magnet synchronous motor drives by online loss minimization approaches. IEEE Trans. Ind. Electron. 2005, 52, 1153–1160. [Google Scholar] [CrossRef]
- Gupta, K.K.; Bhatnagar, P. Multilevel Inverters: Conventional and Emerging Topologies and Their Control; Academic Press: Cambridge, MA, USA, 2017. [Google Scholar]
- Mita, S.; Sujeeth, A.; Aiello, G.; Patti, D.; Gennaro, F.; Scelba, G.; Cacciato, M. Power Loss Modelling of GaN HEMT-based 3L-ANPC Three-Phase Inverter for different PWM Techniques. In Proceedings of the 2022 24th European Conference on Power Electronics and Applications (EPE’22 ECCE Europe), Hanover, Germany, 5–9 September 2022; pp. P.1–P.10. [Google Scholar]
- Schweizer, M.; Kolar, J.W. Design and implementation of a highly efficient three-level T-type converter for low-voltage applications. IEEE Trans. Power Electron. 2012, 28, 899–907. [Google Scholar] [CrossRef]
- Jørgensen, A.B.; Sønderskov, S.H.; Beczkowski, S.; Bidoggia, B.; Munk-Nielsen, S. Analysis of cascaded silicon carbide MOSFETs using a single gate driver for medium voltage applications. IET Power Electron. 2020, 13, 413–419. [Google Scholar] [CrossRef]
- Roig, J.; Gomez, G.; Bauwens, F.; Vlachakis, B.; Rodriguez, J.; Rogina, M.R.; Rodriguez, A.; Lamar, D.G. Series-connected GaN transistors for ultra-fast high-voltage switch (>1kV). In Proceedings of the 2017 IEEE Applied Power Electronics Conference and Exposition (APEC), Tampa, FL, USA, 26–30 March 2017; pp. 3043–3048. [Google Scholar]
- Rodriguez, J.; Lai, J.S.; Peng, F.Z. Multilevel inverters: A survey of topologies, controls, and applications. IEEE Trans. Ind. Electron. 2002, 49, 724–738. [Google Scholar] [CrossRef]
- Testa, A.; Foti, S.; De Caro, S.; Tornello, L.D.; Scelba, G.; Scarcella, G. Optimal Selection of the Voltage Modulation Strategy for an Open Winding Multilevel Inverter. In Proceedings of the 2020 IEEE Energy Conversion Congress and Exposition (ECCE), Detroit, MI, USA, 11–15 October 2020; pp. 2231–2237. [Google Scholar]
- Yu, E.T.; Dang, X.Z.; Yu, L.S.; Qiao, D.; Asbeck, P.M.; Lau, S.S.; Sullivan, G.J.; Boutros, K.S.; Redwing, J.M. Piezoelectric enhancement of Schottky barrier heights in GaN-AlGaN HFET structures. In Proceedings of the 56th Annual Device Research Conference Digest (Cat. No. 98TH8373), Charlottesville, VA, USA, 22–24 June 1998; pp. 116–117. [Google Scholar]
- Gelagaev, R.; Jacqmaer, P.; Driesen, J. A Fast Voltage Clamp Circuit for the Accurate Measurement of the Dynamic ON-Resistance of Power Transistors. IEEE Trans. Ind. Electron. 2015, 62, 1241–1250. [Google Scholar] [CrossRef]
- Zulauf, G.; Guacci, M.; Kolar, J.W. Dynamic on-Resistance in GaN-on-Si HEMTs: Origins, Dependencies, and Future Characterization Frameworks. IEEE Trans. Power Electron. 2020, 35, 5581–5588. [Google Scholar] [CrossRef]
- Schulte, C.; Böcker, J. Co-simulation of an electric traction drive. In Proceedings of the 2013 International Electric Machines & Drives Conference, Chicago, IL, USA, 12–15 May 2013; pp. 974–978. [Google Scholar]
- Ballestín-Bernad, V.; Artal-Sevil, J.S.; Domínguez-Navarro, J.A. Co-Simulation of a Two-Phase Axial-Gap Transverse Flux Machine. In Proceedings of the 2022 International Conference on Electrical Machines (ICEM), Valencia, Spain, 5–8 September 2022; pp. 956–961. [Google Scholar]
- Turrisi, G.; Tornello, L.D.; Scelba, G.; Cacciato, M.; Scarcella, G. Losses Evaluation in Current Source Inverter Topologies for Automotive Traction Applications. In Proceedings of the 2023 25th European Conference on Power Electronics and Applications (EPE’23 ECCE Europe), Aalborg, Denmark, 4–8 September 2023; pp. 1–11. [Google Scholar]
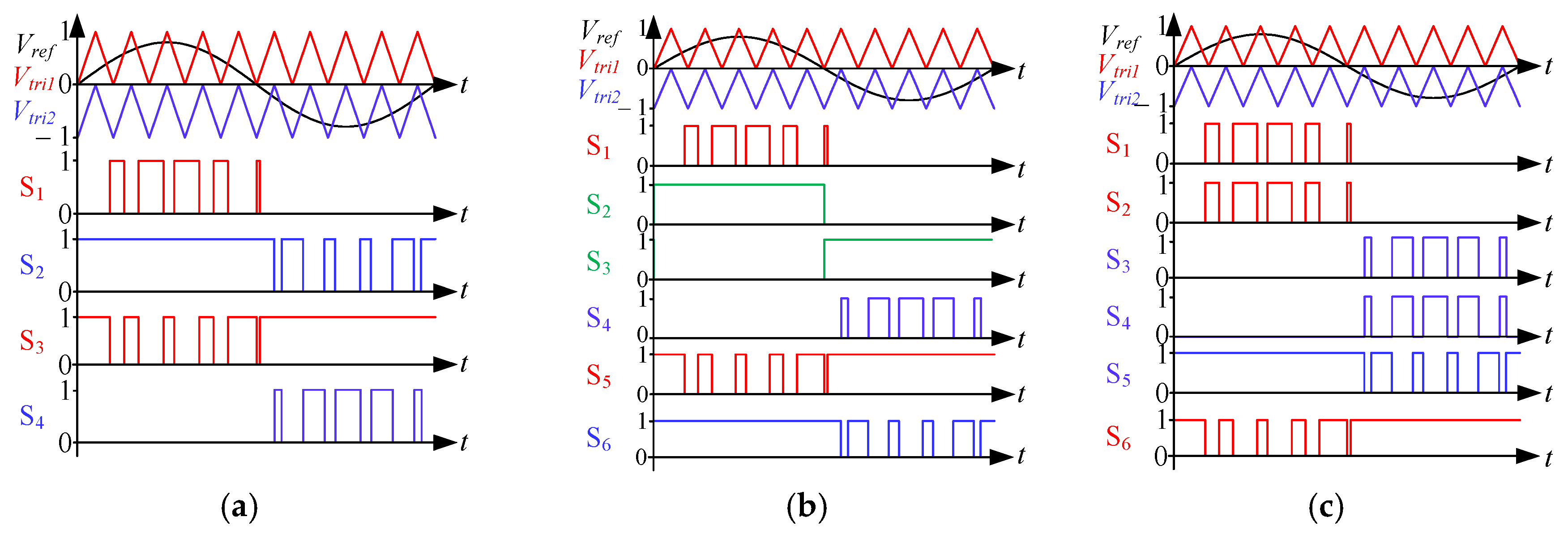
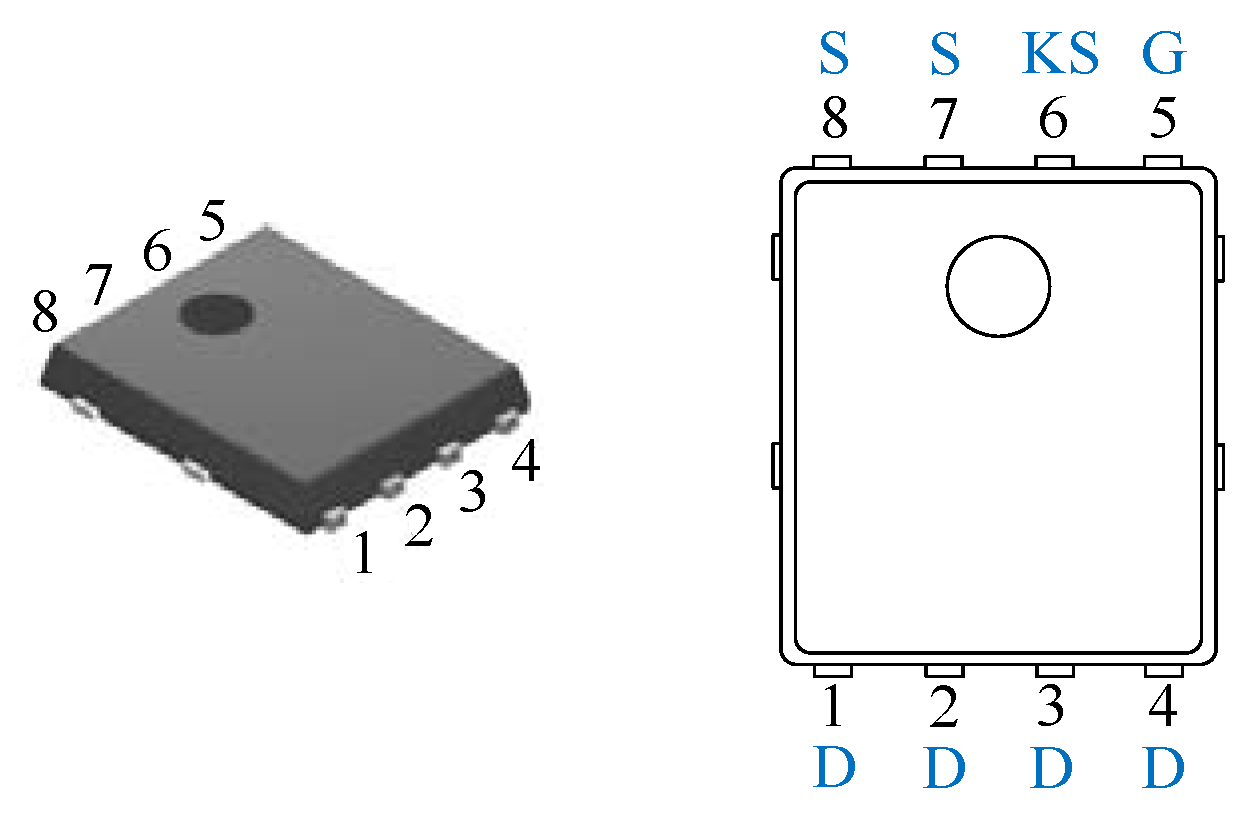
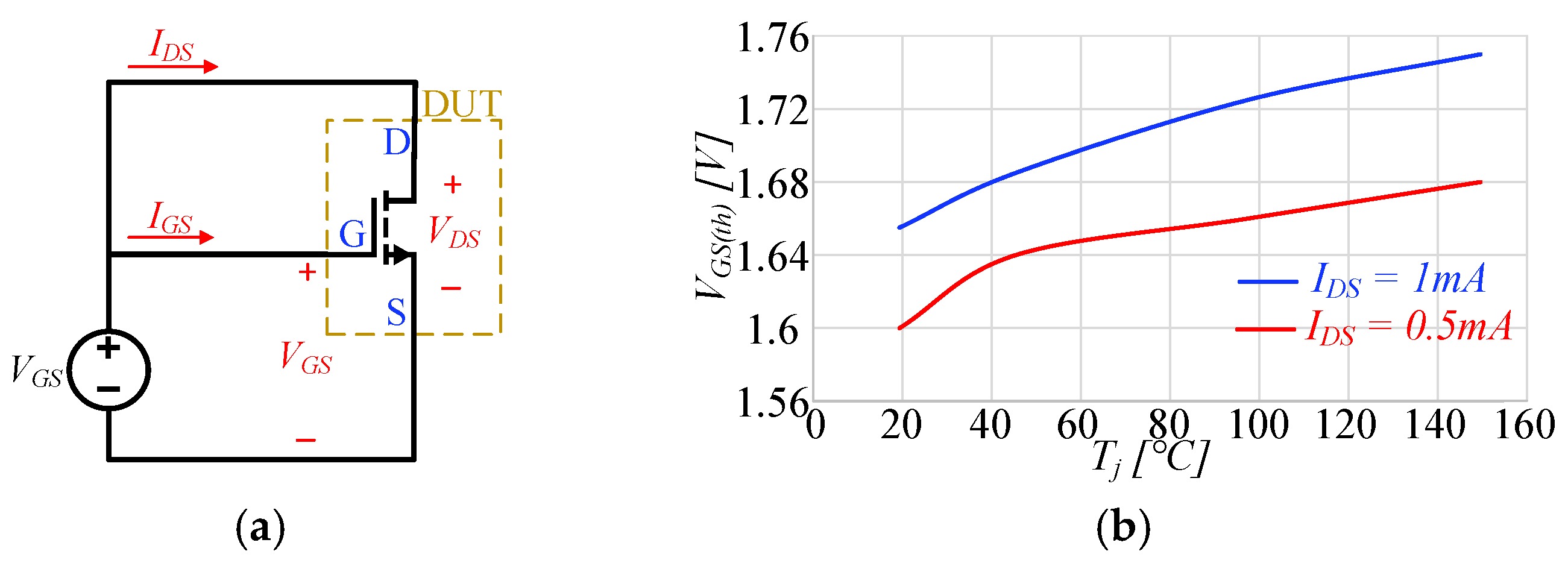
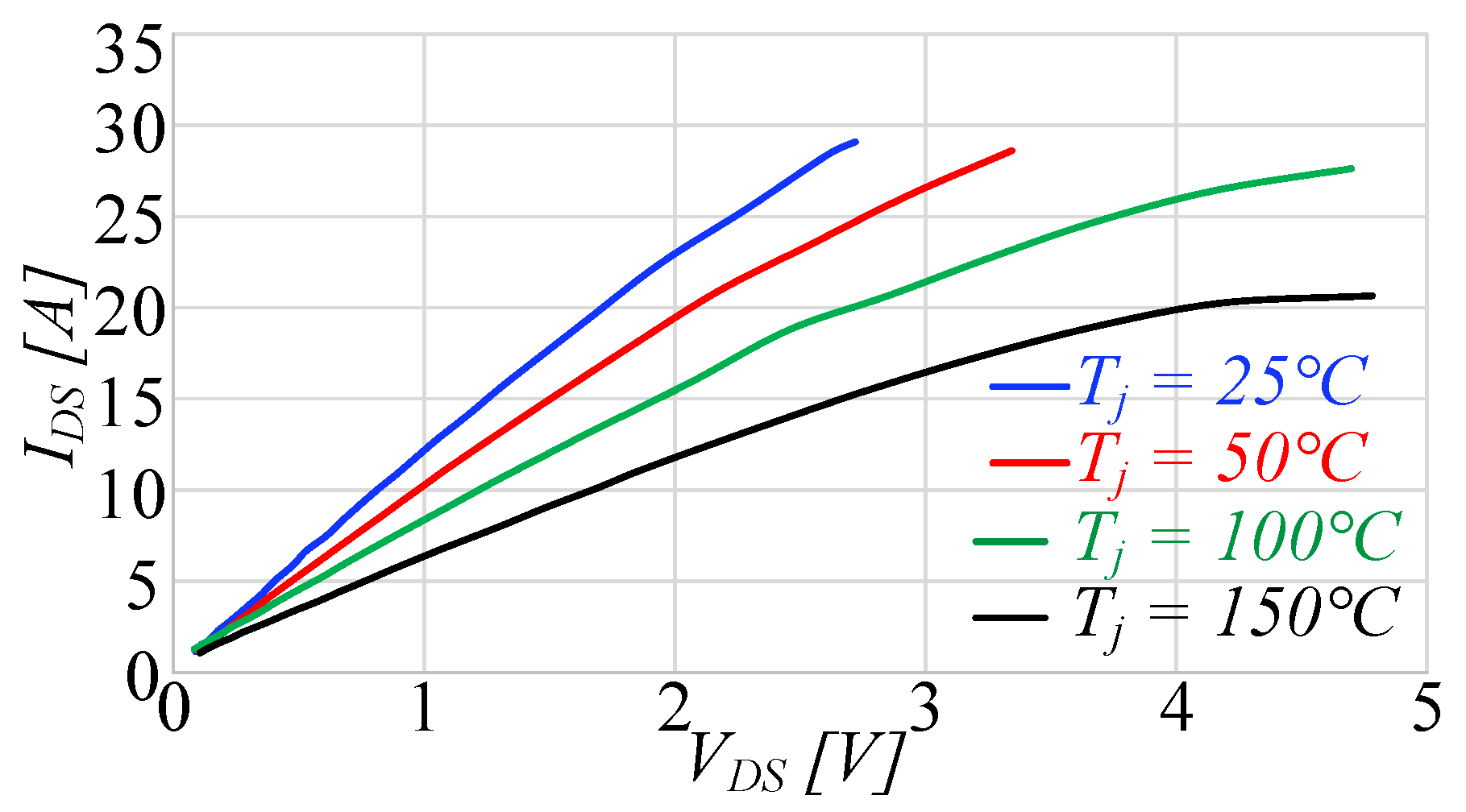







| Parameter | Unit | Si | GaN | SiC |
|---|---|---|---|---|
| Band Gap (Eg) | eV | 1.12 | 3.39 | 3.26 |
| Critical Field (Ecrit) | MV/cm | 0.23 | 3.3 | 2.2 |
| Electron Mobility (µn) | cm2/(V∙s) | 1400 | 1500 | 950 |
| Permittivity (ɛr) | 11.8 | 9 | 9.7 | |
| Thermal Conductivity (λ) | W/(cm∙K) | 1.5 | 1.3 | 3.8 |
| Parameter | Value |
|---|---|
| Maximum power | 110 kW |
| DC bus voltage (VDC) | 800 V |
| Switching frequency (fsw) | 50 kHz |
| Maximum torque @ rated speed | 450 Nm |
| Rated speed (ωrmR) | 250 rad/s |
| Maximum speed (ωrmax) | 350 rad/s |
| Parameter | Symbol | Value |
|---|---|---|
| Stator resistance | Rs | 18.3 mΩ |
| q-axis inductance | Lq | 745 μH |
| d-axis inductance | Ld | 361.4 μH |
| Permanent magnet flux | λpm | 0.45 Wb |
| Equivalent iron loss resistance @ ωrmR | Rc | 50 Ω |
| Parameter | Value |
|---|---|
| Drain-source blocking voltage (V(BL)DSS) | 650 V |
| Continuous drain current (IDS) | 15 A @ Tcase = 25 °C |
| 12 A @ Tcase = 100 °C | |
| Operating junction temperature (Tj) | −50 °C to +150 °C |
| t [µs] | 2.5 | 5 | 7.5 | 10 |
|---|---|---|---|---|
| RDS(on) [mΩ] | 121.3 | 117.8 | 115.5 | 115.5 |
| Topology | Device | δ(θre) | Irms |
|---|---|---|---|
| NPC | S1F, S4F | ||
| S1R, S4R | |||
| S2F, S3F | 1 π < θre < π + φ | ||
| S2R, S3R | |||
| D1, D2 | φ < θre < π π < θre < π + φ | ||
| ANPC | S1F, S4F | ||
| S1R, S4R | |||
| S2F, S3F | 1 | ||
| S2R, S3R | 1 | ||
| S5F, S6F | |||
| S5R, S6R | φ < θre < π | ||
| TNPC | S1F, S4F | φ < θre < π | |
| S1R, S4R | |||
| S2F, S3F | φ < θre < π | ||
| S2R, S3R | |||
| S5F, S6F | φ < θre < π π < θre < π + φ | \ | |
| S5R, S6R | 0 < θre < φ π + φ < θre < 2π |
| Topology | Device | n1 | n2 |
|---|---|---|---|
| NPC | S1, S4 | 0 | n/2 |
| S2, S3 | n/2 | ||
| ANPC | S1, S4 | 0 | n/2 |
| S2, S3 | - | - | |
| S5, S6 | 0 | n/2 | |
| TNPC | S1, S4 | 0 | n/2 |
| S2, S3 | 0 | n/2 | |
| S5, S6 | n/2 | N |
| Rθ [°C/W] | Cθ [mWs/°C] |
|---|---|
| Rθ1 = 0.08 | Cθ1 = 0.03 |
| Rθ2 = 0.9 | Cθ2 = 0.3 |
| Rθ3 = 0.38 | Cθ3 = 3.5 |
| Rθ4 = 0.04 | Cθ4 = 2.6 |
| Topology | Traction Drive Model | Proposed Analytical Approach + RL Circuit | Improvement |
|---|---|---|---|
| NPC, ANPC, TNPC | ≈16 min | ≈5 min | ≈68% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sujeeth, A.; Di Cataldo, A.; Tornello, L.D.; Pulvirenti, M.; Salvo, L.; Sciacca, A.G.; Scelba, G.; Cacciato, M. Power Loss Modelling and Performance Comparison of Three-Level GaN-Based Inverters Used for Electric Traction. Energies 2024, 17, 595. https://doi.org/10.3390/en17030595
Sujeeth A, Di Cataldo A, Tornello LD, Pulvirenti M, Salvo L, Sciacca AG, Scelba G, Cacciato M. Power Loss Modelling and Performance Comparison of Three-Level GaN-Based Inverters Used for Electric Traction. Energies. 2024; 17(3):595. https://doi.org/10.3390/en17030595
Chicago/Turabian StyleSujeeth, Arjun, Angelo Di Cataldo, Luigi Danilo Tornello, Mario Pulvirenti, Luciano Salvo, Angelo Giuseppe Sciacca, Giacomo Scelba, and Mario Cacciato. 2024. "Power Loss Modelling and Performance Comparison of Three-Level GaN-Based Inverters Used for Electric Traction" Energies 17, no. 3: 595. https://doi.org/10.3390/en17030595
APA StyleSujeeth, A., Di Cataldo, A., Tornello, L. D., Pulvirenti, M., Salvo, L., Sciacca, A. G., Scelba, G., & Cacciato, M. (2024). Power Loss Modelling and Performance Comparison of Three-Level GaN-Based Inverters Used for Electric Traction. Energies, 17(3), 595. https://doi.org/10.3390/en17030595









