Oscillation Suppression of Grid-Following Converters by Grid-Forming Converters with Adaptive Droop Control
Abstract
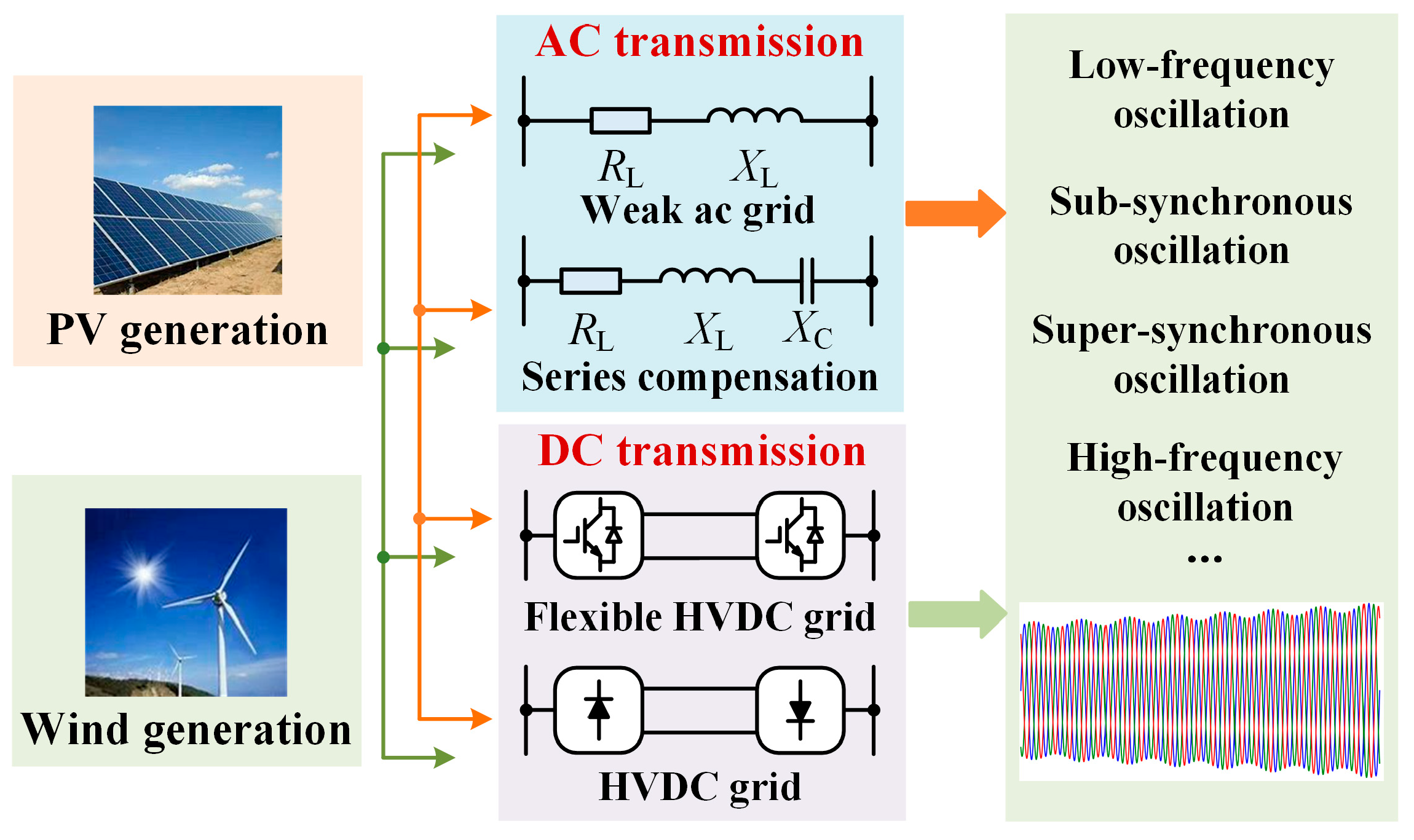
1. Introduction
- (1)
- Extracts ISCR to demonstrate that the stability of the hybrid system combining GFLCs and GFMCs has been improved.
- (2)
- Analyzes the mechanism by which the first-order inertia control unit enhances voltage transient stability.
- (3)
- Proposes the adaptive active power droop control strategy to enhance oscillation suppression capability.
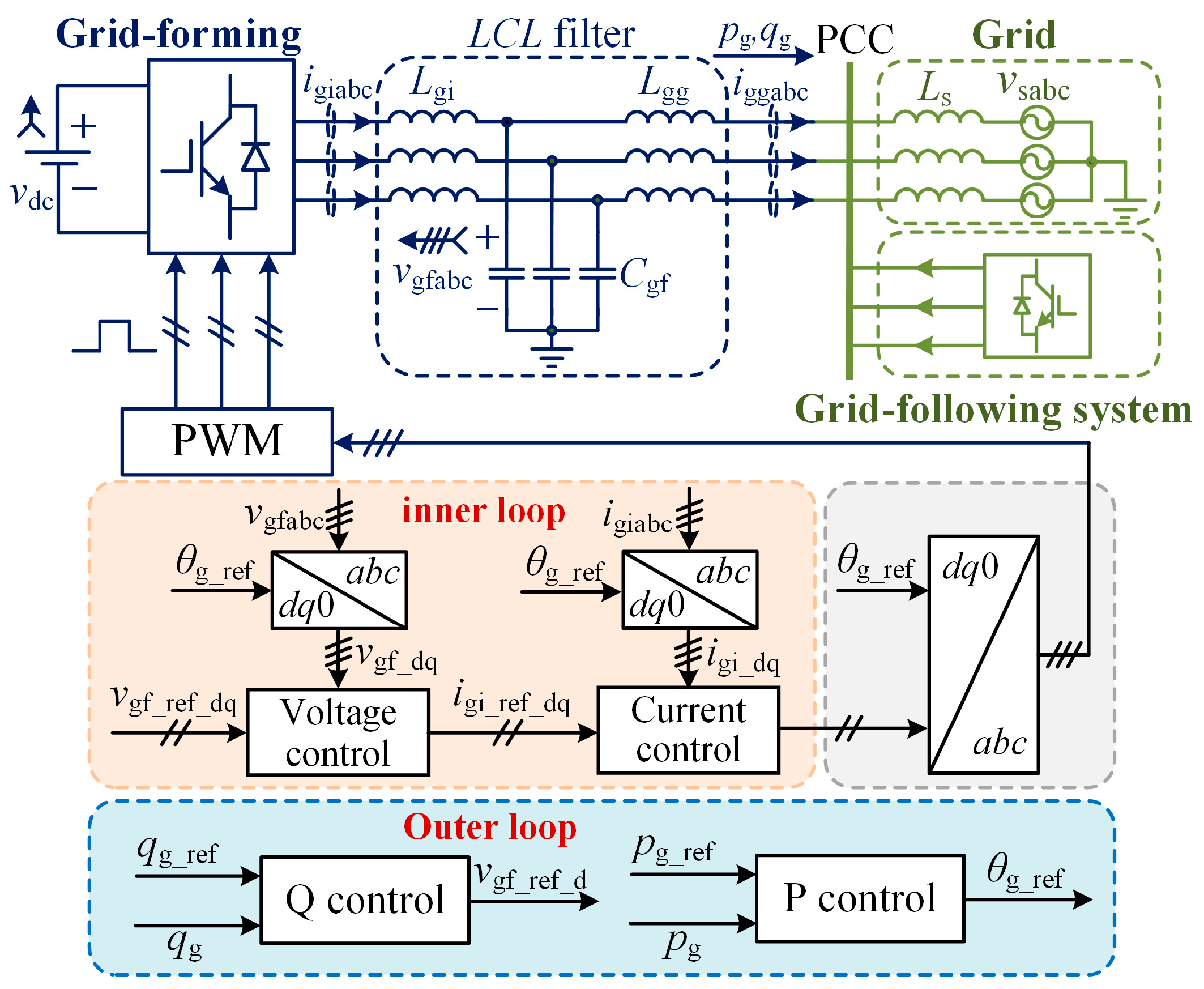
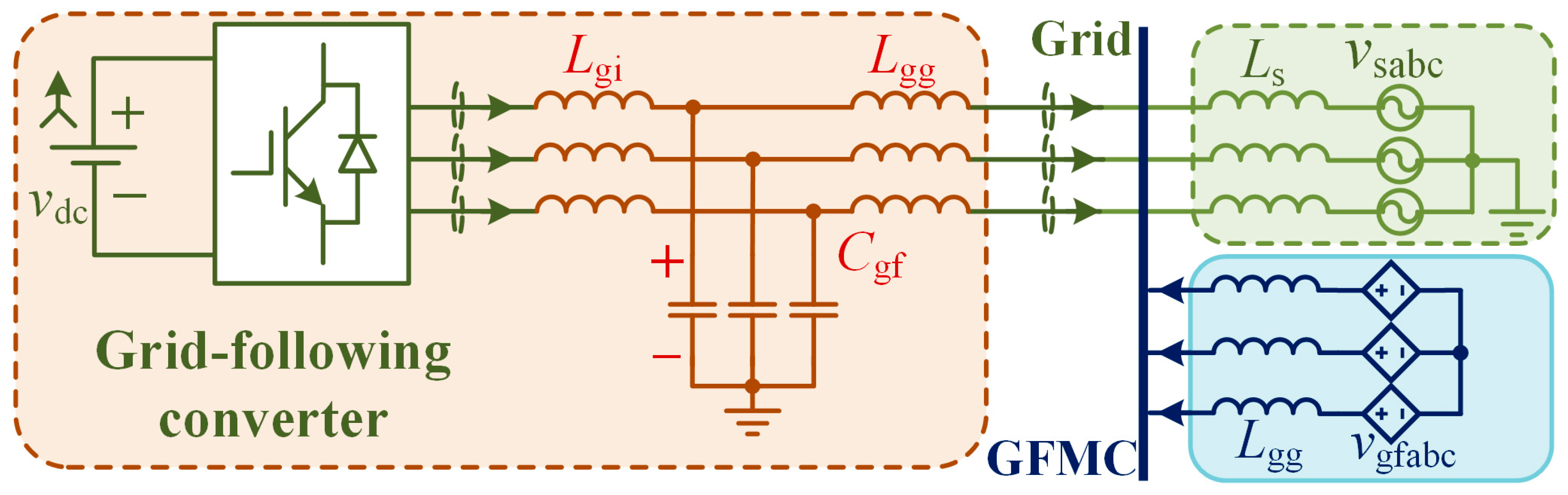
2. System Configuration and Control Structure
3. Principle of the Improved Short Circuit Ratio (ISCR)
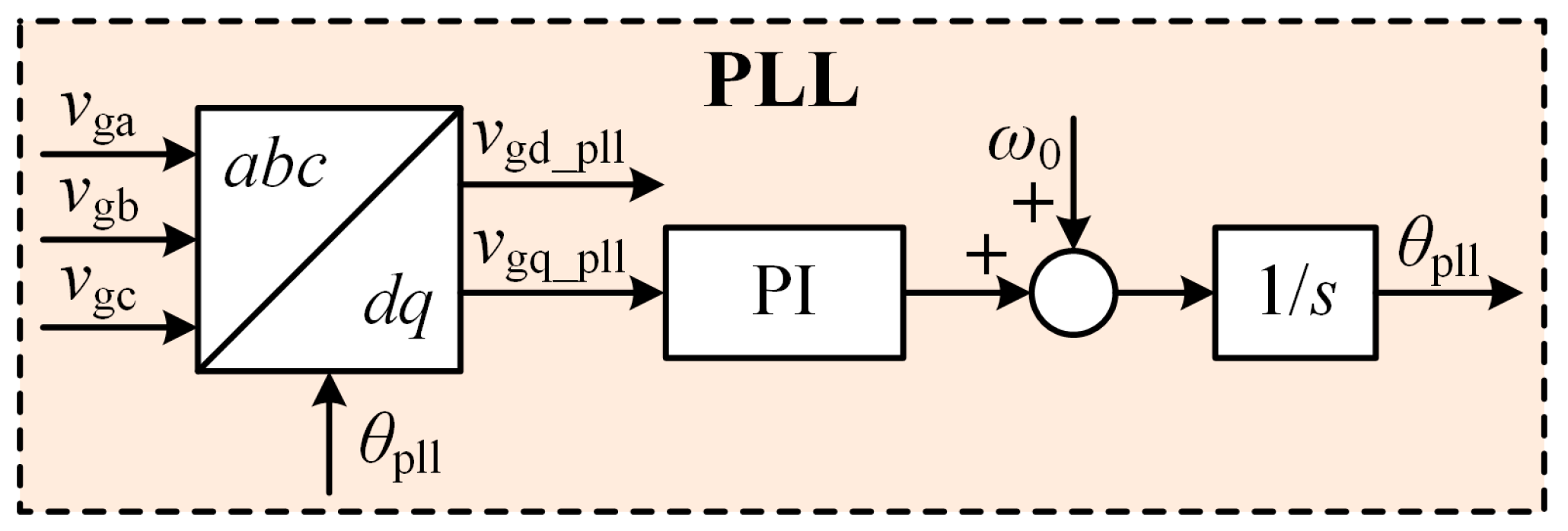
3.1. Conventional Phase-Locked Loop for Grid-Following Converters
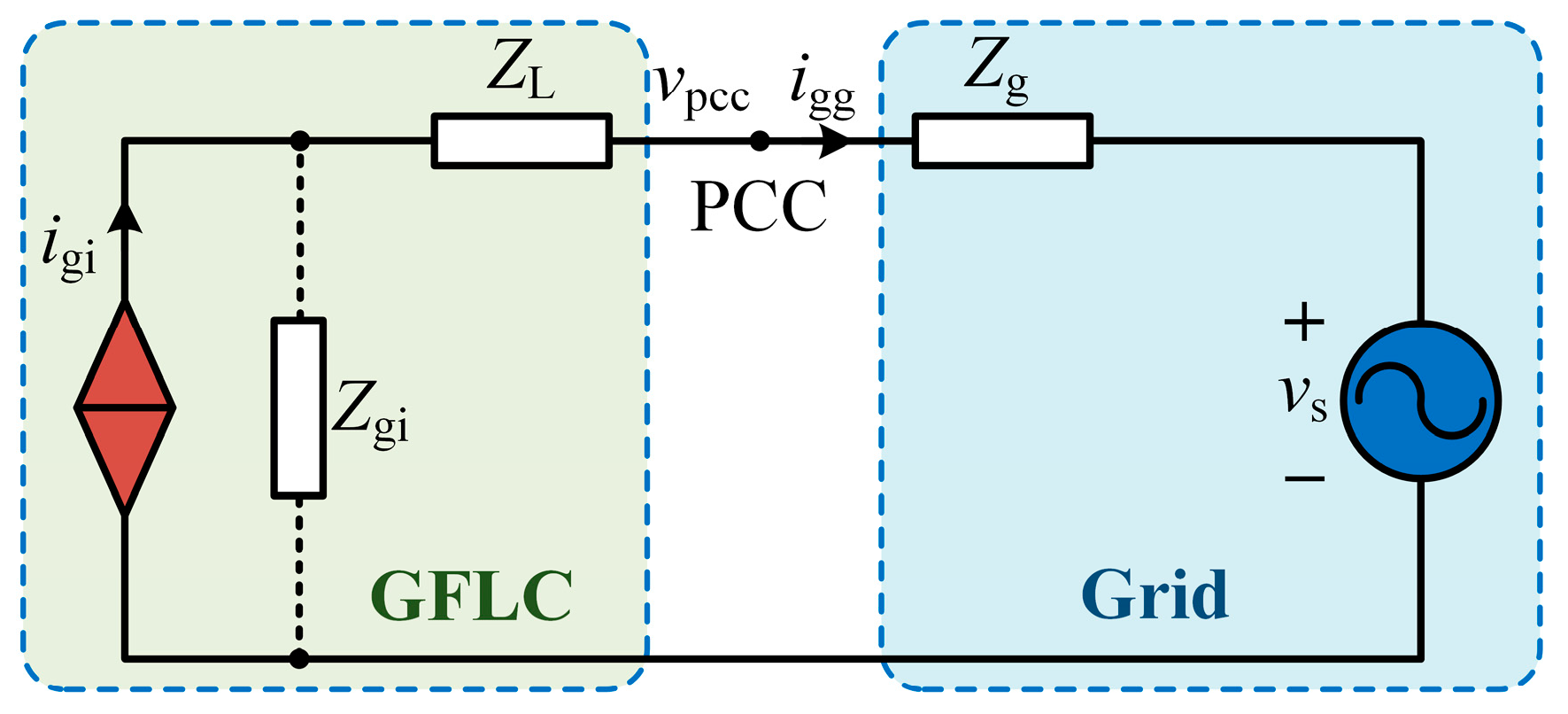
3.2. The Proposed Improved Short Circuit Ratio (ISCR)
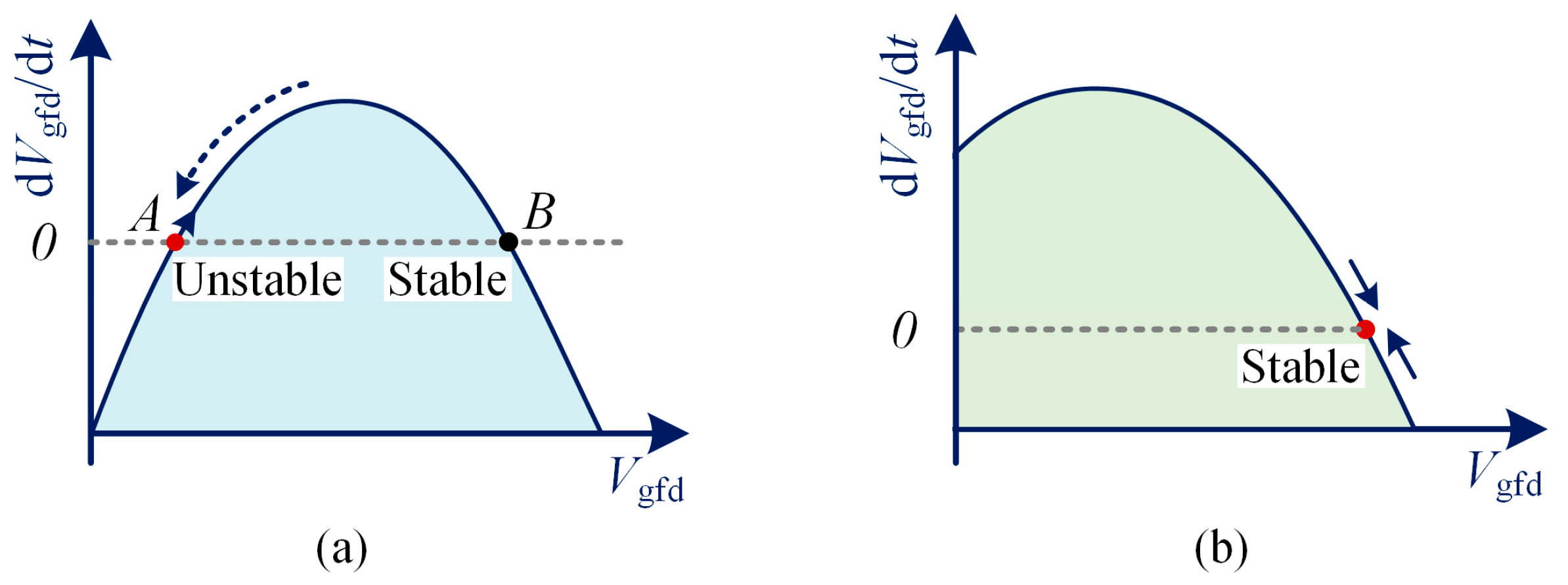
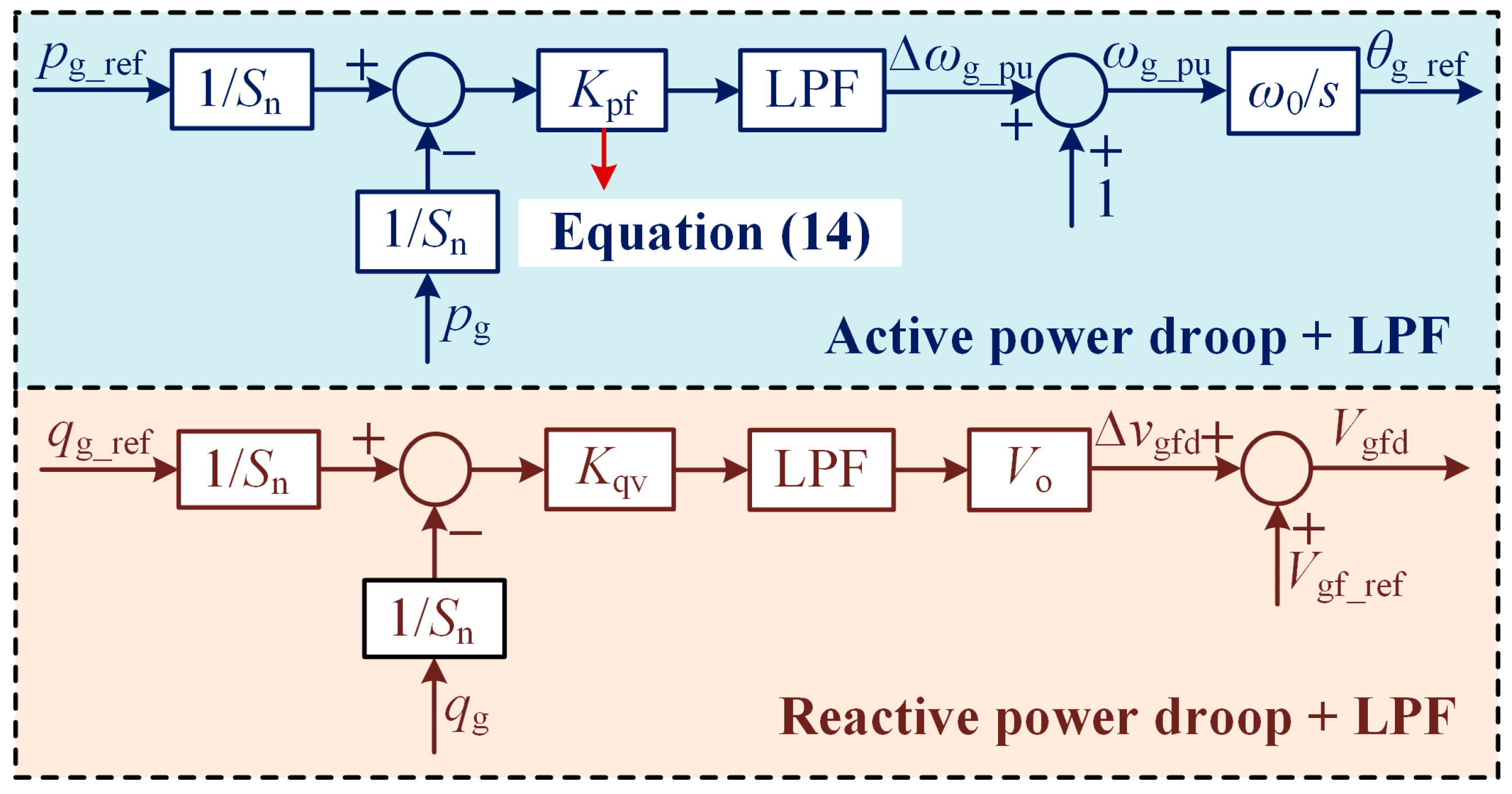
4. The Proposed Wideband Oscillation Suppression Method Based on Adaptive Active Power Droop Control
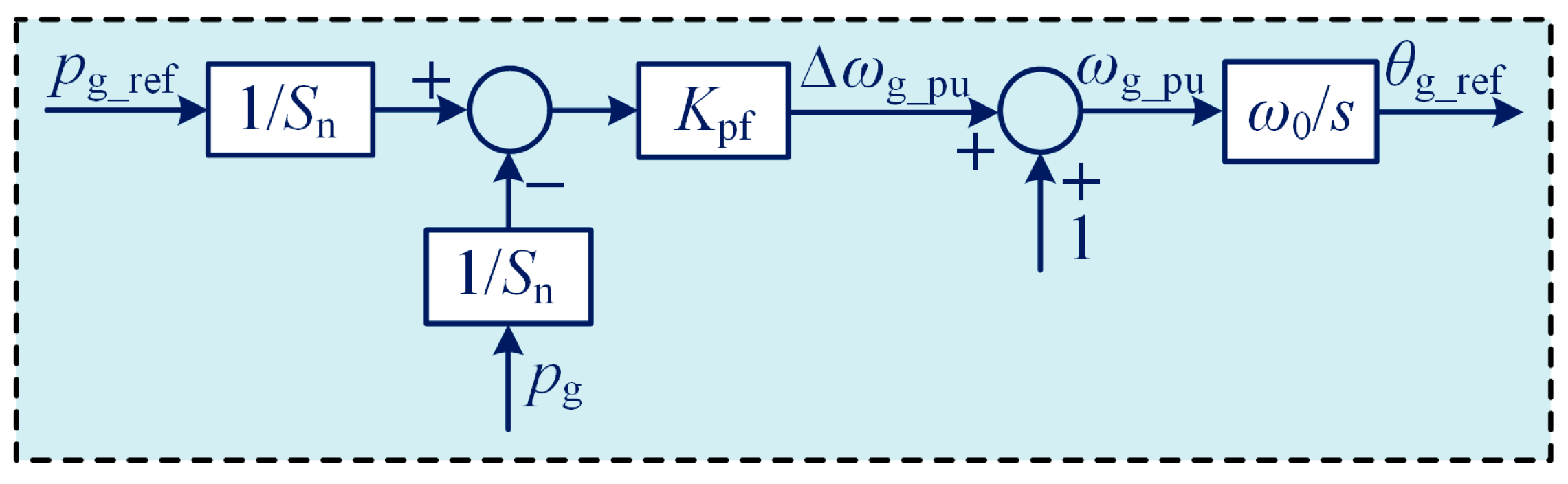
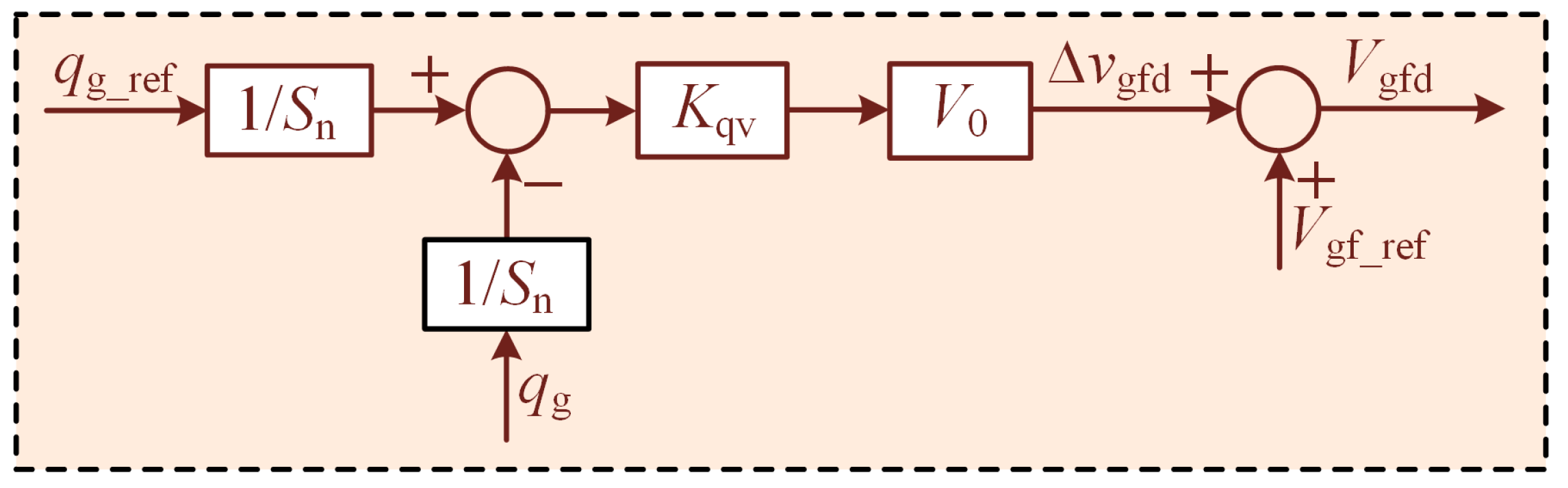
4.1. The Inertia in the Droop Control
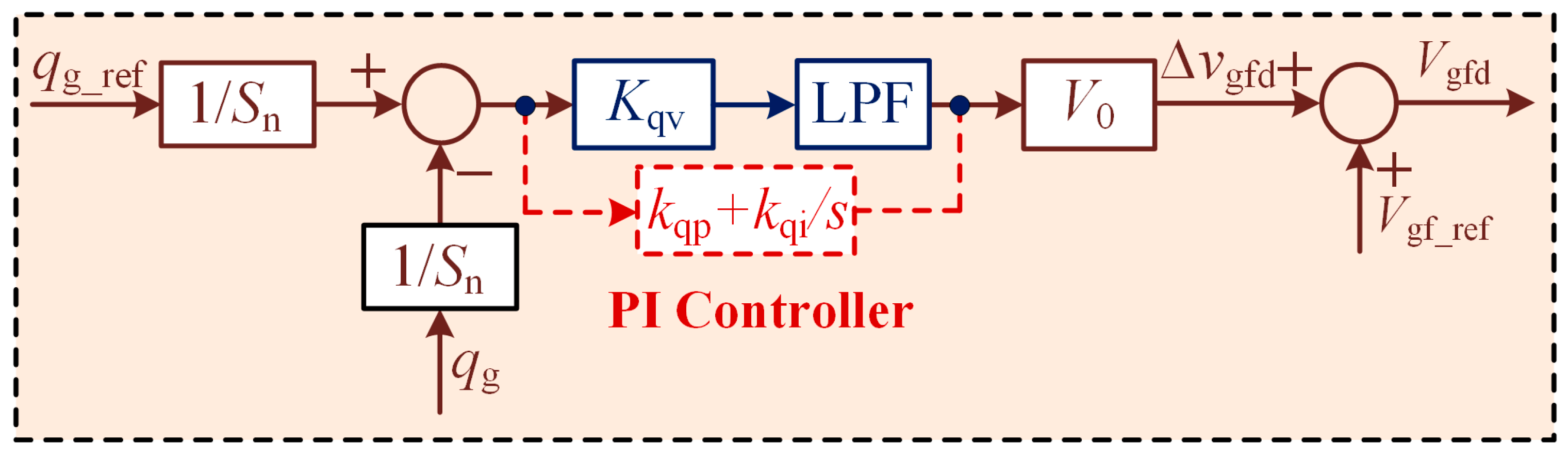
4.2. The Proposed Wideband Oscillation Suppression Method Based on Adaptive Active Power Droop Control
5. Simulation and Experimental Results
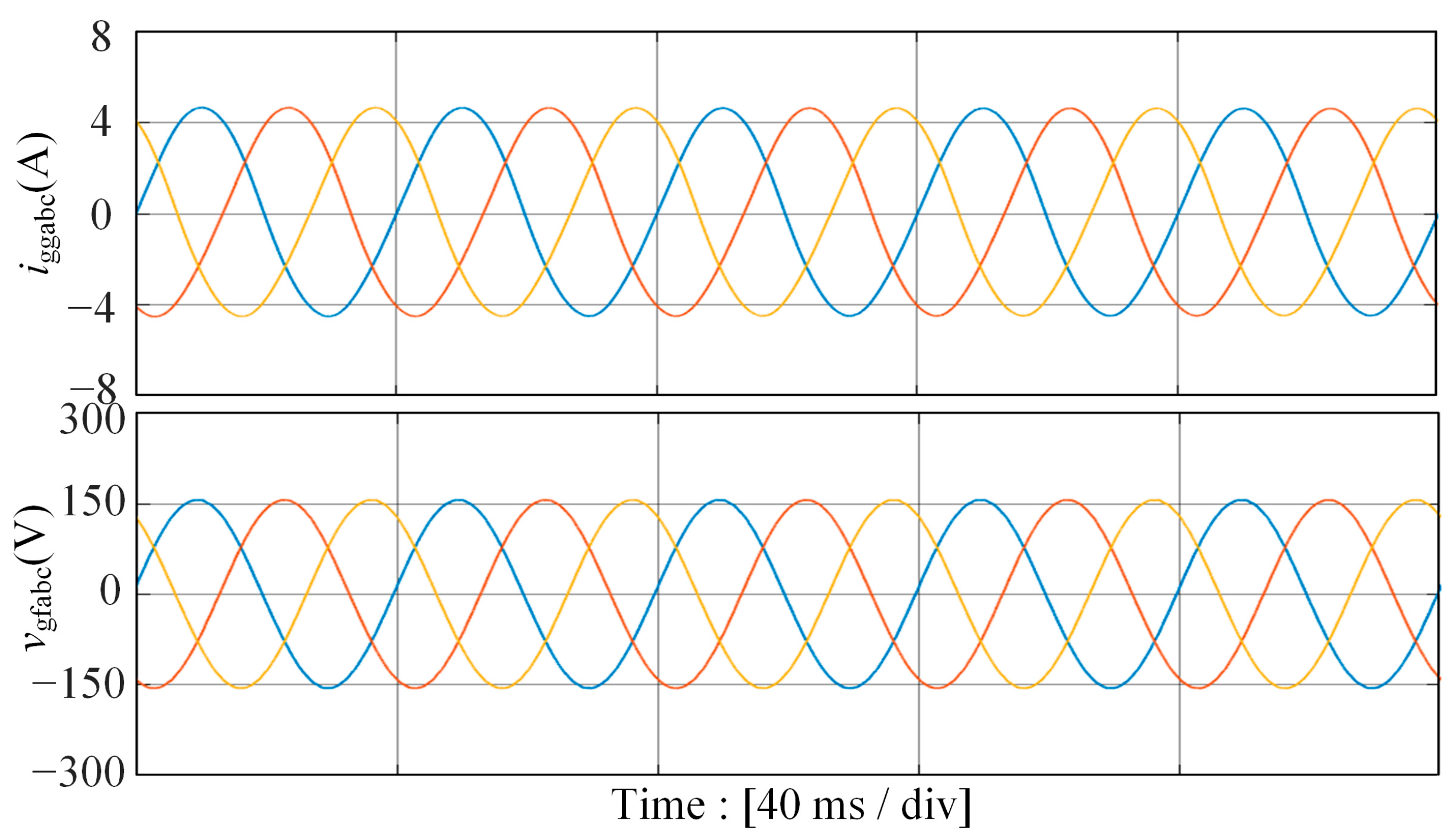
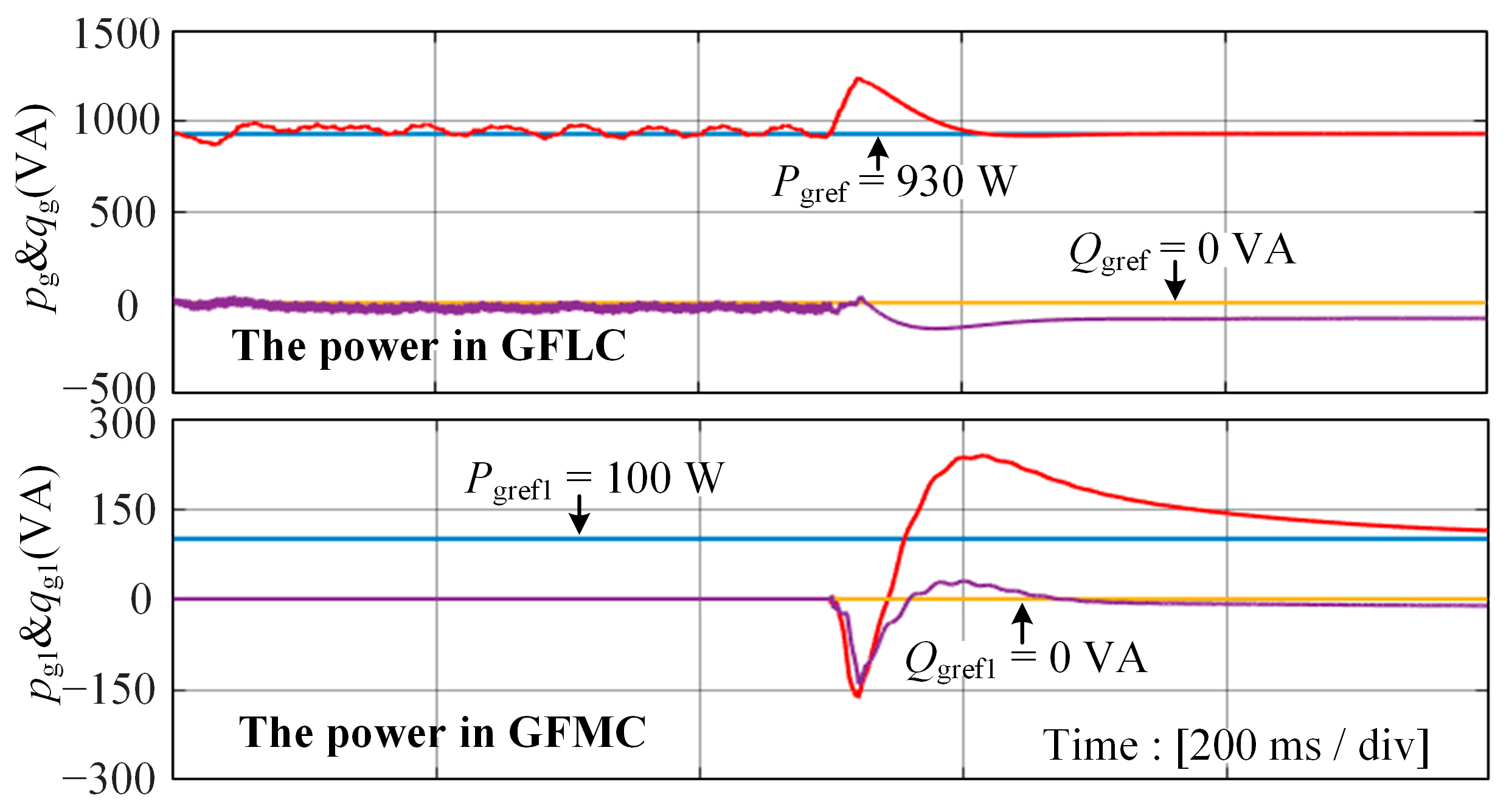
5.1. Simulation Results
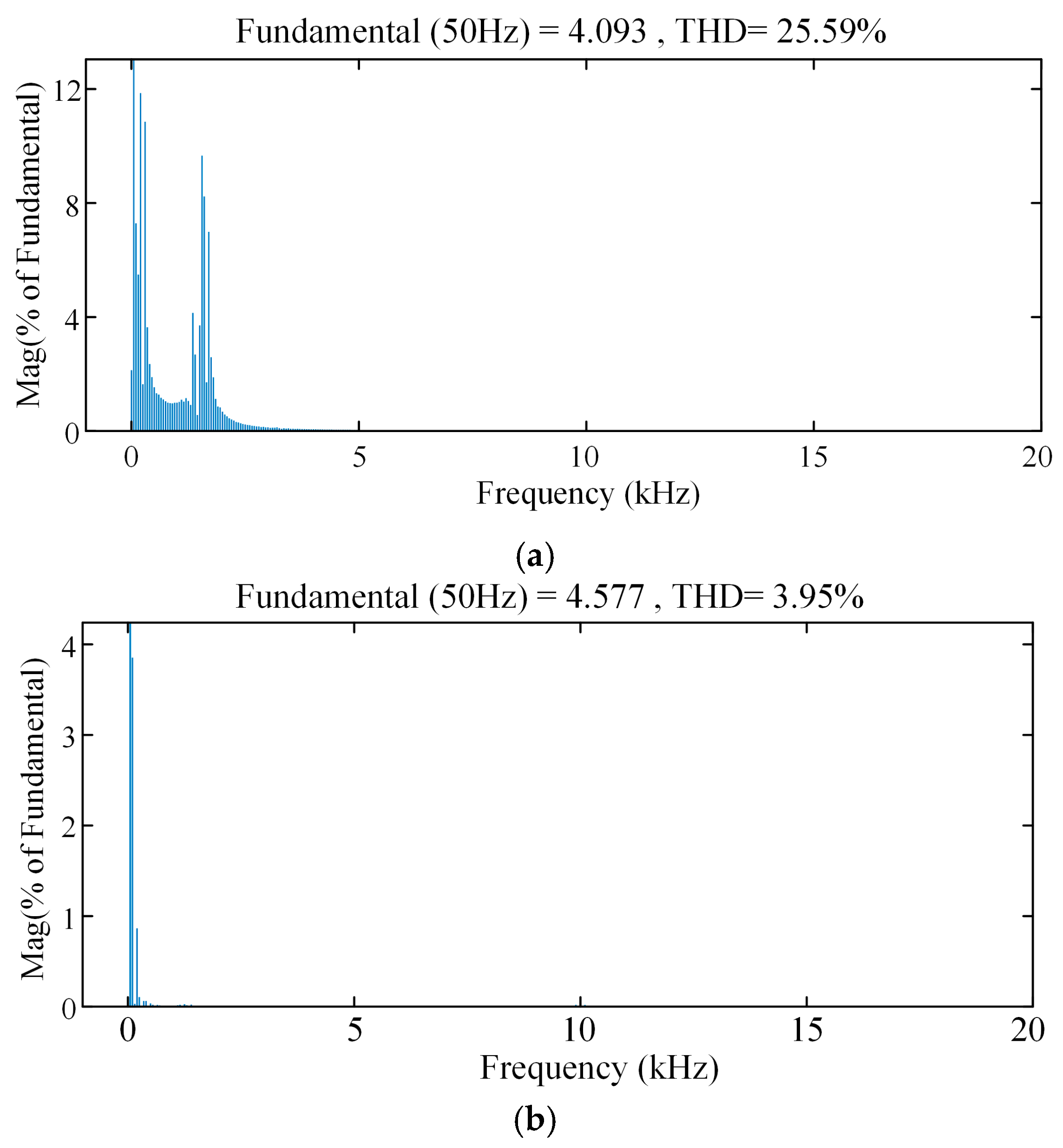
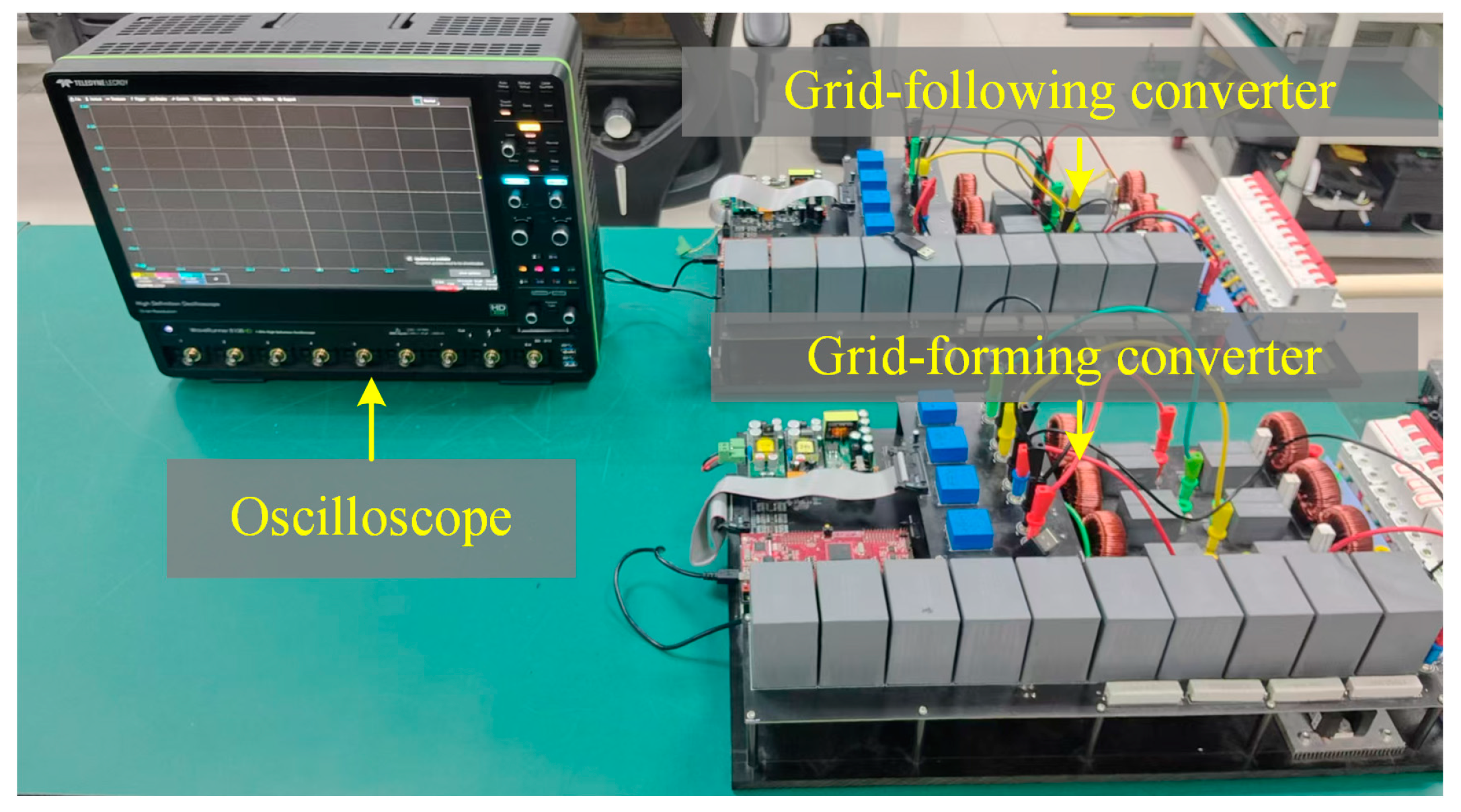
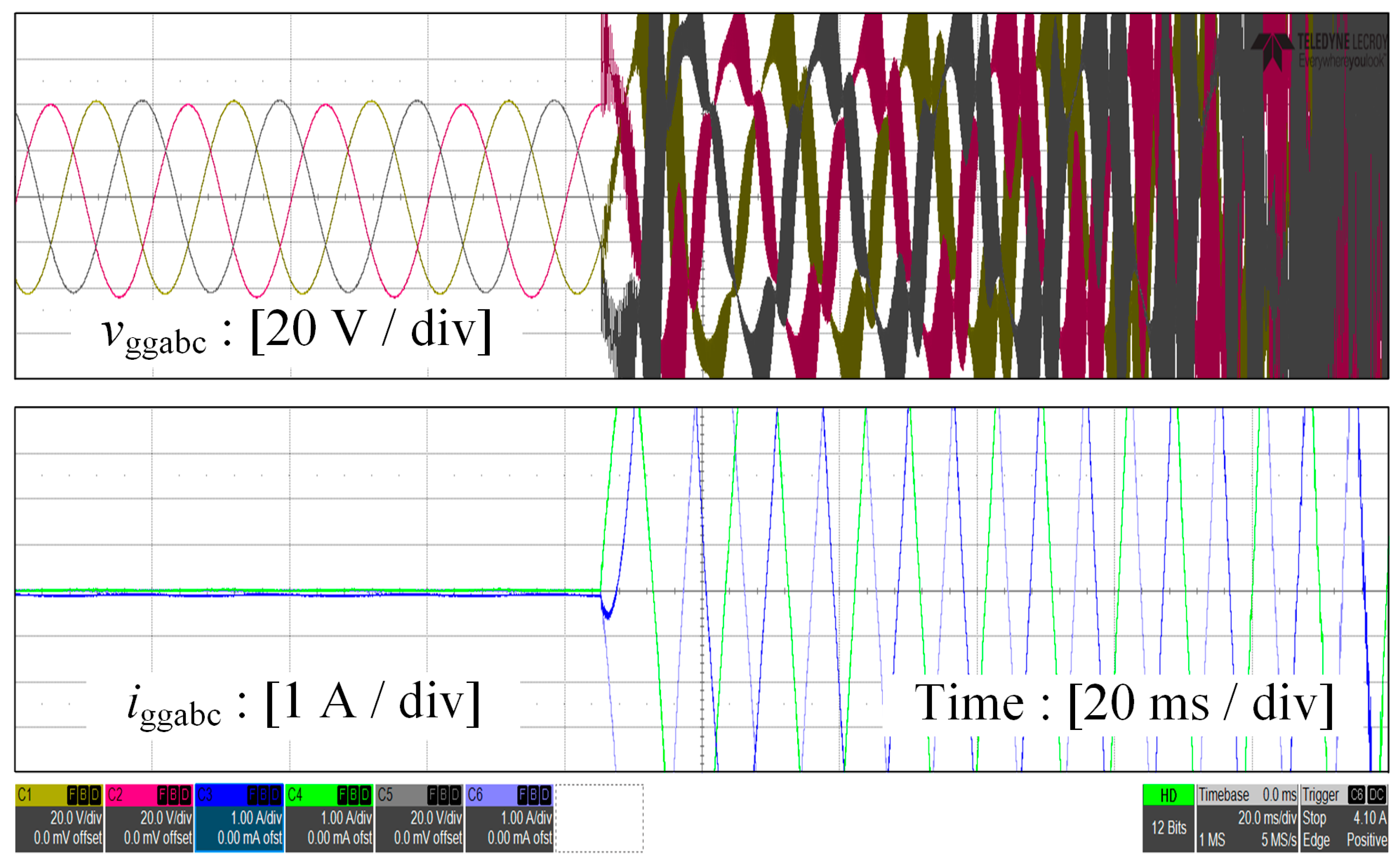
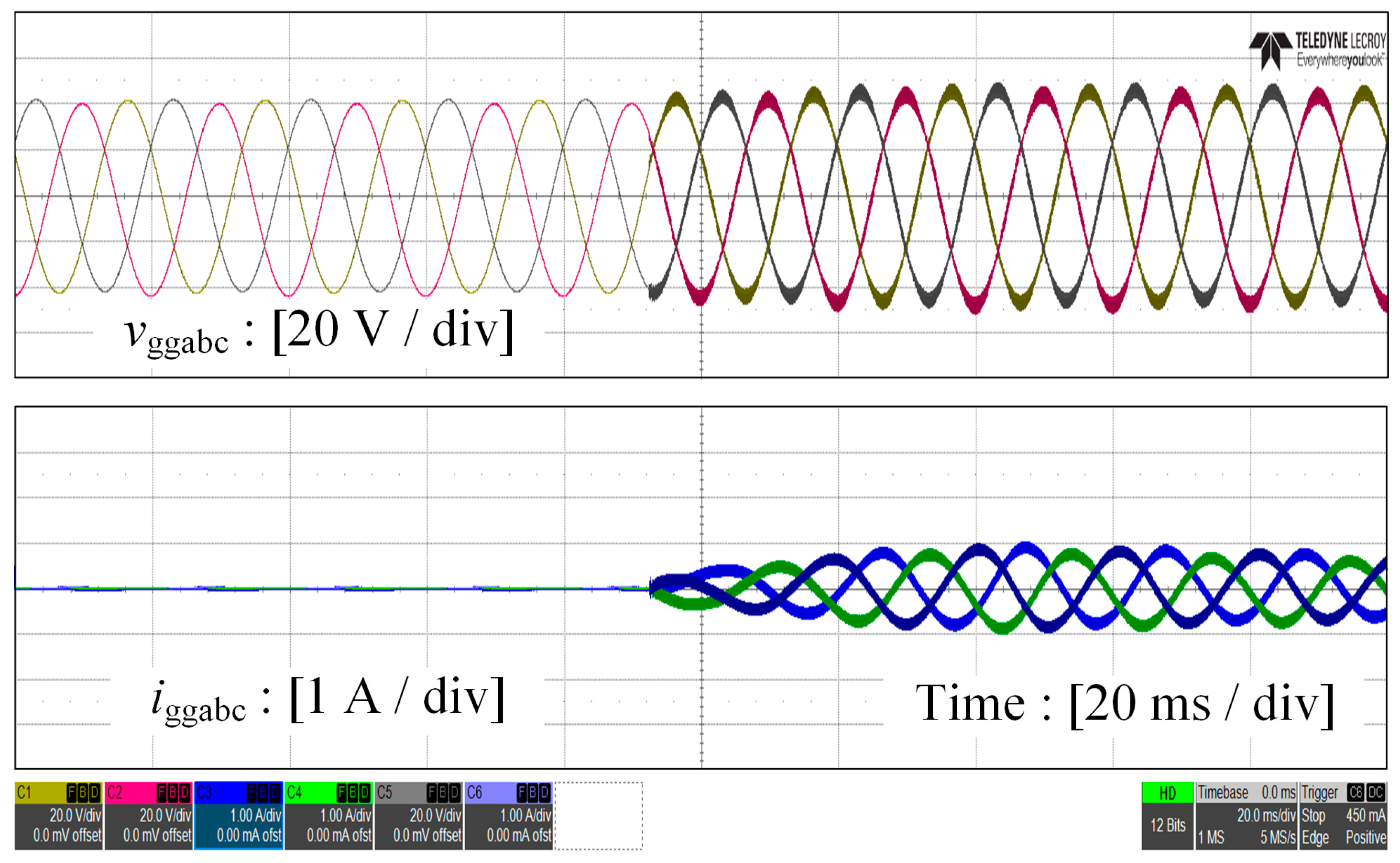
5.2. Experimental Results
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Carrasco, J.M.; Franquelo, L.G.; Bialasiewicz, J.T.; Galvan, E.; PortilloGuisado, R.; Prats, M.A.M.; Leon, J.I.; Moreno-Alfonso, N. Power-electronic systems for the grid integration of renewable energy sources: A survey. IEEE Trans. Ind. Electron. 2006, 53, 1002–1016. [Google Scholar] [CrossRef]
- Bragard, M.; Soltau, N.; Thomas, S.; De Doncker, R.W. The balance of renewable sources and user demands in grids: Power electronics for modular battery energy storage systems. IEEE Trans. Power Electron. 2010, 25, 3049–3056. [Google Scholar] [CrossRef]
- Fang, J. More-Electronics Power Systems: Power Quality and Stability; Springer: Berlin/Heidelberg, Germany, 2020; pp. 135–172. [Google Scholar]
- Fang, J.; Li, X.; Li, H.; Tang, Y. Stability improvement for three-phase grid-connected converters through impedance reshaping in quadrature-axis. IEEE Trans. Power Electron. 2018, 33, 8365–8375. [Google Scholar] [CrossRef]
- Chen, W.; Yang, Y.; Xie, Y.; Chang, X.; Lin, Y.; Yu, H.; Zhang, J. Subsynchronous oscillation mechanism of large-scale photovoltaic power generation connected to the grid through series compensation. Power Syst. Technol. 2021, 45, 4711–4721. [Google Scholar]
- Li, G.; Wang, W.; Guo, J.; Chen, X.; Liu, C.; He, G. Mechanism and analytical methods of wide-band oscillations in wind farms using MMC-HVDC transmission systems. Proc. CSEE 2019, 39, 5281–5297. [Google Scholar]
- Du, W.; Chen, Z.; Schneider, K.P.; Lasseter, R.H.; Nandanoori, S.P.; Tuffner, F.K.; Kundu, S. A comparative study of two widely used grid-forming droop controls on microgrid small-signal stability. IEEE J. Emerg. Sel. Top. Power Electron. 2020, 8, 963–975. [Google Scholar] [CrossRef]
- Pan, D.; Wang, X.; Liu, F.; Shi, R. Transient stability of voltage-source converters with grid-forming control: A design-oriented study. IEEE J. Emerg. Sel. Top. Power Electron. 2020, 8, 1019–1033. [Google Scholar] [CrossRef]
- Chen, M.; Zhou, D.; Blaabjerg, F. Enhanced transient angle stability control of grid-forming converter based on virtual synchronous generator. IEEE Trans. Ind. Electron. 2022, 69, 9133–9144. [Google Scholar] [CrossRef]
- Rosso, R.; Wang, X.; Liserre, M.; Lu, X.; Engelken, S. Grid-forming converters: Control approaches, grid-synchronization, and future trends—A review. IEEE Open J. Ind. Appl. 2021, 2, 93–109. [Google Scholar] [CrossRef]
- Awal, M.A.; Yu, H.; Lukic, S.; Husain, I. Droop and oscillator based grid-forming converter controls: A comparative performance analysis. Front. Energy Res. 2020, 8, 168. [Google Scholar] [CrossRef]
- Liu, P.; Xie, X.; Shair, J. Adaptive hybrid grid-forming and grid-following control of IBRs with enhanced small-signal stability under varying SCRs. IEEE Trans. Power Electron. 2024, 39, 6603–6607. [Google Scholar] [CrossRef]
- Wu, Y.; Wu, H.; Zhao, F.; Li, Z.; Wang, X. Influence of PLL on stability of interconnected grid-forming and grid-following converters. IEEE Trans. Power Electron. 2024, 39, 11980–11985. [Google Scholar] [CrossRef]
- Poolla, B.K.; Gros, D.; Dorfler, F. Placement and implementation of grid-forming and grid-following virtual inertia and fast frequency response. IEEE Trans. Power Syst. 2019, 34, 3035–3046. [Google Scholar] [CrossRef]
- Hoseini, S.M.; Sadeghzadeh, S.M.; Beromi, Y.A. A new method for active power factor correction using a dual-purpose inverter in a flyback converter. Turk. J. Electr. Eng. Comput. Sci. 2016, 24, 4736–4750. [Google Scholar] [CrossRef]
- Fu, S.; Sun, Y.; Li, L.; Liu, Z.; Han, H.; Su, M. Power oscillation suppression in multi-VSG grid by adaptive virtual impedance control. IEEE Syst. J. 2022, 16, 4744–4755. [Google Scholar] [CrossRef]
- Wen, B.; Boroyevich, D.; Burgos, R.; Mattavelli, P.; Shen, Z. Analysis of D-Q small-signal impedance of grid-tied inverters. IEEE Trans. Power Electron. 2016, 31, 675–687. [Google Scholar] [CrossRef]
- Yang, C.; Huang, L.; Xin, H.; Ju, P. Placing grid-forming converters to enhance small signal stability of PLL-integrated power systems. IEEE Trans. Power Syst. 2021, 36, 3563–3573. [Google Scholar] [CrossRef]
- Kawabata, T.; Higashino, S. Parallel operation of voltage source inverters. IEEE Trans. Ind. Appl. 1988, 24, 281–287. [Google Scholar] [CrossRef]
- D’Arco, S.; Suul, J.A. Equivalence of virtual synchronous machines and frequency-droops for converter-based microgrids. IEEE Trans. Smart Grid 2014, 5, 394–395. [Google Scholar] [CrossRef]
- IEEE Standard 2800-2022; IEEE Standard for Interconnection and Interoperability of Inverter Based Resources (IBRs) Interconnecting with Associated Transmission Electric Power Systems. IEEE: Piscataway, NJ, USA, April 2022; pp. 1–180.
- European Connection Conditions. National Grid ESO Grid Code. May 2023. Available online: https://www.neso.energy/document/287051/download (accessed on 17 October 2024).



| Descriptions | Symbols | Values |
|---|---|---|
| DC voltage reference | Vdc | 400 V |
| Grid voltage | Vs | 110 Vrms |
| Converter-side inductance inductance | Lgi | 2 mH |
| Grid-side inductance | Lgg | 2 mH |
| Line inductance | Ls | 10 mH |
| Filter capacitance in GFMC | Cgf1 | 1 μF |
| Filter capacitance in GFLC | Cgf2 | 5 μF |
| Nominal frequency | f0 | 50 Hz |
| Sampling/switching frequency | fs/fsw | 10 kHz |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qiu, L.; Gu, M.; Chen, Z.; Du, Z.; Zhang, L.; Li, W.; Huang, J.; Fang, J. Oscillation Suppression of Grid-Following Converters by Grid-Forming Converters with Adaptive Droop Control. Energies 2024, 17, 5230. https://doi.org/10.3390/en17205230
Qiu L, Gu M, Chen Z, Du Z, Zhang L, Li W, Huang J, Fang J. Oscillation Suppression of Grid-Following Converters by Grid-Forming Converters with Adaptive Droop Control. Energies. 2024; 17(20):5230. https://doi.org/10.3390/en17205230
Chicago/Turabian StyleQiu, Lifeng, Miaosong Gu, Zhongjiang Chen, Zhendong Du, Ligang Zhang, Wenrui Li, Jingyi Huang, and Jingyang Fang. 2024. "Oscillation Suppression of Grid-Following Converters by Grid-Forming Converters with Adaptive Droop Control" Energies 17, no. 20: 5230. https://doi.org/10.3390/en17205230
APA StyleQiu, L., Gu, M., Chen, Z., Du, Z., Zhang, L., Li, W., Huang, J., & Fang, J. (2024). Oscillation Suppression of Grid-Following Converters by Grid-Forming Converters with Adaptive Droop Control. Energies, 17(20), 5230. https://doi.org/10.3390/en17205230







