New Thermochemical Salt Hydrate System for Energy Storage in Buildings
Abstract
1. Introduction
2. Materials and Methods
2.1. Thermogravimetric Analysis of the Salt and Composite
2.2. Sample Preparation
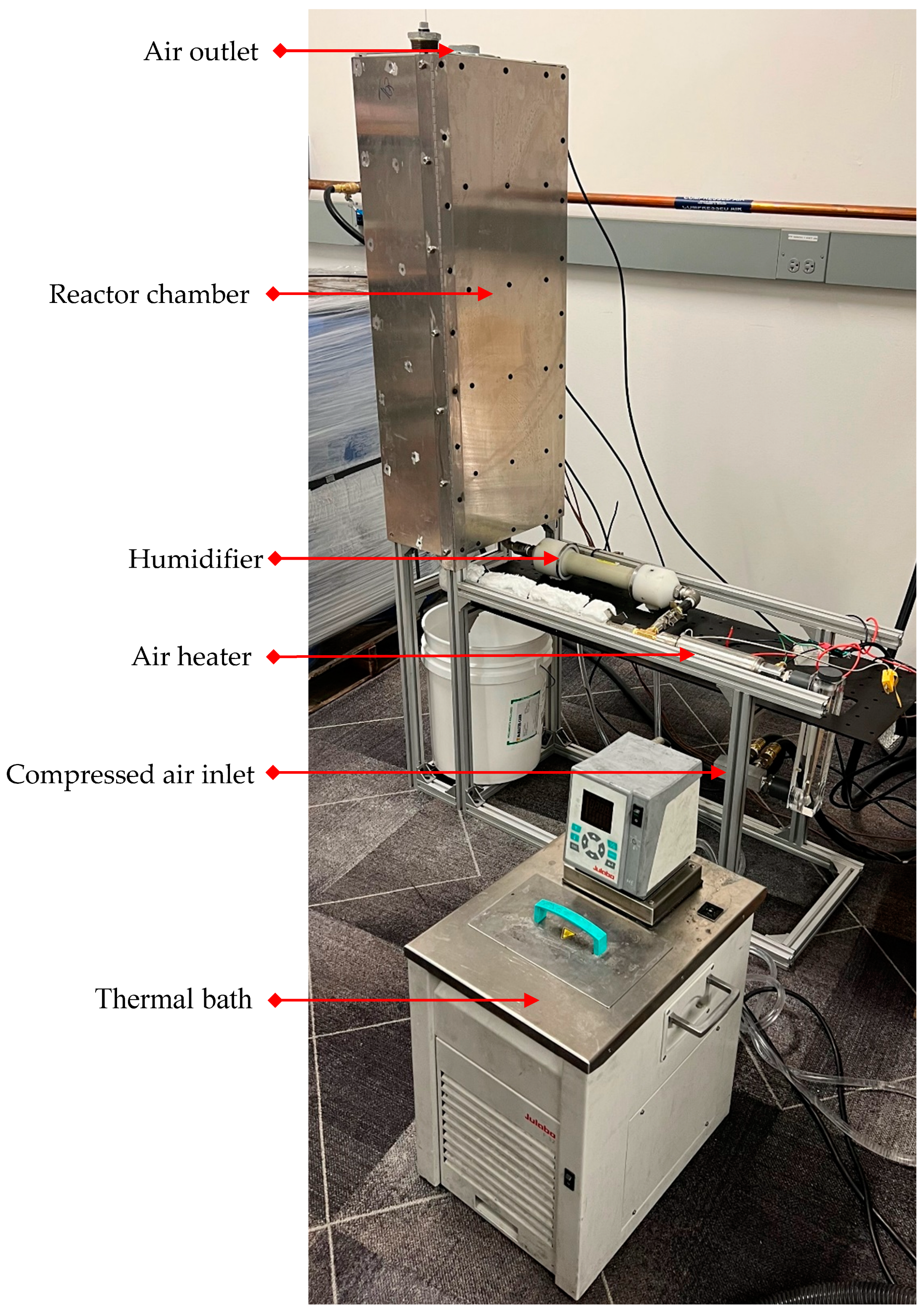
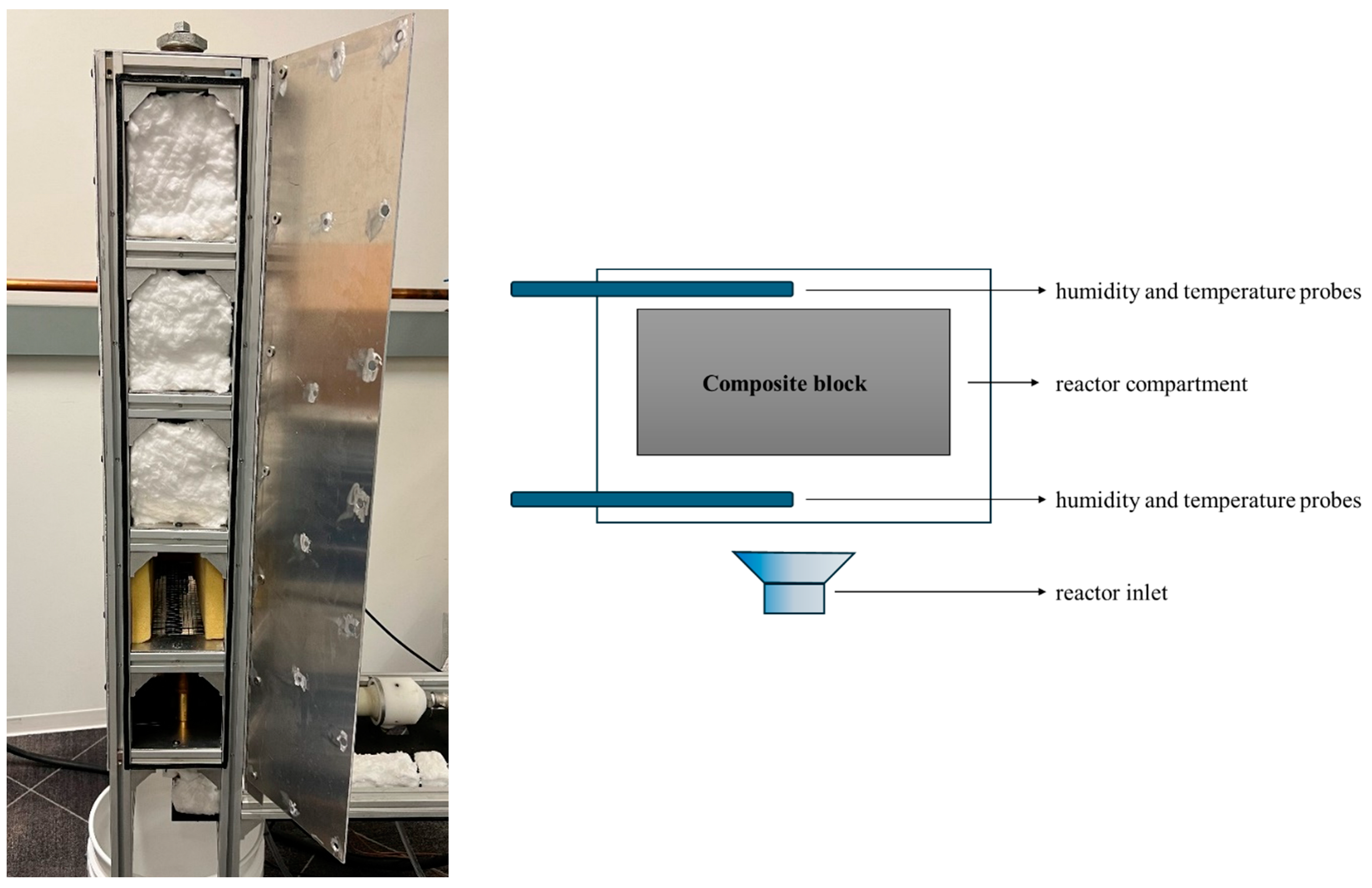
2.3. Thermochemical Reactor Design and Construction
2.4. Energy Analysis
2.4.1. Analysis of the Environmental Chamber
2.4.2. Analysis for the Reactor
Hydration Reaction
Dehydration Reaction
Total Balance
3. Results and Discussion
3.1. Thermogravimetric Analysis of the Salt and Composite
3.2. Composite Block Cycling in the Environmental Test Chamber
- Hydration reaction at 20 °C and 40% RH for 17 h;
- Dehydration reaction at 150 °C overnight;
- Repeat.
3.3. Composite Block Cycling in the Reactor
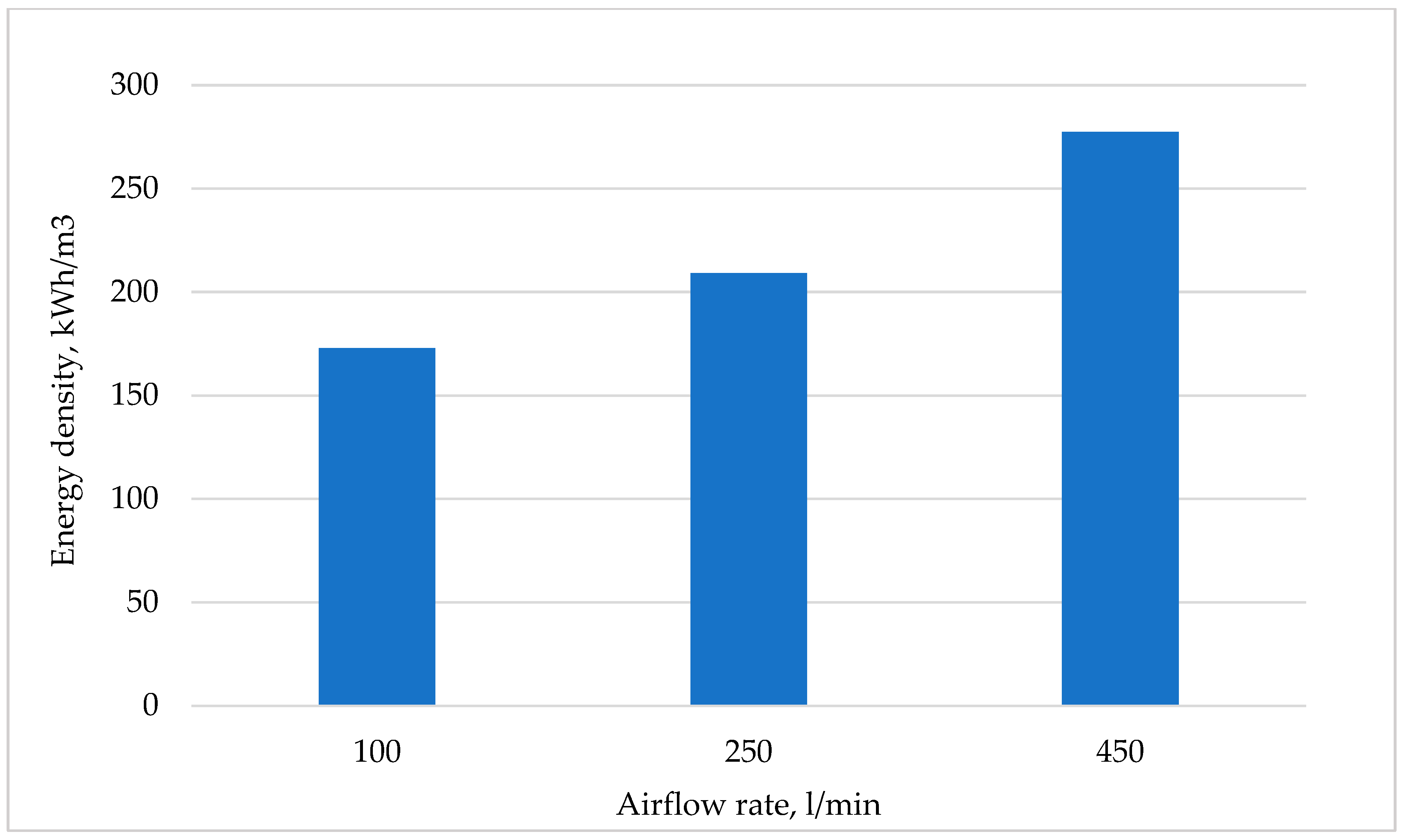
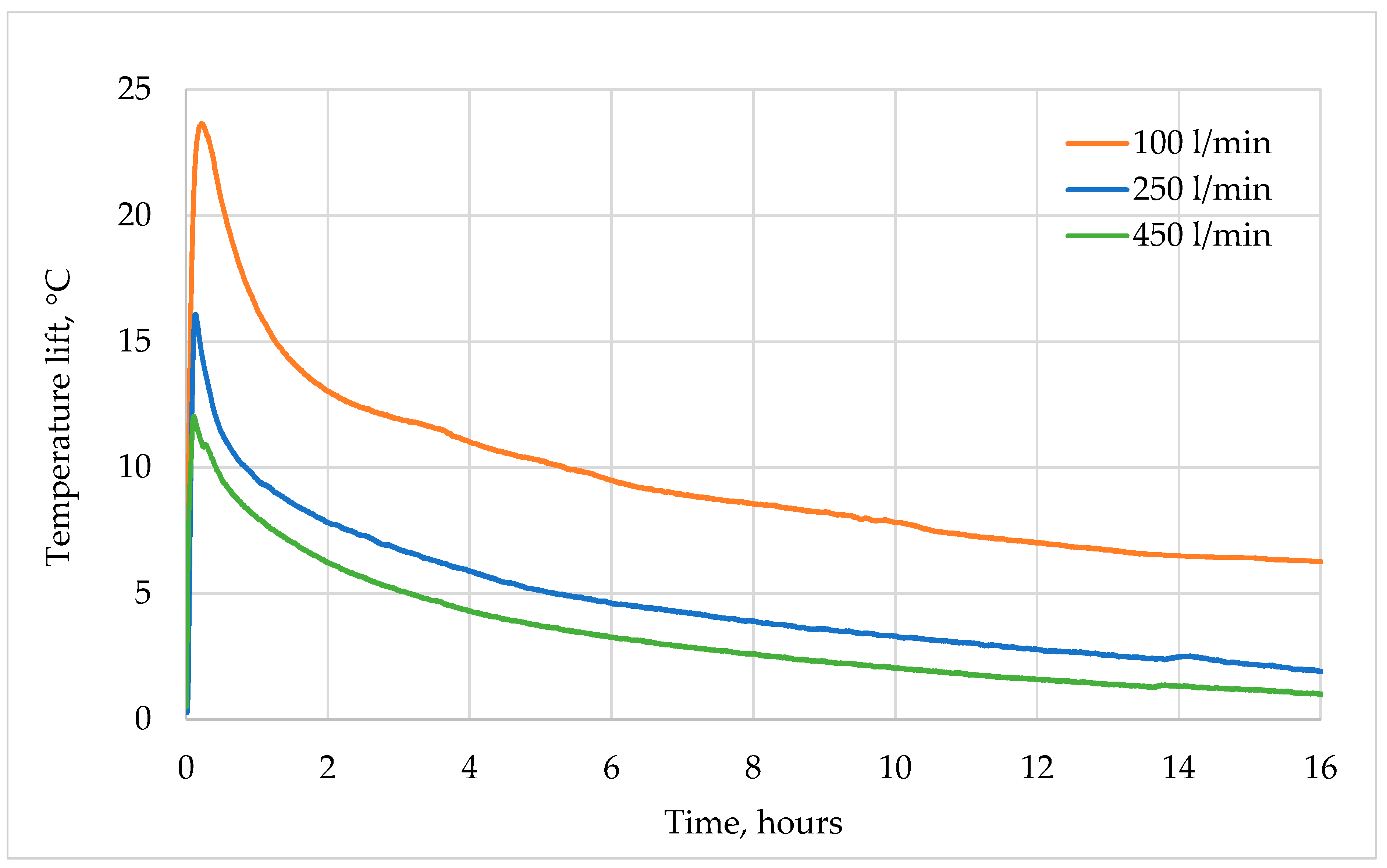
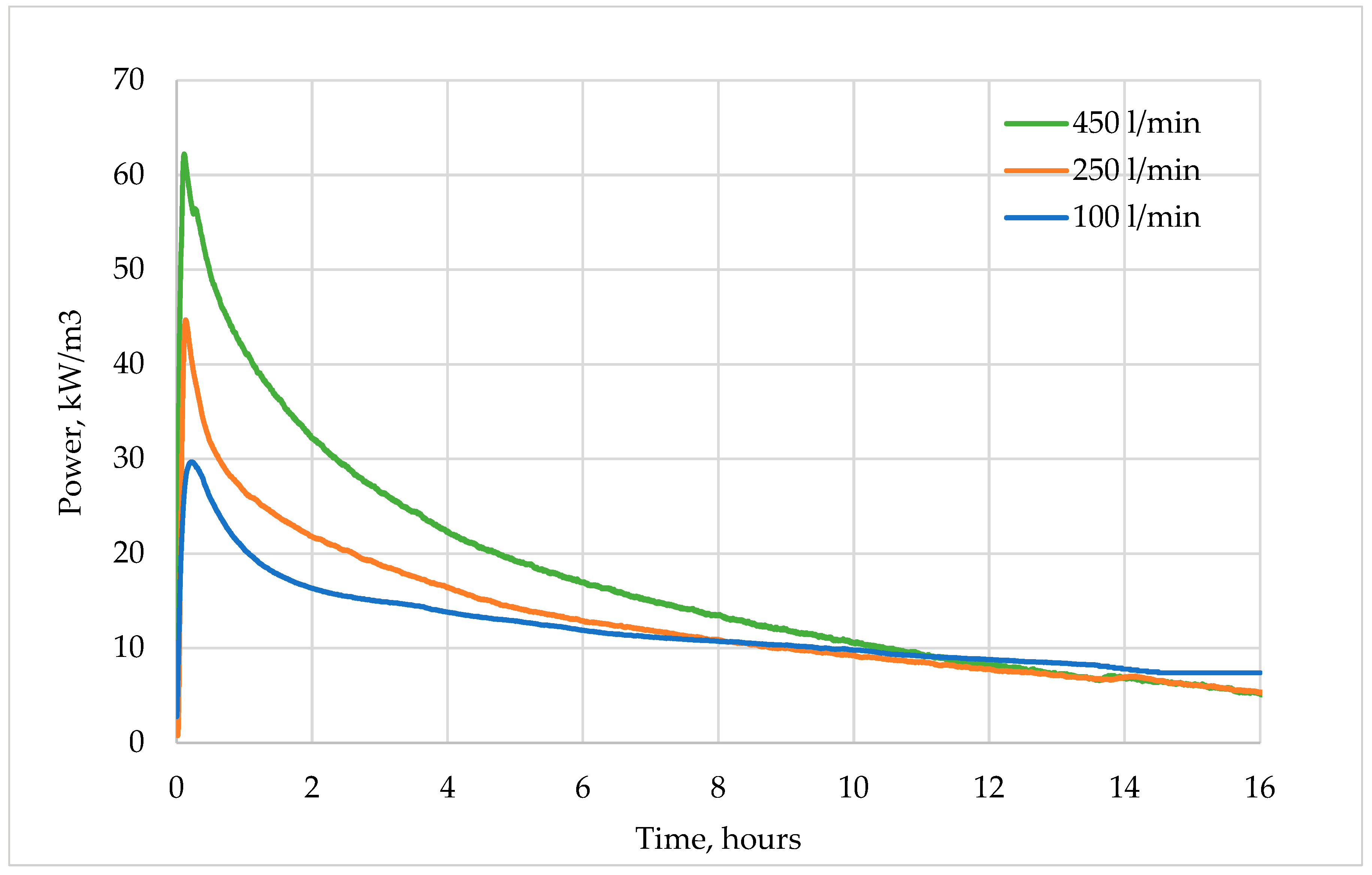
3.3.1. Effect of the Airflow Rate
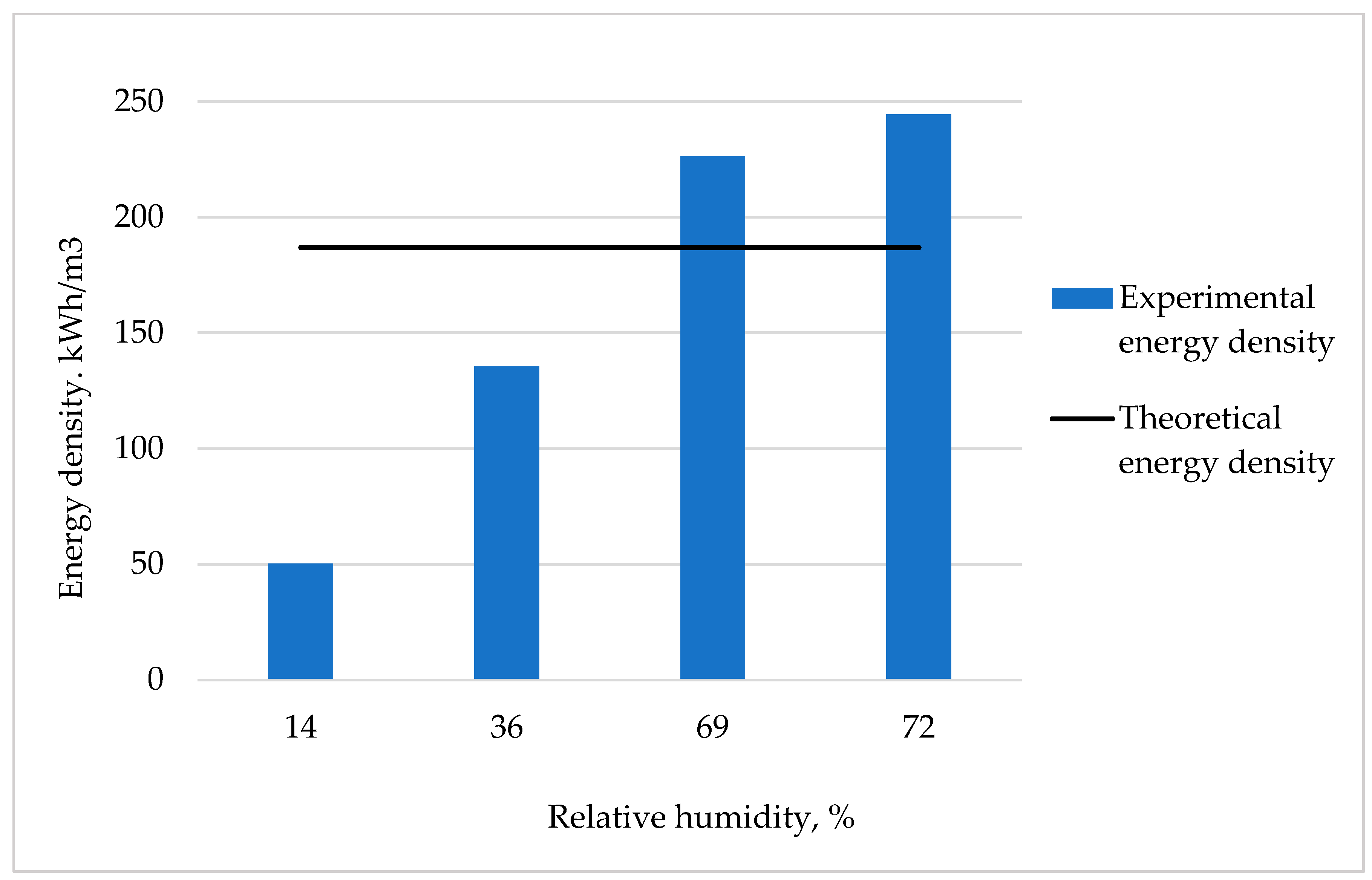
3.3.2. Effect of Relative Humidity
3.3.3. Cycling Performance
3.4. Energy Density and Thermal Efficiency Analysis
3.5. Comparison to the State of the Art
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Qrecovered | Volumetric heat recovered, kW/m3 |
| Qsensible | Volumetric sensible heat, kW/m3 |
| Qloss | Volumetric heat loss, kW/m3 |
| Qinput | Volumetric heat input, kW/m3 |
| Qreaction | Volumetric heat of reaction, kW/m3 |
| Volumetric flow rate, m3/s | |
| Specific volume of dry air, m3/kg | |
| T | Temperature, K, °C |
| Absolute humidity, kgwater/kgair | |
| Volume of material, m3 | |
| m | Mass, kg |
| Specific heat capacity, kJ/kg·K | |
| Etot | Overall energy storage density, kWh/m3 |
| η | Thermal efficiency |
| UA | heat loss coefficient, kW/K |
| Indices | |
| in | Inlet air |
| out | Outlet air |
| amb | Ambient air |
| da | dry air |
| com | composite |
References
- Lind, J.; Möllerström, E.; Averfalk, H.; Ottermo, F. Energy flexibility using the thermal mass of residential buildings. Energy Build. 2023, 301, 113698. [Google Scholar] [CrossRef]
- De Gracia, A.; Cabeza, L.F. Phase change materials and thermal energy storage for buildings. Energy Build. 2015, 103, 414–419. [Google Scholar] [CrossRef]
- Candanedo, J.A.; Dehkordi, V.R.; Stylianou, M. Model-based predictive control of an ice storage device in a building cooling system. Appl. Energy 2013, 111, 1032–1045. [Google Scholar] [CrossRef]
- Toffoletti, G.; Cortella, G.; D’Agaro, P. Thermodynamic and economic seasonal analysis of a transcritical CO2 supermarket with HVAC supply through ice thermal energy storage (ITES). J. Clean. Prod. 2024, 434, 139832. [Google Scholar] [CrossRef]
- Clark, R.J.; Mehrabadi, A.; Farid, M. State of the art on salt hydrate thermochemical energy storage systems for use in building applications. J. Energy Storage 2020, 27, 10114. [Google Scholar] [CrossRef]
- Marín, P.E.; Milian, Y.; Ushak, S.; Cabeza, L.F.; Grágeda, M.; Shire, G.S.F. Lithium compounds for thermochemical energy storage: A state-of-the-art review and future trends. Renew. Sustain. Energy Rev. 2021, 149, 111381. [Google Scholar] [CrossRef]
- Yan, T.; Zhang, H. A critical review of salt hydrates as thermochemical sorption heat storage materials: Thermophysical properties and reaction kinetics. Sol. Energy 2022, 242, 157–183. [Google Scholar] [CrossRef]
- Tregambi, C.; Troiano, M.; Montagnaro, F.; Solimene, R.; Salatino, P. Fluidized Beds for Concentrated Solar Thermal Technologies—A Review. Front. Energy Res. 2021, 9, 618421. [Google Scholar] [CrossRef]
- Farulla, G.A.; Cellura, M.; Guarino, F.; Ferraro, M. A review of thermochemical energy storage systems for power grid support. Appl. Sci. 2020, 10, 3142. [Google Scholar] [CrossRef]
- Michel, B.; Mazet, N.; Neveu, P. Experimental investigation of an open thermochemical process operating with a hydrate salt for thermal storage of solar energy: Local reactive bed evolution. Appl. Energy 2016, 180, 234–244. [Google Scholar] [CrossRef]
- Zsembinszki, G.; Sole, A.; Barreneche, C.; Prieto, C.; Fernández, A.I.; Cabeza, L.F. Review of reactors with potential use in thermochemical energy storage in concentrated solar power plants. Energies 2018, 11, 2358. [Google Scholar] [CrossRef]
- Wokon, M.; Kohzer, A.; Linder, M. Investigations on thermochemical energy storage based on technical grade manganese-iron oxide in a lab-scale packed bed reactor. Sol. Energy 2017, 153, 200–214. [Google Scholar] [CrossRef]
- Dreißigacker, V.; Zunft, S.; Müller-Steinhagen, H. A thermo-mechanical model of packed-bed storage and experimental validation. Appl. Energy 2013, 111, 1120–1125. [Google Scholar] [CrossRef]
- Zhang, Y.N.; Wang, R.Z.; Li, T.X. Experimental investigation on an open sorption thermal storage system for space heating. Energy 2017, 141, 2421–2433. [Google Scholar] [CrossRef]
- Clark, R.J.; Farid, M. Experimental investigation into the performance of novel SrCl2-based composite material for thermochemical energy storage. J. Energy Storage 2021, 36, 102390. [Google Scholar] [CrossRef]
- Courbon, E.; D’Ans, P.; Skrylnyk, O.; Frère, M. New prominent lithium bromide-based composites for thermal energy storage. J. Energy Storage 2020, 32, 101699. [Google Scholar] [CrossRef]
- Bérut, E.; Bois, L.; Touloumet, Q.; Outin, J.; Ondarts, M.; Postole, G.; López, M.J.R.; Auroux, A.; Le Pierrès, N. Characterization of silica-PEG-CaCl2 composite sorbents in an open thermochemical heat storage reactor. J. Energy Storage 2023, 72, 108632. [Google Scholar] [CrossRef]
- Gbenou, T.R.S.; Fopah-Lele, A.; Wang, K. Macroscopic and microscopic investigations of low-temperature thermochemical heat storage reactors: A review. Renew. Sustain. Energy Rev. 2022, 161, 112152. [Google Scholar] [CrossRef]
- Liu, H.; Nagano, K.; Sugiyama, D.; Togawa, J.; Nakamura, M. Honeycomb filters made from mesoporous composite material for an open sorption thermal energy storage system to store low-temperature industrial waste heat. Int. J. Heat Mass Transf. 2013, 65, 471–480. [Google Scholar] [CrossRef]
- Van Essen, V.M.; Gores, J.C.; Bleijendaal, L.P.J.; Zondag, H.A.; Schuitema, R.; Bakker, M.; van Helden, W.G.J. Characterization of salt hydrates for compact seasonal thermochemical storage. In Proceedings of the ASME 3rd International Conference on Energy Sustainability 2009, ES2009, San Francisco, CA, USA, 19–23 July 2009. [Google Scholar] [CrossRef]
- Clark, R.J.; Gholamibozanjani, G.; Woods, J.; Kaur, S.; Odukomaiya, A.; Al-Hallaj, S.; Farid, M. Experimental screening of salt hydrates for thermochemical energy storage for building heating application. J. Energy Storage 2022, 51, 104415. [Google Scholar] [CrossRef]
- N’Tsoukpoe, K.E.; Schmidt, T.; Rammelberg, H.U.; Watts, B.A.; Ruck, W.K.L. A systematic multi-step screening of numerous salt hydrates for low temperature thermochemical energy storage. Appl. Energy 2014, 124, 1–16. [Google Scholar] [CrossRef]
- Courbon, E.; D’Ans, P.; Permyakova, A.; Skrylnyk, O.; Steunou, N.; Degrez, M.; Frère, M. A new composite sorbent based on SrBr2 and silica gel for solar energy storage application with high energy storage density and stability. Appl. Energy 2017, 190, 1184–1194. [Google Scholar] [CrossRef]
- Mills, A.; Farid, M.; Selman, J.R.; Al-Hallaj, S. Thermal conductivity enhancement of phase change materials using a graphite matrix. Appl. Therm. Eng. 2006, 26, 1652–1661. [Google Scholar] [CrossRef]
- Barreneche, C.; Fernández, A.I.; Cabeza, L.F.; Cuypers, R. Thermophysical characterization and thermal cycling stability of two TCM: CaCl2 and zeolite. Appl. Energy 2015, 137, 726–730. [Google Scholar] [CrossRef]
- Donkers, P.A.J.; Adan, O.C.G.; Smeulders, D.M.J. Hydration/Dehydration Processes in Stabilized CaCl2. In Proceedings of the 6th Biot Conference on Poromechanics, Paris, France, 9–13 July 2017. [Google Scholar] [CrossRef]
- Restuccia, G.; Freni, A.; Vasta, S.; Aristov, Y. Selective water sorbent for solid sorption chiller: Experimental results and modelling. Int. J. Refrig. 2004, 27, 284–293. [Google Scholar] [CrossRef]
- Iyimen-Schwarz, Z.; Lechner, M.D. Energiespeicherung durch chemische reaktionen. I. DSC-messungen zur quantitativen verfolgung der enthalpieänderungen von speicherstoffen für die hin- und rückreaktion. Thermochim. Acta 1983, 68, 349–361. [Google Scholar] [CrossRef]
- Lemmon, E.W.; Jacobsen, R.T.; Penoncello, S.G.; Friend, D.G. Thermodynamic properties of air and mixtures of nitrogen, argon, and oxygen from 60 to 2000 K at pressures to 2000 MPa. J. Phys. Chem. Ref. Data 2000, 29, 331–385. [Google Scholar] [CrossRef]
- Dinçer, İ.; Zamfirescu, C. Drying Phenomena: Theory and Applications; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 2015. [Google Scholar] [CrossRef]
- Miao, Q.; Zhang, Y.; Jia, X.; Tan, L.; Ding, Y. MgSO4-expanded graphite composites for mass and heat transfer enhancement of thermochemical energy storage. Solar Energy 2021, 220, 432–439. [Google Scholar] [CrossRef]
- Xu, C.; Yu, Z.; Xie, Y.; Ren, Y.; Ye, F.; Ju, X. Study of the hydration behavior of zeolite-MgSO4 composites for long-term heat storage. Appl. Therm. Eng. 2018, 129, 250–259. [Google Scholar] [CrossRef]
- Michel, B.; Mazet, N.; Neveu, P. Experimental investigation of an innovative thermochemical process operating with a hydrate salt and moist air for thermal storage of solar energy: Global performance. Appl. Energy 2014, 129, 177–186. [Google Scholar] [CrossRef]
- Molenda, M.; Stengler, J.; Linder, M.; Wörner, A. Reversible hydration behavior of CaCl2 at high H2O partial pressures for thermochemical energy storage. Thermochim. Acta 2013, 560, 76–81. [Google Scholar] [CrossRef]
- Farcot, L.; Le Pierrès, N.; Michel, B.; Fourmigué, J.F.; Papillon, P. Numerical investigations of a continuous thermochemical heat storage reactor. J. Energy Storage 2018, 20, 109–119. [Google Scholar] [CrossRef]
- Clark, R.J.; Farid, M. Hydration reaction kinetics of SrCl2 and SrCl2-cement composite material for thermochemical energy storage. Sol. Energy Mater. Sol. Cells 2021, 231, 111311. [Google Scholar] [CrossRef]
- Sögütoglu, L.C.; Birkelbach, F.; Werner, A.; Fischer, H.; Huinink, H.; Adan, O. Hydration of salts as a two-step process: Water adsorption and hydrate formation. Thermochim. Acta 2021, 695, 178819. [Google Scholar] [CrossRef]
- Chen, B.; Johannes, K.; Horgnies, M.; Morin, V.; Kuznik, F. Characterization of an ettringite-based thermochemical energy storage material in an open-mode reactor. J. Energy Storage 2021, 33, 102159. [Google Scholar] [CrossRef]
- Clark, R.J.; Farid, M. Experimental investigation into cascade thermochemical energy storage system using SrCl2-cement and zeolite-13X materials. Appl. Energy 2022, 316, 119145. [Google Scholar] [CrossRef]





| Sample | Impregnation Type | Sample Dimensions, [mm] | EG Density, [kg/m3] | Anhydrous Salt Content, [wt.%] | Perforated Block Volume (Solid), [m3] | Perforated Block Volume (Total), [m3] |
|---|---|---|---|---|---|---|
| Block 1 | molten | 117 × 75 × 75 | 100 | 62 | 0.00047 | 0.000655 |
| Block 2 | molten | 275 × 120 × 73 | 100 | 63 | 0.00171 | 0.00241 |
| Cycle | Energy Density per Solid Volume (Material Level), [kWh/m3] | Energy Density per Volume (Reactor Level), [kWh/m3] |
|---|---|---|
| 2 | 154.9 | 110.1 |
| 6 | 136.5 | 97.0 |
| 10 | 160.6 | 114.1 |
| Sample | Anhydrous Salt Content [wt.%] | Mass of Anhydrous Salt, [kg] | Mass of Water Required for the Formation of Solid Salt, [kg] | Theoretical Heat of Reaction, [kJ/kgwater] | Max Theoretical Energy Density (Material Level), [kWh/m3] | Max Theoretical Energy Density (Reactor Level), [kWh/m3] |
|---|---|---|---|---|---|---|
| Block 2 | 62.9 | 0.3697 | 0.3595 | 3200 [28] | 186.9 | 132.6 |
| Cycle | Energy Density per Solid Volume (Material), [kWh/m3] | Energy Density per Total Volume (Reactor), [kWh/m3] | Absolute Humidity, [kgwater/kgair] | Inlet Temperature, [°C] |
|---|---|---|---|---|
| 6 | 205.1 | 144.8 | 0.009 | 19 |
| 15 | 212.5 | 150.0 | 0.010 | 20 |
| 23 | 222.8 | 157.3 | 0.009 | 18 |
| 31 | 220.3 | 155.5 | 0.010 | 19 |
| 45 | 228.2 | 161.1 | 0.010 | 22 |
| 52 | 224.7 | 158.7 | 0.010 | 21 |
| 60 | 217.5 | 153.5 | 0.010 | 22 |
| 69 | 209.7 | 148.1 | 0.009 | 20 |
| 76 | 222.5 | 157.1 | 0.011 | 21 |
| 84 | 226.3 | 159.8 | 0.010 | 20 |
| Average | 219.0 ± 2.3 | 154.6 ± 1.6 | 0.010 ± 0.0002 | 20 ± 0.4 |
| Cycle | Absolute Humidity, [kgwater/kgair] | Inlet Temperature, [°C] | Airflow Rate, [l/min] | Volumetric Energy Density (Material), [kWh/m3] | Sensible Heat, [kWh/m3] | Heat Loss, [kWh/m3] | Thermal Efficiency | |||
|---|---|---|---|---|---|---|---|---|---|---|
| H * | D ** | H | D | H | D | |||||
| Effect of inlet temperature | ||||||||||
| 38 | 0.0062 | 23 | 400 | 135.5 | 202.2 | 0.2 | 16.7 | 1.9 | 23.7 | 0.8 |
| 36 | 0.0068 | 13 | 400 | 235.5 | 267.3 | −1.3 | 17.1 | −7.7 | 21.8 | 1.0 |
| 40 | 0.0075 | 14 | 400 | 242.3 | 285.9 | −1.2 | 17.1 | −5.9 | 23.1 | 1.0 |
| Effect of relative humidity | ||||||||||
| 38 | 0.0062 | 23 | 400 | 135.5 | 202.2 | 0.2 | 16.7 | 1.9 | 23.7 | 0.8 |
| 18 | 0.0097 | 20 | 400 | 223.8 | 245.0 | −0.2 | 9.9 | −0.5 | 13.8 | 1.0 |
| 83 | 0.0100 | 20 | 400 | 230.2 | 286.8 | −1.8 | 17.0 | −1.7 | 22.1 | 0.9 |
| 77 | 0.0110 | 20 | 400 | 244.4 | - | −0.5 | - | −1.1 | - | - |
| Effect of airflow rate | ||||||||||
| 1 | 0.0091 | 20 | 100 | 172.9 | - | −0.4 | - | 1.9 | - | - |
| 21 | 0.0102 | 22 | 250 | 209.3 | 238.0 | −1.6 | 17.3 | 0.6 | 23.6 | 1.0 |
| 25 | 0.0097 | 21 | 450 | 277.6 | - | 0.0 | - | −0.2 | - | - |
| TCES Material | System | Reactor Energy Density, [kWh/m3] | Number of Cycles | Cost per kg, [USD] | Reference |
|---|---|---|---|---|---|
| Ettringite | Open, fixed bed | 56–104 | - | - | [38] |
| LiBr (53 wt.%), silica gel | Open, fixed bed | 160–175 | 10 | - | [16] |
| CaCl2 (32 wt.%), silica, PEG | Open, fixed bed | 154 | 4 | 220 | [17] |
| Zeolite 13X | Open, fixed bed | 124 | 5 | 1.5 | [39] |
| SrCl2·6H2O (50 wt.%), cement | Open, fixed bed, cascade | 138 | 5 | 0.9 | |
| SrCl2·6H2O (50 wt.%), cement | Open, fixed bed | 132 | 7 | 0.3 | |
| LiCl (14.7 wt.%), activated alumina | Open, fixed bed | 191 | 10 | - | [14] |
| SrBr2 | Open, fixed bed | 203 | 7 | - | [10] |
| CaCl2 (22 wt.%), mesoporous ceramic honeycomb filter | Open, fixed bed | 76 | 25 | - | [19] |
| CaCl2 (63 wt.%), EG | Open, fixed bed | 197 | 90 | 0.6 | Present work |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Galazutdinova, Y.; Clark, R.-J.; Al-Hallaj, S.; Kaur, S.; Farid, M. New Thermochemical Salt Hydrate System for Energy Storage in Buildings. Energies 2024, 17, 5228. https://doi.org/10.3390/en17205228
Galazutdinova Y, Clark R-J, Al-Hallaj S, Kaur S, Farid M. New Thermochemical Salt Hydrate System for Energy Storage in Buildings. Energies. 2024; 17(20):5228. https://doi.org/10.3390/en17205228
Chicago/Turabian StyleGalazutdinova, Yana, Ruby-Jean Clark, Said Al-Hallaj, Sumanjeet Kaur, and Mohammed Farid. 2024. "New Thermochemical Salt Hydrate System for Energy Storage in Buildings" Energies 17, no. 20: 5228. https://doi.org/10.3390/en17205228
APA StyleGalazutdinova, Y., Clark, R.-J., Al-Hallaj, S., Kaur, S., & Farid, M. (2024). New Thermochemical Salt Hydrate System for Energy Storage in Buildings. Energies, 17(20), 5228. https://doi.org/10.3390/en17205228








