Calculation Methods of High-Voltage Direct Current (HVDC) Line Sag Considering Meteorology
Abstract
1. Introduction
2. Calculation Method
2.1. Catenary Equation of Sag
2.2. Temperature Compensation Analysis of Conductor Length
2.2.1. Temperature Distribution of Conductor Strands
2.2.2. Experiment on Temperature Difference between Layers
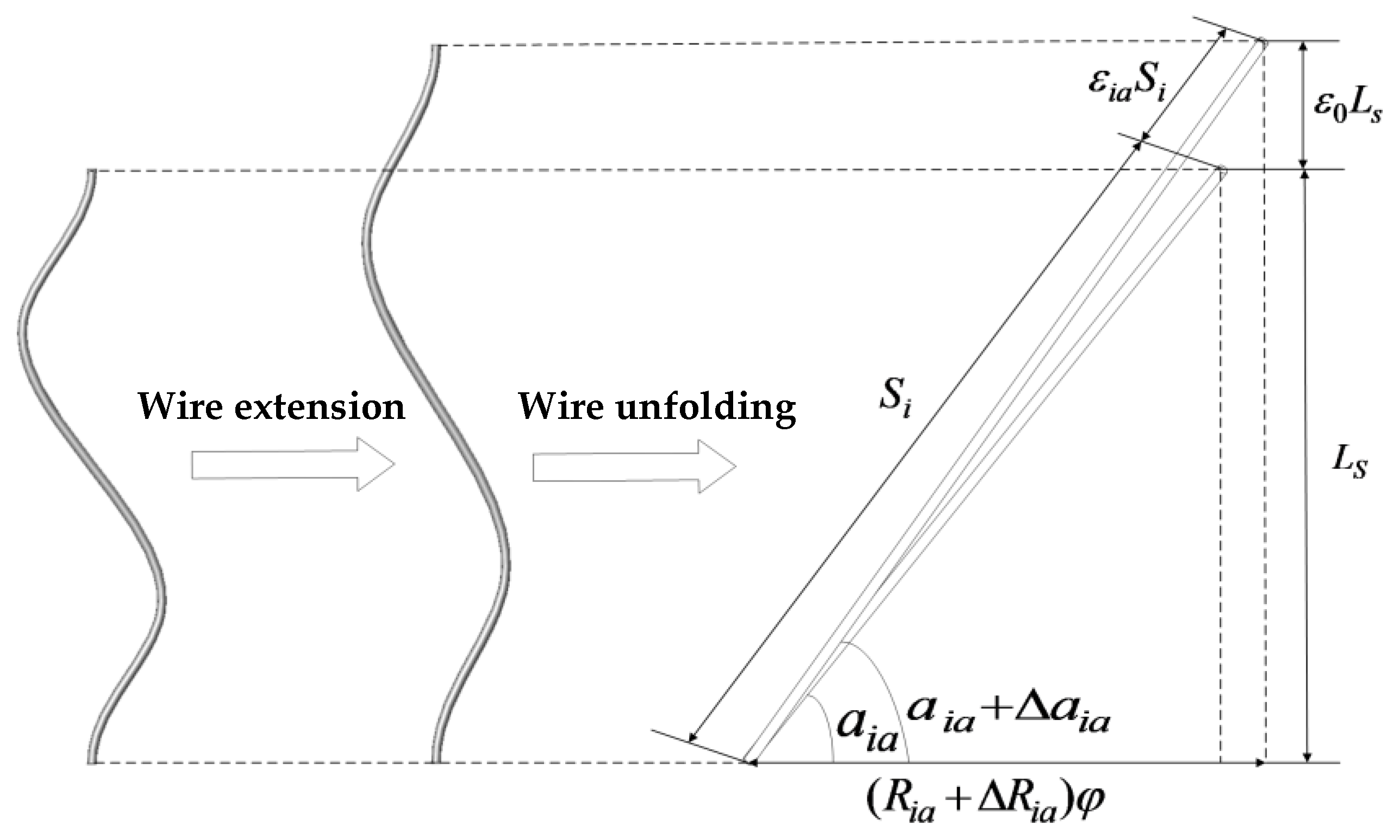
2.2.3. Length Calculation Considering Temperature Distribution
2.3. Correction Calculation Method for Sag
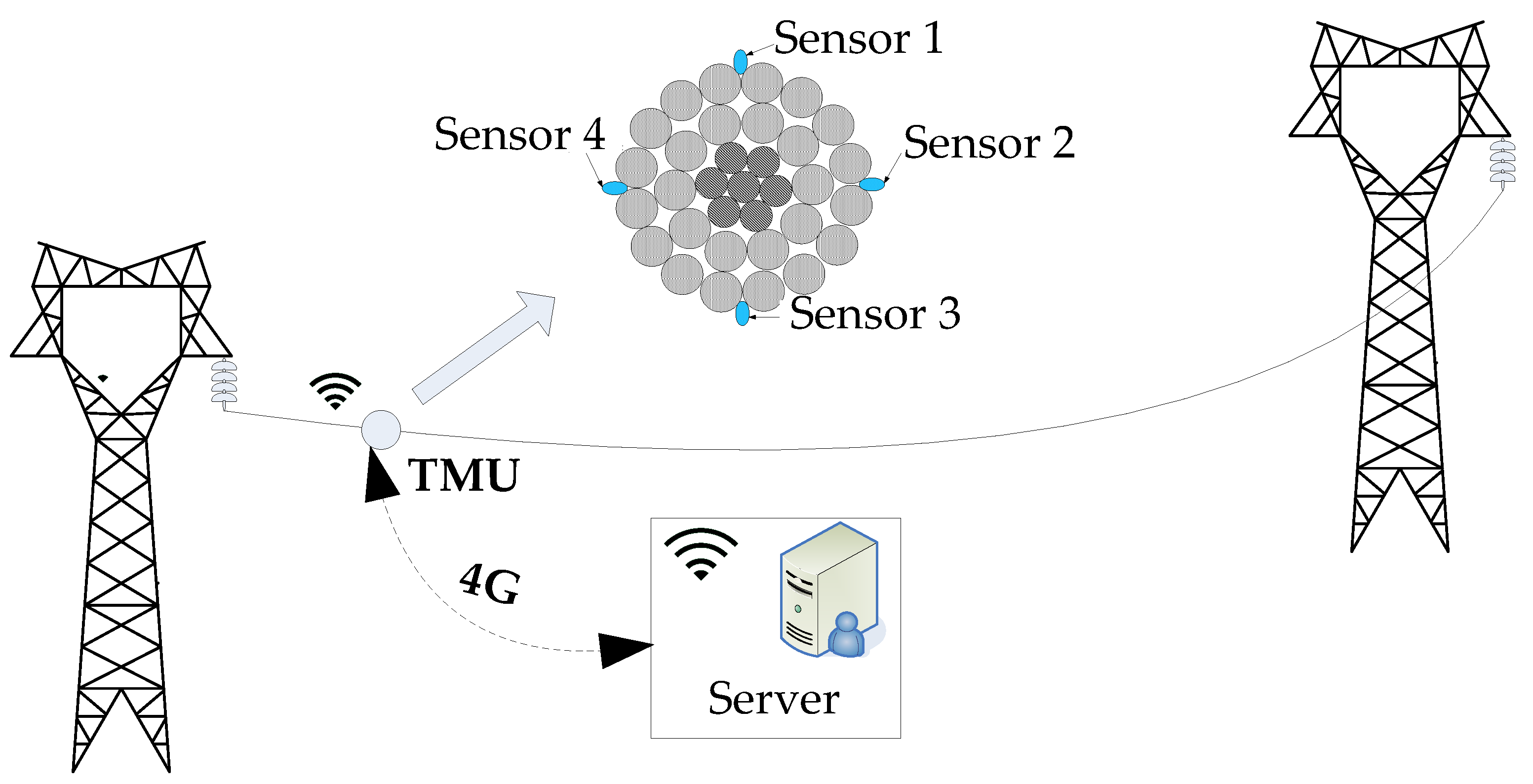
3. Sag Monitoring Method
4. Field Test and Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- IEEE Std 1863–2019; IEEE Guide for Overhead AC Transmission Line Design. IEEE: New York, NY, USA, 11 May 2020; pp. 1–109.
- Oluwajobi, F.I. Effect of sag on transmission line. J. Emerg. Trends Eng. Appl. Sci. 2012, 4, 627–630. [Google Scholar]
- Yan, D.; Liao, Y. Online estimation of power transmission line parameters, temperature and sag. In Proceedings of the North American Power Symposium, Boston, MA, USA, 4–6 August 2011. [Google Scholar]
- Polevoy, A. Impact of Data Errors on Sag Calculation Accuracy for Overhead Transmission Line. IEEE Trans. Power Deliv. 2014, 5, 2040–2045. [Google Scholar] [CrossRef]
- Barrett, J.S.; Dutta, S.; Nigol, O. A New Computer Model of ACSR Conductors. IEEE Trans. Power Appar. Syst. 1983, 3, 614–662. [Google Scholar] [CrossRef]
- Alawar, A.; Bosze, E.J.; Nutt, S.R. A hybrid numerical method to calculate the sag of composite conductors. Electr. Power Syst. Res. 2006, 5, 389–394. [Google Scholar] [CrossRef]
- Bedialauneta, M.T.; Fernandez, E.; Albizu, I. Sag-tension evaluation of high-temperature gap-type conductor in operation. IET Gener. Transm. Distrib. 2022, 1, 19–26. [Google Scholar] [CrossRef]
- Agüero-Rubio, J.; López-Martínez, J.; Gómez-Galán, M.; Callejón-Ferre, Á.-J. A Didactic Procedure to Solve the Equation of Steady-Static Response in Suspended Cables. Mathematics 2020, 8, 1468. [Google Scholar] [CrossRef]
- Kamboj, S.; Dahiya, R. Designing and implementation of overhead conductor altitude measurement system using GPS for sag monitoring. In Proceedings of Intelligent Computing Techniques for Smart Energy Systems; Springer: Singapore, 2019. [Google Scholar]
- Mensah-Bonsu, C.; Krekeler, U.F.; Heydt, G.T.; Hoverson, Y.; Schilleci, J. Application of the Global Positioning System to the measurement of overhead power transmission conductor sag. IEEE Trans. Power Deliv. 2002, 1, 273–278. [Google Scholar] [CrossRef]
- Safdarinezhad, A.; Abdollahifard, M.J.; Ganjali, A. A photogrammetric solution for measurement of power lines sag via integration of image and accelerometer data of a smartphone. Measurement 2022, 199, 111493. [Google Scholar] [CrossRef]
- Song, J.; Qian, J.; Liu, Z.; Jiao, Y.; Zhou, J.; Li, Y.; Chen, Y.; Guo, J.; Wang, Z. Research on Arc Sag Measurement Methods for Transmission Lines Based on Deep Learning and Photogrammetry Technology. Remote Sens. 2023, 15, 2533. [Google Scholar] [CrossRef]
- Hlalele, T.S.; Du, S. Real time monitoring of high voltage transmission line conductor sag: The state-of-the-art. Int. J. Eng. Adv. Technol. 2013, 1, 297–302. [Google Scholar]
- Rodriguez, J.; Franck, C.M. Dynamic Line Rating of overhead transmission lines under natural convective cooling. In Proceedings of the IEEE Eindhoven PowerTech, Eindhoven, The Netherlands, 2 July 2015. [Google Scholar]
- Song, N.; Yangchun, C.; Yuan, D. A new sag calculation method based on the temperature of overhead transmission line. J. North China Electr. Power Univ. 2013, 6, 27–32. [Google Scholar]
- Guo, D.; Wang, P. Investigation of Sag Behaviourfor Aluminium Conductor Steel Reinforced Considering Tensile Stress Distribution. R. Soc. Open Sci. 2021, 8, 2–16. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.; Chen, Z.; Gu, Q. Numerical Algorithms for Calculating Temperature, Layered Stress, and Critical Current of Overhead Conductors. Math. Probl. Eng. 2020, 2020, 6019493. [Google Scholar] [CrossRef]
- Wang, K.; Sun, X.; Sheng, G. Error comparison among three on-line monitoring methods of conductor sag of overhead transmission line. High Volt. Appar. 2014, 4, 27–34. [Google Scholar]
- Ramachandran, P.; Vittal, V.; Heydt, G.T. Mechanical State Estimation for Overhead Transmission Lines with Level Spans. IEEE Trans. Power Syst. 2008, 3, 908–915. [Google Scholar] [CrossRef]
- Dong, X.; Wang, C.; Liang, J.; Han, X.; Zhang, F.; Sun, H. Calculation of Power Transfer Limit Considering Electro-Thermal Coupling of Overhead Transmission Line. IEEE Trans. Power Syst. 2014, 4, 1503–1511. [Google Scholar] [CrossRef]


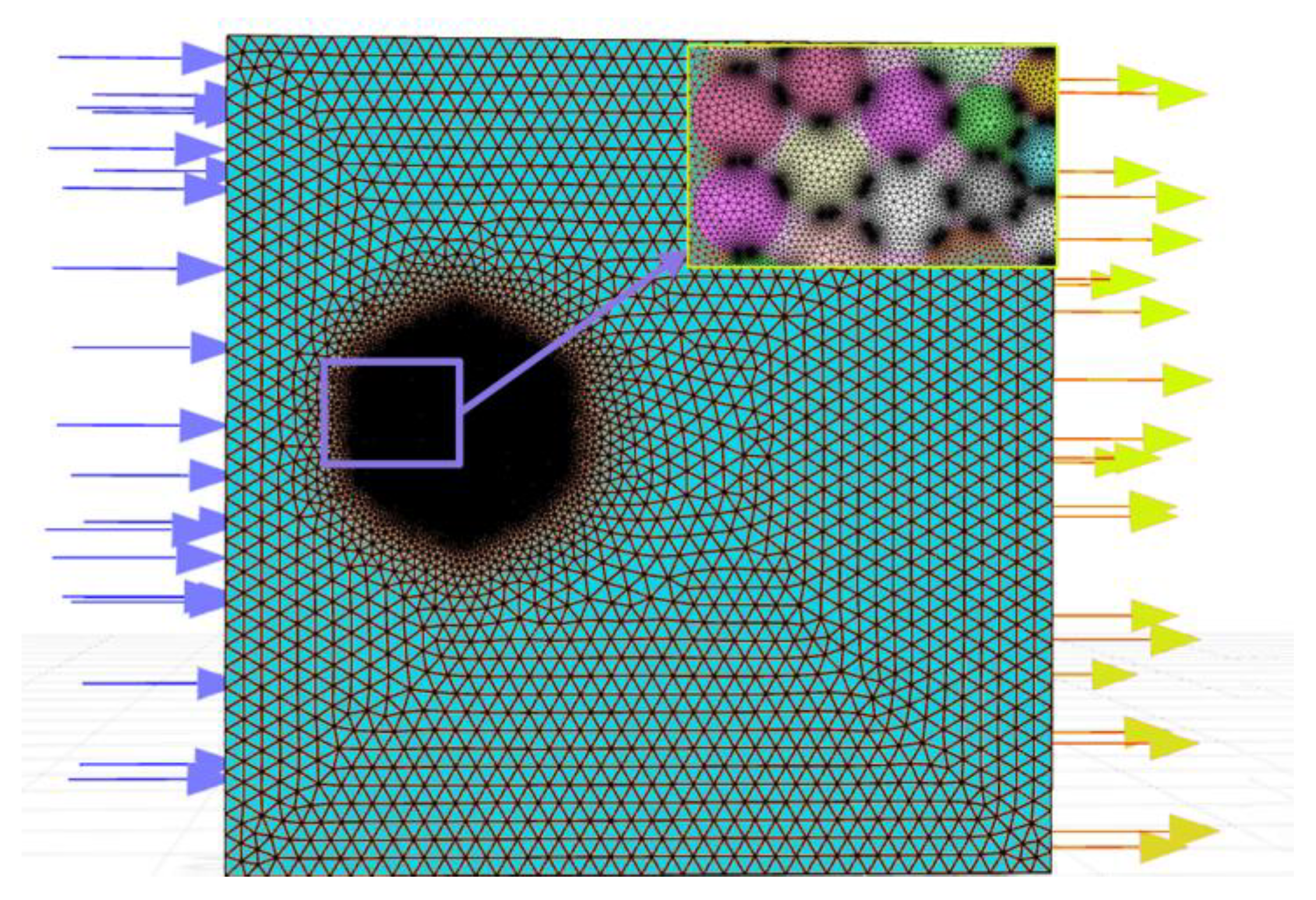
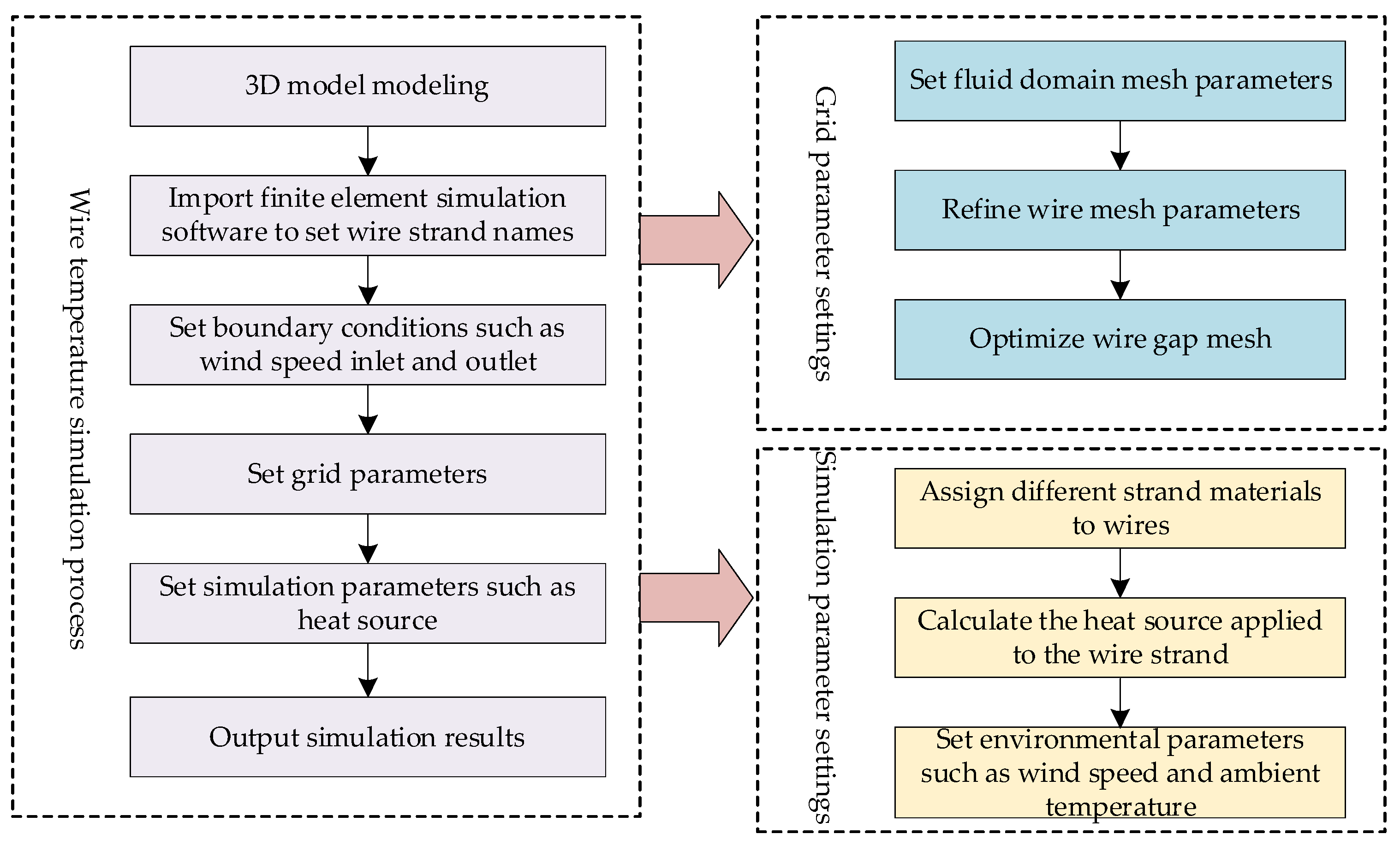
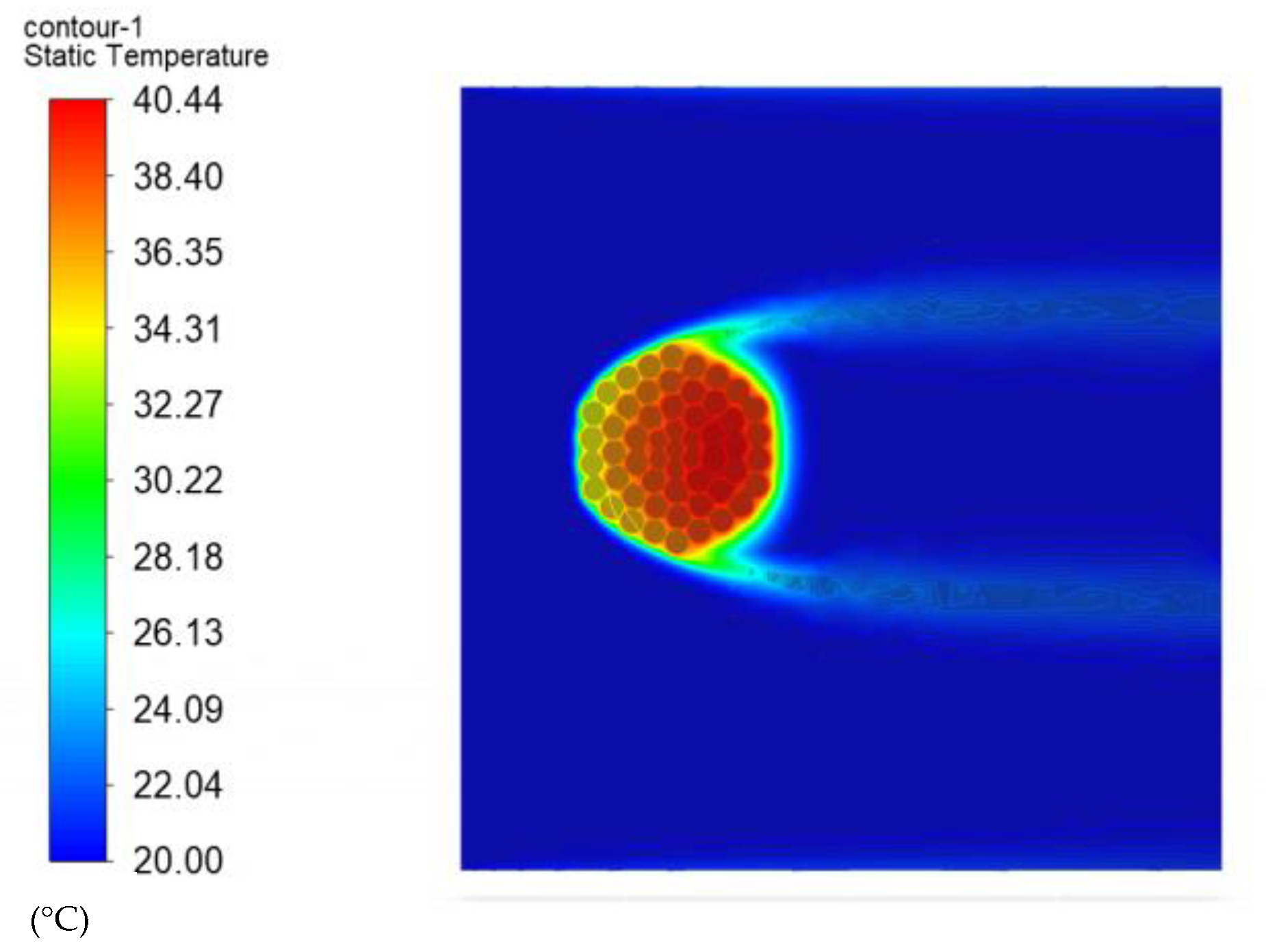

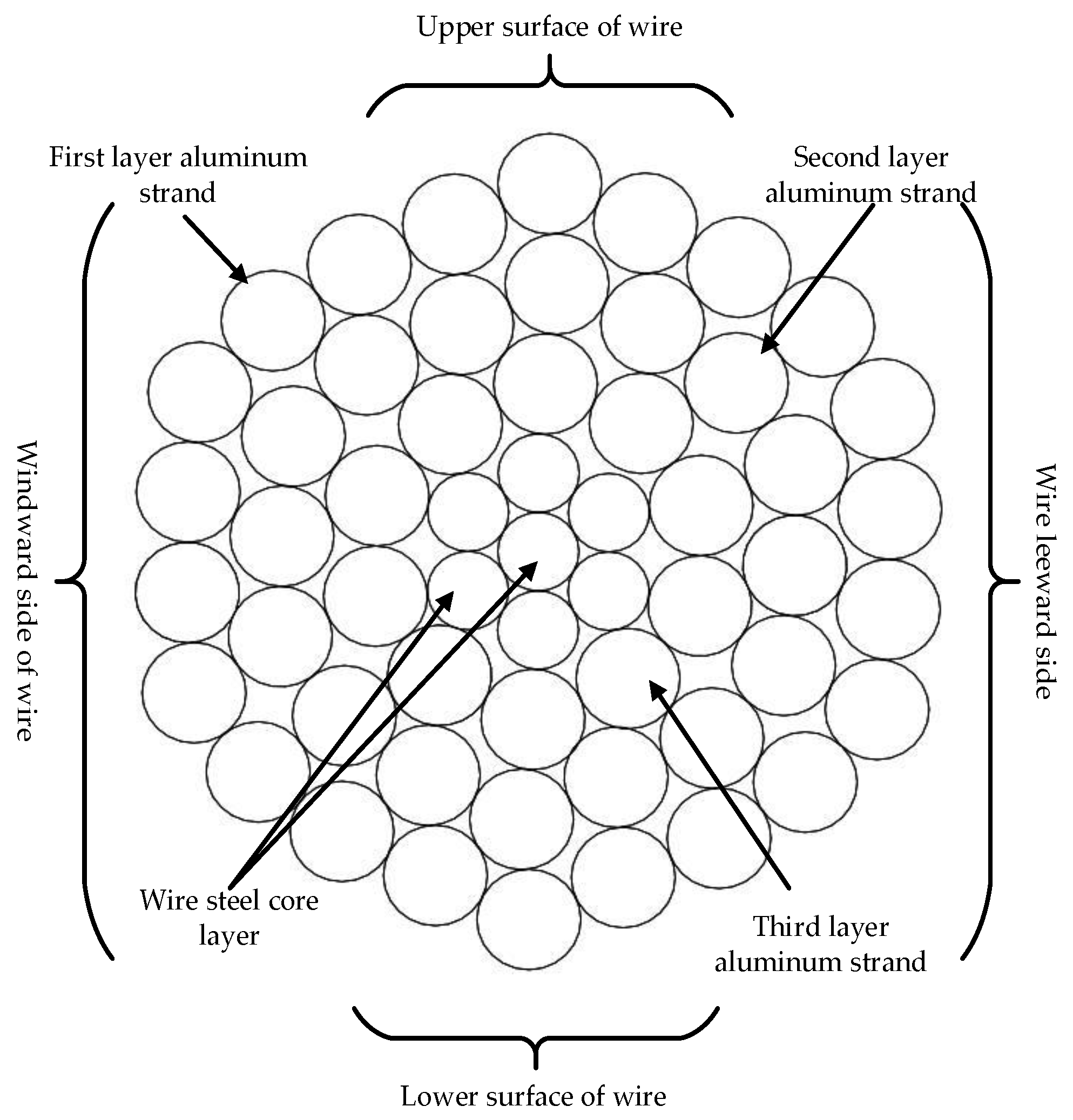
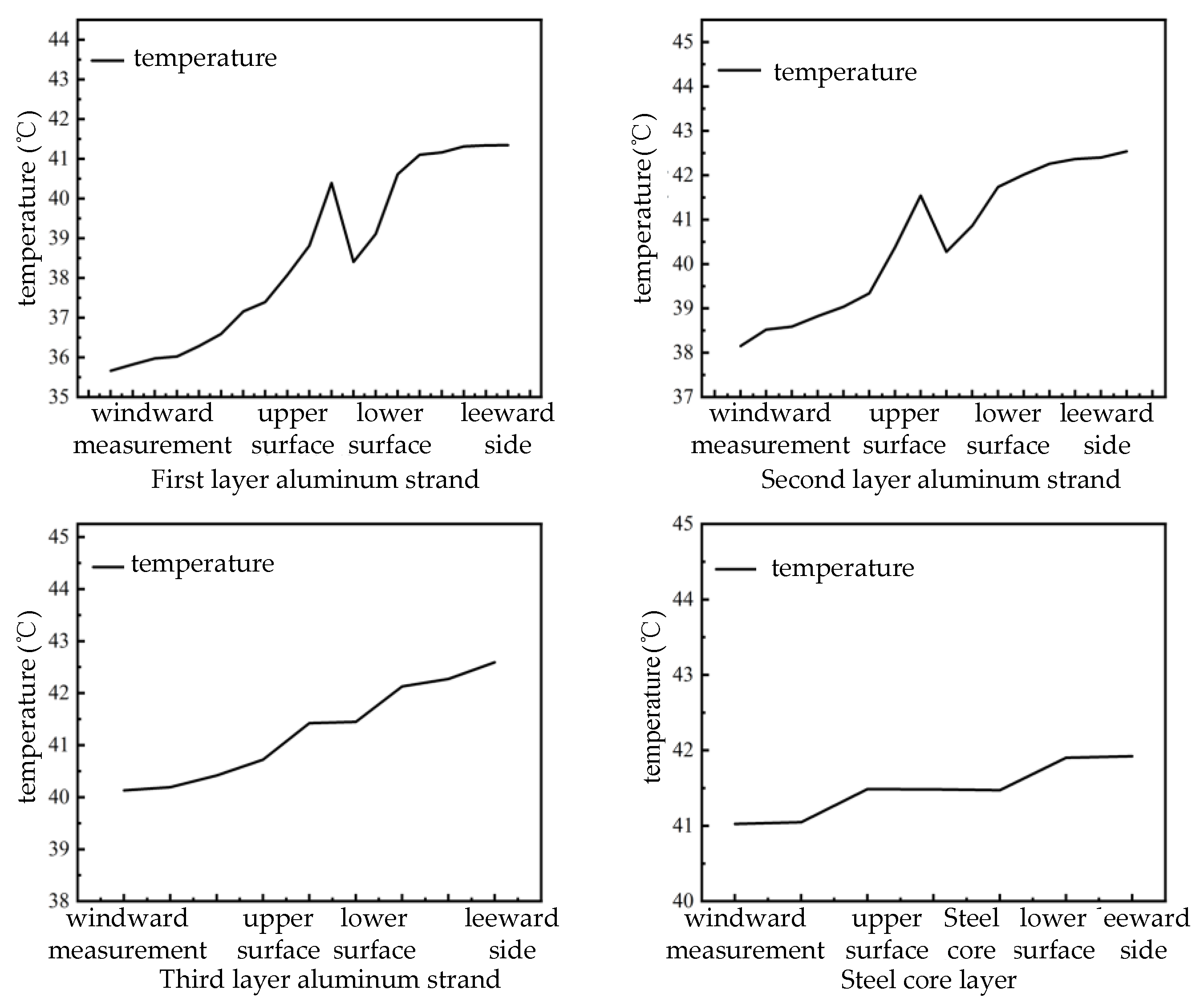


| Area | Windward Side | Upper Surface | Lower Surface | Leeward Side |
|---|---|---|---|---|
| Temp. (°C) | 37.1 | 39.2 | 38.8 | 41.5 |
| Time | Theodolite Measurement (m) | Wind Speed (m/s) | 4-Point Temp. Meas. | 1-Point Temp. Meas. | ||
|---|---|---|---|---|---|---|
| Sag (m) | Relative Error | Sag (m) | Relative Error | |||
| 12:50 | 32.54 | 2.1 | 32.15 | 1.20% | 31.11 | 4.39% |
| 13:50 | 31.66 | 4.8 | 31.20 | 1.45% | 29.17 | 7.86% |
| 14:50 | 33.99 | 0.5 | 33.36 | 1.85% | 33.29 | 2.06% |
| 15:50 | 32.01 | 2.7 | 31.58 | 1.34% | 30.63 | 4.31% |
| 16:50 | 31.51 | 4.0 | 30.98 | 1.68% | 30.04 | 4.67% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, X.; Xie, Z.; Zeng, L.; Zhao, L. Calculation Methods of High-Voltage Direct Current (HVDC) Line Sag Considering Meteorology. Energies 2024, 17, 305. https://doi.org/10.3390/en17020305
Li X, Xie Z, Zeng L, Zhao L. Calculation Methods of High-Voltage Direct Current (HVDC) Line Sag Considering Meteorology. Energies. 2024; 17(2):305. https://doi.org/10.3390/en17020305
Chicago/Turabian StyleLi, Xin, Zuibing Xie, Linping Zeng, and Long Zhao. 2024. "Calculation Methods of High-Voltage Direct Current (HVDC) Line Sag Considering Meteorology" Energies 17, no. 2: 305. https://doi.org/10.3390/en17020305
APA StyleLi, X., Xie, Z., Zeng, L., & Zhao, L. (2024). Calculation Methods of High-Voltage Direct Current (HVDC) Line Sag Considering Meteorology. Energies, 17(2), 305. https://doi.org/10.3390/en17020305







