Enhancing Heat and Mass Transfer in Adsorption Cooling and Desalination Systems Using Ionic Liquid and Graphene Consolidated Composites
Abstract
1. Introduction
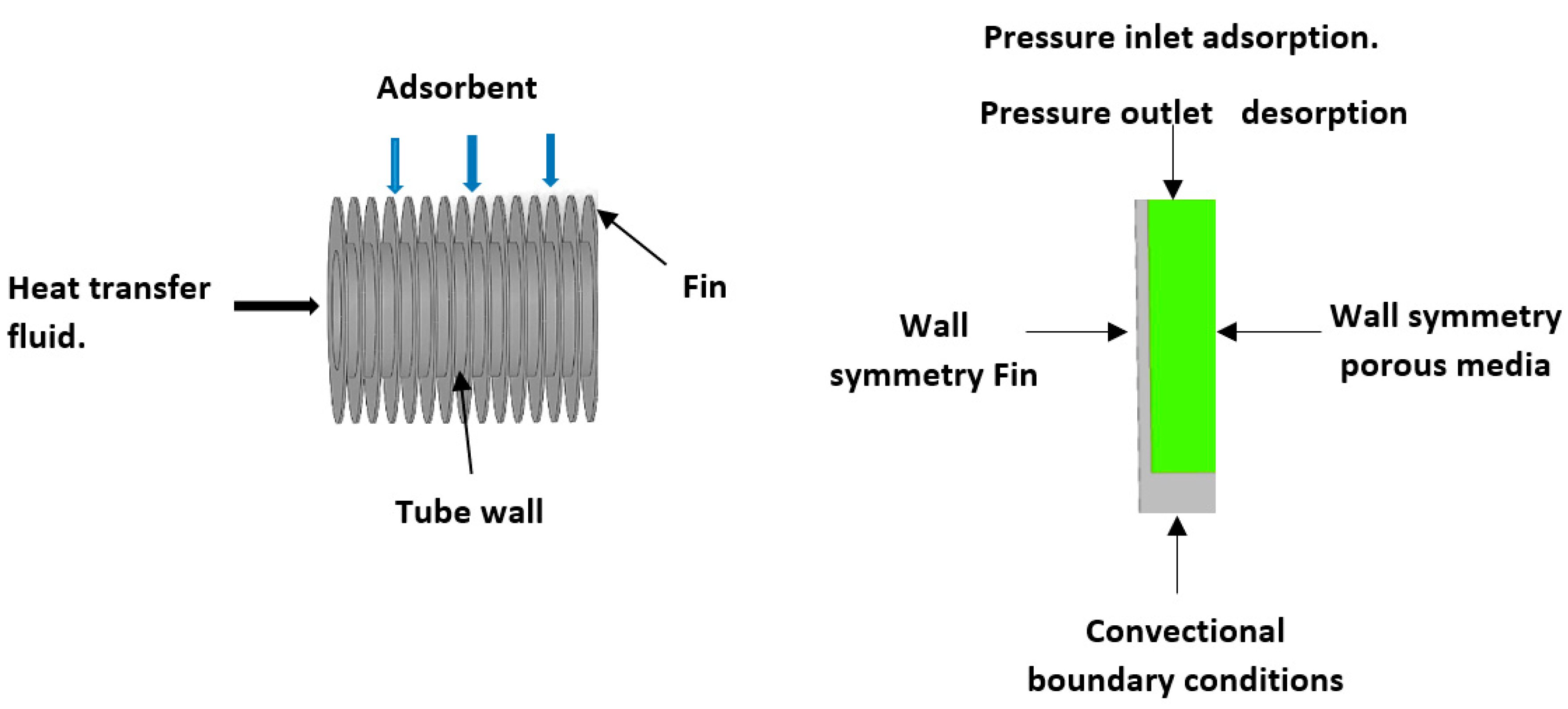
2. Materials and Method
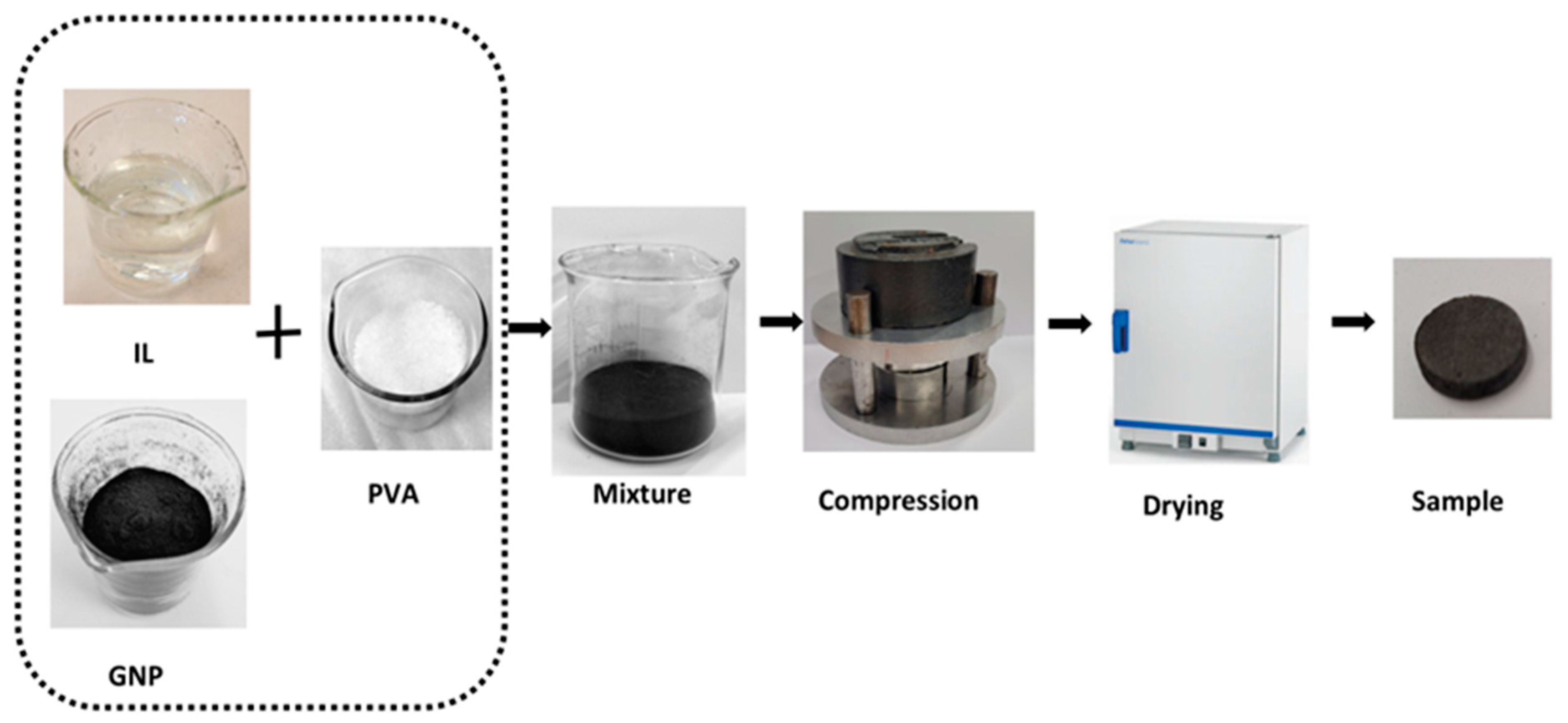
Composite Preparation
3. Composite Experimental Analysis
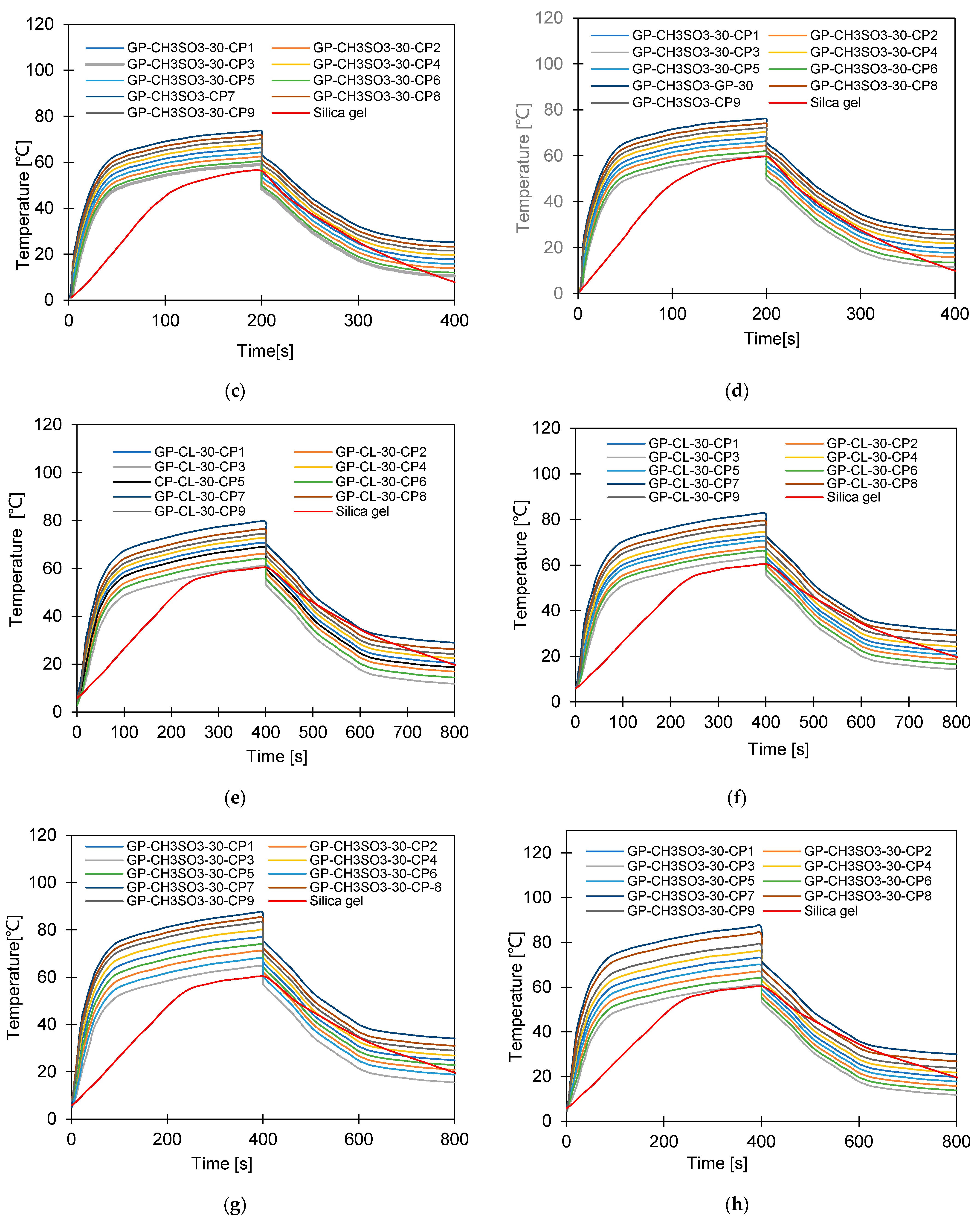
3.1. Heat Transfer Properties
3.2. Composite Adsorption Properties
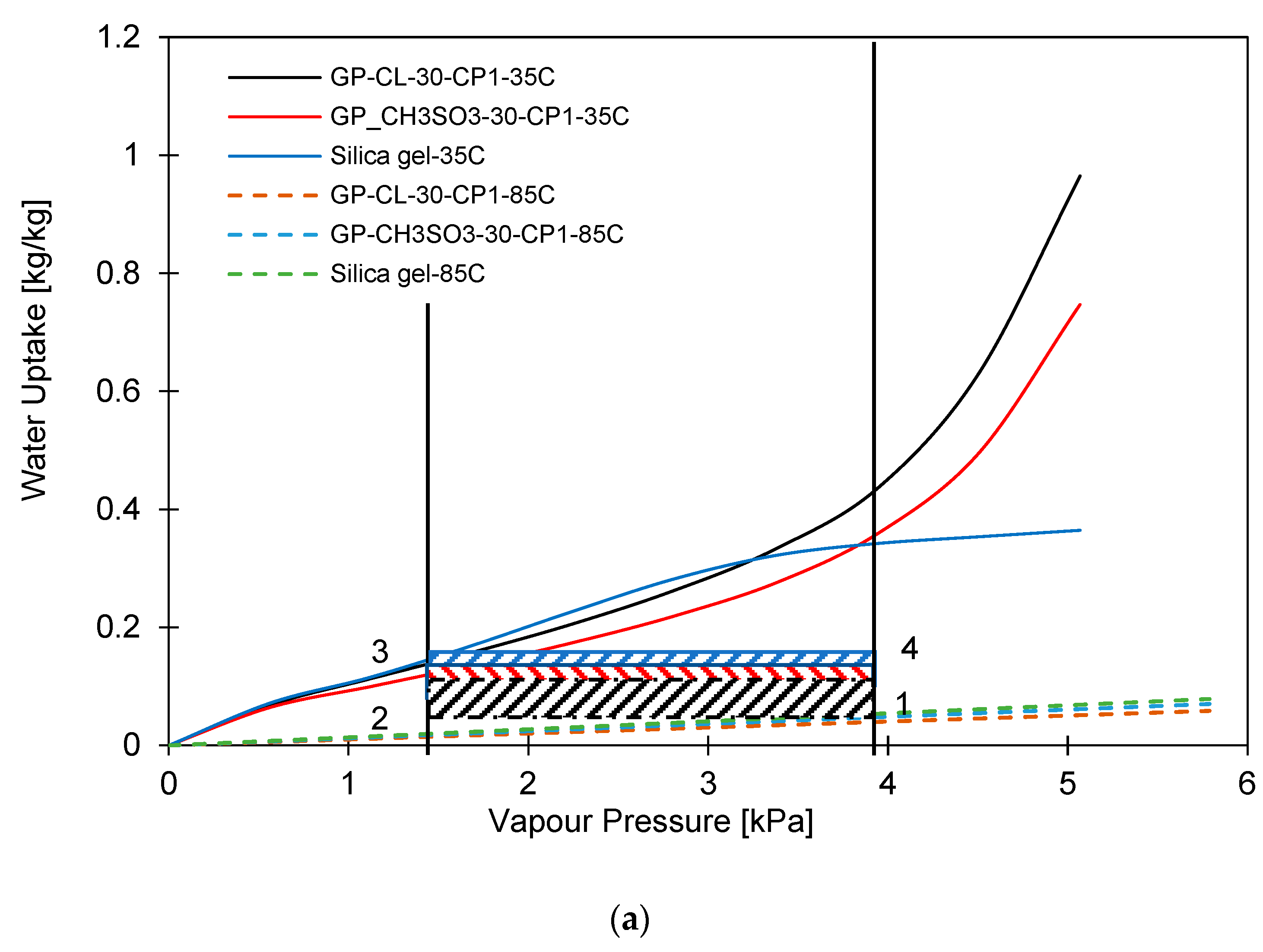
3.2.1. Adsorption Isotherms
3.2.2. Adsorption Kinetics
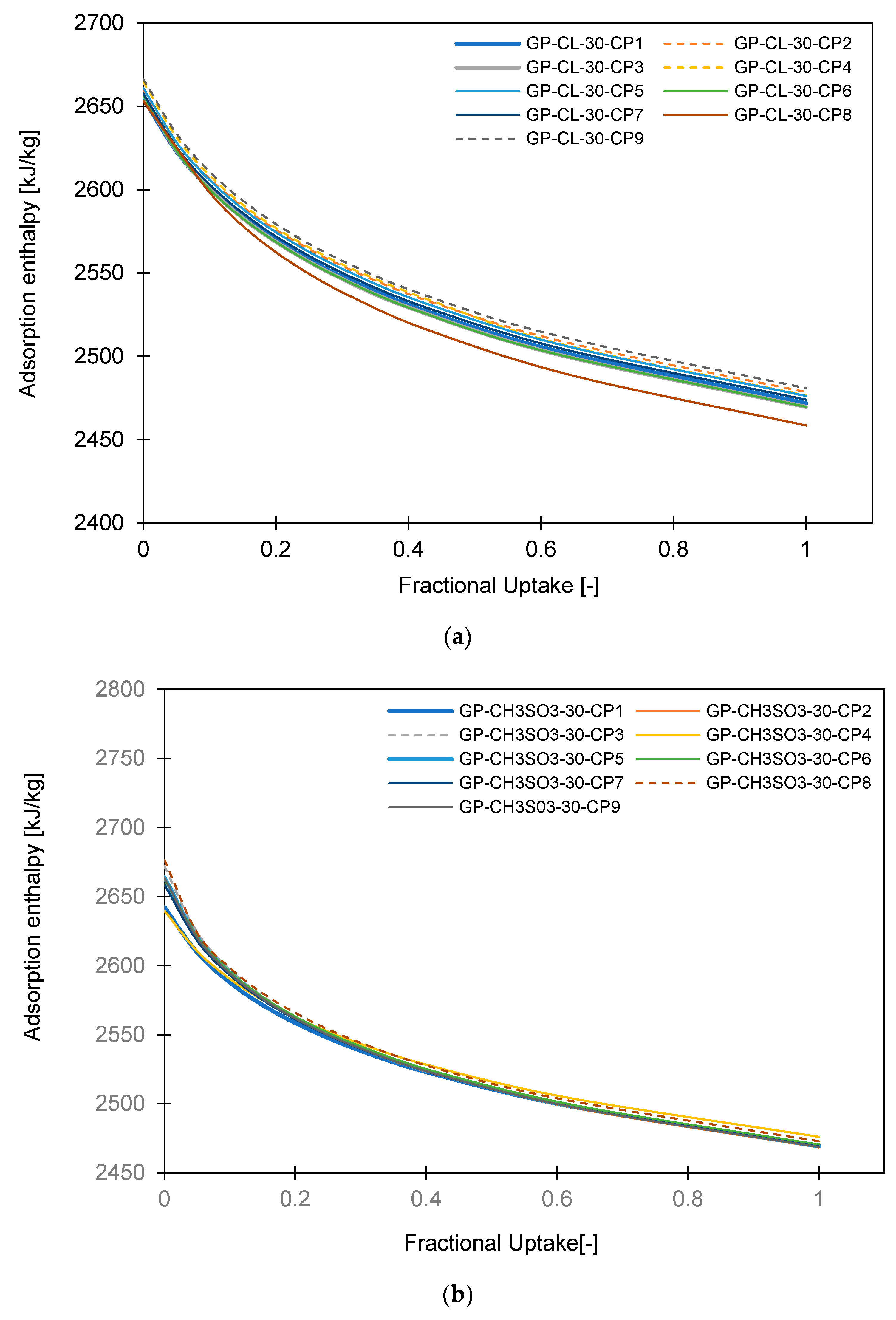
3.2.3. Isosteric Heat of Sorption
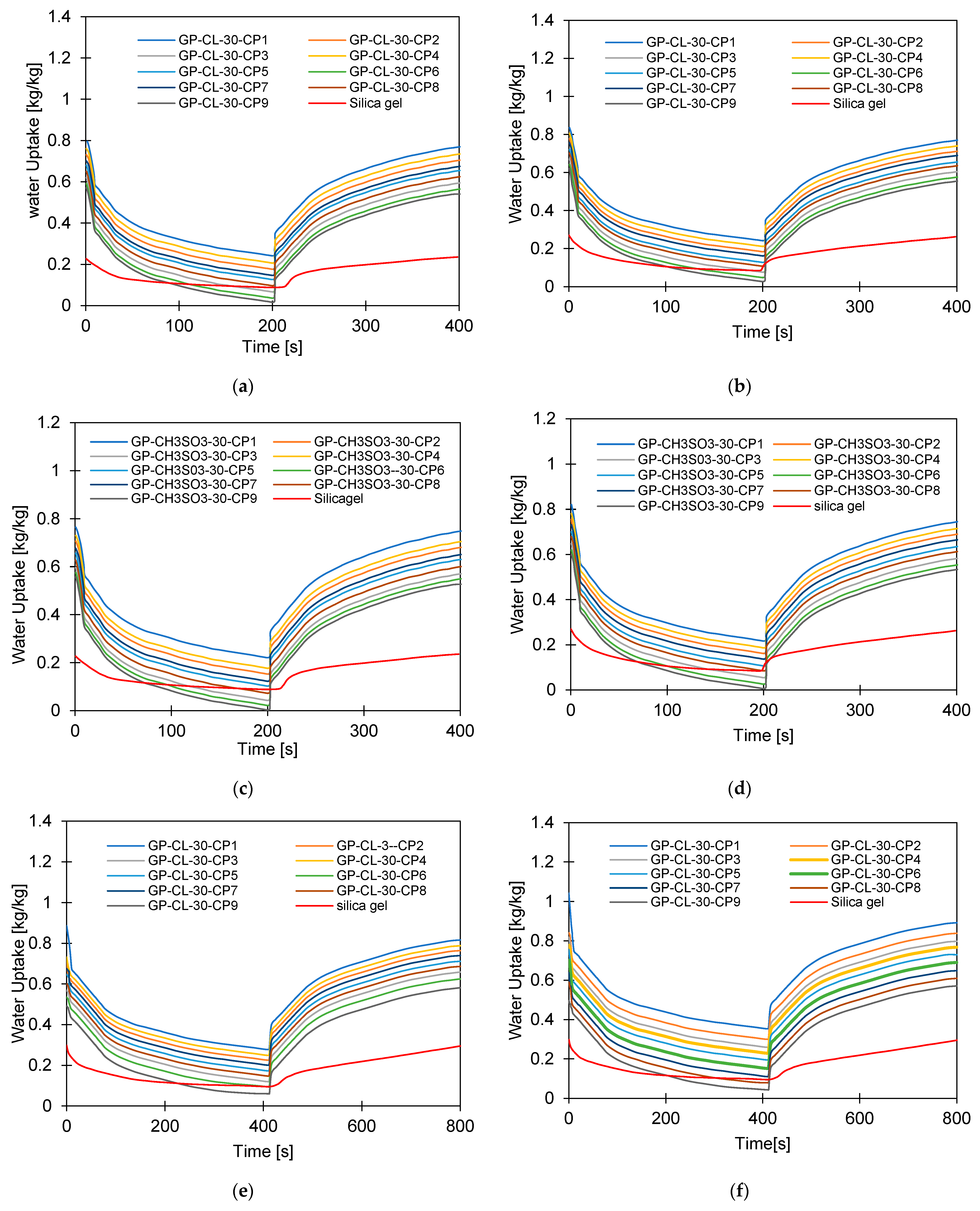
4. Composite Cyclic Performance
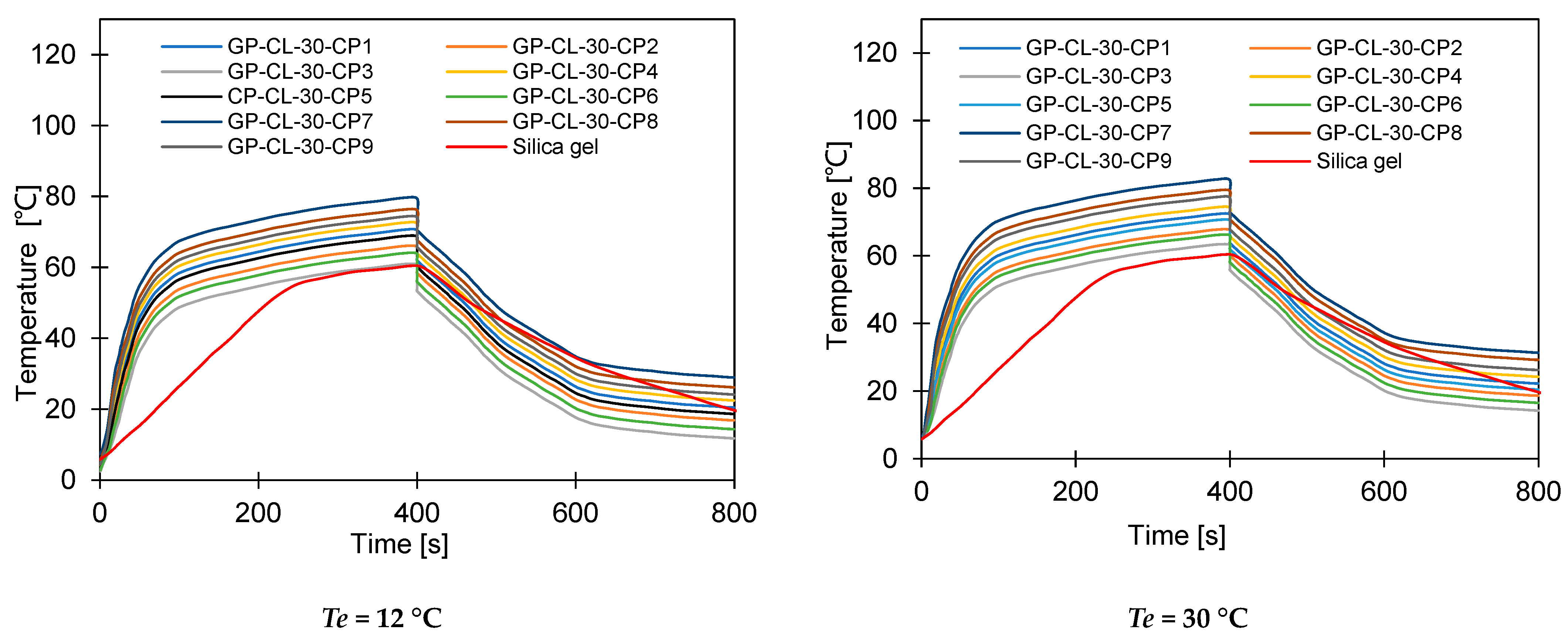
4.1. Material-Level Cyclic Performance
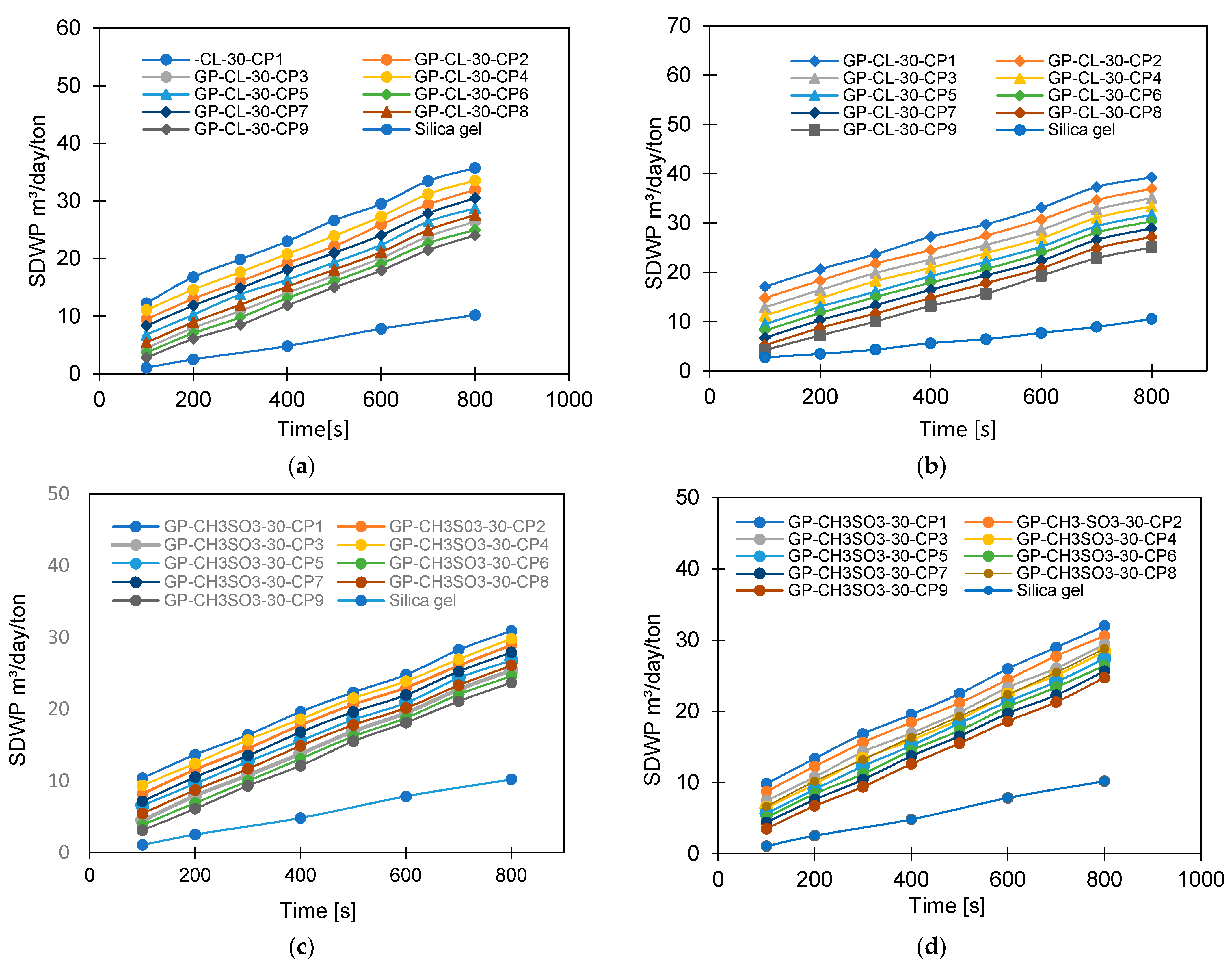
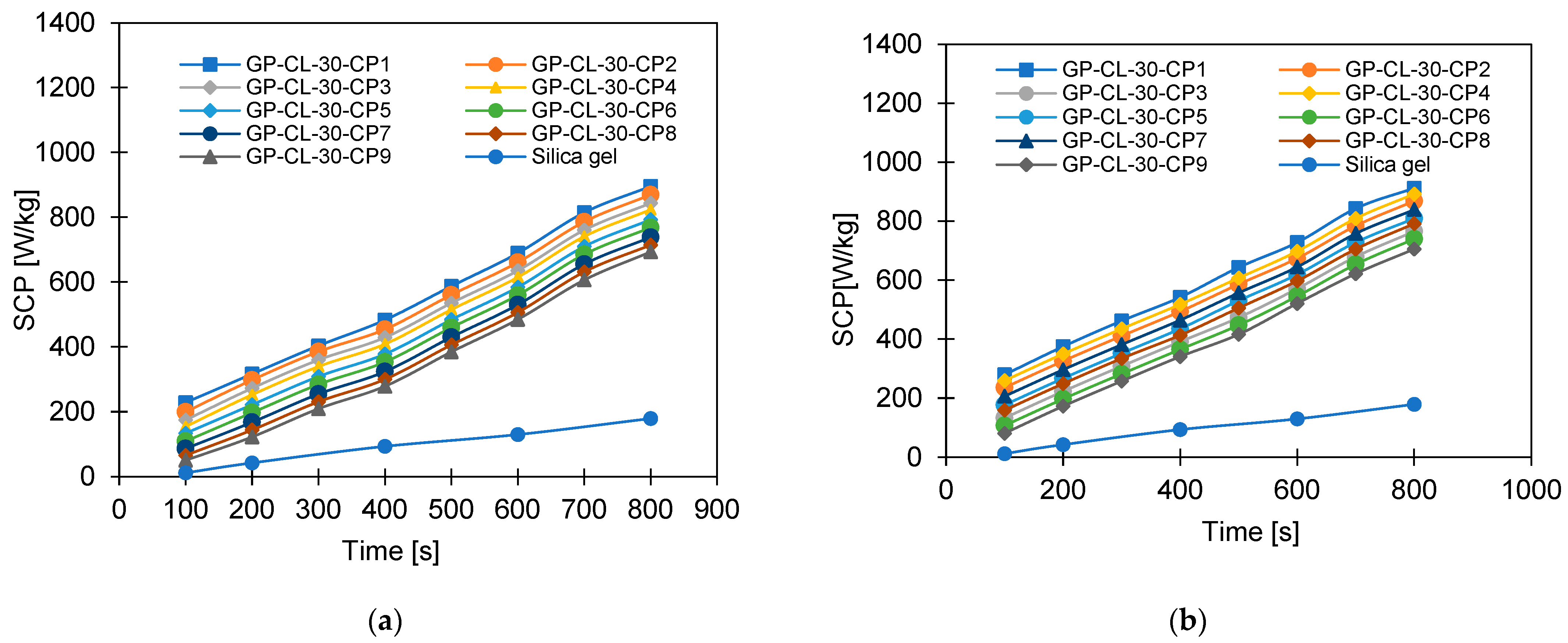
4.2. Component-Level Cyclic Performance
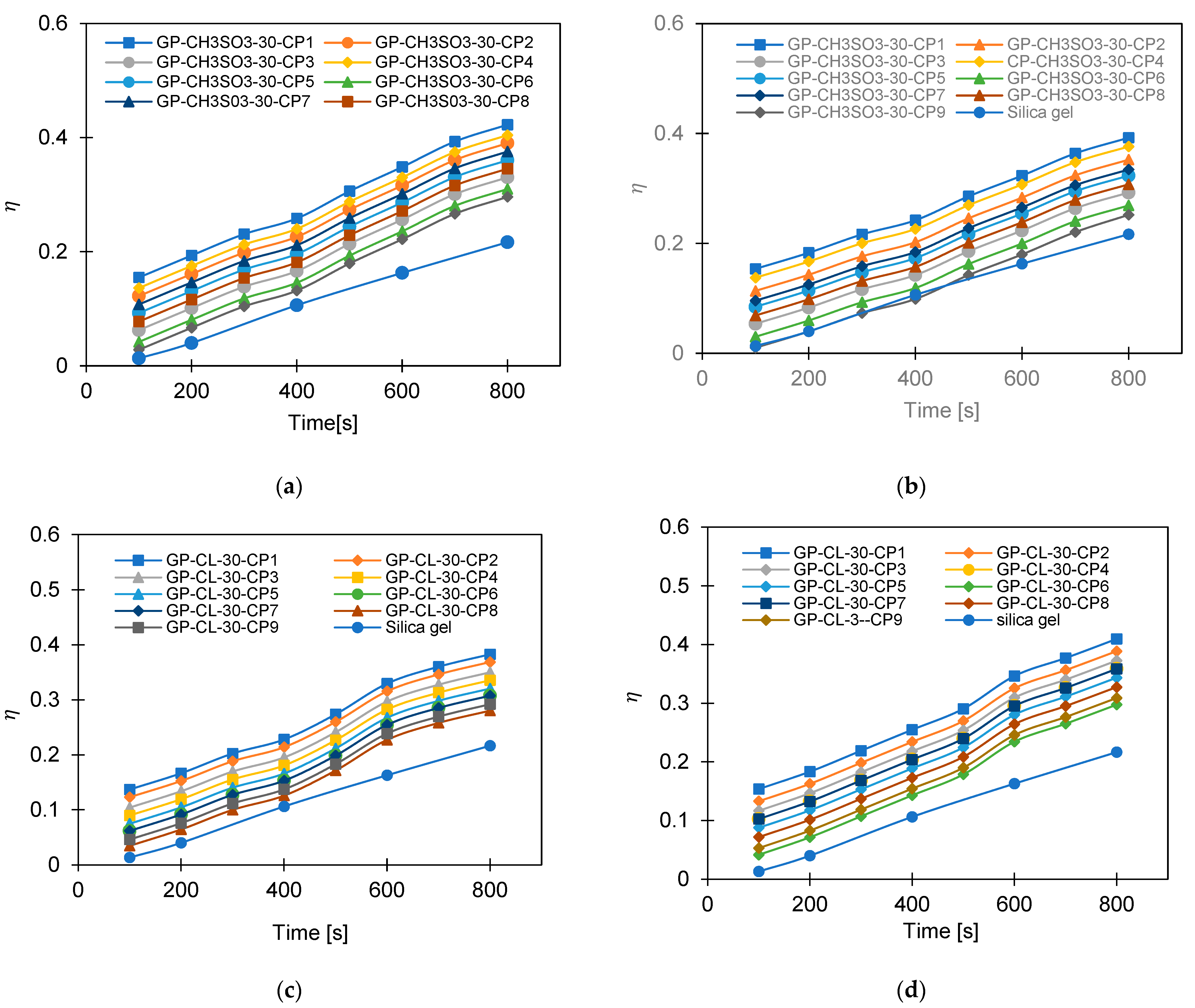
4.3. Exergy Destruction
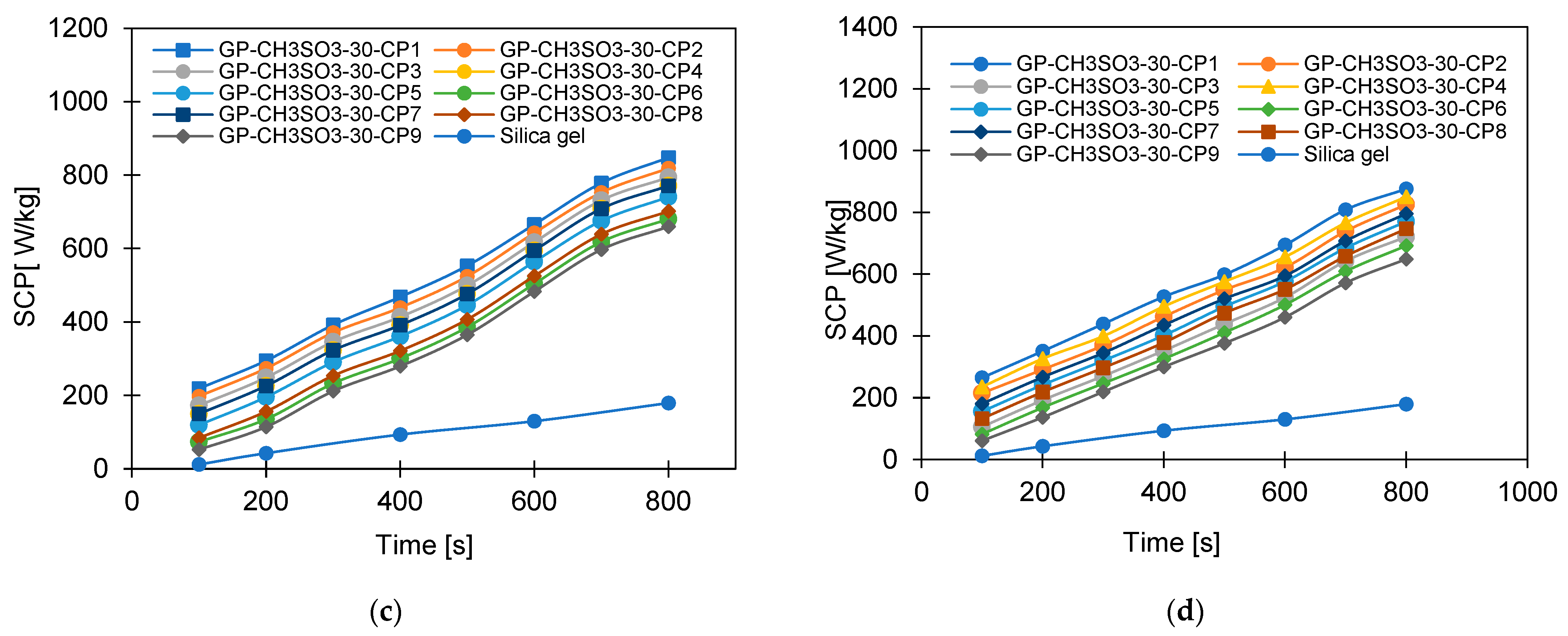
4.4. The Effect of Cycle Time
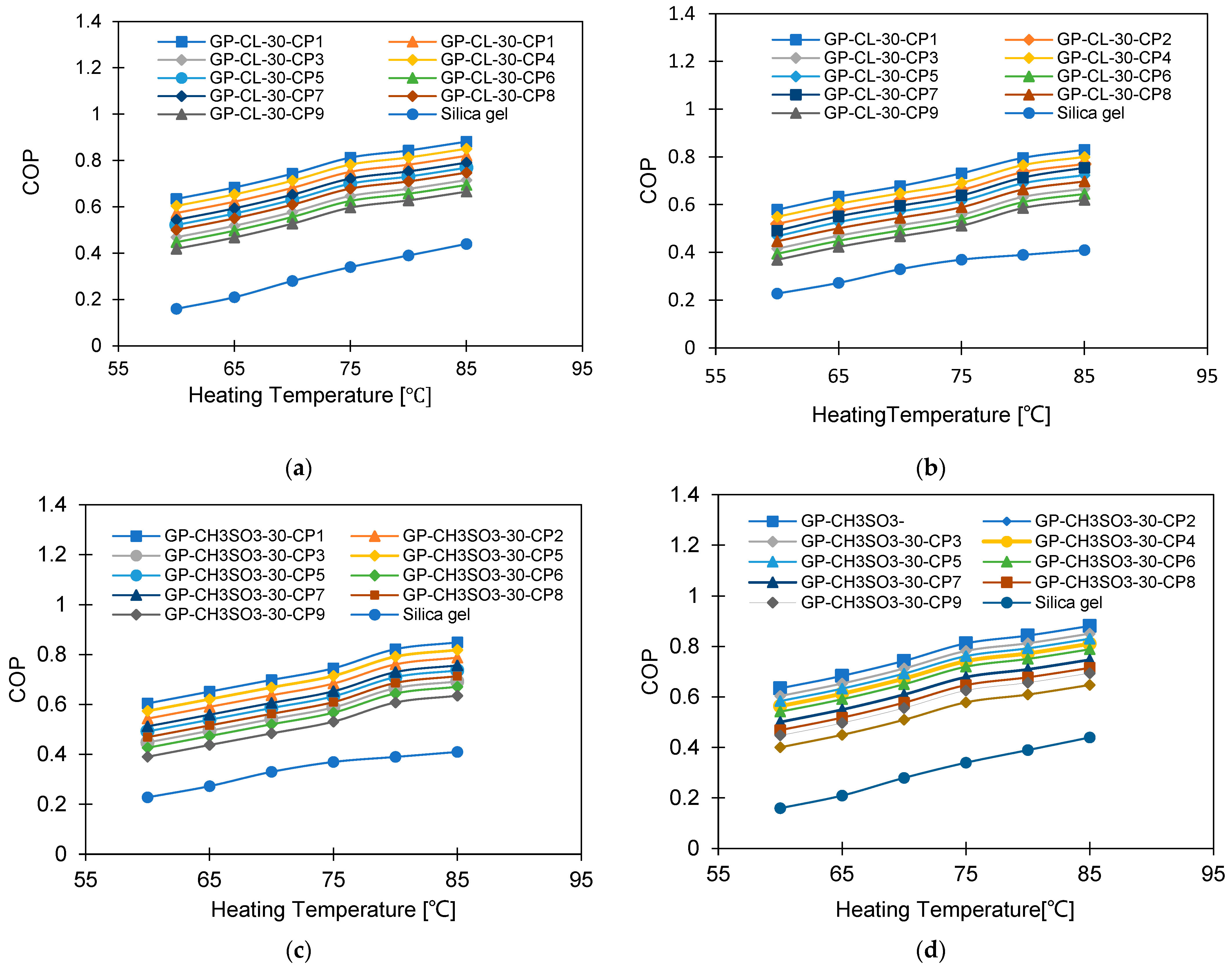
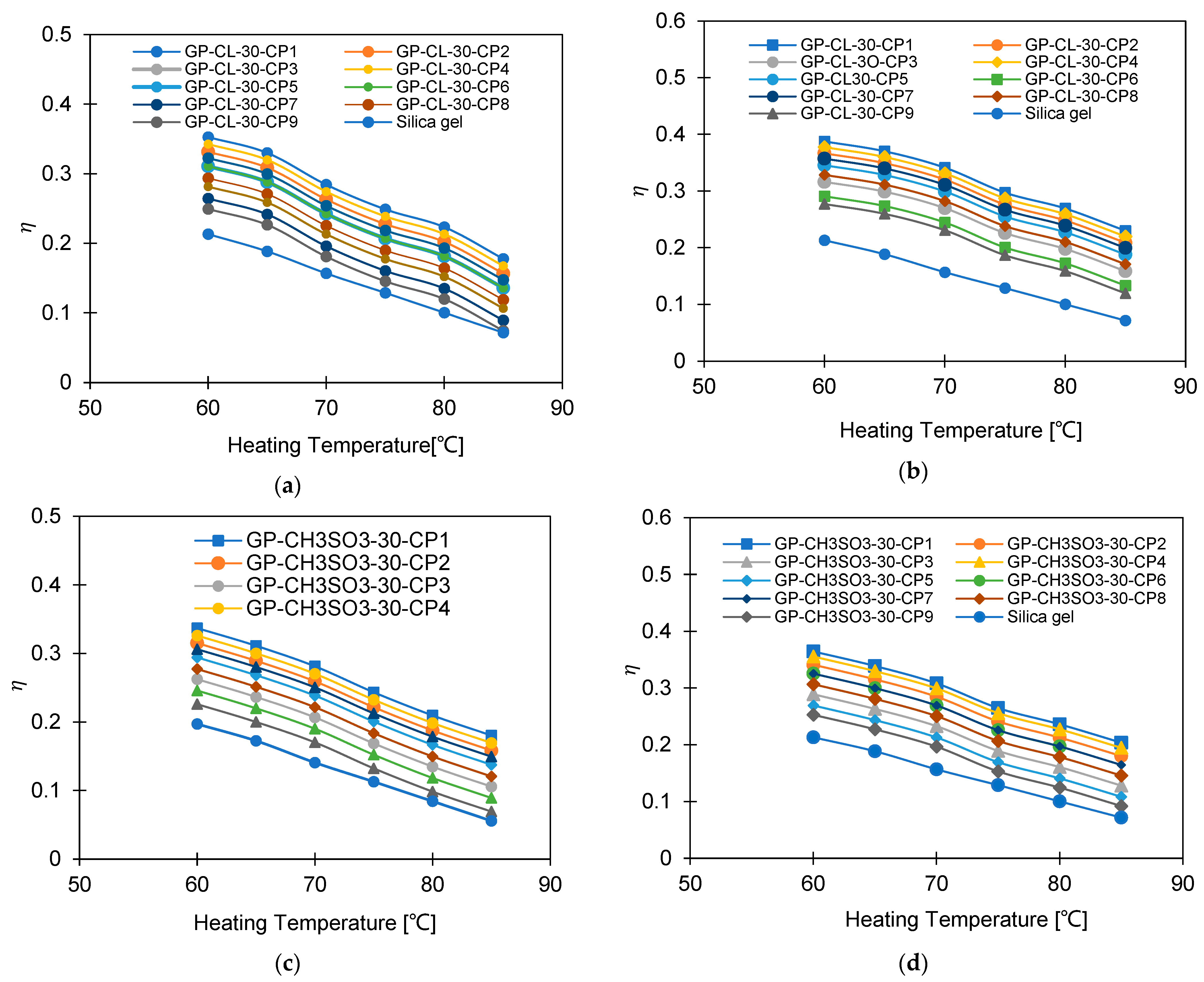
4.5. The Effect of Heat Source Temperature
5. Conclusions
- The developed composites showed significantly improved thermal diffusivity compared to SG, with the GP-CL-30-CP7 composite showing the highest thermal diffusivity of 4.65 mm2/s, a 12.7-fold increase over SG.
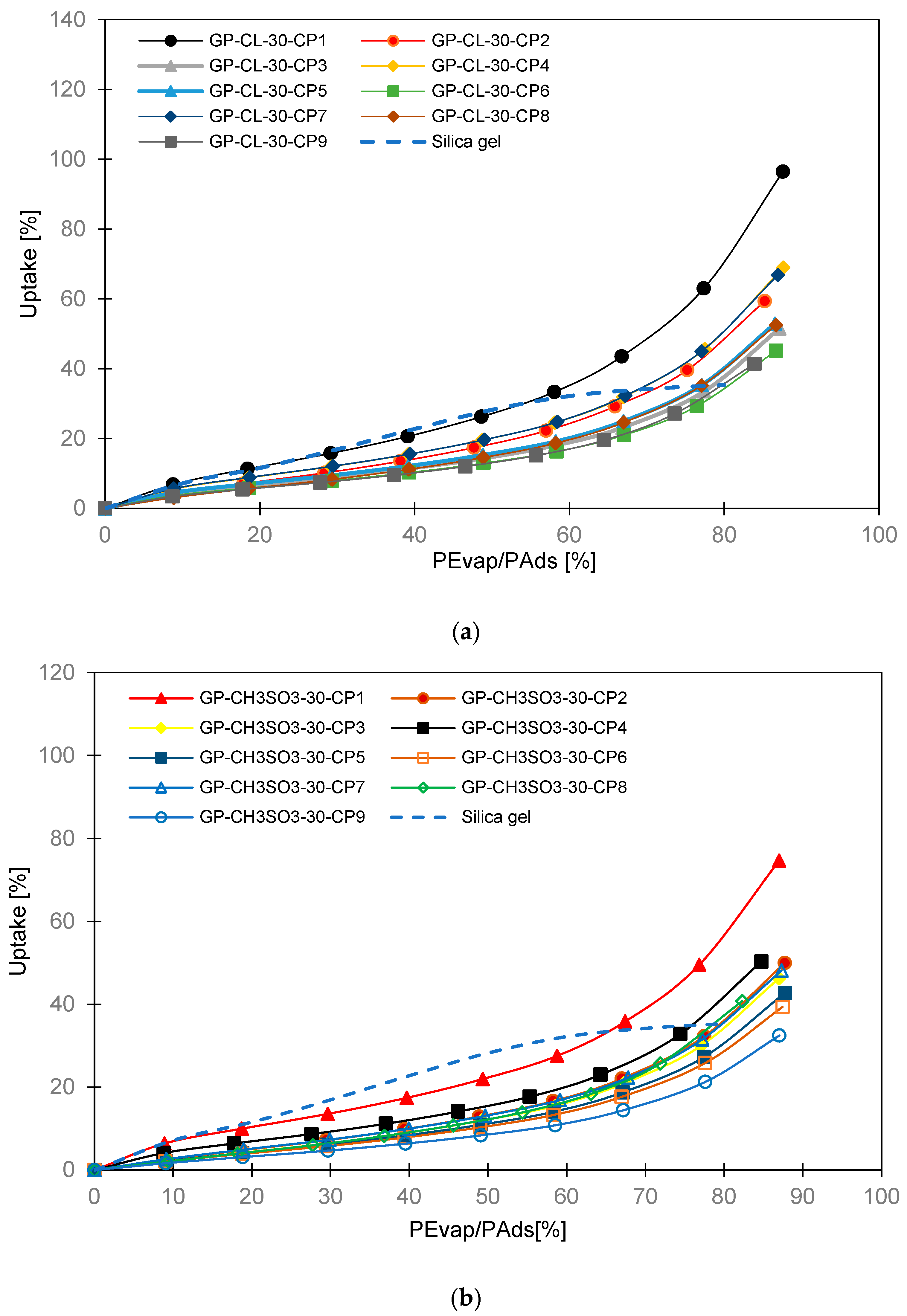
- The composite GP-CL-30-CP1 showed the highest water uptake of 0.9648 kg, a 174% enhancement compared to SG.
- Increasing the cycle time resulted in significantly higher exergy efficiency for GP-CL-30-CP1 compared to SG, attributed to the composites’ high thermal and solvation properties and IL.
- Increasing the heat source temperature improved SDWP, SCP and COP while reducing exergy efficiency. However, the exergy efficiency of the composites, including GP-CL-30-CP1, was higher than SG over the entire range of investigated temperatures by 80.7% and 70% on average at 12 °C.
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Cp | Specific heat capacity | [kJ kg−1 K−1] |
| d | Tube diameter | [mm] |
| D_s | Surface diffusivity | [m2 s−1] |
| D_so | Pre-exponential coefficient | [m2 s−1] |
| Ea | Activation energy | [kJ mol−1] |
| h | Enthalpy | [kJ kg−1] |
| h | Height | [mm] |
| l | Tube length | [mm] |
| K | Thermal conductivity | [W m−1 K−1] |
| Ko | Adsorption constant | [KPa−1] |
| ks αν | Diffusion time constant | [-] |
| M | Mass | [kg] |
| ṁ | Mass flowrate | [kg s−1] |
| P | Pressure | [Pa] |
| p | Fin pitch | [mm] |
| Q | Heat transmitted | [J] |
| Qst | Heat of adsorption | [kJ kg−1] |
| R | Gas law constant | [kJ kmol−1 K−1] |
| t | Time | [s] |
| T | Temperature | [K] [°C] |
| q | Uptake | [kg kg−1] |
| q(o) | Equilibrium uptake | [kg kg−1] |
| R_p | Particle radius | [m] |
| Greek | ||
| µ | Dynamic viscosity | [Pa s] |
| ρ | Density | [kg m−3] |
| α | Thermal diffusivity | [mm2 s−1] |
| δ | Fin thickness | [mm] |
| νg | Specific volume | [m3 kg−1] |
| Subscripts | ||
| ad | adsorbent | |
| ads | adsorption | |
| cw | cooling water | |
| chw | chilled water | |
| cond | condenser | |
| des | desorption | |
| evap | evaporator | |
| f | fin | |
| hex | heat exchanger | |
| hw | heating water | |
| i | inner | |
| in | inlet | |
| o | outer | |
| out | outlet | |
| sat | saturation | |
| SG | silica gel | |
References
- Dhakal, N.; Salinas-Rodriguez, S.G.; Hamdani, J.; Abushaban, A.; Sawalha, H.; Schippers, J.C.; Kennedy, M.D. Is desalination a solution to freshwater scarcity in developing countries? Membranes 2022, 12, 381. [Google Scholar] [CrossRef] [PubMed]
- Hua, W.S.; Xu, H.J.; Xie, W.H. Review on adsorption materials and system configurations of the adsorption desalination applications. Appl. Therm. Eng. 2022, 204, 117958. [Google Scholar] [CrossRef]
- Grabowska, K.; Krzywanski, J.; Zylka, A.; Kulakowska, A.; Skrobek, D.; Sosnowski, M.; Ščurek, R.; Nowak, W.; Czakiert, T. Implementation of Fluidized Bed Concept to Improve Heat Transfer in Ecological Adsorption Cooling and Desalination Systems. Energies 2024, 17, 379. [Google Scholar] [CrossRef]
- Figaj, R.; Żołądek, M.; Homa, M.; Pałac, A. A Novel Hybrid Polygeneration System Based on Biomass, Wind and Solar Energy for Micro-Scale Isolated Communities. Energies 2022, 15, 6331. [Google Scholar] [CrossRef]
- Rezk, A.; Ilis, G.G.; Demir, H. Experimental study on silica gel/ethanol adsorption characteristics for low-grade thermal driven adsorption refrigeration systems. Therm. Sci. Eng. Prog. 2022, 34, 101429. [Google Scholar] [CrossRef]
- Saha, B.B.; El-Sharkawy, I.I.; Shahzad, M.W.; Thu, K.; Ang, L.; Ng, K.C. Fundamental and application aspects of adsorption cooling and desalination. Appl. Therm. Eng. 2016, 97, 68–76. [Google Scholar] [CrossRef]
- Zhang, Y.; Palamara, D.; Palomba, V.; Calabrese, L.; Frazzica, A. Performance analysis of a lab-scale adsorption desalination system using silica gel/LiCl composite. Desalination 2023, 548, 116278. [Google Scholar] [CrossRef]
- Chan, K.C.; Chao, C.Y.H.; Sze-To, G.N.; Hui, K.S. Performance predictions for a new zeolite 13X/CaCl2 composite adsorbent for adsorption cooling systems. Int. J. Heat Mass Transf. 2012, 55, 3214–3224. [Google Scholar] [CrossRef]
- Gordeeva, L.G.; Aristov, Y.I. Composites ‘salt inside porous matrix’ for adsorption heat transformation: A current state-of-the-art and new trends. Int. J. Low Carbon Technol. 2012, 7, 288–302. [Google Scholar] [CrossRef]
- Restucciaa, G.; Freni, A.; Vasta, S.; Aristov, Y. Selective water sorbent for solid sorption chiller: Experimental results and modelling. Int. J. Refrig. 2004, 27, 284–293. [Google Scholar] [CrossRef]
- Hanif, S.; Sultan, M.; Miyazaki, T. Effect of relative humidity on thermal conductivity of zeolite-based adsorbents: Theory and experiments. Appl. Therm. Eng. 2019, 150, 11–18. [Google Scholar] [CrossRef]
- Rouhani, M.; Huttema, W.; Bahrami, M. Thermal conductivity of AQSOA FAM-Z02 packed bed adsorbers in open and closed adsorption thermal energy storage systems. Int. J. Refrig. 2019, 105, 158–168. [Google Scholar] [CrossRef]
- Pal, A.; Shahrom, M.S.R.; Moniruzzaman, M.; Wilfred, C.D.; Mitra, S.; Thu, K.; Saha, B.B. Ionic liquid as a new binder for activated carbon based consolidated composite adsorbents. Chem. Eng. J. 2017, 326, 980–986. [Google Scholar] [CrossRef]
- Oliveira, R.G.; Wang, R.Z. A consolidated calcium chloride-expanded graphite compound for use in sorption refrigeration systems. Carbon 2007, 45, 390–396. [Google Scholar] [CrossRef]
- Cacciola, G.; Restuccia, G.; Mercadante, L. Composites of activated carbon for refrigeration adsorption machines. Carbon 1995, 33, 1205–1210. [Google Scholar] [CrossRef]
- El-Sharkawy, I.I.; Pal, A.; Miyazaki, T.; Saha, B.B.; Koyama, S. A study on consolidated composite adsorbents for cooling application. Appl. Therm. Eng. 2016, 98, 1214–1220. [Google Scholar] [CrossRef]
- Xu, S.Z.; Lemington, Q.; Wang, R.Z.; Wang, L.W.; Zhu, J. A zeolite 13X/magnesium sulfate–water sorption thermal energy storage device for domestic heating. Energy Convers. Manag. 2018, 171, 98–109. [Google Scholar] [CrossRef]
- Li, S.L.; Wu, J.Y.; Xia, Z.Z.; Wang, R.Z. Study on the adsorption isosteres of the composite adsorbent CaCl2 and expanded graphite. Energy Convers. Manag. 2011, 52, 1501–1506. [Google Scholar] [CrossRef]
- Pal, A.; Uddin, K.; Thu, K.; Saha, B.B. Activated carbon and graphene nanoplatelets based novel composite for performance enhancement of adsorption cooling cycle. Energy Convers. Manag. 2019, 180, 134–148. [Google Scholar] [CrossRef]
- Younes, M.M.; El-sharkawy, I.I.; Kabeel, A.E.; Uddin, K.; Pal, A.; Mitra, S.; Thu, K.; Saha, B.B. Synthesis and characterization of silica gel composite with polymer binders for adsorption cooling applications. Int. J. Refrig. 2019, 98, 161–170. [Google Scholar] [CrossRef]
- El-Sharkawy, I.I.; Uddin, K.; Miyazaki, T.; Saha, B.B.; Koyama, S.; Miyawaki, J.; Yoon, S.-H. Adsorption of ethanol onto parent and surface treated activated carbon powders. Int. J. Heat Mass Transf. 2014, 73, 445–455. [Google Scholar] [CrossRef]
- Wang, L.W.; Metcalf, S.J.; Critoph, R.E.; Thorpe, R.; Tamainot-Telto, Z. Development of thermal conductive consolidated activated carbon for adsorption refrigeration. Carbon 2012, 50, 977–986. [Google Scholar] [CrossRef]
- Balandin, A.A.; Ghosh, S.; Bao, W.; Calizo, I.; Teweldebrhan, D.; Miao, F.; Lau, C.N. Superior Thermal Conductivity of Single-Layer Graphene. Nano Lett. 2008, 8, 902. [Google Scholar] [CrossRef]
- Noorunnisa Khanam, P.; AlMaadeed, M.A.; Ouederni, M.; Mayoral, B.; Hamilton, A.; Sun, D. Effect of two types of graphene nanoplatelets on the physico-mechanical properties of linear low–density polyethylene composites. Adv. Manuf. Polym. Compos. Sci. 2016, 2, 67–73. [Google Scholar] [CrossRef]
- Jiménez-Suárez, A.; Prolongo, S. Graphene nanoplatelets. Appl. Sci. 2020, 10, 1753. [Google Scholar] [CrossRef]
- Truong, Q.T.; Pokharel, P.; Song, G.S.; Lee, D.S. Preparation and characterization of graphene nanoplatelets from natural graphite via intercalation and exfoliation with tetraalkylammoniumbromide. Nanosci. Nanotechnol. 2012, 12, 4305–4308. [Google Scholar] [CrossRef] [PubMed]
- Geng, Y.; Wang, S.J.; Kim, J.-K. Preparation of graphite nanoplatelets and graphene sheets. Colloid Interface Sci. 2009, 336, 592–598. [Google Scholar] [CrossRef] [PubMed]
- Rogers, R.D.; Seddon, K.R. Ionic liquids—Solvents of the future? Science 2003, 302, 792–793. [Google Scholar] [CrossRef] [PubMed]
- Joshi, M.D.; Anderson, J.L. Recent advances of ionic liquids in separation science and mass spectrometry. RSC Adv. 2012, 2, 5470–5484. [Google Scholar] [CrossRef]
- Askalany, A.A.; Freni, A.; Santori, G. Supported ionic liquid water sorbent for high throughput desalination and drying. Desalination 2019, 452, 258–264. [Google Scholar] [CrossRef]
- Zhao, Q.; Anderson, J.L.; Liquids, I. 2.11-Ionic Liquids, in Comprehensive Sampling and Sample Preparation; Pawliszyn, J., Ed.; Academic Press: Oxford, UK, 2012; pp. 213–242. [Google Scholar]
- Yuan, J.; Fan, M.; Zhang, F.; Xu, Y.; Tang, H.; Huang, C.; Zhang, H. Amine-functionalized poly(ionic liquid) brushes for carbon dioxide adsorption. Chem. Eng. J. 2017, 316, 903–910. [Google Scholar] [CrossRef]
- Hasib-ur-Rahman, M.; Siaj, M.; Larachi, F. Ionic liquids for CO2 capture—Development and progress. Chem. Eng. Process. Process Intensif. 2010, 49, 313–322. [Google Scholar] [CrossRef]
- Finotello, A.; Bara, J.E.; Camper, D.; Noble, R.D. Room-Temperature Ionic Liquids: Temperature Dependence of Gas Solubility Selectivity. Ind. Eng. Chem. Res. 2008, 47, 3453–3459. [Google Scholar] [CrossRef]
- Tang, J.; Tang, H.; Sun, W.; Radosz, M.; Shen, Y. Poly(ionic liquid)s as new materials for CO2 absorption. J. Polym. Sci. Part A Polym. Chem. 2005, 43, 5477–5489. [Google Scholar] [CrossRef]
- Alsaman, A.S.; Hassan, A.A.; Ali, E.S.; Mohammed, R.H.; Zohir, A.E.; Farid, A.M.; Eraqi, A.M.Z.; El-Ghetany, H.H.; Askalany, A.A. Hybrid Solar-Driven Desalination/Cooling Systems: Current Situation and Future Trend. Energies 2022, 15, 8099. [Google Scholar] [CrossRef]
- Banda, H.; Rupam, T.H.; Rezk, A.; Visak, Z.; Hammerton, J.; Yuan, Q.; Saha, B.B. Preparation and assessment of ionic liquid and few-layered graphene composites to enhance heat and mass transfer in adsorption cooling and desalination systems. Int. J. Heat Mass Transf. 2024, 221, 125095. [Google Scholar] [CrossRef]
- Banda, H.; Rezk, A.; Elsayed, E.; Askalany, A. Experimental and computational study on utilising graphene oxide for adsorption cooling and water desalination. Appl. Therm. Eng. 2023, 229, 120631. [Google Scholar] [CrossRef]
- Rocky, K.A.; Pal, A.; Rupam, T.H.; Palash, M.L.; Saha, B.B. Recent advances of composite adsorbents for heat transformation applications. Therm. Sci. Eng. Prog. 2021, 23, 100900. [Google Scholar] [CrossRef]
- Chua, H.T.; Ng, K.C.; Chakraborty, A.; Oo, N.M.; Othman, M.A. Adsorption characteristics of silica gel+ water systems. Chem. Eng. Data 2002, 47, 1177–1181. [Google Scholar] [CrossRef]
- Wu, C.H.; Hsu, S.H.; Chu, R.Q.; Chen, M.T.; Chung, T.W. Enhancing the thermal conductivity of the heat exchanger in a noncompressive system as a means of energy efficiency improvement of the system. Int. J. Green Energy 2009, 6, 490–507. [Google Scholar] [CrossRef]
- Xie, X.; Li, D.; Tsai, T.-H.; Liu, J.; Braun, P.V.; Cahill, D.G. Thermal Conductivity, Heat Capacity, and Elastic Constants of Water-Soluble Polymers and Polymer Blends. Macromolecules 2016, 49, 972–978. [Google Scholar] [CrossRef]
- Syah, R.; Piri, F.; Elveny, M.; Khan, A. Artificial Intelligence simulation of water treatment using nanostructure composite ordered materials. J. Mol. Liq. 2022, 345, 117046. [Google Scholar] [CrossRef]
- Albadarin, A.B.; Collins, M.N.; Naushad, M.; Shirazian, S.; Walker, G.; Mangwandi, C. Activated lignin-chitosan extruded blends for efficient adsorption of methylene blue. Chem. Eng. J. 2017, 307, 264–272. [Google Scholar] [CrossRef]
- Lin, C.-I.; Wang, L.-H. Rate equations and isotherms for two adsorption models. J. Chin. Inst. Chem. Eng. 2008, 39, 579–585. [Google Scholar] [CrossRef]
- Llano-Restrepo, M.; Mosquera, M.A. Accurate correlation; thermochemistry, and structural interpretation of equilibrium adsorption isotherms of water vapor in zeolite 3A by means of a generalized statistical thermodynamic adsorption model. Fluid Phase Equilibria 2009, 283, 73–88. [Google Scholar] [CrossRef]
- Stoeckli, F.; Jakubov, T.; Lavanchy, A. Water adsorption in active carbons described by the Dubinin–Astakhov equation. J. Chem. Soc. Faraday Trans. 1994, 90, 783–786. [Google Scholar] [CrossRef]
- Malek, A.; Farooq, S. Comparison of isotherm models for hydrocarbon adsorption on activated carbon. AIChE J. 1996, 42, 3191–3201. [Google Scholar] [CrossRef]
- Foo, K.Y.; Hameed, B.H. Insights into the modeling of adsorption isotherm systems. Chem. Eng. J. 2010, 156, 2–10. [Google Scholar] [CrossRef]
- Subramanyam, B.; Das, A. Linearised and non-linearised isotherm models optimization analysis by error functions and statistical means. J. Environ. Health Sci. Eng. 2014, 12, 92. [Google Scholar] [CrossRef] [PubMed]
- Khanam, M.; Jribi, S.; Miyazaki, T.; Saha, B.B.; Koyama, S. Numerical investigation of small-scale adsorption cooling system performance employing activated carbon-ethanol pair. Energies 2018, 11, 1499. [Google Scholar] [CrossRef]
- Zhang, Y.; Palomba, V.; Frazzica, A. Understanding the effect of materials, design criteria and operational parameters on the adsorption desalination performance—A review. Energy Convers. Manag. 2022, 269, 116072. [Google Scholar] [CrossRef]
- Jahan, I.; Rupam, T.H.; Palash, M.L.; Rocky, K.A.; Saha, B.B. Energy efficient green synthesized MOF-801 for adsorption cooling applications. J. Mol. Liq. 2022, 345, 117760. [Google Scholar] [CrossRef]
- Chakraborty, A.; Saha, B.B.; Koyama, S.; Ng, K.C. On the thermodynamic modeling of the isosteric heat of adsorption and comparison with experiments. Appl. Phys. Lett. 2006, 89, 171901. [Google Scholar] [CrossRef]
- Mitra, S.; Thu, K.; Saha, B.B.; Srinivasan, K.; Dutta, P. Modeling study of two-stage, multi-bed air cooled silica gel+water adsorption cooling cum desalination system. Appl. Therm. Eng. 2017, 114, 704–712. [Google Scholar] [CrossRef]
- Sosnowski, M.; Krzywanski, J.; Skoczylas, N. Adsorption Desalination and Cooling Systems: Advances in Design, Modeling and Performance. Energies 2022, 15, 4036. [Google Scholar] [CrossRef]
- Li, M.; Zhao, Y.; Long, R.; Liu, Z.; Liu, W. Computational fluid dynamic study on adsorption-based desalination and cooling systems with stepwise porosity distribution. Desalination 2021, 508, 115048. [Google Scholar] [CrossRef]
- Cao, N.V.; Chung, J.D. Exergy analysis of adsorption cooling systems based on numerical simulation. Energy Technol. 2019, 7, 153–166. [Google Scholar] [CrossRef]
- Youssef, P.G.; Mahmoud, S.M.; Al-Dadah, R.K. Effect of evaporator temperature on the performance of water desalination/refrigeration adsorption system using AQSOA-ZO2. Int. J. Environ. Chem. Ecol. Geol. Eng. 2015, 9, 679–683. [Google Scholar]
- Jin, Y.; Kuznetsov, A.V. Turbulence modeling for flows in wall bounded porous media: An analysis based on direct numerical simulations. Phys. Fluids 2017, 29, 045102. [Google Scholar] [CrossRef]
- Nimvari, M.E.; Maerefat, M.; Jouybari, N.F.; El-hossaini, M.K. Numerical simulation of turbulent reacting flow in porous media using two macroscopic turbulence models. Comput. Fluids 2013, 88, 232–240. [Google Scholar] [CrossRef]
- Jiao, S.; Xu, Z. Selective gas diffusion in graphene oxides membranes: A molecular dynamics simulations study. ACS Appl. Mater. Interfaces 2015, 7, 9052–9059. [Google Scholar] [CrossRef]
- Xia, B.; Sun, D.W. Applications of computational fluid dynamics (CFD) in the food industry: A review. Comput. Electron. Agric. 2002, 34, 5–24. [Google Scholar] [CrossRef]
- Ji, X.; Li, M.; Fan, J.; Zhang, P.; Luo, B.; Wang, L. Structure optimization and performance experiments of a solar-powered finned-tube adsorption refrigeration system. Appl. Energy 2014, 113, 1293–1300. [Google Scholar] [CrossRef]
- Gong, L.X.; Wang, R.Z.; Xia, Z.Z.; Lu, Z.S. Experimental study on an adsorption chiller employing lithium chloride in silica gel and methanol. Int. J. Refrig. 2012, 35, 1950–1957. [Google Scholar] [CrossRef]

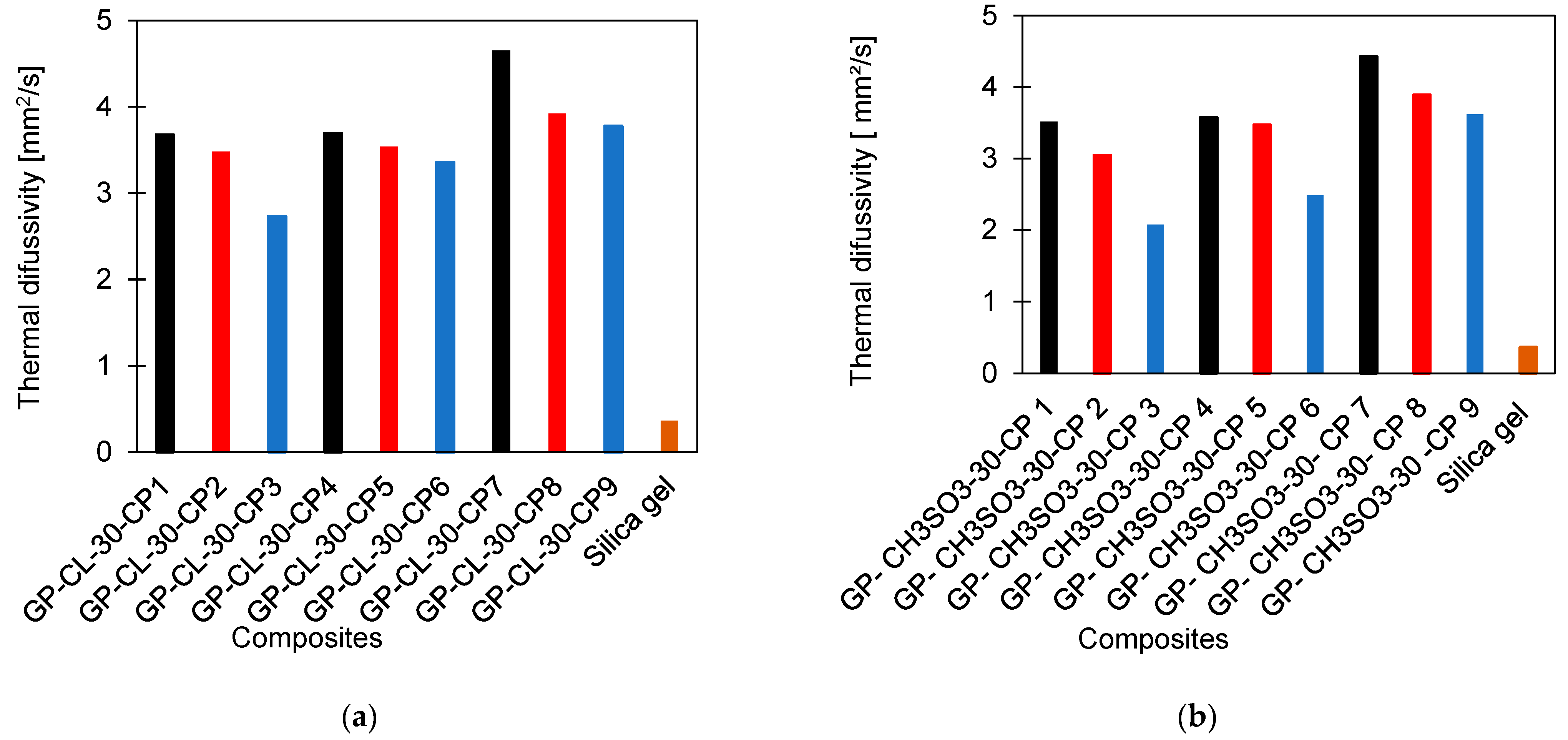


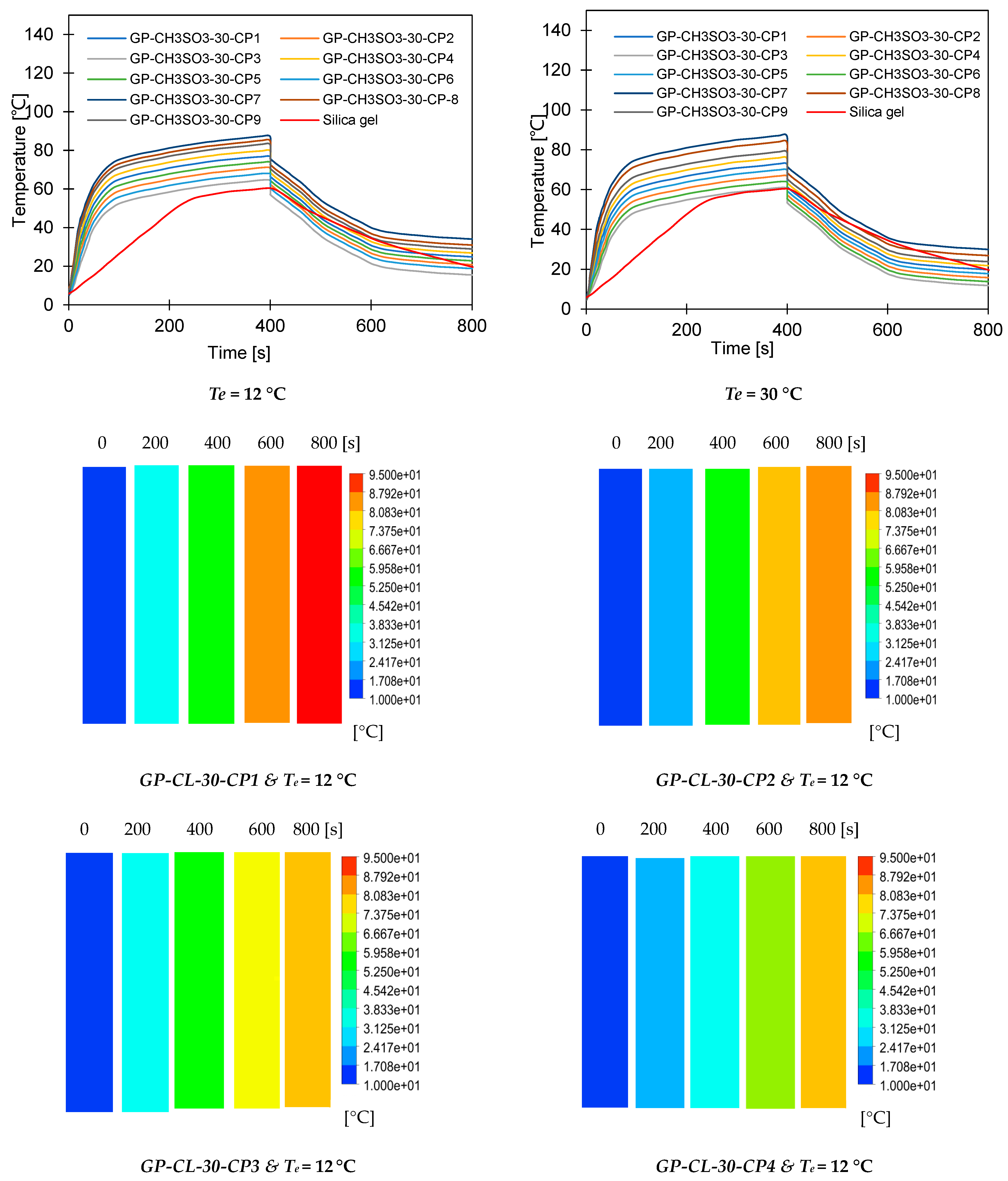
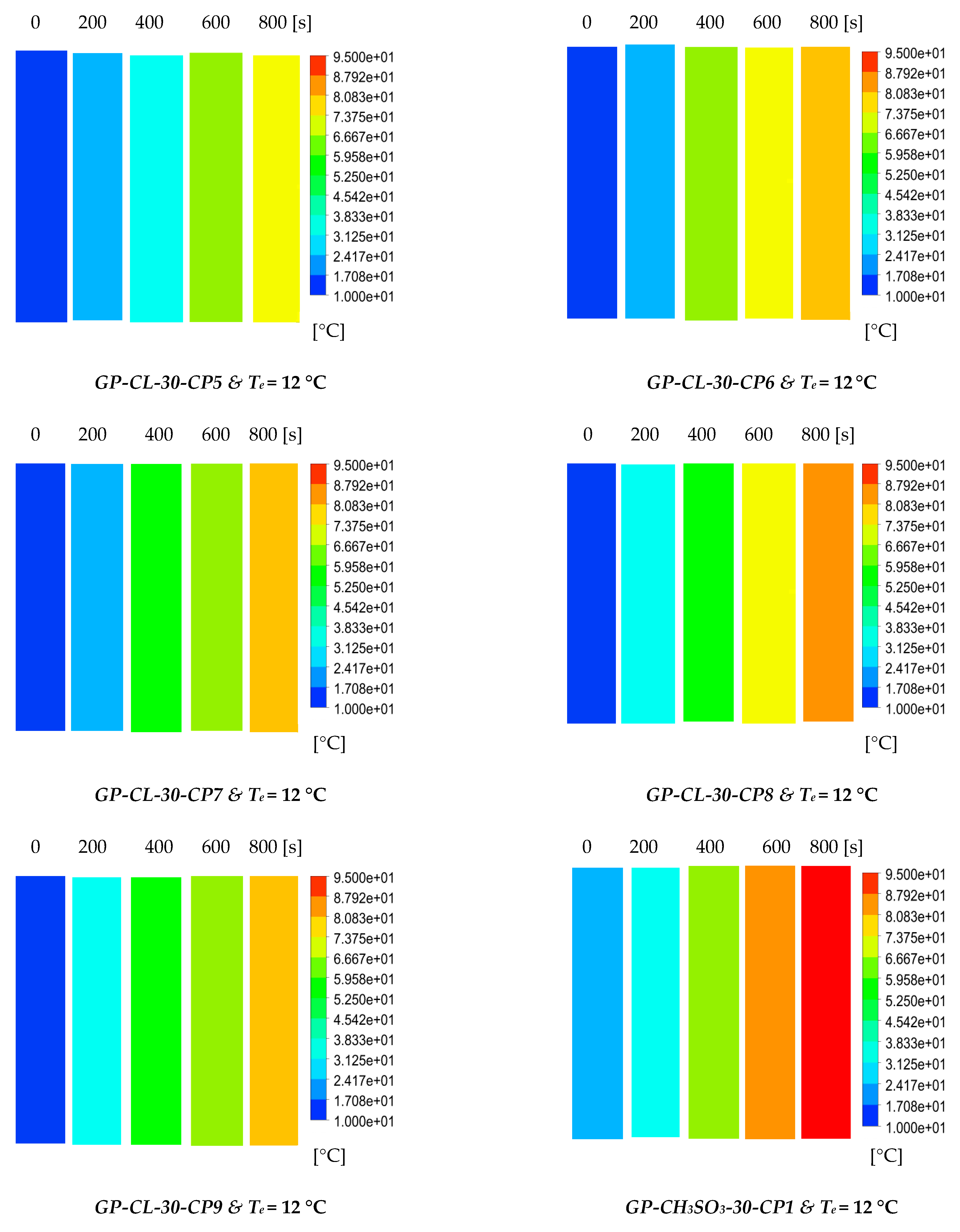
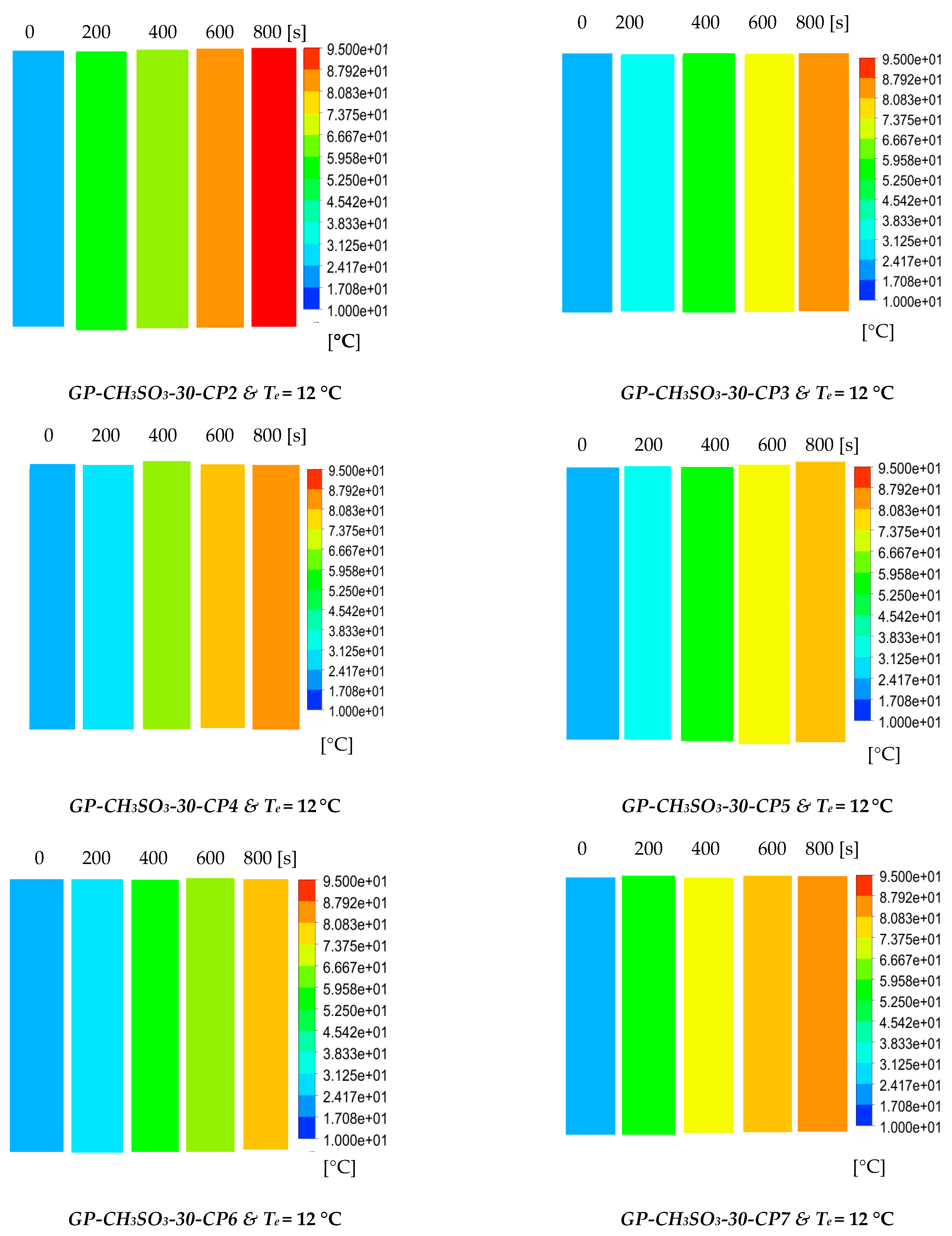


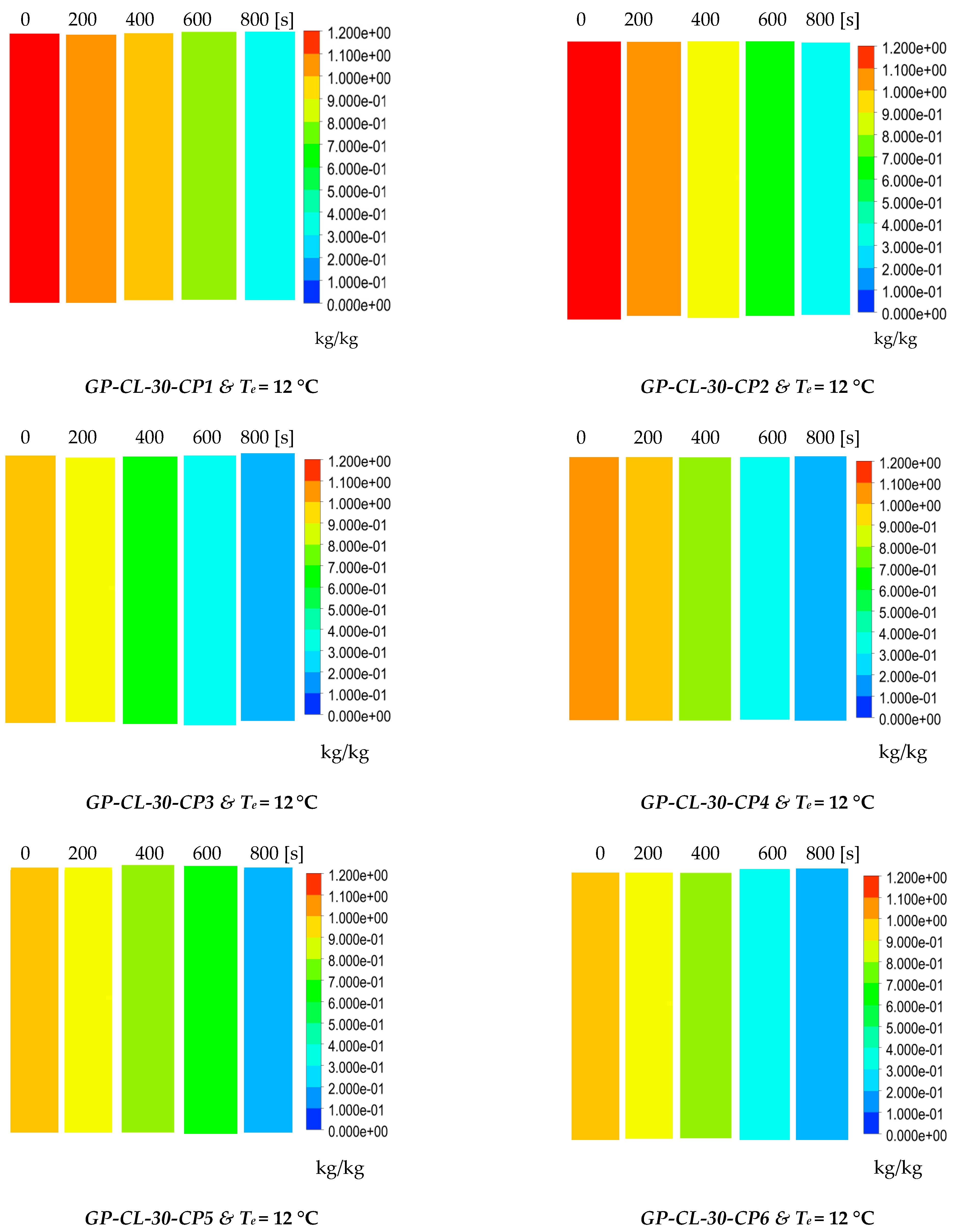







| Composite | Thermal Diffusivity (mm2/s) | Composite | Thermal Diffusivity (mm2/s) | PVA Concentration (%) | Compression Pressure (MPa) |
|---|---|---|---|---|---|
| GP-CL-30-CP1 | 3.679 | GP-CH3SO3-30-CP1 | 3.517 | 2 | 1 |
| GP-CL-30-CP2 | 3.479 | GP-CH3SO3-30-CP2 | 3.052 | 5 | 1 |
| GP-CL-30-CP3 | 2.734 | GP-CH3SO3-30-CP3 | 2.073 | 10 | 1 |
| GP-CL-30-CP4 | 3.694 | GP-CH3SO3-30-CP4 | 3.582 | 2 | 1.5 |
| GP-CL-30-CP5 | 3.540 | GP-CH3SO3-30-CP5 | 3.475 | 5 | 1.5 |
| GP-CL-30-CP6 | 3.362 | GP-CH3SO3-30-CP6 | 2.482 | 10 | 1.5 |
| GP-CL-30-CP7 | 4.652 | GP-CH3SO3-30-CP7 | 4.431 | 2 | 2 |
| GP-CL-30-CP8 | 3.922 | GP-CH3SO3-30-CP8 | 3.895 | 5 | 2 |
| GP-CL-30-CP9 | 3.779 | GP-CH3SO3-30-CP9 | 3.619 | 10 | 2 |
| Silica gel | 0.365 | Silica gel [40] | 0.312 | - | - |
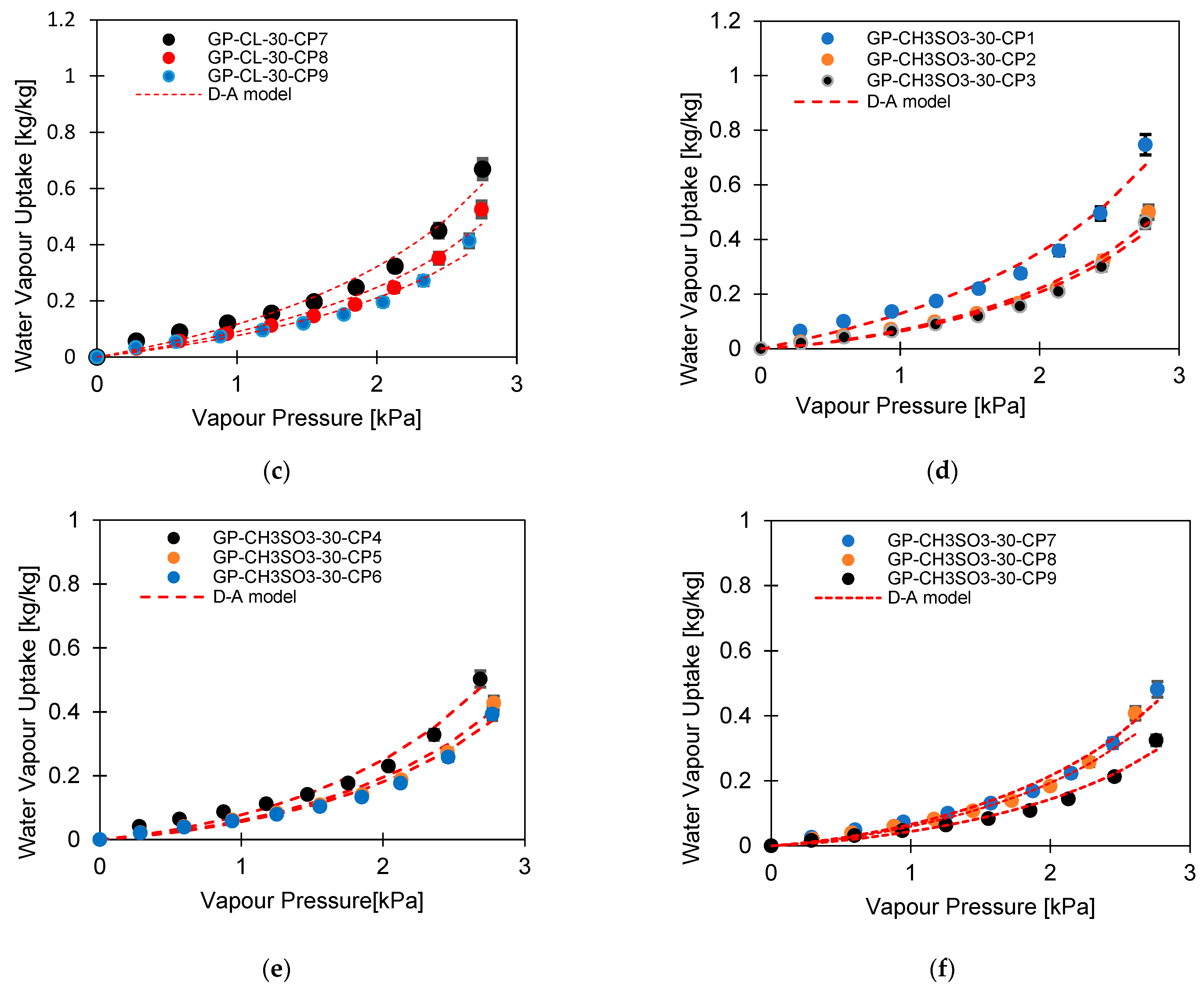
| Adsorbent | D-A Model Fitting Parameters | RMSD [%] | ||
|---|---|---|---|---|
| Maximum Uptake [kg kg−1] | Characteristics Energy (E) [kJ kg−1] | Heterogeneity Parameter (n) [-] | ||
| GP-CL-30-CP1 | 0.62 | 50.626 | 0.68 | 2.80 |
| GP-CL-30-CP2 | 0.657 | 50.626 | 0.68 | 2.33 |
| GP-CL-30-CP3 | 1.01 | 50.626 | 0.68 | 2.69 |
| GP-CL-30-CP4 | 0.79 | 50.626 | 0.68 | 2.31 |
| GP-CL-30-CP5 | 1.12 | 50.626 | 0.68 | 3.33 |
| GP-CL-30-CP6 | 1.03 | 50.626 | 0.68 | 2.96 |
| GP-CL-30-CP7 | 0.67 | 50.626 | 0.68 | 1.91 |
| GP-CL-30-CP8 | 0.84 | 50.626 | 0.68 | 2.57 |
| GP-CL-30-CP9 | 0.75 | 50.626 | 0.68 | 2.61 |
| Adsorbent | D-A Model Fitting Parameters | RMSD [%] | ||
|---|---|---|---|---|
| Maximum Uptake [kg kg−1] | Characteristics Energy [kJ kg−1] | Heterogeneity Parameter [-] | ||
| GP-CH3SO3-30-CP1 | 0.845 | 48.321 | 0.74 | 2.21 |
| GP-CH3SO3-30-CP2 | 0.65 | 48.321 | 0.74 | 1.82 |
| GP-CH3SO3-30-CP3 | 0.75 | 48.321 | 0.74 | 1.92 |
| GP-CH3SO3-30-CP4 | 0.61 | 48.321 | 0.74 | 1.55 |
| GP-CH3SO3-30-CP5 | 0.49 | 48.321 | 0.74 | 1.38 |
| GP-CH3SO3-30-CP6 | 0.70 | 48.321 | 0.74 | 1.86 |
| GP-CH3SO3-30-CP7 | 0.73 | 48.321 | 0.74 | 1.85 |
| GP-CH3SO3-30-CP8 | 0.66 | 48.321 | 0.74 | 2.17 |
| GP-CH3SO3-30-CP9 | 1.48 | 48.321 | 0.74 | 3.95 |
| Parameter | Value | Unit | |
|---|---|---|---|
| GP/IL Composite | Silica Gel | ||
| Dso | 4.4 × 10−4 | 2.54 × 10−4 | m2/s |
| Ea | 48.32–50.62 | 42 | kJ/mol |
| Rp | 2.9 × 10−4 | 0.16 × 10−5 | m |
| Material | Heat of Sorption (J/mol) | Material | Heat of Sorption (J/mol) |
|---|---|---|---|
| GP-CL-30-CP1 | 26,547 | GP-CH3SO3-30-CP1 | 26,424 |
| GP-CL-30-CP2 | 26,596 | GP-CH3SO3-30-CP2 | 26,641 |
| GP-CL-30-CP3 | 26,572 | GP-CH3SO3-30-CP3 | 26,720 |
| GP-CL-30-CP4 | 26,646 | GP-CH3SO3-30-CP4 | 26,398 |
| GP-CL-30-CP5 | 26,609 | GP-CH3SO3-30-CP5 | 26,640 |
| GP-CL-30-CP6 | 26,566 | GP-CH3SO3-30-CP6 | 26,631 |
| GP-CL-30-CP7 | 26,576 | GP-CH3SO3-30-CP7 | 26,587 |
| GP-CL-30-CP8 | 26,533 | GP-CH3SO3-30-CP8 | 26,767 |
| GP-CL-30-CP9 | 26,663 | GP-CH3SO3-30-CP9 | 26,635 |
| Fuji silica gel | 26,078 | Fuji silica gel [55] | 26,790 |
| Parameter | Value |
|---|---|
| Tube outer diameter (d0) | 27 mm |
| Tube inner diameter (di) | 24 mm |
| Fin height (hf) | 10 mm |
| Fin thickness () | 0.54 mm |
| Fin pitch (p) | 3.8 mm |
| Length of the finned tube (l) | 500 mm |
| Parameter | Value | Unit |
|---|---|---|
| MGP/IL | 0.2 | kg |
| MSG | 0.22 | kg |
| Mhex | 2.02 | kg |
| Ads/des bed flow rate | 0.036 | kg/s |
| Cond flow rate | 0.018 | kg/s |
| Evap flow rate | 0.048 | kg/s |
| Tdes | 65–85 | °C |
| Tads | 35 | °C |
| Tchw | 35 | °C |
| Tevap | 12–30 | °C |
| Tcw | 35 | °C |
| Tcond | 35 | °C |
| Cycle time | 400–800 | s |
| Component/ Process | CH3SO3-30-CP1-9 | Silica Gel | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| CP1 | CP2 | CP3 | CP4 | CP5 | CP6 | CP7 | CP8 | CP9 | ||
| Exergy Destruction (kW) | ||||||||||
| Adsorption at 35 °C | 6.05 | 6.88 | 6.55 | 6.21 | 6.70 | 6.87 | 7.4 | 7.26 | 7.19 | 7.17 |
| Desorption at 85 °C | 8.58 | 8.20 | 8.82 | 8.76 | 8.38 | 8.01 | 9.35 | 9.14 | 8.95 | 9.50 |
| Condenser at 35 °C | 1.12 | 1.2 | 1.22 | 1.21 | 1.22 | 1.3 | 1.30 | 1.00 | 1.10 | 1.20 |
| Evaporator at 30 °C | 1.31 | 1.05 | 1.00 | 1.16 | 1.08 | 1.01 | 1.28 | 1.24 | 1.20 | 1.30 |
| System overall | 17.1 | 17.3 | 18.7 | 17.6 | 17.4 | 17.5 | 19.3 | 18.6 | 18.44 | 19.17 |
| Exergy efficiency (%) | ||||||||||
| 40.9 | 37.2 | 30.8 | 38.8 | 34.3 | 29.7 | 35.8 | 32.8 | 28.6 | 16.6 | |
| Component/Process | GP-CL-30-CP1-9 | Silica Gel | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| CP1 | CP2 | CP3 | CP4 | CP5 | CP6 | CP7 | CP8 | CP9 | ||
| Exergy Destruction (kW) | ||||||||||
| Adsorption at 35 °C | 6.37 | 6.69 | 6.04 | 6.87 | 6.48 | 6.20 | 7.41 | 7.24 | 7.01 | 7.17 |
| Desorption at 85 °C | 8.29 | 8.94 | 8.53 | 8.60 | 8.50 | 8.71 | 9.10 | 8.91 | 8.72 | 9.5 |
| Condenser at 35 °C | 1.11 | 1.08 | 1.01 | 1.12 | 1.09 | 1.04 | 1.22 | 1.17 | 1.15 | 1.2 |
| Evaporator at 30 °C | 1.27 | 1.2 | 1.1 | 1.31 | 1.22 | 1.16 | 1.43 | 1.39 | 1.35 | 1.3 |
| System overall | 17.0 | 17.9 | 16.7 | 17.9 | 17.3 | 19.2 | 17.4 | 18.7 | 18.23 | 19.17 |
| Exergy efficiency (%) | ||||||||||
| 42.2 | 39.0 | 32 | 40.4 | 36 | 29.9 | 37.5 | 34.5 | 28.7 | 16.6 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Banda, H.; Rezk, A. Enhancing Heat and Mass Transfer in Adsorption Cooling and Desalination Systems Using Ionic Liquid and Graphene Consolidated Composites. Energies 2024, 17, 4856. https://doi.org/10.3390/en17194856
Banda H, Rezk A. Enhancing Heat and Mass Transfer in Adsorption Cooling and Desalination Systems Using Ionic Liquid and Graphene Consolidated Composites. Energies. 2024; 17(19):4856. https://doi.org/10.3390/en17194856
Chicago/Turabian StyleBanda, Handsome, and Ahmed Rezk. 2024. "Enhancing Heat and Mass Transfer in Adsorption Cooling and Desalination Systems Using Ionic Liquid and Graphene Consolidated Composites" Energies 17, no. 19: 4856. https://doi.org/10.3390/en17194856
APA StyleBanda, H., & Rezk, A. (2024). Enhancing Heat and Mass Transfer in Adsorption Cooling and Desalination Systems Using Ionic Liquid and Graphene Consolidated Composites. Energies, 17(19), 4856. https://doi.org/10.3390/en17194856









