Design, Simulation and Performance of a CSI Converter for Grid-Connected or Islanded Microgrids with High Step-Up Capability in PV Applications
Abstract
:1. Introduction
- Possibility of connection to isolated grids and a distribution grid;
- Connection to a very low voltage photovoltaic system, not exceeding 120 V;
- Drastic scaling of reactive elements;
- Improved performance in terms of harmonic content injected in the grid;
- Improvement of the overall reliability of the conversion system;
- Less susceptibility of the control system to grid disturbances, with particular regard to phenomena known as “harmonic stability” and greater robustness in terms of ability to contribute to grid.
Structure of Paper
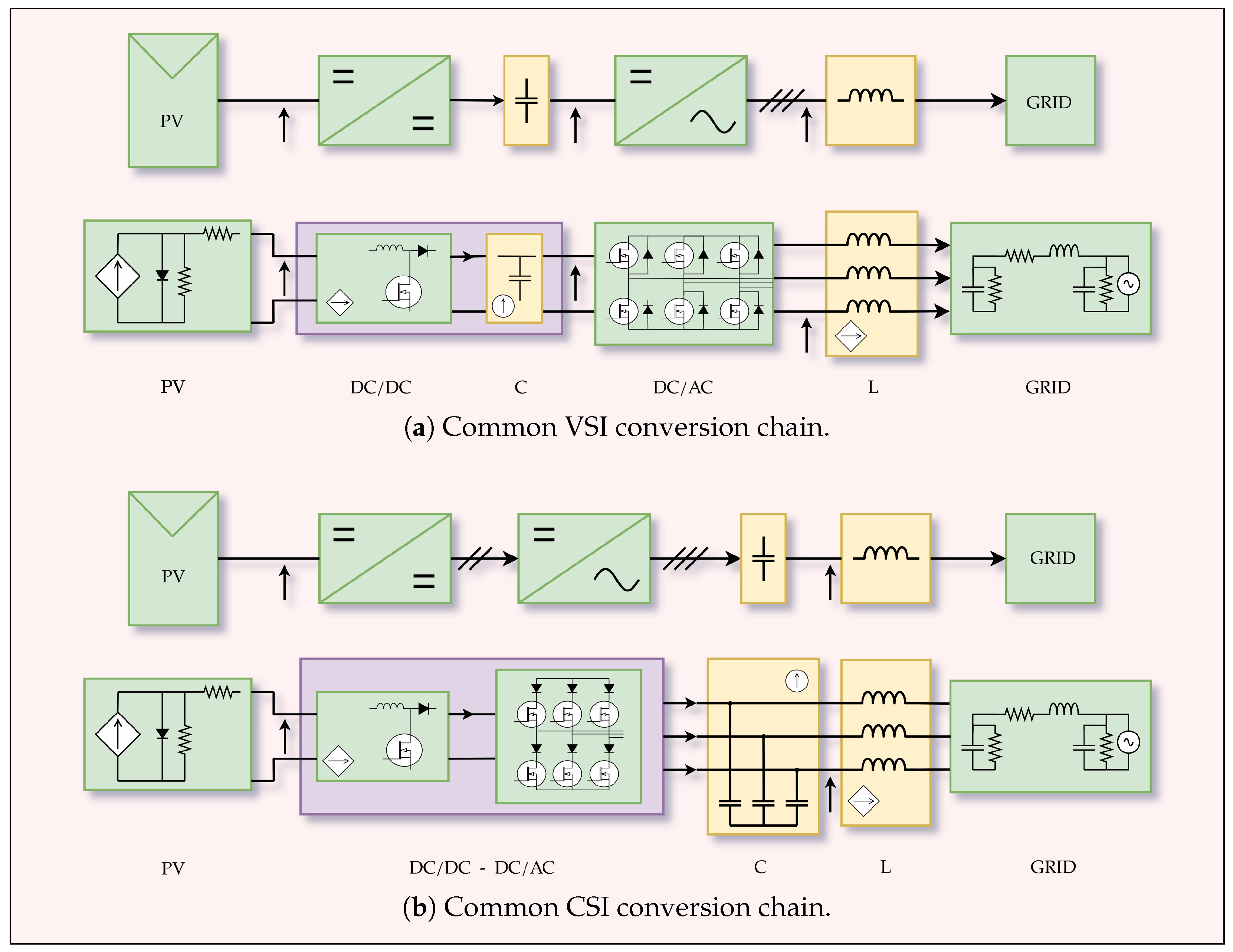
2. Issues and Advantages of Conversion Systems Based on CSI
- The first is that the CSI can be considered, from a strictly electrotechnical point of view, a current generator; as such, it presents two interesting characteristics, which are the ability to have a more stiff control current compared to the VSI and, therefore, to require the presence of reactive elements of much more limited value on the connection to the grid, (C) and (L). This fosters the design of control systems for the current fed into the grid;
- The second is that the inverter requires a current power supply, so the DC/DC converter has no capacity (C), so it is clear that many of the problems related to the control of VSI-based conversion lines, which have been mentioned above, are greatly reduced in the case of CSI. Numerous comparative studies have been done by researchers on these two different types of conversion [20,21,22,23], from which interesting evaluations have emerged that suggest the convenience of adopting one or the other based on specific applications. In particular, in regard to the solution shown in Figure 1b, notable advantages have been highlighted in the implementation and design of the control of the current feeding the grid; furthermore, the stability of the DC-link and the performance of the MPPT are significantly improved. An obvious drawback is the higher losses due to the currents recirculating in the DC-link inductor and in the bridge. The capacity value is lower, even if the DC-link inductor is particularly bulky and requires very careful design to limit conduction losses. Similar to what happens for passive elements, switching losses are better in power devices, but conduction losses are worse.
- The third consideration, perhaps the most relevant, is that the CSI inverter has natural boost characteristics and, therefore, if the DC/DC converter is designed to support these characteristics, it is possible to avoid transformers and also to limit the size of the necessary reactive elements, both on the DC-link and on the grid connection.
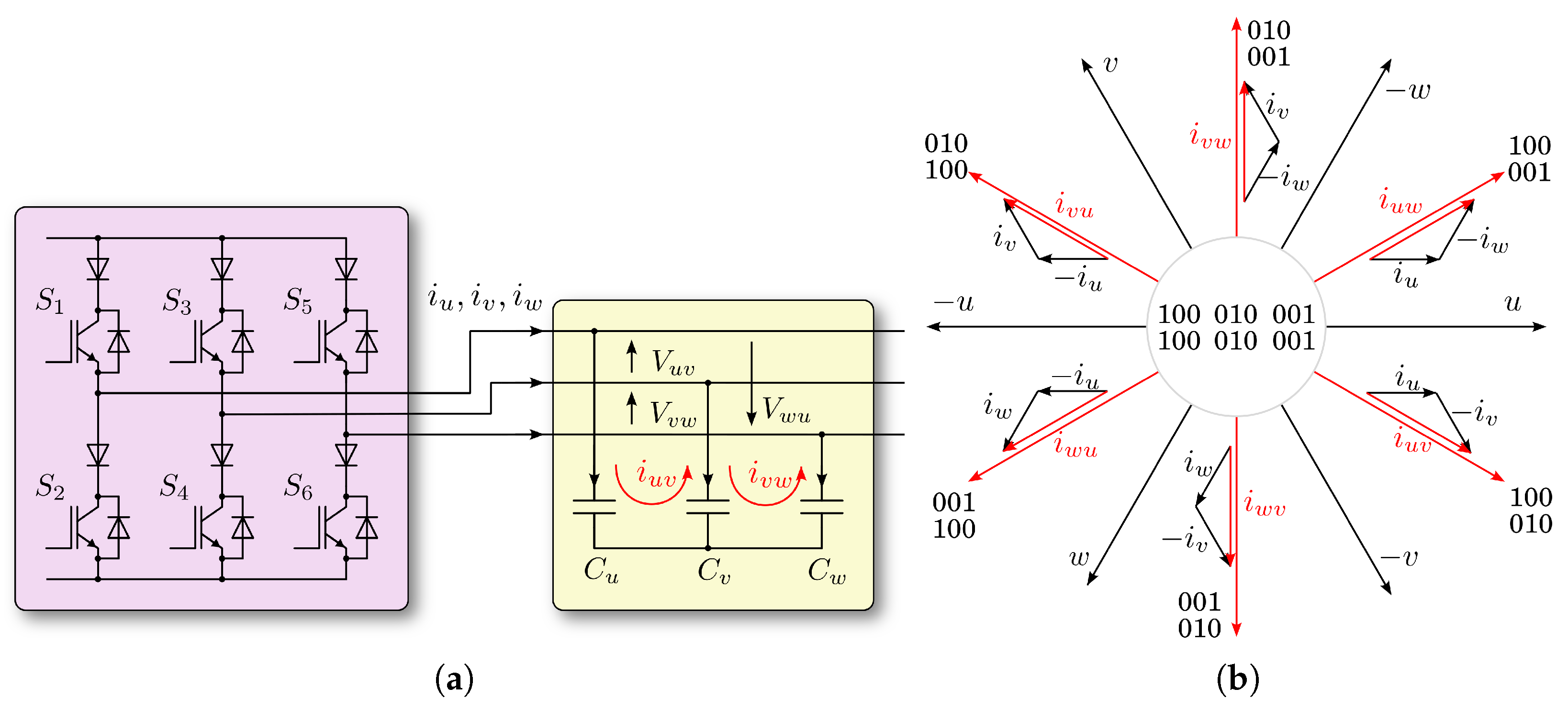
3. CSI Bridge
3.1. CSI Modulation Strategy
- Reduce the complexity of the calculation algorithms given that the entire modulation and control system will have to be implemented on a single microcontroller (Texas Instruments, C2000™ 32-bit MCU F28379D, Dallas, TX, USA). Programming is performed using Simulink Embedded Coder® Support Package (Matlab® release R2024a, from Mathworks Inc., update 3). which offers important advantages for rapid prototyping, software portability, and the production of standardized code for embedded systems. The Simulink® (Matlab® release R2024a, from Mathworks Inc., update 3) models, described below, were programmed according to criteria for the automatic production of code on a microcontroller; therefore, the functions for managing code under interrupt and the discretization of some variables were implemented;
- Modulation with vector current references, therefore of SVM type;
- Use of techniques aimed at the optimal exploitation of the devices, therefore symmetrical modulation in the respective quadrants.
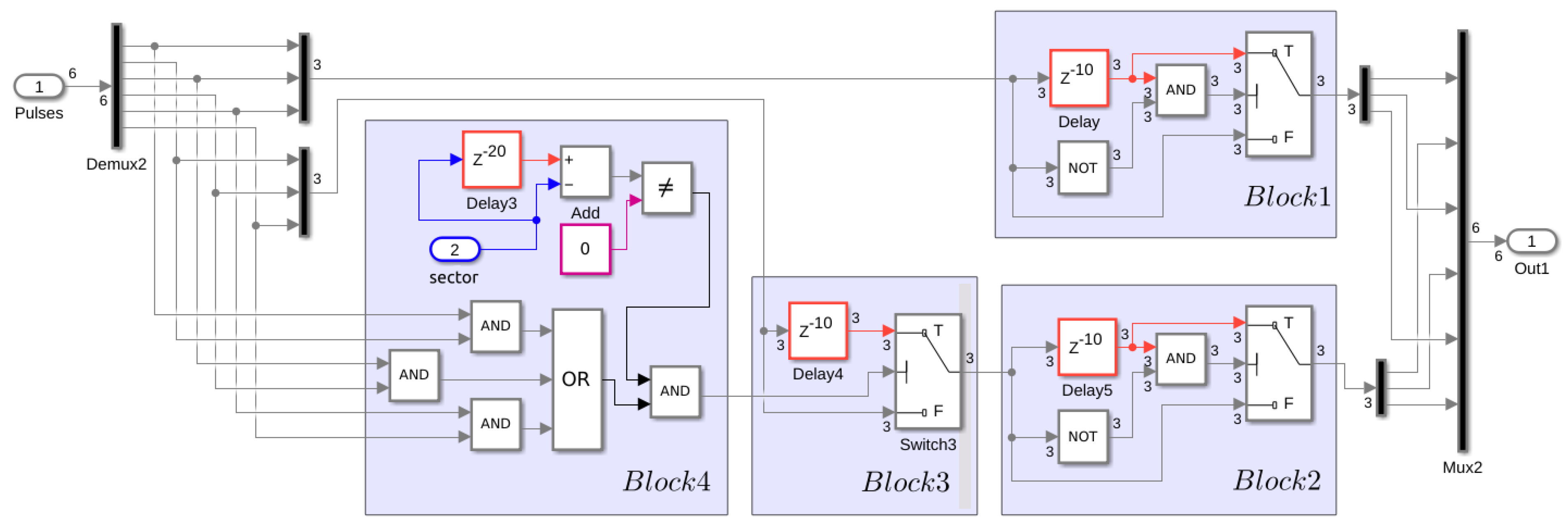
3.2. Dead Time Implementation
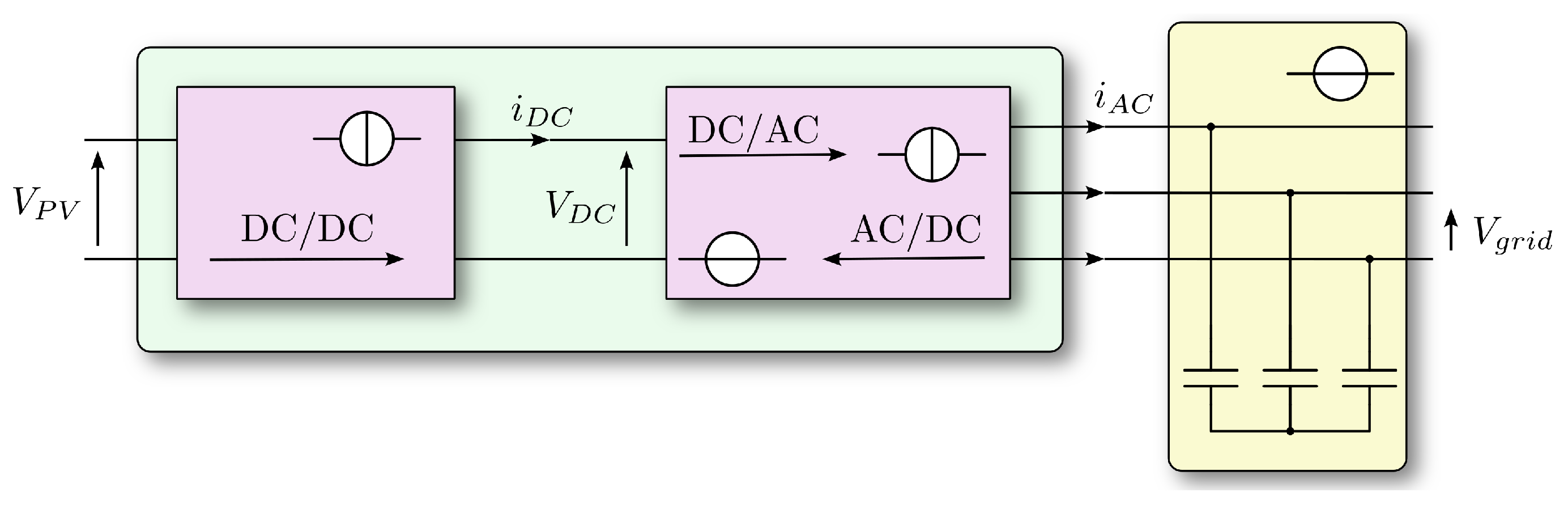
4. Key Considerations on the DC/DC Conversion Stage Structure and Control
4.1. DC/DC Stage Topology Selection for Low Voltage PV Source
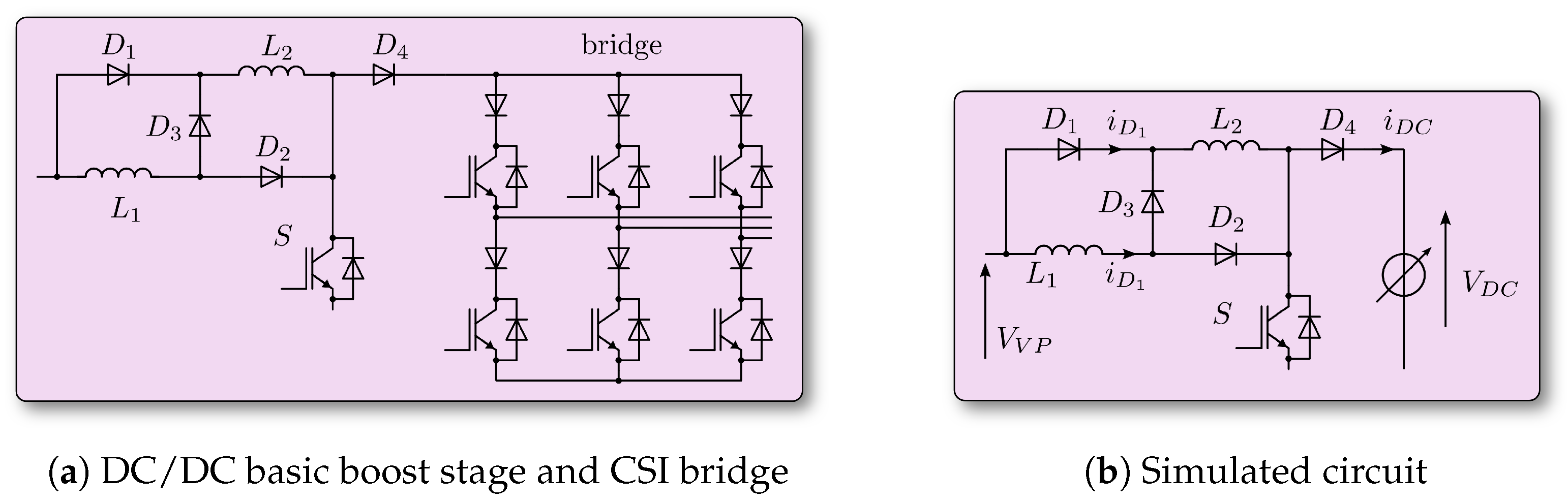
4.2. General Topology of Proposed System and Validation of DC/DC Converter Stage
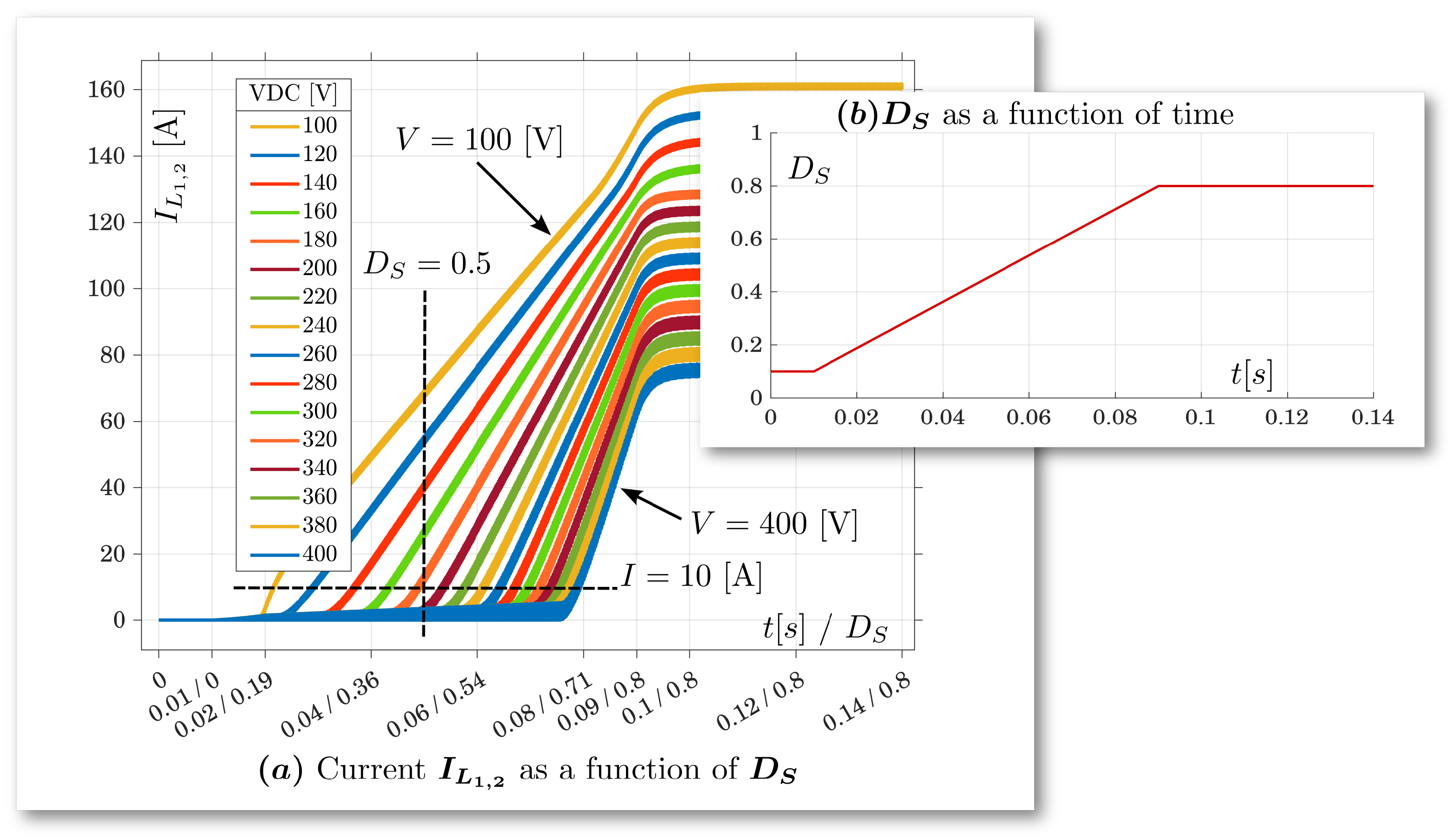
- The circuit configuration of Figure 8 is capable of supplying a minimum value of current only by exceeding a certain DS for each value of the VDC voltage. In order to have a more detailed reference, Table 1 shows the DS value necessary to supply a minimum current of 10 A towards the CSI bridge for different values of the DC-link voltage.
- Beyond this value, the current increases quite linearly up to the maximum DS value, equal to 0.8, which was set in the simulations.
- In Figure 4b, it is worth noting the maximum current values IL1,2 that can be obtained with DS equal to 0.5. This current ranges from 10 A, for a VDC equal to 220 V, to over 80 A for a VDC equal to 100 V. Beyond this limit, it is not possible to obtain current values higher than the minimum limit of 10 A without increasing the DS value.
4.3. Converter Topology and Layout Issues
- Reduce the harmonic content of the voltage generated by the CSI;
- Reduce switching losses;
- Improve dynamic performance;
- Improve the exploitation of switching devices and balance switching and conduction losses.
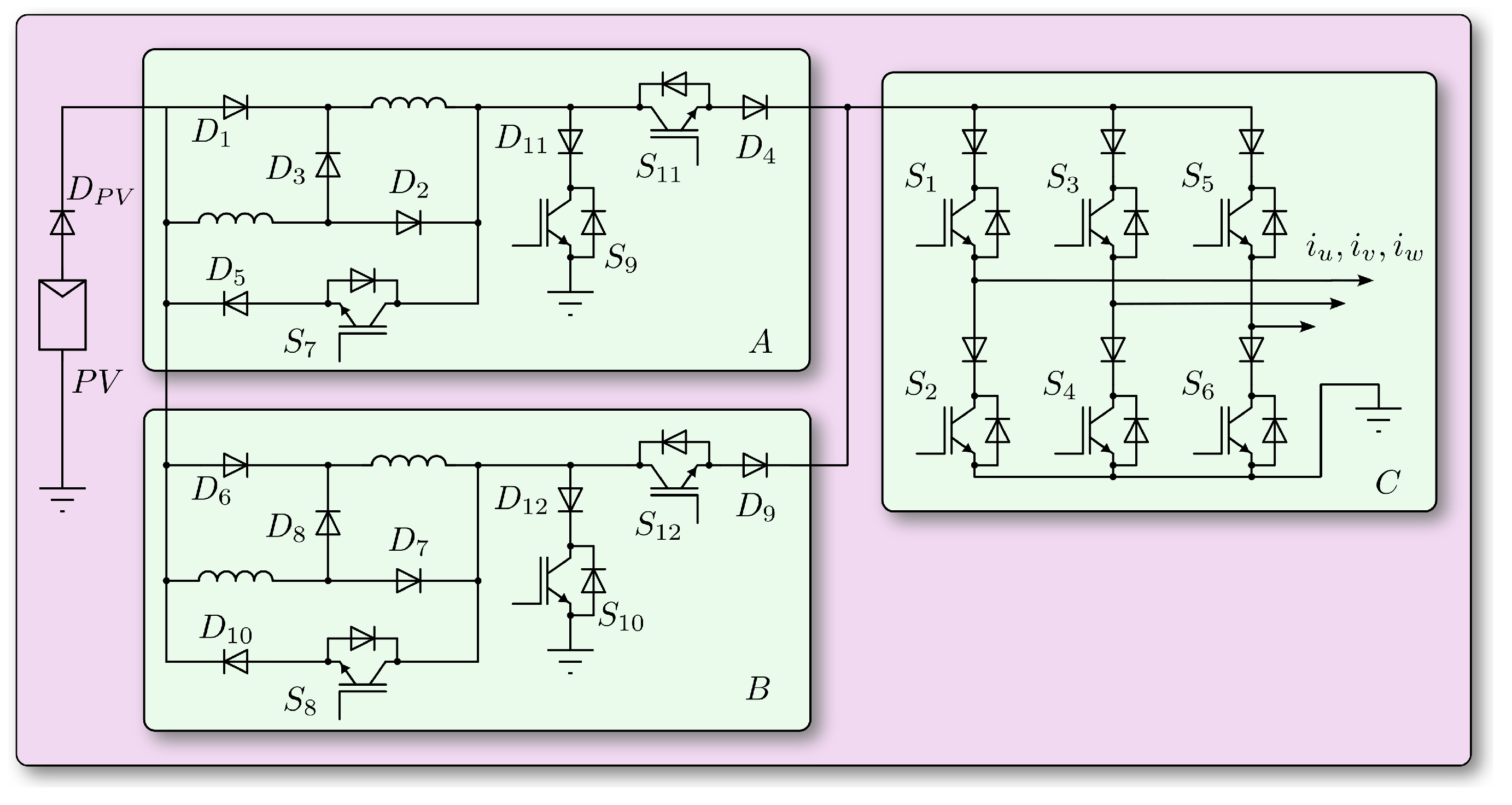
4.4. Compatibility of a Single DC/DC Boost Stage with the CSI Bridge
Doubling Switched-Inductors
5. Simulation Results
6. Discussion
- The topology identified and the modulation technique described allow for an independent regulation of the DC-link current and of the current that the bridge transfers to the grid. In the case of a distributed grid, these choices favor the design of a dynamically more efficient system for the regulation of the power absorbed by the photovoltaic system and therefore the implementation of more performing MPPT strategies. In the case of an isolated grid, the punctual management of the current allows one to respond more quickly to the requests of the connected load, limiting to a minimum the circulation of current inside the conversion system and reducing the conduction losses both in the inductors and in the power devices.
- The data represented in Figure 9, together with the description of the method with which they were obtained, are useful for the characterization of a cascaded SI module with a generic voltage generator in the range of the electrical quantities that were used. Therefore, they are general data useful for the design of systems that adopt this conversion topology.
- The possibility of recirculating the current through the inductors of the DC/DC stage allows one to avoid the use of the so-called fourth leg of the CSI bridge, known in literature with the acronym CSI7 or H7 [33,35,36], since the current coming from the SI modules is zero during the free-wheeling of the bridge, despite this typology being established with a certain frequency in the design of CSI converters and has even been defined as a “universal solution for CSI converters” [34].
Application Possibilities of the Described Conversion System in Fields Other than Photovoltaic Energy Production
- Based on a CSI bridge;
- Reactive power regulation capability;
- High step-up capabilities;
- Not an isolated system;
- Transformerless;
- Allows for managing the energy flow from the low voltage source to a three-phase network;
- Low harmonic content of the current fed into the grid.
7. Patents
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| CSI | Current source inverter |
| VSI | Voltage source inverter |
| SI | Generic switching Inductors module |
| VDC | DC-link voltage |
| S | Generic switch device of DC/DC boost stage |
| DS | Duty-cycle of one stage DC/DC |
| IL | Inductor current |
| SVM | Space vector modulation, or space vector modulator |
| Local mean value of DC-link current | |
| T | Transfer mode of CSI bridge |
| FW | Free-wheeling mode of CSI bridge |
| A-SI | Switching inductors module A |
| B-SI | Switching inductors module B |
| AC | A-SI charging state |
| ACH | A-SI current holding state |
| AT | A-SI current transfer state |
| BC/BCH/BT | The same as above but for the B-SI module |
Appendix A. Three-Dimensional Data Table Containing the Switching Status
| Device | ||||||
|---|---|---|---|---|---|---|
| 1 2 3 4 5 6 7 | 1 2 3 4 5 6 7 | 1 2 3 4 5 6 7 | 1 2 3 4 5 6 7 | 1 2 3 4 5 6 7 | 1 2 3 4 5 6 7 | |
| 1 1 1 0 1 1 1 | 0 1 0 0 0 1 0 | 0 0 0 1 0 0 0 | 1 0 0 0 0 0 1 | 0 0 0 0 0 0 0 | 0 0 1 1 1 0 0 | |
| 1 0 0 0 0 0 1 | 0 0 0 0 0 0 0 | 0 0 1 1 1 0 0 | 1 1 1 0 1 1 1 | 0 1 0 0 0 1 0 | 0 0 0 1 0 0 0 | |
| 0 0 0 0 0 0 0 | 0 0 1 1 1 0 0 | 1 1 1 0 1 1 1 | 0 1 0 0 0 1 0 | 0 0 0 1 0 0 0 | 1 0 0 0 0 0 1 | |
| 0 1 0 0 0 1 0 | 0 0 0 1 0 0 0 | 1 0 0 0 0 0 1 | 0 0 0 0 0 0 0 | 0 0 1 1 1 0 0 | 1 1 1 0 1 1 1 | |
| 0 0 0 1 0 0 0 | 1 0 0 0 0 0 1 | 0 0 0 0 0 0 0 | 0 0 1 1 1 0 0 | 1 1 1 0 1 1 1 | 0 1 0 0 0 1 0 | |
| 0 0 1 1 1 0 0 | 1 1 1 0 1 1 1 | 0 1 0 0 0 1 0 | 0 0 0 1 0 0 0 | 1 0 0 0 0 0 1 | 0 0 0 0 0 0 0 |
References
- Jasińska, E.; Jasiński, M.; Leonowicz, Z.; Martirano, L.; Gono, R.; Jasiński, M. Various Aspects of Energy Transition: Technologies, Economy, and Social Synergies to Sustainable Future. In Proceedings of the 2023 IEEE International Conference on Environment and Electrical Engineering and 2023 IEEE Industrial and Commercial Power Systems Europe (EEEIC/I&CPS Europe), IEEE, Madrid, Spain, 6–9 June 2023; pp. 1–4. [Google Scholar] [CrossRef]
- UN. The Sustainable Development Agenda. Available online: https://www.un.org/sustainabledevelopment/ (accessed on 10 May 2024).
- Semenova, N.; Hassel, L.G. On the Validity of Environmental Performance Metrics. J. Bus. Ethics 2014, 132, 249–258. [Google Scholar] [CrossRef]
- Escrig-Olmedo, E.; Fernández-Izquierdo, M.; Ferrero-Ferrero, I.; Rivera-Lirio, J.; Muñoz-Torres, M. Rating the Raters: Evaluating how ESG Rating Agencies Integrate Sustainability Principles. Sustainability 2019, 11, 915. [Google Scholar] [CrossRef]
- Braccini, A.; Margherita, E. Exploring Organizational Sustainability of Industry 4.0 under the Triple Bottom Line: The Case of a Manufacturing Company. Sustainability 2018, 11, 36. [Google Scholar] [CrossRef]
- Wang, H. Investment Efficiency and Cost Analysis of New Renewable Energy Sources. In Proceedings of the 2020 IEEE Sustainable Power and Energy Conference (iSPEC), Chengdu, China, 23–25 November 2020. [Google Scholar] [CrossRef]
- Yang, Y.; Du, Z.; Zhou, X.; Chen, Y.; Zheng, L.; Xu, Y. Harmonic Stability Analysis of a System Integrated with Photovoltaic Power Generation and Energy Storage Based on Impedance Analysis Method. In Proceedings of the 2023 13th International Conference on Power and Energy Systems (ICPES), Chengdu, China, 8–10 December 2023; pp. 373–378. [Google Scholar] [CrossRef]
- Wang, X.; Blaabjerg, F. Harmonic Stability in Power Electronic-Based Power Systems: Concept, Modeling, and Analysis. IEEE Trans. Smart Grid 2019, 10, 2858–2870. [Google Scholar] [CrossRef]
- Enslin, J.; Heskes, P. Harmonic Interaction Between a Large Number of Distributed Power Inverters and the Distribution Network. IEEE Trans. Power Electron. 2004, 19, 1586–1593. [Google Scholar] [CrossRef]
- Li, Y.; Fan, L.; Miao, Z. Wind in Weak Grids: Low-Frequency Oscillations, Subsynchronous Oscillations, and Torsional Interactions. IEEE Trans. Power Syst. 2020, 35, 109–118. [Google Scholar] [CrossRef]
- Ali, K.; Putranto, L.M.; Imam, A.; Tumiran; Yasirroni, M. Determination of Optimal PV Energy Share Considering Voltage Stability Index. In Proceedings of the 2020 2nd International Conference on Smart Power amp; Internet Energy Systems (SPIES), Bangkok, Thailand, 15–18 September 2020. [CrossRef]
- Uzun, U.E.; Pamuk, N.; Taskin, S. Effect of Solar Photovoltaic Generation Systems on Voltage Stability. In Proceedings of the 2022 Global Energy Conference (GEC), Batman, Turkey, 26–29 October 2022. [Google Scholar] [CrossRef]
- Sun, J. Impedance-Based Stability Criterion for Grid-Connected Inverters. IEEE Trans. Power Electron. 2011, 26, 3075–3078. [Google Scholar] [CrossRef]
- Holmes, D.G.; Lipo, T.A.; McGrath, B.P.; Kong, W.Y. Optimized Design of Stationary Frame Three Phase AC Current Regulators. IEEE Trans. Power Electron. 2009, 24, 2417–2426. [Google Scholar] [CrossRef]
- Midtsund, T.; Suul, J.A.; Undeland, T. Evaluation of current controller performance and stability for voltage source converters connected to a weak grid. In Proceedings of the 2nd International Symposium on Power Electronics for Distributed Generation Systems, Hefei, China, 16–18 June 2010. [Google Scholar] [CrossRef]
- Nasiri, M.; Chandra, S.; Taherkhani, M.; McCormack, S.J. Impact of Input Capacitors in Boost Converters on Stability and Maximum Power Point Tracking in PV systems. In Proceedings of the 2021 IEEE 48th Photovoltaic Specialists Conference (PVSC), Virtual Conference, 20–25 June 2021. [Google Scholar] [CrossRef]
- Santra, S.B.; Chatterjee, D.; Kumar, K.; Bertoluzzo, M.; Sangwongwanich, A.; Blaabjerg, F. Capacitor Selection Method in PV Interfaced Converter Suitable for Maximum Power Point Tracking. IEEE J. Emerg. Sel. Top. Power Electron. 2021, 9, 2136–2146. [Google Scholar] [CrossRef]
- Moradi-Shahrbabak, Z.; Bakhshai, A.; Tabesh, A. Effect of dc-link capacitor on small signal stability of grid connected PV power plants. In Proceedings of the 2018 IEEE 12th International Conference on Compatibility, Power Electronics and Power Engineering (CPE-POWERENG 2018), Doha, Qatar, 10–12 April 2018. [Google Scholar] [CrossRef]
- Yan, G.; Ren, J.; Mu, G.; Jin, L.; Duan, S.; Jia, Q. DC-link voltage stability analysis for single-stage photovoltaic VSIs connected to weak grid. In Proceedings of the 2016 IEEE 8th International Power Electronics and Motion Control Conference (IPEMC-ECCE Asia), Hefei, China, 22–26 May 2016. [Google Scholar] [CrossRef]
- Azmi, S.; Ahmed, K.; Finney, S.; Williams, B. Comparative analysis between voltage and current source inverters in grid-connected application. In Proceedings of the IET Conference on Renewable Power Generation (RPG 2011), Edinburgh, UK, 6–8 September 2011. [Google Scholar] [CrossRef]
- Potdukhe, K.C.; Munshi, A.P.; Munshi, A.A. Reliability prediction of new improved current source inverter (CSI) topology for transformer-less grid connected solar system. In Proceedings of the 2015 IEEE Power, Communication and Information Technology Conference (PCITC), Bhubaneswar, India, 5–17 October 2015; pp. 373–378. [Google Scholar] [CrossRef]
- Rajeev, M.; Agarwal, V. Single Phase Current Source Inverter With Multiloop Control for Transformerless Grid–PV Interface. IEEE Trans. Ind. Appl. 2018, 54, 2416–2424. [Google Scholar] [CrossRef]
- Wang, Z.; Miao, Z.; Fan, L.; Yazdani, A. Weak Grid Operation of A Grid-Following Current-Sourced PV Solar System. In Proceedings of the 2021 North American Power Symposium (NAPS), College Station, TX, USA, 14–16 November 2021; pp. 1–6. [Google Scholar] [CrossRef]
- Cheng, L.; Chen, P.; Yang, A.; Zhang, Q.; Wang, L.; Qian, Z.; Zhang, M.; Zhang, T. A Multilevel Asymmetric Current Source Inverter Topology Using Switched Inductor. In Proceedings of the 2022 4th International Conference on Power and Energy Technology (ICPET), Xining, China, 12–14 May 2022. [Google Scholar] [CrossRef]
- Mao, M.; Zheng, Y.; Chang, L.; Xu, H. A single-stage high gain current source inverter for grid-connected photovoltaic system. In Proceedings of the 2015 9th International Conference on Power Electronics and ECCE Asia (ICPE-ECCE Asia), Seoul, Republic of Korea, 1–5 June 2015; pp. 1902–1907. [Google Scholar] [CrossRef]
- Mao, M.; Li, Y.; Chang, L. Optimal design and experiment validation of switching inductor based tri-state CSI. In Proceedings of the 2017 Chinese Automation Congress (CAC), Jinan, China, 20–22 October 2017; pp. 6923–6927. [Google Scholar] [CrossRef]
- Li, C.; Chang, L.; Mao, M. Improved SVM for High Gain Tri-state CSI to Reduce DC Side Inductor Current Ripple. In Proceedings of the 2019 10th International Conference on Power Electronics and ECCE Asia (ICPE 2019—ECCE Asia), Busan, Republic of Korea, 27–30 May 2019. [Google Scholar] [CrossRef]
- Stephen, A.; Remya, K.P.; Gomathy, S. Review on Non-isolated High Gain DC-DC Converters. In Proceedings of the 2022 Third International Conference on Intelligent Computing Instrumentation and Control Technologies (ICICICT), Kannur, India, 11–12 August 2022. [Google Scholar] [CrossRef]
- Gnanasambandam, K.; Rathore, A.K.; Edpuganti, A.; Srinivasan, D. A Novel Optimal Space Vector Modulation Technique of Current Source Inverter for Solar Power Integration. In Proceedings of the 2018 IEEE International Conference on Power Electronics, Drives and Energy Systems (PEDES), Chennai, India, 18–21 December 2018. [Google Scholar] [CrossRef]
- Liu, Y.; Wang, M.; Xu, Z.; Yan, S. MPC Control of Three-Phase CSI in Unbalanced Grid. In Proceedings of the 2022 International Conference on Power Energy Systems and Applications (ICoPESA), Singapore, 25–27 February 2022; pp. 357–361. [Google Scholar] [CrossRef]
- Aguirre, M.P.; Calvino, L.; Valla, M.I. Multilevel Current-Source Inverter with FPGA Control. IEEE Trans. Ind. Electron. 2013, 60, 3–10. [Google Scholar] [CrossRef]
- Guo, X.; Xu, D.; Wu, B. Four-Leg Current-Source Inverter with a New Space Vector Modulation for Common-Mode Voltage Suppression. IEEE Trans. Ind. Electron. 2015, 62, 6003–6007. [Google Scholar] [CrossRef]
- Anand, S.; Gundlapalli, S.K.; Fernandes, B.G. Transformer-Less Grid Feeding Current Source Inverter for Solar Photovoltaic System. IEEE Trans. Ind. Electron. 2014, 61, 5334–5344. [Google Scholar] [CrossRef]
- Wang, W.; Gao, F.; Rui, S. Operation and modulation of H7 current source inverter with hybrid SiC and Si semiconductor switches. In Proceedings of the 2015 9th International Conference on Power Electronics and ECCE Asia (ICPE-ECCE Asia), Seoul, Republic of Korea, 1–5 June 2015. [Google Scholar] [CrossRef]
- Lorenzani, E.; Immovilli, F.; Migliazza, G.; Frigieri, M.; Bianchini, C.; Davoli, M. CSI7: A Modified Three-Phase Current-Source Inverter for Modular Photovoltaic Applications. IEEE Trans. Ind. Electron. 2017, 64, 5449–5459. [Google Scholar] [CrossRef]
- Bendre, A.; Wallace, I.; Nord, J.; Venkataramanan, G. A current source PWM inverter with actively commutated SCRs. IEEE Trans. Power Electron. 2002, 17, 461–468. [Google Scholar] [CrossRef]
- Di Fazio, A.R.; Russo, M.; Valeri, S.; De Santis, M. LV distribution system modelling for distributed energy resources. In Proceedings of the 2016 IEEE 16th International Conference on Environment and Electrical Engineering (EEEIC), Florence, Italy, 7–10 June 2016; pp. 1–6. [Google Scholar] [CrossRef]
- Catellani, S.; Bier, A.; Martin, J.; Gabriel, L.; Barruel, F. Characterization of 1.2 kV Silicon Carbide (SiC) semiconductors in hard switching mode for three-phase Current Source Inverter (CSI) prototyping in. In Proceedings of the PCIM Europe 2015, International Exhibition and Conference for Power Electronics, Intelligent Motion, Renewable Energy and Energy Management, Nuremberg, Germany, 19–20 May 2015; pp. 1–8. [Google Scholar]
- Lee, Y.; Castellazzi, A. Discrete-time optimization of current-sensor-less control for a high-frequency all-SiC CSI converter. In Proceedings of the 2022 25th International Conference on Electrical Machines and Systems (ICEMS), Chiang Mai, Thailand, 29 November–2 December 2022; pp. 1–5. [Google Scholar] [CrossRef]
- Sahan, B.; Araújo, S.V.; Nöding, C.; Zacharias, P. Comparative Evaluation of Three-Phase Current Source Inverters for Grid Interfacing of Distributed and Renewable Energy Systems. IEEE Trans. Power Electron. 2011, 26, 2304–2318. [Google Scholar] [CrossRef]
- Singh, A.; Mirafzal, B. A low-voltage generator-converter topology for Direct Drive Wind Turbines. In Proceedings of the 2016 IEEE 7th International Symposium on Power Electronics for Distributed Generation Systems (PEDG), Vancouver, BC, Canada, 27–30 June 2016; Volume 24, pp. 1–6. [Google Scholar] [CrossRef]
- Kavousi, A.; Fathi, S.H.; Milimonfared, J.; Soltani, M.N. Application of Boost Converter to Increase the Speed Range of Dual-Stator Winding Induction Generator in Wind Power Systems. IEEE Trans. Power Electron. 2018, 33, 9599–9610. [Google Scholar] [CrossRef]
- Wang, H.; Nayar, C.; Su, J.; Ding, M. Control and interfacing of a grid-connected small scale wind turbine generator. In Proceedings of the 2009 Australasian Universities Power Engineering Conference, Adelaide, Australia, 27–30 September 2009; pp. 1–5. [Google Scholar]
- Killeen, P.; Ghule, A.N.; Ludois, D.C. A Medium-Voltage Current Source Inverter for Synchronous Electrostatic Drives. IEEE J. Emerg. Sel. Top. Power Electron. 2022, 10, 1597–1608. [Google Scholar] [CrossRef]
- Fernández, E.; Coello, M. Control drive for SMPMSM in CSI converter with SiC devices. In Proceedings of the 2017 IEEE 37th Central America and Panama Convention (CONCAPAN XXXVII), Managua, Nicaragua, 15–17 November 2017; pp. 1–6. [Google Scholar] [CrossRef]
- Lee, Y.; Castellazzi, A. Implementation options of a fully SiC Buck-CSI for advanced motor drive application. In Proceedings of the 2022 24th European Conference on Power Electronics and Applications (EPE’22 ECCE Europe), Hanover, Germany, 5–9 September 2022; pp. P.1–P.11. [Google Scholar]
- Tang, L.; Su, G.J. Boost mode test of a current-source-inverter-fed permanent magnet synchronous motor drive for automotive applications. In Proceedings of the 2010 IEEE 12th Workshop on Control and Modeling for Power Electronics (COMPEL), Boulder, CO, USA, 28–30 June 2010; Volume 28, pp. 1–8. [Google Scholar] [CrossRef]
- Su, G.J.; Tang, L.; Wu, Z. Extended constant-torque and constant-power speed range control of permanent magnet machine using a current source inverter. In Proceedings of the 2009 IEEE Vehicle Power and Propulsion Conference, Dearborn, MI, USA, 7–10 September 2009; Volume 137, pp. 109–115. [Google Scholar] [CrossRef]
- Ma, F.; Kuang, Y.; Wang, Z.; Huang, G.; Kuang, D.; Zhang, C. Multi-Port and -Functional Power Conditioner and Its Control Strategy with Renewable Energy Access for a Railway Traction System. Energies 2021, 14, 6146. [Google Scholar] [CrossRef]
- Chen, J.; Hu, H.; Wang, M.; Ge, Y.; Wang, K.; Huang, Y.; Yang, K.; He, Z.; Xu, Z.; Li, Y.R. Power Flow Control-Based Regenerative Braking Energy Utilization in AC Electrified Railways: Review and Future Trends. IEEE Trans. Intell. Transp. Syst. 2024, 25, 6345–6365. [Google Scholar] [CrossRef]








| VDC | 100 V | 120 V | 140 V | 160 V | 180 V | 200 V | 220 V | 240 V |
| DS | 0.187 | 0.27 | 0.335 | 0.38 | 0.425 | 0.47 | 0.51 | 0.547 |
| IDC | 0.813 A | 0.73 A | 0.665 A | 0.62 A | 0.575 A | 0.53 A | 0.49 A | 0.453 A |
| VDC | 260 V | 280 V | 300 V | 320 V | 340 V | 360 V | 380 V | 400 V |
| DS | 0.58 | 0.6 | 0.625 | 0.65 | 0.67 | 0.685 | 0.695 | 0.7 |
| IDC | 0.42 A | 0.4 A | 0.375 A | 0.35 A | 0.33 A | 0.315 A | 0.305 A | 0.3 A |
| Devices and Bridge Status | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| ON | OFF | OFF | OFF | OFF | ON | ON | OFF | OFF | |
| OFF | ON | ON | OFF | OFF | OFF | OFF | ON | ON | |
| OFF | OFF | OFF | ON | ON | OFF | OFF | OFF | OFF | |
| OFF | OFF | ON | ON | OFF | OFF | OFF | OFF | ON | |
| OFF | OFF | OFF | OFF | ON | ON | OFF | OFF | OFF | |
| ON | ON | OFF | OFF | OFF | OFF | ON | ON | OFF | |
| T | True | True | False | True | True | False | True | True | False |
| False | False | True | False | False | True | False | False | True |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Di Stefano, R.; Marignetti, F.; Pellini, F. Design, Simulation and Performance of a CSI Converter for Grid-Connected or Islanded Microgrids with High Step-Up Capability in PV Applications. Energies 2024, 17, 4787. https://doi.org/10.3390/en17194787
Di Stefano R, Marignetti F, Pellini F. Design, Simulation and Performance of a CSI Converter for Grid-Connected or Islanded Microgrids with High Step-Up Capability in PV Applications. Energies. 2024; 17(19):4787. https://doi.org/10.3390/en17194787
Chicago/Turabian StyleDi Stefano, Roberto, Fabrizio Marignetti, and Fabio Pellini. 2024. "Design, Simulation and Performance of a CSI Converter for Grid-Connected or Islanded Microgrids with High Step-Up Capability in PV Applications" Energies 17, no. 19: 4787. https://doi.org/10.3390/en17194787
APA StyleDi Stefano, R., Marignetti, F., & Pellini, F. (2024). Design, Simulation and Performance of a CSI Converter for Grid-Connected or Islanded Microgrids with High Step-Up Capability in PV Applications. Energies, 17(19), 4787. https://doi.org/10.3390/en17194787








