Review of Switched Reluctance Motor Converters and Torque Ripple Minimisation Techniques for Electric Vehicle Applications
Abstract
1. Introduction
- High power and torque density (/kg and /A);
- High torque to inertia ratio;
- High efficiency;
- Compact size and low weight;
- Low cost;
- Robust (electrically and mechanically) and fault tolerant;
- Wide constant torque/speed range;
- Wide temperature operating range;
- Low vibration and acoustic noise.
2. SRM Power Converters
2.1. Introduction
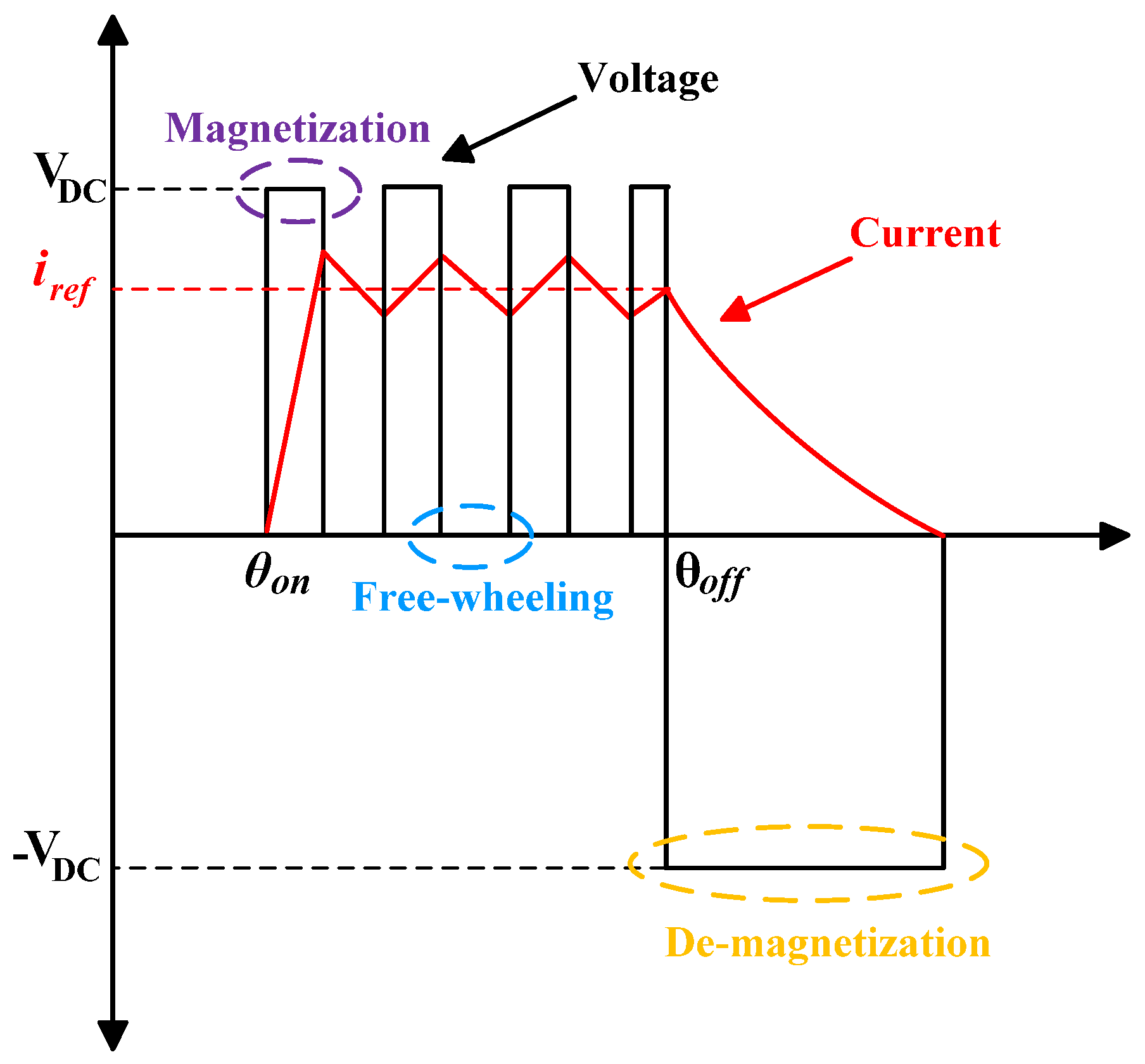
- Fast magnetisation and demagnetisation of SRM phases: The converter should facilitate fast magnetisation to quickly reach the reference current in the phase winding, thereby increasing the SRM base speed. It should also minimise demagnetisation time by rapidly extinguishing phase current to eliminate the current tail, preventing the SRM from entering the negative torque production region. These conditions can be enhanced by boosting the DC supply voltage during magnetisation and demagnetisation periods.
- Phase overlap: The converter must be able to simultaneously energise the incoming phase and de-energise the outgoing phase.
- High efficiency: To improve SRM drive efficiency, the converter should efficiently return demagnetisation energy to the supply or store it for use in energising another phase rather than dissipating it as heat in winding resistance.
- Minimum cost: An economical converter provides excellent performance with a minimal number of switching devices. Fewer switches reduces switching losses, the number of gate drive circuits, and overall converter cost.
- Fault tolerance: For critical applications, a high-reliability converter is necessary to ensure continuous SRM rotation even after a phase failure.
- Low complexity: Simple design and control algorithms are preferred for the SRM converter.
2.2. Classification of SRM Power Converters
2.3. Hard-Switching Converters
2.3.1. Bridge Converters
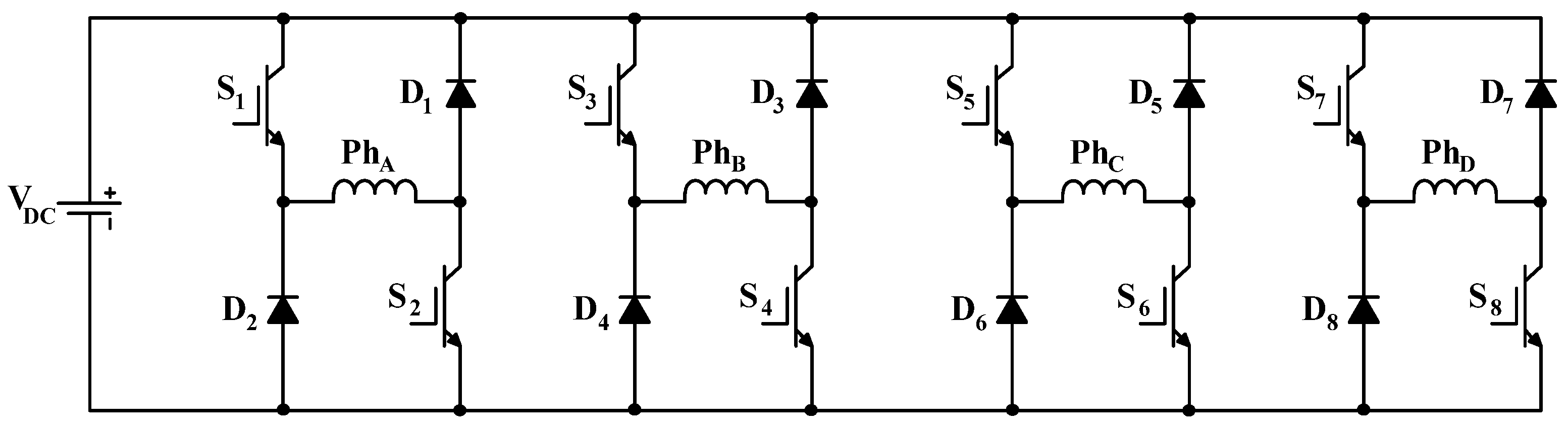
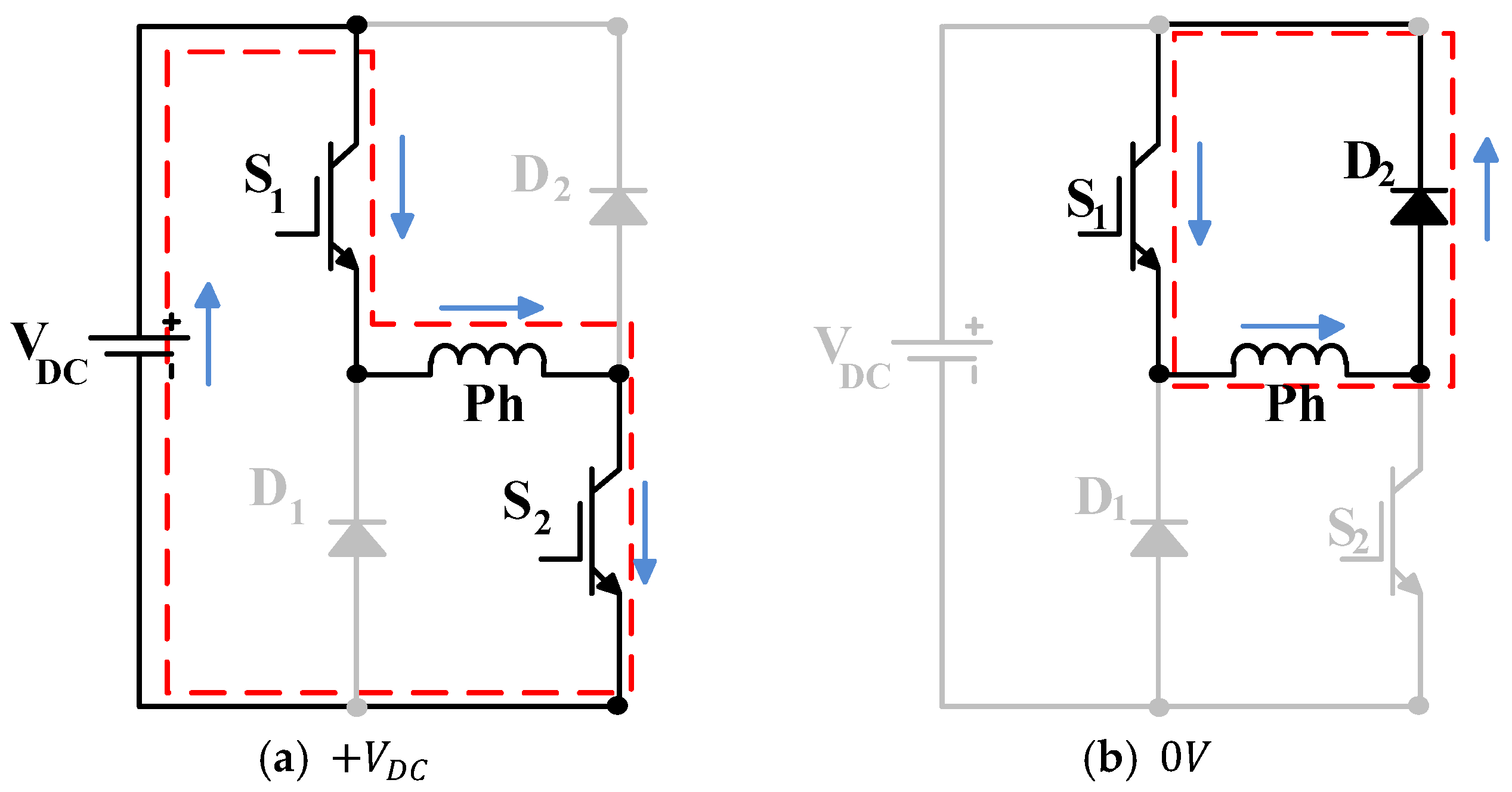
Asymmetric Half Bridge
- Independent control of each phase without overlap.
- Three available voltage levels, namely .
- Low complexity.
- High fault tolerance, with no link switch ‘shoot through’ path.
- High efficiency as stored magnetic energy in phase winding is fed back to the DClink during demagnetisation.
- Increase in semiconductor device number, as each phase requires two switches and two diodes.
- More conduction losses since two semiconductors are connected in series with the conducting phase winding.
- The relatively low magnetisation and demagnetisation voltage limits motor base speed.
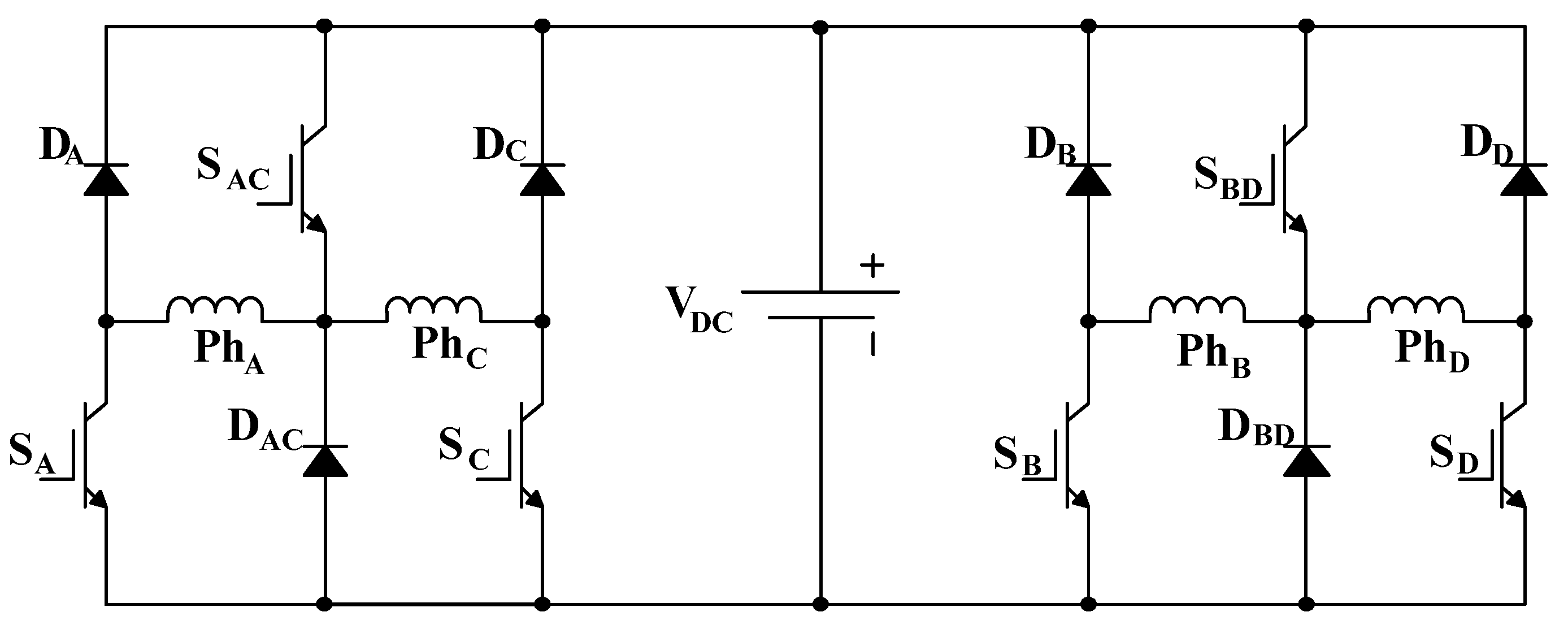
Common-Phase Converter
Common Switch Converter
2.3.2. Dissipative Converters
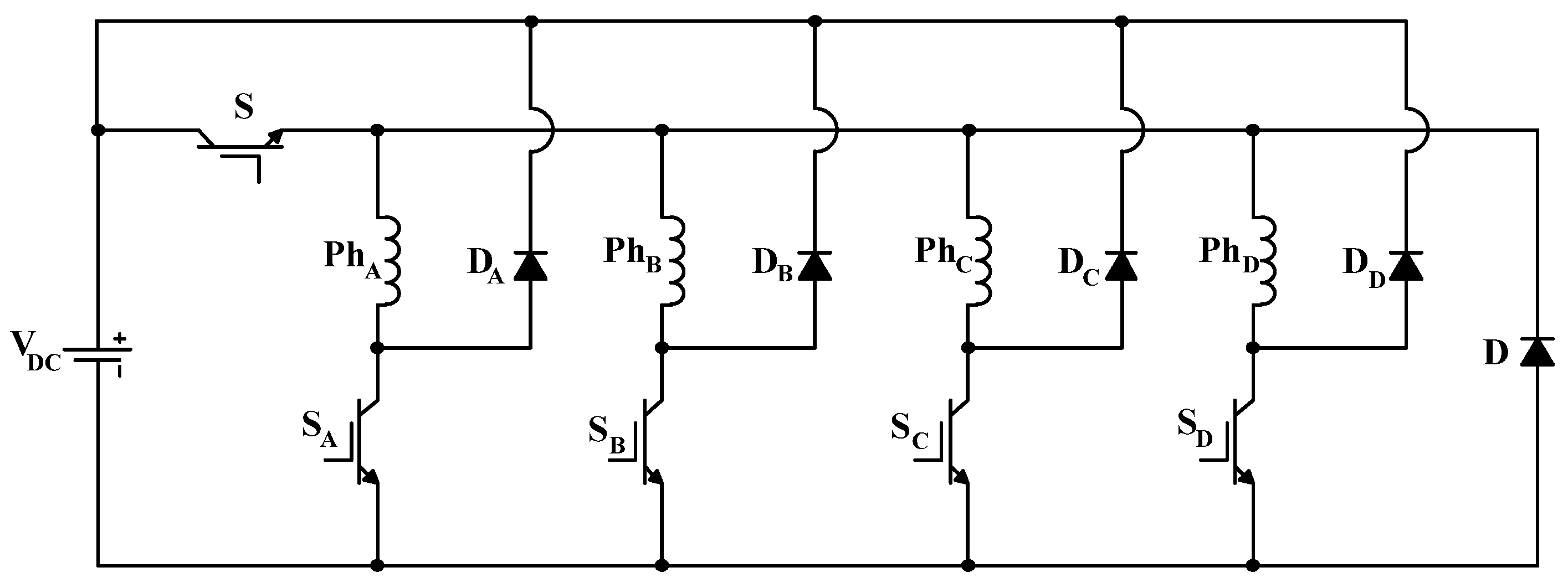
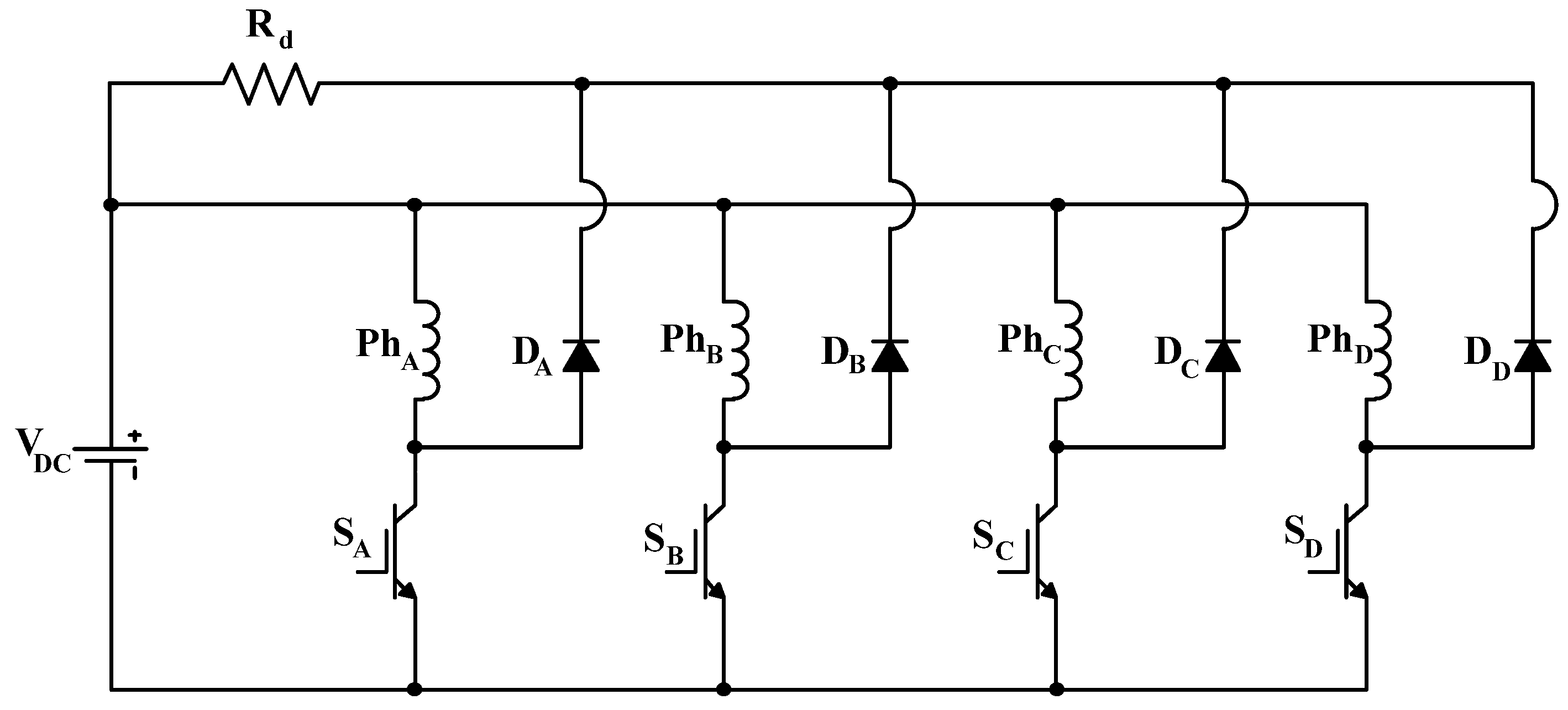
R-Dump Converter
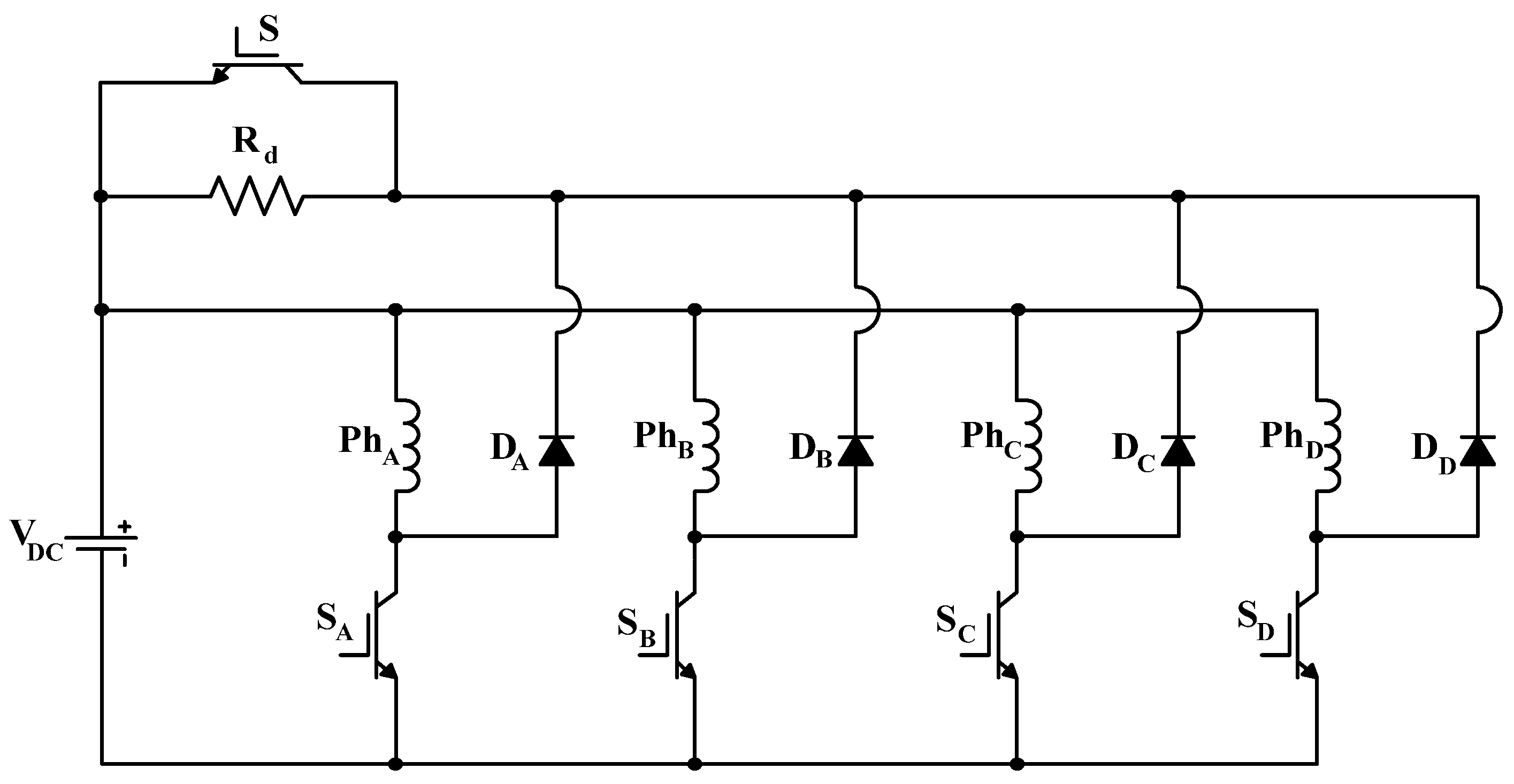
Modified R-Dump Converter
2.3.3. Magnetic Converters
2.3.4. Capacitive Converters
C-Dump Converter
Modified C-Dump Converter
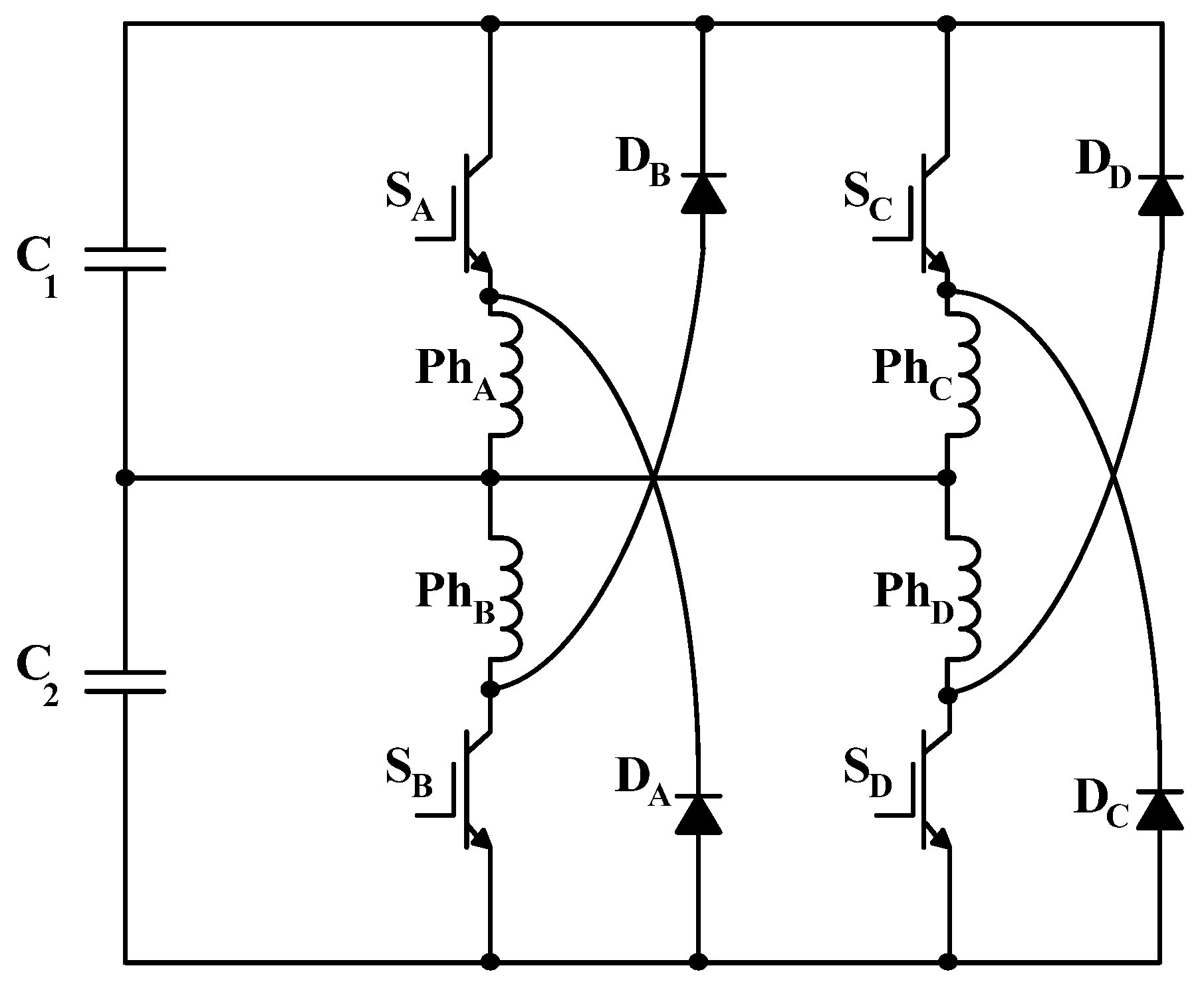
Split DC Link Converter
Passive Boost Converter
2.4. Soft-Switching and Advanced Converters
2.5. Comparing SRM Power Converters
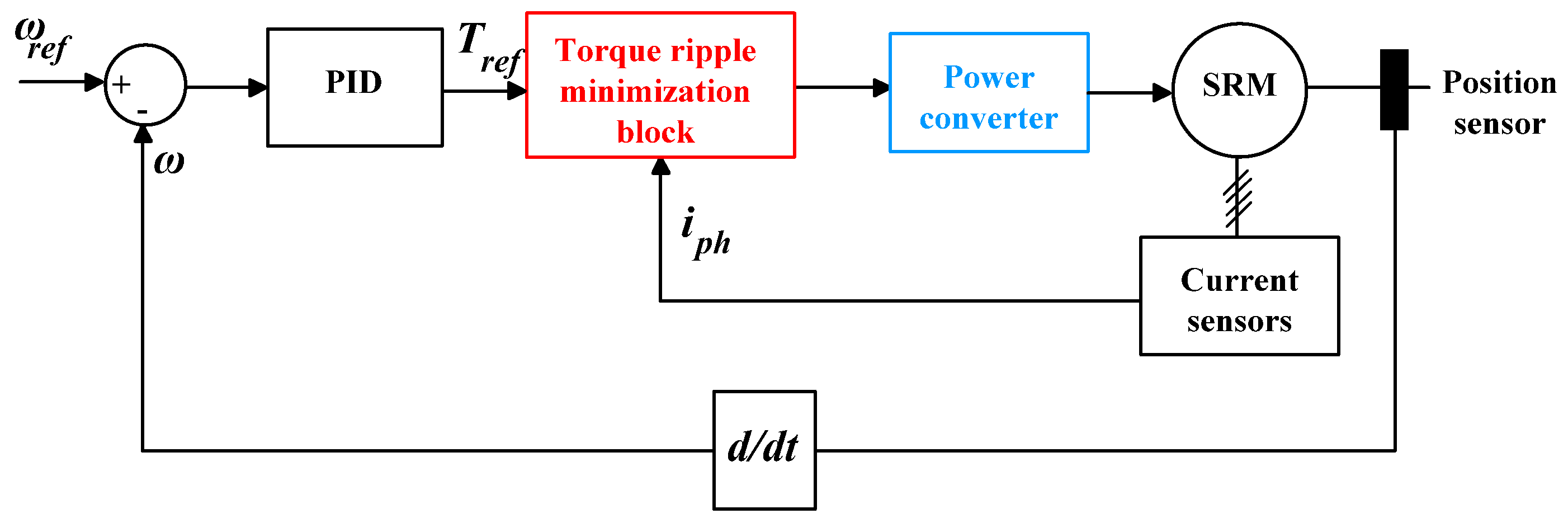
3. Torque Ripple Minimisation Approaches
3.1. Introduction to TR Approaches
3.2. Classification of Torque Ripple Minimisation Approaches
3.2.1. Machine Design Approach
3.2.2. Control Approach
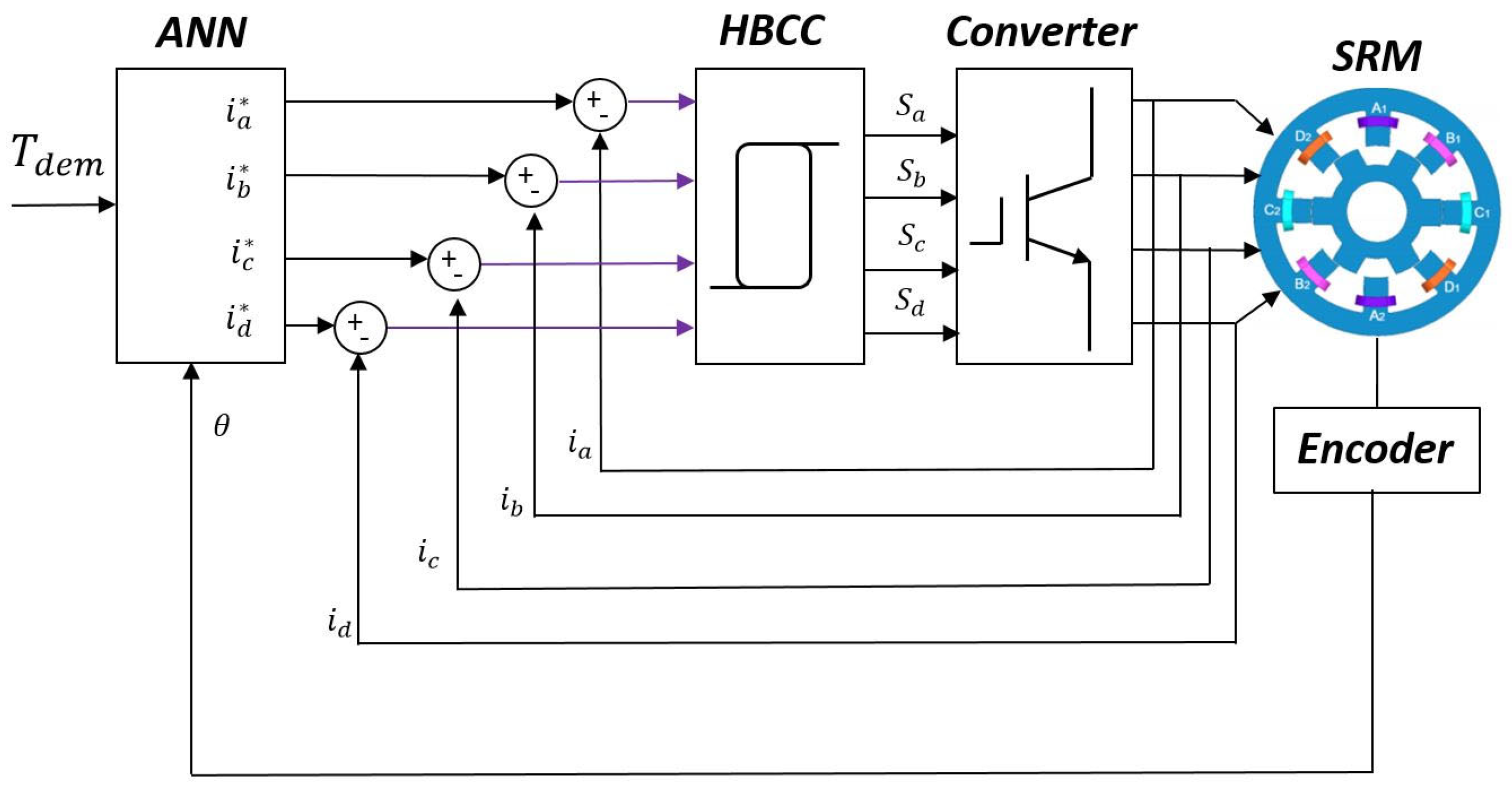
Current Profiling
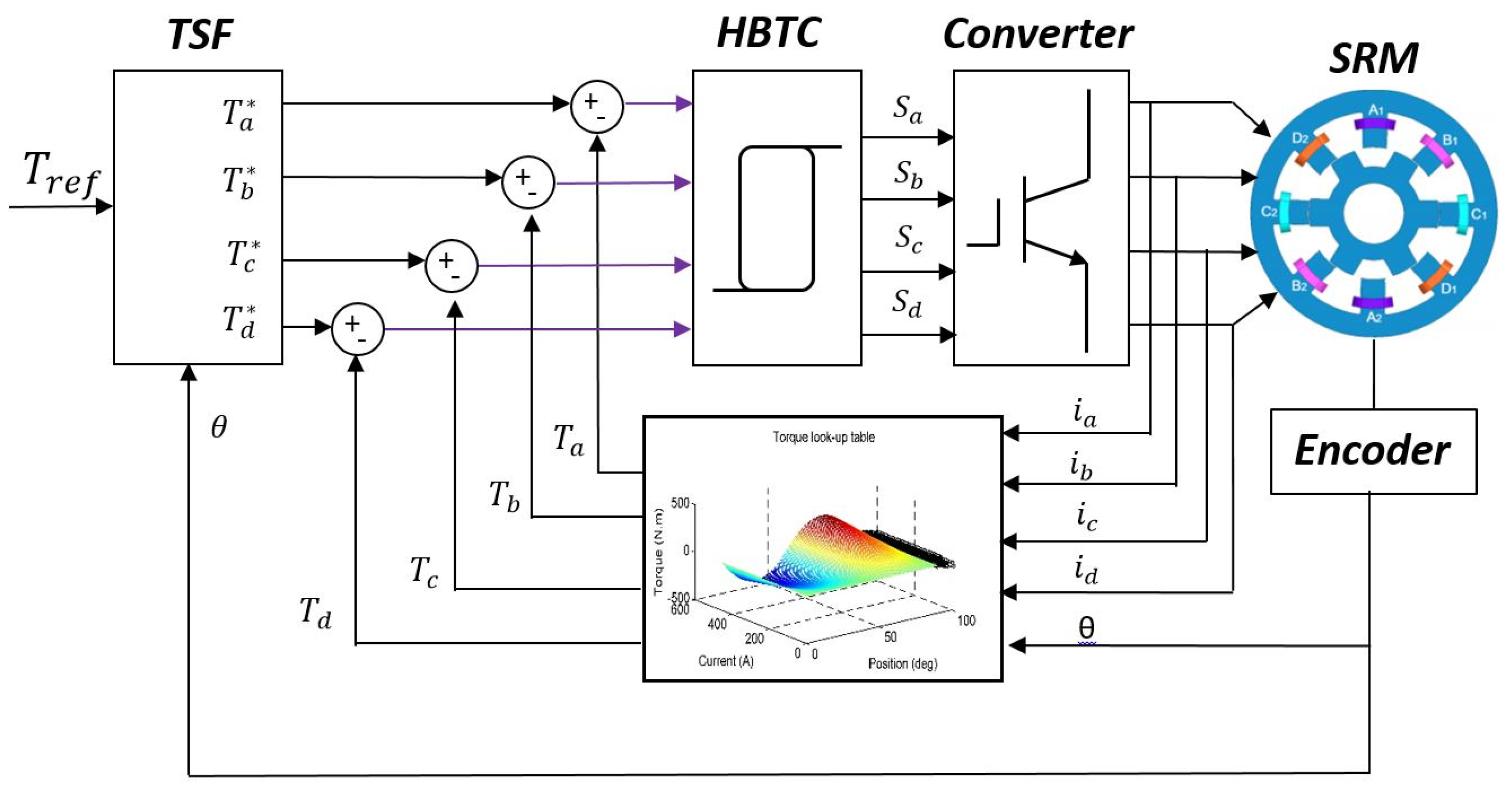
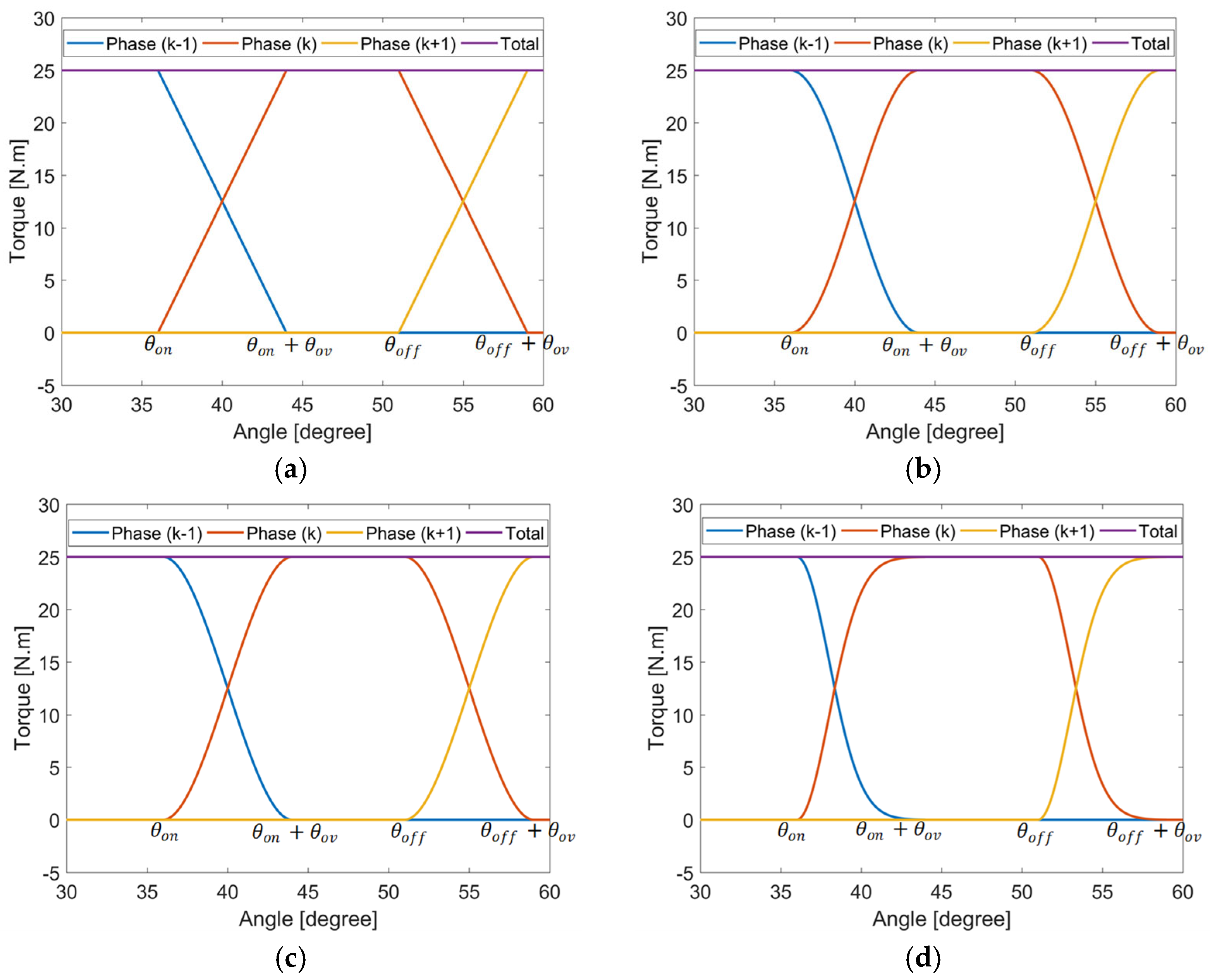
Torque Sharing Function
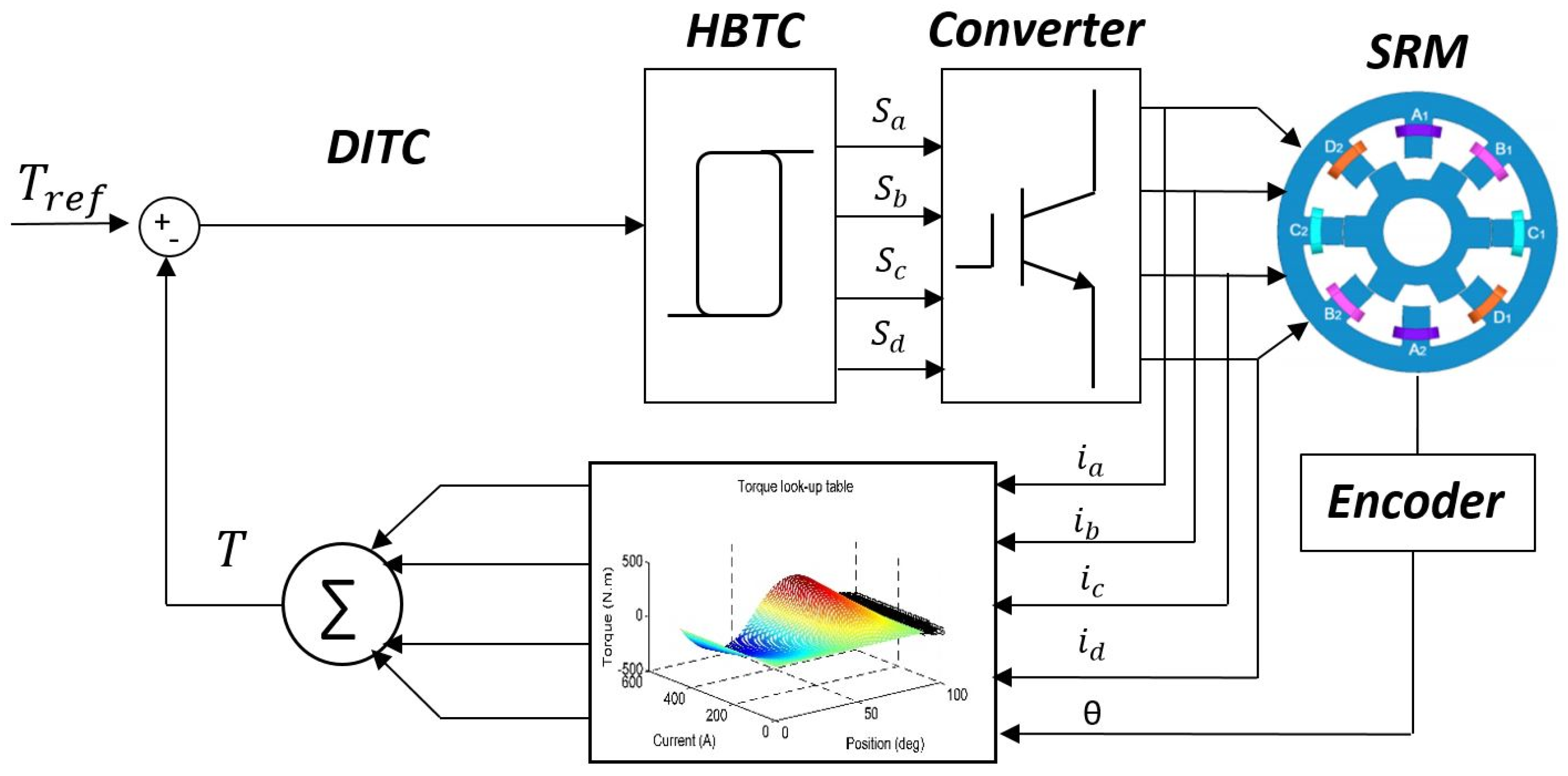
Direct Instantaneous Torque Control
3.3. Comparison of Torque Ripple Minimisation Approaches
4. Discussion and Conclusions
- Investigating the performance of SRM power converters using SiC and GaN semiconductor devices.
- Investigating and developing torque ripple reduction techniques for converter fault conditions, including open and short circuit faults, using fewer additional power switches.
- Developing control schemes to reduce torque ripple over a wide speed range.
Author Contributions
Funding
Conflicts of Interest
References
- Habib, S.; Khan, M.M.; Abbas, F.; Sang, L.; Shahid, M.U.; Tang, H. A Comprehensive Study of Implemented International Standards, Technical Challenges, Impacts and Prospects for Electric Vehicles. IEEE Access 2018, 6, 13866–13890. [Google Scholar] [CrossRef]
- Bostanci, E.; Moallem, M.; Parsapour, A.; Fahimi, B. Opportunities and Challenges of Switched Reluctance Motor Drives for Electric Propulsion: A Comparative Study. IEEE Trans. Transp. Electrif. 2017, 3, 58–75. [Google Scholar] [CrossRef]
- Ramesh, P.; Lenin, N.C. High Power Density Electrical Machines for Electric Vehicles—Comprehensive Review Based on Material Technology. IEEE Trans. Magn. 2019, 55, 0900121. [Google Scholar] [CrossRef]
- Chiba, A.; Kiyota, K. Review of research and development of switched reluctance motor for hybrid electrical vehicle. In Proceedings of the 2015 IEEE Workshop on Electrical Machines Design, Control and Diagnosis (WEMDCD), Turin, Italy, 26–27 March 2015; pp. 127–131. [Google Scholar] [CrossRef]
- Zeraoulia, M.; Benbouzid, M.E.H.; Diallo, D. Electric Motor Drive Selection Issues for HEV Propulsion Systems: A Comparative Study. IEEE Trans. Veh. Technol. 2006, 55, 1756–1764. [Google Scholar] [CrossRef]
- Bianchi, N.; Bolognani, S.; Carraro, E.; Castiello, M.; Fornasiero, E. Electric Vehicle Traction Based on Synchronous Reluctance Motors. IEEE Trans. Ind. Appl. 2016, 52, 4762–4769. [Google Scholar] [CrossRef]
- Kumar, G.; Chuang, C.; Lu, M.; Liaw, C. Development of an Electric Vehicle Synchronous Reluctance Motor Drive. IEEE Trans. Veh. Technol. 2020, 69, 5012–5024. [Google Scholar] [CrossRef]
- Rahman, K.; Fahimi, B.; Suresh, G.; Rajarathnam, A.; Ehsani, M. Advantages of switched reluctance motor applications to EV and HEV: Design and control issues. IEEE Trans. Ind. Appl. 2000, 36, 111–121. [Google Scholar] [CrossRef]
- Wang, S.; Zhan, Q.; Ma, Z.; Zhou, L. Implementation of a 50-kW four-phase switched reluctance motor drive system for hybrid electric vehicle. IEEE Trans. Magn. 2005, 41, 501–504. [Google Scholar] [CrossRef]
- Chiba, A.; Kiyota, K.; Hoshi, N.; Takemoto, M.; Ogasawara, S. Development of a Rare-Earth-Free SR Motor with High Torque Density for Hybrid Vehicles. IEEE Trans. Energy Convers. 2015, 30, 175–182. [Google Scholar] [CrossRef]
- Kiyota, K.; Kakishima, T.; Chiba, A. Comparison of Test Result and Design Stage Prediction of Switched Reluctance Motor Competitive with 60-kW Rare-Earth PM Motor. IEEE Trans. Ind. Electron. 2014, 61, 5712–5721. [Google Scholar] [CrossRef]
- Takeno, M.; Chiba, A.; Hoshi, N.; Ogasawara, S.; Takemoto, M.; Rahman, M.A. Test Results and Torque Improvement of the 50-kW Switched Reluctance Motor Designed for Hybrid Electric Vehicles. IEEE Trans. Ind. Appl. 2012, 48, 1327–1334. [Google Scholar] [CrossRef]
- Vukosavic, S.; Stefanovic, V. SRM inverter topologies: A comparative evaluation. IEEE Trans. Ind. Appl. 1991, 27, 1034–1047. [Google Scholar] [CrossRef]
- Ellabban, O.; Abu-Rub, H. Switched reluctance motor converter topologies: A review. In Proceedings of the 2014 IEEE International Conference on Industrial Technology (ICIT), Busan, Republic of Korea, 26 February–1 March 2014; pp. 840–846. [Google Scholar]
- Hu, Y.; Wang, T.; Ding, W. Performance Evaluation on a Novel Power Converter with Minimum Number of Switches for a Six-Phase Switched Reluctance Motor. IEEE Trans. Ind. Electron. 2019, 66, 1693–1702. [Google Scholar] [CrossRef]
- Pollock, C.; Williams, B.W. Power converter circuits for switched reluctance motors with the minimum number of switches. IEE Proc. B Electr. Power Appl. 1990, 137, 373–384. [Google Scholar] [CrossRef]
- Jang, D.H.; Husain, I.; Ehsani, M. Modified (n+1) switch converter for switched reluctance motor drives. In Proceedings of the PESC ‘95—Power Electronics Specialist Conference, Atlanta, GA, USA, 18–22 June 1995; Volume 2, pp. 1121–1127. [Google Scholar]
- Krishnan, R.; Materu, P.N. Analysis and design of a low cost converter for switched reluctance motor drives. IEEE Trans. Ind. Appl. 1993, 29, 320–327. [Google Scholar] [CrossRef]
- Ehsani, M.; Husain, I.; Ramani, K.R.; Galloway, J.H. Dual-decay converter for switched reluctance motor drives in low-voltage applications. IEEE Trans. Power Electron. 1993, 8, 224–230. [Google Scholar] [CrossRef]
- Miller, T.J. Converter Volt-Ampere Requirements of the Switched Reluctance Motor Drive. IEEE Trans. Ind. Appl. 1985, IA-21, 1136–1144. [Google Scholar] [CrossRef]
- Mir, S.; Husain, I.; Elbuluk, M.E. Energy-efficient C-dump converters for switched reluctance motors. IEEE Trans. Power Electron. 1997, 12, 912–921. [Google Scholar] [CrossRef]
- Hava, A.M.; Blasko, V.; Lipo, T.A. A modified C-dump converter for variable-reluctance machines. IEEE Trans. Ind. Appl. 1992, 28, 1017–1022. [Google Scholar] [CrossRef]
- Ryoo, H.-J.; Kim, W.-H.; Rim, G.-H.; Kang, W.; Park, J.-H.; Won, C.-Y. A new split source type converter for SRM drives. In Proceedings of the PESC 98 Record. 29th Annual IEEE Power Electronics Specialists Conference (Cat. No.98CH36196), Fukuoka, Japan, 22 May 1998; Volume 2, pp. 1290–1294. [Google Scholar]
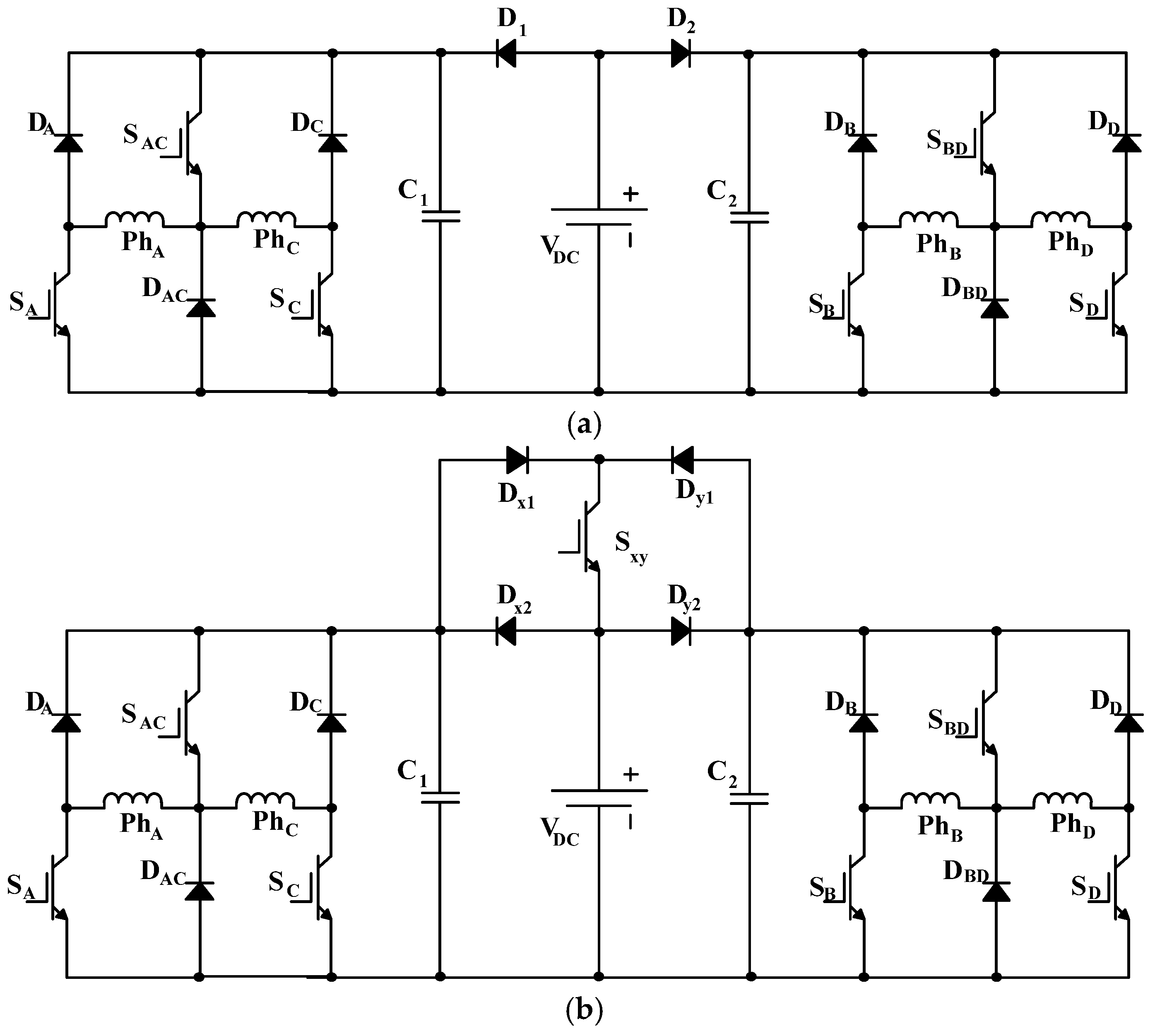
- Dessouky, Y.; Williams, B.; Fletcher, J. A novel power converter with voltage-boosting capacitors for a four-phase SRM drive. IEEE Trans. Ind. Electron. 1998, 45, 815–823. [Google Scholar] [CrossRef]
- Abdel-Aziz, A.A.; Ahmed, K.H.; Wang, S.; Massoud, A.M.; Williams, B.W. A Neutral-Point Diode-Clamped Converter with Inherent Voltage-Boosting for a Four-Phase SRM Drive. IEEE Trans. Ind. Electron. 2020, 67, 5313–5324. [Google Scholar] [CrossRef]
- Cabezuelo, D.; Andreu, J.; Kortabarria, I.; Ibarra, E.; Garate, I. SRM converter topologies for EV application: State of the technology. In Proceedings of the 2017 IEEE 26th International Symposium on Industrial Electronics (ISIE), Edinburgh, UK, 19–21 June 2017; pp. 861–866. [Google Scholar]
- Xu, S.; Chen, H.; Yang, J.; Dong, F. Performance Evaluation and Reliability Enhancement of Switched Reluctance Drive System by a Novel Integrated Power Converter. IEEE Trans. Power Electron. 2019, 34, 11090–11102. [Google Scholar] [CrossRef]
- Deng, X.; Mecrow, B.C.; Wu, H.; Martin, R.; Gai, Y. Cost-Effective and High-Efficiency Variable-Speed Switched Reluctance Drives with Ring-Connected Winding Configuration. IEEE Trans. Energy Convers. 2019, 34, 120–129. [Google Scholar] [CrossRef]
- Sun, Q.; Wu, J.; Gan, C.; Guo, J. Modular Full-Bridge Converter for Three-Phase Switched Reluctance Motors with Integrated Fault-Tolerance Capability. IEEE Trans. Power Electron. 2019, 34, 2622–2634. [Google Scholar] [CrossRef]
- Chen, Q.; Xu, D.; Xu, L.; Wang, J.; Lin, Z.; Zhu, X. Fault-Tolerant Operation of a Novel Dual-Channel Switched Reluctance Motor Using Two 3-Phase Standard Inverters. IEEE Trans. Appl. Supercond. 2018, 28, 1–5. [Google Scholar] [CrossRef]
- Song, S.; Xia, Z.; Zhang, Z.; Liu, W. Control Performance Analysis and Improvement of a Modular Power Converter for Three-Phase SRM with Y-Connected Windings and Neutral Line. IEEE Trans. Ind. Electron. 2016, 63, 6020–6030. [Google Scholar] [CrossRef]
- Martin, R.; Widmer, J.D.; Mecrow, B.C.; Kimiabeigi, M.; Mebarki, A.; Brown, N.L. Electromagnetic Considerations for a Six-Phase Switched Reluctance Motor Driven by a Three-Phase Inverter. IEEE Trans. Ind. Appl. 2016, 52, 3783–3791. [Google Scholar] [CrossRef]
- Elmutalab, M.A.; Elrayyah, A.; Husain, T.; Sozer, Y. Extending the Speed Range of a Switched Reluctance Motor Using a Fast-Demagnetizing Technique. IEEE Trans. Ind. Appl. 2018, 54, 3294–3304. [Google Scholar] [CrossRef]
- Jung, C. Power Up with 800-V Systems: The benefits of upgrading voltage power for battery-electric passenger vehicles. IEEE Electrif. Mag. 2017, 5, 53–58. [Google Scholar] [CrossRef]
- Gaafar, M.A.; Abdelmaksoud, A.; Orabi, M.; Chen, H.; Dardeer, M. Performance Investigation of Switched Reluctance Motor Driven by Quasi-Z-Source Integrated Multiport Converter with Different Switching Algorithms. Sustainability 2021, 13, 9517. [Google Scholar] [CrossRef]
- Pires, V.F.; Cordeiro, A.; Foito, D.; Pires, A.J. Fault-Tolerant Multilevel Converter to Feed a Switched Reluctance Machine. Machines 2022, 10, 35. [Google Scholar] [CrossRef]
- Borecki, J.; Orlik, B. Novel, multilevel converter topology for fault-tolerant operation of switched reluctance machines. In Proceedings of the 2017 11th IEEE International Conference on Compatibility, Power Electronics and Power Engineering (CPE-POWERENG), Cadiz, Spain, 4–6 April 2017; pp. 375–380. [Google Scholar]
- Peng, F.; Ye, J.; Emadi, A. An asymmetric three-level neutral point diode clamped converter for switched reluctance motor drives. IEEE Trans. Power Electron. 2017, 32, 8618–8631. [Google Scholar] [CrossRef]
- Gan, C.; Sun, Q.; Wu, J.; Kong, W.; Shi, C.; Hu, Y. MMC-based SRM drives with decentralized battery energy storage system for hybrid electric vehicles. IEEE Trans. Power Electron. 2019, 34, 2608–2621. [Google Scholar] [CrossRef]
- Yuan, K.; Ma, M.; Wang, Z.; Wang, R.; Yang, S. A new five-level power converter for switched reluctance motor drive. In Proceedings of the Power Electronics and Application Conference and Exposition (PEAC), Shenzhen, China, 4–7 November 2018; pp. 1–6. [Google Scholar]
- Han, G.; Chen, H. Improved Power Converter of SRM Drive for Electric Vehicle with Self-Balanced Capacitor Voltages. IEEE Trans. Transp. Electrif. 2021, 7, 1339–1348. [Google Scholar] [CrossRef]
- Cai, J.; Zhao, X. An On-Board Charger Integrated Power Converter for EV Switched Reluctance Motor Drives. IEEE Trans. Ind. Electron. 2020, 68, 3683–3692. [Google Scholar] [CrossRef]
- Chithrabhanu, A.; Vasudevan, K. Analysis and Design Considerations of a Buck-Boost Energy Recovery-Based Power Converter for SRM Drive. IEEE J. Emerg. Sel. Top. Power Electron. 2023, 11, 886–900. [Google Scholar] [CrossRef]
- Rana, K.; Teja, A.V.R. Fast Discharging (N+1) Switch Converter with Regenerative Flyback Operation for N-Phase SRM Drives. IEEE Trans. Power Electron. 2022, 37, 8359–8368. [Google Scholar] [CrossRef]
- Guan, M.; Liu, C.; Han, S.; Sun, X. Analysis of Midpoint Current Characteristics for Novel Six-Phase N+2 Power Converter in Different Working Condition. IEEE Access 2020, 8, 105104–105117. [Google Scholar] [CrossRef]
- Dong, J.; Jiang, J.W.; Howey, B.; Li, H.; Bilgin, B.; Callegaro, A.D.; Emadi, A. Hybrid acoustic noise analysis approach of conventional and mutually coupled switched reluctance motors. IEEE Trans. Energy Convers. 2017, 32, 1042–1051. [Google Scholar] [CrossRef]
- Almirante, I.; Lorenzani, E. Simple Strategy for Torque Ripple Minimization in Switched Reluctance Motor Drives. Energies 2023, 16, 6885. [Google Scholar] [CrossRef]
- Rana, A.K.; Teja, A.V.R. A Mathematical Torque Ripple Minimization Technique Based on a Nonlinear Modulating Factor for Switched Reluctance Motor Drives. IEEE Trans. Ind. Electron. 2022, 69, 1356–1366. [Google Scholar] [CrossRef]
- Gan, C.; Wu, J.; Sun, Q.; Kong, W.; Li, H.; Hu, Y. A review on machine topologies and control techniques for low-noise switched reluctance motors in electric vehicle applications. IEEE Access 2018, 6, 31430–31443. [Google Scholar] [CrossRef]
- Hamouda, M.; Al-Amyal, F.; Odinaev, I.; Ibrahim, M.N.; Számel, L. A Novel Universal Torque Control of Switched Reluctance Motors for Electric Vehicles. Mathematics 2022, 10, 3833. [Google Scholar] [CrossRef]
- Deng, X.; Mecrow, B.; Wu, H.; Martin, R. Design and development of low torque ripple variable-speed drive system with six-phase switched reluctance motors. IEEE Trans. Energy Convers. 2017, 33, 420–429. [Google Scholar] [CrossRef]
- Jiang, J.; Bilgin, B.; Emadi, A. Three-phase 24/16 switched reluctance machine for a hybrid electric powertrain. IEEE Trans. Transp. Electrif. 2017, 3, 76–85. [Google Scholar] [CrossRef]
- Desai, P.C.; Krishnamurthy, M.; Schofield, N.; Emadi, A. Novel switched reluctance machine configuration with higher number of rotor poles than stator poles: Concept to implementation. IEEE Trans. Ind. Electron. 2010, 57, 649–659. [Google Scholar] [CrossRef]
- Bilgin, B.; Emadi, A.; Krishnamurthy, M. Design considerations for switched reluctance machines with a higher number of rotor poles. IEEE Trans. Ind. Electron. 2012, 59, 3745–3756. [Google Scholar] [CrossRef]
- Bilgin, B.; Emadi, A.; Krishnamurthy, M. Comprehensive evaluation of the dynamic performance of a 6/10 SRM for traction application in PHEVs. IEEE Trans. Ind. Electron. 2013, 60, 2564–2575. [Google Scholar] [CrossRef]
- Lin, J.; Schofield, N.; Emadi, A. External-rotor 6-10 switched reluctance motor for an electric bicycle. IEEE Trans. Transp. Electrif. 2015, 1, 348–356. [Google Scholar] [CrossRef]
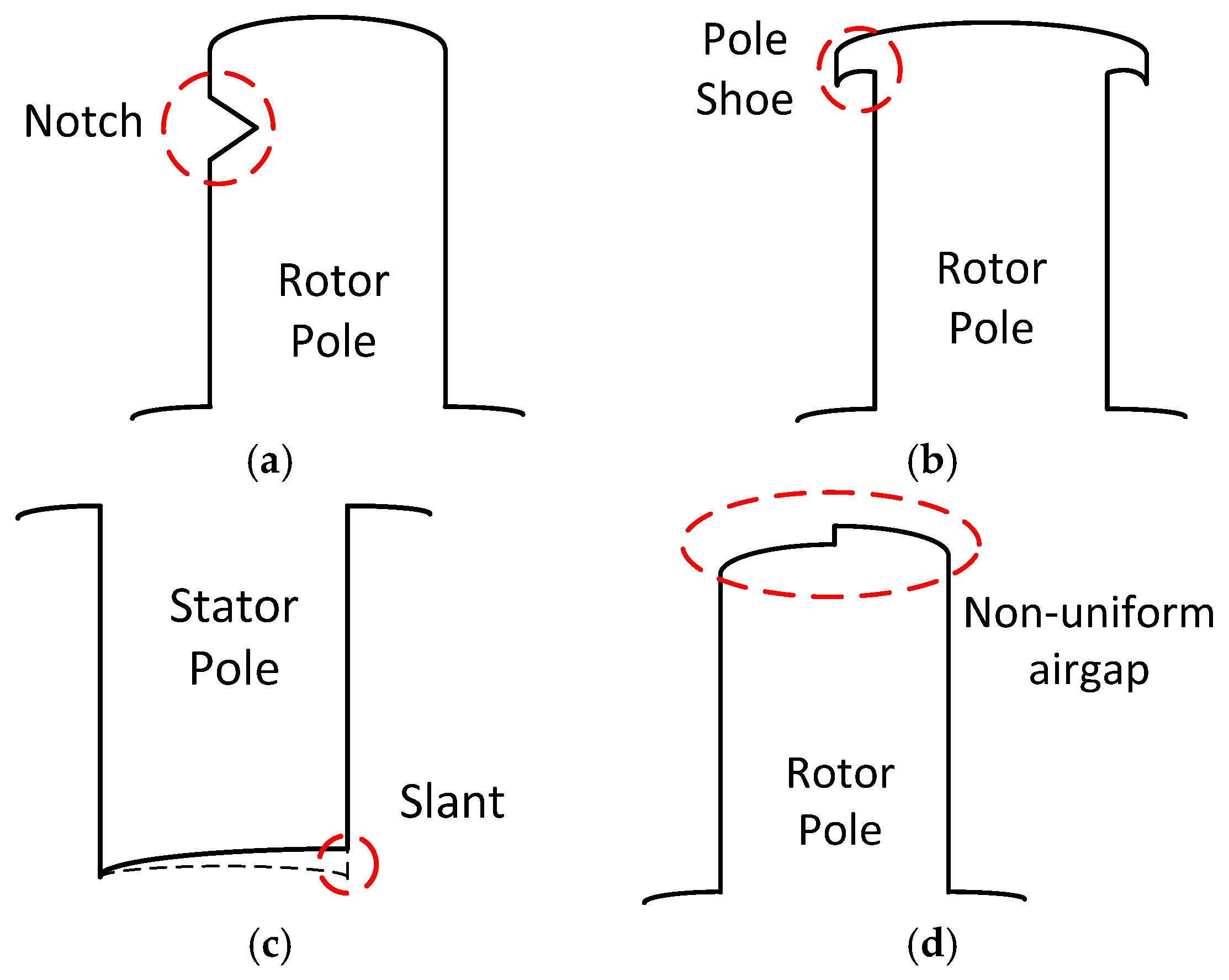
- Lee, J.W.; Kim, H.S.; Kwon, B.I.; Kim, B.T. New rotor shape design for minimum torque ripple of SRM using FEM. IEEE Trans. Magn. 2004, 40, 754–757. [Google Scholar] [CrossRef]
- Li, G.; Ojeda, J.; Hlioui, S.; Hoang, E.; Lecrivain, M.; Gabsi, M. Modification in rotor pole geometry of mutually coupled switched reluctance machine for torque ripple mitigating. IEEE Trans. Magn. 2011, 48, 2025–2034. [Google Scholar] [CrossRef]
- Choi, Y.K.; Yoon, H.S.; Koh, C.S. Pole-shape optimization of a switched-reluctance motor for torque ripple reduction. IEEE Trans. Magn. 2007, 43, 1797–1800. [Google Scholar] [CrossRef]
- Sheth, N.; Rajagopal, K. Torque profiles of a switched reluctance motor having special pole face shapes and asymmetric stator poles. IEEE Trans. Magn. 2004, 40, 2035–2037. [Google Scholar] [CrossRef]
- Lee, D.H.; Pham, T.H.; Ahn, J.W. Design and operation characteristics of four-two pole high-speed SRM for torque ripple reduction. IEEE Trans. Ind. Electron. 2013, 60, 3637–3643. [Google Scholar] [CrossRef]
- Sheth, N.; Rajagopal, K. Optimum pole arcs for a switched reluctance motor for higher torque with reduced ripple. IEEE Trans. Magn. 2003, 39, 3214–3216. [Google Scholar] [CrossRef]
- Jing, B.; Dang, X.; Liu, Z.; Ji, J. Torque Ripple Suppression of Switched Reluctance Motor with Reference Torque Online Correction. Machines 2023, 11, 179. [Google Scholar] [CrossRef]
- He, X.; Yao, Y. An Improved Hybrid Control Scheme of a Switched Reluctance Motor for Torque Ripple Reduction. Appl. Sci. 2022, 12, 12283. [Google Scholar] [CrossRef]
- Dúbravka, P.; Rafajdus, P.; Makyš, P.; Szabó, L. Control of switched reluctance motor by current profiling under normal and open phase operating condition. IET Electr. Power Appl. 2017, 11, 548–556. [Google Scholar] [CrossRef]
- Schulz, S.; Rahman, K. High-performance digital PI current regulator for EV switched reluctance motor drives. IEEE Trans. Ind. Appl. 2003, 39, 1118–1126. [Google Scholar] [CrossRef]
- Gobbi, R.; Ramar, K. Optimization techniques for a hysteresis current controller to minimize torque ripple in switched reluctance motors. IET Electr. Power Appl. 2009, 3, 453–460. [Google Scholar] [CrossRef]
- Lin, Z.; Reay, D.; Williams, B.; He, X. High-performance current control for switched reluctance motors based on on-line estimated parameters. IET Electr. Power Appl. 2010, 4, 67–74. [Google Scholar] [CrossRef]
- Mikail, R.; Husain, I.; Sozer, Y.; Islam, M.S.; Sebastian, T. A fixed switching frequency predictive current control method for switched reluctance machines. IEEE Trans. Ind. Appl. 2014, 50, 3717–3726. [Google Scholar] [CrossRef]
- Ye, J.; Malysz, P.; Emadi, A. A fixed-switching-frequency integral sliding mode current controller for switched reluctance motor drives. IEEE J. Emerg. Sel. Top. Power Electron. 2015, 3, 381–394. [Google Scholar] [CrossRef]
- Li, X.; Shamsi, P. Model predictive current control of switched reluctance motors with inductance auto-calibration. IEEE Trans. Ind. Electron. 2016, 63, 3934–3941. [Google Scholar] [CrossRef]
- Peng, F.; Ye, J.; Emadi, A. A digital PWM current controller for switched reluctance motor drives. IEEE Trans. Power Electron. 2016, 31, 7087–7098. [Google Scholar]
- Zhang, X.; Yang, Q.; Ma, M.; Lin, Z.; Yang, S. A switched reluctance motor torque ripple reduction strategy with deadbeat current control and active thermal management. IEEE Trans. Veh. Technol. 2020, 69, 317–327. [Google Scholar] [CrossRef]
- Mikail, R.; Husain, I.; Sozer, Y.; Islam, M.; Sebastian, T. Torque-ripple minimization of switched reluctance machines using current profiling. IEEE Trans. Ind. Appl. 2013, 49, 1258–1267. [Google Scholar] [CrossRef]
- Mikail, R.; Husain, I.; Islam, M.; Sozer, Y.; Sebastian, T. Four quadrant torque ripple minimization of switched reluctance machine through current profiling with mitigation of rotor eccentricity problem and sensor errors. IEEE Trans. Ind. Appl. 2015, 51, 2079–2104. [Google Scholar] [CrossRef]
- Chapman, P.; Sudhoff, S. Design and precise realization of optimized current waveforms for an 8/6 switched reluctance drive. IEEE Trans. Power Electron. 2002, 17, 76–83. [Google Scholar] [CrossRef]
- Henriques, L.; Branco, P.C.; Rolim, L.; Suemitsu, W. Proposition of an offline learning current modulation for torque-ripple reduction in switched reluctance motors: Design and experimental evaluation. IEEE Trans. Ind. Electron. 2002, 49, 665–676. [Google Scholar] [CrossRef]
- Lin, Z.; Reay, D.; Williams, B.; He, X. Torque ripple reduction in switched reluctance motor drives using B-spline neural networks. IEEE Trans. Ind. Appl. 2006, 42, 1445–1453. [Google Scholar] [CrossRef]
- Sahoo, S.; Panda, S.; Xu, J. Iterative learning-based high-performance current controller for switched reluctance motors. IEEE Trans. Energy Convers. 2004, 19, 491–498. [Google Scholar] [CrossRef]
- Sahoo, S.; Panda, S.; Xu, J.-X. Indirect torque control of switched reluctance motors using iterative learning control. IEEE Trans. Power Electron. 2005, 20, 200–208. [Google Scholar] [CrossRef]
- Inderka, R.; De Doncker, R. High-dynamic direct average torque control for switched reluctance drives. IEEE Trans. Ind. Appl. 2003, 39, 1040–1045. [Google Scholar] [CrossRef]
- Cheng, H.; Chen, H.; Yang, Z. Average torque control of switched reluctance machine drives for electric vehicles. IET Electr. Power Appl. 2015, 9, 459–468. [Google Scholar] [CrossRef]
- Wong, K.; Cheng, K.; Ho, S. On-line instantaneous torque control of a switched reluctance motor based on co-energy control. IET Electr. Power Appl. 2009, 3, 257–264. [Google Scholar] [CrossRef]
- Wong, K.; Cheng, K.; Ho, S. Four-quadrant instantaneous torque control of switched reluctance machine at low speed based on co-energy control. IET Electr. Power Appl. 2009, 3, 431–444. [Google Scholar] [CrossRef]
- Cheok, A.; Fukuda, Y. A new torque and flux control method for switched reluctance motor drives. IEEE Trans. Power Electron. 2002, 17, 543–557. [Google Scholar] [CrossRef]
- Ai-de, X.; Xianchao, Z.; Kunlun, H.; Yuzhao, C. Torque ripple reduction of SRM using optimized voltage vector in DTC. IET Electr. Syst. Transp. 2018, 8, 35–43. [Google Scholar] [CrossRef]
- Zhang, W.; Xu, A.; Han, L.; Wang, S. Minimizing torque ripple of SRM by applying DB-DTFC. IET Electr. Power Appl. 2019, 13, 1883–1890. [Google Scholar] [CrossRef]
- Reddy, P.; Ronaki, D.; Perumal, P. Efficiency improvement and torque ripple minimization of four-phase switched reluctance motor drive using new direct torque control strategy. IET Electr. Power Appl. 2020, 14, 52–61. [Google Scholar] [CrossRef]
- Yan, N.; Cao, X.; Deng, Z. Direct torque control for switched reluctance motor to obtain high torque–ampere ratio. IEEE Trans. Ind. Electron. 2018, 66, 5144–5152. [Google Scholar] [CrossRef]
- Xu, A.; Shang, C.; Chen, J.; Zhu, J.; Han, L. A new control method based on DTC and MPC to reduce torque ripple in SRM. IEEE Access 2019, 7, 68584–68593. [Google Scholar] [CrossRef]
- Ye, J.; Bilgin, B.; Emadi, A. An Extended-Speed Low-Ripple Torque Control of Switched Reluctance Motor Drives. IEEE Trans. Power Electron. 2015, 30, 1457–1470. [Google Scholar] [CrossRef]
- Ye, J.; Bilgin, B.; Emadi, A. An Offline Torque Sharing Function for Torque Ripple Reduction in Switched Reluctance Motor Drives. IEEE Trans. Power Electron. 2015, 30, 726–735. [Google Scholar] [CrossRef]
- Li, H.; Bilgin, B.; Emadi, A. An Improved Torque Sharing Function for Torque Ripple Reduction in Switched Reluctance Machines. IEEE Trans. Power Electron. 2019, 34, 1635–1644. [Google Scholar] [CrossRef]
- Cai, H.; Wang, H.; Li, M.; Shen, S.; Feng, Y.; Zheng, J. Torque Ripple Reduction for Switched Reluctance Motor with Optimized PWM Control Strategy. Energies 2018, 11, 3215. [Google Scholar] [CrossRef]
- Huang, L.; Zhu, Z.Q.; Feng, J.; Guo, S.; Li, Y.; Shi, J.X. Novel Current Profile of Switched Reluctance Machines for Torque Density Enhancement in Low-Speed Applications. IEEE Trans. Ind. Electron. 2020, 67, 9623–9634. [Google Scholar] [CrossRef]
- Han, G.; Zhu, H.; Zhang, L.; Hong, J.; Zhu, B. Model-Free Current Predictive Control Method for Switched Reluctance Motors. IEEE Trans. Ind. Electron. 2024, 1–10. [Google Scholar] [CrossRef]
- Hagan, M.T.; Demuth, H.B.; Beale, M. Neural Networks Design; Oklahoma State University: Stillwater, OK, USA, 2014. [Google Scholar]
- Beale, H.M.; Demuth, H. Neural Networks Toolbox User’s Guide; Mathworks: Portola Valley, CA, USA, 1992. [Google Scholar]
- Reay, D.; Green, T.; Williams, B. Application of associative memory neural networks to the control of a switched reluctance motor. Proc. IECON 1993, 1, 200–206. [Google Scholar]
- Alharkan, H. Torque Ripple Minimization of Variable Reluctance Motor Using Reinforcement Dual NNs Learning Architecture. Energies 2023, 16, 4839. [Google Scholar] [CrossRef]
- Song, S.; Huang, S.; Zhao, Y.; Zhao, X.; Duan, X.; Ma, R.; Liu, W. Torque Ripple Reduction of Switched Reluctance Machine with Torque Distribution and Online Correction. IEEE Trans. Ind. Electron. 2023, 70, 8842–8852. [Google Scholar] [CrossRef]
- Ling, X.; Zhou, C.; Yang, L.; Zhang, J. Torque Ripple Suppression Method of Switched Reluctance Motor Based on an Improved Torque Distribution Function. Electronics 2022, 11, 1552. [Google Scholar] [CrossRef]
- Xia, Z.; Bilgin, B.; Nalakath, S.; Emadi, A. A New Torque Sharing Function Method for Switched Reluctance Machines with Lower Current Tracking Error. IEEE Trans. Ind. Electron. 2021, 68, 10612–10622. [Google Scholar] [CrossRef]
- Jing, B.; Dang, X.; Liu, Z.; Long, S. Torque Ripple Suppression of Switched Reluctance Motor Based on Fuzzy Indirect Instant Torque Control. IEEE Access 2022, 10, 75472–75481. [Google Scholar] [CrossRef]
- Xue, X.D.; Cheng, K.W.E.; Ho, S.L. Optimization and Evaluation of Torque-Sharing Functions for Torque Ripple Minimization in Switched Reluctance Motor Drives. IEEE Trans. Power Electron. 2009, 24, 2076–2090. [Google Scholar] [CrossRef]
- Vujičić, V.P. Minimization of Torque Ripple and Copper Losses in Switched Reluctance Drive. IEEE Trans. Power Electron. 2012, 27, 388–399. [Google Scholar] [CrossRef]
- Lee, D.-H.; Liang, J.; Lee, Z.-G.; Ahn, J.-W. A Simple Nonlinear Logical Torque Sharing Function for Low-Torque Ripple SR Drive. IEEE Trans. Ind. Electron. 2009, 56, 3021–3028. [Google Scholar] [CrossRef]
- Gan, C.; Wu, J.; Sun, Q.; Yang, S.; Hu, Y.; Jin, L. Low-cost direct instantaneous torque control for switched reluctance motors with bus current detection under soft-chopping mode. IET Power Electron. 2016, 9, 482–490. [Google Scholar] [CrossRef]
- Sun, Q.; Wu, J.; Gan, C.; Hu, Y.; Si, J. OCTSF for torque ripple minimization in SRMs. IET Power Electron. 2016, 9, 2741–2750. [Google Scholar] [CrossRef]
- Al-Amyal, F.; Számel, L. Research on Novel Hybrid Torque Sharing Function for Switched Reluctance Motors. IEEE Access 2022, 10, 91306–91315. [Google Scholar] [CrossRef]
- Ge, L.; Fan, Z.; Huang, J.; Cheng, Q.; Zhao, D.; Song, S.; De Doncker, R.W. Model Predictive Control of Switched Reluctance Machines with Online Torque Sharing Function Based on Optimal Flux-linkage Curve. IEEE Trans. Transp. Electrif. 2023. [Google Scholar] [CrossRef]
- Feng, L.; Sun, X.; Yang, Z.; Diao, K. Optimal Torque Sharing Function Control for Switched Reluctance Motors Based on Active Disturbance Rejection Controller. IEEE/ASME Trans. Mechatron. 2023, 28, 2600–2608. [Google Scholar] [CrossRef]
- Inderka, R.; De Doncker, R. DITC-direct instantaneous torque control of switched reluctance drives. IEEE Trans. Ind. Appl. 2003, 39, 1046–1051. [Google Scholar] [CrossRef]
- Sun, X.; Wu, J.; Lei, G.; Guo, Y.; Zhu, J. Torque Ripple Reduction of SRM Drive Using Improved Direct Torque Control with Sliding Mode Controller and Observer. IEEE Trans. Ind. Electron. 2021, 68, 9334–9345. [Google Scholar] [CrossRef]
- Ronanki, D.; Pittam, K.R.; Dekka, A.; Perumal, P.; Beig, A.R. Phase Current Reconstruction Method with an Improved Direct Torque Control of SRM Drive for Electric Transportation Applications. IEEE Trans. Ind. Appl. 2022, 58, 7648–7657. [Google Scholar] [CrossRef]
- Hu, Y.; Gu, C.; Zhang, Z.; Kang, Z.; Li, Y. Torque Ripple Minimization of Six-Phase Switched Reluctance Motor Based on Enhanced Direct Instantaneous Torque Control. IEEE Trans. Transp. Electrif. 2023. [Google Scholar] [CrossRef]
- Mohanraj, D.; Gopalakrishnan, J.; Chokkalingam, B.; Ojo, J.O. An Enhanced Model Predictive Direct Torque Control of SRM Drive Based on a Novel Modified Switching Strategy for Low Torque Ripple. IEEE J. Emerg. Sel. Top. Power Electron. 2024, 12, 2203–2213. [Google Scholar] [CrossRef]
- Fuengwarodsakul, N.H.; Menne, M.; Inderka, R.B.; De Doncker, R.W. High-dynamic four-quadrant switched reluctance drive based on DITC. IEEE Trans. Ind. Appl. 2005, 41, 1232–1242. [Google Scholar] [CrossRef]
- Brauer, H.J.; Hennen, M.D.; De Doncker, R.W. Control for Polyphase Switched Reluctance Machines to Minimize Torque Ripple and Decrease Ohmic Machine Losses. IEEE Trans. Power Electron. 2012, 27, 370–378. [Google Scholar] [CrossRef]
- Liang, J.; Lee, D.H.; Ahn, J.W. Direct instantaneous torque control of switched reluctance machines using 4-level converters. IET Electr. Power Appl. 2009, 3, 313–323. [Google Scholar] [CrossRef]
- Zeng, H.; Chen, H.; Shi, J. Direct instantaneous torque control with wide operating range for switched reluctance motors. IET Electr. Power Appl. 2015, 9, 578–585. [Google Scholar] [CrossRef]
- Li, W.; Cui, Z.; Ding, S.; Chen, F.; Guo, Y. Model Predictive Direct Torque Control of Switched Reluctance Motors for Low-Speed Operation. IEEE Trans. Energy Convers. 2022, 37, 1406–1415. [Google Scholar] [CrossRef]
- Thirumalasetty, M.; Narayanan, G. PWM-Based Predictive Direct Torque Control of Switched Reluctance Machine for Accurate Torque Tracking with Minimization of Phase RMS Currents. IEEE Trans. Ind. Appl. 2024, 1–13. [Google Scholar] [CrossRef]
- Cai, Y.; Dong, Z.; Liu, H.; Liu, Y.; Wu, Y. Direct Instantaneous Torque Control of SRM Based on a Novel Multilevel Converter for Low Torque Ripple. World Electr. Veh. J. 2023, 14, 140. [Google Scholar] [CrossRef]
- Sun, Q.; Wu, J.; Gan, C. Optimized Direct Instantaneous Torque Control for SRMs with Efficiency Improvement. IEEE Trans. Ind. Electron. 2021, 68, 2072–2082. [Google Scholar] [CrossRef]
- Sheng, L.; Wang, G.; Fan, Y.; Liu, J.; Liu, D.; Mu, D. An Improved Direct Predictive Torque Control for Torque Ripple and Copper Loss Reduction in SRM Drive. Appl. Sci. 2023, 13, 5319. [Google Scholar] [CrossRef]







| SCIM | PMSM | SRM | |
|---|---|---|---|
| Size | Moderate | Moderate | Compact |
| Weight | Moderate | Moderate | Low |
| Cost | Low | High | Low |
| Ruggedness | High | Low | High |
| Power density | Moderate | High | Moderate |
| Constant torque speed range | Moderate | Wide | Wide |
| Efficiency | Low | High | Moderate |
| Permanent magnets | No | Yes | No |
| Power factor | Low | High | Low |
| Cooling | Complicated | Moderate | Simple |
| Fault tolerance | Low | Low | High |
| Torque ripple | Low | Low | High |
| Noise and vibration | Low | Low | High |
| Power converter | Modular | Modular | Specific |
| Bifilar [20] | R-Dump [18,19] | C-Dump [21,22] | Split DC Link [23] | Common Switch [17] | Common Phase [16] | ASHB [15] | Boost [24] | Boost and Reg. [40] | |
|---|---|---|---|---|---|---|---|---|---|
| Number of switches | n | n | n + 1 | n | n + 1 | n + 2 | 2n | n + 2 | n + 3 |
| Rating of switch | 2 | ||||||||
| Control complexity | simple | Simple | Complex | Simple | Simple | Simple | Simple | Simple | Moderate |
| SRM modification | Yes | No | No | No | No | No | No | No | No |
| Fault-tolerance | High | High | High | Low | Low | Moderate | High | Moderate | Moderate |
| Performance | Medium | Low | High | Medium | Low | Moderate | High | High | High |
| Current build up | Medium | Slow | Medium | Slow | Slow | Medium | Medium | Fast | Fast |
| Efficiency | Medium | Low | High | High | Low | Low | High | High | High |
| Voltage levels | |||||||||
| Snubber circuits | Yes | No | No | No | No | No | No | No | No |
| Number of phases | Any | Any | Any | Even | Any | Even | Any | Even | Even |
| Current Profiling [75] | TSF [105] | DITC [113] | |
|---|---|---|---|
| Control parameter | Current | Phase torque | Total torque |
| SRM modification | No | No | No |
| Prior knowledge of SRM parameters | Yes | Yes | Yes |
| Current profiles memory storage | Yes | No | No |
| Current sensors | Required | Required | Required |
| Position sensor | Required | Required | Required |
| Number of overlapping phases | Limited to two | Limited to two | Any number |
| Methodology | Offline | Offline | Any |
| Torque–speed range | Moderate | Moderate | Wide |
| RMS current optimisation | Yes | Yes | Yes |
| Torque ripple percentage | Medium | Medium | Low |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abdel-Aziz, A.; Elgenedy, M.; Williams, B. Review of Switched Reluctance Motor Converters and Torque Ripple Minimisation Techniques for Electric Vehicle Applications. Energies 2024, 17, 3263. https://doi.org/10.3390/en17133263
Abdel-Aziz A, Elgenedy M, Williams B. Review of Switched Reluctance Motor Converters and Torque Ripple Minimisation Techniques for Electric Vehicle Applications. Energies. 2024; 17(13):3263. https://doi.org/10.3390/en17133263
Chicago/Turabian StyleAbdel-Aziz, Ali, Mohamed Elgenedy, and Barry Williams. 2024. "Review of Switched Reluctance Motor Converters and Torque Ripple Minimisation Techniques for Electric Vehicle Applications" Energies 17, no. 13: 3263. https://doi.org/10.3390/en17133263
APA StyleAbdel-Aziz, A., Elgenedy, M., & Williams, B. (2024). Review of Switched Reluctance Motor Converters and Torque Ripple Minimisation Techniques for Electric Vehicle Applications. Energies, 17(13), 3263. https://doi.org/10.3390/en17133263






