Integrated Underground Analyses as a Key for Seasonal Heat Storage and Smart Urban Areas
Abstract
1. Introduction
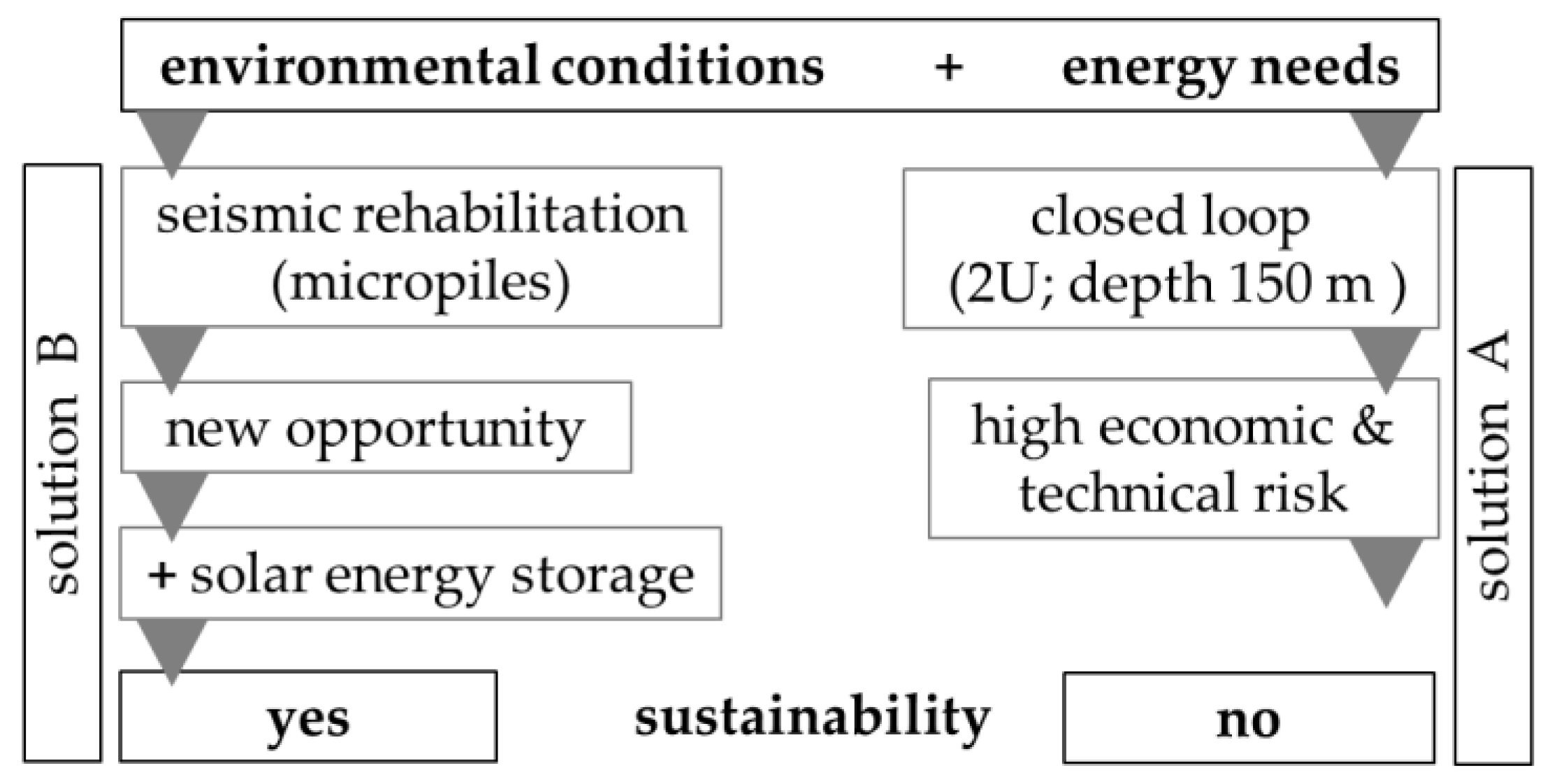
Major Objectives
- (a)
- the winter heating energy requirement had to be satisfied by designing a low enthalpy geothermal system (‘stable renewable resource’) consisting of double U probes, each 150 m deep, at an equidistance of 8 m from each other and in a rectangular geometry; the geometric characteristics of the layout were conditioned by the logistics of the area surrounding the building;
- (b)
- the electrical energy devoted to feeding the geothermal heat pump had to be provided by the installation of photovoltaic panels on the roof of the building.
2. Materials and Methods
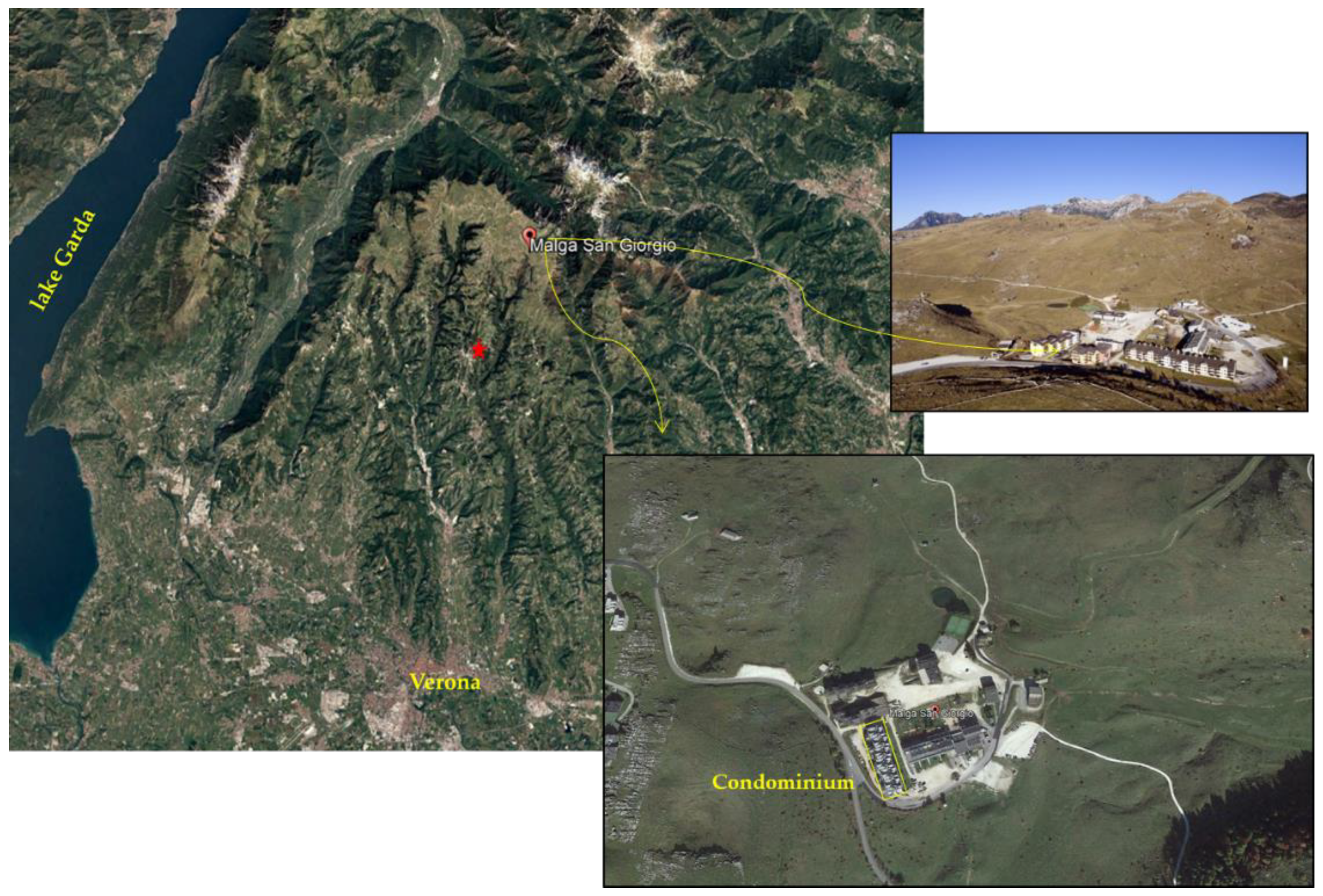
2.1. The Case Study
2.2. Bulding Energy Needs
2.3. Air Temperature Data
2.4. Geological and Hydrogeological Investigation
In-Hole Measurements
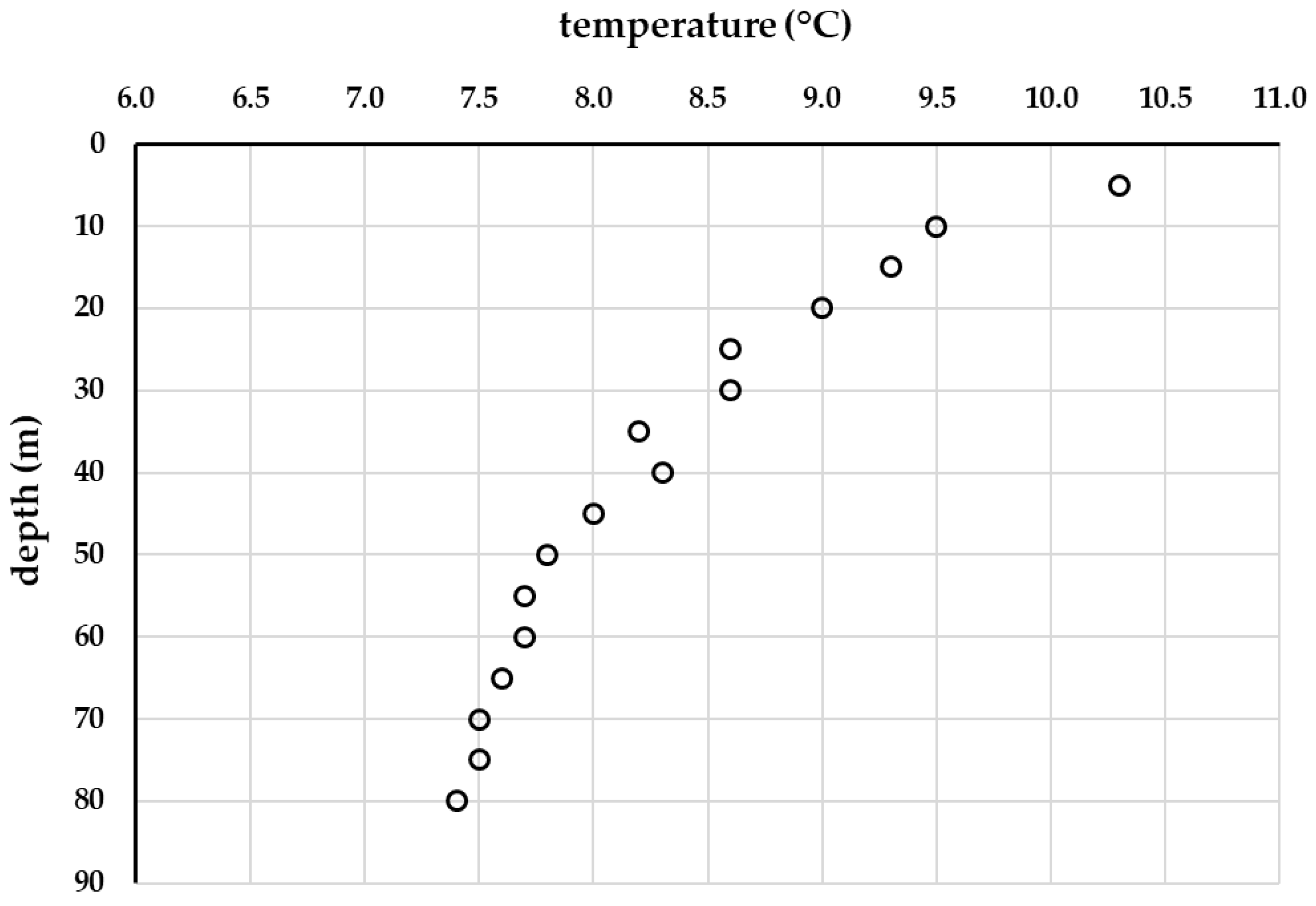
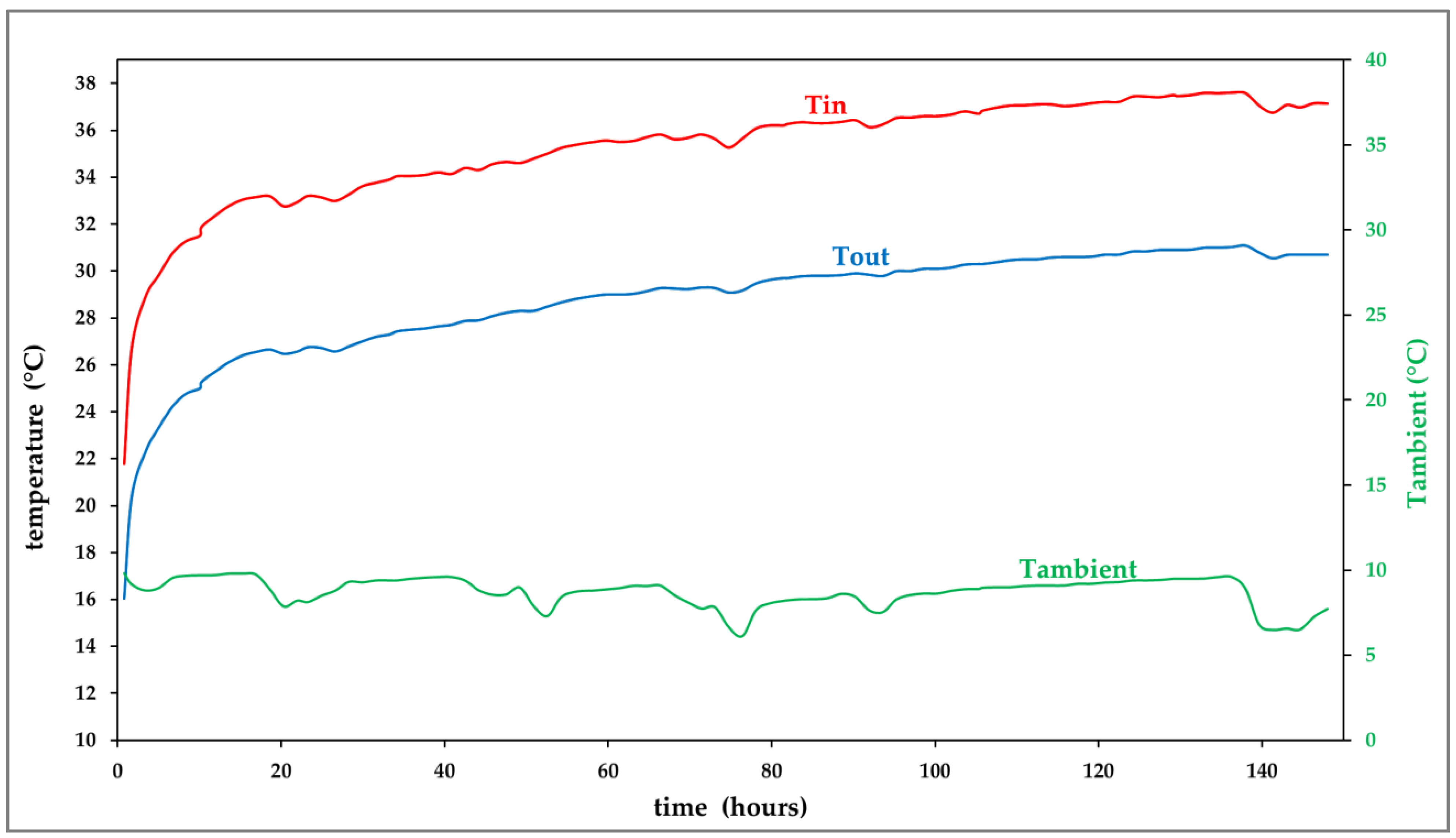
2.5. Undisturbed Ground Temperature and Thermal Response Test
2.6. Numerical Model and Calculation of Stored Heat in the Ground
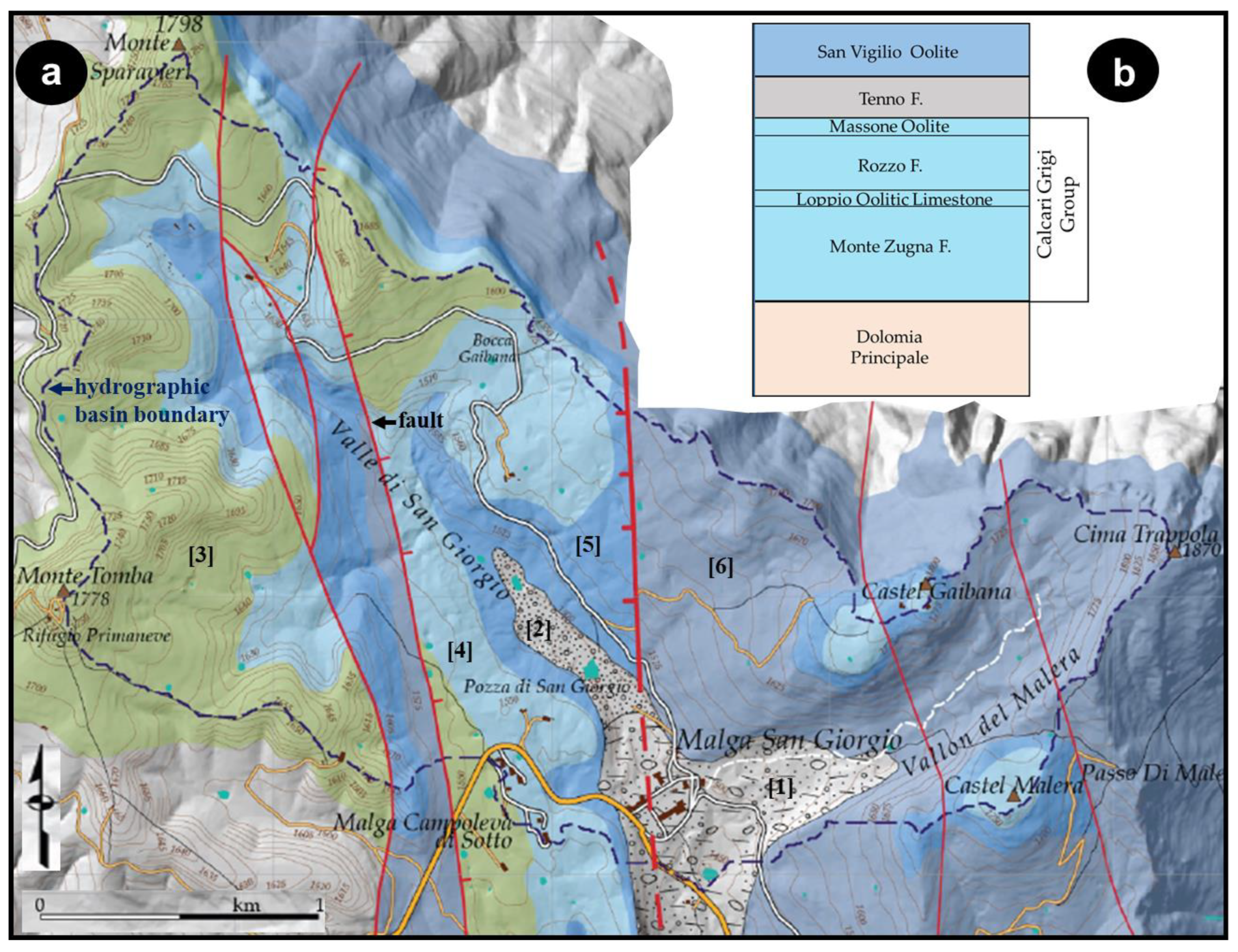
3. Geological Model
3.1. Local Geological Model and Underground Circulation Conditions
- (a)
- medium coarse gravel; gravel with limestone clasts (diameter 0.5–5.0 cm), with sub-rounded pebbles, to a lesser extent, of varying diameter from 6 to 10 cm, with medium-fine gravel matrices in significant quantities (20–35%);
- (b)
- pebble gravel composed of gravel clasts of variable diameter (3 and 8 cm), with a medium-fine gravel matrix (generally <15%, but locally up to 30–35%); the clasts are calcareous, heterogeneous with angular or slightly rounded shapes;
- (c)
- gravel pebble slightly sandy deposits consisting of gravel clasts (diameter 4–10 cm), with angular to sub-rounded shapes, with a gravel–sandy matrix (35–40%) containing even a small amount of silty fraction (example in Figure 5a);
- (d)
- medium gravel weak sands consisting of poorly classed gravels, with limestone clasts (diameter 0.5–2.0 cm), with a matrix composed of fine gravel and coarse sand;
- (e)
- silty sandy gravel deposits consisting of limestone clasts (diameter 0.5–2.0 cm), generally not rounded, rarely containing sub-angular limestone pebbles up to 6 cm, with abundant (40–45%) sandy–silty matrices (example in Figure 5a);
- (f)
- gravelly–sandy silt unit formed by an abundant yellowish silty–clayey matrix, slightly sandy, with the presence of a few clasts (diameter 1–2 cm; example in Figure 5a);
- (g)
- blocks of calcarenite and bioclastic limestone with bivalves varying in dimension between 5 and 20 cm;
- (h)
- oolitic limestones (25.6 to 32.0 m) belonging to the Massone Formation (example in Figure 5c);
- (i)
- pelitic limestones (32.0 to 120.0 m) belonging to the Rotzo Formation, representing a medium to low hydrodynamic energy lagoon environment, with frequent biostromes formed by the accumulation of Lithiotis (example in Figure 5b); this unit is highly heterogeneous and locally highly fractured.
3.2. Hydraulic Parameters
4. Design Changes and Modelling
4.1. Choice and Design of the Geothermal Solution
- (a)
- the drilling of a second deep (80 m) pilot borehole to verify the lithological sequences directly underlying the building; to measure the temperature in relation to the depth in order to define the undisturbed temperature of the subsoil, conditioned by the building presence above it. This investigation allowed us to verify that the Quaternary deposits have a local total thickness of 15 m overlying the carbonate bedrock down to the borehole bottom.
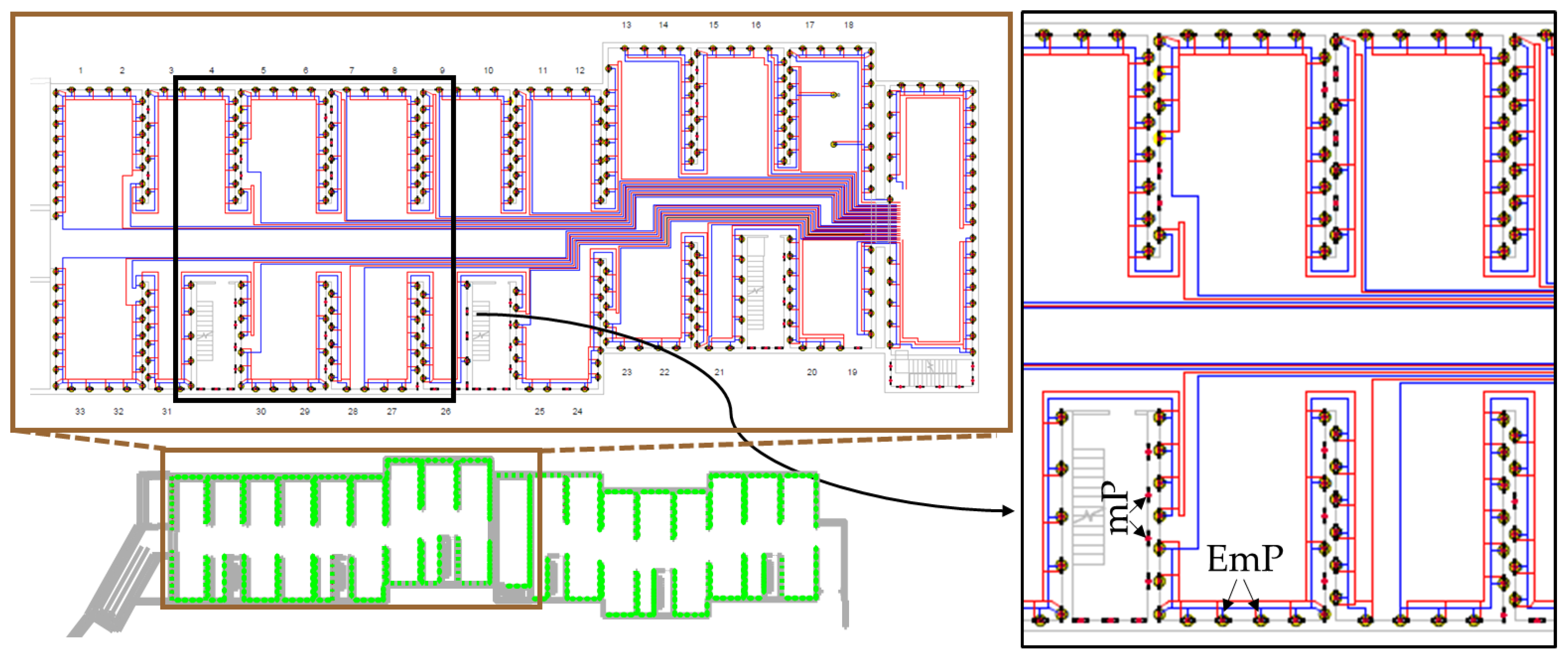
- (b)
- in one of the 20 m-deep energy micropile probes, a thermal response test was carried out to define the thermophysical parameters of the shallow subsoil.
4.2. Thermophysical Parameters of the Underground
4.3. Numerical Model
4.3.1. Model Creation and Domain Discretization
4.3.2. Simulation of the Initial State
5. Conclusions
- (a)
- conceive/introduce the energy micropiles (EmPs) by combining/integrating geotechnical solutions (i.e., foundation micropiles) with geothermal ones, and hence selecting coaxial geothermal probes in accordance with the concept of circular economy;
- (b)
- install the EmPs inside the garage of the building without any additional inclusion of soil or hydraulic works;
- (c)
- conceive and design an integrated (hybrid) renewable energy supply system (geothermal and solar) to provide the thermal energy necessary for heating the building.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lund, H.; Østergaard, P.A.; Connolly, D.; Mathiesen, B.V. Smart energy and smart energy systems. Energy 2017, 137, 556–565. [Google Scholar] [CrossRef]
- Emiliano Corà—EUREC, BE. (Ed.) 2050 Vision for 100% Renewable Heating and Cooling in Europe; Bruxelles, Belgium, 2020. Available online: https://www.rhc-platform.org/content/uploads/2019/10/RHC-VISION-2050-WEB.pdf (accessed on 23 February 2024).
- Directive (EU) 2023/2413 of the European Parliament and of the Council of 18 October 2023 Amending Directive (EU) 2018/2001, Regulation (EU) 2018/1999 and Directive 98/70/EC as Regards the Promotion of Energy from Renewable Sources, and Repealing Council Directive (EU) 2015/652. Available online: https://eur-lex.europa.eu/legal-content/EN/TXT/?uri=CELEX%3A32023L2413&qid=1699364355105 (accessed on 23 February 2024).
- European Climate Foundation. Available online: https://europeanclimate.org/wp-content/uploads/2022/03/ecf-building-emissions-problem-march2022.pdf (accessed on 23 February 2024).
- Directive 2010/31/EU of the European Parliament and of the Council of 19 May 2010 on the Energy Performance of Buildings (Recast). Available online: https://eur-lex.europa.eu/legal-content/EN/TXT/HTML/?uri=CELEX:32010L0031 (accessed on 23 February 2024).
- Commission Recommendation (EU) 2016/1318 of 29 July 2016 on Guidelines for the Promotion of Nearly Zero-Energy Buildings and Best Practices to Ensure That, by 2020, All New Buildings Are Nearly Zero-Energy Buildings. Available online: https://eur-lex.europa.eu/legal-content/EN/TXT/PDF/?uri=CELEX:32016H1318&from=EN (accessed on 23 February 2024).
- Paksoy, H.O.; Andersson, O.; Abaci, S.; Evliya, H.; Turgut, B. Heating and cooling of a hospital using solar energy coupled with seasonal thermal energy storage in an aquifer. Renew. Energy 2000, 19, 117–122. [Google Scholar] [CrossRef]
- Rapti, D.; Marchetti, A.; Andreotti, M.; Neri, I.; Caputo, R. GeoTh: An Experimental Laboratory Set-Up for the Measurement of the Thermal Conductivity of Granular Materials. Soil Syst. 2021, 6, 88. [Google Scholar] [CrossRef]
- Liuzzo-Scorpo, A.M.; Nordell, B.; Gehlin, S. Influence of regional groundwater flow on ground temperature around heat extraction boreholes. Geothermics 2015, 56, 119–127. [Google Scholar] [CrossRef]
- Zilong, Z.; Lin, Y.-F.; Stumpf, A.; Wang, X. Assessing impacts of groundwater on geothermal heat exchangers: A review of methodology and modeling. Renew. Energy 2022, 190, 121–147. [Google Scholar] [CrossRef]
- Cunha, R.P.; Bourne-Webb, P.J. A critical review on the current knowledge of geothermal energy piles to sustainably climatize buildings. Renew. Sustain. Energy Rev. 2022, 158, 112072. [Google Scholar] [CrossRef]
- Mohamad, Z.; Fardoun, F.; Meftah, F. A review on energy piles design, evaluation, and optimization. J. Clean. Prod. 2021, 292, 125802. [Google Scholar] [CrossRef]
- Da Silva Oliveira Morais, T.; de Hollanda Cavalcanti Tsuha, C.; Bandeira Neto, L.A.; Martand Singh, R. Effects of seasonal variations on the thermal response of energy piles in an unsaturated Brazilian tropical soil. Energy Build. 2020, 216, 109971. [Google Scholar] [CrossRef]
- Lizzi, F. First Patents on Root Piles and Reticulated Root. Piles, Fundedile. 1950-52, Naples. Patent no. 497736, March 1952. [Google Scholar]
- Armour, T.; Groneck, P.; Keeley, J.; Sharma, S. Micropile Design and Construction Guidelines Implementation Manual; Priority Technologies Program (PTP) Project. Report No. FHWASA-97-070; Federal Highway Administration: Washington, DC, USA, 2000; pp. 1–376. [Google Scholar]
- EN 14199:2015; Execution of Special Geotechnical Works—Micropiles. CEN—European Committee for Standardization: Brussels, Belgium, 2015.
- Sabatini, P.J.; Tanyu, B.; Armour, T.; Groneck, P.; Keeley, J. Micropile Design and Construction Guidelines; FHWA: Washington, DC, USA, 2005; p. 380.
- El Kamash, W.; El Naggar, H.; To, P.; Sivakugan, N. The Effect of Long-Term Consolidation on Foundations Underpinned by Micropiles in Soft Clay. Ain Shams Eng. J. 2022, 13, 101487. [Google Scholar] [CrossRef]
- Alsaleh, H.; Shahrour, I. Influence of Plasticity on the Seismic Soil-Micropiles-Structure Interaction. Soil Dyn. Earthq. Eng. 2009, 29, 574–578. [Google Scholar] [CrossRef]
- Xie, W.; Limin, S. Assessment and Mitigation on Near-Fault Earthquake Wave Effects on Seismic Responses and Pile-Soil Interactions of Soil-Pile-Bridge Model. Soil Dyn. Earthq. Eng. 2021, 143, 106596. [Google Scholar] [CrossRef]
- Capatti, M.C.; Dezzi, F.; Carbonari, S. Full-Scale Experimental Assessment of the Dynamic Horizontal Behavior of Micropiles in Alluvial Silty Soils. Soil Dyn. Earthq. Eng. 2018, 113, 58–74. [Google Scholar] [CrossRef]
- Traylor, R.P.; Cadden, A.W.; Bruce, D.A. High capacity micropiles in karst: Challenges and opportunities. In Deep Foundations 2002: An International Perspective on Theory, Design, Construction, and Performance; ASCE: Reston, VA, USA, 2002; pp. 743–759. [Google Scholar]
- Bivens, M.J.; Siegel, T.C. Case histories of micropile in karst: The influence of installation on design performance. In Contemporary Issues in Deep Foundations; ASCE: Reston, VA, USA, 2007. [Google Scholar] [CrossRef]
- European Commission. The European Green Deal; Communication (Document 52019DC0640); European Commission: Brussels, Belgium, 2019; Available online: https://eur-lex.europa.eu/legal-content/EN/TXT/HTML/?uri=CELEX:52019DC0640 (accessed on 22 February 2024).
- Department of Civil Protection as at 31 March 2022. Available online: https://rischi.protezionecivile.gov.it/static/c7650fa8eac014a28ef074236bb234f7/mappa-classificazione-sismica-aggiornata-al-31-marzo-2022-provincia.pdf (accessed on 20 February 2024).
- Sauro, U.; Bidese, E.; Bonomi, E. Cimbri dei Monti Lessini; Grafica, L., Ed.; La Grafica: Verona, Italy, 2017; 408p, ISBN 978-88-6947-154-4. [Google Scholar]
- ARPAV Database. Available online: https://www.arpa.veneto.it/dati-ambientali/dati-storici/aria/ (accessed on 1 April 2023).
- UNI 10349:1994/EC; Riscaldamento e Raffrescamento Degli Edifici, Dati Climatici. Ente Nazionale Italiano di Unificazione: Milan, Italy, 1997. (In Italian)
- Masetti, D.; Fantoni, R.; Romano, R.; Sartorio, D.; Trevisani, E. Tectonostratigraphic evolution of the Jurassic extensional basins of the eastern Southern Alps and Adriatic foreland based on an integrated study of surface and subsurface data. Am. Ass. Petrol. Geol. Bull. 2012, 96, 2065–2089. [Google Scholar] [CrossRef]
- Sauro, U. Geomorfological Map of the Upper Part of the Lessini (Prealpi Venete); scale: 1:25.000; Istituto Geografico Militare (autorizzazione n. 506 del13-12-1971), Litografia Artstica Cartografica: Firenze, Italy, 1973. [Google Scholar]
- Zampieri, D.; Zorzin, R. Carta geologica dei Lessini Centro-Occidentali tra la Valpantena e la Val d’Illasi. In Geologia, Idrogeologia e Qualità dei Principali Acquiferi Veronesi; A cura di Memoria del Museo Civico di Storia Naturale di Verona, 2. Serie, sezione Scienze della Terra, B4; Gutenberg: Povegliano Veronese, Italy, 1993. [Google Scholar]
- Preto, N.; Breda, A.; Dal Corso, J.; Franceschi, M.; Rocca, F.; Spada, C.; Roghi, G. The Loppio Oolitic Limestone (Early Jurassic, Southern Alps): A prograding oolitic body with high original porosity originated by a carbonate platform crisis and recovery. Mar. Pet. Geol. 2017, 79, 394–411. [Google Scholar] [CrossRef]
- Posenato, R.; Masetti, D. Environmental control and dynamics of Lower Jurassic bivalve build-ups in the Trento Platform (Southern Alps, Italy). Palaeogeogr. Palaeoclimatol. Palaeoecol. 2012, 361–362, 1–13. [Google Scholar] [CrossRef]
- Lefranc, E. Procédé de mesure de la perméabilité des sols dans les nappes aquifères et application au calcul du débit des puits. Le Génie Civ. 1936, 109, 306–308. [Google Scholar]
- Lefranc, E. La théorie des poches absorbantes et son application à la détermination du coefficient de perméabilité en place et au calcul du débit des nappes d’eau. Le Génie Civ. 1937, 111, 409–413. [Google Scholar]
- Hvorslev, M.J. Time-Lag and Soil Permeability in Ground-Water Observations; Bulletin 36; U.S. Army Engineer Waterways Experiment Station: Vicksburg, MI, USA, 1951; p. 50. [Google Scholar]
- Chapuis, R.P. Overdamped slug test in monitoring wells: Review of interpretation methods with mathematical, physical, and numerical analysis of storativity influence. Can. Geotech. J. 1998, 35, 697–719. [Google Scholar] [CrossRef]
- Association Française de Normalisation (AFNOR). Recognition and Tests, LEFRANC Water Test; Norme Française NF P 94-132 Sols; AFNOR: La Plaine Saint-Denis Cedex, France, 2000. (In French) [Google Scholar]
- Lugeon, M. Barrages et Géologie; Dunod: Paris, France, 1933. [Google Scholar]
- Wang, T.T.; Zhan, S.S.; Huang, T.H. Determining transmissivity of fracture sets with statistical significance using single-borehole hydraulic tests: Methodology and implementation at Heshe well site in Central Taiwan. Eng. Geol. 2015, 198, 1–15. [Google Scholar] [CrossRef]
- Shahbazi, A.; Saeidi, A.; Chesnaux, R. A review of existing methods used to evaluate the hydraulic conductivity of a fractured rock mass. Eng. Geol. 2020, 265, 105438. [Google Scholar] [CrossRef]
- Vaskou, P.; de Quadros, E.F.; Kanji, M.A.; Johnson, T.; Ekmekci, M. ISRM Suggested Method for the Lugeon Test. Rock Mech. Rock Eng. 2019, 52, 4155–4174. [Google Scholar] [CrossRef]
- Houlsby, A. Routine Interpretation of the Lugeon Water-Test. Q. J. Eng. Geol. 1976, 9, 303–313. [Google Scholar] [CrossRef]
- Nonveiller, E. Grouting Theory and Practice, Development of Geotechnical Engineering; Elsevier: Amsterdam, The Netherlands, 1989. [Google Scholar]
- Dou, J.X.; Zhang, G.J.; Zhou, M.X.; Wang, Z.L.; Gyatso, N.; Jiang, M.Q.; Safari, P.; Liu, J.Q. Curtain grouting experiment in a dam foundation: Case study with the main focus on the Lugeon and grout take tests. Bull. Eng. Geol. Environ. 2020, 79, 4527–4547. [Google Scholar] [CrossRef]
- Deere, D.U. Technical description of rock cores for engineering purposes. Rock Mech. Eng. Geol. 1963, 1, 18–22. [Google Scholar]
- El-Naqa, A. The hydraulic conductivity of the fractures intersecting Cambrian sandstone rock masses, central Jordan. Environ. Geol. 2001, 40, 973–982. [Google Scholar] [CrossRef]
- Jiang, X.W.; Wan, L.; Wang, X.S.; Wu, X.; Zhang, X. Estimation of rock mass deformation modulus using variation sin transmissivity and RQD with depth. Int. J. Rock Mech. Min. Sci. 2009, 46, 1370–1377. [Google Scholar] [CrossRef]
- Tinti, F.; Barbaresi, A.; Benni, S.; Torreggiani, D.; Bruno, R.; Tassinari, P. Experimental analysis of shallow underground temperature for the assessment of energy efficiency potential of underground wine cellars. Energy Build. 2015, 80, 451–460. [Google Scholar] [CrossRef]
- Baggs, S.A. Remote prediction of ground temperature in Australian soils and mapping its distribution. Sol. Energy 1983, 30, 351–366. [Google Scholar] [CrossRef]
- Kavanaugh, S.P.; Xie, L.; Martin, C. Investigation of Methods for Determining Soil and Rock Formation Thermal Properties from Short-Term Field Tests; Final Report for ASHRAE 1118 RP-1118; American Society of Heating, Refrigerating and Air-Conditioning Engineers, Inc.: Peachtree Corners, GA, USA, 2000; p. 77. [Google Scholar]
- Carslaw, H.G.; Jaeger, J.C. Conduction of Heat in Solids; Oxford University Press: Oxford, UK, 1959; ISBN 0198533683. [Google Scholar]
- Mogensen, P. Fluid to duct wall heat transfer in duct system heat storage. In Proceedings of the International Conference on Subsurface Heat Storage in Theory and Practice, Stockholm, Sweden, 6–8 June 1983; Ed. Swedish Council for Building Research, Sweden, 1983. pp. 652–657. [Google Scholar]
- Eskilson, P. Thermal Analysis of Heat Extraction Boreholes. Ph.D. Thesis, Department of Mathematical Physics, University of Lund, Lund, Sweden, 1987; p. 264. [Google Scholar]
- Bandos, T.V.; Montero, Á.; Fernández, E.; Santander, J.L.G.; Isidro, J.M.; Pérez, J.; de Córdoba, P.J.F.; Urchueguía, J.F. Finite Line-Source Model for Borehole Heat Exchangers: Effect of Vertical Temperature Variations. Geothermics 2009, 38, 263–270. [Google Scholar] [CrossRef]
- Spitler, J.D.; Gehlin, S.E.A. Thermal response testing for ground source heat pump systems—An historical review. Renew. Sustain. Energy Rev. 2015, 50, 1125–1137. [Google Scholar] [CrossRef]
- Al-Khoury, R.; Bonnier, P.G.; Brinkgreve, R.B.J. Efficient finite element formulation for geothermal heating systems: Part I: Steady state. Int. J. Num. Methods Eng. 2005, 63, 988–1013. [Google Scholar] [CrossRef]
- Al-Khoury, R.; Bonnier, P.G. Efficient finite element formulation for geothermal heating systems: Part II: Transient. Int. J. Num. Methods Eng. 2006, 67, 725–745. [Google Scholar] [CrossRef]
- Quiñones-Rozo, C. Lugeon test interpretation, revisited. In Collaborative Management of Integrated Watersheds, US Society of Dams, Proceedings of the 30th Annual Conference; Sacramento, CA, USA, 12–16 April 2010, Hosted by Bureau of Reclamation: Washington, DC, USA, 2010; pp. 405–414. [Google Scholar]
- Chiasson, A.; Rees, S.J.; Spitler, J.D. A preliminary assessment of the effects of ground-water flow on closed-loop ground-source systems. ASHRAE Trans. 2000, 106, 380–393. [Google Scholar]
- Verein Deutscher Ingenieure-VDI. VDI 4640 Part 1. In Thermal Use of the Underground: Fundamentals, Approvals, Environmental Aspects; Verein Deutscher Ingenieure: Düsseldorf, Germany, 2010.











| Stage | Pressure (bar) |
|---|---|
| 1st | 6.7 |
| 2nd | 8.7 |
| 3rd | 10.7 |
| 4th | 8.7 |
| 5th | 6.7 |
| RQD (%) | Rock Mass Quality |
|---|---|
| <25 | very poor |
| 25–50 | poor |
| 50–75 | fair |
| 75–90 | good |
| 90–100 | excellent |
| Parameters | Injected Power | Power per Unit Length | Flow Rate | Duration |
|---|---|---|---|---|
| units | (W) | (W/m) | (L/s) | (h) |
| value | 1500 | 75 | 0.05 | 148 |
| Symbol | Variable | Unit |
|---|---|---|
| D | depth | m |
| T | temperature | °C |
| k | hydraulic conductivity | m/s |
| RQD | Rock Quality Designation Index | % |
| P | power | W |
| t | time | days |
| Construction Feature | Symbol | Value | Unit |
|---|---|---|---|
| depth of the EmP head | Dhead | 5 | m |
| EmP length | Lpile | 20 | m |
| EmP diameter | dpile | 0.14 | m |
| grout thermal conductivity | λgrout | 2 | W/(m·K) |
| diameter of the external pipe | dpipe,e | 0.06 | m |
| thickness of the external pipe | thpipe,e | 0.008 | m |
| thermal conductivity of the external pipe | λpipe,e | 290 | W/(m·K) |
| diameter of the internal pipe | dpipe,i | 0.025 | m |
| thickness of the internal pipe | thpipe,i | 0.002 | m |
| thermal conductivity of the internal pipe | λpipe,i | 0.42 | W/(m·K) |
| heat capacity of the heat transfer fluid | cfluid | 4.016 | MJ/(m3·K) |
| thermal conductivity of the heat transfer fluid | λfluid | 0.526 | W/(m·K) |
| dynamic viscosity of the heat transfer fluid | ηfluid | 6.4 | g/(m·s) |
| density of the heat transfer fluid | ρfluid | 1.004 | t/m3 |
| Depth Interval | Geologic Unit | Hydraulic Conductivity | Porosity | Thermal Conductivity | Heat Capacity [59] |
|---|---|---|---|---|---|
| m | - | m/d | (-) | W/(m·K) | MJ/(m3·K) |
| 0–15 | Quaternary deposits | 1.1232 | 0.3 | 1.00 | 1.75 |
| 16–120 | Mesozoic limestone | 0.0915 | 0.3 | 3.03 | 2.28 |
| Layer | Depth | Layer | Depth |
|---|---|---|---|
| Number | m | Number | m |
| 1 | 0 | 12 | 35 |
| 2 | 1 | 13 | 40 |
| 3 | 2 | 14 | 45 |
| 4 | 3 | 15 | 50 |
| 5 | 4 | 16 | 55 |
| 6 | 5 | 17 | 60 |
| 7 | 10 | 18 | 65 |
| 8 | 15 | 19 | 70 |
| 9 | 20 | 20 | 75 |
| 10 | 25 | 21 | 80 |
| 11 | 30 | 22 | 120 |
| Month | Daily Operating Hours (Full Month) | Monthly Operating Days (Full Day) | |
|---|---|---|---|
| h | Days | ||
| extraction prevailing | January | 11.98 | 15.48 |
| February | 8.93 | 10.42 | |
| March | 0.65 | 0.84 | |
| injection prevailing | April | 7.90 | 9.88 |
| May | 15.44 | 19.94 | |
| June | 18.74 | 23.42 | |
| July | 20.52 | 26.51 | |
| August | 17.26 | 22.30 | |
| September | 11.28 | 14.10 | |
| extraction prevailing | October | 2.36 | 3.05 |
| November | 7.19 | 8.99 | |
| December | 11.90 | 15.37 |
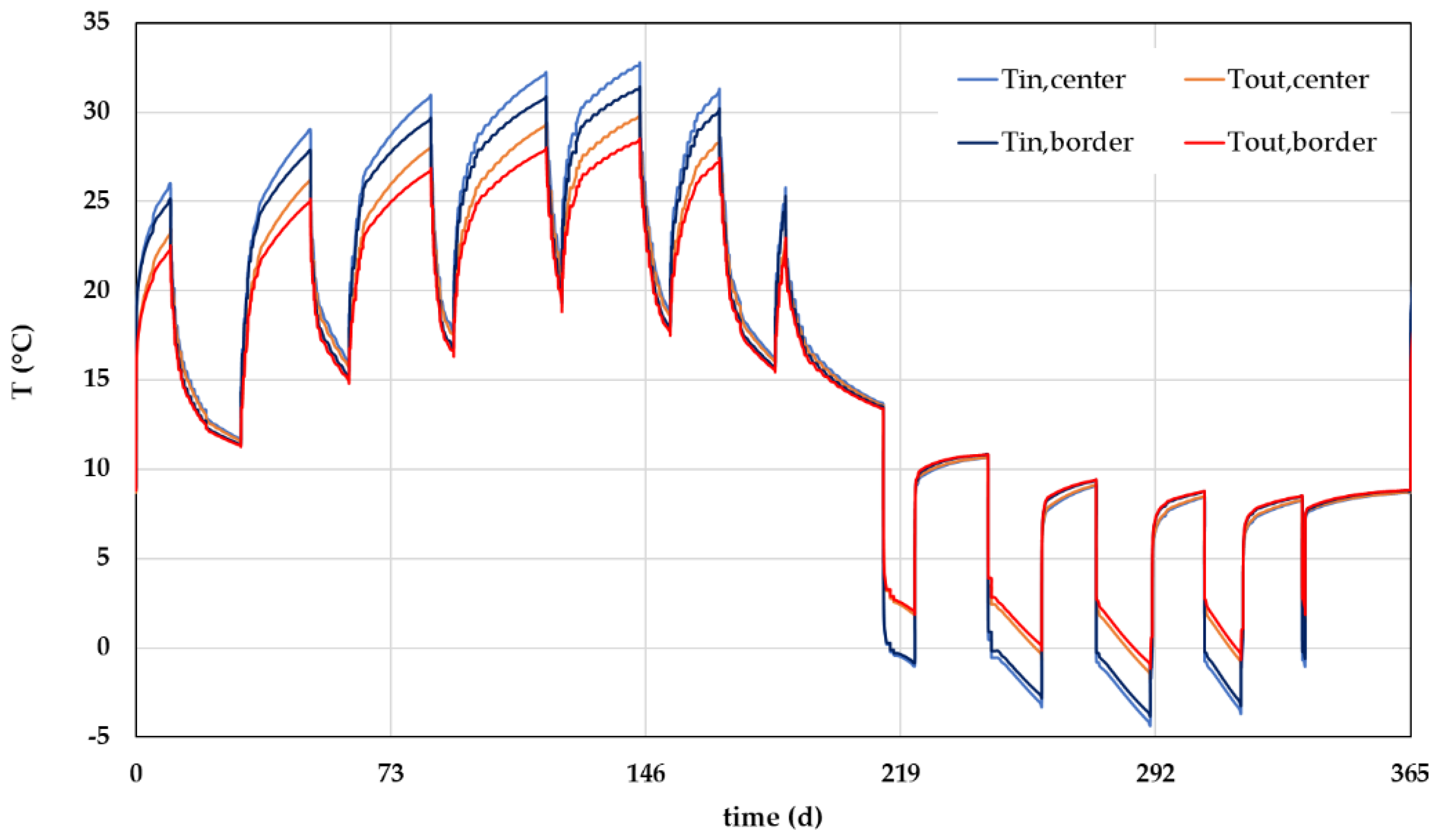
| EmP in the Middle of the Geothermal Field | EmP at the Border of the Geothermal Field | |||
|---|---|---|---|---|
| Years | min T (°C) | max T (°C) | min T (°C) | max T (°C) |
| 1 | −4.34 | 32.77 | −3.81 | 31.25 |
| 2 | −4.05 | 32.94 | −3.53 | 31.43 |
| 3 | −3.97 | 33.07 | −3.44 | 31.74 |
| 4 | −3.99 | 33.38 | −3.45 | 32.03 |
| 5 | −4.04 | 32.97 | −3.50 | 31.84 |
| variation 1–5 years | +0.3 °C | +0.2 °C | +0.3 °C | +0.6 °C |
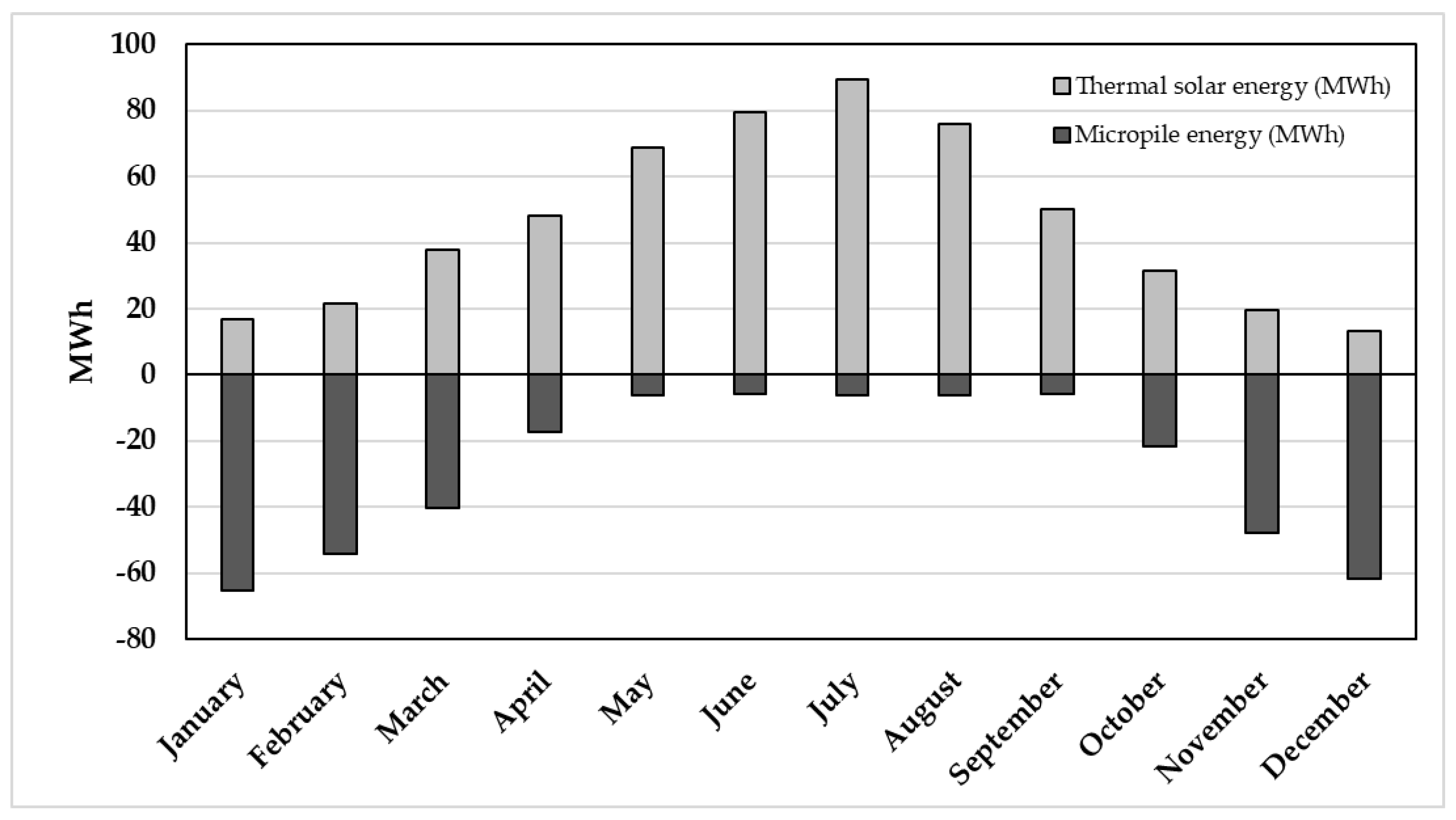
| Month | Extracted/Injected Energy (MWh) |
|---|---|
| January | −24.22 |
| February | −18.80 |
| March | −7.55 |
| April | +11.75 |
| May | +34.36 |
| June | +40.00 |
| July | +41.81 |
| August | +29.74 |
| September | +19.27 |
| October | −8.45 |
| November | −16.90 |
| December | −23.96 |
| Depth (m) | th (m) | cg (MJ/m3·K) | r (m) | Tg,i (°C) | Tg,f (°C) | ΔTg (K) | Qst (MJ) | Qst (MWh) |
|---|---|---|---|---|---|---|---|---|
| 5 | 5 | 1.75 | 10 | 9.4 | 24.4 | 15 | 41,233.40 | 11.45 |
| 10 | 5 | 1.75 | 10 | 8.9 | 29.9 | 21 | 57,726.77 | 16.04 |
| 15 | 5 | 1.75 | 10 | 8.7 | 29.7 | 21 | 115,453.50 | 32.07 |
| 20 | 5 | 2.28 | 10 | 8.4 | 29.1 | 20.7 | 148,270.61 | 41.19 |
| total | 20 | 362,684.31 | 100.75 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rapti, D.; Tinti, F.; Caputo, C.A. Integrated Underground Analyses as a Key for Seasonal Heat Storage and Smart Urban Areas. Energies 2024, 17, 2533. https://doi.org/10.3390/en17112533
Rapti D, Tinti F, Caputo CA. Integrated Underground Analyses as a Key for Seasonal Heat Storage and Smart Urban Areas. Energies. 2024; 17(11):2533. https://doi.org/10.3390/en17112533
Chicago/Turabian StyleRapti, Dimitra, Francesco Tinti, and Carlo Antonio Caputo. 2024. "Integrated Underground Analyses as a Key for Seasonal Heat Storage and Smart Urban Areas" Energies 17, no. 11: 2533. https://doi.org/10.3390/en17112533
APA StyleRapti, D., Tinti, F., & Caputo, C. A. (2024). Integrated Underground Analyses as a Key for Seasonal Heat Storage and Smart Urban Areas. Energies, 17(11), 2533. https://doi.org/10.3390/en17112533






