Comparative Irradiated Dimensional Change Strain Analyses of Two Types of Graphite Components in a Thorium Molten Salt Reactor
Abstract
1. Introduction
2. Methodology
2.1. Constitutive Equations for UMAT
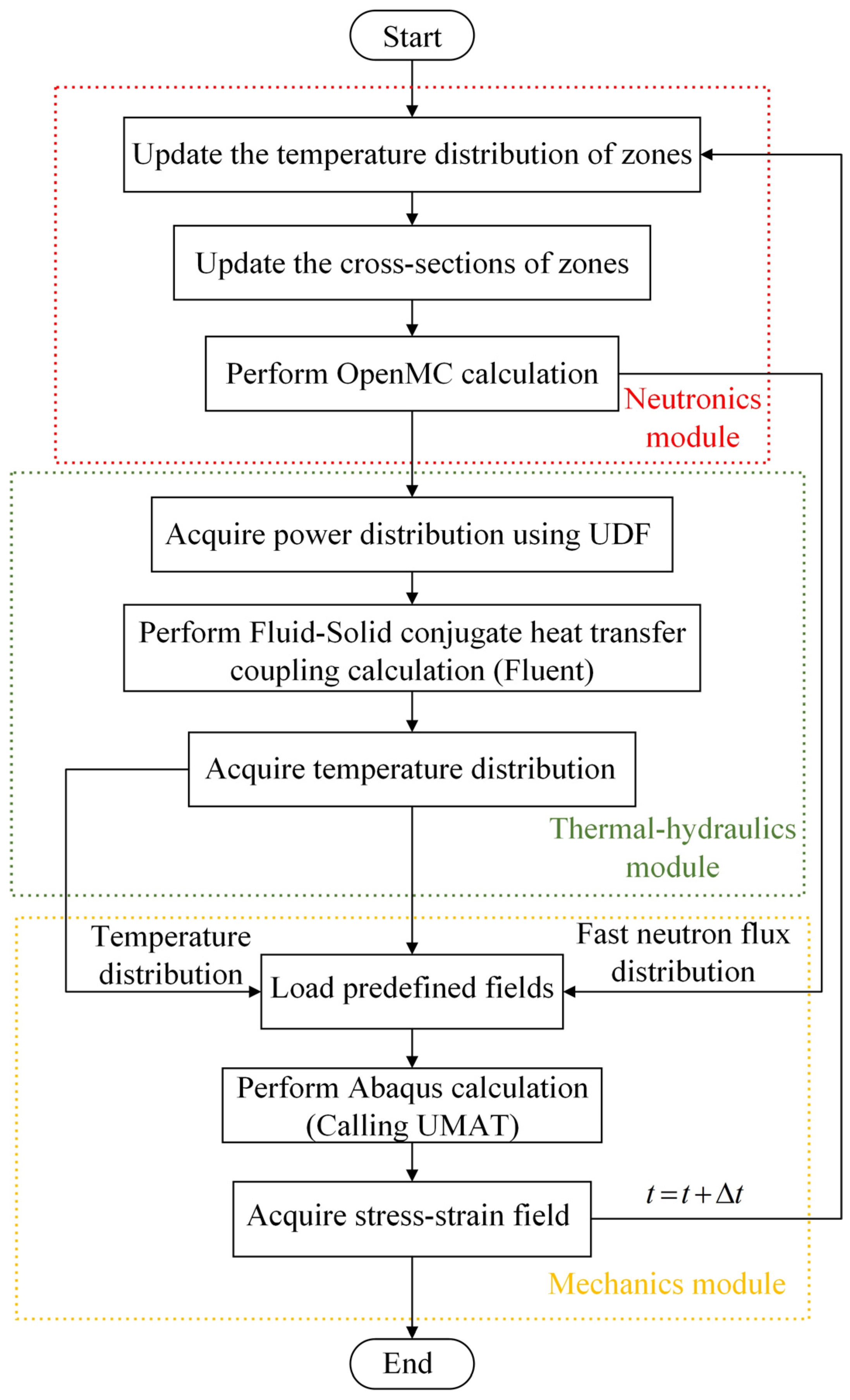
2.2. Coupling Method for Dimensional Change Strain
- OpenMC ran the coupled neutron–photon transport simulation to get the relative power distribution, which was then normalized and multiplied by the known total power to get the actual power density. A power density array was then written to a UDF file as the heat source for Fluent [47]. The fast neutron flux distribution (E > 0.1 MeV) was also tallied.
- After compiling the UDF file, Fluent was launched to start the thermal-hydraulic calculation. The calculation concludes once the temperature and velocity fields satisfy the residual convergence criteria defined by the user. At this point, a precise temperature distribution was derived.
- The array containing the temperature distribution with 3D coordinates was saved into a CSV file. The same processing method was used for the fast neutron flux distribution. Then, the two files were loaded as predefined fields in the load module of Abaqus. Finally, a calculation job was submitted that calls the UMAT subroutine.
- In the neutronics calculation model, the HPA was divided into 320 regions, as shown in Figure 6. The same processing method was used for the RCA. In the neutronics calculation model, each region is composed of multiple meshes for thermal-hydraulic calculation using Fluent. The average temperature for each region was calculated based on the specific material number assigned in DAG-OpenMC [48]. Each region is assigned a unique material number for reference. DAG-OpenMC then read the temperature data to update the properties and cross-sections, assuming that cross sections are accessible at temperatures , and a region material is designated a temperature , where . Statistical interpolation is implemented in the following manner: A random number is generated from a uniform distribution within the range of 0 to 1. If , the cross sections at temperature were utilized. Otherwise, the cross sections at were utilized.
- The above iteration was carried out until calculations were manually stopped.
2.3. Neutronics Model
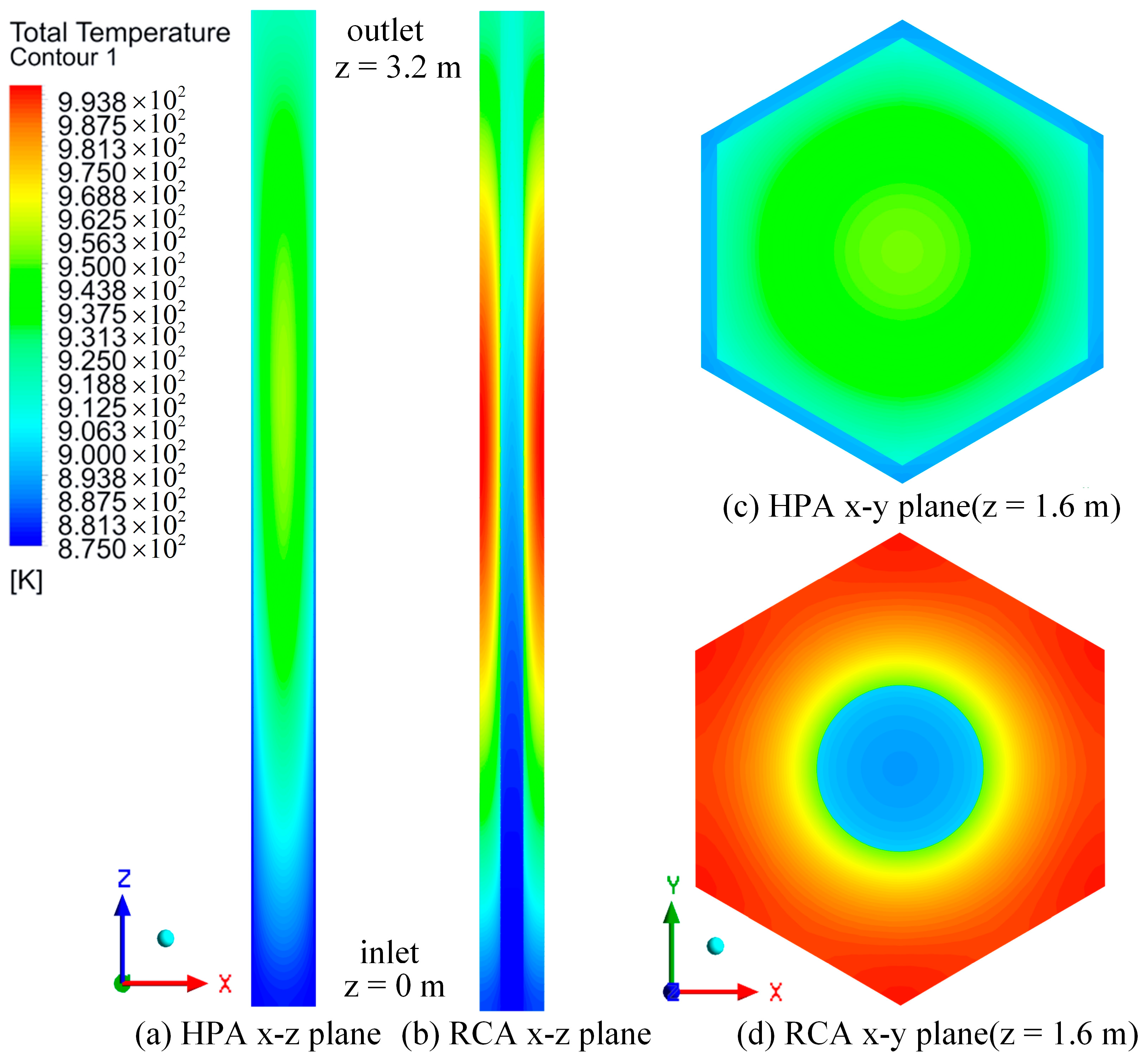
2.4. Thermal-Hydraulics Model
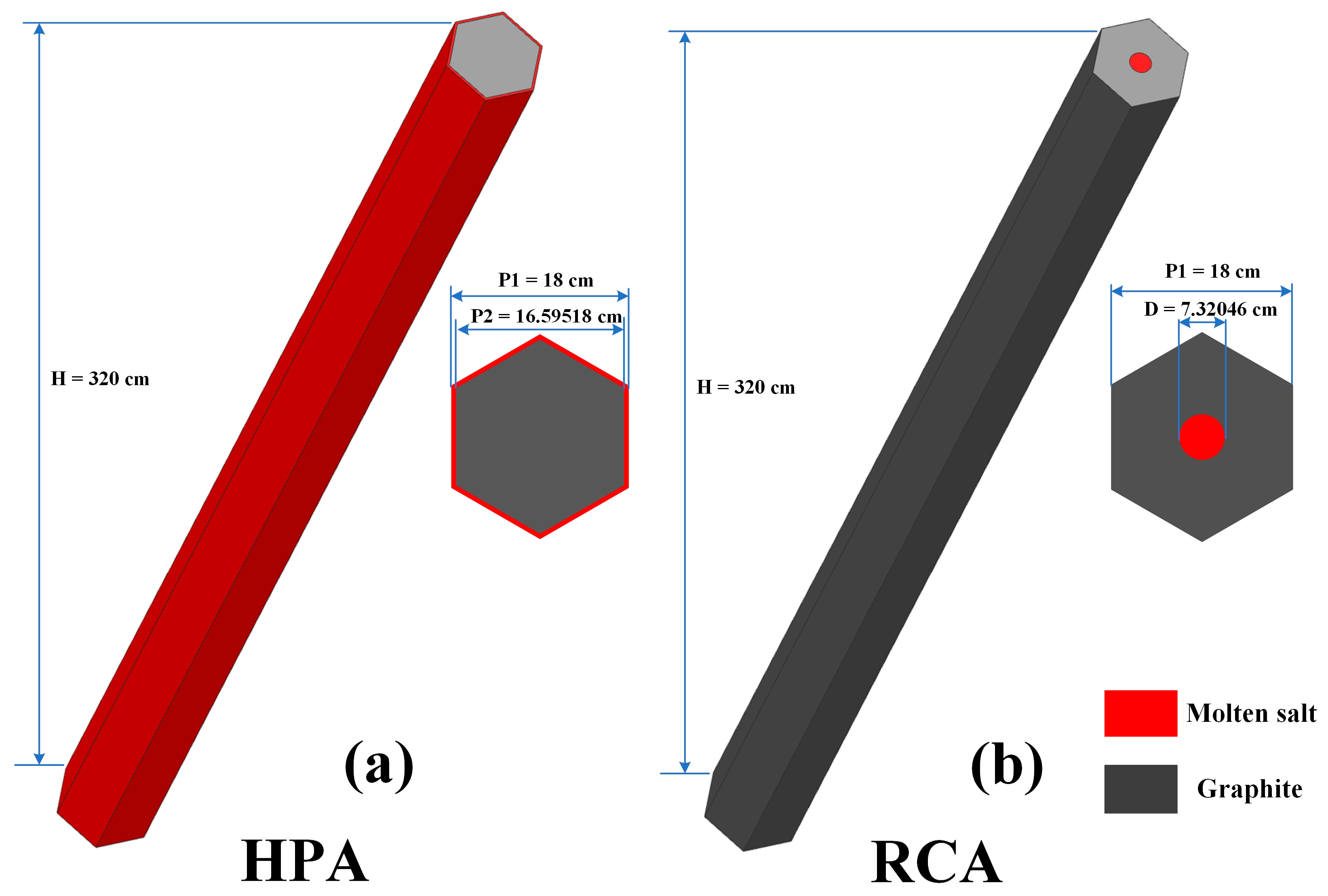
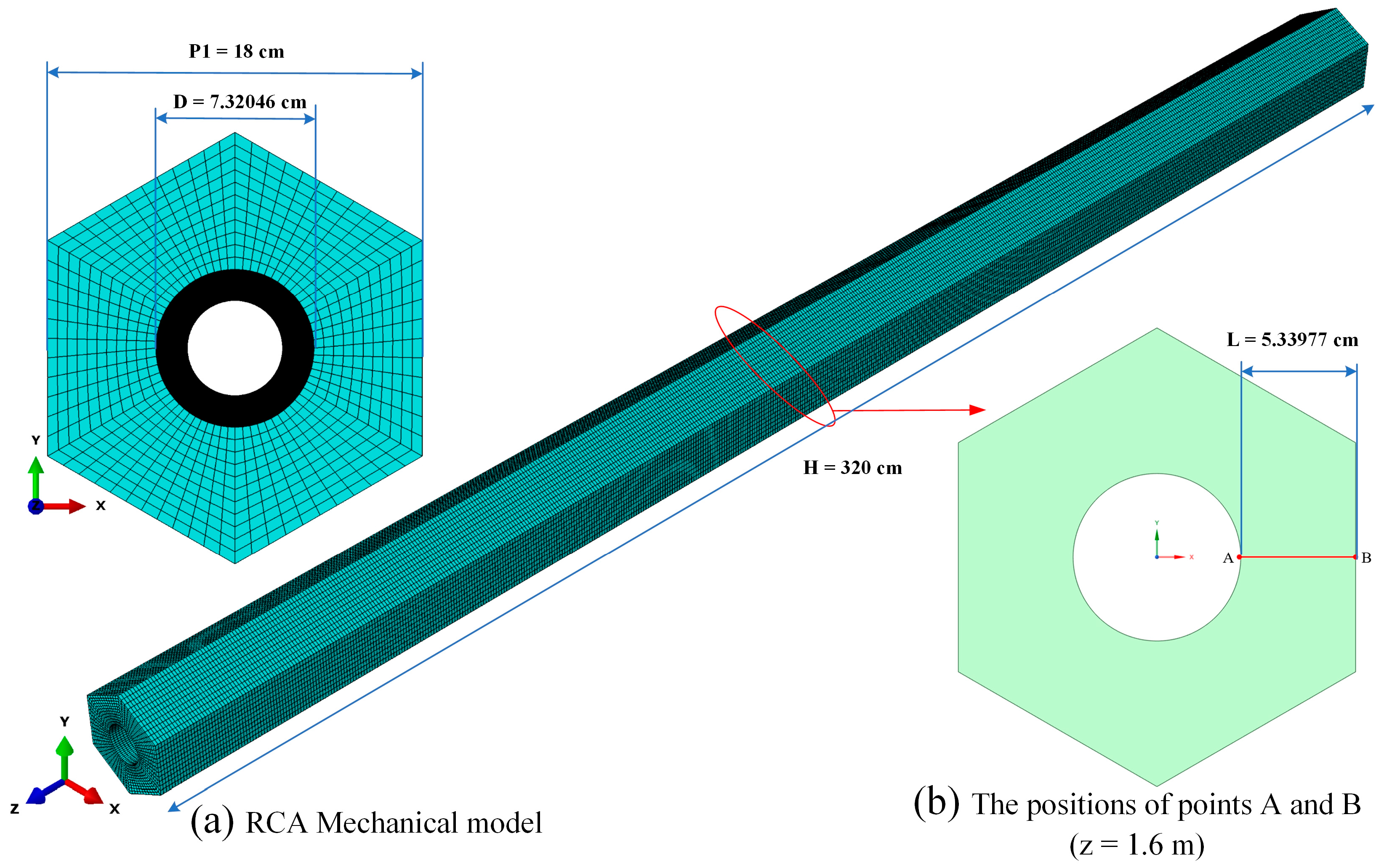
2.5. Mechanical Model
3. Analysis and Discussion
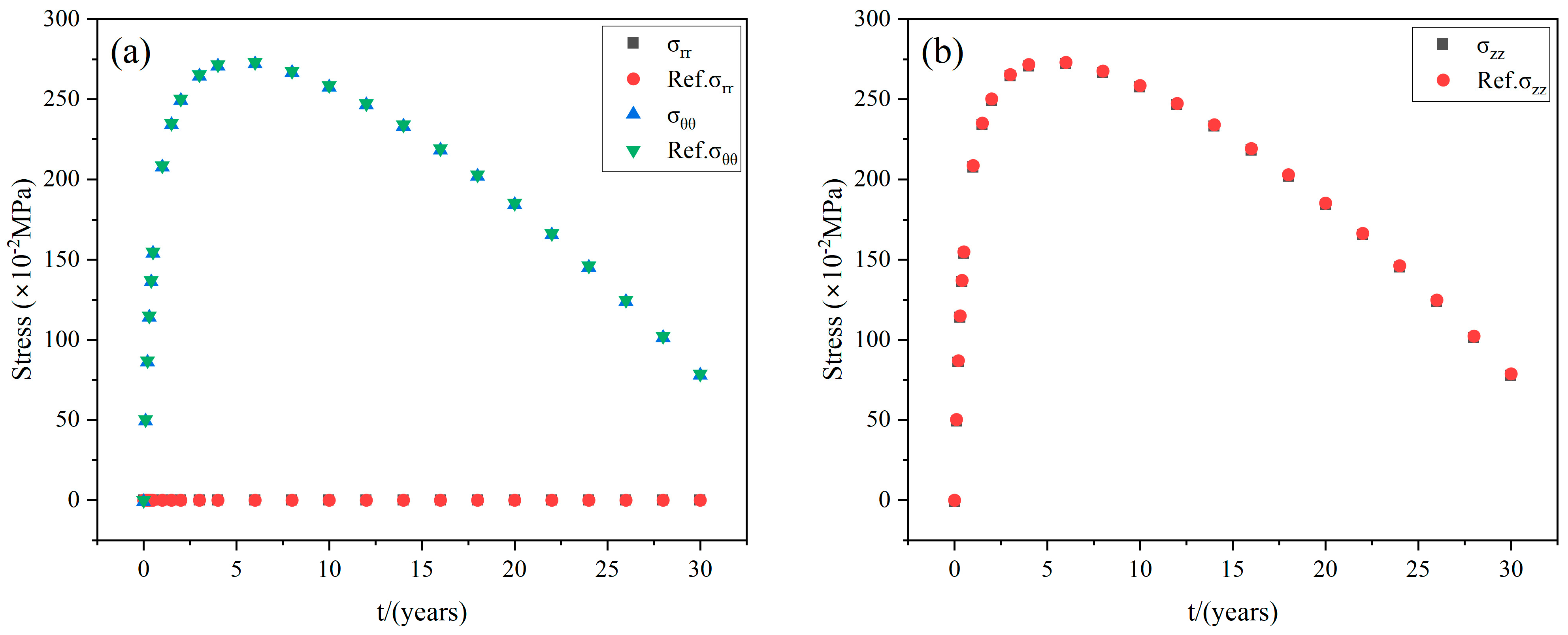
3.1. Verification of UMAT
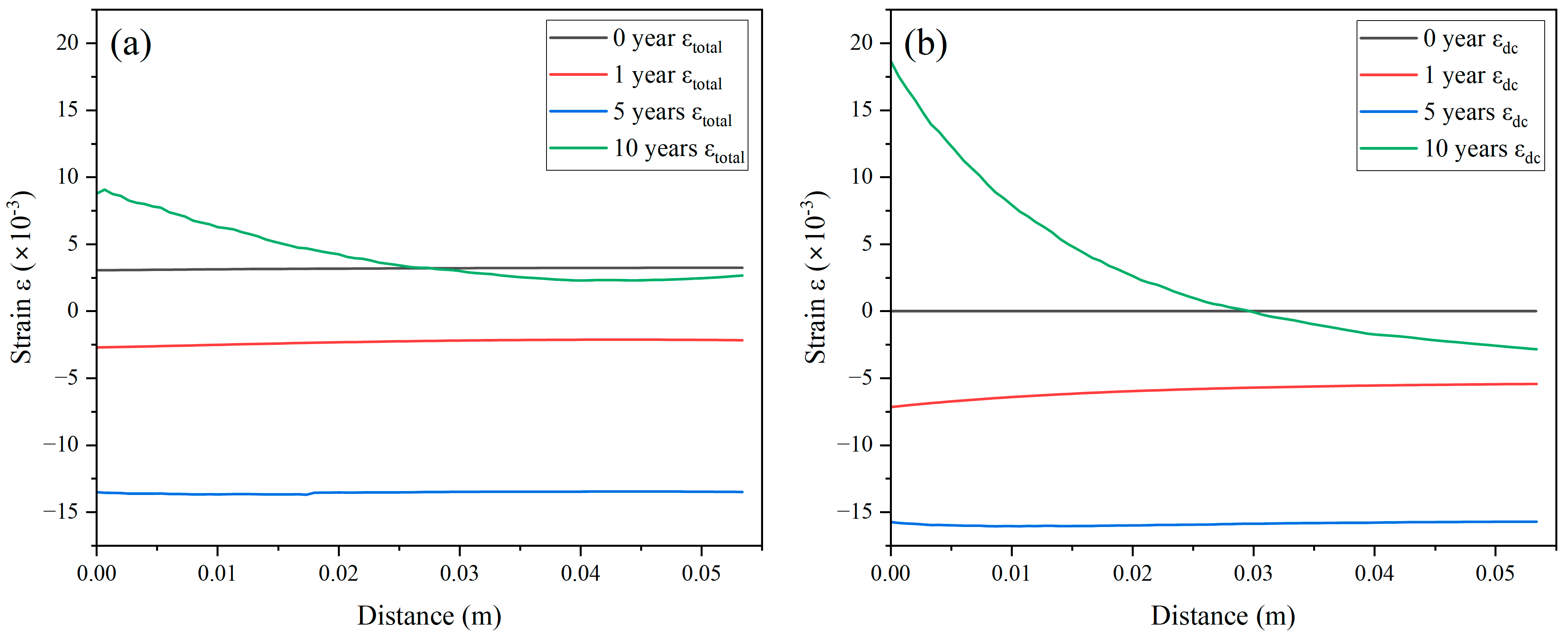
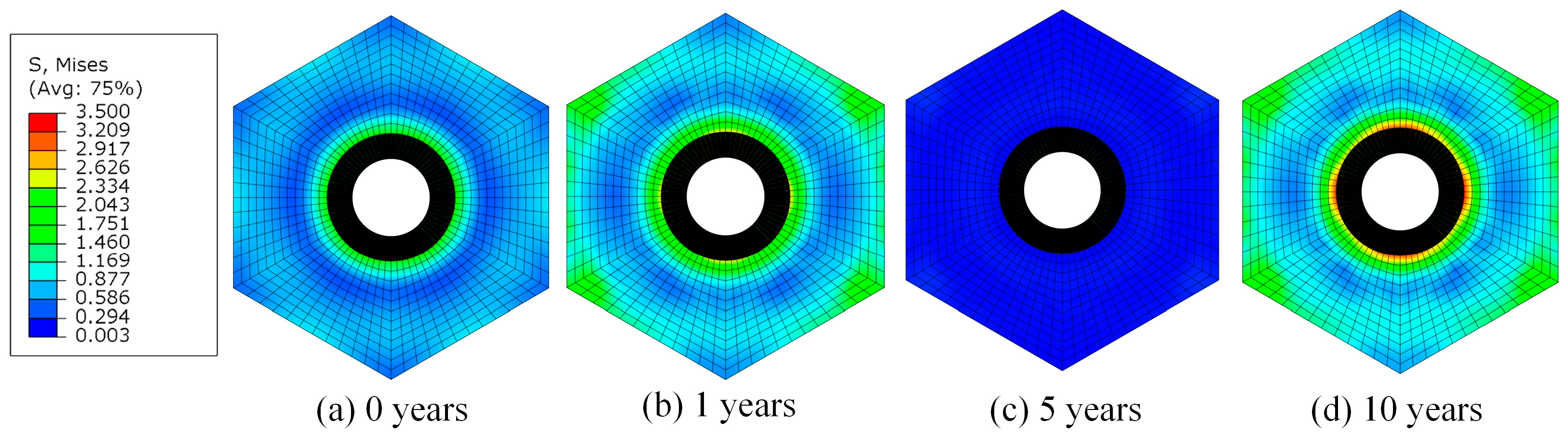
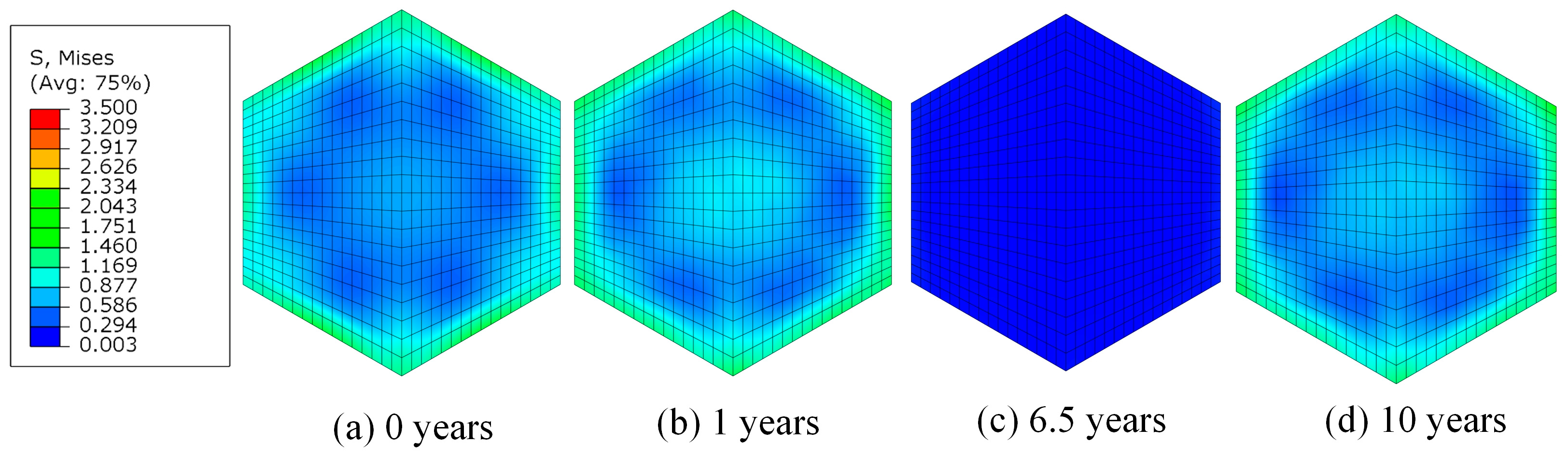
3.2. Analysis of Stress–Strain Field
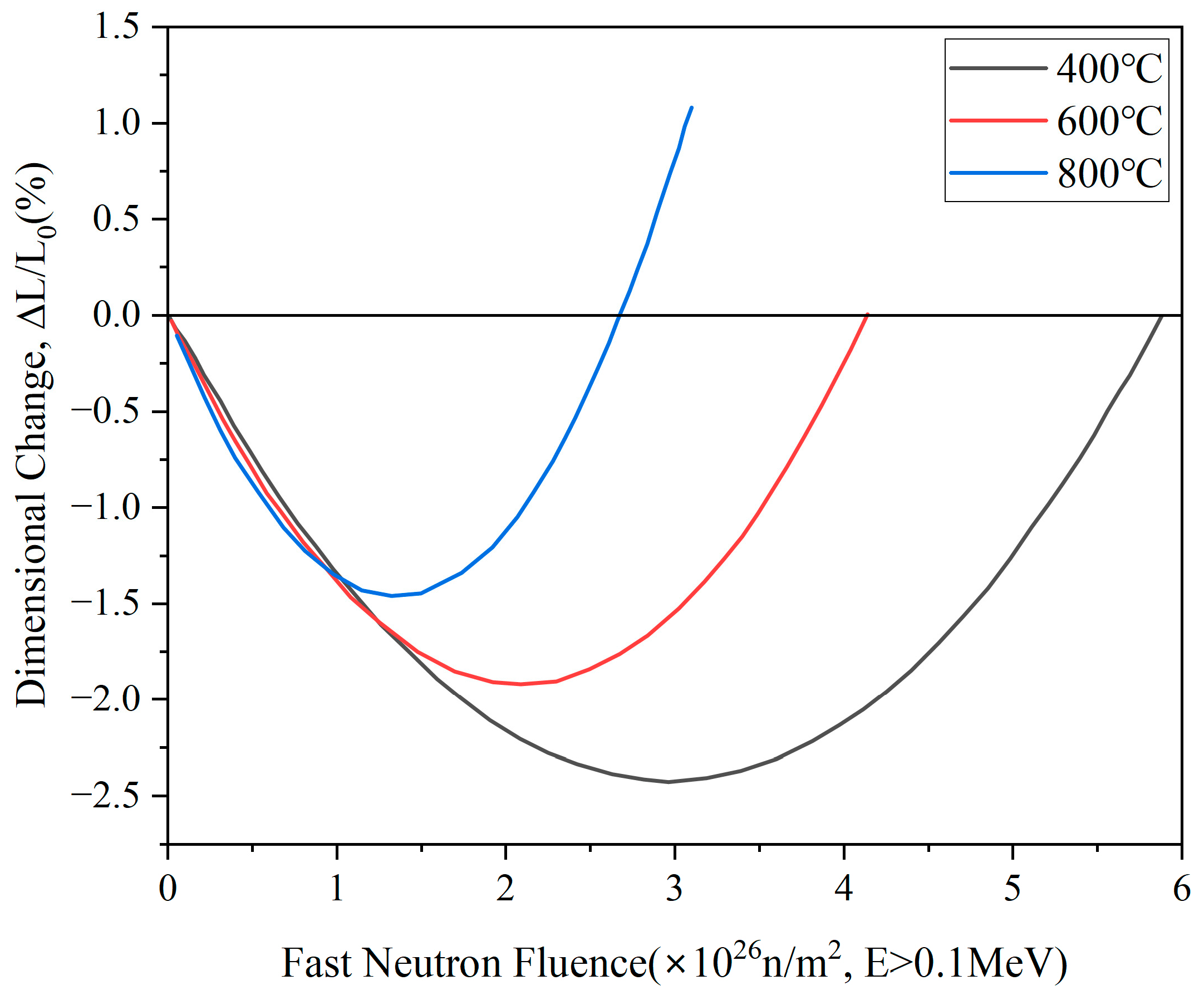
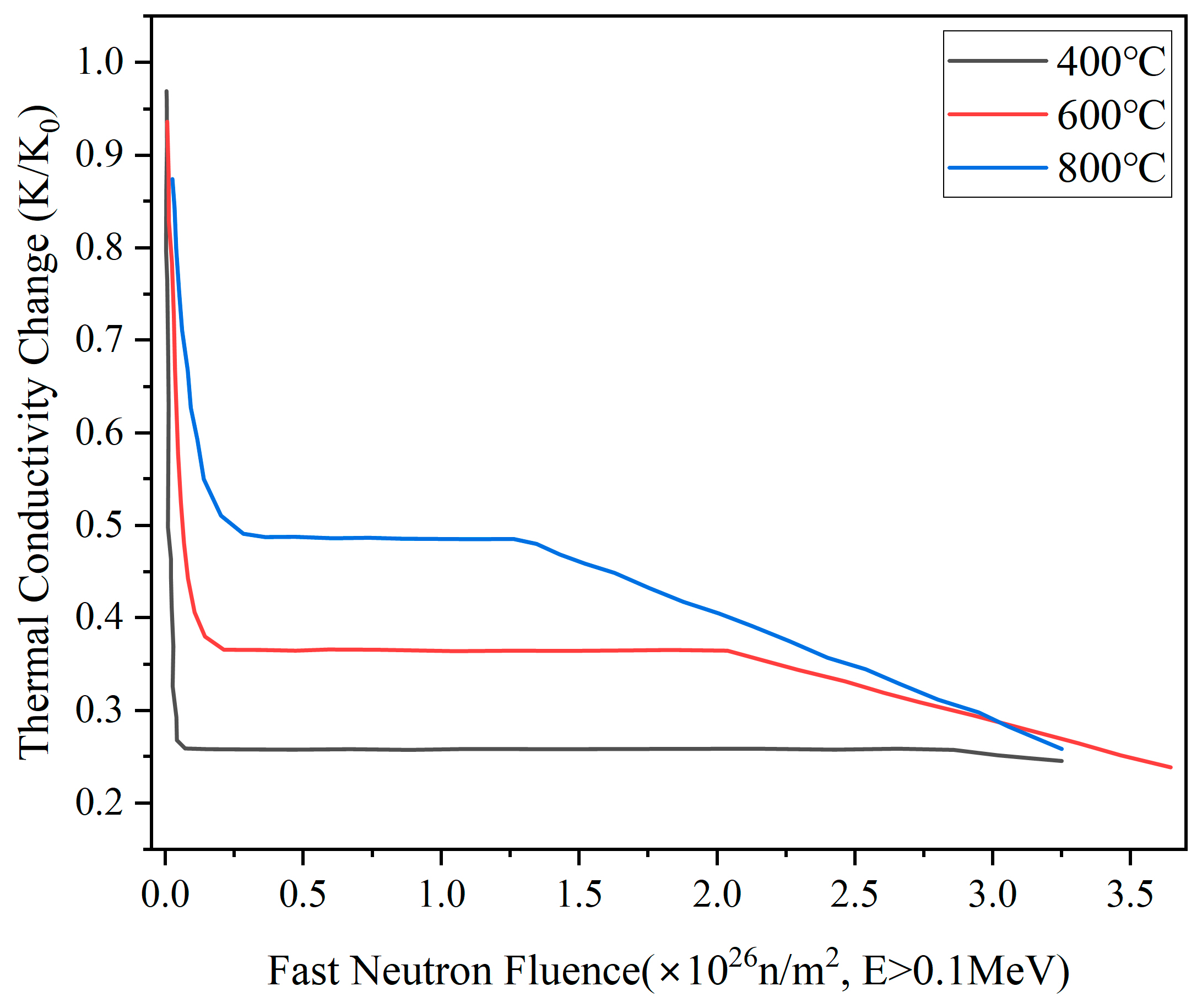
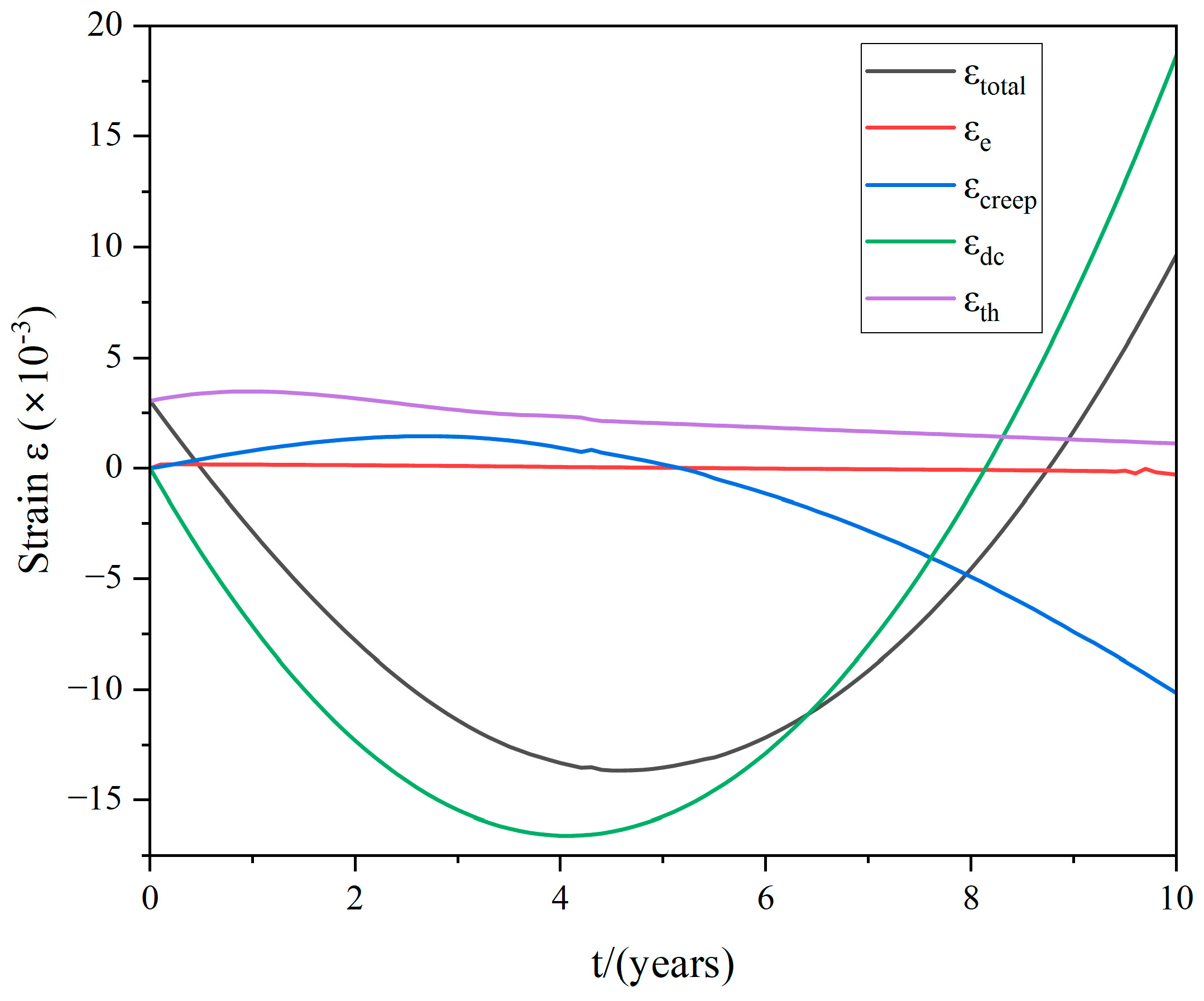
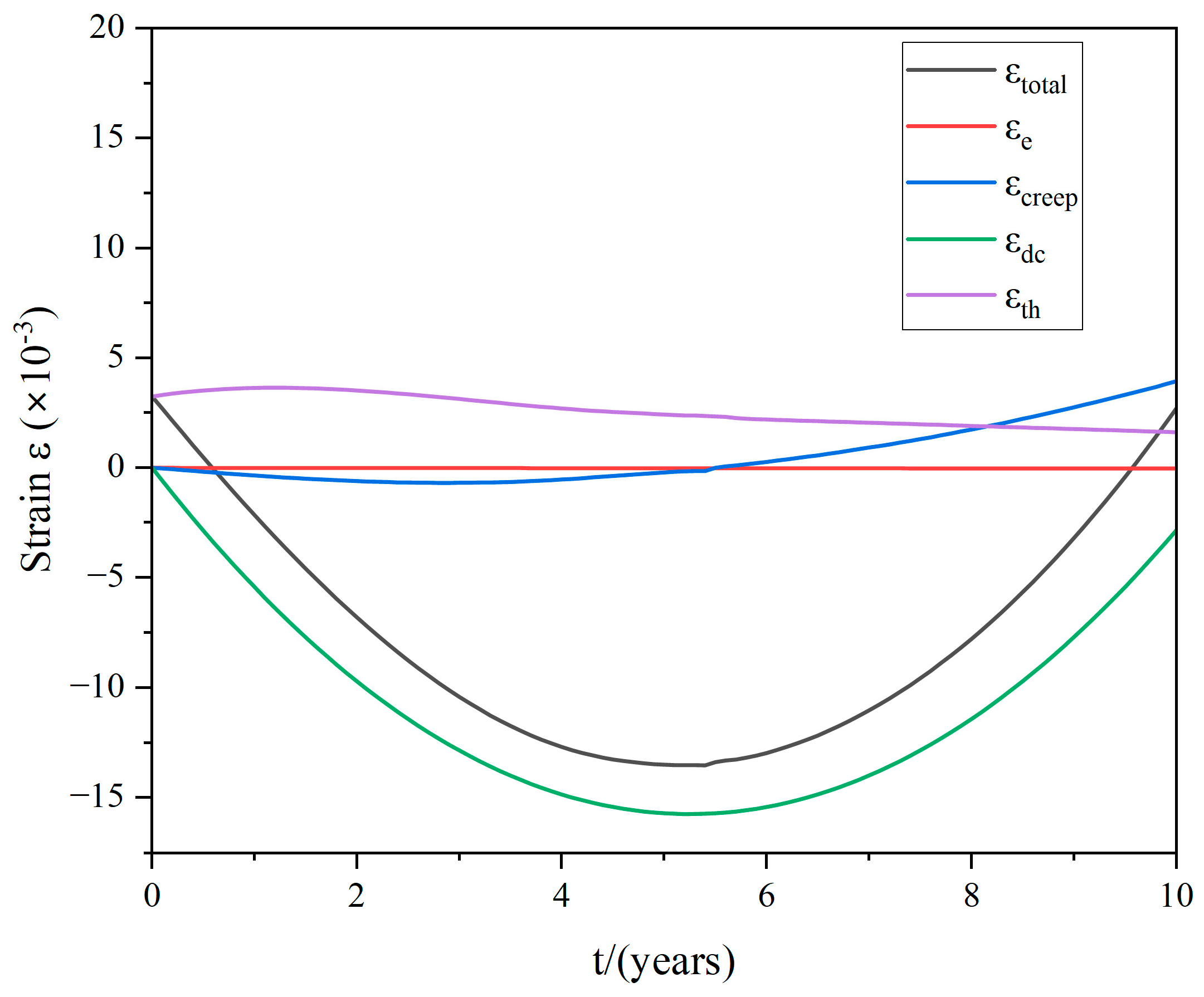
3.3. Analysis of Graphite Lifespan
4. Conclusions
- The factors influencing graphite deformation under high temperatures and irradiation are numerous. These include thermal expansion, irradiation creep, changes in thermo-mechanical physical parameters, and dimensional changes. Simply analyzing graphite lifespan based solely on dimensional changes may lead to inaccuracies. By utilizing an improved method that incorporates a graphite material constitutive model, all these factors can be comprehensively considered. As a result, the calculated graphite lifespan using the improved method is likely to be more accurate. The HPA graphite lifespan is 10 years, and the RCA graphite lifespan is 8.8 years.
- An examination of the behavior of stress–strain and lifespan indicates that dimensional changes play a crucial role in graphite deformation, significantly impacting the overall trend. Furthermore, irradiation creep is shown to reduce stress significantly. The stress field undergoes significant changes as irradiation neutron fluence increases. The peak stress within the graphite component also increases with higher levels of irradiation neutron fluence. When the volume decreases to a minimum, the center of the component experiences uniform deformation with the least amount of stress. For HPA graphite, this stage is expected to be reached in approximately 6.5 years. However, RCA graphite will reach that stage more rapidly.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Serp, J.; Allibert, M.; Beneš, O.; Delpech, S.; Feynberg, O.; Ghetta, V.; Heuer, D.; Holcomb, D.; Ignatiev, V.; Kloosterman, J.L.; et al. The Molten Salt Reactor (MSR) in Generation IV: Overview and Perspectives. Prog. Nucl. Energy 2014, 77, 308–319. [Google Scholar] [CrossRef]
- Ignatiev, V.V. Molten Salt Reactors. In Encyclopedia of Nuclear Energy; Greenspan, E., Ed.; Elsevier: Oxford, UK, 2021; pp. 553–568. ISBN 978-0-12-819732-5. [Google Scholar]
- Doe, U.S. A Technology Roadmap for Generation IV Nuclear Energy Systems. 2002. Available online: https://www.gen-4.org/gif/jcms/c_40481/technology-roadmap (accessed on 10 April 2024).
- Locatelli, G.; Mancini, M.; Todeschini, N. Generation IV Nuclear Reactors: Current Status and Future Prospects. Energy Policy 2013, 61, 1503–1520. [Google Scholar] [CrossRef]
- Kelly, J.E. Generation IV International Forum: A Decade of Progress through International Cooperation. Prog. Nucl. Energy 2014, 77, 240–246. [Google Scholar] [CrossRef]
- Jorgensen, L. 19—ThorCon Reactor. In Molten Salt Reactors and Thorium Energy; Dolan, T.J., Ed.; Woodhead Publishing: Sawston, UK, 2017; pp. 557–564. ISBN 978-0-08-101126-3. [Google Scholar]
- Zhu, G.-F.; Yan, R.; Peng, H.-H.; Ji, R.-M.; Yu, S.-H.; Liu, Y.-F.; Tian, J.; Xu, B. Application of Monte Carlo Method to Calculate the Effective Delayed Neutron Fraction in Molten Salt Reactor. Nucl. Sci. Tech. 2019, 30, 34. [Google Scholar] [CrossRef]
- Carter, J.P.; Borrelli, R.A. Integral Molten Salt Reactor Neutron Physics Study Using Monte Carlo N-Particle Code. Nucl. Eng. Des. 2020, 365, 110718. [Google Scholar] [CrossRef]
- Wu, J.; Chen, J.; Cai, X.; Zou, C.; Yu, C.; Cui, Y.; Zhang, A.; Zhao, H. A Review of Molten Salt Reactor Multi-Physics Coupling Models and Development Prospects. Energies 2022, 15, 8296. [Google Scholar] [CrossRef]
- He, L.; Chen, L.; Xia, S.; Zou, Y. Minor Actinides Transmutation and 233U Breeding in a Closed Th-U Cycle Based on Molten Chloride Salt Fast Reactor. Energies 2022, 15, 9472. [Google Scholar] [CrossRef]
- Peng, M.; Liu, Y.; Zou, Y.; Dai, Y. Preliminary Design and Study of a Small Modular Chlorine Salt Fast Reactor Cooled by Supercritical Carbon Dioxide. Energies 2023, 16, 4862. [Google Scholar] [CrossRef]
- Yu, K.; Cheng, M.; Zuo, X.; Dai, Z. Transmutation and Breeding Performance Analysis of Molten Chloride Salt Fast Reactor Using a Fuel Management Code with Nodal Expansion Method. Energies 2022, 15, 6299. [Google Scholar] [CrossRef]
- Yang, P.; Lin, Z.-K.; Wan, W.; Zhu, G.-F.; Yu, X.-H.; Dai, Z.-M. Preliminary Neutron Study of a Thorium-Based Molten Salt Energy Amplifier. Nucl. Sci. Tech. 2020, 31, 41. [Google Scholar] [CrossRef]
- Zou, C.; Yu, C.; Wu, J.; Cai, X.; Chen, J. Transition to Thorium Fuel Cycle in a Small Modular Molten Salt Reactor Based on a Batch Reprocessing Mode. Ann. Nucl. Energy 2020, 138, 107163. [Google Scholar] [CrossRef]
- Zou, C.; Zhu, G.; Yu, C.; Zou, Y.; Chen, J. Preliminary Study on TRUs Utilization in a Small Modular Th-Based Molten Salt Reactor (smTMSR). Nucl. Eng. Des. 2018, 339, 75–82. [Google Scholar] [CrossRef]
- Zou, C.Y.; Cai, C.Z.; Yu, C.G.; Wu, J.H.; Chen, J.G. Transition to Thorium Fuel Cycle for TMSR. Nucl. Eng. Des. 2018, 330, 420–428. [Google Scholar] [CrossRef]
- Bei, X.; Dai, Y.; Yu, K.; Cheng, M. Three-Dimensional Surrogate Model Based on Back-Propagation Neural Network for Key Neutronics Parameters Prediction in Molten Salt Reactor. Energies 2023, 16, 4044. [Google Scholar] [CrossRef]
- Engel, J.R.; Bauman, H.F.; Dearing, J.F.; Grimes, W.R.; McCoy, H.E.; Rhoades, W.A. Conceptual Design Characteristics of a Denatured Molten-Salt Reactor with Once-Through Fueling; Oak Ridge National Lab: Oak Ridge, TN, USA, 1980.
- Honma, Y.; Shimazu, Y.; Narabayashi, T. Optimization of Flux Distribution in a Molten-Salt Reactor with a 2-Region Core for Plutonium Burning. Prog. Nucl. Energy 2008, 50, 257–261. [Google Scholar] [CrossRef]
- Nuttin, A.; Heuer, D.; Billebaud, A.; Brissot, R.; Le Brun, C.; Liatard, E.; Loiseaux, J.-M.; Mathieu, L.; Meplan, O.; Merle-Lucotte, E.; et al. Potential of Thorium Molten Salt Reactorsdetailed Calculations and Concept Evolution with a View to Large Scale Energy Production. Prog. Nucl. Energy 2005, 46, 77–99. [Google Scholar] [CrossRef]
- Nagy, K.; Kloosterman, J.L.; Lathouwers, D.; van der Hagen, T.H.J.J. The Effects of Core Zoning on the Graphite Lifespan and Breeding Gain of a Moderated Molten Salt Reactor. Ann. Nucl. Energy 2012, 43, 19–25. [Google Scholar] [CrossRef]
- Mitachi, K.; Yamamoto, T.; Yoshioka, R. Three-Region Core Design for 200-MW(Electric) Molten-Salt Reactor with Thorium-Uranium Fuel. Nucl. Technol. 2007, 158, 348–357. [Google Scholar] [CrossRef]
- Neighbour, G.B. Modelling of Dimensional Changes in Irradiated Nuclear Graphites. J. Phys. D Appl. Phys. 2000, 33, 2966. [Google Scholar] [CrossRef]
- Marsden, B.J.; Haverty, M.; Bodel, W.; Hall, G.N.; Jones, A.N.; Mummery, P.M.; Treifi, M. Dimensional Change, Irradiation Creep and Thermal/Mechanical Property Changes in Nuclear Graphite. Int. Mater. Rev. 2016, 61, 155–182. [Google Scholar] [CrossRef]
- Campbell, A.A.; Burchell, T.D. 3.11—Radiation Effects in Graphite☆. In Comprehensive Nuclear Materials, 2nd ed.; Konings, R.J.M., Stoller, R.E., Eds.; Elsevier: Oxford, UK, 2020; pp. 398–436. ISBN 978-0-08-102866-7. [Google Scholar]
- Shibata, T.; Sawa, K.; Eto, M.; Kunimoto, E.; Shiozawa, S.; Oku, T.; Maruyama, T. Draft of Standard for Graphite Core Components in High Temperature Gas-Cooled Reactor; Japan Atomic Energy Agency: Tokai, Japan, 2010.
- Kasten, P.R.; Bettis, E.S.; Cook, W.H.; Eatherly, W.P.; Holmes, D.K.; Kedl, R.J.; Kennedy, C.R.; Kirslis, S.S.; McCoy, H.E.; Perry, A.M.; et al. Graphite Behavior and Its Effects on MSBR Performance. Nucl. Eng. Des. 1969, 9, 157–195. [Google Scholar] [CrossRef]
- Kelly, B.T. Irradiation Behavior of Fine-Grain Graphites. Fusion Technol. 1989, 16, 96–103. [Google Scholar] [CrossRef]
- Zhu, G.; Guo, W.; Kang, X.; Zou, C.; Zou, Y.; Yan, R.; Dai, Y. Neutronic Effect of Graphite Dimensional Change in a Small Modular Molten Salt Reactor. Int. J. Energy Res. 2021, 45, 11976–11991. [Google Scholar] [CrossRef]
- Wang, Y.; Guo, W.; Zhu, G.; Dai, Y.; Zhong, Y.; Zou, Y.; Chen, J.; Cai, X. A New Structure Design to Extend Graphite Assembly Lifespan in Small Modular Molten Salt Reactors. Int. J. Energy Res. 2021, 45, 12247–12257. [Google Scholar] [CrossRef]
- Platonov, P.A.; Chugunov, O.K.; Manevsky, V.N.; Karpukhin, V.I. Radiation Damage and Life-Time Evaluation of RBMK Graphite Stack. In Proceedings of the Graphite Moderator Lifecycle Behaviour, Bath, UK, 24–27 September 1995; pp. 79–90. [Google Scholar]
- Oku, T.; Ishihara, M. Lifetime Evaluation of Graphite Components for HTGRs. Nucl. Eng. Des. 2004, 227, 209–217. [Google Scholar] [CrossRef]
- Marsden, B.J.; Jones, A.N.; Hall, G.N.; Treifi, M.; Mummery, P.M. 14—Graphite as a Core Material for Generation IV Nuclear Reactors. In Structural Materials for Generation IV Nuclear Reactors; Yvon, P., Ed.; Woodhead Publishing: Sawston, UK, 2017; pp. 495–532. ISBN 978-0-08-100906-2. [Google Scholar]
- Chang, T.Y.; Rashid, Y.R. Viscoelastic Response of Graphitic Materials in Irradiation Environments. Nucl. Eng. Des. 1970, 14, 181–190. [Google Scholar] [CrossRef]
- Smith, P.D.; Pelessone, D. Consistent Linearization Method for Finite-Element Analysis of Viscoelastic Materials. In Proceedings of the 7th International Conference on Structural Mechanics in Reactor Technology, Chicago, IL, USA, 22–26 August 1983. [Google Scholar]
- Elcoate, C.D.; Payne, J.F.B. Pile Grade A Graphite—Constitutive Modelling. 2001. Available online: https://repository.lib.ncsu.edu/server/api/core/bitstreams/261d0537-a980-4f59-b32d-264fa2c08d51/content (accessed on 10 April 2024).
- Li, H.; Marsden, B.J.; Fok, S.L. Relationship between Nuclear Graphite Moderator Brick Bore Profile Measurement and Irradiation-Induced Dimensional Change. Nucl. Eng. Des. 2004, 232, 237–247. [Google Scholar] [CrossRef]
- Smith, M. ABAQUS/Standard User’s Manual, Version 6.9; 2009. Available online: http://orpheus.nchc.org.tw:2080/v6.12/index.html (accessed on 10 April 2024).
- Tsang, D.K.L.; Marsden, B.J. The Development of a Stress Analysis Code for Nuclear Graphite Components in Gas-Cooled Reactors. J. Nucl. Mater. 2006, 350, 208–220. [Google Scholar] [CrossRef]
- Tsang, D.K.L.; Marsden, B.J. Effects of Dimensional Change Strain in Nuclear Graphite Component Stress Analysis. Nucl. Eng. Des. 2007, 237, 897–904. [Google Scholar] [CrossRef]
- Mohanty, S.; Majumdar, S. Finite Element Based Stress Analysis of Graphite Component in High Temperature Gas Cooled Reactor Core Using Linear and Nonlinear Irradiation Creep Models. Nucl. Eng. Des. 2015, 292, 32–38. [Google Scholar] [CrossRef]
- Fahad, M.; McNally, K.; Yates, T.; Warren, N.; Marsden, B.J.; Mummery, P.M.; Hall, G.N. Finite Element Modelling of Multilayer Advanced Gas-Cooled Reactor Bricks and Creep Interaction. Nucl. Eng. Des. 2017, 324, 390–401. [Google Scholar] [CrossRef]
- McNally, K.; Yates, T.; Fahad, M.; Marsden, B.J.; Warren, N.; Hall, G.N. Design and Analysis of a Computer Experiment for the Study of the Distortion of an Advanced Gas-Cooled Reactor Moderator Brick. Nucl. Eng. Des. 2020, 367, 110766. [Google Scholar] [CrossRef]
- Gao, J.; Yao, W.; Ma, Y. Analytical and Numerical Study of Graphite IG110 Parts in Advanced Reactor under High Temperature and Irradiation. Nucl. Eng. Des. 2016, 305, 421–432. [Google Scholar] [CrossRef]
- Yang, X.; Gao, Y.-T.; Zhong, Y.; Ding, D.; Tsang, D.-K.-L. Stress Analysis of the TMSR Graphite Component under Irradiation Conditions. Nucl. Sci. Tech. 2018, 29, 173. [Google Scholar] [CrossRef]
- Romano, P.K.; Horelik, N.E.; Herman, B.R.; Nelson, A.G.; Forget, B.; Smith, K. OpenMC: A State-of-the-Art Monte Carlo Code for Research and Development. Ann. Nucl. Energy 2015, 82, 90–97. [Google Scholar] [CrossRef]
- ANSYS, Inc. ANSYS FLUENT Theory Guide; ANSYS Inc Canonsburg: Canonsburg, PA, USA, 2013; pp. 90311–90312. [Google Scholar]
- Wilson, P.P.H.; Tautges, T.J.; Kraftcheck, J.A.; Smith, B.M.; Henderson, D.L. Acceleration Techniques for the Direct Use of CAD-Based Geometry in Fusion Neutronics Analysis. Fusion Eng. Des. 2010, 85, 1759–1765. [Google Scholar] [CrossRef]
- Kirk, B.S.; Peterson, J.W.; Stogner, R.H.; Carey, G.F. libMesh: A C++ Library for Parallel Adaptive Mesh Refinement/Coarsening Simulations. Eng. Comput. 2006, 22, 237–254. [Google Scholar] [CrossRef]
- Brown, D.A.; Chadwick, M.B.; Capote, R.; Kahler, A.C.; Trkov, A.; Herman, M.W.; Sonzogni, A.A.; Danon, Y.; Carlson, A.D.; Dunn, M. ENDF/B-VIII. 0: The 8th Major Release of the Nuclear Reaction Data Library with CIELO-Project Cross Sections, New Standards and Thermal Scattering Data. Nucl. Data Sheets 2018, 148, 1–142. [Google Scholar] [CrossRef]
- Kahler III, A.C.; Macfarlane, R. NJOY2016; Los Alamos National Lab (LANL): Los Alamos, NM, USA, 2016.
- Sabharwall, P.; Aufiero, M.; Fratoni, M. 11—Heat Transfer and Computational Fluid Dynamics for Molten Salt Reactor Technologies. In Advances of Computational Fluid Dynamics in Nuclear Reactor Design and Safety Assessment; Joshi, J.B., Nayak, A.K., Eds.; Woodhead Publishing Series in Energy; Woodhead Publishing: Sawston, UK, 2019; pp. 801–834. ISBN 978-0-08-102337-2. [Google Scholar]






| Temperature () | ||
|---|---|---|
| 400 | 0.279 | −1.64 |
| 600 | 0.450 | −1.86 |
| 800 | 0.821 | −2.19 |
| Temperature () | ||||||
|---|---|---|---|---|---|---|
| 400 | 0.133 | −0.683 | 0.800 | −0.103 | 1.070 | 2.81 |
| 600 | 0.264 | −0.983 | 0.796 | −0.134 | 1.010 | 2.05 |
| 800 | 0.628 | −1.570 | 0.738 | −0.164 | 0.896 | 1.30 |
| Temperature () | ||||||
|---|---|---|---|---|---|---|
| 400 | −0.1690 | 1.160 | −0.456 | −3.92 | 2.29 | 2.7 |
| 600 | −0.0745 | 0.889 | −0.684 | −2.75 | 1.66 | 1.8 |
| 800 | −0.0403 | 0.726 | −0.845 | −1.93 | 1.04 | 1.4 |
| Parameter | HPA | RCA |
|---|---|---|
| Fuel salt | LiF-BeF2-ThF4-UF4 (68–26–5–1 mol%) | LiF-BeF2-ThF4-UF4 (68–26–5–1 mol%) |
| Power density (MW/m3) | 100 | 100 |
| Graphite density (kg/m3) | 1780 | 1780 |
| Pitch size (cm) | 18 | 18 |
| Fuel volume fraction (%) | 15 | 15 |
| Height (cm) | 320 | 320 |
| Parameter | Graphite | Fuel Salt |
|---|---|---|
| Type | IG-110 | LiF-BeF2-ThF4-UF4 (68–26–5–1 mol%) |
| Density (kg/m3) | 1780 | |
| Specific heat (J/kg/K−1) | 710 | 2207.49 |
| Thermal conductivity (W/m/K−1) | 116 (BOL) | |
| Viscosity (Pa·s) | ||
| Poisson’s ratio | 0.3 | |
| Thermal expansion coefficient (K−1) | 4.5 10−6 | |
| Elastic modulus (GPa) | 9.8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhong, Y.; Zou, C.; Wang, Q.; Zhu, G.; Guo, W.; Wang, Z. Comparative Irradiated Dimensional Change Strain Analyses of Two Types of Graphite Components in a Thorium Molten Salt Reactor. Energies 2024, 17, 2469. https://doi.org/10.3390/en17112469
Zhong Y, Zou C, Wang Q, Zhu G, Guo W, Wang Z. Comparative Irradiated Dimensional Change Strain Analyses of Two Types of Graphite Components in a Thorium Molten Salt Reactor. Energies. 2024; 17(11):2469. https://doi.org/10.3390/en17112469
Chicago/Turabian StyleZhong, Yu, Chunyan Zou, Qi Wang, Guifeng Zhu, Wei Guo, and Zhichao Wang. 2024. "Comparative Irradiated Dimensional Change Strain Analyses of Two Types of Graphite Components in a Thorium Molten Salt Reactor" Energies 17, no. 11: 2469. https://doi.org/10.3390/en17112469
APA StyleZhong, Y., Zou, C., Wang, Q., Zhu, G., Guo, W., & Wang, Z. (2024). Comparative Irradiated Dimensional Change Strain Analyses of Two Types of Graphite Components in a Thorium Molten Salt Reactor. Energies, 17(11), 2469. https://doi.org/10.3390/en17112469







