Magnetic and Thermal Behavior of a Planar Toroidal Transformer
Abstract
1. Introduction
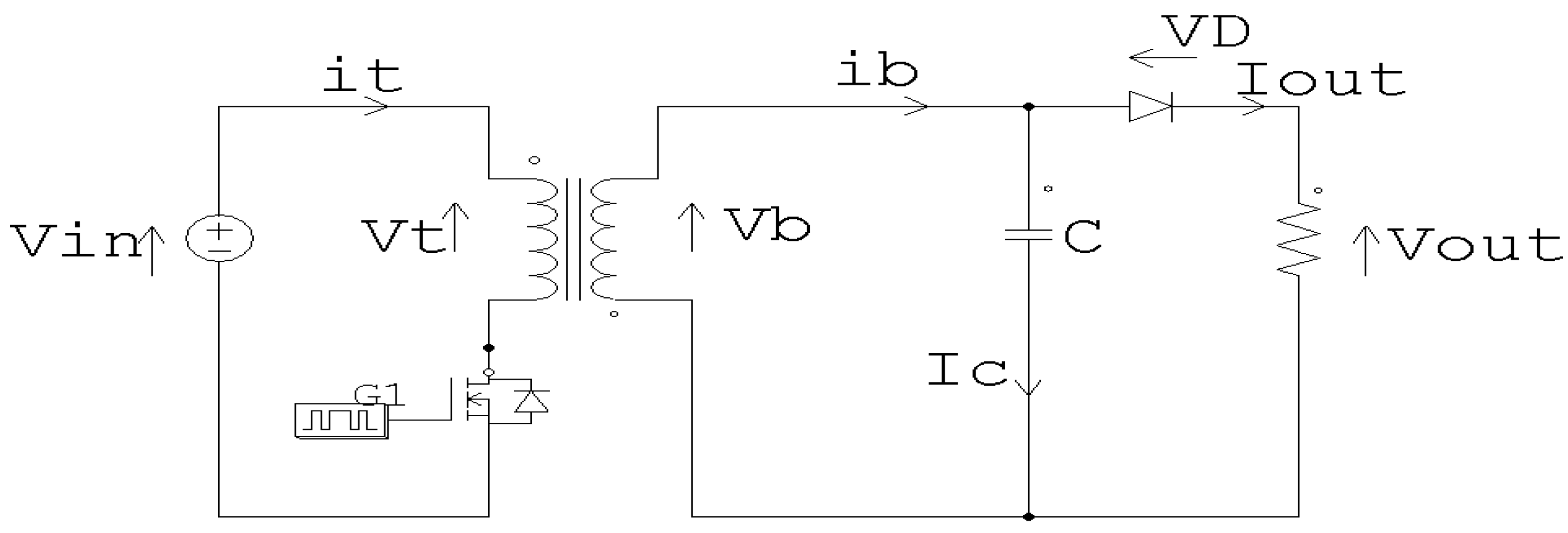
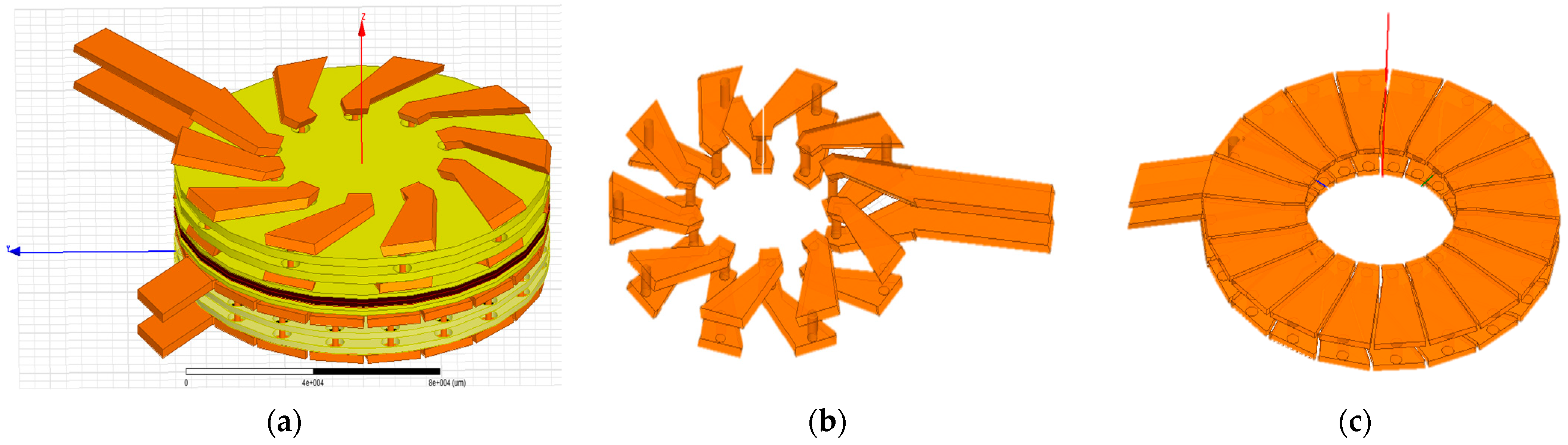
2. Materials and Methods
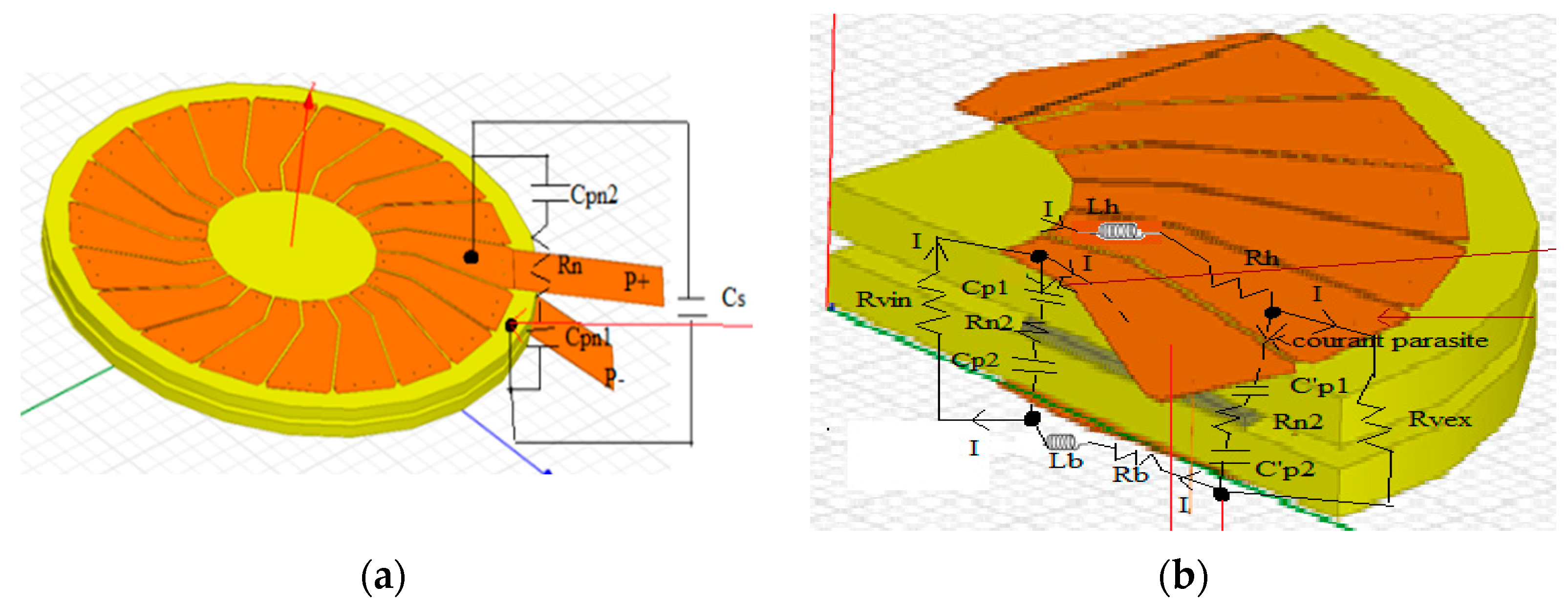
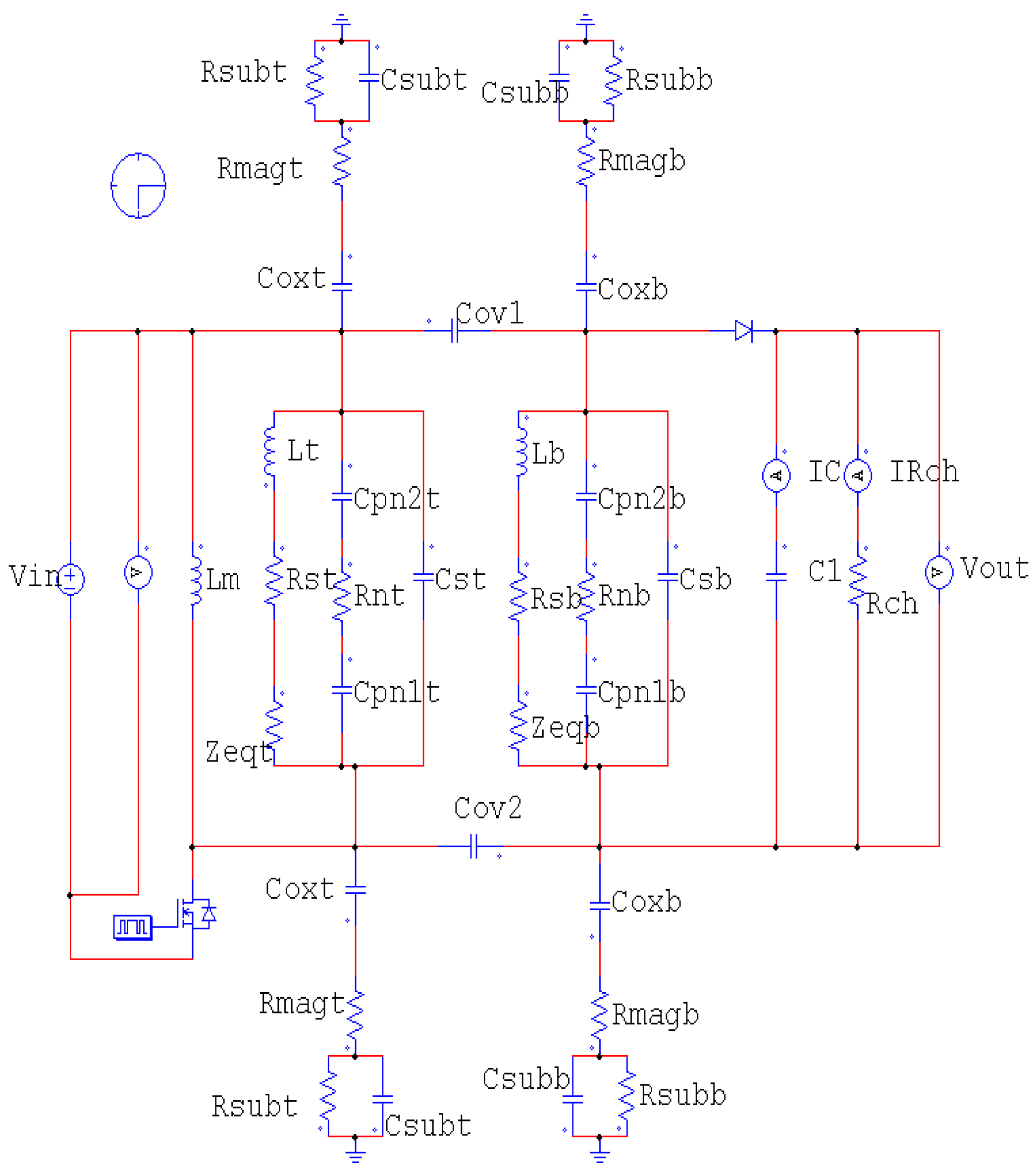
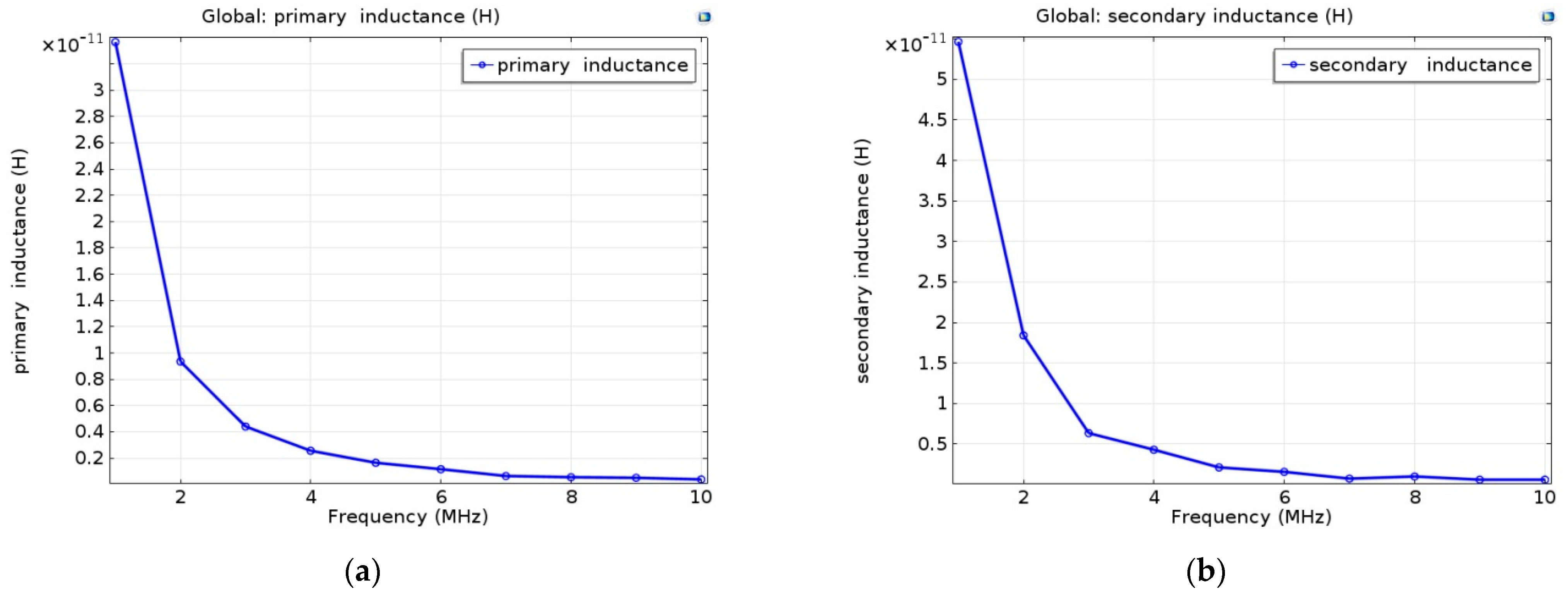
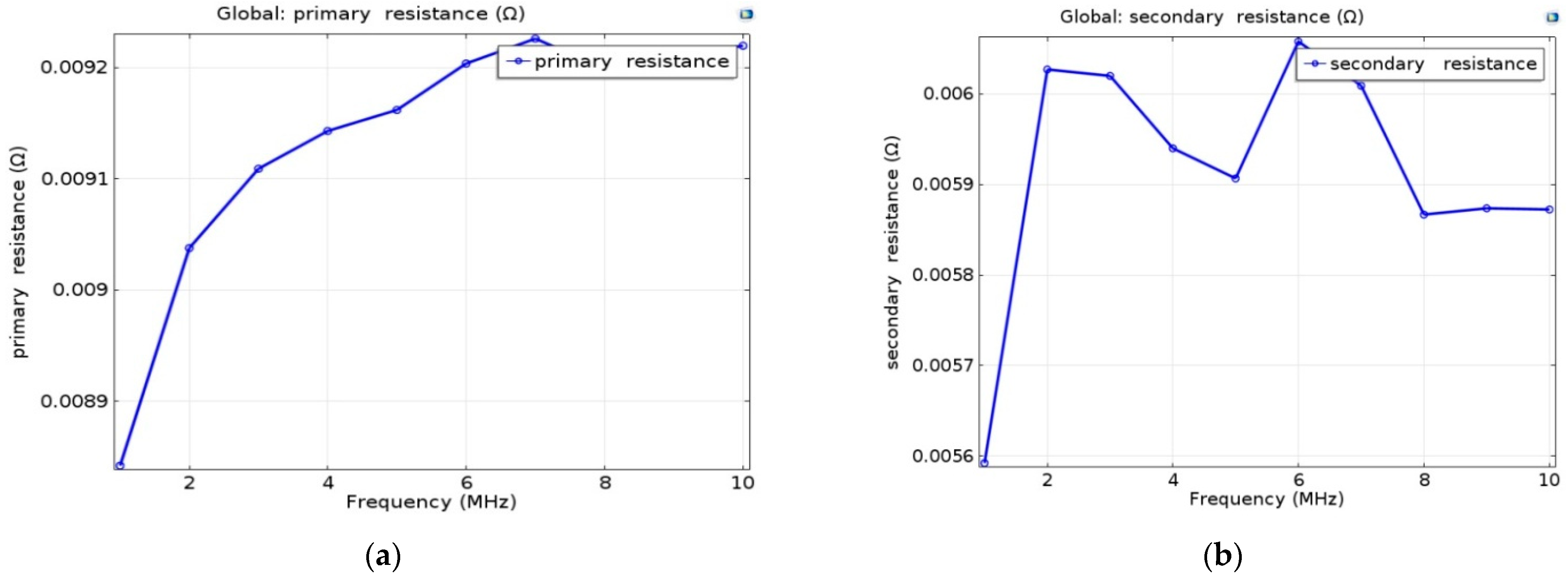
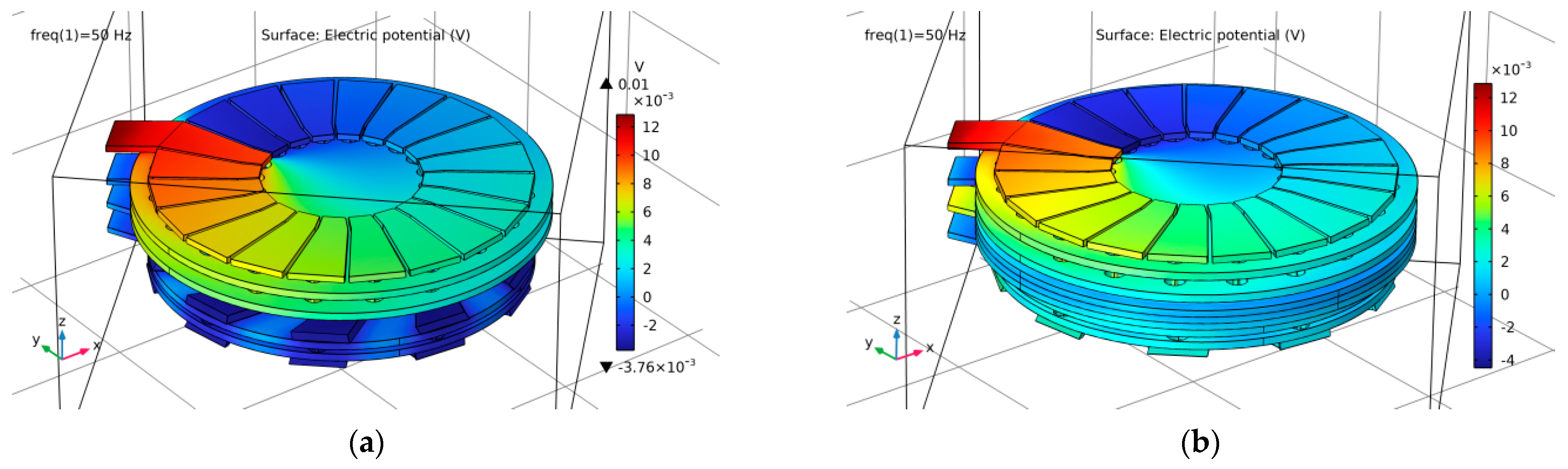
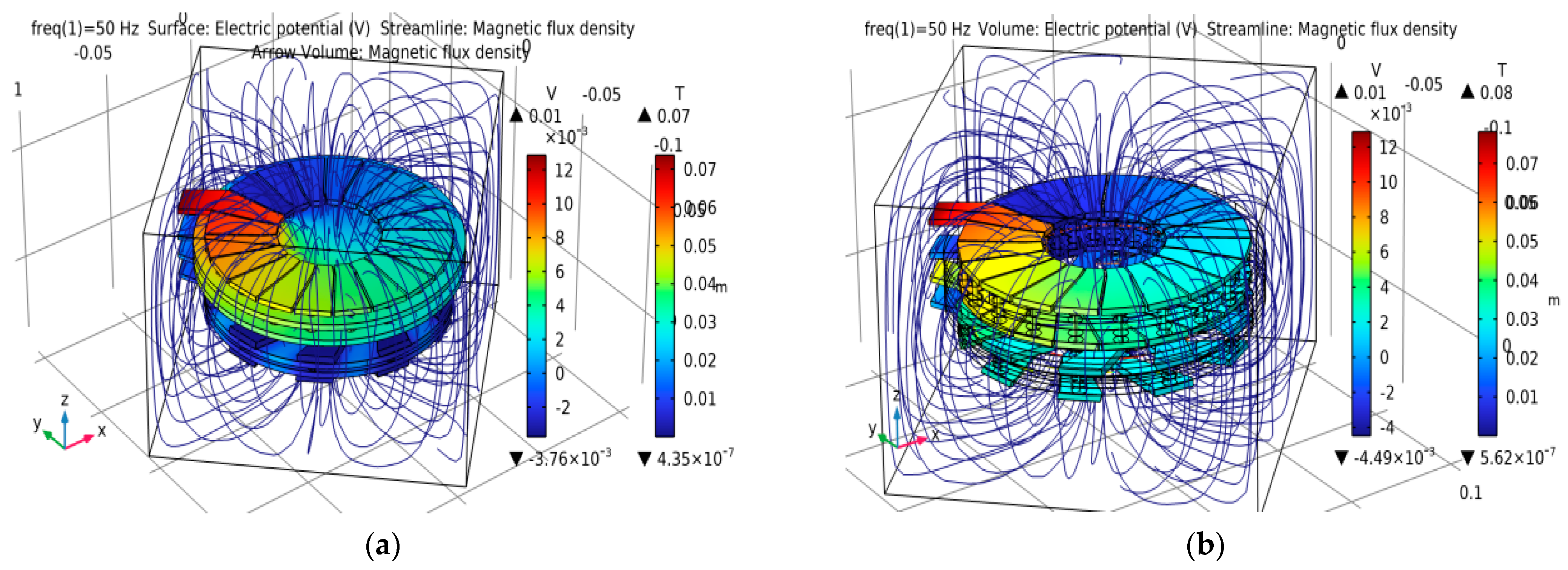
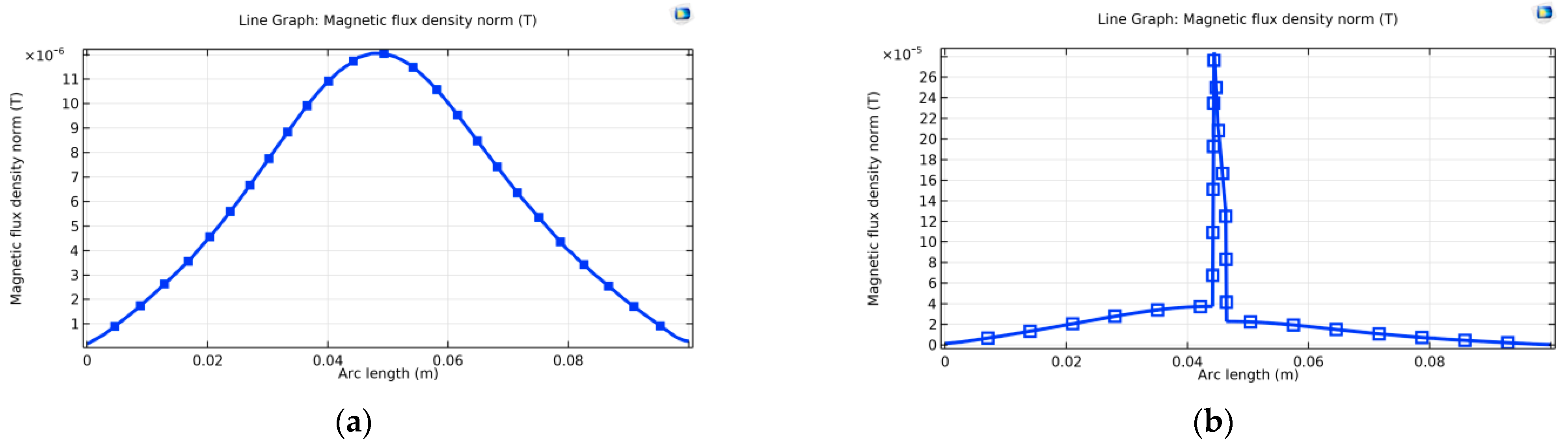
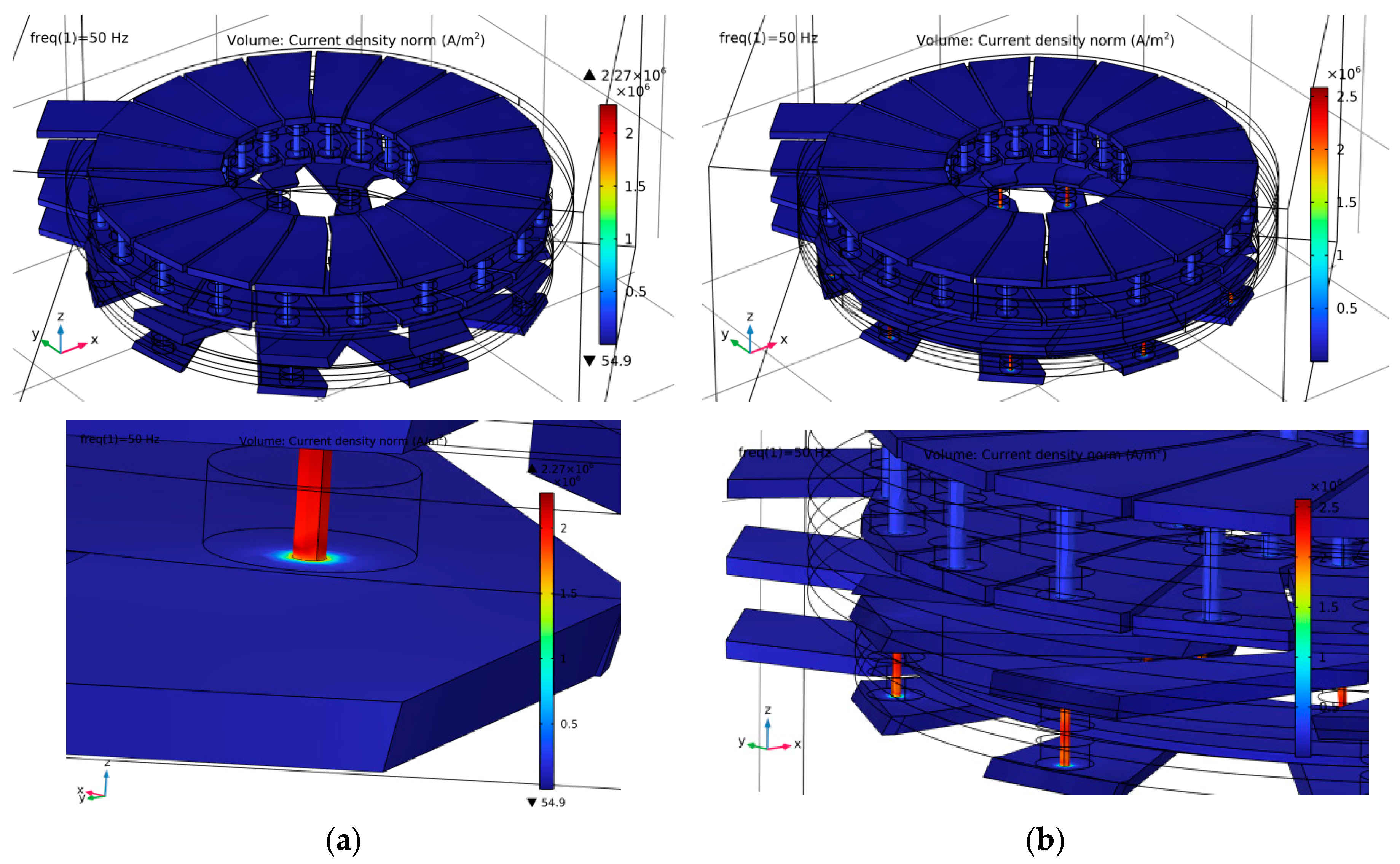
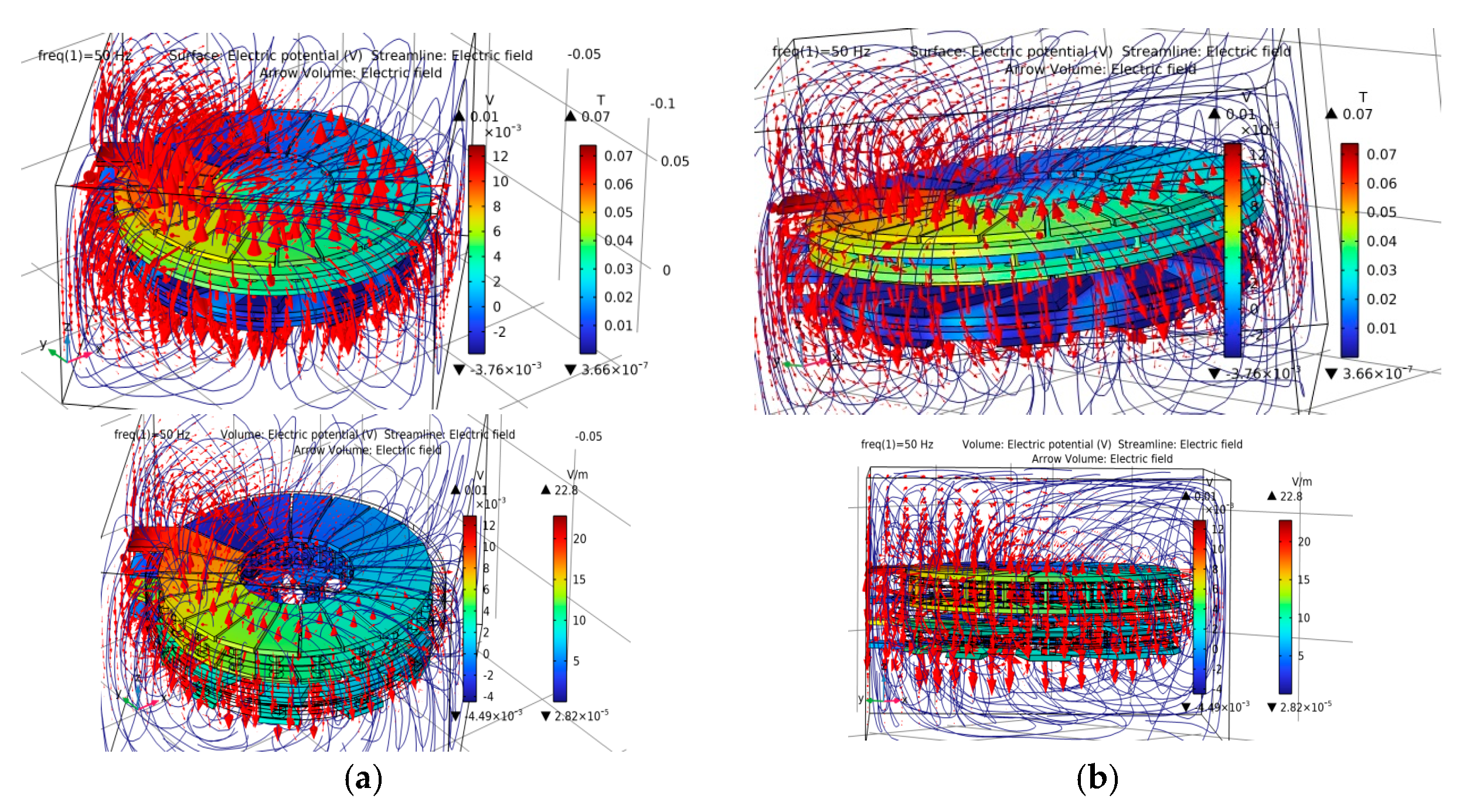
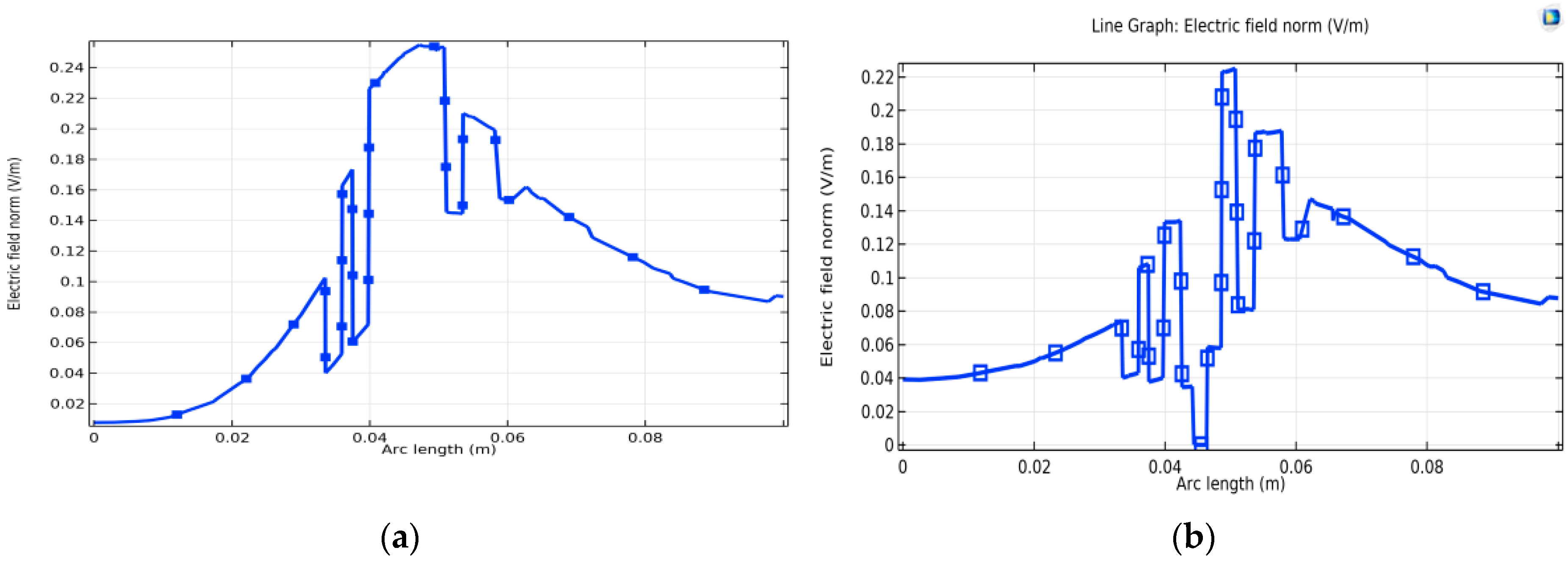
3. Simulating the Magnetic Behavior
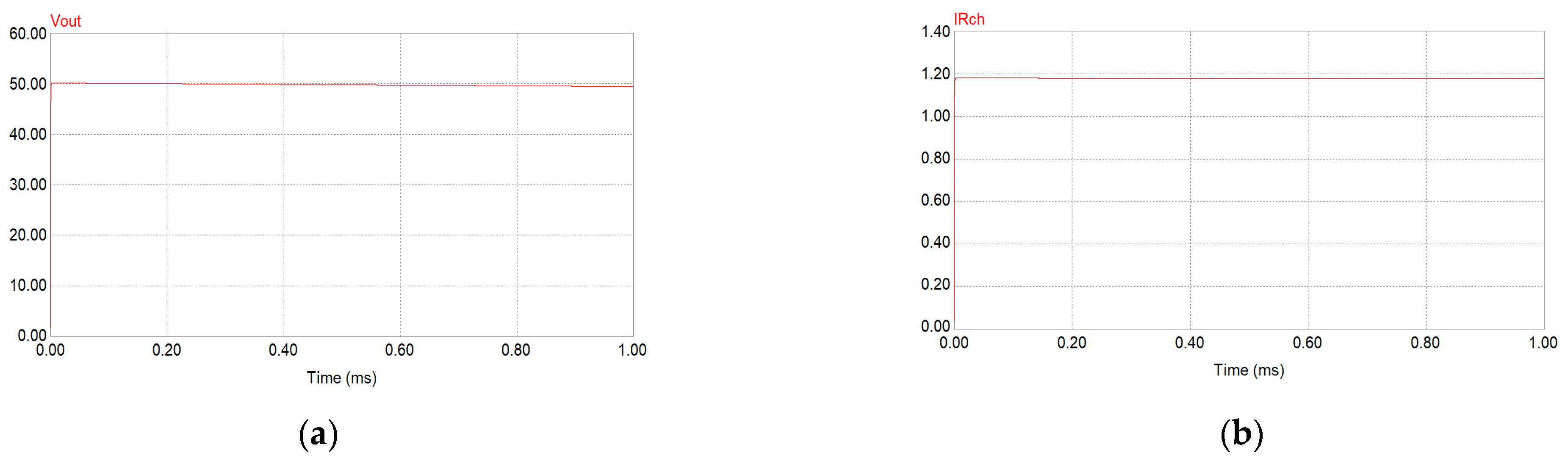
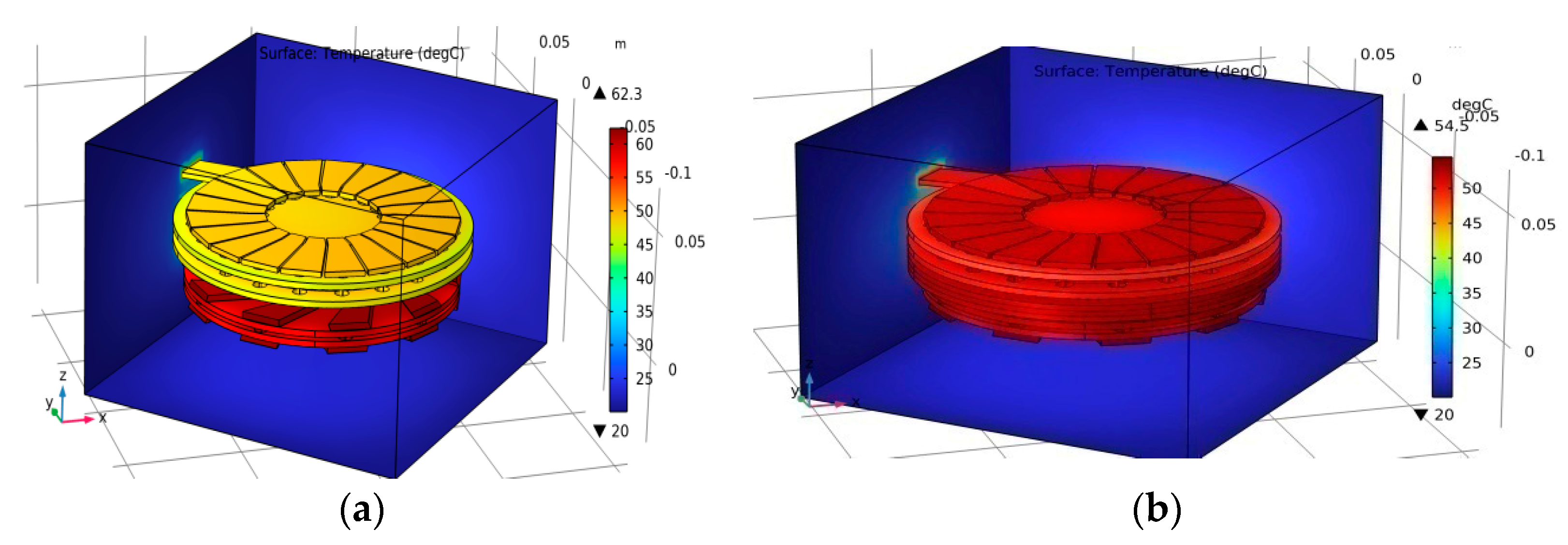
4. Thermal Behavior of the Planar Transformer
5. The Thermal Behavior
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Benzidane, M.R.; Melati, R.; Benyamina, M.; Meskine, S.; Spiteri, P.; Boukortt, A.; Adda Benattia, T. Miniaturization and optimization of a DC–DC boost converter for photovoltaic application by designing an integrated dual-layer inductor model. Trans. Electr. Electron. Mater. 2022, 23, 462–475. [Google Scholar] [CrossRef]
- Luna, M. High-Efficiency and High-Performance Power Electronics for Power Grids and Electrical Drives. Energies 2022, 15, 5844. [Google Scholar] [CrossRef]
- Raghavendra, K.V.G.; Zeb, K.; Muthusamy, A.; Krishna, T.N.V.; Kumar, S.V.P.; Kim, D.H.; Kim, M.S.; Cho, H.G.; Kim, H.J. A comprehensive review of DC–DC converter topologies and modulation strategies with recent advances in solar photovoltaic systems. Electronics 2019, 9, 31. [Google Scholar] [CrossRef]
- Aggas, J.M. Planar Magnetics Design for Low Voltage High Power DC-DC Converters. Doctoral Dissertation, Auburn University, Auburn, AL, USA, 2014. [Google Scholar]
- Bose, B.K. Global energy scenario and impact of power electronics in 21st century. IEEE Trans. Ind. Electron. 2012, 60, 2638–2651. [Google Scholar] [CrossRef]
- Melati, R.; Hamid, A.; Thierry, L.; Derkaoui, M. Design of a new electrical model of a ferromagnetic planar inductor for its integration in a micro-converter. Math. Comput. Model. 2013, 57, 200–227. [Google Scholar] [CrossRef]
- Derkaoui, M.D.M.; Hamid, A.; Lebey, T.L.T.; Melati, R. Design and modeling of an integrated micro-transformer in a flyback converter. IEEE Trans. Power Electron. 2013, 11, 669–682. [Google Scholar] [CrossRef][Green Version]
- Li, Q.; Wolfs, P. A review of the single phase photovoltaic module integrated converter topologies with three different DC link configurations. IEEE Trans. Power Electron. 2008, 23, 1320–1333. [Google Scholar]
- Shawky, A.; Ahmed, M.; Orabi, M.; Aroudi, A.E. Classification of three-phase grid-tied microinverters in photovoltaic applications. Energies 2020, 13, 2929. [Google Scholar] [CrossRef]
- Liu, Y.; Liu, X.; Lu, Q.; Zhang, T.; Yin, X. A T-model with parameter extraction method for modeling 3-D spiral inductor. IEEE Microw. Wirel. Compon. Lett. 2021, 32, 37–40. [Google Scholar] [CrossRef]
- Kouril, L.; Pospisilik, M.; Adamek, M.; Jasek, R. Coil optimization with aid of flat coil optimizer. In Proceedings of the 5th WSEAS Congress on Applied Computing Conference, and Proceedings of the 1st International Conference on Biologically Inspired Computation. World Scientific and Engineering Academy and Society (WSEAS), Faro, Portugal, 2–4 May 2012. [Google Scholar]
- Senhadji, N.; Hamid, A.; Bley, V.; Leby, T. Design and integration of planar inductances on PCB application passive type filters. Trans. Electr. Electron. Mater. 2020, 21, 123–137. [Google Scholar] [CrossRef]
- Derkaoui, M.; Benhadda, Y.; Hamid, A. Modeling and simulation of an integrated octagonal planar transformer for RF systems. SN Appl. Sci. 2020, 2, 656. [Google Scholar] [CrossRef]
- Ferrell, J.; Lai, J.S.; Nergaard, T.; Huang, X.; Zhu, L.; Davis, R. The role of parasitic inductance in high-power planar transformer design and converter integration. In Proceedings of the Nineteenth Annual IEEE Applied Power Electronics Conference and Exposition, 2004, APEC’04, Anaheim, CA, USA, 22–26 February 2004; Volume 1, pp. 510–515. [Google Scholar]
- Wang, S.; Xu, C. Design theory and implementation of a planar EMI filter based on annular integrated inductor–capacitor unit. IEEE Trans. Power Electron. 2012, 28, 1167–1176. [Google Scholar] [CrossRef]
- Yunas, J.; Majlis, B.Y. Comparative study of stack interwinding micro-transformers on silicon monolithic. Microelectron. J. 2008, 39, 1564–1567. [Google Scholar] [CrossRef]
- Zhang, Y.; Li, E.; Long, H.; Feng, Z. Accurate model for micromachined microwave planar spiral inductors. Int. J. RF Microw. Comput.-Aided Eng. 2003, 13, 229–238. [Google Scholar] [CrossRef]
- Lewaiter, A.; Ackermann, B. A thermal model for planar transformers. In Proceedings of the 4th IEEE International Conference on Power Electronics and Drive Systems, IEEEPEDS2001-Indonesia, Proceedings (Cat. No. 01TH8594), Denpasar, Indonesia, 25–25 October 2001; IEEE: Piscataway, NJ, USA, 2001; Volume 2, pp. 669–673. [Google Scholar]
- Allaoui, A.; Hamid, A.; Spitéri, P.; Bley, V.; Lebey, T. Thermal modeling of an integrated inductor in a micro-converter. J. LowPower Electron. 2015, 11, 63–73. [Google Scholar] [CrossRef]
- Benhadda, Y.; Hamid, A.; Lebey, T. Thermal modeling of an integrated circular inductor. J. Nano Electron. 2017, 9, 1004. [Google Scholar] [CrossRef]
- Hu, J. Analyses of the temperature field in a bar-shaped piezoelectric transformer operating in longitudinal vibration mode. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2003, 50, 594–600. [Google Scholar]
- Joo, H.W.; Lee, C.H.; Rho, J.S.; Jung, H.K. Analysis of temperature rise for piezoelectric transformer using finite-element method. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2006, 53, 1449–1457. [Google Scholar] [CrossRef]
- Susa, D.; Lehtonen, M.; Nordman, H. Dynamic thermal modelling of power transformers. IEEE Trans. Power Deliv. 2005, 20, 197–204. [Google Scholar] [CrossRef]
- Rajnak, M.; Wu, Z.; Dolnik, B.; Paulovicova, K.; Tothova, J.; Cimbala, R.; Timko, M. Magnetic field effect on thermal, dielectric, and viscous properties of a transformer oil-based magnetic nanofluid. Energies 2019, 12, 4532. [Google Scholar] [CrossRef]
- Benhadda, Y.; Derkaoui, M.; Kharbouch, H.; Hamid, A.; Spiteri, P. Numerical Simulation for Cooling of Integrated Toroidal Octagonal Inductor Using Nanofluid in a Microchannel Heat Sink. Metall. Mater. Eng. 2024, 30, 17–44. [Google Scholar] [CrossRef]
- Mokhefi, A.; Rossi di Schio, E. Effect of a magnetic field on the Couette forced convection of a Buongiorno’s nanofluid over an embedded cavity. JP J. Heat Mass Transf. 2022, 30, 89–104. [Google Scholar] [CrossRef]
- Caillaud, R.; Buttay, C.; Mrad, R.; Le Lesle, J.; Morel, F.; Degrenne, N.; Mollov, S. Comparison of planar and Toroidal PCB integrated inductors for a multi-cellular 3.3 kW PFC. In Proceedings of the 2017 IEEE International Workshop on Integrated Power Packaging (IWIPP), Delft, The Netherlands, 5–7 April 2017; pp. 1–5. [Google Scholar]
- Pasko, S.W.; Kazimierczuk, M.K.; Grzesik, B. Self-capacitance of coupled toroidal inductors for EMI filters. IEEE Trans. Electromagn. Compat. 2015, 57, 216–223. [Google Scholar] [CrossRef]
- Wheeler, H.A. Simple inductance formulas for radio coils. Proc. Inst. Radio 1928, 16, 1398–1400. [Google Scholar] [CrossRef]
- Mohan, S.S.; del Mar Hershenson, M.; Boyd, S.P.; Lee, T.H. Simple accurate expressions for planar spiral inductances. IEEE J. Solid-State Circuits 1999, 34, 1419–1424. [Google Scholar] [CrossRef]






| Material | Cu | NiFe | FR4 |
|---|---|---|---|
| Permeability μ | 1 | 900 | 1 |
| Resistivity σ (Ω m) | 1.75 × 10−8 | 20 × 10−8 | |
| Permittivity ε | 1 | 11.8 | 4.5 |
| Geometrical Parameter | Value | Electrical Parameter | Value |
|---|---|---|---|
| External diameter: dout | 63 mm | Primary inductance lt | 12.5 µH |
| Internal diameter din | 37 mm | Secondary inductance lb | 3.125 µH |
| Core thickness: eNiFe | 0.4 mm | Primary serial resistance Rst | 66.64 mΩ |
| Skin thickness: δ | 82.24 µm | Secondary serial resistance Rsb | 18.54 mΩ |
| Number of primary turns: nt | 20 | Primary oxide capacitance Coxt | 44.14 mF |
| Number of secondary turns: nb | 10 | Secondary oxide capacitance Coxb | 39.66 mF |
| Width of the primary conductor: ωmoyt | 5.66 mm | Primary magnetic resistance: Rmagt | 27.18 × 10−9 Ω |
| Width of the secondary conductor: ωmoyb | 10.17 mm | Secondary magnetic resistance: Rmagb | 30.25 × 10−9 Ω |
| Thickness of the primary conductor: tt | 70 µm | Primary capacitance of du substrate Cst | 44.14 mF |
| Thickness of the secondary conductor: tb | 70 µm | Secondary capacitance of substrate Csb | 39.66 mF |
| Primary spacing: St | 2.19 mm | Capacitance inter-spacing of primary Covt | 0.0047 pF |
| Secondary spacing: Sb | 5.53 mm | Capacitance inter-spacing of secondary Covb | 0.111 pF |
| Primary total length: lTt | 1040 mm | Coupling capacitance between the primary and secondary coils Cov1,2 | 74.79 mF |
| Secondary total length: lTb | 520 mm | Coupling capacitance between the secondary and primary coils Cov2 | 14.81 mF |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Benamer, K.; Hamid, A.; Rossi di Schio, E.; Mokhefi, A.; Melati, R.; Valdiserri, P. Magnetic and Thermal Behavior of a Planar Toroidal Transformer. Energies 2024, 17, 2454. https://doi.org/10.3390/en17112454
Benamer K, Hamid A, Rossi di Schio E, Mokhefi A, Melati R, Valdiserri P. Magnetic and Thermal Behavior of a Planar Toroidal Transformer. Energies. 2024; 17(11):2454. https://doi.org/10.3390/en17112454
Chicago/Turabian StyleBenamer, Kahina, Azzedine Hamid, Eugenia Rossi di Schio, Abderrahim Mokhefi, Rabia Melati, and Paolo Valdiserri. 2024. "Magnetic and Thermal Behavior of a Planar Toroidal Transformer" Energies 17, no. 11: 2454. https://doi.org/10.3390/en17112454
APA StyleBenamer, K., Hamid, A., Rossi di Schio, E., Mokhefi, A., Melati, R., & Valdiserri, P. (2024). Magnetic and Thermal Behavior of a Planar Toroidal Transformer. Energies, 17(11), 2454. https://doi.org/10.3390/en17112454








