Influence of Wind-Turbine-Generator Power Control on the Performance of a Virtual Synchronous Machine
Abstract
1. Introduction
- −
- Region 1: Stopped (Wind speed is lower than the cut-in wind speed),
- −
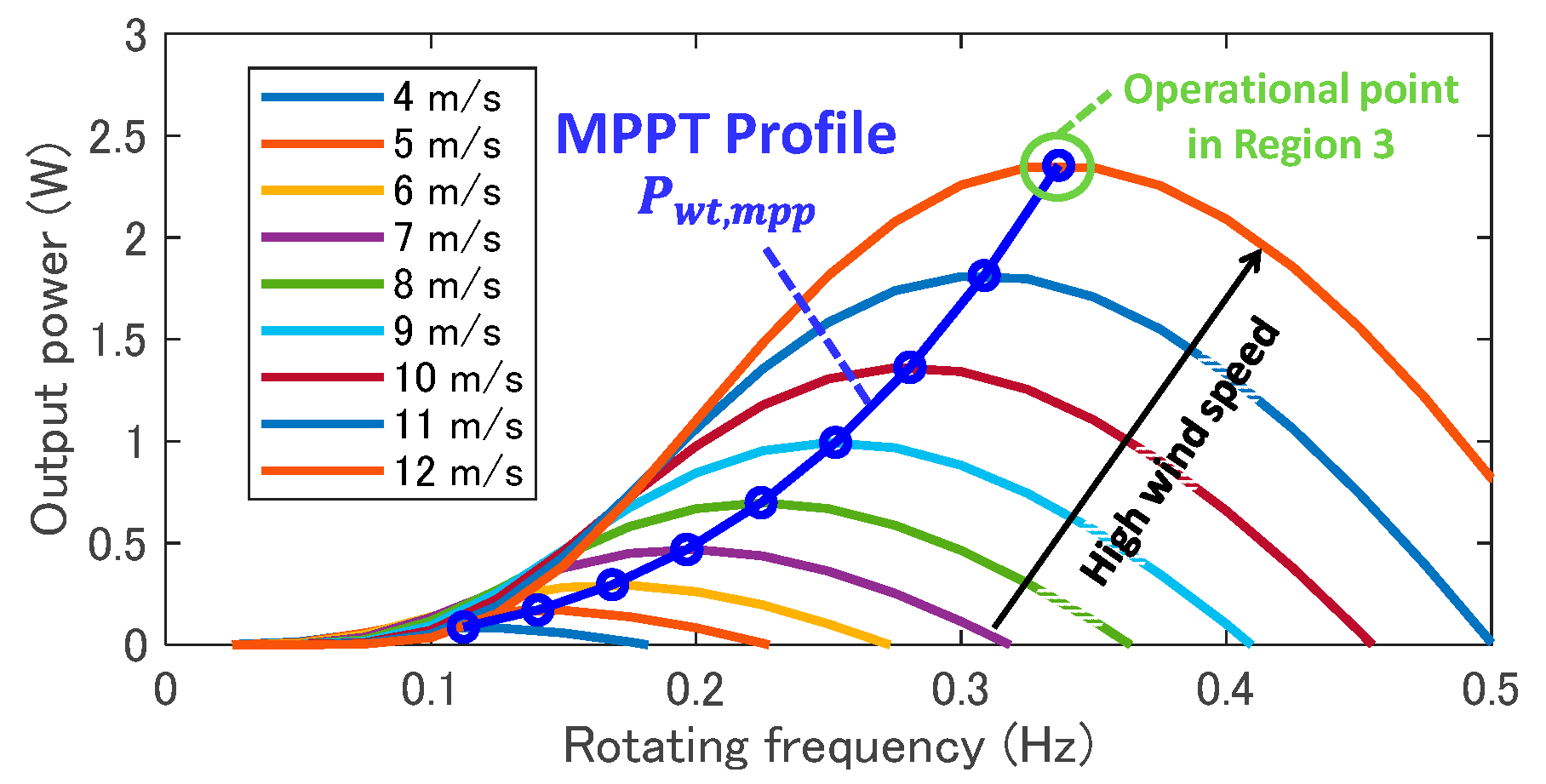
- Region 2: MPPT operation (Wind speed is between the cut-in and rated wind speed),
- −
- Region 3 Rated output operation (Wind speed is between the rated and cut-out wind speed),
- −
- Region 4: Stopped (Wind speed is larger than the cut-out wind speed).
2. Model of Wind Power Generation with Virtual Inertia Control
2.1. Wind Turbine
2.2. Wind Turbine Controller
2.3. Rotor Side Converter (RSC) Control
2.4. Grid Side Converter (GSC) Control
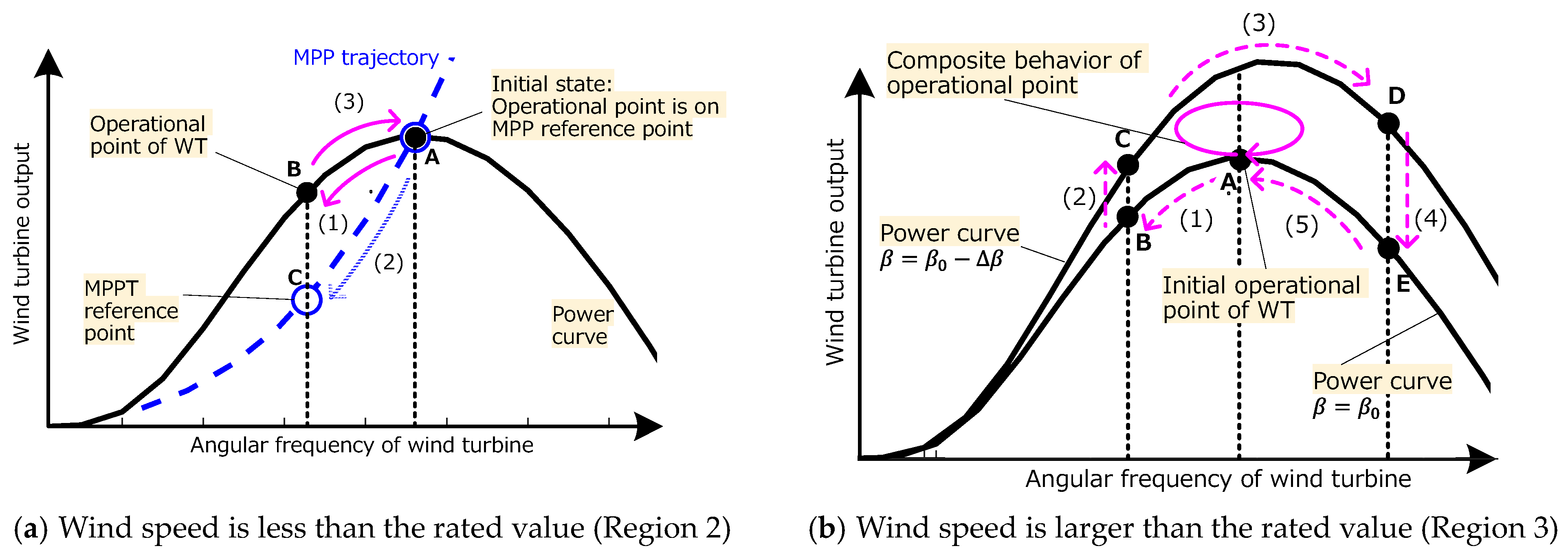
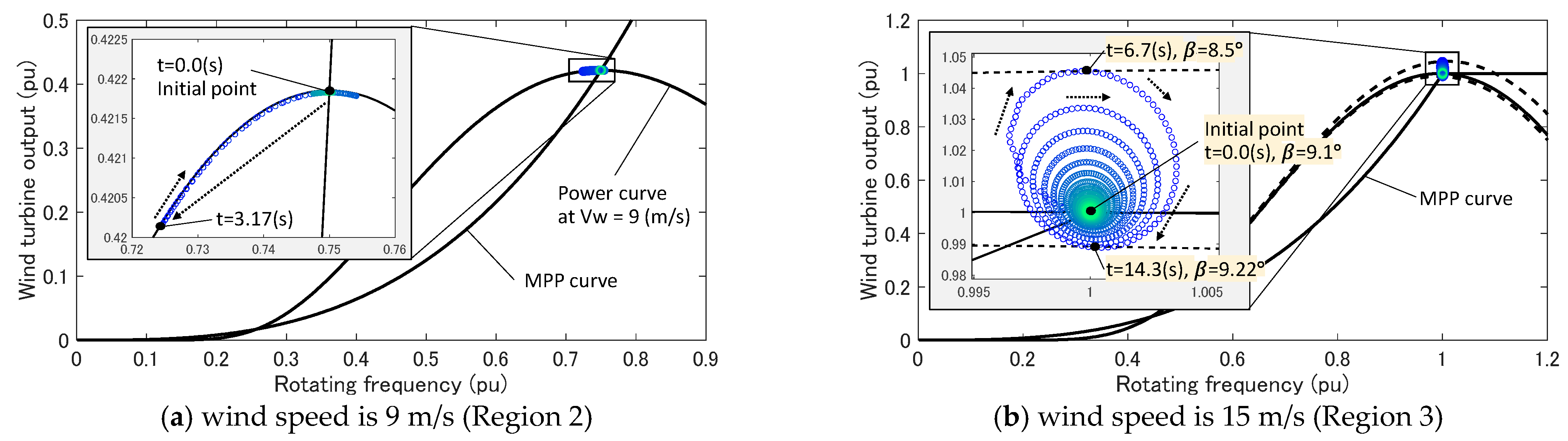
3. Influence of Wind Turbine Control on the VSM Performance
4. Simulation Study
4.1. Power System Model
4.2. Simulation Conditions
5. Simulation Results
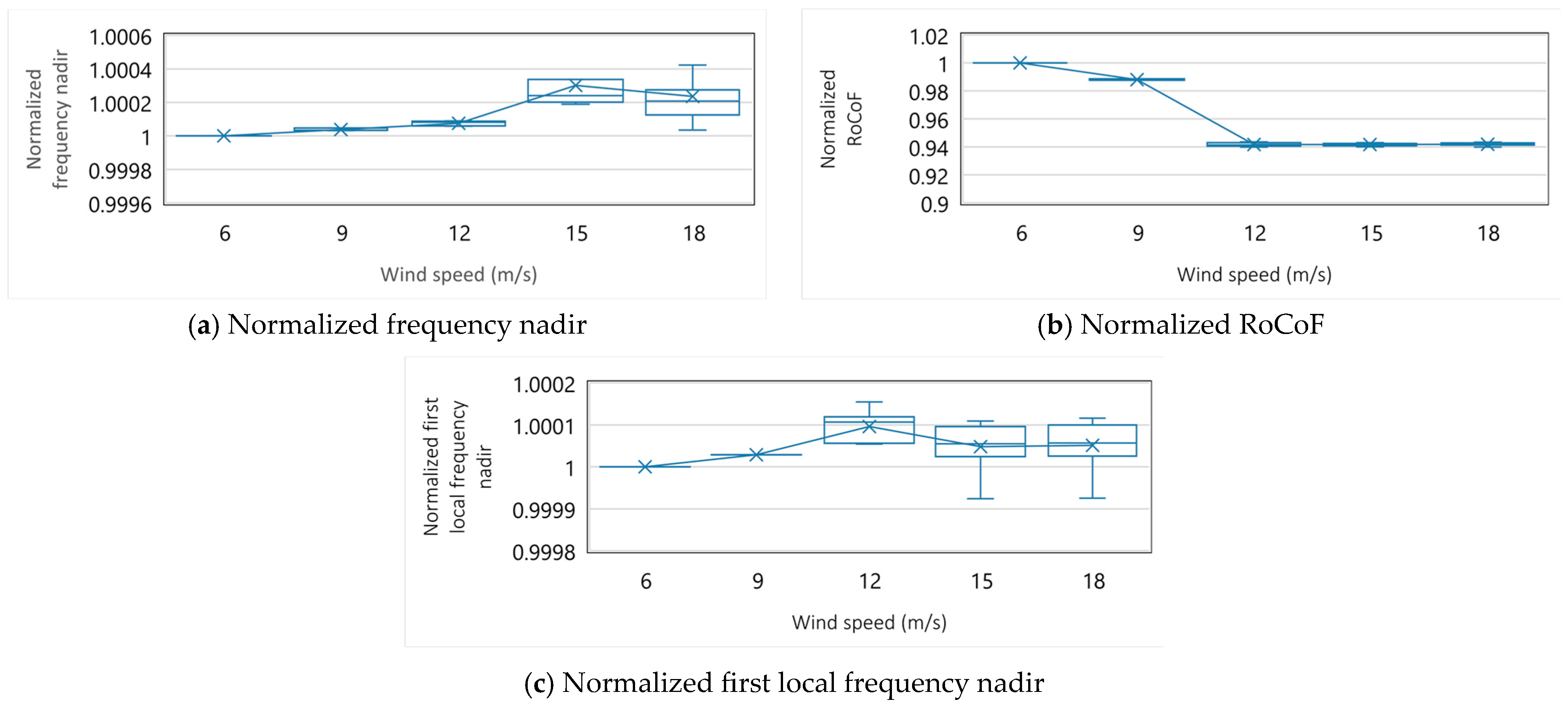
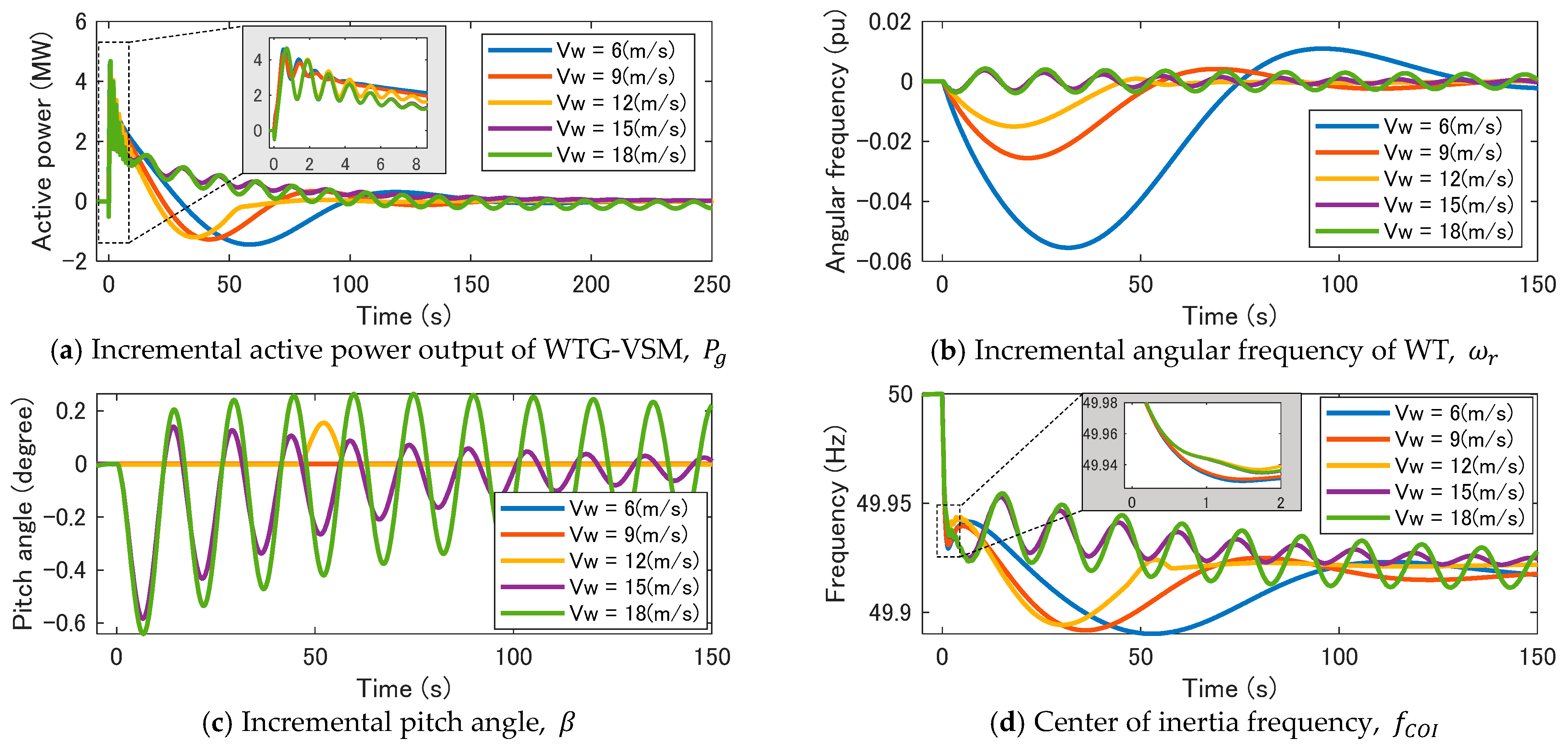
5.1. Influence of Wind Speed on VSM Performance
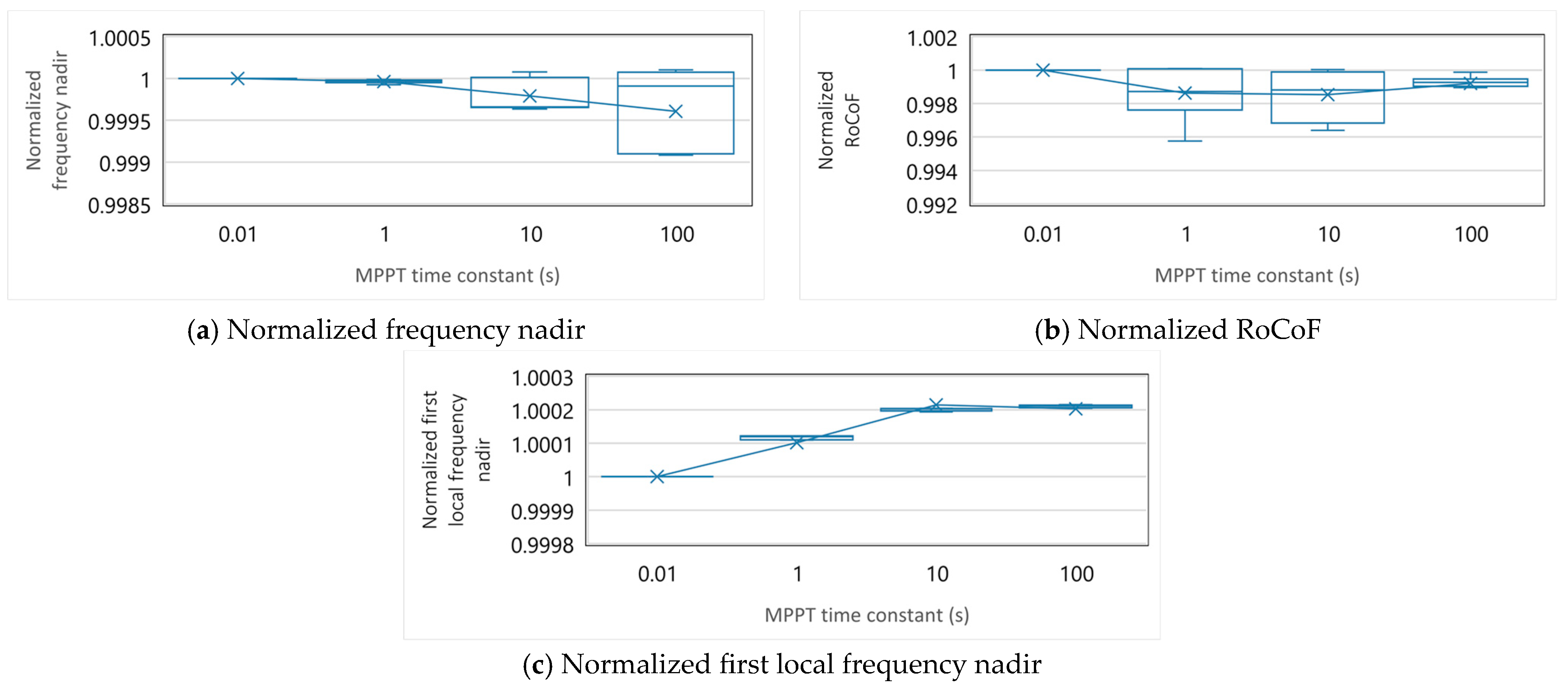
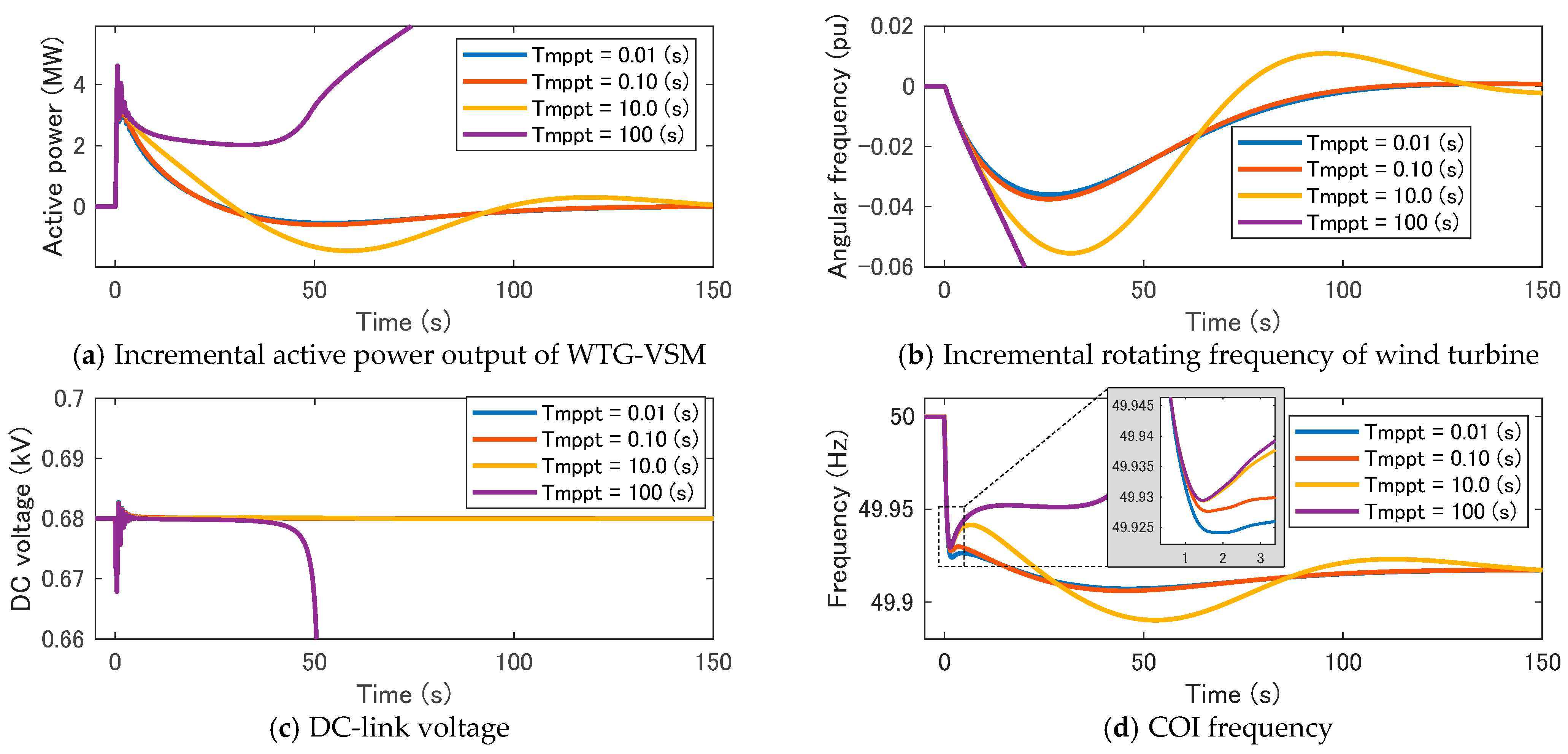
5.2. Influence of Time Constant of MPPT Function
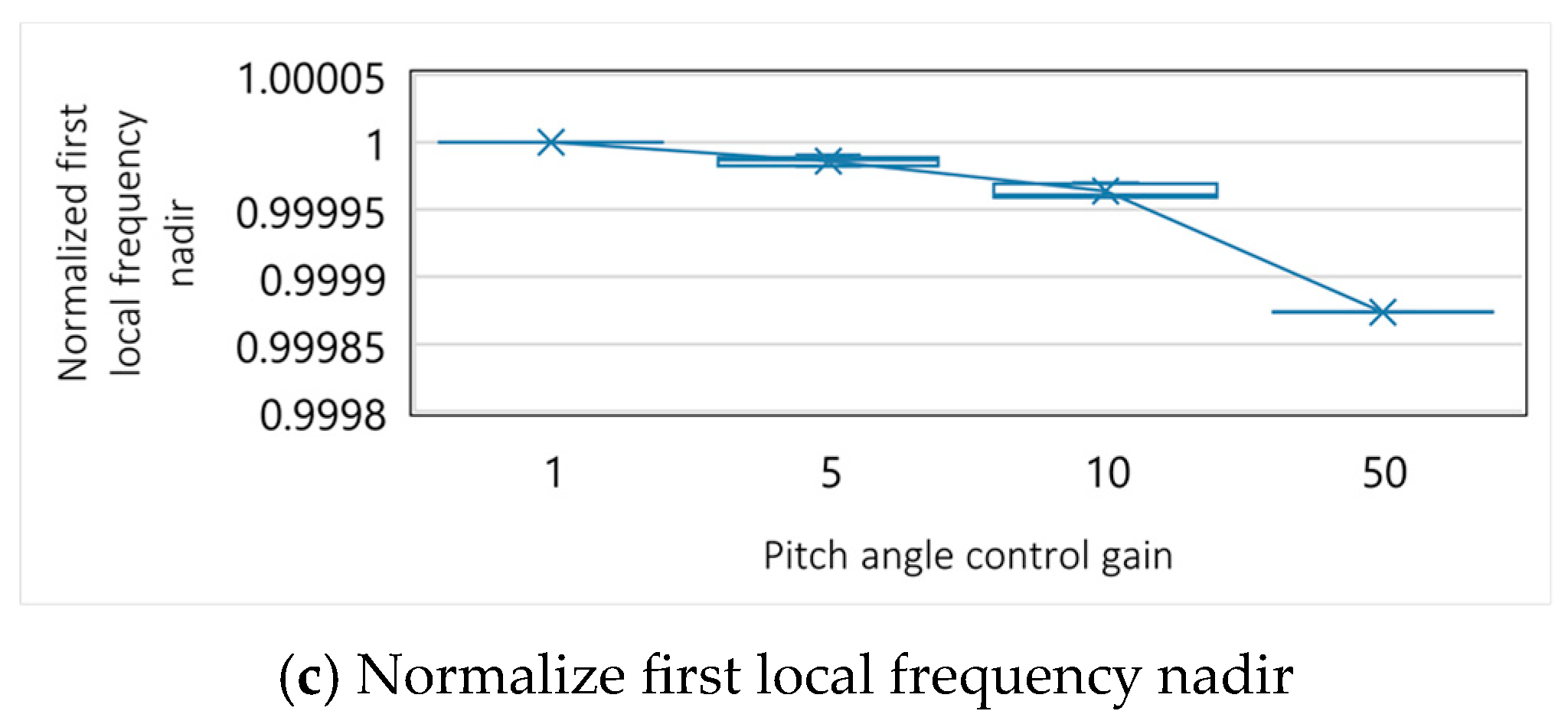
5.3. Influence of Pitch Angle Control Gain on VSM Performance
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
| Parameter | Source A | Source D | PMSG (WTG) |
|---|---|---|---|
| Rated capacity (MVA) | 120 | 10.0 | 100 |
| Rated voltage (kV) | 16.5 | 16.5 | 0.40 |
| Rated frequency (Hz) | 50.0 | 50.0 | 55.5 |
| Inertia constant (s) | 4.0 | 4.0 | 4.0 |
| d-axis unsaturated reactance | 1.7 | 1.7 | 0.36 |
| q-axis unsaturated reactance | 1.6 | 1.6 | 0.25 |
| d-axis unsaturated transient reactance | 0.23 | 0.23 | 0.15 |
| d-axis unsaturated sub-transient reactance | 0.23 | 0.23 | 0.15 |
| q-axis unsaturated sub-transient reactance | 0.23 | 0.23 | 0.15 |
| d-axis unsaturated transient time | 5.9 | 5.9 | 9.0 |
| d-axis unsaturated sub-transient time | 0.010 | 0.010 | 0.010 |
| q-axis unsaturated sub-transient time | 0.010 | 0.010 | 0.010 |
| Armature time constant | 0.40 | 0.40 | 0.33 |
| Potier reactance | 0.095 | 0.095 | 0.080 |
| Parameter | Symbol | Value |
|---|---|---|
| MPPT PI control proportional gain | 0.30 | |
| MPPT PI control integral time (s) | 30.0 | |
| DC voltage control (RSC) proportional gain | 5.0 | |
| DC voltage control (RSC) integral time (s) | 0.20 | |
| Reactive power control (RSC) proportional gain | 0.20 | |
| Reactive power control (RSC) integral time (s) | 0.10 | |
| Grid voltage control (GSC) proportional gain | 0.01 | |
| Grid voltage control (GSC) integral time (s) | 1.0 | |
| Voltage droop control (GSC) gain | 0.20 | |
| Voltage droop control (GSC) time constant (s) | 0.020 | |
| VSM (GSC) inertia constant (s) | 8.0 | |
| VSM (GSC) damping coefficient | 25.0 | |
| VSM (GSC) virtual resistance (pu) | 0.20 | |
| VSM (GSC) virtual reactance (pu) | 0.30 |
Appendix B
| Wind Speed (m/s) | Pitch Angle Control Gain | MPPT Time Constant (s) | |||
|---|---|---|---|---|---|
| 0.01 | 1 | 10 | 100 | ||
| 6 | 1 | Stable | Stable | Stable | Unstable |
| 5 | Stable | Stable | Stable | Unstable | |
| 10 | Stable | Stable | Stable | Unstable | |
| 50 | Stable | Stable | Stable | Unstable | |
| 9 | 1 | Stable | Stable | Stable | Unstable |
| 5 | Stable | Stable | Stable | Unstable | |
| 10 | Stable | Stable | Stable | Unstable | |
| 50 | Stable | Stable | Stable | Unstable | |
| 12 | 1 | Stable | Stable | Stable | Stable |
| 5 | Stable | Stable | Stable | Stable | |
| 10 | Stable | Stable | Stable | Stable | |
| 50 | Stable | Stable | Stable | Stable | |
| 15 | 1 | Stable | Stable | Stable | Unstable |
| 5 | Stable | Stable | Stable | Stable | |
| 10 | Stable | Stable | Stable | Stable | |
| 50 | Stable | Stable | Stable | Stable | |
| 18 | 1 | Stable | Stable | Unstable | Unstable |
| 5 | Stable | Stable | Unstable | Unstable | |
| 10 | Stable | Stable | Stable | Stable | |
| 50 | Stable | Stable | Stable | Stable | |

References
- Beck, H.-P.; Hesse, R. Virtual Synchronous Machine. In Proceedings of the 9th International Conference on Electrical Power Quality and Utilisation, Barcelona, Spain, 9–11 October 2007. [Google Scholar] [CrossRef]
- Ekanayake, J.B.; Jenkins, N.; Strbac, G. Frequency Response from Wind Turbines. Wind Eng. 2008, 32, 573–586. [Google Scholar] [CrossRef]
- Karbouj, H.; Rather, Z.H.; Flynn, D.; Qazi, H.W. Non-synchronous Fast Frequency Reserves in Renewable Energy Integrated Power Systems: A Critical Review. Int. J. Electr. Power Energy Syst. 2019, 106, 488–501. [Google Scholar] [CrossRef]
- Janssens, N.A.; Lambin, G.; Bragard, N. Active Power Control Strategies of DFIG Wind Turbines. In Proceedings of the 2007 IEEE Lausanne Power Tech, Lausanne, Switzerland, 1–5 July 2007; IEEE Publications Lausanne Power Tech: Lausanne, Switzerland, 2007. [Google Scholar] [CrossRef]
- Moutis, P.; Loukarakis, E.; Papathanasiou, S.; Hatziargyriou, N.D. Primary Load-Frequency Control from Pitch-Controlled Wind Turbines. In Proceedings of the 2009 IEEE Bucharest PowerTech, Bucharest, Romania, 28 June–2 July 2009. [Google Scholar] [CrossRef]
- Nguyen, X.H.; Nakajima, T.; Ota, Y. Droop-Based Grid-Forming Function by Type IV Wind Farm for Fast Frequency Control. In Proceedings of the 2021 IEEE PES Innovative Smart Grid Technologies-Asia (ISGT Asia), Brisbane, Australia, 5–8 December 2021. [Google Scholar] [CrossRef]
- Moutis, P.; Papathanassiou, S.A.; Hatziargyriou, N.D. Improved Load-Frequency Control Contribution of Variable Speed Variable Pitch Wind Generators. Renew. Energy 2012, 48, 514–523. [Google Scholar] [CrossRef]
- Lyu, X.; Subotić, I.; Groß, D. Unified Grid-Forming Control of PMSG Wind Turbines for Fast Frequency Response and MPPT. In Proceedings of the IREP Bulk Power System Dynamics and Control Symposium, Banff, AB, Canada, 25–30 July 2022. [Google Scholar] [CrossRef]
- Wind Energy Generation Systems–Part 21–1: Measurement and Assessment of Electrical Characteristics–Wind Turbines, IEC 61400-21-1. Available online: https://webstore.iec.ch/publication/29528. (accessed on 29 December 2023).
- Ullah, N.R.; Thiringer, T.; Karlsson, D. Temporary Primary Frequency Control Support by Variable Speed Wind Turbines—Potential and Applications. IEEE Trans. Power Syst. 2008, 23, 601–612. [Google Scholar] [CrossRef]
- El Itani, S.; Annakkage, U.D.; Joos, G. Short-Term Frequency Support Utilizing Inertial Response of DFIG Wind Turbines. In Proceedings of the 2011 IEEE Power and Energy Society General Meeting, Detroit, MI, USA, 24–28 July 2011; pp. 1–8. [Google Scholar] [CrossRef]
- Kang, M.; Muljadi, E.; Hur, K.; Kang, Y.C. Stable Adaptive Inertial Control of a Doubly Fed Induction Generator. IEEE Trans. Smart Grid. 2016, 7, 2971–2979. [Google Scholar] [CrossRef]
- Aho, J.; Buckspan, A.; Laks, J.; Fleming, P.; Jeong, Y.; Dunne, F.; Churchfield, M.; Pao, L.; Johnson, K. A Tutorial of Wind Turbine Control for Supporting Grid Frequency Through Active Power Control. In Proceedings of the American Control Conference (ACC), Montreal, QC, Canada, 27–29 June 2012. [Google Scholar] [CrossRef]
- Anaya-Lara, O.; Jenkins, N.; Ekanayake, J.B.; Cartwright, P.; Hughes, M. Wind Energy Generation: Modelling and Control; John Wiley & Sons: Chichester, UK, 2009. [Google Scholar]
- Nguyen, T.; Vu, T.; Paudyal, S.; Blaabjerg, F.; Long Vu, T.L. Grid-Forming Inverter-Based Wind Turbine Generators: Comprehensive Review, Comparative Analysis, and Recommendations. arXiv 2022, arXiv:2203.02105. [Google Scholar] [CrossRef]
- Wang, Y.; Meng, J.; Zhang, X.; Xu, L. Control of PMSG-Based Wind Turbines for System Inertial Response and Power Oscillation Damping. IEEE Trans. Sustain. Energy 2015, 6, 565–574. [Google Scholar] [CrossRef]
- Kheshti, M.; Ding, L.; Nayeripour, M.; Wang, X.; Terzija, V. Active Power Support of Wind Turbines for Grid Frequency Events Using a Reliable Power Reference Scheme. Renew. Energy 2019, 139, 1241–1254. [Google Scholar] [CrossRef]
- Zhou, B.; Bian, X.; Zhang, J.; Lou, P.; Zhang, L.; Wang, X. Dynamic Frequency Regulation of Microgrid Based on Double-Layer Adaptive Virtual Inertia Control of DFIG; IEEE: Shanghai, China, 2022; pp. 1073–1081. [Google Scholar] [CrossRef]
- Kang, M.; Lee, J.; Kang, Y.C. Modified Stepwise Inertial Control Using the Mechanical Input and Electrical Output Curves of a Doubly Fed Induction Generator. In Proceedings of the 9th International Conference on Power Electronics and ECCE Asia (ICPE-ECCE Asia), Seoul, Republic of Korea, 1–5 June 2015. [Google Scholar] [CrossRef]
- Heidary Yazdi, S.S.; Milimonfared, J.; Fathi, S.H.; Rouzbehi, K.; Rakhshani, E. Analytical Modeling and Inertia Estimation of VSG-Controlled Type 4 WTGs: Power System Frequency Response Investigation. Int. J. Electr. Power Energy Syst. 2019, 107, 446–461. [Google Scholar] [CrossRef]
- Sun, C.; Ali, S.Q.; Joos, G.; Bouffard, F. Improved VSG Control for Type-IV Wind Turbine Generator Considering Operation Limitations. In Proceedings of the IEEE Energy Conversion Congress and Exposition (ECCE), Baltimore, MD, USA, 29 September–3 October 2019. [Google Scholar] [CrossRef]
- Zhu, J.; Hu, J.; Hung, W.; Wang, C.; Zhang, X.; Bu, S.; Li, Q.; Urdal, H.; Booth, C.D. Synthetic Inertia Control Strategy for Doubly Fed Induction Generator Wind Turbine Generators Using Lithium-Ion Supercapacitors. IEEE Trans. Energy Convers. 2018, 33, 773–783. [Google Scholar] [CrossRef]
- Li, Y.; Yuan, X.; Li, J.; Xiao, H.; Xu, Z.; Du, Z. Novel Grid-Forming Control of PMSG-Based Wind Turbine for Integrating Weak AC Grid Without Sacrificing Maximum Power Point Tracking. IET Gener. Transm. Distrib. 2021, 15, 1613–1625. [Google Scholar] [CrossRef]
- Li, S.; Hu, J.; Wang, S.; He, H.; Wang, L.; Liu, G. A Novel Inertial Control Strategy for Full-Capacity Wind Turbine with PLL by Optimizing Internal Potential Response. In Proceedings of the 17th International Conference on Electrical Machines and Systems (ICEMS), Hangzhou, China, 22–25 October 2014. [Google Scholar] [CrossRef]
- Jiang, Q.; Zeng, X.; Li, B.; Wang, S.; Liu, T.; Chen, Z.; Wang, T.; Zhang, M. Time-Sharing Frequency Coordinated Control Strategy for PMSG-Based Wind Turbine. IEEE J. Emerg. Sel. Top. Circuits Syst. 2022, 12, 268–278. [Google Scholar] [CrossRef]
- Li, Y.; Xu, Z.; Wong, K.P. Advanced Control Strategies of PMSG-Based Wind Turbines for System Inertia Support. IEEE Trans. Power Syst. 2017, 32, 3027–3037. [Google Scholar] [CrossRef]
- Ackermann, T. Wind Power in Power Systems, 2nd ed.; John Willey & Sons Ltd: Chichester, UK, 2012; pp. 827–828. [Google Scholar]
- Pourbeik, P.; Ellis, A.; Sanchez-Gasca, J.; Kazachkov, Y.; Muljadi, E.; Senthil, J.; Davies, D. Generic Stability Models for Type 3 & 4 Wind Turbine Generators for WECC. In Proceedings of the 2013 IEEE Power & Energy Society General Meeting, Vancouver, BC, Canada, 21–25 July 2013; pp. 1–5. [Google Scholar] [CrossRef]
- Wu, B.; Lang, Y.; Zargari, N.; Kouro, S. Power Conversion and Control of Wind Energy System; Wiley-IEEE Press: Hoboken, NJ, USA, 2011. [Google Scholar] [CrossRef]
- Rowan, T.M.; Kerkman, R.J. A New Synchronous Current Regulator and an Analysis of Current-Regulated PWM Inverters. IEEE Trans. Ind. Appl. 1986, IA-22, 678–690. [Google Scholar] [CrossRef]
- Institution of Electrical Engineering in Japan. Japanese Power System Model. Available online: http://www.iee.or.jp/pes/model/english/ (accessed on 29 December 2023).
- Orihara, D.; Kikusato, H.; Hashimoto, J.; Otani, K.; Takamatsu, T.; Oozeki, T.; Taoka, H.; Matsuura, T.; Miyazaki, S.; Hamada, H.; et al. Contribution of Voltage Support Function to Virtual Inertia Control Performance of Inverter-Based Resource in Frequency Stability. Energies 2021, 14, 4220. [Google Scholar] [CrossRef]
- Zhao, J.; Tang, Y.; Terzija, V. Robust Online Estimation of Power System Center of Inertia Frequency. IEEE Trans. Power Syst. 2019, 34, 821–825. [Google Scholar] [CrossRef]
- Entso, E. Technical Requirements for Fast Frequency Reserve Provision in the Nordic Synchronous Area-External Document. Available online: https://www.statnett.no/globalassets/for-aktorer-i-kraftsystemet/marked/reservemarkeder/ffr/technical-requirements-for-ffr-v1.1.pdf (accessed on 29 December 2023).
- FINGRID. The Technical Requirements and the Pre-Qualification Process of Fast Frequency Reserve (FFR). Available online: https://www.fingrid.fi/globalassets/dokumentit/fi/sahkomarkkinat/reservit/the-technical-requirements-and-the-prequalification-process-of-fast-frequency-reserve-ffr-as-of-22-may-2023.pdf (accessed on 29 December 2023).



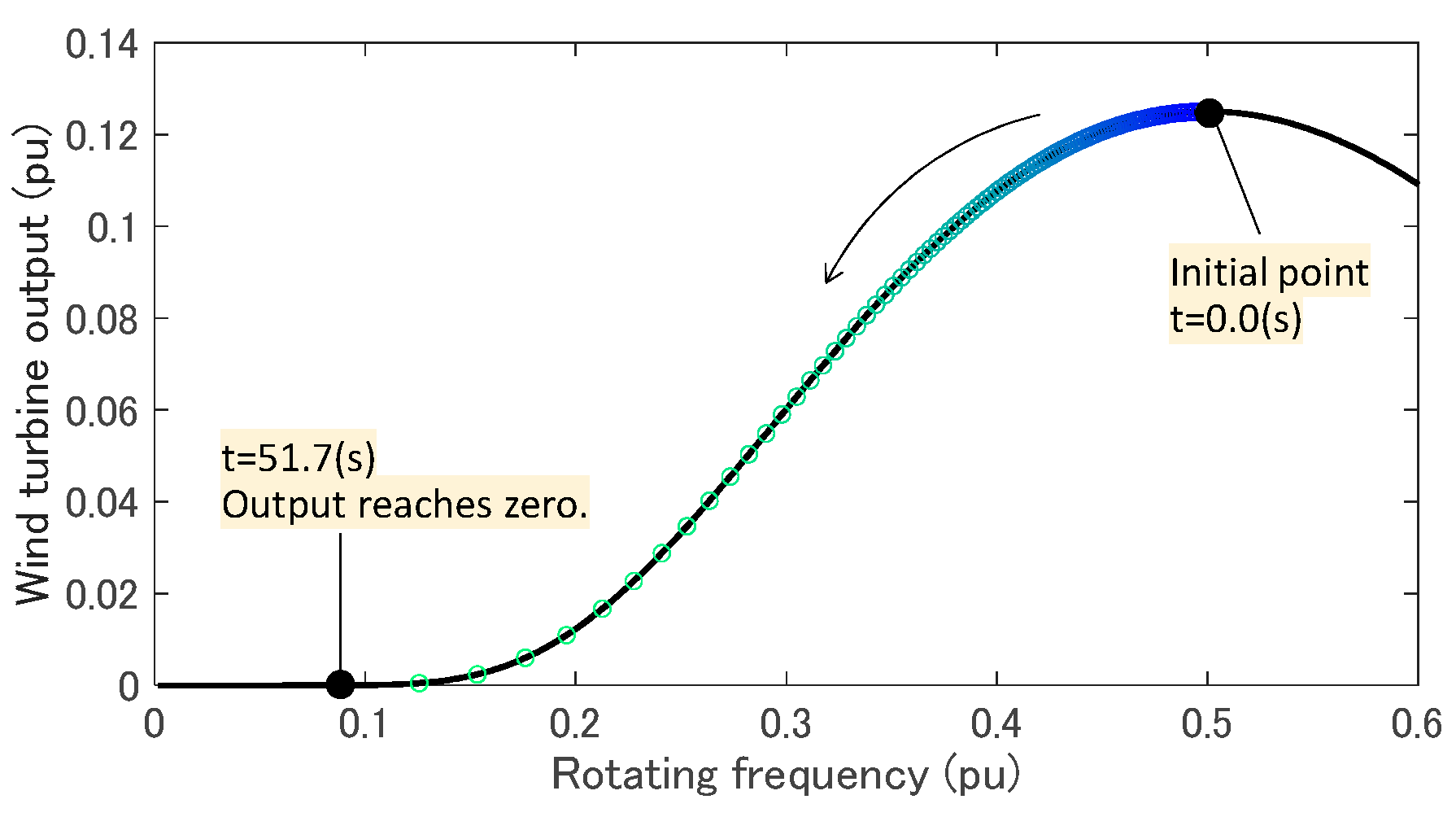
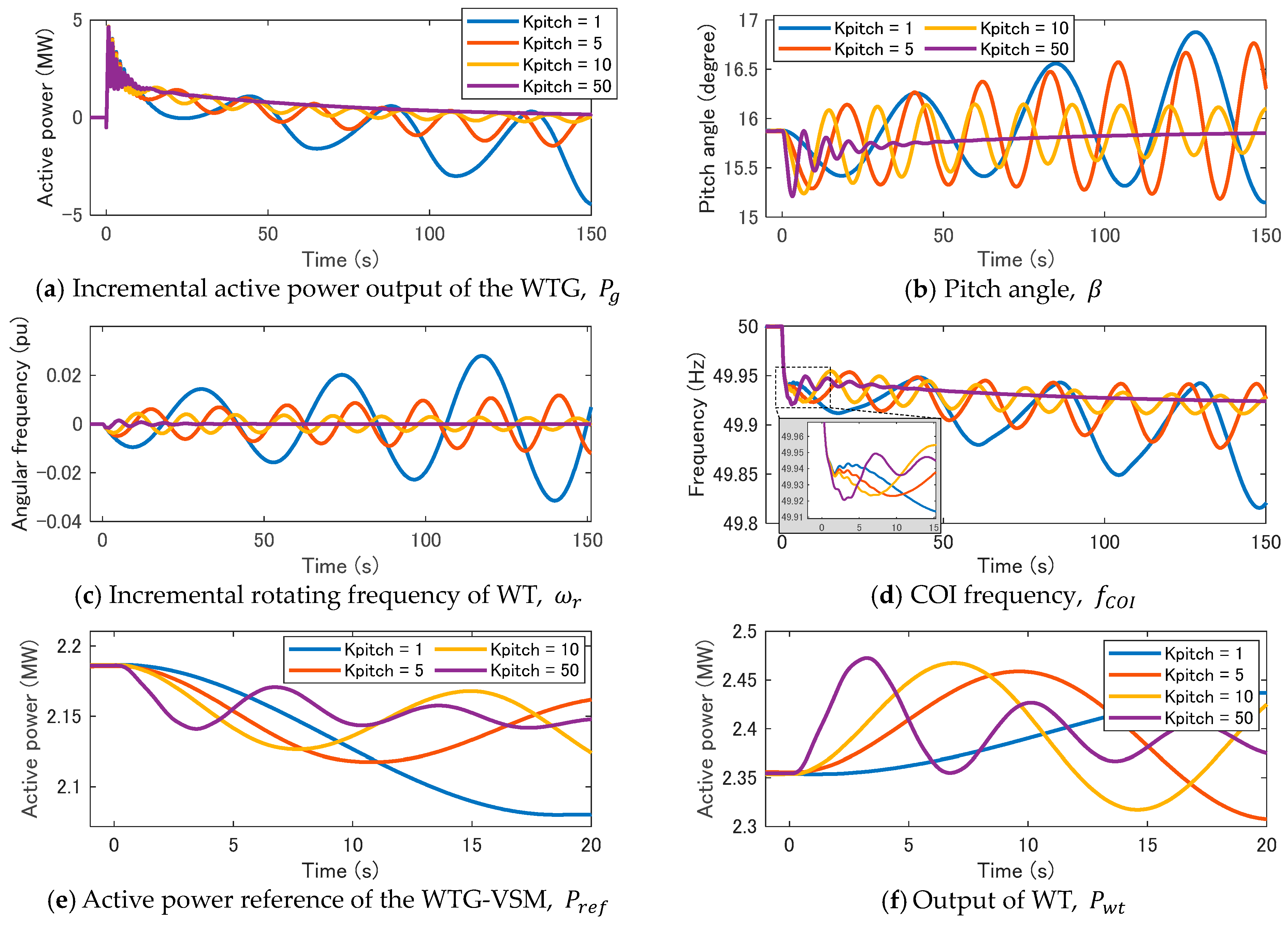

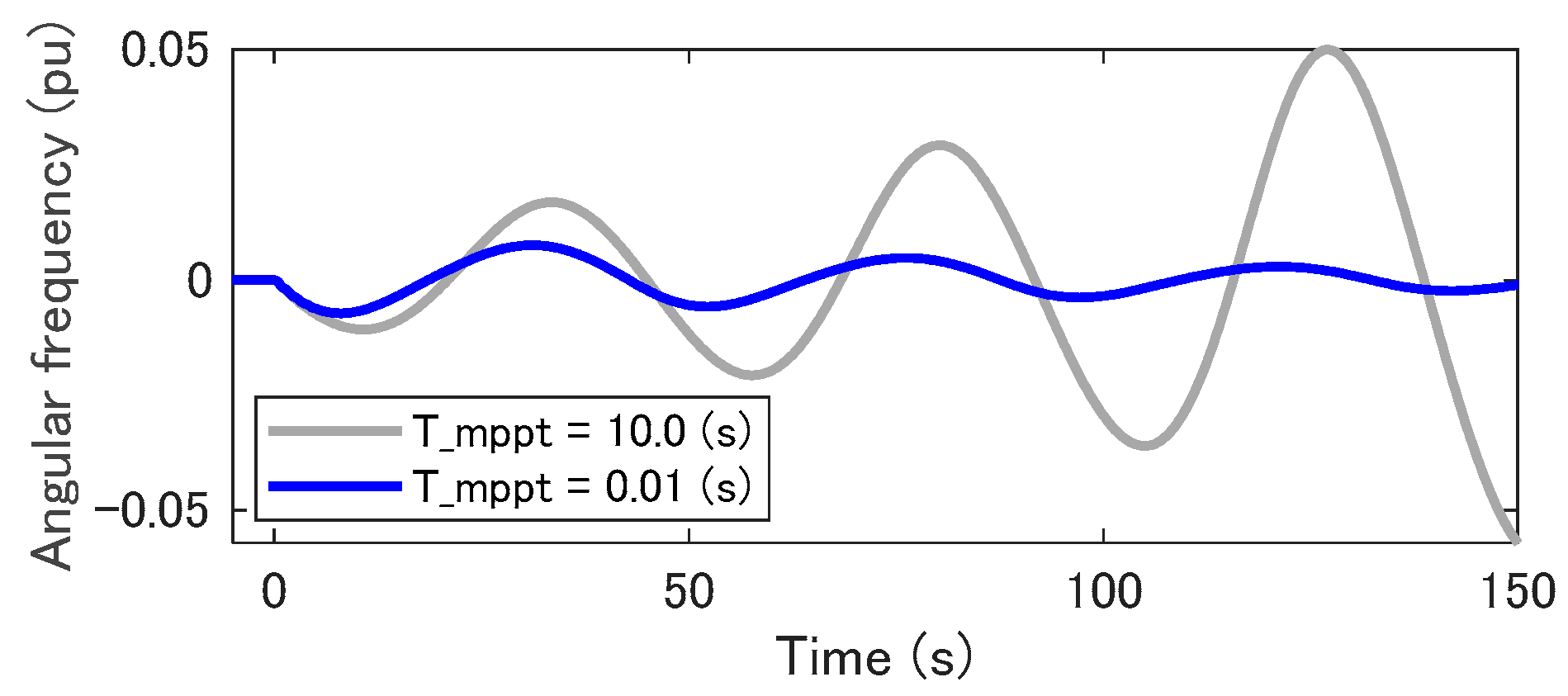
| Wind turbine | |
|---|---|
| Radius of windmill | 40 [m] |
| Rated frequency of windmill | 0.3 [Hz] |
| Cut in/ Rated/ Cut out wind speed | 4/12/25 [m/s] |
| Power coefficient c1, c2, …, c7 | {0.73, 151, 0.58, 0.002, 2.14, 13.2, 18.4} |
| Gear ratio | 55 |
| Permanent magnet synchronous generator (PMSG) | |
| Rated frequency of PMSG | 55.5 [Hz] |
| Rated voltage of PMSG | 400 [V] |
| Inertia constant | 4.0 [s] |
| Damping factor | 0.01 [pu] |
| Interconnection | |
| Rated voltage of DC-link | 680 [V] |
| Capacitance in DC-link | 10 [mF] |
| Rated AC voltage of GSC | 400 [V] |
| Transformer leakage reactance | 0.06 [pu] (GSC, RSC) |
| Transformer voltage ratio | 400/400 [V] (GSC, RSC) |
| Parameter | Symbol | Value |
|---|---|---|
| Wind speed (m/s) | VW | 6, 9, 12, 15, 18 |
| Time constant of MPPT (s) | Tmppt | 0.01, 1, 10, 100 |
| Pitch angle control gain | Kpitch | 1, 5, 10, 50 |
| Wind Speed (m/s) | ||||||
|---|---|---|---|---|---|---|
| Region 2 | Region 3 | |||||
| 6 | 9 | 12 | 15 | 18 | ||
| (Hz) | 49.890 | 49.892 | 49.894 | 49.922 | 49.911 | |
| (Hz) | 49.929 | 49.931 | 49.937 | 49.935 | 49.935 | |
| RoCoF (Hz/s) | −0.116 | −0.115 | −0.110 | −0.110 | −0.110 | |
| MPPT Time Constant (s) | ||||
|---|---|---|---|---|
| 0.01 | 1 | 10 | 100 | |
| (Hz) | 49.907 | 49.906 | 49.890 | –*1 |
| (Hz) | 49.924 | 49.928 | 49.929 | –*1 |
| RoCoF (Hz/s) | −0.117 | −0.116 | −0.116 | –*1 |
| Pitch Angle Control Gain | ||||
|---|---|---|---|---|
| 1 | 5 | 10 | 50 | |
| (Hz) | –*1 | –*1 | 49.911 | 49.921 |
| (Hz) | –*1 | –*1 | 49.935 | 49.921 |
| RoCoF (Hz/s) | –*1 | –*1 | −0.110 | −0.110 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Orihara, D.; Taoka, H.; Otani, K. Influence of Wind-Turbine-Generator Power Control on the Performance of a Virtual Synchronous Machine. Energies 2024, 17, 234. https://doi.org/10.3390/en17010234
Orihara D, Taoka H, Otani K. Influence of Wind-Turbine-Generator Power Control on the Performance of a Virtual Synchronous Machine. Energies. 2024; 17(1):234. https://doi.org/10.3390/en17010234
Chicago/Turabian StyleOrihara, Dai, Hisao Taoka, and Kenji Otani. 2024. "Influence of Wind-Turbine-Generator Power Control on the Performance of a Virtual Synchronous Machine" Energies 17, no. 1: 234. https://doi.org/10.3390/en17010234
APA StyleOrihara, D., Taoka, H., & Otani, K. (2024). Influence of Wind-Turbine-Generator Power Control on the Performance of a Virtual Synchronous Machine. Energies, 17(1), 234. https://doi.org/10.3390/en17010234






