A Tensile Rotary Airborne Wind Energy System—Modelling, Analysis and Improved Design
Abstract
1. Introduction
1.1. A Brief History of Daisy-Kite AWE Rotary Kite Turbine
1.2. Motivation and Main Work Organisation
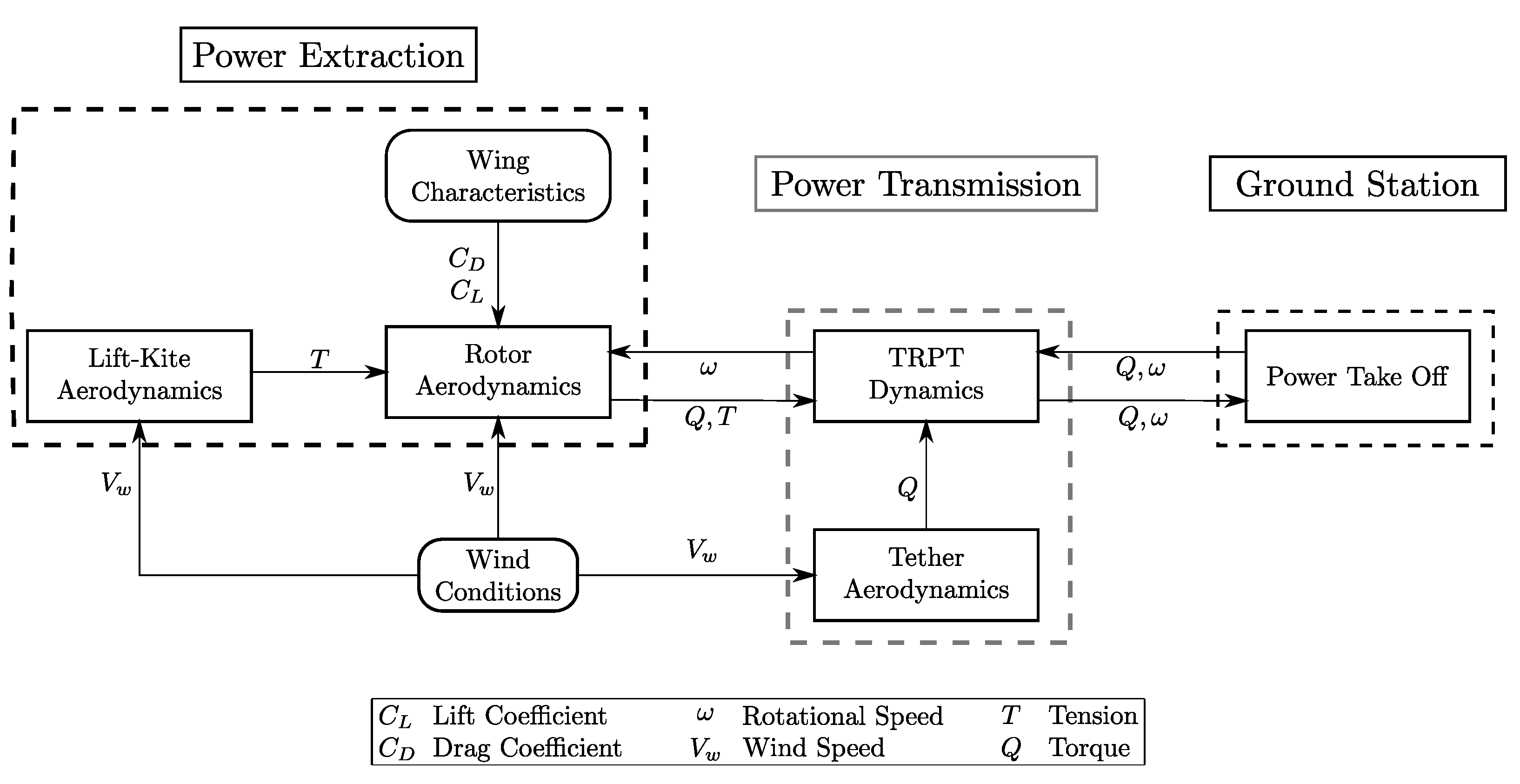
2. Modelling Framework
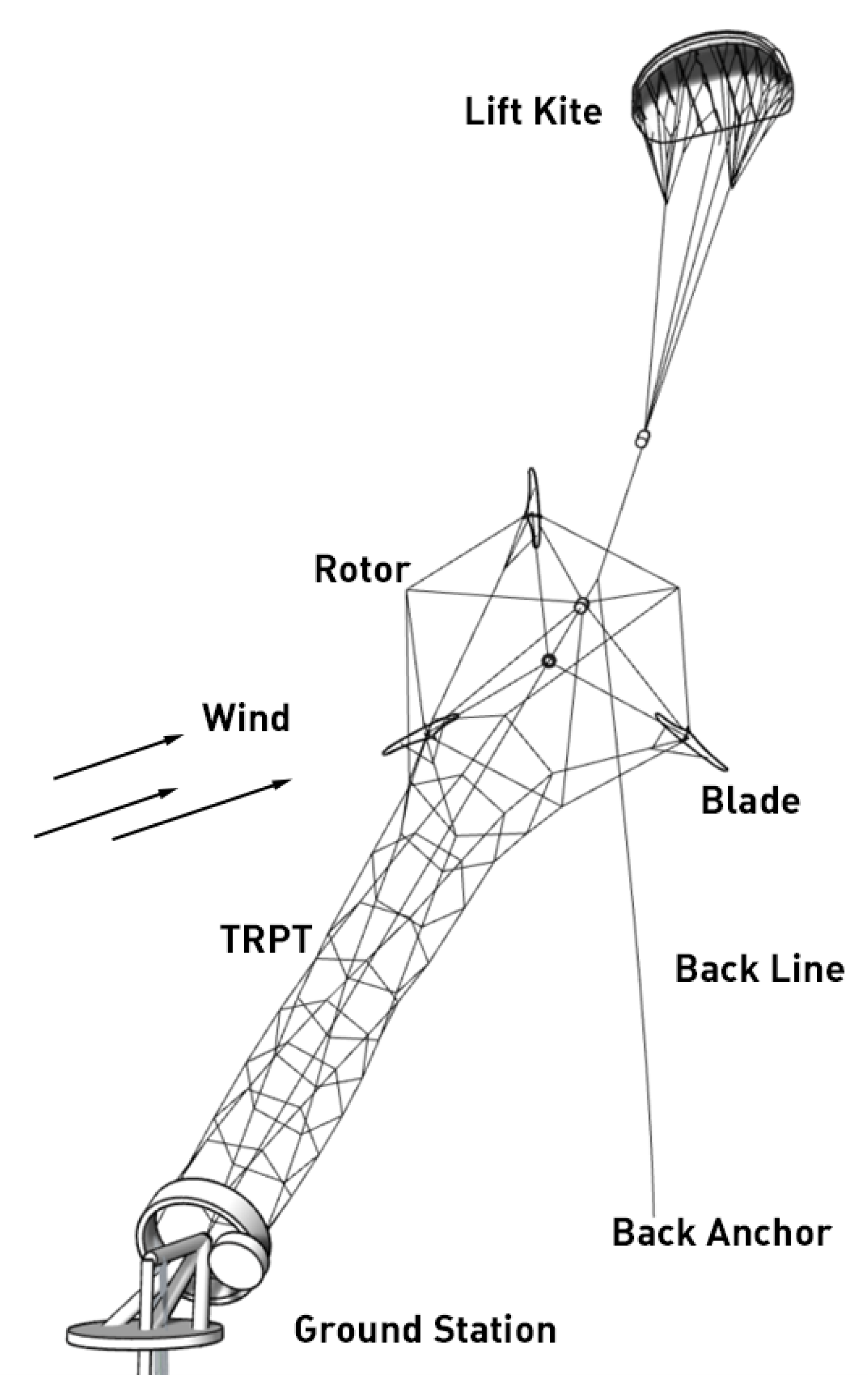
2.1. Overall System Configuration
2.2. Power Extraction
2.2.1. Rotor Aerodynamics
2.2.2. Wing Characteristics
2.2.3. Lift-Kite Aerodynamics
2.2.4. Wind Models
- (1)
- The first is the uniform and constant wind speed used to analyse steady-state performance.
- (2)
- The second wind model assumes that the wind speed varies with time but is uniform in the plane perpendicular to the wind’s direction. This model is used for the simulation of dynamic system responses.
- (3)
- The third wind shear model accounts for the variations in wind speed in both time and altitude. The variation in wind speed with altitude is calculated following the power law [25]. This wind shear model is used for the entire system to integrate all modules into the same modelling scheme.
2.3. Ground Station—Power Take Off
3. Power Transmission—TRPT Representations and Tether Drag Models
3.1. Steady State TRPT Model
- Wind reference frame. It is defined as (), in which is parallel to the wind velocity vector, , which is parallel to the ground; is perpendicular to the wind vector and also parallel to the ground; and is perpendicular to the plane.
- Rotating reference frame for the lower ring. It is defined as (), with the origin at . lies on the system’s axis of rotation, and are in the plane of the lower ring, and is towards point A.
- Rotating reference frame for the upper ring. It is denoted by (), the origin is at , lies on the axis of rotation, and are in the plane of the upper ring, and is towards point B.
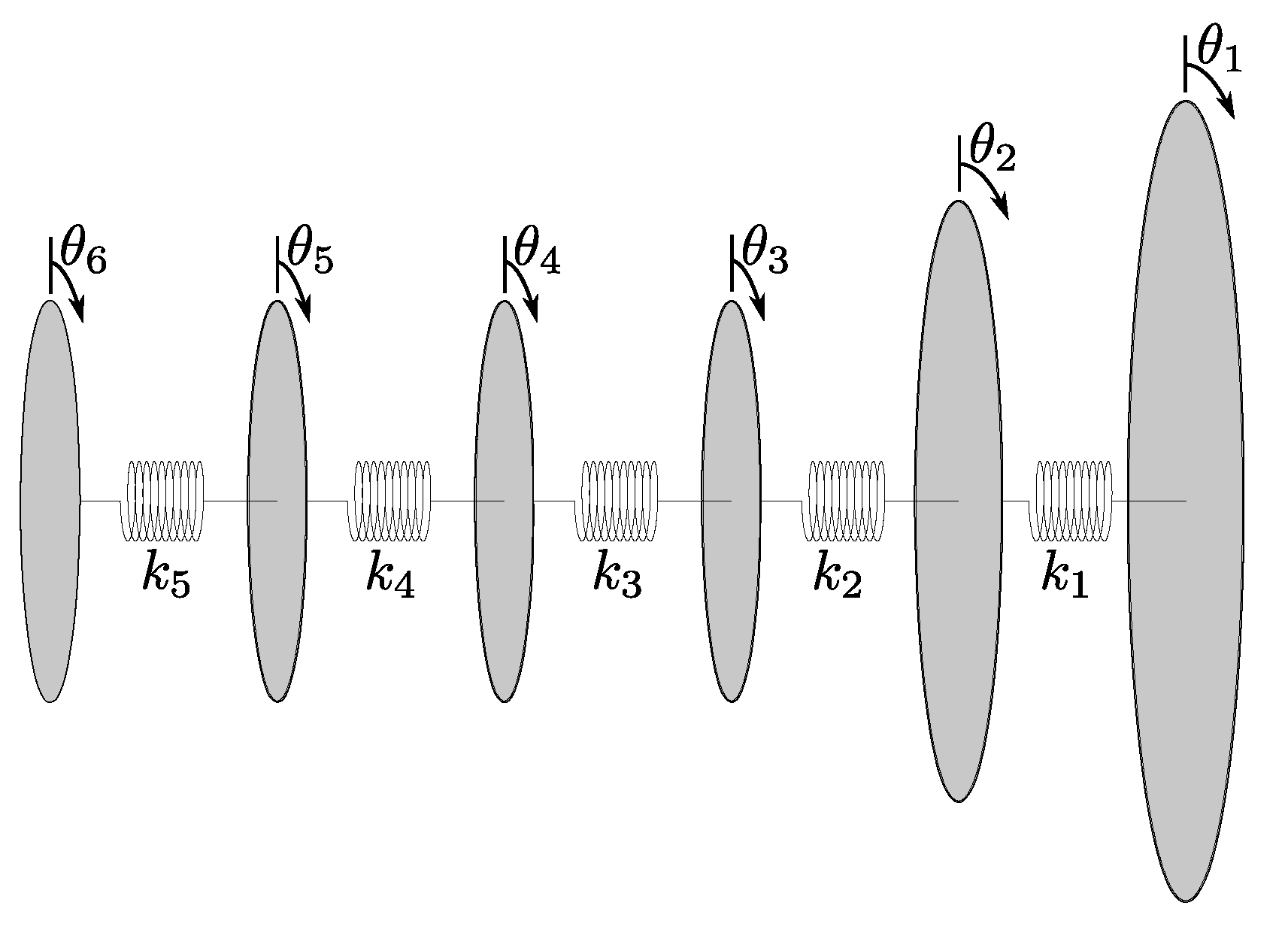
3.2. TRPT Dynamic Model 1: Spring—Disc Representation
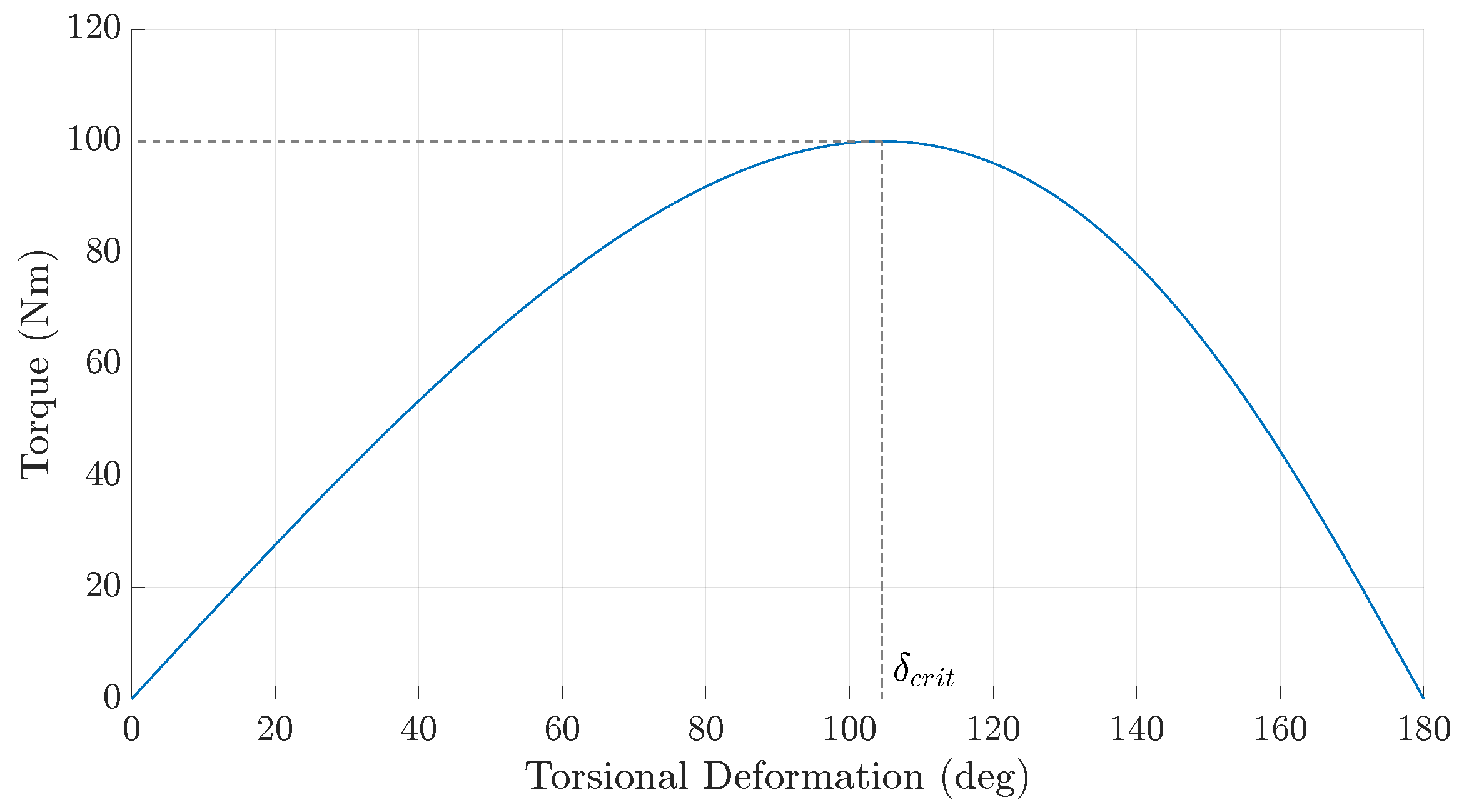
3.3. TRPT Dynamic Model 2: Multi-Spring Representation
3.4. Tether Drag Models-Calculation of Torque Loss in TRPT
3.4.1. Simple Tether-Drag Model for Steady-State TRPT Representation
3.4.2. Improved Tether Drag Model for Dynamic TRPT Representations
4. Model Validation and Modifications
4.1. Steady State Model
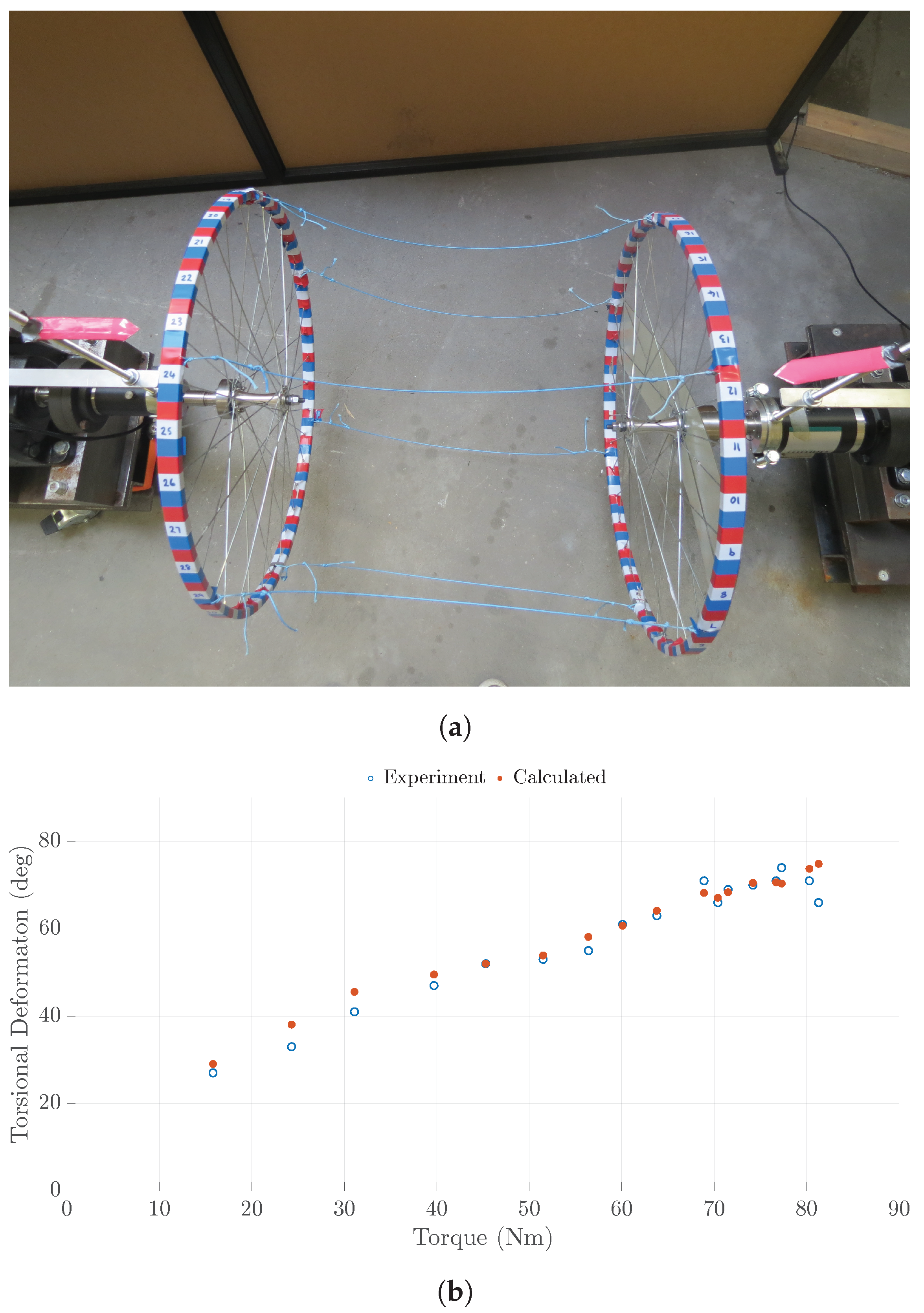
4.2. Spring-Disc Representation Compared to Field-Testing Data
4.2.1. Steady-State Response Testing
4.2.2. Dynamic Response Testing
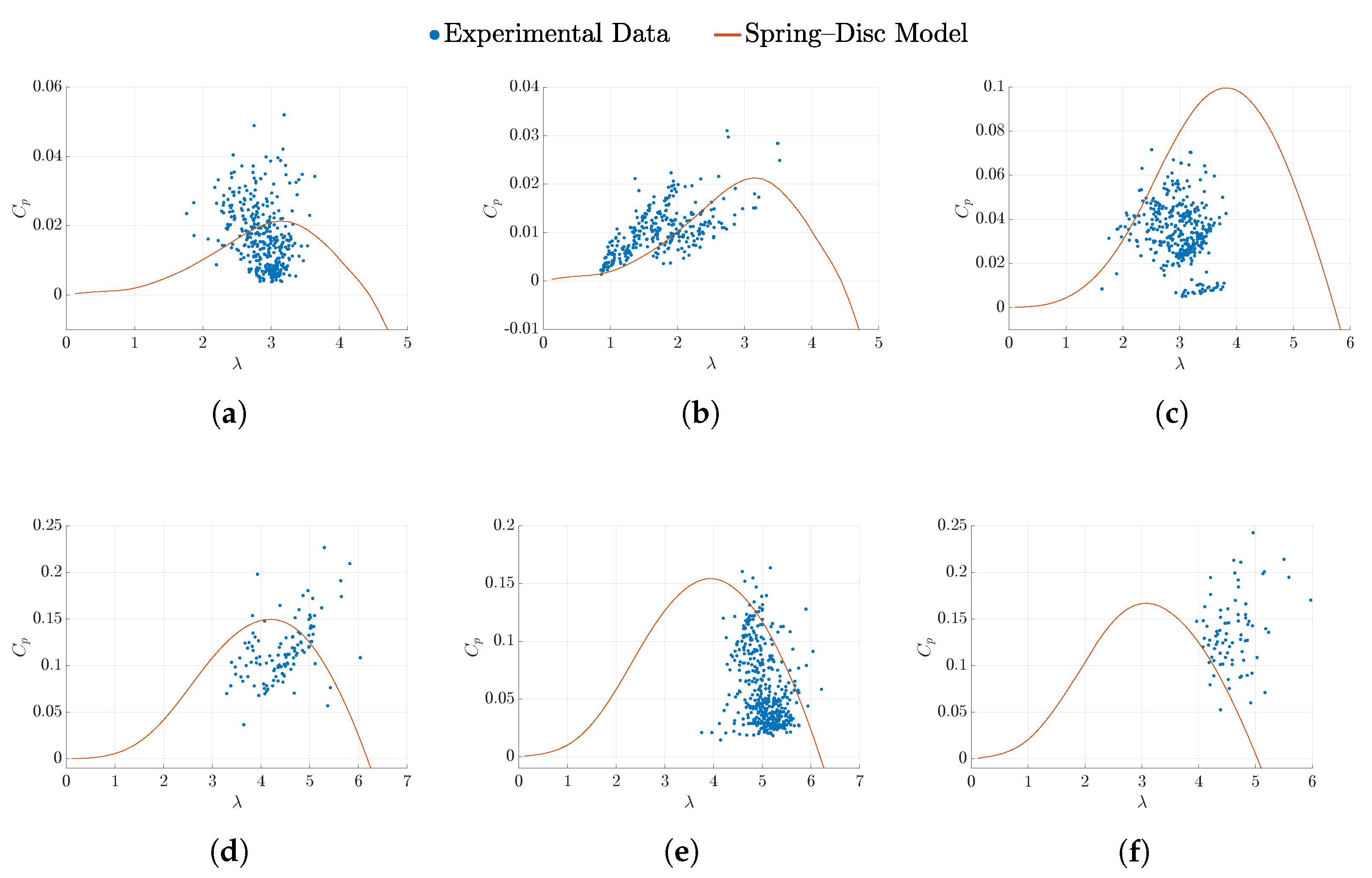
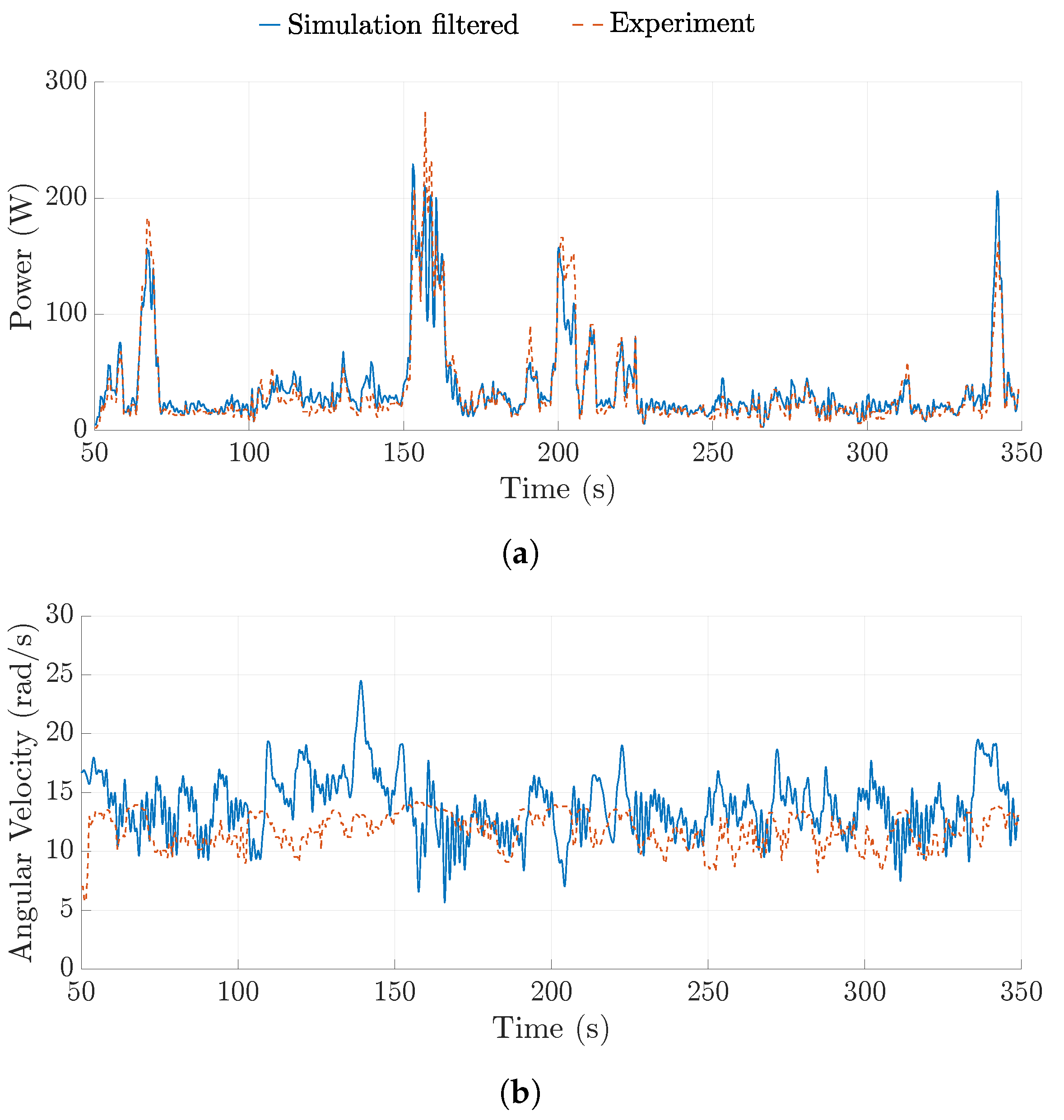
4.3. Multi-Spring Representation Compared to Field-Testing Data
4.3.1. Improving Computational Efficiency with Assumption of Rigid Wings
4.3.2. Multi-Spring Model Compared to Experimental Data
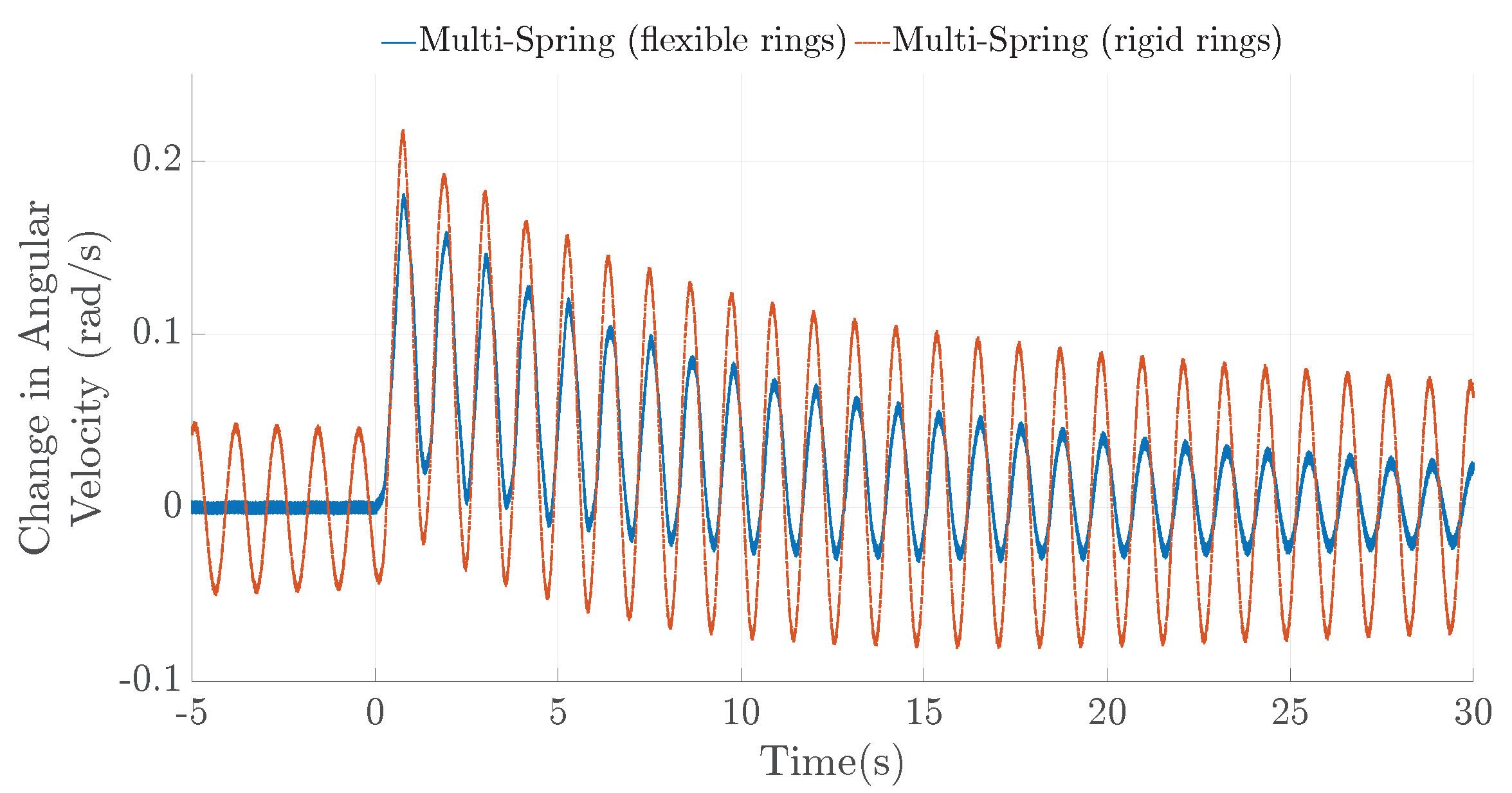
4.4. Comparison of Spring-Disc and Multi-Spring TRPT Models
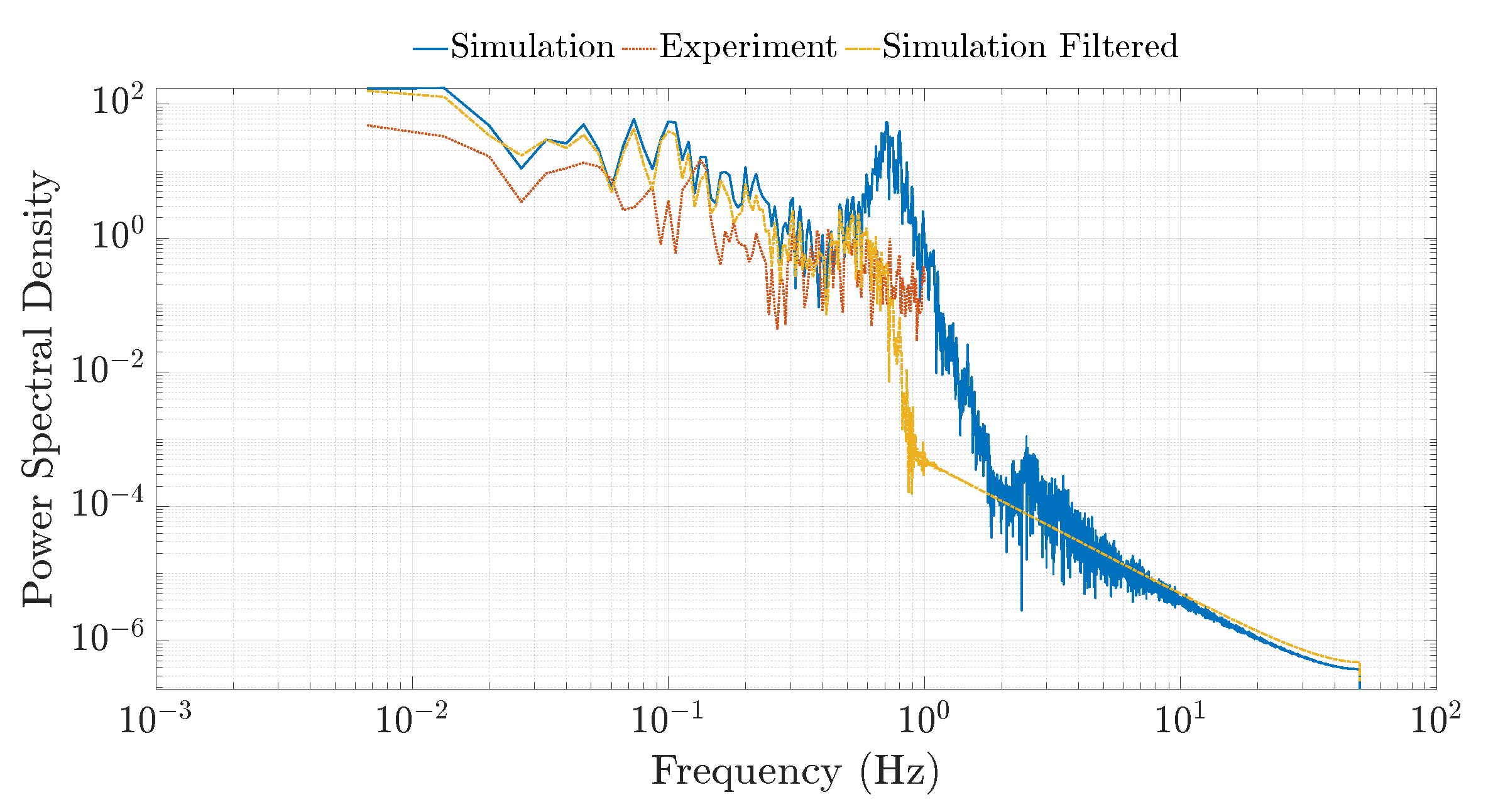
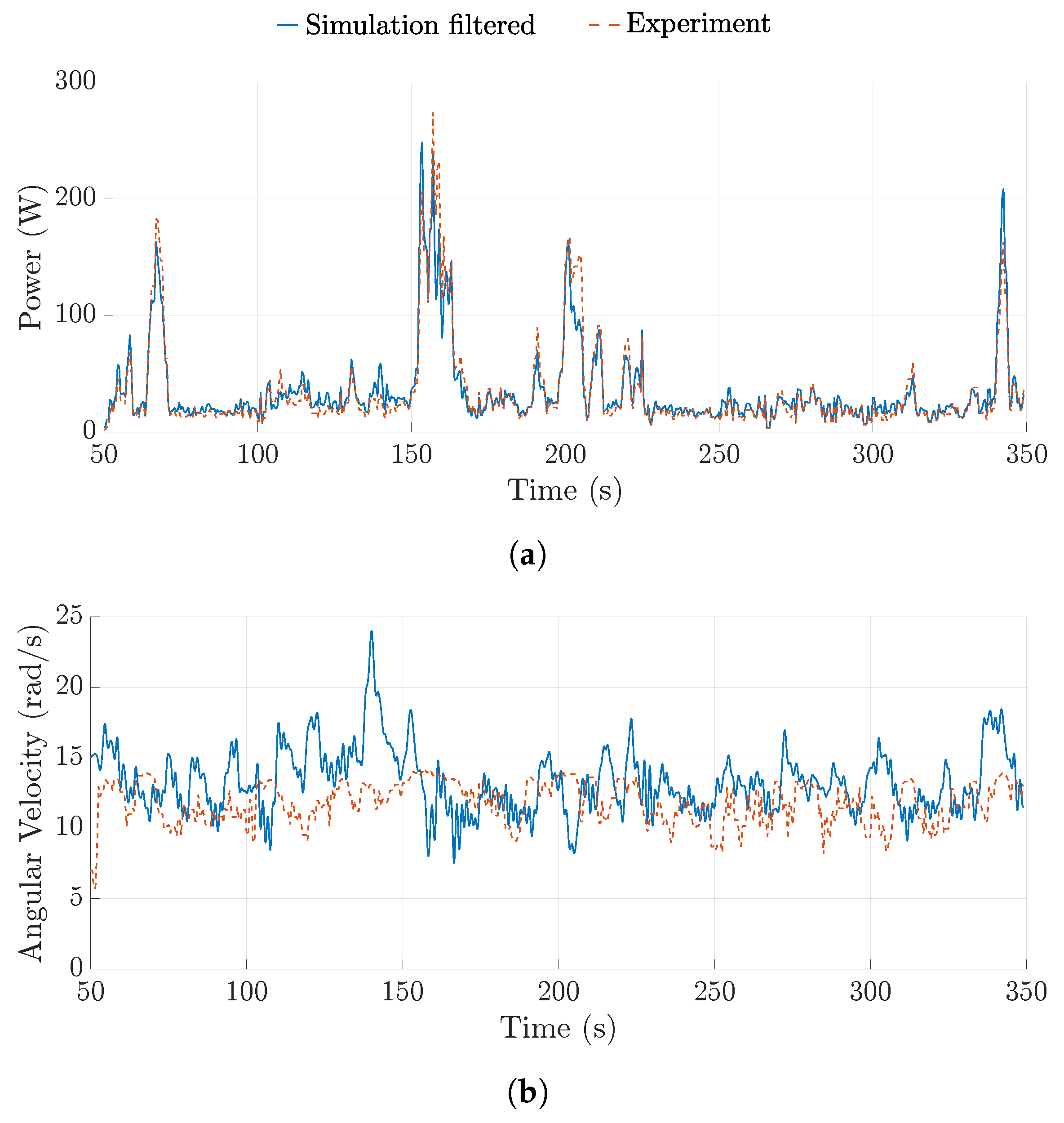
4.4.1. Response to Short-Term Step Changes in Torque and Tension
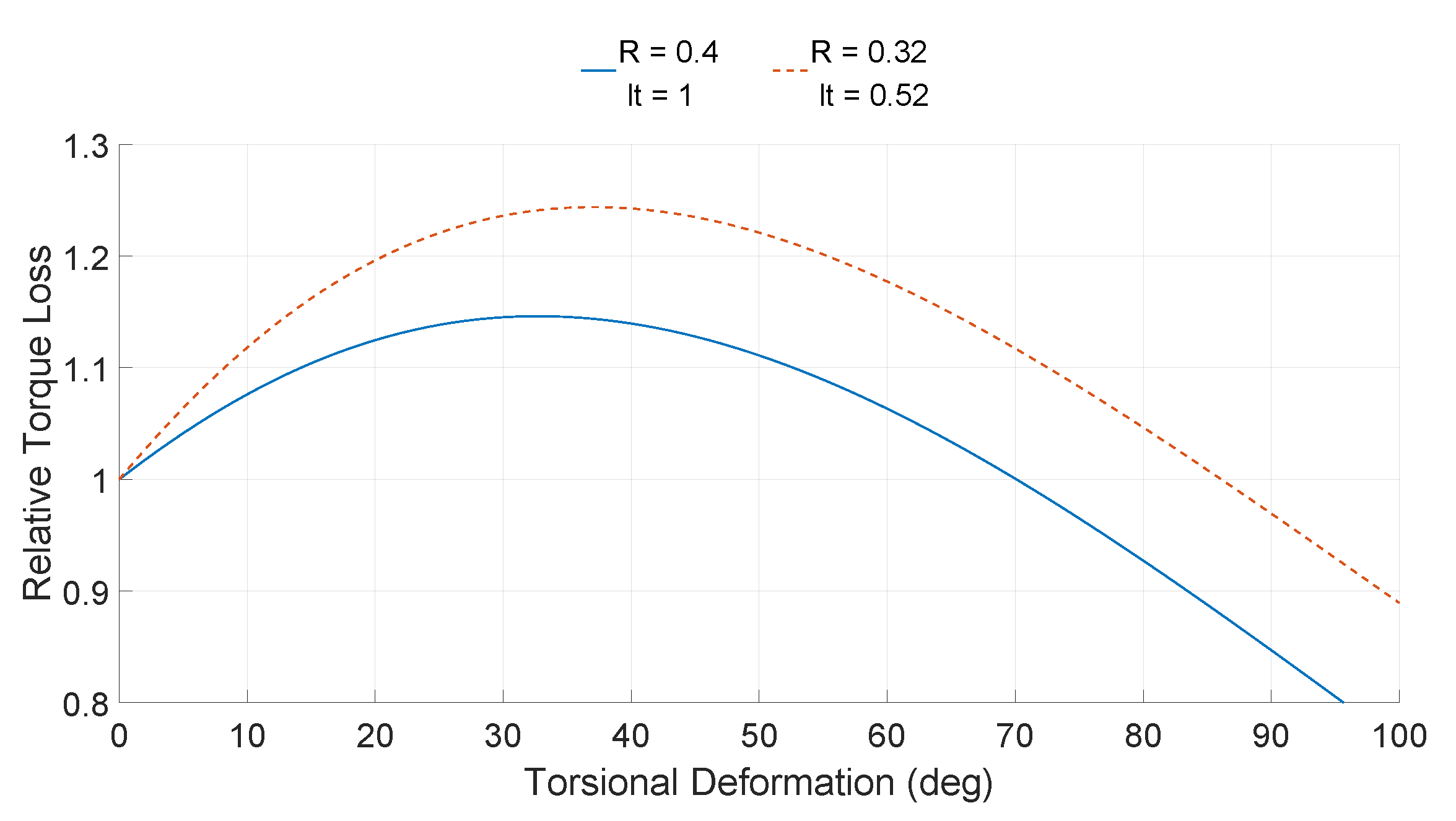
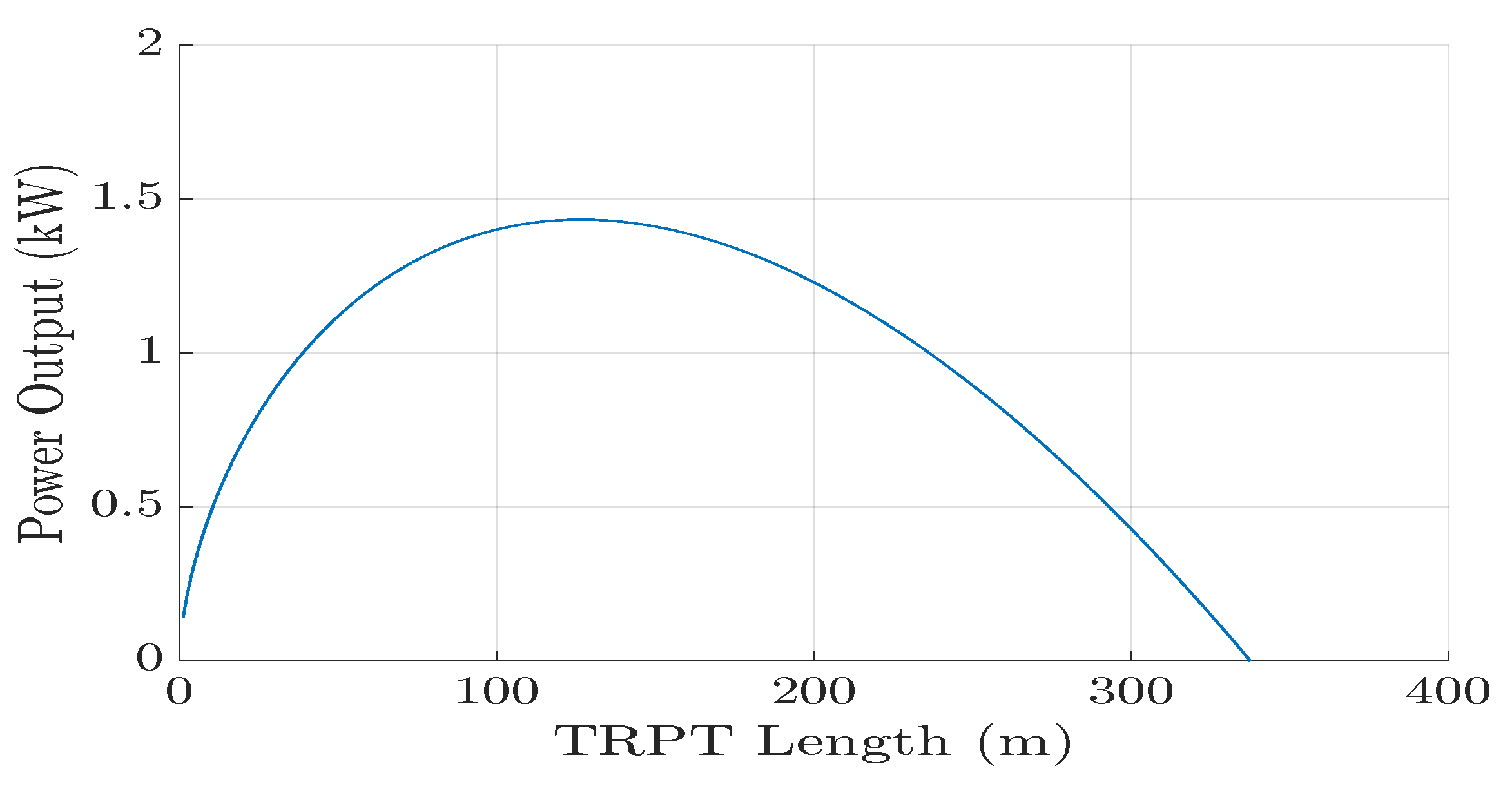
4.4.2. Impact of TRPT Length
4.4.3. A Few Remarks
5. System Analysis and Improved/Optimised Design
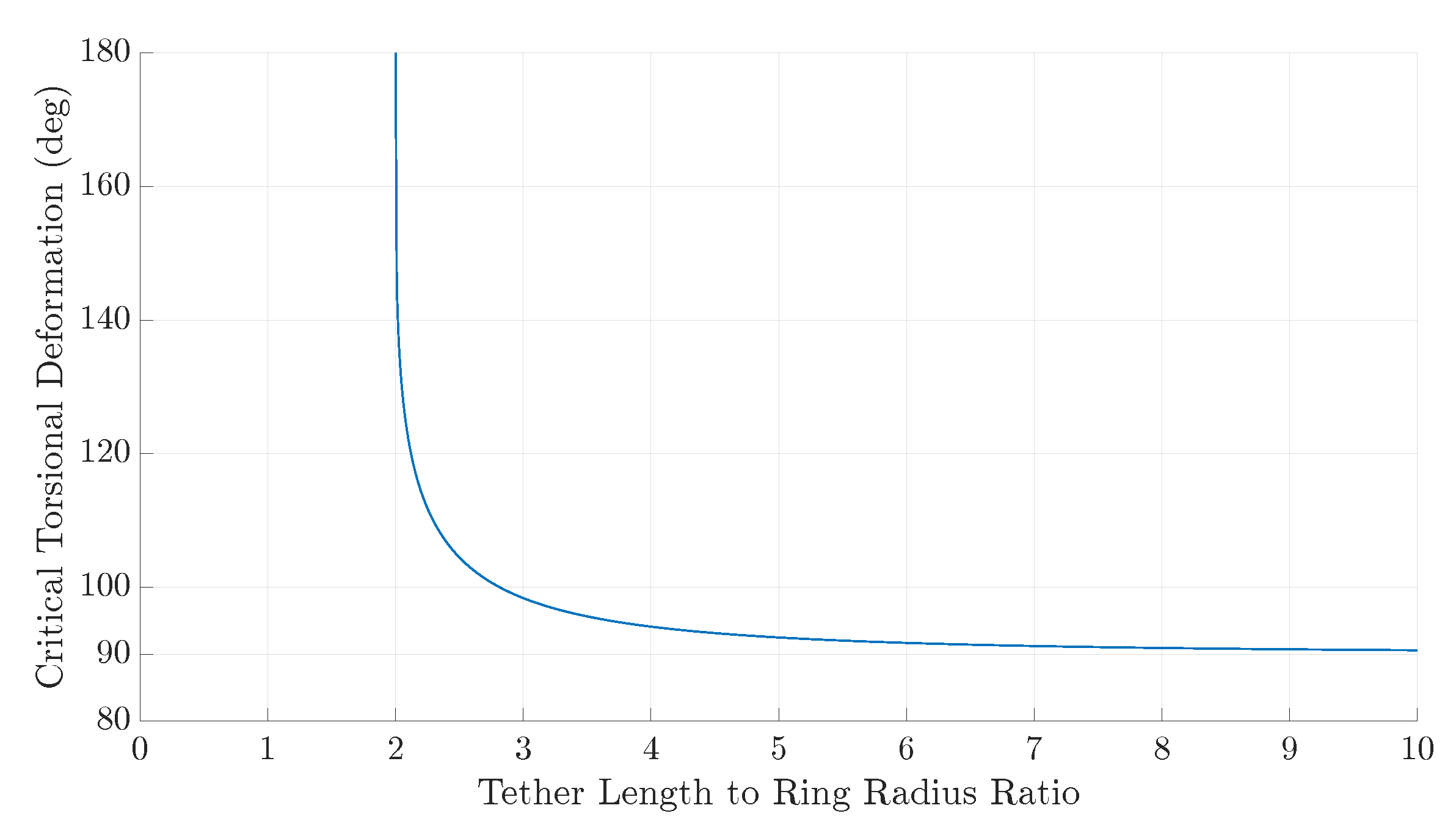
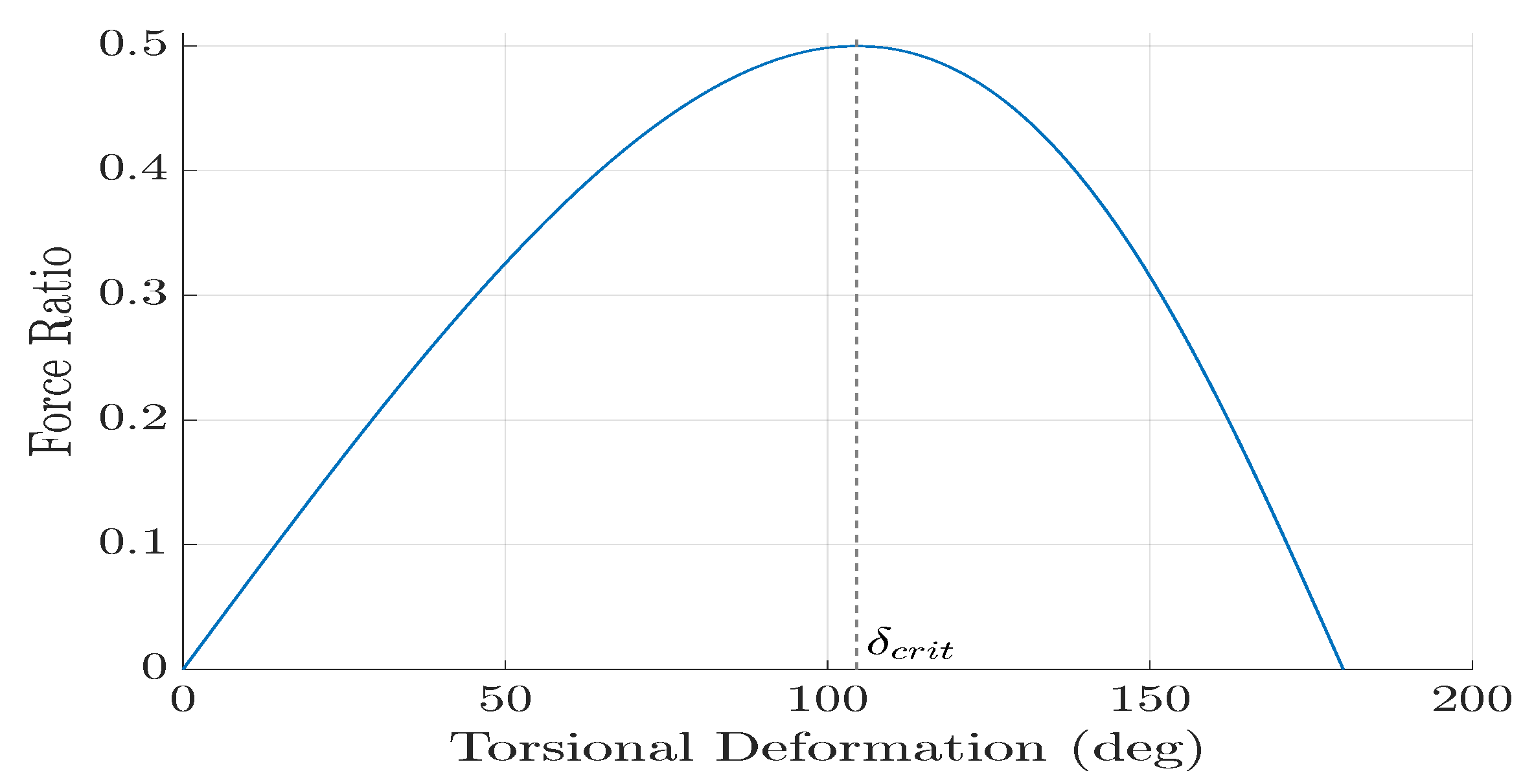
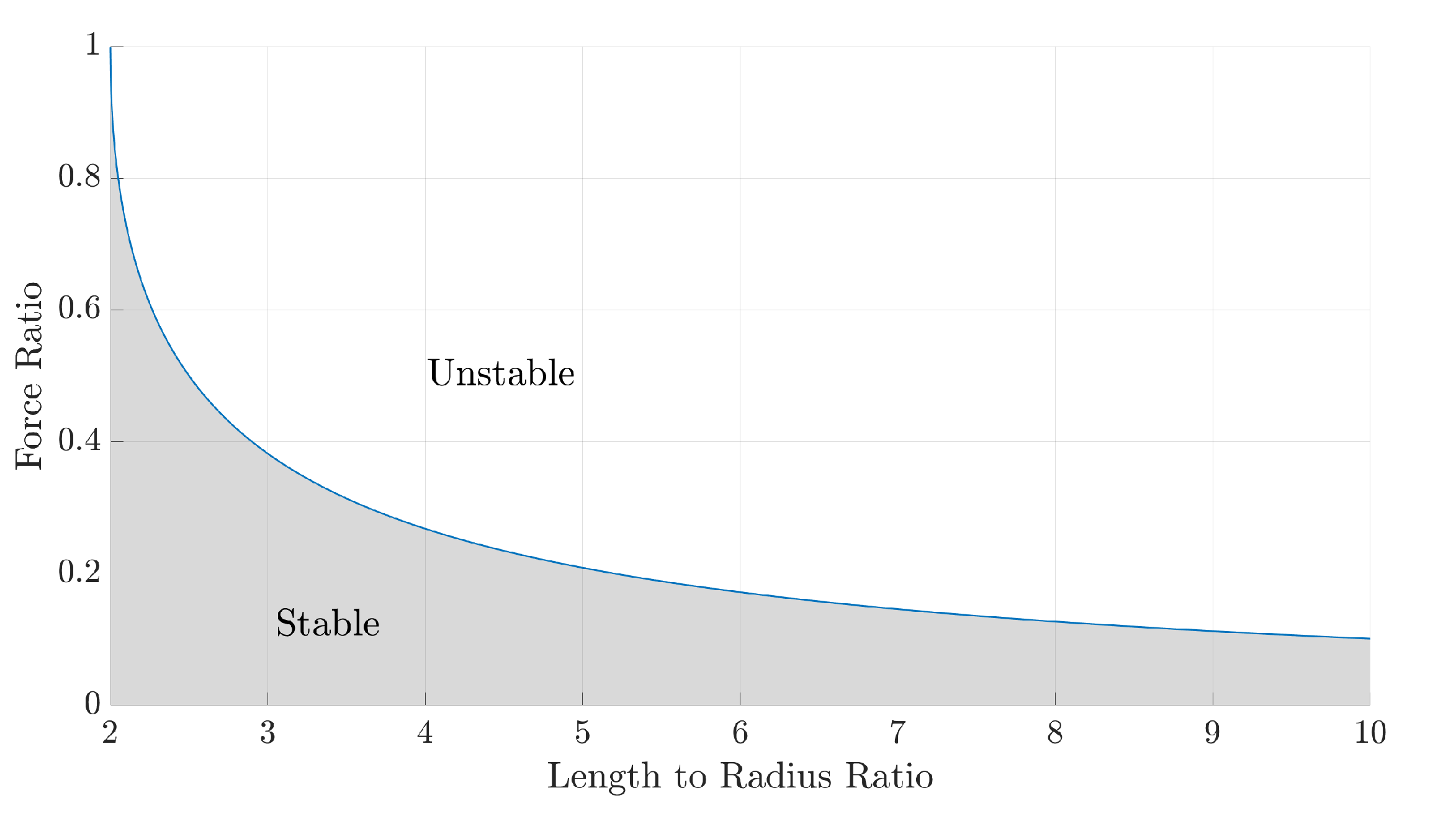
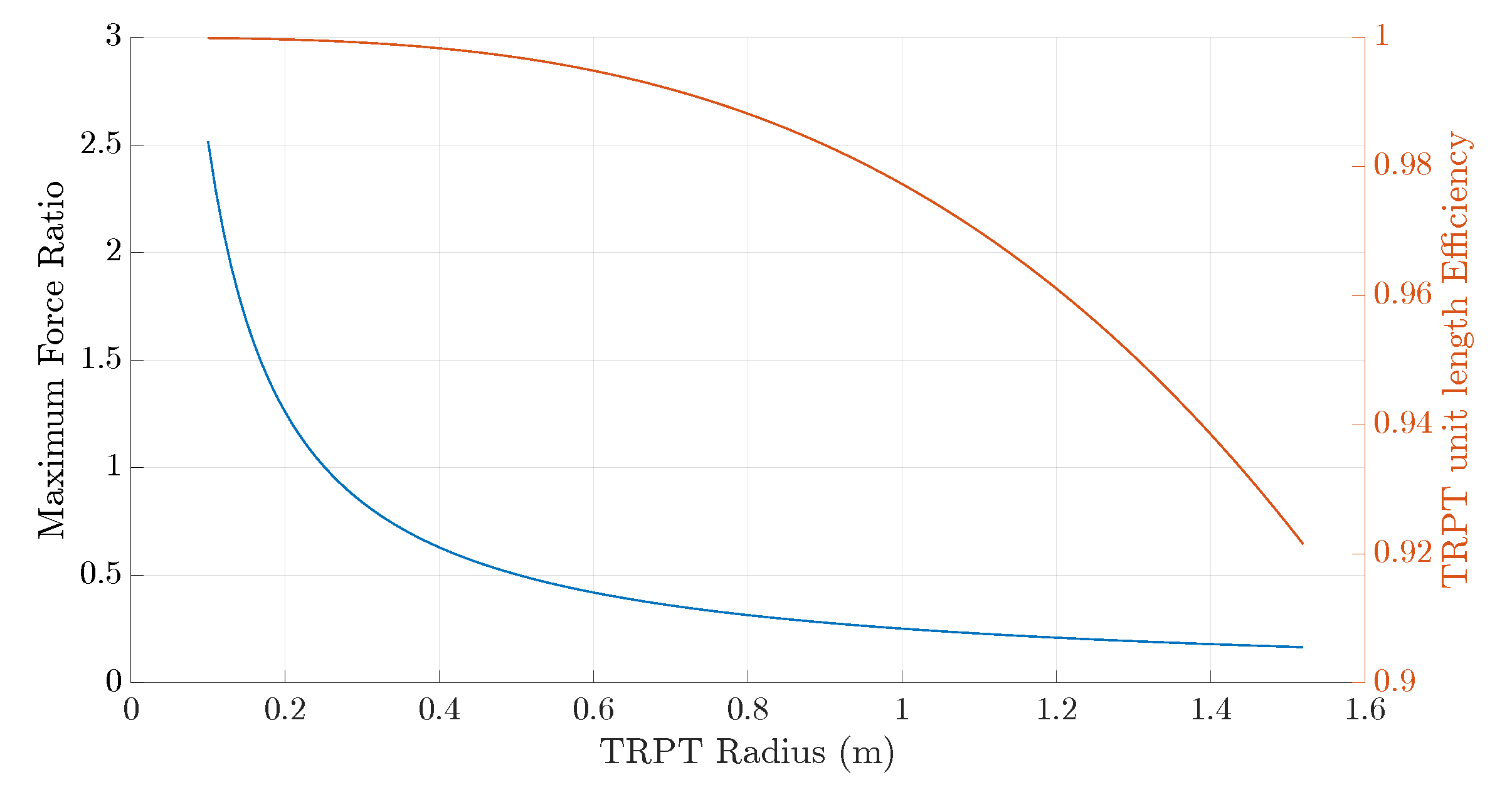
5.1. TRPT Design Analysis
5.2. Rotor Design Analysis
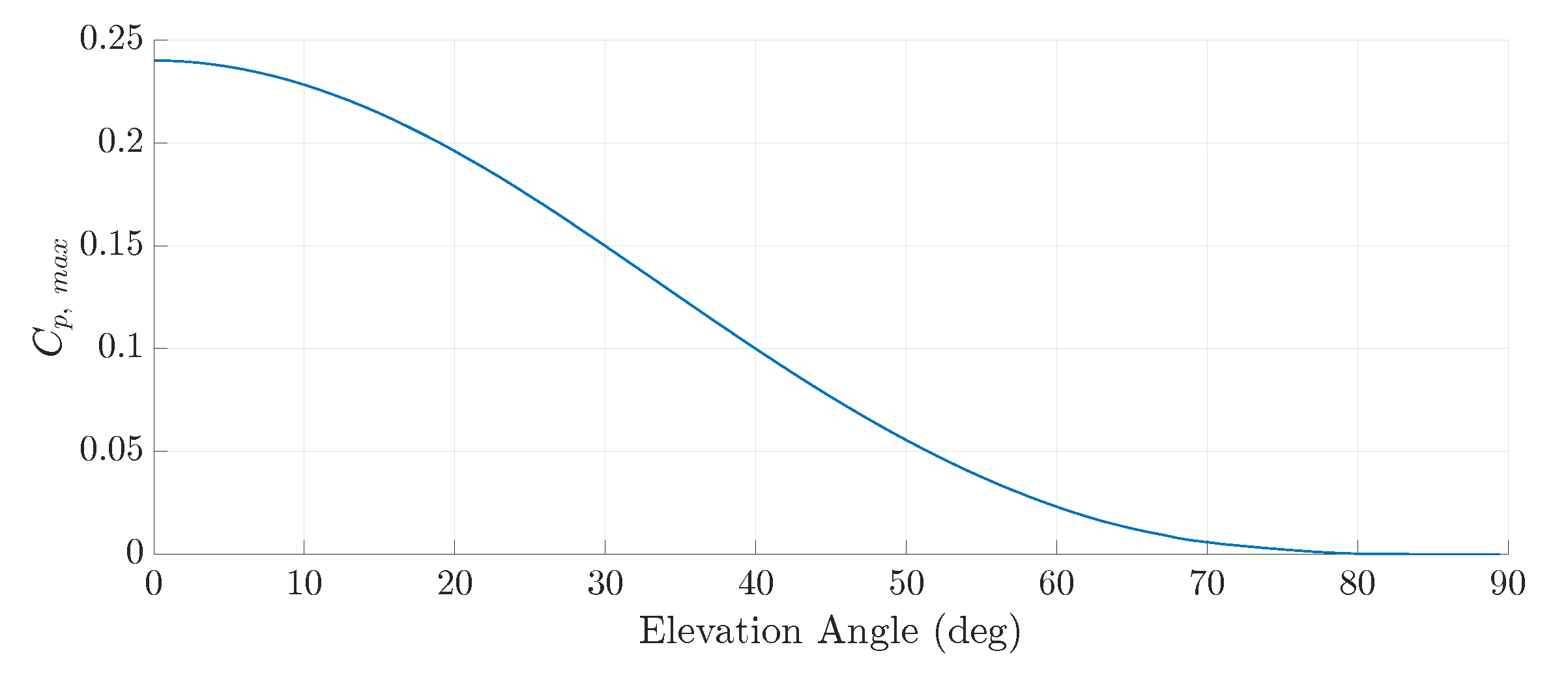
5.2.1. System Elevation Angle
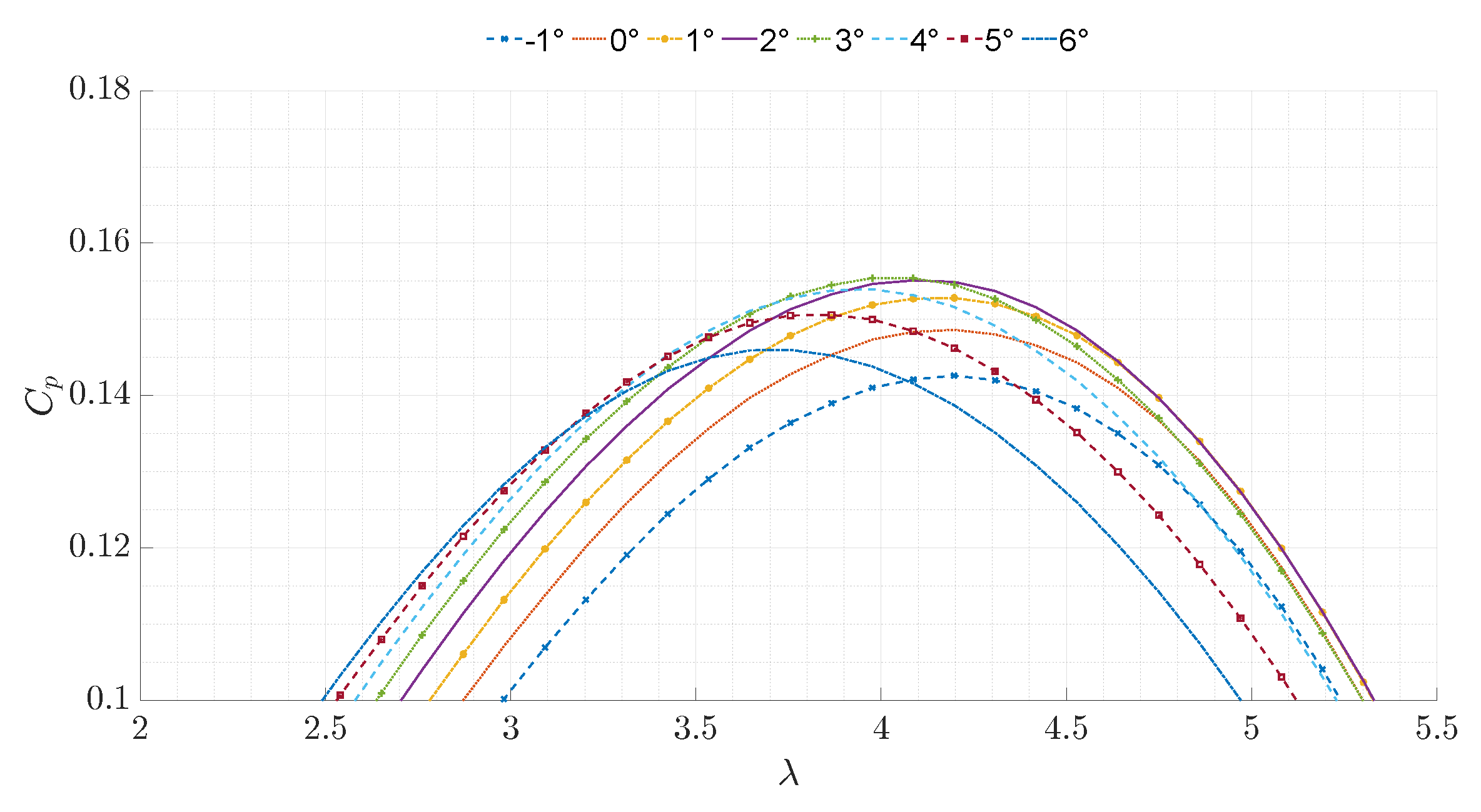
5.2.2. Blade Pitch Angle
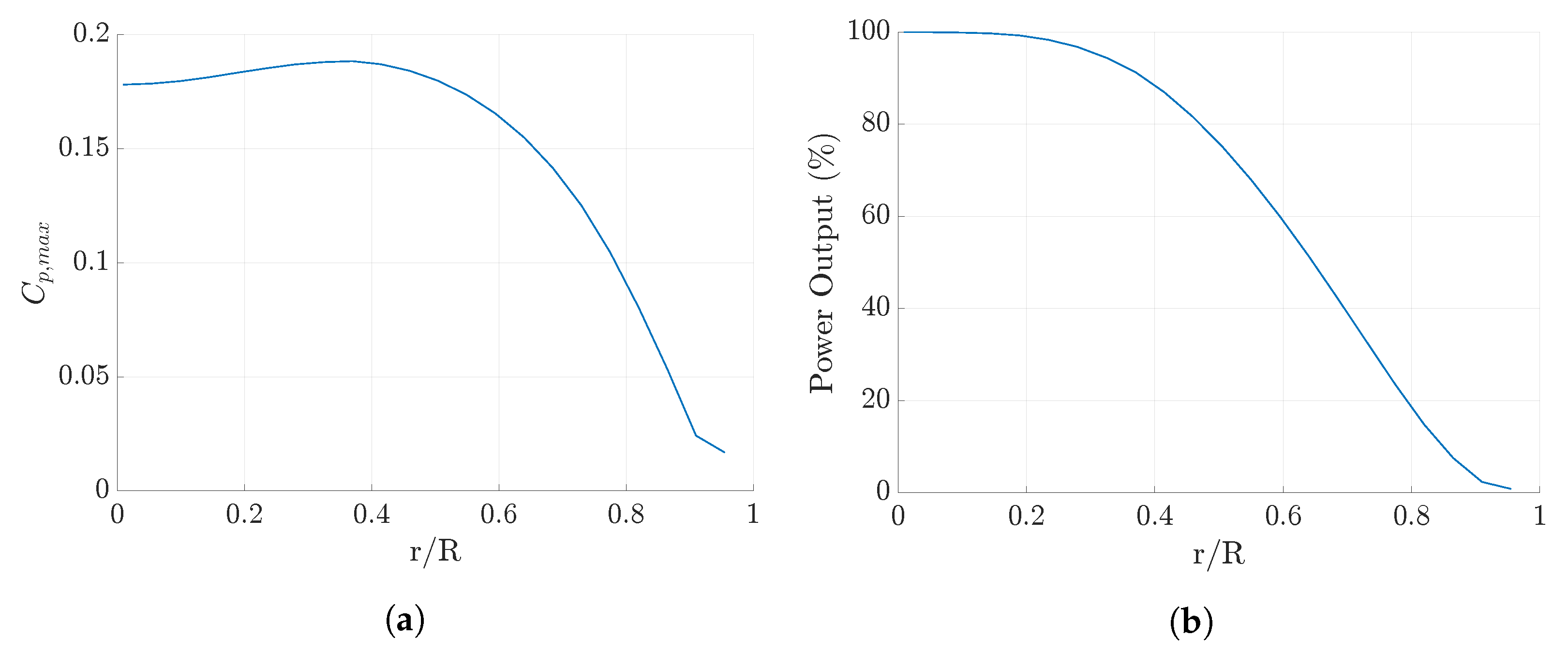
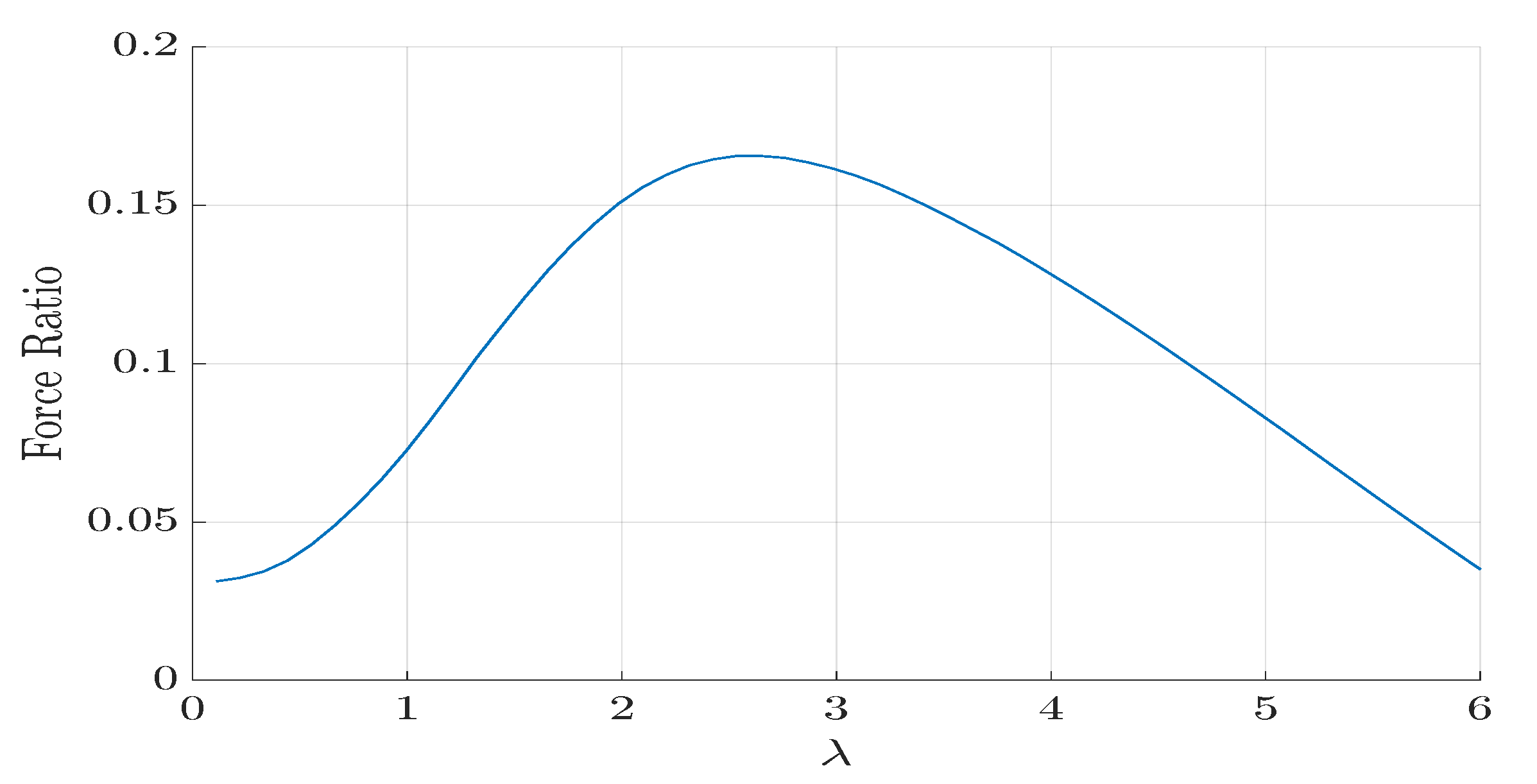
5.2.3. Blade Length
5.3. Tether-Drag Analysis
5.3.1. Analysis with Simple Tether-Drag Model
5.3.2. Analysis with Improved Tether-Drag Model
5.4. Optimised/Improved Design
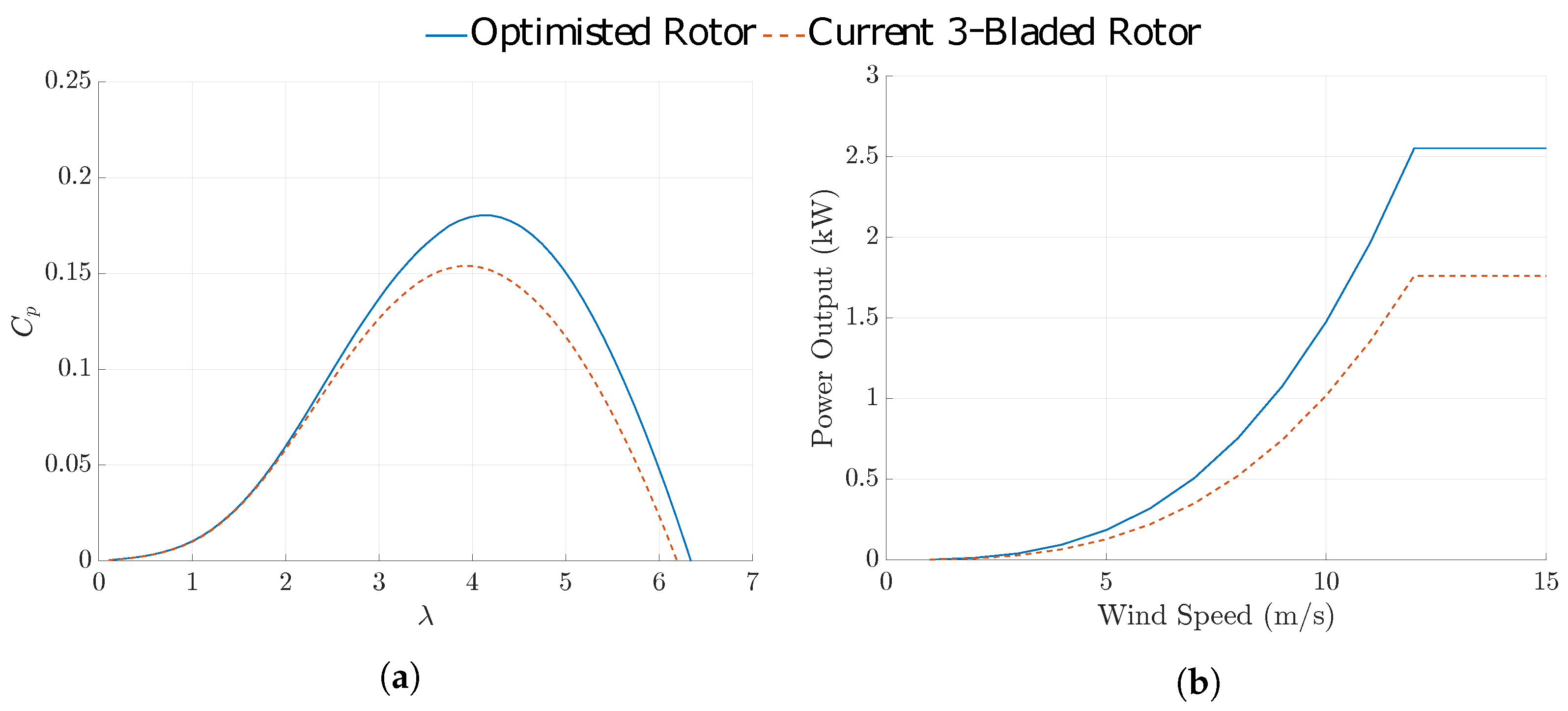
5.4.1. Optimised Rotor Design
5.4.2. Optimised TRPT Design
5.4.3. Optimised Elevation Angle and Tether Length
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| AWE(S) | Airborne wind energy (system) | BEM | Blade element momentum |
| DoF | Degree of freedom | EOM | Equation of motion |
| RMSE | Root mean square error | TRPT | Tensile rotary power Transmission |
Appendix A. Pseudo Codes of Model Development
Appendix A.1. Spring–Disc TRPT Modelling
| Inputs | Wind speed , TRPT geometry R and , elevation angle , initial conditions and , and generator torque |
| Line 1 | Find and |
| Line 2 | Find , and |
| Line 3 | Find , and |
| Line 4 | Find and |
| Line 5 | |
| Line 6 | |
| Line 7 | , |
| Line 8 | For each time step, i |
| Line 9 | Find , and |
| Line 10 | Find , and update |
| Line 11 | Find and |
| Line 12 | |
| Line 13 | |
| Line 14 | , |
| Line 15 | End For |
| Outputs | , , , , , , , |
Appendix A.2. Multi-Spring TRPT Modelling
| Inputs | Wind speed , TRPT geometry R and , elevation angle , initial conditions and , generator torque , and number of tethers . |
| Line 1 | Find and |
| Line 2 | Find , and |
| Line 3 | Find , and |
| Line 4 | Find |
| Line 5 | |
| Line 6 | |
| Line 7 | , |
| Line 8 | For each time step, i |
| Line 9 | Find , and |
| Line 10 | Find , and |
| Line 11 | Find |
| Line 12 | |
| Line 13 | |
| Line 14 | , |
| Line 15 | End For |
| Outputs | , , , , , , , , |
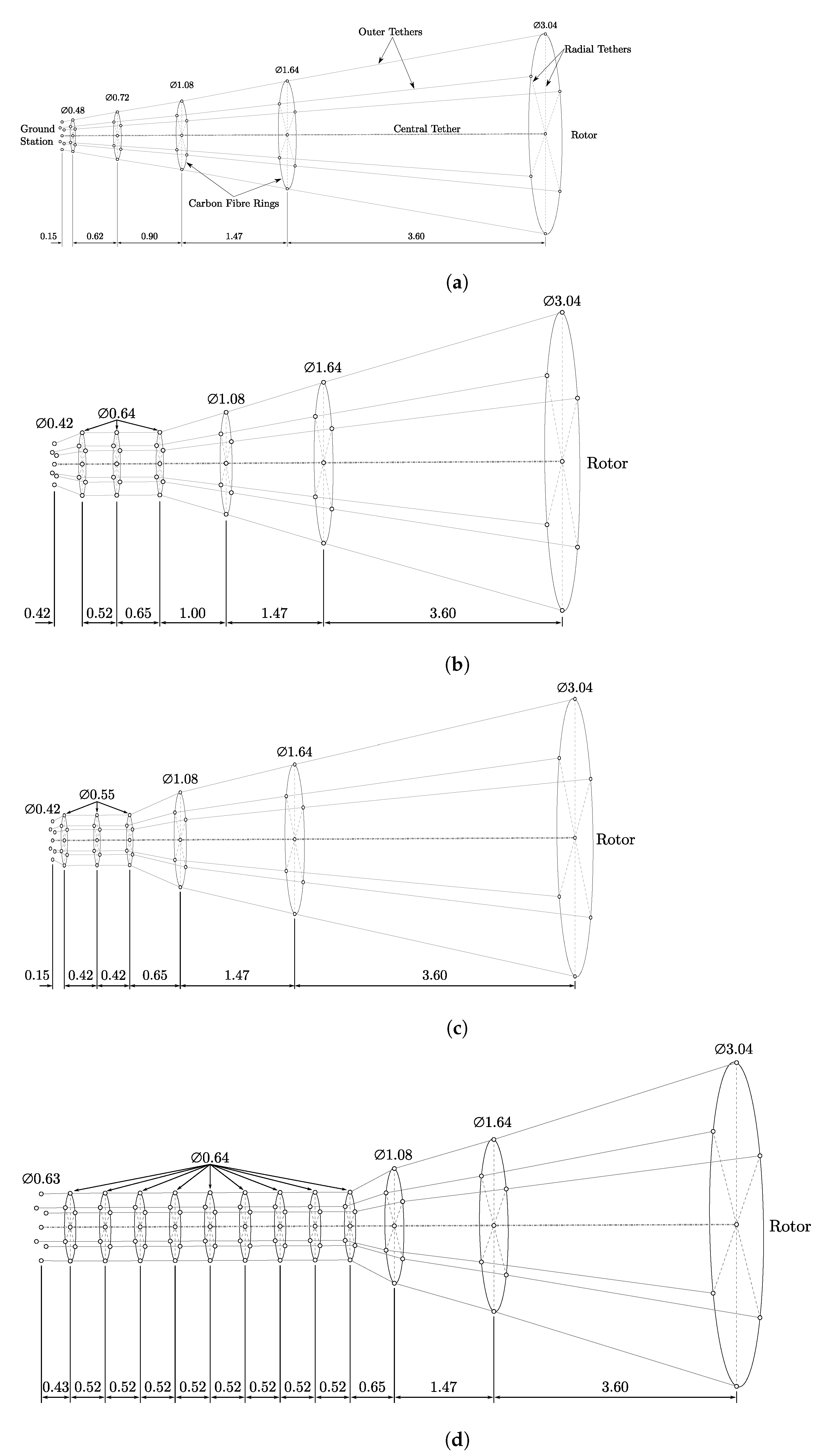
Appendix B. Four TRPT Configurations
Appendix C. Comparison of Multi-Spring Model and Experimental Data

References
- Bramwell, A.R.S.; Done, G.; Blamford, D. Bramwell’s Helicopter Dynamics; Butterworth-Heinemann: Oxford, UK, 2001. [Google Scholar]
- Read, R. Windswept & Interesting Ltd. Available online: https://www.windswept-and-interesting.co.uk/ (accessed on 10 January 2020).
- Read, R. Kite Networks for Harvesting Wind Energy. In Airborne Wind Energy: Advances in Technology Development and Research; Schmehl, R., Ed.; Springer: Singapore, 2018; pp. 515–537. [Google Scholar] [CrossRef]
- Tulloch, O. Modelling and Analysis of Rotary Airborne Wind Energy Systems—A Tensile Rotary Power Transmission Design. Ph.D. Thesis, University of Strathclyde, Glasgow, UK, 2021. [Google Scholar] [CrossRef]
- Malz, E. Airborne Wind Energy—To Fly or Not to Fly? A Study on the Power Production of Airborne Wind Energy Systems and Their Integration in the Electricity Generation System. Ph.D. Thesis, Chalmers University of Technology, Göteberg, Sweden, 2020. [Google Scholar] [CrossRef]
- De Schutter, J.; Leuthold, R.; Bronnenmeyer, T.; Paelinck, R.; Diehl, M. Optimal control of stacked multi-kite systems for utility-scale airborne wind energy. In Proceedings of the 2019 IEEE 58th Conference on Decision and Control (CDC), Nice, France, 11–13 December 2019; pp. 4865–4870. [Google Scholar] [CrossRef]
- Rimkus, S.; Das, T. An application of the autogyro theory to airborne wind energy extraction. In Proceedings of the ASME 2013 Dynamic Systems and Control Conference, DSCC 2013, American Society of Mechanical Engineers (ASME), Palo Alto, CA, USA, 21–23 October 2013; Volume 3. [Google Scholar] [CrossRef]
- Mackertich, S.; Das, T. A quantitative energy and systems analysis framework for airborne wind energy conversion using autorotation. In Proceedings of the 2016 American Control Conference (ACC). American Automatic Control Council (AACC), Boston, MA, USA, 6–8 July 2016; pp. 4996–5001. [Google Scholar] [CrossRef]
- Rancourt, D.; Bolduc-Teasdale, F.; Bouchard, E.D.; Anderson, M.J.; Mavris, D.N. Design space exploration of gyrocopter-type airborne wind turbines. Wind Energy 2016, 19, 895–909. [Google Scholar] [CrossRef]
- Benhaïem, P.; Schmehl, R. Airborne wind energy conversion using a rotating reel system. In Airborne Wind Energy: Advances in Technology Development and Research; Schmehl, R., Ed.; Springer: Singapore, 2018; pp. 539–577. [Google Scholar] [CrossRef]
- Roberts, B.W. Quad-rotorcraft to harness high-altitude wind energy. In Airborne Wind Energy: Advances in Technology Development and Research; Schmehl, R., Ed.; Springer: Singapore, 2018. [Google Scholar] [CrossRef]
- Schutter, J.D.; Leuthold, R.; Diehl, M. Optimal control of a rigid-wing rotary kite system for airborne wind energy. In Proceedings of the 2018 European Control Conference (ECC), Limassol, Cyprus, 12–15 June 2018; pp. 1734–1739. [Google Scholar] [CrossRef]
- Beaupoil, C. Practical experiences with a torsion based rigid blade rotary airborne wind energy system with ground based power generation. In Proceedings of the 8th International Airborne Wind Energy Conference (AWEC2019), Glasgow, UK, 15–16 October 2019; Schmehl, R., Tulloch, O., Eds.; Delft University of Technology and University of Strathclyde: Glasgow, UK, 2019; pp. 146–147. [Google Scholar]
- Selsam, D. Selsam—Innovation in Alternative Energy. Available online: http://www.selsam.com (accessed on 4 December 2017).
- Sky WindPower. Available online: https://www.skywindpower.com (accessed on 27 March 2019).
- Ippolito, M. KiteGen Carousel. Available online: http://www.kitegen.com/en/products/kite-gen-carousel (accessed on 21 March 2021).
- Castellani, F.; Astolfi, D.; Natili, F.; Mari, F. The yawing behavior of horizontal-axis wind turbines: A numerical and experimental analysis. Machines 2019, 7, 15. [Google Scholar] [CrossRef]
- Micallef, D.; Kloosterman, M.; Ferreira, C.S.; Sant, T.; van Bussel, G.; Micallef, D. Comparison and validation of BEM and free wake unsteady panel model with the Mexico rotor experiment. In Proceedings of the Euromech, Madrid, Spain, 20–22 October 2009; Volume 508. Available online: http://resolver.tudelft.nl/uuid:33950a07-dbaf-492b-bfec-8833984afd2f (accessed on 21 March 2021).
- Rahimi, H.; Hartvelt, M.; Peinke, J.; Schepers, J. Investigation of the current yaw engineering models for simulation of wind turbines in BEM and comparison with CFD and experiment. J. Phys. Conf. Ser. 2016, 753, 022016. [Google Scholar] [CrossRef]
- Micallef, D.; Sant, T. A review of wind turbine yaw aerodynamics. In Wind Turbines; Aissaoui, A.G., Tahour, A., Eds.; IntechOpen: Rijeka, Croatia, 2016; Chapter 2. [Google Scholar] [CrossRef]
- Ghoreyshi, M.; Bergeron, K.; Seidel, J.; Jirasek, A.; Lofthouse, A.J.; Cummings, R.M. Prediction of aerodynamic characteristics of ram-air parachutes. J. Aircr. 2016, 53, 1802. [Google Scholar] [CrossRef]
- Hansen, C. AirfoilPrep. Available online: https://nwtc.nrel.gov/AirFoilPrep (accessed on 4 July 2020).
- Ostowari, C.; Naik, D. Post-Stall Wind Tunnel Data for NACA 44xx Series Airfoil Sections; Technical Report; Department of Aerospace Engineering, Texas A and M University: College Station, TX, USA, 1985. [Google Scholar] [CrossRef]
- NACA 4412 (naca4412-il) Xfoil Prediction Polar at RE=200,000 Ncrit=5. Available online: http://airfoiltools.com/polar/details?polar=xf-naca4412-il-200000-n5 (accessed on 4 April 2020).
- Manwell, J.; McGowan, J.; Rogers, A. Wind Energy Explained; Wiley: Hoboken, NJ, USA, 2002. [Google Scholar]
- Jonkman, B.; Kilcher, L. TurbSim User’s Guide: Version 1.06. 00; National Renewable Energy Laboratory: Golden, CO, USA, 2012.
- IEC 61400-1; Wind Turbines–Part 1: Design Requirements. IEC: Geneva, Switzerland, 2005.
- Tulloch, O.; Amiri, A.K.; Yue, H.; Feuchtwang, J.; Read, R. Tensile rotary power transmission model development for airborne wind energy systems. J. Phys. Conf. Ser. 2020, 1618, 032001. [Google Scholar] [CrossRef]
- Chopra, A.K. Dynamics of Structures Theory and Applications to Earthquake Engineering; Prentice Hall: Hoboken, NJ, USA, 1995. [Google Scholar] [CrossRef]
- Zanon, M.; Gros, S.; Andersson, J.; Diehl, M. Airborne wind energy based on dual airfoils. IEEE Trans. Control Syst. Technol. 2013, 21, 1215–1222. [Google Scholar] [CrossRef]
- Dunker, S. Tether and bridle line drag in airborne wind energy applications. In Airborne Wind Energy: Advances in Technology Development and Research; Schmehl, R., Ed.; Springer: Singapore, 2018; pp. 29–56. [Google Scholar] [CrossRef]
- Bootle, W. Forces on an inclined circular cylinder in supercritical flow. AIAA J. 1971, 9, 514–516. [Google Scholar] [CrossRef]
- DSM. Dyneema. Available online: https://www.dsm.com/products/dyneema/en_GB/home.html (accessed on 17 February 2020).
- Carbon Fibre Profiles. Available online: https://www.carbonfibreprofiles.com/ (accessed on 17 February 2020).
- Sanborn, B.; Dileonardi, A.M.; Weerasooriya, T. Tensile properties of dyneema SK76 single fibers at multiple loading rates using a direct gripping method. J. Dyn. Behav. Mater. 2014, 1, 4–14. [Google Scholar] [CrossRef]









| Case | Test Date | Wing | TRPT | Wind Speed (m/s) | Power Output (w) | 1st Natural Frequency (Hz) |
|---|---|---|---|---|---|---|
| 1 | 8 September 2019 | Rigid | 4 | 5.3 | 35 | 0.74 |
| 2 | 20 September 2018 | Rigid | 3 | 6.1 | 50 | 1.43 |
| 3 | 27 August 2018 | Rigid | 3 | 2.7 | 10 | 0.73 |
| 4 | 6 May 2018 | Soft | 2 | 5.8 | 10 | 1.47 |
| 5 | 18 June 2017 | Soft | 1 | 5.5 | 15 | 1.52 |
| Wind Speed (m/s) | Change in Torque RMSE | Change in Tension RMSE |
|---|---|---|
| 6 | 0.056 | 0.332 |
| 8 | 0.038 | 0.271 |
| 10 | 0.019 | 0.186 |
| 12 | 0.019 | 0.119 |
| Models | Torque Loss (Nm) | TRPT–4 Efficiency (%) |
|---|---|---|
| Simple tether-drag model | 7.6 | 83.2 |
| Improved tether-drag model ( neglected) | 4.9 | 89.2 |
| Improved tether-drag model | 5.1 | 88.6 |
| Rotor Radius | Blade Length | TRPT Radius | TRPT Section Length | Elevation Angle | TRPT Total Length | Tip Speed Ratio |
|---|---|---|---|---|---|---|
| 2.22 m | 1.4 m | 0.5 m | 1.25 m | 18.5 | 190 m | 3.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tulloch, O.; Yue, H.; Kazemi Amiri, A.M.; Read, R. A Tensile Rotary Airborne Wind Energy System—Modelling, Analysis and Improved Design. Energies 2023, 16, 2610. https://doi.org/10.3390/en16062610
Tulloch O, Yue H, Kazemi Amiri AM, Read R. A Tensile Rotary Airborne Wind Energy System—Modelling, Analysis and Improved Design. Energies. 2023; 16(6):2610. https://doi.org/10.3390/en16062610
Chicago/Turabian StyleTulloch, Oliver, Hong Yue, Abbas Mehrad Kazemi Amiri, and Roderick Read. 2023. "A Tensile Rotary Airborne Wind Energy System—Modelling, Analysis and Improved Design" Energies 16, no. 6: 2610. https://doi.org/10.3390/en16062610
APA StyleTulloch, O., Yue, H., Kazemi Amiri, A. M., & Read, R. (2023). A Tensile Rotary Airborne Wind Energy System—Modelling, Analysis and Improved Design. Energies, 16(6), 2610. https://doi.org/10.3390/en16062610









