Polymer-Coated Nanoparticles and Pickering Emulsions as Agents for Enhanced Oil Recovery: Basic Studies Using a Porous Medium Model †
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
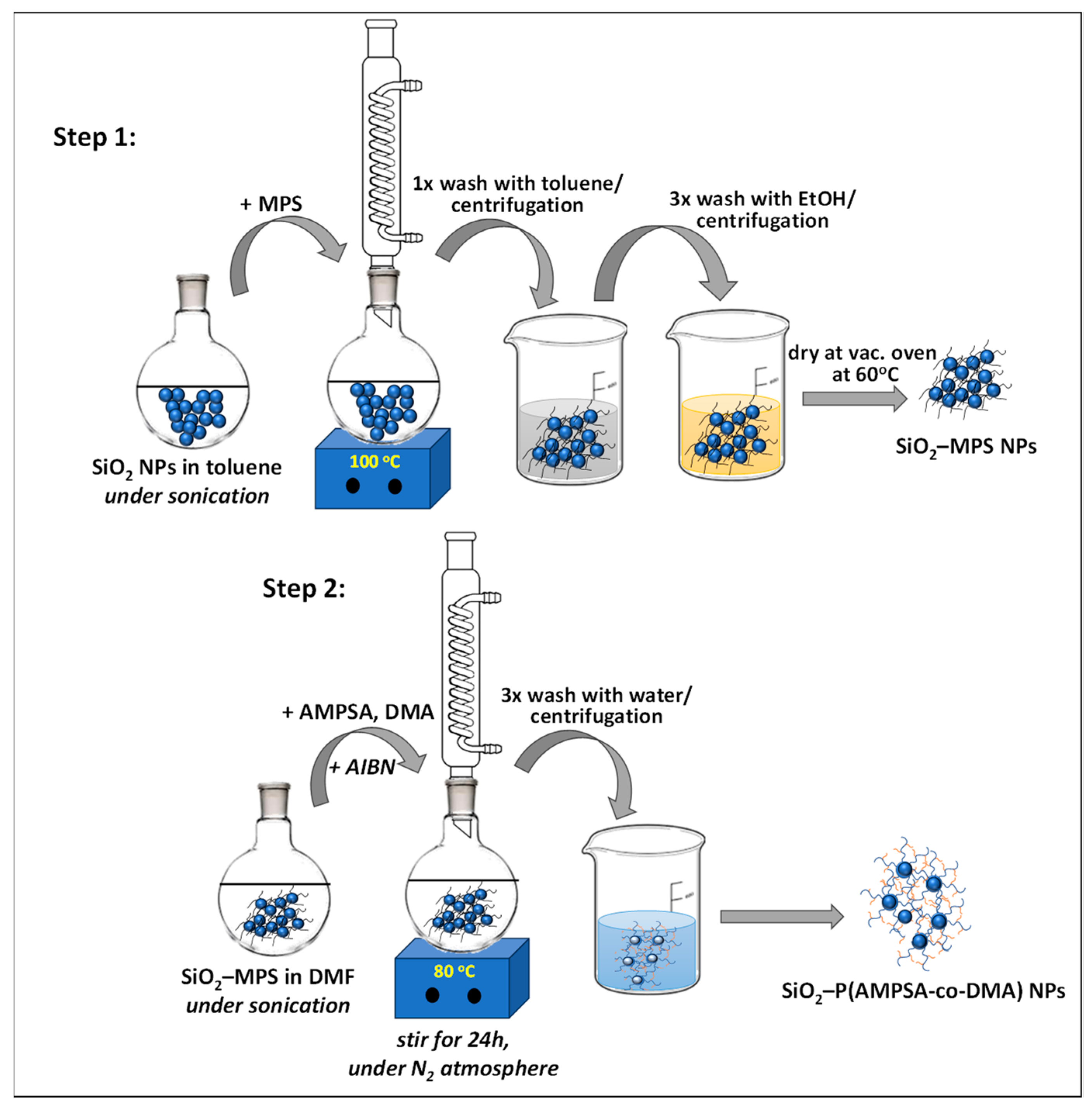
2.2. Functionalization of SiO2 NPs with 3-(trimethoxysilyl)-propyl methacrylate (SiO2-MPS)
2.3. Copolymerization of AMPSA and DMA onto the Modified SiO2-MPS NPs (SiO2-P(AMPSA-co-DMA))
2.4. Characterization of PNPs and Dispersions
2.5. Synthesis and Characterization of Pickering Emulsions
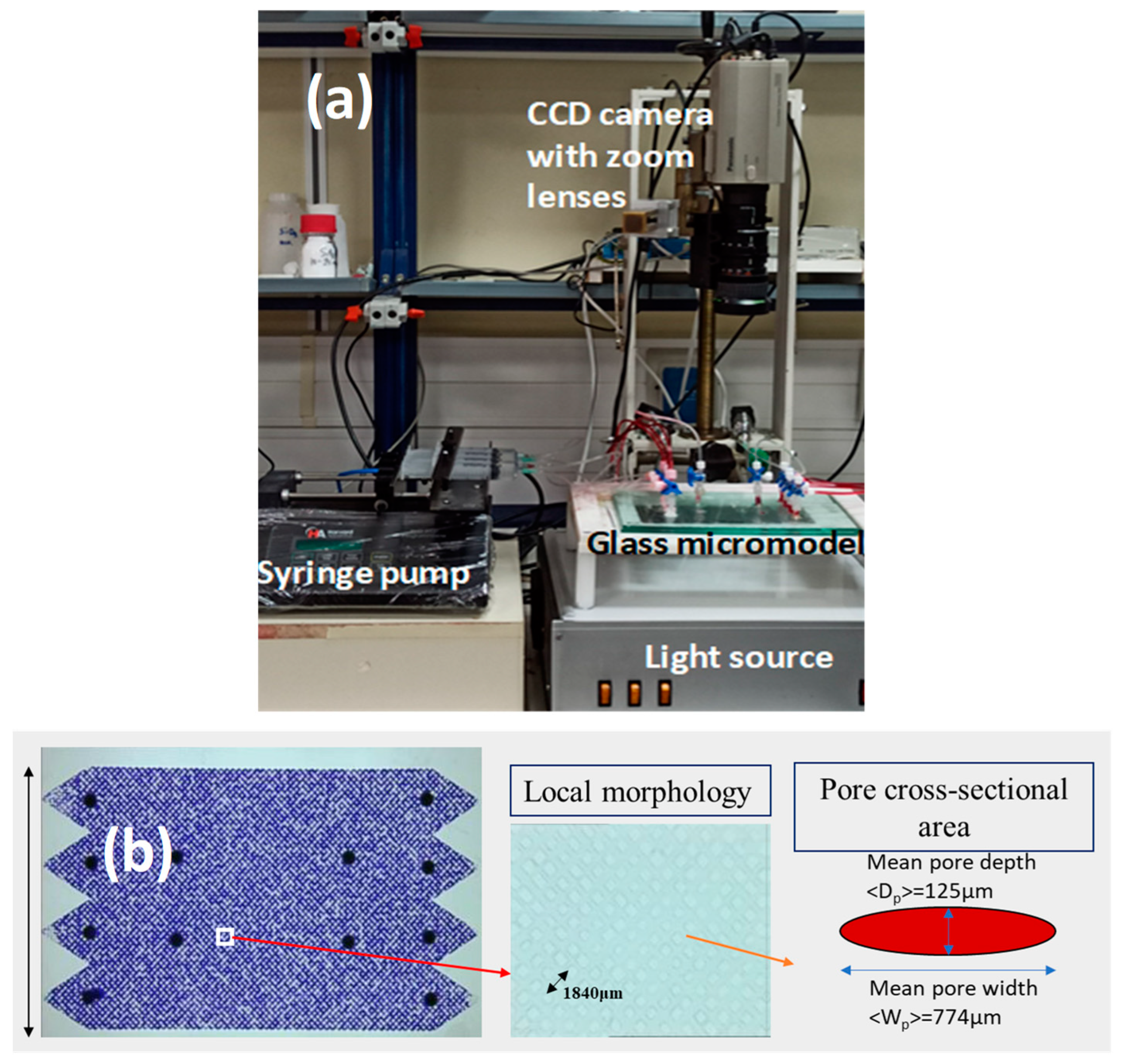
2.6. Visualization EOR Tests in a Glass-Etched Pore Network
3. Results and Discussion
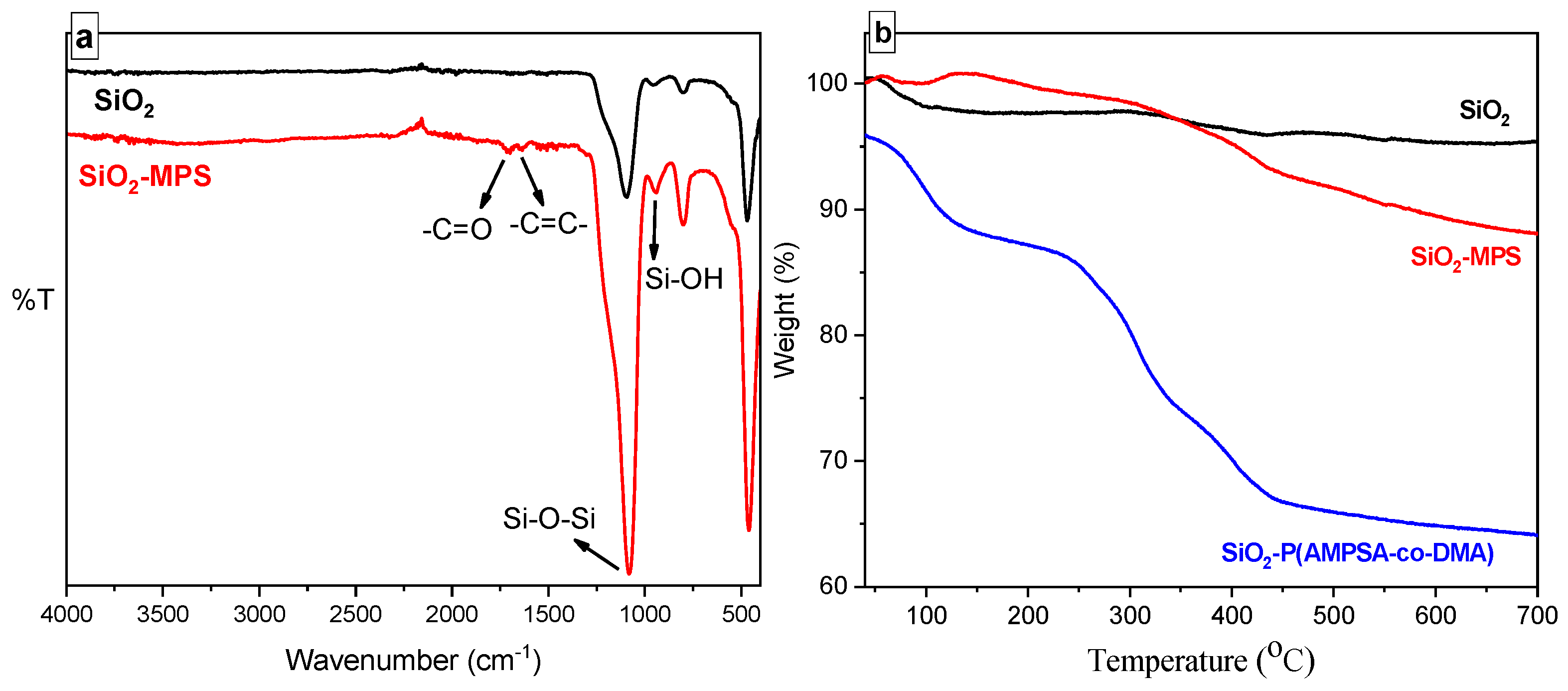
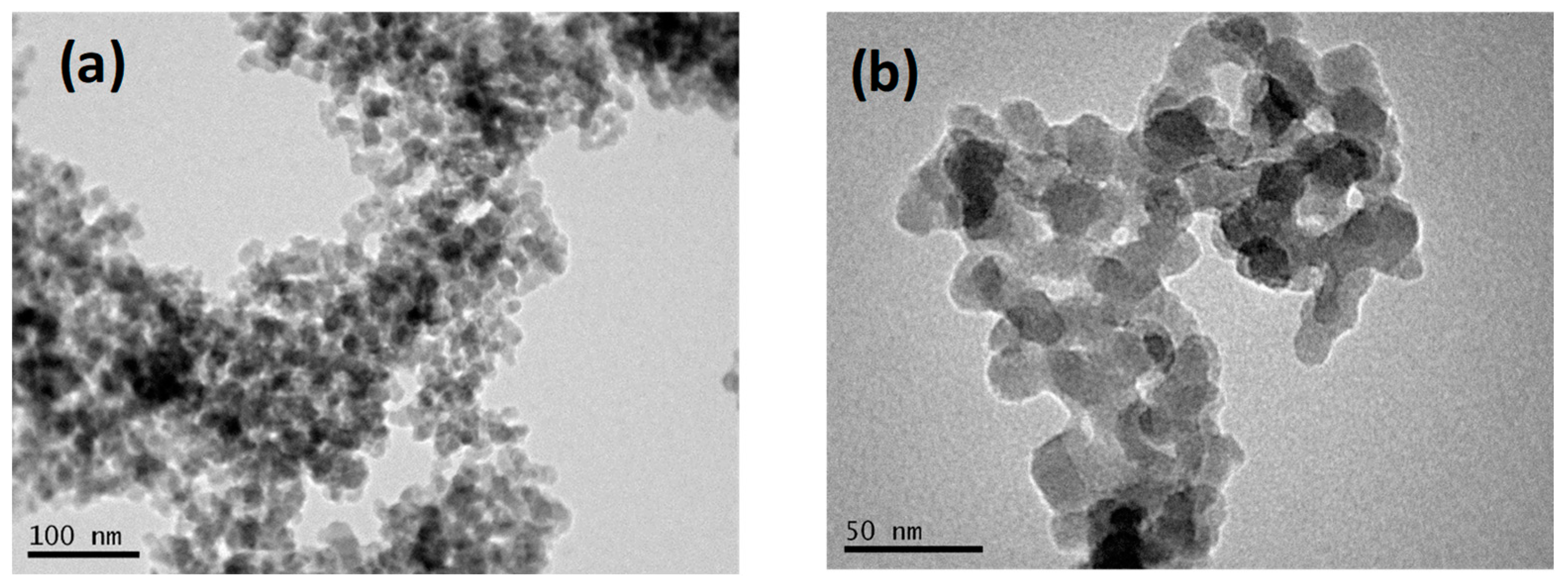
3.1. Physicochemical Properties of PNPs
3.2. Stability of PNP Dispersions
3.3. Interfacial Properties and Wettability of PNP Dispersions
3.4. Stability of Pickering Emulsions
3.5. Rheology of Pickering Emulsions
3.6. Flow of Shear-Thinning Fluid through a Pore
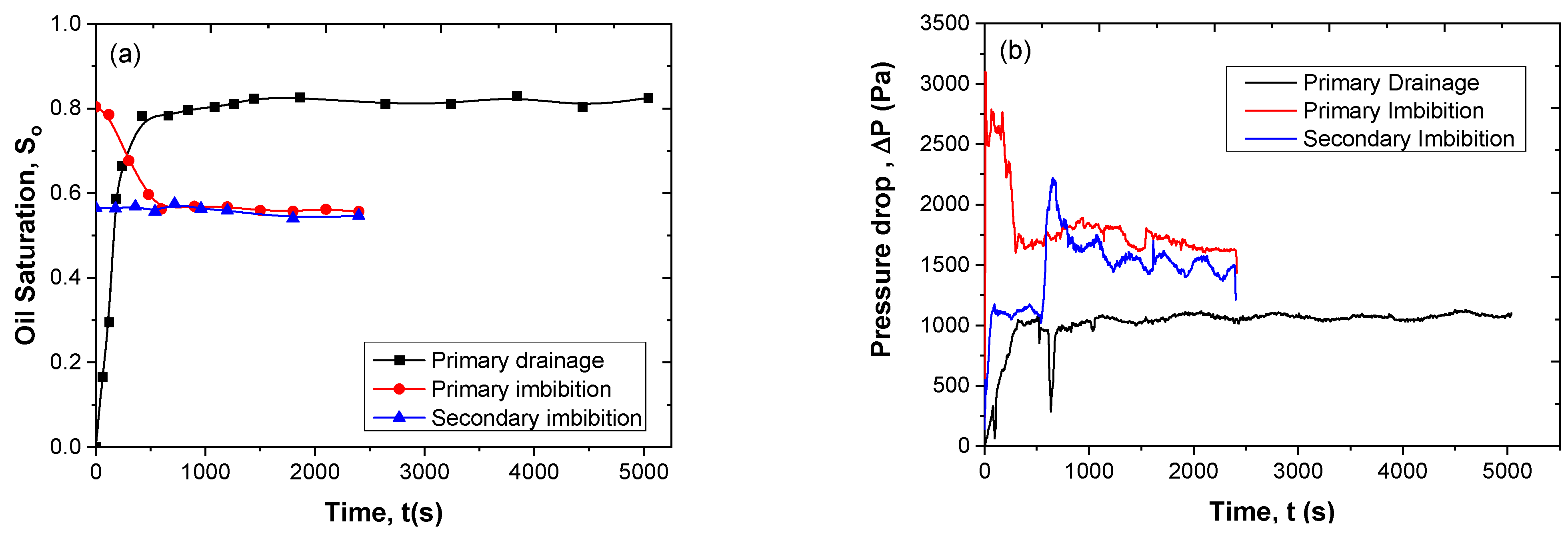
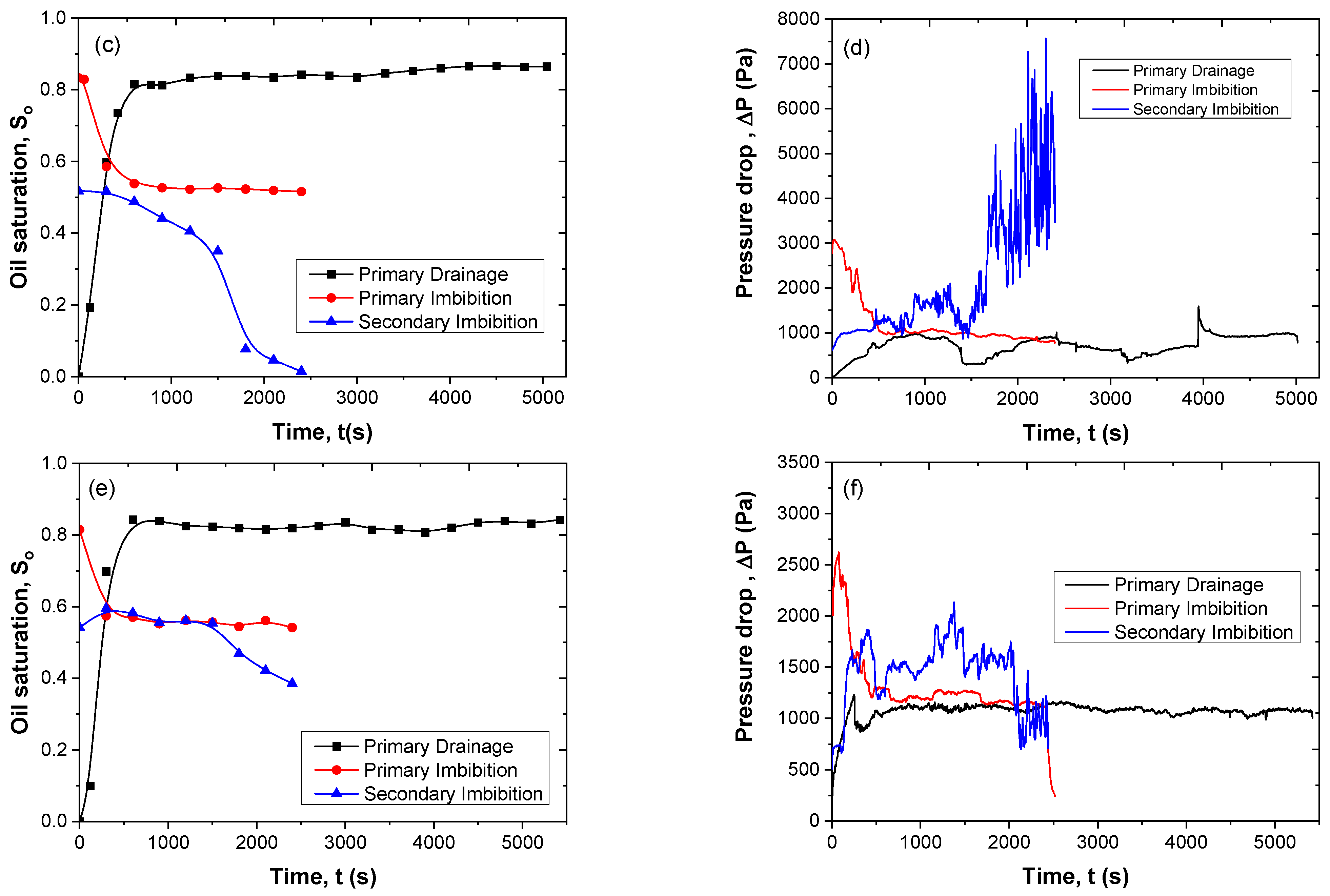
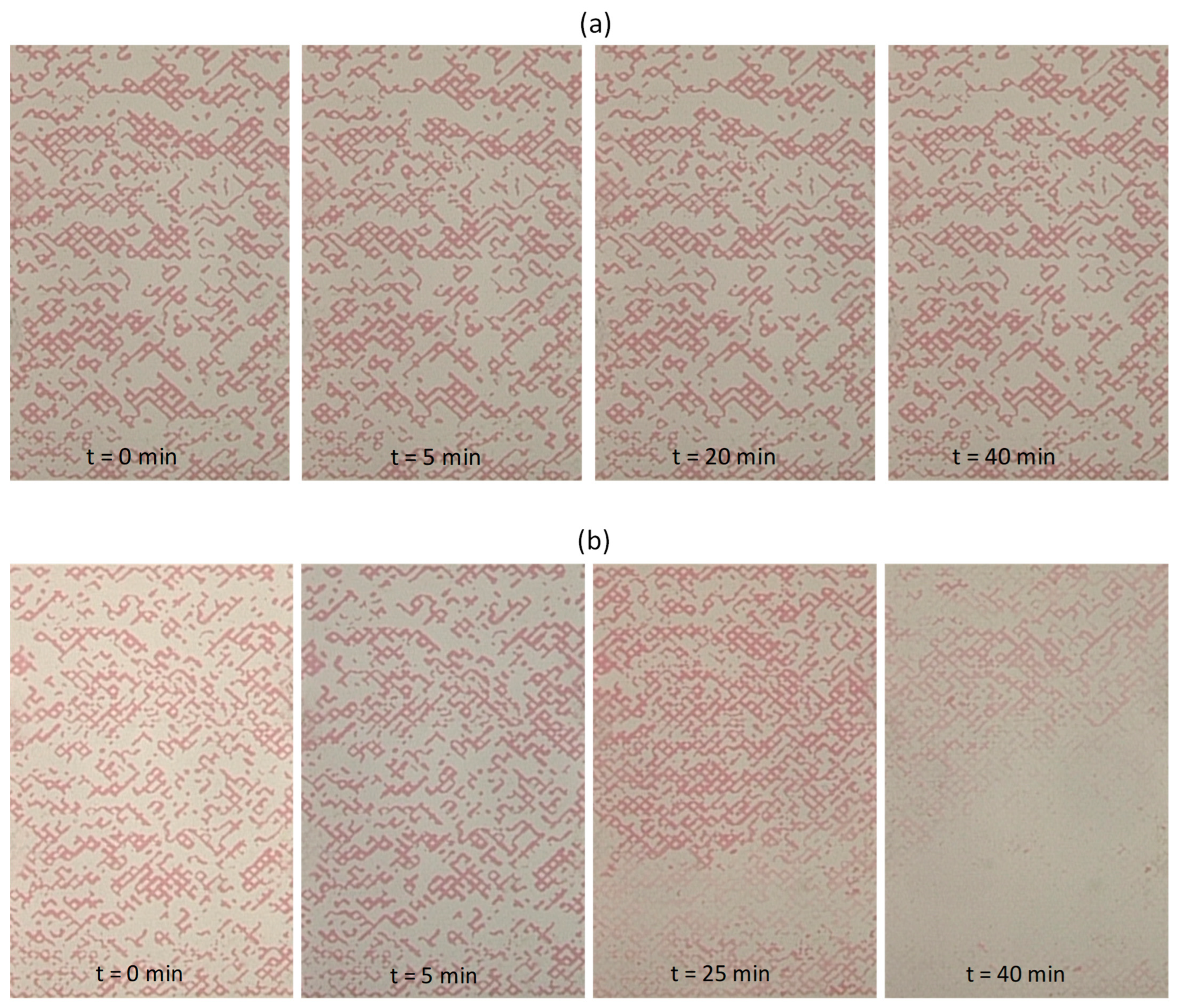
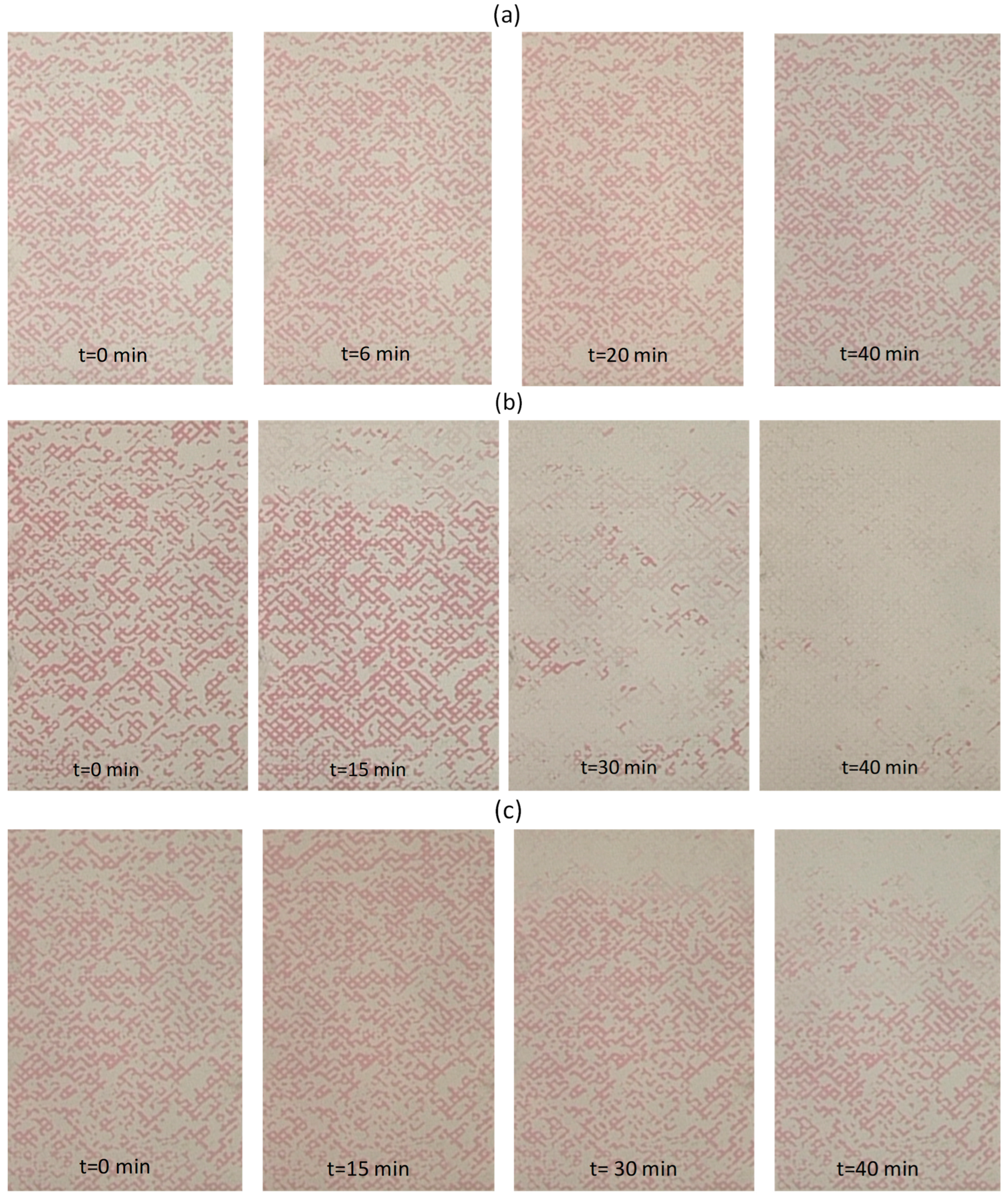
3.7. Immiscible Displacement in the Pore Network
3.8. Two-Phase Flow Patterns
3.9. Energy Efficiency of Secondary Imbibition
4. Conclusions
- The surfaces of SiO2 NPs were coated with the polymer through the copolymerization of the monomers AMPSA and DMA on the SiO2-MPS NPs, via free radical polymerization.
- SiO2-P(AMPSA-co-DMA) NPs remain stable even at high temperatures (~200–250 °C), which are higher than those prevailing at an oil reservoir (<150 °C).
- SiO2-P(AMPSA-co-DMA) NPs demonstrated excellent stability in salt solutions at a high ionic strength.
- SiO2-P(AMPSA-co-DMA) NPs dispersed in salt solutions result in stable oil-in-water Pickering emulsions with a power law shear-thinning rheology.
- The injection of SiO2-P(AMPSA-co-DMA) NP dispersions facilitates successive events of ganglia mobilization and coalescence that lead to fluid redistribution and a weak-to-moderate increase in the oil recovery.
- The higher ionic strength and presence of divalent ions lead to larger oil droplet sizes and more viscous Pickering emulsions, which are able to attain a higher oil-recovery efficiency.
- As one goes from PNP dispersions to Pickering emulsions, the increase in the capillary number and viscosity ratio favor the transition of the flow pattern from capillary fingering to frontal drive and a higher oil-recovery efficiency.
- In terms of the oil-recovery efficiency, the most viscous Pickering emulsion is selected, but in terms of energy efficiency, the less viscous Pickering emulsion is preferable.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Golas, P.L.; Louie, S.; Lowry, G.V.; Matyjaszewski, K.; Tilton, R.D. Compartive study of polymeric stabilizers for magnetic nanoparticles using ATRP. Langmuir 2010, 26, 16890–16900. [Google Scholar] [CrossRef]
- Wang, L.; Xu, H.; Gao, J.; Yao, J.; Zhang, Q. Recent progress in metal-organic frameworks-based hydrogels and aerogels and their applications. Coord. Chem. Rev. 2019, 398, 213016. [Google Scholar] [CrossRef]
- Wu, Y.; Li, Y.; Zhao, T.; Wang, X.; Isaeva, V.I.; Kustov, L.M.; Yao, J.; Gao, J. Bimetal-organic framework-derived nanotube@cellulose aerogels for peroxymonosulfate (PMS) activation. Carbohydr. Polym. 2022, 296, 119969. [Google Scholar] [CrossRef]
- Wei, Β.; Li, Q.; Ning, J.; Wang, Y.; Sun, L.; Pu, W. Macro- and micro-scale observations of a surface-functionalized nanocellulose based aqueous nanofluids in chemical enhanced oil recovery (C-EOR). Fuel 2019, 236, 1321–1333. [Google Scholar] [CrossRef]
- Husein, N.; Yunan, M.H.; Ismail, I.; Wan Sulaiman, W.R.; Boyou, N. Enhanced Oil Recovery by Alkaline-Surfactant-Polymer Alternating with Waterflooding. Chem. Eng. Transact. 2018, 63, 823–828. [Google Scholar]
- Levitt, D.B.; Pope, G.A. Selection and Screening of Polymers for Enhanced-Oil Recovery. In Proceedings of the SPE Symposium on Improved Oil Recovery, Tulsa, OK, USA, 20–23 April 2008. [Google Scholar] [CrossRef]
- Al-Shakry, B.; Shiran, B.S.; Skauge, T.; Skauge, A. Polymer Injectivity: Influence of Permeability in the Flow of EOR Polymers in Porous Media. In Proceedings of the SPE Europec featured at 81st EAGE Conference and Exhibition, London, UK, 3–6 June 2019. [Google Scholar] [CrossRef]
- Puerto, M.; Hirasaki, G.J.; Miller, C.A.; Barnes, J.R. Surfactant Systems for EOR in High-Temperature, High-Salinity Environments. SPE J. 2011, 17, 11–19. [Google Scholar] [CrossRef]
- Machale, J.; Majumder, K.S.; Ghosh, P.; Sen, T.K. Role of chemical additives and their rheological properties in enhanced oil recovery. Rev. Chem. Eng. 2020, 26, 789–830. [Google Scholar] [CrossRef]
- Sircar, A.; Rayavarapu, K.; Bist, N.; Yadav, K.; Singh, S. Applications of nanoparticles in enhanced oil recovery. Petrol. Res. 2022, 7, 77–90. [Google Scholar] [CrossRef]
- Ponnapati, R.; Karazincir, O.; Dao, E.; Ng, R.; Mohanty, K.K.; Krihnamoorti, R. Polymer-functionalized nanoparticles for improving waterfllod sweep effciiency: Characterization and transport properties. Ind. Eng. Chem. Res. 2011, 50, 13030–13036. [Google Scholar] [CrossRef]
- Liu, L.; Pu, X.; Tao, H.; Chen, K.; Guo, W.; Luo, D.; Ren, Z. Pickering emulsion stabilized by organoclay and interediately hydrophobic nanosilica for high temperature conditions. Coll. Surf. A Physicochem. Eng. Asp. 2021, 610, 125694. [Google Scholar] [CrossRef]
- Harati, S.; Bayat, A.E.; Sarvestani, M.T. Assessing the effects of different gas types on stability of SiO2 nanopartciles foam for enhanced oil recovery purpose. J. Mol. Liq. 2020, 313, 113521. [Google Scholar] [CrossRef]
- Morrow, N.R.; Buckley, J. Improved oil recovery by low salinitywaterflooding. J. Petrol. Technol. 2011, 63, 106–112. [Google Scholar] [CrossRef]
- Yuan, H.; Shapiro, A.A. Induced migration of fines during waterlooding in communicating layer-cake reervoirs. J. Petrol. Sci. Eng. 2011, 78, 618–626. [Google Scholar] [CrossRef]
- Arab, D.; Pourafshary, P. Nanoparticles-assisted surface charge modification of the porous medium to treat colloidal particles migration induced by low salinity water flooding. Coll. Surf. A Physicochem. Eng. Asp. 2013, 436, 803–814. [Google Scholar] [CrossRef]
- Maghzi, A.; Kharrat, R.; Mohebbi, A.; Ghazanfari, M.H. The impact of silica nanoparticles on the performance of polymer solution in presence of salts in polymer flooding for heavy oil recovery. Fuel 2014, 123, 123–132. [Google Scholar] [CrossRef]
- ShamsiJazeyi, H.; Miller, C.A.; Wong, M.S.; Tour, J.M.; Verduzco, R. Polymer-coated nanoparticles for enhanced oil recovery. J. Appl. Polym. Sci. 2014, 131, 123–132. [Google Scholar] [CrossRef]
- Hu, X.; Ke, Y.; Zhao, Y.; Yu, C.; Lu, S.; Peng, F. Preparation and properties of nanocomposites of β-cyclodextrin- functionalized polyacrylamide and its application for enhancing oil recovery. RSC Adv. 2018, 8, 30491–30501. [Google Scholar] [CrossRef]
- Gbadamosi, A.O.; Junin, R.; Manan, M.A.; Yekeen, N.; Agi, A.; Oseh, J.O. Recent advances and prospects in polymeric nanofluids application for enhanced oil recovery. J. Ind Eng. Chem. 2018, 66, 1–19. [Google Scholar] [CrossRef]
- Tabar, M.A.; Alaei, M.; Bazmi, M.; Khojasteh, R.R.; Koolivand-Salooki, M.; Motiee, F.; Rashidi, A.M. Facile and economical preparation method of nanoporous graphene/silica nanohybrid and evaluation of its Pickering emulsion properties for chemical enhanced oil recovery (C-EOR). Fuel 2017, 206, 453–466. [Google Scholar]
- Zhang, Y.; Geng, J.; Liu, J.; Bai, B.; He, X.; Wei, M.; Deng, W. Direct Pore-Level Visualization and Verification of In Situ Oil-in-Water Pickering Emulsification during Polymeric Nanogel Flooding for EOR in a Transparent Three-Dimensional Micromodel. Langmuir 2021, 37, 13353–13364. [Google Scholar] [CrossRef]
- Chen, X.; Li, Y.; Liu, Z.; Trivedi, J.; Tang, Y.; Sui, M. Visualized investigation of the immiscible displacemnet: Influencing factors, improved method, and EOR effect. Fuel 2023, 331, 125841. [Google Scholar] [CrossRef]
- Haruna, M.A.; Gardy, J.; Yao, G.; Hu, Z.; Hondow, N.; Dongsheng, W.D. Nanoparticle modified polyacrylamide for enhanced oil recovery at harsh conditions. Fuel 2020, 268, 117186. [Google Scholar] [CrossRef]
- Ranka, M.; Brown, P.; Hatton, T.A. Responsive Stabilization of Nanoparticles for Extreme Salinity and High Temperature Reservoir Applications. ACS Appl. Mater. Interfaces 2015, 7, 19651–19658. [Google Scholar] [CrossRef]
- Lee, J.; Moesari, E.; Dandamudi, C.B.; Beniah, G.; Chang, B.; Iqbal, M.; Fei, Y.; Zhou, N.; Ellison, C.J.; Johnston, K.P. Ultra-Stable Silica Nanoparticles as Nano-plugging Additive for Shale Exploitation in Harsh Environments. Macromolecules 2019, 50, 7699–7711. [Google Scholar] [CrossRef]
- Wu, Y.; Wang, Z.; Zhang, T.; Bai, Y.; Wang, P.; Luo, P.; Gou, S.; Guo, Q. Poly(2-acrylamide-2-methylpropanesulfonic acid)-modified SiO2 nanoparticles for water-based drilling fluids. Ind. Eng. Chem. Res. 2016, 56, 168–174. [Google Scholar] [CrossRef]
- Park, H.; Lim, H.S.; Yang, J.; Kwak, C.; Kim, J.; Kim, J.; Choi, S.S.; Kim, C.B.; Lee, J. A Systematic Investigation on the Properties of Silica Nanoparticles “Multipoint”-Grafted with Poly(2-acrylamido-2-methylpropanesulfonate-co-acrylic Acid) in Extreme Salinity Brines and Brine-Oil Interfaces. Langmuir 2020, 36, 3174–3183. [Google Scholar] [CrossRef]
- Hendraningrat, L.; Torsaeter, O. A Stabilizer that Enhances the Oil Recovery Process Using Silica-Based Nanofluids. Transp. Porous Media 2015, 108, 679–696. [Google Scholar] [CrossRef]
- Ehtesabi, H.; Ahadia, M.M.; Taghikhani, V.; Ghazanfari, M.H. Enhanced Heavy Oil Recovery in Sandstone Cores Using TiO2 Nanofluids. Energy Fuels 2013, 28, 423–430. [Google Scholar] [CrossRef]
- Yadav, U.S.; Kumar, H.; Roy, V.; Juyal, S.; Tripathi, A.; Shanker, A. Experimental evaluation of partially hydrolyzed polyacrylamide and silica nanoparticles solutions for enhanced oil recovery. J. Pet. Explor. Prod. Technol. 2020, 10, 1109–1114. [Google Scholar] [CrossRef]
- Liu, J.X.; Zhu, H.J.; Wang, P.; Pan, J.M. Recent studies of Pickering emulsion system in petroleum treatment: The role of particles. Petrol. Sci. 2021, 18, 1551–1563. [Google Scholar] [CrossRef]
- DiCarlo, D.A.; Aminzadeh, B.; Roberts, M.; Chung, D.H.; Bryant, S.L.; Huh, C. Mobility control through spontaneous formation of nanoparticle stabilized emulsions. Geopys. Res. Lett. 2011, 38, 1–5. [Google Scholar] [CrossRef]
- Ding, B.; Ahmadi, S.H.; Babak, P.; Bryant, S.L.; Kantzas, A. On the Stability of Pickering and Classical Nanoemulsions: Theory and Experiments. Langmuir 2023, 39, 6975–6991. [Google Scholar] [CrossRef]
- Arab, D.; Kantzas, A.; Bryant, S.L. Nanoparticle stabilized oil in water emulsions: A critical review. J. Pet. Sci. Eng. 2018, 163, 217–242. [Google Scholar] [CrossRef]
- Yousufi, M.M.; Dzulkarnain, I.B.; Mohyaldinn Elhaj, M.E.; Ahmed, S. A perspective on the prospect of Pickering emulsion in reservoir conformance control with insight into the influential parameters and characterization techniques. Processes 2023, 11, 2672. [Google Scholar] [CrossRef]
- Ntente, C.; Iatridi, Z.; Theodoropoulou, M.; Bokias, G.; Tsakiroglou, C. Anionic amphiphilic copolymers as potential agents for enhanced oil recovery. React. Funct. Polym. 2023, 184, 105521. [Google Scholar] [CrossRef]
- Berry, J.D.; Neeson, M.J.; Dagastine, R.R.; Chan, D.Y.C.; Tabor, R.F. Measurment of surface and interfacial tension using pendant drop tensiometry. J. Colloid Interface Sci. 2015, 454, 226–237. [Google Scholar] [CrossRef]
- Pantoja, M.; Díaz-Benito, B.; Velasco, F.; Abenojar, J.; Del Real, J.C. Analysis of hydrolysis process of γ-methacryloxypropyltrimethoxysilane and its influence on the formation of silane coatings on aluminum alloy. Appl. Surf. Sci. 2009, 255, 6386–6390. [Google Scholar] [CrossRef]
- Zhang, H.; Li, C.; Guo, J.; Zang, L.; Luo, J. In Situ Synthesis of Poly(methyl methacrylate)/SiO2 Hybrid Nanocomposites via “Grafting Onto” Strategy Based on UV Irradiation in the Presence of Iron Aqueous Solution. J. Nanomater. 2012, 2012, 217412. [Google Scholar] [CrossRef]
- Kunst, S.R.; Beltrami, L.V.R.; Cardoso, H.R.P.; Santana, J.A.; Sarmento, V.H.V.; Muller, I.L.; Malfatti, F. Characterization of siloxane-poly(methyl methacrylate) hybrid films obtained on a tinplate substrate modified by the addition of organic and inorganic acids. Mater. Res. 2015, 201518, 151–163. [Google Scholar] [CrossRef]
- Lopes, I.M.F.; Abersfelder, K.; Oliveira, P.W.; Mousavi, S.H.; Junqueira, R.M.R. Flower-like silicon dioxide/polymer composite particles synthesized by dispersion polymerization route. J. Mater. Sci. 2018, 53, 11367–11377. [Google Scholar] [CrossRef]
- Hartwig, A.; Sebald, M.; Kleemeier, M. Cross-linking of cationically polymerised epoxides by nanoparticles. Polymer 2005, 46, 2029–2039. [Google Scholar] [CrossRef]
- Jiang, J.; Wang, W.; Shen, H.; Wang, J.; Cao, J. Characterization of silica particles modified with γ-methacryloxypropyltrimethoxysilane. Appl. Surf. Sci. 2017, 397, 104–111. [Google Scholar] [CrossRef]
- Massoumi, B.; Abbasian, M.; Jahanban-Esfahlan, R.; Motamedi, S.; Samadian, H.; Rezaei, A.; Derakhshankhah, H.; Farnudiyan-Habibi, A.; Jaymand, M. PEGylated hollow pH-responsive polymeric nanocapsules for controlled drug delivery. Polym. Int. 2020, 69, 519–527. [Google Scholar] [CrossRef]
- Jouenne, S. Polymer flooding in high temperature, high salinity conditions: Selection of polymer type and polymer chemistry, thermal stability. J. Pet. Sci. Eng. 2020, 195, 107545. [Google Scholar] [CrossRef]
- Kozicki, W.; Chou, C.H.; Tiu, C. Non-Newtonian flow in ducts of arbitrary cross-sectional shape. Chem. Eng. Sci. 1966, 21, 665–679. [Google Scholar] [CrossRef]
- Tsakiroglou, C.D. A methodology for the derivation of non-Darcian models for the flow of generalized Newtonian fluids in porous media. J. Non-Newt. Fluid Mech. 2002, 105, 79–110. [Google Scholar] [CrossRef]
- Payatakes, A.C.; Dias, M.M. Immiscible Microdisplacement and Ganglion Dynamics in Porous Media. Rev. Chem. Eng. 1984, 2, 85–174. [Google Scholar] [CrossRef]
- Sygouni, V.; Tsakiroglou, C.D.; Payatakes, A.C. Using wavelets to characterize the wettability of porous materials. Phys. Rev. E 2007, 76, 056304. [Google Scholar] [CrossRef]
- Yuan, H.H.; Swanson, B.F. Resolving Pore-Space Characteristics by Rate-Controlled Porosimetry. SPE Form. Eval. 1989, 4, 17–24. [Google Scholar] [CrossRef]
- Moradi, M.; Kazempour, M.; French, J.T.; Alvarado, V. Dynamic flow response of crude oil-in-water emulsion during flow through porous media. Fuel 2014, 135, 38–45. [Google Scholar] [CrossRef]
- Lenormand, R.; Touboul, E.; Zarcone, C. Numerical models and experiments on immiscible displacements in porous media. J. Fluid Mech. 1988, 189, 165–187. [Google Scholar] [CrossRef]
- Tsakiroglou, C.D.; Theodoropoulou, M.; Karoutsos, V. Non-equilibrium capillary pressure and relative permeability curves of porous media. AIChE J. 2003, 49, 2472–2486. [Google Scholar] [CrossRef]
- Tsakiroglou, C.D. Correlation of the two-phase flow coefficients of porous media with the rheology of shear-thinning fluids. J. Non-Newtonian Fluid Mech. 2004, 117, 1–23. [Google Scholar] [CrossRef]
- Tsakiroglou, C.D.; Theodoropoulou, M.; Karoutsos, V.; Papanicolaou, D.; Sygouni, V. Experimental study of the immiscible displacement of shear-thinning fluids in pore networks. J. Coll. Interface Sci. 2003, 267, 217–232. [Google Scholar] [CrossRef]
- Ntente, C.; Strekla, A.; Iatridi, Z.; Theodoropoulou, M.; Bokias, G.; Tsakiroglou, C.D. Polymer-functionalized nanoparticles as agents for the in situ remediation of oil-contaminated soils. IOP Conf. Ser. Earth Environ. Sci. 2022, 1123, 012064. [Google Scholar] [CrossRef]


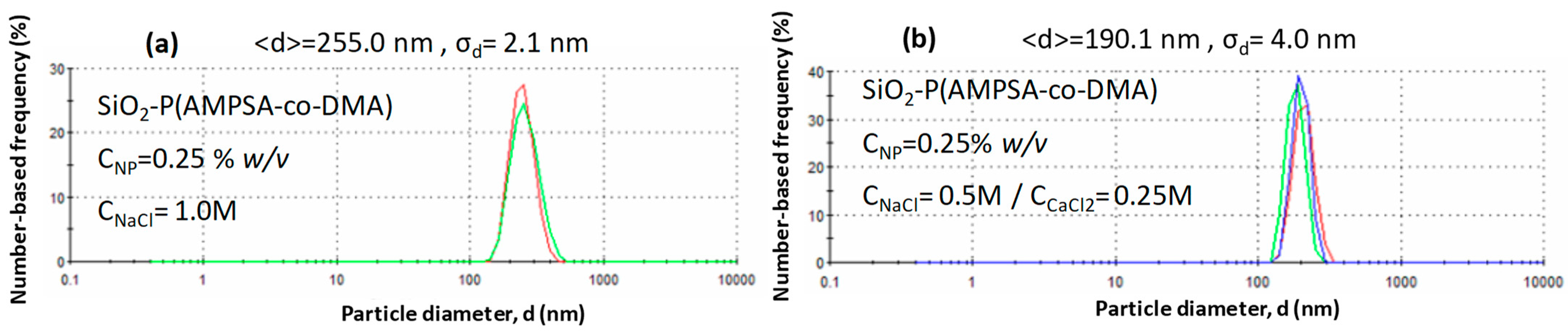
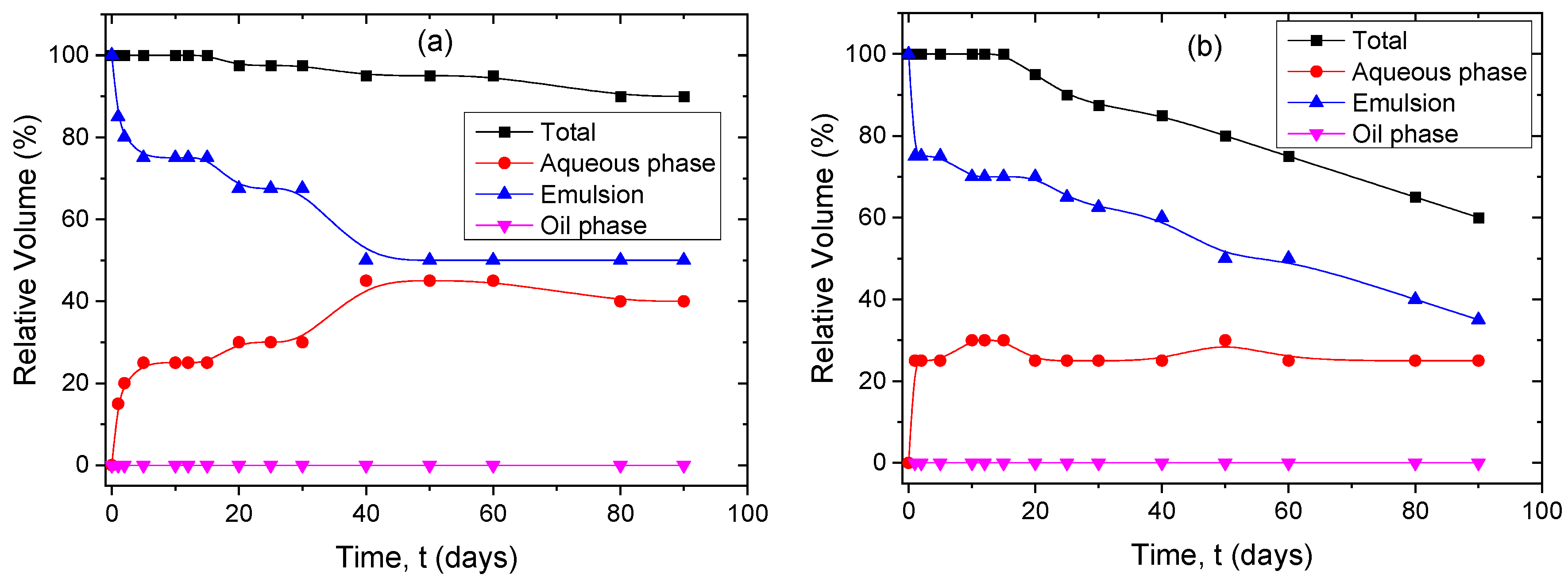
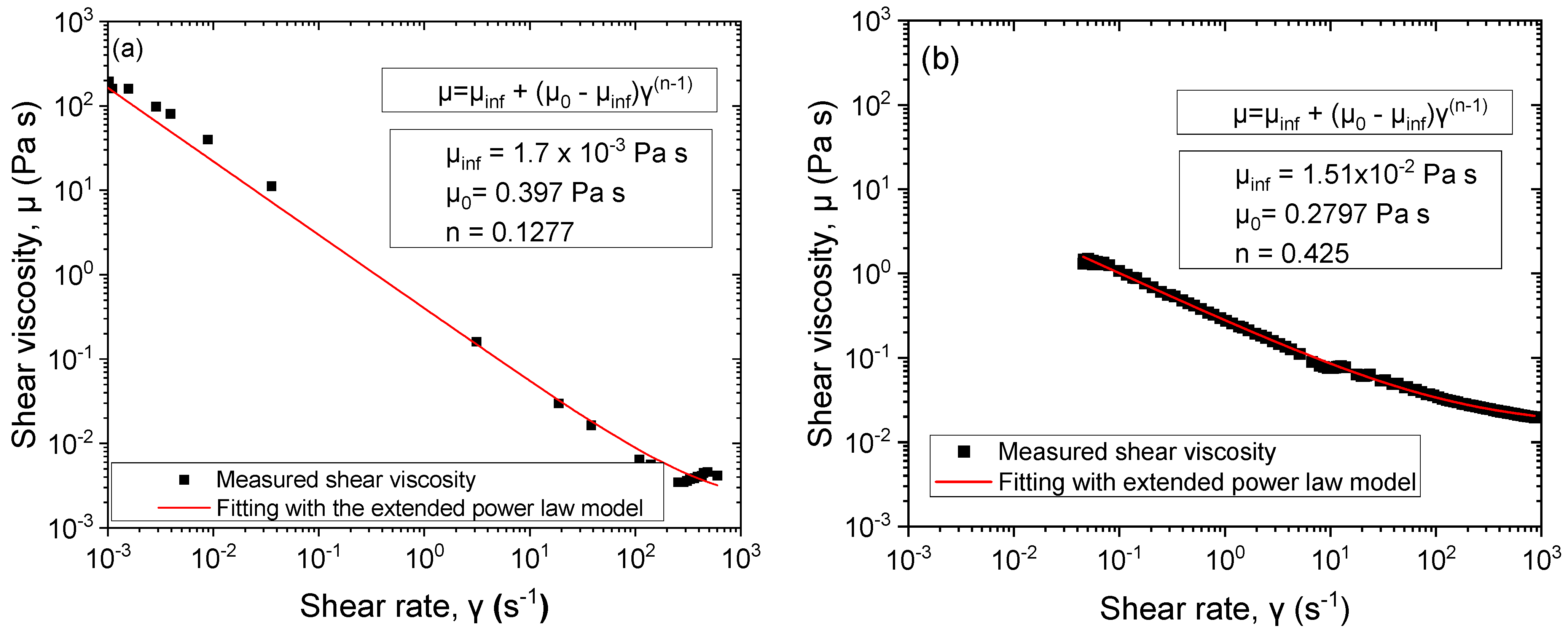
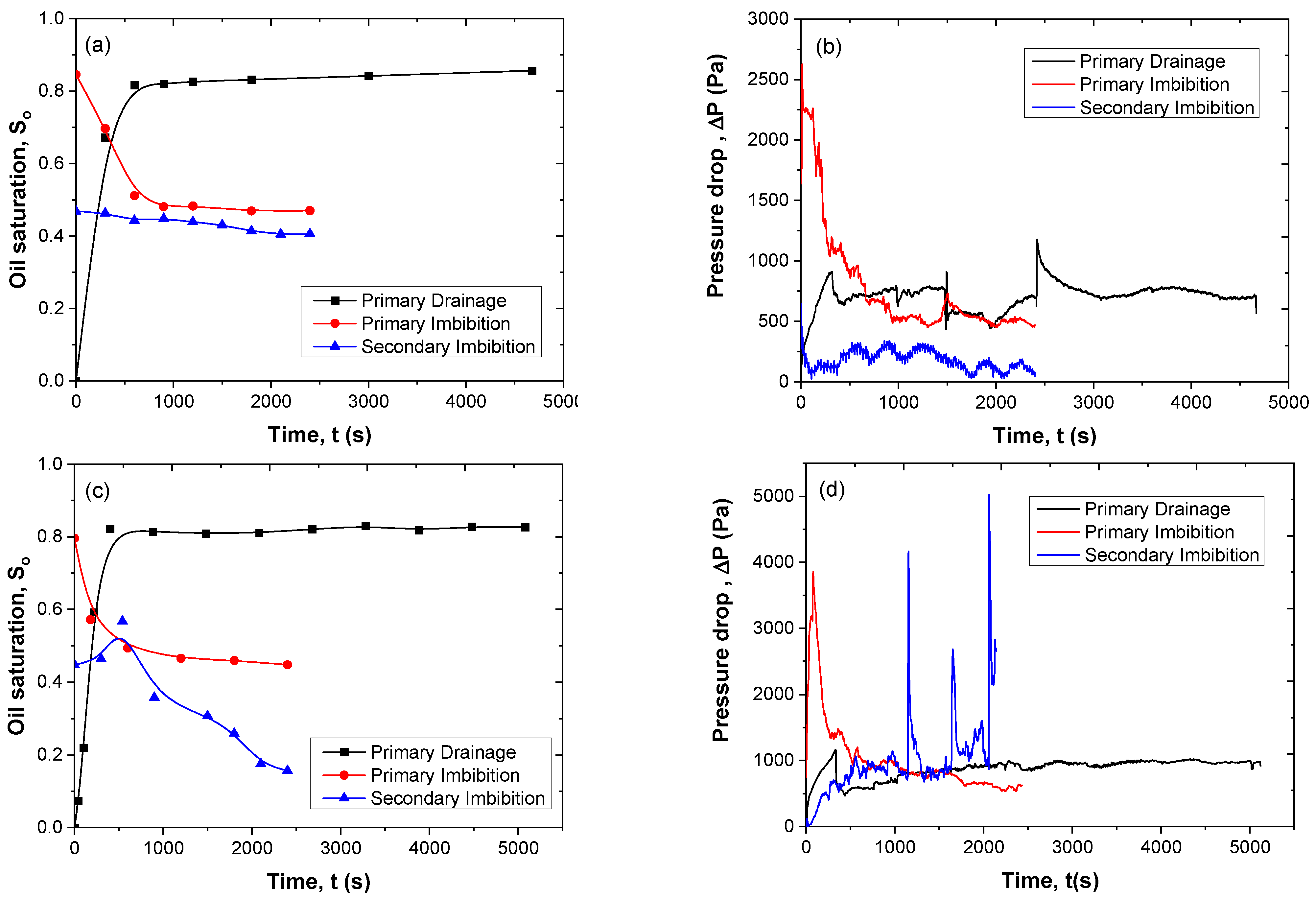
| Aqueous Phase | (mN/m) | (mN/m) | (mN/m) |
|---|---|---|---|
| 3DW | 72.40 ± 0.20 | 49.92 | 34.76 |
| NaCl 1.0 M | 73.70 ± 0.15 | 48.48 | 34.02 |
| CNP = 0.25% w/v in NaCl 1.0 M | 53.07 ± 0.46 | 26.88 | 30.28 |
| NaCl 0.5 M + CaCl2 0.25 M | 71.05 ± 0.23 | 43.55 | 34.13 |
| CNP = 0.25% w/v in NaCl 0.5 M + CaCl2 0.25 M | 55.40 ± 0.21 | 33.25 | 28.04 |
| NP (% w/v) | Salt (M) | Contact Angle (ο) | Image | Contact Angle (ο) | Image |
| Fluid system | NP aqueous dispersion/air | NP aqueous dispersion/n-C10 | |||
| 1.0 | 3DW | 45.00 ± 0.05 |  | 66.30 ± 0.14 |  |
| 0.5 | 3DW | 50.00 ± 0.71 |  | 67.35 ± 1.91 |  |
| 0.25 | 3DW | 46.35 ± 0.49 |  | 63.95 ± 0.05 |  |
| 0.25 | NaCl 0.25 M | 38.40 ± 0.14 |  | 68.60 ± 1.41 |  |
| 0.25 | NaCl 0.5 M | 48.80 ± 1.41 |  | 71.70 ± 1.41 |  |
| 0.25 | NaCl 1.0 M | 63.80 ± 0.28 |  | 71.50 ± 1.56 |  |
| 0.25 | NaCl 0.5 M +CaCl2 0.25 M | 59.10 ± 0.99 |  | 71.15 ± 0.07 |  |
| Fluid system | NP aqueous dispersion/paraffin oil | ||||
| 0.0 | 3DW | 60.32 ± 2.13 | |||
| 0.0 | NaCl 1.0 M | 54.4 ± 1.03 |  | ||
| 0.25 | NaCl 1.0 M | 62.75 ± 0.05 |  | ||
| 0.0 | NaCl 0.5 M + CaCl2 0.25 M | 54.2 ± 0.09 |  | ||
| 0.25 | NaCl 0.5 M + CaCl2 0.25 M | 79.00 ± 1.56 |  | ||
| Type of Displacement | Displaced Fluid | Injected Fluid | Flow Rate Q (mL/min) | Injected Vol (mL) | Oil Saturation | Oil Removal Efficiency Reff (%) |
|---|---|---|---|---|---|---|
| Drainage | 1 M NaCl | Paraffin oil | 0.08 | 7.6 | 0.86 | - |
| Primary Imbibition | Residual paraffin oil | 1 M NaCl | 0.2 | 8 | 0.47 | 45.3 |
| Secondary Imbibition | Residual paraffin oil | 0.25% SiO2-P(AMPSA-co-DMA) w/v in 1 M NaCl (Dispersion 1) | 0.2 | 8 | 0.40 | 14.9 |
| Drainage | 1 M NaCl | Paraffin oil | 0.08 | 8 | 0.83 | - |
| Primary Imbibition | Residual paraffin oil | 1 M NaCl | 0.2 | 8 | 0.45 | 45.8 |
| Secondary Imbibition | Residual paraffin oil | 0.25% SiO2-P(AMPSA-co-DMA) w/v in 1 M NaCl (Emulsion 1) | 0.2 | 8 | 0.16 | 64.4 |
| Drainage | NaCl 0.5 M–CaCl2 0.25 M | Paraffin oil | 0.08 | 8 | 0.82 | - |
| Primary Imbibition | Residual paraffin oil | 0.5 M NaCl, 0.25 M CaCl2 | 0.2 | 8 | 0.56 | 30.2 |
| Secondary Imbibition | Residual paraffin oil | 0.25% SiO2-P(AMPSA-co-DMA) w/v in 0.5 M NaCl/0.25 M CaCl2 (Dispersion 2) | 0.2 | 8 | 0.55 | 1.7 |
| Drainage | 0.5 M NaCl–0.25 M CaCl2 | Paraffin oil | 0.08 | 8 | 0.86 | - |
| Primary Imbibition | Residual paraffin oil | 0.5 M NaCl, 0.25 M CaCl2 | 0.2 | 8 | 0.52 | 39.5 |
| Secondary Imbibition | Residual paraffin oil | 0.25% SiO2-P(AMPSA-co-DMA) w/v in 0.5 M NaCl / 0.25 M CaCl2 (Emulsion 2) | 0.2 | 8 | 0.014 | 97.3 |
| Drainage | 0.5 M NaCl–0.25 M CaCl2 | Paraffin oil | 0.08 | 8.0 | 0.843 | - |
| Primary Imbibition | Residual paraffin oil | 0.5 M NaCl, 0.25 M CaCl2 | 0.2 | 8.0 | 0.541 | 35.8 |
| Secondary Imbibition | Residual paraffin oil | 0.25% SiO2-P(AMPSA-co-DMA) w/v in 0.5 M NaCl/0.25 M CaCl2 (Emulsion 2) | 0.1 | 4.0 | 0.385 | 28.8 |
| 0.25% SiO2-P(AMPSA-co-DMA) w/v in 0.5 M NaCl /0.25 M CaCl2 (Dispersion 2) | 0.1 | 4.0 |
| Displacement | Defender | Invader | Ca × 105 | κ | CaL1 | CaLN |
|---|---|---|---|---|---|---|
| Drainage | 1 M NaCl (μ = 0.97 × 10−3 Pa s) | Paraffin oil (μ = 0.02 Pa s) | 0.424 | 20.6 | 0.018 | 1.365 |
| Primary imbibition | Paraffin oil (μ = 0.02 Pa s) | 1 M NaCl | 0.0516 | 0.0485 | 0.00218 | 0.165 |
| Secondary imbibition | Paraffin oil (μ = 0.02 Pa s) | CNP = 0.25% w/v in 1.0 M NaCl | 0.0579 | 0.0485 | 0.00245 | 0.186 |
| Secondary imbibition | Paraffin oil (μ = 0.02 Pa s) | Pickering emulsion (μ = 0.05367 Pa s) | 3.208 | 2.683 | 0.1359 | 10.32 |
| Drainage | 0.5 M NaCl 0.25 M CaCl2 (μ = 0.93 × 10−3 Pa s) | Paraffin oil (μ = 0.02 Pa s) | 0.425 | 21.5 | 0.0180 | 1.367 |
| Primary imbibition | Paraffin oil (μ = 0.02 Pa s) | 0.5 M NaCl 0.25 M CaCl2 (μ = 0.93 × 10−3 Pa s) | 0.0493 | 0.0465 | 0.00209 | 0.159 |
| Secondary imbibition | Paraffin oil (μ = 0.02 Pa s) | CNP = 0.25% w/v in 0.5 M NaCl 0.25 M CaCl2 | 0.06 | 0.0465 | 0.00255 | 0.193 |
| Secondary imbibition | Paraffin oil (μ = 0.02 Pa s) | Pickering emulsion (μ = 0.07814 Pa s) | 5.054 | 3.907 | 0.214 | 16.27 |
| Secondary imbibition | Paraffin oil (μ = 0.02 Pa s) | Pickering emulsion (μ = 0.07814 Pa s) | 2.527 | 3.907 | 0.107 | 8.13 |
| Secondary imbibition | Paraffin oil (μ = 0.02 Pa s) | CNP = 0.25% w/v in 0.5 M NaCl 0.25 M CaCl2 | 0.03 | 0.0465 | 0.00127 | 0.0965 |
| Injected Fluid | Energy Efficiency, EE (% μW−1) | Oil Removal Efficiency, Reff (%) |
|---|---|---|
| Dispersion 1 | 24.83 | 14.9 |
| Emulsion 1 | 20.06 | 64.4 |
| Dispersion 2 | 0.349 | 1.7 |
| Emulsion 2 | 13.68 | 97.3 |
| Emulsion 2 + Dispersion 2 | 6.03 | 28.8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ntente, C.; Strekla, A.; Iatridi, Z.; Theodoropoulou, M.; Bokias, G.; Tsakiroglou, C.D. Polymer-Coated Nanoparticles and Pickering Emulsions as Agents for Enhanced Oil Recovery: Basic Studies Using a Porous Medium Model. Energies 2023, 16, 8043. https://doi.org/10.3390/en16248043
Ntente C, Strekla A, Iatridi Z, Theodoropoulou M, Bokias G, Tsakiroglou CD. Polymer-Coated Nanoparticles and Pickering Emulsions as Agents for Enhanced Oil Recovery: Basic Studies Using a Porous Medium Model. Energies. 2023; 16(24):8043. https://doi.org/10.3390/en16248043
Chicago/Turabian StyleNtente, Christina, Anastasia Strekla, Zacharoula Iatridi, Maria Theodoropoulou, Georgios Bokias, and Christos D. Tsakiroglou. 2023. "Polymer-Coated Nanoparticles and Pickering Emulsions as Agents for Enhanced Oil Recovery: Basic Studies Using a Porous Medium Model" Energies 16, no. 24: 8043. https://doi.org/10.3390/en16248043
APA StyleNtente, C., Strekla, A., Iatridi, Z., Theodoropoulou, M., Bokias, G., & Tsakiroglou, C. D. (2023). Polymer-Coated Nanoparticles and Pickering Emulsions as Agents for Enhanced Oil Recovery: Basic Studies Using a Porous Medium Model. Energies, 16(24), 8043. https://doi.org/10.3390/en16248043











