Power Receiving Unit for High-Power Resonant Wireless Power Transfer
Abstract
:1. Introduction
2. GaN HEMT Based Class E Resonant WPT
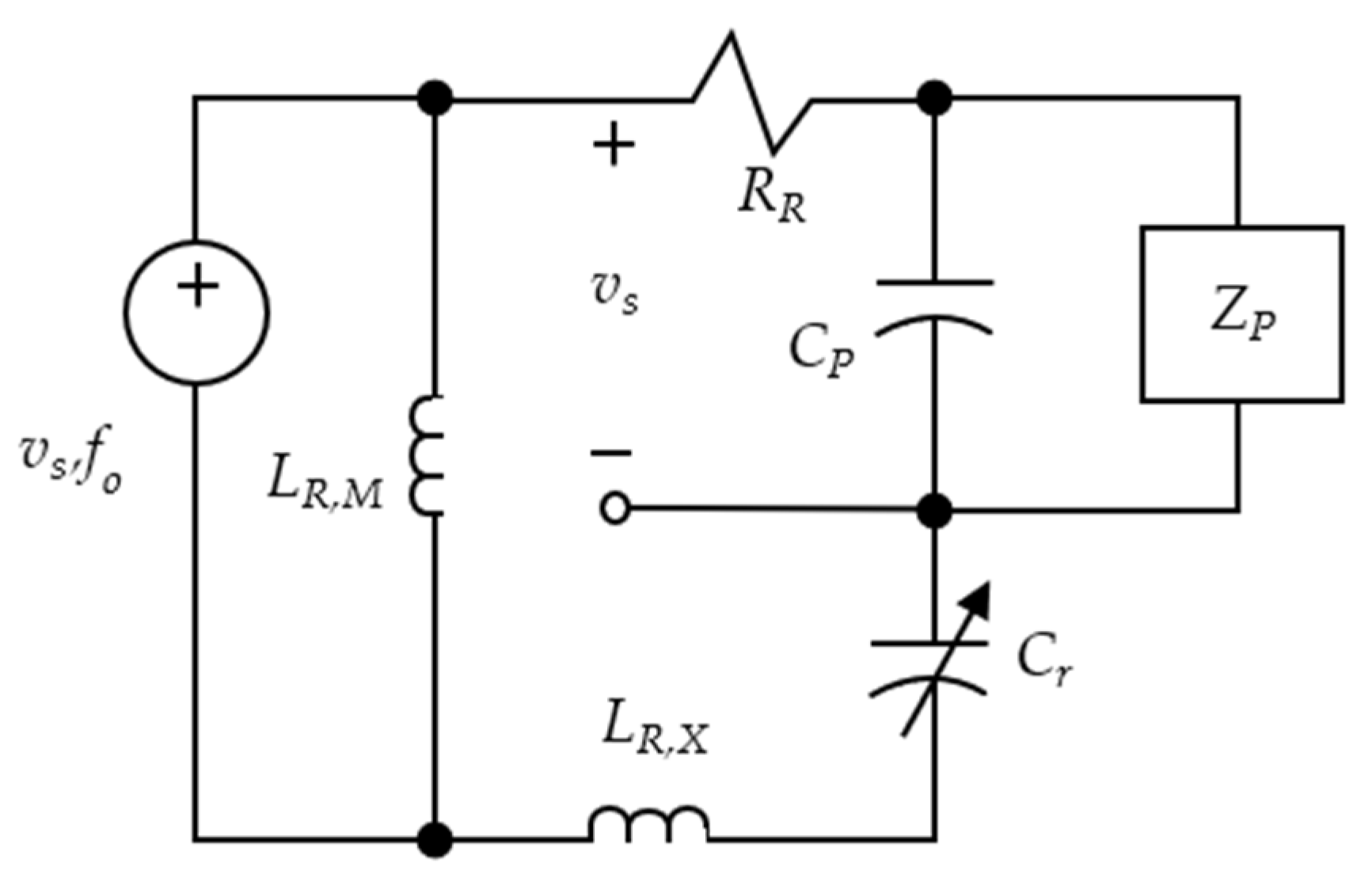
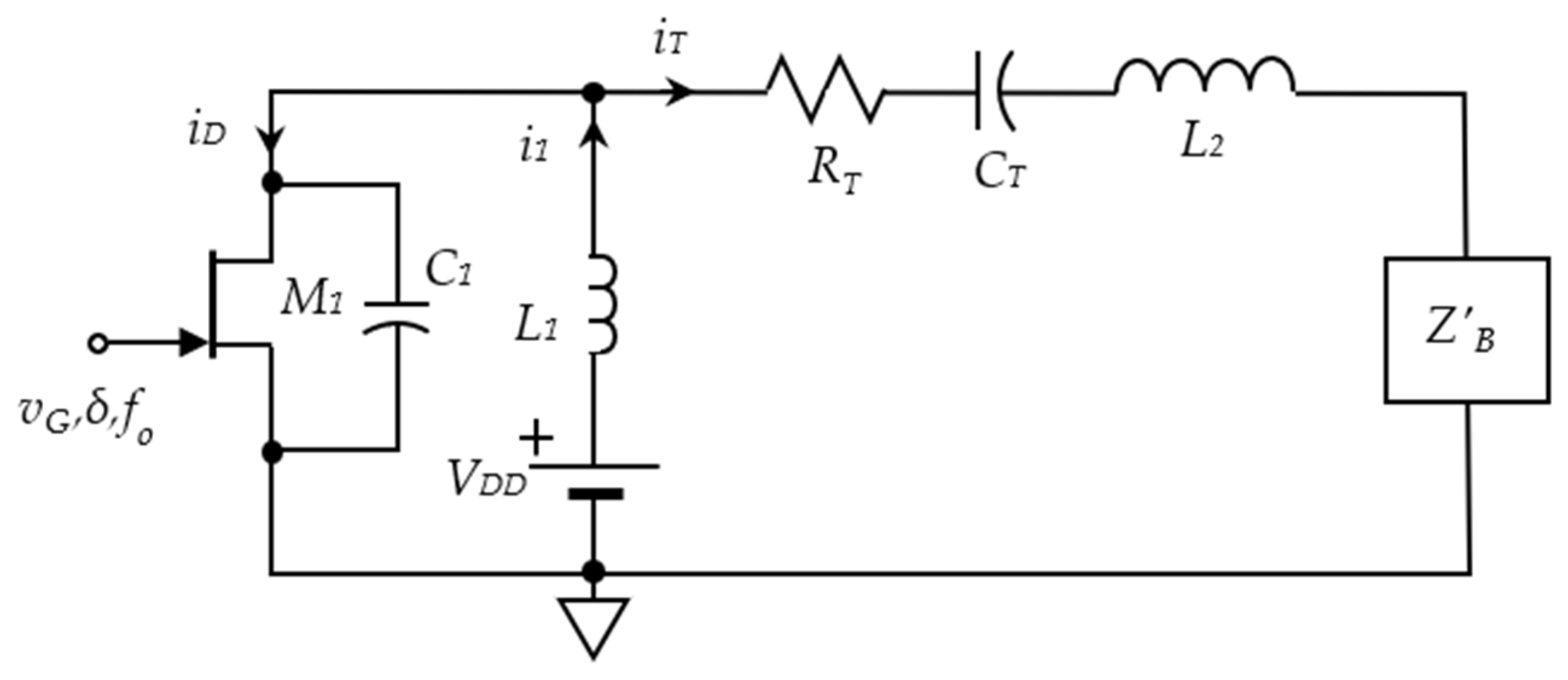
2.1. Power Transmitting Unit (PTU)
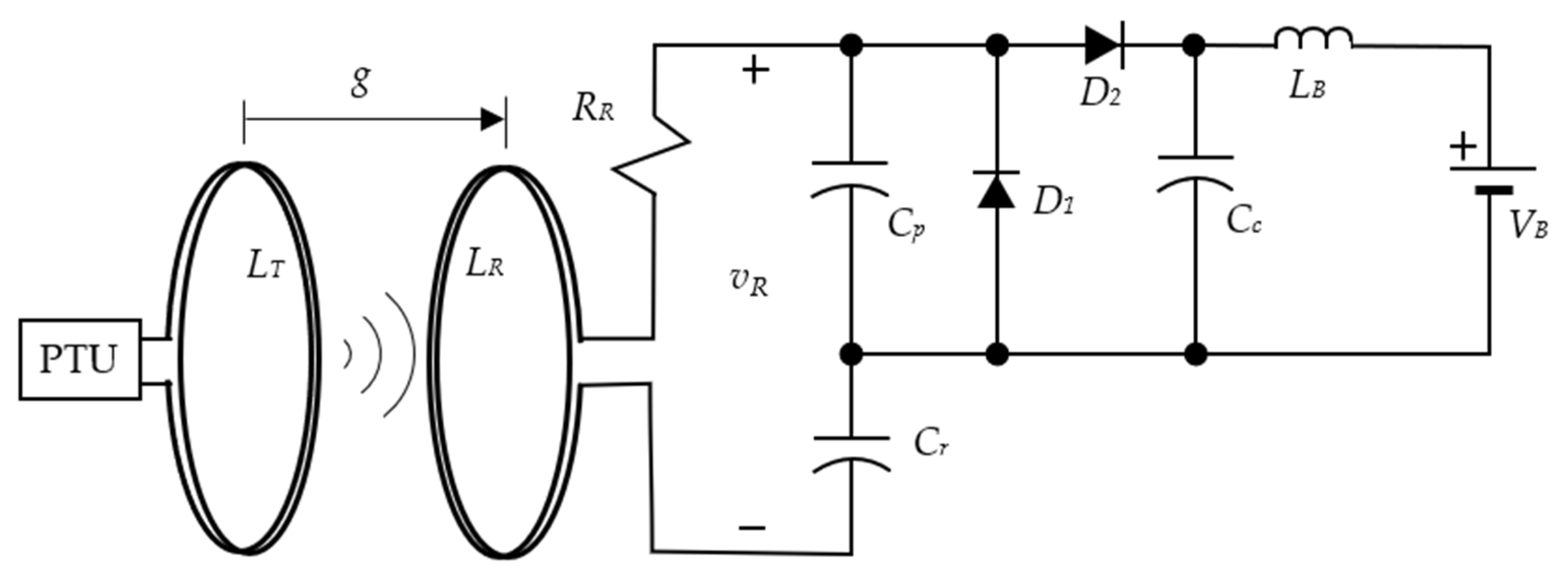
2.2. Power Receiving Unit (PRU)
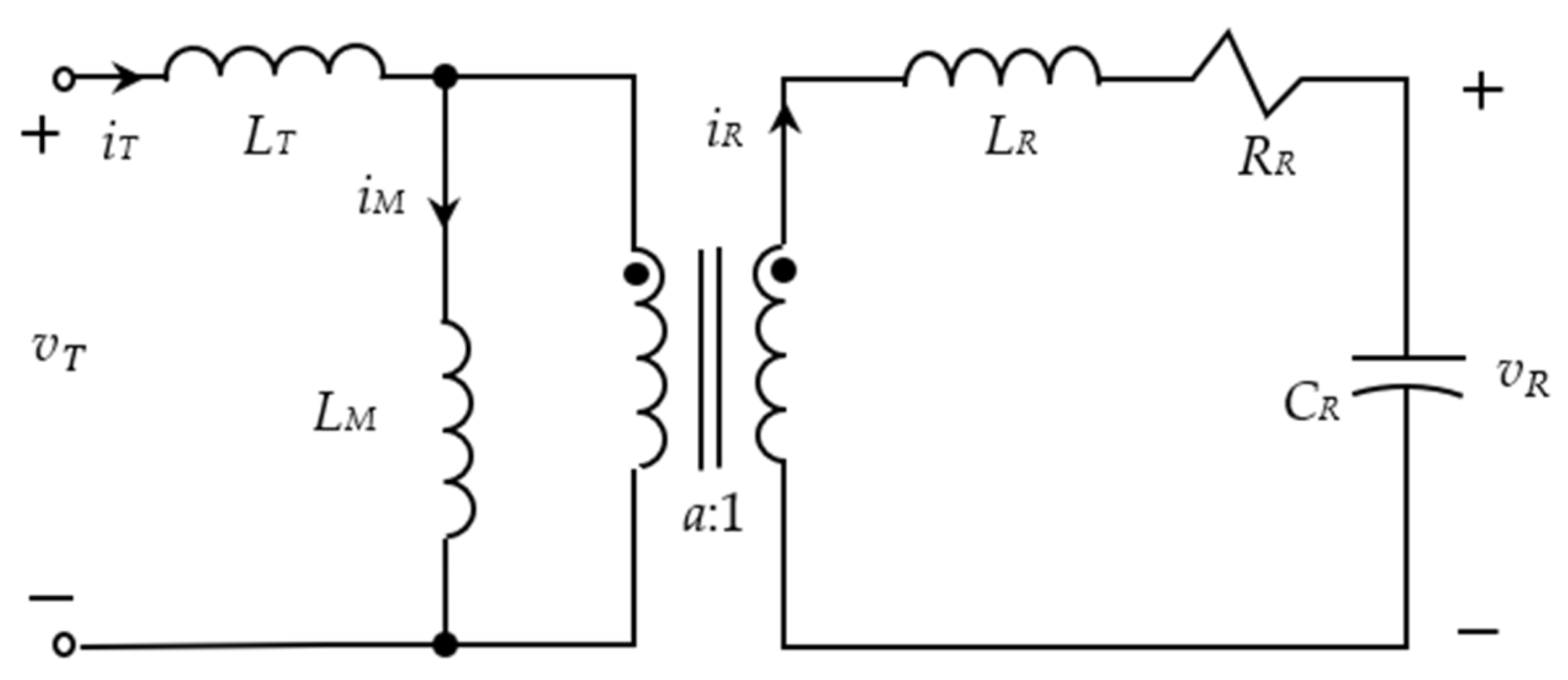
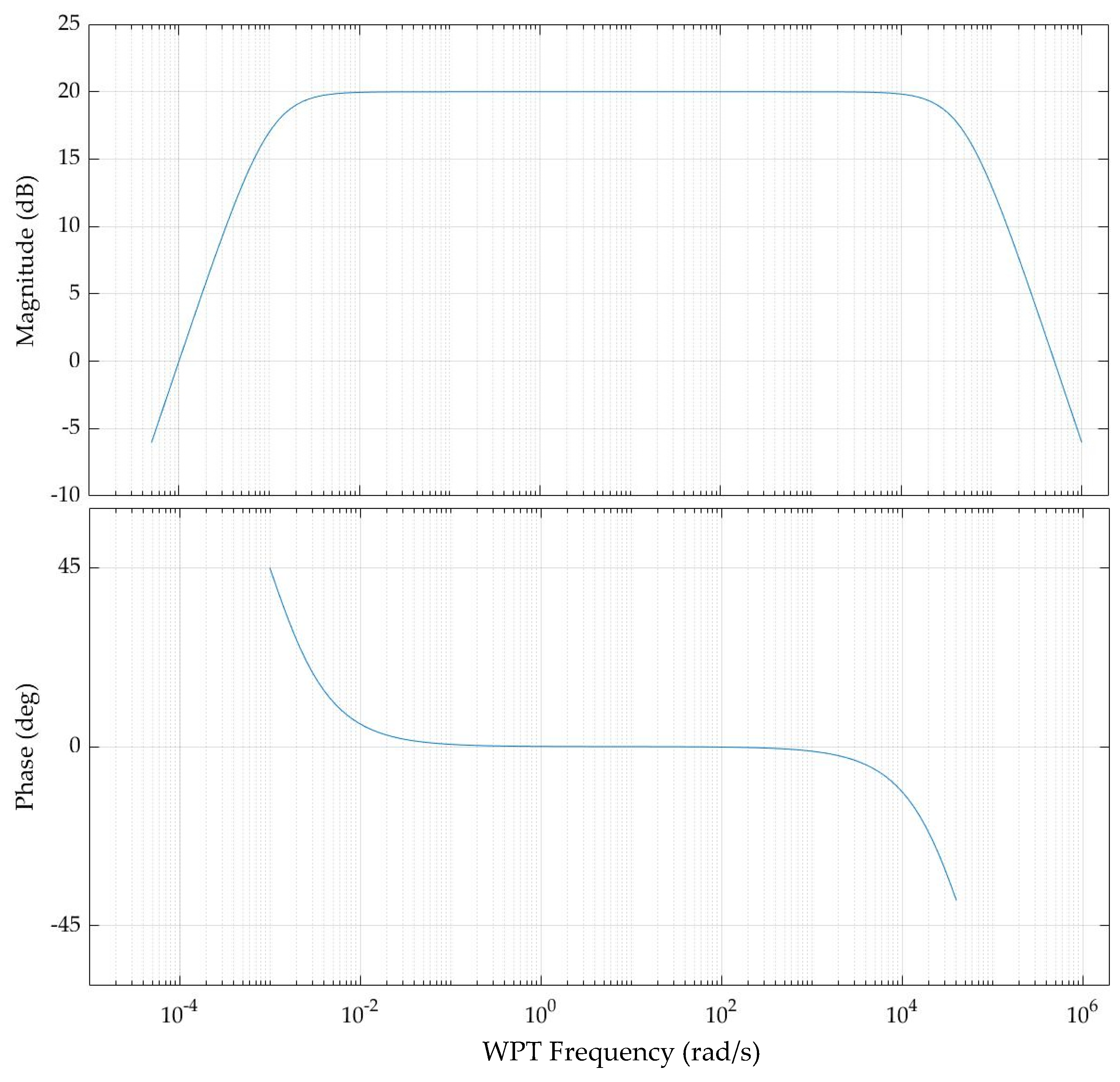
2.3. Transformer Model for WPT
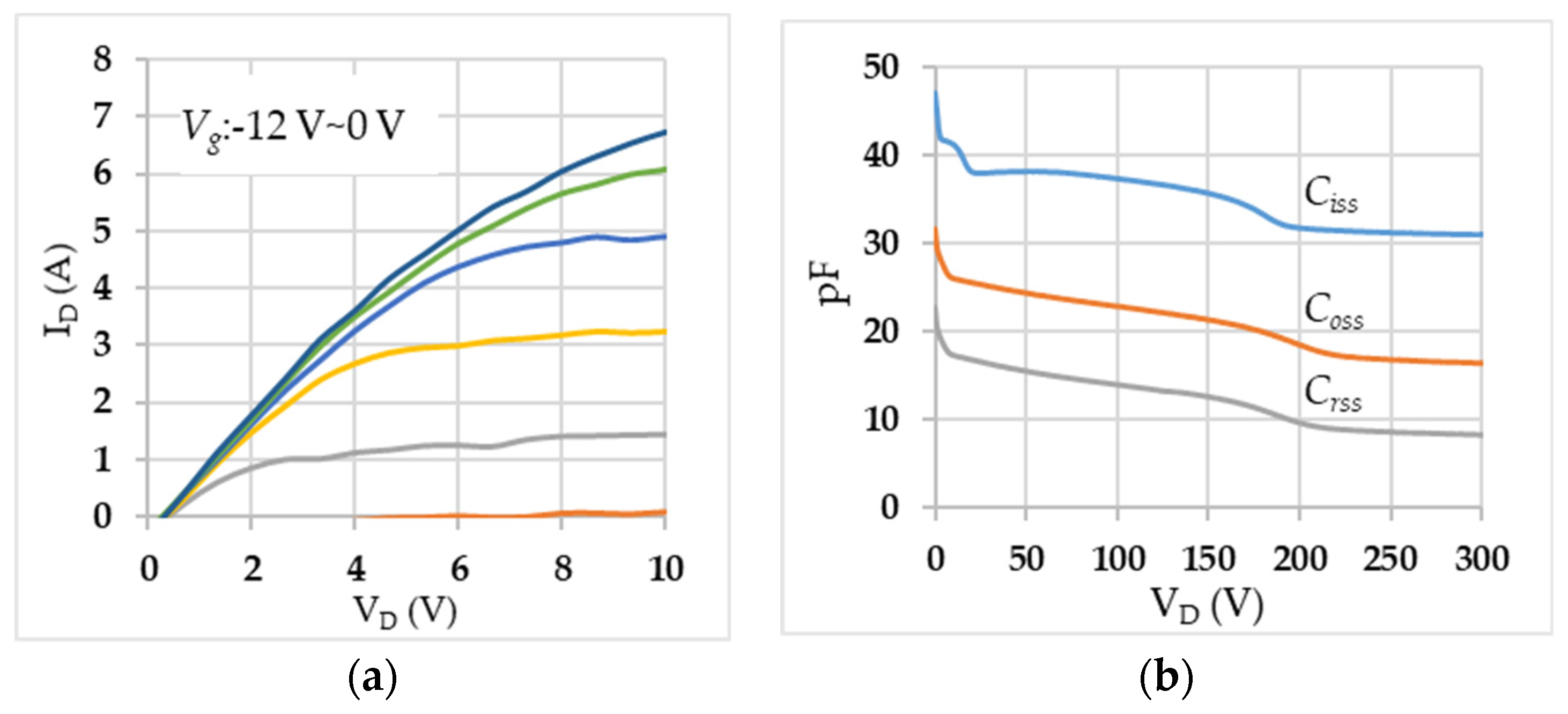
2.4. GaN HEMT Module
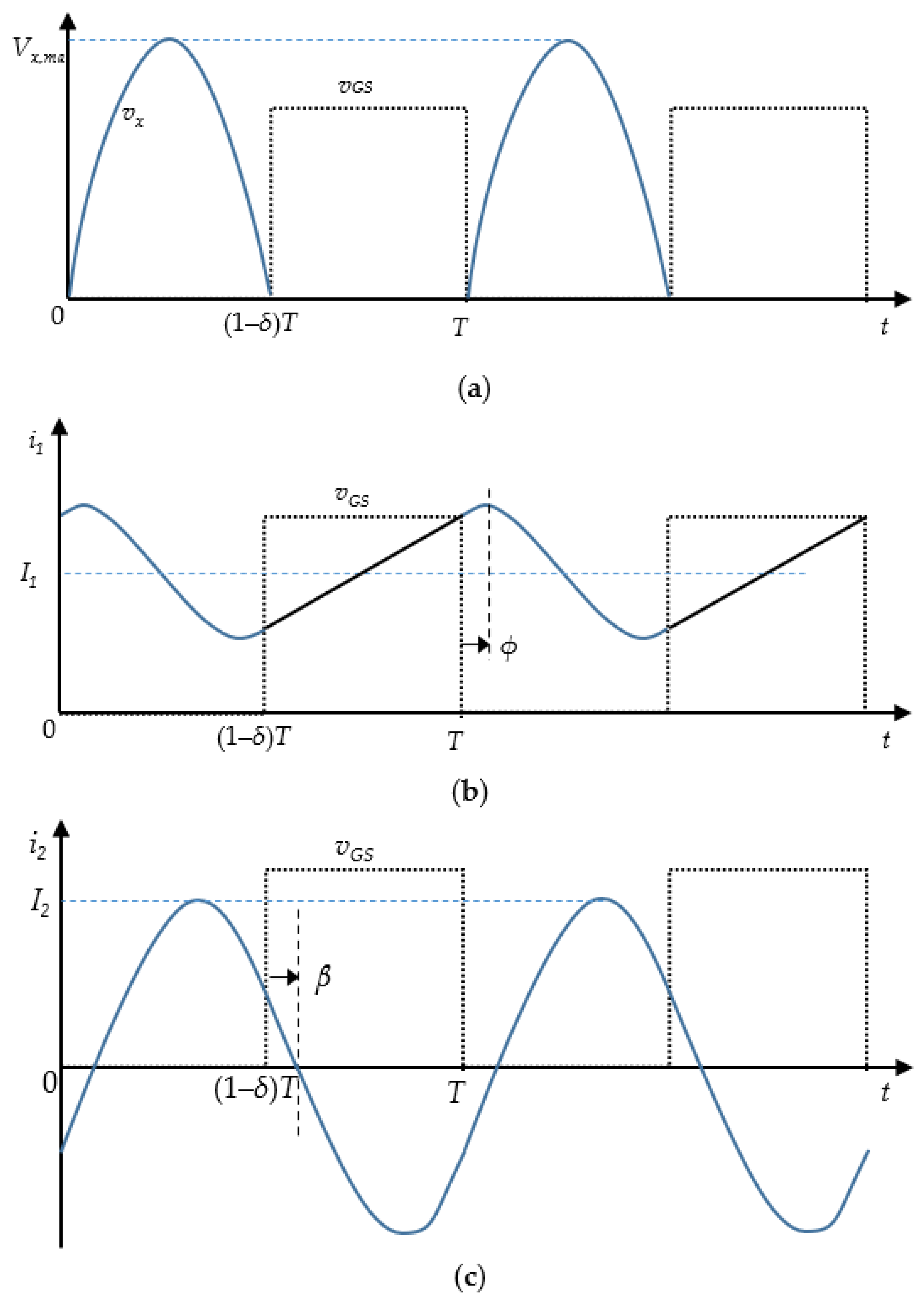
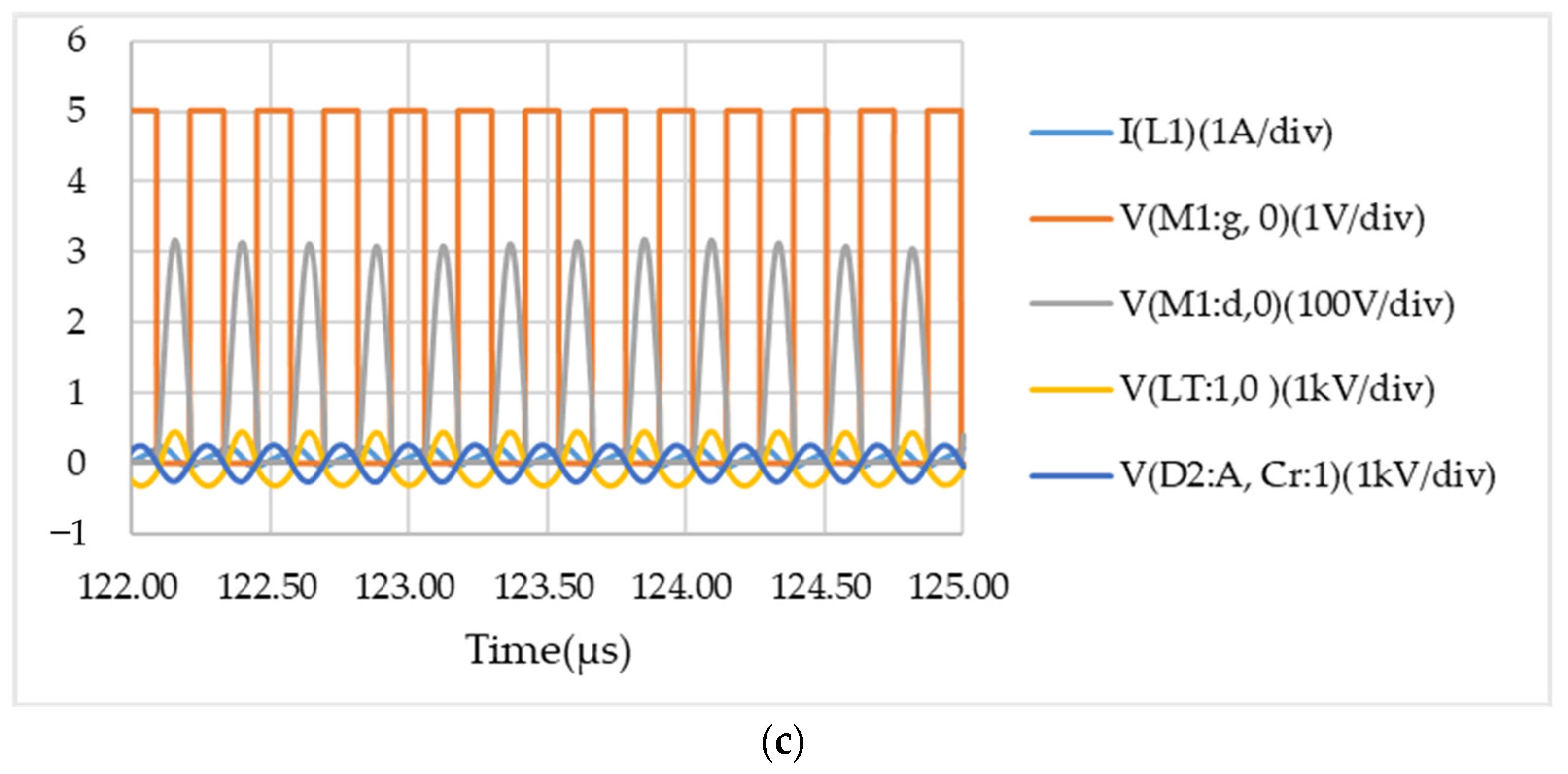
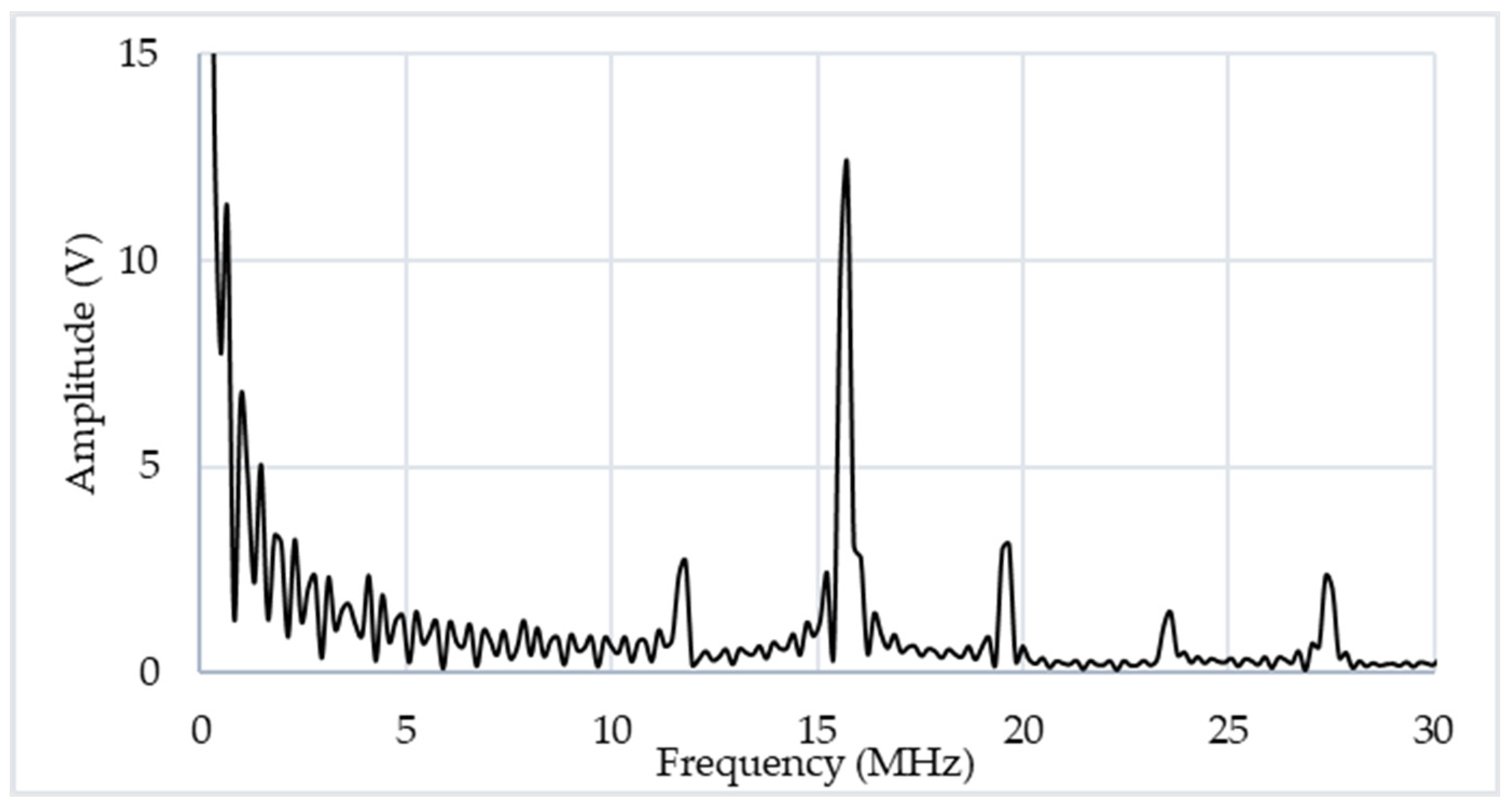
2.5. Subharmonic Oscillation
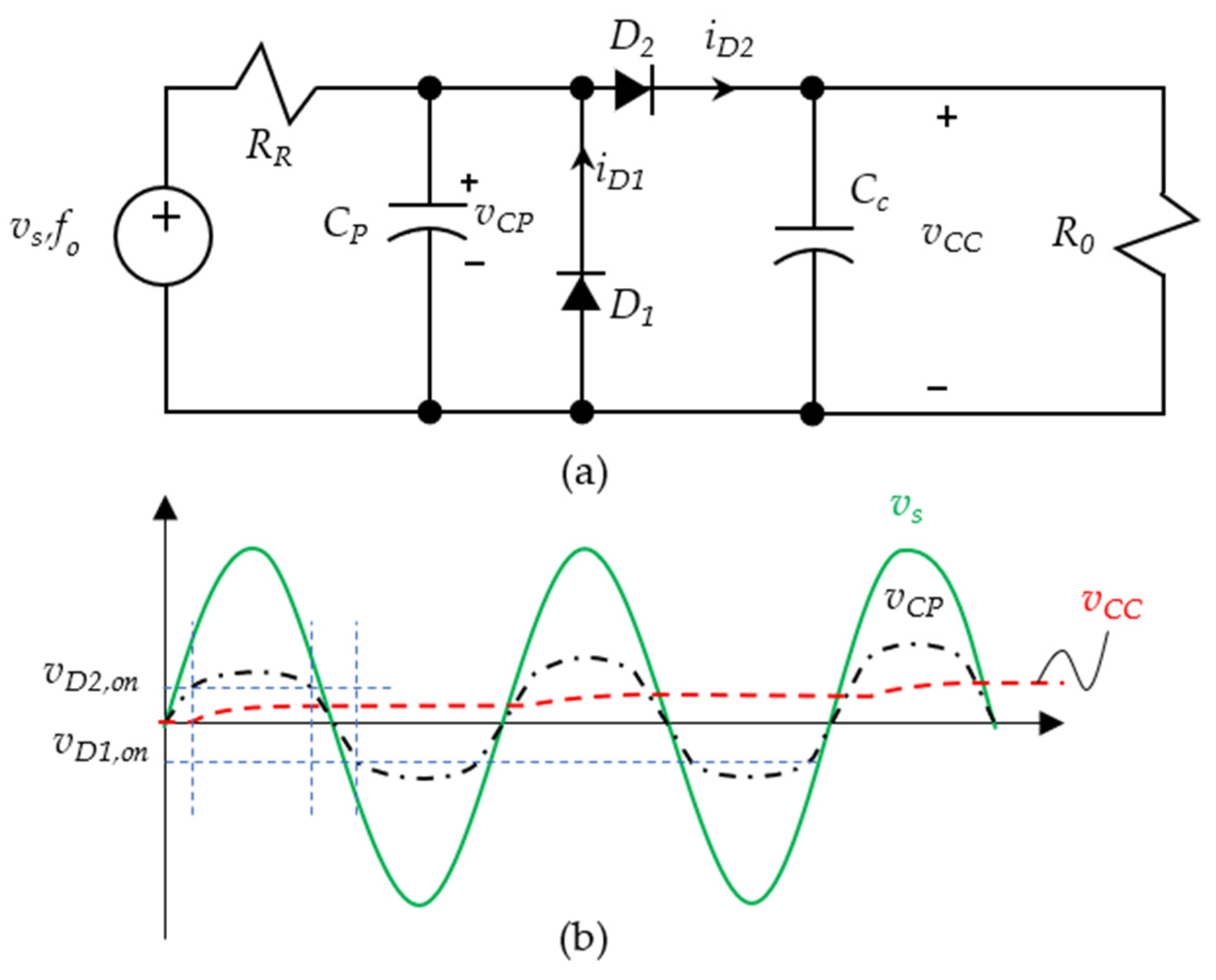
3. PRU Module for Battery Charging
3.1. AC-DC Converter
3.2. Resonant Capacitive Voltage Divider
3.3. Battery Charger Using a Flat-Band Band-Pass Filter
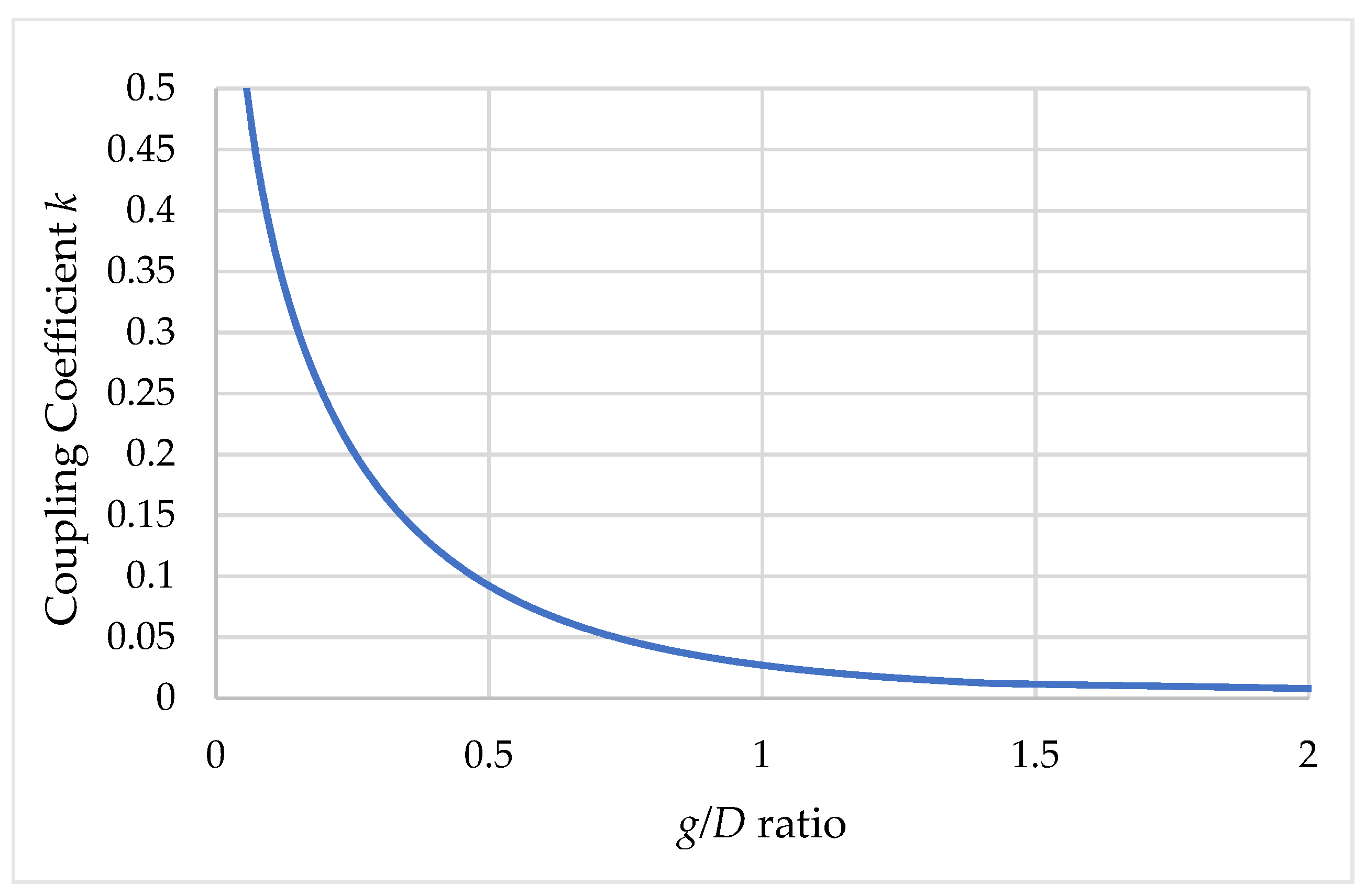
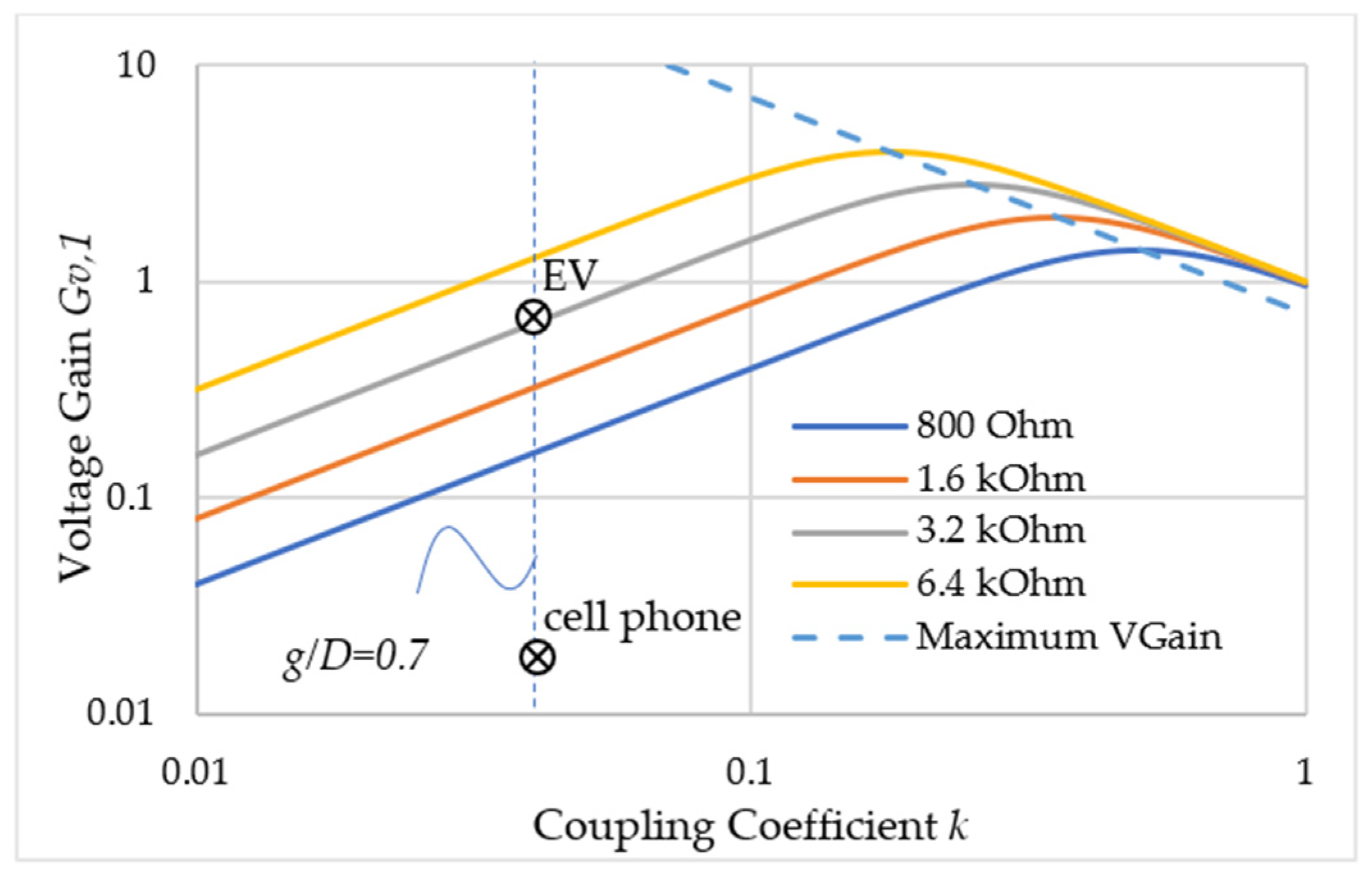
3.4. Magnetic Coupling Coefficient
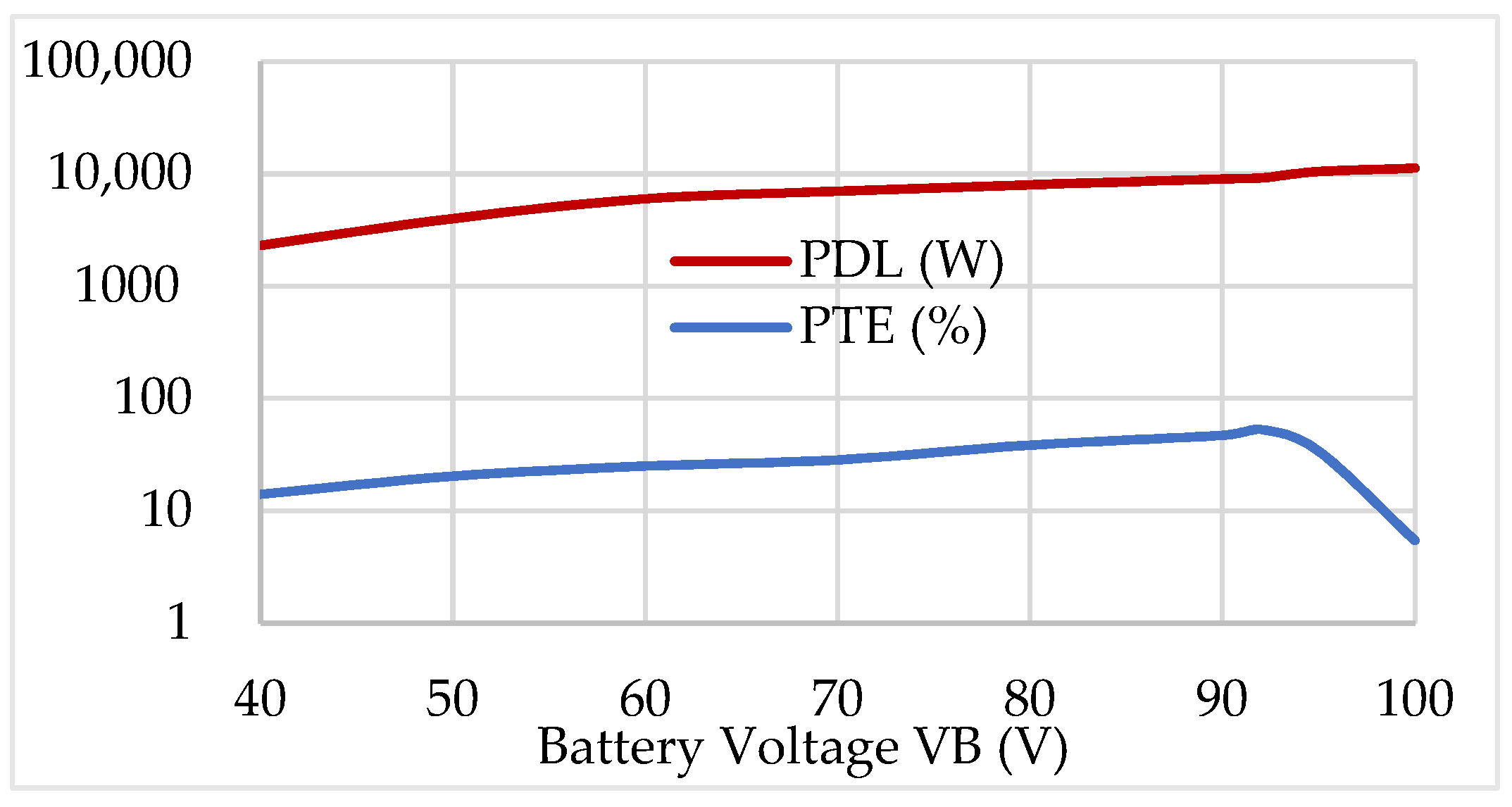
4. Simulation and Experiment
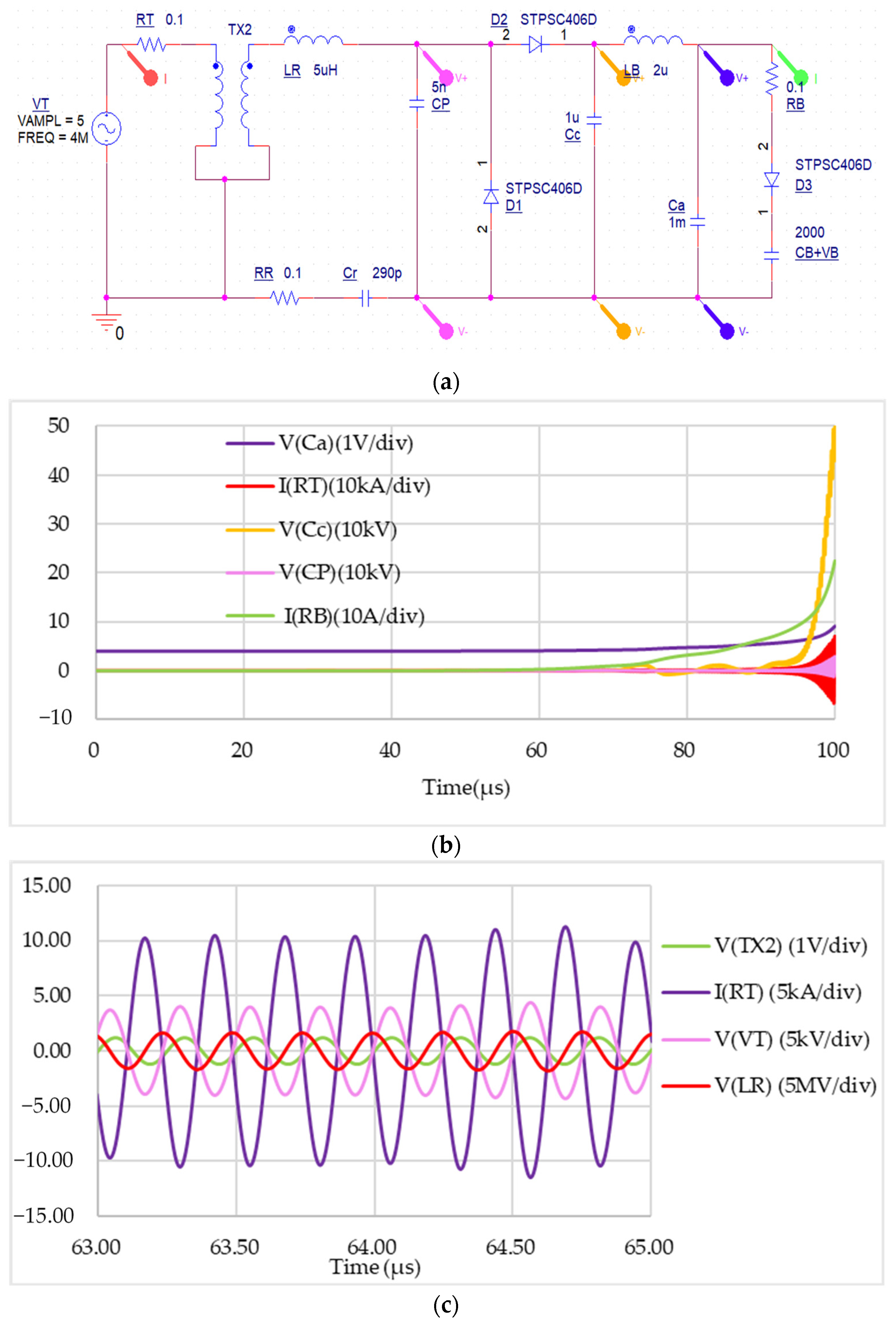
4.1. Simulation
- (1)
- Assigning the voltage of the battery ;
- (2)
- Performing OrCAD PSpice analysis with proper selection of the run-to-time, around 300 us in our study, and step-size, 1 ns in our study;
- (3)
- Exporting the trace data of the power dissipation elements such as diodes, resistors, and transistors;
- (4)
- Exporting the trace data of the battery to calculate the output power;
- (5)
- Selecting the maximum current, 100 A in this study, to define the valid trace data range;
- (6)
- Performing the calculation of the output power by multiplying the input current with the battery and the voltage of the battery itself;
- (7)
- Performing the calculation of the input power by adding the output power together with the power losses from all of the power dissipation elements.
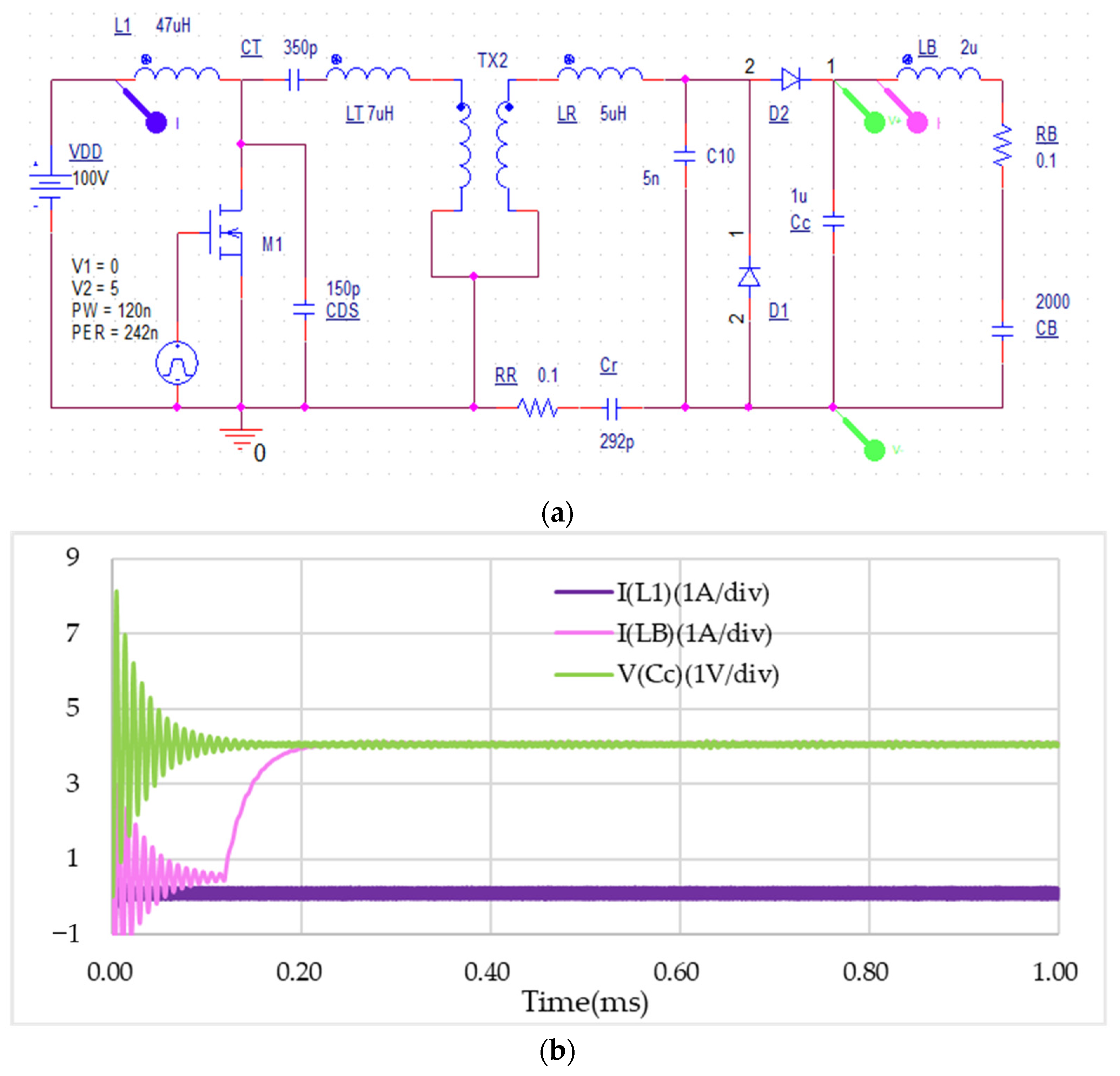
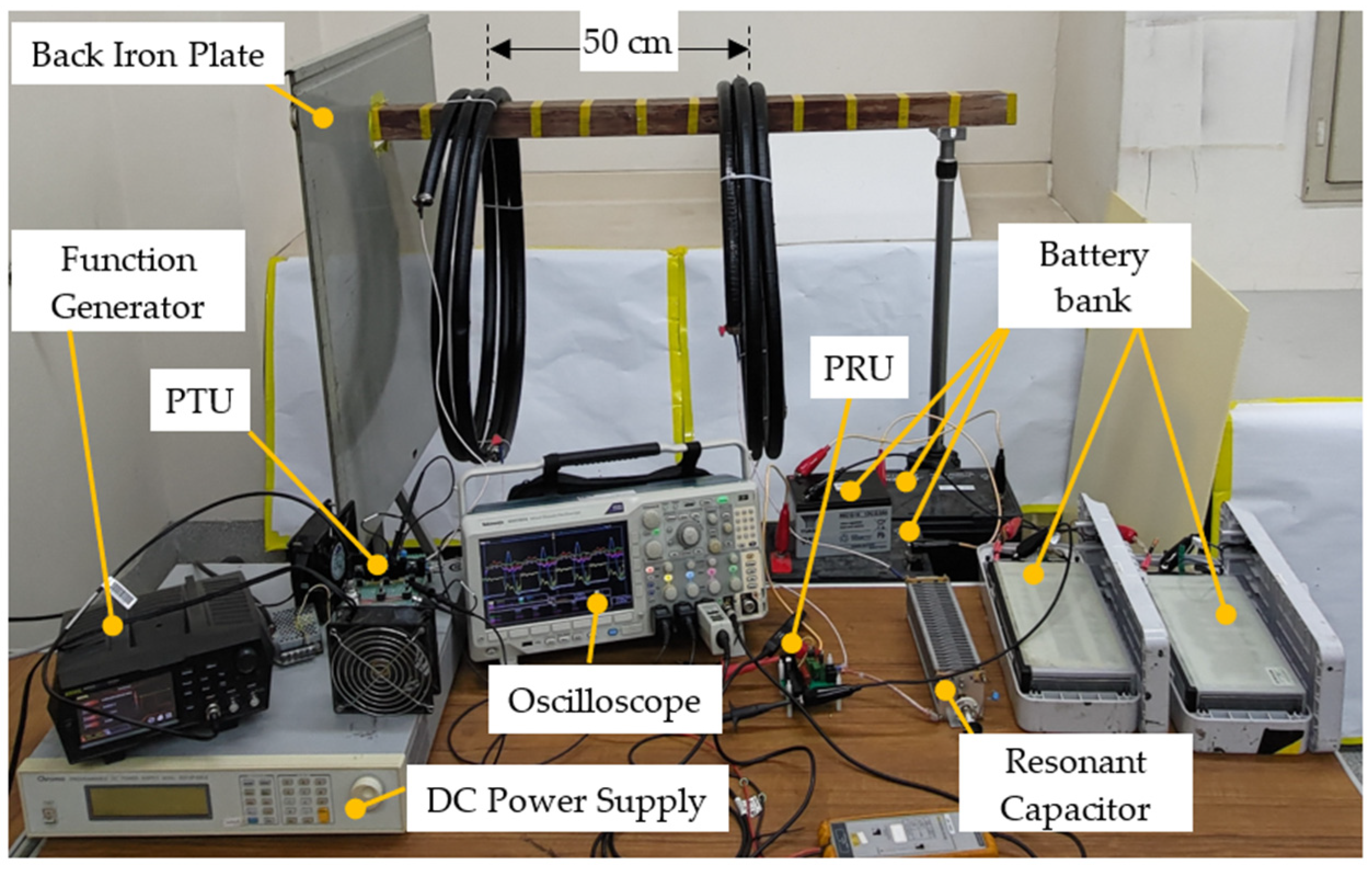
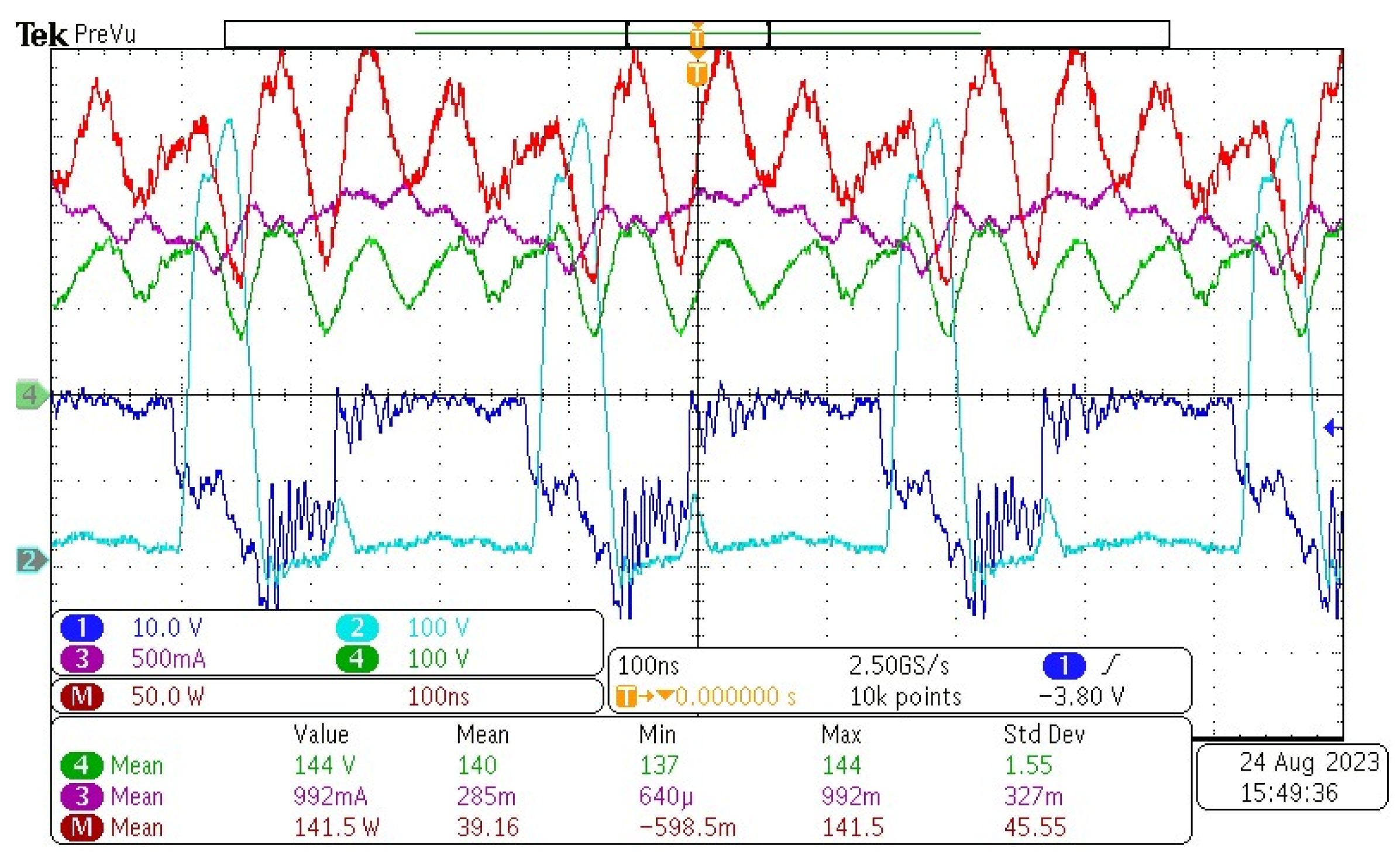
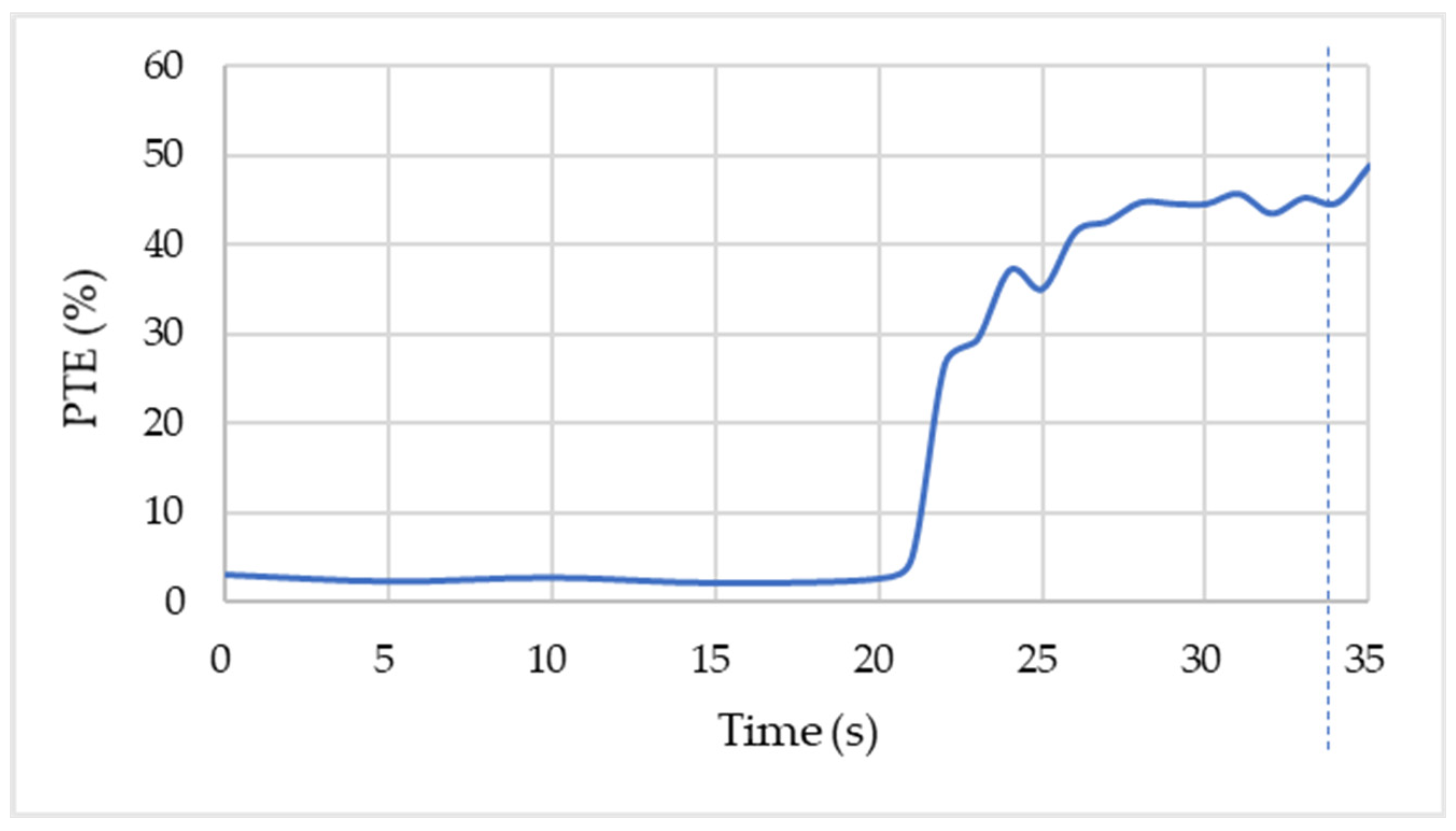
4.2. Experiment
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zhang, Z.; Pang, H.; Georgiadis, A.; Cecati, C. Wireless power transfer—An overview. IEEE Trans. Ind. Electron. 2018, 66, 1044–1058. [Google Scholar] [CrossRef]
- Wang, Y.; Sun, Z.; Guan, Y.; Xu, D. Overview of Megahertz Wireless Power Transfer. Proc. IEEE 2023, 111, 528–554. [Google Scholar] [CrossRef]
- Ahmad, A.; Alam, M.S.; Chabaan, R. A Comprehensive Review of Wireless Charging Technologies for Electric Vehicles. IEEE Trans. Transp. Electrif. 2018, 4, 38–63. [Google Scholar] [CrossRef]
- Yan, Y.; Shi, W.; Zhang, X. Design of UAV wireless power transmission system based on coupling coil structure optimization. EURASIP J. Wirel. Commun. Netw. 2020, 2020, 67. [Google Scholar] [CrossRef]
- Khan, S.R.; Pavuluri, S.K.; Cummins, G.; Desmulliez, M. Wireless Power Transfer Techniques for Implantable Medical Devices: A Review. Sensors 2020, 20, 3487. [Google Scholar] [CrossRef] [PubMed]
- Liu, C.-Y.; Wang, G.-B.; Wu, C.-C.; Chang, E.Y.; Cheng, S.; Chieng, W.-H. Derivation of the Resonance Mechanism for Wireless Power Transfer Using Class-E Amplifier. Energies 2021, 14, 632. [Google Scholar] [CrossRef]
- Paolucci, M.; Green, P.B. Benefits of GaN e-Mode HEMTs in Wireless Power Transfer—GaN Power Devices in Resonant Class D and Class E Radio Frequency Power Amplifiers. Rev 1.0. Infineon, White Paper. Available online: infineon.com (accessed on 1 October 2018).
- Choi, J.; Tsukiyama, D.; Tsuruda, Y.; Davila, J.M.R. High-frequency, high-power resonant inverter with eGaN FET for wireless power transfer. IEEE Trans. Power Electron. 2017, 33, 1890–1896. [Google Scholar] [CrossRef]
- Sinha, S.; Regensburger, B.; Kumar, A.; Afridi, K. A very-high-power-transfer-density GaN-based capacitive wireless power transfer system. In Proceedings of the 2017 IEEE 5th Workshop on Wide Bandgap Power Devices and Applications (WiPDA), Albuquerque, NM, USA, 30 October–1 November 2017; pp. 360–365. [Google Scholar]
- Abou Houran, M.; Yang, X.; Chen, W. Free Angular-Positioning Wireless Power Transfer Using a Spherical Joint. Energies 2018, 11, 3488. [Google Scholar] [CrossRef]
- Jolani, F.; Yu, Y.-Q.; Chen, Z. A planar positioning-free magnetically-coupled resonant wireless power transfer. In Proceedings of the 2015 IEEE Wireless Power Transfer Conference (WPTC), Boulder, CO, USA, 13–15 May 2015; pp. 1–3. [Google Scholar] [CrossRef]
- Liu, C.-Y.; Wu, C.-C.; Tang, L.-C.; Shieh, Y.-T.; Chieng, W.-H.; Chang, E.-Y. Resonant Mechanism for a Long-Distance Wireless Power Transfer Using Class E PA and GaN HEMT. Energies 2023, 16, 3657. [Google Scholar] [CrossRef]
- Li, J.; Qin, R.; Sun, J.; Costonett, D. Systematic Design of a 100-W 6.78-MHz Wireless Charging Station Covering Multiple Devices and a Large Charging Area. IEEE Trans. Power Electron. 2022, 37, 4877–4889. [Google Scholar] [CrossRef]
- Huang, X.; Yu, Z.; Dou, Y.; Lin, S.; Ouyang, Z.; Andersen, M.A.E. Load-Independent Push–Pull Class E 2 Topology with Coupled Inductors for MHz-WPT Applications. IEEE Trans. Power Electron. 2022, 37, 8726–8737. [Google Scholar] [CrossRef]
- Liu, J.; Liu, Z.; Chen, W.; Sun, Z.; Su, H. An Optimized Coil Array and Passivity-Based Control for Receiving Side Multilevel Connected DC-DC Converter of Dynamic Wireless Charging. IEEE Trans. Veh. Technol. 2022, 71, 3715–3726. [Google Scholar] [CrossRef]
- Hou, X.; Hu, H.; Su, Y.; Liu, Z.; Deng, Z.; Deng, R. A Multirelay Wireless Power Transfer System With Double-Sided LCC Compensation Network for Online Monitoring Equipment. IEEE J. Emerg. Sel. Top. Power Electron. 2022, 11, 1262–1271. [Google Scholar] [CrossRef]
- Li, J.; Wang, C.; Wang, L.; Ding, Y.; Yu, T.; Cheng, Y. A comparative study on transmission performance of multistage wireless power transfer systems using SPS compensation and LCC compensation. Int. J. Circuit Theory Appl. 2022, 51, 1625–1641. [Google Scholar] [CrossRef]
- Larky, A. Negative-impedance converters. IRE Trans. Circuit Theory 1957, 4, 124–131. [Google Scholar] [CrossRef]
- Yoon, S.H.; Kim, T.H.; Yook, J.G.; Yun, G.H.; Lee, W.Y. High Q-factor WPT system with negative impedance converter. In Proceedings of the 2017 IEEE Wireless Power Transfer Conference (WPTC), Taipei, Taiwan, 10–12 May 2017; pp. 1–4. [Google Scholar]
- Kim, T.H.; Yun, G.H.; Lee, W.; Yook, J.G. Highly efficient WPT system with negative impedance converter for Q-factor improvement. IEEE Access 2019, 7, 108750–108760. [Google Scholar] [CrossRef]
- Abramov, E.; Schultz, Y.; Evzelman, M.; Peretz, M.M. Analysis and design of post-regulation stages for resonant capacitively-coupled wireless power systems. In Proceedings of the 2022 IEEE Applied Power Electronics Conference and Exposition (APEC), Houston, TX, USA, 20–24 March 2022; pp. 492–499. [Google Scholar]
- Harmonic and Subharmonic Oscillations. Available online: https://phys.libretexts.org/bookshelves/classical_mechanics/essential_graduate_physics_-_classical_mechanics_(likharev)/05%3a_oscillations/5.08%3a_harmonic_and_subharmonic_oscillations (accessed on 17 September 2023).
- Hayashi, C. Subharmonic oscillations in nonlinear systems. J. Appl. Phys. 1953, 24, 521–529. [Google Scholar] [CrossRef]
- Weng, Y.-C.; Wu, C.-C.; Chang, E.Y.; Chieng, W.-H. Minimum Power Input Control for Class-E Amplifier Using Depletion-Mode Gallium Nitride High Electron Mobility Transistor. Energies 2021, 14, 2302. [Google Scholar] [CrossRef]
- Ke, C.-Y.; Wang, W.-C.; Ker, M.-D.; Yang, C.-Y.; Chang, E.Y. Investigation on ESD robustness of 1200-V D- mode GaN MIS-HEMTs with HBM ESD test and TLP measurement. In Proceedings of the 2023 International VLSI Symposium on Technology, Systems and Applications (VLSI-TSA/VLSI-DAT), Hsinchu, Taiwan, 17–20 April 2023; pp. 1–2. [Google Scholar] [CrossRef]
- Preuss, V.H.B. A Software Package for the Steady-State Simulation of Autonomous Circuits Using the Harmonic Balance Method. Master’s Thesis, Federal University of Santa Catarina, Florianópolis, Brazil, 2021. [Google Scholar]
- Keysight PathWave Advanced Design System (ADS), Chapter 3 Harmonic Balance (HB) Simulation. Available online: https://www.keysight.com/us/en/products/software/pathwave-design-software/pathwave-advanced-design-system.html (accessed on 17 November 2023).
- Donati Guerrieri, S.; Ramella, C.; Catoggio, E.; Bonani, F. Bridging the Gap between Physical and Circuit Analysis for Variability-Aware Microwave Design: Modeling Approaches. Electronics 2022, 11, 860. [Google Scholar] [CrossRef]
- Shieh, Y.-T.; Wu, C.-C.; Liu, C.-Y.; Chieng, W.-H.; Su, Y.-S.; Jeng, S.-L.; Chang, E.-Y. Lithium Battery Model and Its Application to Parallel Charging. Energies 2022, 15, 4767. [Google Scholar] [CrossRef]





| Symbol | Description | Unit | VDS | |
|---|---|---|---|---|
| 0 V | 600 V | |||
| Coss | Output capacitance | pF | 31 | 17 |
| Ciss | Input capacitance | pF | 46 | 31 |
| Crss | Feedback capacitance | pF | 23 | 8 |
| Vth | Gate turn on voltage | V | −9 | |
| VGS | Maximum | V | 10~−30 | |
| RDS,on | On resistance | 900 | ||
| VBD | Breakdown voltage | V | 600 | |
| iD,cont. | Continuous drain current | A | 3 | |
| Item | Description | Unit | Value |
|---|---|---|---|
| PTU coil resistance | Ω | 0.1 | |
| PRU coil resistance | Ω | 0.1 | |
| Battery resistance | Ω | 0.1 | |
| Leakage inductance of WPT transformer | uH | 5 | |
| Magnetization inductance of WPT transformer | uH | 0.1 | |
| Band pass filter inductance | uH | 2 | |
| High voltage, fast recovery diode; SiC | STPSC406D | ||
| PRU resonance capacitance | pF | 290 | |
| PRU AC voltage divider capacitance | nF | 5 | |
| Battery capacitance | F | 2000 | |
| PRU AC-DC output capacitance | uF | 1 | |
| PRU stabilization capacitance | mF | 1 | |
| k | Magnetic coupling coefficient | 1 | |
| Battery voltage | V | 4 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, C.-Y.; Tang, H.-C.; Shieh, Y.-T.; Wu, C.-C.; Chieng, W.-H.; Chang, E.-Y.; Ueda, D. Power Receiving Unit for High-Power Resonant Wireless Power Transfer. Energies 2023, 16, 7856. https://doi.org/10.3390/en16237856
Liu C-Y, Tang H-C, Shieh Y-T, Wu C-C, Chieng W-H, Chang E-Y, Ueda D. Power Receiving Unit for High-Power Resonant Wireless Power Transfer. Energies. 2023; 16(23):7856. https://doi.org/10.3390/en16237856
Chicago/Turabian StyleLiu, Ching-Yao, Hsien-Chung Tang, Yueh-Tsung Shieh, Chih-Chiang Wu, Wei-Hua Chieng, Edward-Yi Chang, and Daisuke Ueda. 2023. "Power Receiving Unit for High-Power Resonant Wireless Power Transfer" Energies 16, no. 23: 7856. https://doi.org/10.3390/en16237856
APA StyleLiu, C.-Y., Tang, H.-C., Shieh, Y.-T., Wu, C.-C., Chieng, W.-H., Chang, E.-Y., & Ueda, D. (2023). Power Receiving Unit for High-Power Resonant Wireless Power Transfer. Energies, 16(23), 7856. https://doi.org/10.3390/en16237856






