Prediction and Measurement of the Heat Transfer Coefficient in Direct, Oil-Cooled Batteries
Abstract
:1. Introduction
2. A Direct, Oil-Cooled Battery Pack
2.1. Conceptual Development
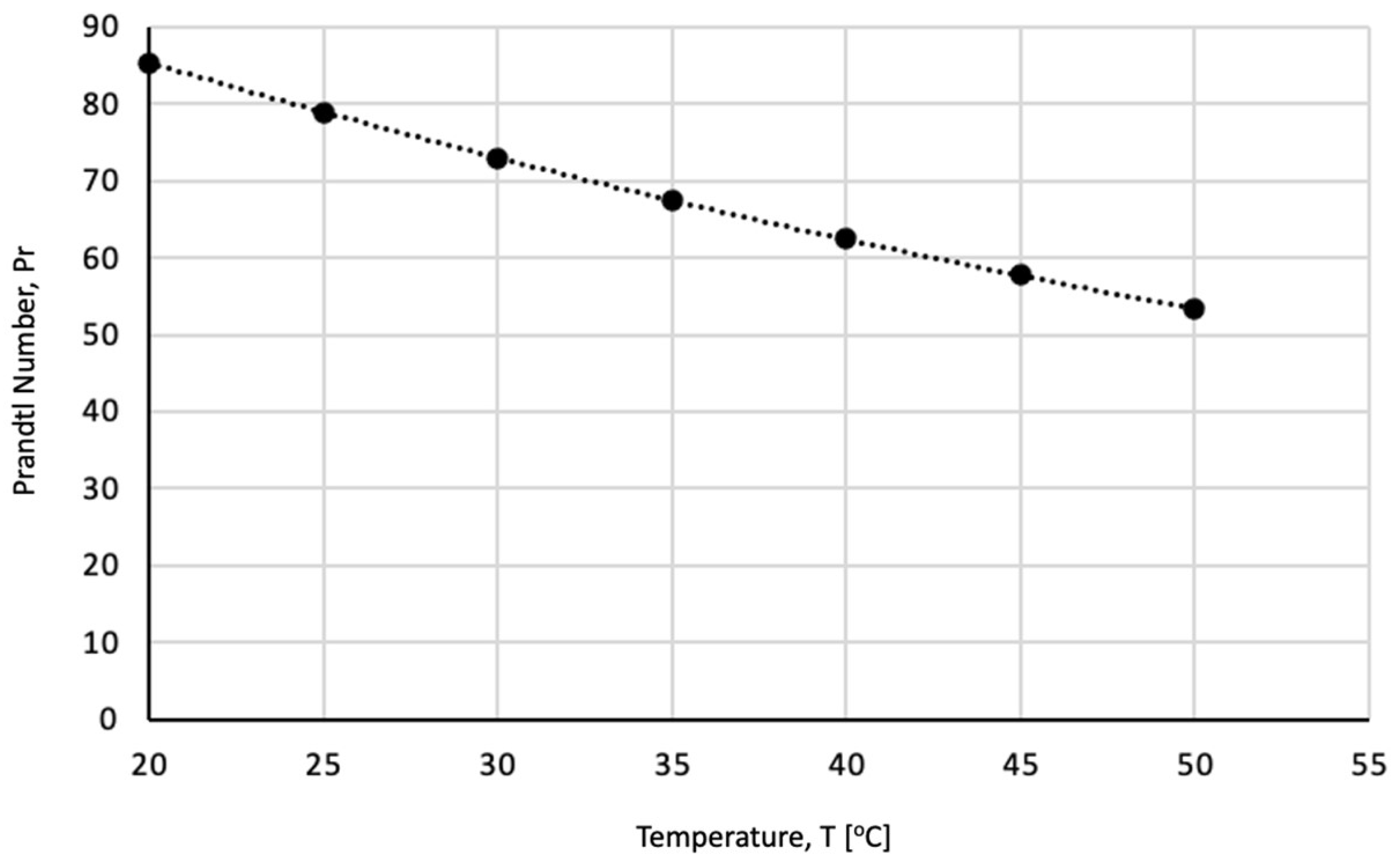
2.2. Empirical Correlations
3. Experimental Work
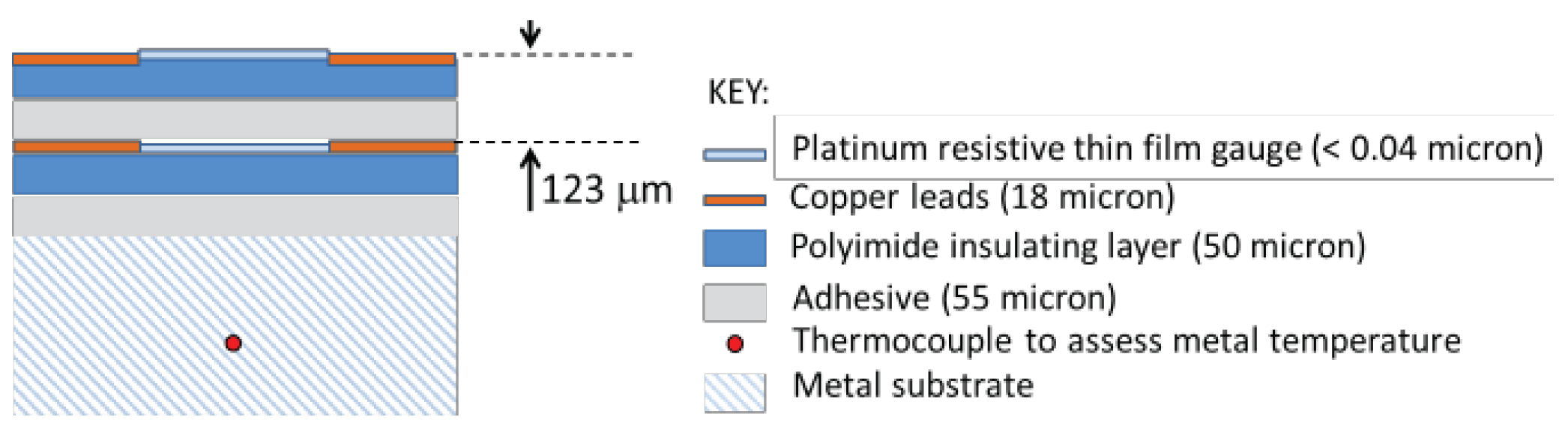
3.1. Heat Flux Measurement: Application in Oil Cooling
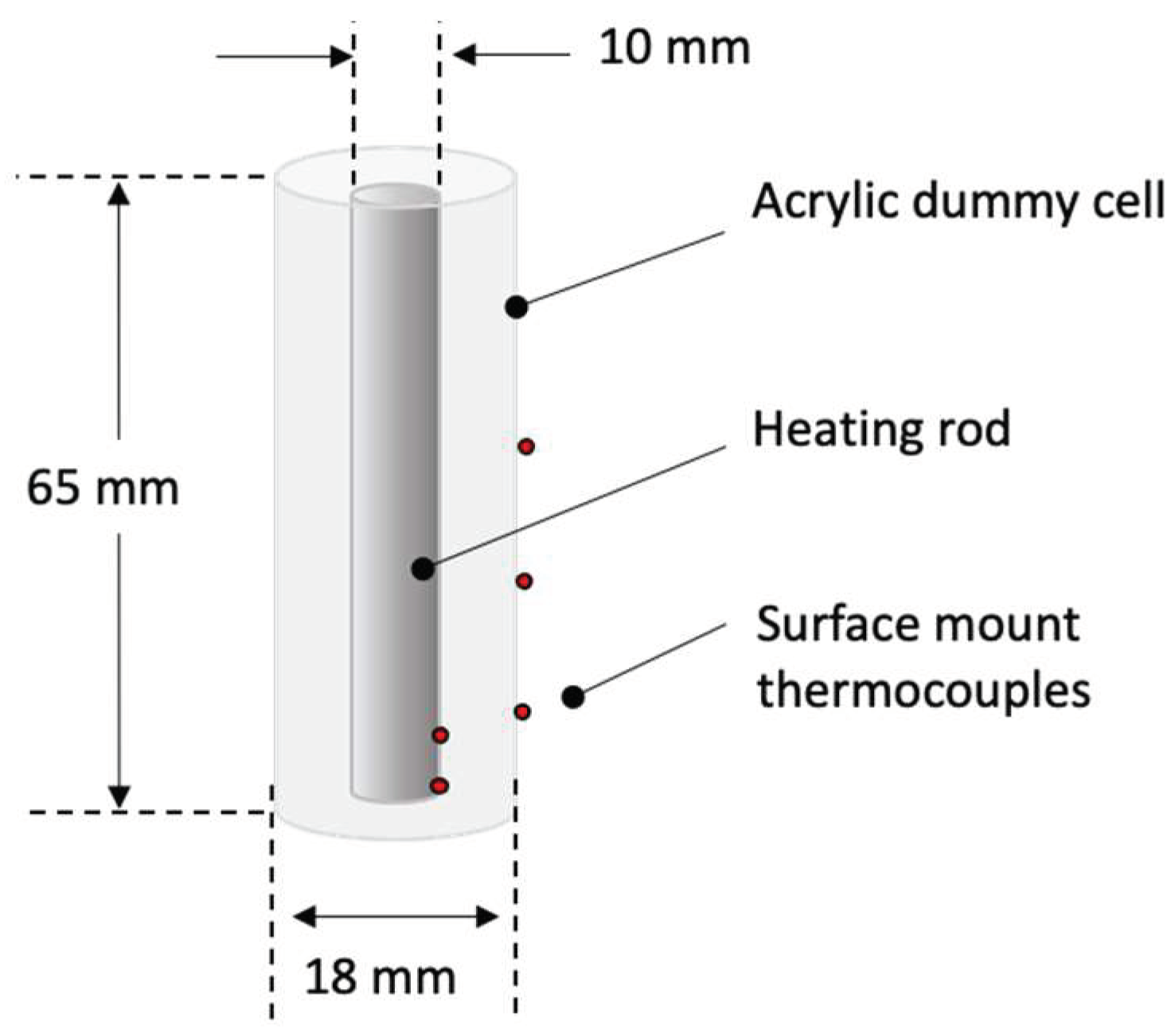
3.2. The Instrumented Cell
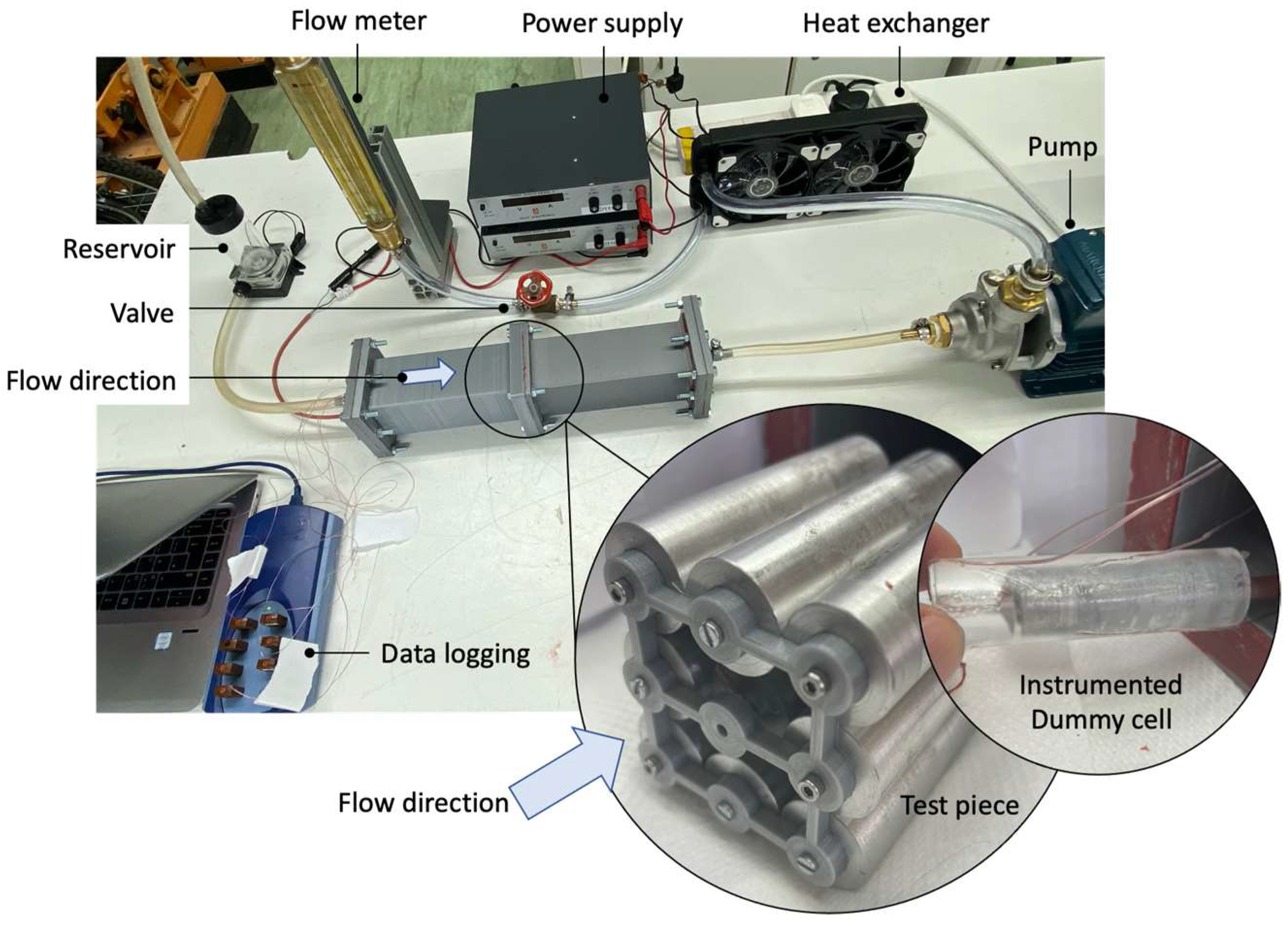
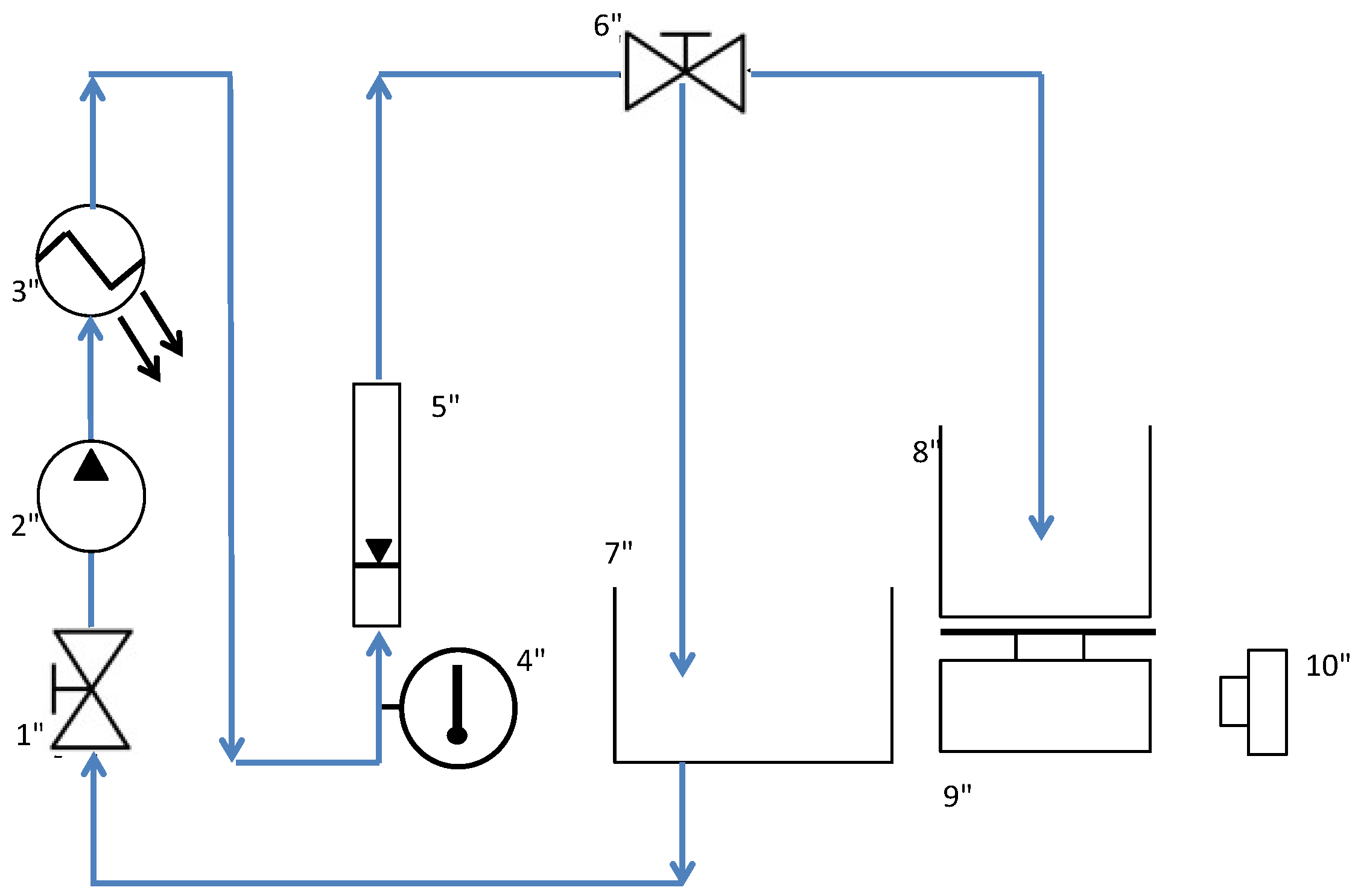
3.3. The Flow Test Rig
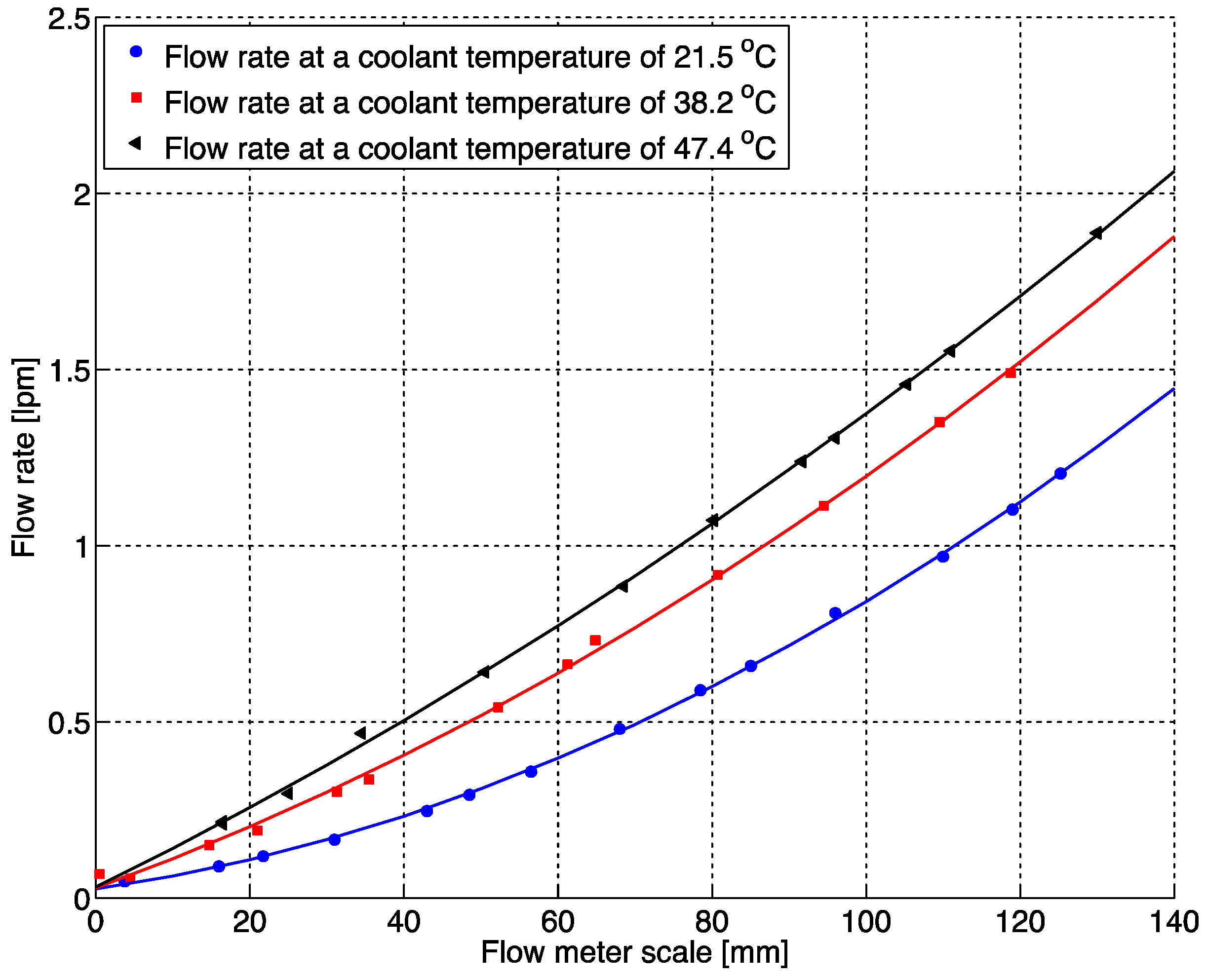
3.4. Flow Calibration
3.5. Uncertainty Analysis
4. Test Results and Discussion
4.1. Analysis
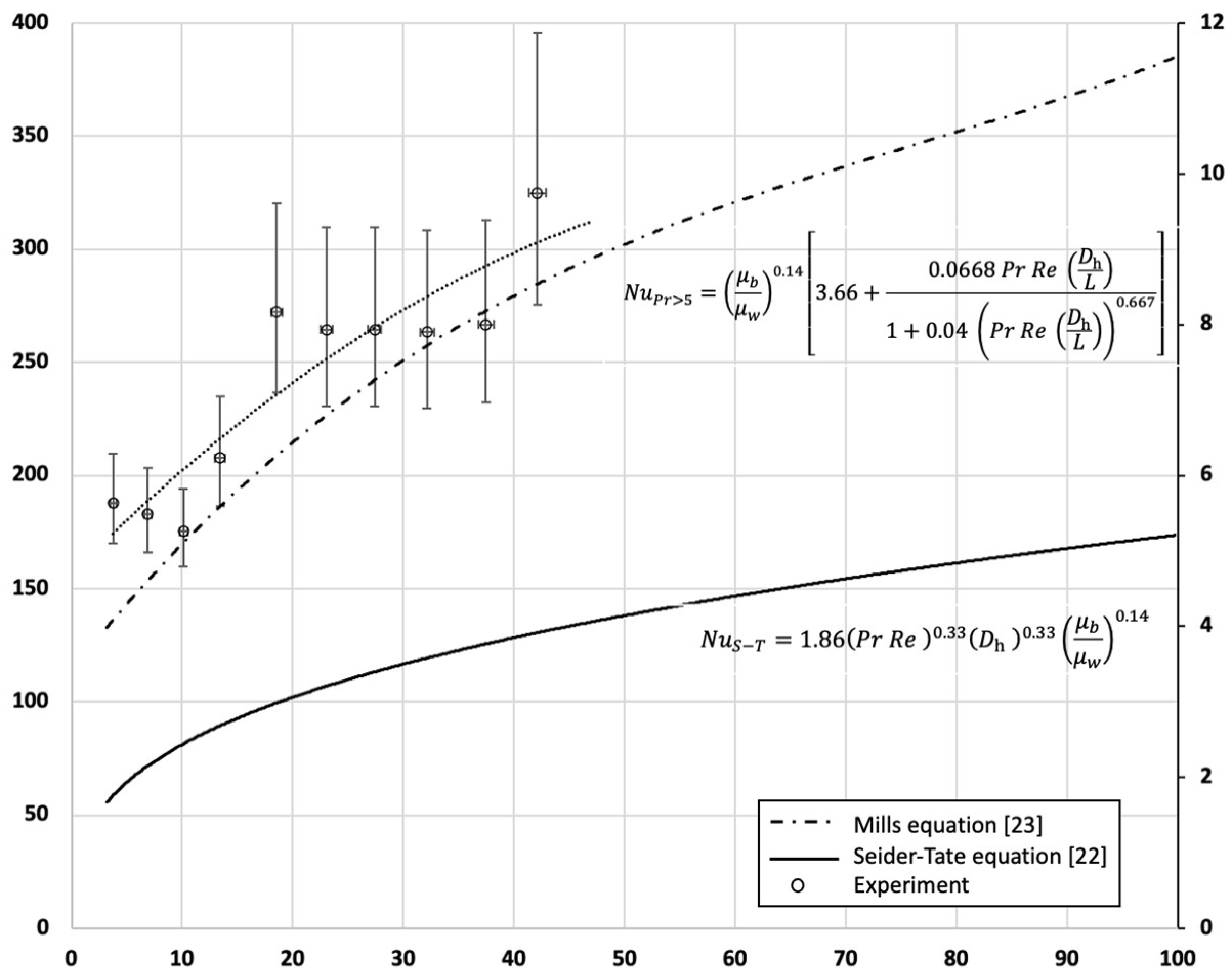
4.2. Comparison of Empirical and Experimental Results
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Xia, G.; Cao, L.; Bi, G. A review on battery thermal management in electrical vehicle application. J. Power Sources 2017, 367, 90–105. [Google Scholar] [CrossRef]
- Growth of Li-Ion Battery Manufacturing Capacity in Key EV Markets. S&P Global. Available online: https://www.spglobal.com/mobility/en/research-analysis/growth-of-liion-battery-manufacturing-capacity.html (accessed on 2 February 2023).
- Armand, M.; Tarascon, J.M. Building better batteries. Nature 2008, 451, 652–657. [Google Scholar] [CrossRef] [PubMed]
- He, F.; Ma, L. Thermal Management on Hybrid Power Systems Using Cylindrical and Prismatic Battery Cells. Heat Transf. Eng. 2015, 37, 581–590. [Google Scholar] [CrossRef]
- Bandhauer, T.M.; Garimella, S.; Fuller, T.F. A critical review of thermal issues in lithium-ion batteries. J. Electrochem. Soc. 2015, 138, R1–R25. [Google Scholar] [CrossRef]
- Pesaran, A.; Keyser, M.; Kim, G.; Santhanagopalan, S.; Smith, K. Tools for designing thermal management of batteries in electric drive vehicles. In Proceedings of the Advanced Automotive Battery Conference, Pasadena, CA, USA, 4–8 February 2013; Available online: https://www.nrel.gov/docs/fy13osti/57747.pdf (accessed on 10 January 2023).
- Offer, G.; Patel, Y.; Hales, A.; Diaz, L.B.; Marzook, M. Cool metric for lithium-ion batteries could spur progress. Nature 2020, 582, 485–487. [Google Scholar] [CrossRef]
- Tennessen, A.P.T.; Weingtraub, J.; Herman, W.A. Battery Coolant Jacket. U.S. Patent 20130004820A1, 11 February 2014. Available online: https://patents.google.com/patent/US20130004820A1/en (accessed on 20 December 2022).
- Chan, B.J.; Rao, V. Vehicle Battery Module with Cooling and Safety Features. U.S. Patent 9,620,830, 11 April 2017. Available online: https://patents.google.com/patent/US9620830B2/en (accessed on 20 December 2022).
- Ahn, J.; Woo, H.; Jung, D.Y.; Namgoong, J.E. Cooling System for Battery Packs. U.S. Patent 7,560,190, 14 July 2009. Available online: https://patents.google.com/patent/US7560190B2/en (accessed on 20 December 2022).
- Fan, L.; Khodadadi, J.M.; Pesaran, A.A. A parametric study on thermal management of an air-cooled lithium-ion battery module for plug-in hybrid electric vehicles. J. Power Sources 2013, 238, 301–312. [Google Scholar] [CrossRef]
- Yang, N.; Zhang, X.; Li, G.; Hua, D. Assessment of the forced air-cooling performance for cylindrical lithium-ion battery packs: A comparative analysis between aligned and staggered cell arrangements. Appl. Therm. Eng. 2015, 80, 55–65. [Google Scholar] [CrossRef]
- Wang, T.; Tseng, K.J.; Zhao, J. Development of efficient air-cooling strategies for lithium-ion battery module based on empirical heat source model. Appl. Therm. Eng. 2015, 90, 521–529. [Google Scholar] [CrossRef]
- Hermann, W.A. Liquid Cooling Manifold with Multi-Function Thermal Interface. U.S. Patent 20100104938A1, 11 September 2012. Available online: https://patents.google.com/patent/US8263250B2/en (accessed on 20 December 2022).
- Kandlikar, S.G.; Hayner, C.N. Liquid cooled cold plates for industrial high power electronic devices—Thermal design and Manufacturing Considerations. Heat Transf. Eng. 2010, 30, 918–930. [Google Scholar] [CrossRef]
- Sawani, M.; Camilleri, R. Investigating the temperature profile of Li-ion battery cells under various cooling technologies. In Proceedings of the 16th UK Heat Transfer Conference (UKHTC2019), Nottingham, UK, 8–10 September 2019. [Google Scholar]
- Spitthoff, L.; Shearing, P.R.; Burheim, O.S. Temperature, Ageing and Thermal Management of Lithium-Ion Batteries. Energies 2021, 14, 1248–1278. [Google Scholar] [CrossRef]
- Leng, F.; Tan, C.M.; Pecht, M. Effect of Temperature on the Aging rate of Li ion Battery Operating above Room Temperature. Nat. Sci. Rep. 2015, 5, 12967. [Google Scholar] [CrossRef]
- Roe, C.; Feng, X.; White, G.; Li, R.; Wang, H.; Rui, X.; Wu, B. Immersion cooling for lithium-ion batteries—A review. J. Power Sources 2022, 525, 231094. [Google Scholar] [CrossRef]
- Jayarajan, S.A.; Azimov, U. CFD Modelling and thermal Analysis of a Cold Plate Design with a Zig-Zag Serpentine Flow Pattern for Li-Ion Batteries. Energies 2023, 16, 5243. [Google Scholar] [CrossRef]
- Dubey, P.; Pulugundla, G.; Srouji, A.K. Direct Comparison of Immersion and Cold-Plate Based Cooling for Automotive Battery Modules. Energies 2021, 14, 1259. [Google Scholar] [CrossRef]
- Anisha; Kumar, A. Identification and Mitigation of Shortcomings in Direct and Indirect Liquid Cooling-Based Battery Thermal Management System. Energies 2023, 16, 3857. [Google Scholar] [CrossRef]
- Camilleri, R.; Howey, D.A.; McCulloch, M.D. Predicting the temperature and flow distribution in a direct oil-cooled electrical machine with segmented stator. IEEE Trans. Ind. Electron. 2015, 63, 82–91. [Google Scholar] [CrossRef]
- Camilleri, R.; McCulloch, M.D. Assessing the Temperature and Current Density of Flat Winding Coils for Concentrated Windings Within a Segmented Stator Machine. IEEE Trans. Ind. Appl. 2021, 57, 2440–2448. [Google Scholar] [CrossRef]
- Camilleri, R.; McCulloch, M.D. Integrating a Heat Sink into Concentrated Wound Coils to Improve the Current Density of an Axial Flux, Direct Liquid Cooled Electrical Machine with Segmented Stator. Energies 2021, 14, 3619. [Google Scholar] [CrossRef]
- Özdemir, T.; Amini, A.; Ekici, Ö.; Köksal, M. Experimental Assessment of the Lumped Lithium-Ion Battery Model at Different Operating Conditions. Heat Transf. Eng. 2021, 43, 314–325. [Google Scholar] [CrossRef]
- Incropera, F.; Dewitt, D.P.; Bergman, T.L.; Lavine, A.S. Internal Flow: Laminar flow in circular tubes. In Introduction to Heat Transfer, 5th ed.; Wiley & Sons Inc.: New York, NY, USA, 2007; pp. 482–483+514. [Google Scholar]
- Mills, A.F.; Transfer, H.; Hall, P.; Jersey, N.; Mills, A.F. Heat Transfer, 2nd ed.; Prentice Hall: Hoboken, NJ, USA, 1999. [Google Scholar]
- Schultz, D.L.; Jones, T.V. Heat Transfer Measurements in Short-Duration Hypersonic Facilities; AGARD-AG-165; Advisory Group for Aerospace Research & Development (AGARD): Paris, France, 1973. [Google Scholar]
- Astarita, T.; Cardone, G.; Calomagno, G.M. Infrared thermography: An optical method in heat transfer and fluid flow visualization. Opt. Lasers Eng. 2006, 44, 261–281. [Google Scholar] [CrossRef]
- Collins, M.; Chana, K.; Povey, T. New Technique for the fabrication of miniature thin film heat flux gauges. Meas. Sci. Technol. 2015, 26, 025303. [Google Scholar] [CrossRef]
- Doorly, J.E.; Oldfield, M.L.G. The theory of advanced multi-layer thin film heat transfer gauges. Int. J. Heat Mass Transf. 1987, 30, 1159–1168. [Google Scholar] [CrossRef]
- Jones, T.V. The thin film heat transfer gauges—A history and new developments. In Proceedings of the 4th national UK Heat Transfer Conference, IMechE Conference Transaction, Manchester, UK, 26–27 September 1995. [Google Scholar]
- Oldfield, M.L.G. Impulse response processing of transient heat transfer gauge signals. ASME J. Turbomach. 2008, 130, 21–23. [Google Scholar] [CrossRef]
- Camilleri, R.; Beard, P.; Howey, D.A.; McCulloch, M.D. Prediction and measurement of the heat transfer coefficient in a direct oil-cooled electrical machine with segmented stator. IEEE Trans. Ind. Electron. 2018, 65, 94–102. [Google Scholar] [CrossRef]
- DSI. Opticool Fluid. Available online: http://dsiventures.com/electronics-cooling/opticool-fluid/ (accessed on 6 March 2015).
- Bell, S. A Beginner’s Guide to Uncertainty of Measurement; Measurement Good Practice Guide No. 11. Iss. 2; National Physical Laboratory: London, UK, 2001. [Google Scholar]



| Fluid Property | Equation | |
|---|---|---|
| Density, [kg/m3] | (8) | |
| Dynamic viscosity, [Pa.s] | (9) | |
| Specific heat capacity, c [J/kgK] | (10) | |
| Thermal conductivity, k [W/mK] | (11) | |
| Prandtl number, Pr | (12) |
| Test No. | Flow Rate [m3/s] ×10−6 | Coolant Inlet Temp. [°C] | Cell Internal Temp. [°C] | Cell Surface Temp. [°C] |
|---|---|---|---|---|
| 1 | 0.196 | 31.30 | 52.25 | 33.27 |
| 2 | 0.371 | 32.27 | 53.08 | 34.67 |
| 3 | 0.576 | 32.54 | 52.89 | 34.97 |
| 4 | 0.818 | 33.06 | 53.48 | 35.48 |
| 5 | 1.062 | 33.73 | 54.5 | 36.15 |
| 6 | 1.348 | 33.97 | 54.14 | 36.32 |
| 7 | 1.676 | 32.73 | 54.38 | 35.81 |
| 8 | 2.017 | 33.57 | 55.78 | 37.22 |
| 9 | 2.422 | 34.06 | 56.23 | 37.56 |
| 10 | 2.857 | 33.85 | 55.84 | 37.26 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Camilleri, R.; Meignen-Viaud, N. Prediction and Measurement of the Heat Transfer Coefficient in Direct, Oil-Cooled Batteries. Energies 2023, 16, 7725. https://doi.org/10.3390/en16237725
Camilleri R, Meignen-Viaud N. Prediction and Measurement of the Heat Transfer Coefficient in Direct, Oil-Cooled Batteries. Energies. 2023; 16(23):7725. https://doi.org/10.3390/en16237725
Chicago/Turabian StyleCamilleri, Robert, and Nolan Meignen-Viaud. 2023. "Prediction and Measurement of the Heat Transfer Coefficient in Direct, Oil-Cooled Batteries" Energies 16, no. 23: 7725. https://doi.org/10.3390/en16237725
APA StyleCamilleri, R., & Meignen-Viaud, N. (2023). Prediction and Measurement of the Heat Transfer Coefficient in Direct, Oil-Cooled Batteries. Energies, 16(23), 7725. https://doi.org/10.3390/en16237725








