Numerical Prediction on In-Cylinder Mixture Formation and Combustion Characteristics for SIDI Engine Fueled with Hydrogen: Effect of Injection Angle and Equivalence Ratio
Abstract
:1. Introduction
2. Materials and Methods
Reference Engine Specification
3. 1D Simulation
4. 3D CFD Simulation
5. Boundary Conditions
6. Results and Discussion
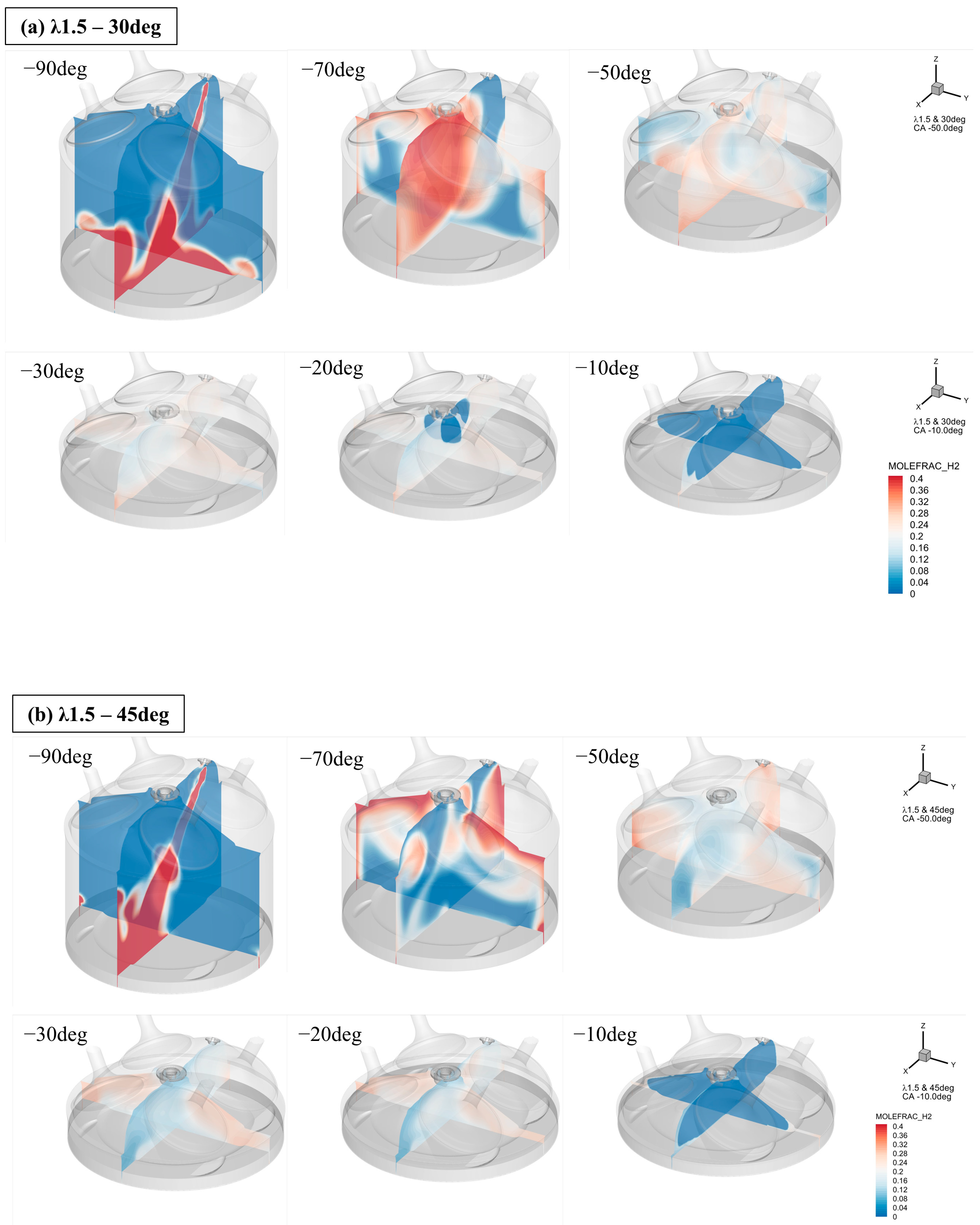
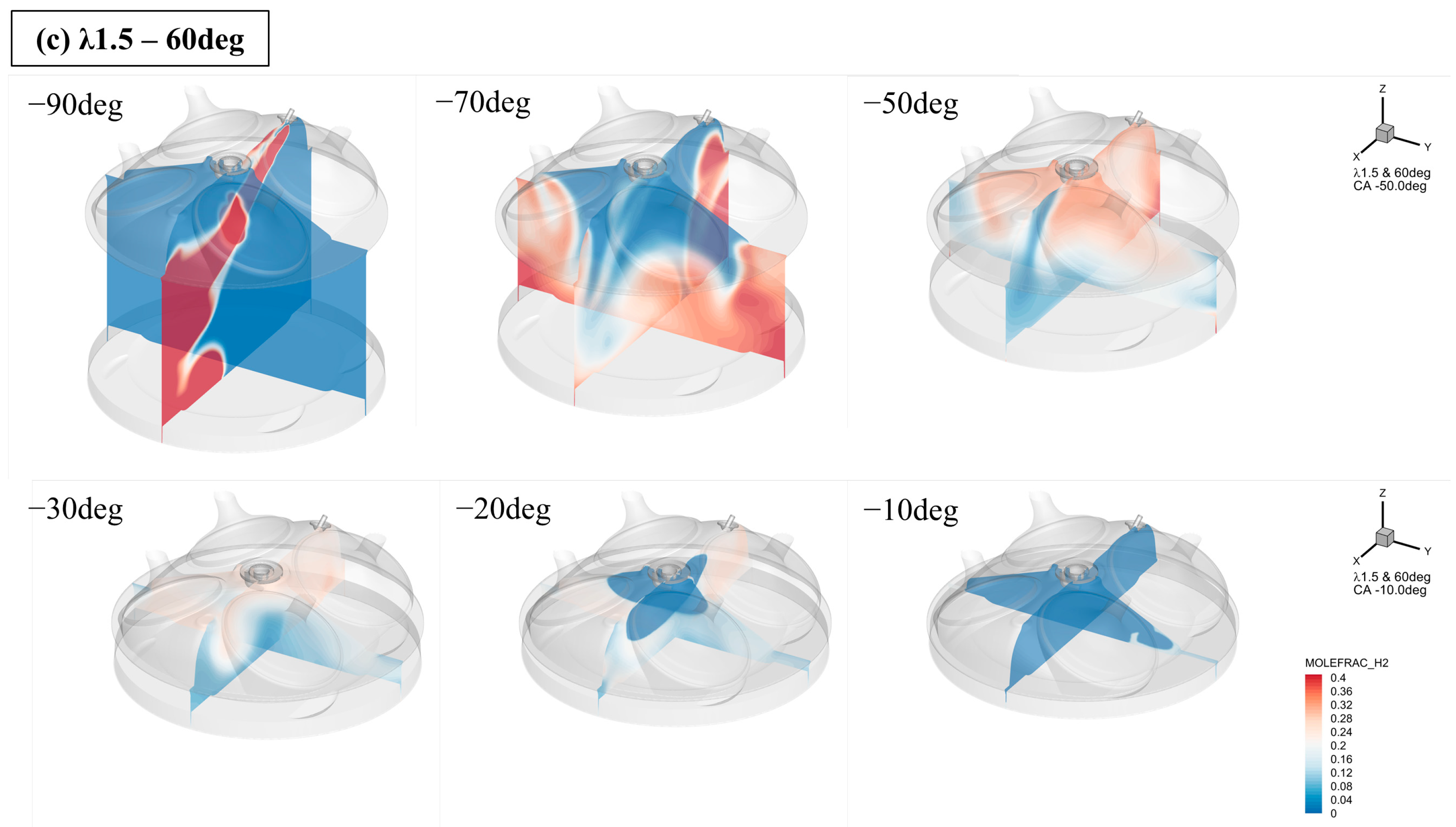
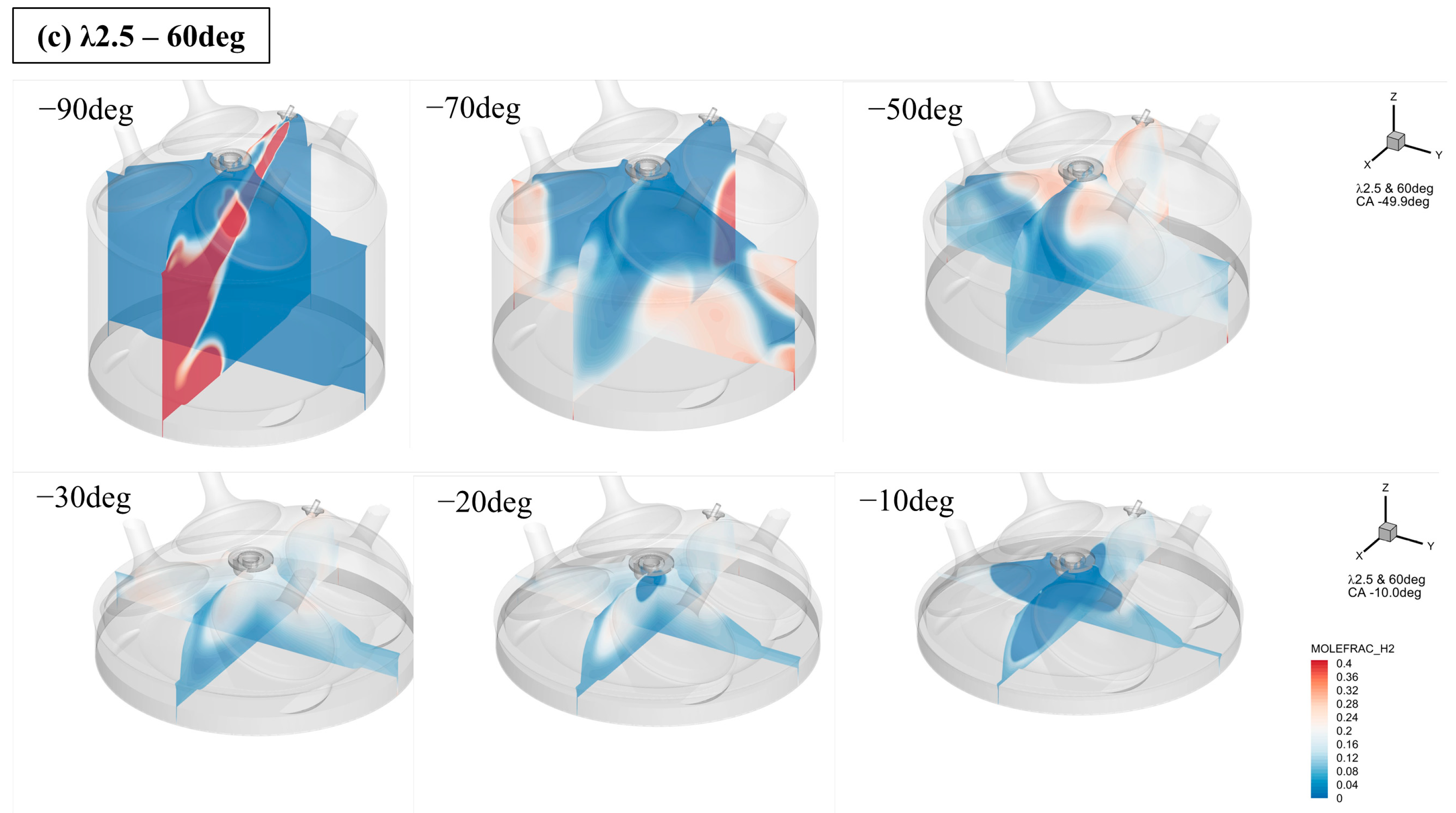
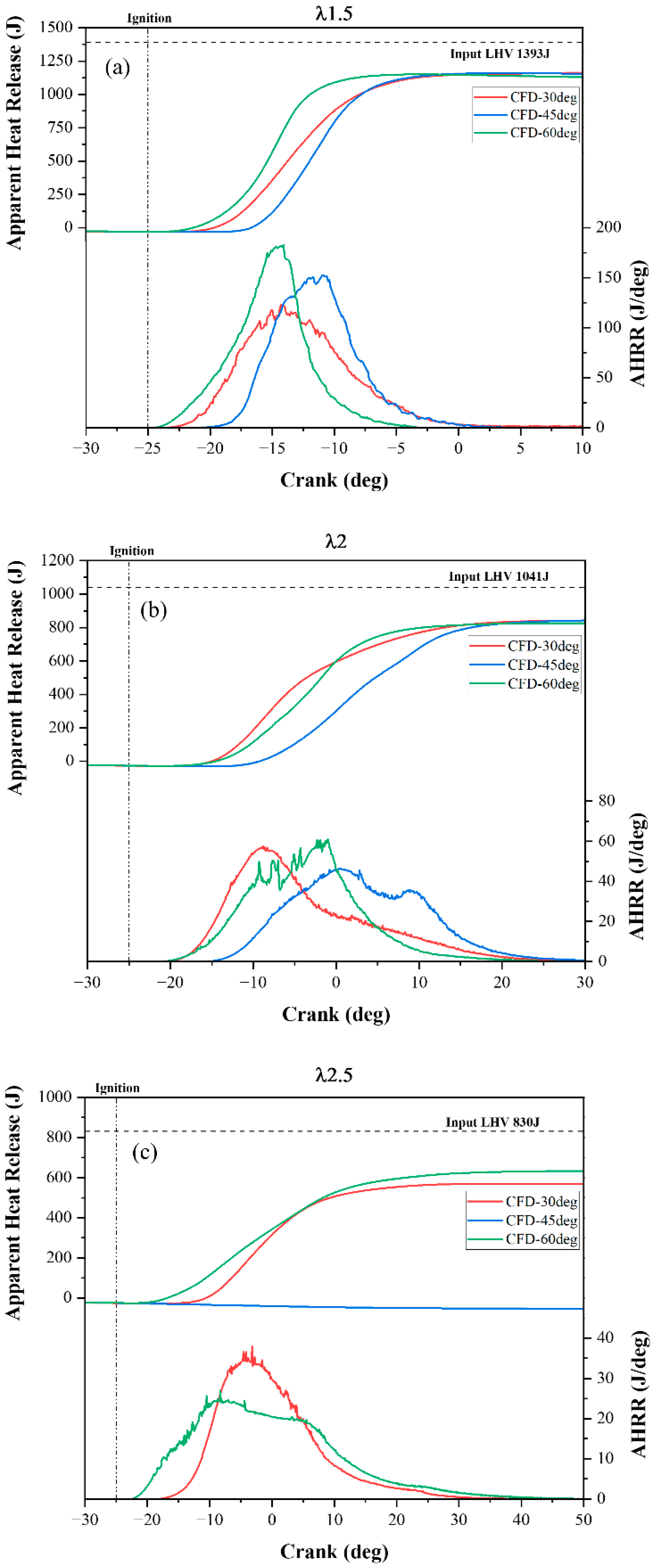
Effect of Injection Angle on Combustion Characteristics
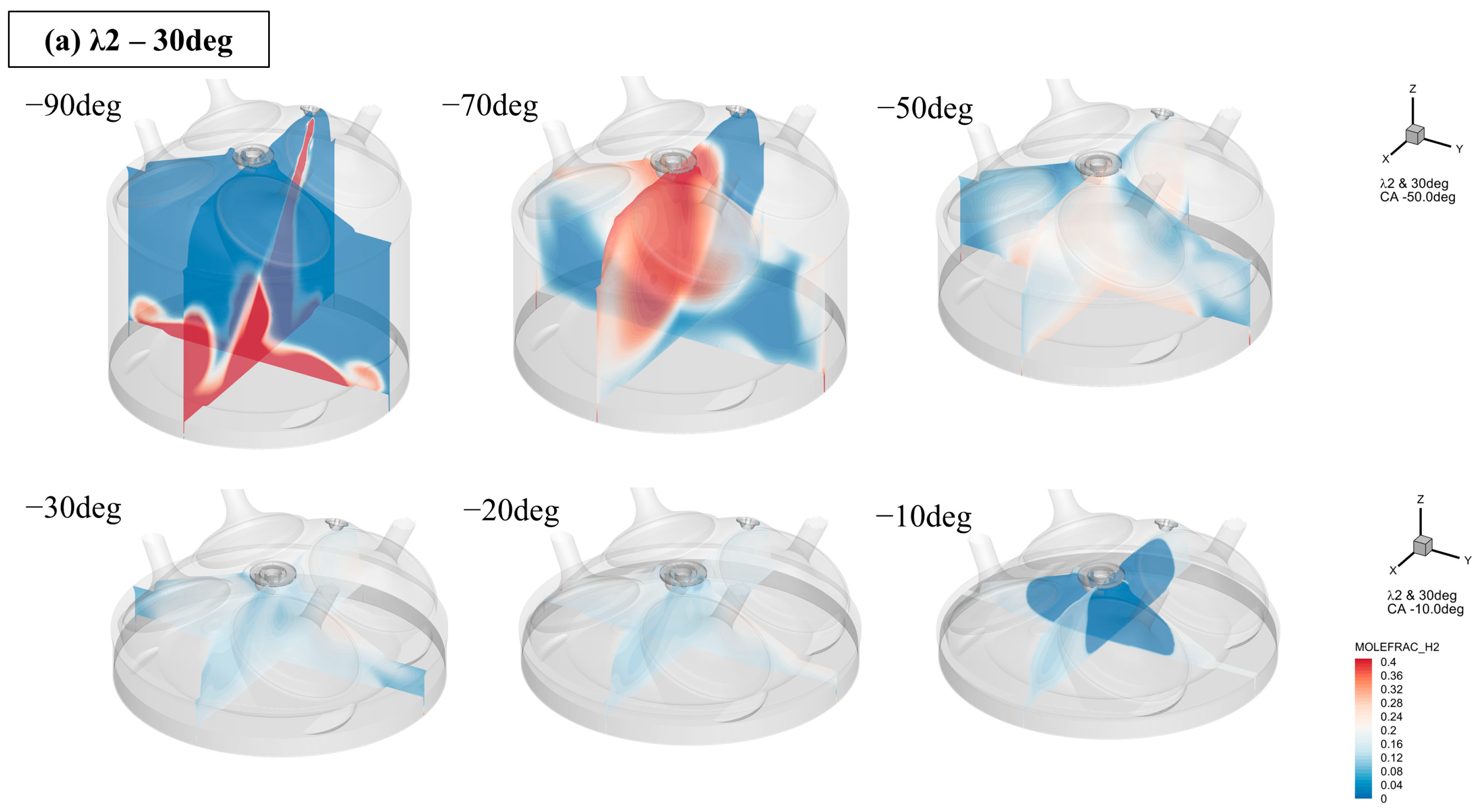
7. Effect of Equivalence Ratio on Combustion Characteristics
8. Conclusions
- The in-cylinder mixer formation and combustion characteristics in each case were investigated by varying the spray angle (30°, 45°, and 60°) and the excess air ratio. Different spray angles showed different combustion characteristics. Although there was no specific trend as the spray angle increased from 30° to 60°, there was a trend as the excess air ratio increased.
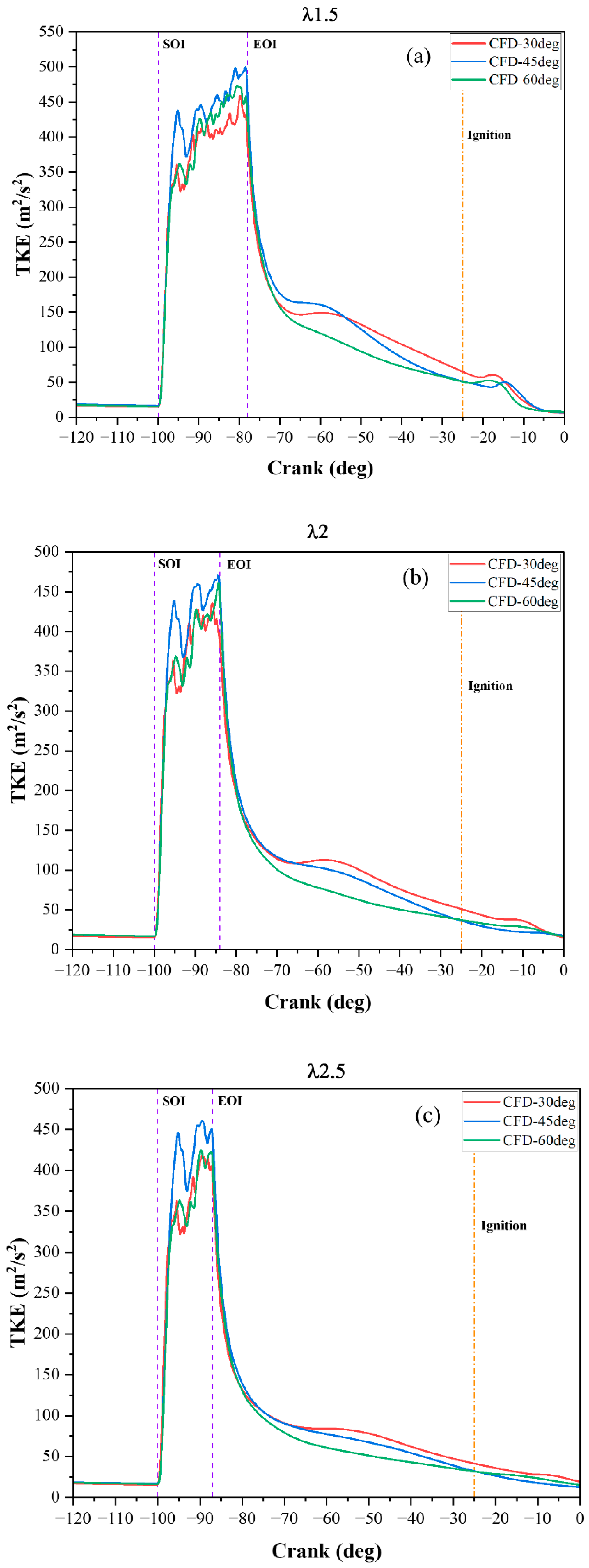
- A spray angle of 45° showed the highest TKE value compared to the other cases for all the equivalence ratio conditions. The cylinder achieved high turbulence intensity, but misfires occurred under λ2.5 conditions. This confirms that the turbulence intensity and spray behavior as functions of the spray angle have a considerable impact on the combustion characteristics under lean conditions.
- At spray angles of 30° and 45°, the SOI spray impinged on the cylinder liner and piston crown, respectively, and separated into two streams, each forming two plumes. The formed plumes could interact with each other by forming a vortex, especially around the spark plug, forming lean or rich regions depending on the spray angle.
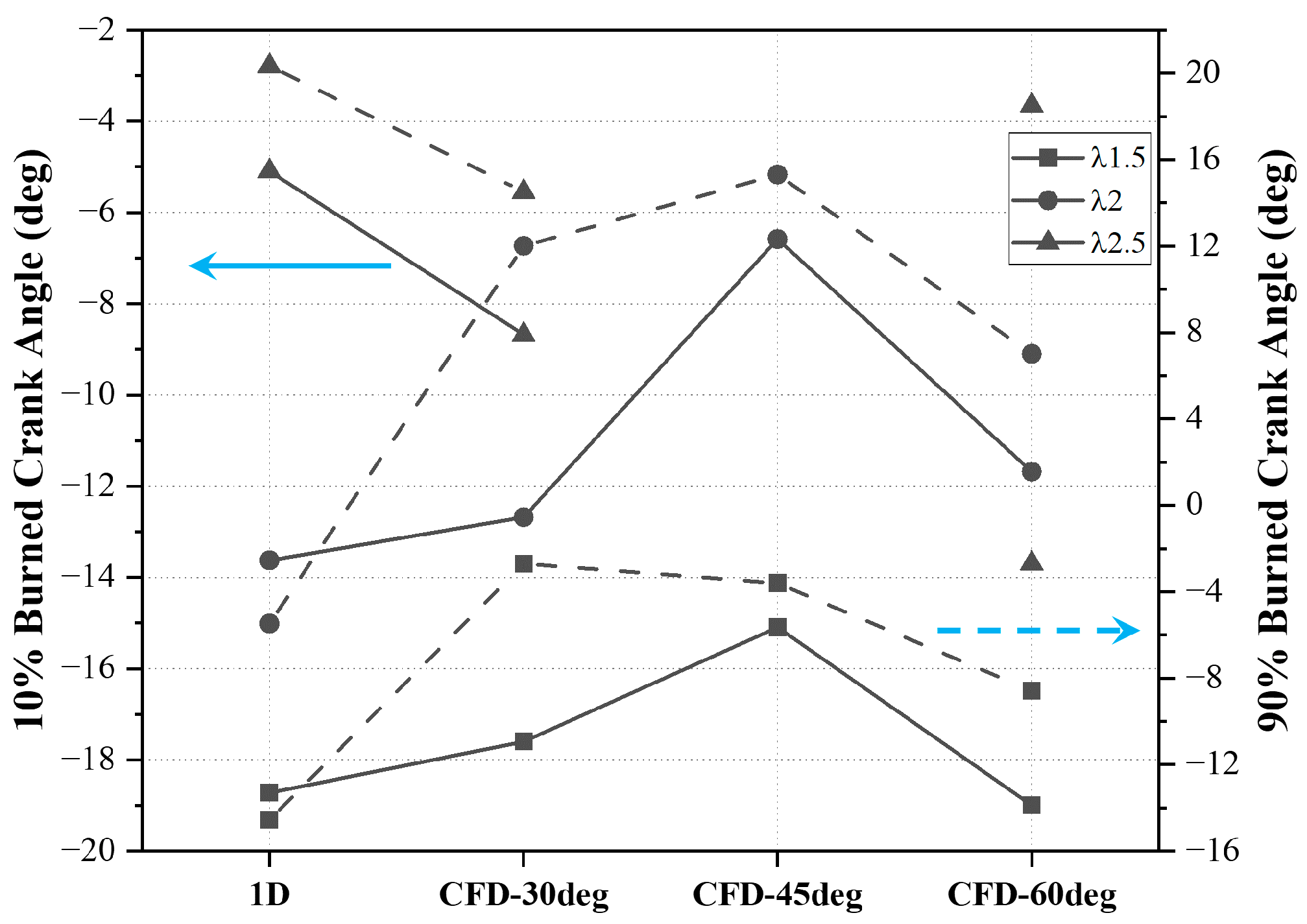
- Lean and rich regions formed around the spark plug, resulting in differences in the initial flame development rate for each spray angle. Spray angles of 30° and 60° showed a similar trend for MFB 10%, but at 45°, misfires were detected, more so in lean conditions, and misfires occurred in λ2.5.
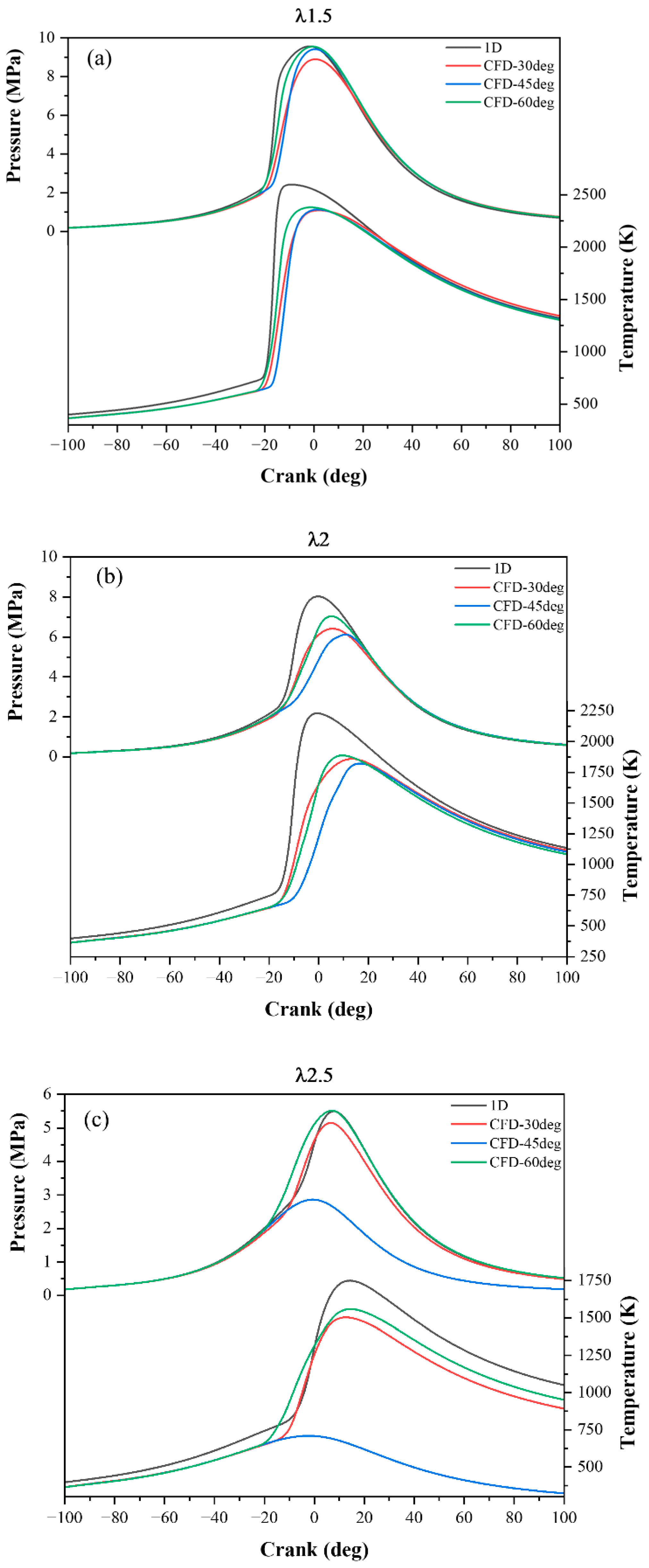
- After the initial flame development, the flame propagation was the fastest at a spray angle of 60° and was significantly retarded at other spray angles. In particular, the initial flame development angle was perceived at a 45° spray angle; however, the flame termination stage of MFB90 exhibited a faster burning rate than the perceived initial flame development.
- Depending on the spray angle, the combustion pressure and temperature were also affected by the differences in the initial flame development and flame propagation velocity, resulting in different combustion characteristics depending on the mixer formation conditions in the cylinder. For all excess air ratios, the combustion pressure and temperature were the highest at a spray angle of 60°.
- As the excess air ratio increased, the NOx emissions decreased significantly as the combustion pressure and temperature decreased, especially at λ2.5, which showed low NOx emissions of 28.6 ppm at a 30-degree spray angle and 160.96 ppm at a 60-degree spray angle. It is believed that the NOx emissions at a 60-degree spray angle were high because the combustion pressure and temperature were relatively high.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| laminar flame velocity | |
| maximum laminar velocity | |
| laminar velocity roll-off value | |
| the equivalence ratio | |
| equivalence ratio at maximum velocity | |
| unburned gas temperature | |
| 298 K | |
| pressure | |
| 101,325 Pa | |
| temperature exponent | |
| pressure exponent | |
| residual mass fraction in the unburned zone | |
| Me | trapped mass |
| burned mass | |
| turbulent flame speed multiplier | |
| Taylor length scale multiplier | |
| flame kernel growth multiplier | |
| turbulent flame speed | |
| flame radius | |
| surface area at the flame front | |
| unburned density | |
| represents time | |
| Taylor microscale length or excess air ratio | |
| integral length scale | |
| turbulent intensity | |
| turbulent Reynolds number | |
| unburned zone dynamic viscosity |
References
- BP. Statistical Review of World Energy. 2022. Available online: https://www.bp.com/en/global/corporate/energy-economics/statistical-review-of-world-energy.html (accessed on 28 June 2022).
- Gómez, D.R.; Watterson, J.D.; Americano, B.B.; Ha, C.; Marland, G.; Matsika, E.; Namayanga, L.N.; Osman-Elasha, B.; Saka, J.D.K.; Treanton, K.; et al. Guidelines for National Greenhouse Gas Inventories; Intergovernmental Panel on Climate Change (IPCC): Geneva, Switzerland, 2006. [Google Scholar]
- Andres, R.J.; Boden, T.A.; Bréon, F.M.; Ciais, P.; Davis, S.; Erickson, D.; Gregg, J.S.; Jacobson, A.; Marland, G.; Miller, J.; et al. A synthesis of carbon dioxide emissions from fossil-fuel combustion. Biogeosciences 2012, 9, 1845–1871. [Google Scholar] [CrossRef]
- Falkner, R. The Paris Agreement and the new logic of international climate politics. Int. Aff. 2016, 92, 1107–1125. [Google Scholar] [CrossRef]
- Schleussner, C.F.; Rogelj, J.; Schaeffer, M.; Lissner, T.; Licker, R.; Fischer, E.M.; Knutti, R.; Levermann, A.; Frieler, K.; Hare, W. Science and policy characteristics of the Paris Agreement temperature goal. Nat. Clim. Change 2016, 6, 827–835. [Google Scholar] [CrossRef]
- Chen, L.; Msigwa, G.; Yang, M.; Osman, A.I.; Fawzy, S.; Rooney, D.W.; Yap, P.S. Strategies to achieve a carbon neutral society: A review. Environ. Chem. Lett. 2022, 20, 2277–2310. [Google Scholar] [CrossRef]
- IEA. Transport Sector CO2 Emissions by Mode in the Sustainable Development Scenario; IEA: Paris, France, 2019; pp. 2000–2030. [Google Scholar]
- Abdel-Rahman, A. On the emissions from internal-combustion engines: A review. Int. J. Energy Res. 1998, 22, 483–513. [Google Scholar] [CrossRef]
- Alagumalai, A. Internal combustion engines: Progress and prospects. Renew. Sustain. Energy Rev. 2014, 38, 561–571. [Google Scholar] [CrossRef]
- Afanaseva, O.; Bezyukov, O.; Pervukhin, D. Perspective Technical Solutions for the Cylinder-Piston Group Vibrations Reduction; Taylor& Francis Group: Abingdon, UK, 2018; pp. 293–300. [Google Scholar]
- Afanaseva, O.; Bezyukov, O.; Pervukhin, D.; Tukeev, D. Experimental Study Results Processing Method for the Marine Diesel Engines Vibration Activity Caused by the Cylinder-Piston Group Operations. Inventions 2023, 8, 71. [Google Scholar] [CrossRef]
- Ji, C.; Wang, S. Effect of hydrogen addition on combustion and emissions performance of a spark ignition gasoline engine at lean conditions. Int. J. Hydrogen Energy 2009, 34, 7823–7834. [Google Scholar] [CrossRef]
- Kim, J.; Chun, K.M.; Song, S. Thermal efficiency improvement of a range extender based on the T-GDI engine for a medium-size electric bus using on-board gasoline fuel reforming with steam addition. Fuel 2021, 300, 120965. [Google Scholar] [CrossRef]
- Yu, X.; Li, G.; Du, Y.; Guo, Z.; Shang, Z.; He, F.; Shen, Q.; Li, D.; Li, Y. A comparative study on effects of homogeneous or stratified hydrogen on combustion and emissions of a gasoline/hydrogen SI engine. Int. J. Hydrogen Energy 2019, 44, 25974–25984. [Google Scholar] [CrossRef]
- Cho, J.; Song, S. Prediction of hydrogen-added combustion process in T-GDI engine using artificial neural network. Appl. Therm. Eng. 2020, 181, 115974. [Google Scholar] [CrossRef]
- Park, J.; Cha, H.; Song, S.; Chun, K.M. A numerical study of a methane-fueled gas engine generator with addition of hydrogen using cycle simulation and DOE method. Int. J. Hydrogen Energy 2011, 36, 5153–5162. [Google Scholar] [CrossRef]
- Zhang, R.; Chen, L.; Pan, J.; Wei, H.; Zhou, L.; Liu, C. Effects of direct-injected hydrogen addition on methane combustion performance in an optical SI engine with high compression-ratio. Int. J. Hydrogen Energy 2020, 45, 3284–3293. [Google Scholar] [CrossRef]
- Zhen, X.; Li, X.; Wang, Y.; Liu, D.; Tian, Z. Comparative study on combustion and emission characteristics of methanol/hydrogen, ethanol/hydrogen and methane/hydrogen blends in high compression ratio SI engine. Fuel 2020, 267, 117193. [Google Scholar] [CrossRef]
- Seelam, N.; Gugulothu, S.K.; Reddy, R.V.; Bhasker, B.; Kumar Panda, J. Exploration of engine characteristics in a CRDI diesel engine enriched with hydrogen in dual fuel mode using toroidal combustion chamber. Int. J. Hydrogen Energy 2022, 47, 13157–13167. [Google Scholar] [CrossRef]
- Frost, J.; Tall, A.; Sheriff, A.M.; Schönborn, A.; Hellier, P. An experimental and modelling study of dual fuel aqueous ammonia and diesel combustion in a single cylinder compression ignition engine. Int. J. Hydrogen Energy 2021, 46, 35495–35510. [Google Scholar] [CrossRef]
- Li, H.; Karim, G.A. Exhaust emissions from an SI engine operating on gaseous fuel mixtures containing hydrogen. Int. J. Hydrogen Energy 2005, 30, 1491–1499. [Google Scholar] [CrossRef]
- Chaichan, M.T. EGR effects on hydrogen engines performance and emissions. Int. J. Sci. Eng. Res. 2016, 7, 80–90. [Google Scholar]
- Dhyani, V.; Subramanian, K.A. Control of backfire and NOx emission reduction in a hydrogen fueled multi-cylinder spark ignition engine using cooled EGR and water injection strategies. Int. J. Hydrogen Energy 2019, 44, 6287–6298. [Google Scholar] [CrossRef]
- Xin, G.; Ji, C.; Wang, S.; Meng, H.; Chang, K.; Yang, J. Effect of ammonia addition on combustion and emission characteristics of hydrogen-fueled engine under lean-burn condition. Int. J. Hydrogen Energy 2022, 47, 9762–9774. [Google Scholar] [CrossRef]
- Chai, W.S.; Bao, Y.; Jin, P.; Tang, G.; Zhou, L. A review on ammonia, ammonia-hydrogen and ammonia-methane fuels. Renew. Sustain. Energy Rev. 2021, 147, 111254. [Google Scholar] [CrossRef]
- Kiesgen, G.; Klüting, M.; Bock, C.; Fischer, H. The New 12-Cylinder Hydrogen Engine in the 7 Series-The H2 ICE Age Has Begun. SAE Tech. Pap. Ser. 2006, 1, 0431. [Google Scholar]
- Tang, X.; Kabat, D.M.; Natkin, R.J.; Stockhausen, W.F. Ford P2000 Hydrogen Engine Dynamometer Development. SAE Tech. Pap. Ser. 2022, 111, 631–642. [Google Scholar]
- Jaura, A.K.; Ortmann, W.; Stuntz, R.; Natkin, B.; Grabowski, T. Ford’s H2RV-An Industry First HEV Propelled with a H2 Fueled Engine-A Fuel Efficient and Clean Solution for Sustainable Mobility. SAE Tech. Pap. Ser. 2004, 1, 0058. [Google Scholar]
- Lee, S.; Kim, G.; Bae, C. Behavior of hydrogen hollow-cone spray depending on the ambient pressure. Int. J. Hydrogen Energy 2021, 46, 4538–4554. [Google Scholar] [CrossRef]
- Gamma Technologies. Engine_Performance Application Manual; Version 2022; Gamma Technologies: Westmont, IL, USA, 2022. [Google Scholar]
- Han, Z.; Reitz, R.D. Turbulence modeling of internal combustion engines using RNG κ-ε models. Combust. Sci. Technol. 1995, 106, 267–295. [Google Scholar] [CrossRef]
- Yakhot, V.; Orszag, S.A. Renormalization group analysis of turbulence. I. Basic theory. J. Sci. Comput. 1986, 1, 3–51. [Google Scholar] [CrossRef]
- Lucchini, T.; D’Errico, G.; Fiocco, M. Multi-Dimensional Modeling of Gas Exchange and Fuel-Air Mixing Processes in a Direct-Injection, Gas Fueled Engine. SAE Tech. Pap. Ser. 2011, 24, 0036. [Google Scholar]
- Convergent Science. CONVERGE 3.0 Manual; Version 3.0; Convergent Science: Madison, WI, USA, 2021. [Google Scholar]

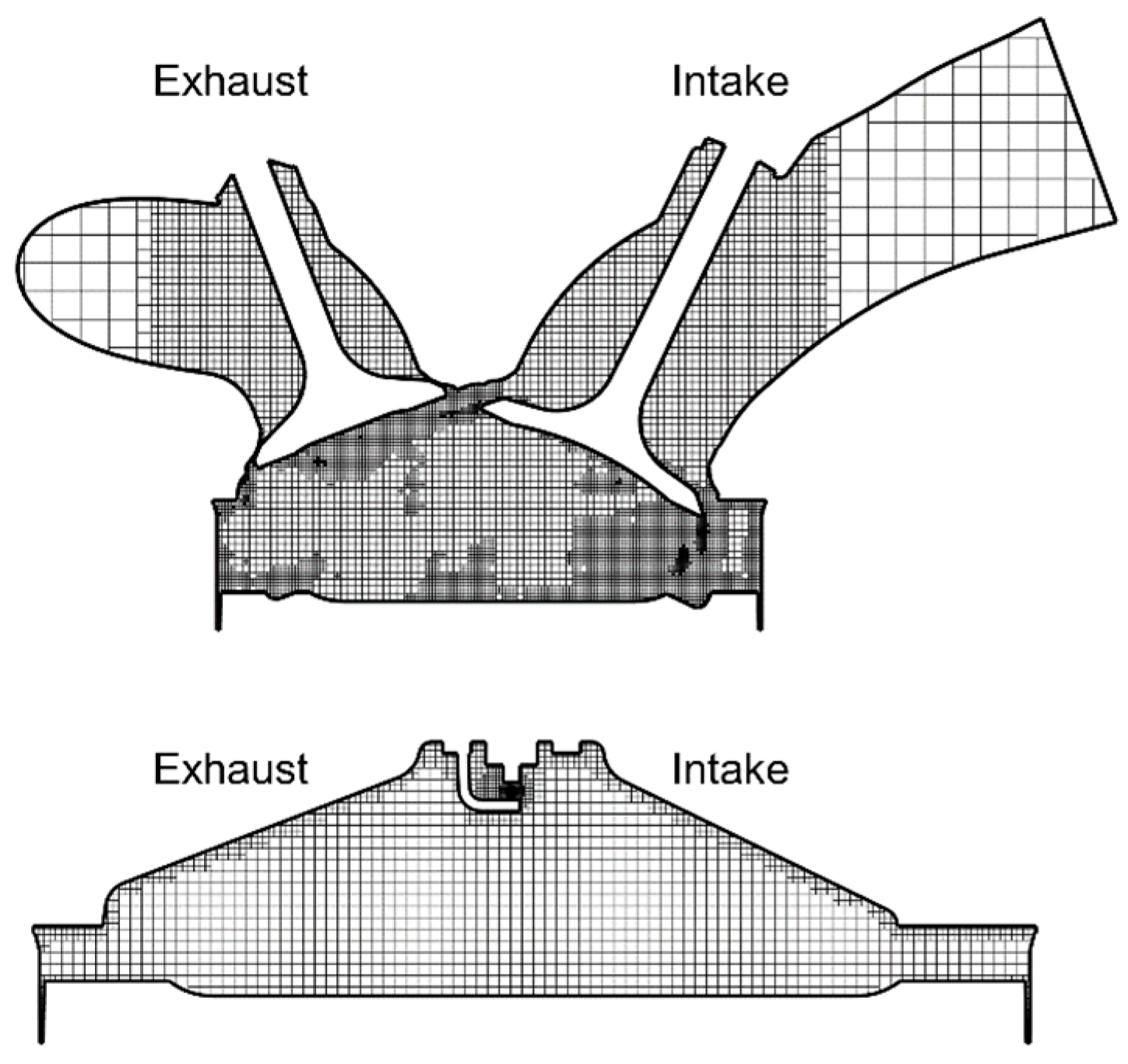





| Parameter | Specification |
|---|---|
| Virtual engine type | 4cylinder DI-SI engine |
| Bore × Stroke [mm] | 86 × 86 |
| Displacement [cc] | 1998 |
| Compression ratio | 10:1 |
| Intake valve timing | 2aTDC—70aBDC |
| Exhaust valve timing | 13bBDC—38aTDC |
| RPM | 2000 |
| Start of injection (SOI) | −100 aTDC |
| Injection pressure [MPa] | 2.5 |
| Boosting system | Turbocharger |
| Boost pressure [MPa] | 0.125 |
| Excess air ratio [λ] | 1.5–2.5 |
| Spark timing (ST) | −25 aTDC |
| Region | Strategy | Embedding Level |
|---|---|---|
| In-cylinder | AMR (velocity, temperature) Fixed embedding (cylinder) | 3 2 |
| Injector | Fixed embedding (cylinder) | 3 |
| Spark plug | Fixed embedding (sphere) | 5, 6 |
| Piston head | Fixed embedding (boundary) | 3 |
| Piston skirt | Fixed embedding (boundary) | 3 |
| Cylinder head | Fixed embedding (boundary) | 3 |
| Parameter | 1D | 3D-CFD |
|---|---|---|
| Inlet pressure | 1.25 bar | |
| Inlet temperature | 300 K | |
| Outlet pressure | 1.01325 bar | |
| Outlet temperature | 800 K | |
| Cylinder temperature | 800 K | |
| Liner temperature | 350 K | |
| Head temperature | 350 K | |
| Fuel temperature | 300 K | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Oh, S.; Park, J. Numerical Prediction on In-Cylinder Mixture Formation and Combustion Characteristics for SIDI Engine Fueled with Hydrogen: Effect of Injection Angle and Equivalence Ratio. Energies 2023, 16, 7509. https://doi.org/10.3390/en16227509
Oh S, Park J. Numerical Prediction on In-Cylinder Mixture Formation and Combustion Characteristics for SIDI Engine Fueled with Hydrogen: Effect of Injection Angle and Equivalence Ratio. Energies. 2023; 16(22):7509. https://doi.org/10.3390/en16227509
Chicago/Turabian StyleOh, Sehyun, and Jungsoo Park. 2023. "Numerical Prediction on In-Cylinder Mixture Formation and Combustion Characteristics for SIDI Engine Fueled with Hydrogen: Effect of Injection Angle and Equivalence Ratio" Energies 16, no. 22: 7509. https://doi.org/10.3390/en16227509
APA StyleOh, S., & Park, J. (2023). Numerical Prediction on In-Cylinder Mixture Formation and Combustion Characteristics for SIDI Engine Fueled with Hydrogen: Effect of Injection Angle and Equivalence Ratio. Energies, 16(22), 7509. https://doi.org/10.3390/en16227509






