A Finite Element Analysis Model-Based Study on the Effect of the Frame on Membrane Stresses in Proton Exchange Membrane Fuel Cells
Abstract
:1. Introduction
2. Finite Element Simulations
2.1. Model and Assumption
2.2. Material Properties
3. Finite Element Analyses Results
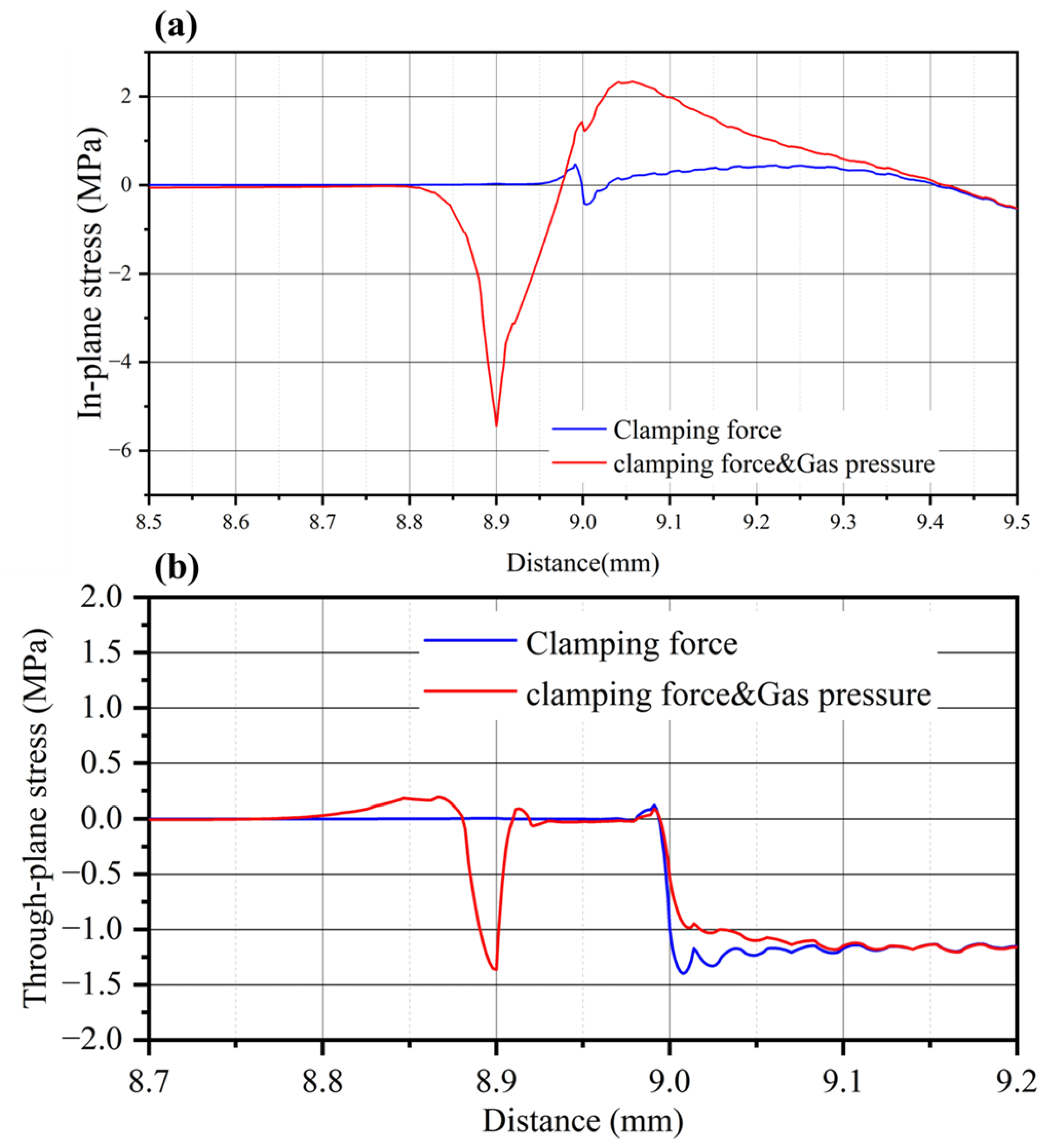
3.1. Stress–Strain Response on Membrane
3.2. Analysis of Influencing Factors
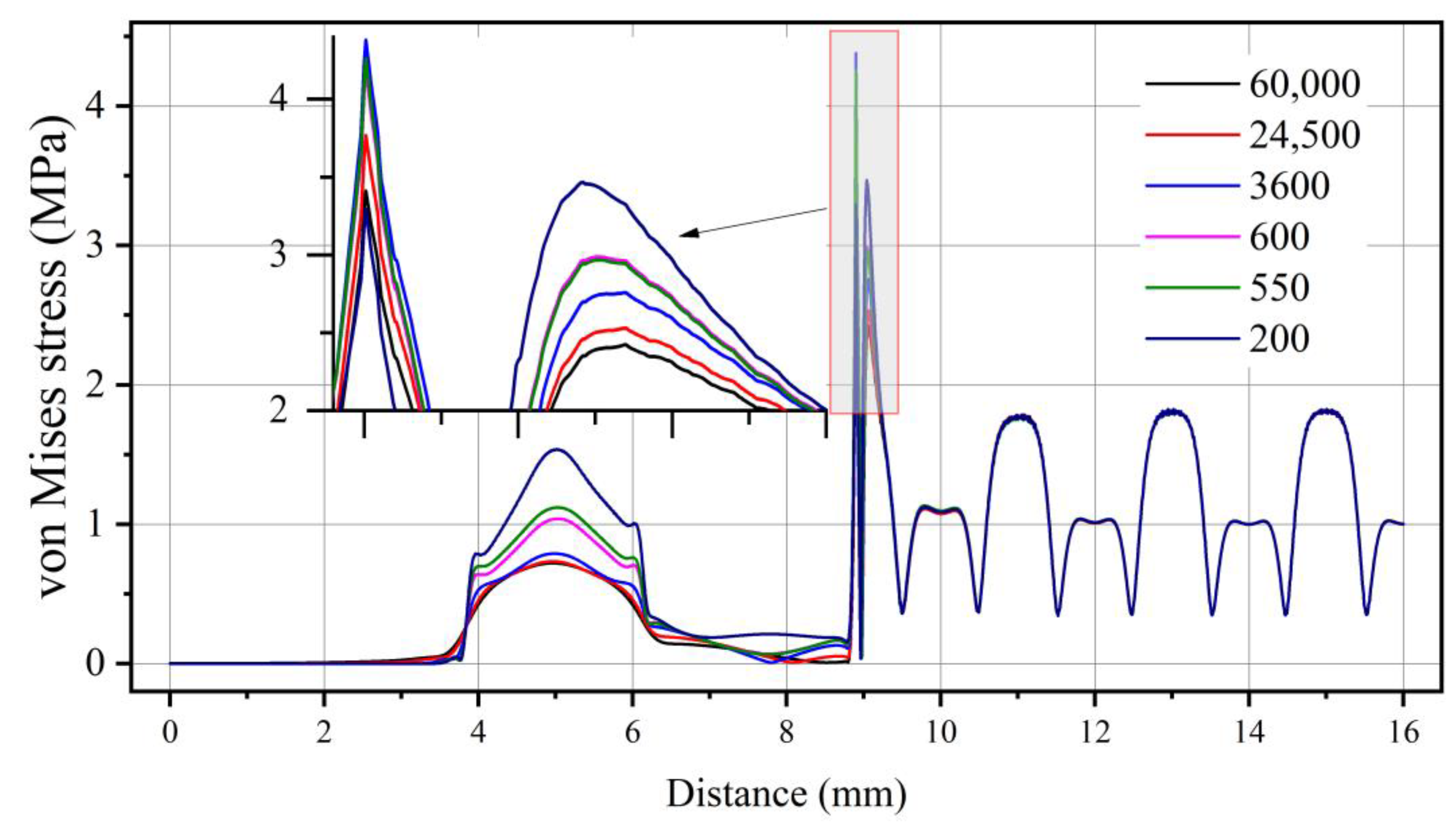
3.2.1. Frame Material
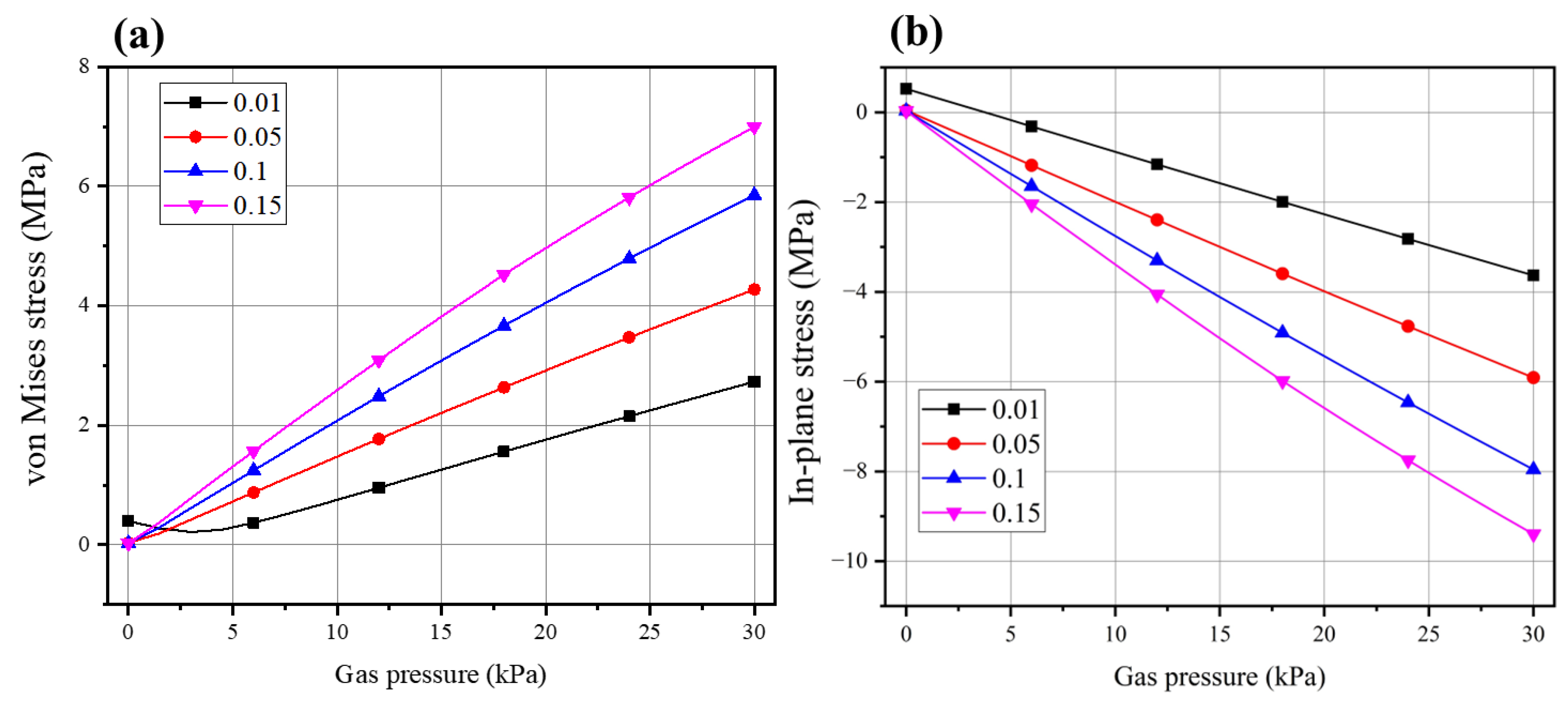
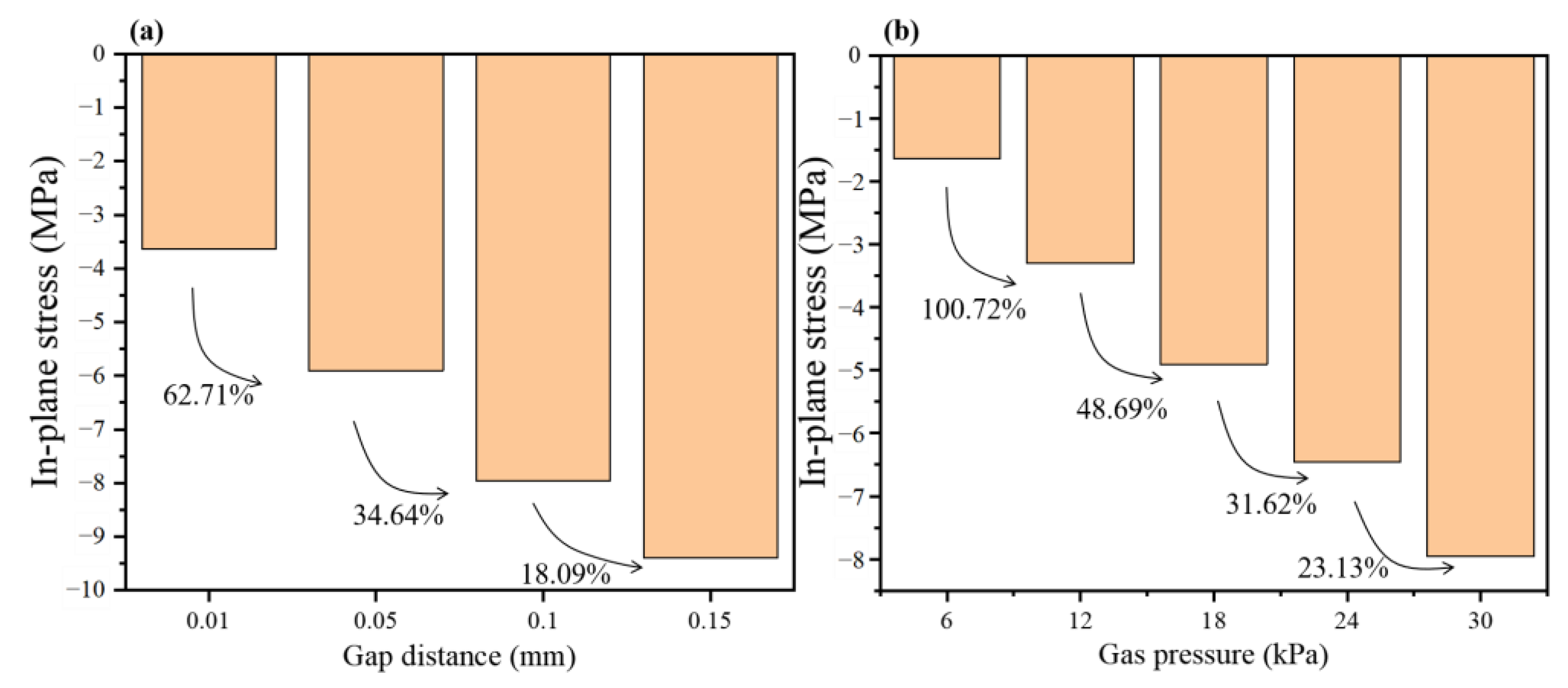
3.2.2. Gas Pressure and Gap Width
3.3. Double-Layer Frame Structure
- Frequent gas pressure changes and large gas pressure differences between the anode and cathode may have caused early damage to the membrane at the gap.
- The higher the modulus of elasticity, the stronger the ability to bear the installation clamping force, the smaller the stress of the membrane under the seal and the smaller the degree of deflection of the frame caused by the air pressure difference. The greater the rigidity of frame material, the more likely it is to cause stress concentration at the end of the frame.
- As the air pressure difference increased, the length of the gap at the joint also increased and the stress on the membrane will increase accordingly. The stress on the membrane at the joint is more sensitive to the air pressure difference. By changing the frame material, reducing the length of the transfer area can reduce the impact of the pressure difference to a certain extent. However, this does not fundamentally solve the problem. Therefore, appropriate control strategies should be adopted to avoid frequent changes in air pressure and large air pressure difference between anode and cathode during fuel cell operation. Measures should be taken to eliminate the gaps in the joint area to avoid direct exposure of the membrane to the harsh environment of fuel cell operation.
- The double-layer frame is a better structure compared to the single-layer frame. Modifying the frame based on existing processing technology is a structure that can achieve better performance. The double-layer frame eliminates the gap at the joint and can effectively reduce the impact of pressure difference. The stress level on the membrane at the frame is smaller than the active area and the distribution is more even.
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Jewell, J.; McCollum, D.; Emmerling, J.; Bertram, C.; Gernaat, D.; Krey, V.; Paroussos, L.; Berger, L.; Fragkiadakis, K.; Keppo, I.; et al. Limited emission reductions from fuel subsidy removal except in energy-exporting regions. Nature 2018, 554, 229–233. [Google Scholar] [CrossRef]
- Razi, F.; Dincer, I. Renewable energy development and hydrogen economy in MENA region: A review. Renew. Sustain. Energy Rev. 2022, 168, 112763. [Google Scholar] [CrossRef]
- Squadrito, G.; Maggio, G.; Nicita, A. The green hydrogen revolution. Renew. Energy 2023, 216, 119041. [Google Scholar]
- Sun, C.; Negro, E.; Vezzù, K.; Pagot, G.; Cavinato, G.; Nale, A.; Herve Bang, Y.; Di Noto, V. Hybrid inorganic-organic proton-conducting membranes based on SPEEK doped with WO3 nanoparticles for application in vanadium redox flow batteries. Electrochim. Acta 2019, 309, 311–325. [Google Scholar]
- Jiao, K.; Xuan, J.; Du, Q.; Bao, Z.; Xie, B.; Wang, B.; Zhao, Y.; Fan, L.; Wang, H.; Hou, Z.; et al. Designing the next generation of proton-exchange membrane fuel cells. Nature 2021, 595, 361–369. [Google Scholar]
- Wu, S.; Salmon, N.; Li, M.; Bañares-Alcántara, R.; Tsang, S. Energy Decarbonization via Green H2 or NH3? ACS Energy Lett. 2022, 7, 1021–1033. [Google Scholar] [CrossRef]
- Li, J.; Fang, C.; Xu, L. Current status and trends of the research and development for fuel cell vehicles. J. Automot. Saf. Energy 2014, 5, 17. [Google Scholar]
- Thompson, S.; Wilson, A.; Zelenay, P.; Myers, D.; More, K.; Neyerlin, K.; Papageorgopoulos, D. ElectroCat: DOE’s approach to PGM-free catalyst and electrode R&D. Solid State Ion. 2018, 319, 68–76. [Google Scholar]
- Mustain, W.; Chatenet, M.; Page, M.; Kim, Y.S. Durability challenges of anion exchange membrane fuel cells. Energy Environ. Sci. 2020, 13, 2805–2838. [Google Scholar]
- Yan, S.; Yang, M.; Sun, C.; Xu, S. Liquid Water Characteristics in the Compressed Gradient Porosity Gas Diffusion Layer of Proton Exchange Membrane Fuel Cells Using the Lattice Boltzmann Method. Energies 2023, 16, 6010. [Google Scholar] [CrossRef]
- Zhang, S.; Yuan, X.; Wang, H.; Mérida, W.; Zhu, H.; Shen, J.; Wu, S.; Zhang, J. A review of accelerated stress tests of MEA durability in PEM fuel cells. Int. J. Hydrogen Energy 2009, 34, 388–404. [Google Scholar] [CrossRef]
- Stariha, S.; Macauley, N.; Sneed, B.; Langlois, D.; More, K.; Mukundan, R.; Borup, R. Recent advances in catalyst accelerated stress tests for polymer electrolyte membrane fuel cells. J. Electrochem. Soc. 2018, 165, F492. [Google Scholar] [CrossRef]
- Pahon, E.; Hissel, D.; Yousfi-Steiner, N. A review of accelerated stress tests dedicated to proton exchange membrane fuel cells—Part I: Fuel cell component level. J. Power Sources 2022, 546, 231895. [Google Scholar]
- Lim, C.; Ghassemzadeh, L.; Van Hove, F.; Lauritzen, M.; Kolodziej, J.; Wang, G.G.; Holdcroft, S.; Kjeang, E. Membrane degradation during combined chemical and mechanical accelerated stress testing of polymer electrolyte fuel cells. J. Power Sources 2014, 257, 102–110. [Google Scholar] [CrossRef]
- Yang, W.; Guo, H.; Niu, F.; Wang, B.; Huang, B.; Niu, S.; Liu, J.; Yang, S.; Yang, Y. A novel strategy for accelerating degradation of proton exchange membranes in fuel cell. Renew. Energy 2023, 213, 38–46. [Google Scholar] [CrossRef]
- Shimizu, R.; Tsuji, J.; Sato, N.; Takano, J.; Itami, S.; Kusakabe, M.; Miyatake, K.; Iiyama, A.; Uchida, M. Durability and degradation analysis of hydrocarbon ionomer membranes in polymer electrolyte fuel cells accelerated stress evaluation. J. Power Sources 2017, 367, 63–71. [Google Scholar] [CrossRef]
- Crum, M.; Liu, W. Effective Testing Matrix for Studying Membrane Durability in PEM Fuel Cells: Part 2. Mechanical Durability and Combined Mechanical and Chemical Durability. ECS Trans. 2006, 3, 541. [Google Scholar] [CrossRef]
- Zhang, Y.; Gao, Z.; Wei, L.; Su, J. Improving the Performance of PEM Fuel Cells: Form a Patterned Hydrophobic Catalyst Layer. J. Electrochem. Soc. 2023, 170, 054508. [Google Scholar] [CrossRef]
- Qiu, D.; Peng, L.; Liang, P.; Yi, P.; Lai, X. Mechanical degradation of proton exchange membrane along the MEA frame in proton exchange membrane fuel cells. Energy 2018, 165, 210–222. [Google Scholar] [CrossRef]
- Kusoglu, A.; Karlsson, A.M.; Santare, M.H.; Cleghorn, S.; Johnson, W.B. Mechanical behavior of fuel cell membranes under humidity cycles and effect of swelling anisotropy on the fatigue stresses. J. Power Sources 2007, 170, 345–358. [Google Scholar] [CrossRef]
- Li, B.; Kim, Y.S.; Mukundan, R.; Wilson, M.S.; Welch, C.; Fenton, J.; Borup, R.L. Mixed Hydrocarbon/Fluoropolymer Membrane/Ionomer MEAs for Durablity Studies. ECS Trans. 2010, 33, 913. [Google Scholar] [CrossRef]
- Bates, A.; Mukherjee, S.; Hwang, S.; Lee, S.C.; Kwon, O.; Choi, G.H.; Park, S. Simulation and experimental analysis of the clamping pressure distribution in a PEM fuel cell stack. Int. J. Hydrogen Energy 2013, 38, 6481–6493. [Google Scholar] [CrossRef]
- Alizadeh, E.; Barzegari, M.M.; Momenifar, M.; Ghadimi, M.; Saadat, S.H.M. Investigation of contact pressure distribution over the active area of PEM fuel cell stack. Int. J. Hydrogen Energy 2016, 41, 3062–3071. [Google Scholar] [CrossRef]
- Kusoglu, A.; Santare, M.H.; Karlsson, A.M. Aspects of fatigue failure mechanisms in polymer fuel cell membranes. J. Polym. Sci. Part B Polym. Phys. 2011, 49, 1506–1517. [Google Scholar] [CrossRef]
- Khattra, N.S.; Karlsson, A.M.; Santare, M.H.; Walsh, P.; Busby, F.C. Effect of time-dependent material properties on the mechanical behavior of PFSA membranes subjected to humidity cycling. J. Power Sources 2012, 214, 365–376. [Google Scholar] [CrossRef]
- Liu, D.; Peng, L.; Lai, X. Effect of assembly error of bipolar plate on the contact pressure distribution and stress failure of membrane electrode assembly in proton exchange membrane fuel cell. J. Power Sources 2010, 195, 4213–4221. [Google Scholar] [CrossRef]
- Liang, P.; Qiu, D.; Peng, L.; Yi, P.; Lai, X.; Ni, J. Structure failure of the sealing in the assembly process for proton exchange membrane fuel cells. Int. J. Hydrogen Energy 2017, 42, 10217–10227. [Google Scholar] [CrossRef]
- Ye, D.H.; Zhan, Z.G.; Lee, Y.J.; Tu, Z.K.; Zhang, Y.; Pan, M. Effects of Frame Materials and Structures on Stress Concentration of Membrane Electrode Assembly of PEMFCs. Fuel Cells 2013, 13, 1205–1212. [Google Scholar] [CrossRef]
- Yao, J.; Yan, F.-Y.; Pei, X.-J. Bionic flow field research and optimization of PEMFC with multi-branch veins. Chem. Pap. 2023, 77, 935–946. [Google Scholar] [CrossRef]
- Stefancu, A.; Melenciuc, S.; Budescu, M. Construction. Architecture Section, Penalty Based Algorithms for Frictional Contact Problems. Bull. Polytech. Inst. Jassy Constr. 2011, 57, 119–130. [Google Scholar]
- Lu, Z.; Kim, C.; Karlsson, A.M.; Cross, J.C.; Santare, M.H. Effect of gas diffusion layer modulus and land–groove geometry on membrane stresses in proton exchange membrane fuel cells. J. Power Sources 2011, 196, 4646–4654. [Google Scholar] [CrossRef]
- Liu, W.; Qiu, D.; Peng, L.; Yi, P.; Lai, X. Mechanical degradation of proton exchange membrane during assembly and running processes in proton exchange membrane fuel cells with metallic bipolar plates. Int. J. Energy Res. 2020, 44, 8622–8634. [Google Scholar] [CrossRef]
- Wei, C.; Lu, A.; Sun, S.; Wei, X.; Zho, X.; Sun, J. Establishment of Constitutive Model of Silicone Rubber Foams Based on Statistical Theory of Rubber Elasticity. Chin. J. Polym. Sci. 2018, 36, 1077–1083. [Google Scholar] [CrossRef]
- Peng, X.; Han, L.; Li, L. A consistently compressible Mooney-Rivlin model for the vulcanized rubber based on the Penn’s experimental data. Polym. Eng. Sci. 2021, 61, 2287–2294. [Google Scholar] [CrossRef]
- Wu, B.; Zhao, M.; Shi, W.; Liu, W.; Liu, J.; Xing, D.; Yao, Y.; Hou, Z.; Ming, P.; Gu, J.; et al. The degradation study of Nafion/PTFE composite membrane in PEM fuel cell under accelerated stress tests. Int. J. Hydrogen Energy 2014, 39, 14381–14390. [Google Scholar] [CrossRef]
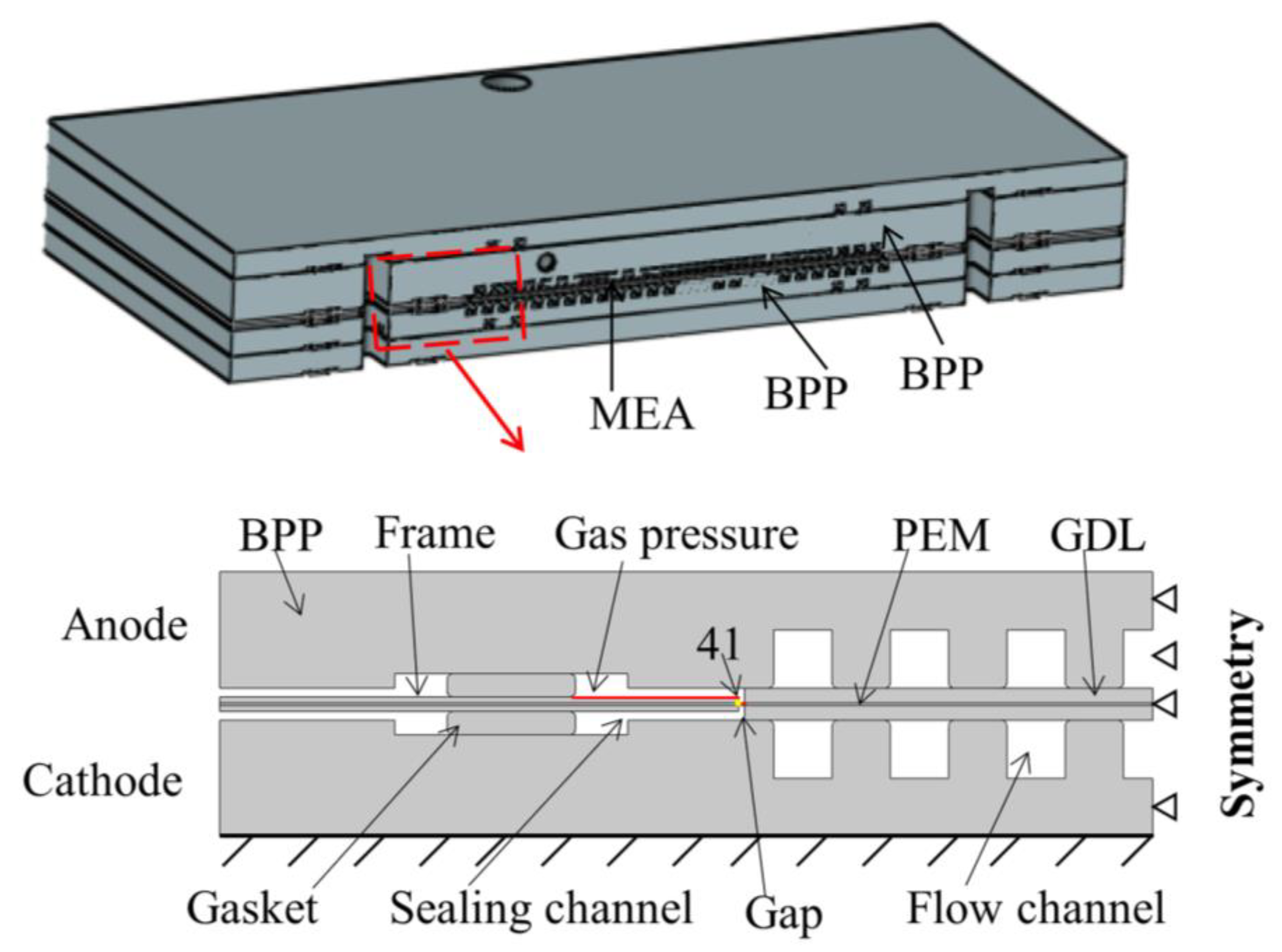



| Parameters | Value/(mm) | |
|---|---|---|
| BPP | Thickness | 2 |
| Flow channel/ridge width | 1 | |
| Flow channel/ridge height | 1 | |
| Sealing channel width | 4 | |
| Gasket | Width | 2.2 |
| Height | 0.4 | |
| GDL thickness | 0.25 | |
| Frame thickness | 0.1 | |
| Membrane thickness | 0.05 | |
| Component | Young’s Modulus/(MPa) | Poisson’s Ratio | Density/(kg/m3) |
|---|---|---|---|
| BPP | 10,000 | 0.3 | 1800 |
| Frame [29] | 600 | 0.3 | 900 |
| GDL [28] | 9 | 0.09 | 400 |
| Membrane [19] | 197 | 0.25 | 2000 |
| Parameter | ||
|---|---|---|
| Value/(MPa) | 0.59949 | 0.26034 |
| Material Types | Young’s Modulus/(MPa) | Poisson’s Ratio | Density/(kg/m3) |
|---|---|---|---|
| Composite material | 60,000 | 0.3 | 2540 |
| Composite material | 24,500 | 0.3 | 2000 |
| Macromolecular material | 3600 | 0.38 | 1200 |
| Macromolecular material | 600 | 0.3 | 900 |
| Macromolecular material | 550 | 0.46 | 930 |
| Macromolecular material | 200 | 0.25 | 1900 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Z.; Tan, Y.; Yang, D.; Chu, T.; Li, B. A Finite Element Analysis Model-Based Study on the Effect of the Frame on Membrane Stresses in Proton Exchange Membrane Fuel Cells. Energies 2023, 16, 7044. https://doi.org/10.3390/en16207044
Zhang Z, Tan Y, Yang D, Chu T, Li B. A Finite Element Analysis Model-Based Study on the Effect of the Frame on Membrane Stresses in Proton Exchange Membrane Fuel Cells. Energies. 2023; 16(20):7044. https://doi.org/10.3390/en16207044
Chicago/Turabian StyleZhang, Zikuan, Yongle Tan, Daozeng Yang, Tiankuo Chu, and Bing Li. 2023. "A Finite Element Analysis Model-Based Study on the Effect of the Frame on Membrane Stresses in Proton Exchange Membrane Fuel Cells" Energies 16, no. 20: 7044. https://doi.org/10.3390/en16207044
APA StyleZhang, Z., Tan, Y., Yang, D., Chu, T., & Li, B. (2023). A Finite Element Analysis Model-Based Study on the Effect of the Frame on Membrane Stresses in Proton Exchange Membrane Fuel Cells. Energies, 16(20), 7044. https://doi.org/10.3390/en16207044





