Research on the Modulation and Control Strategy for a Novel Single-Phase Current Source Inverter
Abstract
:1. Introduction
2. Topology and Operating Modes of the Proposed Single-Phase CSI
2.1. Analysis of the Proposed Single-Phase CSI’s Operating Modes
2.2. Comparison of Different CSI Topologies
3. Modulation Strategy Based on DC-Link Current Control
4. Calculation for the Optimal Reference of DC-Link Current
5. Control Strategy of the Output Voltage
6. Experimental Results
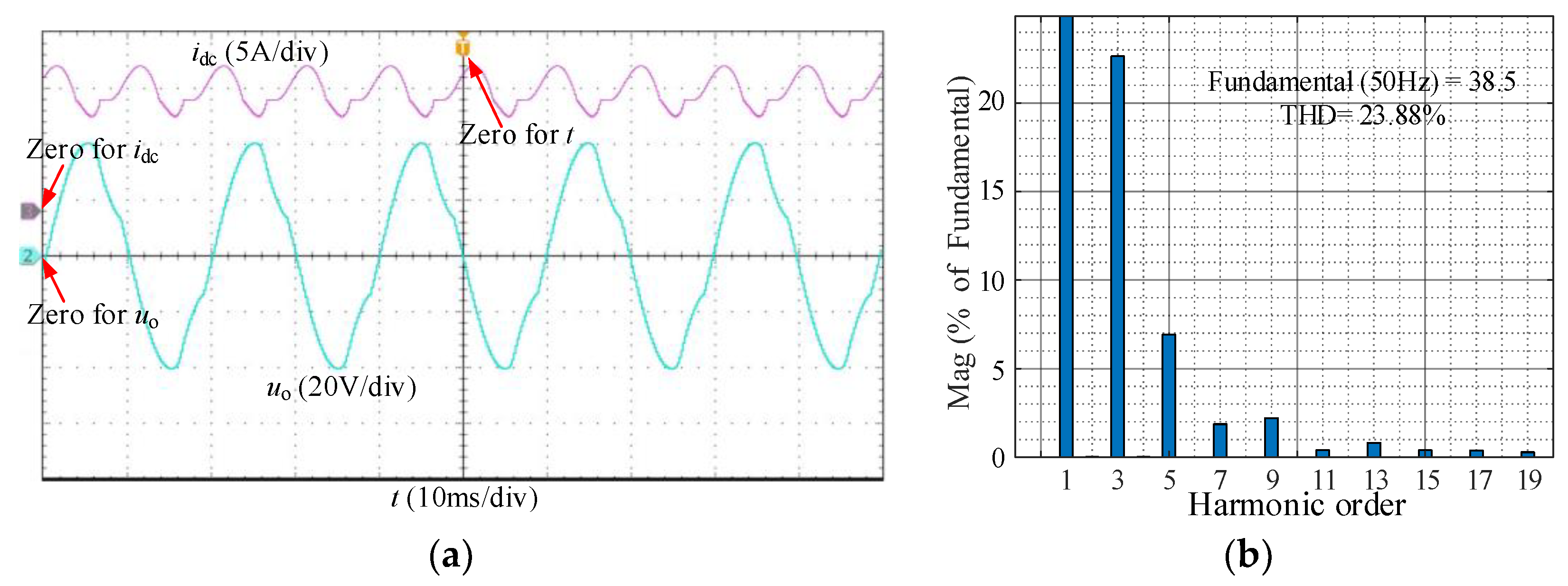
6.1. Experimental Results of Steady-State
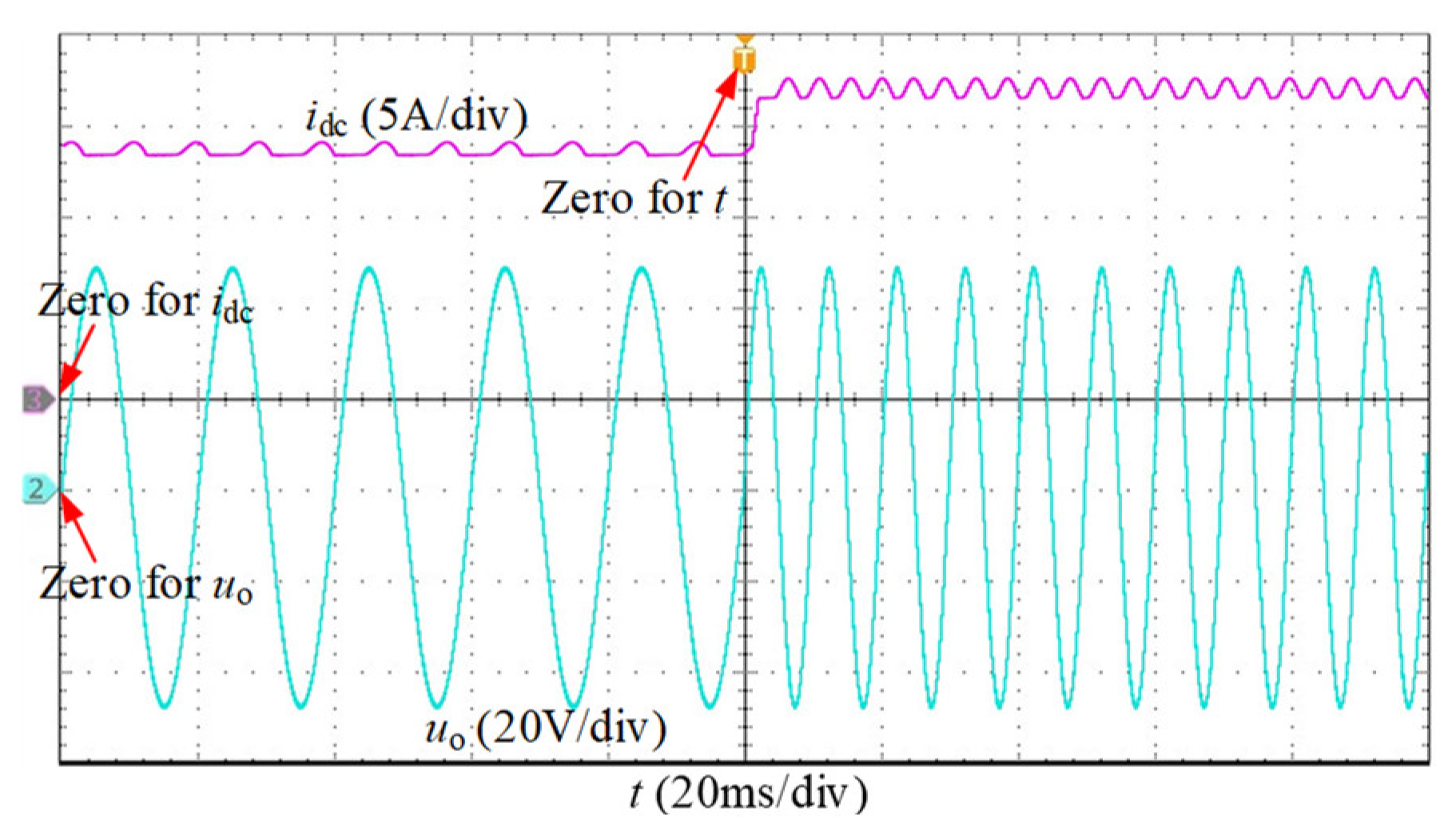
6.2. Experimental Results of Dynamic-State
7. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| VSI | Voltage Source Inverter |
| CSI | Current Source Inverter |
| HVDC | High Voltage Direct Current |
| Ldc | DC-link inductance (mH) |
| C | AC side’s filter capacitor (μF) |
| R | AC side’s resistive load (Ω) |
| idc | DC side’s current (A) |
| io | Output current on the resistive load R (A) |
| udc | DC side’s voltage (V) |
| uo | Output voltage on the resistive load R (A) |
| p/q | Switching functions for S0, S1, S2, S3, and S4 (-) |
| d | Duty cycle (%) |
| Reference of the output current io (A) | |
| Reference of the DC side’s current idc (A) | |
| ∆idc_down | Decrement of idc when discharging (A) |
| ∆idc_up | Increment of idc when charging (A) |
| Ts | Switching period (s) |
| U | Fundamental amplitude of uo (V) |
| I | Fundamental amplitude of io (A) |
| θ | Initial phase of io (rad) |
| ω | Fundamental frequency of io (Hz) |
| ∆idcmax | Maximum reduction of idc (A) |
| uod/uod | d-q frame components of uo |
| Reference of uod/uod | |
| iod/iod | d-q frame components of io |
| Reference of iod/iod |
References
- Chakrabarti, A.; Sarkar, K.; Kasari, P.R.; Das, B.; Biswas, S.K. A CB-PWM Technique for Eliminating CMV in Multilevel Multiphase VSI. IEEE Trans. Ind. Electron. 2022, 70, 8666–8675. [Google Scholar] [CrossRef]
- He, J.; Zhang, C.; Lei, M.; Han, J.; Guo, P.; Li, Y. An Active Bypass Pulse Injection-Based Low Switching Frequency PWM Approach for Harmonic Compensation of Current-Source Converters. IEEE Trans. Power Electron. 2021, 36, 1614–1625. [Google Scholar] [CrossRef]
- Rajeev, M.; Agarwal, V. Single phase current source inverter with multiloop control for transformerless grid–PV interface. IEEE Trans. Ind. Appl. 2018, 54, 2416–2424. [Google Scholar] [CrossRef]
- Titus, J.; Harikrishnan, P.; Hatua, K. An SCR-Based CSI-Fed Induction Motor Drive for High Power Medium Voltage Applications. IEEE Trans. Ind. Electron. 2020, 68, 4657–4666. [Google Scholar] [CrossRef]
- Guo, X.-Q.; Sui, S.; Wang, B.; Zhang, W. A Current-Based Approach for Short-Circuit Fault Diagnosis in Closed-Loop Current Source Inverter. IEEE Trans. Ind. Electron. 2019, 67, 7941–7950. [Google Scholar] [CrossRef]
- Miao, Y.; Liao, W.; Huang, S.; Liu, P.; Wu, X.; Song, P.; Li, G. DC-Link Current Minimization Scheme for IM Drive System Fed by Bidirectional DC Chopper-Based CSI. IEEE Trans. Transp. Electrif. 2023, 9, 2839–2850. [Google Scholar] [CrossRef]
- Li, Y.W.; Pande, M.; Zargari, N.R.; Wu, B. DC-link current minimization for high-power current-source motor drives. IEEE Trans. Power Electron. 2008, 24, 232–240. [Google Scholar]
- Geng, Y.; Yang, K.; Lai, Z.; Zheng, P.; Liu, H.; Deng, R. A Novel Low Voltage Ride Through Control Method for Current Source Grid-Connected Photovoltaic Inverters. IEEE Access 2019, 7, 51735–51748. [Google Scholar] [CrossRef]
- Wang, W.; Gao, F.; Yang, Y.; Blaabjerg, F. Operation and modulation of H7 current-source inverter with hybrid SiC and Si semiconductor switches. IEEE J. Emerg. Sel. Top. Power Electron. 2017, 6, 387–399. [Google Scholar] [CrossRef]
- Yang, S.; Peng, F.Z.; Lei, Q.; Inoshita, R.; Qian, Z. Current-fed quasi-Z-source inverter with voltage Buck–Boost and regeneration capability. IEEE Trans. Ind. Appl. 2010, 47, 882–892. [Google Scholar] [CrossRef]
- Guo, X.; Wang, N.; Zhang, J.; Wang, B.; Nguyen, M.-K. A Novel Transformerless Current Source Inverter for Leakage Current Reduction. IEEE Access 2019, 7, 50681–50690. [Google Scholar] [CrossRef]
- Nascimento, A.J.P.; de Menezes, B.F.; de Mesquita, S.J.; Costa, K.R.; Tofoli, F.L.; Daher, S.; Antunes, F.L.M. Bidirectional Isolated Asymmetrical Multilevel Inverter. IEEE Trans. Circuits Syst. II Express Briefs 2022, 70, 151–155. [Google Scholar] [CrossRef]
- Sun, R.; Wang, X.; Ye, Y. Seventeen-Level Inverter Based on Switched-Capacitor and Flying-Capacitor-Fed T-type Unit. IEEE Access 2022, 10, 33561–33570. [Google Scholar] [CrossRef]
- Ding, L.; Li, Y.W. Simultaneous DC current balance and common-mode voltage control with multilevel current source inverters. IEEE Trans. Power Electron. 2018, 33, 9188–9197. [Google Scholar] [CrossRef]
- Guo, Q.; Zhou, C.; Li, S. A Multiple Loops Control Strategy Based on DC Link Voltage of Current Source PWM Rectifiers. Trans. China Electrotech. Soc. 2022, 37, 2051–2063. [Google Scholar]
- Wu, F.; Feng, F.; Luo, L.; Duan, J.; Sun, L. Sampling period online adjusting-based hysteresis current control without band with constant switching frequency. IEEE Trans. Ind. Electron. 2014, 62, 270–277. [Google Scholar] [CrossRef]
- Wang, Z.; Wu, B.; Xu, D.; Cheng, M.; Xu, L. DC-link current ripple mitigation for current-source grid-connected converters under unbalanced grid conditions. IEEE Trans. Ind. Electron. 2016, 63, 4967–4977. [Google Scholar] [CrossRef]




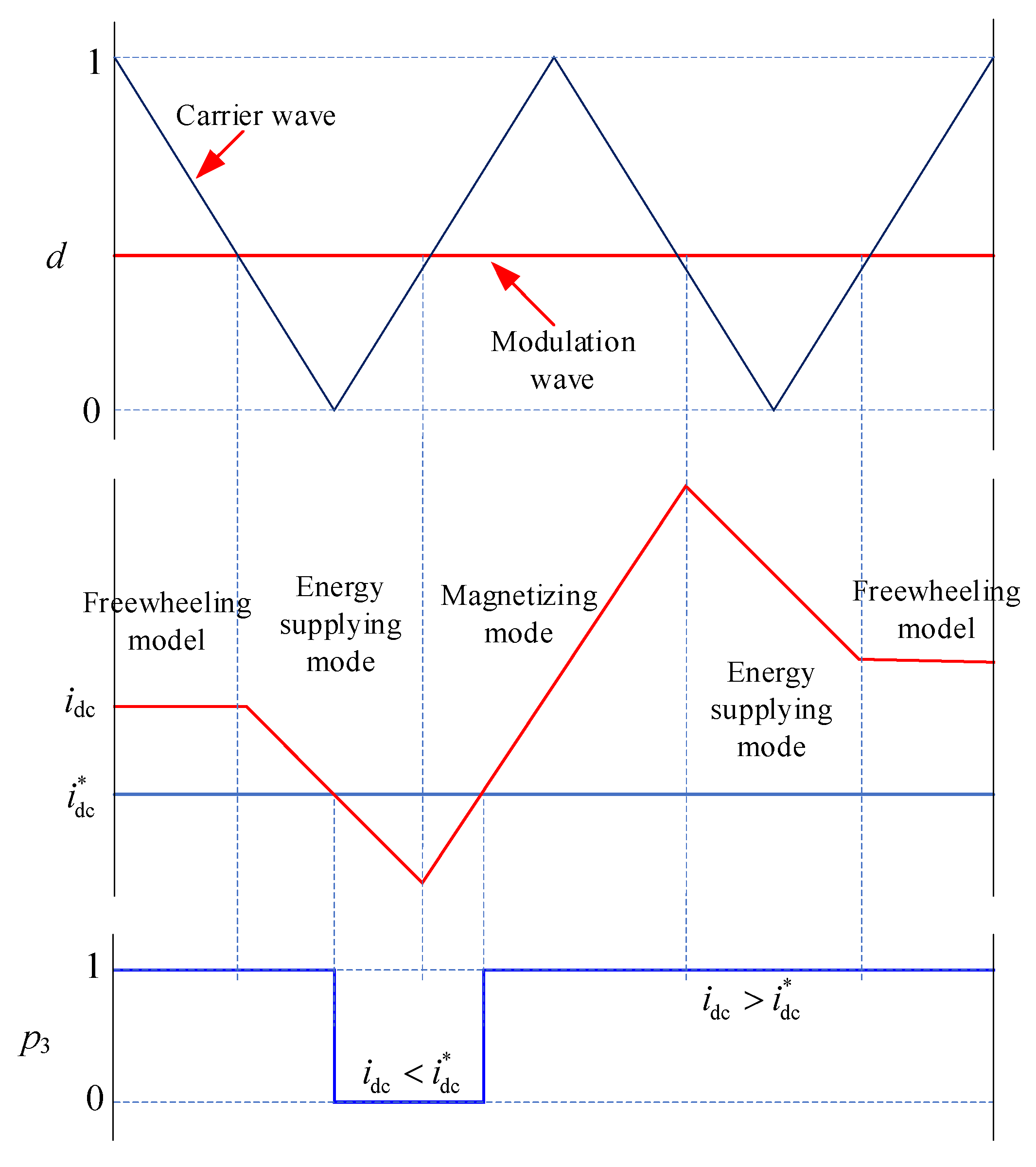

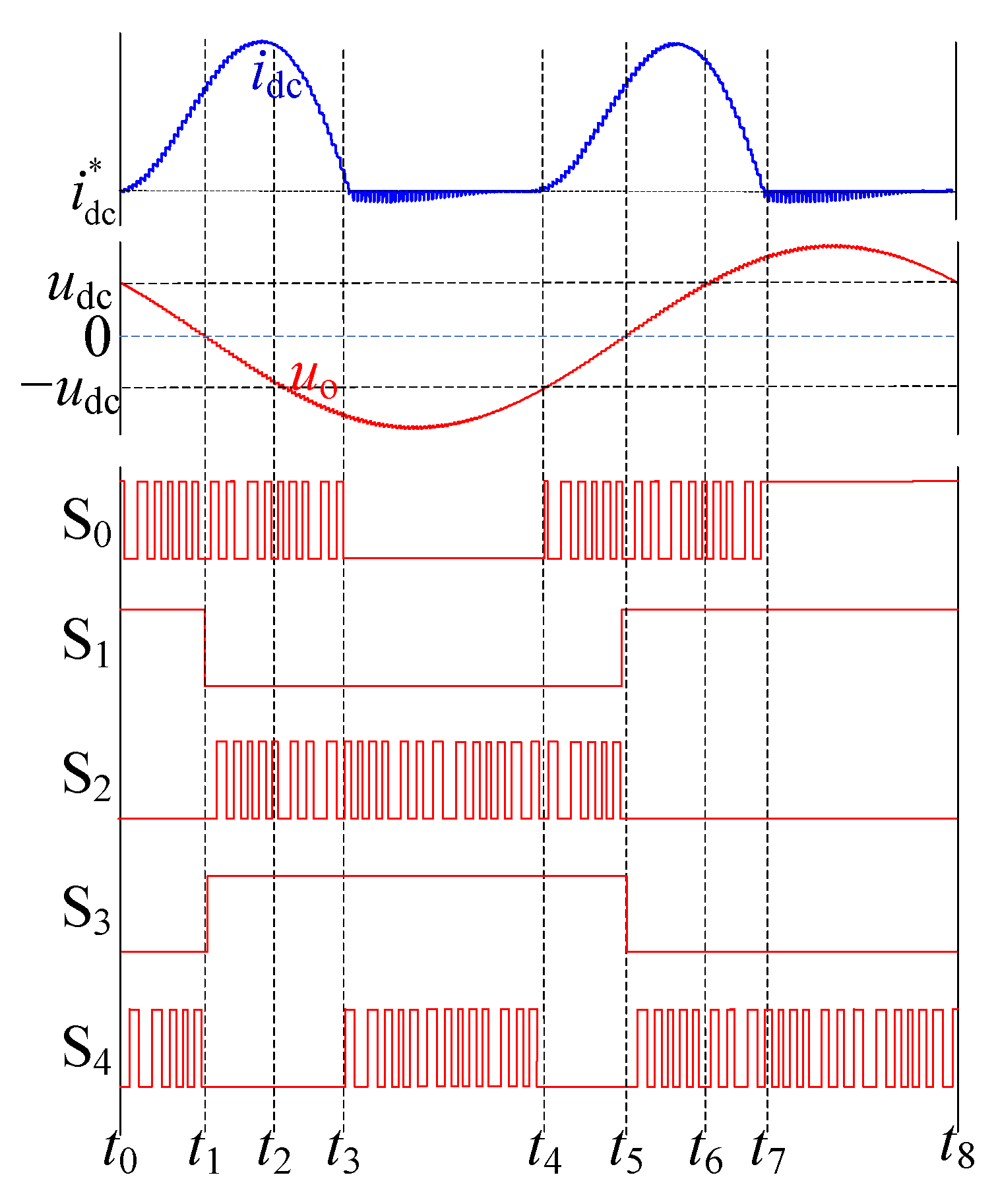
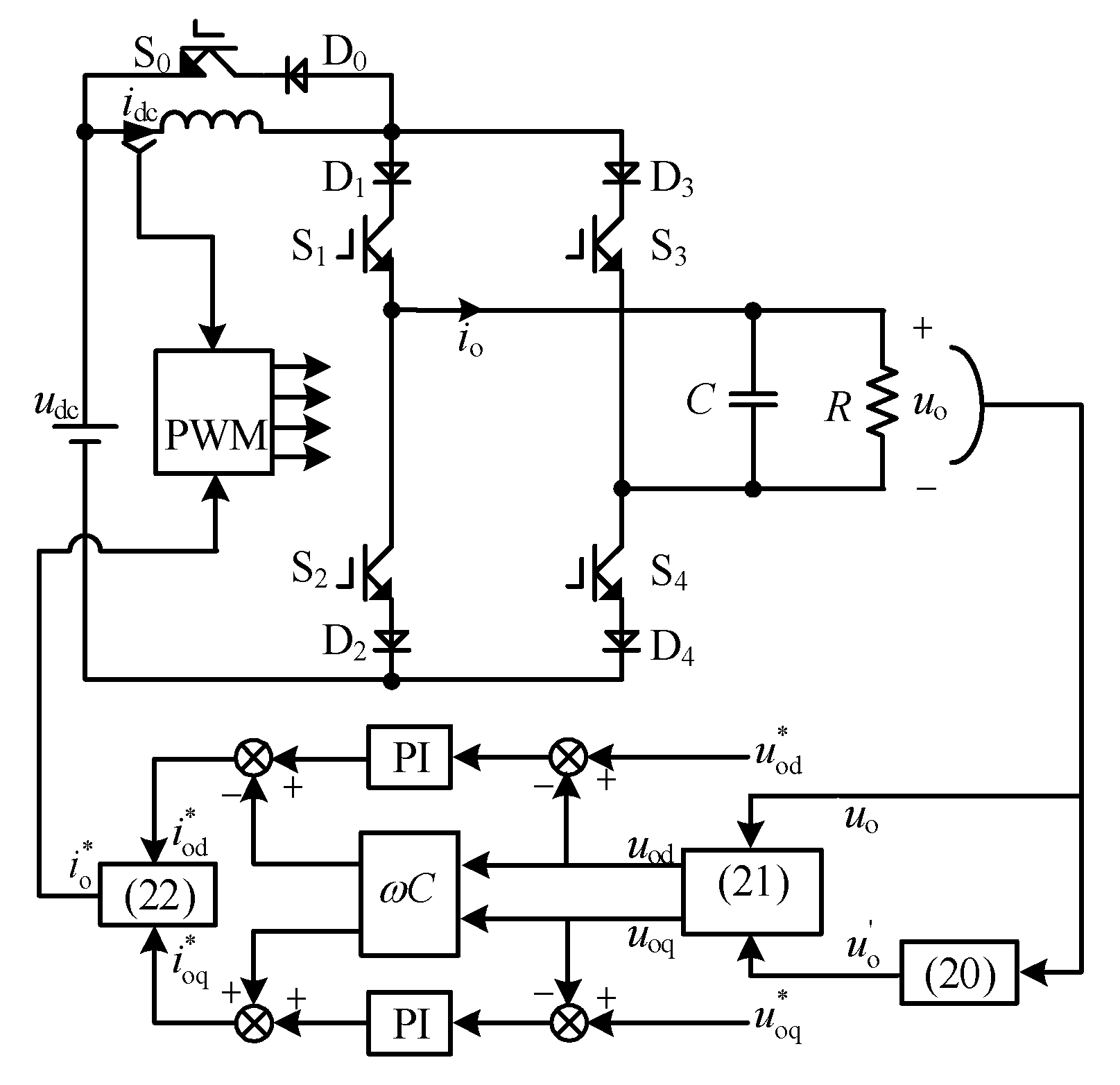
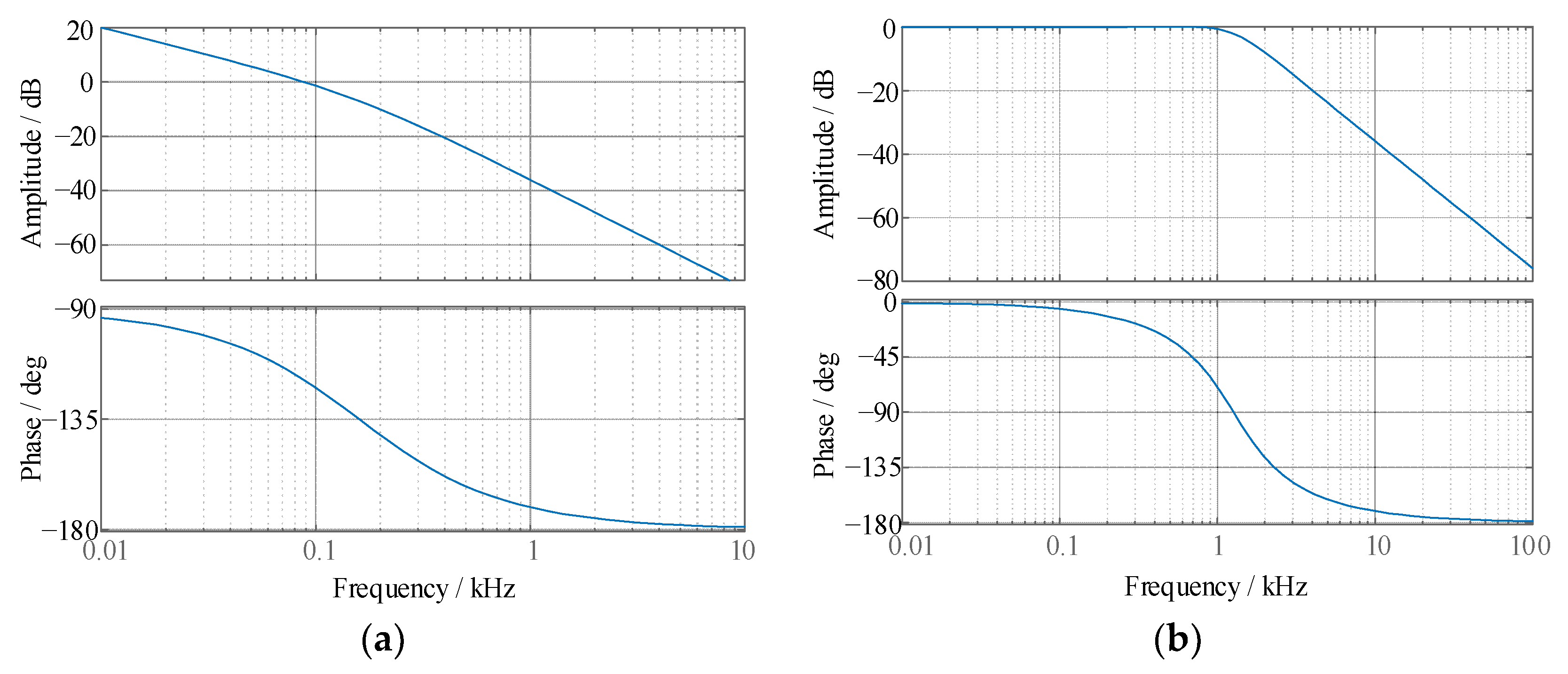





| Operating Modes | Switching State | Switching Function p and q |
|---|---|---|
| magnetizing mode | S1 and S2 ON, S0, S3, and S4 OFF | p = 0, q = 1 |
| energy-supplying mode I | S1 and S4 ON, S0, S2, and S3 OFF | p = 1, q = 0 |
| energy-supplying mode II | S2 and S3 ON, S0, S1, and S4 OFF | p = −1, q = 0 |
| freewheeling model | S0 ON, S1, S2, S3, and S4 OFF | p = 0, q = 0 |
| Operating Mode | Withstand Voltage S0 | Withstand Voltage S1 | Withstand Voltage S2 | Withstand Voltage S3 | Withstand Voltage S4 |
|---|---|---|---|---|---|
| Magnetizing mode | udc | 0 | 0 | −uo | uo |
| Energy-supplying mode | udc − uo | 0 | −uo | −uo | 0 |
| Freewheeling model | 0 | uo | −udc − uo | 0 | −udc |
| Parameter | Current Source Rectifier | Buck Converter | Quasi-Z Source Network | Bidirectional DC Chopper | Proposed Single-Phase CSI |
|---|---|---|---|---|---|
| NV | 1 | 1 | 1 | 1 | 1 |
| NUS | 0 | 0 | 0 | 0 | 0 |
| NBS | 8 | 5 | 4 | 6 | 5 |
| ND | 8 | 5 | 5 | 6 | 5 |
| NC | 2 | 1 | 2 | 1 | 1 |
| Ndrv | 8 | 5 | 4 | 6 | 5 |
| NT | 0 | 0 | 1 | 0 | 0 |
| Nl | 3 | 3 | 3 | 3 | 3 |
| CF | 11.33 | 7 | 6.67 | 8.33 | 7 |
| Input H Bridge | Yes | No | No | Yes | No |
| Modulation | PWM | PWM | PWM | PWM | PWM |
| Name | Value |
|---|---|
| DC input voltage (V) | 25 |
| AC output voltage (V) | 30~150 |
| Load power (W) | ≤103 |
| Output frequency (Hz) | ≤500 Hz |
| Switching frequency (Hz) | 10 k |
| Sampling frequency (Hz) | 10 k |
| Ldc (mH) | 4 |
| C (μF) | 265 |
| R (Ω) | 25 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Y.; Yang, T.; Miao, Y. Research on the Modulation and Control Strategy for a Novel Single-Phase Current Source Inverter. Energies 2023, 16, 6729. https://doi.org/10.3390/en16186729
Zhang Y, Yang T, Miao Y. Research on the Modulation and Control Strategy for a Novel Single-Phase Current Source Inverter. Energies. 2023; 16(18):6729. https://doi.org/10.3390/en16186729
Chicago/Turabian StyleZhang, Yi, Tao Yang, and Yiru Miao. 2023. "Research on the Modulation and Control Strategy for a Novel Single-Phase Current Source Inverter" Energies 16, no. 18: 6729. https://doi.org/10.3390/en16186729
APA StyleZhang, Y., Yang, T., & Miao, Y. (2023). Research on the Modulation and Control Strategy for a Novel Single-Phase Current Source Inverter. Energies, 16(18), 6729. https://doi.org/10.3390/en16186729







