Next Article in Journal
Review, Comprehensive Analysis and Derivation of Analytical Power Loss Calculation Equations for Two- to Three-Level Midpoint Clamped Inverter Topologies with Hybrid Switch Configurations
Previous Article in Journal
The Design, Analysis, and Optimization of a New Pitch Mechanism for Small Wind Turbines
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Energies
Volume 16
Issue 18
10.3390/en16186707
energies-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Improving Efficiency of a Pole-Changing Vernier Machine Considering Residual Magnetic Flux Density

by
Sung-Hyun Lee
,
Jung-Woo Kwon
and
Byung-Il Kwon
*
Department of Electrical and Electronic Engineering, Hanyang University, Ansan 15588, Republic of Korea
*
Author to whom correspondence should be addressed.
Energies 2023, 16(18), 6707; https://doi.org/10.3390/en16186707
Submission received: 18 August 2023 / Revised: 12 September 2023 / Accepted: 18 September 2023 / Published: 19 September 2023
(This article belongs to the Section F: Electrical Engineering)
Browse Figures
Versions Notes

Abstract

:
This paper presents the efficiency improvement of a pole-changing vernier machine (PCVM) by considering the residual magnetic flux density ( B r ) of low coercivity force (LCF) permanent magnets (PMs). The PCVM operates in two modes: vernier machine (VM) mode and permanent magnet synchronous machine (PMSM) mode, achieved through pole-changing. Pole-changing involves reversing the magnetic flux direction of LCF PM to alter the number of rotor pole pairs. By changing the number of rotor pole pairs, the PCVM operates as a VM mode at low speeds, providing high torque, and as a PMSM mode at high speeds, offering high efficiency. To achieve this, a combination of high coercivity force (HCF) PM and LCF PM is utilized in a single structure. The magnetic flux direction in the LCF PM is determined by B r , and the highest efficiency is achieved when B r reaches its maximum value | B r m |. This paper focuses on improving efficiency by obtaining B r m in VM mode and − B r m in PMSM mode through the design process. Additionally, finite element analysis (FEA) is employed to compare the performance of the improved model, which considers B r , with that of the conventional model, designed without considering B r . The improved model achieves higher B r values in each mode compared to the conventional model, resulting in increased torque density. Consequently, this leads to improved efficiency.

1. Introduction

Permanent magnet synchronous machines (PMSMs) have gained significant attention due to their high torque density and efficiency [1,2,3]. However, the constant airgap flux of permanent magnets (PMs) presents a challenge for the operation of the machine over a wide speed range [4]. Designing a machine solely for low-speed operation results in decreased efficiency at higher speeds due to high iron losses. Conversely, optimizing for high-speed operation leads to lower efficiency and torque at low speeds as the magnetic flux density decreases due to high copper losses [5,6].
Vernier machines (VMs) have emerged as a viable option for low-speed, high-torque applications like wind turbines. VMs offer a magnetic gear effect that provides high torque density and efficiency at low speeds [7,8,9]. The VM generates a back electromotive force (B−EMF) approximately 1.5–3 times larger than that of a typical PMSM, resulting in substantial torque even at low speeds. As a result, VMs allow for compact size and high efficiency in the low-speed range [10,11,12,13]. However, VMs typically have a higher number of pole pairs compared to conventional PMSMs, resulting in increased core losses. These losses escalate as the speed rises, leading to decreased efficiency at high speeds. Consequently, VMs have primarily been studied and optimized for low-speed applications, limiting their advantages [14,15].
Efforts have been made to apply the concept of pole-changing, based on the memory machine principle [16,17,18,19], to VMs to combine the advantages of high torque at low speeds from VMs and high efficiency at high speeds from PMSMs within a single machine structure [20,21,22,23]. One such development is the pole-changing vernier machine (PCVM) [20], where pole-changing is achieved by utilizing both low coercivity force (LCF) and high coercivity force (HCF) PMs on the rotor. By reversing the magnetic flux direction of the LCF PM, the number of rotor poles can be altered, enabling the PCVM to operate as a VM at low speeds, providing high torque, and as a PMSM at high speeds, offering high efficiency. Consequently, the PCVM exhibits high efficiency across a wide speed range. A PCVM with high efficiency across a wide speed range can offer significant advantages in applications with wide speed ranges and multiple loads, such as washing machines and electric vehicles (EVs) [24,25,26].
However, previous studies [20] focused primarily on proposing the pole-changing topology under the ideal assumption that the residual magnetic flux density ( B r ) of the LCF PM would reach its maximum value, | B r m |. Consequently, the efficiency of the conventional PCVM model, designed without considering B r of the LCF PM, decreased across the wide speed range due to low magnetic flux density when B r   of the LCF PM failed to attain B r m or − B r m   in each mode.
This paper aims to enhance the efficiency of the PCVM by improving the magnetic flux density through design considerations for B r of the LCF PM. Section 2 presents an overview of the PCVM structure, the operating principles of its two modes achieved through pole-changing, and the pole-changing process based on variations in B r of the LCF PM. Section 3 selects the design parameters for the PCVM, considering B r of the LCF PM, and determines the range of these parameters. The final values of the design parameters are obtained through the pole-changing process within the established range. In Section 4, the improved model is validated to achieve B r m in VM mode and − B r m in PMSM mode. The efficiency improvement is then verified by comparing the performance of the improved model, designed with consideration of B r of the LCF PM, with that of the conventional model, designed without such consideration. Finally, Section 5 concludes the paper.

2. Operating Principle and Pole-Changing Process

2.1. Structure of PCVM

The PCVM structure, as depicted in Figure 1, comprises 36 slots with concentrated three-phase armature windings in the stator slots. To achieve pole-changing, two types of PMs with different coercivities, namely HCF PMs and LCF PMs, are utilized. In the consequent pole structure, half of the HCF PMs and LCF PMs are replaced with an iron pole [27]. Pole-changing is accomplished by reversing the magnetic flux direction of the LCF PM, thereby altering the number of rotor pole pairs.

2.2. Operating Principle of Two Modes

Figure 2 shows the 12-segment structure of PCVM and indicates the magnetic flux direction of PMs with arrows.
The VM mode operates based on the principle described in Equation (1), which utilizes the magnetic gear effect.
Z r = Z s ± p
Here, Z r represents the number of rotor pole pairs, Z s is the number of stator slots, and p denotes the number of armature winding pole pairs. As shown in Figure 2a, the PCVM operates in VM mode when the magnetic flux direction of HCF PM and LCF PM is the same. This condition is achieved by satisfying Equation (1) with Z s = 36, Z r = 24, and p = 12.
The PMSM mode operates based on the principle described by Equation (2), where torque is generated through the interaction between rotor pole pairs and armature winding pole pairs.
Z r = p
As depicted in Figure 2b, the PCVM operates in PMSM mode when the magnetic flux directions of the HCF PM and LCF PM are different. This condition is achieved by satisfying Equation (2) with Z r = 12, p = 12, due to the pole-changing that results in a different direction of the LCF PMs.

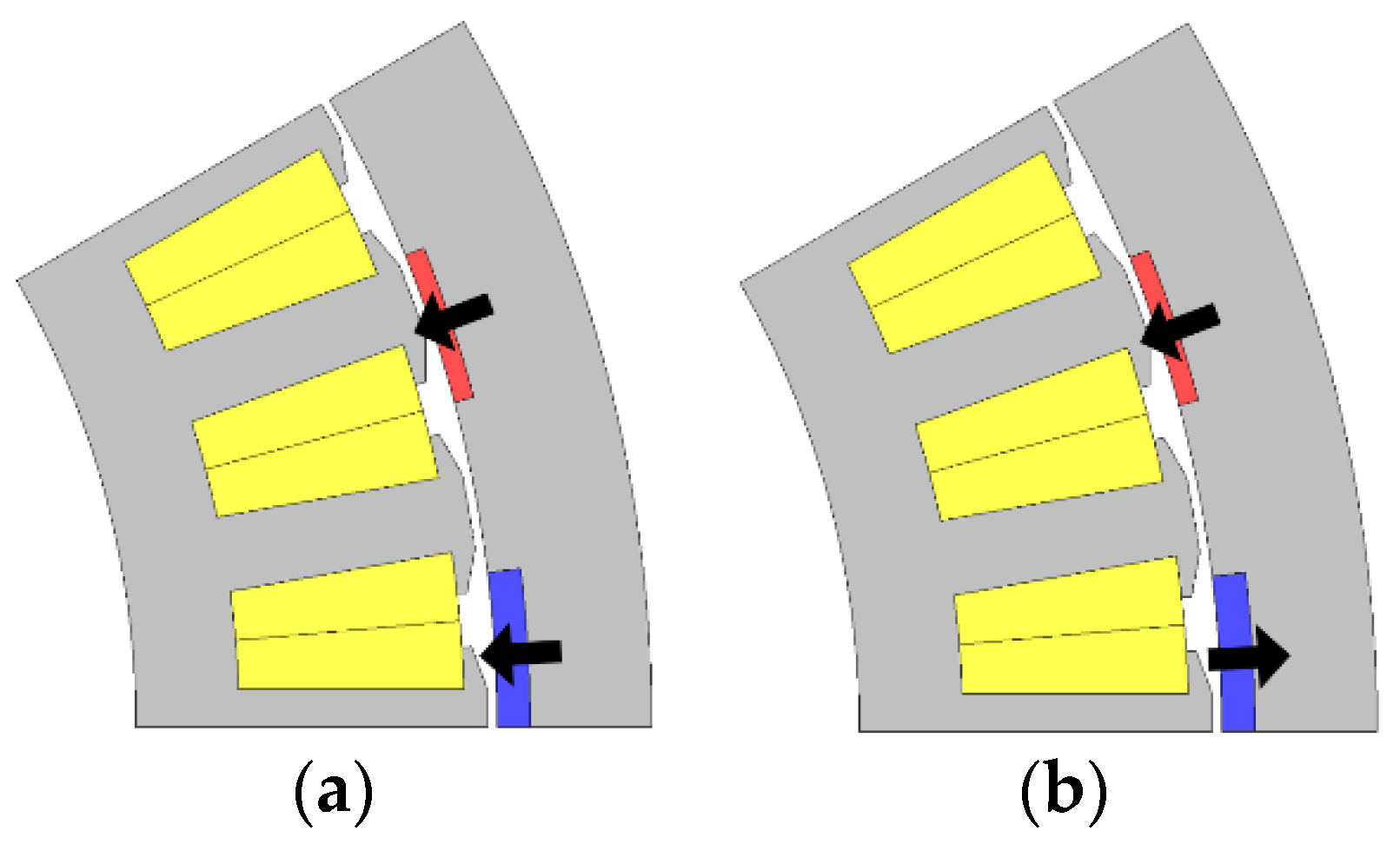
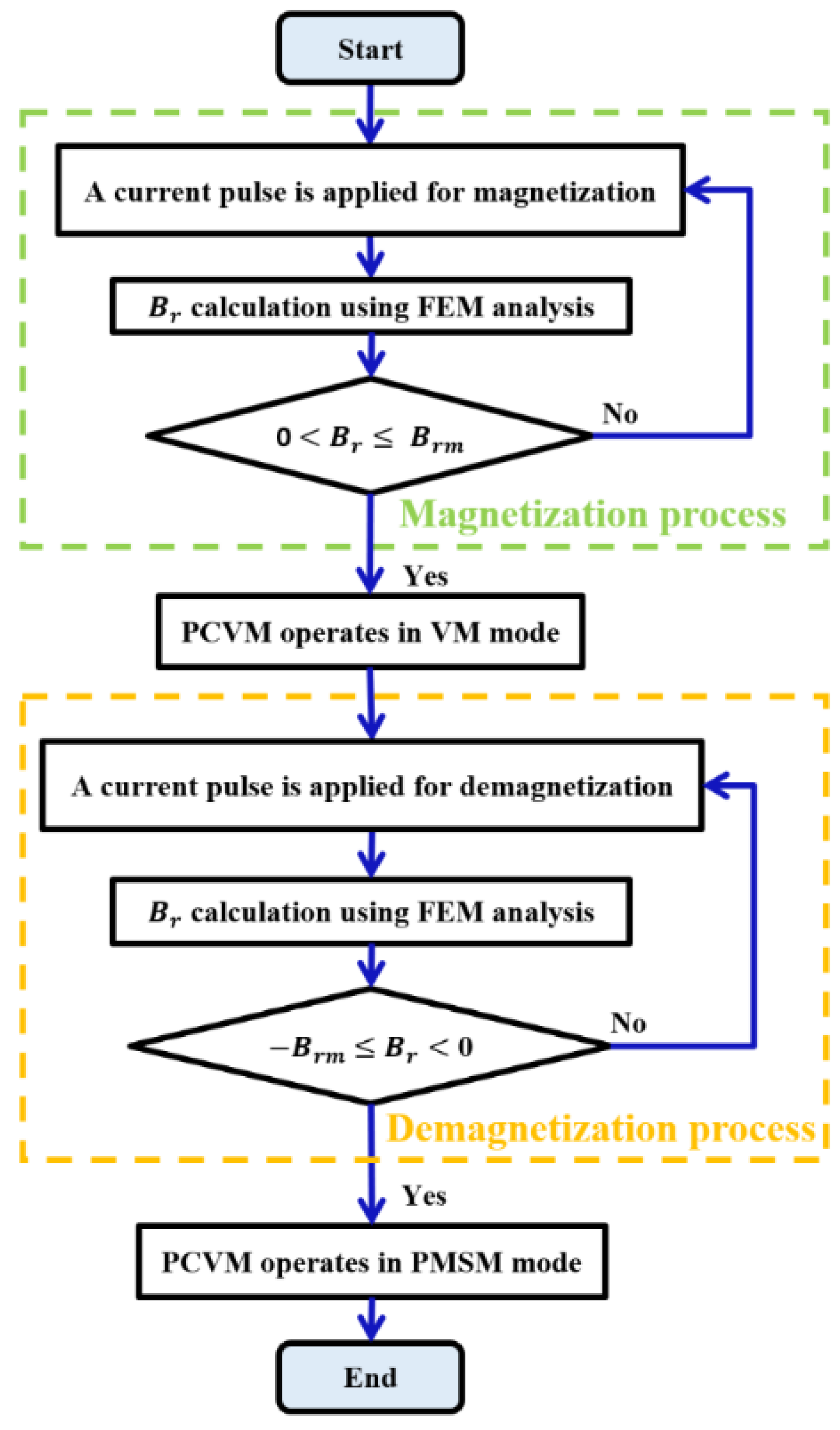
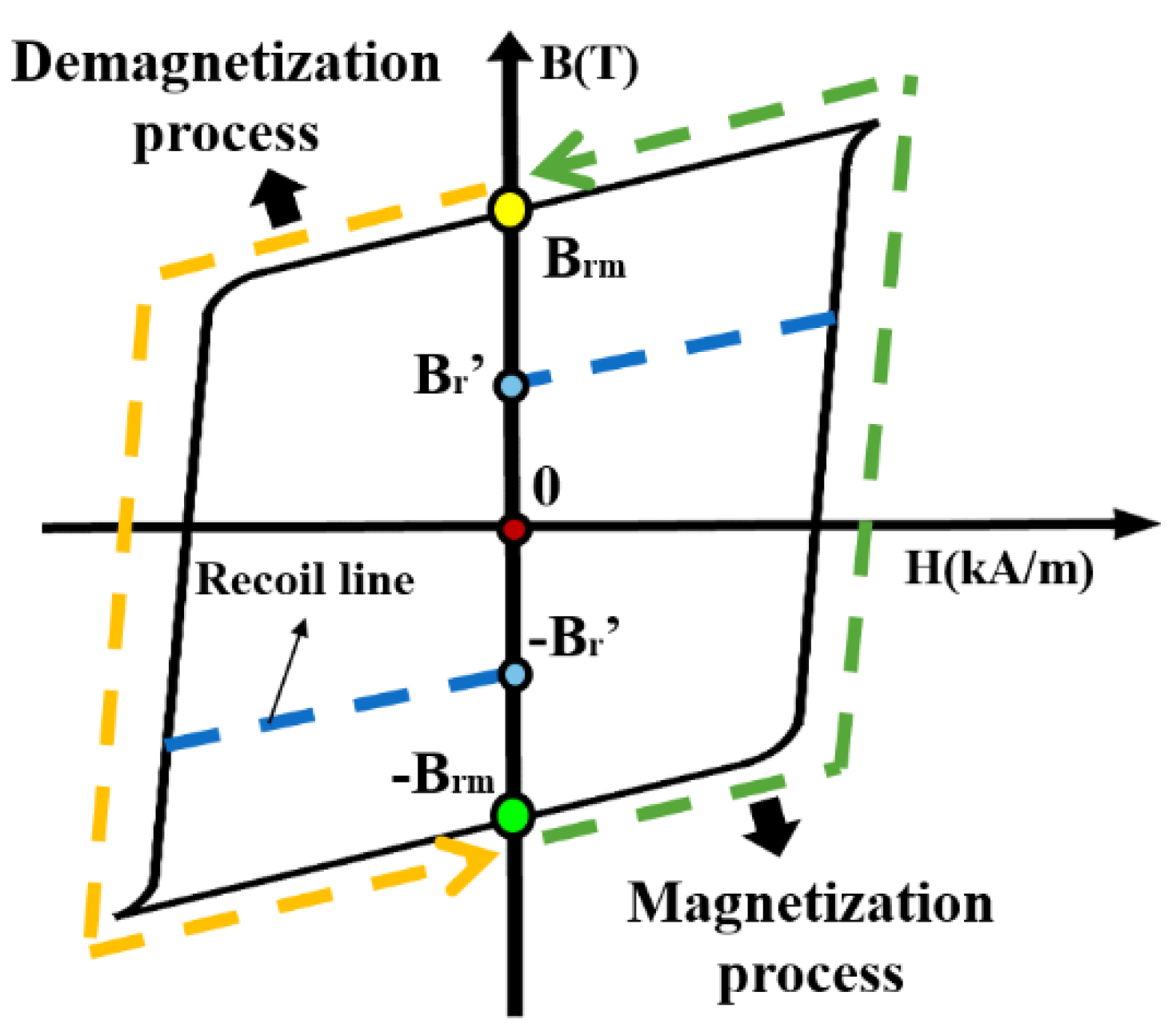
2.3. Pole-Changing Process Using B r of the LCF PM

Figure 3 illustrates the flowchart of the pole-changing process using B r of the LCF PM, while Figure 4 represents the B−H curve of the LCF PM. The process begins by applying a current pulse for magnetization to obtain a positive value of B r in the LCF PM. Furthermore, B r of the LCF PM changes according to the magnetization process, as indicated by the green line on the B−H curve in Figure 4. The B r value is calculated using finite element analysis (FEA). If B r falls within the range of “ 0 < B r ≤ B r m ”, the PCVM operates in VM mode, as depicted in Figure 2a. If B r is not within the specified range, the magnetization process is repeated by returning to the application of current pulses for magnetization, as indicated in the flowchart in Figure 3.
Next, a current pulse is applied for demagnetization to obtain a negative value of B r in the LCF PM. B r of the LCF PM changes according to the demagnetization process, represented by the orange line on the B−H curve in Figure 4. The B r value is calculated using FEA. If B r falls within the range of “ − B r m ≤ B r < 0 ”, the PCVM operates in PMSM mode, as shown in Figure 2b. If B r is not within the specified range, the demagnetization process is repeated by returning to the application of current pulses for demagnetization, following the flowchart in Figure 3.

3. Design That Considers B r of the LCF PM

3.1. Conventional Model without Considering B r

The conventional model focused primarily on proposing a pole-changing topology, assuming an ideal condition where all areas of the LCF PM exhibit either B r m or − B r m , as shown by the B−H curve in Figure 4. However, this design overlooked the B r of the LCF PM, resulting in reduced efficiency due to lower torque density when the B r of LCF PMs fell along the recoil line, such as B r ′ or − B r ′ in Figure 4. The specifications of the conventional model, designed without considering B r of the LCF PM, are summarized in Table 1.

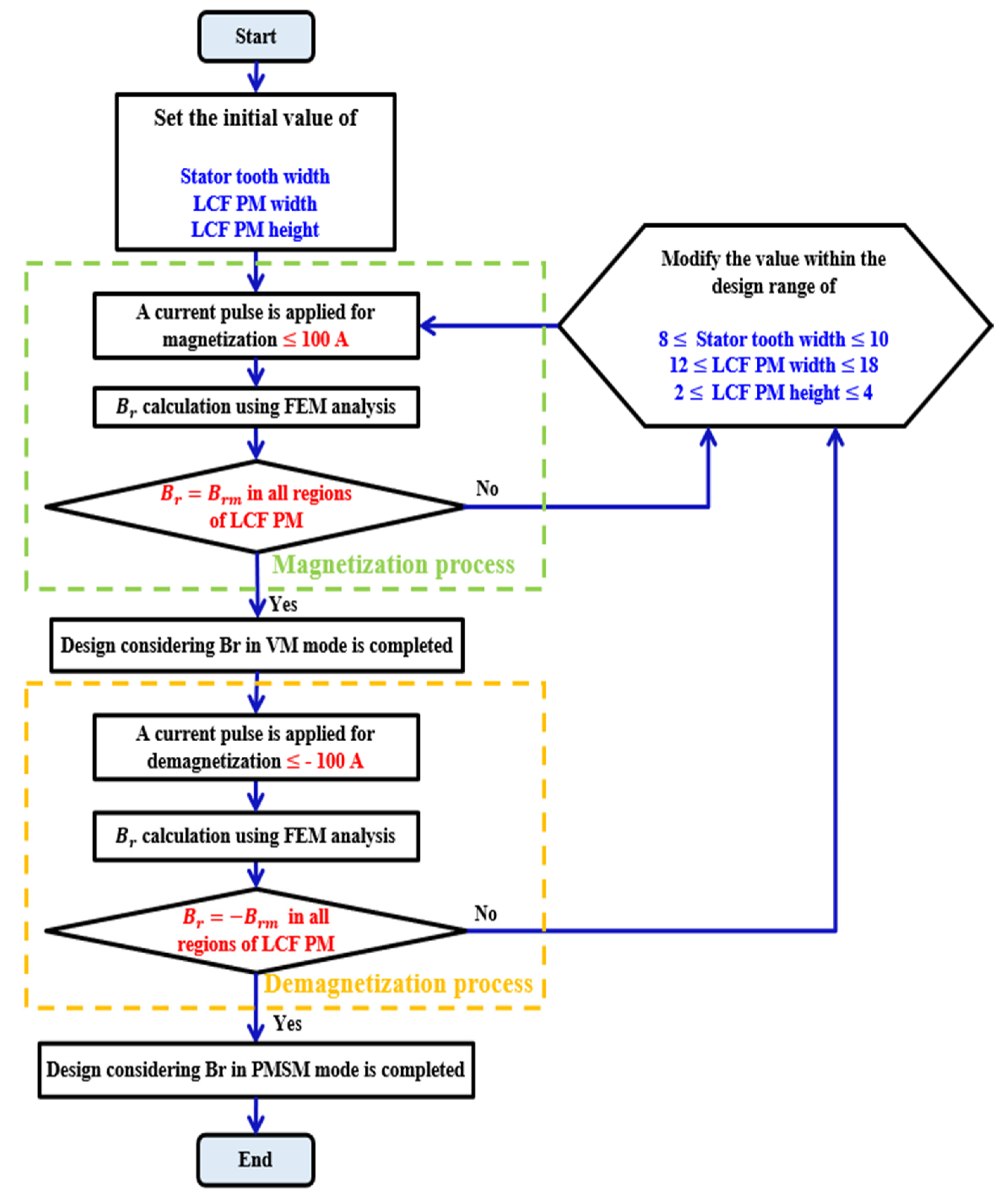
3.2. Selection and Range of Design Parameters

Designing with consideration of the B r of LCF PM during the pole-changing process can improve efficiency by achieving B r m in VM mode and − B r m in PMSM mode. As seen in Figure 3, a current pulse is applied to achieve | B r m |. However, the magnitude of the current pulses is limited by the inverter capacity. For the research objective of high efficiency over a wide speed range in applications such as washing machines and EVs, an inverter capacity of 55 kW was adopted in this study. The magnitude of the current pulses is <100 A due to current limitations imposed by the inverter capacity.
To obtain B r m or − B r m within the limited magnitude of the current pulse, specific design parameters were chosen, and their ranges were determined. These design parameters were identified based on previous research [28], which investigated the impact of design parameter variations on the current pulse magnitude. The selected design parameters include stator tooth width, LCF PM width, and LCF PM height. The stator tooth width governs the flux path for current pulse magnetization and demagnetization, while the LCF PM width and height correspond to the regions where these processes occur. Consequently, increasing the stator tooth width and decreasing the LCF PM width and height lead to a reduction in the current pulse magnitude [28,29].
Figure 5 depicts the magnitude of the current pulse with variations in the design parameters. Feasible ranges for the design parameters were determined as shown on the x-axis in Figure 5a–c, considering manufacturing constraints, slot fill factor, and prevention of PM scattering. In Figure 5, the effect of a single design parameter on the current pulse magnitude is examined while keeping the other design parameters constant. For example, in Figure 5a, when the stator tooth width changes, the LCF PM width and height remain constant. The values at which the design parameters are kept constant are as follows: The stator tooth width is set to its maximum value of 10 mm, while the LCF PM width and height are set to their minimum values of 12 and 2 mm, respectively. These settings are chosen to minimize the current pulse magnitude, as explained earlier. Furthermore, since magnetization current pulses are typically larger than demagnetization pulses [30], the current pulses shown on the y-axis in Figure 5 represent the magnitude of the magnetization current pulse.
As a result, the range of the design parameters is determined based on a maximum current pulse magnitude of 100 A due to current limitations imposed by the inverter capacity. In Figure 5, a current pulse size of 100A has been represented by a red dashed line to indicate the limitation on current pulse magnitude. Additionally, current pulse magnitudes based on design parameters are depicted as blue dots. In Figure 5a, the design parameter range for the stator tooth width is determined as 8–10 mm, with the condition that the magnitude of the current pulses is less than 100 A. In Figure 5b, the design parameter range for the LCF PM width is determined as 12–18 mm, with the condition that the magnitude of the current pulses is less than 100 A. Additionally, in Figure 5c, the design parameter range for the LCF PM height is determined as 2–4 mm, with the condition that the magnitude of the current pulses is less than 100 A.

3.3. Determining the Final Values of Design Parameters

Figure 6 depicts a flowchart illustrating the process of determining the final values of the design parameters according to the pole-changing process and the range of the design parameters. In this process, as described in Section 2, the magnitude of the current pulses is limited, and the objective has been modified from achieving B r to B r m   for efficiency improvement.
The flowchart is explained in three parts: the magnetization process, the demagnetization process, and the determination of the final values of design parameters. Initially, the minimum value from the range of each design parameter is selected as the initial value. The stator tooth width is set to 8 mm, the LCF PM width is set to 12 mm, and the LCF PM height is set to 2 mm. Subsequently, the magnetization process is performed by applying a current pulse limited to 100 A. If any region of the LCF PM fails to achieve B r m , the values of the design parameters within their respective ranges are adjusted, and the magnetization process is repeated. Next, the demagnetization process is conducted by applying a current pulse limited to −100 A. If any region of the LCF PM fails to attain − B r m , the values of the design parameters are modified again within their respective ranges, and the magnetization process is repeated. Finally, a combination of design parameter values that satisfies both the magnetization and demagnetization processes is derived. The combination of design parameter values that achieves the highest efficiency in each mode is selected from the derived combinations. In this manner, the design for the VM and PMSM modes with the highest efficiency is completed in the PCVM. This design, considering B r , improves the efficiency of each mode, enabling high efficiency over a wide speed range.
The final values of the design parameters for the conventional and improved models are summarized in Table 2.

4. Performance Evaluation

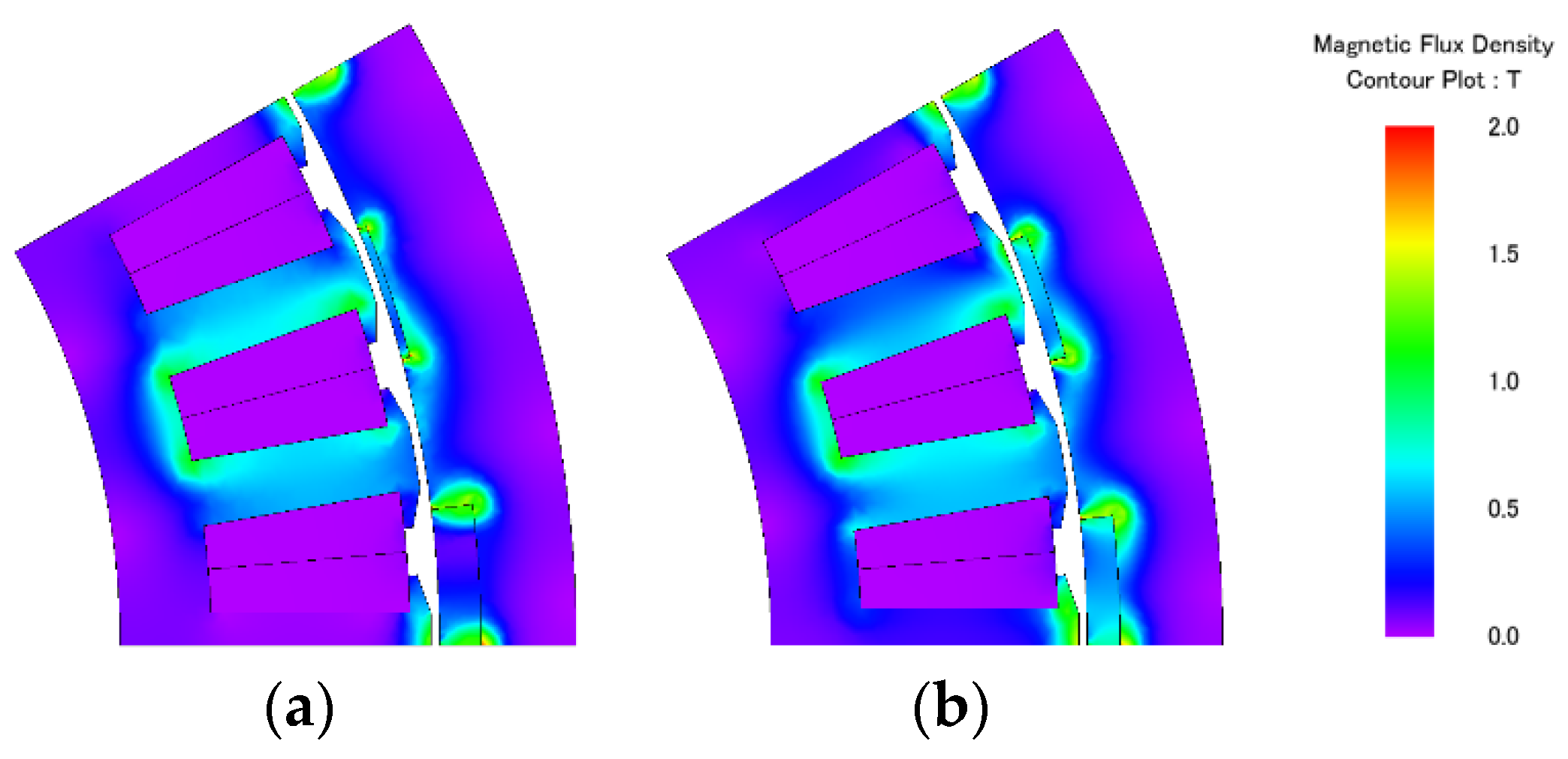
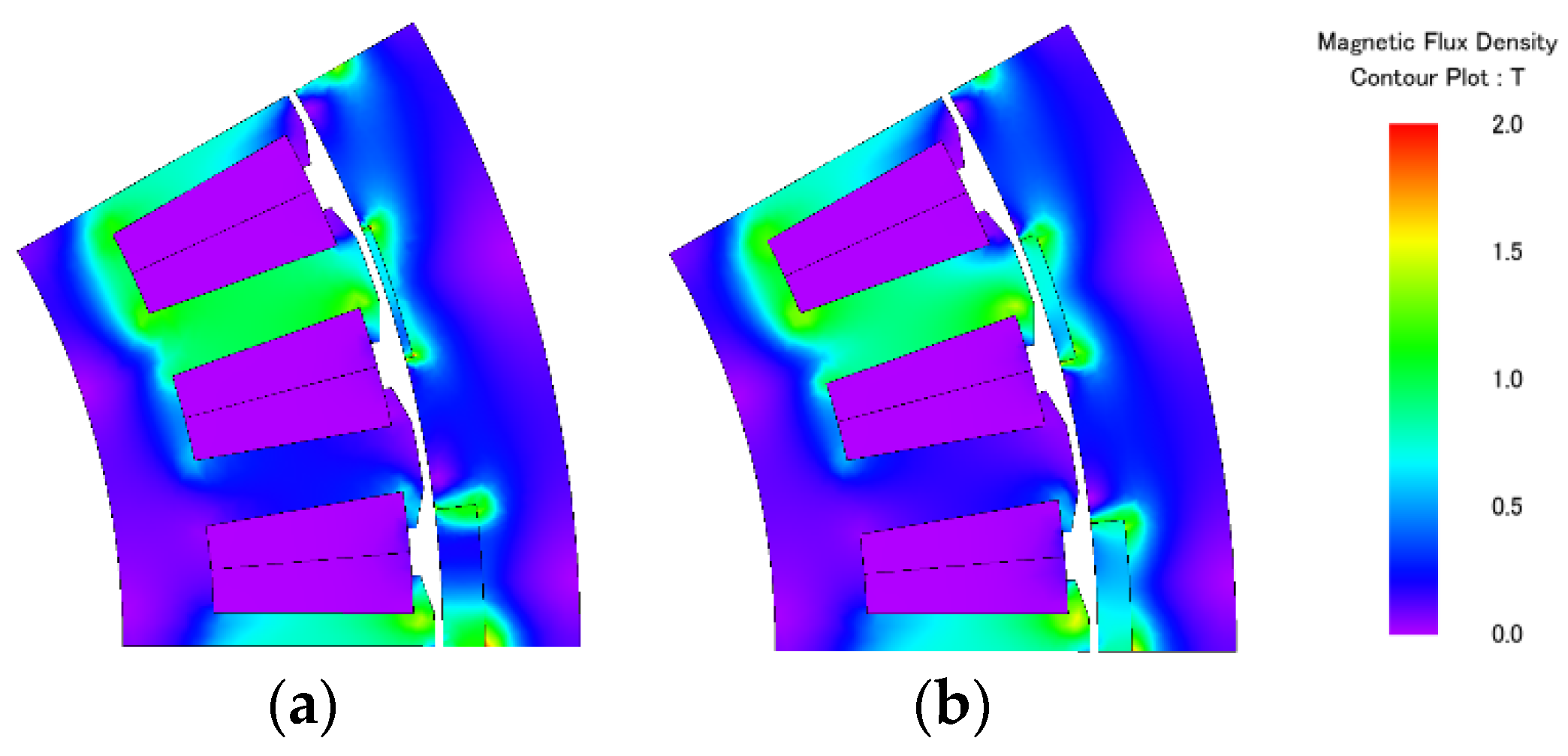
4.1. Confirmation of B r m with the Pole-Changing Process

To confirm that B r of the improved model’s LCF PM obtains B r m in VM mode and − B r m in PMSM mode, FEA was performed using JMAG Version 22 to apply a current pulse of the pole-changing process and calculate B r in each mode. It has been verified in numerous studies [31,32] that the commercial software JMAG provides values that are highly consistent with experimental results.
The LCF PMs are located as indicated in Figure 7a. Furthermore, a current pulse is applied to magnetize the LCF PMs in the opposite direction, resulting in a change in the magnetic flux direction of all LCF PMs. The B r value of the LCF PM changes along the magnetization process, as shown by the green line on the B−H curve in Figure 4, where its value is “ 0 < B r ≤ B r m ”. Consequently, as shown in Figure 7b, the machine operates in VM mode. The LCF PMs move at a mechanical angle of 15° and then are fixed, as shown in Figure 7c. Moreover, a current pulse is applied for demagnetization in the opposite direction to the magnetization of the LCF PMs, changing the Then, a current pulse is applied for demagnetization in the opposite direction to the magnetic flux direction of the LCF PMs. The B r value of the LCF PM changes along the demagnetization process, as shown by the orange line on the B−H curve in Figure 4, where its value is “ − B r m ≤ B r < 0 ”. Therefore, as shown in Figure 7d, the machine operates in PMSM mode.
As shown in Figure 7a,c, a magnetic field of approximately 0.7 T affects all regions of the LCF PM in the magnetic flux density distribution. Therefore, it was confirmed that the design considering B r for efficiency improvement was completed since the LCF PM has B r m in the VM mode and − B r m in the PMSM mode. Furthermore, it can be confirmed that the magnetic flux direction of the LCF PMs has changed by observing the alteration of the flux lines depicted in Figure 7b,d. Therefore, the number of rotor pole pairs changes, confirming that pole-changing is complete.

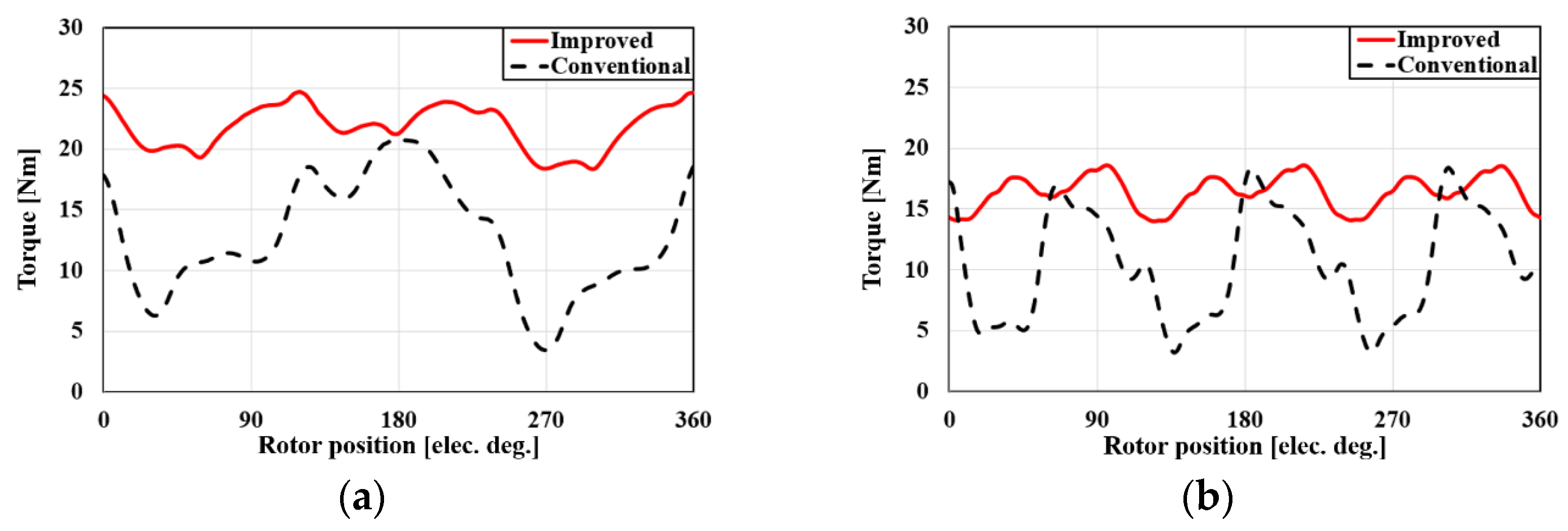
4.2. Comparison of Performances

The performance of the improved model designed considering the B r of LCF PM was compared with the conventional model designed without considering the B r of LCF PM. The B−EMF comparison of the conventional and improved models of the PCVM during VM and PMSM modes is shown in Figure 8. Figure 8a shows that B−EMF in VM mode is 51.6 V r m s in the conventional model and 77.5 V r m s in the improved model. Figure 8b shows that B−EMF in PMSM mode is 40.7 V r m s in the conventional model and 58.3 V r m s in the improved model. Therefore, the B−EMF of each mode was improved by design, considering the B r of LCF PM.
The output torque comparison of the conventional and improved models of the PCVM during VM and PMSM modes is shown in Figure 9. Figure 9a shows that the torque in VM mode is 12.7 Nm in the conventional model and 21.8 Nm in the improved model. Figure 9b shows that the torque in PMSM mode is 10.5 Nm in the conventional model and 16.4 Nm in the improved model. Therefore, the torque of each mode was improved by design, considering the B r of LCF PM.
To compare the magnetic flux density distribution in the VM mode of the improved model shown in Figure 7b with the conventional model, it is presented in Figure 10. Figure 10a shows that the LCF PM could not obtain B r m because the design considering the B r of LCF PM was not performed. Figure 10b shows that the LCF PM has a high magnetic flux density by obtaining a B r m because the design considering the B r of LCF PM was performed.
To compare the magnetic flux density distribution in the PMSM mode of the improved model shown in Figure 7d with the conventional model, it is presented in Figure 11. Figure 11a shows that the LCF PM could not obtain a − B r m because the design considering the B r of LCF PM was not performed. Figure 11b shows that the LCF PM has a high magnetic flux density by obtaining a − B r m because the design considering the B r of LCF PM was performed.
To confirm the efficiency improvement through the design, considering the B r of LCF PM, the efficiency of each mode is calculated. The efficiency is calculated using Equation (3).
η = P o u t P o u t + P c + P i × 100 %
where P o u t ,   P c   , and P i are the output power, copper loss, and iron loss, respectively. The efficiency of the VM mode calculated using Equation (3) is 86.9% for the conventional model and 90.7% for the improved model. Furthermore, the PMSM mode is 92.5% for the conventional model and 94.9% for the improved model.
Table 3 presents a performance comparison of the conventional and improved models. As shown in Figure 8 and Figure 9, it is observed that the improved model for each mode shows enhanced performance compared to the conventional model under the same operating conditions. The improved model achieved higher torque density by obtaining B r m in the VM mode and − B r m in the PMSM mode, considering the design with B r . Moreover, when operating in PMSM mode, the number of rotor pole pairs is reduced by half compared to VM mode due to pole-changing. This reduction in pole pairs leads to a decrease in iron losses, thereby enhancing efficiency at high speeds. The improved model and the conventional model have the same current conditions, so they have the same copper losses. In the improved model, as a result of considering B r in the design process, there was a slight increase in iron losses due to the increase in stator tooth width compared to the conventional model. Nevertheless, the substantial increase in B r significantly enhances torque density. Therefore, the efficiency of the improved model is enhanced by having a high magnetic flux density in each mode as the design considering the B r of LCF PM is performed.

5. Conclusions

In this study, a design considering the B r of LCF PMs was used to improve the efficiency of the PCVM. To verify the efficiency improvement, the efficiencies of the improved model, designed considering the B r of the LCF PM, and the conventional model, designed without considering the B r of the LCF PM, were compared in each mode. In VM mode, the efficiency of the conventional model was 86.9%, while the efficiency of the improved model was 90.7%. This represents a 3.8% increase in efficiency compared to the conventional model. In PMSM mode, the efficiency of the conventional model was 92.5%, while that of the improved model was 94.9%. This corresponds to a 2.4% increase in efficiency compared to the conventional model.
The improved model, in the design process that considered B r , resulted in a slight increase in iron losses compared to the conventional model due to the increase in the stator tooth width. However, the substantial increase in B r significantly enhances torque density. The improved model increased torque density by obtaining B r m in VM mode and − B r m in PMSM mode through the design considering B r . When operating in PMSM mode, the number of rotor pole pairs is reduced by half compared to VM mode due to pole-changing. This reduction in pole pairs decreases the frequency at high speeds, resulting in reduced iron losses and further enhancing efficiency. Therefore, PCVM achieved high torque in VM mode at low speeds and high efficiency in PMSM mode at high speeds, owing to the design considerations of LCF PM’s B r . This improved efficiency across a wide speed range.
PCVM has been proposed previously, but research on this relatively new structure has not been extensively conducted. This study has contributed to improving the efficiency of PCVM by carefully considering the characteristics of LCF PM. PCVM used only two operating points: low speed and high speed. Future research can expand into applications that utilize multiple loads in wide speed ranges, similar to EVs, allowing for performance improvement.

Author Contributions

S.-H.L. conceived the initial idea, performed the model design, analyzed the data, and wrote this paper; J.-W.K. analyzed the model and assisted with English correction; B.-I.K. supervised the overall research. All authors have read and agreed to the published version of the manuscript.

Funding

This work was supported by the National Research Foundation of Korea (NRF) grant funded by the Korean government (MSIT) (No. NRF-2020R1A2B5B01002400).

Data Availability Statement

The data that support the findings of this study are available on request from the corresponding author, B.-I.K., upon reasonable request.

Conflicts of Interest

The authors declare no conflict of interest.

References

  1. Fang, L.; Jung, J.W.; Hong, J.P.; Lee, J.H. Study on High-Efficiency Performance in Interior Permanent-Magnet Synchronous Motor with Double-Layer PM Design. IEEE Trans. Magn. 2008, 44, 4393–4396. [Google Scholar] [CrossRef]
  2. Ba, X.; Gong, Z.; Guo, Y.; Zhang, C.; Zhu, J. Development of Equivalent Circuit Models of Permanent Magnet Synchronous Motors Considering Core Loss. Energies 2022, 15, 1995. [Google Scholar] [CrossRef]
  3. Hou, L.; Ma, J.; Wang, W. Sliding Mode Predictive Current Control of Permanent Magnet Synchronous Motor with Cascaded Variable Rate Sliding Mode Speed Controller. IEEE Access 2022, 10, 33992–34002. [Google Scholar] [CrossRef]
  4. Tapia, J.; Leonardi, F.; Lipo, T. Consequent-pole permanent-magnet machine with extended field-weakening capability. IEEE Trans. Ind. Appl. 2003, 39, 1704–1709. [Google Scholar] [CrossRef]
  5. Kwon, J.-W.; Kwon, B.-I. High-Efficiency Dual Output Stator-PM Machine for the Two-Mode Operation of Washing Machines. IEEE Trans. Energy Convers. 2018, 33, 2050–2059. [Google Scholar] [CrossRef]
  6. Baloch, N.; Kwon, J.-W.; Ayub, M.; Kwon, B.-I. Low-Cost Dual-Mechanical-Port Dual-Excitation Machine for Washing Machine Application. IEEE Access 2019, 7, 87141–87149. [Google Scholar] [CrossRef]
  7. Toba, A.; Lipo, T.A. Generic torque-maximizing design methodology of surface permanent-magnet vernier machine. IEEE Trans. Ind. Appl. 2000, 36, 1539–1546. [Google Scholar] [CrossRef]
  8. Kim, B.; Lipo, T.A. Analysis of a PM Vernier Motor with Spoke Structure. IEEE Trans. Ind. Appl. 2015, 52, 217–225. [Google Scholar] [CrossRef]
  9. Kim, B. Characteristic analysis of a vernier PM motor considering adjustable speed control. In Proceedings of the 2016 IEEE Transportation Electrification Conference and Expo, Asia-Pacific (ITEC Asia-Pacific), Busan, Republic of Korea, 1–4 June 2016; pp. 671–676. [Google Scholar] [CrossRef]
  10. Zou, T.; Li, D.; Qu, R.; Jiang, D.; Li, J. Advanced High Torque Density PM Vernier Machine with Multiple Working Harmonics. IEEE Trans. Ind. Appl. 2017, 53, 5295–5304. [Google Scholar] [CrossRef]
  11. Du, K.; Xu, L.; Zhao, W.; Liu, G. Analysis and Design of a Fault-Tolerant Permanent Magnet Vernier Machine with Improved Power Factor. IEEE Trans. Ind. Electron. 2021, 69, 4353–4363. [Google Scholar] [CrossRef]
  12. Zhao, W.; Sun, X.; Ji, J.; Liu, G. Design and Analysis of New Vernier Permanent-Magnet Machine with Improved Torque Capability. IEEE Trans. Appl. Supercond. 2016, 26, 1–5. [Google Scholar] [CrossRef]
  13. Xu, L.; Zhao, W.; Liu, G.; Song, C. Design Optimization of a Spoke-Type Permanent-Magnet Vernier Machine for Torque Density and Power Factor Improvement. IEEE Trans. Veh. Technol. 2019, 68, 3446–3456. [Google Scholar] [CrossRef]
  14. Wang, H.; Fang, S.; Yang, H.; Lin, H.; Li, Y.; Qin, L.; Zhou, Y. Loss Calculation and Temperature Field Analysis of Consequent-Pole Hybrid Excited Vernier Machine. IEEE Trans. Magn. 2017, 53, 1–5. [Google Scholar] [CrossRef]
  15. Zhao, F.; Kim, M.-S.; Kwon, B.-I.; Baek, J.-H. A Small Axial-Flux Vernier Machine with Ring-Type Magnets for the Auto-Focusing Lens Drive System. IEEE Trans. Magn. 2016, 52, 1–4. [Google Scholar] [CrossRef]
  16. Ostovic, V. Memory motors-a new class of controllable flux PM machines for a true wide speed operation. In Proceedings of the Conference Record of the 2001 IEEE Industry Applications Conference. 36th IAS Annual Meeting (Cat. No.01CH37248), Chicago, IL, USA, 30 September–4 October 2002; Volume 4, pp. 2577–2584. [Google Scholar] [CrossRef]
  17. Ostovic, V. Pole-changing permanent-magnet machines. IEEE Trans. Ind. Appl. 2002, 38, 1493–1499. [Google Scholar] [CrossRef]
  18. Yang, H.; Zhu, Z.Q.; Lin, H.; Wu, D.; Hua, H.; Fang, S.; Huang, Y.-K. Novel High-Performance Switched Flux Hybrid Magnet Memory Machines with Reduced Rare-Earth Magnets. IEEE Trans. Ind. Appl. 2016, 52, 3901–3915. [Google Scholar] [CrossRef]
  19. Yang, H.; Zheng, H.; Lin, H.; Zhu, Z.-Q.; Fu, W.; Liu, W.; Lei, J.; Lyu, S. Investigation of Hybrid-Magnet-Circuit Variable Flux Memory Machines with Different Hybrid Magnet Configurations. IEEE Trans. Ind. Appl. 2020, 57, 340–351. [Google Scholar] [CrossRef]
  20. Baloch, N.; Kwon, B.-I. A pole changing vernier machine with consequent pole rotor. Int. J. Appl. Electromagn. Mech. 2019, 59, 931–941. [Google Scholar] [CrossRef]
  21. Lee, S.-H.; Baloch, N.; Kwon, B.-I. Design and analysis of a double consequent pole changing vernier machine. Int. J. Appl. Electromagn. Mech. 2020, 64, 941–949. [Google Scholar] [CrossRef]
  22. Baloch, N.; Atiq, S.; Kwon, B.-I. A Wound-Field Pole-Changing Vernier Machine for Electric Vehicles. IEEE Access 2020, 8, 91865–91875. [Google Scholar] [CrossRef]
  23. Baloch, N.; Kwon, B.-I. A Distributed Winding Wound Field Pole-Changing Vernier Machine for Variable Speed Application. IEEE Trans. Manag. 2019, 55, 1–6. [Google Scholar] [CrossRef]
  24. Maekawa, S.; Yuki, K.; Matsushita, M.; Nitta, I.; Hasegawa, Y.; Shiga, T.; Hosoito, T.; Nagai, K.; Kubota, H. Study of the Magnetization Method Suitable for Fractional-Slot Concentrated-Winding Variable Magnetomotive-Force Memory Motor. IEEE Trans. Power Electron. 2013, 29, 4877–4887. [Google Scholar] [CrossRef]
  25. Asgar, M.; Afjei, E.; Behbahani, A.; Siadatan, A. A 12/8 double-stator switched reluctance motor for washing machine application. In Proceedings of the The 6th Power Electronics, Drive Systems & Technologies Conference (PEDSTC2015), Tehran, Iran, 3–4 February 2015; pp. 168–172. [Google Scholar] [CrossRef]
  26. Li, F.; Chau, K.T.; Liu, C. Pole-Changing Flux-Weakening DC-Excited Dual-Memory Machines for Electric Vehicles. IEEE Trans. Energy Convers. 2015, 31, 27–36. [Google Scholar] [CrossRef]
  27. Chung, S.-U.; Moon, S.-H.; Kim, D.-J.; Kim, J.-M. Development of a 20-Pole–24-Slot SPMSM with Consequent Pole Rotor for In-Wheel Direct Drive. IEEE Trans. Ind. Electron. 2015, 63, 302–309. [Google Scholar] [CrossRef]
  28. Ibrahim, M.; Masisi, L.; Pillay, P. Design of Variable Flux Permanent-Magnet Machine for Reduced Inverter Rating. IEEE Trans. Ind. Appl. 2015, 51, 3666–3674. [Google Scholar] [CrossRef]
  29. Limsuwan, N.; Kato, T.; Akatsu, K.; Lorenz, R.D. Design and Evaluation of a Variable-Flux Flux-Intensifying Interior Permanent-Magnet Machine. IEEE Trans. Ind. Appl. 2014, 50, 1015–1024. [Google Scholar] [CrossRef]
  30. Hua, H.; Zhu, Z.Q.; Pride, A.; Deodhar, R.P.; Sasaki, T. A Novel Variable Flux Memory Machine with Series Hybrid Magnets. IEEE Trans. Ind. Appl. 2017, 53, 4396–4405. [Google Scholar] [CrossRef]
  31. Jandaghi, B.; Dinavahi, V. Prototyping of Nonlinear Time-Stepped Finite Element Simulation for Linear Induction Machines on Parallel Reconfigurable Hardware. IEEE Trans. Ind. Electron. 2017, 64, 7711–7720. [Google Scholar] [CrossRef]
  32. Yang, H.; Lin, H.; Zhu, Z.Q.; Wang, D.; Fang, S.; Huang, Y. A Variable-Flux Hybrid-PM Switched-Flux Memory Machine for EV/HEV Applications. IEEE Trans. Ind. Appl. 2016, 52, 2203–2214. [Google Scholar] [CrossRef]
Energies 16 06707 g001
Figure 1. Structure of PCVM.
Figure 1. Structure of PCVM.
Energies 16 06707 g001
Energies 16 06707 g002
Figure 2. Magnetic flux direction of PMs: (a) VM mode; (b) PMSM mode.
Figure 2. Magnetic flux direction of PMs: (a) VM mode; (b) PMSM mode.
Energies 16 06707 g002
Energies 16 06707 g003
Figure 3. Flowchart of the pole-changing process.
Figure 3. Flowchart of the pole-changing process.
Energies 16 06707 g003
Energies 16 06707 g004
Figure 4. B−H curve of LCF PM.
Figure 4. B−H curve of LCF PM.
Energies 16 06707 g004
Energies 16 06707 g005
Figure 5. Magnitude of current pulse with variation in (a) stator tooth width, (b) LCF PM width, and (c) LCF PM height.
Figure 5. Magnitude of current pulse with variation in (a) stator tooth width, (b) LCF PM width, and (c) LCF PM height.
Energies 16 06707 g005
Energies 16 06707 g006
Figure 6. Flowchart for determining the final values of design parameters according to the pole-changing process and the range of the design parameters.
Figure 6. Flowchart for determining the final values of design parameters according to the pole-changing process and the range of the design parameters.
Energies 16 06707 g006
Energies 16 06707 g007
Figure 7. Magnetic flux density distribution and flux line according to the pole-changing process of the improved model: (a) PMSM mode to VM mode; (b) VM mode; (c) VM mode to PMSM mode; and (d) PMSM mode.
Figure 7. Magnetic flux density distribution and flux line according to the pole-changing process of the improved model: (a) PMSM mode to VM mode; (b) VM mode; (c) VM mode to PMSM mode; and (d) PMSM mode.
Energies 16 06707 g007
Energies 16 06707 g008
Figure 8. Comparison of the B−EMF of the conventional model and the improved model: (a) VM mode and (b) PMSM mode.
Figure 8. Comparison of the B−EMF of the conventional model and the improved model: (a) VM mode and (b) PMSM mode.
Energies 16 06707 g008
Energies 16 06707 g009
Figure 9. Comparison of the output torque of the conventional model and the improved model: (a) VM mode and (b) PMSM mode.
Figure 9. Comparison of the output torque of the conventional model and the improved model: (a) VM mode and (b) PMSM mode.
Energies 16 06707 g009
Energies 16 06707 g010
Figure 10. Comparison of the magnetic flux density distribution of the VM mode: (a) conventional model and (b) improved model.
Figure 10. Comparison of the magnetic flux density distribution of the VM mode: (a) conventional model and (b) improved model.
Energies 16 06707 g010
Energies 16 06707 g011
Figure 11. Comparison of the magnetic flux density distribution of the PMSM mode: (a) conventional model and (b) improved model.
Figure 11. Comparison of the magnetic flux density distribution of the PMSM mode: (a) conventional model and (b) improved model.
Energies 16 06707 g011
Table 1. Design specifications of conventional model.
Table 1. Design specifications of conventional model.
ItemUnitValue
Rotor outer diametermm300
Stator outer diametermm265
Air gap lengthmm1
Number of slots--36
Rotor pole pairs (VM mode)--24
Rotor pole pairs (PMSM mode)--12
HCF/LCF PM coercivitykA/m650/318
HCF/LCF PM remanenceT0.87/0.7
Table 2. Final values of the design parameters.
Table 2. Final values of the design parameters.
ParameterUnitConventionalImproved
Stator tooth widthmm79
LCF PM widthmm1716
LCF PM heightmm54
Table 3. Performance comparison of conventional and improved model.
Table 3. Performance comparison of conventional and improved model.
ItemUnitConventionalImproved
Machine mode -- VMPMSMVMPMSM
Speedrpm300
B−EMFV51.640.877.558.3
Terminal voltageV104.265.6141.486.8
Average torqueNm12.710.521.816.4
Copper lossW6.96.96.96.9
Iron lossW53.419.862.920.7
Efficiency%86.992.590.794.9
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

Share and Cite

MDPI and ACS Style

Lee, S.-H.; Kwon, J.-W.; Kwon, B.-I. Improving Efficiency of a Pole-Changing Vernier Machine Considering Residual Magnetic Flux Density. Energies 2023, 16, 6707. https://doi.org/10.3390/en16186707

AMA Style

Lee S-H, Kwon J-W, Kwon B-I. Improving Efficiency of a Pole-Changing Vernier Machine Considering Residual Magnetic Flux Density. Energies. 2023; 16(18):6707. https://doi.org/10.3390/en16186707

Chicago/Turabian Style

Lee, Sung-Hyun, Jung-Woo Kwon, and Byung-Il Kwon. 2023. "Improving Efficiency of a Pole-Changing Vernier Machine Considering Residual Magnetic Flux Density" Energies 16, no. 18: 6707. https://doi.org/10.3390/en16186707

APA Style

Lee, S. -H., Kwon, J. -W., & Kwon, B. -I. (2023). Improving Efficiency of a Pole-Changing Vernier Machine Considering Residual Magnetic Flux Density. Energies, 16(18), 6707. https://doi.org/10.3390/en16186707

Note that from the first issue of 2016, this journal uses article numbers instead of page numbers. See further details here.

Article Metrics

Back to TopTop