Optimal Generation Capacity Allocation and Droop Control Design for Current Sharing in DC Microgrids †
Abstract
:1. Introduction
1.1. Background and Motivation
1.2. Literature Review and Research Gaps
1.3. Contributions
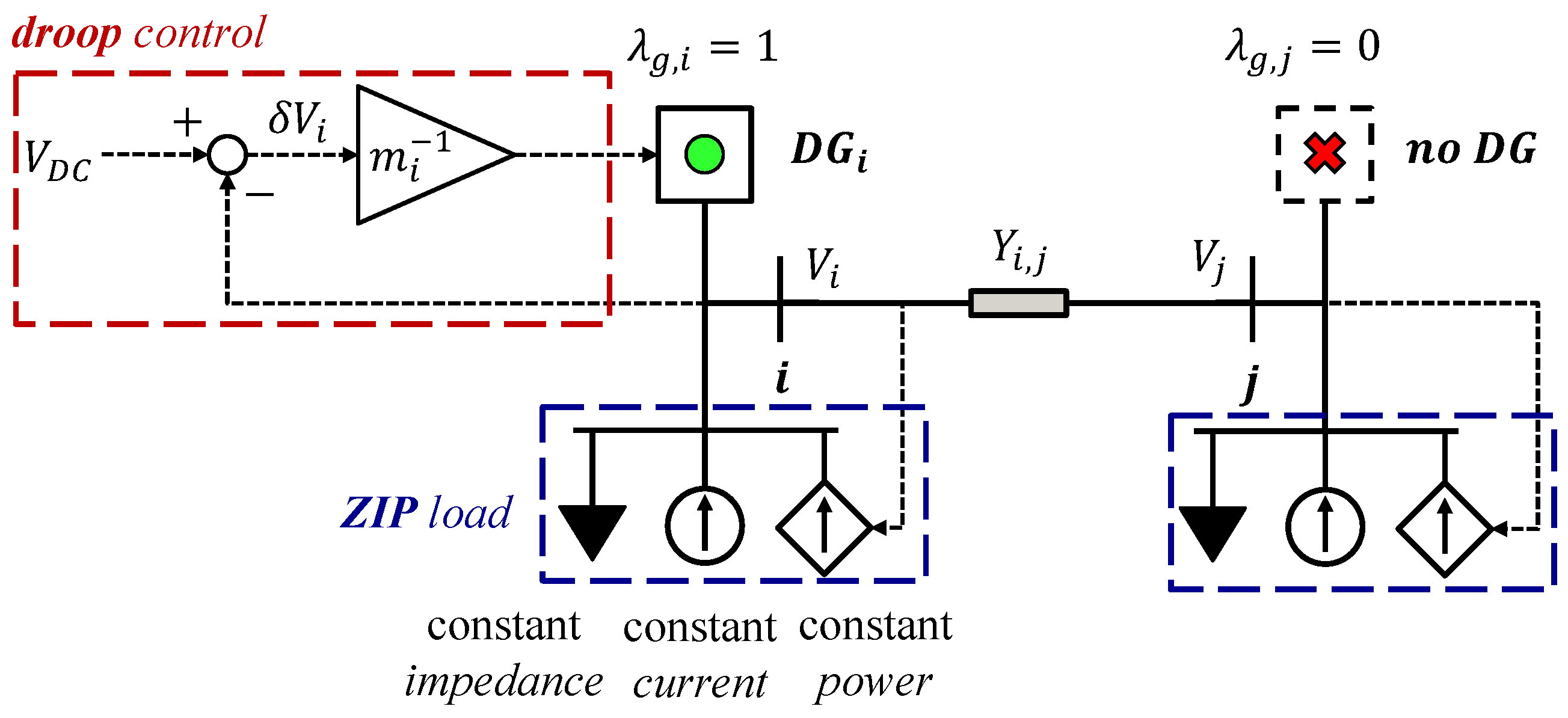
2. Simultaneous Optimal DG Placement Strategy and Droop Design Methodology
2.1. Optimal DG Allocation and Control Design as a Mixed-Integer Linear Programming Problem
2.1.1. Objective Function Formulation
2.1.2. DG Capacity Allocation Modeling through Linear Inequalities
2.1.3. Microgrid Operation and Power Flow Modeling for Mixed-Integer Formulations
2.1.4. Non-Linear Terms and Globally Valid Approximations
2.1.5. DGs Availability Constraint
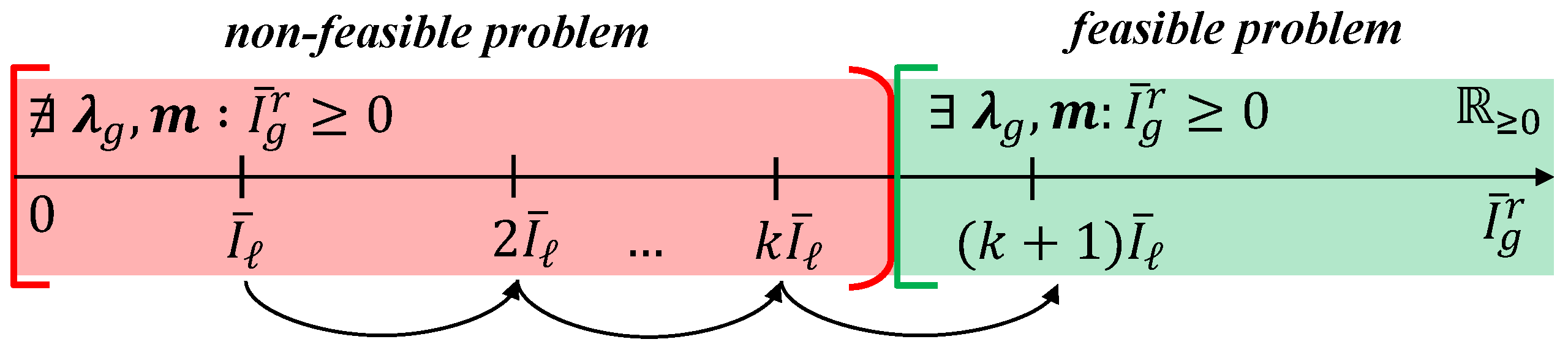
2.1.6. Constraints Related to Aggregated Generation Capacity
3. Results of Optimal and Concurrent DG Capacity Allocation and Droop Design
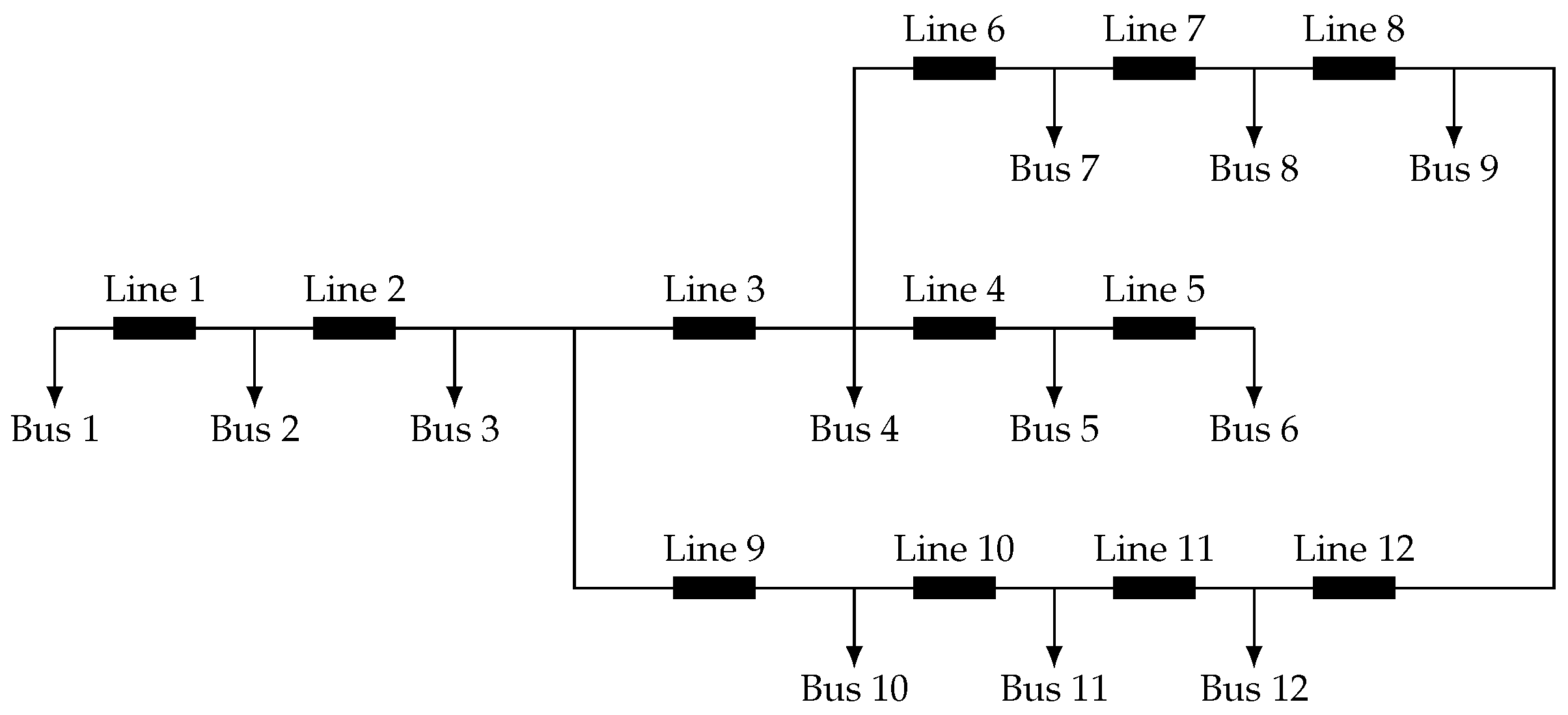
3.1. DC Microgrid Case Study
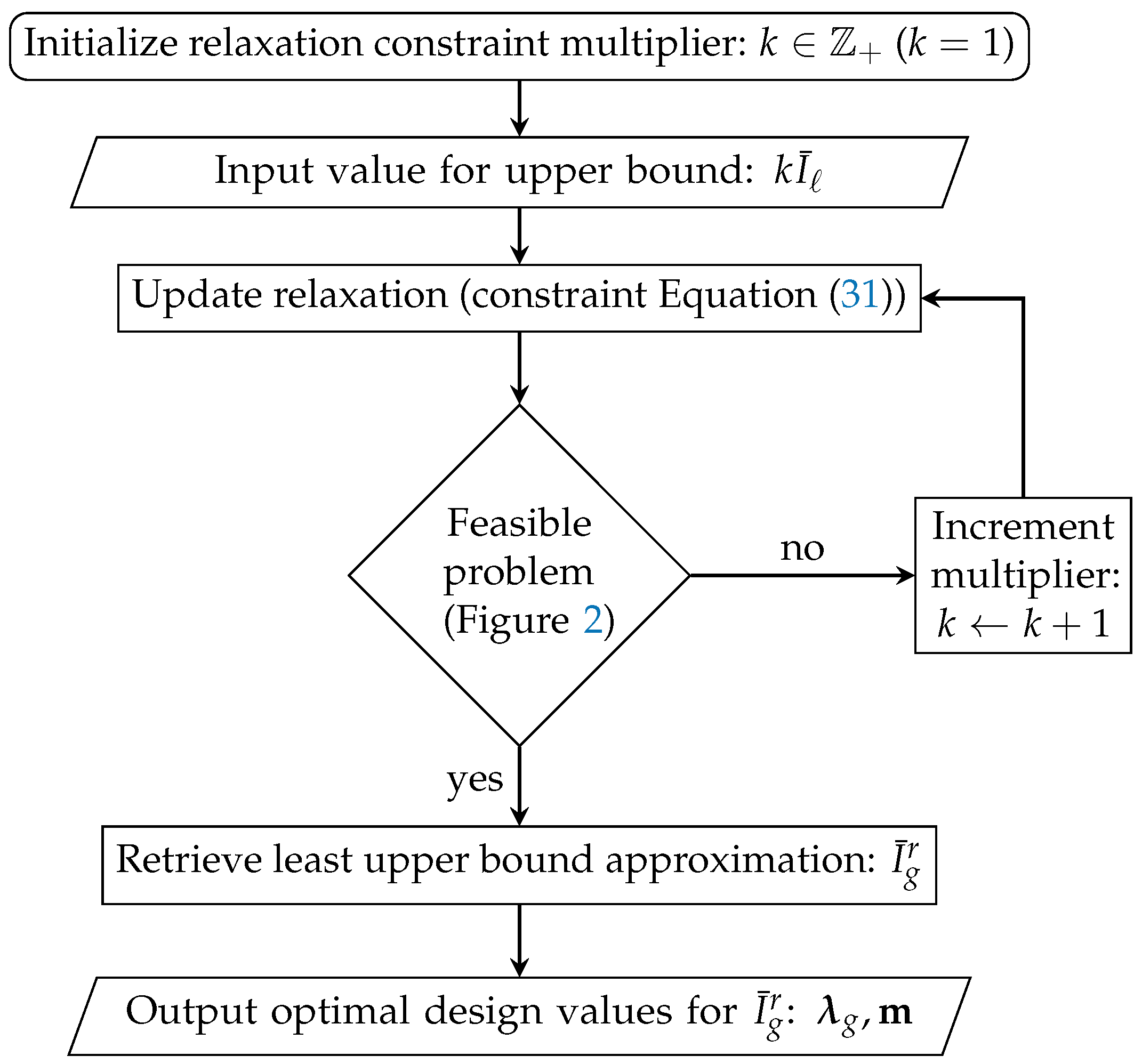
3.2. Problem Solution Approach
3.2.1. DG Availability and Related Grid Patterns
3.2.2. Optimal Droop Control Design
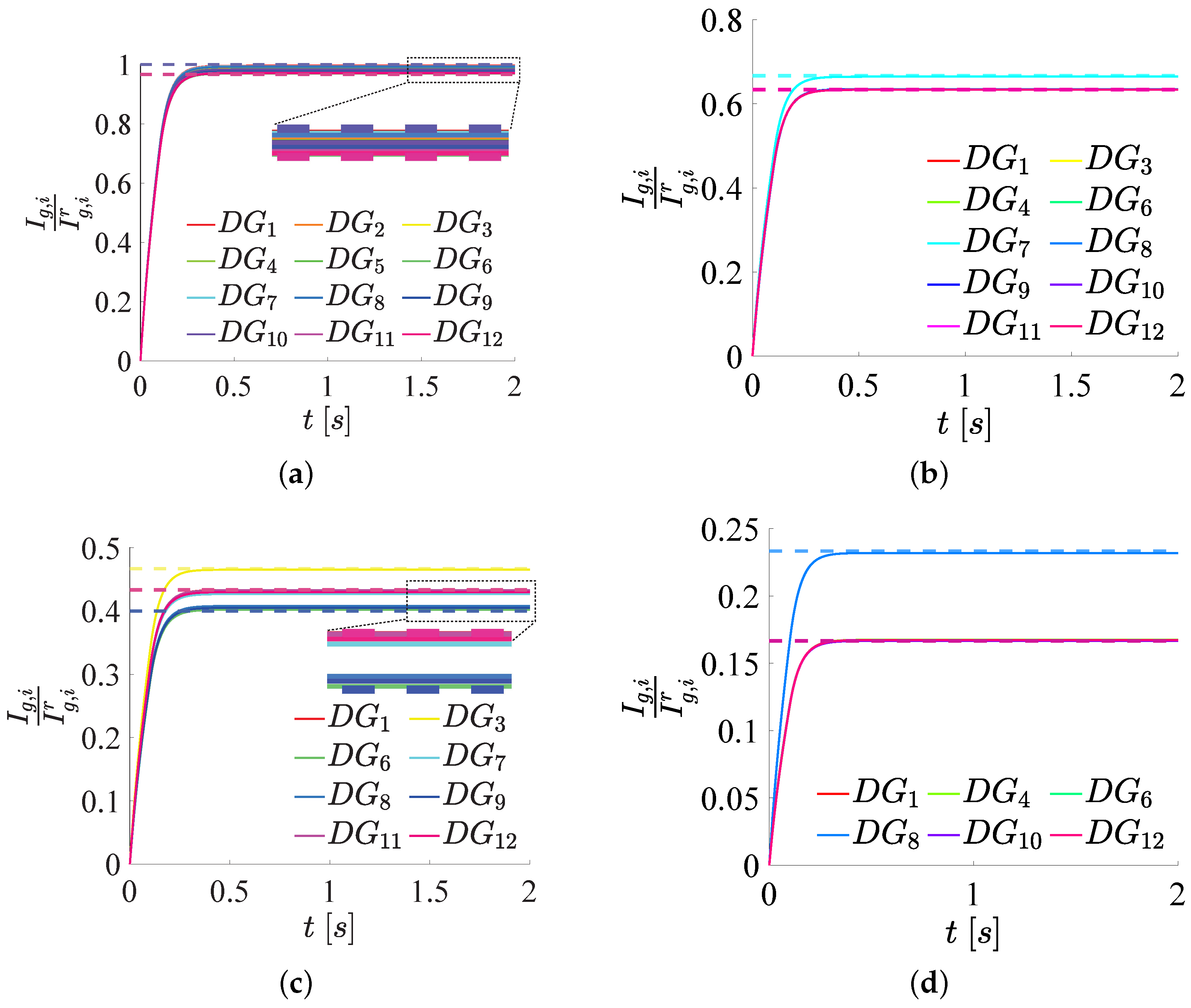
3.3. Verification of the Optimal Design with Time Domain Simulations
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
- The following abbreviations are used in this manuscript:
| DG | Distributed generator |
| MG | Microgrid |
| RES | Renewable energy source |
| ESS | Energy storage system |
| PCC | Point of common coupling |
| SOS | Special ordered set |
| LP | Linear programming |
| IP | Integer programming |
| MILP | Mixed-integer linear programming |
| MISOCP | Mixed-integer second-order optimization |
| MICVXO | Mixed-integer convex optimization |
| MetH | Meta-heuristic |
| TLBO | Teaching-learning-based optimization |
Appendix A
References
- Chapaloglou, S.; Alves, E.; Trovato, V.; Tedeschi, E. Optimal Energy Management in Autonomous Power Systems with Probabilistic Security Constraints and Adaptive Frequency Control. IEEE Trans. Power Syst. 2023, 1–12. [Google Scholar] [CrossRef]
- Ton, D.T.; Smith, M.A. The U.S. Department of Energy’s Microgrid Initiative. Electr. J. 2012, 25, 84–94. [Google Scholar] [CrossRef]
- Hatziargyriou, N.; Asano, H.; Iravani, R.; Marnay, C. Microgrids. IEEE Power Energy Mag. 2007, 5, 78–94. [Google Scholar] [CrossRef]
- Meng, L.; Shafiee, Q.; Trecate, G.F.; Karimi, H.; Fulwani, D.; Lu, X.; Guerrero, J.M. Review on Control of DC Microgrids and Multiple Microgrid Clusters. IEEE J. Emerg. Sel. Top. Power Electron. 2017, 5, 928–948. [Google Scholar]
- Al-Ismail, F.S. DC Microgrid Planning, Operation, and Control: A Comprehensive Review. IEEE Access 2021, 9, 36154–36172. [Google Scholar] [CrossRef]
- Lotfi, H.; Khodaei, A. AC versus DC Microgrid Planning. IEEE Trans. Smart Grid 2017, 8, 296–304. [Google Scholar] [CrossRef]
- Albea-Sanchez, C. Hybrid dynamical control based on consensus algorithm for current sharing in DC-bus microgrids. Nonlinear Anal. Hybrid Syst. 2021, 39, 100972. [Google Scholar] [CrossRef]
- Han, Y.; Ning, X.; Yang, P.; Xu, L. Review of Power Sharing, Voltage Restoration and Stabilization Techniques in Hierarchical Controlled DC Microgrids. IEEE Access 2019, 7, 149202–149223. [Google Scholar] [CrossRef]
- Abdolmaleki, B.; Shafiee, Q.; Sadabadi, M.S.; Dragicevic, T. Economical Secondary Control of DC Microgrids. In Proceedings of the 2020 IEEE 11th International Symposium on Power Electronics for Distributed Generation Systems (PEDG), Dubrovnik, Croatia, 28 September–1 October 2020; pp. 304–308. [Google Scholar] [CrossRef]
- Abdolmaleki, B.; Bergna-Diaz, G. Distributed Control and Optimization of DC Microgrids: A Port-Hamiltonian Approach. IEEE Access 2022, 10, 64222–64233. [Google Scholar] [CrossRef]
- Abdolmaleki, B.; Bergna-Diaz, G. A Nonlinear Control Framework for Optimal Load-Sharing and Voltage Containment in DC Networks. IEEE Trans. Power Syst. 2023, 38, 976–979. [Google Scholar] [CrossRef]
- Georgilakis, P.S.; Hatziargyriou, N.D. Optimal Distributed Generation Placement in Power Distribution Networks: Models, Methods, and Future Research. IEEE Trans. Power Syst. 2013, 28, 3420–3428. [Google Scholar] [CrossRef]
- Gupta, Y.; Doolla, S.; Chatterjee, K.; Pal, B.C. Optimal DG Allocation and Volt–Var Dispatch for a Droop-Based Microgrid. IEEE Trans. Smart Grid 2021, 12, 169–181. [Google Scholar] [CrossRef]
- Chapaloglou, S.; Abdolmaleki, B.; Tedeschi, E. Optimal Sizing and Placement of Droop-based Converters in DC Microgrids with ZIP Loads. In Proceedings of the 2022 Second International Conference on Sustainable Mobility Applications, Renewables and Technology (SMART), Virtual, 23–25 November 2022; pp. 1–8. [Google Scholar] [CrossRef]
- Iqbal, S.; Khan, H.A.; Nasir, M. Sizing of Low-Power DC Microgrids with Intermittent AC Grids in Developing Regions. In Proceedings of the 2019 IEEE Power Energy Society General Meeting (PESGM), Atlanta, GA, USA, 4–8 August 2019; pp. 1–5. [Google Scholar]
- Nasir, M.; Iqbal, S.; Khan, H.A. Optimal Planning and Design of Low-Voltage Low-Power Solar DC Microgrids. IEEE Trans. Power Syst. 2018, 33, 2919–2928. [Google Scholar] [CrossRef]
- Phurailatpam, C.; Rajpurohit, B.S.; Wang, L. Planning and optimization of autonomous DC microgrids for rural and urban applications in India. Renew. Sustain. Energy Rev. 2018, 82, 194–204. [Google Scholar] [CrossRef]
- Che, L.; Zhang, X.; Shahidehpour, M.; Alabdulwahab, A.; Al-Turki, Y. Optimal Planning of Loop-Based Microgrid Topology. IEEE Trans. Smart Grid 2017, 8, 1771–1781. [Google Scholar] [CrossRef]
- Wang, P.; Zhang, L.; Xu, D. Optimal Sizing of Distributed Generations in DC Microgrids with Lifespan Estimated Model of Batteries. In Proceedings of the 2018 21st International Conference on Electrical Machines and Systems (ICEMS), Jeju, Republic of Korea, 7–10 October 2018; pp. 2045–2049. [Google Scholar]
- Molina-Martin, F.; Montoya, O.D.; Grisales-Noreña, L.F.; Hernández, J.C. A Mixed-Integer Conic Formulation for Optimal Placement and Dimensioning of DGs in DC Distribution Networks. Electronics 2021, 10, 176. [Google Scholar] [CrossRef]
- Grisales-Noreña, L.F.; Montoya, O.D.; Ramos-Paja, C.A.; Hernandez-Escobedo, Q.; Perea-Moreno, A.J. Optimal Location and Sizing of Distributed Generators in DC Networks Using a Hybrid Method Based on Parallel PBIL and PSO. Electronics 2020, 9, 1808. [Google Scholar] [CrossRef]
- Zhou, J.; Xu, Z. Optimal sizing design and integrated cost-benefit assessment of stand-alone microgrid system with different energy storage employing chameleon swarm algorithm: A rural case in Northeast China. Renew. Energy 2023, 202, 1110–1137. [Google Scholar] [CrossRef]
- Wu, X.; Wang, Z.; Ding, T.; Li, Z. Hybrid AC/DC Microgrid Planning with Optimal Placement of DC Feeders. Energies 2019, 12, 1751. [Google Scholar] [CrossRef] [Green Version]
- Krishnamurthy, N.K.; Sabhahit, J.N.; Jadoun, V.K.; Gaonkar, D.N.; Shrivastava, A.; Rao, V.S.; Kudva, G. Optimal Placement and Sizing of Electric Vehicle Charging Infrastructure in a Grid-Tied DC Microgrid Using Modified TLBO Method. Energies 2023, 16, 1781. [Google Scholar] [CrossRef]
- Khon, K.; Chhlonh, C.; Vai, V.; Alvarez-Herault, M.C.; Raison, B.; Bun, L. Comprehensive Low Voltage Microgrid Planning Methodology for Rural Electrification. Sustainability 2023, 15, 2841. [Google Scholar] [CrossRef]
- Alanazi, A.; Lotfi, H.; Khodaei, A. Optimal Energy Storage Sizing and Siting in Hybrid AC/DC Microgrids. In Proceedings of the 2018 North American Power Symposium (NAPS), Fargo, ND, USA, 9–11 September 2018; pp. 1–6. [Google Scholar] [CrossRef]
- Jabr, R.A. Mixed-Integer Convex Optimization for DC Microgrid Droop Control. IEEE Trans. Power Syst. 2021, 36, 5901–5908. [Google Scholar] [CrossRef]
- Shi, L.; Liu, Q.; Shao, J.; Cheng, Y.; Zheng, W.X. A Cooperation-Competition Evolutionary Dynamic Model Over Signed Networks. IEEE Trans. Autom. Control 2023, 1–8. [Google Scholar] [CrossRef]
- Shi, L.; Li, W.; Shi, M.; Shi, K.; Cheng, Y. Opinion Polarization Over Signed Social Networks with Quasi Structural Balance. IEEE Trans. Autom. Control 2023, 1–8. [Google Scholar] [CrossRef]
- IEEE Std 1547-2018 (Revision of IEEE Std 1547-2003); IEEE Standard for Interconnection and Interoperability of Distributed Energy Resources with Associated Electric Power Systems Interfaces. IEEE: Piscataway Township, NJ, USA, 2018; pp. 1–138. [CrossRef]
- D’Ambrosio, C.; Lodi, A.; Martello, S. Piecewise linear approximation of functions of two variables in MILP models. Oper. Res. Lett. 2010, 38, 39–46. [Google Scholar] [CrossRef]
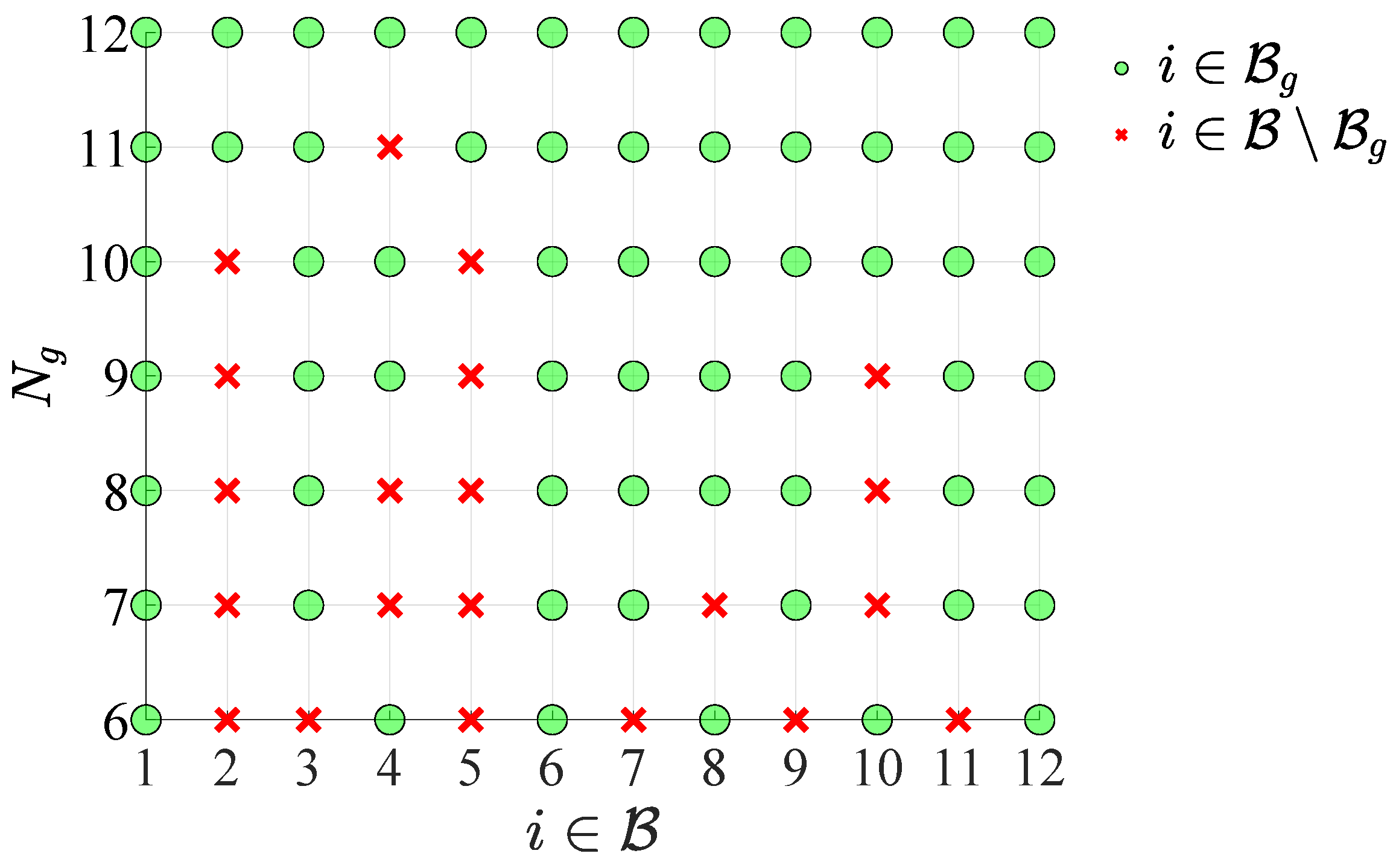

| Ref No. | Sizing | Placement | Droop Design | Method |
|---|---|---|---|---|
| [15] | ✓ | × | × | LP |
| [16] | ✓ | × | × | LP |
| [17] | ✓ | × | × | HOMER-based |
| [18] | ✓ | × | × | IP |
| [19] | ✓ | × | × | MetH |
| [22] | ✓ | × | × | MetH |
| [23] | × | ✓ | × | MILP |
| [24] | ✓ | ✓ | × | MetH |
| [25] | ✓ | ✓ | × | MILP + Clustering |
| [26] | ✓ | ✓ | × | MILP |
| [20] | ✓ | ✓ | × | MISOCP |
| [21] | ✓ | ✓ | × | MetH |
| [27] | × | × | ✓ | MICVXO |
| This Work | ✓ | ✓ | ✓ | MILP |
| Line / | ||||
|---|---|---|---|---|
| 1 | 0.250 | 0.300 | 2.00 | 39.0963 |
| 2 | 0.150 | 0.200 | 3.00 | 26.0642 |
| 3 | 0.200 | 0.250 | 5.00 | 32.5802 |
| 4 | 0.250 | 0.100 | 1.00 | 13.0321 |
| 5 | 0.300 | 0.150 | 1.50 | 19.5481 |
| 6 | 0.350 | 0.300 | 2.50 | 39.0963 |
| 7 | 0.150 | 0.400 | 3.00 | 52.1284 |
| 8 | 0.250 | 0.300 | 1.50 | 39.0963 |
| 9 | 0.400 | 0.200 | 5.00 | 26.0642 |
| 10 | 0.250 | 0.150 | 2.00 | 19.5481 |
| 11 | 0.350 | 0.300 | 2.00 | 39.0963 |
| 12 | 0.650 | 0.200 | 3.00 | 26.0642 |
| 1 | 218.60 | 218.60 | 1.000 | 0.1680 | 0.3497 | −0.3509 |
| 2 | 147.40 | 147.40 | 1.000 | 0.1680 | 0.3497 | 0.2578 |
| 3 | 185.50 | 191.90 | 0.967 | 0.1980 | −4 | |
| 4 | 73.20 | 73.20 | 1.000 | 0.5018 | 0.3497 | −0.3509 |
| 5 | 109.80 | 113.59 | 0.967 | 0.3345 | ||
| 6 | 219.10 | 226.66 | 0.967 | 0.1677 | ||
| 7 | 291.80 | 291.80 | 1.000 | 0.1259 | 0.3497 | −0.3509 |
| 8 | 218.10 | 218.10 | 1.000 | 0.1684 | 0.3497 | −0.3509 |
| 9 | 149.40 | 154.55 | 0.967 | 0.2459 | ||
| 10 | 110.30 | 110.30 | 1.000 | 0.3330 | 0.3497 | −0.3509 |
| 11 | 218.60 | 218.14 | 0.967 | 0.1680 | ||
| 12 | 147.40 | 152.48 | 0.967 | 0.2492 |
| 1 | 344.06 | 2.064 | 0.1667 | 0.0178 | 0.0541 | −0.0541 |
| 4 | 362.73 | 2.176 | 0.1667 | 0.0169 | 0.0541 | −0.0541 |
| 6 | 269.84 | 1.619 | 0.1667 | 0.0227 | 0.0541 | −0.0541 |
| 8 | 526.38 | 2.256 | 0.2333 | 0.0144 | 0.3236 | −0.3247 |
| 10 | 307.61 | 1.846 | 0.1667 | 0.0199 | 0.0541 | −0.0541 |
| 12 | 282.15 | 1.693 | 0.1667 | 0.0217 | 0.0541 | −0.0541 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chapaloglou, S.; Abdolmaleki, B.; Tedeschi, E. Optimal Generation Capacity Allocation and Droop Control Design for Current Sharing in DC Microgrids. Energies 2023, 16, 4583. https://doi.org/10.3390/en16124583
Chapaloglou S, Abdolmaleki B, Tedeschi E. Optimal Generation Capacity Allocation and Droop Control Design for Current Sharing in DC Microgrids. Energies. 2023; 16(12):4583. https://doi.org/10.3390/en16124583
Chicago/Turabian StyleChapaloglou, Spyridon, Babak Abdolmaleki, and Elisabetta Tedeschi. 2023. "Optimal Generation Capacity Allocation and Droop Control Design for Current Sharing in DC Microgrids" Energies 16, no. 12: 4583. https://doi.org/10.3390/en16124583
APA StyleChapaloglou, S., Abdolmaleki, B., & Tedeschi, E. (2023). Optimal Generation Capacity Allocation and Droop Control Design for Current Sharing in DC Microgrids. Energies, 16(12), 4583. https://doi.org/10.3390/en16124583










