Active Power Control to Mitigate Frequency Deviations in Large-Scale Grid-Connected PV System Using Grid-Forming Single-Stage Inverters
Abstract
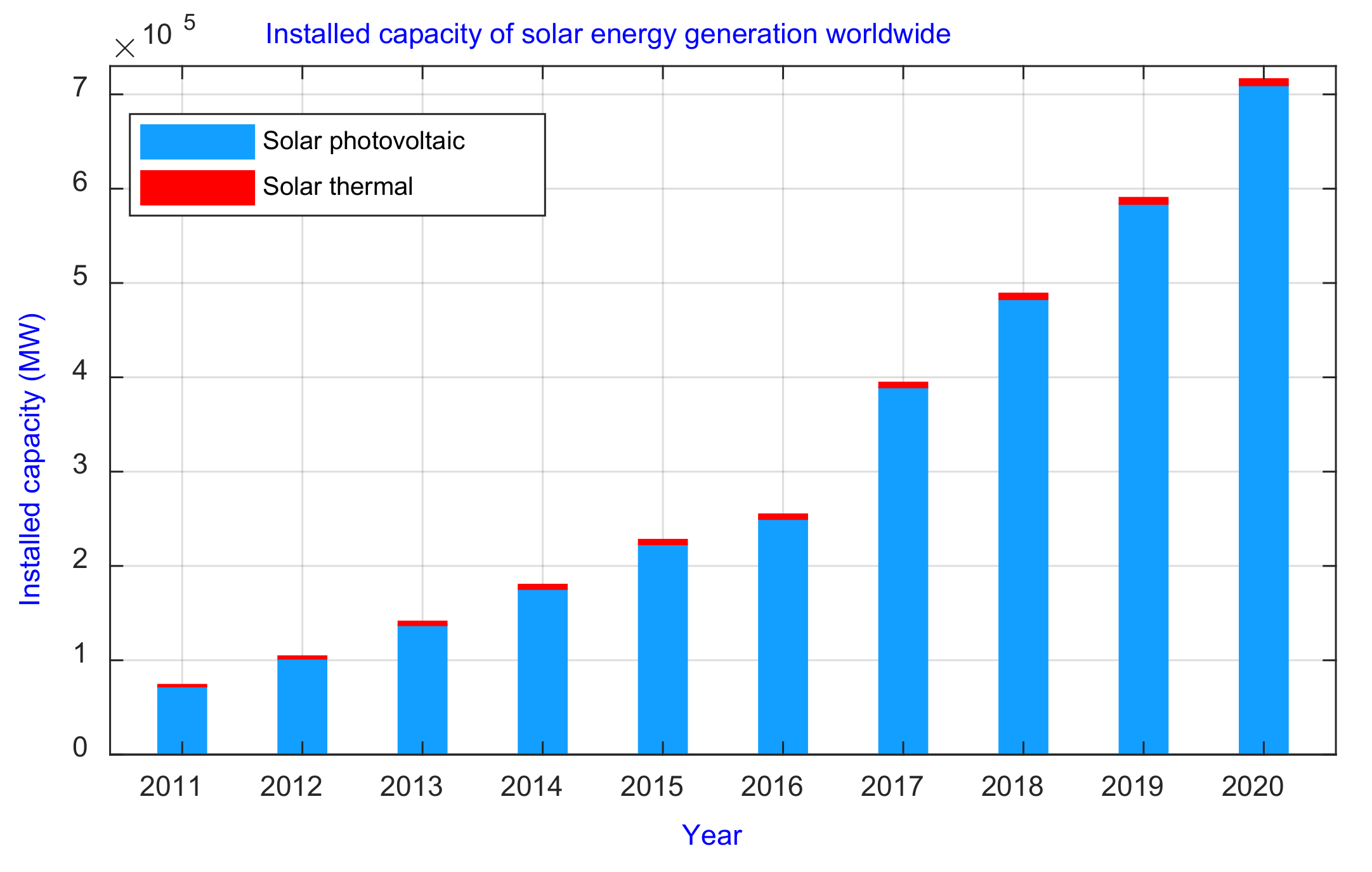
:1. Introduction
- To develop a complete control method that will allow large-scale PVPPs to tolerate frequency increases by rapidly reducing the necessary active power under a variety of frequency deviation scenarios to meet the standard criteria (GCs).
- To design power control for frequency support at the grid during frequency deviation and enforce the PVPPs to behave as traditional power plants towards smooth integration and grid stability.
- To add a new and simple control aspect to the existing single-stage inverter controller to work at normal and abnormal conditions without additional or extra elements. The controller is aimed at mitigating the frequency deviation according to the recent integration requirements during abnormal conditions.
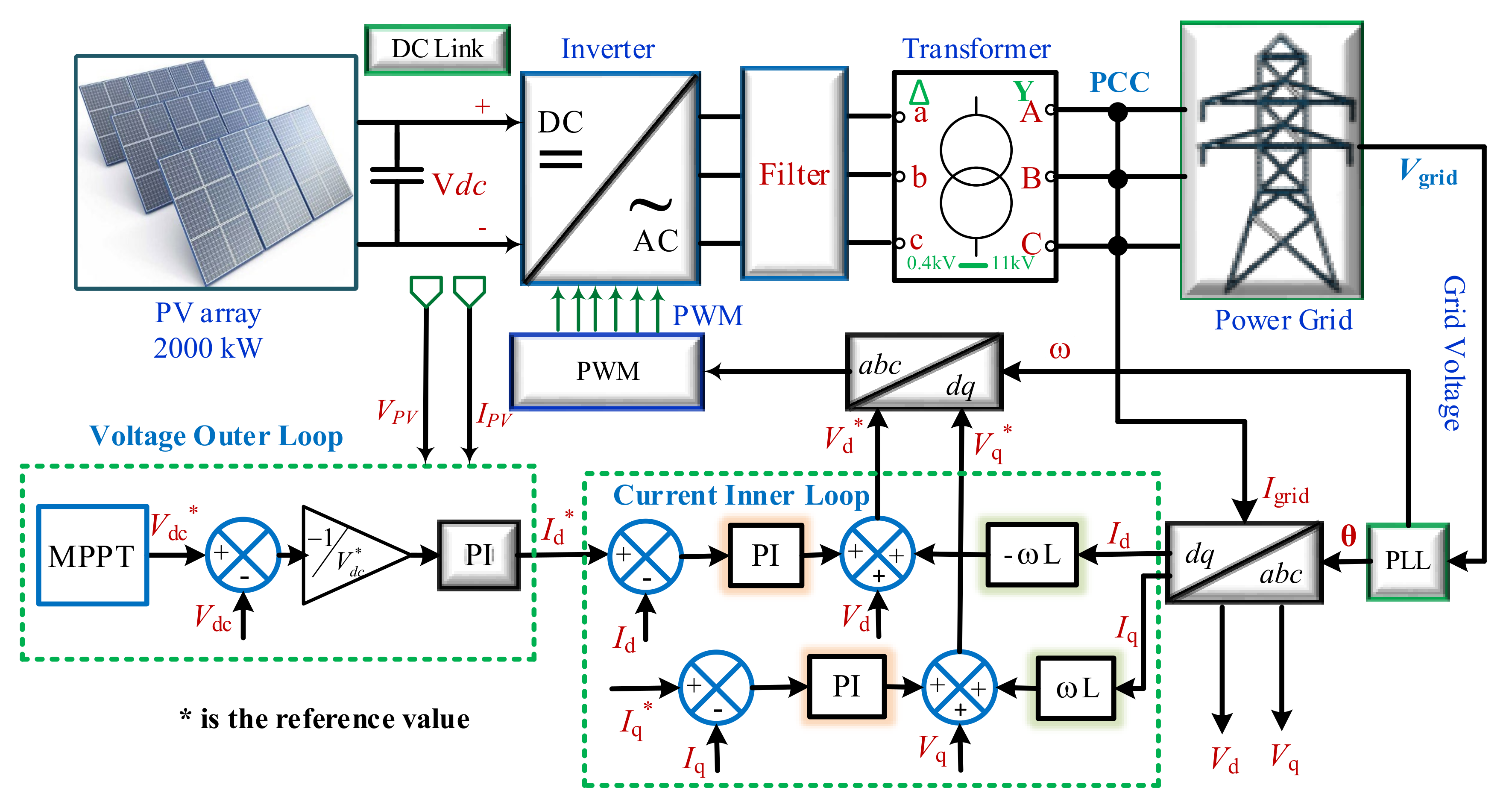
2. Grid-Connected PV System
2.1. PV Array Modeling and Sizing
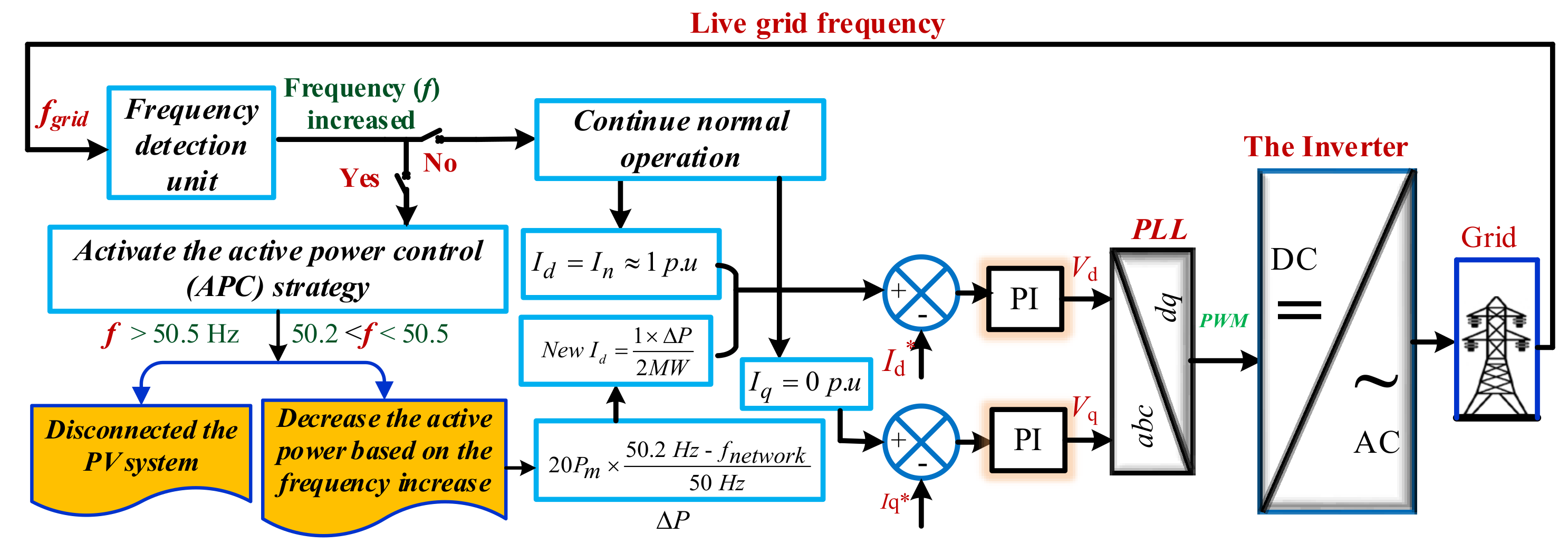
2.2. Control Strategy of PV Inverter
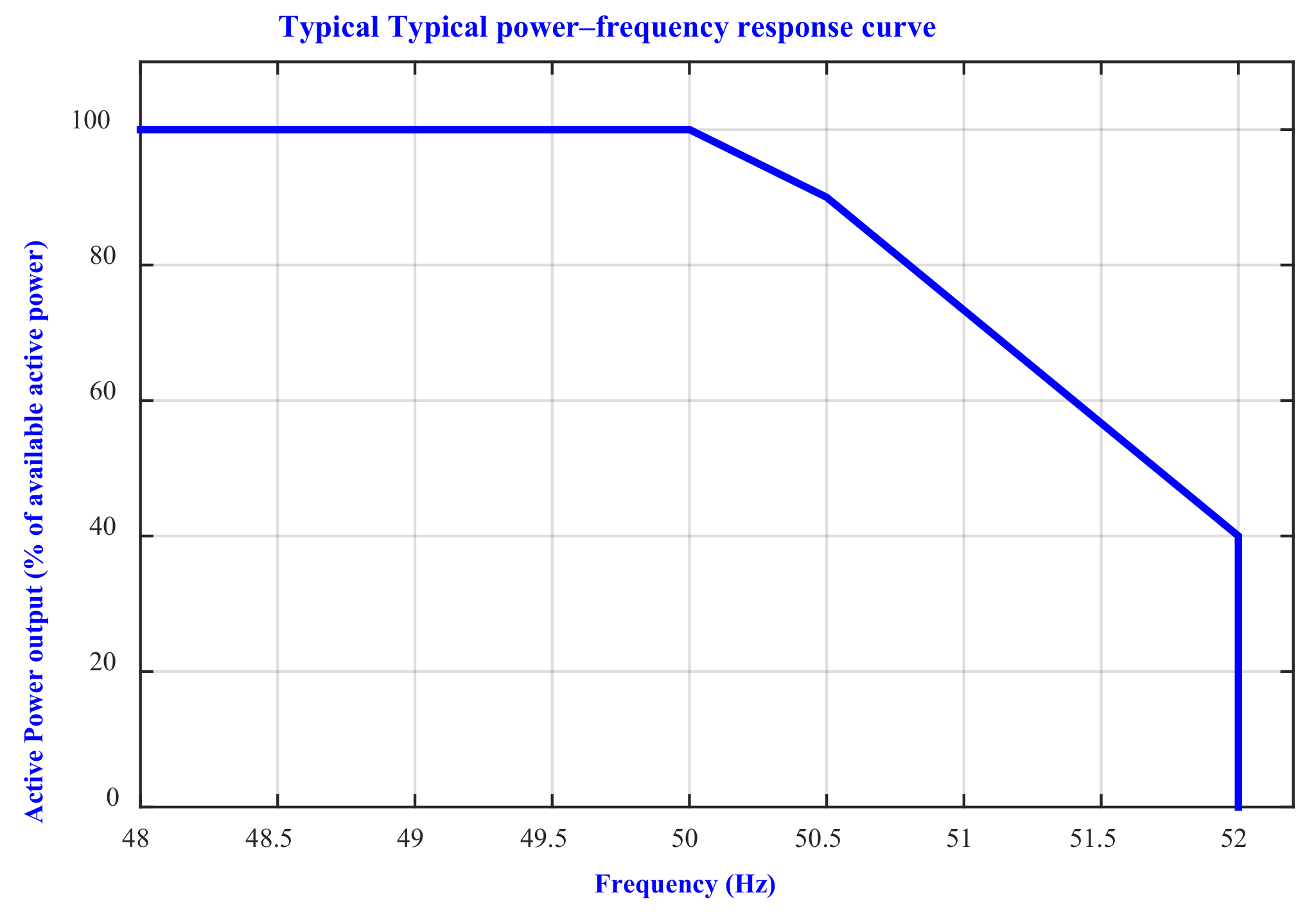
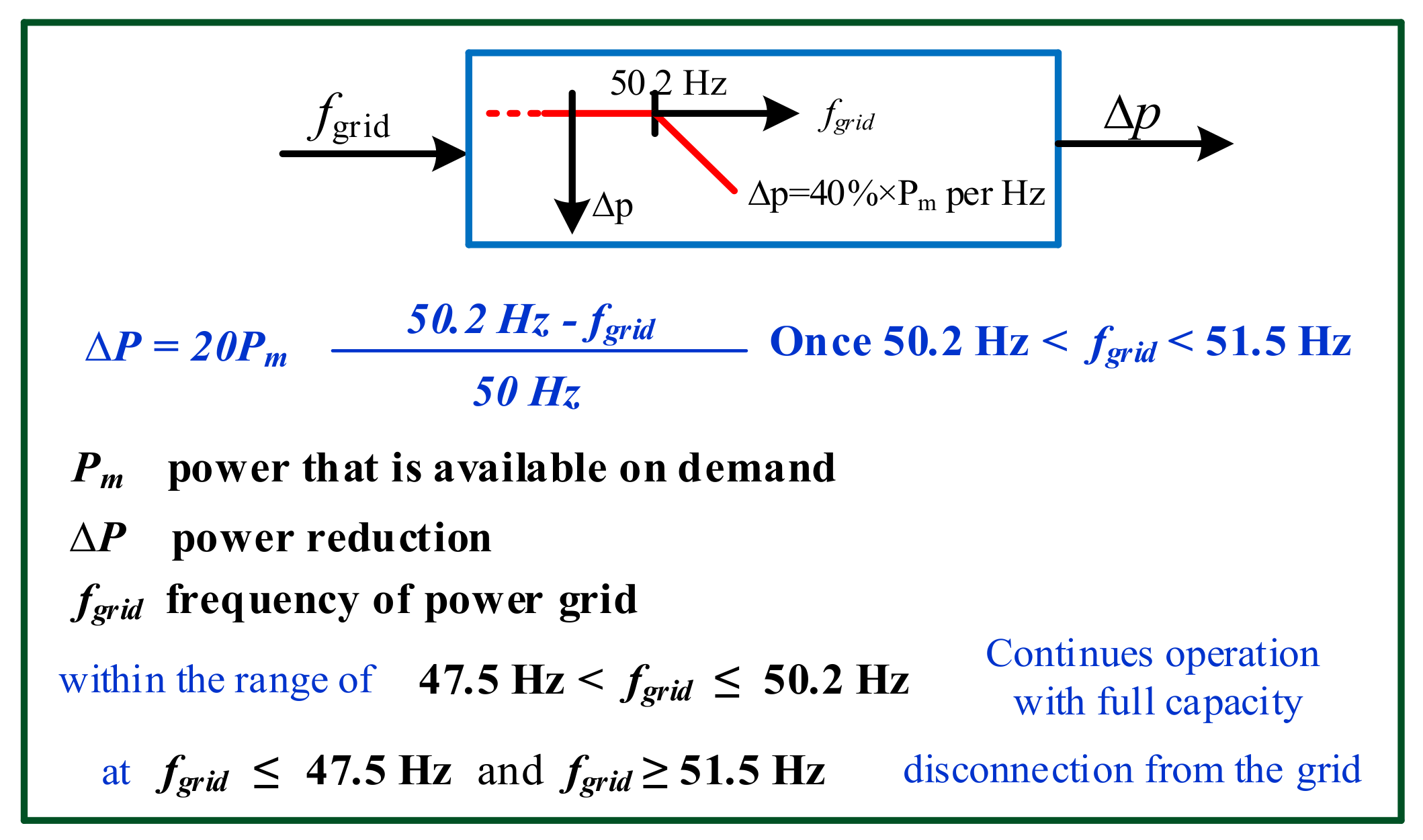
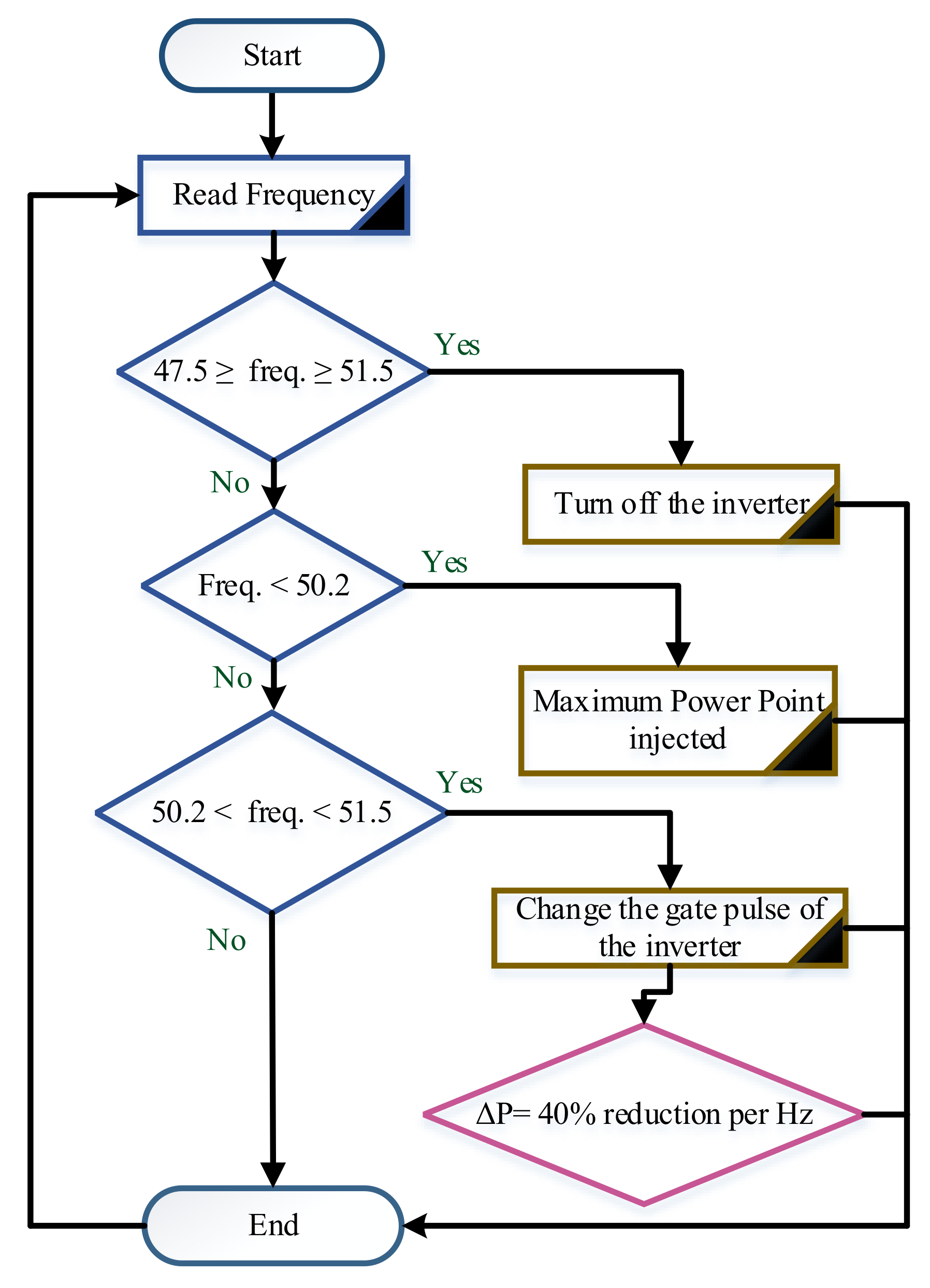
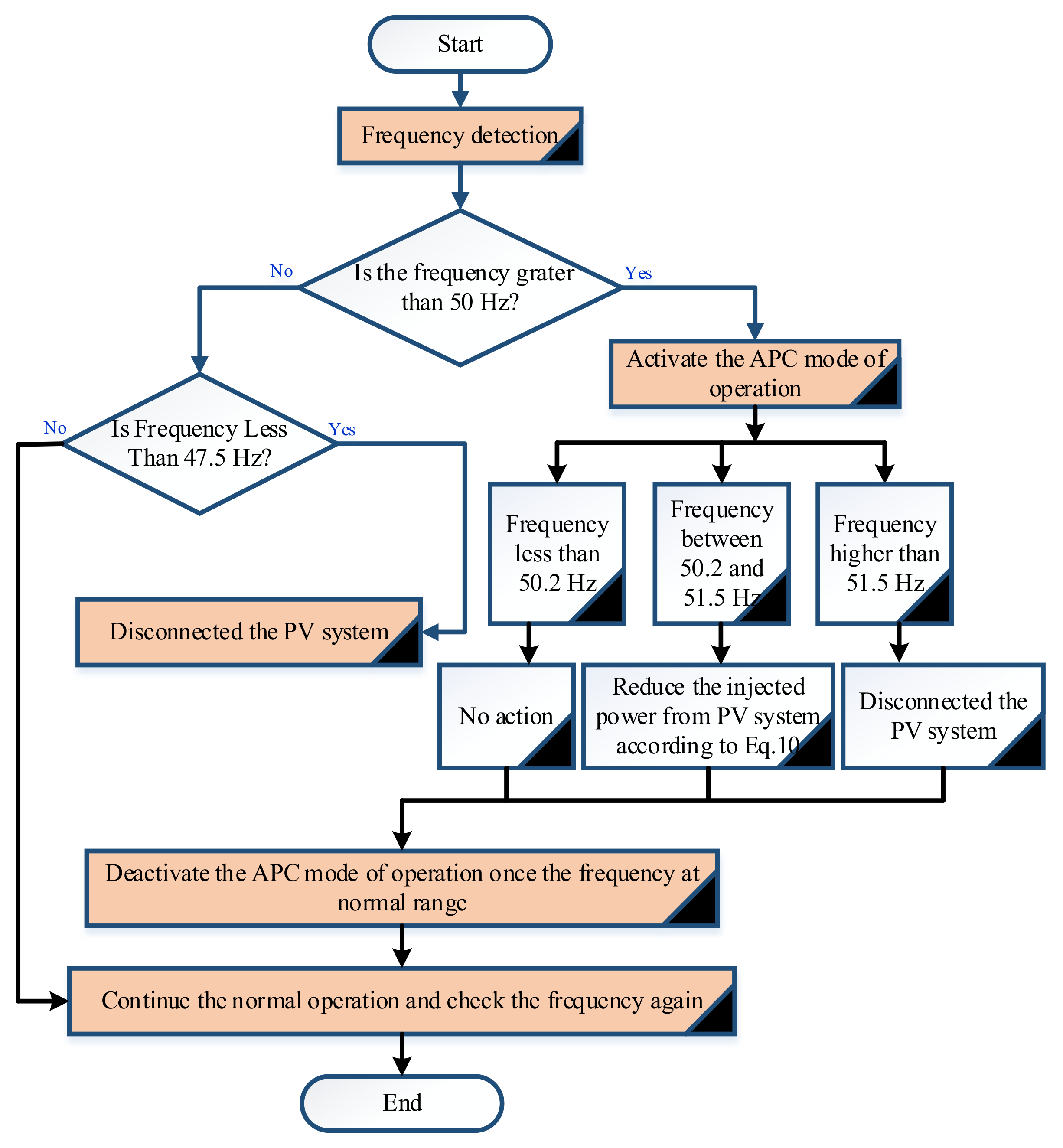
3. Active Power Control
- First case: if the line frequency is less than 50.2 Hz and greater than 47.5 Hz, the PV output power is totally inverted and supplied into the utility grid.
- Second case: if the main line frequency increased above 50.2 Hz until 51.5 Hz, the quantity of PV generated injected power into the grid must be decreased according to the following equation:
- Third case: if the main line frequency goes below 47.5 Hz or above 51.5 Hz, the DC power inverted is totally cut.
4. Results and Discussion
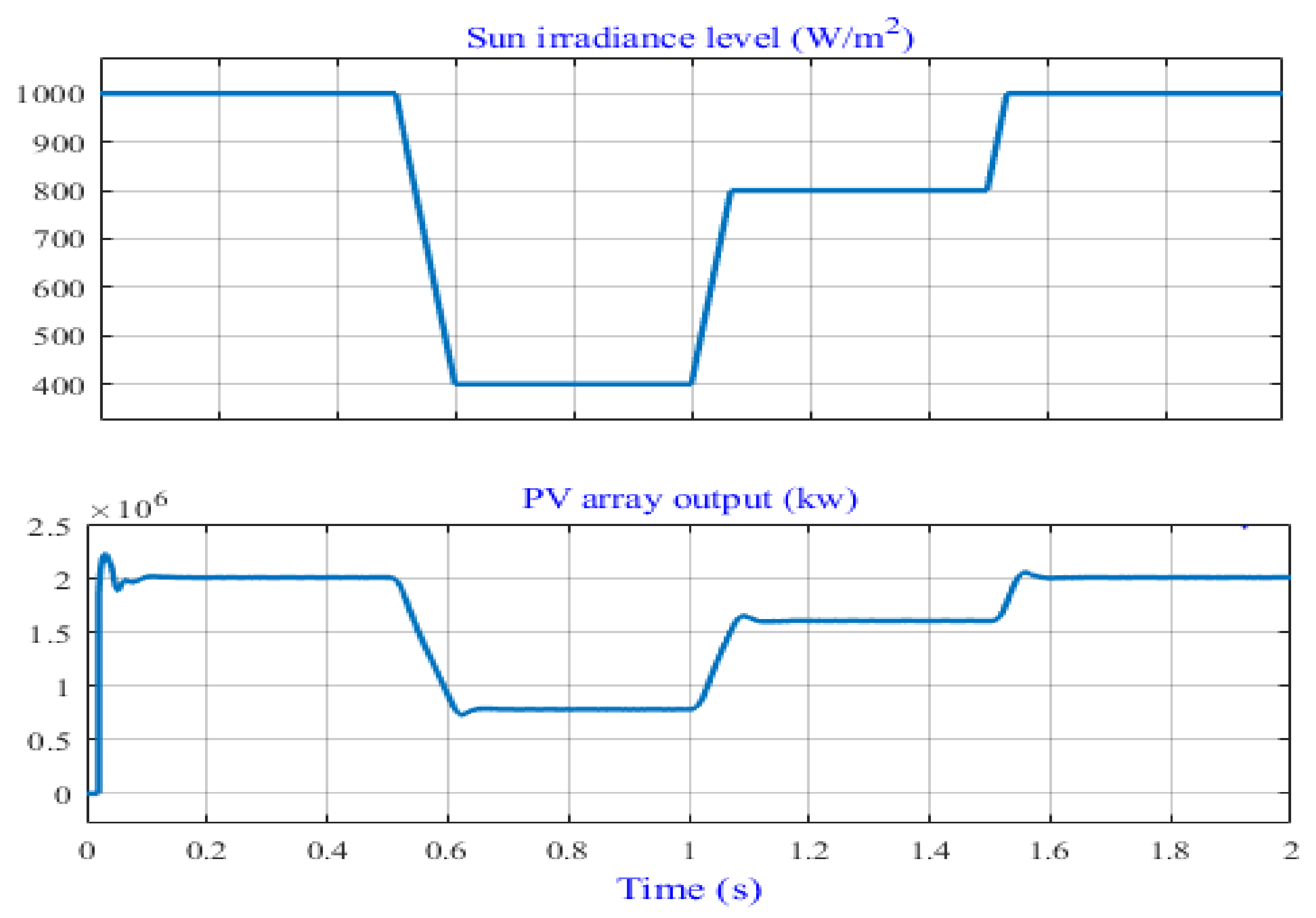
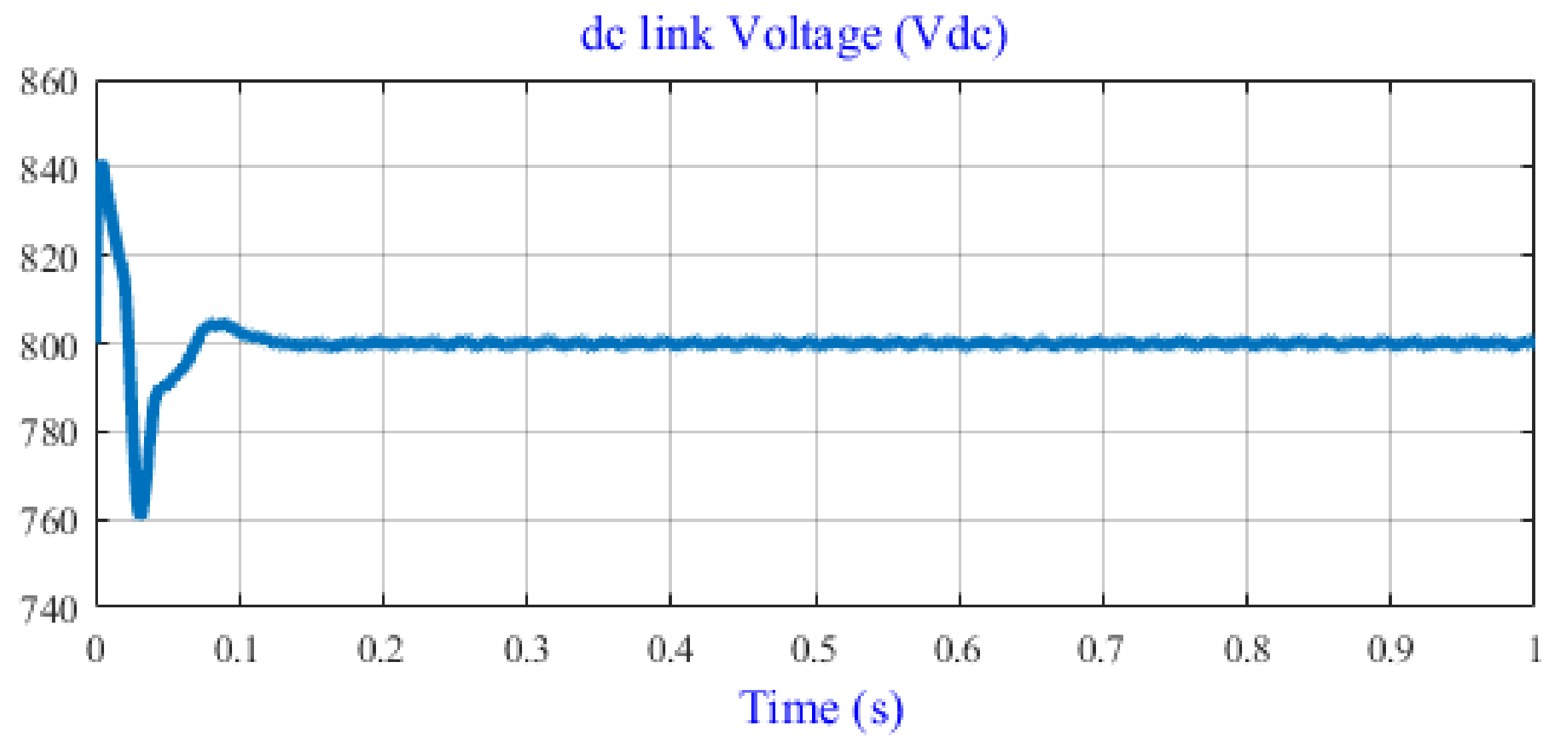
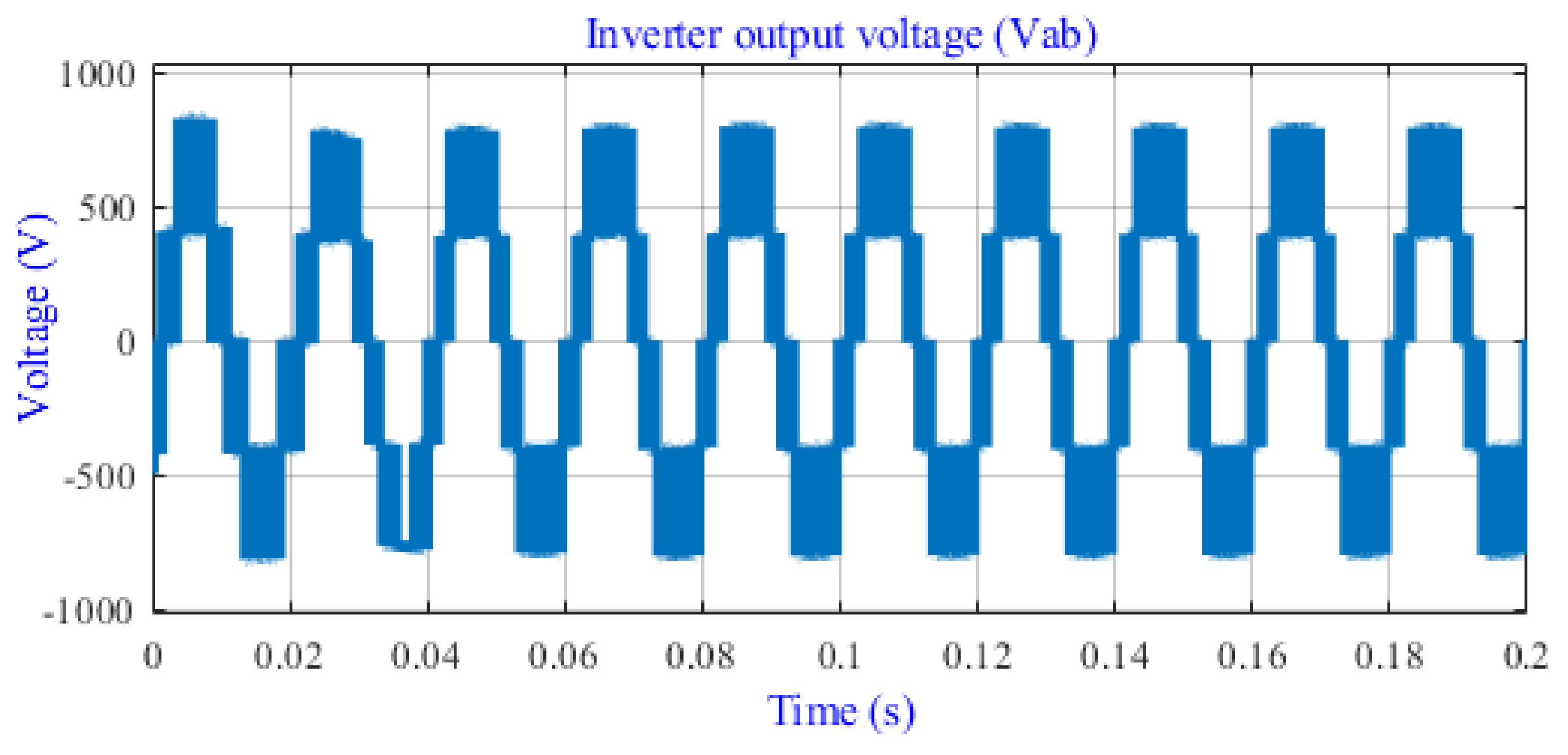
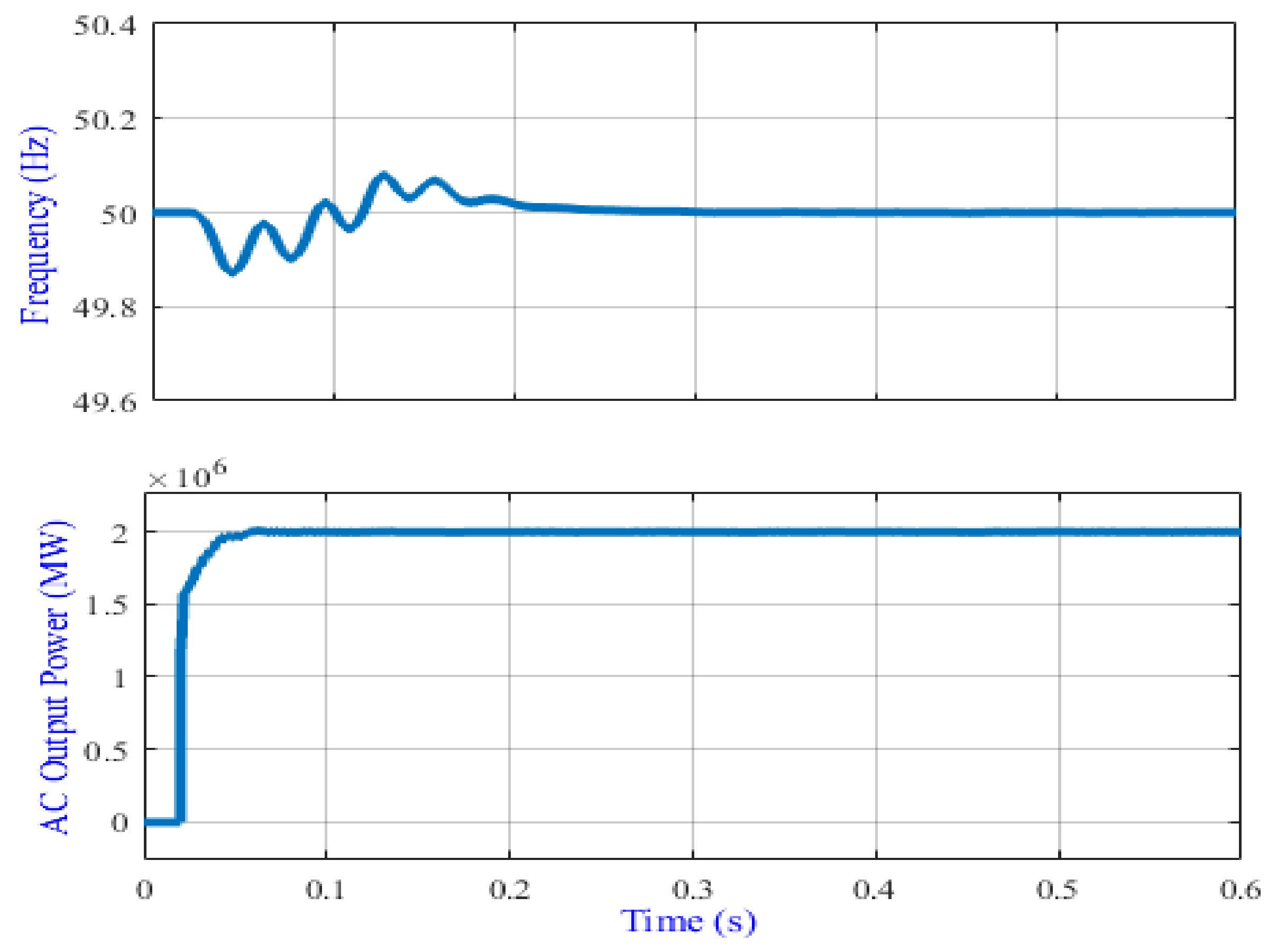
4.1. Simulation Results of the Large-Scale PVPP
4.2. Simulation Results of the APC
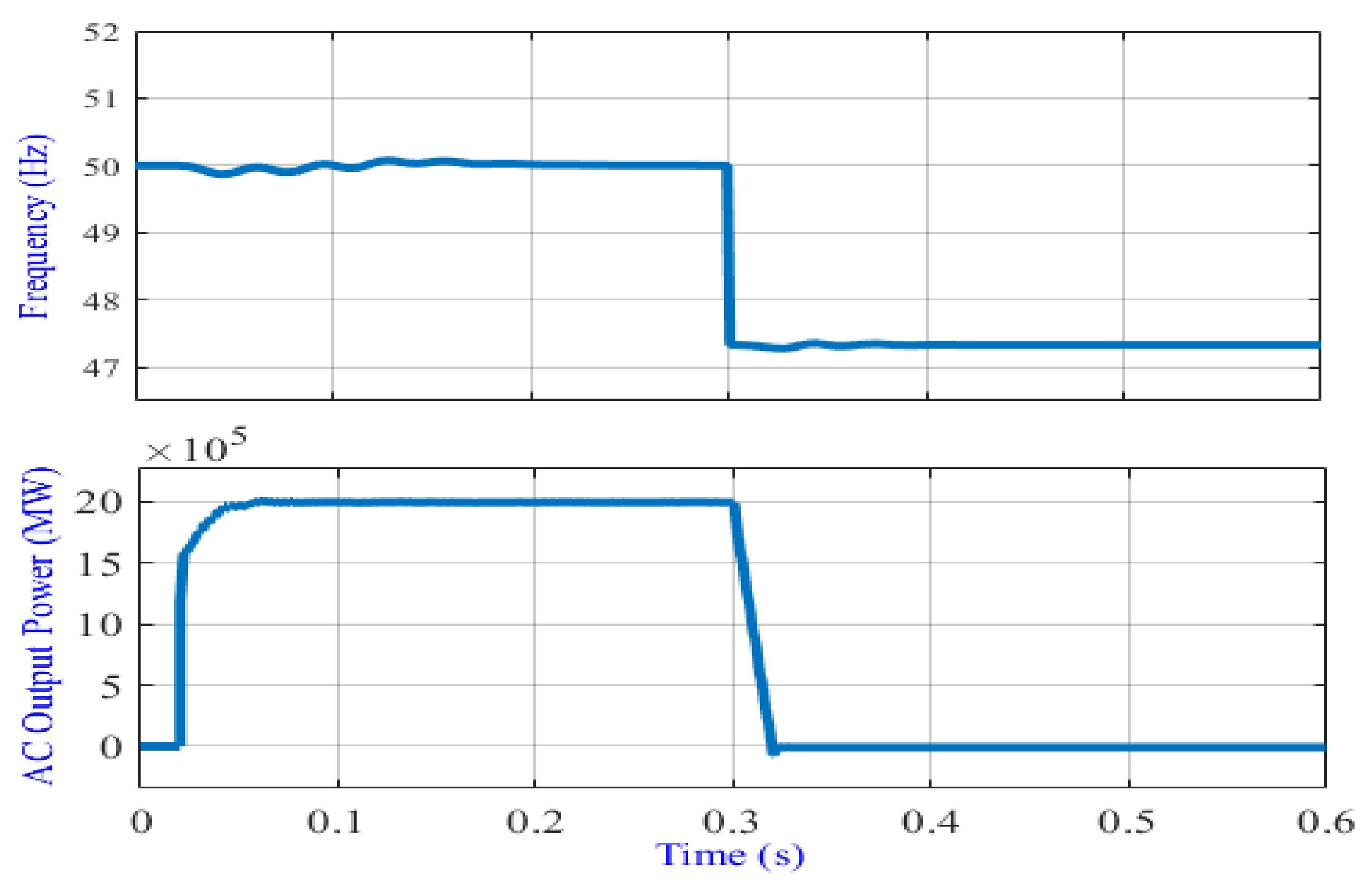
4.2.1. Normal Operation
4.2.2. Frequency Less Than 47.5 Hz
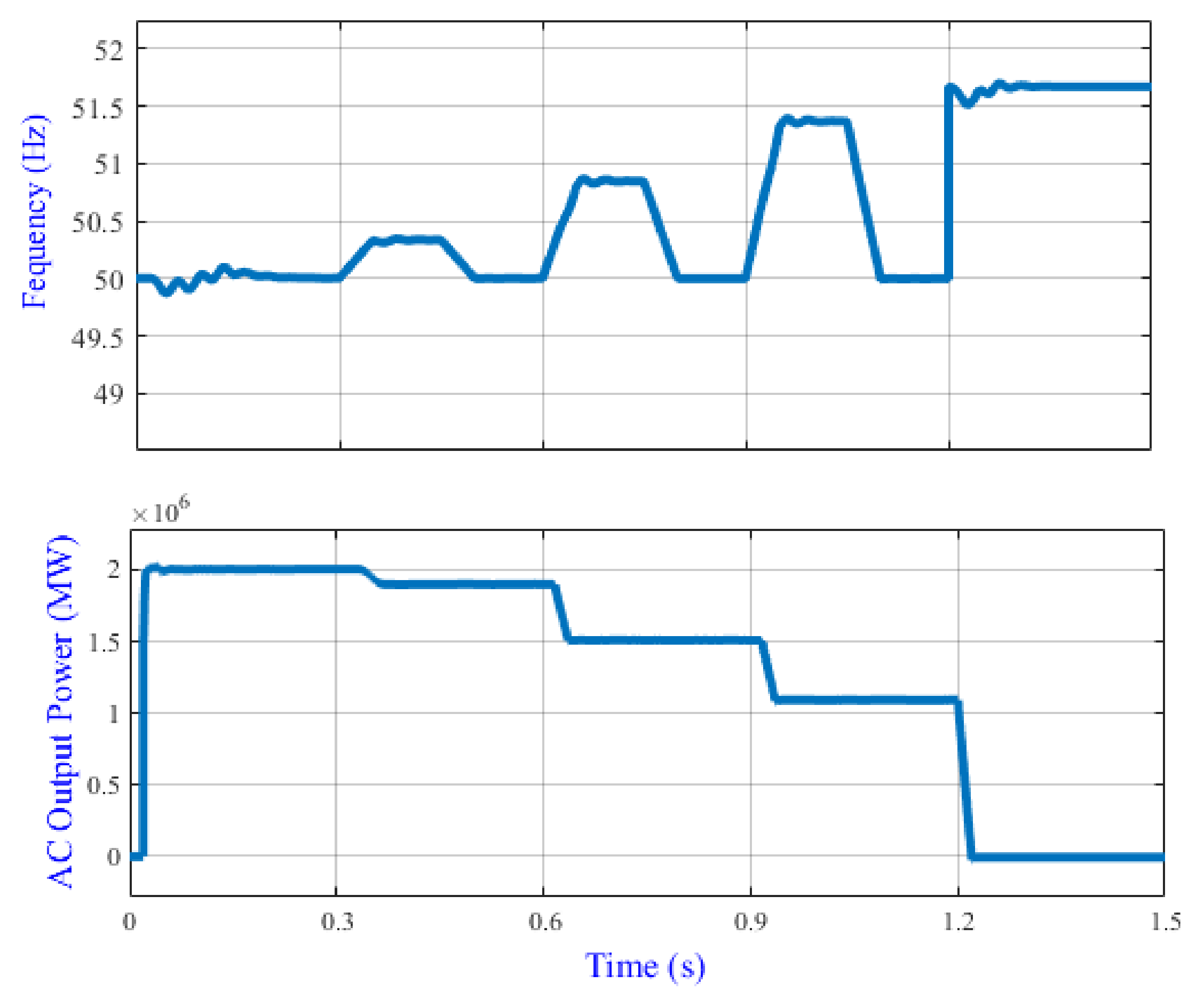
4.2.3. Frequency Ranging from 50.2–51.5 Hz
- (1)
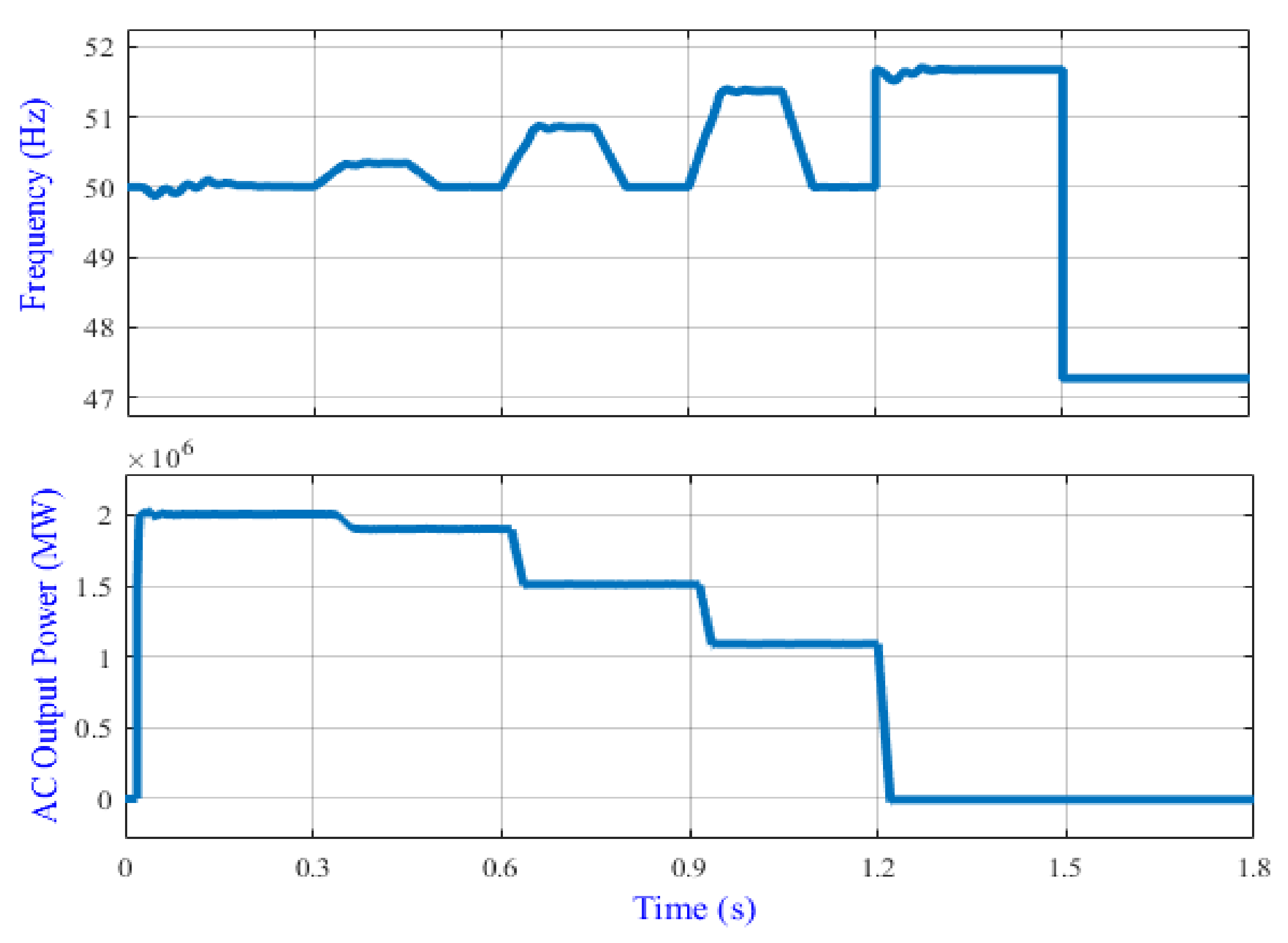
- From 0 to 0.3 s, the frequency is in the range of 50 Hz and therefore there is no action done by the controller.
- (2)
- From 0.3 s to 0.5 s the frequency is increased up to 50.3 Hz, so the APC is activated, and the output power is reduced by around 80 kW and the PV power plant supplies the grid with 1.92 MW. Thus, the frequency recovers to nominal operation between 0.5 s and 0.6 s.
- (3)
- From 0.6 s to 0.8 s the frequency is increased up to 50.8 Hz, so the APC is activated, and the output power is reduced by around 480 kW and the PV power plant supplies the grid with 1.52 MW. Thus, the frequency recovers to nominal value between 0.8 s and 0.9 s.
- (4)
- From 0.9 s to 1.1 s the frequency is increased up to 51.3 Hz, so the APC is activated, and the output power is reduced by around 880 kW and the PV power plant supplies the grid with 1.12 MW. Thus, the frequency recovers to nominal value between 1.1 s and 1.2 s.
- (5)
- At 1.2 s, the frequency is increased to above 51.5 Hz, and then the APC is activated to disconnect the PV power plant.
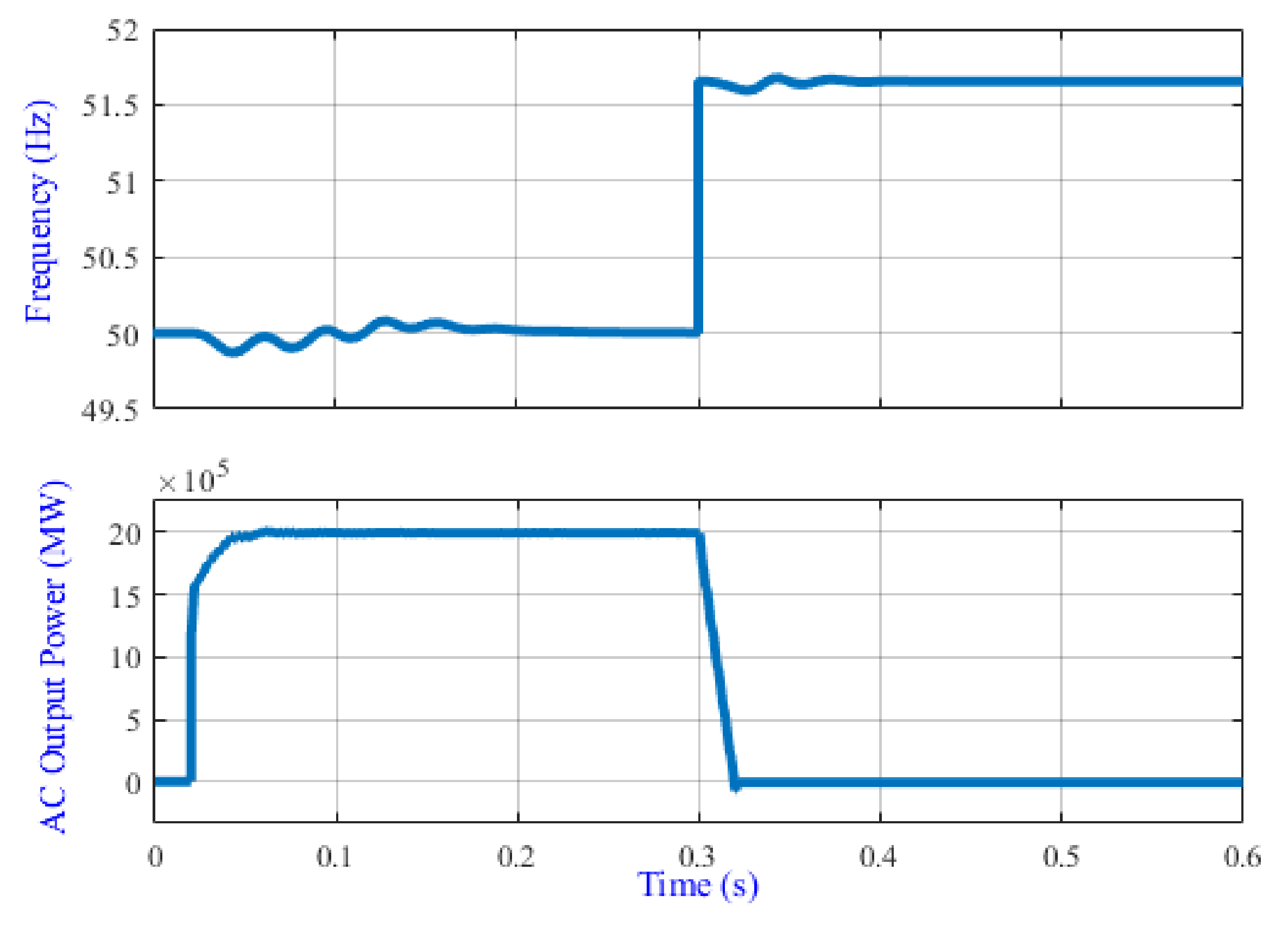
4.2.4. Frequency Higher Than 51.5 Hz
4.2.5. Different Rates of Changes of the Frequency at Same Time
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Nkiriki, J.; Ustun, T.S. Mini-grid policy directions for decentralized smart energy models in Sub-Saharan Africa. In Proceedings of the 2017 IEEE PES Innovative Smart Grid Technologies Conference Europe (ISGT-Europe), Turin, Italy, 26–29 September 2017; pp. 1–6. [Google Scholar]
- Javed, K.; Ashfaq, H.; Singh, R.; Hussain, S.M.S.; Ustun, T.S. Design and Performance Analysis of a Stand-alone PV System with Hybrid Energy Storage for Rural India. Electronics 2019, 8, 952. [Google Scholar] [CrossRef] [Green Version]
- Dos Santos, F.C.; Thornburg, J.; Ustun, T.S. Automated Planning of Rooftop PV Systems with Aerial Image Processing. In Proceedings of the 2018 IEEE PES Asia-Pacific Power and Energy Engineering Conference (APPEEC), Kota Kinabalu, Malaysia, 7–10 October 2018; pp. 736–740. [Google Scholar]
- Oteng, D.; Zuo, J.; Sharifi, E. A scientometric review of trends in solar photovoltaic waste management research. Sol. Energy 2021, 224, 545–562. [Google Scholar] [CrossRef]
- International Renewable Energy Agency. IRENA Renewable Power Capacity Growth. Available online: https://bit.ly/3Gs9jRJ (accessed on 1 February 2022).
- Japan Electrical Safety and Environment Technology Laboratories, Low-Voltage Grid-Connected Inverter Certification. Available online: https://www.jet.or.jp/en/products/protection/index.html (accessed on 4 February 2022).
- Panigrahi, R.; Mishra, S.K.; Srivastava, S.C.; Srivastava, A.K.; Schulz, N.N. Grid integration of small-scale photovoltaic systems in secondary distribution network—A review. IEEE Trans. Ind. Appl. 2020, 56, 3178–3195. [Google Scholar] [CrossRef]
- Al-Shetwi, A.Q.; Sujod, M.Z. Grid-connected photovoltaic power plants: A review of the recent integration requirements in modern grid codes. Int. J. Energy Res. 2018, 42, 1849–1865. [Google Scholar] [CrossRef]
- Ustun, T.S.; Sugahara, S.; Suzuki, M.; Hashimoto, J.; Otani, K. Power Hardware in-the-Loop Testing to Analyze Fault Behavior of Smart Inverters in Distribution Networks. Sustainability 2020, 12, 9365. [Google Scholar] [CrossRef]
- Cheng, Y.; Azizipanah-Abarghooee, R.; Azizi, S.; Ding, L.; Terzija, V. Smart frequency control in low inertia energy systems based on frequency response techniques: A review. Appl. Energy 2020, 279, 115798. [Google Scholar] [CrossRef]
- Tayyebi, A.; Groß, D.; Anta, A.; Kupzog, F.; Dörfler, F. Frequency stability of synchronous machines and grid-forming power converters. IEEE J. Emerg. Sel. Top. Power Electron. 2020, 8, 1004–1018. [Google Scholar] [CrossRef] [Green Version]
- Hashimoto, J.; Ustun, T.S.; Suzuki, M.; Sugahara, S.; Hasegawa, M.; Otani, K. Advanced Grid Integration Test Platform for Increased Distributed Renewable Energy Penetration in Smart Grids. IEEE Access 2021, 9, 34040–34053. [Google Scholar] [CrossRef]
- Honrubia-Escribano, A.; Ramirez, F.J.; Gómez-Lázaro, E.; Garcia-Villaverde, P.M.; Ruiz-Ortega, M.J.; Parra-Requena, G. Influence of solar technology in the economic performance of PV power plants in Europe. A comprehensive analysis. Renew. Sustain. Energy Rev. 2018, 82, 488–501. [Google Scholar] [CrossRef]
- Christie, R.D.; Bose, A. Load frequency control issues in power system operations after deregulation. IEEE Trans. Power Syst. 1996, 11, 1191–1200. [Google Scholar] [CrossRef]
- Datta, M.; Senjyu, T.; Yona, A.; Funabashi, T.; Kim, C.-H. A frequency-control approach by photovoltaic generator in a PV–diesel hybrid power system. IEEE Trans. Energy Convers. 2011, 26, 559–571. [Google Scholar] [CrossRef]
- Liu, L.; Li, H.; Xue, Y.; Liu, W. Decoupled active and reactive power control for large-scale grid-connected photovoltaic systems using cascaded modular multilevel converters. IEEE Trans. Power Electron. 2015, 30, 176–187. [Google Scholar]
- Stimoniaris, D.; Tsiamitros, D.; Dialynas, E. Improved energy storage management and PV-active power control infrastructure and strategies for microgrids. IEEE Trans. Power Syst. 2016, 31, 813–820. [Google Scholar] [CrossRef]
- Latif, A.; Hussain, S.M.S.; Das, D.C.; Ustun, T.S. Optimum Synthesis of a BOA Optimized Novel Dual-Stage PI − (1 + ID) Controller for Frequency Response of a Microgrid. Energies 2020, 13, 3446. [Google Scholar] [CrossRef]
- Bullich-Massagué, E.; Aragüés-Peñalba, M.; Sumper, A.; Boix-Aragones, O. Active power control in a hybrid PV-storage power plant for frequency support. Sol. Energy 2017, 144, 49–62. [Google Scholar] [CrossRef] [Green Version]
- Yang, Y.; Enjeti, P.; Blaabjerg, F.; Wang, H. Suggested grid code modifications to ensure wide-scale adoption of photovoltaic energy in distributed power generation systems. In Proceedings of the Industry Applications Society Annual Meeting, Lake Buena Vista, FL, USA, 6–11 October 2013; pp. 1–8. [Google Scholar]
- Troester, E. New German grid codes for connecting PV systems to the medium voltage power grid. In Proceedings of the 2nd International Workshop on Concentrating Photovoltaic Power Plants: Optical Design, Production, Grid Connection, Darmstadt, Germany, 9–10 March 2009; pp. 9–10. [Google Scholar]
- Gevorgian, V.; Booth, S. Review of Technical Requirements for Interconnecting Wind and Solar Generation; National Renewable Energy Laboratory: Golden, CO, USA, 2013. [Google Scholar]
- Energy Commission Malaysia (ECM). Grid Code for Peninsular Malaysia. Available online: http://st.gov.my (accessed on 16 June 2021).
- Wu, Y.-K.; Chang, S.-M.; Mandal, P. Grid-connected wind power plants: A survey on the integration requirements in modern grid codes. IEEE Trans. Ind. Appl. 2019, 55, 5584–5593. [Google Scholar] [CrossRef]
- Liu, Y.; Zhu, L.; Zhan, L.; Gracia, J.R.; King, T., Jr.; Liu, Y. Active power control of solar PV generation for large interconnection frequency regulation and oscillation damping. Int. J. Energy Res. 2016, 40, 353–361. [Google Scholar] [CrossRef]
- Zhang, Y.; Ma, L.; Zheng, T.Q. Application of feedback linearization strategy in voltage fault ride-through for photovoltaic inverters. In Proceedings of the IECON 2011-37th Annual Conference on IEEE Industrial Electronics Society, Melbourne, VIC, Australia, 7–10 November 2011; pp. 4666–4671. [Google Scholar]
- Jayakrishnan, R.; Sruthy, V. In Fault ride through augmentation of microgrid, Advancements in Power and Energy (TAP Energy). In Proceedings of the 2015 International Conference on Technological Advancements in Power and Energy (TAP Energy), Kollam, India, 24–26 June 2015; pp. 357–362. [Google Scholar]
- Rath, D.; Kar, S.; Patra, A.K. Harmonic distortion assessment in the single-phase photovoltaic (PV) system based on spwm technique. Arab. J. Sci. Eng. 2021, 41, 9601–9615. [Google Scholar] [CrossRef]
- Nour, A.M.; Helal, A.A.; El-Saadawi, M.M.; Hatata, A.Y. A control scheme for voltage unbalance mitigation in distribution network with rooftop pv systems based on distributed batteries. Int. J. Electr. Power Energy Syst. 2021, 124, 106375. [Google Scholar] [CrossRef]
- Yang, F.; Ling, Z.; Wei, M.; Mi, T.; Yang, H.; Qiu, R.C. Real-time static voltage stability assessment in large-scale power systems based on spectrum estimation of phasor measurement unit data. Int. J. Electr. Power Energy Syst. 2021, 124, 106196. [Google Scholar] [CrossRef]
- Hoke, A.F.; Shirazi, M.; Chakraborty, S.; Muljadi, E.; Maksimovic, D. Rapid active power control of photovoltaic systems for grid frequency support. IEEE J. Emerg. Sel. Top. Power Electron. 2017, 5, 1154–1163. [Google Scholar] [CrossRef]
- Kikusato, H.; Ustun, T.S.; Hashimoto, J.; Otani, K. Aggregate Modeling of Distribution System with Multiple Smart Inverters. In Proceedings of the 2019 International Conference on Smart Energy Systems and Technologies (SEST), Porto, Portugal, 9–11 September 2019; pp. 1–6. [Google Scholar]
- Ustun, T.S.; Aoto, Y. Analysis of Smart Inverter’s Impact on the Distribution Network Operation. IEEE Access 2019, 7, 9790–9804. [Google Scholar] [CrossRef]
- Long, J.; Qu, L.; Zhang, S.; Li, L. In Frequency control strategy and test technology of photovoltaic power plant. In Proceedings of the 2019 IEEE 3rd Conference on Energy Internet and Energy System Integration (EI2), Changsha, China, 8–10 November 2019; pp. 1695–1698. [Google Scholar]
- Chen, Z.; Liu, J.; Lin, Z.; Duan, Z. Closed-loop active power control of wind farm based on frequency domain analysis. Electr. Power Syst. Res. 2019, 170, 13–24. [Google Scholar] [CrossRef]
- Madorell-Batlle, Q.; Bullich-Massagué, E.; Cheah-Mañé, M.; Gomis-Bellmunt, O. Over-frequency support in large-scale photovoltaic power plants using non-conventional control architectures. Int. J. Electr. Power Energy Syst. 2021, 127, 106679. [Google Scholar] [CrossRef]
- Padhy, S.; Sahu, P.R.; Khadanga, R.K.; Prusty, B.R.; Panda, S. MPA-Tuned Fractional Order PID Controller for Frequency Control of Interconnected Smart Grid Power System. In Proceedings of the 2021 Innovations in Power and Advanced Computing Technologies (i-PACT), Kuala Lumpur, Malaysia, 27–29 November 2021; pp. 1–5. [Google Scholar]
- Latif, A.; Hussain, S.S.; Das, D.C.; Ustun, T.S.; Iqbal, A. A review on fractional order (FO) controllers’ optimization for load frequency stabilization in power networks. Energy Rep. 2021, 7, 4009–4021. [Google Scholar] [CrossRef]
- Howlader, A.M.; Sadoyama, S.; Roose, L.R.; Chen, Y. Active power control to mitigate voltage and frequency deviations for the smart grid using smart PV inverters. Appl. Energy 2020, 258, 114000. [Google Scholar] [CrossRef]
- Olita, F. Advanced Control and Condition Monitoring PV Systems. Master Thesis, Aalborg University Institute of Energy Technology Denmark, Aalborg, Denmark, 2012. [Google Scholar]
- Jana, J.; Saha, H.; Bhattacharya, K.D. A review of inverter topologies for single-phase grid-connected photovoltaic systems. Renew. Sustain. Energy Rev. 2016, 72, 1256–1270. [Google Scholar] [CrossRef]
- Al-Shetwi, A.Q. Design and economic evaluation of electrification of small villages in rural area in Yemen using stand-alone PV system. Int. J. Renew. Energy Res. (IJRER) 2016, 6, 289–298. [Google Scholar]
- Menzi, D.; Kolar, J.W.; Anderson, J.A.; Kasper, M.J. New third-harmonic injection modulation reducing the dc-link energy buffer requirement of phase-modular three-phase isolated pfc ac/dc converter systems. In Proceedings of the 2021 IEEE 22nd Workshop on Control and Modelling of Power Electronics (COMPEL), Cartagena, Colombia, 2–5 November 2021. [Google Scholar]
- Basso, T. IEEE 1547 and 2030 Standards for Distributed Energy Resources Interconnection and Interoperability with the Electricity Grid; National Renewable Energy Lab. (NREL): Golden, CO, USA, 2014. [Google Scholar]
- Orłowska-Kowalska, T.; Blaabjerg, F.; Rodríguez, J. Advanced and Intelligent Control in Power Electronics and Drives; Springer: New York, NY, USA, 2014; Volume 531. [Google Scholar]
- Villalva, M.G.; Gazoli, J.R.; Ruppert Filho, E. Comprehensive approach to modeling and simulation of photovoltaic arrays. IEEE Trans. Power Electron. 2009, 24, 1198–1208. [Google Scholar] [CrossRef]
- Nwaigwe, K.; Mutabilwa, P.; Dintwa, E. An overview of solar power (PV systems) integration into electricity grids. Mater. Sci. Energy Technol. 2019, 2, 629–633. [Google Scholar] [CrossRef]
- Ali, Q.; Muhamad Zahim, S.; Noor Lina, R. A review of the fault ride through requirements in different grid codes concerning penetration of PV system to the electric power network. ARPN J. Eng. Appl. Sci. 2015, 10, 9906–9912. [Google Scholar]
- Lee, J.-S.; Lee, K.B. Variable dc-link voltage algorithm with a wide range of maximum power point tracking for a two-string PV system. Energies 2013, 6, 58–78. [Google Scholar] [CrossRef] [Green Version]
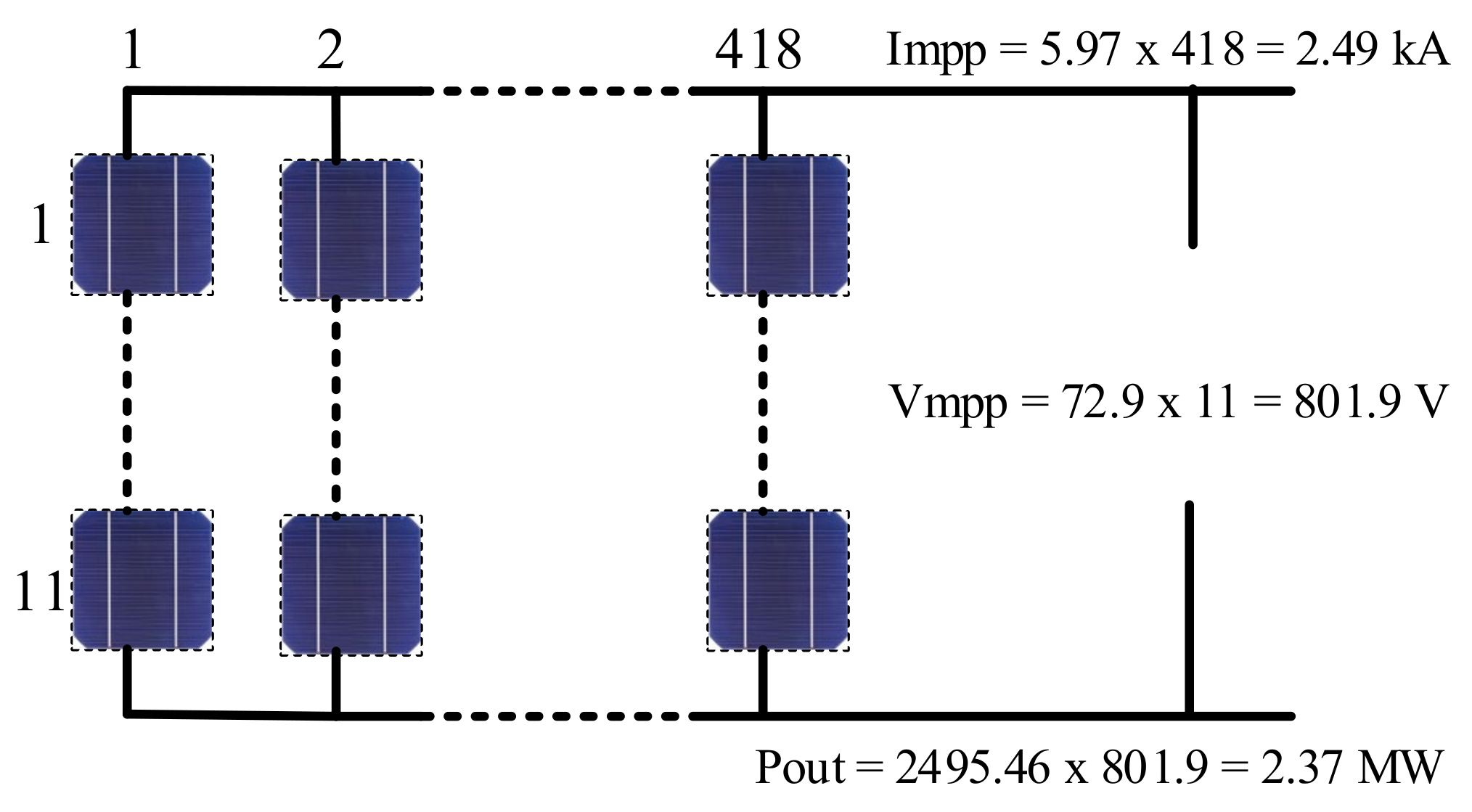



| The Parameters | The Value | The Parameters | The Value |
|---|---|---|---|
| Maximum power | Pmax = 435.21 W | Numbers of cells per module | Ncell = 128 |
| Maximum current | Imp = 5.97 A | Temperature coefficient of Isc | αi = 0.0307/Cº |
| Maximum voltage | Vmp = 72.9 V | Temperature coefficient of Voc | αv = −0.0229/Cº |
| Short-circuit current | Ish = 6.43 A | Parallel and series resistance (Rp,Rs) | 419.77 Ω, 0.537 Ω |
| Open-circuit voltage | Voc = 85.6 V | Ideally factor of the diode | m = 0.872 |
| Inverter Parameters | The Value |
|---|---|
| Grid voltage (Vg) | 11 kV |
| Grid frequency (f) | 50 Hz |
| Voltage of DC-link (Vdc) | 800 V |
| Capacitor of DC-link (Cdc) | 0.2130 F |
| Grid angular frequency (ɷ) | 2π × 50 rad/s |
| Filter parameters (R and L) | 1.25 Ω, 0.1 mH |
| inverter Switching frequency (fs) | 2 kHz |
| Current loop parameter of PI (Kp, Ki) | 0.3, 20 |
| Voltage loop parameter of PI (Kp, Ki) | 2, 400 |
| Transformer (n = Vp/Vs) | 0.4/11 kV |
| Ref. | Controller | Main Finding | Limitation/Remarks |
|---|---|---|---|
| [19] | Direct ramp rate control | The frequency drop is supported using an energy storage device (battery to fulfill grid requirements). | Power oscillations could occur. Power ripple in the MPP. Extra device and then extra cost. Low response |
| [34] | frequency droop control strategy | PV power plant has a good performance on frequency droop control. | ACP concept according to the standard requirements not taken into consideration. |
| [35] | Close-loop control | Enhance the wind turbine disturbance suppression capability and power tracking dynamic performance during frequency deviation at the connection point. | Studied the effect of frequency impacts on the operation of wind turbine. Overlooked the power reduction based on grid frequency deviation. |
| [36] | Hierarchical control architecture | The proposed control obtained a fast and accurate response and was robust against communication failures. | More focus is paid on the fast response rather than the achievement of grid-code requirements and APC. Cannot support the operation under different faults. |
| Proposed APC | Active power control using grid-forming inverters | Enforce the PV system to behave similar to a traditional power plant during frequency deviation towards frequency stability. | The proposed APC is effective to help the PVPP behave similar to a traditional power plant for a stable, reliable, and efficient future of PV systems-connected power grid. |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Al-Shetwi, A.Q.; Issa, W.K.; Aqeil, R.F.; Ustun, T.S.; Al-Masri, H.M.K.; Alzaareer, K.; Abdolrasol, M.G.M.; Abdullah, M.A. Active Power Control to Mitigate Frequency Deviations in Large-Scale Grid-Connected PV System Using Grid-Forming Single-Stage Inverters. Energies 2022, 15, 2035. https://doi.org/10.3390/en15062035
Al-Shetwi AQ, Issa WK, Aqeil RF, Ustun TS, Al-Masri HMK, Alzaareer K, Abdolrasol MGM, Abdullah MA. Active Power Control to Mitigate Frequency Deviations in Large-Scale Grid-Connected PV System Using Grid-Forming Single-Stage Inverters. Energies. 2022; 15(6):2035. https://doi.org/10.3390/en15062035
Chicago/Turabian StyleAl-Shetwi, Ali Q., Walid K. Issa, Raed F. Aqeil, Taha Selim Ustun, Hussein M. K. Al-Masri, Khaled Alzaareer, Maher G. M. Abdolrasol, and Majid A. Abdullah. 2022. "Active Power Control to Mitigate Frequency Deviations in Large-Scale Grid-Connected PV System Using Grid-Forming Single-Stage Inverters" Energies 15, no. 6: 2035. https://doi.org/10.3390/en15062035
APA StyleAl-Shetwi, A. Q., Issa, W. K., Aqeil, R. F., Ustun, T. S., Al-Masri, H. M. K., Alzaareer, K., Abdolrasol, M. G. M., & Abdullah, M. A. (2022). Active Power Control to Mitigate Frequency Deviations in Large-Scale Grid-Connected PV System Using Grid-Forming Single-Stage Inverters. Energies, 15(6), 2035. https://doi.org/10.3390/en15062035










