1. Introduction
A pair of closely spaced vertical-axis wind turbines (VAWTs) can yield more power than two isolated VAWTs [
1]. This idea was extended to a wind farm where many pairs of small-sized VAWTs were placed in a limited area in order to yield a high power density [
2]. VAWTs accept wind from all directions; thus, it is possible to place them in proximity to each other. In order to investigate the performance and characteristics of such closely spaced VAWTs, wind tunnel experiments [
3,
4,
5,
6,
7,
8] and computational fluid dynamics (CFD) simulations of a pair of VAWTs were performed in two dimensions [
9,
10,
11,
12,
13,
14], as well as in three dimensions [
6,
15].
In Refs. [
4,
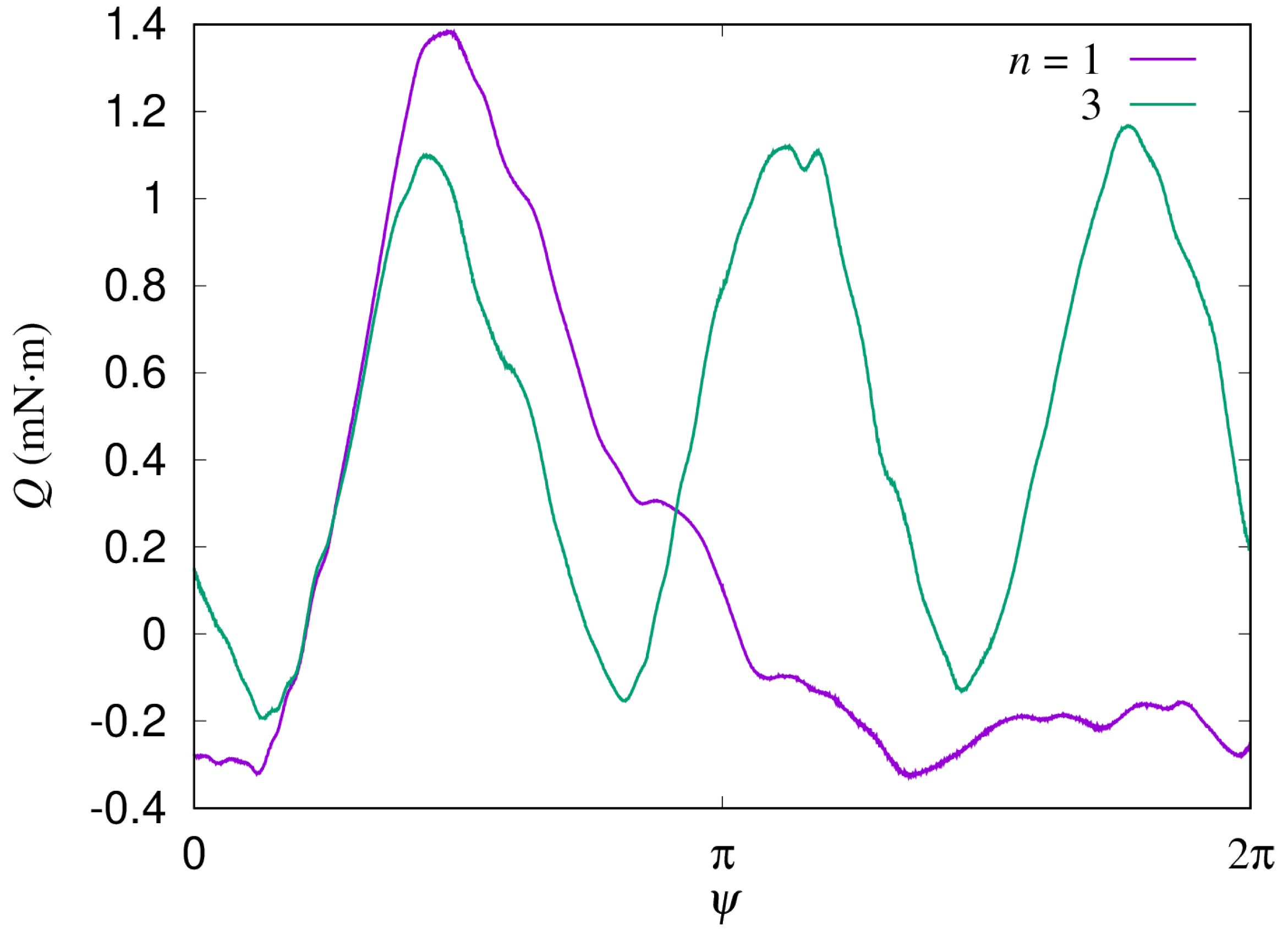
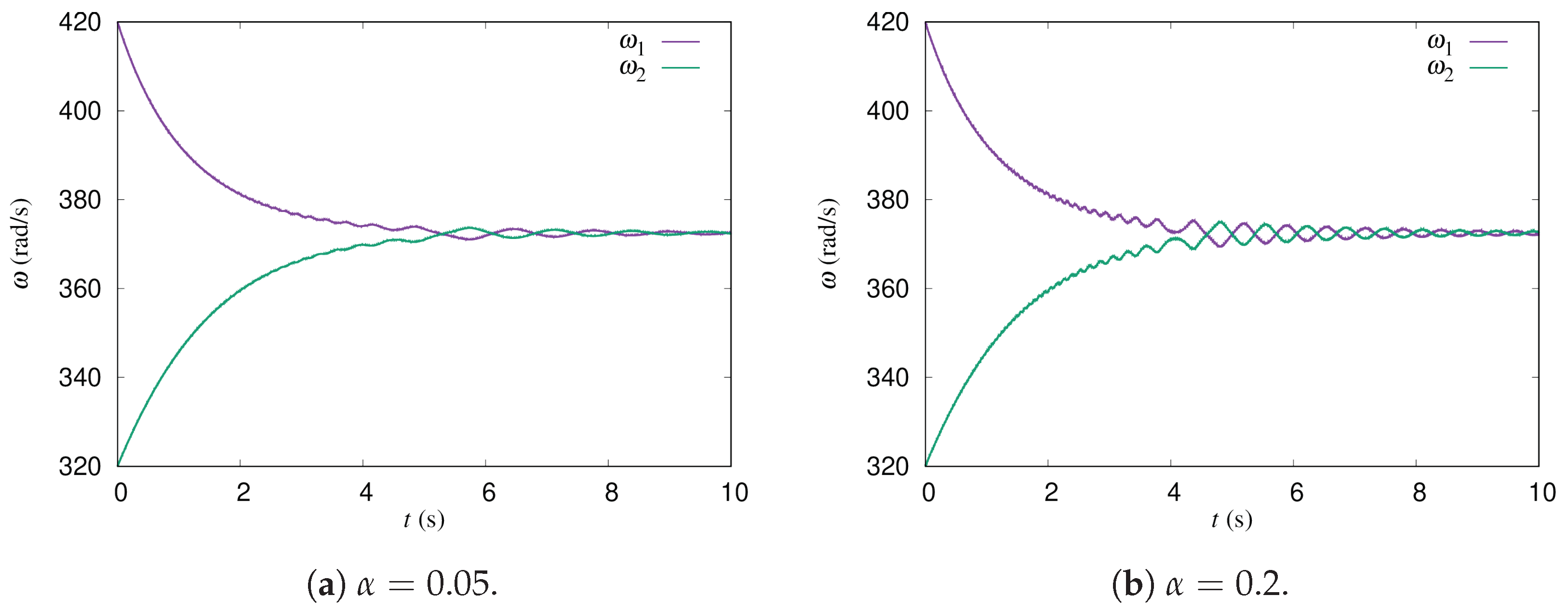
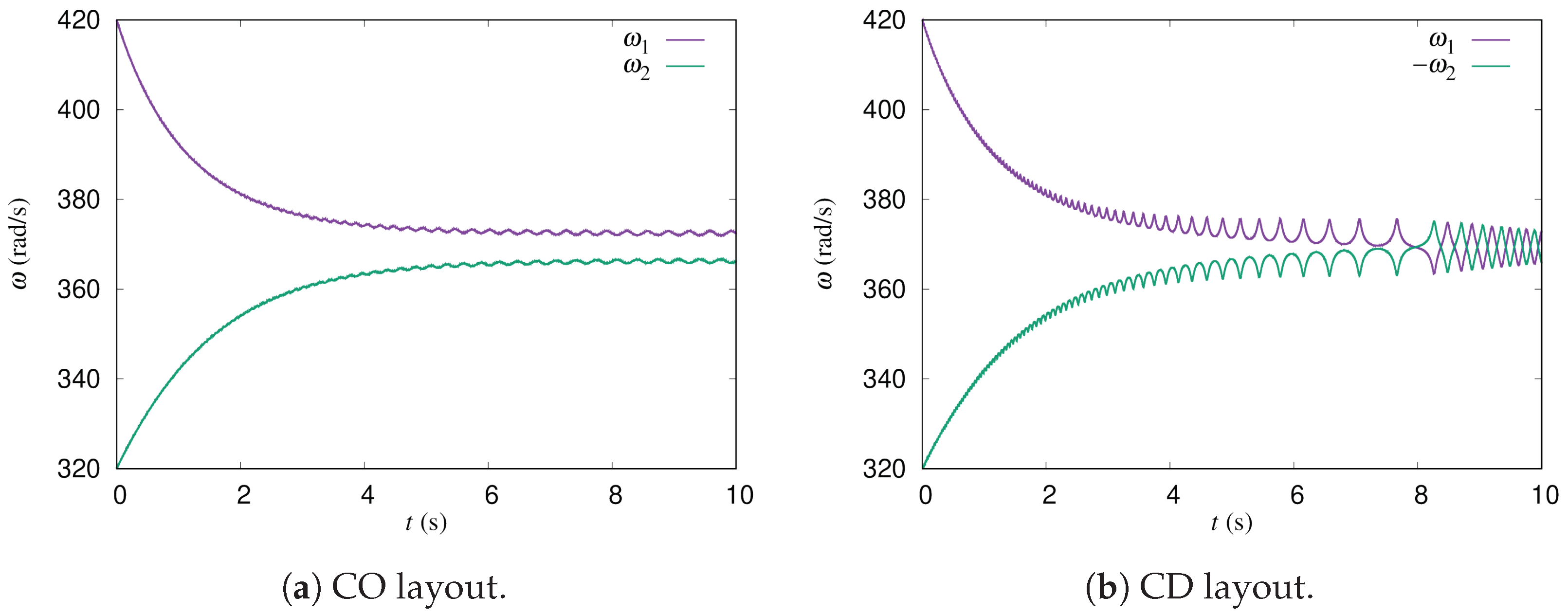
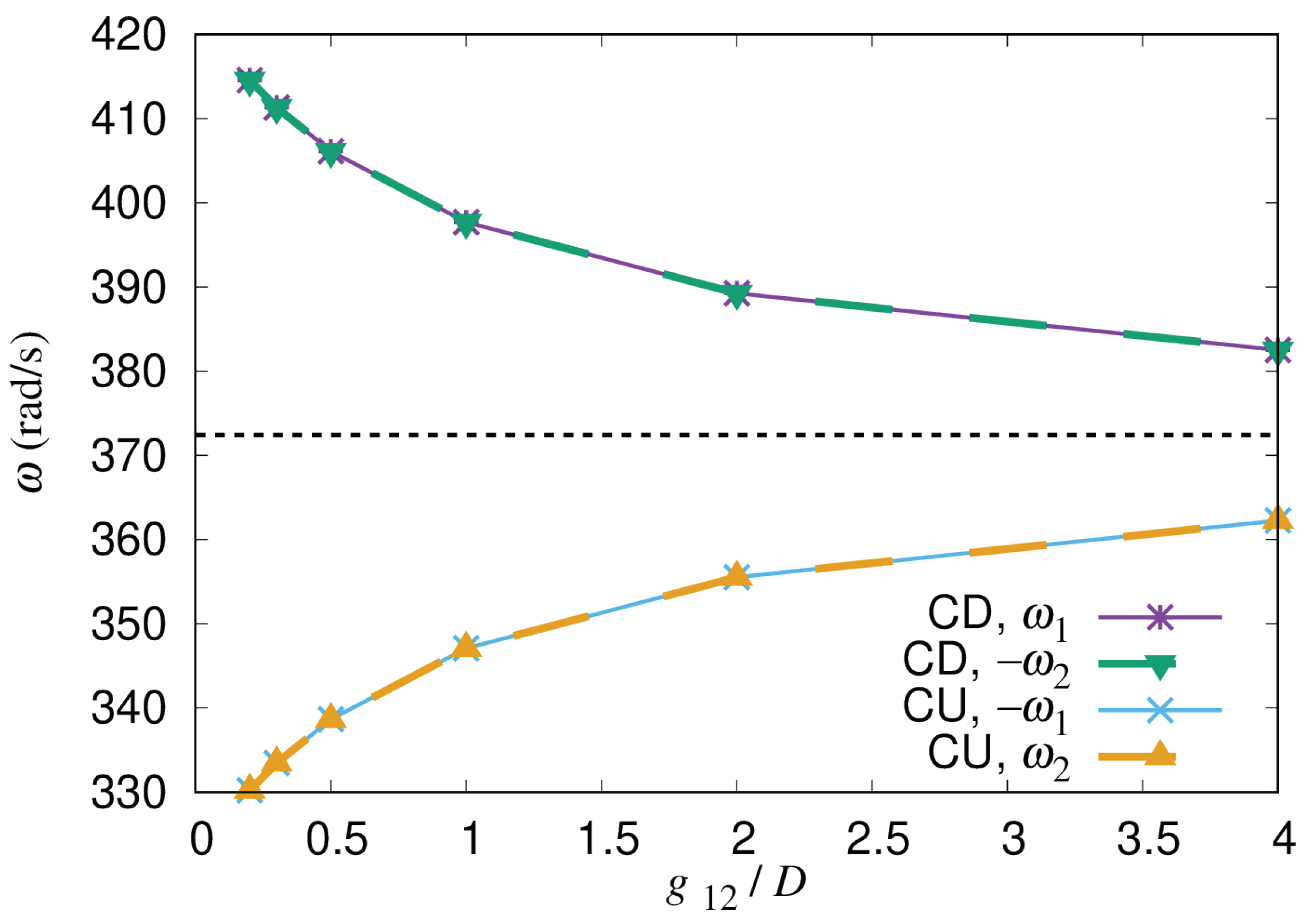
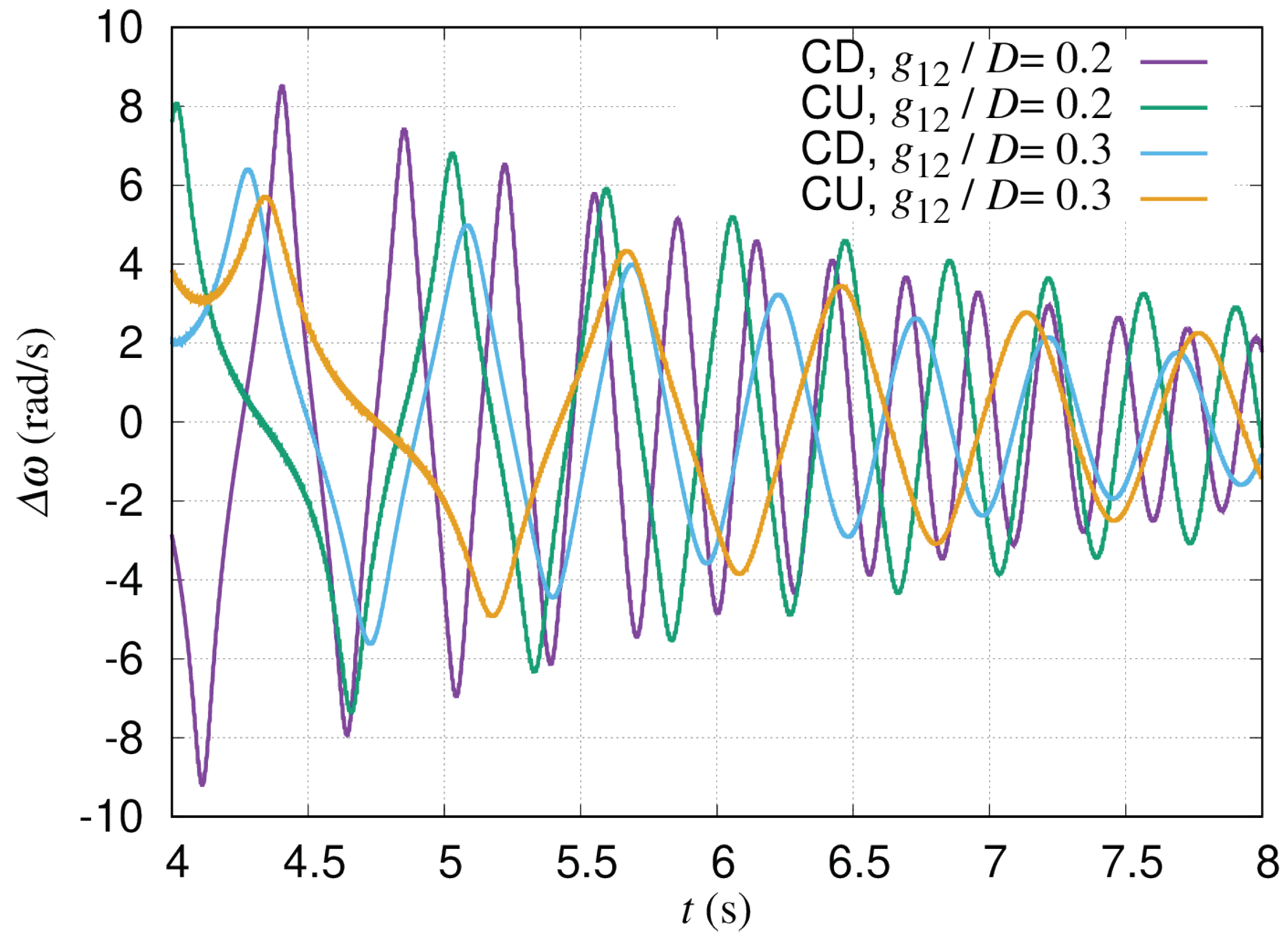
5], wind tunnel experiments of a pair of two-bladed H-type Darrieus turbines were reported, and synchronization of the rotations was found in both counter-down (CD) and counter-up (CU) layouts. Note that, in the CD layout, the blades of the two rotors move in the downwind direction in the gap region between the rotors. In the CU layout, the blades move in the upwind direction in the gap region. It was found that the rotational speeds equalize and the power output increases. Let us call this phenomenon phase synchronization. It was also found that the phase difference between the rotors oscillates around a mean value, and converges to it during the phase synchronization period. However, the mechanism of phase synchronization was not discussed in their papers.
Jodai et al. performed wind tunnel experiments on a pair of closely spaced, small-sized rotors made by a 3D printer and found that phase synchronization occurs when the distance between the rotors is sufficiently small in the CD layout [
7,
8]. It was found that the rotational speed with phase synchronization was 13% greater than that for a single rotor under the extremely small gap condition; the gap distance was 10% of the rotor diameter. This was the first report on the steep rise of the rotational speed under phase synchronization of a pair of closely spaced VAWTs with the extremely small gap. The oscillation of the phase difference was not reported in their papers.
Hara et al. performed a two-dimensional CFD analysis [
13,
14] to simulate the experiments by Jodai et al. They adopted a dynamic fluid–body interaction (DFBI) model that enabled the angular velocities of the rotors to change dynamically. The simulation results showed that phase synchronization also occurred in the CFD analysis for both the CD and CU layouts. They also found that the angular velocities of the rotors oscillated around the mean value during phase synchronization. Note that the oscillation of the angular velocities around the mean value means the same as the oscillation of the phase difference. From the observations of the CFD simulation results, it was proposed that phase synchronization and the oscillation of the angular velocities occur because of the interaction torques generated by pressure fluctuations in the gap between the rotors. In fact, they found that the velocity increases and the pressure decreases according to Bernoulli’s law when blades of the two rotors come closer together in the gap region.
In this study, we developed an analytical model for the interaction torques that can be included in evolution equations of the angular velocities of rotors considered as solid bodies. Such a model is useful to understand the physics of the observed phenomena. Here, we present the details of the derivation, as well as numerical results showing the phase synchronization and the oscillation of the angular velocities. We also perform a simulation of a pair of rotors with slightly different torque characteristics and show that phase synchronization also occurs if interaction torques exist.
This paper is organized as follows. The details of the model, including the derivation of the interaction torques, are explained in
Section 2. Then,
Section 3 shows the numerical results based on the model. In particular, in
Section 3.2, we report that the phase synchronization and oscillation of the angular velocities occur when using our model. We also report the dependence of the angular velocities in the phase synchronization regime on the gap in
Section 3.3. The oscillations of the difference in angular velocities are shown in
Section 3.4. The conclusions are given in
Section 4.
Appendix A shows the verification of our model based on comparison with CFD as well as experimental results, while
Appendix B summarizes normalized expressions of our model.
2. Model
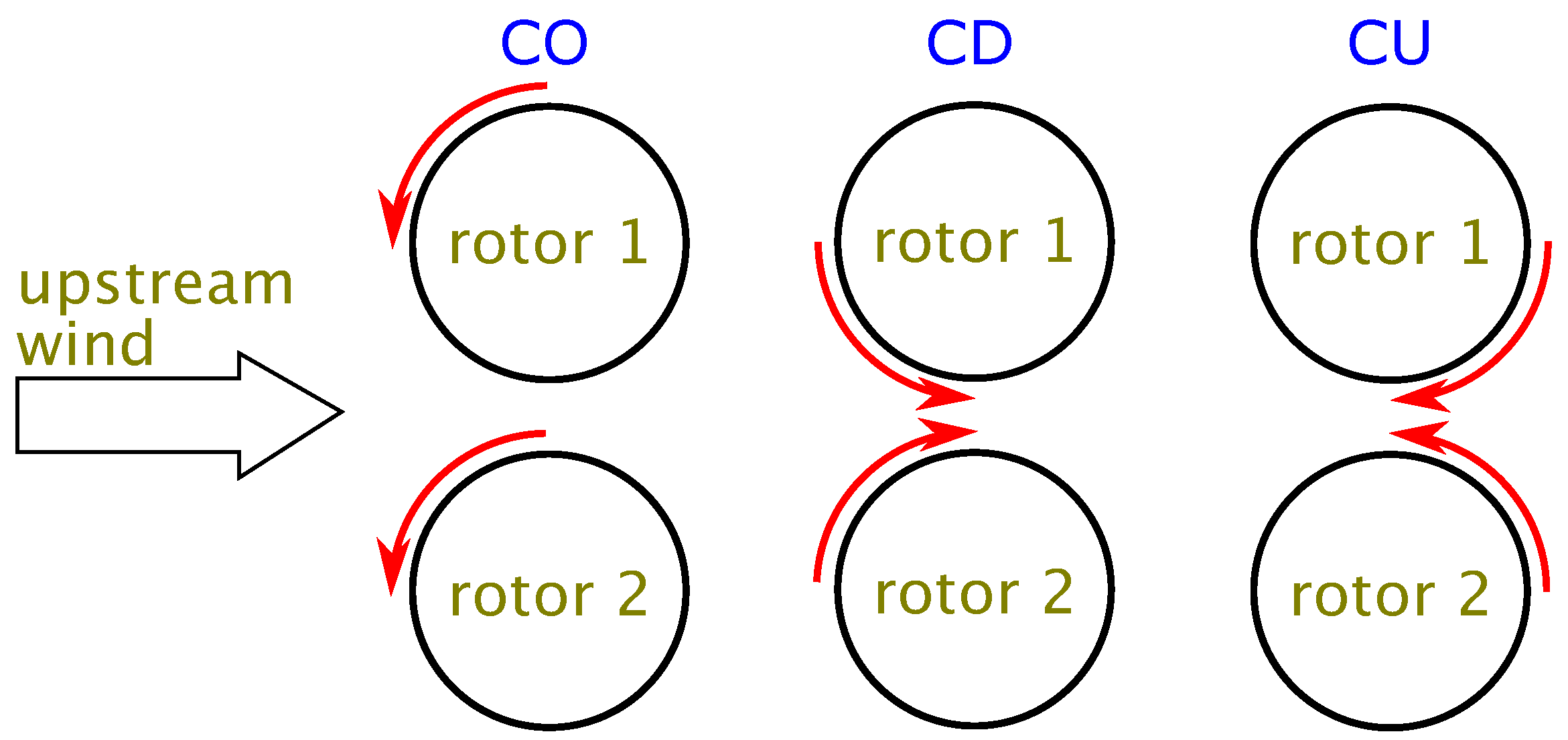
Let us consider a pair of VAWTs. The geometry of the layout is shown in
Figure 1. The upstream wind flows from the left of the figure. The rotation directions of the rotors are shown by the arrows. Co-rotating, Counter Down, and Counter Up layouts are written as CO, CD, and CU, respectively. In the CD layout, the blades of the rotors move in the downwind direction in the gap region between the rotors. In contrast, in the CU layout, the blades move in the upwind direction.
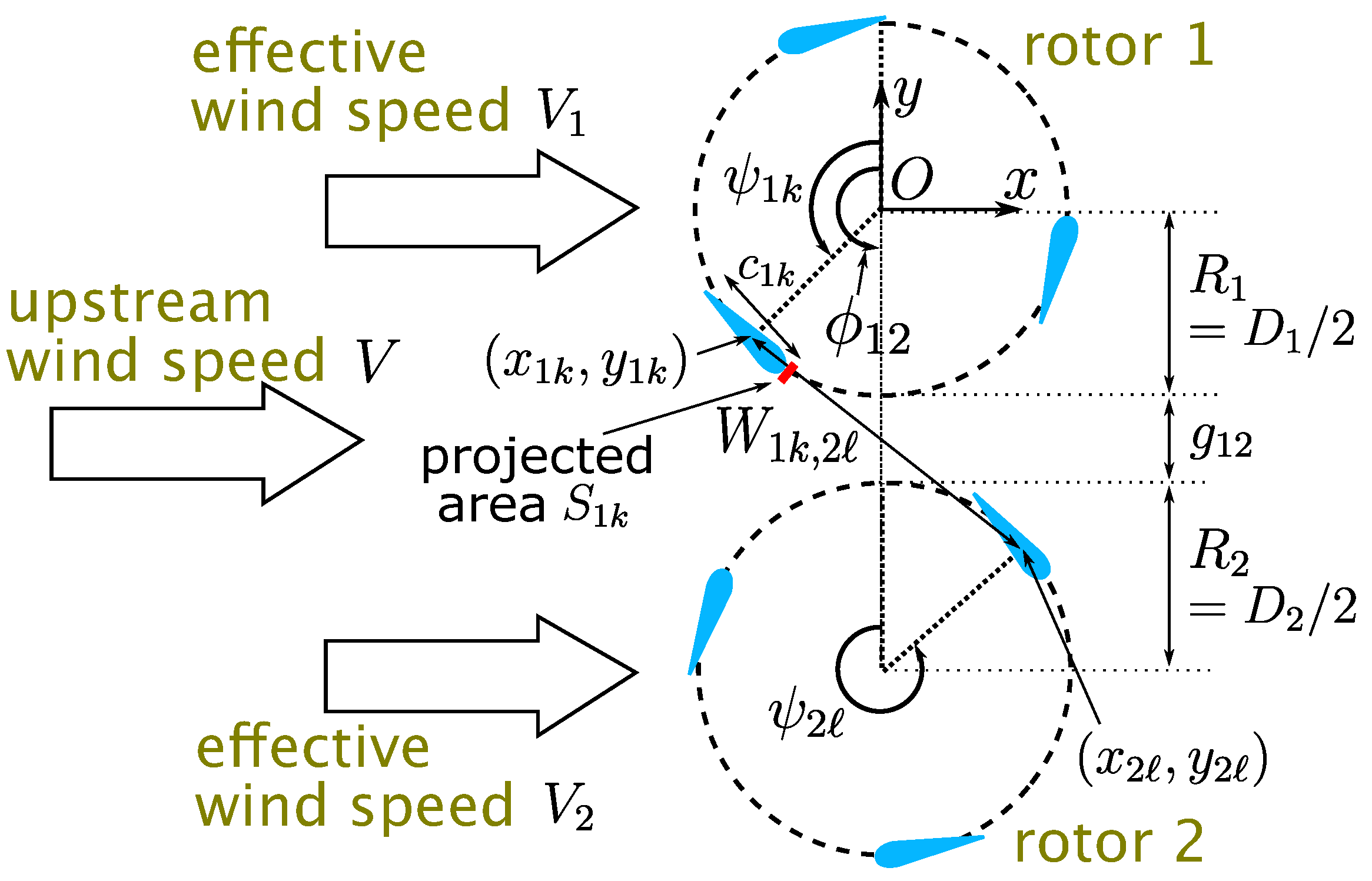
Figure 2 explains the definitions of variables. The diameter and the radius of a rotor
i are denoted by
and
, respectively. The gap between rotors
i and
j is given by
. In this study, we only considered a pair of rotors. A rotor
i has
blades, and the position of a blade
k is expressed as
in the two-dimensional plane, of which the origin is at the center of rotor 1. An azimuthal angle of a blade
k of a rotor
i is expressed by
, which is zero in the direction of the
y axis and increases in the counterclockwise direction. The blades of a rotor are equally spaced in the azimuthal angle, and thus the angle between the neighboring blades is
. The chord length and the area projected along the rotation direction of a blade
k of a rotor
i are denoted
and
, respectively.
The distance between the blade
k of the rotor
i and the blade
ℓ of the rotor
j is written as
, which varies in time due to the rotation. As we explain later in this section, we adopted a model to describe a change in the flow velocity according to the temporal change of
, leading to a pressure fluctuation between the blades according to Bernoulli’s law. The center of the rotor
j is in the direction of an angle
seen from the center of rotor
i. As with the azimuthal angle
, the angle
is also zero in the direction of the
y axis and increases in the counterclockwise direction. The angle of the center of the rotor
i seen from the center of the rotor
j is
, although only
with
and
is shown in
Figure 2.
The wind flows in the direction of the x axis. The upstream speed is denoted by V. The flow velocity experienced by the rotor differs for each rotor. The effective flow velocity immediately in front of rotor i is written as .
As mentioned above, we only considered a pair of rotors. The evolution equations governing the rotation are
where
represent the two rotors,
is the angular velocity,
is the azimuthal angle of a representative blade,
is the moment of inertia,
is the rotor torque,
is the load torque, and
is the torque due to the interaction with the other rotor
through pressure fluctuation in the gap between them. Note that
is not necessarily
but can be another
with
; the meaning is the same for any choice of
.
In the following section, we explain our torque models. First, we take the rotor torque
as
This torque, without the last term
, simulates the torque characteristics in the two-dimensional CFD by Hara et al., as shown in Figure 2 of Ref. [
14]. Note that the rotor is operated at an angular velocity, in a sense of average over phase, where the torque in Equation (
3) is balanced by a load torque introduced below. Since the balanced state occurs at an angular velocity larger than that at the maximum torque in the present choice of the load torque for a given flow velocity, the rotor torque characteristics at small angular velocity regime is not essential. Here,
expresses a modulation in the rotor torque depending on the angle of the blades, which we explain shortly. Without this modulation, or setting
,
is a cubic function of
for a given
and takes a maximum value
when
on the positive
side. When
at the positive
side, the rotor torque becomes zero. Thus,
is sometimes called no-load angular velocity.
Here, we take
where
and
are constants. As shown in
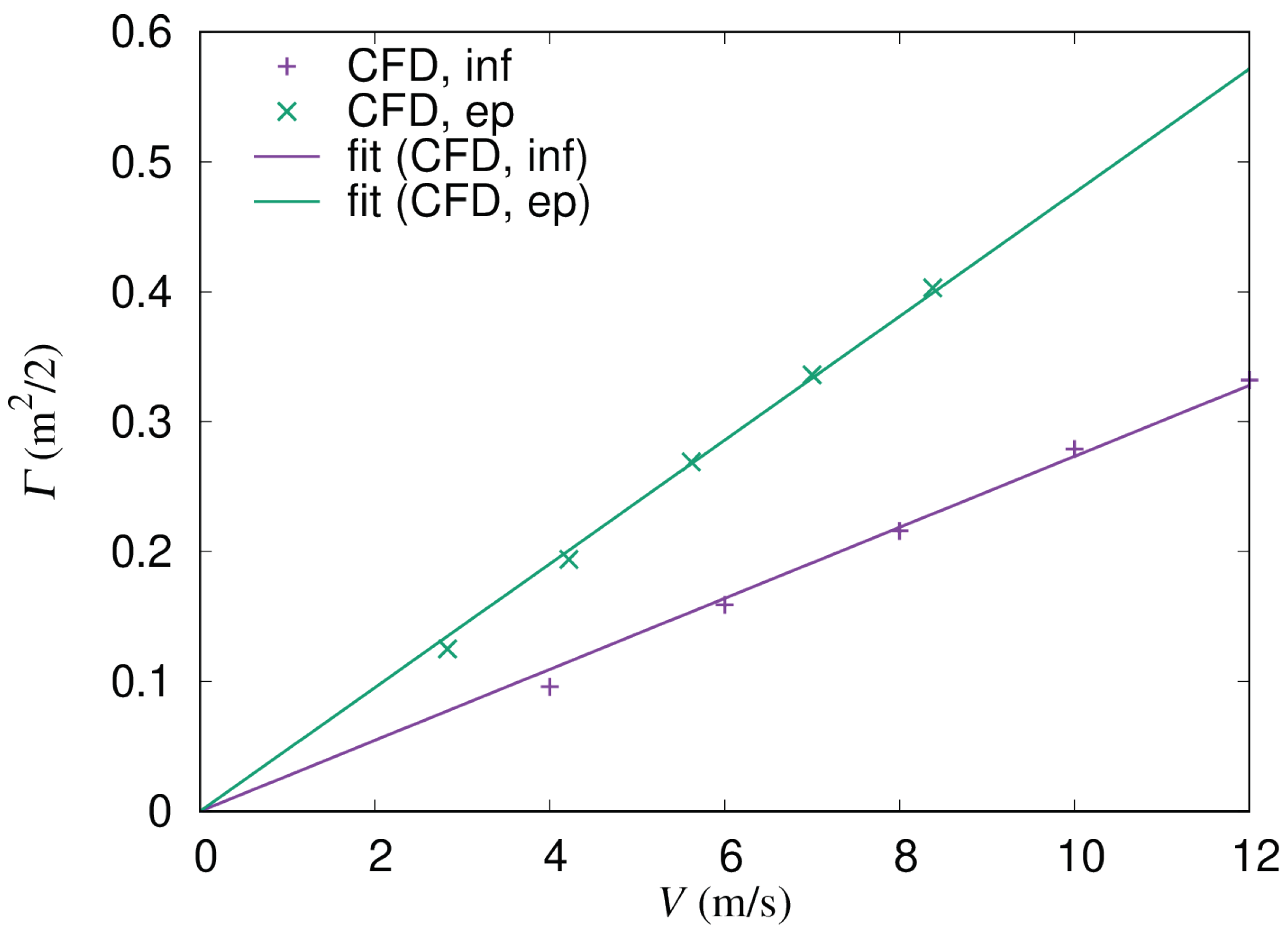
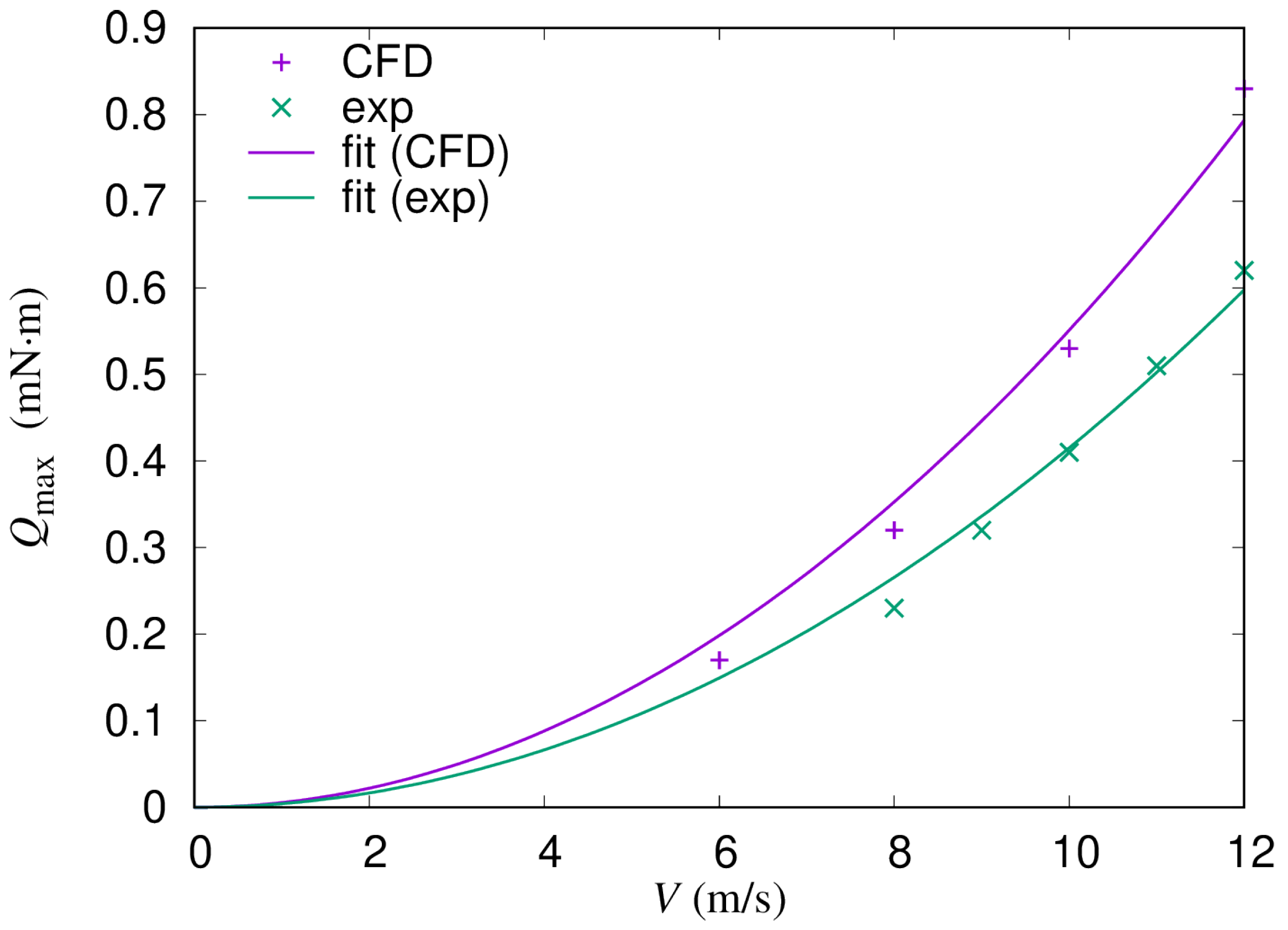
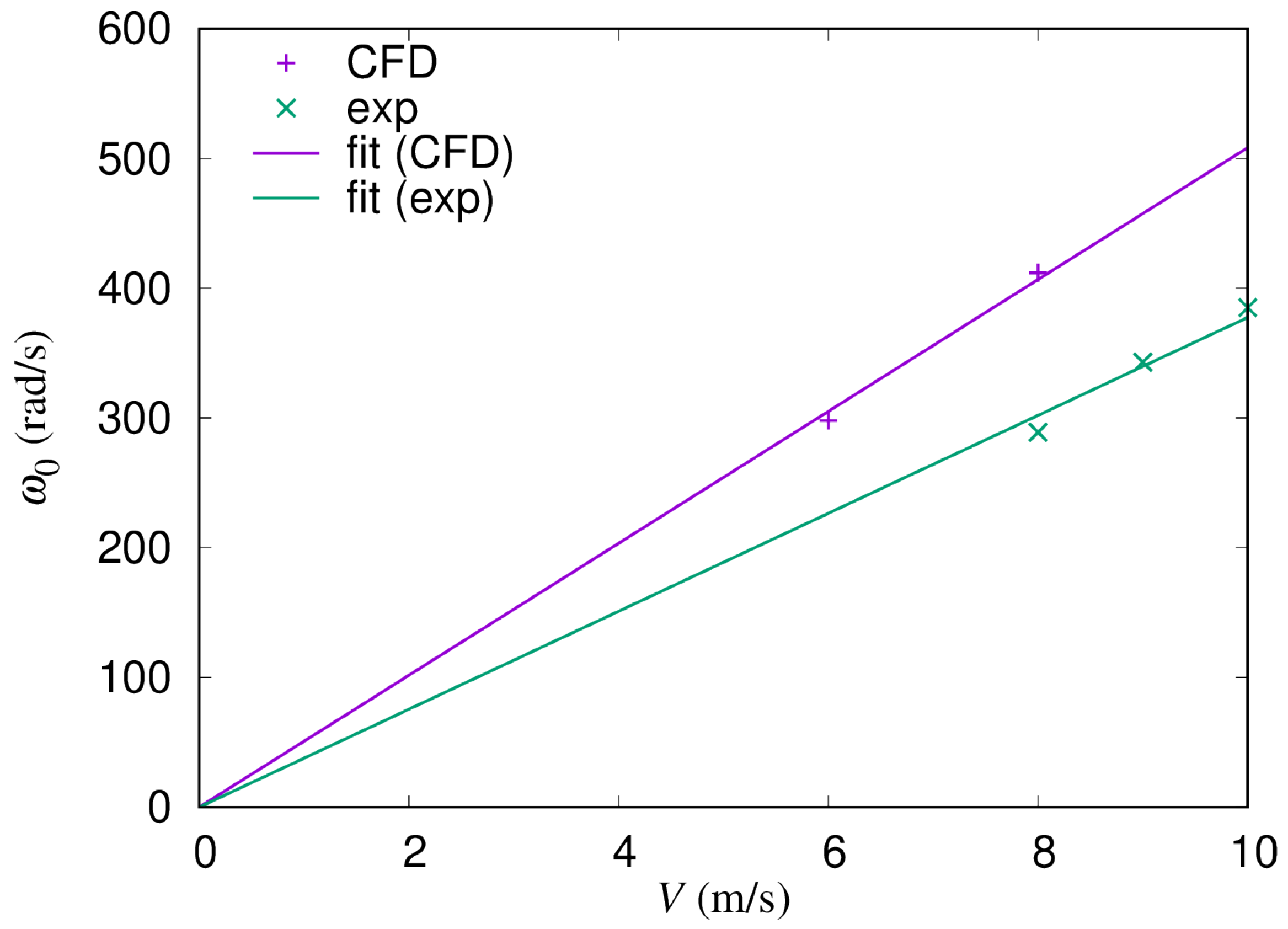
Appendix A, we chose these expressions by observing the CFD [
13,
14], as well as the experimental [
7,
8] data. The actual values used in our simulation are given in the next section. Note that normalized expressions of Equations (
3)–(5), as well as equations to appear below are given in
Appendix B, where it is shown that the normalized rotor torque
is expressed by a tip–speed ratio
for the rotor
i and normalized
and
only.
The average of
over
is taken to be unity. As shown in
Appendix A, it is known that the rotor torque by a single blade is finite at the upstream side and becomes maximal when a blade comes to the position
. It decreases to almost zero at the downstream side when the rotor solidity
is large. We assume that
for a single blade.
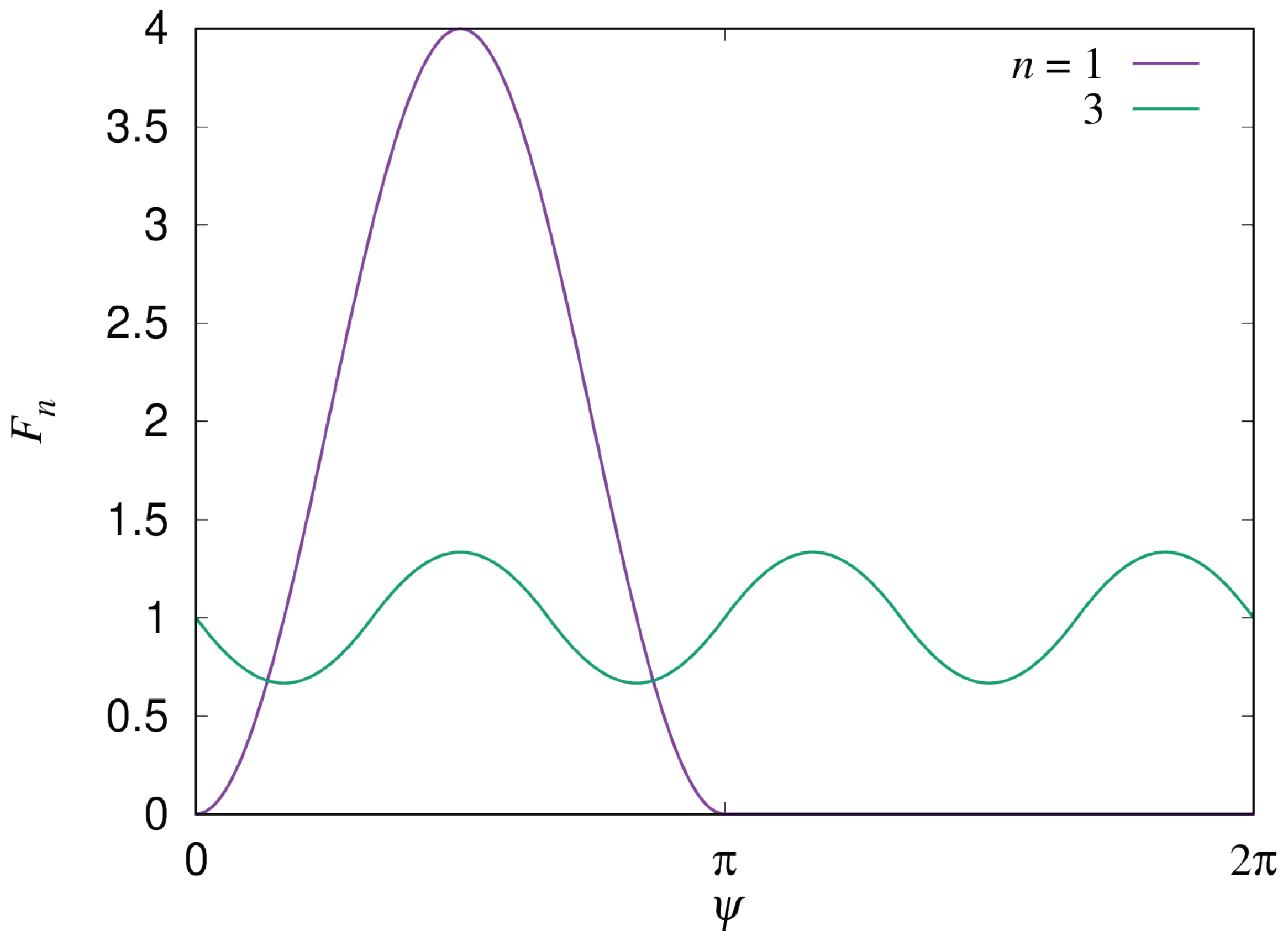
Figure 3 shows the azimuthal angle
dependence of
. In the figure,
plots Equation (
6). The curve of
plots a summation of the modulation function for
over three blades equally spaced in the azimuthal angle and normalized such that
. Note that the function for the modulation can be rather flexibly chosen since it is enough to simulate qualitative aspects of the torque modulation. Any function with its maximum at
and almost zero in the downstream half may be acceptable.
For the effective wind speed, we use
where
is a coefficient of self-induced velocity, and
is the circulation of rotor
j. The second term expresses the velocity induced by another rotor. This assumption, assigning a circulation for a rotor, is similar to the one in Ref. [
16]. We take
where
is a constant. This dependence was also obtained by the CFD results as shown in
Appendix A. By using the effective wind speed
, the rotor torque on rotor
i can be calculated. Note that
in Equation (
7), especially its mutually induced velocity in the second term of the right-hand side, is evaluated by using the coordinate of the center of rotor
i. In reality, the effective flow velocity is different for each blade, and the summation of the torques on every blade of a rotor determines the rotor torque. However, in this study, the effective velocity
is used for calculating the rotor torque as an average of the effective velocities for all blades in the rotor, and we assume that
is the flow velocity immediately in front of rotor
i. Instead of considering the torques on each blade, we take into account the torque modulation of the rotor torque via
. The modulation expresses the fact that the blade experiences significantly smaller flow than the upstream when it is in the downstream half of the rotation.
Secondly, we take the load torque as
The dependence, square of angular velocity, is known as the ideal load torque to obtain highest power at each instance [
17]. The CFD simulations by Hara et al. [
13,
14] also adopted the ideal load torque of the same form. Within our torque model, it is shown that the load torque in Equation (
9) works to keep the highest power as follows. According to the rotor torque in Equation (
3), the power
takes a maximum value when
, and the corresponding rotor torque is
. By using Equations (4) and (5), these are written such that the maximum power is obtained when
and the corresponding torque is
. The ideal operation of the turbine is achieved by balancing the rotor torque
by the load torque
for whatever
. This is realized by setting
, which is obtained by eliminating
in
Q by using
. The coefficient
is determined so that the load torque takes 95% of
when the power becomes maximal for a given
in
Section 3.1.
Finally, we come to the torque caused by pressure fluctuations between the blades. The positions of the blades of rotors 1 and 2 are given by
The distance
between two blades at
and
is
Here, we consider the flow to be incompressible and assume that
where
is the average flow velocity, and
expresses the change in the flow velocity between the two blades due to a change in distance between them. Note that the calculation of
may be improved by taking not only the
x component of the flow velocity but also the
y component, or taking the distance in the
y direction between the blades
k and
ℓ instead of the distance
itself. Let us leave this refinement as a future issue. We roughly estimate the change of velocity
in this study. Then, we obtain the pressure fluctuation
using Bernoulli’s law
as
Here, the air pressure is written as
p. Note that we neglected the effects of viscous force and unsteadiness of the flow. We adopted the Bernoulli’s law to explain interactions between the blades due to pressure fluctuation through the increase in the flow velocity in the
x direction observed in the CFD results, as shown in Figure 20 of Ref. [
14]. The force on a blade due to this pressure fluctuation is calculated by integrating
with the blade volume. The necessary force component is that along the rotation direction
for the blade
k of the rotor
i. As an example, for
, this is calculated as
By using Equation (
15),
Then, we can obtain
The torque on a blade due to pressure fluctuations may be approximated by multiplying the blade volume
by the rotor radius
. Now, we also assume that the total torque on a rotor can be obtained by adding contributions from all blades. Then, we obtain
Here, a coefficient
is included to control the strength of the interaction between the blades due to the pressure fluctuation, since the summation over all blades may be rather rough. Readers may think that it is strange to add a contribution from a blade at the opposite side of the gap between the rotors; it is natural to count only contributions from blades in the gap region. We also considered a model where rotors are taken as solid cylinders and only considered a narrowed channel between the gap region. This may not be a bad choice for high solidity rotors; however, the flow goes into the region surrounded by the blades of a rotor in reality. Thus, we decided to consider all combinations of blades and to add contributions from all pairs of blades in our model as an average in a sense, although big contributions must arise when two blades come to the gap region, and thus, other contributions may not play major roles. From a numerical computation viewpoint, this assumption, summation over all blades, is far simpler than taking the azimuthal angle of each blade into account to judge whether we should add its contribution or not. The on/off nature of the contributions can yield abrupt changes in torque, leading to a strange time evolution. Thus, we avoid this confusion.