A Perturbation-Based Methodology to Estimate the Equivalent Inertia of an Area Monitored by PMUs
Abstract
:1. Introduction
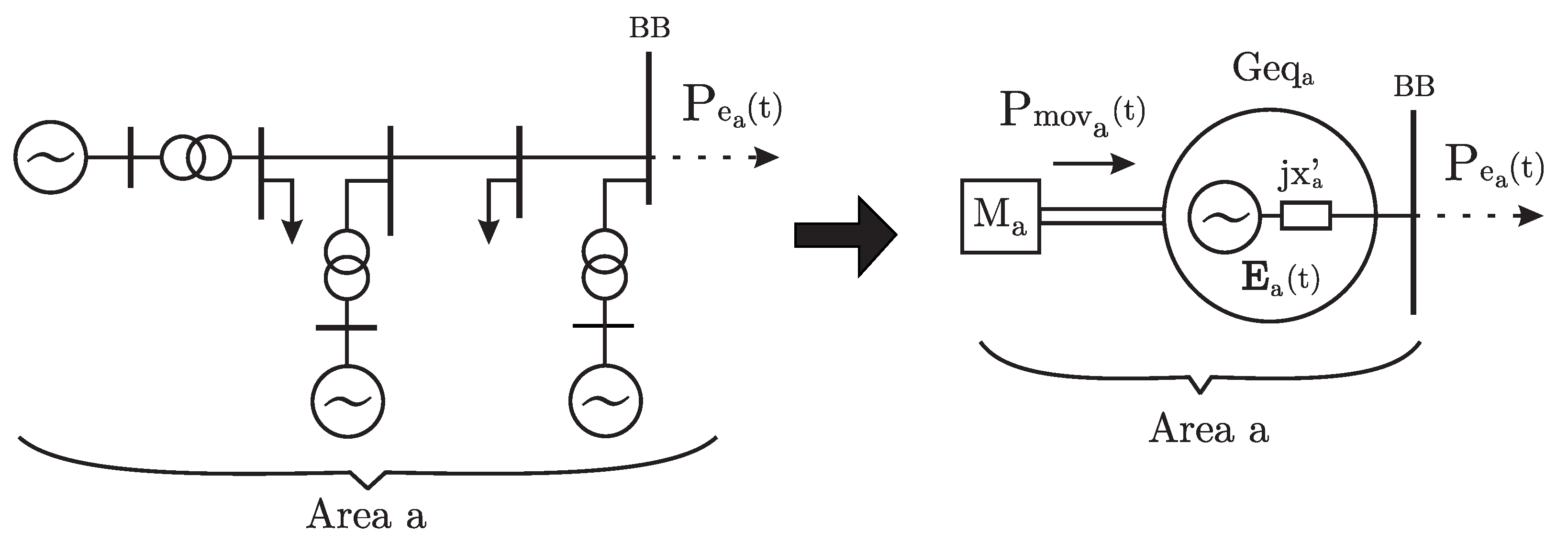
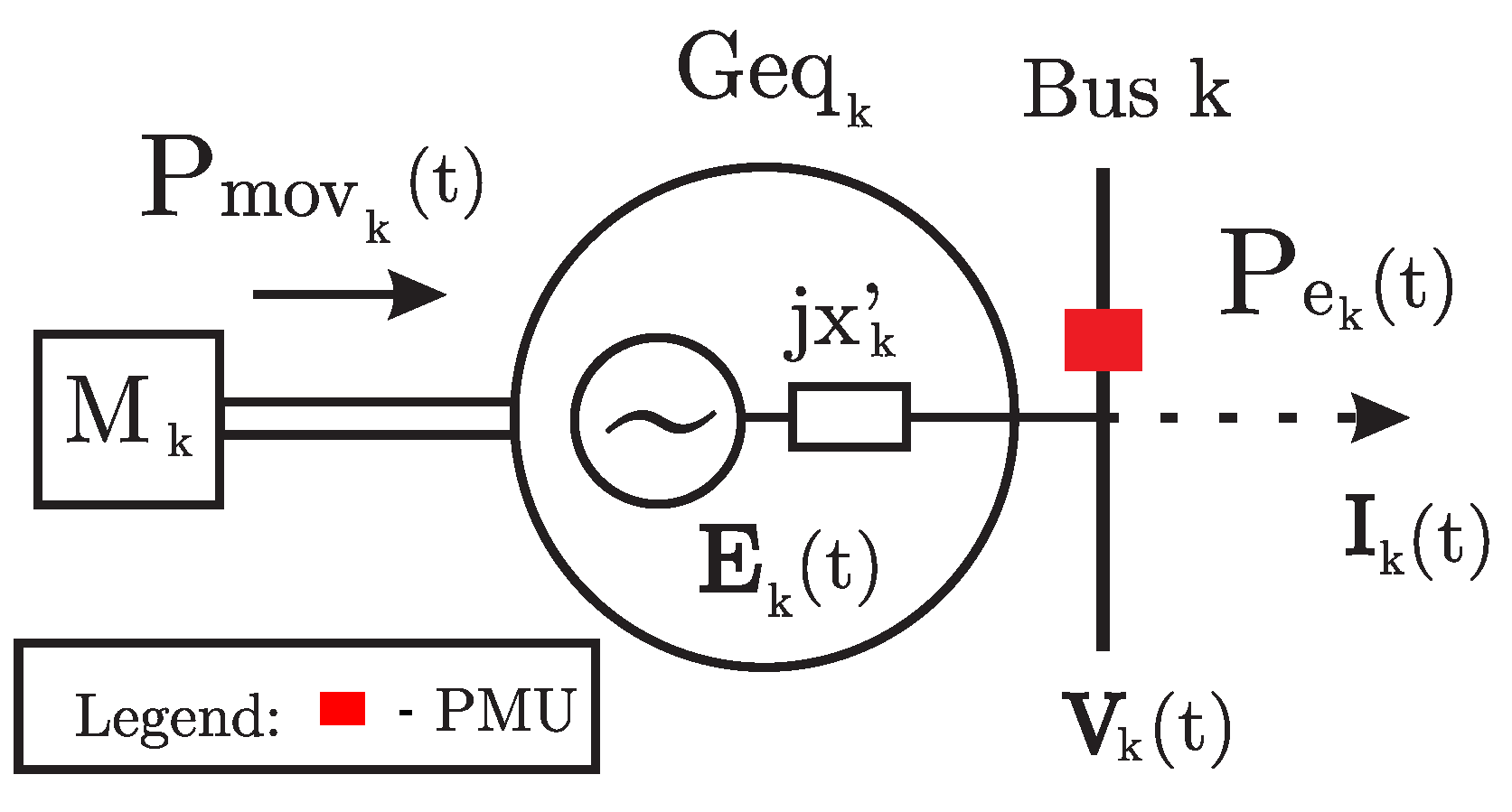
2. The Dynamic Behavior of an Area
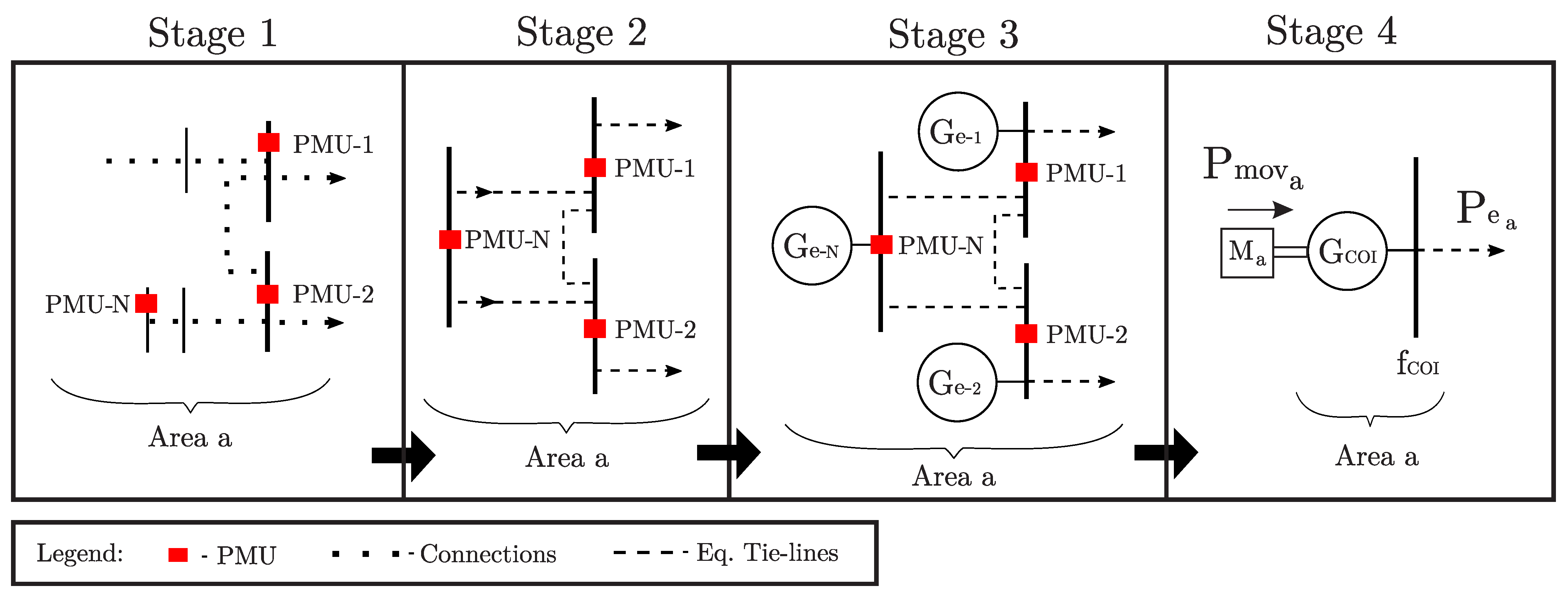
3. Methodology
3.1. Determination of the COI Dynamics
3.2. Determination of the Equivalent Moving Power
3.2.1. Estimation of the Dynamic Behavior of the Loads
3.2.2. Estimation of the Equivalent Power Generated
3.2.3. Estimation of the Equivalent Mechanical Power
3.3. Estimation of Inertia
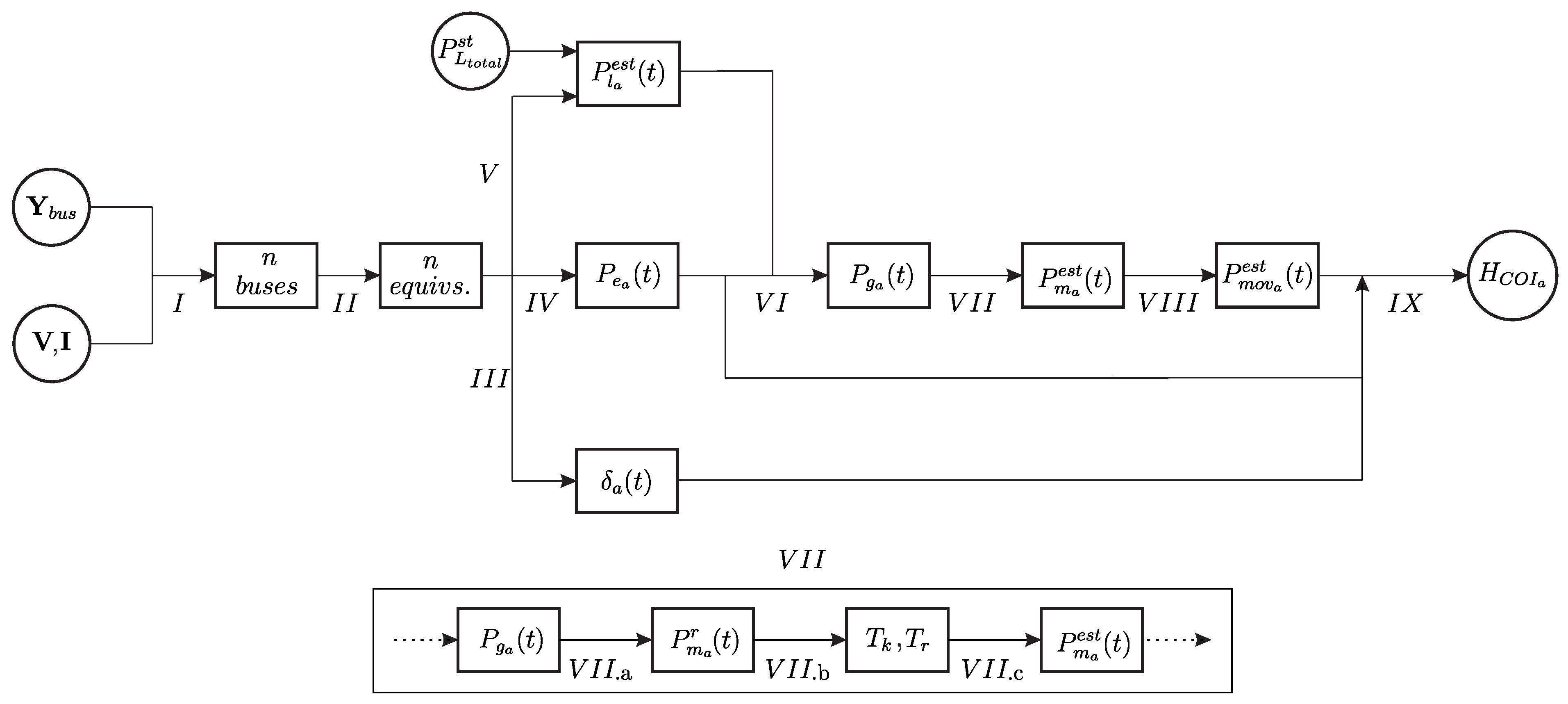
3.4. Summary of the Methodology
- I
- –Ward Equivalent method to reduce the system around the n buses where PMUs are installed (described in Section 3.1).
- II
- –Estimation of the n equivalent generators using the “Variance method” (described in Section 3.1).
- III
- –Calculation of through Equation (22).
- IV
- –Obtainment of the power injected from the PMUs at the boundaries.
- V
- –Estimation of the equivalent load through Equation (25).
- VI
- –Estimation of through Equation (29).
- VII
- –Estimation of the equivalent moving power
- (a)
- –Estimation of through data-fitting (described in Section 3.2.3).
- (b)
- –Estimation of and through model identification (Section 3.2.3).
- (c)
- –Estimation of through Equation (33).
- VIII
- –Estimation of through Equation (34).
- IX
- –Estimation of through Equation (35).
4. Results
4.1. Validation Study
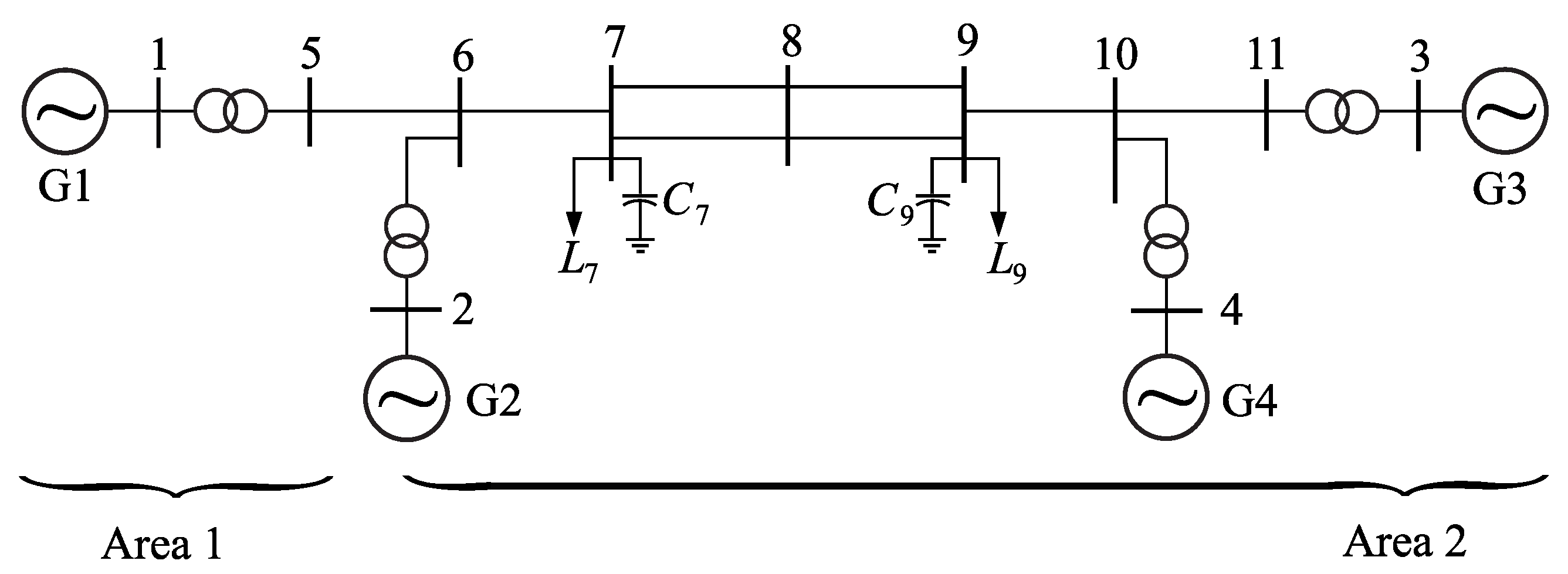
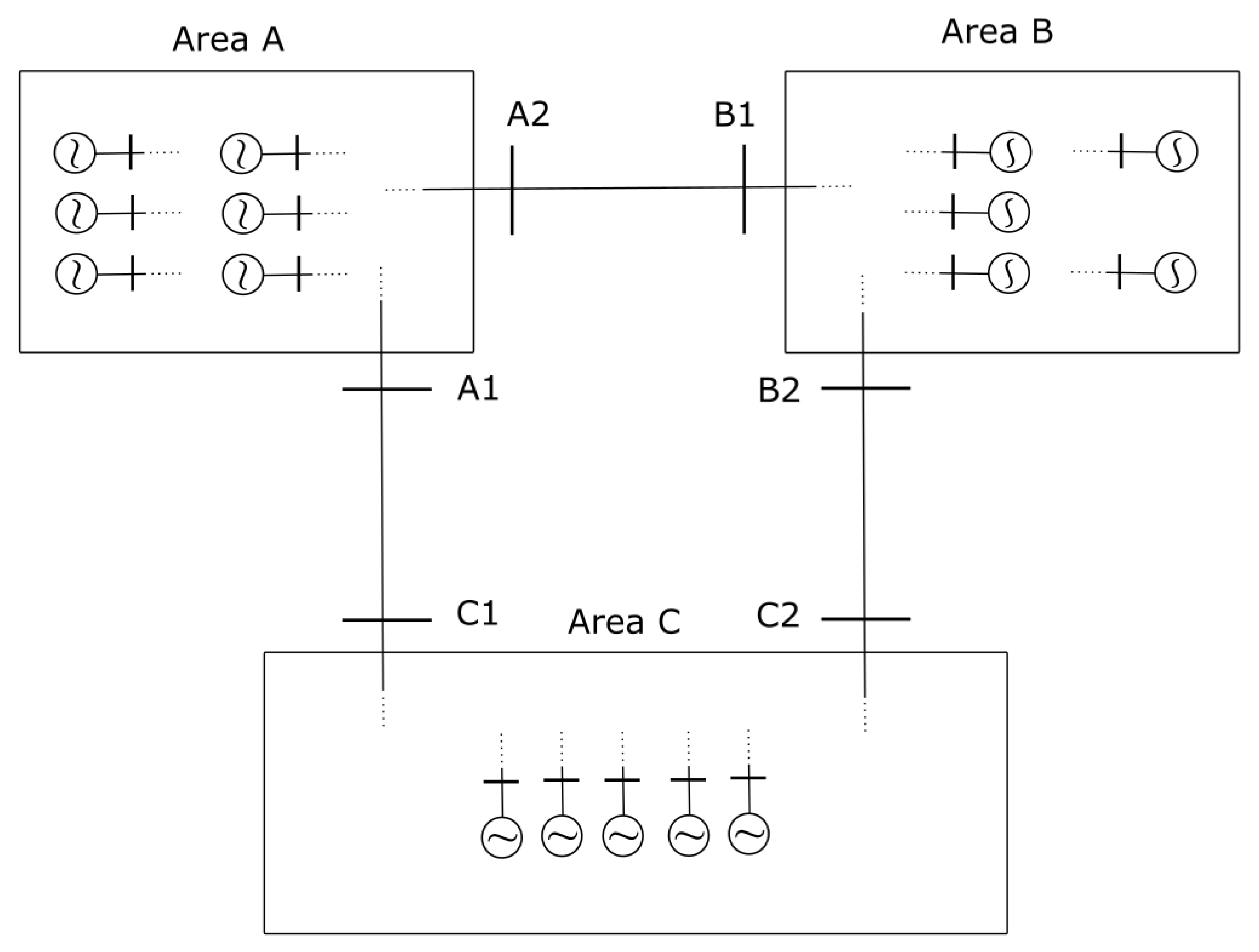
4.1.1. Test System
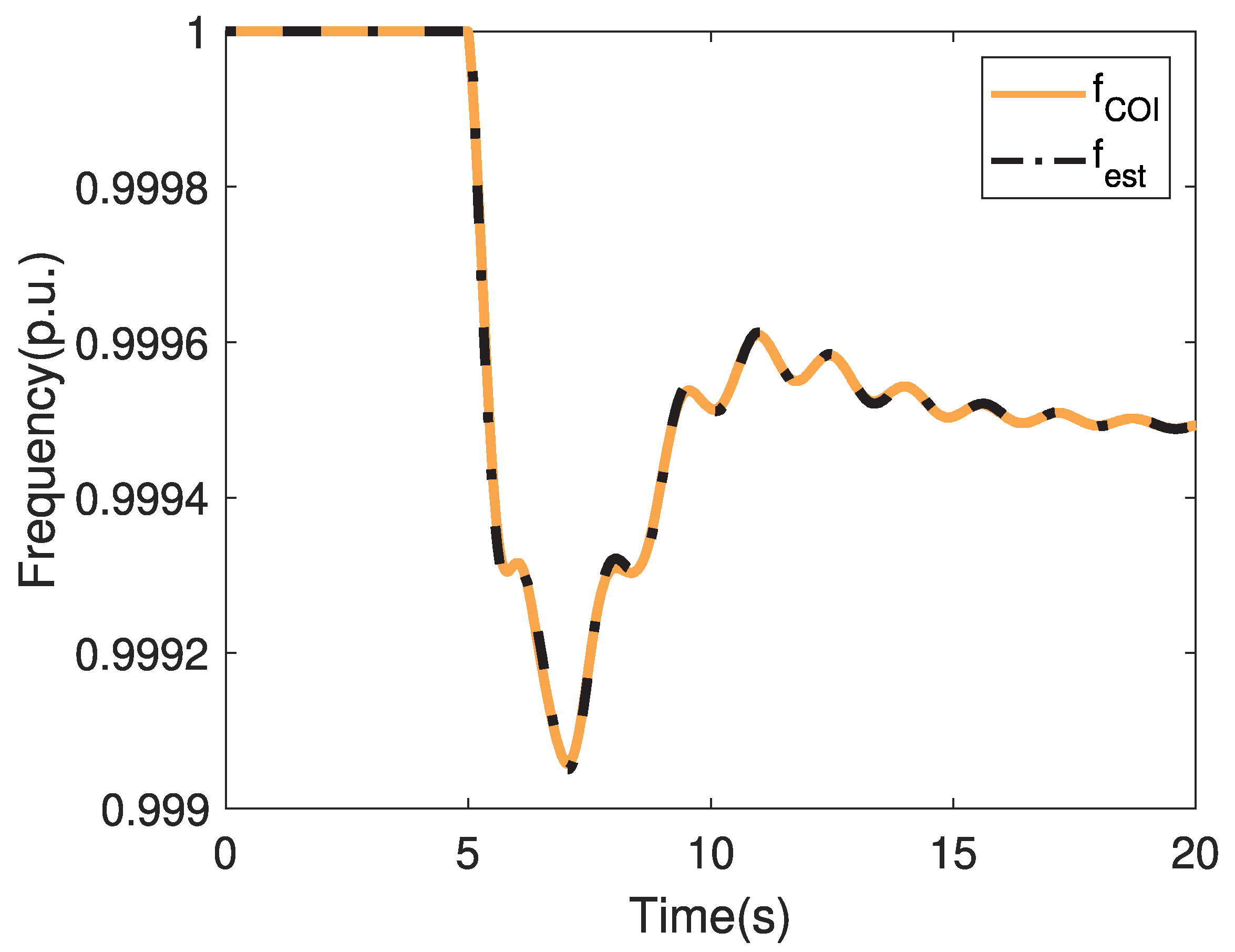
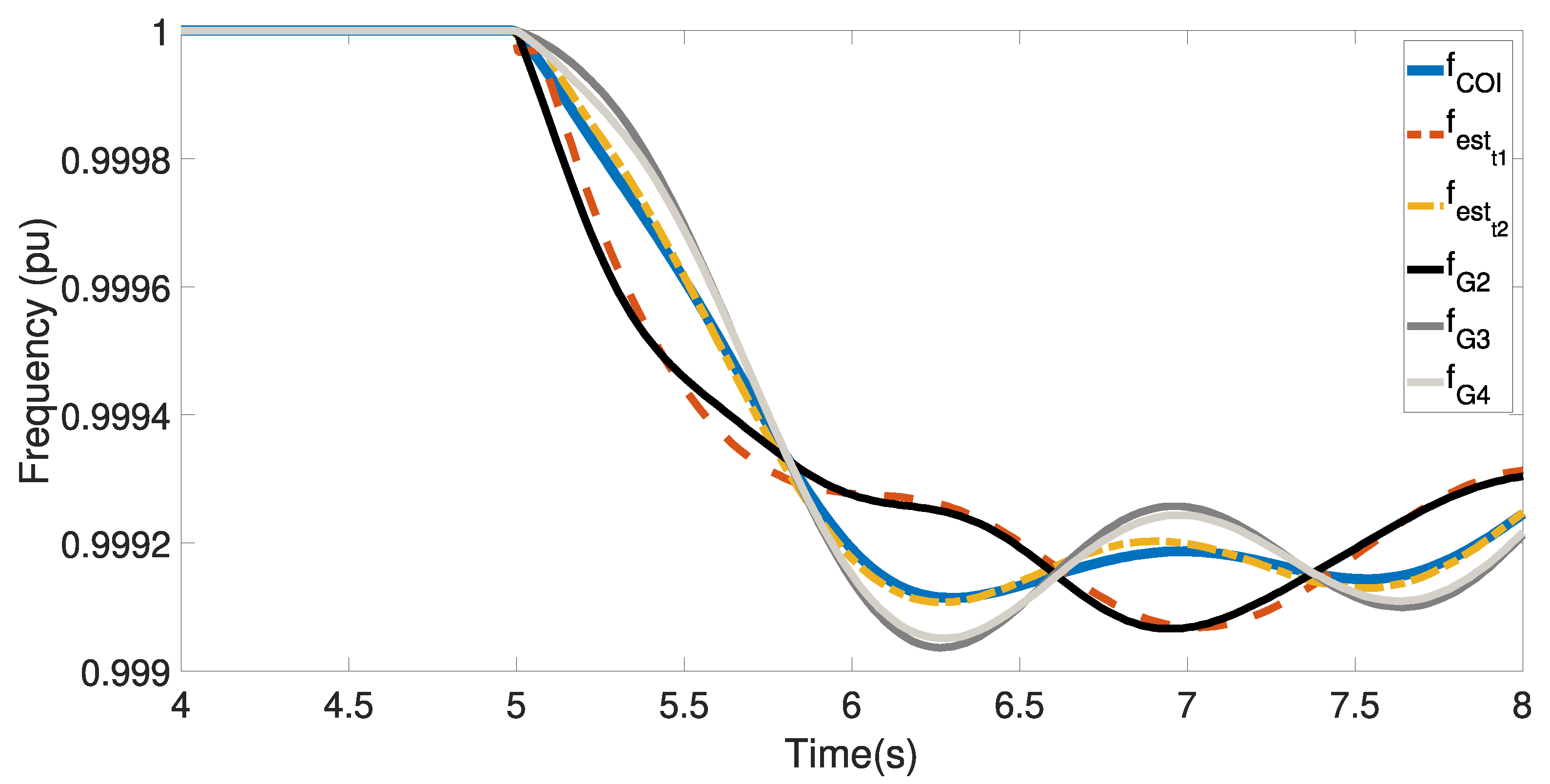
4.1.2. Estimation of the Mean Frequency
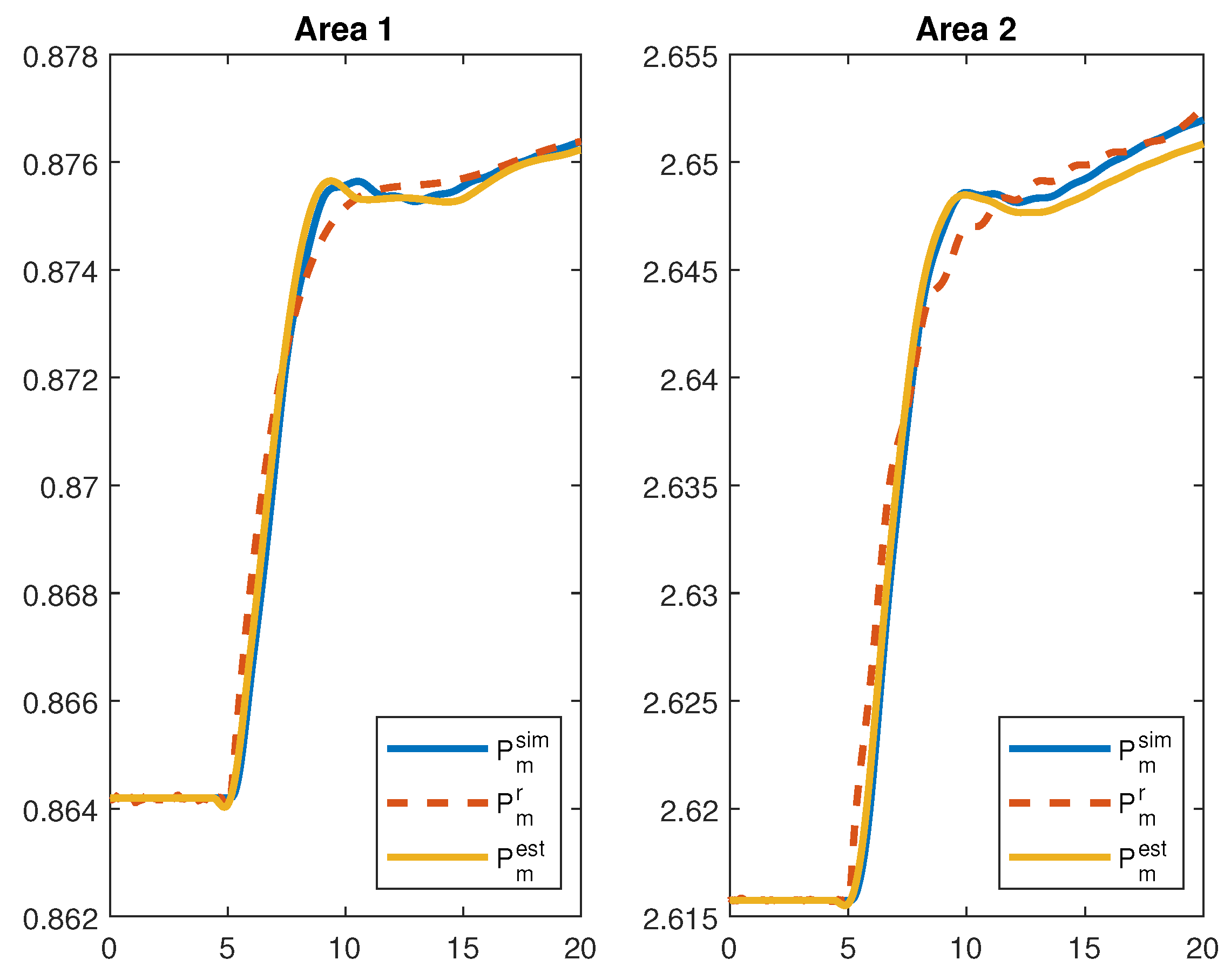
4.1.3. Estimation of the Moving Power
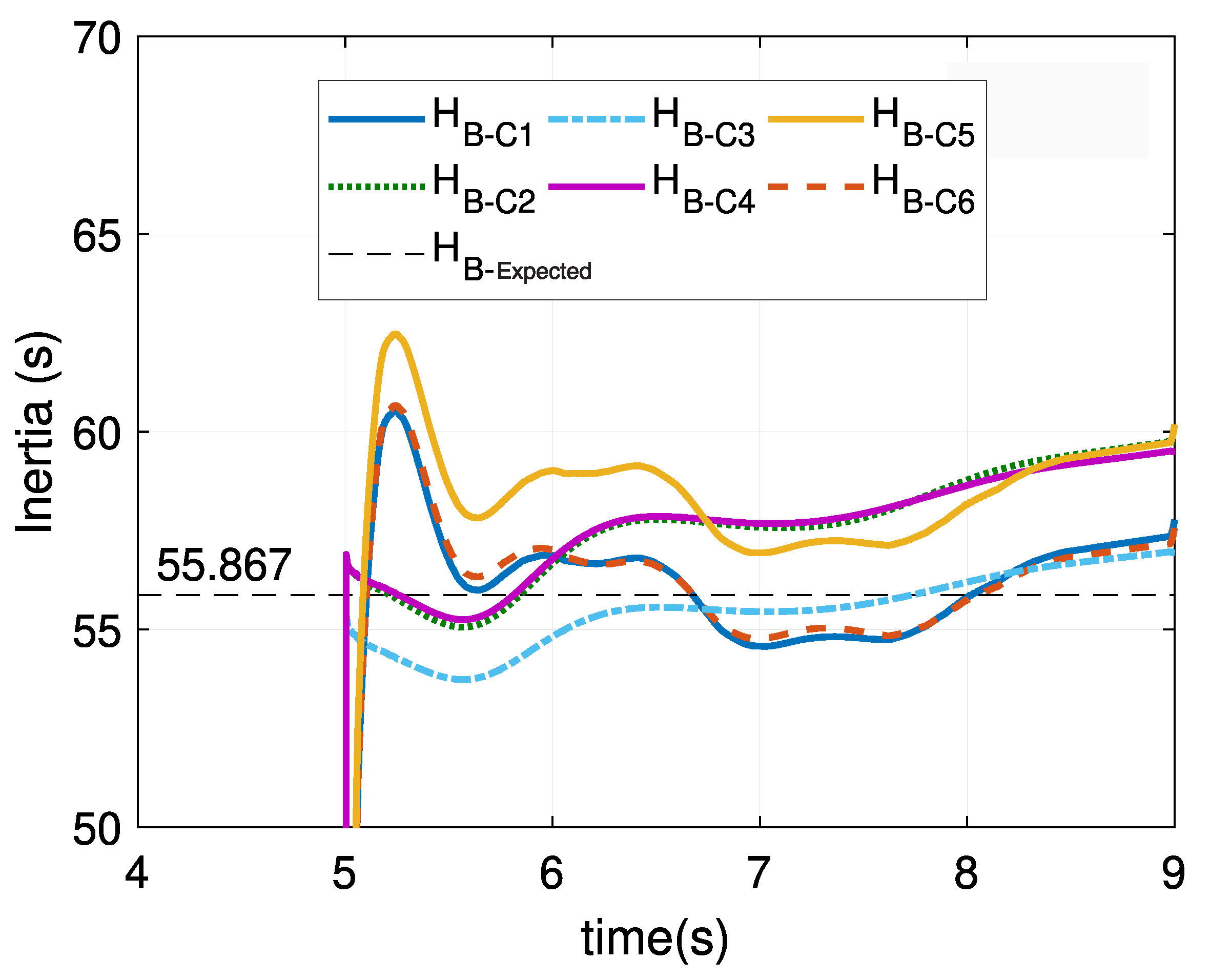
4.1.4. Inertia Estimation
4.1.5. Inertia Variation
4.2. Performance Evaluation Studies
4.2.1. Test System and Study Cases
4.2.2. Results
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| PMU | Phasor Measurement Unit |
| LSM | Least-Squares Method |
| RES | Renewable Energy Sources |
| TSO | Transmission System Operator |
| COI | Center of Inertia |
| WP | Wind Power |
| BB | Boundary Bus |
| RoCoF | Rate of Change of Frequency |
References
- Arani, M.F.M.; El-Saadany, E.F. Implementing Virtual Inertia in DFIG-Based Wind Power Generation. IEEE Trans. Power Syst. 2013, 28, 1373–1384. [Google Scholar] [CrossRef]
- Bolzoni, A.; Terlizzi, C.; Perini, R. Analytical Design and Modelling of Power Converters Equipped with Synthetic Inertia Control. In Proceedings of the 2018 20th European Conference on Power Electronics and Applications (EPE’18 ECCE Europe), Riga, Latvia, 17–21 September 2018. [Google Scholar]
- Rezkalla, M.; Pertl, M.; Marinelli, M. Electric power system inertia: Requirements, challenges and solutions. Electr. Eng. 2018, 100, 2677–2693. [Google Scholar] [CrossRef] [Green Version]
- AEMO. Inertia Requirements Methodology; Technical Report; Australian Energy Market Operator (AEMO): Melbourne, Australia, 2018. [Google Scholar]
- Malik, O.P. Evolution of Power Systems into Smarter Networks. J. Control Autom. Electr. Syst. 2013, 24, 139–147. [Google Scholar] [CrossRef]
- Kim, D.I.; Chun, T.Y.; Yoon, S.H.; Lee, G.; Shin, Y.J. Wavelet-Based Event Detection Method Using PMU Data. IEEE Trans. Smart Grid 2017, 8, 1154–1162. [Google Scholar] [CrossRef]
- Bosisio, A.; Berizzi, A.; Moraes, G.R.; Nebuloni, R.; Giannuzzi, G.; Zaottini, R.; Maiolini, C. Combined use of PCA and Prony Analysis for Electromechanical Oscillation Identification. In Proceedings of the 2019 International Conference on Clean Electrical Power (ICCEP), Otranto, Italy, 2–4 July 2019; IEEE: Piscataway, NJ, USA, 2019. [Google Scholar] [CrossRef]
- Chow, J.H.; Chakrabortty, A.; Vanfretti, L.; Arcak, M. Estimation of Radial Power System Transfer Path Dynamic Parameters Using Synchronized Phasor Data. IEEE Trans. Power Syst. 2008, 23, 564–571. [Google Scholar] [CrossRef] [Green Version]
- Phadke, A.G.; Bi, T. Phasor measurement units, WAMS, and their applications in protection and control of power systems. J. Mod. Power Syst. Clean Energy 2018, 6, 619–629. [Google Scholar] [CrossRef] [Green Version]
- Chow, J.H. Power System Coherency and Model Reduction; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Fan, L.; Miao, Z.; Wehbe, Y. Application of Dynamic State and Parameter Estimation Techniques on Real-World Data. IEEE Trans. Smart Grid 2013, 4, 1133–1141. [Google Scholar] [CrossRef] [Green Version]
- Wall, P.; Terzija, V. Simultaneous Estimation of the Time of Disturbance and Inertia in Power Systems. IEEE Trans. Power Deliv. 2014, 29, 2018–2031. [Google Scholar] [CrossRef]
- Ashton, P.M.; Taylor, G.A.; Carter, A.M.; Bradley, M.E.; Hung, W. Application of phasor measurement units to estimate power system inertial frequency response. In Proceedings of the 2013 IEEE Power & Energy Society General Meeting, Vancouver, BC, Canada, 21–25 July 2013; pp. 1–5. [Google Scholar] [CrossRef]
- Huang, Z.; Du, P.; Kosterev, D.; Yang, B. Application of extended Kalman filter techniques for dynamic model parameter calibration. In Proceedings of the 2009 IEEE Power & Energy Society General Meeting, Calgary, AB, Canada, 26–30 July 2009; pp. 1–8. [Google Scholar] [CrossRef]
- Cao, X.; Stephen, B.; Abdulhadi, I.F.; Booth, C.D.; Burt, G.M. Switching Markov Gaussian Models for Dynamic Power System Inertia Estimation. IEEE Trans. Power Syst. 2016, 31, 3394–3403. [Google Scholar] [CrossRef] [Green Version]
- Petra, N.; Petra, C.G.; Zhang, Z.; Constantinescu, E.M.; Anitescu, M. A Bayesian Approach for Parameter Estimation With Uncertainty for Dynamic Power Systems. IEEE Trans. Power Syst. 2017, 32, 2735–2743. [Google Scholar] [CrossRef]
- Zhao, J.; Tang, Y.; Terzija, V. Robust Online Estimation of Power System Center of Inertia Frequency. IEEE Trans. Power Syst. 2019, 34, 821–825. [Google Scholar] [CrossRef]
- Zografos, D.; Ghandhari, M.; Eriksson, R. Power system inertia estimation: Utilization of frequency and voltage response after a disturbance. Electr. Power Syst. Res. 2018, 161, 52–60. [Google Scholar] [CrossRef]
- Lugnani, L.; Dotta, D.; Lackner, C.; Chow, J. ARMAX-based method for inertial constant estimation of generation units using synchrophasors. Electr. Power Syst. Res. 2020, 180, 106097. [Google Scholar] [CrossRef]
- Garcia-Valle, R.; Yang, G.Y.; Martin, K.E.; Nielsen, A.H.; Ostergaard, J. DTU PMU laboratory development–Testing and validation. In Proceedings of the 2010 IEEE PES Innovative Smart Grid Technologies Conference Europe (ISGT Europe), Gothenburg, Sweden, 11–13 October 2010; IEEE: Piscataway, NJ, USA, 2010. [Google Scholar] [CrossRef] [Green Version]
- Angioni, A.; Lipari, G.; Pau, M.; Ponci, F.; Monti, A. A Low Cost PMU to Monitor Distribution Grids. In Proceedings of the 2017 IEEE International Workshop on Applied Measurements for Power Systems (AMPS), Liverpool, UK, 20–22 September 2017; IEEE: Piscataway, NJ, USA, 2017. [Google Scholar] [CrossRef] [Green Version]
- Laverty, D.M.; Vanfretti, L.; Khatib, I.A.; Applegreen, V.K.; Best, R.J.; Morrow, D.J. The OpenPMU Project: Challenges and perspectives. In Proceedings of the 2013 IEEE Power & Energy Society General Meeting, Vancouver, BC, Canada, 21–25 July 2013; IEEE: Piscataway, NJ, USA, 2013. [Google Scholar] [CrossRef]
- Smart Grid Investment Grant Program. Factors Affecting PMU Installation Costs; Technical Report; U.S. Department of Energy–Electricity Delivery & Energy Reliability: Washington, DC, USA, 2014.
- Schofield, D.; Gonzalez-Longatt, F.; Bogdanov, D. Design and Implementation of a Low-Cost Phasor Measurement Unit: A Comprehensive Review. In Proceedings of the 2018 Seventh Balkan Conference on Lighting (BalkanLight), Varna, Bulgaria, 20–22 September 2018; IEEE: Piscataway, NJ, USA, 2018. [Google Scholar] [CrossRef] [Green Version]
- Wehbe, Y.; Fan, L.; Miao, Z. Least squares based estimation of synchronous generator states and parameters with phasor measurement units. In Proceedings of the 2012 North American Power Symposium (NAPS), Champaign, IL, USA, 9–11 September 2012; pp. 1–6. [Google Scholar] [CrossRef]
- Kundur, P.; Balu, N.J.; Lauby, M.G. Power System Stability and Control; McGraw-Hill: New York, NY, USA, 1994; Volume 7. [Google Scholar]
- Gonzalez-Longatt, F.; Rueda, J.L. Power Factory Applications for Power System Analysis; Springer Publishing Company: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
- Fan, L.; Wehbe, Y. Extended Kalman filtering based real-time dynamic state and parameter estimation using PMU data. Electr. Power Syst. Res. 2013, 103, 168–177. [Google Scholar] [CrossRef]
- Kalsi, K.; Sun, Y.; Huang, Z.; Du, P.; Diao, R.; Anderson, K.K.; Li, Y.; Lee, B. Calibrating multi-machine power system parameters with the extended Kalman filter. In Proceedings of the IEEE Power and Energy Society General Meeting, Detroit, MI, USA, 24–28 July 2011; pp. 1–8. [Google Scholar] [CrossRef]
- Machowski, J.; Bialek, J.W.; Bumby, J.R. Power System Dynamics, Stability and Control; Wiley: Hoboken, NJ, USA, 2012. [Google Scholar]
- Byrd, R.H.; Schnabel, R.B.; Shultz, G.A. Approximate solution of the trust region problem by minimization over two-dimensional subspaces. Math. Program. 1988, 40, 247–263. [Google Scholar] [CrossRef]
- Regulski, P.; Wall, P.; Rusidovic, Z.; Terzija, V. Estimation of load model parameters from PMU measurements. In Proceedings of the IEEE PES Innovative Smart Grid Technologies, Istanbul, Turkey, 12–15 October 2014; IEEE: Piscataway, NJ, USA, 2014; pp. 1–6. [Google Scholar]
- Alinejad, B.; Akbari, M.; Kazemi, H. PMU-based distribution network load modelling using Harmony Search Algorithm. In Proceedings of the 2012 Proceedings of 17th Conference on Electrical Power Distribution, Tehran, Iran, 2–3 May 2012; IEEE: Piscataway, NJ, USA, 2012; pp. 1–6. [Google Scholar]
- Polykarpou, E.; Asprou, M.; Kyriakides, E. Dynamic load modelling using real time estimated states. In Proceedings of the 2017 IEEE Manchester PowerTech, Manchester, UK, 18–22 June 2017; IEEE: Piscataway, NJ, USA, 2017; pp. 1–6. [Google Scholar]
- Zografos, D.; Ghandhari, M. Power system inertia estimation by approaching load power change after a disturbance. In Proceedings of the 2017 IEEE Power & Energy Society General Meeting, Chicago, IL, USA, 16–20 July 2017; IEEE: Piscataway, NJ, USA; pp. 1–5. [Google Scholar]
- Bliznyuk, D.; Berdin, A.; Romanov, I. PMU data analysis for load characteristics estimation. In Proceedings of the 2016 57th International Scientific Conference on Power and Electrical Engineering of Riga Technical University (RTUCON), Riga, Latvia, 13–14 October 2016; IEEE: Piscataway, NJ, USA, 2016; pp. 1–5. [Google Scholar]
- Vignesh, V.; Chakrabarti, S.; Srivastava, S.C. Power system load modelling under large and small disturbances using phasor measurement units data. IET Gener. Transm. Distrib. 2015, 9, 1316–1323. [Google Scholar]
- Kuivaniemi, M.; Laasonen, M.; Elkington, K.; Danell, A.; Modig, N.; Bruseth, A.; Jansson, E.A.; Orum, E. Estimation of System Inertia in the Nordic Power System Using Measured Frequency Disturbances. In Proceedings of the Lund Symposium on across Borders–Integrating Systems and Markets–CIGRE, Lund, Zweden, 27–28 May 2015; pp. 27–28. [Google Scholar]
- Gao, W.; Ning, J. Wavelet-Based Disturbance Analysis for Power System Wide-Area Monitoring. IEEE Trans. Smart Grid 2011, 2, 121–130. [Google Scholar] [CrossRef]
- Cui, M.; Wang, J.; Tan, J.; Florita, A.R.; Zhang, Y. A Novel Event Detection Method Using PMU Data With High Precision. IEEE Trans. Power Syst. 2019, 34, 454–466. [Google Scholar] [CrossRef]
- Shams, N.; Wall, P.; Terzija, V. Active Power Imbalance Detection, Size and Location Estimation Using Limited PMU Measurements. IEEE Trans. Power Syst. 2019, 34, 1362–1372. [Google Scholar] [CrossRef]
- Proceedings of the IEEE Guide for Synchronous Generator Modeling Practices and Applications in Power System Stability Analyses; IEEE Std 1110-2002 (Revision of IEEE Std 1110-1991); IEEE: Piscataway, NJ, USA, 2003; 72p. [CrossRef]
- Arif, A.; Wang, Z.; Wang, J.; Mather, B.; Bashualdo, H.; Zhao, D. Load Modeling—A Review. IEEE Trans. Smart Grid 2018, 9, 5986–5999. [Google Scholar] [CrossRef]
- Tellez, A.P. Modelling Aggregate Loads in Power Systems. Master’s Thesis, KTH Royal Institute of Technology, Stockholm, Sweden, 2017. [Google Scholar]
- Wall, P.; Gonzalez-Longatt, F.; Terzija, V. Estimation of generator inertia available during a disturbance. In Proceedings of the IEEE Power and Energy Society General Meeting, San Diego, CA, USA, 22–26 July 2012; pp. 1–8. [Google Scholar] [CrossRef]


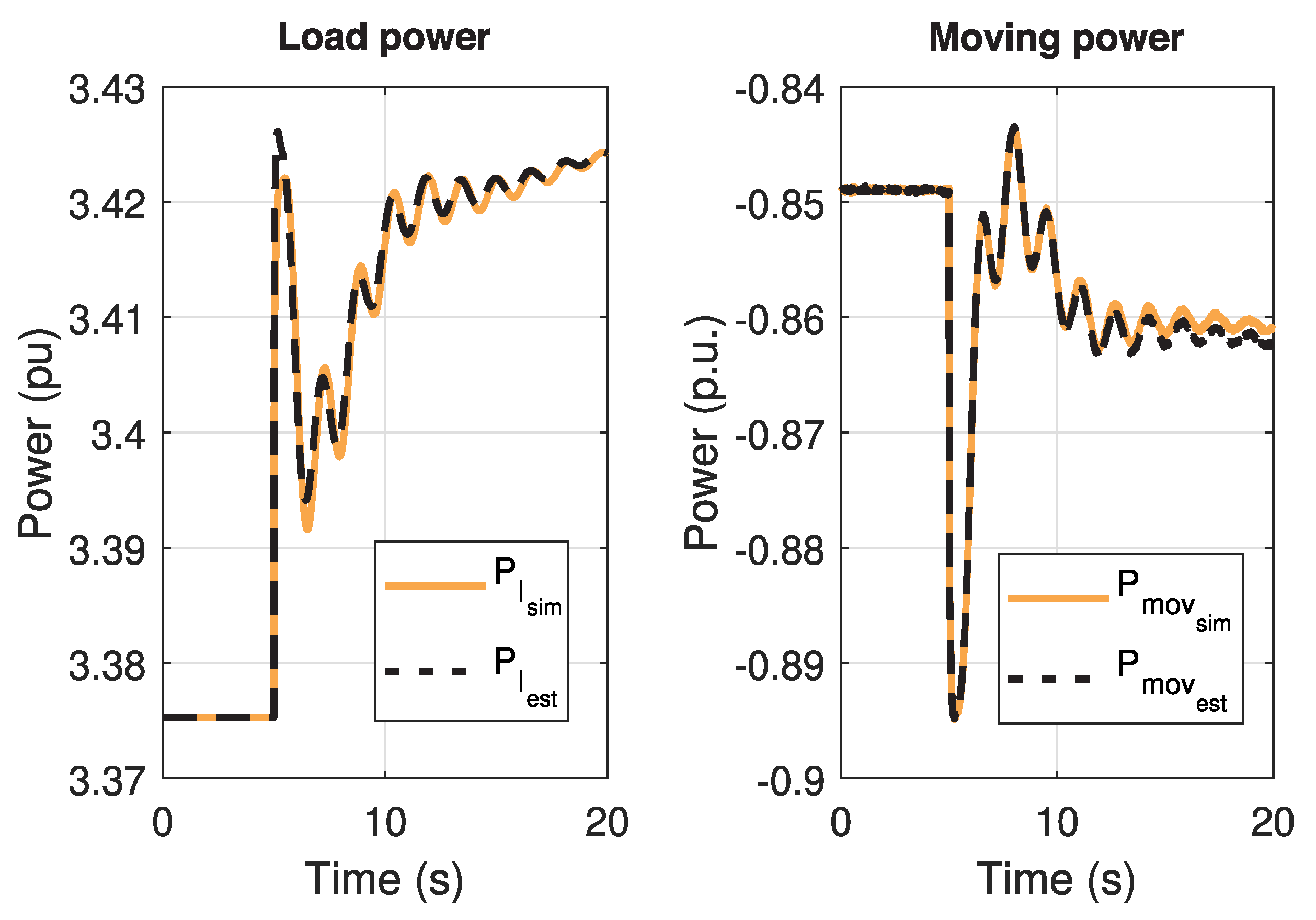
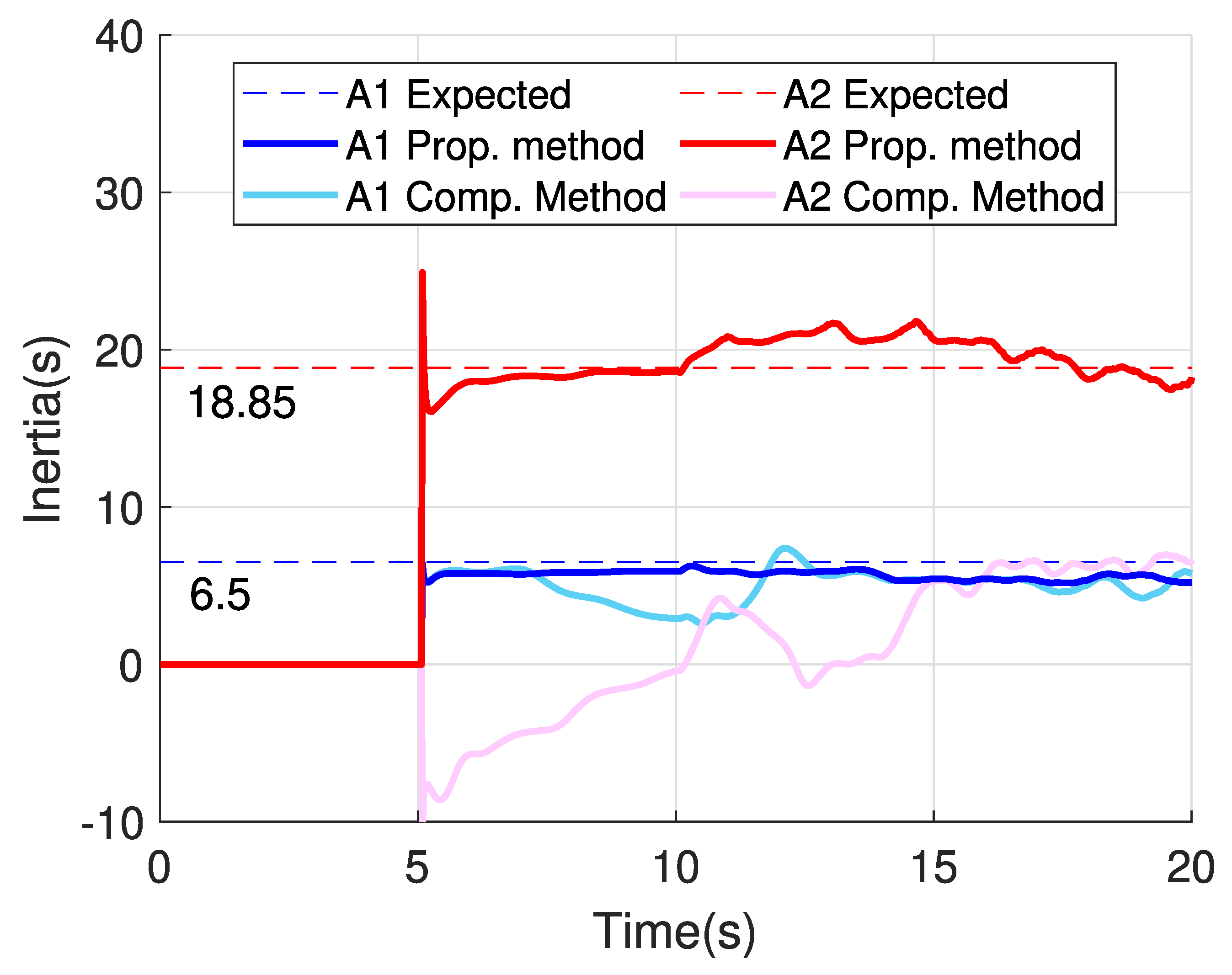
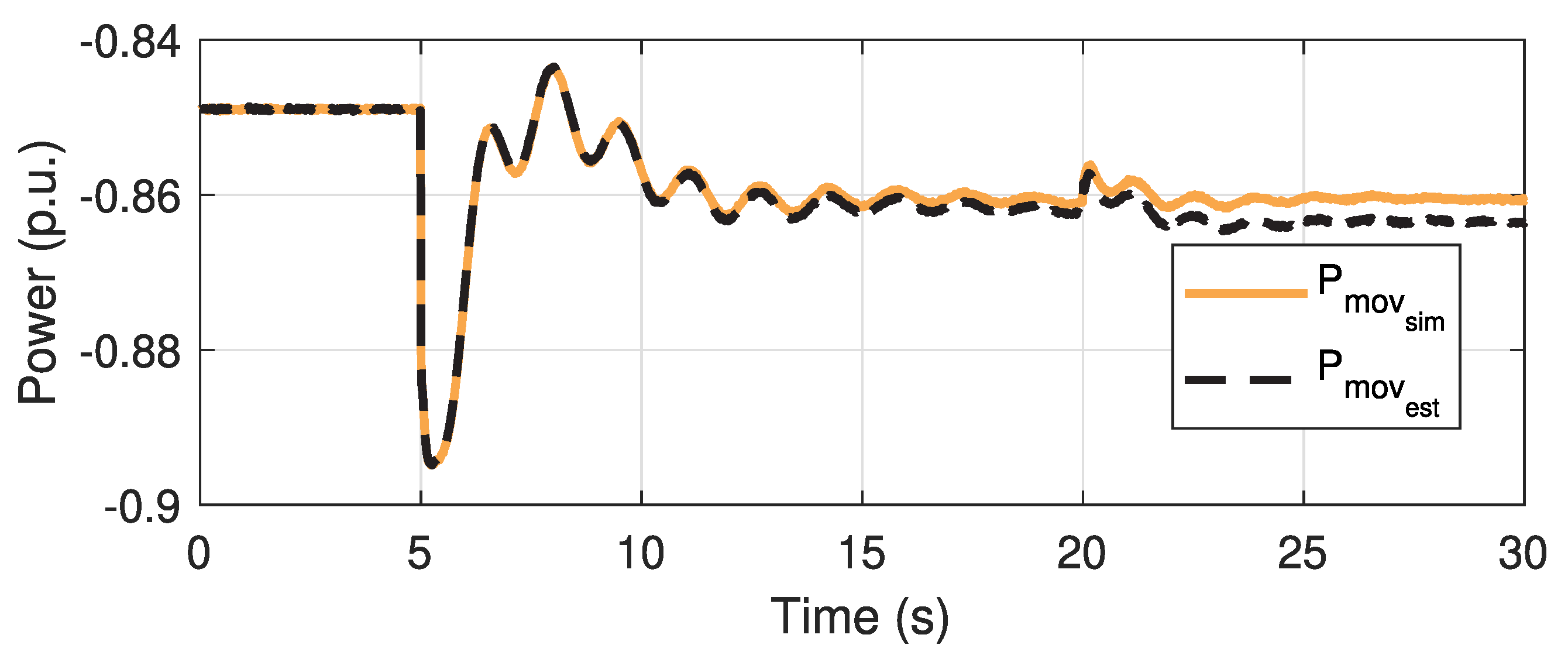
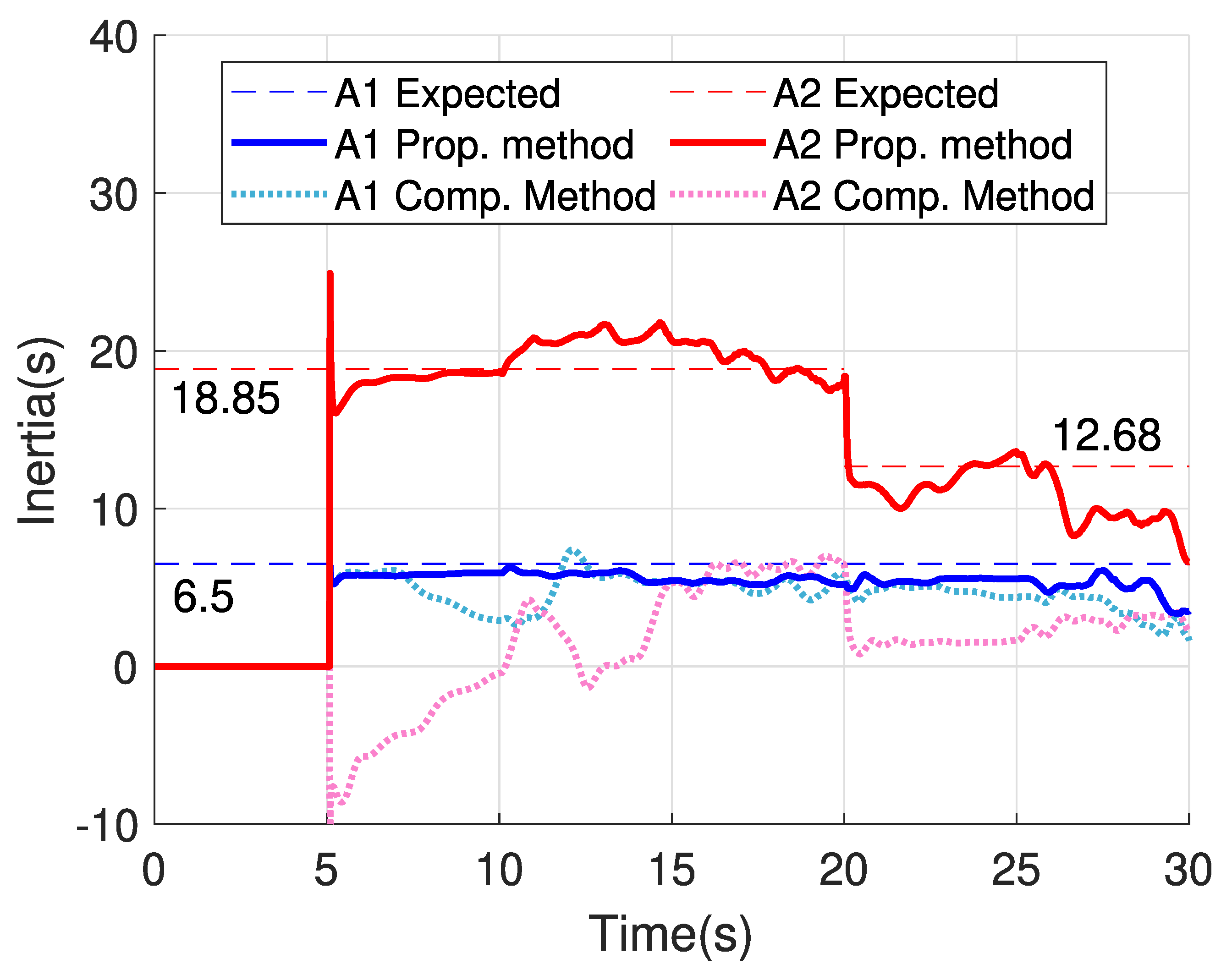
| Case 1 | Estimated | Simulated | Simulated |
| Case 2 | Simulated | Estimated | Simulated |
| Case 3 | Simulated | Simulated | Estimated |
| Case 4 | Simulated | Estimated | Estimated |
| Case 5 | Estimated | Estimated | Estimated |
| Case 6 | Estimated | Estimated | Estimated |
| Study 1: Loss of Generation | Study 2: Load Shedding | |||||
|---|---|---|---|---|---|---|
| Case 1 | −0.54 | −1.07 | −3.38 | −4.81 | −3.20 | −8.11 |
| Case 2 | 4.86 | 2.05 | 10.79 | 2.54 | 4.88 | −8.29 |
| Case 3 | 4.59 | 4.64 | 8.51 | 7.31 | 4.16 | −2.15 |
| Case 4 | 9.41 | 1.85 | 11.14 | 8.79 | 4.86 | −7.07 |
| Case 5 | 12.44 | −4.47 | 9.35 | 6.06 | −1.97 | −2.90 |
| Case 6 | 6.87 | 1.59 | 0.95 | 3.71 | −3.30 | −1.22 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rossetto Moraes, G.; Ilea, V.; Berizzi, A.; Pisani, C.; Giannuzzi, G.; Zaottini, R. A Perturbation-Based Methodology to Estimate the Equivalent Inertia of an Area Monitored by PMUs. Energies 2021, 14, 8477. https://doi.org/10.3390/en14248477
Rossetto Moraes G, Ilea V, Berizzi A, Pisani C, Giannuzzi G, Zaottini R. A Perturbation-Based Methodology to Estimate the Equivalent Inertia of an Area Monitored by PMUs. Energies. 2021; 14(24):8477. https://doi.org/10.3390/en14248477
Chicago/Turabian StyleRossetto Moraes, Guido, Valentin Ilea, Alberto Berizzi, Cosimo Pisani, Giorgio Giannuzzi, and Roberto Zaottini. 2021. "A Perturbation-Based Methodology to Estimate the Equivalent Inertia of an Area Monitored by PMUs" Energies 14, no. 24: 8477. https://doi.org/10.3390/en14248477
APA StyleRossetto Moraes, G., Ilea, V., Berizzi, A., Pisani, C., Giannuzzi, G., & Zaottini, R. (2021). A Perturbation-Based Methodology to Estimate the Equivalent Inertia of an Area Monitored by PMUs. Energies, 14(24), 8477. https://doi.org/10.3390/en14248477









