Estimation of the Compressive Strength of Corrugated Cardboard Boxes with Various Openings
Abstract
:1. Introduction
2. Materials and Methods
2.1. Ultimate Compressive Strength of a Single Panel
2.2. Buckling—McKee’s Formula
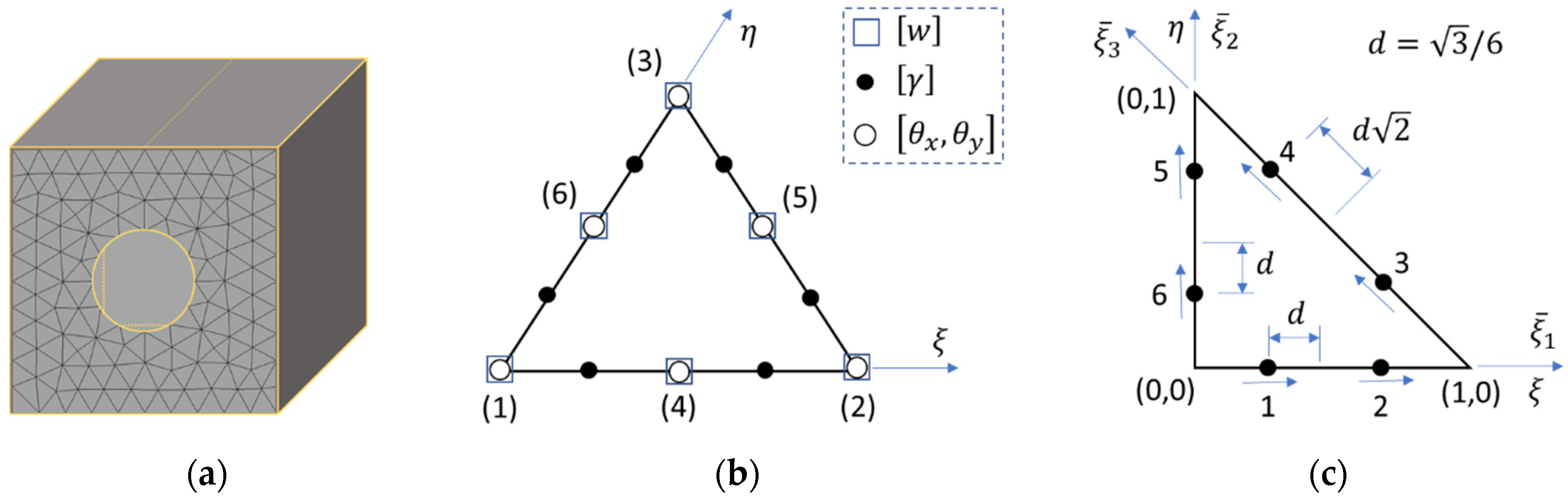
2.3. Buckling—Finite Element Method
- discretize the panel with finite elements (FE), see Figure 2a,
- compute the stiffness matrix of each element,
- assemble the global stiffness matrix of a whole panel,
- compute nodal forces representing initial loading configuration , i.e., for loading multiplier (one-parameter loading assumed ),
- take boundary conditions into account,
- solve equation to obtain nodal displacements in pre-buckling state:
- extract element displacements (from displacements of the system ) and compute in each element the generalized stresses .
- generate initial geometrical stress matrices for each element and the whole panel ,
- formulate initial buckling problem:
- solve the eigenproblem to determine the pairs , where is a number of degrees of freedom, is th eigenvalue, is th eigenvector (post-buckling deformation mode).
2.4. Box Compression Strength—McKee’s Formula
2.5. Box Compression Strength—General Case
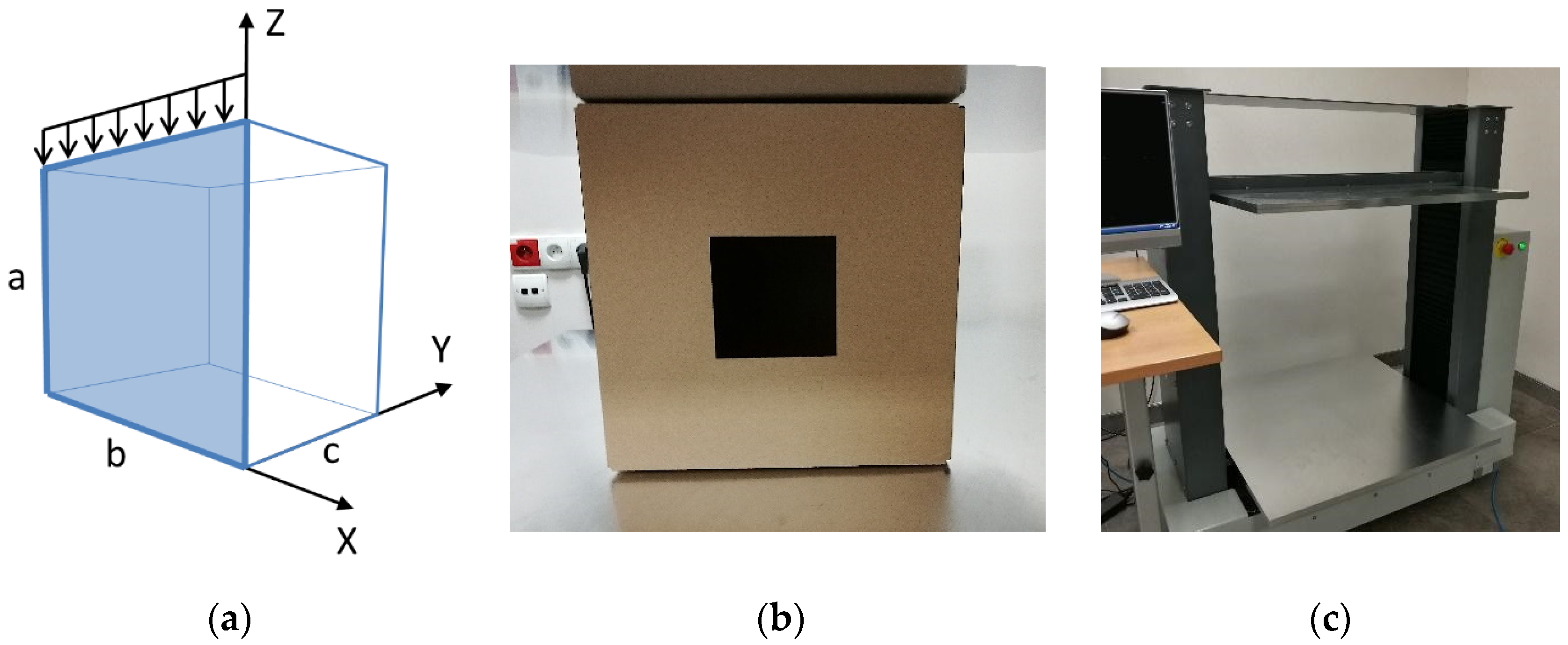
2.6. Practical Considerations
- (a)
- Equation (4)—for orthotropic rectangular plates without holes (analytical),
- (b)
- Equation (8)—for orthotropic rectangular plates with various openings (numerical – FEM).
3. Results
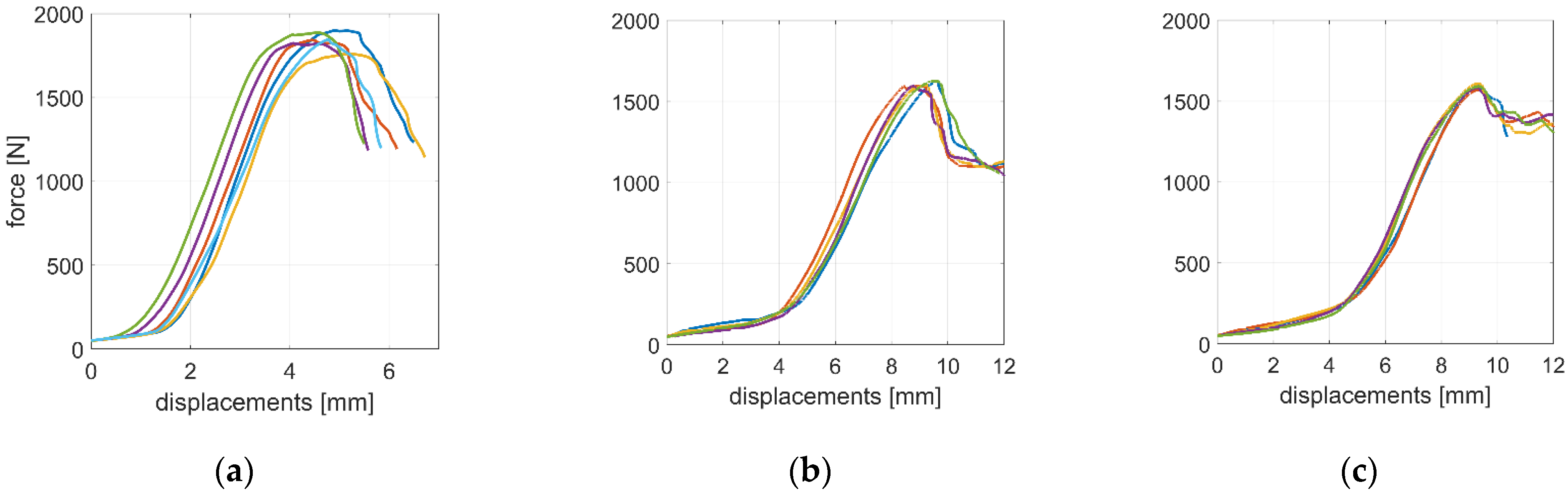
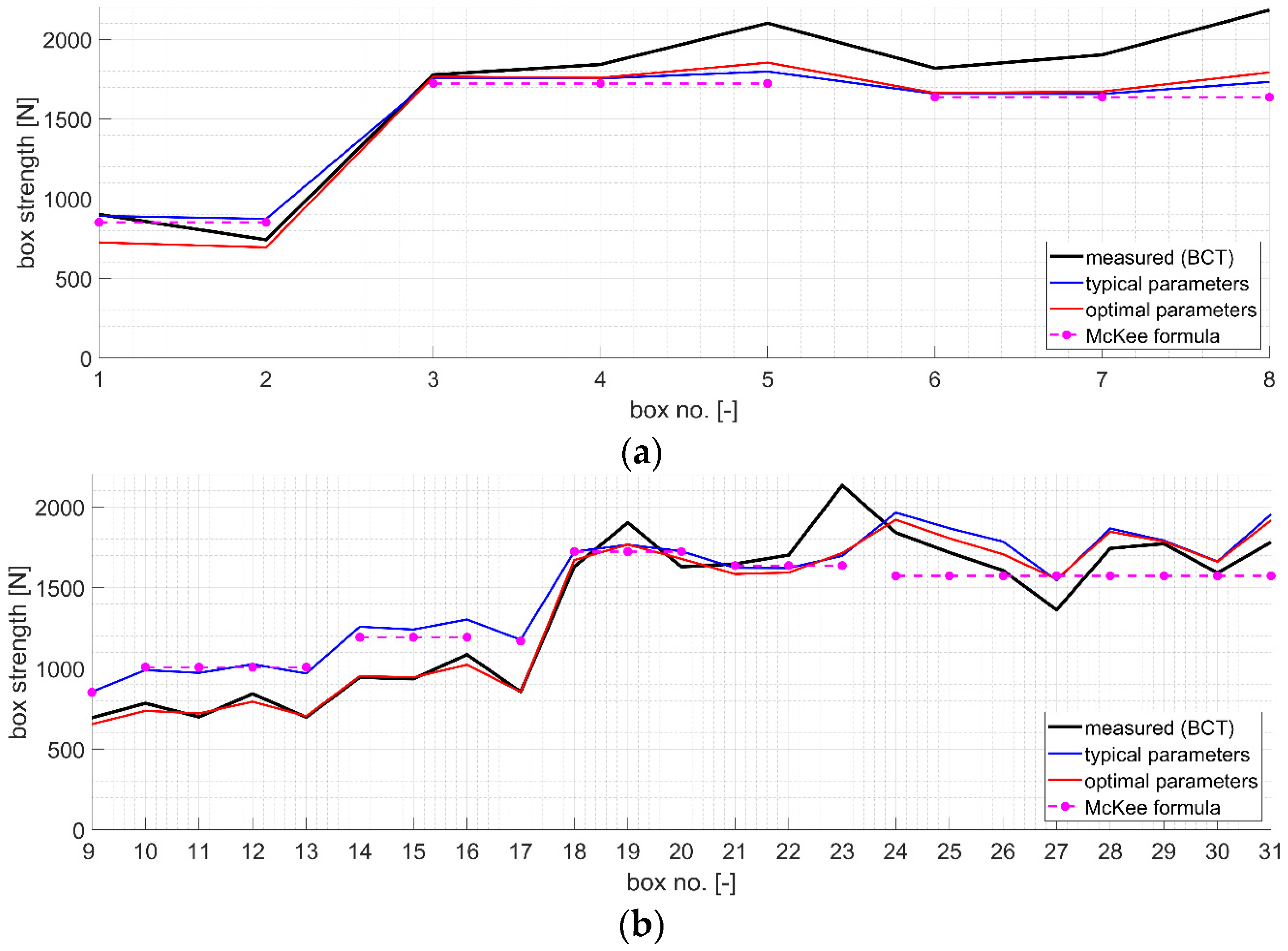
3.1. Box Compression Strength—Experiment vs. Estimation
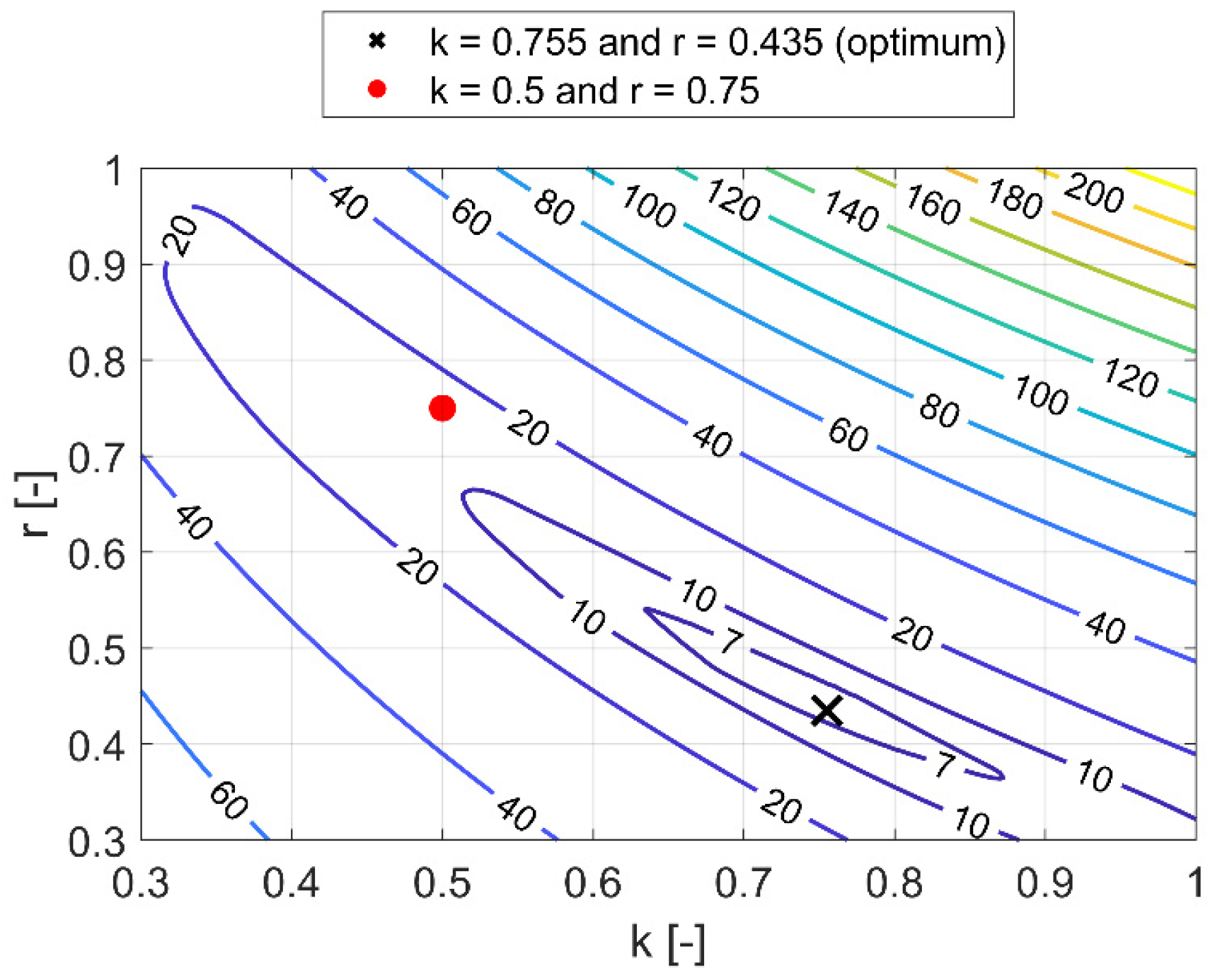
3.2. Reduction of the Estimation Error—Optimal Parameters
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
Appendix A
Derivation of the Substitute Transverse Shear Strain Matrix
References
- Sohrabpour, V.; Hellström, D. Models and software for corrugated board and box design. In Proceedings of the 18th International Conference on Engineering Design (ICED 11), Copenhagen, Denmark, 15–18 October 2011. [Google Scholar]
- Kellicutt, K.; Landt, E. Development of design data for corrugated fiberboard shipping containers. Tappi J. 1952, 35, 398–402. [Google Scholar]
- Maltenfort, G. Compression strength of corrugated containers. Fibre Contain. 1956, 41, 106–121. [Google Scholar]
- McKee, R.C.; Gander, J.W.; Wachuta, J.R. Compression strength formula for corrugated boxes. Paperboard Packag. 1963, 48, 149–159. [Google Scholar]
- Allerby, I.M.; Laing, G.N.; Cardwell, R.D. Compressive strength—From components to corrugated containers. Appita Conf. Notes 1985, 1–11. [Google Scholar]
- Schrampfer, K.E.; Whitsitt, W.J.; Baum, G.A. Combined Board Edge Crush (ECT) Technology; Institute of Paper Chemistry: Appleton, WI, USA, 1987. [Google Scholar]
- Batelka, J.J.; Smith, C.N. Package Compression Model; Institute of Paper Science and Technology: Atlanta, GA, USA, 1993. [Google Scholar]
- Urbanik, T.J.; Frank, B. Box compression analysis of world-wide data spanning 46 years. Wood Fiber Sci. 2006, 38, 399–416. [Google Scholar]
- Garbowski, T.; Gajewski, T.; Grabski, J.K. The role of buckling in the estimation of compressive strength of corrugated cardboard boxes. Materials 2020, 13, 4578. [Google Scholar] [CrossRef]
- Avilés, F.; Carlsson, L.A.; May-Pat, A. A shear-corrected formulation of the sandwich twist specimen. Exp. Mech. 2012, 52, 17–23. [Google Scholar] [CrossRef]
- Garbowski, T.; Gajewski, T.; Grabski, J.K. Role of transverse shear modulus in the performance of corrugated materials. Materials 2020, 13, 3791. [Google Scholar] [CrossRef]
- Garbowski, T.; Gajewski, T.; Grabski, J.K. Torsional and transversal stiffness of orthotropic sandwich panels. Materials 2020, 13, 5016. [Google Scholar] [CrossRef]
- Urbanik, T.J.; Saliklis, E.P. Finite element corroboration of buckling phenomena observed in corrugated boxes. Wood Fiber Sci. 2003, 35, 322–333. [Google Scholar]
- Nordstrand, T. Basic Testing and Strength Design of Corrugated Board and Containers. Ph.D. Thesis, Lund University, Lund, Sweden, 2003. [Google Scholar]
- Nordstrand, T.; Carlsson, L. Evaluation of transverse shear stiffness of structural core sandwich plates. Comp. Struct. 1997, 37, 145–153. [Google Scholar] [CrossRef]
- Pozorski, Z.; Wojciechowski, S. The influence of symmetrical boundary conditions on the structural behaviour of sandwich panels subjected to torsion. Symmetry 2020, 12, 2093. [Google Scholar] [CrossRef]
- Yan, J.; Wang, G.; Li, Q.; Zhang, L.; Yan, J.D.; Chen, C.; Fang, Z. A comparative study on damage mechanism of sandwich structures with different core materials under lightning strikes. Energies 2017, 10, 1594. [Google Scholar] [CrossRef] [Green Version]
- Zhang, X.; Jin, X.; Xie, G.; Yan, H. Thermo-fluidic comparison between sandwich panels with tetrahedral lattice cores fabricated by casting and metal sheet folding. Energies 2017, 10, 906. [Google Scholar] [CrossRef] [Green Version]
- Duan, Y.; Zhang, H.; Sfarra, S.; Avdelidis, N.P.; Loutas, T.H.; Sotiriadis, G.; Kostopoulos, V.; Fernandes, H.; Petrescu, F.I.; Ibarra-Castanedo, C.; et al. On the use of infrared thermography and acousto-ultrasonics NDT techniques for ceramic-coated sandwich structures. Energies 2019, 12, 2537. [Google Scholar] [CrossRef] [Green Version]
- Garbowski, T.; Jarmuszczak, M. Homogenization of corrugated paperboard. Part 1. Analytical homogenization. Pol. Pap. Rev. 2014, 70, 345–349. (In Polish) [Google Scholar]
- Garbowski, T.; Jarmuszczak, M. Homogenization of corrugated paperboard. Part 2. Numerical homogenization. Pol. Pap. Rev. 2014, 70, 390–394. (In Polish) [Google Scholar]
- Hohe, J. A direct homogenization approach for determination of the stiffness matrix for microheterogeneous plates with application to sandwich panels. Compos. Part B 2003, 34, 615–626. [Google Scholar] [CrossRef]
- Buannic, N.; Cartraud, P.; Quesnel, T. Homogenization of corrugated core sandwich panels. Comp. Struct. 2003, 59, 299–312. [Google Scholar] [CrossRef] [Green Version]
- Biancolini, M.E. Evaluation of equivalent stiffness properties of corrugated board. Comp. Struct. 2005, 69, 322–328. [Google Scholar] [CrossRef]
- Abbès, B.; Guo, Y.Q. Analytic homogenization for torsion of orthotropic sandwich plates: Application. Comp. Struct. 2010, 92, 699–706. [Google Scholar] [CrossRef]
- Marek, A.; Garbowski, T. Homogenization of sandwich panels. Comput. Assist. Methods Eng. Sci. 2015, 22, 39–50. [Google Scholar]
- Garbowski, T.; Marek, A. Homogenization of corrugated boards through inverse analysis. In Proceedings of the 1st International Conference on Engineering and Applied Sciences Optimization, Kos Island, Greece, 4–6 June 2014; pp. 1751–1766. [Google Scholar]
- Singh, J.; Olsen, E.; Singh, S.P.; Manley, J.; Wallace, F. The effect of ventilation and hand holes on loss of compression strength in corrugated boxes. J. Appl. Packag. Res. 2008, 2, 227–238. [Google Scholar]
- Fadiji, T.; Coetzee, C.J.; Opara, U.L. Compression strength of ventilated corrugated paperboard packages: Numerical modelling, experimental validation and effects of vent geometric design. Biosyst. Eng. 2016, 151, 231–247. [Google Scholar] [CrossRef]
- Fadiji, T.; Ambaw, A.; Coetzee, C.J.; Berry, T.M.; Opara, U.L. Application of the finite element analysis to predict the mechanical strength of ventilated corrugated paperboard packaging for handling fresh produce. Biosyst. Eng. 2018, 174, 260–281. [Google Scholar] [CrossRef]
- Heimerl, G.J. Determination of plate compressive strengths. In National Advisory Committee for Aeronautics; Technical Note Number 1480; National Advisory Committee for Aeronautics (NACA): Washington, DC, USA, 1947. [Google Scholar]
- FEMat Systems. Available online: http://www.fematsystems.pl/en/systems/bct/ (accessed on 26 March 2020).
- Ventsel, E.; Krauthammer, T. Thin Plates and Shells. Theory, Analysis, and Applications; Marcel Dekker, Inc.: New York, NY, USA; Basel, Switzerland, 2001. [Google Scholar]
- Norstrand, T. On buckling loads for edge-loaded orthotropic plates including transverse shear. Comp. Struct. 2004, 65, 1–6. [Google Scholar] [CrossRef]
- Wang, C.M.; Wang, C.Y.; Reddy, J.N. Exact Solutions for Buckling of Structural Members; CRC Press: Boca Ratton, FL, USA, 2005. [Google Scholar]
- Zienkiewicz, O.C.; Taylor, R.L.; Papadopoulus, P.; Onate, E. Plate bending elements with discrete constraints: New Triangular Elements. Comput. Struct. 1990, 35, 505–522. [Google Scholar] [CrossRef]
- Zienkiewicz, O.C.; Taylor, R.L. The Finite Element Method for Solid and Structural Mechanics, 6th ed.; Butterworth-Heinemann: Oxford, UK, 2005. [Google Scholar]
- Onate, E.; Castro, J. Derivation of plate elements based on assumed shear strain fields. In Recent Advances on Computational Structural Mechanics; Ladeveze, P., Zienkiewicz, O.C., Eds.; Elsevier Pub: Amsterdam, The Netherlands, 1991. [Google Scholar]
- Buchanan, J.S.; Draper, J.; Teague, G.W. Combined board characteristics that determine box performance. Paperboard Packag. 1964, 49, 74–85. [Google Scholar]
- Shick, P.E.; Chari, N.C.S. Top-to-bottom compression for double wall corrugated boxes. Tappi J. 1965, 48, 423–430. [Google Scholar]
- Wolf, M. New equation helps pin down box specifications. Package Eng. 1972, 17, 66–67. [Google Scholar]
- FEMat Systems. Available online: http://www.fematsystems.pl/en/systems/bse/ (accessed on 26 September 2020).
- Garbowski, T.; Borysiewicz, A. The stability of corrugated board packages. Pol. Pap. Rev. 2014, 70, 452–458. (In Polish) [Google Scholar]
- Hughes, T.R.J. The finite element method. In Linear Static and Dynamic Analysis; Prentice Hall: Upper Saddle River, NJ, USA, 1987. [Google Scholar]
- Onate, E. Structural Analysis with the Finite Element Method. Vol. 1: Basis and Solids; Springer-CIMNE: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Onate, E.; Zienkiewicz, O.C.; Suarez, B.; Taylor, R.L. A general methodology for deriving shear constrained Reissner-Mindlin plate elements. Int. J. Numer. Meth. Eng. 1992, 33, 345–367. [Google Scholar] [CrossRef]
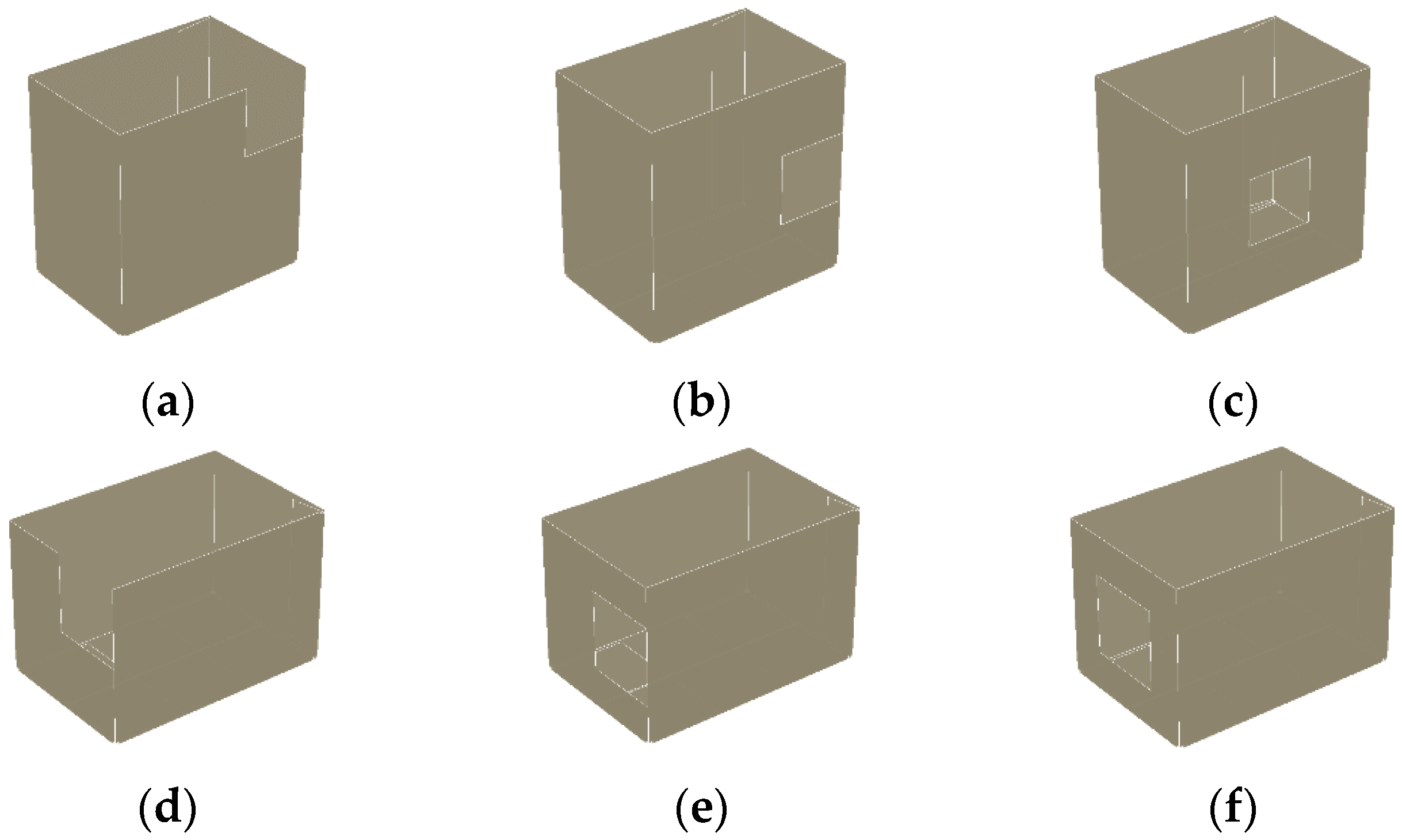
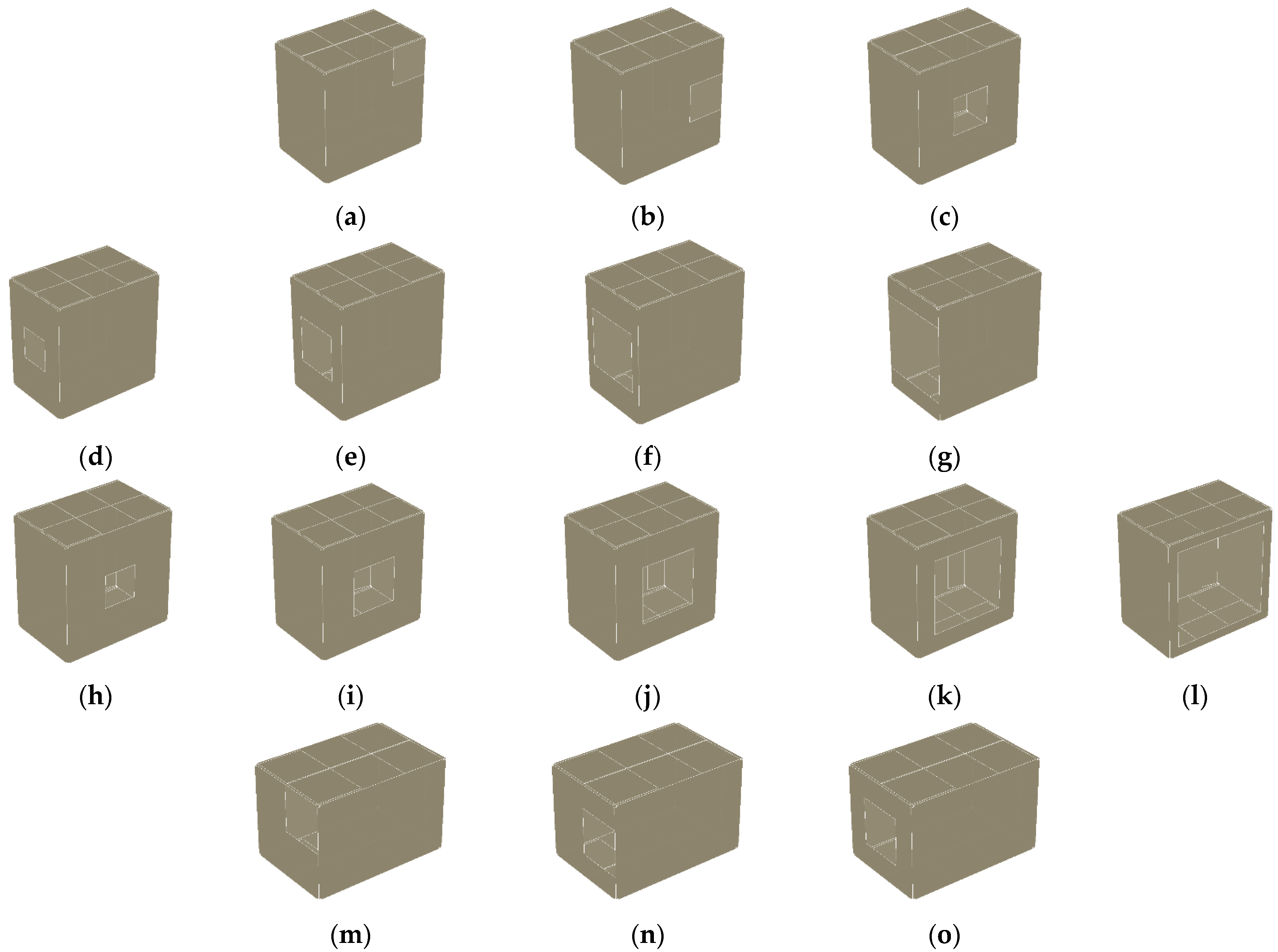
| No. | Opening Type | Box Height [mm] | Cardboard Quality | Box Strength [N] |
|---|---|---|---|---|
| 1 | O3B1S | 200 | 3E350-3 | 901.4 |
| 2 | O2B1S | 200 | 3E350-3 | 742.3 |
| 3 | O2B1S | 200 | 3B400A1-1 | 1777.9 |
| 4 | O1B1S | 200 | 3B400A1-1 | 1842.7 |
| 5 | O3B1S | 200 | 3B400A1-1 | 2101.6 |
| 6 | O1L1S | 300 | 3B400A1-3 | 1819.4 |
| 7 | O2L1S | 300 | 3B400A1-3 | 1903.0 |
| 8 | O3L1S | 300 | 3B400A1-3 | 2184.3 |
| No. | Opening Type | Box Height [mm] | Cardboard Quality | Box Strength [N] |
|---|---|---|---|---|
| 9 | O1B1S | 200 | 3E350-3 | 694.3 |
| 10 | O3B1S | 200 | 3E350-5 | 783.8 |
| 11 | O1L1S | 300 | 3E350-5 | 699.3 |
| 12 | O3L1S | 300 | 3E350-5 | 842.9 |
| 13 | O2B1S | 200 | 3E350-5 | 697.5 |
| 14 | O3B1S | 200 | 3E380A2-1 | 944.6 |
| 15 | O2L1S | 300 | 3E380A2-1 | 935.7 |
| 16 | O3L1S | 300 | 3E380A2-1 | 1085.3 |
| 17 | O1L1S | 300 | 3E380A2-2 | 854.8 |
| 18 | O1B1S | 200 | 3B400A1-1 | 1630.0 |
| 19 | O3B1S | 200 | 3B400A1-1 | 1902.9 |
| 20 | O2B1S | 200 | 3B400A1-1 | 1629.0 |
| 21 | O1L1S | 300 | 3B400A1-3 | 1647.5 |
| 22 | O2L1S | 300 | 3B400A1-3 | 1701.9 |
| 23 | O3L1S | 300 | 3B400A1-3 | 2133.6 |
| 24 | O3B1S1a | 300 | 3B480-1 | 1841.1 |
| 25 | O3B1S1b | 300 | 3B480-1 | 1717.9 |
| 26 | O3B1S1c | 300 | 3B480-1 | 1606.6 |
| 27 | O3L1S1e | 300 | 3B480-1 | 1362.9 |
| 28 | O3L1S1b | 300 | 3B480-1 | 1743.5 |
| 29 | O3L1S1c | 300 | 3B480-1 | 1772.4 |
| 30 | O3L1S1d | 300 | 3B480-1 | 1591.3 |
| 31 | O3L1S1a | 300 | 3B480-1 | 1782.3 |
| No. | Cardboard Quality | Thickness [mm] | ECT | |||||
|---|---|---|---|---|---|---|---|---|
| 1 | 3B400A1-1 | 2.82 | 5.79 | 3484.8 | 1789.8 | 2262.1 | 7.73 | 12.78 |
| 2 | 3B400A1-3 | 2.80 | 5.50 | 3443.5 | 1565.5 | 2115.5 | 6.09 | 11.30 |
| 3 | 3B480-1 | 2.82 | 5.29 | 3491.2 | 1820.1 | 2359.9 | 5.66 | 12.40 |
| 4 | 3E350-3 | 1.49 | 3.96 | 958.9 | 431.5 | 870.2 | 2.49 | 2.79 |
| 5 | 3E350-5 | 1.49 | 4.68 | 878.6 | 376.9 | 904.0 | 2.86 | 2.97 |
| 6 | 3E380A2-1 | 1.59 | 5.41 | 1272.1 | 505.4 | 1084.5 | 3.83 | 3.86 |
| 8 | 3E380A2-2 | 1.52 | 5.31 | 1042.9 | 445.1 | 963.2 | 3.39 | 3.70 |
| Case | k [—] | r [—] | Mean Error [%] |
|---|---|---|---|
| McKee formula | 0.4215 | 0.746 | 15.5 |
| method proposed (typical parameters) | 0.500 | 0.750 | 14.9 |
| method proposed (optimal parameters) | 0.755 | 0.435 | 6.5 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Garbowski, T.; Gajewski, T.; Grabski, J.K. Estimation of the Compressive Strength of Corrugated Cardboard Boxes with Various Openings. Energies 2021, 14, 155. https://doi.org/10.3390/en14010155
Garbowski T, Gajewski T, Grabski JK. Estimation of the Compressive Strength of Corrugated Cardboard Boxes with Various Openings. Energies. 2021; 14(1):155. https://doi.org/10.3390/en14010155
Chicago/Turabian StyleGarbowski, Tomasz, Tomasz Gajewski, and Jakub Krzysztof Grabski. 2021. "Estimation of the Compressive Strength of Corrugated Cardboard Boxes with Various Openings" Energies 14, no. 1: 155. https://doi.org/10.3390/en14010155
APA StyleGarbowski, T., Gajewski, T., & Grabski, J. K. (2021). Estimation of the Compressive Strength of Corrugated Cardboard Boxes with Various Openings. Energies, 14(1), 155. https://doi.org/10.3390/en14010155







