Phase Synchronization Stability of Non-Homogeneous Low-Voltage Distribution Networks with Large-Scale Distributed Generations
Abstract
:1. Introduction
- (1)
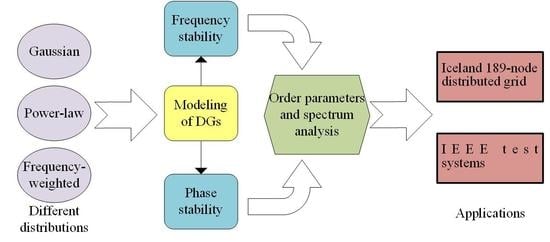
- We derive non-homogeneous models for simulating different weighted distribution methods in a large-scale islanded distribution network.
- (2)
- We analytically study the effects of weighted distribution methods on the phase synchronization stability by means of explicit synchronization conditions. We illustrate our insightful results and the utility of our approach through the critical coupling strength formula.
- (3)
- Different from other studies focusing on the weighted distribution methods which only associate with the inherent properties of the network, this paper adopts several realistic distribution styles as weighted distribution methods to research the synchronization stability in the large-scale distribution network.
2. Correlation Theory
2.1. Graph Theory
2.2. Synchronization Conditions
2.3. Critical Coupling Strength
3. Weighted Distribution
3.1. Star Topology
3.2. The General Formula for Weighted Distribution
3.3. Weighted Distribution Model
3.3.1. Gaussian Distribution
3.3.2. Power-Law Distribution
3.3.3. Frequency-Weighted Network
3.4. Critical Coupling Strength Formula
4. Simulation
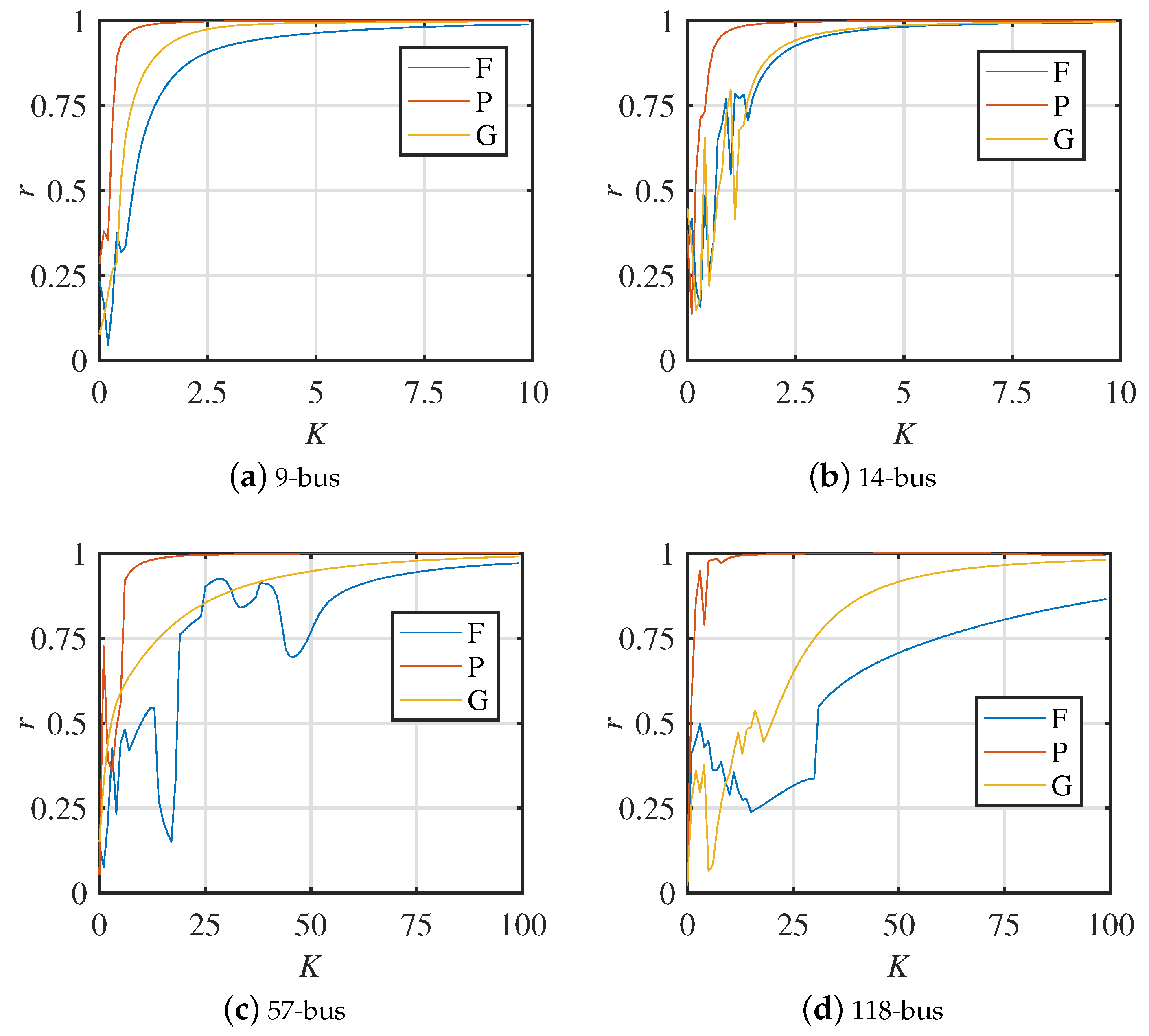
4.1. Case 1
4.1.1. Weighted Undirected Network
4.1.2. Weighted Directed Network
4.2. Case 2
4.2.1. Weighted Undirected Network
4.2.2. Weighted Directed Network
4.3. Case 3
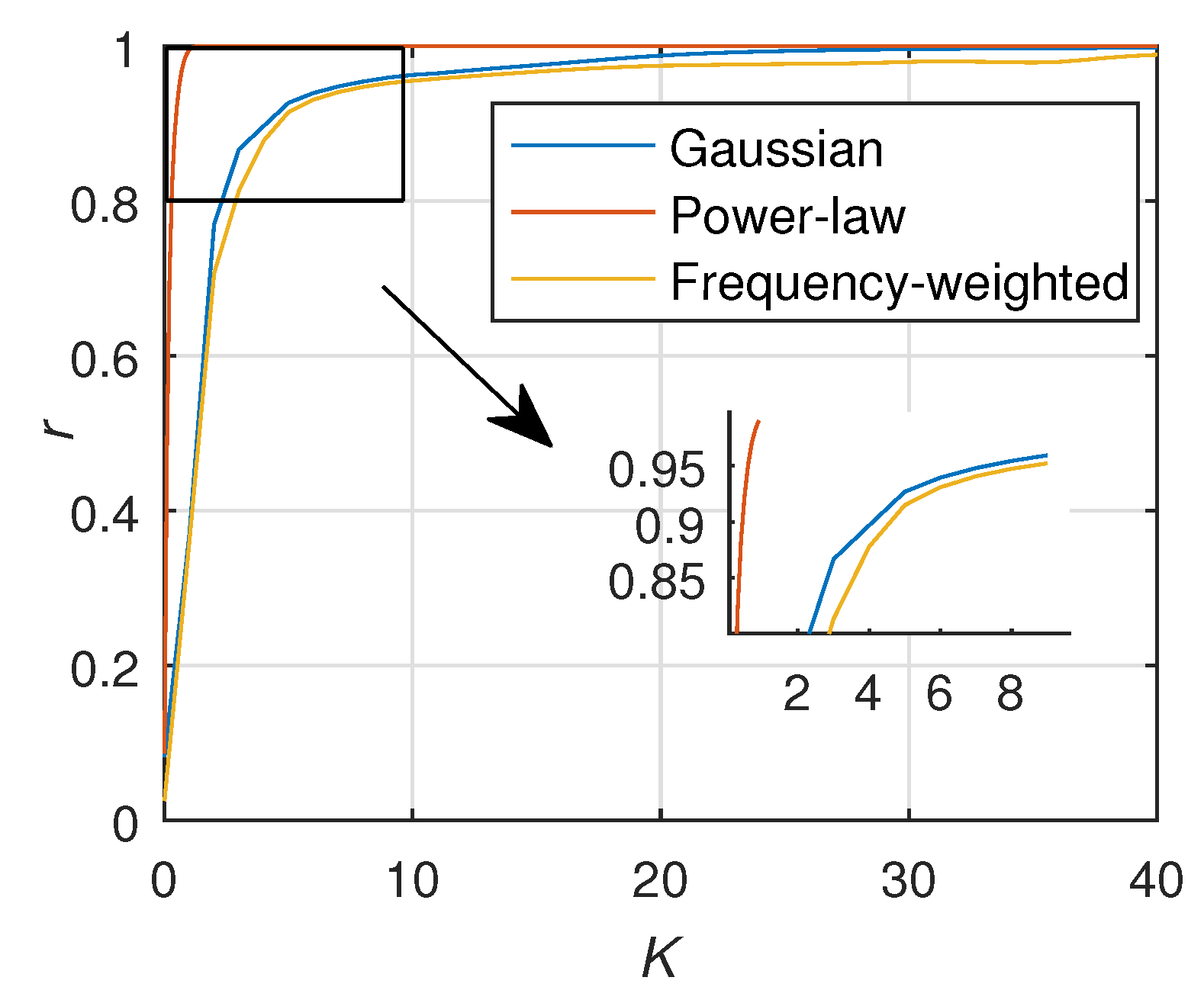
4.4. Stability Analysis
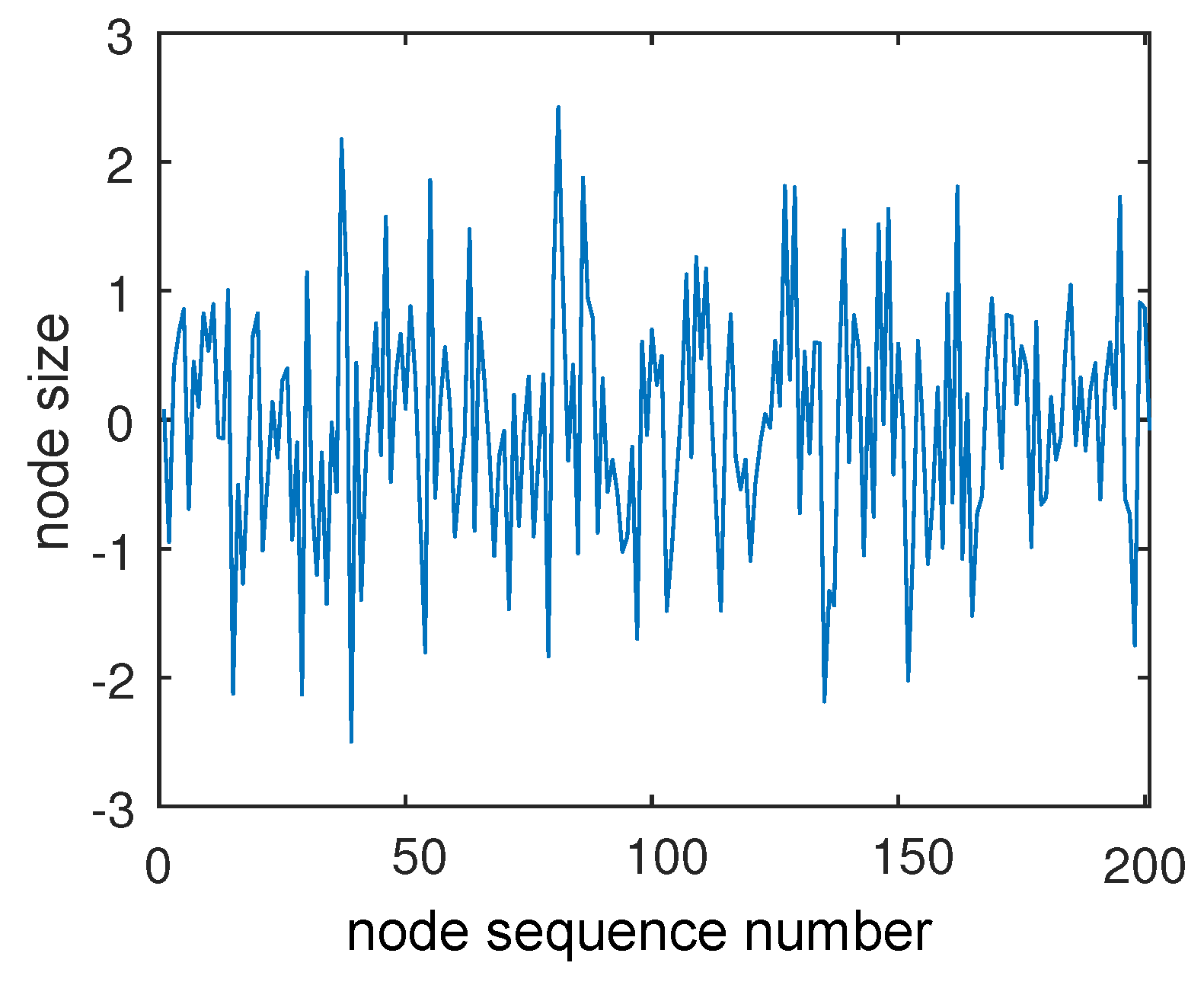
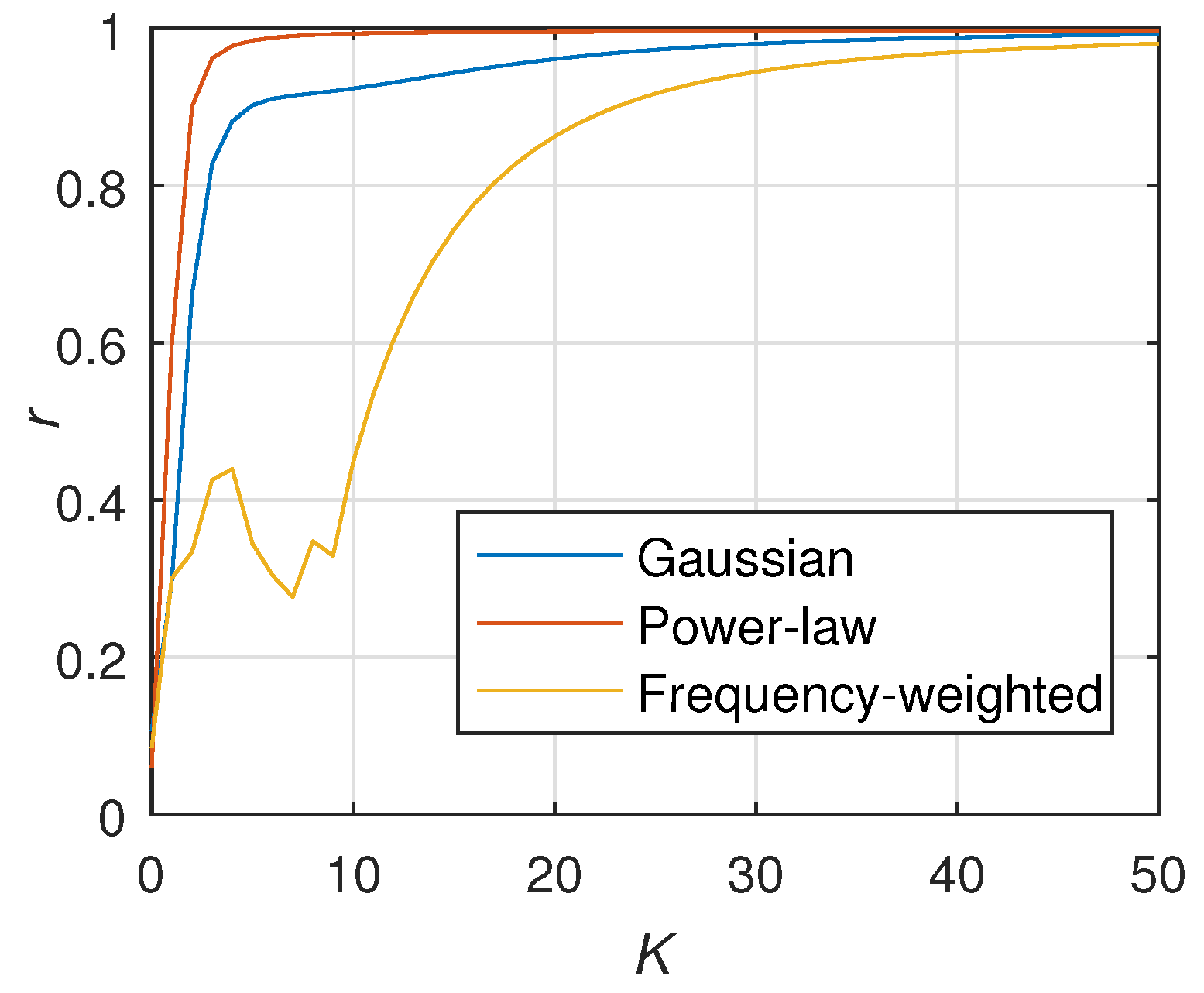
4.5. Further Analysis of Weighted Network in the Actually Distributed Network
4.5.1. Dynamic IEEE Test Systems
4.5.2. Iceland 189-Nodes Distribution Grid
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Uriarte, F.M.; Smith, C.; VanBroekhoven, S.; Hebner, R.E. Microgrid ramp rates and the inertial stability margin. IEEE Trans. Power Syst. 2015, 30, 3209–3216. [Google Scholar] [CrossRef]
- Mendis, N.; Muttaqi, K.M.; Perera, S. Management of battery-supercapacitor hybrid energy storage and synchronous condenser for isolated operation of PMSG based variable-speed wind turbine generating systems. IEEE Trans. Smart Grid 2014, 5, 944–953. [Google Scholar] [CrossRef]
- Lai, J.; Lu, X.; Yu, X.; Monti, A. Cluster-Oriented Distributed Cooperative Control for Multiple AC Microgrids. IEEE Trans. Ind. Inform. 2019, 15, 5906–5918. [Google Scholar] [CrossRef]
- Lai, J.; Lu, X.; Yu, X.; Monti, A. Stochastic Distributed Secondary Control for AC Microgrids via Event-Triggered Communication. IEEE Trans. Smart Grid 2020. to be published. [Google Scholar] [CrossRef]
- Lai, J.; Lu, X.; Yu, X.; Monti, A.; Zhou, H. Distributed Voltage Regulation for Cyber-Physical Microgrids with Coupling Delays and Slow Switching Topologies. IEEE Trans. Syst. Man Cybernet. Syst. 2020, 50, 100–110. [Google Scholar] [CrossRef]
- Lai, J.; Lu, X. Nonlinear Mean-Square Power Sharing Control for AC Microgrids under Distributed Event Detection. IEEE Trans. Ind. Inform. 2020. to be published. [Google Scholar] [CrossRef]
- Refaat, S.S.; Abu-Rub, H.; Sanfilippo, A.P.; Mohamed, A. Impact of grid-tied large-scale photovoltaic system on dynamic voltage stability of electric power grids. IET Renew. Power Gener. 2018, 12, 157–164. [Google Scholar] [CrossRef]
- Byshkin, M.; Stivala, A.; Mira, A.; Robins, G.; Lomi, A. Fast Maximum Likelihood estimation via Equilibrium Expectation for Large Network Data. Nature 2013, 31, 1. [Google Scholar] [CrossRef]
- Brockmann, D.; Helbing, D. The Hidden Geometry of Complex, Network-Driven Contagion Phenomena. Science 2013, 342, 1337–1342. [Google Scholar] [CrossRef] [Green Version]
- Centola, D. The Spread of Behavior in an Online Social Network Experiment. Science 2010, 329, 1194–1197. [Google Scholar] [CrossRef]
- Li, X. The role of degree-weighted couplings in the synchronous onset of Kuramoto oscillator networks. Physica A Stat. Mech. Its Appl. 2008, 387, 6624–6630. [Google Scholar] [CrossRef]
- Wu, C.W. Synchronizability of networks of chaotic systems coupled via a graph with a prescribed degree sequence. Phys. Lett. A 2005, 346, 281–287. [Google Scholar] [CrossRef]
- Sorrentino, F.; di Bernardo, M.; Garofalo, F. Synchronizability In addition, Synchronization Dynamics Of Weighed And Unweighed Scale Free Networks With Degree Mixing. Int. J. Bifurc. Chaos 2007, 17, 2419–2434. [Google Scholar] [CrossRef] [Green Version]
- Leyva, I.; Sendinanadal, I.; Almendral, J.A. Explosive synchronization in weighted complex networks. Phys. Rev. E Stat. Nonlinear Soft Matter Phys. 2013, 88, 042808. [Google Scholar] [CrossRef] [Green Version]
- Zhu, L.; Tian, L.; Zhu, C.; Shi, D. Effects of coupling-frequency correlations on synchronization of complete graphs. Phys. Lett. A 2013, 377, 2749–2753. [Google Scholar] [CrossRef]
- Chavez, M.; Hwang, D.U.; Amann, A. Synchronization is enhanced in weighted complex networks. Phys. Rev. Lett. 2005, 94, 218701. [Google Scholar] [CrossRef]
- Jalili, M.; Mazloomian, A. Weighted coupling for geographical networks: Application to reducing consensus time in sensor networks. Phys. Lett. A 2010, 374, 3920–3925. [Google Scholar] [CrossRef]
- Qin, B.Z.; Lu, X.B. Adaptive approach to global synchronization of directed networks with fast switching topologies. Phys. Lett. A 2010, 374, 3942–3950. [Google Scholar] [CrossRef]
- Dong, Z.; Tian, M.; Lu, Y.; Lai, J.; Tang, R.; Li, X. Impact of core-periphery structure on cascading failures in interdependent scale-free networks. Phys. Lett. A 2019, 383, 607–616. [Google Scholar] [CrossRef]
- Tian, M.; Dong, Z.; Cui, M.; Wang, J.; Wang, X.; Zhao, L. Energy-supported cascading failure model on interdependent networks considering control nodes. Physica A Stat. Mech. Its Appl. 2019, 522, 195–204. [Google Scholar] [CrossRef]
- Dorfler, F.; Chertkov, M.; Bullo, F. Synchronization in complex oscillator networks and smart grids. Proc. Natl. Acad. Sci. USA 2013, 110, 2005–2010. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bidram, A.; Davoudi, A.; Lewis, F.L.; Nasirian, V. Cooperative Synchronization in Distributed Microgrid Control; Springer: Cham, Switzerland, 2017. [Google Scholar]
- Fazlyab, M.; Dorfler, F.; Preciado, V.M. Optimal Design for Synchronization of Kuramoto Oscillators in Tree Networks. Physics 2005, 92, 181–189. [Google Scholar]
- Gu, Y.Q.; Shao, C.; Fu, X.C. Complete synchronization and stability of star-shaped complex networks. Chaos Solitons Fractals 2006, 28, 480–488. [Google Scholar] [CrossRef]
- Dekker, A.H.; Taylor, R. Synchronisation Properties of Trees in the Kuramoto Model. Siam J. Appl. Dynam. Syst. 2012, 12, 97–100. [Google Scholar]
- Zhai, M. Transmission characteristics of low-voltage distribution networks in china under the smart grids environment. IEEE Trans. Power Deliv. 2011, 26, 173–180. [Google Scholar] [CrossRef]
- Dorfler, F.; Bullo, F. Synchronization in complex networks of phase oscillators: A survey. Automatica 2014, 50, 1539–1564. [Google Scholar] [CrossRef] [Green Version]
- Simpson-Porco, J.; Dorfler, F.; Bullo, F. Synchronization and power sharing for droop-controlled inverters in islanded microgrids. Automatica 2013, 49, 2603–2611. [Google Scholar] [CrossRef] [Green Version]
- Pagani, G.; Aiello, M. Towards decentralization: A topological investigation of the medium and low voltage grids. IEEE Trans. Smart Grid 2011, 2, 538–547. [Google Scholar] [CrossRef]
- Wu, Y.; Lee, C.; Liu, L. Study of reconfiguration for the distribution system with distributed generators. IEEE Trans. Power Deliv. 2010, 25, 1678–1685. [Google Scholar] [CrossRef]
- Li, C.; Chen, G. Network connection strengths: Another power-law? arXiv 2003, arXiv:cond-mat/0311333. [Google Scholar]
- Barrat, A.; Barth, M.; Pastorsatorras, R. The architecture of complex weighted networks. Proc. Natl. Acad. Sci. USA 2004, 101, 3747–3752. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Dynamic IEEE Test Systems. 2019. Available online: https://www.kios.ucy.ac.cy/testsystems/index.php/dynamic-ieee-test-systems (accessed on 13 October 2019).
- Power Systems Test Case Archive. 2013. Available online: http://www.maths.ed.ac.uk/optenergy/NetworkData/iceland/ (accessed on 13 October 2019).
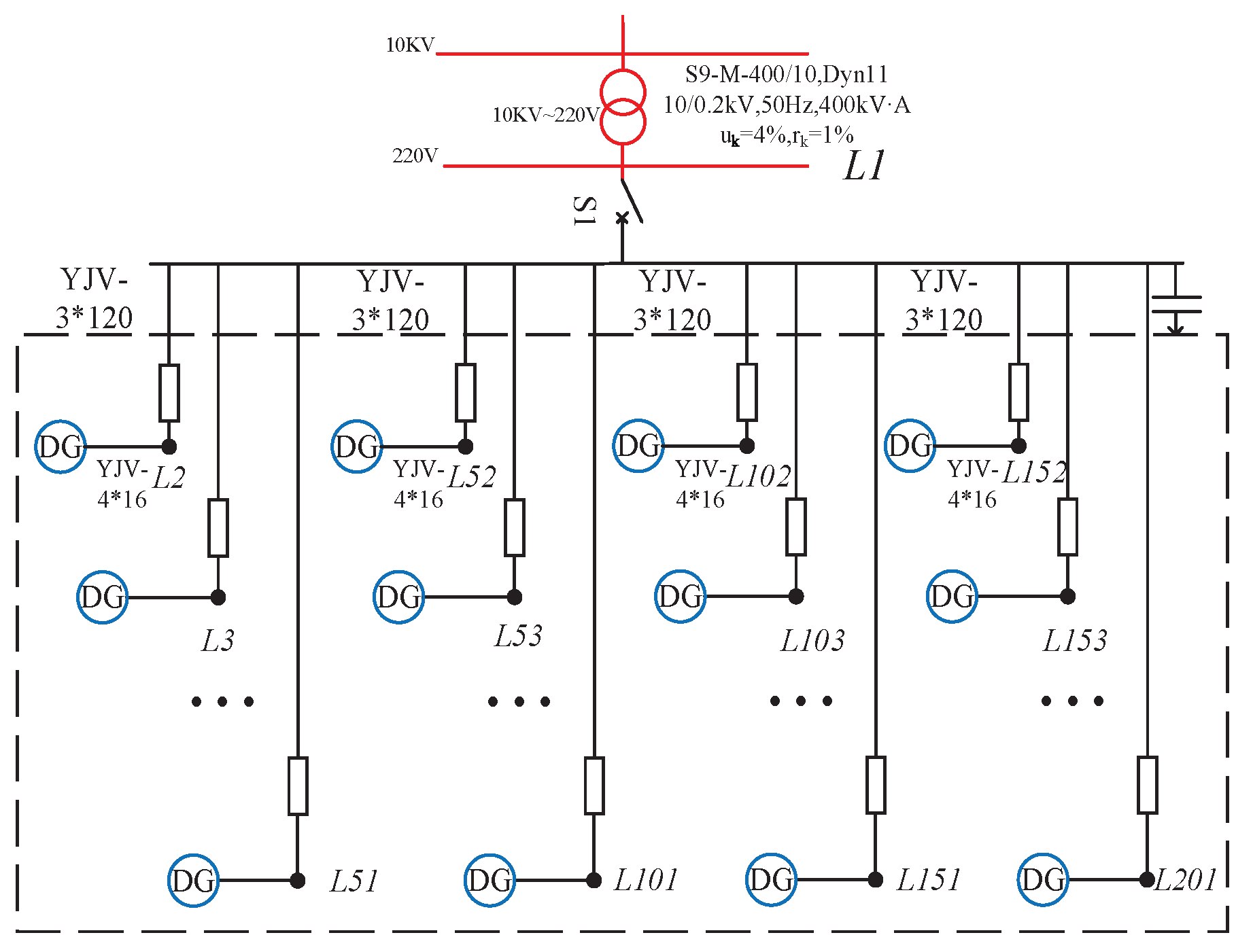
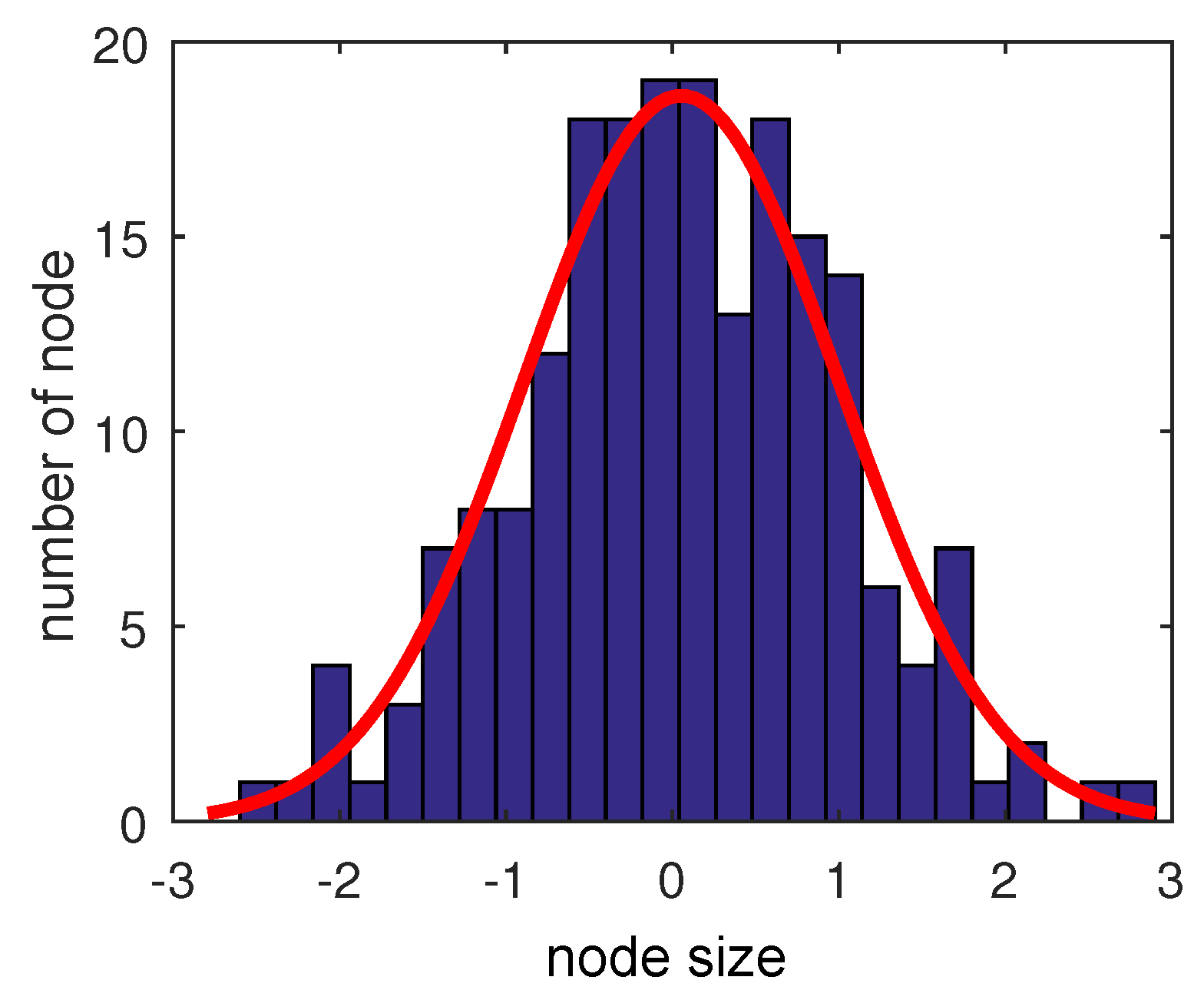

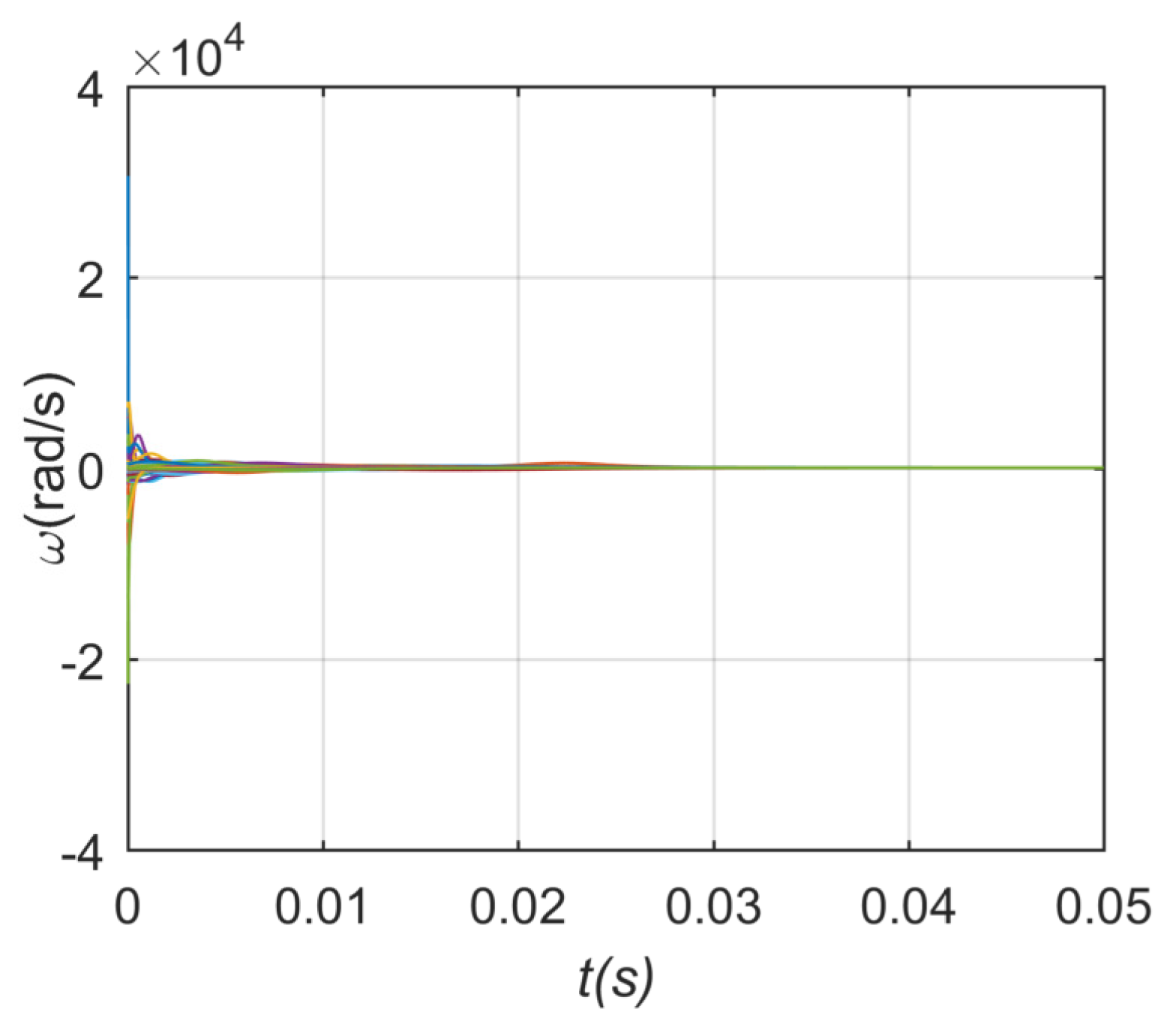

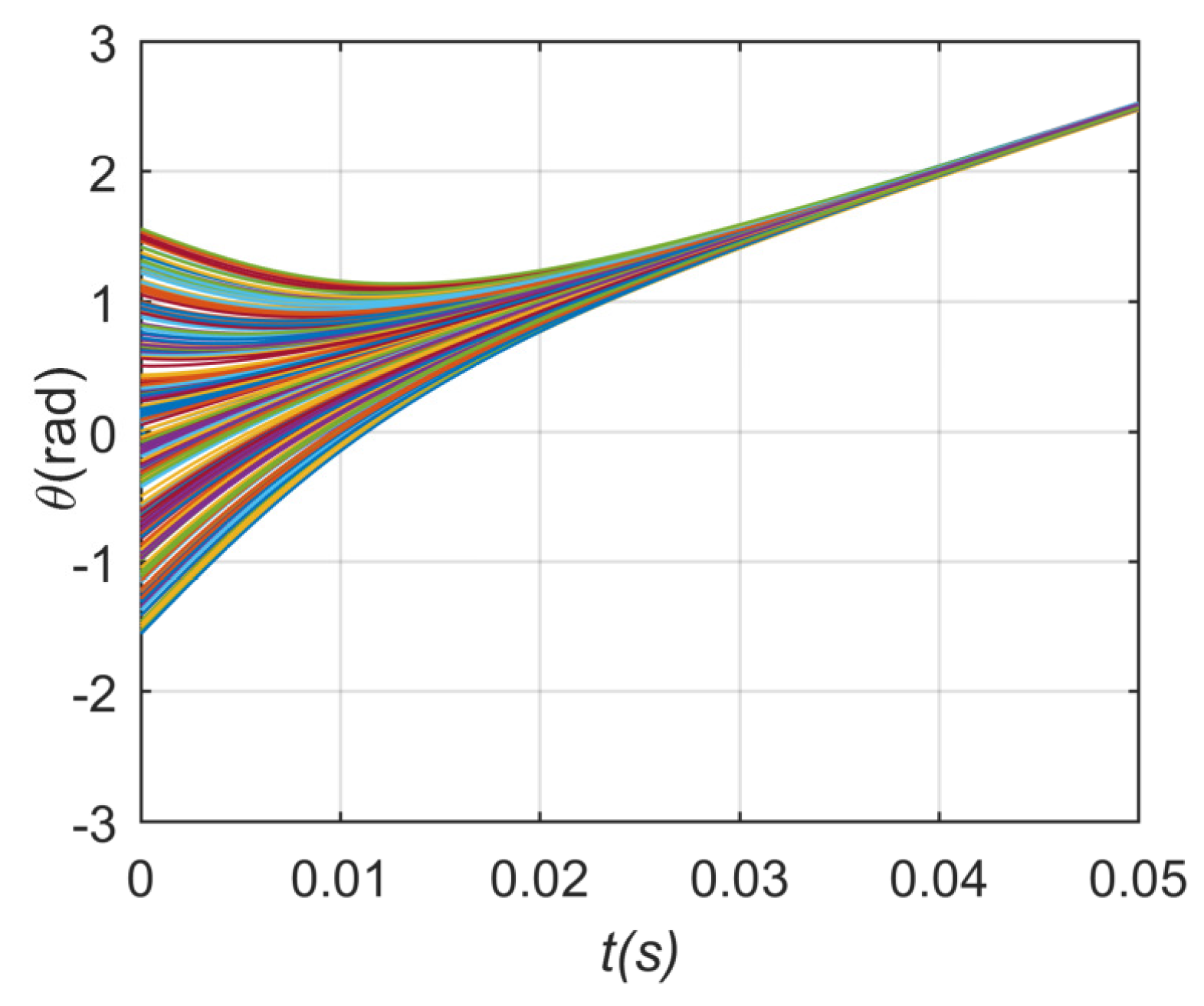
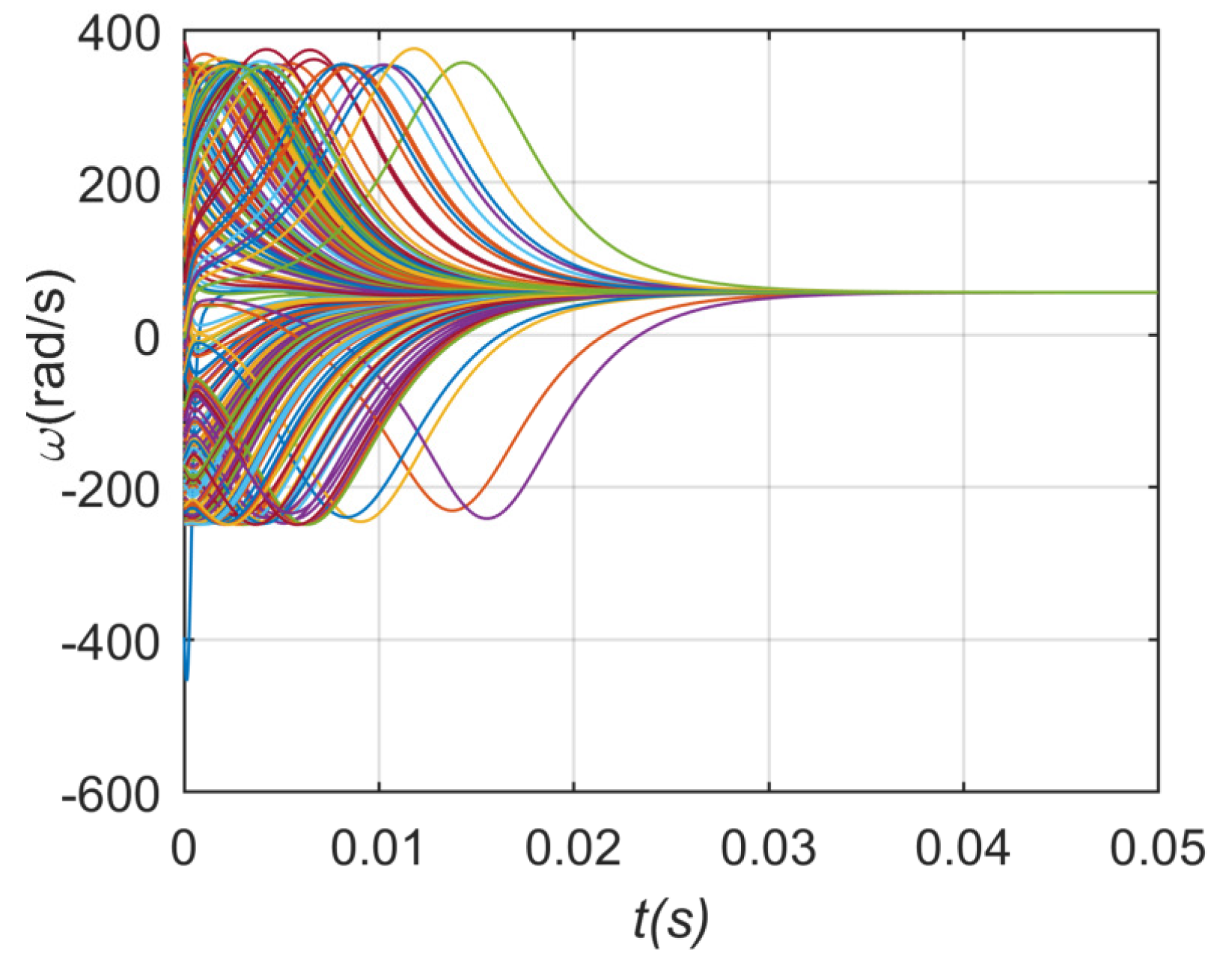
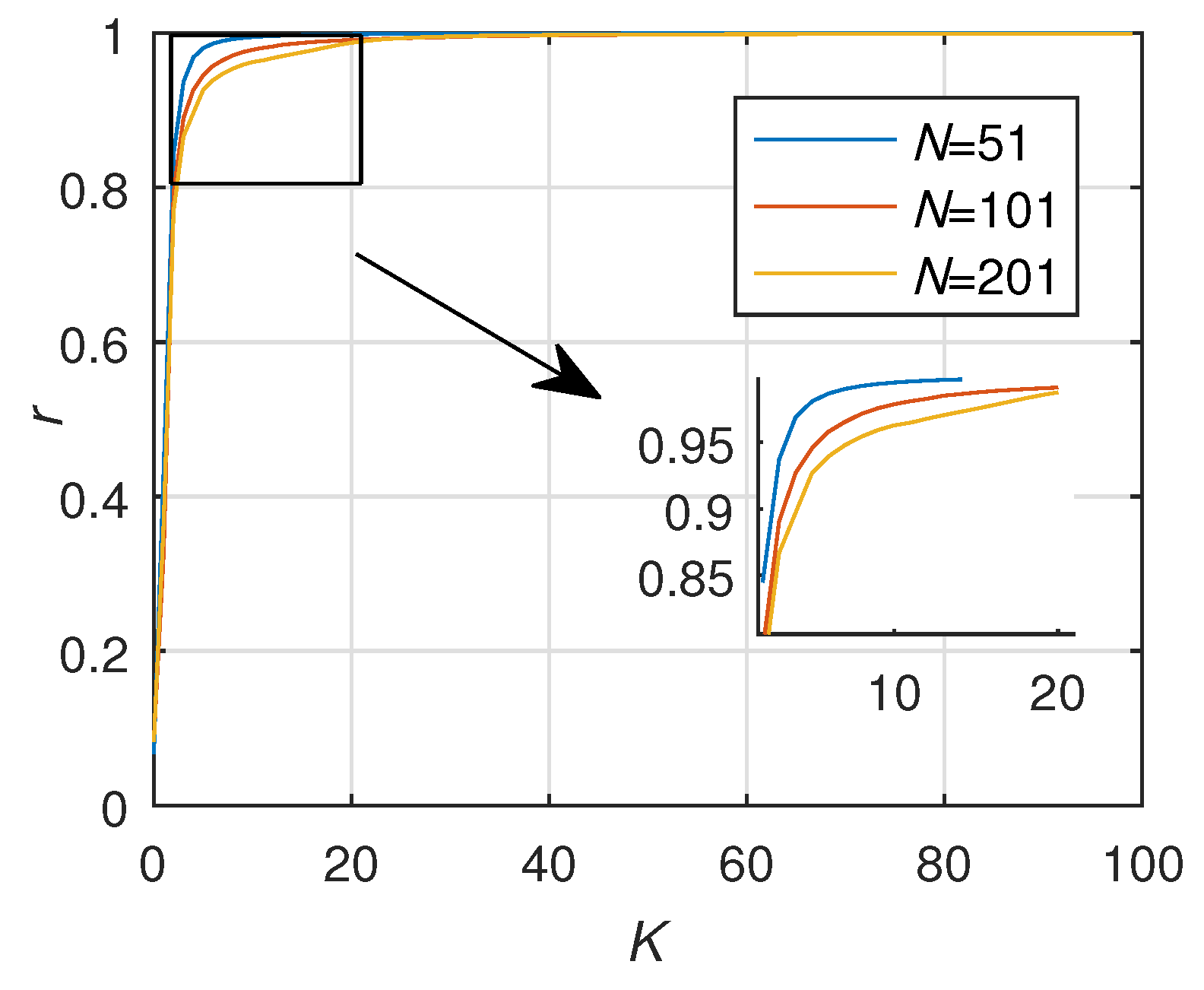
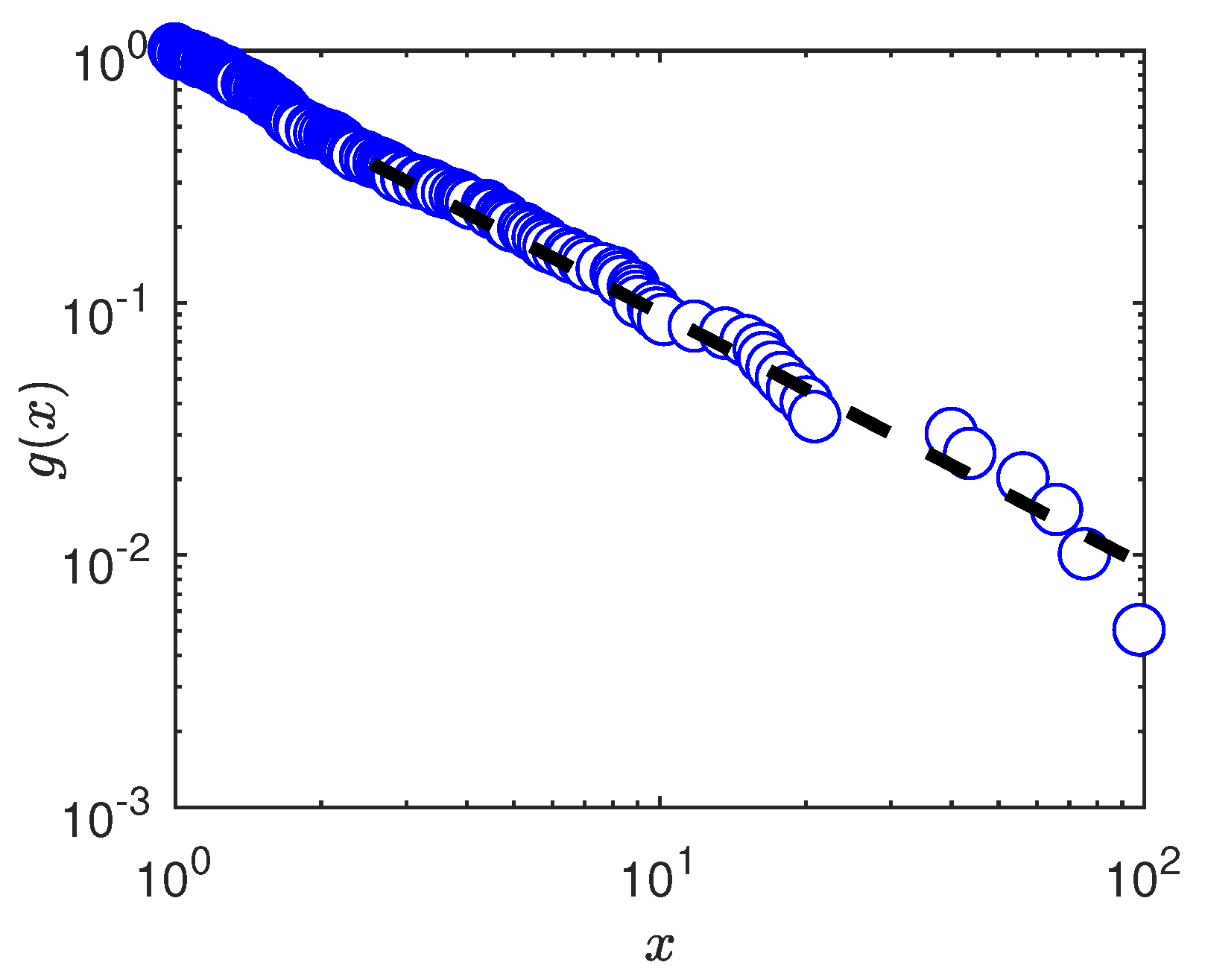



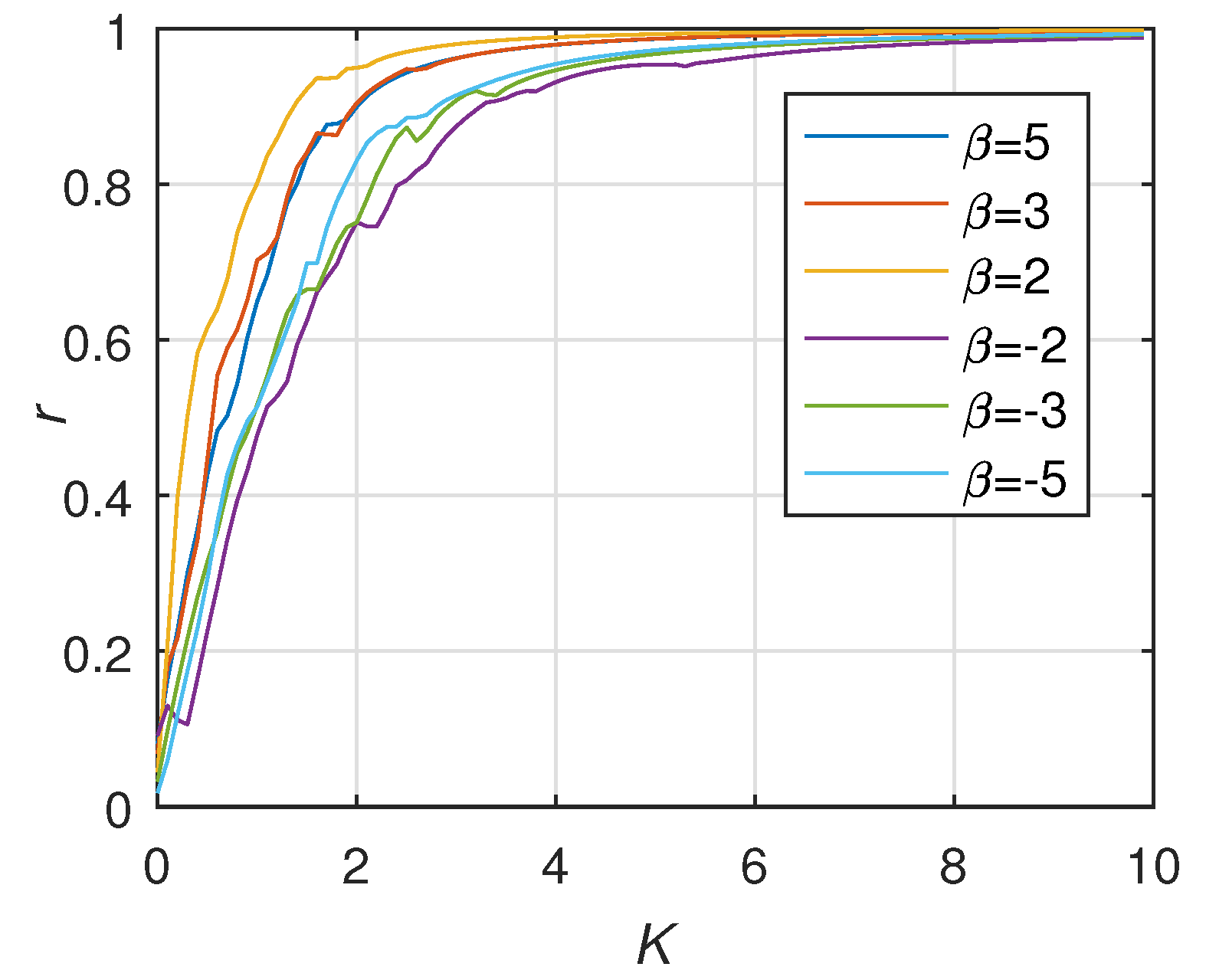
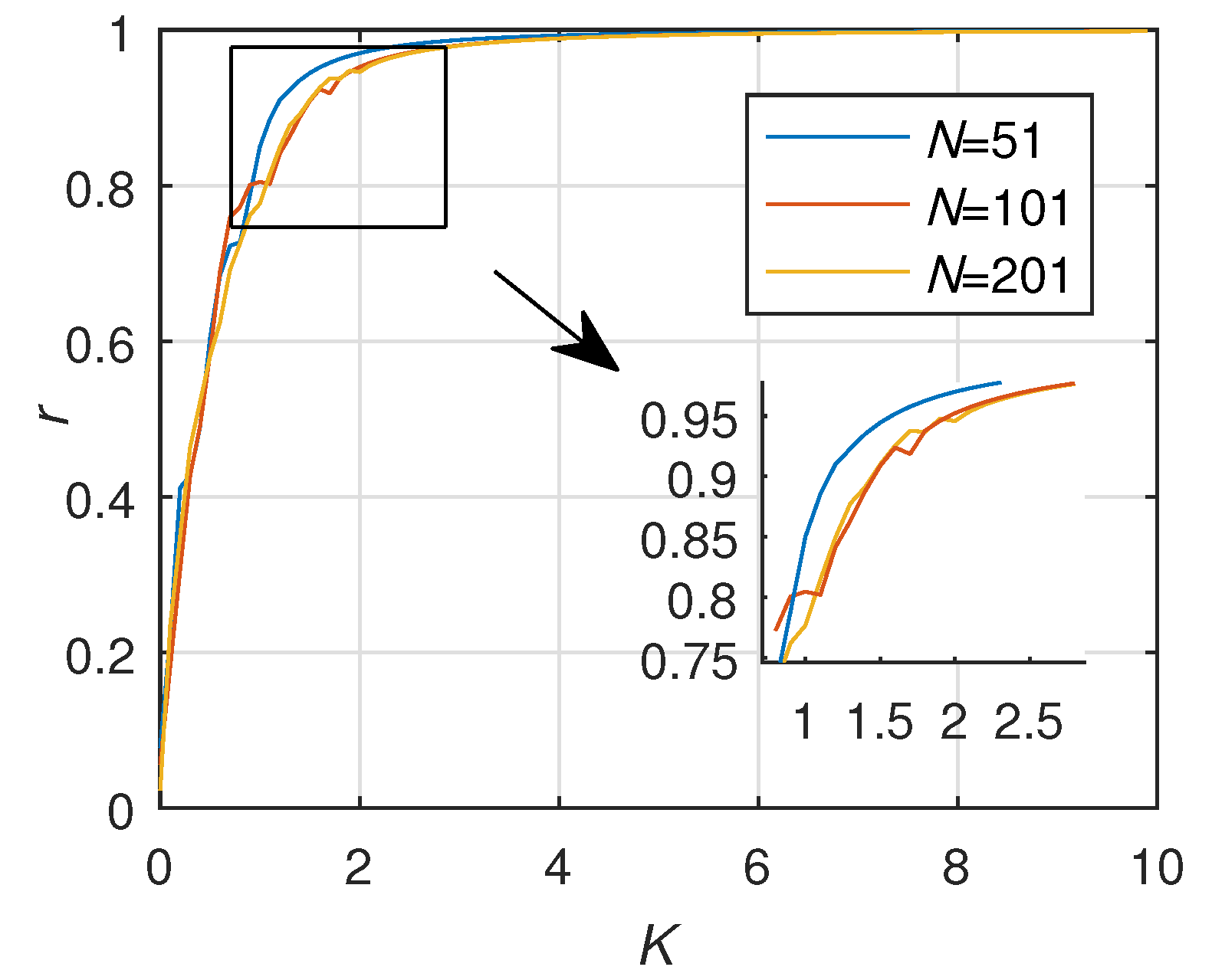

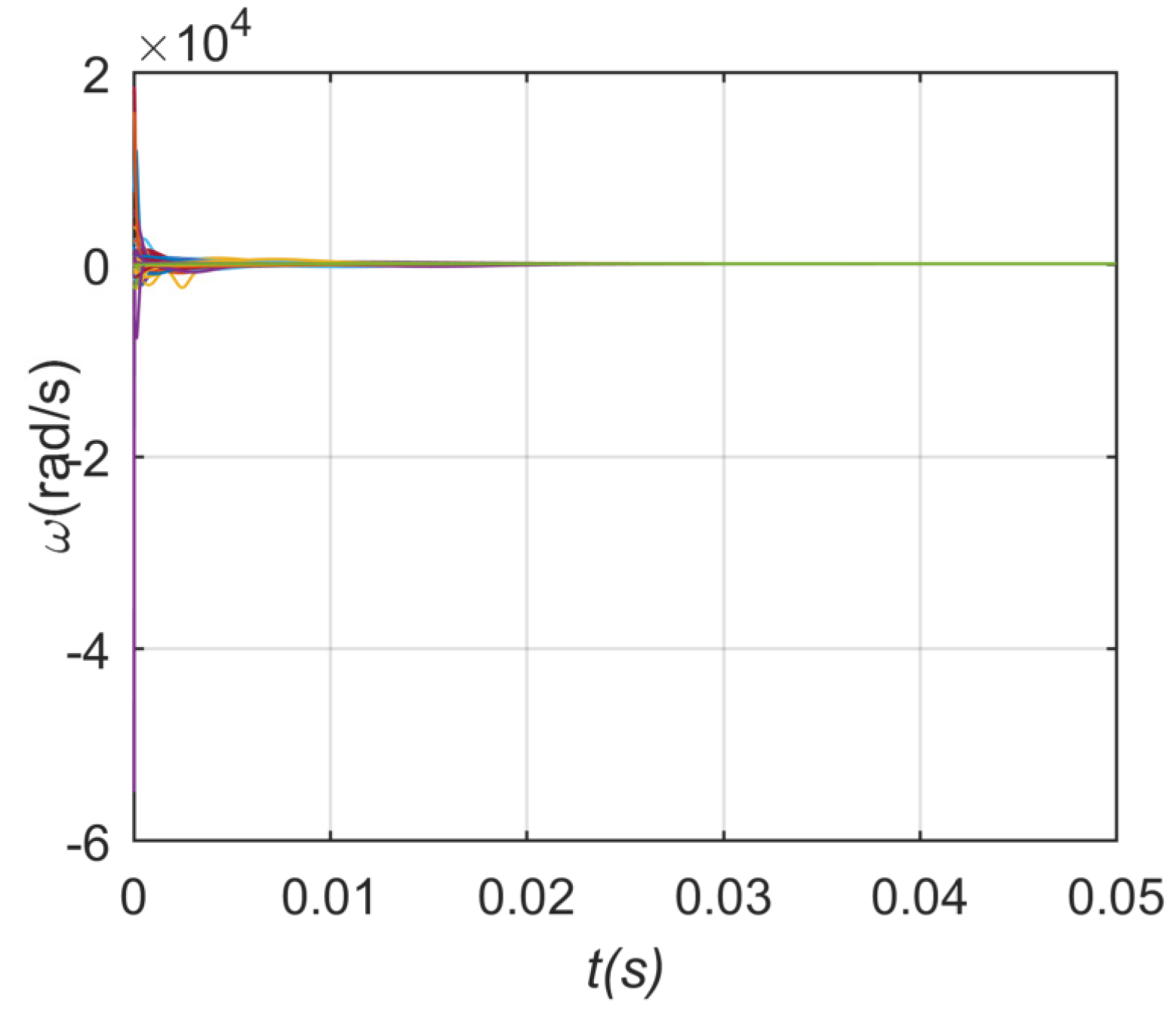
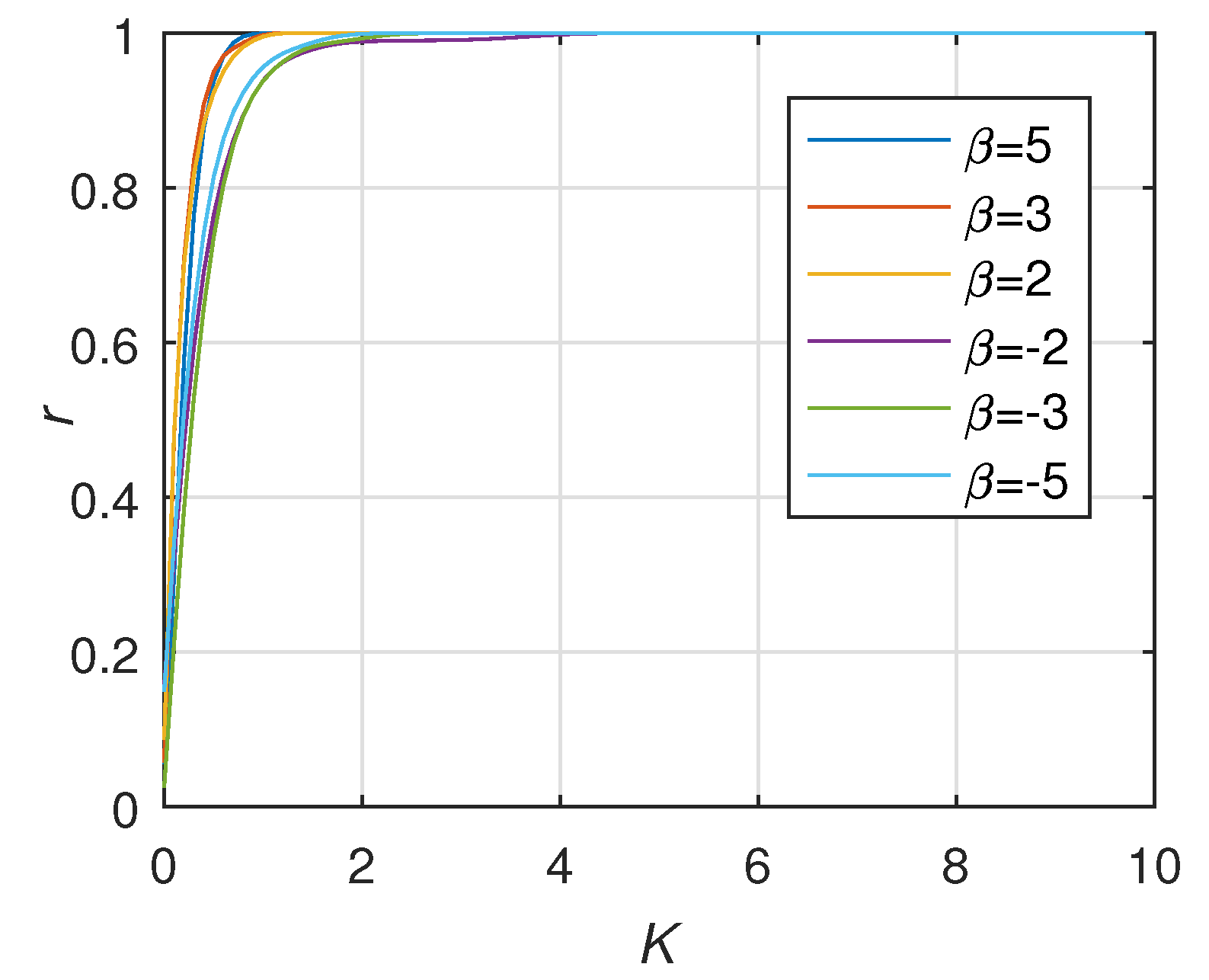


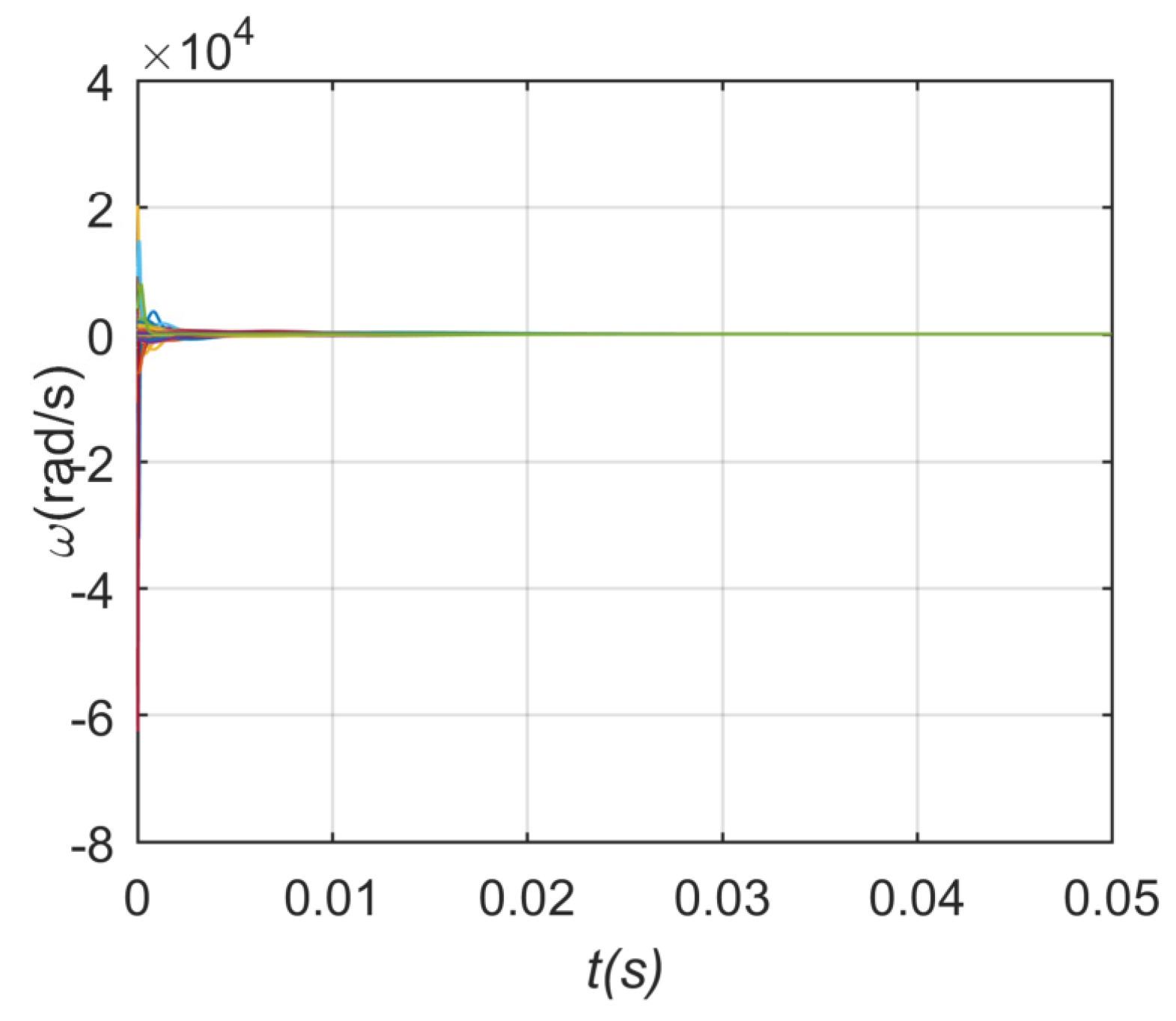
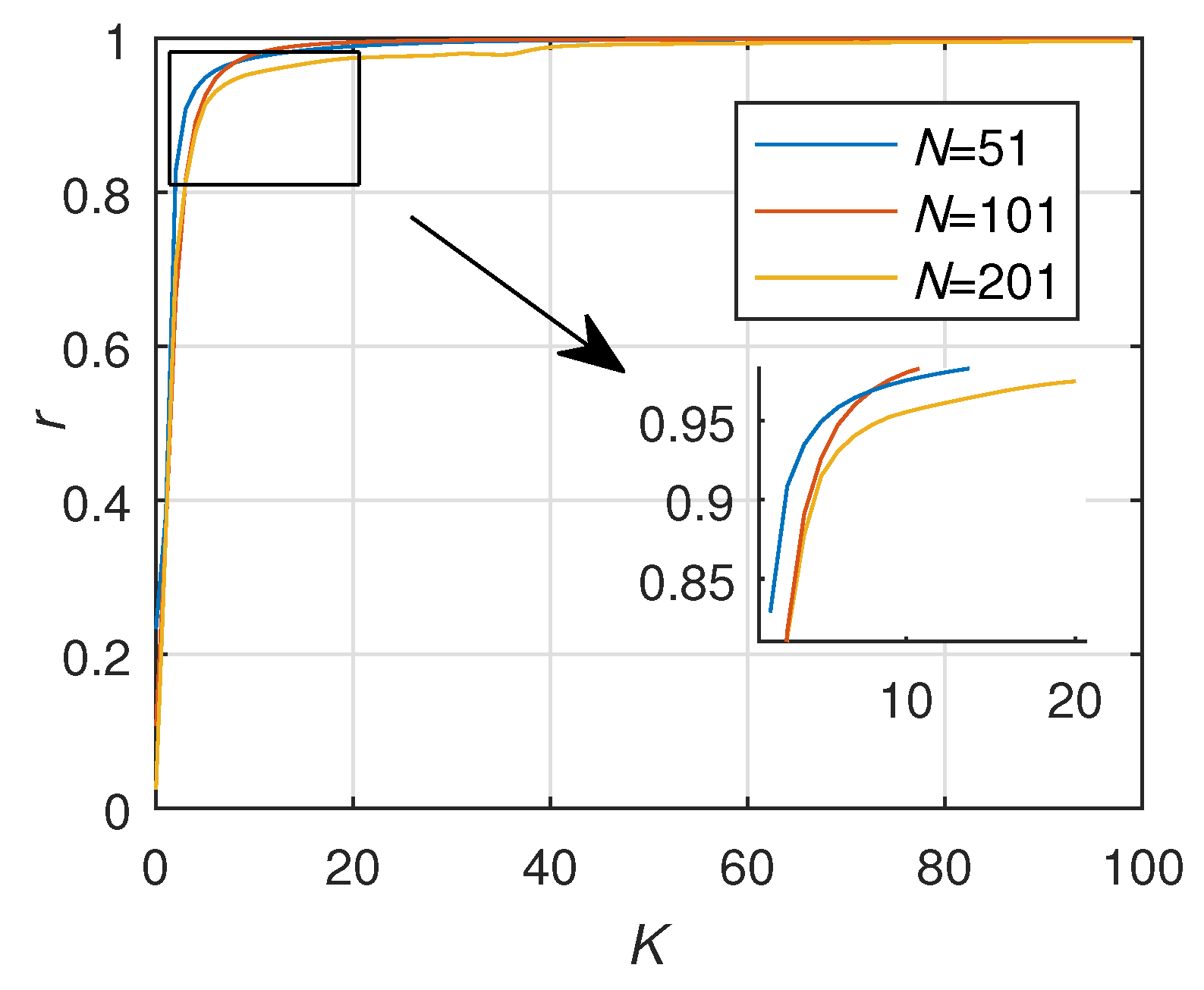
| Type | Parameter |
|---|---|
| wire type | YJV-3 |
| wire section | 3 × 120 mm |
| wire resistance | 0.153/km |
| wire length | 100 km |
| number of DG | 51/101/201 |
| wire type in inverter | YJV-4 |
| wire section in inverter | 4 × 16 mm |
| wire resistance in inverter | 1.15/km |
| wire length in inverter | 10 km |
| rated frequency of inverter | 50 Hz |
| distribution of inverter rated frequency | Gaussian distribution |
| distribution of weight index | Gaussian distribution |
| voltage classes | 220 V |
| Type | Parameter |
|---|---|
| wire type | YJV-3 |
| wire section | 3 × 120 mm |
| wire resistance | 0.153/km |
| wire length | 100 km |
| number of DG | 51/101/201 |
| wire type in inverter | YJV-4 |
| wire section in inverter | 4 × 16 mm |
| wire resistance in inverter | 1.15/km |
| wire length in inverter | 10 km |
| rated frequency of inverter | 50 Hz |
| distribution of inverter rated frequency | Gaussian distribution |
| distribution of weight index | Power-law distribution |
| voltage classes | 220 V |
| Distribution Mode | |||
|---|---|---|---|
| Gaussian distribution | –1.005 | –557.16 | 554.38 |
| Power-law distribution | –1.006 | –436.59 | 433.98 |
| Frequency-weighted | –1.0007 | –686.99 | 686.51 |
| IEEE Test Systems | 9-bus | 14-bus | 57-bus | 118-bus |
|---|---|---|---|---|
| Gaussian distribution | 3.4 | 4.2 | 47 | 56.1 |
| Power-law distribution | 1.1 | 1.5 | 10.7 | 16.1 |
| Frequency-weighted | 4.5 | 4.9 | 78.4 | 113.4 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, S.; Zhou, H.; Lai, J.; Zhou, Y.; Yu, C. Phase Synchronization Stability of Non-Homogeneous Low-Voltage Distribution Networks with Large-Scale Distributed Generations. Energies 2020, 13, 1257. https://doi.org/10.3390/en13051257
Chen S, Zhou H, Lai J, Zhou Y, Yu C. Phase Synchronization Stability of Non-Homogeneous Low-Voltage Distribution Networks with Large-Scale Distributed Generations. Energies. 2020; 13(5):1257. https://doi.org/10.3390/en13051257
Chicago/Turabian StyleChen, Shi, Hong Zhou, Jingang Lai, Yiwei Zhou, and Chang Yu. 2020. "Phase Synchronization Stability of Non-Homogeneous Low-Voltage Distribution Networks with Large-Scale Distributed Generations" Energies 13, no. 5: 1257. https://doi.org/10.3390/en13051257
APA StyleChen, S., Zhou, H., Lai, J., Zhou, Y., & Yu, C. (2020). Phase Synchronization Stability of Non-Homogeneous Low-Voltage Distribution Networks with Large-Scale Distributed Generations. Energies, 13(5), 1257. https://doi.org/10.3390/en13051257