Effects of Tip Clearance Size on Energy Performance and Pressure Fluctuation of a Tidal Propeller Turbine
Abstract
1. Introduction
2. Turbine Model
2.1. Physical Model of Propeller Turbine
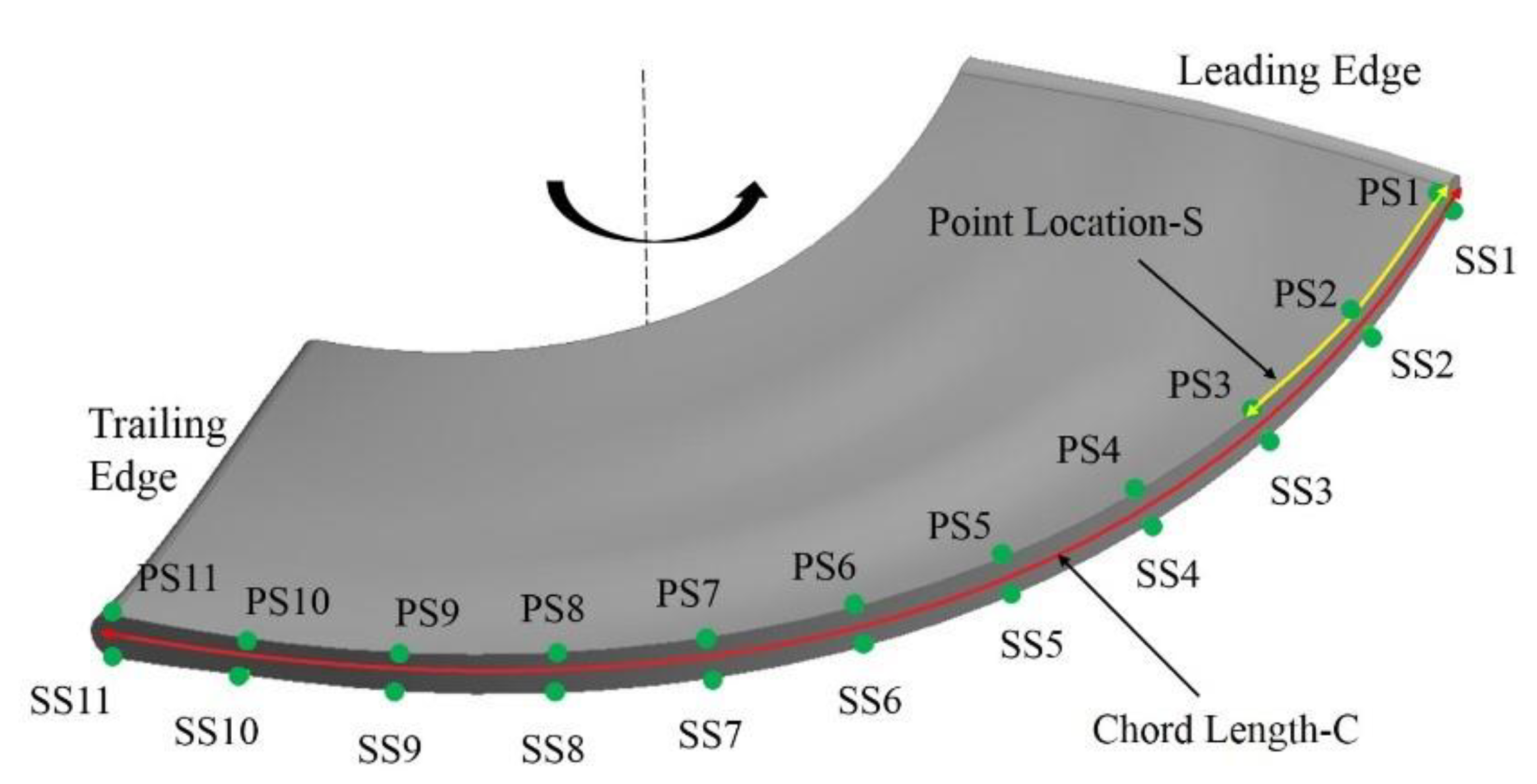
2.2. Tip-Clearance Configurations and Monitoring Points
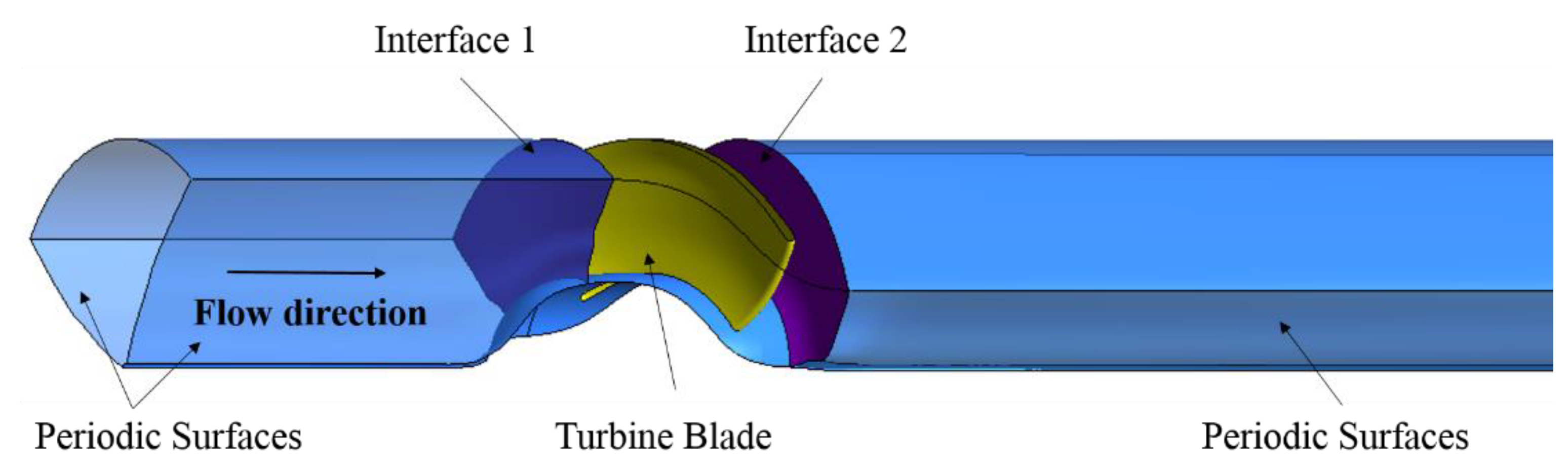
3. Numerical Method
3.1. Turbulence Model and Boundary Conditions
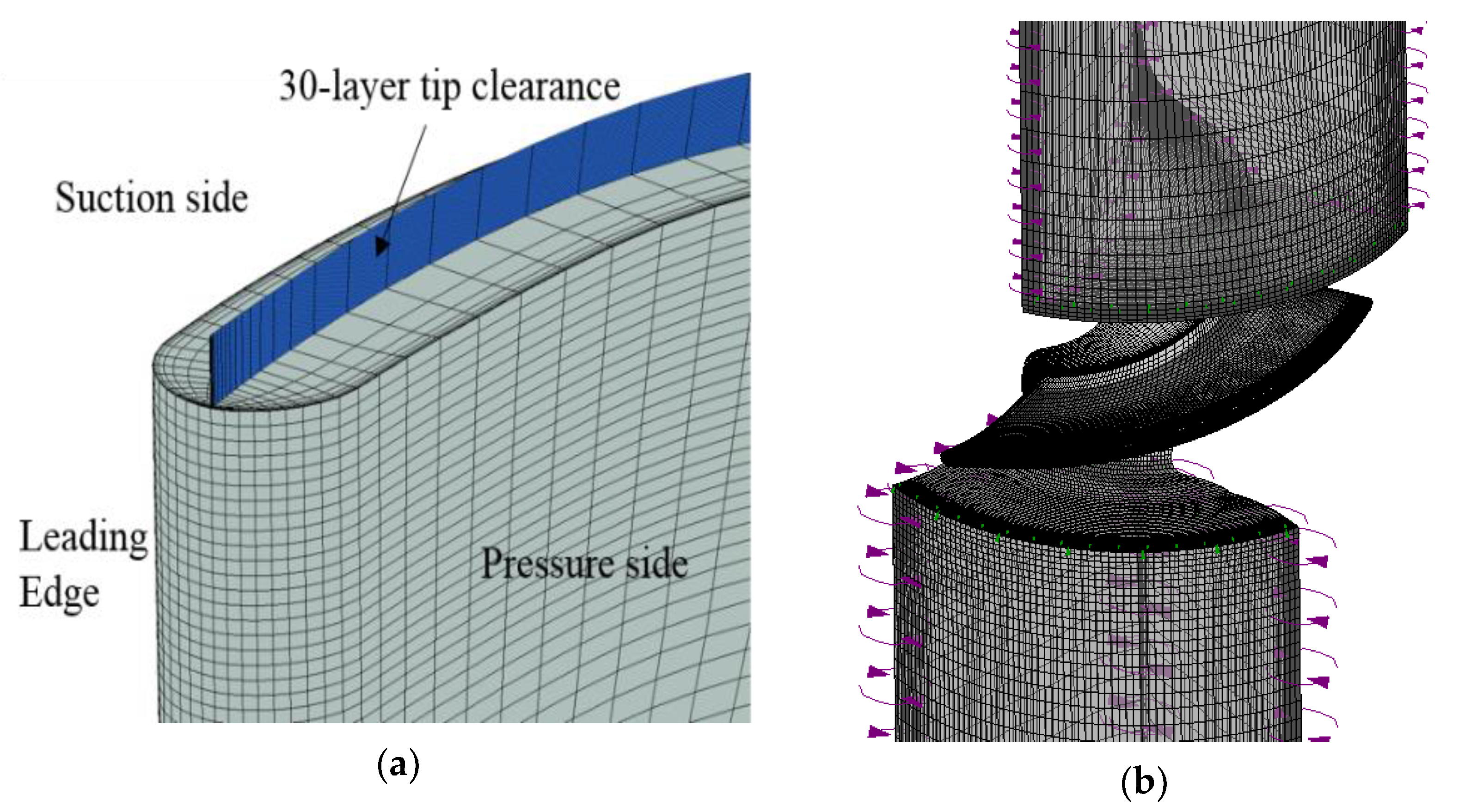
3.2. Mesh Strategy and Grid Independent Analysis
3.3. Numerical Results Verification
4. Results and Discussion
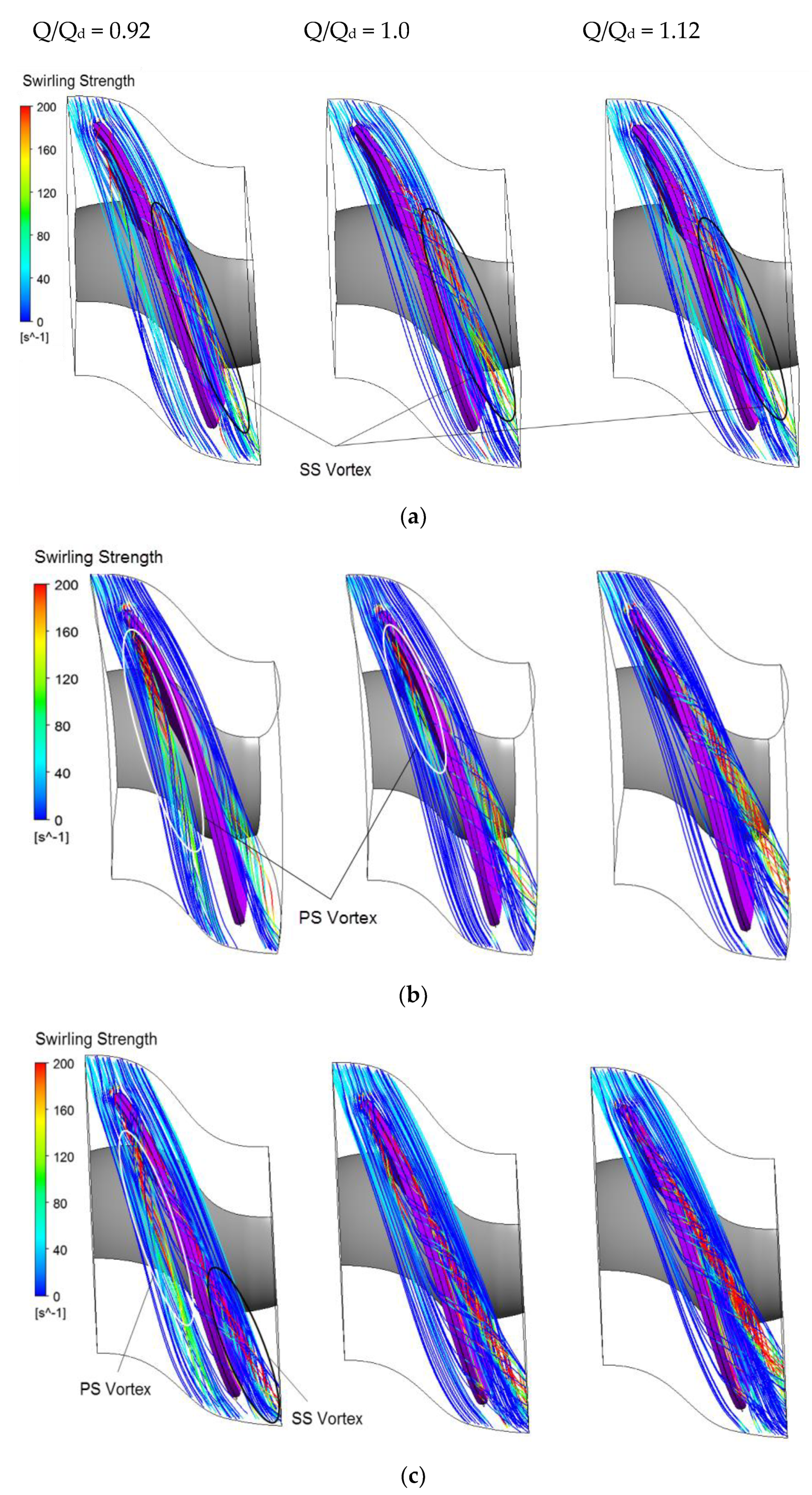
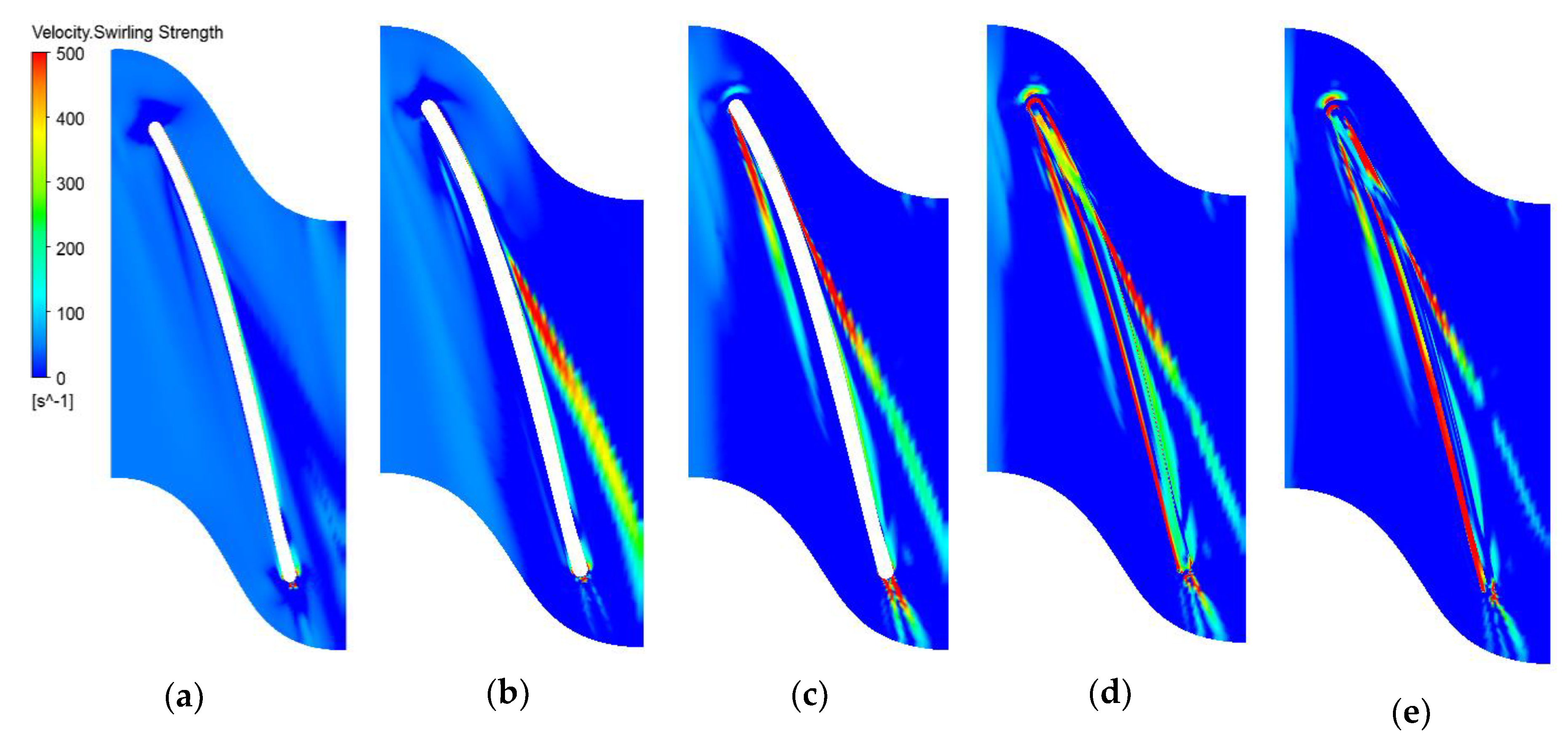
4.1. Trajectories of Tip-Leakage Vortex at Different Tip-Clearance Sizes and Various Flow Conditions
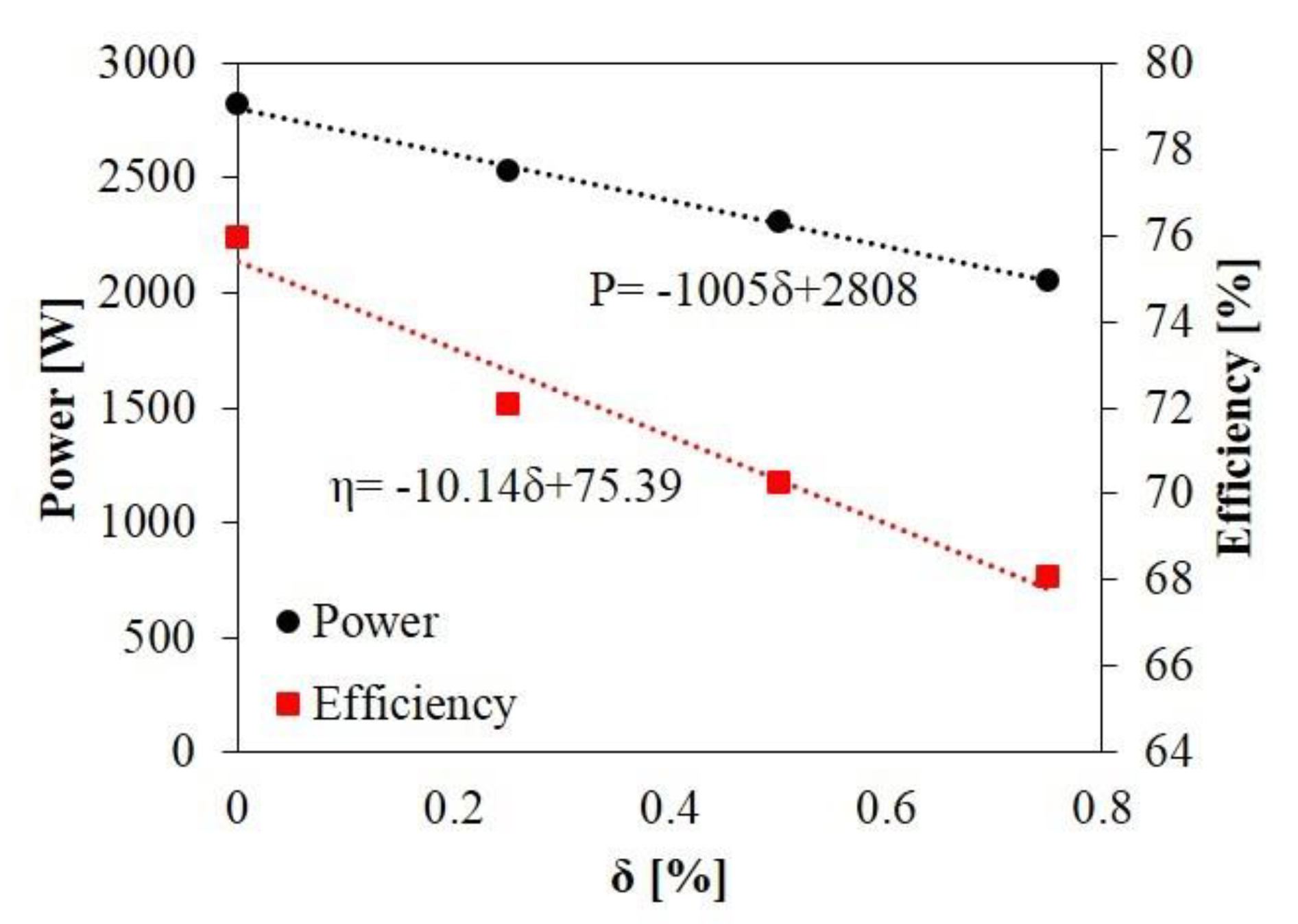
4.2. Effects of Tip-Leakage Flow on Turbine Performance
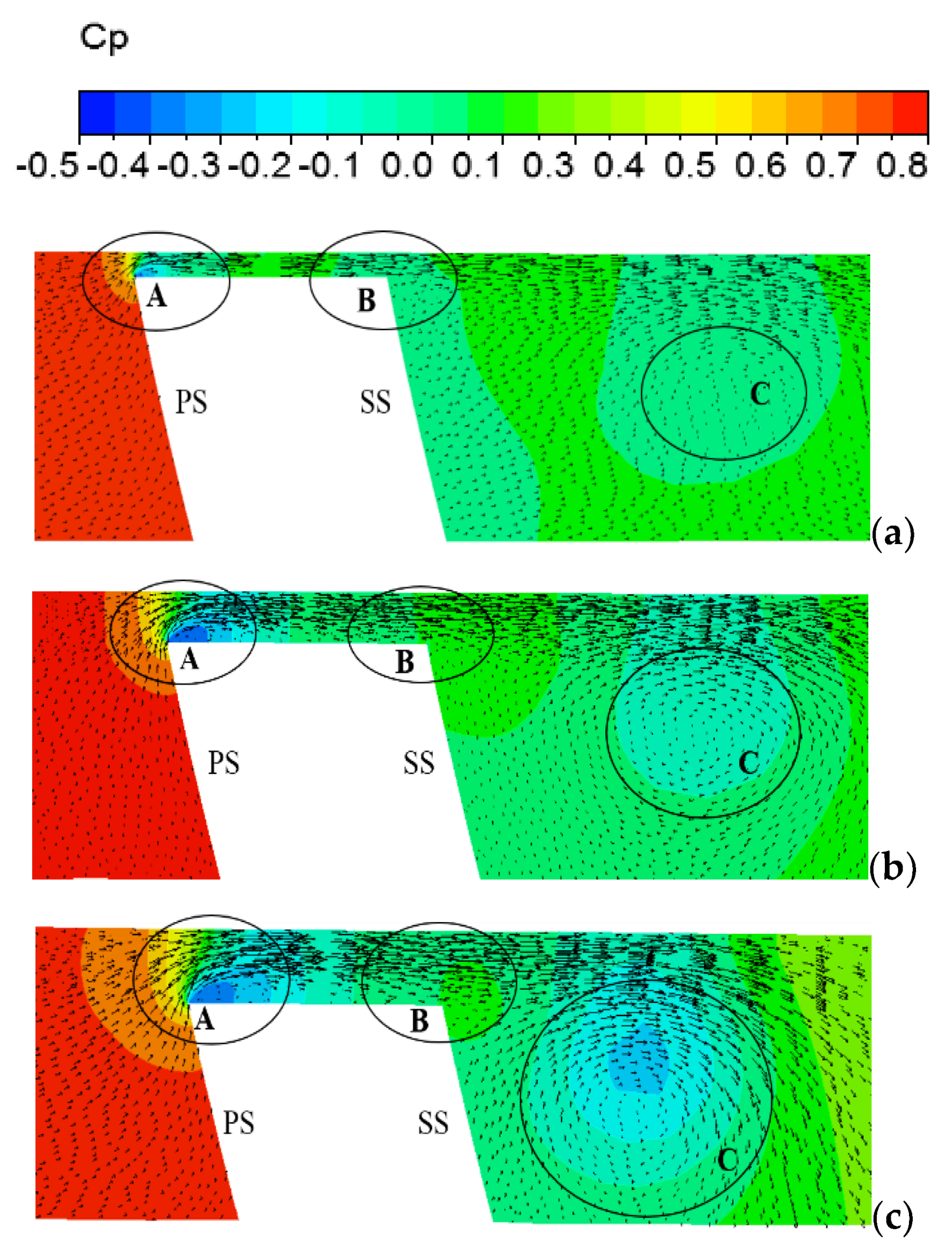
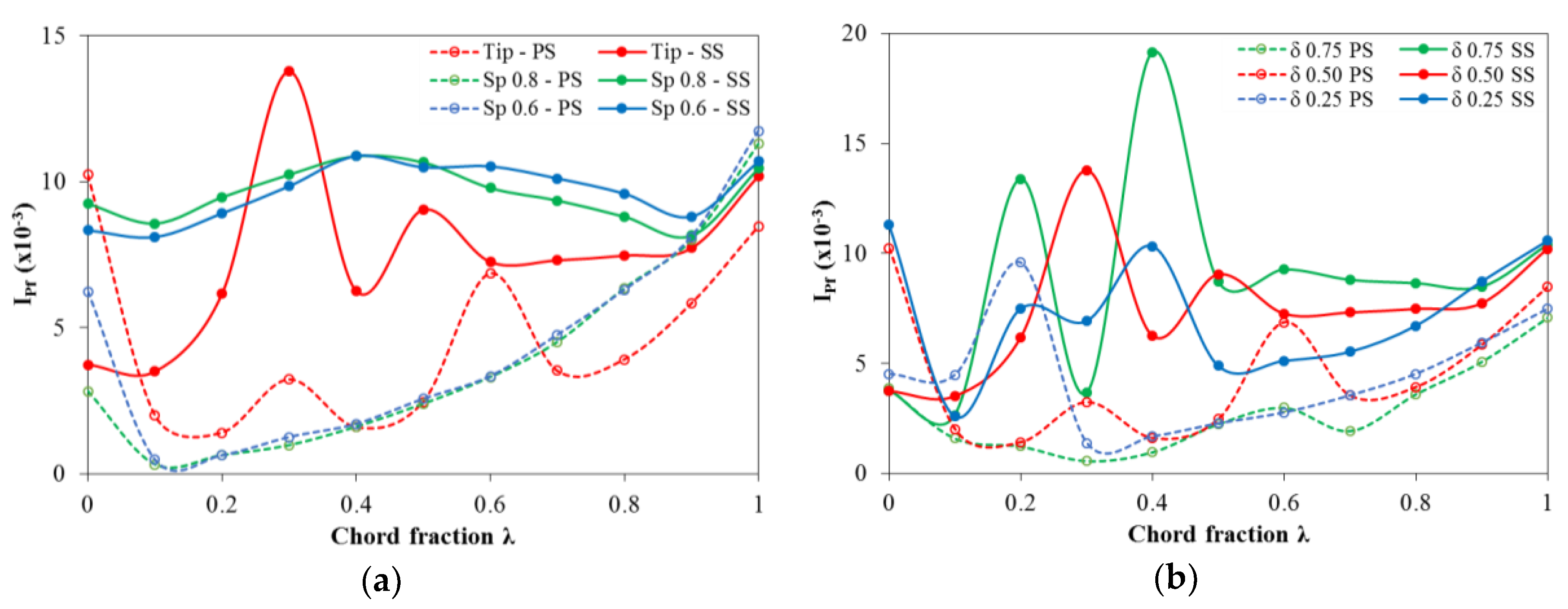
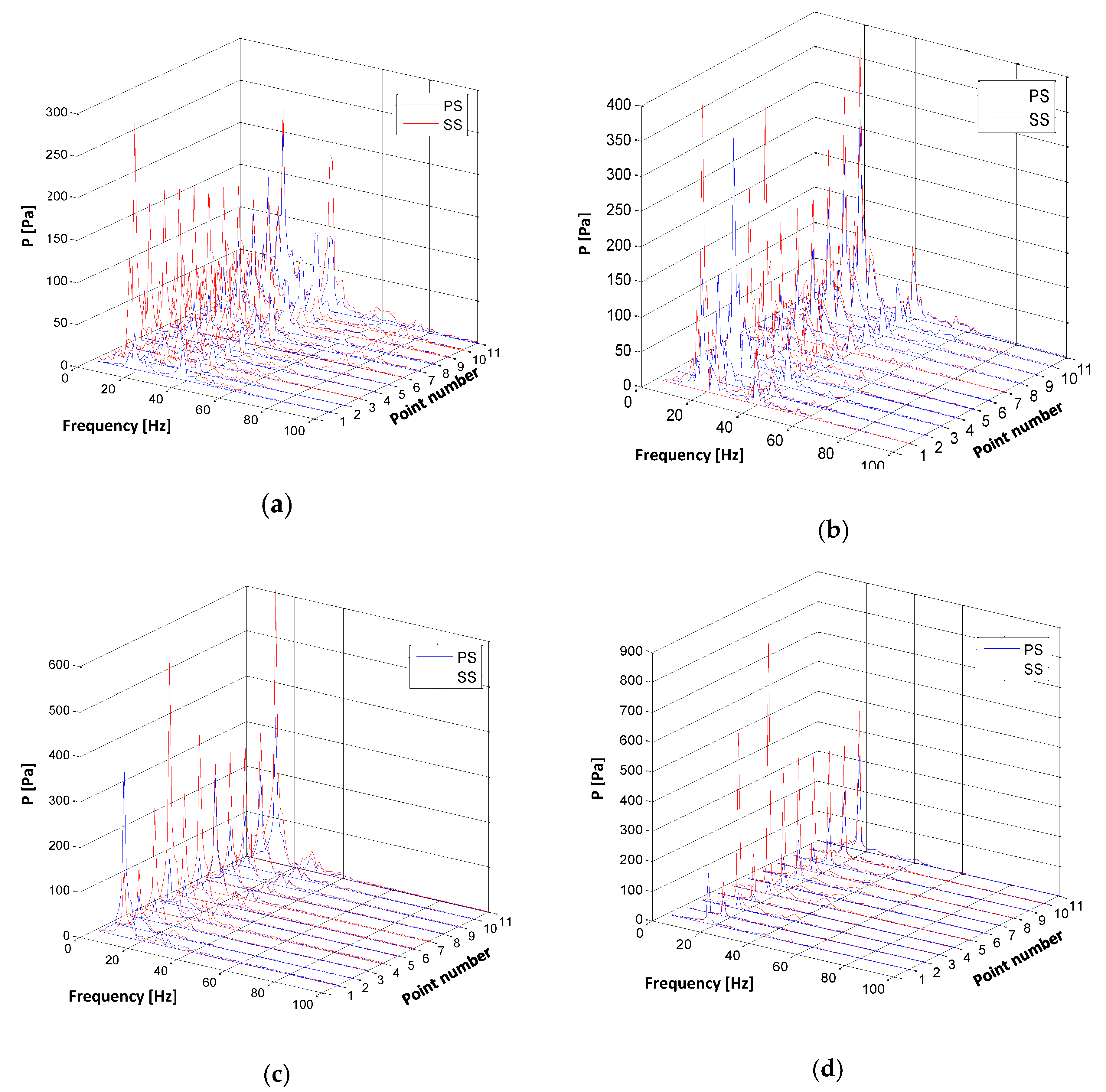
4.3. Analysis of the Pressure Fluctuations on Turbine Blade
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| P | Power output | H | Effective head |
| η | Efficiency | T | Torque |
| Qd | Design flow rate | pi | Pressure at each time step |
| N | Rotational speed | Ipf | Dimensionless of pressure fluctuation intensity |
| Dt | Tip diameter | μt | Turbulence viscosity |
| Dh | Hub diameter | k | Turbulence kinetic energy |
| Z | Number of blades | ω | Turbulence frequency |
| δ | Dimensionless of tip clearance | F2 | Blending function |
| λ | Blade chord fraction | α1 | Model constant |
| C | Blade chord length | β′ | Model constant |
| S | Monitoring point position | ∆V | Leakage volume |
| CP | Pressure coefficient | TCS | Tip-clearance size |
| Plocal | Local static pressure | TLV | Tip-leakage vortex |
| Utip | Velocity of the blade tip | SST | Shear-stress transport |
| ρ | Fluid density | PS | Pressure side |
| Ph | Hydraulic power | SS | Suction side |
| Pmec | Mechanical power |
References
- Rains, D.A. Tip Clearance Flows in Axial Compressors and Pumps. Ph.D. Thesis, California Institute of Technology, Pasadena, CA, USA, 1954. [Google Scholar]
- Khalid, S.A.; Khalsa, A.S.; Waitz, I.A.; Tan, C.S.; Greitzer, E.M.; Cumpsty, N.A. Endwall blockage in axial compressors. J. Turbomach. 1999, 121, 499–509. [Google Scholar] [CrossRef]
- Denton, J.D. The 1993 IGTI scholar lecture: Loss mechanisms in turbomachines. J. Turbomach. 1993, 115, 621–656. [Google Scholar] [CrossRef]
- Hsiao, C.T.; Chahine, G.L. Scaling of tip vortex cavitation inception noise with a bubble dynamics model accounting for nuclei size distribution. J. Fluids Eng. 2005, 127, 55–65. [Google Scholar] [CrossRef]
- Wernet, M.P.; Zante, D.V.; Strazisar, T.J.; John, W.T.; Prahst, P.S. Characterization of the tip clearance flow in an axial compressor using 3D digital PIV. Exp. Fluids 2005, 39, 743–753. [Google Scholar] [CrossRef]
- Wu, H.; Miorini, R.L.; Katz, J. Measurements of the tip leakage vortex structures and turbulence in the meridional plane of an axial water-jet pump. Exp. Fluids 2011, 50, 989–1003. [Google Scholar] [CrossRef]
- Wu, H.; Miorini, R.L.; Tan, D.; Katz, J. Turbulence within the tip-leakage vortex of an axial waterjet pump. AIAA J. 2012, 50, 2574–2587. [Google Scholar] [CrossRef]
- Miorini, R.L.; Wu, H.; Katz, J. The internal structure of the tip leakage vortex within the rotor of an axial waterjet pump. J. Turbomach. 2012, 134, 031018. [Google Scholar] [CrossRef]
- Jang, C.M. Analysis of vortical flow field in a propeller fan by LDV measurements and LES-Part I: Three-dimensional vortical flow structures. J. Fluids Eng. 2001, 123, 748–754. [Google Scholar] [CrossRef]
- Zhang, D.S.; Shi, W.; Esch, B.P.M.B.; Shi, L.; Dubuisson, M. Numerical and experimental investigation of tip leakage vortex trajectory and dynamics in an axial flow pump. Comput. Fluids 2015, 112, 61–71. [Google Scholar] [CrossRef]
- Liu, Y.; Tan, L.; Hao, Y.; Xu, Y. Energy performance and flow patterns of a mixed flow pump with different tip clearance sizes. Energies 2017, 10, 191. [Google Scholar] [CrossRef]
- Xu, Y.; Tan, L.; Liu, Y.; Cao, S. Pressure fluctuation and flow pattern of a mixed-flow pump with different blade tip clearances under cavitation condition. Adv. Mech. Eng. 2017, 9, 1–12. [Google Scholar] [CrossRef]
- Ji, L.; Li, W.; Shi, W.; Chang, H.; Yang, Z. Energy characteristics of mixed-flow pump under different tip clearances based on entropy production analysis. Energy 2020, 199, 117447. [Google Scholar] [CrossRef]
- Lu, L.; Pan, G.; Wei, J.; Pan, Y. Numerical simulation of tip clearance impact on a pumpjet propulsor. Int. J. Nav. Arch. Ocean Eng. 2016, 8, 219–227. [Google Scholar] [CrossRef]
- Meng, F.; Zheng, Q.; Gao, J.; Fu, W. Effect of tip clearance on flow field and heat transfer characteristics in a large meridional expansion turbine. Energies 2019, 12, 162. [Google Scholar] [CrossRef]
- Qiu, C.; Huang, Q.; Pan, G.; Shi, Y.; Dong, X. Numerical simulation of hydrodynamic and cavitation performance of pumpjet propulsor with different tip clearances in oblique flow. Ocean Eng. 2020, 209, 107285. [Google Scholar] [CrossRef]
- Moghadam, S.M.A.; Meinke, M.; Schröder, W. Analysis of tip-leakage flow in an axial fan at varying tip-gap sizes and operating conditions. Comput. Fluids 2019, 183, 107–129. [Google Scholar] [CrossRef]
- Tran, B.N.; Kim, J.H. Design and analysis of a pico propeller hydro turbine applied in fish farms using CFD and experimental method. J. Korean Soc. Mar. Environ. Saf. 2019, 25, 373–380. [Google Scholar] [CrossRef]
- Singh, P.; Nestmann, F. Experimental optimization of a free vortex propeller runner for micro hydro application. Exp. Therm. Fluid Sci. 2009, 33, 991–1002. [Google Scholar] [CrossRef]
- Muis, A.; Sutikno, P. Design and simulation of very low head axial hydraulic turbine with variation of swirl velocity criterion. Int. J. Fluid Mach. Syst. 2014, 7, 68–79. [Google Scholar] [CrossRef]
- Menter, F.R. Review of the shear-stress transport turbulence model experience from an industrial perspective. Int. J. Comput. Fluid. Dyn. 2009, 23, 305–316. [Google Scholar] [CrossRef]
- Bardina, J.E.; Huang, P.G.; Coakley, T.J. Turbulence Modeling Validation, Testing, and Development; NASA Technical Memorandum 110446; Ames Research Center: Moffett Field, CA, USA, 1997; pp. 15–25.
- Chen, Z.; Kim, J.C.; Im, M.H.; Choi, Y.D. Analysis on the performance and internal flow of a tubular type hydro turbine for vessel cooling system. J. Korean Soc. Mar. Eng. 2014, 38, 1244–1250. [Google Scholar] [CrossRef]
- ANSYS, Inc. ANSYS CFX-Solver Theory Guide, Release 15.0; SAS IP, Inc.: Pittsburgh, PA, USA, 2013; pp. 89–90. [Google Scholar]
- Blazek, J. Computational Fluid Dynamics: Principles and Applications; Elsevier: London, UK, 2001; pp. 353–392. [Google Scholar]
- Samora, I.; Hasmatuchi, V.; Münch-Allign, C.; Franca, M.J.; Schleiss, A.J.; Ramos, H.M. Experimental characterization of a five blade tubular propeller turbine for pipe inline installation. Renew. Energy 2016, 95, 356–366. [Google Scholar] [CrossRef]
- Zhou, J.; Adrian, R.J.; Balachandar, S.; Kendall, T.M. Mechanisms for generation coherent packets of hairpin vortices in channel flow. J. Fluid Mech. 1999, 387, 353–396. [Google Scholar] [CrossRef]
- Jung, J.H.; Joo, W.G. Effect of tip clearance, winglets, and shroud height on the tip leakage in axial flow fans. Int. J. Refrig. 2018, 93, 195–204. [Google Scholar] [CrossRef]
- Yu, H.; Zhang, Z.; Hua, H. Numerical investigation of tip clearance effects on propulsion performance and pressure fluctuation of a pump-jet propulsor. Ocean Eng. 2019, 192, 106500. [Google Scholar] [CrossRef]
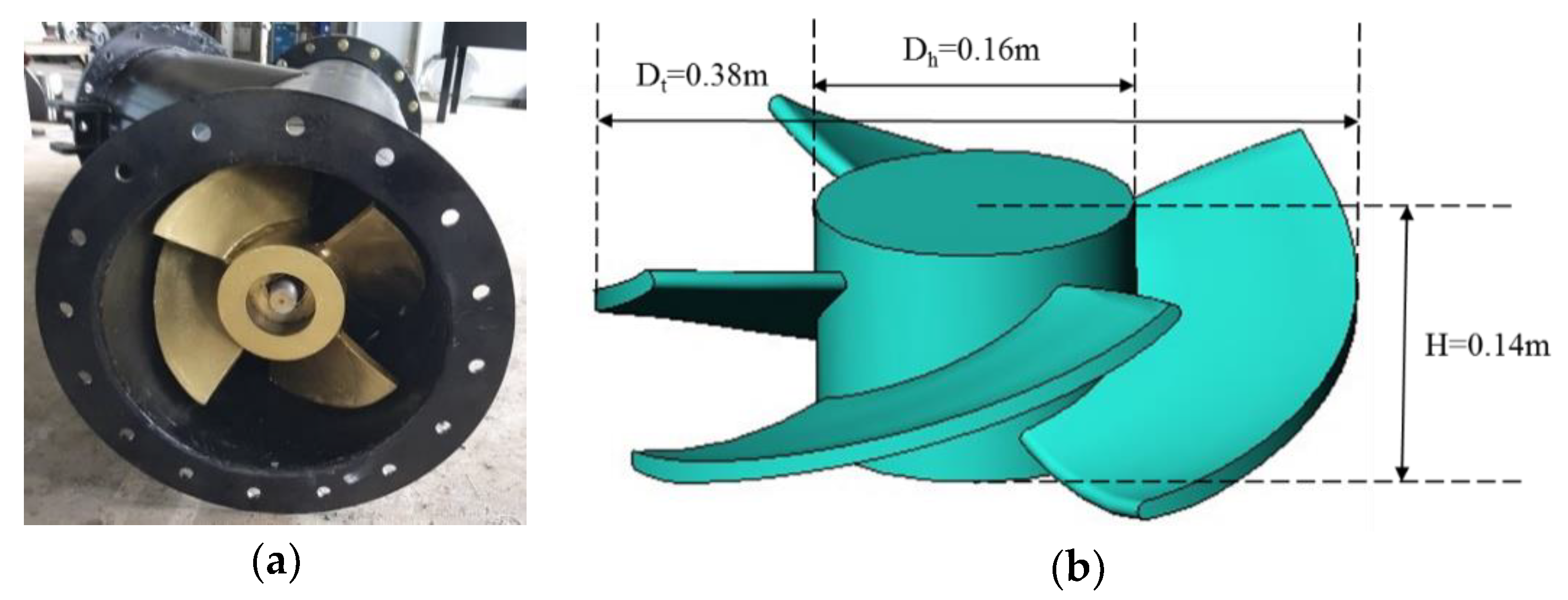



| Parameters | Symbol | Unit | Value |
|---|---|---|---|
| Power output | P | kW | 3 |
| Gross head | H | m | 2 |
| Designed flow rate | Qd | m3/s | 0.25 |
| Rotational speed | N | RPM | 450 |
| Tip diameter | Dt | m | 0.38 |
| Hub diameter | Dh | m | 0.16 |
| Hub-to-tip ratio | Dh/Dt | - | 0.4 |
| Number of blades | Z | - | 4 |
| Domains | M1 | M2 | M3 | M4 | M5 |
|---|---|---|---|---|---|
| Suction | 67,600 | 114,920 | 188,825 | 25,220 | 318,825 |
| Runner | 391,560 | 512,040 | 624,990 | 730,410 | 820,770 |
| Discharge | 148,512 | 277,440 | 395,080 | 527,680 | 667,080 |
| Mesh size | 607,672 | 904,400 | 1,208,895 | 1,510,290 | 1,806,675 |
| p/P1 | 1 | 1.002414 | 1.003535 | 1.00368 | 1.004445 |
| η/η1 | 1 | 0.999802 | 0.999746 | 0.999604 | 0.999472 |
| Monitor Points | Maximal Amplitude of Pressure Fluctuation [Pa] | Dominant Frequency [Hz] | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| δ = 0% | δ = 0.25% | δ = 0.5% | δ = 0.75% | δ = 0% | δ = 0.25% | δ = 0.5% | δ = 0.75% | |||||
| PS | SS | PS | SS | PS | SS | PS | SS | |||||
| P1 | 45 | 286 | 161 | 398 | 390 | 150 | 165 | 167 | 18 | 18 | 16 | 17 |
| P2 | 20 | 180 | 164 | 82 | 75 | 136 | 70 | 120 | 18 | 18 | 16 | 17 |
| P3 | 25 | 190 | 345 | 276 | 56 | 250 | 51 | 588 | 18 | 18 | 16 | 17 |
| P4 | 30 | 185 | 46 | 250 | 125 | 560 | 25 | 159 | 18 | 18 | 16 | 17 |
| P5 | 48 | 178 | 60 | 365 | 63 | 254 | 46 | 842 | 18 | 18 | 16 | 17 |
| P6 | 51 | 175 | 72 | 173 | 90 | 366 | 100 | 370 | 18 | 18 | 16 | 17 |
| P7 | 75 | 166 | 98 | 180 | 262 | 295 | 130 | 406 | 18 | 18 | 16 | 17 |
| P8 | 90 | 157 | 125 | 202 | 139 | 300 | 79 | 381 | 18 | 18 | 16 | 17 |
| P9 | 120 | 140 | 170 | 246 | 148 | 302 | 161 | 375 | 18 | 18 | 16 | 17 |
| P10 | 160 | 42 | 218 | 314 | 220 | 318 | 223 | 370 | 18 | 18 | 16 | 17 |
| P11 | 215 | 230 | 275 | 370 | 335 | 600 | 315 | 465 | 18 | 18 | 16 | 17 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tran, B.N.; Jeong, H.; Kim, J.-H.; Park, J.-S.; Yang, C. Effects of Tip Clearance Size on Energy Performance and Pressure Fluctuation of a Tidal Propeller Turbine. Energies 2020, 13, 4055. https://doi.org/10.3390/en13164055
Tran BN, Jeong H, Kim J-H, Park J-S, Yang C. Effects of Tip Clearance Size on Energy Performance and Pressure Fluctuation of a Tidal Propeller Turbine. Energies. 2020; 13(16):4055. https://doi.org/10.3390/en13164055
Chicago/Turabian StyleTran, Bao Ngoc, Haechang Jeong, Jun-Ho Kim, Jin-Soon Park, and Changjo Yang. 2020. "Effects of Tip Clearance Size on Energy Performance and Pressure Fluctuation of a Tidal Propeller Turbine" Energies 13, no. 16: 4055. https://doi.org/10.3390/en13164055
APA StyleTran, B. N., Jeong, H., Kim, J.-H., Park, J.-S., & Yang, C. (2020). Effects of Tip Clearance Size on Energy Performance and Pressure Fluctuation of a Tidal Propeller Turbine. Energies, 13(16), 4055. https://doi.org/10.3390/en13164055






