Comparison of Control Strategies to Realize Synthetic Inertia in Converters
Abstract
:1. Introduction
2. Strategies to Realize Synthetic Inertia
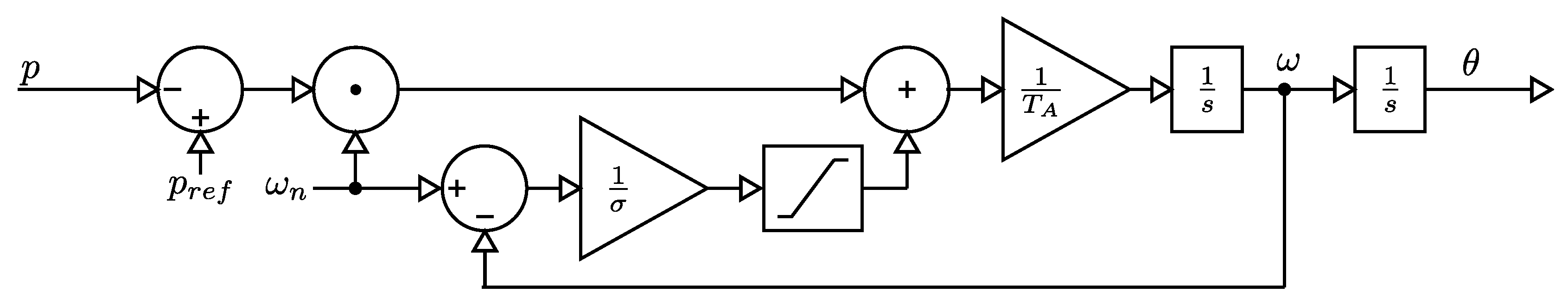
- “Active synthetic inertia”, realized by using a grid-forming control strategy that relies on determining the grid angle based on Equation (5) and
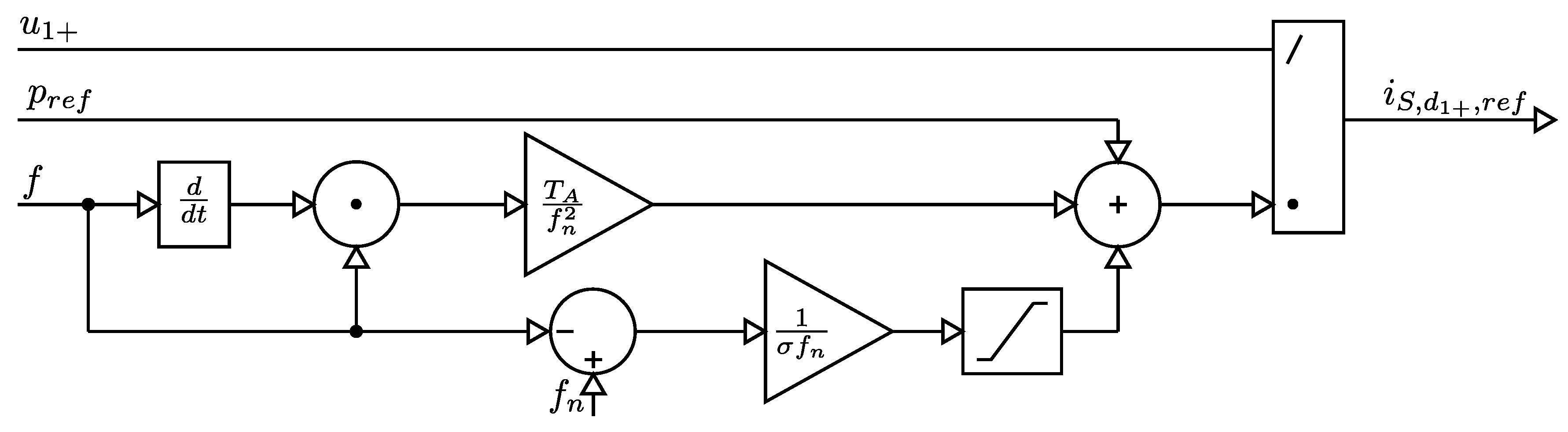
- “Passive synthetic inertia”, realized by calculating a reference power according to Equation (4) that is used in the control of a grid-following converter.
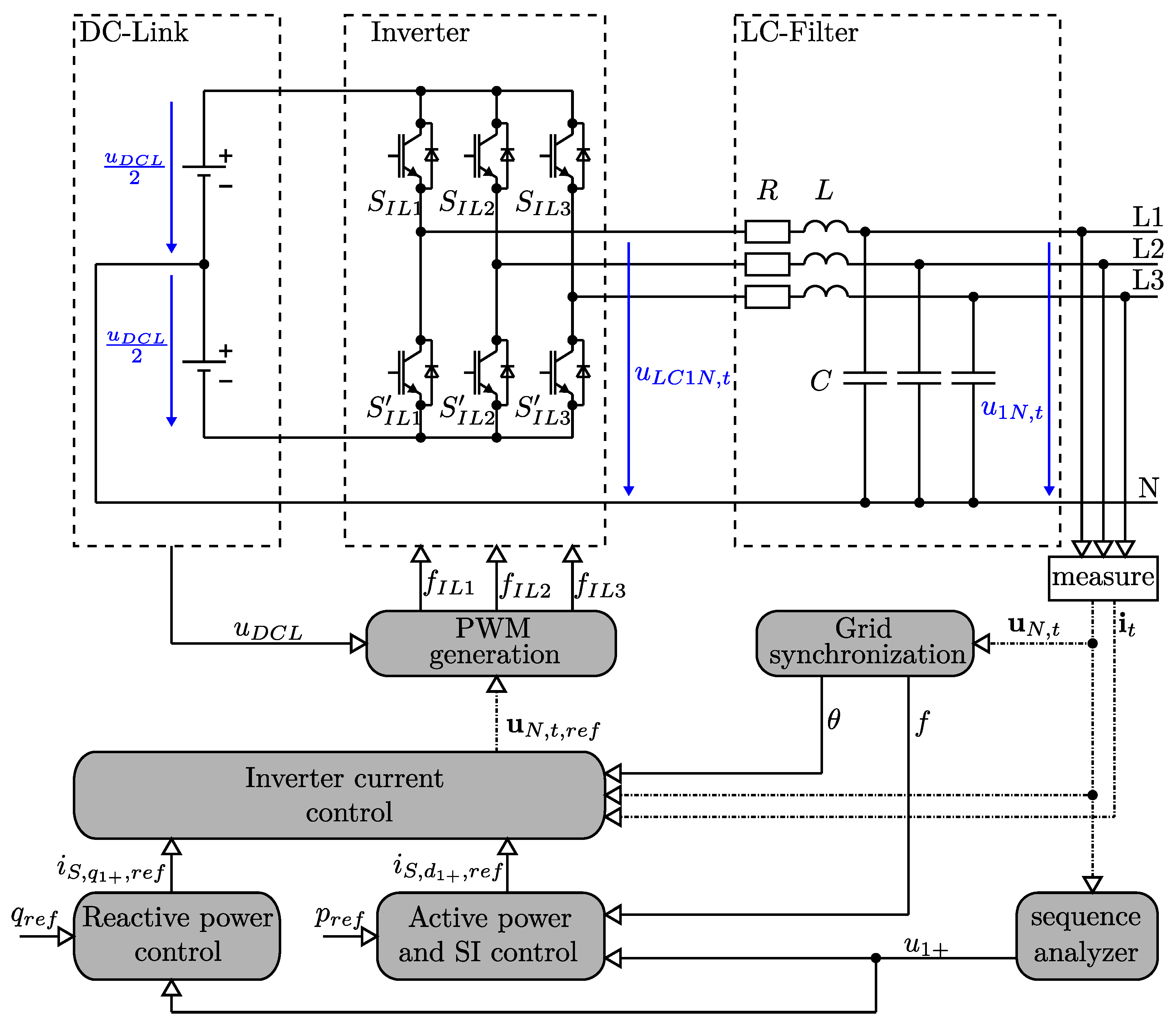
3. Model Description
3.1. Design Considerations for the Output Filter
3.2. Model of a Grid-Forming Converter with Active Synthetic Inertia
3.2.1. Power Calculation
3.2.2. SI Control
3.2.3. Cascaded Voltage- and Current Control
3.2.4. Tuning Considerations
3.2.5. PWM Generation
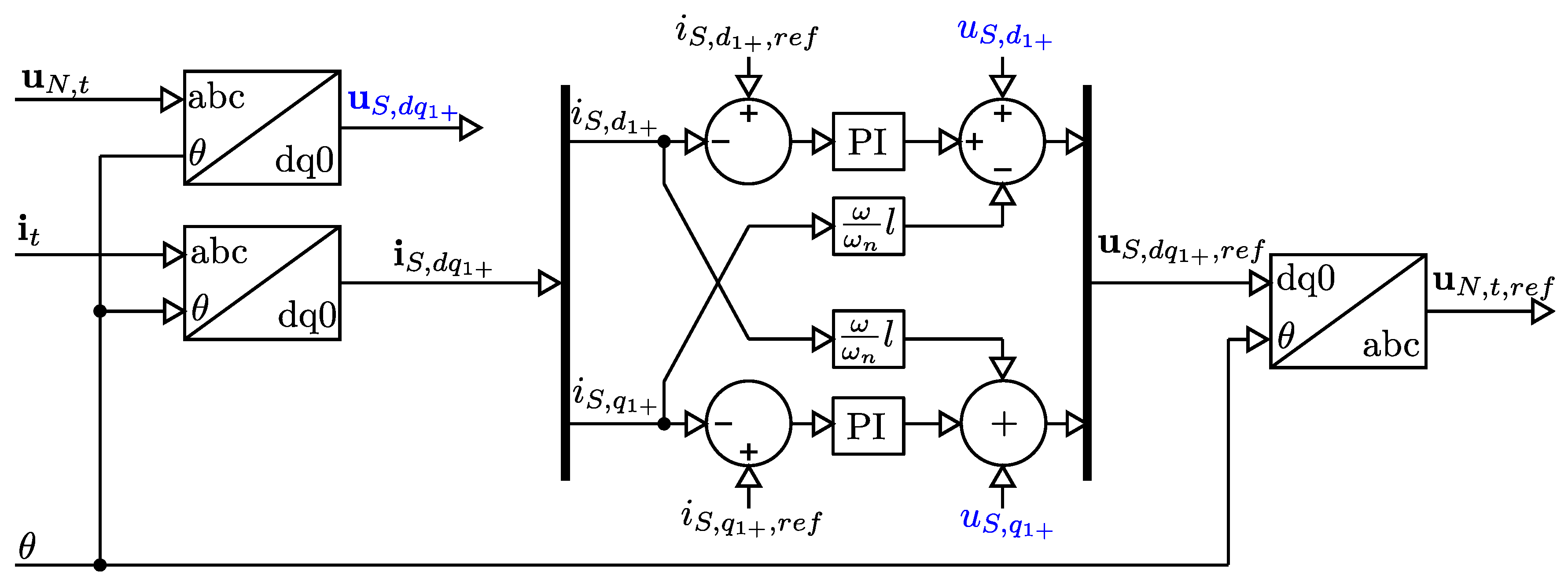
3.3. Model of a Grid-Following Converter with Passive Synthetic Inertia
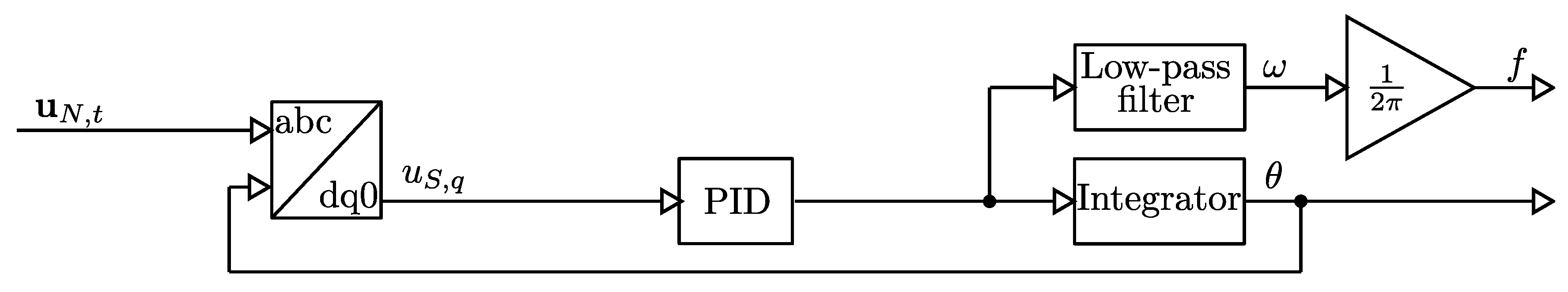
3.3.1. Grid Synchronization and Sequence Analyzer
3.3.2. Active Power and SI Control
3.3.3. Reactive Power Control
3.3.4. Inverter Current Control
3.3.5. Tuning Considerations
4. Simulation
4.1. Simulation Set-Up
4.2. Results
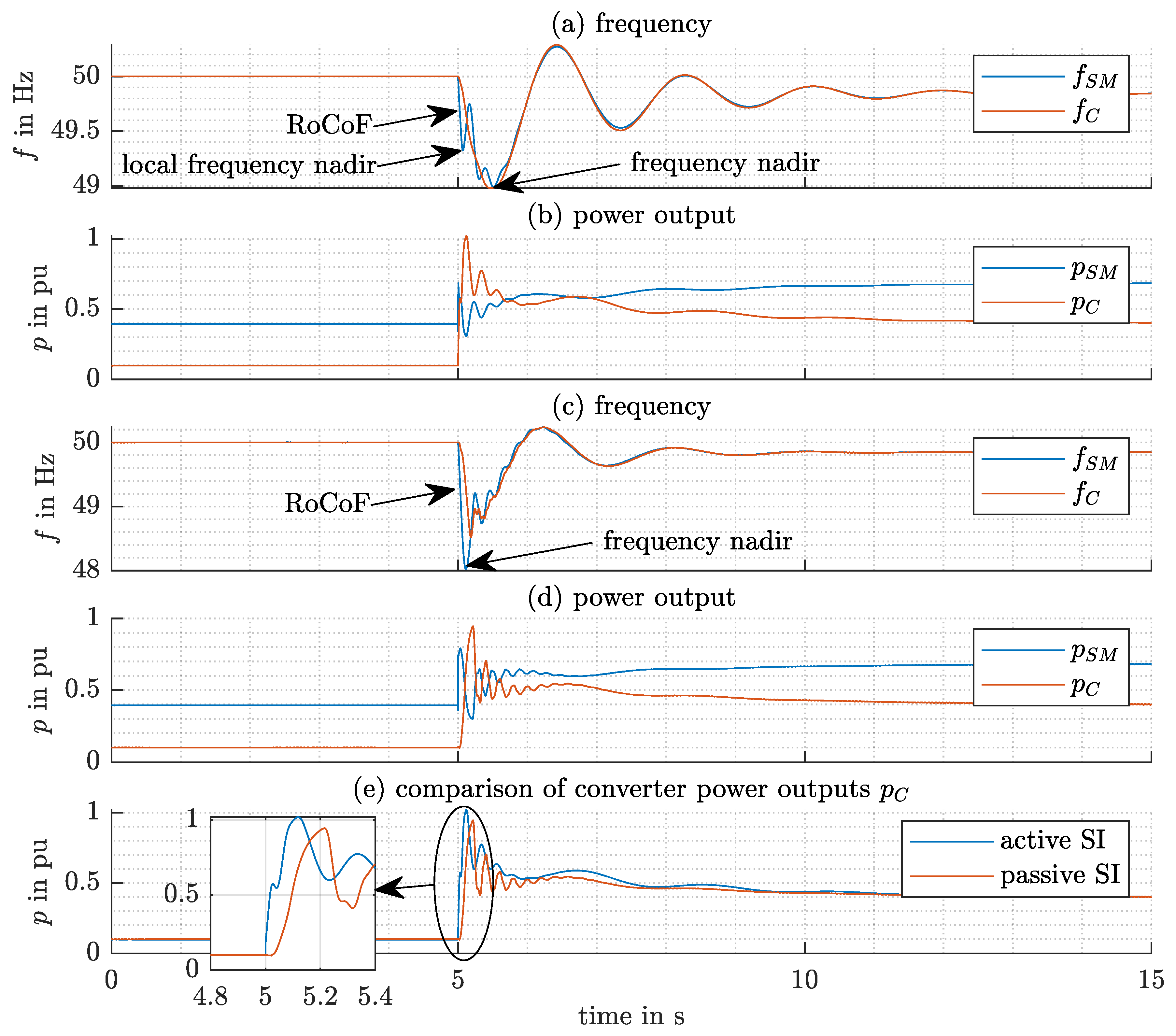
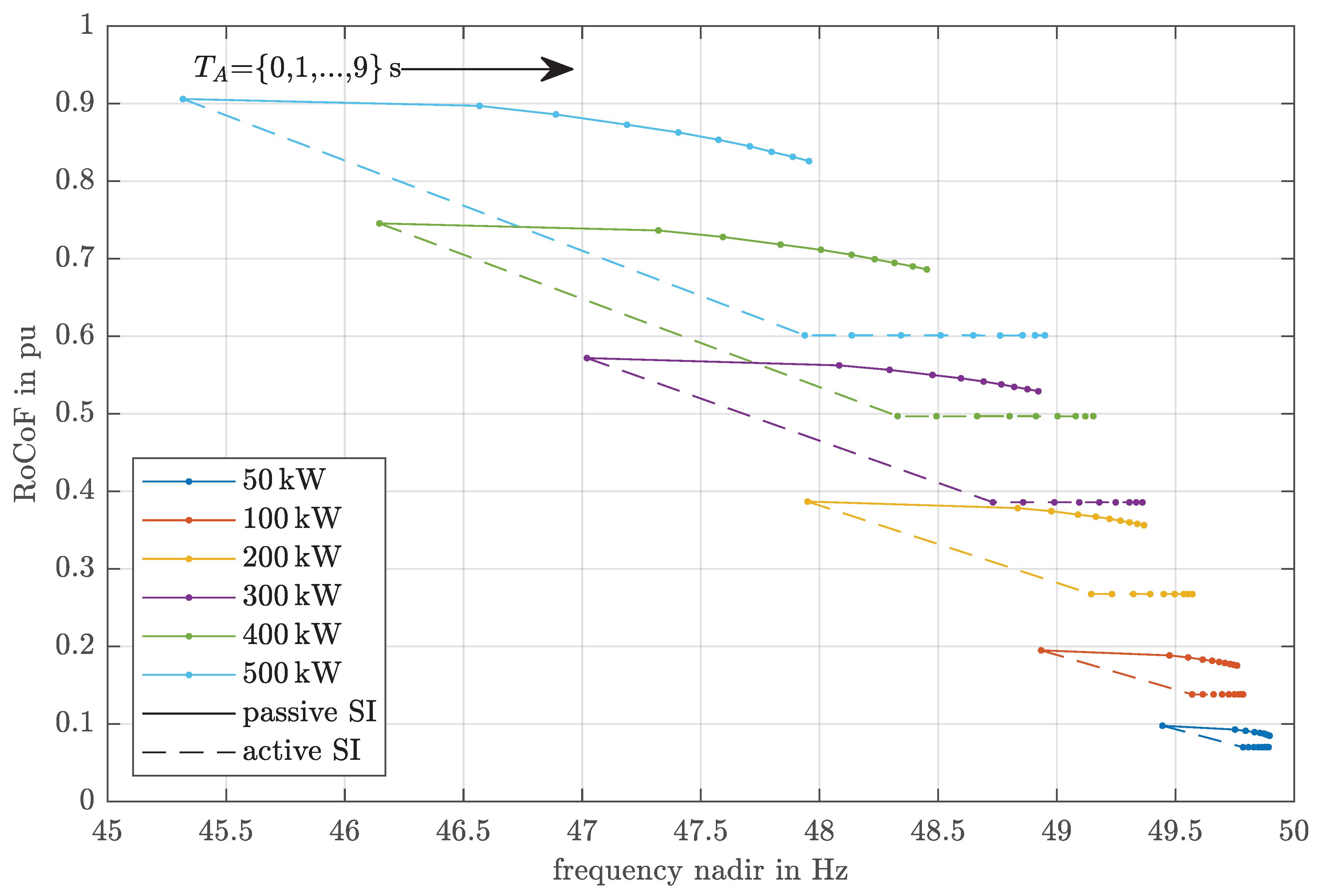
4.2.1. Simulation of a Single Load-Step for Both Models
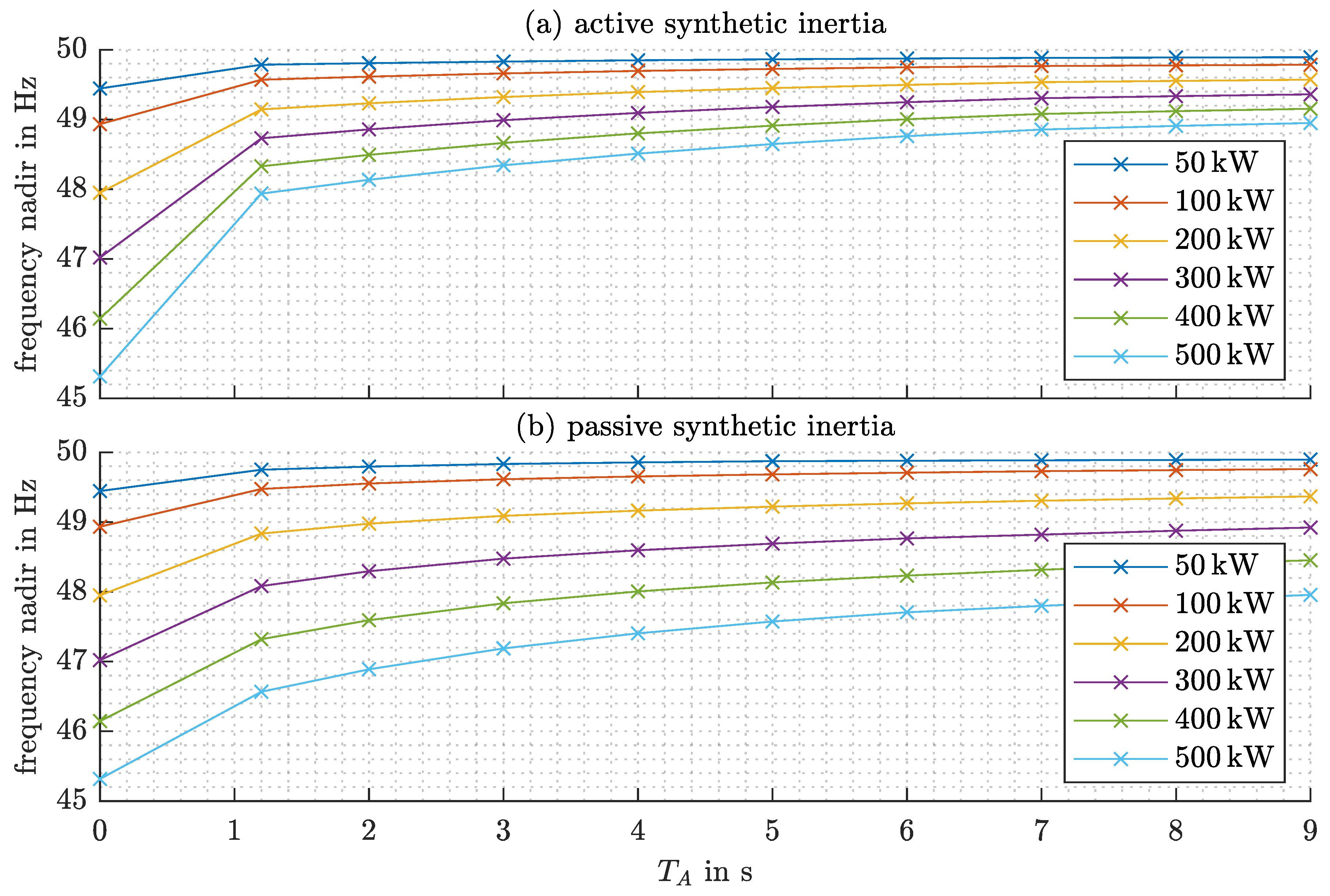
4.2.2. Sensitivity Analysis
4.3. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
List of Symbols
| normalized instantaneous current vector | |
| instantaneous value of the current in phase L1 | |
| instantaneous value of the current in phase L2 | |
| instantaneous value of the current in phase L3 | |
| current space vector in the -plane | |
| current space vector in the dq-plane | |
| reference value of the direct/active, positive-sequence component of the normalized current space vector | |
| reference value of the quadrature/reactive, positive-sequence component of the normalized current space vector | |
| direct/active, positive-sequence component of the normalized current output space vector | |
| quadrature/reactive, positive-sequence component of the normalized current output space vector | |
| quadrature/reactive, positive-sequence component of the normalized current output space vector | |
| phase-to-phase root-mean-square voltage vector | |
| nominal phase-to-phase voltage | |
| normalized phase-to-phase root-mean-square voltage vector | |
| normalized phase-to-phase root-mean-square voltage between L1-L2 | |
| normalized phase-to-phase root-mean-square voltage between L2-L3 | |
| normalized phase-to-phase root-mean-square voltage between L3-L1 | |
| normalized phase-to-neutral instantaneous voltage vector | |
| normalized phase-to-neutral instantaneous reference voltage vector | |
| normalized phase-to-neutral instantaneous voltage in L1 | |
| normalized phase-to-neutral instantaneous voltage in L2 | |
| normalized phase-to-neutral instantaneous voltage in L3 | |
| normalized voltage space vector in the dq-plane | |
| direct/active, positive-sequence component of the normalized voltage output space vector | |
| quadrature/reactive, positive-sequence component of the normalized voltage output space vector | |
| reference value of the direct/active, positive-sequence component of the normalized voltage output space vector | |
| reference value of the quadrature/reactive, positive-sequence component of the normalized voltage output space vector | |
| complex value of the normalized positive-sequence voltage | |
| magnitude of the normalized positive-sequence voltage | |
| angle of the normalized positive-sequence voltage | |
| DC-link voltage | |
| instantaneous phase-to-neutral voltage in L1 at the inverter output | |
| instantaneous phase-to-neutral voltage in L2 at the inverter output | |
| instantaneous phase-to-neutral voltage in L3 at the inverter output | |
| normalized voltage space vector at the inverter output in the dq-plane | |
| mechanical power | |
| P | electrical power |
| p | normalized active power output of the converter |
| q | normalized reactive power output of the converter |
| reference value of the active power | |
| reference value of the reactive power | |
| angular frequency | |
| nominal angular frequency | |
| L | inductance of the LC filter |
| l | normalized inductance of the LC filter |
| R | resistance of the LC filter |
| C | capacitance of the LC filter |
| c | normalized capacitance of the LC filter |
| time constant of the current control loop | |
| proportional controller gain of the current control loop | |
| integral controller gain of the current control loop | |
| proportional controller gain of the voltage control loop | |
| integral controller gain of the voltage control loop | |
| switching frequency of the switching control | |
| maximum current ripple at the converter output | |
| nominal apparent power of the converter | |
| H | inertia constant |
| time constant of generator governor | |
| starting time constant | |
| droop | |
| J | rotation inertia |
| M | electrical torque |
| mechanical torque | |
| phase margin | |
| transfer function of the current controller | |
| process transfer function of the current control loop | |
| loop gain of the current control loop | |
| transfer function of the voltage controller | |
| process transfer function of the voltage control loop | |
| loop gain of the voltage control loop | |
| switching signal for the switch | |
| switching signal for the switch | |
| switching signal for the switch | |
| switching signal for the switch | |
| switching signal for the switch | |
| switching signal for the switch |
References
- Milano, F.; Dörfler, F.; Hug, G.; Hill, D.J.; Verbič, G. Foundations and challenges of low-inertia systems. In Proceedings of the 2018 IEEE Power Systems Computation Conference (PSCC), Dublin, Ireland, 11–15 June 2018; pp. 1–25. [Google Scholar]
- Bevrani, H.; Raisch, J. On virtual inertia application in power grid frequency control. Energy Procedia 2017, 141, 681–688. [Google Scholar] [CrossRef]
- Forkasiewicz, K.; Coldwell, M.; Cross, A.; Strickland, D. Meeting frequency response requirements with uncertain system inertia—A UK perspective. In Proceedings of the 2016 IEEE International Conference on Renewable Energy Research and Applications (ICRERA), Birmingham, UK, 20–23 November 2016; pp. 538–543. [Google Scholar]
- ENTSO-E. Fast Frequency Reserve—Solution to the Nordic Inertia Challenge. Technical Report. 2019. Available online: epressi.com/media/userfiles/107305/1576157646/fast-frequency-reserve-solution-to-the-nordic-inertia-challenge-1.pdf (accessed on 23 June 2020).
- RG-CE System Protection and Dynamics Sub Group. Frequency Stability Evaluation Criteria for the Synchronous Zone of Continental Europe. Technical Report. 2016. Available online: https://eepublicdownloads.blob.core.windows.net/public-cdn-container/clean-documents/SOC%20documents/RGCE_SPD_frequency_stability_criteria_v10.pdf (accessed on 23 June 2020).
- Bevrani, H.; François, B.; Ise, T. Microgrid Dynamics and Control; John Wiley & Sons: Hoboken, NJ, USA, 2017. [Google Scholar]
- Liu, J.; Miura, Y.; Bevrani, H.; Ise, T. Enhanced virtual synchronous generator control for parallel inverters in microgrids. IEEE Trans. Smart Grid 2016, 8, 2268–2277. [Google Scholar] [CrossRef]
- Gawlik, W.; Lechner, A.; Schürhuber, R. Inertia Certificates-Bedeutung und Wert von Momentanreserve für den Verbundnetzbetrieb. In Proceedings of the Vortrag: IEWT Internationale Energiewirtschaftstagung TU Wien, Wien, Austria, 15–17 February 2017. [Google Scholar]
- Poolla, B.K.; Bolognani, S.; Na, L.; Dörfler, F. A market mechanism for virtual inertia. IEEE Trans. Smart Grid 2020, 11, 3570–3579. [Google Scholar] [CrossRef] [Green Version]
- Tamrakar, U.; Shrestha, D.; Maharjan, M.; Bhattarai, B.P.; Hansen, T.M.; Tonkoski, R. Virtual inertia: Current trends and future directions. Appl. Sci. 2017, 7, 654. [Google Scholar] [CrossRef]
- Mehrizi-Sani, A.; Iravani, R. Potential-function based control of a microgrid in islanded and grid-connected modes. IEEE Trans. Power Syst. 2010, 25, 1883–1891. [Google Scholar] [CrossRef]
- Katiraei, F.; Iravani, M.R. Power management strategies for a microgrid with multiple distributed generation units. IEEE Trans. Power Syst. 2006, 21, 1821–1831. [Google Scholar] [CrossRef]
- Tabesh, A.; Iravani, R. Multivariable dynamic model and robust control of a voltage-source converter for power system applications. IEEE Trans. Power Deliv. 2008, 24, 462–471. [Google Scholar] [CrossRef]
- Lin, Y.; Johnson, B.; Gevorgian, V.; Purba, V.; Dhople, S. Stability assessment of a system comprising a single machine and inverter with scalable ratings. In Proceedings of the 2017 North American Power Symposium (NAPS), Morgantown, WV, USA, 17–19 September 2017; pp. 1–6. [Google Scholar]
- Beck, H.P.; Hesse, R. Virtual synchronous machine. In Proceedings of the 2007 9th International Conference on Electrical Power Quality and Utilisation, Barcelona, Spain, 9–11 October 2007; pp. 1–6. [Google Scholar]
- Zhong, Q.C.; Nguyen, P.L.; Ma, Z.; Sheng, W. Self-synchronized synchronverters: Inverters without a dedicated synchronization unit. IEEE Trans. Power Electron. 2013, 29, 617–630. [Google Scholar] [CrossRef]
- Zhong, Q.C.; Hornik, T. Control of Power Inverters in Renewable Energy and Smart Grid Integration; John Wiley & Sons: Hoboken, NJ, USA, 2012; Volume 97. [Google Scholar]
- Bevrani, H.; Ise, T.; Miura, Y. Virtual synchronous generators: A survey and new perspectives. Int. J. Electr. Power Energy Syst. 2014, 54, 244–254. [Google Scholar] [CrossRef]
- D’Arco, S.; Suul, J.A. Virtual synchronous machines—Classification of implementations and analysis of equivalence to droop controllers for microgrids. In Proceedings of the 2013 IEEE Grenoble Conference, Grenoble, France, 16–20 June 2013; pp. 1–7. [Google Scholar]
- Tayyebi, A.; Dörfler, F.; Kupzog, F.; Miletic, Z.; Hribernik, W. Grid-Forming Converters–Inevitability, Control Strategies and Challenges in Future Grids Application. In Proceedings of the CIRED 2018 Ljubljana Workshop on Microgrids and Local Energy Communities, Ljubljana, Slovenia, 7–8 June 2018. [Google Scholar]
- Teodorescu, R.; Liserre, M.; Rodriguez, P. Grid Converters for Photovoltaic and Wind Power Systems; John Wiley & Sons: Hoboken, NJ, USA, 2011; Volume 29. [Google Scholar]
- Tayyebi, A.; Groß, D.; Anta, A.; Kupzog, F.; Dörfler, F. Frequency Stability of Synchronous Machines and Grid-Forming Power Converters. IEEE J. Emerg. Sel. Top. Power Electron. 2020, 8, 1004–1018. [Google Scholar] [CrossRef] [Green Version]
- Emanuel, H.; Brombach, J.; Rosso, R.; Pierros, K. Requirements for control strategies of grid-connected converters in the future power system. IET Renew. Power Gener. 2020, 14, 1288–1295. [Google Scholar] [CrossRef]
- Zhong, Q.C.; Weiss, G. Synchronverters: Inverters that mimic synchronous generators. IEEE Trans. Ind. Electron. 2010, 58, 1259–1267. [Google Scholar] [CrossRef]
- Beres, R.N.; Wang, X.; Liserre, M.; Blaabjerg, F.; Bak, C.L. A review of passive power filters for three-phase grid-connected voltage-source converters. IEEE J. Emerg. Sel. Top. Power Electron. 2015, 4, 54–69. [Google Scholar] [CrossRef] [Green Version]
- Henninger, S. Netzdienliche Integration Regenerativer Energiequellen über Stromrichtergekoppelte Einspeisenetze Mit Integrierten Energiespeichern. Ph.D. Thesis, FAU Erlangen, Erlangen, Germany, 2019. [Google Scholar]
- Yazdani, A.; Iravani, R. Voltage-Sourced Converters in Power Systems: Modeling, Control, and Applications; John Wiley & Sons: Hoboken, NJ, USA, 2010. [Google Scholar]
- The MathWorks, Inc. Documentation; Simulation and Model-Based Design. 2020. Available online: https://www.mathworks.com/products/simulink.html (accessed on 23 June 2020).
- Fischer, M.; Engelken, S.; Mihov, N.; Mendonca, A. Operational experiences with inertial response provided by type 4 wind turbines. IET Renew. Power Gener. 2016, 10, 17–24. [Google Scholar] [CrossRef]
- Miller, N.; Clark, K.; Walling, R. WindINERTIA: Controlled inertial response from GE wind turbine generators. In Proceedings of the 45th Annual Minnesota Power Systems Conference, Minneapolis, MN, USA, 3–5 November 2009. [Google Scholar]
- Wachtel, S.; Beekmann, A. Contribution of wind energy converters with inertia emulation to frequency control and frequency stability in power systems. In Proceedings of the 8th International Workshop on Large-Scale Integration of Wind Power Into Power Systems as Well as on Transmission Networks for Offshore Wind Farms, Bremen, Germany, 14–15 October 2009; Volume 113. [Google Scholar]
- Sterner, M.; Stadler, I. Handbook of Energy Storage: Demand, Technologies, Integration; Springer: Berlin, Germany, 2019. [Google Scholar]
- Wurm, M.; Jonke, P.; Marchgraber, J.; Gawlik, W.; Vitovec, W. Ortsnetz-Inselbetriebsversuch mit einem 2,5-MVA/2,2-MWh-Batteriespeicher: Messergebnisse und Vergleich mit einem Controller Hardware-in-the-loop Setup. e & i Elektrotechnik und Informationstechnik 2019, 136, 368–376. [Google Scholar]
- Eriksson, R.; Modig, N.; Elkington, K. Synthetic inertia versus fast frequency response: A definition. IET Renew. Power Gener. 2017, 12, 507–514. [Google Scholar] [CrossRef]
- Rezkalla, M.; Zecchino, A.; Martinenas, S.; Prostejovsky, A.M.; Marinelli, M. Comparison between synthetic inertia and fast frequency containment control based on single phase EVs in a microgrid. Appl. Energy 2018, 210, 764–775. [Google Scholar] [CrossRef] [Green Version]
- Poolla, B.K.; Groß, D.; Dörfler, F. Placement and implementation of grid-forming and grid-following virtual inertia and fast frequency response. IEEE Trans. Power Syst. 2019, 34, 3035–3046. [Google Scholar] [CrossRef] [Green Version]
- OVE. OVE EN 50160:2011. Voltage Characteristics of Electricity Supplied by Public Electricity Networks. 2016, p. 36. Available online: https://shop.austrian-standards.at/action/de/public/details/383226/OEVE_OENORM_EN_50160_2011_03_01 (accessed on 23 June 2020).
- ENTSO-E. ENTSO-E Regional Groups. Available online: https://www.entsoe.eu/about/system-operations/#regional-groups (accessed on 23 June 2020).


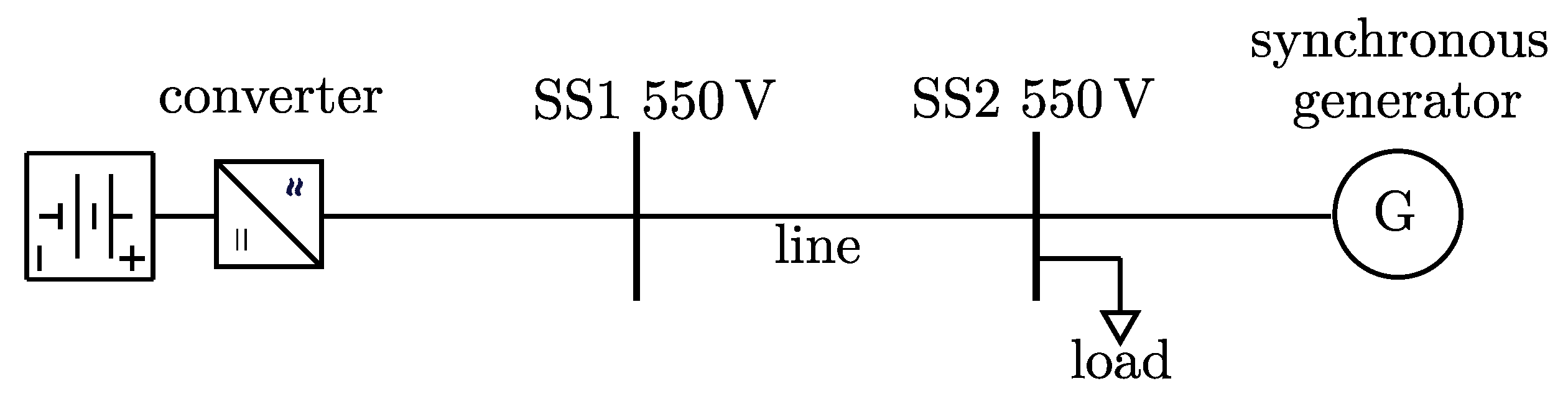
| Converter | Generator | Line | |||
|---|---|---|---|---|---|
| Parameter | Value | Parameter | Value | Parameter | Value |
| 550 | 550 | R | |||
| 650 kVA | 1 MVA | L | |||
| 900 | |||||
| L | 260 | ||||
| R | 1 | ||||
| C | 342 | ||||
| 5 | |||||
| pu | |||||
| 10 pu | |||||
| 60 | |||||
| pu | |||||
| 658 pu | |||||
| pu | pu | ||||
| 10 | |||||
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Marchgraber, J.; Alács, C.; Guo, Y.; Gawlik, W.; Anta, A.; Stimmer, A.; Lenz, M.; Froschauer, M.; Leonhardt, M. Comparison of Control Strategies to Realize Synthetic Inertia in Converters. Energies 2020, 13, 3491. https://doi.org/10.3390/en13133491
Marchgraber J, Alács C, Guo Y, Gawlik W, Anta A, Stimmer A, Lenz M, Froschauer M, Leonhardt M. Comparison of Control Strategies to Realize Synthetic Inertia in Converters. Energies. 2020; 13(13):3491. https://doi.org/10.3390/en13133491
Chicago/Turabian StyleMarchgraber, Jürgen, Christian Alács, Yi Guo, Wolfgang Gawlik, Adolfo Anta, Alexander Stimmer, Martin Lenz, Manuel Froschauer, and Michaela Leonhardt. 2020. "Comparison of Control Strategies to Realize Synthetic Inertia in Converters" Energies 13, no. 13: 3491. https://doi.org/10.3390/en13133491
APA StyleMarchgraber, J., Alács, C., Guo, Y., Gawlik, W., Anta, A., Stimmer, A., Lenz, M., Froschauer, M., & Leonhardt, M. (2020). Comparison of Control Strategies to Realize Synthetic Inertia in Converters. Energies, 13(13), 3491. https://doi.org/10.3390/en13133491





