Solving the Stochastic Generation and Transmission Capacity Planning Problem Applied to Large-Scale Power Systems Using Generalized Shift-Factors
Abstract
:1. Introduction
1.1. Technical Literature Review
1.2. Contributions
2. Introducing the Stochastic Capacity Expansion Planning Formulation
The Proposed Formulation Applied to an Example System (4-Bus)
3. Simulation Results
3.1. Garver Test Power System
3.1.1. Static Deterministic Analysis
3.1.2. Multi-Stage Deterministic and Stochastic Analyses Using an Hourly Load Modeling
3.2. IEEE 300-Bus Test Power System
- For the power unit candidates, we include fifteen new power generation options with a maximum number of = 1.
- For the transmission network candidates, there are thirty investments options where each line has a maximum power flow of 390 MW and the maximum number of new elements is .
3.3. The PEGASE 1354-Bus Test Power System
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Mass, P.; Gibrat, R. Application of linear programming to investment in the electric industry. Manag. Sci. 1957, 3, 149–166. [Google Scholar] [CrossRef]
- Akhavizadegan, F.; Wang, L.; McCalley, J. Scenario selection for iterative stochastic transmission expansion planning. Energies 2020, 13, 1203. [Google Scholar] [CrossRef] [Green Version]
- Quintero, J.; Zhang, H.; Chakhchoukh, Y.; Vittal, V.; Heydt, G. Next generation transmission expansion planning framework: Models, tools, and educational opportunities. IEEE Trans. Power Syst. 2014, 29, 1911–1918. [Google Scholar] [CrossRef]
- Khodaei, A.; Shahidehpour, M. Microgrid-based co-optimization of generation and transmission planning in power systems. IEEE Trans. Power Syst. 2013, 28, 1582–1590. [Google Scholar] [CrossRef]
- Aravena, I.; Cárdenas, R.; Gil, E.; Hinojosa, V.; Araneda, J.C.; Reyes, P. Co-optimization of generation and transmission investment decisions under hydro uncertainty using stochastic mixed-integer programming. In Proceedings of the 10th Latin-American Congress on Electric Power Generation, Transmission and Distribution (CLAGTEE 2013), Vina del Mar, Chile, 6–9 October 2013; pp. 1–8. [Google Scholar]
- Villanasa, R. Transmission Network Planning Using Linear and Mixed Linear Integer Programming. Ph.D. Thesis, Ressenlaer Polythechnic Institute, Troy, NY, USA, 1984. [Google Scholar]
- Moreira, A.; Pozo, D.; Street, A.; Sauma, E. Reliable renewable generation and transmission expansion planning: Co-optimizing system’s resources for meeting renewable targets. IEEE Trans. Power Syst. 2017, 32, 3246–3257. [Google Scholar] [CrossRef]
- Lumbreras, S.; Ramos, A.; Banez-Chicharro, F. Optimal transmission network expansion planning in real-sized power systems with high renewable penetration. Electr. Power Syst. Res. 2017, 149, 76–88. [Google Scholar] [CrossRef]
- Hinojosa, V.H.; Velásquez, J. Improving the mathematical formulation of security-constrained generation capacity expansion planning using power transmission distribution factors and line outage distribution factors. Electr. Power Syst. Res. 2016, 140, 391–400. [Google Scholar] [CrossRef]
- Hinojosa, V.H.; Velásquez, J. Stochastic security-constrained generation expansion planning based on linear distribution factors. Electr. Power Syst. Res. 2016, 140, 139–146. [Google Scholar] [CrossRef]
- Hinojosa, V.H. A generalized stochastic N-m security-constrained generation expansion planning methodology using partial transmission distribution factors. In Proceedings of the 2017 IEEE Power & Energy Society General Meeting, Chicago, IL, USA, 16–20 July 2017. [Google Scholar]
- Hinojosa, V.H. Comparing Corrective and Preventive Security-Constrained DCOPF Problems Using Linear Shift-Factors. Energies 2020, 13, 516. [Google Scholar] [CrossRef] [Green Version]
- Hinojosa, V.H.; Gonzales-Longatt, F. Preventive security-constrained DC-OPF formulation using power transmission distribution factors and line outage distribution factors. Energies 2018, 11, 1497. [Google Scholar] [CrossRef] [Green Version]
- Wood, A.J.; Wollenberg, B.F. Power Generation, Operation and Control; John Wiley & Sons: Hoboken, NJ, USA, 1996. [Google Scholar]
- Rahmani, M.; Kargarian, A.; Hug, G. Comprehensive power transfer distribution factor for large-scale transmission expansion planning. IET Gen. Trans. Dist. 2016, 10, 2981–2989. [Google Scholar] [CrossRef]
- Hinojosa, V.H.; Sepúlveda, J. Solving the stochastic transmission expansion capacity planning methodology based on a generalized partial transmission distribution factors. In Proceedings of the IEEE PowerTech Conference, Milan, Italy, 23–27 June 2019. [Google Scholar]
- Liang, Z.; Chen, H.; Wang, X.; Idris, I.I.; Tan, B.; Zhang, C. An extreme scenario method for robust transmission expansion planning with wind power uncertainty. Energies 2018, 11, 2116. [Google Scholar] [CrossRef] [Green Version]
- Ryan, S.; McCalley, J.; Woodruff, D. Long Term Resource Planning for Electric Power Systems under Uncertainty. 2011. Available online: http://works.bepress.com/sarah_m_ryan/27/ (accessed on 30 June 2020).
- Birge, J.; Louveuax, F. Introduction to Stochastic Programming; ser. Springer Series in Operations Reserach and Financial Engineering; Springer: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- García-Cerezo, A.; Baringo, L.; García-Bertrand, R. Representative days for expansion decisions in power systems. Energies 2020, 13, 335. [Google Scholar] [CrossRef] [Green Version]
- Gurobi Optimization. Available online: https://www.gurobi.com/ (accessed on 1 June 2020).
- Matlab. Available online: https://www.mathworks.com/ (accessed on 1 June 2020).
- Garver, L.L. Transmission network estimation using linear programming. IEEE Trans. Power Apparat. Syst. 1970, PAS-89, 1688–1697. [Google Scholar] [CrossRef]
- Hinojosa, V.H.; Galleguillos, N.; Nuques, B. A simulated rebounding algorithm applied to the multi-stage security-constrained transmission expansion planning in power systems. Int. J. Elect. Power Energy Syst. 2013, 47, 168–180. [Google Scholar] [CrossRef]
- Sepúlveda, J. Planificación de la Capacidad de Generación y Transmission Usando Factores de Sensibilidad Considerando Incertidumbre en las Variables de Entrada, Contingencias y Restricciones de Flexibilidad. Bachelor’s Thesis, Departamento of Electrical Engineering, Universidad Técnica Federico Santa María, Valparaíso, Chile, 2019. (In Spanish). [Google Scholar]
- Zimmerman, R.D.; Murillo-Sanchez, C.E.; Thomas, R.J. MATPOWER: Steady-state operations, planning, and analysus tools for power systems research and education. IEEE Trans. Power Syst. 2011, 26, 12–19. [Google Scholar] [CrossRef] [Green Version]
- Fliscounakis, S.; Panciatici, P.; Capitanescu, F.; Wehenkel, L. Contingency ranking with respect to overloads in very large power systems taking into account uncertainty, preventive and corrective actions. IEEE Trans. Power Syst. 2013, 28, 4909–4917. [Google Scholar] [CrossRef] [Green Version]
- Majidi-Qadikolai, M.; Baldick, R. A generalized decomposition framework for large-scale transmission expansion planning. IEEE Trans. Power Syst. 2018, 33, 1635–1649. [Google Scholar] [CrossRef]
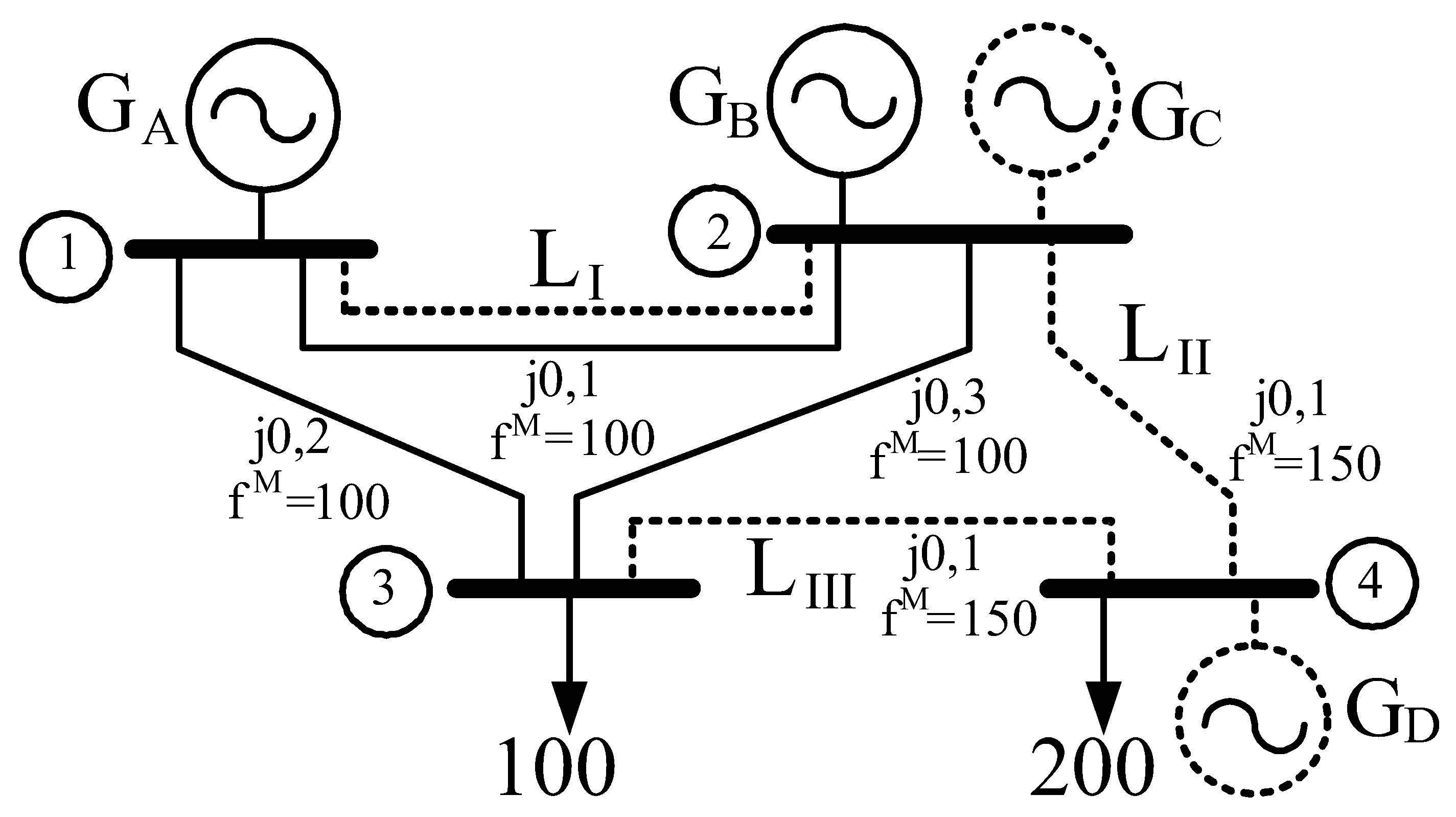



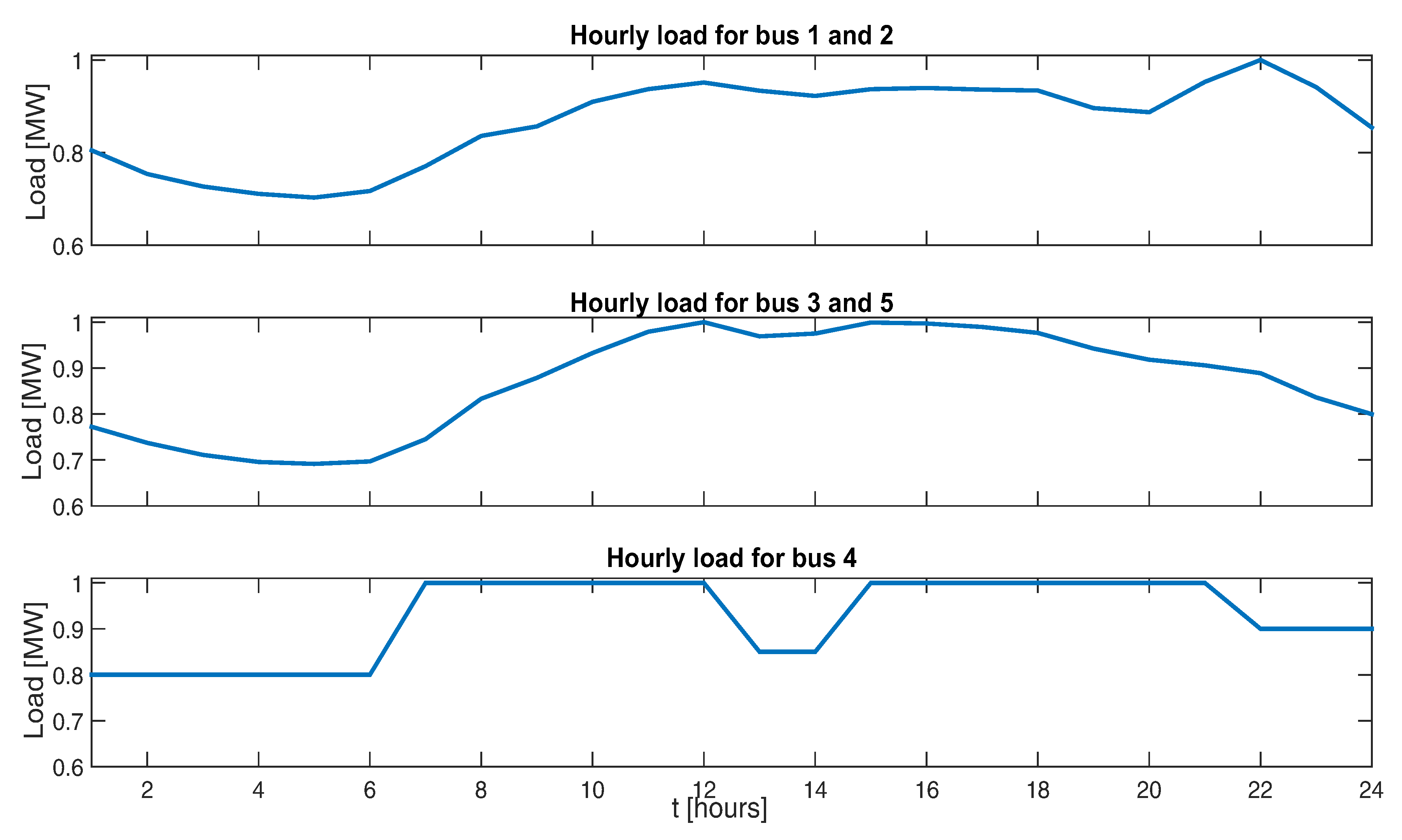
| # | ||||
|---|---|---|---|---|
| (MW) | ($) | ($/MWh) | ||
| 250 | existing unit | 10 | - | |
| 100 | existing unit | 14 | - | |
| 150 | 3,000,000 | 16 | 2 | |
| 110 | 2,500,000 | 8 | 3 |
| # | () | |||
|---|---|---|---|---|
| ($) | ||||
| 1 | 2 | 10,000,000 | 1 | |
| 2 | 4 | 6,000,000 | 2 | |
| 3 | 4 | 5,000,000 | 3 |
| Line | ||||
|---|---|---|---|---|
| 1–2 | 0 | −0.420 | −0.317 | −0.360 |
| 1–3 | 0 | −0.159 | −0.366 | −0.283 |
| 2–4 | 0 | 0.034 | −0.138 | −0.069 |
| 2–4 | 0 | 0.062 | −0.248 | −0.324 |
| 3–4 | 0 | −0.041 | 0.166 | −0.117 |
| # | Bus | ||||
|---|---|---|---|---|---|
| (MW) | ($/MW) | ($/MW) | ($/MWh) | ||
| 1 | 3 × 30 | existing unit | - | 14.08 | |
| 1 | 1 × 60 | existing unit | - | 22.11 | |
| 3 | 2 × 60 | existing unit | - | 25.95 | |
| 3 | 2 × 120 | 300,000 | 9000 | 20.41 | |
| 6 | 1 × 120 | 250,000 | 7500 | 25.95 | |
| 6 | 2 × 240 | 350,000 | 10,500 | 14.08 | |
| – | 2,4,5 | 999 | virtual unit | - |
| Model | v | (s) | |||
|---|---|---|---|---|---|
| GSF | 236 | 123 | 8213 | 475,809,470.91 | 0.030 |
| CD | 269 | 132 | 778 | 475,809,470.91 | 0.021 |
| Model | E | v | (s) | |||
|---|---|---|---|---|---|---|
| GSF | 1 | 56,908 | 16,895 | 1,959,394 | 621,509,628.5 | 979.55 |
| CD | 1 | 55,938 | 18,830 | 187,419 | 621,509,628.5 | 92.39 |
| GSF | 5 | 282,355 | 82,109 | 9,790,325 | 625,989,931.6 | 8793 |
| CD | 5 | 277,545 | 91,790 | 932,616 | 625,989,931.6 | 1043 |
| GSF | 10 | 564,153 | 163,624 | 19,578,388 | 629,964,367.3 | 35,692 |
| CD | 10 | 554,543 | 182,990 | 1,863,994 | 629,964,367.3 | 9801 |
| Model | E | v | (s) | ||
|---|---|---|---|---|---|
| GSF | 1 | 43,470 | 312,900 | 52,024,910,450.4 | 4032 |
| CD | 1 | 214,110 | 627,690 | 52,024,910,450.4 | 87,797 |
| GSF | 5 | 217,350 | 1,567,500 | 257,487,249,145.5 | 272,240 |
| CD | 5 | 1,070,550 | 3,141,450 | - | - |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hinojosa, V.H.; Sepúlveda, J. Solving the Stochastic Generation and Transmission Capacity Planning Problem Applied to Large-Scale Power Systems Using Generalized Shift-Factors. Energies 2020, 13, 3327. https://doi.org/10.3390/en13133327
Hinojosa VH, Sepúlveda J. Solving the Stochastic Generation and Transmission Capacity Planning Problem Applied to Large-Scale Power Systems Using Generalized Shift-Factors. Energies. 2020; 13(13):3327. https://doi.org/10.3390/en13133327
Chicago/Turabian StyleHinojosa, Victor H., and Joaquín Sepúlveda. 2020. "Solving the Stochastic Generation and Transmission Capacity Planning Problem Applied to Large-Scale Power Systems Using Generalized Shift-Factors" Energies 13, no. 13: 3327. https://doi.org/10.3390/en13133327
APA StyleHinojosa, V. H., & Sepúlveda, J. (2020). Solving the Stochastic Generation and Transmission Capacity Planning Problem Applied to Large-Scale Power Systems Using Generalized Shift-Factors. Energies, 13(13), 3327. https://doi.org/10.3390/en13133327





