Controlled Impedance-Admittance-Torque Nonlinear Modeling and Analysis of Modern Power Systems
Abstract
:1. Introduction
2. Power Grid Network Formulation
2.1. Impedance-Admittance (IA) Modeling of a Power Grid
- 1.
- The link impedance representation is
- 2.
- The node or bus admittance representation can be obtained as
- 1.
- matrices and are constant, symmetric, and positive definite,
- 2.
- damping matrices and are constant, symmetric, and at least positive semi-definite,
- 3.
- the skew-symmetric matrices and are linearly dependent from the synchronous angular frequency, , of the rotating system reference frame,
- 4.
- all external uncontrolled inputs of the system are either voltage or current sources and are included in the vector .
2.2. Controlled Impedance-Admittance (CIA) Modeling of Power Grids with Active Grid Components
2.3. Complete Generalized Controlled Impedance-Admittance-Torque (CIAT) Modeling with the Rotating Electromechanical Dynamics Included
- 1.
- the matrix at the left side of Equation (13) is always constructed as a block diagonal matrix with all involved matrices , , and are constant, symmetric positive definite,
- 2.
- the first matrix at the right side of Equation (13) can be always written as a sum of a constant negative definite (or negative semi-definite) damping matrix , and a generally non-constant skew-symmetric matrix ,
- 3.
- the last vector at the right side of Equation (13) is now extended to include all the external mechanical torque inputs that act on the rotating machine masses. It is also noted that in the generalized model, vectors and I may contain controlled components; in this case the vector is split into two distinct vectors, the first to include the controlled parts of and I and the second to include all the external uncontrolled inputs that are considered all bounded (known or unknown tending to constants in steady state).
3. Analysis of System Stability and State Convergence to Equilibrium
3.1. Basic Notations, Preliminaries, and Recent Results for ISS Systems
3.2. Stability and State-Convergence Analysis for CIAT Models
- there exist nondecreasing functions such that, for almost all
- the functions , of Assumption 1.1 are bounded and B is independent of x.
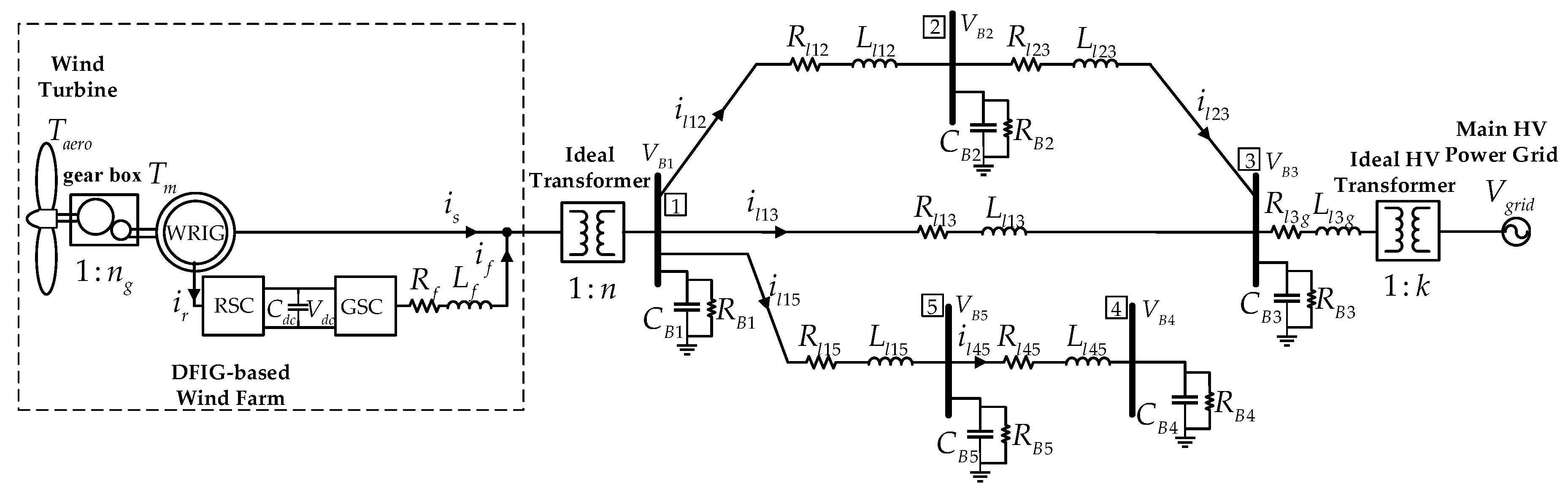
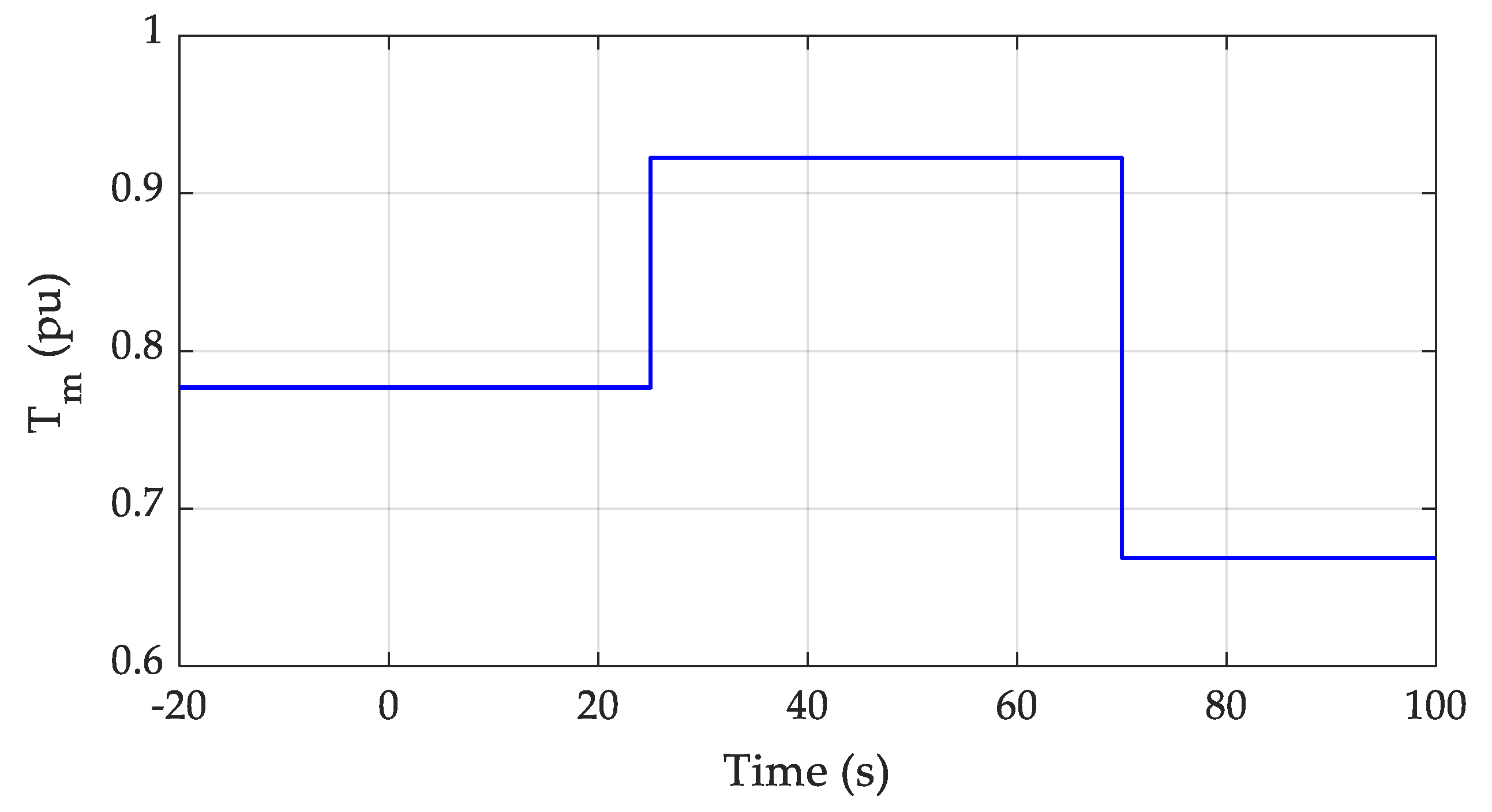
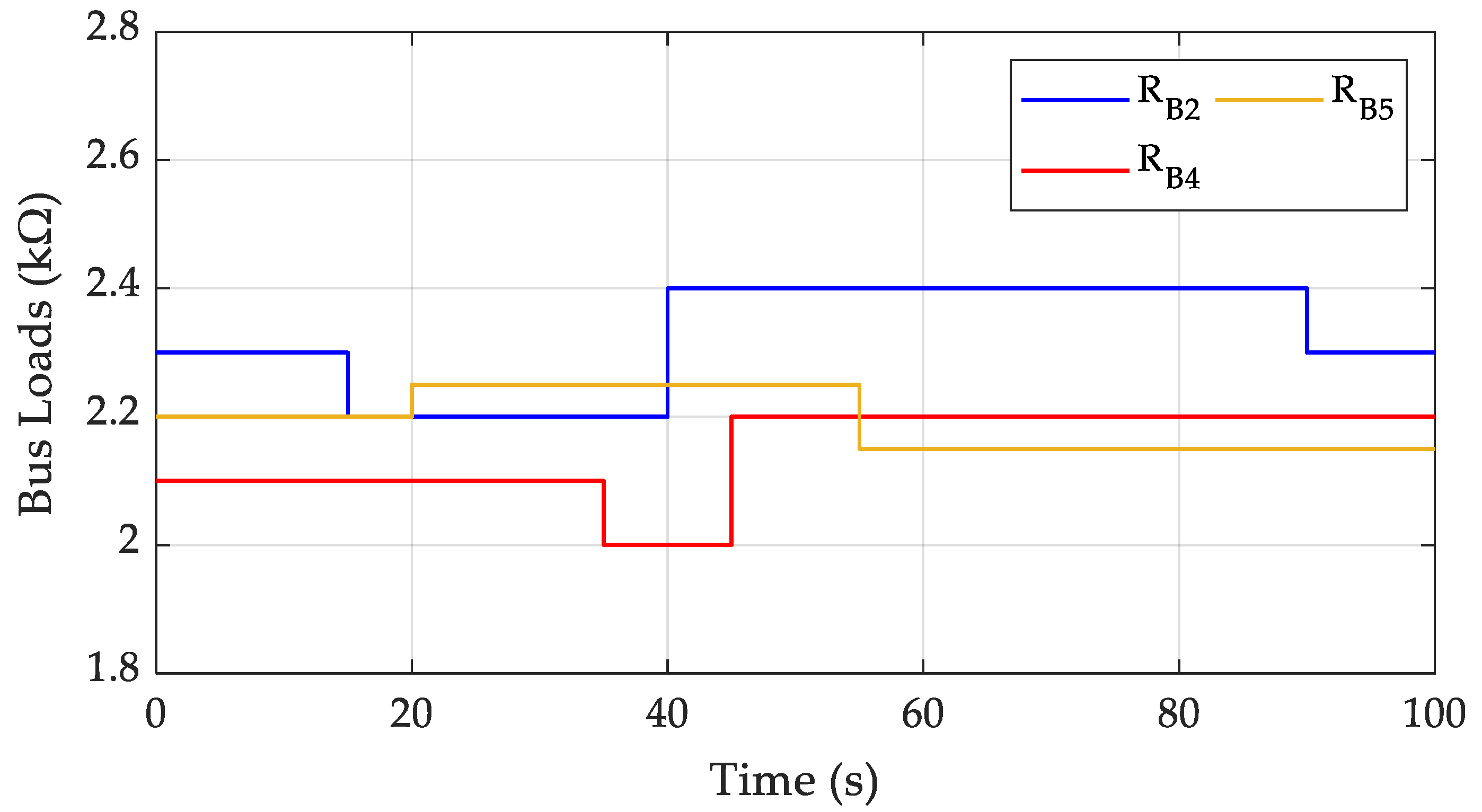
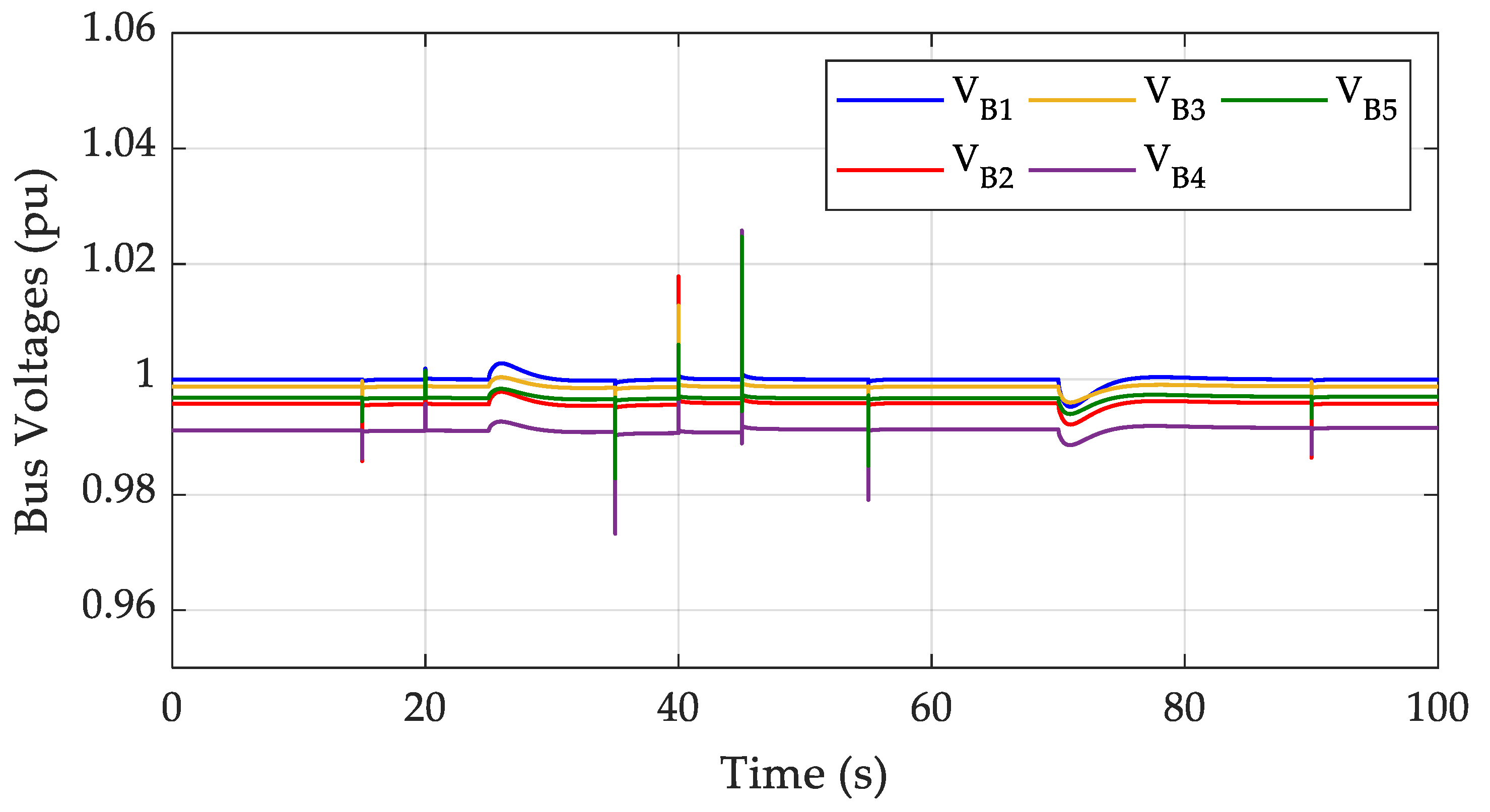
4. Demonstrating the CIAT Modeling and Analysis Results on a DG-Based Power System
Demonstrating the CIAT Model Description for a DG-Based Power System
- For the impedance part of the CIAT formulation
- 2.
- For the admittance part of the CIAT formulation
- 3.
- For the torque part of the CIAT formulation
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| Inductive impedance matrix | |
| Capacitance matrix | |
| Resistance matrix | |
| Conductance matrix | |
| External voltage input vector | |
| External current input vector | |
| Generalized Damping matrices (IA-CIA) | |
| Generalized Inductance matrix (IA-CIA) | |
| Generalized Capacitance matrix (IA-CIA) | |
| Skew-symmetric coupling matrices (IA-CIA) | |
| Power converter duty-ratio controlled input vector | |
| Rotor inertia matrix/total rotor inertia | |
| Mechanical damping matrix | |
| External torque input vector | |
| Block-diagonal damping matrix (CIAT) | |
| Skew-symmetric coupling matrix (CIAT) | |
| Block-diagonal matrix (CIAT)/sub-matrix of | |
| State vector | |
| Input vector | |
| Nominal DFIG power | |
| Nominal stator voltage | |
| Stator resistance | |
| Rotor resistance | |
| Stator inductance | |
| Rotor inductance | |
| Mutual inductance | |
| Rotor angular velocity | |
| Pole pairs | |
| Rotor friction coefficient | |
| Gearbox ratio | |
| Blade radius | |
| GSC-side filter resistance | |
| GSC-side filter inductance | |
| Dc-link capacitance | |
| Ideal transformer ratios | |
| Power grid frequency/angular velocity |
Appendix A
References
- Jiayi, H.; Chuanwen, J.; Rong, X. A review on distributed energy resources and MicroGrid. Renew. Sustain. Energy Rev. 2008, 12, 2472–2483. [Google Scholar] [CrossRef]
- Fang, J.; Li, H.; Tang, Y.; Blaabjerg, F. On the Inertia of Future More-Electronics Power Systems. IEEE J. Emerg. Sel. Top. Power Electron. 2019, 7, 2130–2146. [Google Scholar] [CrossRef]
- Olivares, D.E.; Mehrizi-Sani, A.; Etemadi, A.H.; Canizares, C.A.; Iravani, R.; Kazerani, M. Trends in Microgrid Control. IEEE Trans. Smart Grid 2014, 5, 1905–1919. [Google Scholar] [CrossRef]
- Spanias, C.; Lestas, I. A System Reference Frame Approach for Stability Analysis and Control of Power Grids. IEEE Trans. Power Syst. 2019, 34, 1105–1115. [Google Scholar] [CrossRef]
- Pagani, G.A.; Aiello, M. The Power Grid as a complex network: A survey. Phys. A Stat. Mech. Its Appl. 2013, 392, 2688–2700. [Google Scholar] [CrossRef] [Green Version]
- Faloutsos, M.; Faloutsos, P.; Faloutsos, C. On power-law relationships of the internet topology. In Proceedings of the Conference on Applications, Technologies, Architectures, and Protocols for Computer Communication; ACM: Cambridge, MA, USA, August 1999; p. 262. [Google Scholar]
- Albert, R.; Jeong, H.; Barabási, A.L. Internet: Diameter of the World-Wide Web. Nature 1999, 401, 130–131. [Google Scholar] [CrossRef] [Green Version]
- Boss, M.; Elsinger, H.; Summer, M.; Thurner, S. The network topology of the interbank market. Quant. Financ. 2004, 4, 677–684. [Google Scholar] [CrossRef]
- Yang, C.; Guan, Z.H.; Liu, Z.W.; Chen, J.; Chi, M.; Zheng, G.-L. Wide-area multiple line-outages detection in power complex networks. Electr. Power Energy Syst. 2016, 79, 132–141. [Google Scholar] [CrossRef]
- Arianos, S.; Bompard, E.; Carbone, A.; Xue, F. Power grid vulnerability: A complex network approach. CHAOS 2009, 19, 1–6. [Google Scholar] [CrossRef]
- Papageorgiou, P.C.; Alexandridis, A.T. A suitable to the microgrid analysis approach for nonlinear modeling and control of an inverter interface. In Proceedings of the 25th Mediterranean Conference on Control and Automation (MED-2017), Valletta, Malta, 3–6 July 2017; pp. 522–527. [Google Scholar]
- Fiaz, S.; Zonetti, D.; Ortega, R.; Scherpen, J.M.A.; van der Schaft, A.J. A port-Hamiltonian approach to power network modeling and analysis. Eur. J. Control 2013, 19, 477–485. [Google Scholar] [CrossRef]
- Konstantopoulos, G.C.; Alexandridis, A.T. Generalized nonlinear stabilizing controllers for Hamiltonian passive systems with switching devices. IEEE Trans. Control Syst. Technol. 2013, 21, 1479–1488. [Google Scholar] [CrossRef]
- Kundur, P. Power System Stability and Control; McGraw-Hill: New York, NY, USA, 1994. [Google Scholar]
- Vu, T.L.; Turitsyn, K. Lyapunov functions family approach to transient stability assessment. IEEE Trans. Power Syst. 2016, 31, 1269–1277. [Google Scholar] [CrossRef] [Green Version]
- Slotine, J.-J.E.; Li, W. Applied Nonlinear Control, 1st ed.; Prentice-Hall: Englewood Cliffs, NJ, USA, 1991. [Google Scholar]
- Ortega, R.; Loría Perez, J.A.; Nicklasson, P.J.; Sira-Ramirez, H. Passivity-Based Control of Euler-Lagrange Systems, 1st ed.; Springer: London, UK, 1998. [Google Scholar]
- Watson, J.; Ojo, Y.; Lestas, I.; Spanias, C. Stability of power networks with grid-forming converters. In Proceedings of the 2019 IEEE Milan PowerTech, Milan, Italy, 23–27 June 2019; pp. 1–6. [Google Scholar]
- Ghandhari, M.; Andersson, G.; Hiskens, I.A. Control Lyapunov functions for controllable series devices. IEEE Trans. Power Syst. 2001, 16, 689–694. [Google Scholar] [CrossRef]
- Alexandridis, A.T.; Papageorgiou, P.C. A complex network deployment suitable for modern power distribution analysis at the primary control level. IFAC-PapersOnLine 2017, 50, 9186–9191. [Google Scholar] [CrossRef]
- Alexandridis, A.T. Studying State Convergence of Input-to-State Stable Systems with Applications to Power System Analysis. Energies 2020, 13, 92. [Google Scholar] [CrossRef] [Green Version]
- Berger, T.; Halikias, G.; Karcanias, N. Effects on dynamic and non-dynamic element changes in RC and RL networks. Int. J. Circuit Theory Appl. 2015, 43, 36–59. [Google Scholar] [CrossRef] [Green Version]
- Glover, J.D.; Sarma, M.S.; Overbye, T.T. Power System Analysis & Design, 5th ed.; Cengage Learning, 200 First Stamford Place: Stamford, CT, USA, 2012. [Google Scholar]
- Krause, P.C.; Wasynczuk, O.; Sudhoff, S.D. Analysis of Electric Machinery and Drive Systems, 2nd ed.; Wiley-IEEE Press: Piscataway, NJ, USA, 2002. [Google Scholar]
- Wang, B.; Cai, G.; Yang, D.; Wang, L.; Yu, Z. Investigation on Dynamic Response of Grid-Tied VSC During Electromechanical Oscillations of Power Systems. Energies 2020, 13, 94. [Google Scholar] [CrossRef] [Green Version]
- Krein, P.T.; Bentsman, J.; Bass, R.M.; Lesieutre, B.L. On the Use of Averaging for the Analysis of Power Electronic Systems. IEEE Trans. Power Electron. 1990, 5, 182–190. [Google Scholar] [CrossRef]
- Sira-Ramirez, H.; Silva-Ortigoza, R. Control Design Techniques in Power Electronics Devices; Springer: London, UK, 2006. [Google Scholar]
- Yazdani, A.; Iravani, R. Voltage-Sourced Converters in Power Systems; Wiley Press: Hoboken, NJ, USA, 2010. [Google Scholar]
- Kundur, P.; Paserba, J.; Ajjarapu, V.; Andersson, G.; Bose, A.; Canizares, C.; Hatziargyriou, N.; Hill, D.; Stankovic, A.; Taylor, C.; et al. Definition and classification of power system stability IEEE/CIGRE joint task force on stability terms and definitions. IEEE Trans. Power Syst. 2004, 19, 1387–1401. [Google Scholar]
- Marquez, H.J. Nonlinear Control Systems; Wiley & Sons, Inc.: Hoboken, NJ, USA, 2003. [Google Scholar]
- Sontag, E.D.; Wang, Y. New characterizations of input-to-state stability. IEEE Trans. Autom. Control 1996, 41, 1283–1294. [Google Scholar] [CrossRef] [Green Version]
- Khalil, H.K. Nonlinear Systems, 3rd ed.; Prentice-Hall: Upper Saddle River, NJ, USA, 2002. [Google Scholar]
- Sontag, E.D. Input to state stability: Basic concepts and results. In Nonlinear and Optimal Control Theory. Lecture Notes in Mathematics, 1st ed.; Nistri, P., Stefani, G., Eds.; Springer: Berlin, Germany, 2008; Volume 1932, pp. 163–220. [Google Scholar]
- Konstantopoulos, G.C.; Alexandridis, A.T. Stability and convergence analysis for a class of nonlinear passive systems. In Proceedings of the 50th Conference on Decision and Control and European. Control Conference (CDC-ECC), Orlando, FL, USA, 12–15 December 2011; pp. 1753–1758. [Google Scholar]
- Panteley, E.; Loria, A.; Teel, A. Relaxed persistency of excitation for uniform asymptotic stability. IEEE Trans. Autom. Control 2001, 46, 1874–1886. [Google Scholar] [CrossRef]
- Loria, A.; Panteley, E.; Teel, A. A new persistency of excitation condition for UGAS of NLTV systems: Application to stabilization of nonholonomic systems. In Proceedings of the 5th European Control Conference, Karlsruhe, Germany, 31 August–3 September 1991. Paper No. 500. [Google Scholar]
- Bourdoulis, M.K.; Alexandridis, A.T. Direct Power Control of DFIG Wind Systems Based on Nonlinear Modeling and Analysis. IEEE J. Emerg. Sel. Top. Power Electron. 2014, 2, 764–775. [Google Scholar] [CrossRef]




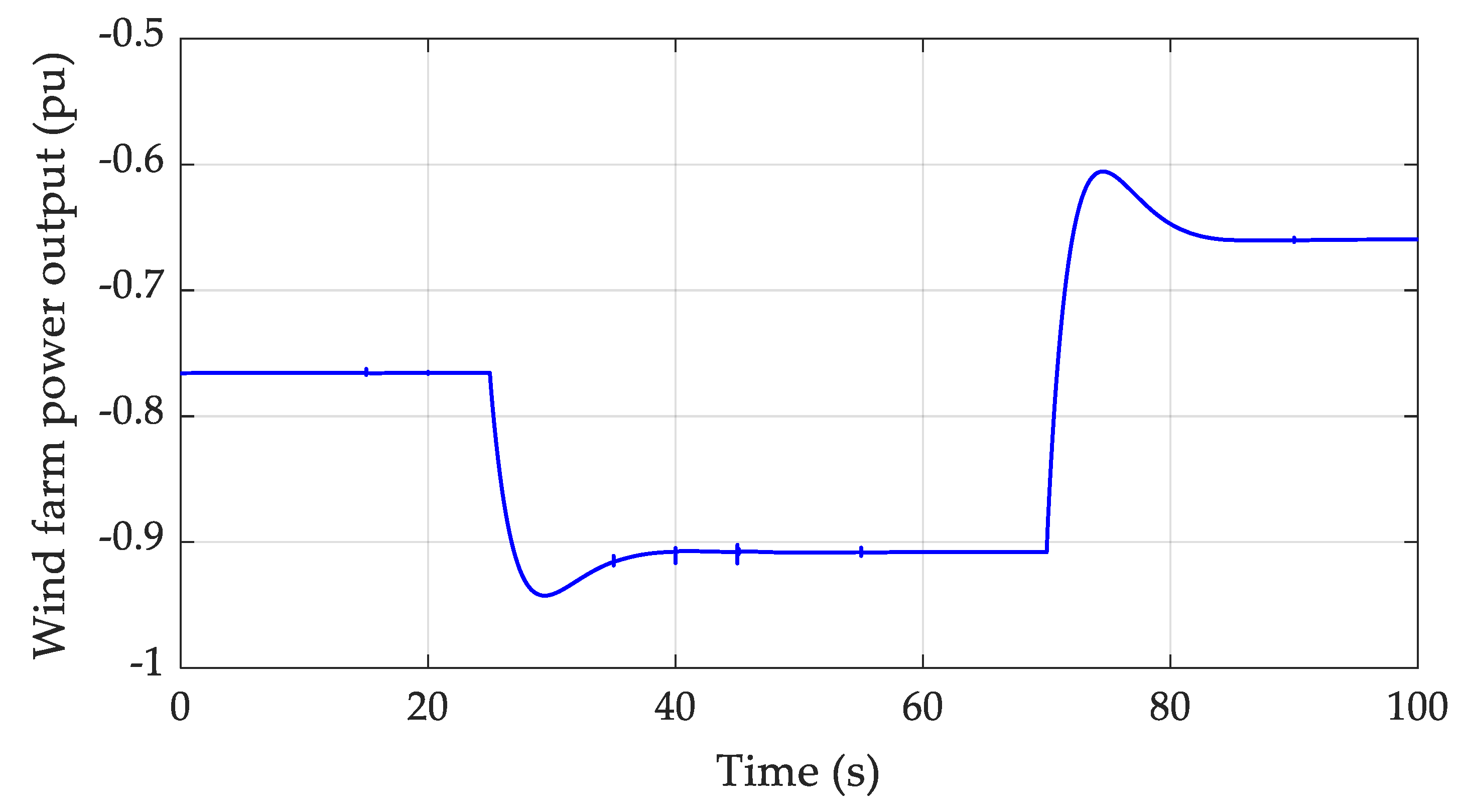
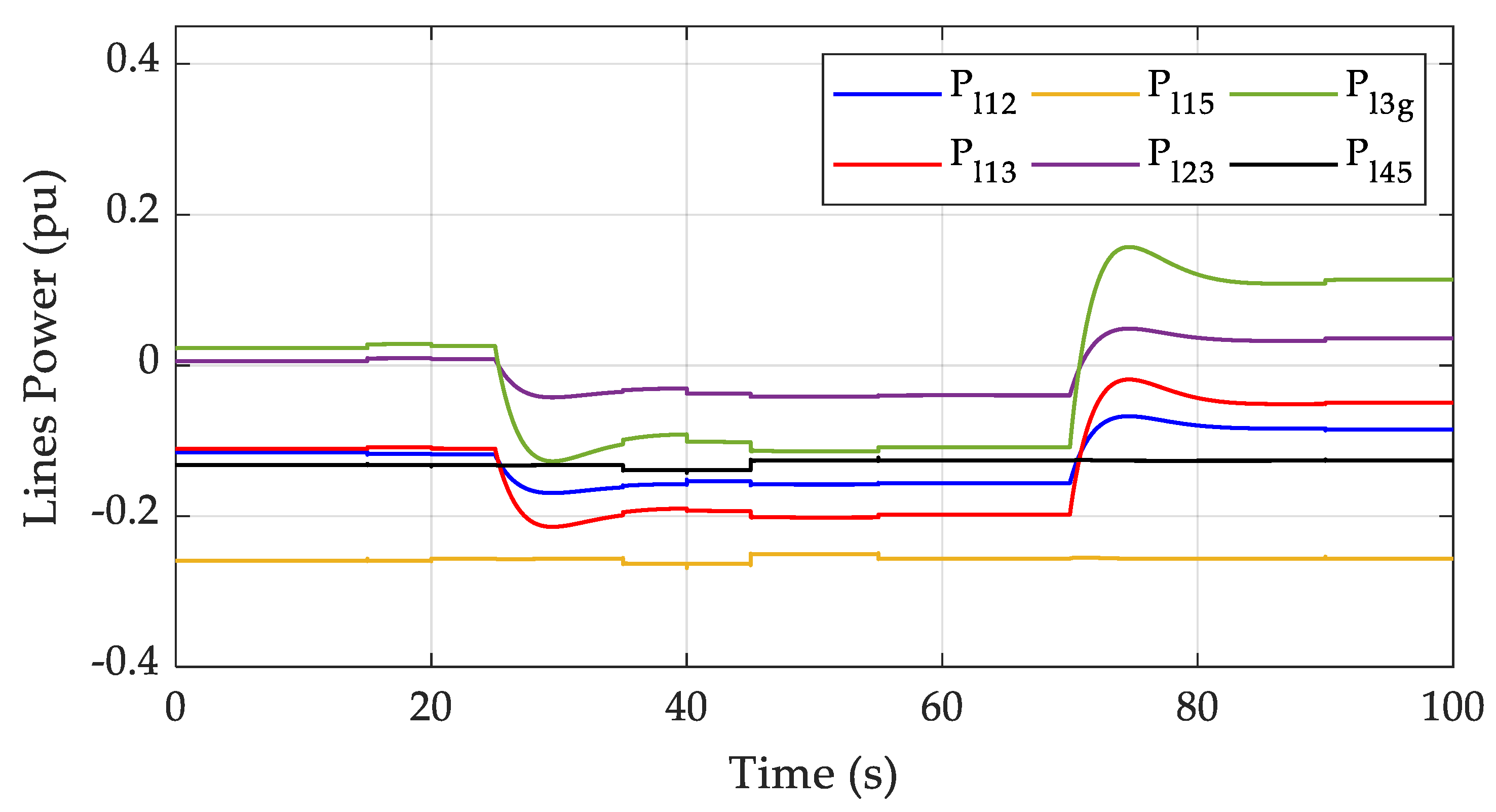
| Parameters/Values | ||
|---|---|---|
| Parameters/Values | |
|---|---|
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
C. Papageorgiou, P.; Alexandridis, A.T. Controlled Impedance-Admittance-Torque Nonlinear Modeling and Analysis of Modern Power Systems. Energies 2020, 13, 2461. https://doi.org/10.3390/en13102461
C. Papageorgiou P, Alexandridis AT. Controlled Impedance-Admittance-Torque Nonlinear Modeling and Analysis of Modern Power Systems. Energies. 2020; 13(10):2461. https://doi.org/10.3390/en13102461
Chicago/Turabian StyleC. Papageorgiou, Panos, and Antonio T. Alexandridis. 2020. "Controlled Impedance-Admittance-Torque Nonlinear Modeling and Analysis of Modern Power Systems" Energies 13, no. 10: 2461. https://doi.org/10.3390/en13102461
APA StyleC. Papageorgiou, P., & Alexandridis, A. T. (2020). Controlled Impedance-Admittance-Torque Nonlinear Modeling and Analysis of Modern Power Systems. Energies, 13(10), 2461. https://doi.org/10.3390/en13102461






