Effect of Viscous Dissipation in Heat Transfer of MHD Flow of Micropolar Fluid Partial Slip Conditions: Dual Solutions and Stability Analysis
Abstract
:1. Introduction
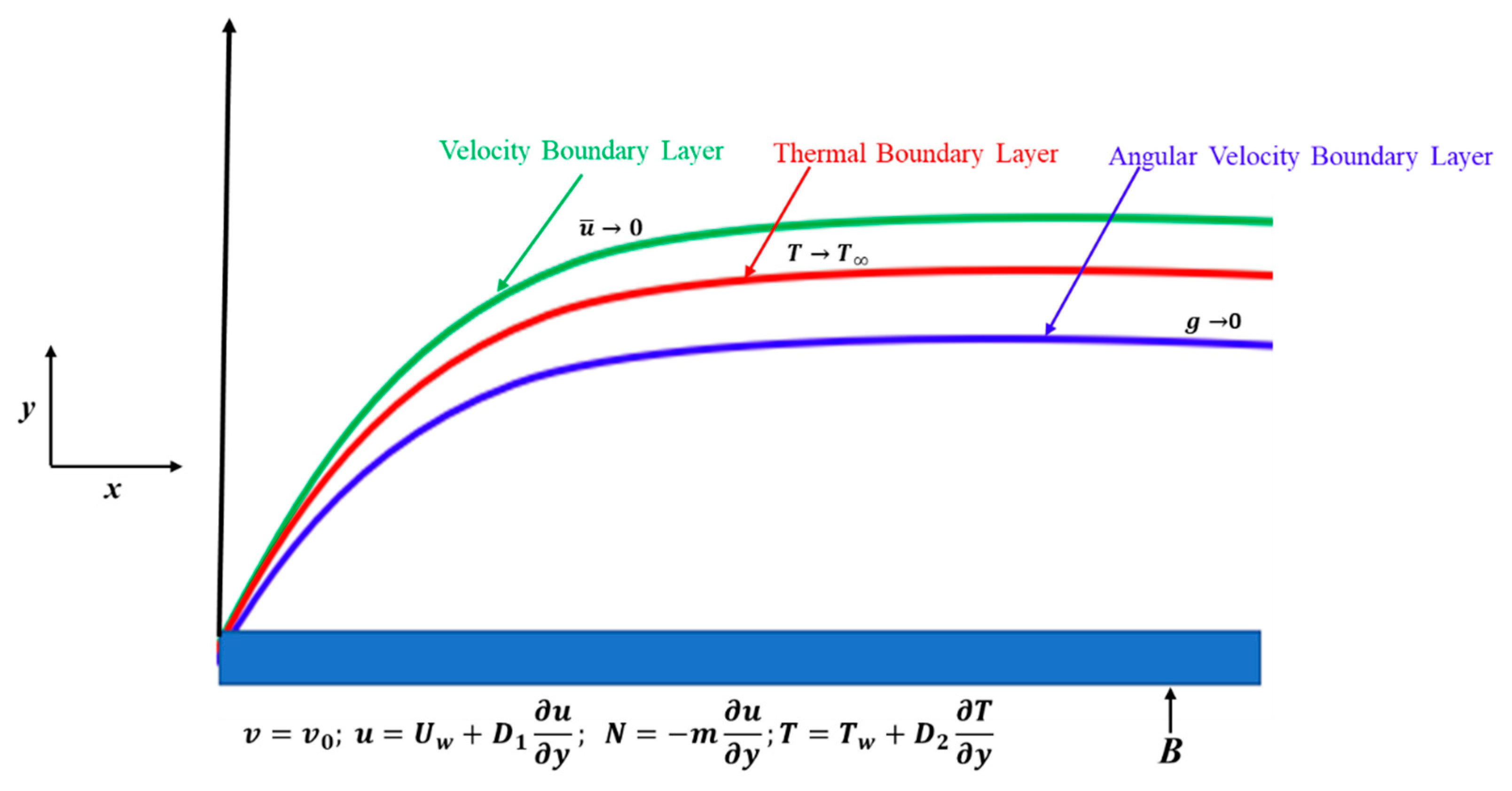
2. Problem Formulation
3. Stability Analysis
4. Numerical Method
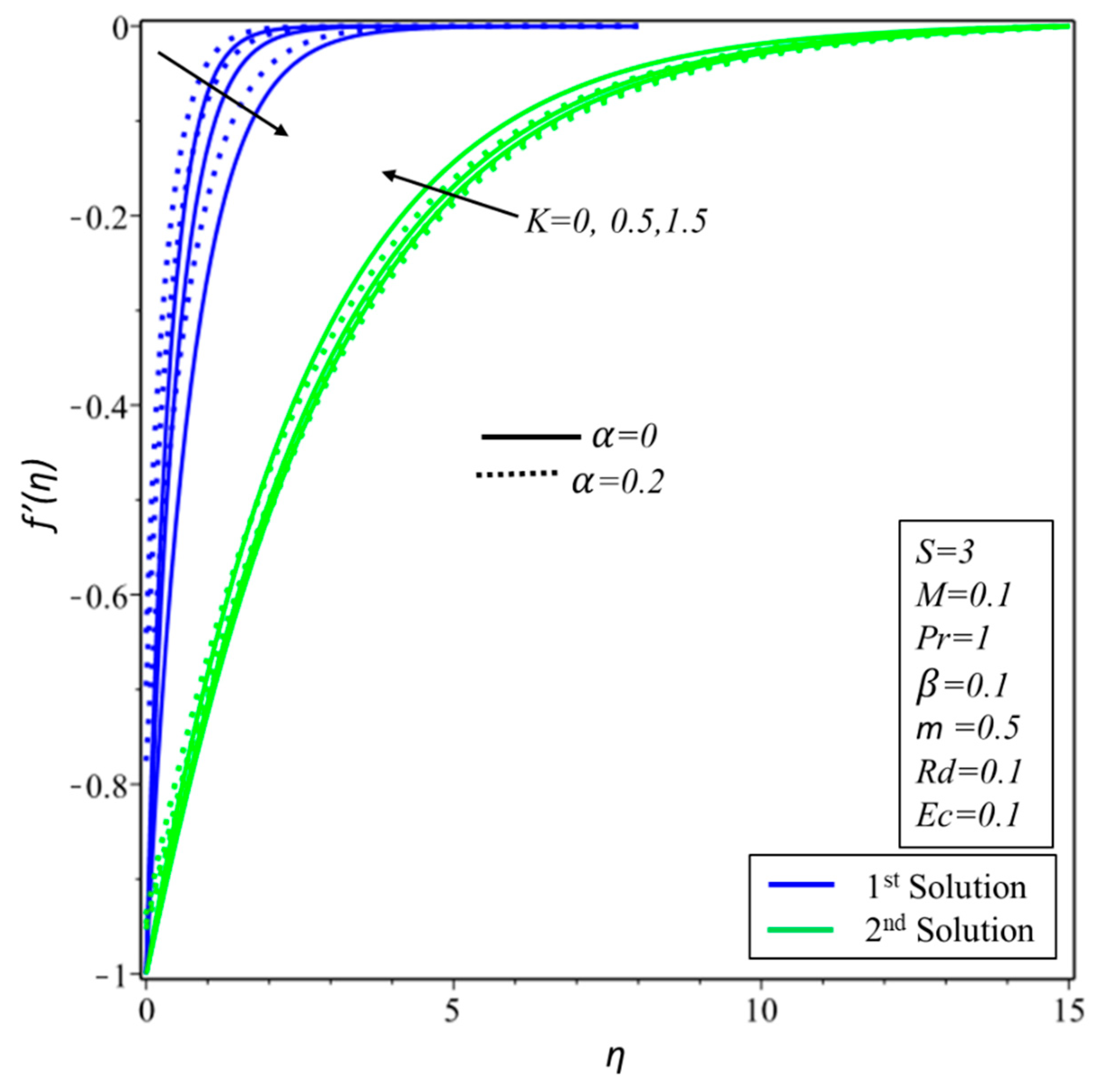
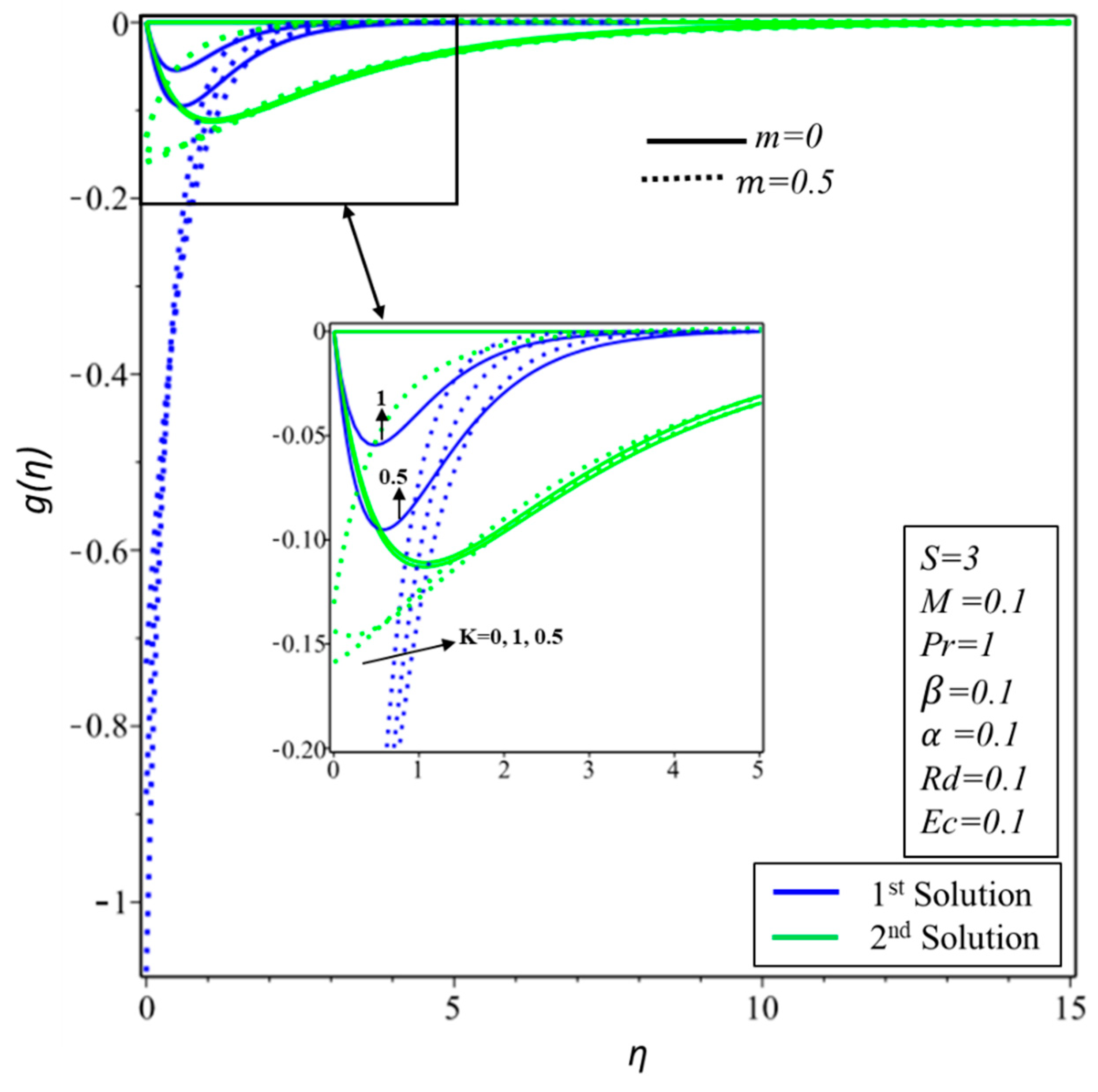
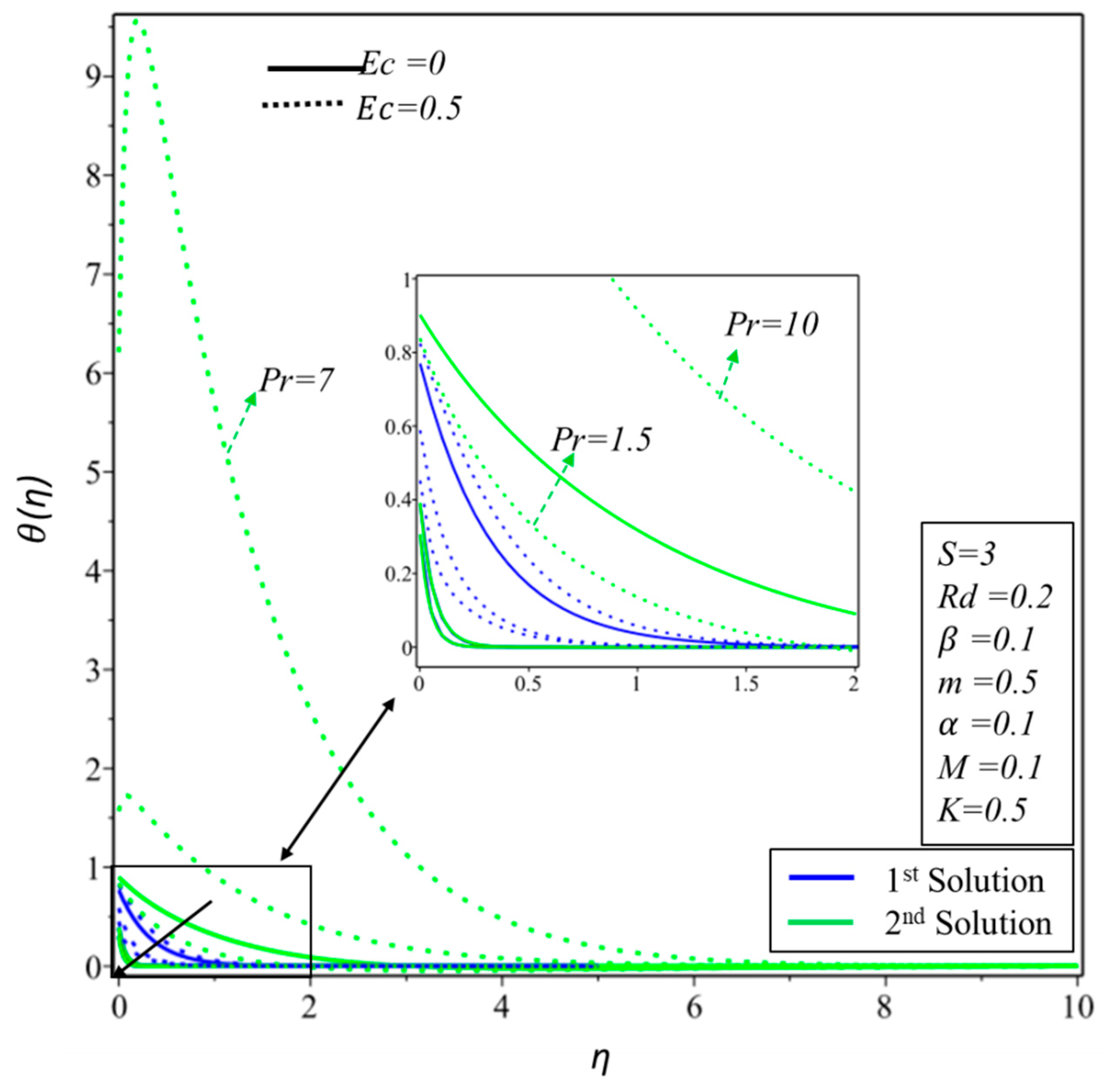
5. Results and Discussion
6. Conclusions
- (1)
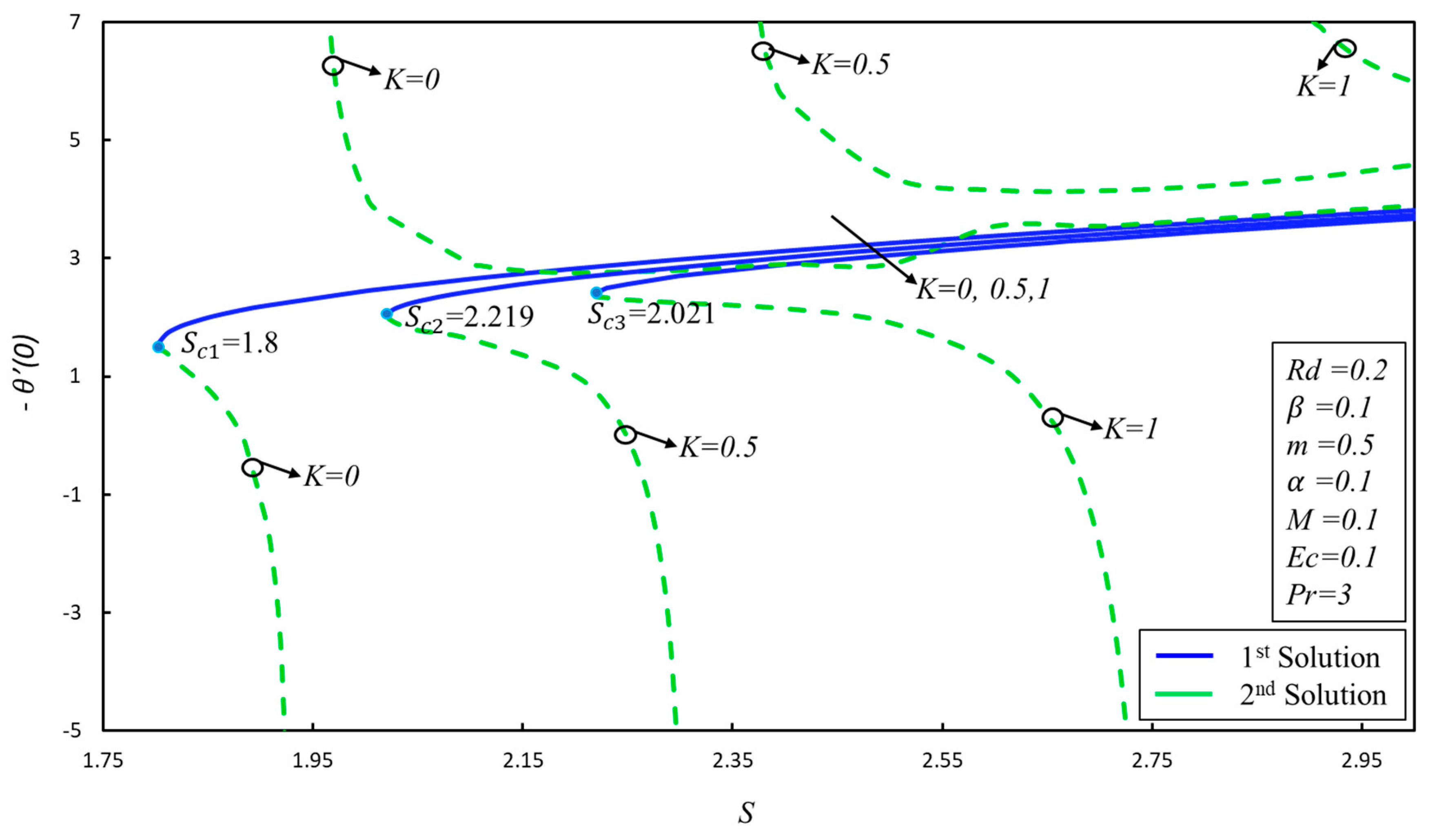
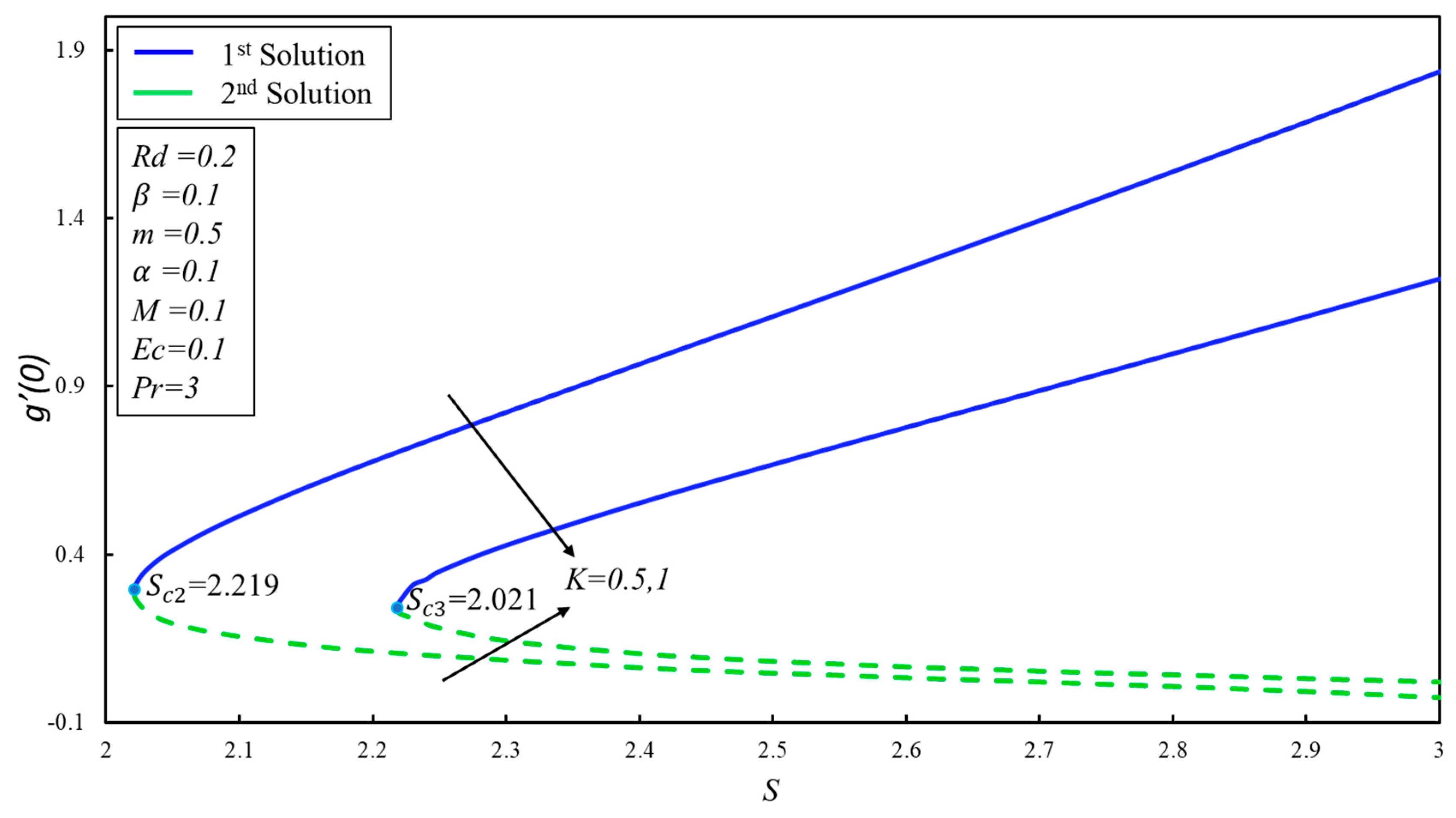
- Dual solutions exist over the permeable shrinking sheet and the critical values of suction parameter depends on material parameter .
- (2)
- Velocity field and the thickness of hydrodynamic boundary layer increase (decrease) in the first solution and decrease (increases) in the second solution when is increased.
- (3)
- Both solutions increase (decrease) for the strong effect of and ().
- (4)
- The material parameter and mass suction parameter are inversely proportional to each other’s.
- (5)
- The results of numerical solutions are closed to the exact solutions.
- (6)
- The results of the smallest eigenvalues show that second solution is not stable as compared to the first solution.
- (7)
- The realizable flow situation is only possible for the first solution.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| a constant | |
| ambient temperature | |
| angular velocity | |
| current density | |
| dissipation function | |
| dynamic viscosity | |
| Eckert number | |
| electrical conductivity | |
| gradient operator | |
| gyration parameter | |
| Hartmann number | |
| Laplacian operator | |
| local Nusselt number | |
| local Reynolds number | |
| Lorentz force | |
| Magnetic field vector | |
| mass transfer velocity | |
| mean absorption coefficient, | |
| Positive constant | |
| Prandtl number | |
| pressure | |
| Rosseland approximation | |
| skin friction coefficient | |
| slip factors of temperature | |
| slip factors of velocity | |
| smallest eigen value | |
| spin gradient viscosity | |
| Stability transformed variable | |
| Stefan-Boltzmann constant | |
| T | Temperature |
| thermal radiation | |
| thermal slip parameter | |
| time | |
| transformed variable | |
| variable temperature of surface | |
| u, v | velocity components |
| Velocity of shrinking surface | |
| velocity slip parameter | |
| Velocity vector | |
| vortex viscosity |
References
- Dero, S.; Rohni, A.M.; Saaban, A. MHD micropolar nanofluid flow over an exponentially stretching/shrinking surface: Triple solutions. J. Adv. Res. Fluid Mech. Therm. Sci 2019, 56, 165–174. [Google Scholar]
- Hayat, T.; Khan, M.I.; Waqas, M.; Alsaedi, A.; Khan, M.I. Radiative flow of micropolar nanofluid accounting thermophoresis and Brownian moment. Int. J. Hydrog. Energy 2017, 42, 16821–16833. [Google Scholar] [CrossRef]
- Zubair, M.; Shah, Z.; Dawar, A.; Islam, S.; Kumam, P.; Khan, A. Entropy generation optimization in squeezing magnetohydrodynamics flow of casson nanofluid with viscous dissipation and joule heating effect. Entropy 2019, 21, 747. [Google Scholar] [CrossRef] [Green Version]
- Qing, J.; Bhatti, M.; Abbas, M.; Rashidi, M.; Ali, M. Entropy generation on MHD Casson nanofluid flow over a porous stretching/shrinking surface. Entropy 2016, 18, 123. [Google Scholar] [CrossRef]
- Aurangzaib Bhattacharyya, K.; Shafie, S. Effect of partial slip on an unsteady MHD mixed convection stagnation-point flow of a micropolar fluid towards a permeable shrinking sheet. Alex. Eng. J. 2016, 55, 1285–1293. [Google Scholar] [CrossRef] [Green Version]
- OAlzahrani, E.; Shah, Z.; Alghamdi, W.; Zaka Ullah, M. Darcy–Forchheimer Radiative Flow of Micropoler CNT Nanofluid in Rotating Frame with Convective Heat Generation/Consumption. Processes 2019, 7, 666. [Google Scholar] [CrossRef] [Green Version]
- Omori, T.; Ishikawa, T. Swimming of Spermatozoa in a Maxwell Fluid. Micromachines 2019, 10, 78. [Google Scholar] [CrossRef] [Green Version]
- Zafar, M.; Ahmad, B.; Rana, M.A.; Zahid, M. Mathematical Analysis of the Coating Process Over a Porous Web Lubricated with Upper Convected Maxwell Fluid. Coatings 2019, 9, 458. [Google Scholar] [CrossRef] [Green Version]
- Guo, X.; Qi, H. Analytical solution of electro-osmotic peristalsis of fractional Jeffreys fluid in a micro-channel. Micromachines 2017, 8, 341. [Google Scholar] [CrossRef] [Green Version]
- Hayat, T.; Qayyum, S.; Imtiaz, M.; Alsaedi, A. Impact of Cattaneo-Christov heat flux in Jeffrey fluid flow with homogeneous-heterogeneous reactions. PLoS ONE 2016, 11, e0148662. [Google Scholar] [CrossRef]
- Khan, W.A.; Irfan, M.; Khan, M.; Alshomrani, A.S.; Alzahrani, A.K.; Alghamdi, M.S. Impact of chemical processes on magneto nanoparticle for the generalized Burgers fluid. J. Mol. Liq. 2017, 234, 201–208. [Google Scholar] [CrossRef]
- Maleki, H.; Safaei, M.R.; Togun, H.; Dahari, M. Heat transfer and fluid flow of pseudo-plastic nanofluid over a moving permeable plate with viscous dissipation and heat absorption/generation. J. Therm. Anal. Calorim. 2019, 135, 1643–1654. [Google Scholar] [CrossRef]
- Eringen, A.C. Theory of micropolar fluids. J. Math. Mech. 1966, 16, 1–18. [Google Scholar] [CrossRef]
- Bhattacharjee, B.; Chakraborti, P.; Choudhuri, K. Evaluation of the performance characteristics of double-layered porous micropolar fluid lubricated journal bearing. Tribol. Int. 2019. [Google Scholar] [CrossRef]
- Koriko, O.K.; Animasaun, I.L.; Omowaye, A.J.; Oreyeni, T. The combined influence of nonlinear thermal radiation and thermal stratification on the dynamics of micropolar fluid along a vertical surface. Multidiscip. Modeling Mater. Struct. 2019, 15, 133–155. [Google Scholar] [CrossRef]
- Lakshmi, R.V.; Sarojamma, G.; Sreelakshmi, K.; Vajravelu, K. Heat Transfer Analysis in a Micropolar Fluid with Non-Linear Thermal Radiation and Second-Order Velocity Slip. In Applied Mathematics and Scientific Computing; Birkhäuser: Cham, Switzerland, 2019; pp. 385–395. [Google Scholar]
- Mozaffari, M.; D’Orazio, A.; Karimipour, A.; Abdollahi, A.; Safaei, M.R. Lattice Boltzmann method to simulate convection heat transfer in a microchannel under heat flux: Gravity and inclination angle on slip-velocity. Int. J. Numer. Methods Heat Fluid Flow 2019. [Google Scholar] [CrossRef]
- Khan, A.A.; Batool, R.; Kousar, N. MHD Micropolar Fluid over Curved Stretching Surface with modified Fourier law. Sci. Iran. 2019. [Google Scholar] [CrossRef] [Green Version]
- Maleki, H.; Safaei, M.R.; Alrashed, A.A.; Kasaeian, A. Flow and heat transfer in non-Newtonian nanofluids over porous surfaces. J. Therm. Anal. Calorim. 2019, 135, 1655–1666. [Google Scholar] [CrossRef]
- Gholamalizadeh, E.; Pahlevanzadeh, F.; Ghani, K.; Karimipour, A.; Nguyen, T.K.; Safaei, M.R. Simulation of water/FMWCNT nanofluid forced convection in a microchannel filled with porous material under slip velocity and temperature jump boundary conditions. Int. J. Numer. Methods Heat Fluid Flow 2019. [Google Scholar] [CrossRef]
- Nikkhah, Z.; Karimipour, A.; Safaei, M.R.; Forghani-Tehrani, P.; Goodarzi, M.; Dahari, M.; Wongwises, S. Forced convective heat transfer of water/functionalized multi-walled carbon nanotube nanofluids in a microchannel with oscillating heat flux and slip boundary condition. Int. Commun. Heat Mass Transf. 2015, 68, 69–77. [Google Scholar] [CrossRef]
- Abidi, A.; Raizah, Z.; Madiouli, J. Magnetic Field Effect on the Double Diffusive Natural Convection in Three-Dimensional Cavity Filled with Micropolar Nanofluid. Appl. Sci. 2018, 8, 2342. [Google Scholar] [CrossRef] [Green Version]
- Magodora, M.; Mondal, H.; Sibanda, P. Dual solutions of a micropolar nanofluid flow with radiative heat mass transfer over stretching/shrinking sheet using spectral quasilinearization method. Multidiscip. Modeling Mater. Struct. 2019. [Google Scholar] [CrossRef]
- Karimipour, A.; Nezhad, A.H.; D’Orazio, A.; Esfe, M.H.; Safaei, M.R.; Shirani, E. Simulation of copper–water nanofluid in a microchannel in slip flow regime using the lattice Boltzmann method. Eur. J. Mech. B Fluids 2015, 49, 89–99. [Google Scholar] [CrossRef]
- Zaib, A.; Haq, R.U. Magnetohydrodynamics mixed convective flow driven through a static wedge including TiO2 nanomaterial with micropolar liquid: Similarity dual solutions via finite difference method. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2019. [Google Scholar] [CrossRef]
- Lund, L.A.; Omar, Z.; Dero, S.; Khan, I. Linear stability analysis of MHD flow of micropolar fluid with thermal radiation and convective boundary condition: Exact solution. Heat Transf. Asian Res. 2019, 1–16. [Google Scholar] [CrossRef]
- Ishak, A.; Nazar, R.; Pop, I. Magnetohydrodynamic (MHD) flow of a micropolar fluid towards a stagnation point on a vertical surface. Comput. Math. Appl. 2008, 56, 3188–3194. [Google Scholar] [CrossRef] [Green Version]
- Raza, J.; Rohni, A.M.; Omar, Z. Rheology of micropolar fluid in a channel with changing walls: Investigation of multiple solutions. J. Mol. Liq. 2016, 223, 890–902. [Google Scholar] [CrossRef]
- Lund, L.A.; Omar, Z.; Khan, I. Quadruple Solutions of Mixed Convection Flow of Magnetohydrodynamic Nanofluid Over Exponentially Vertical Shrinking and Stretching Surfaces: Stability Analysis. Comput. Methods Programs Biomed. 2019. [Google Scholar] [CrossRef]
- Schlichting, H. Boundary Layer Theory; McGraw-Hill Inc.: New York, NY, USA, 1979. [Google Scholar]
- Soid, S.K.; Ishak, A.; Pop, I. MHD flow and heat transfer over a radially stretching/shrinking disk. Chin. J. Phys. 2018, 56, 58–66. [Google Scholar] [CrossRef]
- Khan, M.; Irfan, M.; Ahmad, L.; Khan, W.A. Simultaneous investigation of MHD and convective phenomena on time-dependent flow of Carreau nanofluid with variable properties: Dual solutions. Phys. Lett. A 2018, 34, 2334–2342. [Google Scholar] [CrossRef]
- Raju, C.S.K.; Sandeep, N.; Babu, M.J.; Sugunamma, V. Dual solutions for three-dimensional MHD flow of a nanofluid over a nonlinearly permeable stretching sheet. Alex. Eng. J. 2016, 55, 151–162. [Google Scholar] [CrossRef] [Green Version]
- Lok, Y.Y.; Ishak, A.; Pop, I. Oblique stagnation slip flow of a micropolar fluid towards a stretching/shrinking surface: A stability analysis. Chin. J. Phys. 2018, 56, 3062–3072. [Google Scholar] [CrossRef]
- Sandeep, N.; Sulochana, C. Dual solutions for unsteady mixed convection flow of MHD micropolar fluid over a stretching/shrinking sheet with non-uniform heat source/sink. Eng. Sci. Technol. Int. J. 2015, 18, 738–745. [Google Scholar] [CrossRef] [Green Version]
- Yacob, N.A.; Ishak, A.; Pop, I. Melting heat transfer in boundary layer stagnation-point flow towards a stretching/shrinking sheet in a micropolar fluid. Comput. Fluids 2011, 47, 16–21. [Google Scholar] [CrossRef]
- Turkyilmazoglu, M. A note on micropolar fluid flow and heat transfer over a porous shrinking sheet. Int. J. Heat Mass Transf. 2014, 72, 388–391. [Google Scholar] [CrossRef]
- Bhattacharyya, K.; Mukhopadhyay, S.; Layek, G.C.; Pop, I. Effects of thermal radiation on micropolar fluid flow and heat transfer over a porous shrinking sheet. Int. J. Heat Mass Transf. 2012, 55, 2945–2952. [Google Scholar] [CrossRef]
- Wilks, G.; Bramley, J.S. Dual solutions in mixed convection. Proc. R. Soc. Edinb. Sect. A Math. 1981, 87, 349–358. [Google Scholar] [CrossRef]
- Merkin, J.H. On dual solutions occurring in mixed convection in a porous medium. J. Eng. Math. 1986, 20, 171–179. [Google Scholar] [CrossRef]
- Ali, F.M.; Naganthran, K.; Nazar, R.; Pop, I. MHD mixed convection boundary layer stagnation-point flow on a vertical surface with induced magnetic field. Int. J. Numer. Methods Heat Fluid Flow 2019. [Google Scholar] [CrossRef]
- Lund, L.A.; Omar, Z.; Khan, I. Analysis of dual solution for MHD flow of Williamson fluid with slippage. Heliyon 2019, 5, e01345. [Google Scholar] [CrossRef] [Green Version]
- Khan, M.; Alshomrani, A.S.; Haq, R.U. Investigation of dual solutions in flow of a non-Newtonian fluid with homogeneous–heterogeneous reactions: Critical points. Eur. J. Mech. B Fluids 2018, 68, 30–38. [Google Scholar]
- Jusoh, R.; Nazar, R.; Pop, I. Impact of heat generation/absorption on the unsteady magnetohydrodynamic stagnation point flow and heat transfer of nanofluids. Int. J. Numer. Methods Heat Fluid Flow 2019. [Google Scholar] [CrossRef]
- Lund, L.A.; Ching, D.L.C.; Omar, Z.; Khan, I.; Nisar, K.S. Triple Local Similarity Solutions of Darcy-Forchheimer Magnetohydrodynamic (MHD) Flow of Micropolar Nanofluid Over an Exponential Shrinking Surface: Stability Analysis. Coatings 2019, 9, 527. [Google Scholar] [CrossRef] [Green Version]
- Khan, Z.H.; Qasim, M.; Haq, R.U.; Al-Mdallal, Q.M. Closed form dual nature solutions of fluid flow and heat transfer over a stretching/shrinking sheet in a porous medium. Chin. J. Phys. 2017, 55, 1284–1293. [Google Scholar] [CrossRef]
- Raza, J.; Rohni, A.M.; Omar, Z.; Awais, M. Heat and mass transfer analysis of MHD nanofluid flow in a rotating channel with slip effects. J. Mol. Liq. 2016, 219, 703–708. [Google Scholar] [CrossRef]
- Rohni, A.M.; Ahmad, S.; Pop, I. Flow and heat transfer at a stagnation-point over an exponentially shrinking vertical sheet with suction. Int. J. Therm. Sci. 2014, 75, 164–170. [Google Scholar] [CrossRef]
- Weidman, P.D.; Kubitschek, D.G.; Davis, A.M.J. The effect of transpiration on self-similar boundary layer flow over moving surfaces. Int. J. Eng. Sci. 2006, 44, 730–737. [Google Scholar] [CrossRef]
- Lund, L.A.; Omar, Z.; Khan, I. Steady incompressible magnetohydrodynamics Casson boundary layer flow past a permeable vertical and exponentially shrinking sheet: A stability analysis. Heat Transf. Asian Res. 2019. [Google Scholar] [CrossRef]
- Harris, S.D.; Ingham, D.B.; Pop, I. Mixed convection boundary-layer flow near the stagnation point on a vertical surface in a porous medium: Brinkman model with slip. Transp. Porous Media 2009, 77, 267–285. [Google Scholar] [CrossRef]
- Ali, L.L.; Omar, Z.; Khan, I.; Raza, J.; Bakouri, M.; Tlili, I. Stability Analysis of Darcy-Forchheimer Flow of Casson Type Nanofluid Over an Exponential Sheet: Investigation of Critical Points. Symmetry 2019, 11, 412. [Google Scholar]

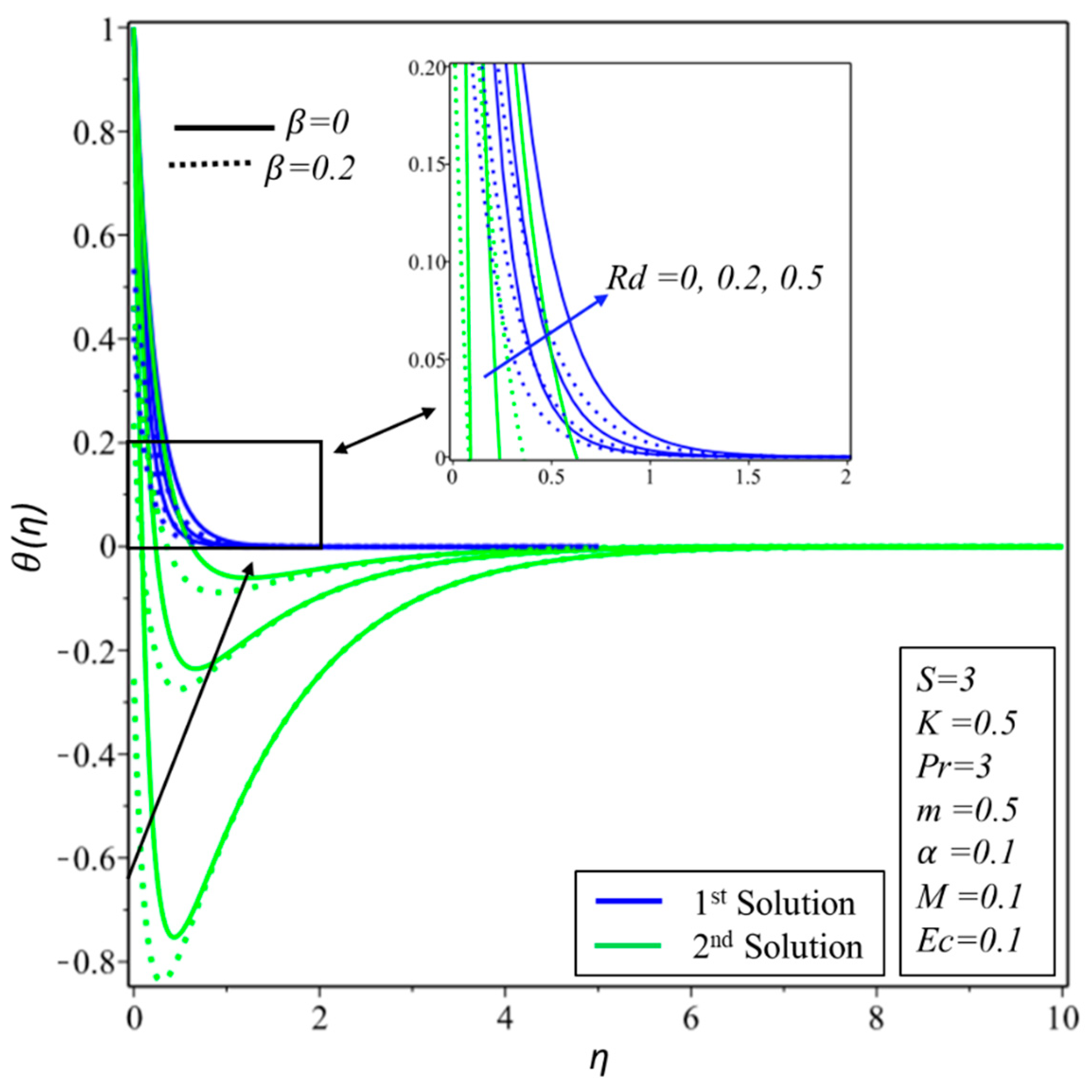
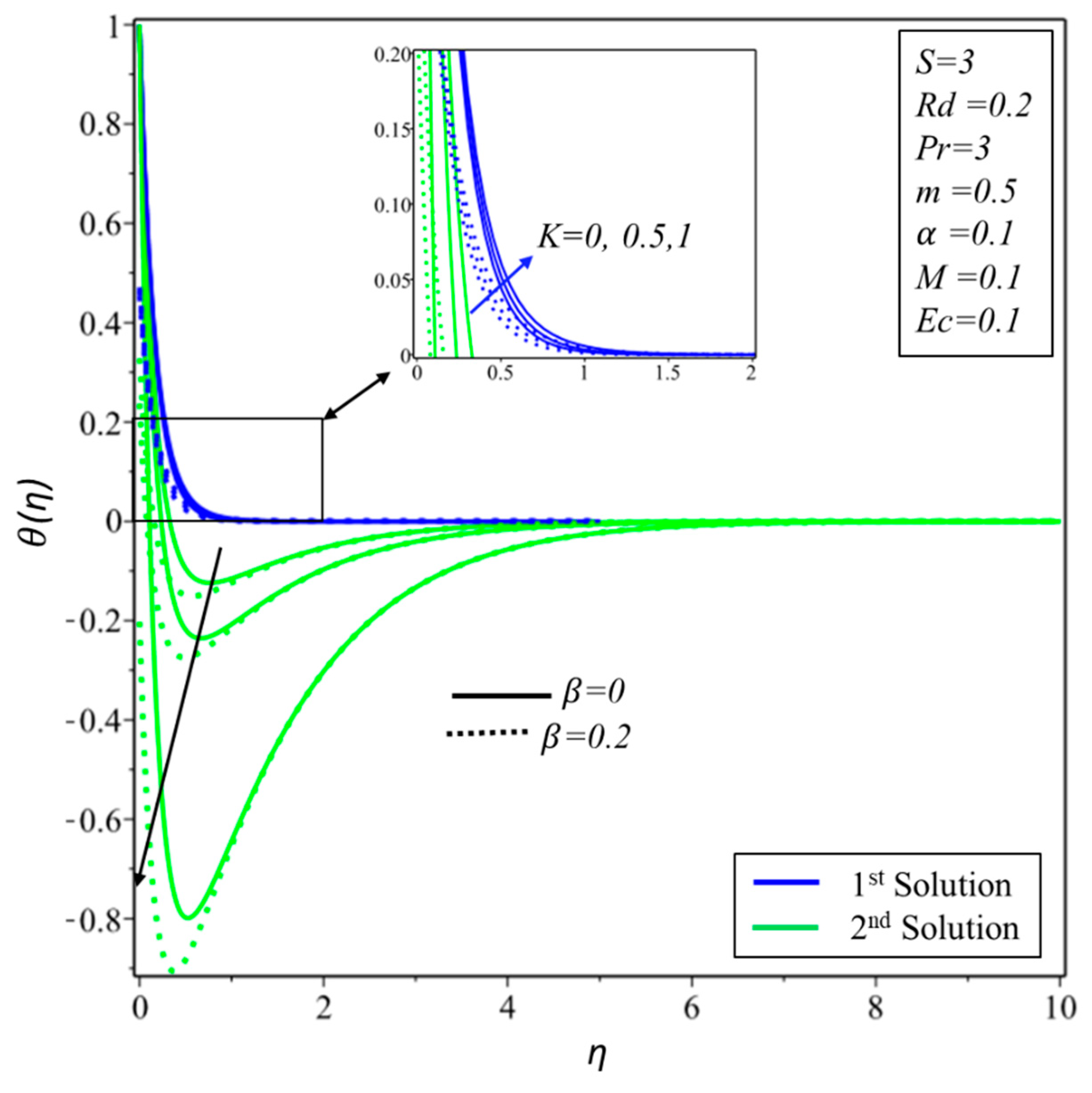
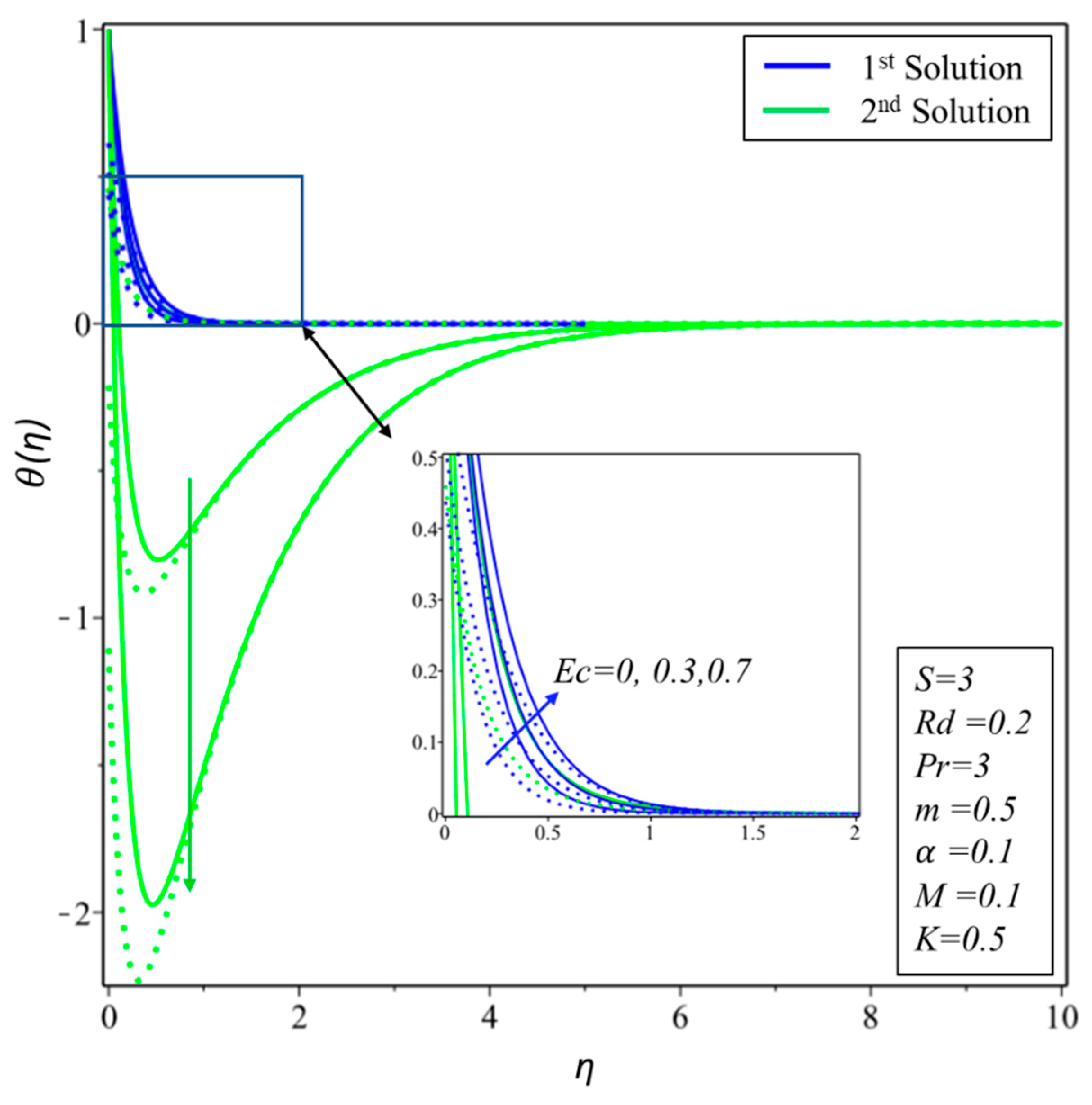
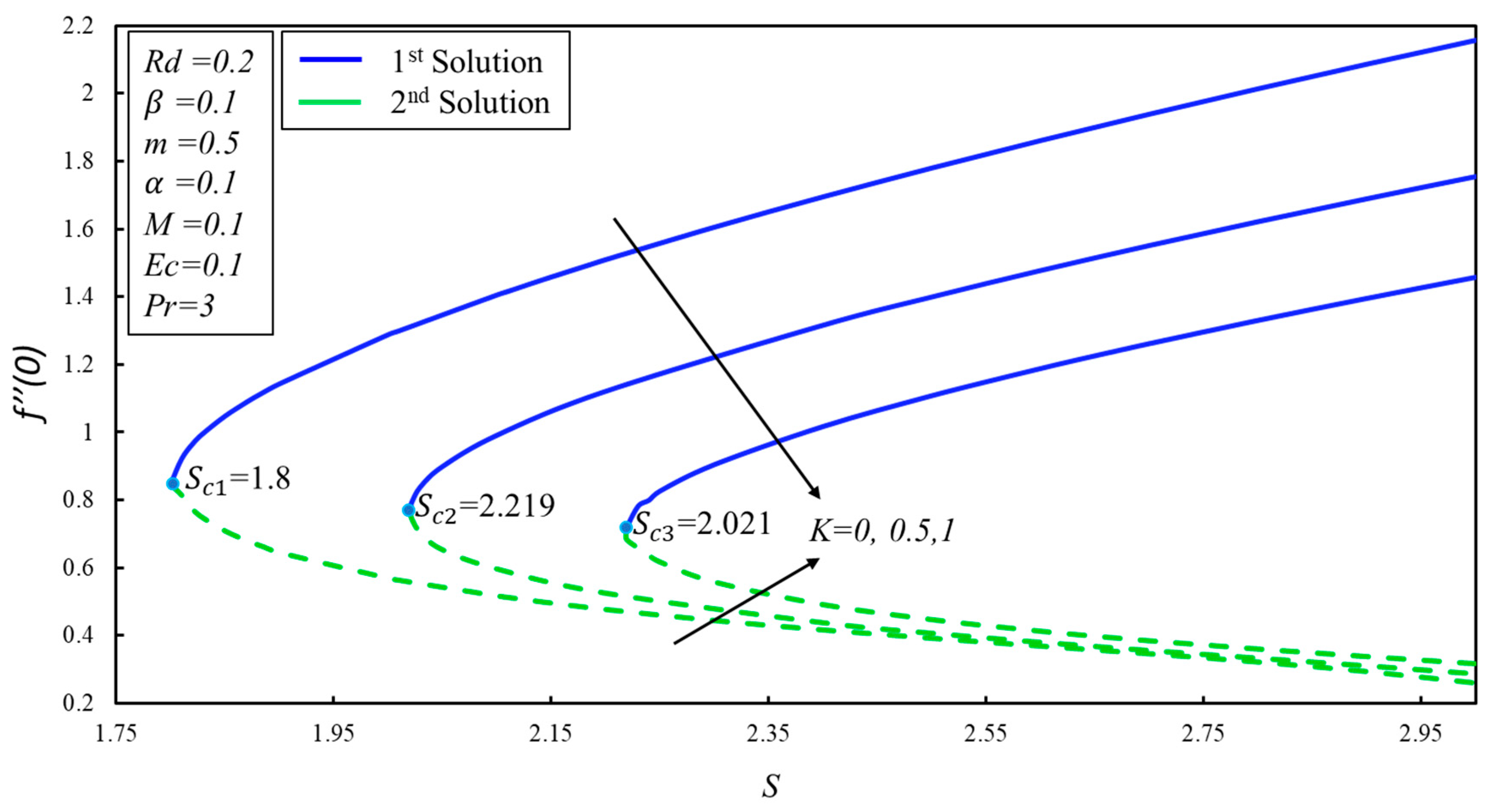
| K | S | ||
|---|---|---|---|
| 1st Solution | 2nd Solution | ||
| 0 | 1.85 | 0.43203 | −0.02392 |
| 1.95 | 0. 83673 | −0.2750 | |
| 2.35 | 1.0874 | −0.92698 | |
| 1 | 2.35 | 0.3292 | −0.2685 |
| 2.5 | 0.7038 | −0.4163 | |
| K | S | ||||||
|---|---|---|---|---|---|---|---|
| 1st Solution | 2nd Solution | ||||||
| Exact | Numerical | Absolute Error | Exact | Numerical | Absolute Error | ||
| 0 | 2 | 1.00 | 1.00009998 | -- | -- | -- | |
| 2.5 | 2.00 | 1.99999999 | 0.50 | 0.49710246 | |||
| 0.1 | 3 | 2.47185249 | 2.47185249 | 0 | 0.38529036 | 0.38486773 | |
| 3.5 | 3.01773913 | 3.01773913 | 0 | 0.31559419 | 0.31386568 | ||
| 0.5 | 3 | 2.00 | 2.00000000 | 0 | 0.40 | 0.39990306 | |
| 3.5 | 2.47703296 | 2.47703306 | 0.32296703 | 0.31959198 | |||
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lund, L.A.; Omar, Z.; Khan, I.; Kadry, S.; Rho, S.; Mari, I.A.; Nisar, K.S. Effect of Viscous Dissipation in Heat Transfer of MHD Flow of Micropolar Fluid Partial Slip Conditions: Dual Solutions and Stability Analysis. Energies 2019, 12, 4617. https://doi.org/10.3390/en12244617
Lund LA, Omar Z, Khan I, Kadry S, Rho S, Mari IA, Nisar KS. Effect of Viscous Dissipation in Heat Transfer of MHD Flow of Micropolar Fluid Partial Slip Conditions: Dual Solutions and Stability Analysis. Energies. 2019; 12(24):4617. https://doi.org/10.3390/en12244617
Chicago/Turabian StyleLund, Liaquat Ali, Zurni Omar, Ilyas Khan, Seifedine Kadry, Seungmin Rho, Irshad Ali Mari, and Kottakkaran Sooppy Nisar. 2019. "Effect of Viscous Dissipation in Heat Transfer of MHD Flow of Micropolar Fluid Partial Slip Conditions: Dual Solutions and Stability Analysis" Energies 12, no. 24: 4617. https://doi.org/10.3390/en12244617
APA StyleLund, L. A., Omar, Z., Khan, I., Kadry, S., Rho, S., Mari, I. A., & Nisar, K. S. (2019). Effect of Viscous Dissipation in Heat Transfer of MHD Flow of Micropolar Fluid Partial Slip Conditions: Dual Solutions and Stability Analysis. Energies, 12(24), 4617. https://doi.org/10.3390/en12244617









