Duality Based Risk Mitigation Method for Construction of Joint Hydro-Wind Coordination Short-Run Marginal Cost Curves
Abstract
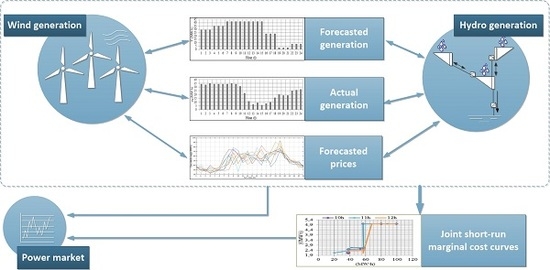
1. Introduction
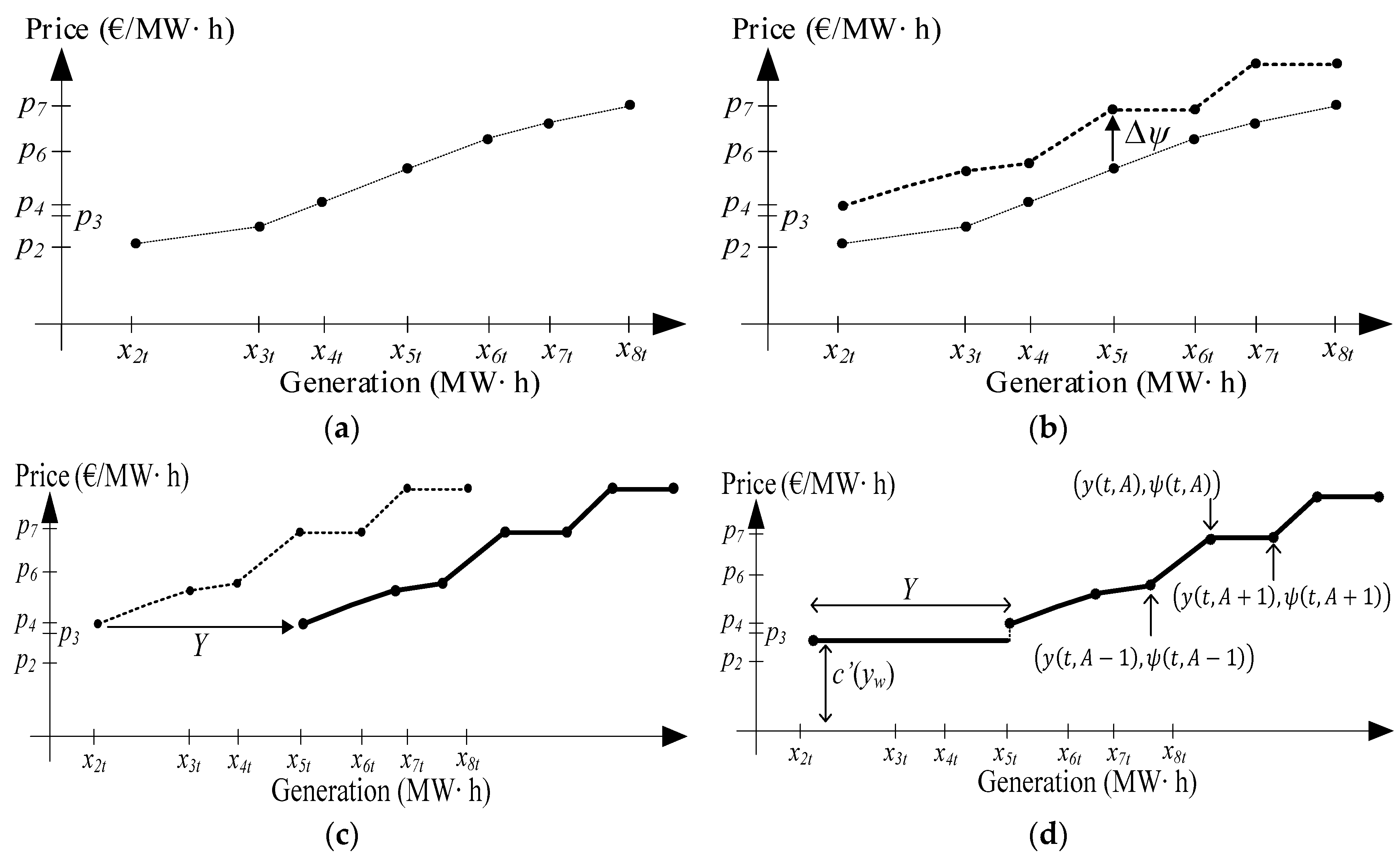
2. Construction of the Joint Short-Run Marginal Cost Curve
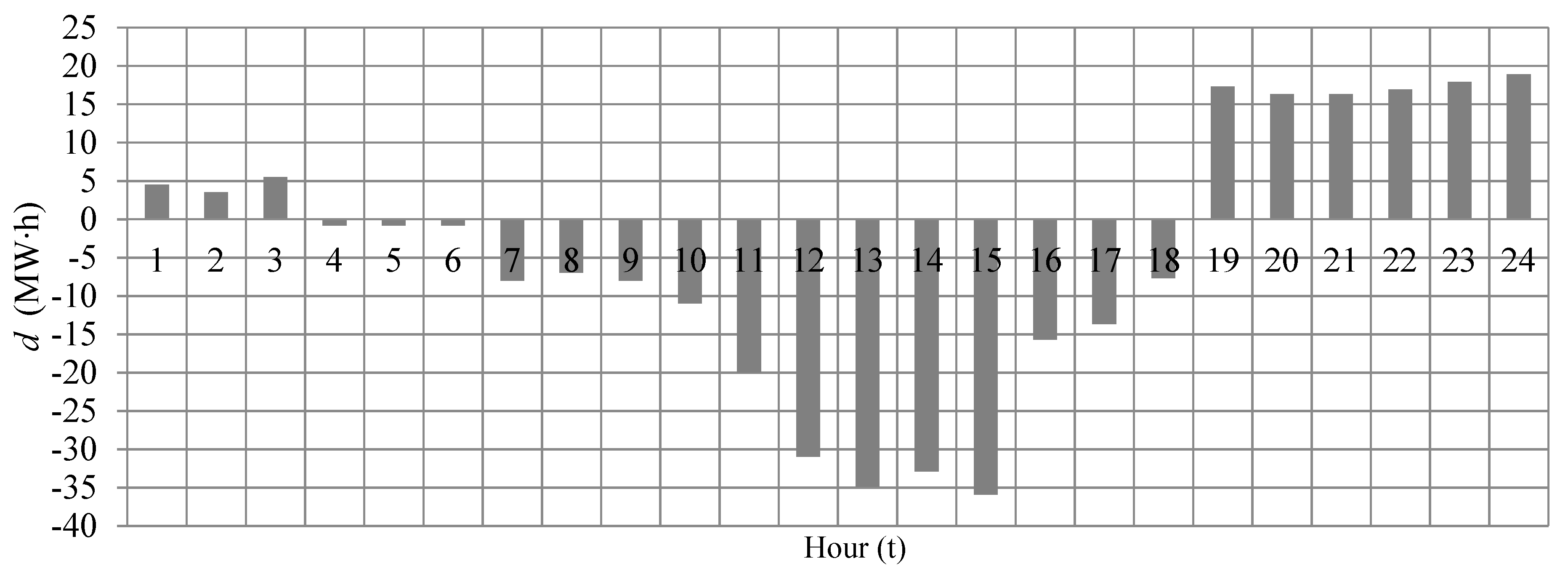
3. Case Study and Results
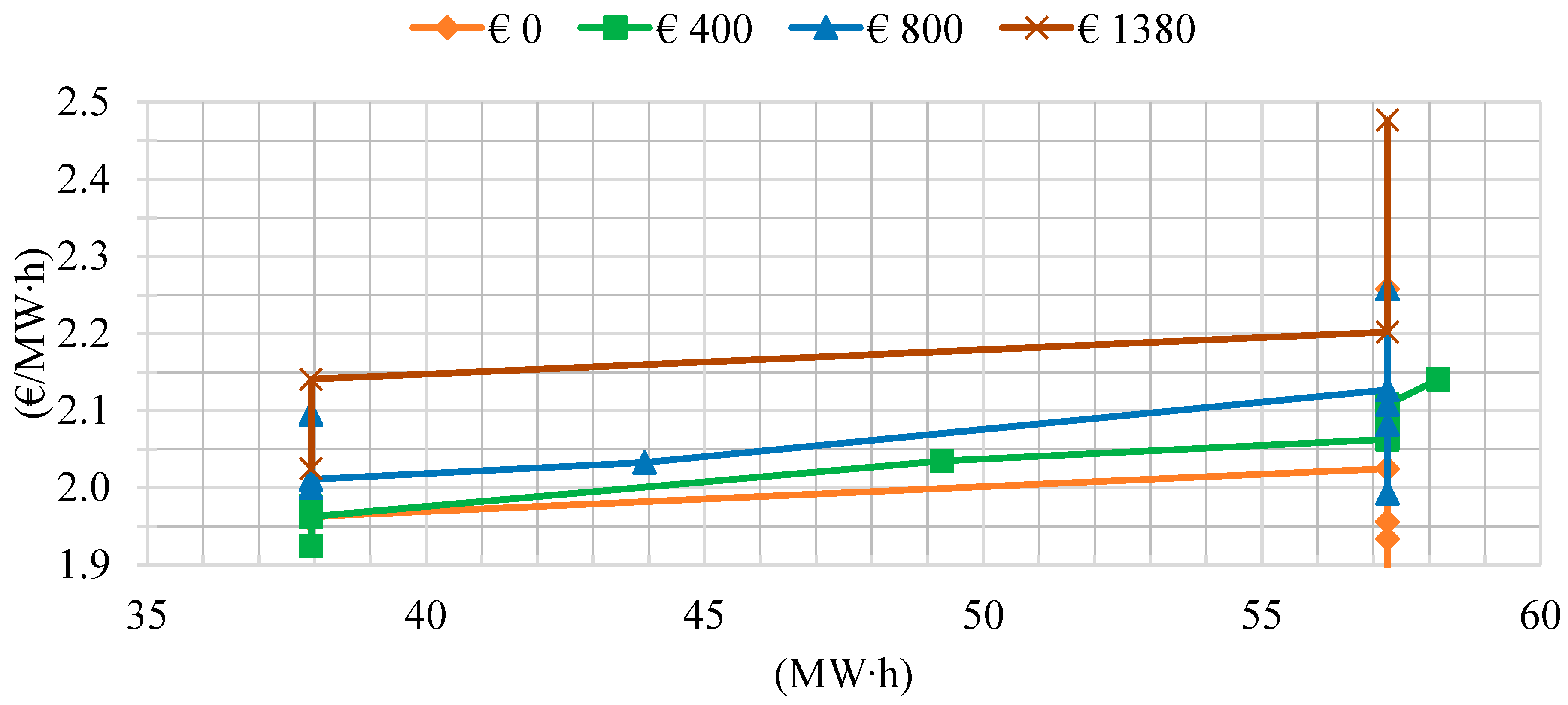
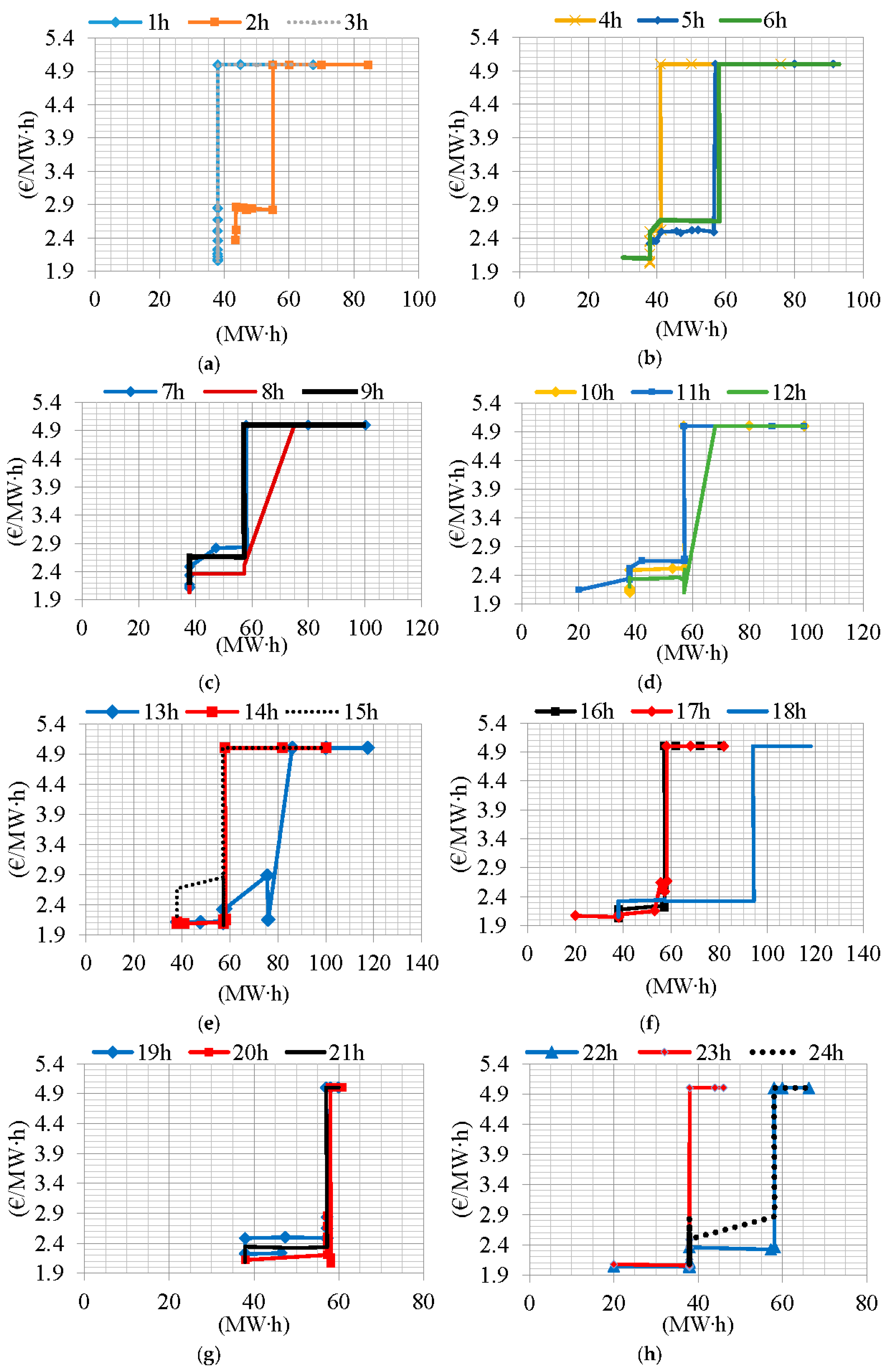
3.1. Results
3.2. Discussion
4. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
Nomenclature
| Symbols | |
| A | An event from the family of all events , . |
| Short-run marginal cost function (€/MW∙h). | |
| Difference between forecasted and actual wind generation (MW). | |
| Positive wind difference (MW). | |
| Negative wind difference (MW). | |
| Natural water inflow (MW). | |
| Special function used for risk shaping of CVaR (€). | |
| Sigma algebra which defines all possible events in hour . | |
| Net outflow of hydro generation (MW) | |
| Hourly revenue (€). | |
| Maximal capacity of reservoir (MW∙h). | |
| Parameter used for risk exposure reduction in risk shaping procedure (€). | |
| Hydro turbine maximal capacity (MW). | |
| Wind turbine maximal capacity (MW). | |
| Minimal capacity of reservoir (MW∙h). | |
| Hydro turbine minimal capacity (MW). | |
| Wind turbine minimal capacity (MW). | |
| Probability of the event and consequently probability of realization of the price | |
| Energy stock, amount of water in reservoir in t, (MW∙h). | |
| Energy stock at the beginning of planning interval (MW∙h) | |
| Energy stock surplus or deficit at the end of planning interval (MWh) | |
| Hydro generation (MW). | |
| Y | Contracted wind generation (MW). |
| Actual wind generation (MW). | |
| Greek | |
| Percentile used for the CVaR where 1- defines the worst events (%). | |
| Shadow prices associated with constraint of CVaR’s helping variable (€). | |
| Shadow prices associated with CVaR’s hourly revenue constraint. | |
| The decision variable which defines the Value at Risk (€). | |
| Variable used for obtainment of the CVaR (€). | |
| Shadow price of hydro generation maximum capacity (€/MW∙h). | |
| Shadow price of reservoir maximum capacity (€/MW∙h). | |
| Shadow price of energy stock surplus/deficit at the end of operation(€/MW∙h). | |
| Shadow price of hydro generation minimum capacity (€/MW∙h). | |
| Shadow price of reservoir minimum capacity (€/MW∙h). | |
| Shadow price of risk mitigation capability (dimensionless). | |
| Price of electricity (€/MW∙h). | |
| Shadow price of water (€/MW∙h). | |
| Spaces | |
| Planning interval, subspace of the real line . | |
References
- Ilak, P.; Rajšl, I.; Krajcar, S.; Delimar, M. The impact of a wind variable generation on the hydro generation water. Appl. Energy 2015, 154, 197–208. [Google Scholar] [CrossRef]
- Horsley, A.; Wrobel, A.J. Efficiency Rents of Storage Plants in Peak-Load Pricing, II: Hydroelectricty; London School of Economics & Political Science (LSE): London, UK, 1999. [Google Scholar]
- Horsley, A.; Wrobel, A.J. Efficiency Rents of Storage Plants in Peak-Load Pricing, I: Pumped Storage; London School of Economics & Political Science (LSE): London, UK, 1996. [Google Scholar]
- Ilak, P.; Krajcar, S.; Rajšl, I.; Delimar, M. Pricing Energy and Ancillary Services in a Day-Ahead Market for a Price-Taker Hydro Generating Company Using a Risk-Constrained Approach. Energies 2014, 7, 2317–2342. [Google Scholar] [CrossRef]
- Rockafellar, R.T. Conjugate Duality and Optimization; Siam: Philadelphia, PA, USA, 1974. [Google Scholar]
- Kaldellis, J.K.; Kapsali, M.; Kavadis, K.A. Energy balance analysis of wind-based pumped hydro storage systems in remote island electrical networks. Appl. Energy 2010, 87, 2427–2437. [Google Scholar] [CrossRef]
- Varkani, A.K.; Daraeepour, A.; Monsef, H.A. A new self-scheduling strategy for integrated operation of wind and pumped-storage power plants in power markets. Appl. Energy 2011, 88, 5002–5012. [Google Scholar] [CrossRef]
- Rajšl, I.; Ilak, P.; Delimar, M.; Krajcar, S. Dispatch Method for Independently Owned Hydropower Plants in the Same River Flow. Energies 2012, 5, 3674–3690. [Google Scholar] [CrossRef]
- Angarita, J.M.; Usaola, J.G. Combining hydro-generation and wind energy: Biddings and operation on electricity spot markets. Electr. Power Syst. Res. 2007, 77, 393–400. [Google Scholar] [CrossRef]
- Angarita, J.L.; Usaola, J.; Martínez-Crespo, J. Combined hydro-wind generation bids in a pool-based electricity market. Electr. Power Syst. Res. 2009, 79, 1038–1046. [Google Scholar] [CrossRef]
- Bahrami, S.; Amini, M.H. A decentralized trading algorithm for an electricity market with generation uncertainty. Appl. Energy 2018, 218, 520–532. [Google Scholar] [CrossRef]
- Bahrami, S.; Toulabi, M.; Ranjbar, S.; Moeini-Aghtaie, M.; Ranjbar, A.M. A Decentralized Energy Management Framework for Energy Hubs in Dynamic Pricing Markets. IEEE Trans. Smart Grid 2017. [Google Scholar] [CrossRef]
- Samadi, P.; Bahrami, S.; Wong, V.W.S.; Schober, R. Power dispatch and load control with generation uncertainty. In Proceedings of the 2015 IEEE Global Conference on Signal and Information Processing (GlobalSIP), Orlando, FL, USA, 14–16 December 2015; pp. 1126–1130. [Google Scholar]
- Mohammadi, A.; Mehrtash, M.; Kargarian, A. Diagonal Quadratic Approximation for Decentralized Collaborative TSO+DSO Optimal Power Flow. IEEE Trans. Smart Grid 2018. [Google Scholar] [CrossRef]
- Elsaiah, S.; Cai, N.; Benidris, M.; Mitra, J. Fast economic power dispatch method for power system planning studies. IET Gener. Transm. Distrib. 2015, 9, 417–426. [Google Scholar] [CrossRef]
- Boroojeni, K.G.; Amini, M.H.; Nejadpak, A.; Iyengar, S.S.; Hoseinzadeh, B.; Bak, C.L. A theoretical bilevel control scheme for power networks with large-scale penetration of distributed renewable resources. In Proceedings of the 2016 IEEE International Conference on Electro Information Technology (EIT), Grand Forks, ND, USA, 19–21 May 2016; pp. 0510–0515. [Google Scholar]
- Vieira, B.; Viana, A.; Matos, M.; Pedro, J. A multiple criteria utility-based approach for unit commitment with wind power and pumped storage hydro. Electr. Power Syst. Res. 2016, 131, 244–254. [Google Scholar] [CrossRef]
- Duque, J.; Castronuovo, E.D.; Sánchez, I.; Usaola, J. Optimal operation of a pumped-storage hydro plant that compensates the imbalances of a wind power producer. Electr. Power Syst. Res. 2011, 81, 1767–1777. [Google Scholar] [CrossRef]
- Rockafellar, R.T.; Uryasev, S. Optimization of conditional value-at-risk. J. Risk 1998, 2, 21–41. [Google Scholar] [CrossRef]
- Artzner, P.; Delbaen, F.; Eber, J.M.; Heath, D. Thinking coherently. Risk 1997, 10, 68–91. [Google Scholar]
- Acerbi, C. Spectral measures of risk: A coherent representation of subjective risk aversion. J. Bank. Financ. 2002, 26, 1505–1518. [Google Scholar] [CrossRef]
- Follmer, H.; Schied, A. Convex and Coherent Risk Measures; Humboldt University: Berlin, Germany, 2008. [Google Scholar]
- Rockafellar, R.T. Coherent Approaches to Risk in Optimization Under Uncertanty. Tutor. Oper. Res. Inf. 2007, 3, 38–61. [Google Scholar]
- Rockafellar, R.T.; Uryasev, S. Conditional Value-at-Risk for General Loss Distributions. J. Bank. Financ. 2002, 26, 1443–1471. [Google Scholar] [CrossRef]
- Perekhodtsev, D.; Lave, L. Efficient Bidding for Hydro Power Plants in Markets for Energy and Ancillary Services; MIT Center for Energy and Environmental Policy Research: Cambridge, MA, USA, 2005. [Google Scholar]
- Koopmans, T.C. Water Storage Policy in a Simplified Hydroelectric System. In Proceedings of the First International Conference on Operational Research, Oxford, UK, 2–5 September 1957. [Google Scholar]
- Warford, J.; Munasinghe, M. Electricity Pricing: Theory and Case Studies; International Bank for Reconstruction and Development: Baltimore, MD, USA; London, UK, 1982. [Google Scholar]
- Zhang, H.; Gao, F.; Wu, J.; Liu, K.; Liu, X. Optimal Bidding Strategies for Wind Power Producers in the Day-ahead Electricity Market. Energies 2012, 5, 4804–4823. [Google Scholar] [CrossRef]
- Ilak, P.; Krajcar, S.; Rajšl, I.; Delimar, M. Profit Maximization of a Hydro Producer in a Day-Ahead Energy Market and Ancillary Service Markets. In Proceedings of the IEEE Region 8 Conference EuroCon, Zagreb, Croatia, 1–4 July 2013. [Google Scholar]
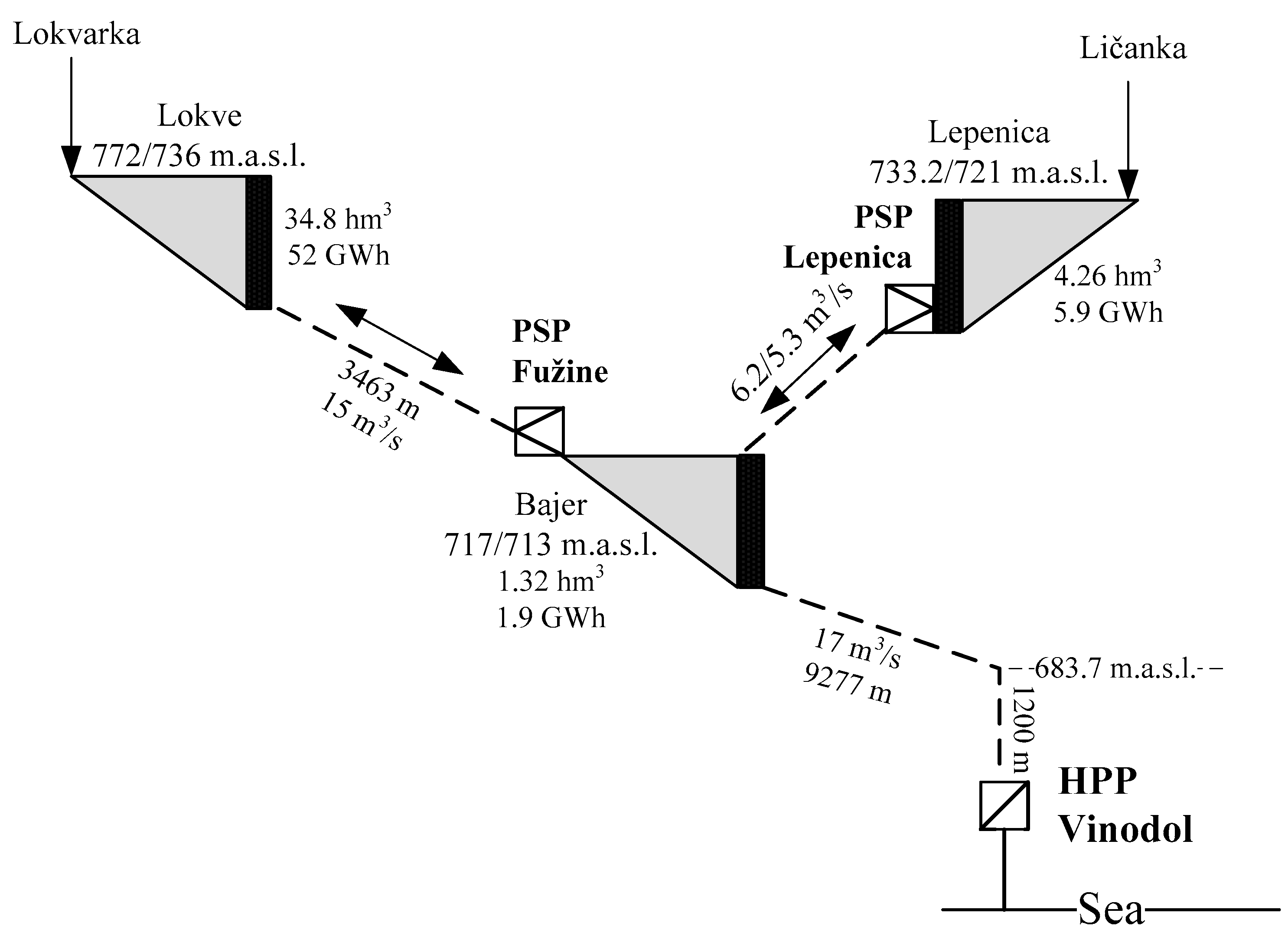
| Reservoir | kSt (GWh) | Power Plant | kTu/nTu (m3/s) | kTu/nTu (MW) |
|---|---|---|---|---|
| Lokve | 52 | PSP Fužine | 10/9 | 4.6/4.8 |
| Bajer | 1.9 | HP Vinodol | 18.6 | 94.5 |
| Lepenica | 5.9 | PSP Lepenica | 6.2/5.3 | 1.14/1.25 |
| Reservoir | e (m3/s) | ||
|---|---|---|---|
| Lokve | 66 | 46 | 0.68 |
| Bajer | 64 | 64 | 0.89 |
| Lepenica | 58 | 58 | 0.21 |
| Revenue with the Proposed Method (€) | Revenue in “Bussines as Usual” Case (€) | (€) |
|---|---|---|
| 98,885 | 89,351 | 0 |
| 97,995 | 88,461 | 400 |
| 97,100 | 87,566 | 800 |
| 96,332 | 86,798 | 1380 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ilak, P.; Rajšl, I.; Đaković, J.; Delimar, M. Duality Based Risk Mitigation Method for Construction of Joint Hydro-Wind Coordination Short-Run Marginal Cost Curves. Energies 2018, 11, 1254. https://doi.org/10.3390/en11051254
Ilak P, Rajšl I, Đaković J, Delimar M. Duality Based Risk Mitigation Method for Construction of Joint Hydro-Wind Coordination Short-Run Marginal Cost Curves. Energies. 2018; 11(5):1254. https://doi.org/10.3390/en11051254
Chicago/Turabian StyleIlak, Perica, Ivan Rajšl, Josip Đaković, and Marko Delimar. 2018. "Duality Based Risk Mitigation Method for Construction of Joint Hydro-Wind Coordination Short-Run Marginal Cost Curves" Energies 11, no. 5: 1254. https://doi.org/10.3390/en11051254
APA StyleIlak, P., Rajšl, I., Đaković, J., & Delimar, M. (2018). Duality Based Risk Mitigation Method for Construction of Joint Hydro-Wind Coordination Short-Run Marginal Cost Curves. Energies, 11(5), 1254. https://doi.org/10.3390/en11051254