Normal Pursuit-System Limitations—First Discovered in Infantile Nystagmus Syndrome
Abstract
:Introduction
Methods
Recording
Stimuli
Protocol
Subjects
Analysis and Simulations
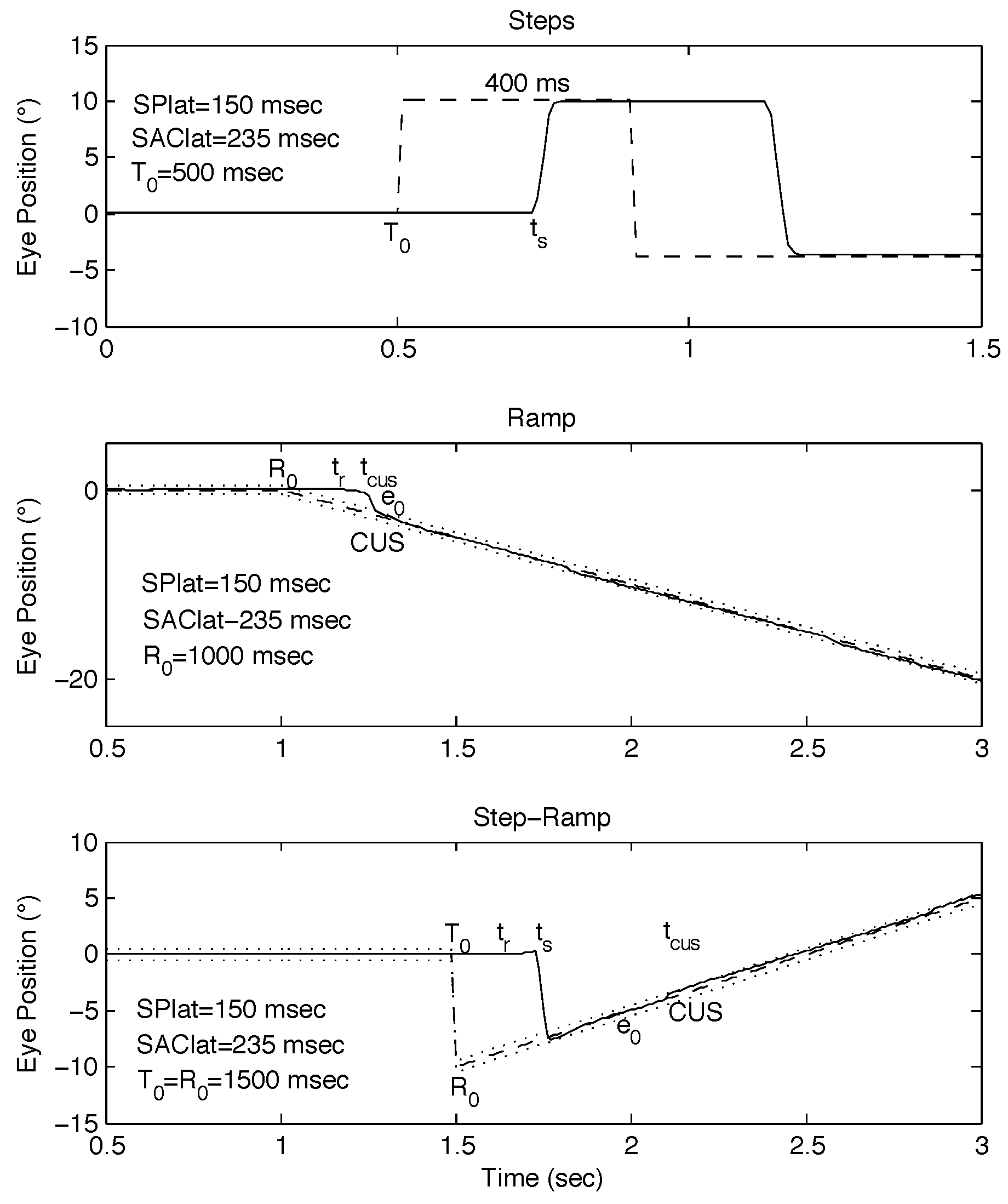
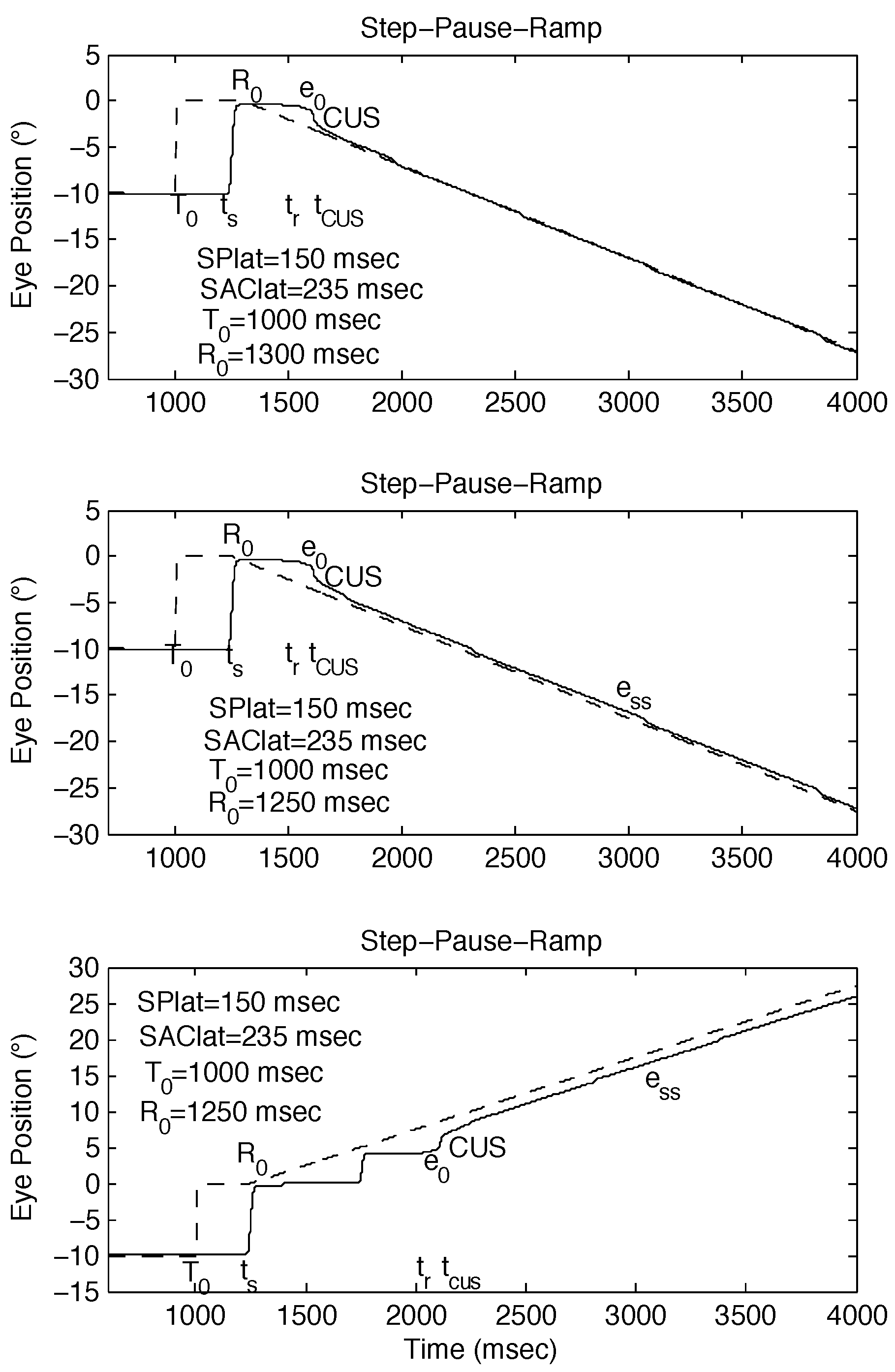
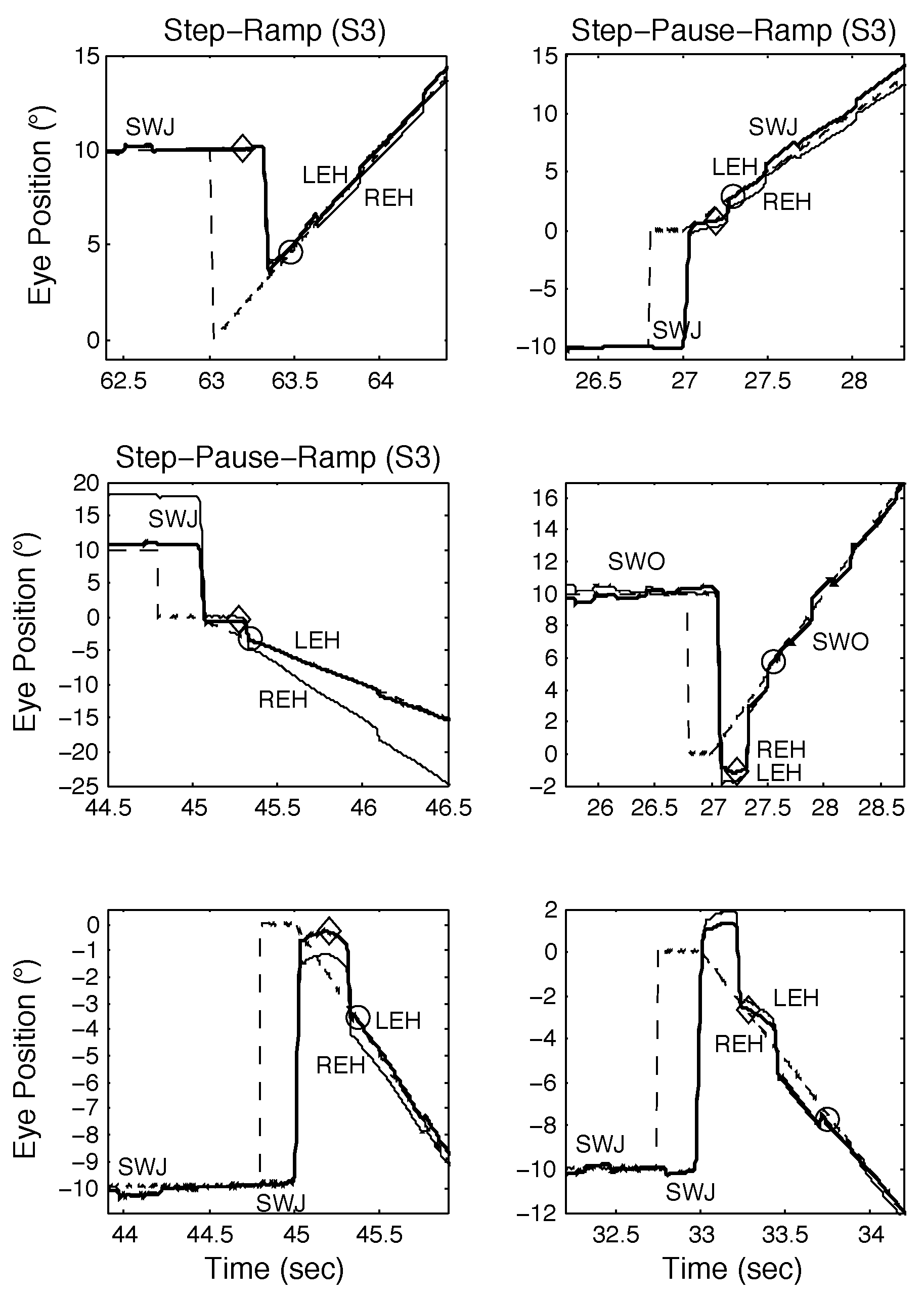
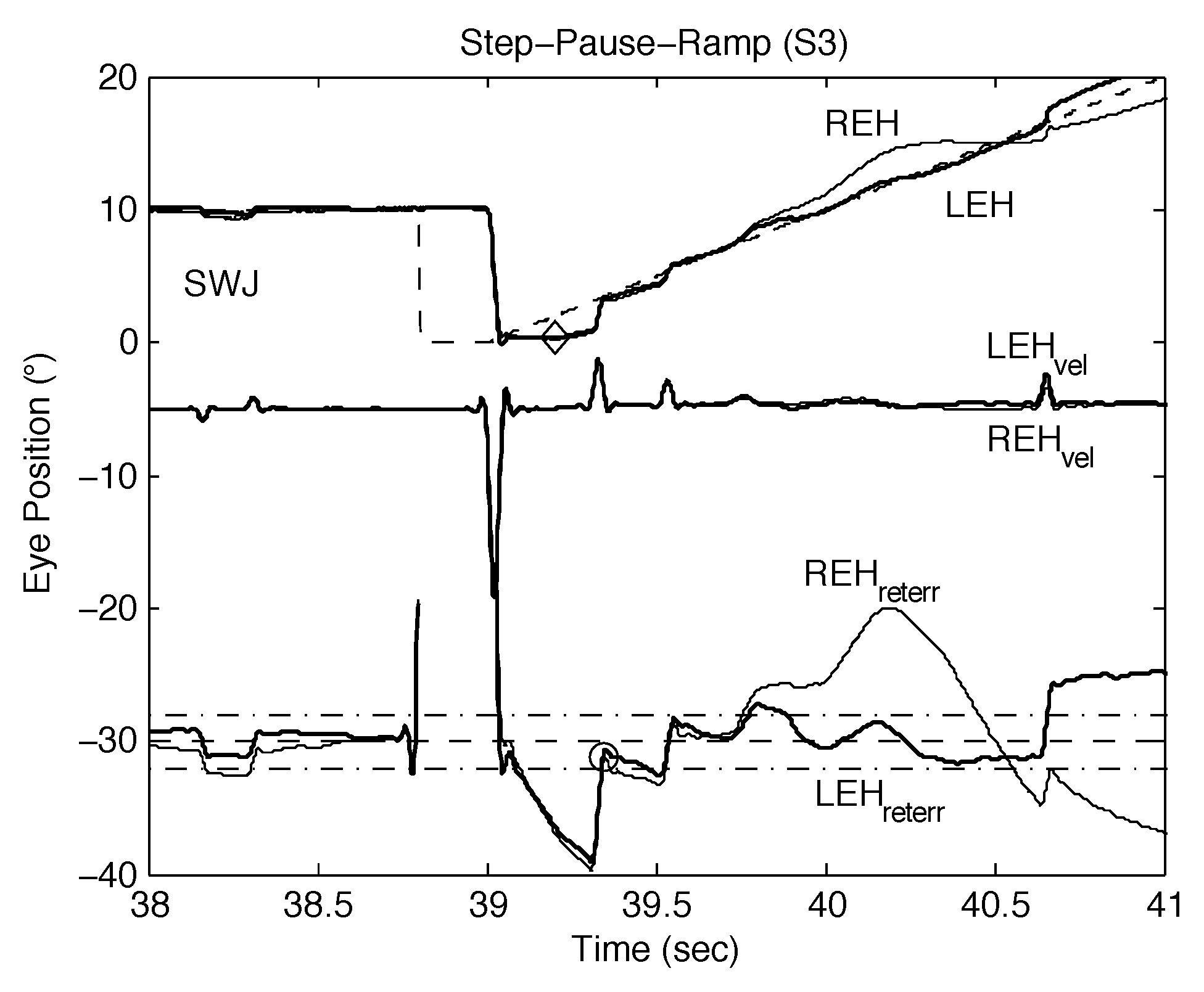
Results
Model Simulations
Subject Data
Discussion
Conclusions
Acknowledgments
APPENDIX
References
- Abel, L. A., L. F. Dell’Osso, and R. B. Daroff. 1978. Analog model for gaze-evoked nystagmus. Institute of Electrical and Electronics Engineers Transactions of Biomedical Engineering BME, 25: 71–75. [Google Scholar]
- Awater, H., and M. Lappe. 2006. Mislocalization of perceived saccade target position induced by perisaccadic visual stimulation. Journal of Neuroscience 26: 12–20. [Google Scholar] [CrossRef] [PubMed]
- CEMAS_Working_Group. 2001. A National Eye Institute Sponsored Workshop and Publication on The Classification of Eye Movement Abnormalities and Strabismus (CEMAS). In The National Eye Institute Publications (www.nei.nih.gov). Bethesda, MD: National Institutes of Health, National Eye Institute. [Google Scholar]
- Dell’Osso, L. F. 1968. A Dual-Mode Model for the Normal Eye Tracking System and the System with Nystagmus. (Ph.D. Dissertation). University of Wyoming, Laramie. [Google Scholar]
- Dell’Osso, L. F. 1986. Evaluation of smooth pursuit in the presence of congenital nystagmus. Neuro-ophthalmology 6: 383–406. [Google Scholar]
- Dell’Osso, L. F. 1994. Evidence suggesting individual ocular motor control of each eye (muscle). Journal of Vestibular Research 4: 335–345. [Google Scholar] [PubMed]
- Dell’Osso, L. F. 1995. The analysis of ocular motor dysfunction: Insights into disease processes and normal function. Institute of Electrical and Electronics Engineers/Engineering in Medicine and Biology Special Issue: Advances in Vision Research 14: 22–29. [Google Scholar]
- Dell’Osso, L. F. 2006. Biologically relevant models of infantile nystagmus syndrome: the requirement for behavioral ocular motor system models. Seminars in Ophthalmology 21, 2: 71–77. [Google Scholar]
- Dell’Osso, L. F., and R. B. Daroff. 1975. Congenital nystagmus waveforms and foveation strategy. Documenta Ophthalmologica 39: 155–182. [Google Scholar]
- Dell’Osso, L. F., R. W. Hertle, R. W. Williams, and J. B. Jacobs. 1999. A new surgery for congenital nystagmus: effects of tenotomy on an achiasmatic canine and the role of extraocular proprioception. Journal of the American Academy of Pediatric Ophthalmology and Strabismus 3: 166–182. [Google Scholar]
- Dell’Osso, L. F., D. A. Robinson, and R. B. Daroff. 1974. Optokinetic asymmetry in internuclear ophthalmoplegia. Archives of Neurology 31: 138–139. [Google Scholar]
- Dell’Osso, L. F., J. Van der Steen, R. M. Steinman, and H. Collewijn. 1992. Foveation dynamics in congenital nystagmus II: Smooth pursuit. Documenta Ophthalmologica 79: 25–49. [Google Scholar] [CrossRef]
- Dell’Osso, L. F., Z. Wang, R. J. Leigh, and J. B. Jacobs. 2006. Hypothetical explanation for the role of proprioception in the damping of infantile nystagmus by tenotomy surgery: The small-signal gain hypothesis. In Proceedngs of the World Multiconference on Systemics, Cybernetics and Informatics 2006 Conference. Edited by N. Callaos. Orlando, FL: pp. 39–44. [Google Scholar]
- Dell’Osso, L. F., and R. W. Williams. 1995. Ocular motor abnormalities in achiasmatic mutant Belgian sheepdogs: Unyoked eye movements in a mammal. Vision Research 35: 109–116. [Google Scholar] [CrossRef] [PubMed]
- Dell’Osso, L. F., R. W. Williams, J. B. Jacobs, and D. M. Erchul. 1998. The congenital and see-saw nystagmus in the prototypical achiasma of canines: comparison to the human achiasmatic prototype. Vision Research 38: 1629–1641. [Google Scholar] [CrossRef] [PubMed]
- Fleuriet, J., S. Hugues, L. Perrinet, and L. Goffart. 2011. Saccadic foveation of a moving visual target in the rhesus monkey. Journal of Neurophysiology 105, 2: 883–895. [Google Scholar] [CrossRef] [PubMed]
- Hamker, F. H., M. Zirnsak, and M. Lappe. 2008. About the influence of postsaccadic mechanisms for visual stability on peri-saccadic compression of object location. Journal of Vision 8, 14: 1 1–13. [Google Scholar] [CrossRef]
- Jacobs, J. B., and L. F. Dell’Osso. 2004. Congenital nystagmus: hypothesis for its genesis and complex waveforms within a behavioral ocular motor system model. Journal of Vision 4, 7: 604–625. [Google Scholar] [CrossRef]
- Jacobs, J. B., L. F. Dell’Osso, Z. I. Wang, G. M. Acland, and J. Bennett. 2009. Using the NAFX to measure the effectiveness over time of gene therapy in canine LCA. Investigative Ophthalmology and Visual Science 50, 10: 4685–4692. [Google Scholar] [CrossRef]
- Krauzlis, R. J. 2004. Recasting the smooth pursuit eye movement system. Journal of Neurophysiology 91: 591–603. [Google Scholar] [CrossRef]
- Krauzlis, R. J. 2005. The control of voluntary eye movements: new perspectives. Neuroscientist 11, 2: 124–137. [Google Scholar] [CrossRef]
- Kurzan, R., and U. Büttner. 1989. Smooth pursuit mechanisms in congenital nystagmus. Neuro ophthalmology 9: 313–325. [Google Scholar] [CrossRef]
- Latour, P. L. 1962. Visual threshold during eye movements. Vision Research 2: 261–262. [Google Scholar] [CrossRef]
- Lisberger, S. G. 1998. Postsaccadic enhancement of initiation of smooth pursuit eye movements in monkeys. Journal of Neurophysiology 79, 4: 1918–1930. [Google Scholar] [PubMed]
- Liston, D., and R. J. Krauzlis. 2005. Shared decision signal explains performance and timing of pursuit and saccadic eye movements. Journal of Vision 5, 9: 678–689. [Google Scholar] [PubMed]
- Orban de Xivry, J. J., and P. Lefevre. 2007. Saccades and pursuit: two outcomes of a single sensorimotor process. Journal of Physiology 584, Pt 1: 11–23. [Google Scholar]
- Rashbass, C. 1961. The relationship between saccadic and smooth tracking eye movements. Journal of Physiology (London) 159: 326–338. [Google Scholar] [CrossRef]
- Volkmann, F. C. 1962. Vision during voluntary saccadic eye movement. Journal of the Optical Society of America 52: 571–578. [Google Scholar]
- Volkmann, F. C., A. M. L. Schick, and L. A. Riggs. 1969. Time course of visual inhibition during voluntary saccades. Journal of the Optical Society of America 58: 562–569. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z. I., and L. F. Dell’Osso. 2007. Being “slow to see” is a dynamic visual function consequence of infantile nystagmus syndrome: Model predictions and patient data identify stimulus timing as its cause. Vision Research 47, 11: 1550–1560. [Google Scholar]
- Wang, Z. I., and L. F. Dell’Osso. 2009. Factors influencing pursuit ability in infantile nystagmus syndrome: Target timing and foveation capability. Vision Research 49: 182–189. [Google Scholar]
- Wang, Z. I., and L. F. Dell’Osso. 2011a. Smooth-pursuit changes after the tenotomy and reattachment procedure for infantile nystagmus syndrome: model predictions and patient data. Journal of Pediatric Ophthalmology and Strabismus 48: 1–8. [Google Scholar]
- Wang, Z. I., and L. F. Dell’Osso. 2011b. A unifying model-based hypothesis for the diverse waveforms of infantile nystagmus syndrome. Journal of Eye Movement Research 4, 1: 1, 1–18. [Google Scholar] [CrossRef]
- Zhou, W., and W. M. King. 1997a. Binocular eye movements not coordinated during REM sleep. Experimental Brain Research 117: 153–160. [Google Scholar] [PubMed]
- Zhou, W., and W. M. King. 1997b. Monocular rather than conjugate saccade burst generators in paramedian pontine reticular formation (PPRF). Society of Neuroscience Abstracts 23: 7. [Google Scholar]
- Zhou, W., and W. M. King. 1998. Premotor commands encode monocular eye movements. Nature 393: 692–695. [Google Scholar] [PubMed]
- Zuber, B. L., and L. Stark. 1966. Saccadic suppression: elevation of visual thresholds associated with saccadic eye movements. Experimental Neurology 16: 65–79. [Google Scholar]






 |
 |
Copyright © 2013. This article is licensed under a Creative Commons Attribution 4.0 International License.
Share and Cite
Dell’Osso, L.F.; Jacobs, J.B. Normal Pursuit-System Limitations—First Discovered in Infantile Nystagmus Syndrome. J. Eye Mov. Res. 2013, 6, 1-24. https://doi.org/10.16910/jemr.6.1.2
Dell’Osso LF, Jacobs JB. Normal Pursuit-System Limitations—First Discovered in Infantile Nystagmus Syndrome. Journal of Eye Movement Research. 2013; 6(1):1-24. https://doi.org/10.16910/jemr.6.1.2
Chicago/Turabian StyleDell’Osso, Louis F., and Jonathan B. Jacobs. 2013. "Normal Pursuit-System Limitations—First Discovered in Infantile Nystagmus Syndrome" Journal of Eye Movement Research 6, no. 1: 1-24. https://doi.org/10.16910/jemr.6.1.2
APA StyleDell’Osso, L. F., & Jacobs, J. B. (2013). Normal Pursuit-System Limitations—First Discovered in Infantile Nystagmus Syndrome. Journal of Eye Movement Research, 6(1), 1-24. https://doi.org/10.16910/jemr.6.1.2



