The Importance of Uncertainty Analysis and Traceable Measurements in Routine Quantitative 90Y-PET Molecular Radiotherapy: A Multicenter Experience
Abstract
1. Introduction
- Three PET scanners available at three Italian centers were calibrated with the aim to recover the 90Y activity from 90Y-PET images. For all the PET scanners, the calibration procedure was performed using a water phantom uniformly filled with a known concentration of 18F-FDG to correlate the count rate to the phantom activity (Section 2.1).
- After the calibration, for each scanner, a uniform cylindrical phantom containing 90Y was prepared with the aim to assess the quantitative accuracy of the scanner in reference conditions. Each uniform phantom was prepared following a traceable calibration methodology (Section 2.2). For the first two centers (GH, IRST), accurate activity concentration measurements of a stock 90Y radionuclidic solution were performed directly at the hospital using the ENEA-INMRI portable Triple-to-Double-Coincidence Ratio (TDCR). For one center (SMG), the activity concentration of the stock solution was measured using the on-site dose calibrator, traceable to a primary standard (Section 2.3).
- Finally, the ability of each scanner to recover the activity concentration on the uniform phantom was assessed taking into account all possible correction factors (Section 2.4) and sources of uncertainty in the quantification processes (Section 2.5). The two TOF PET scanners available at the GH and IRST sites directly supported 90Y as a viable PET radionuclide, while 90Y was not present in the list of radionuclides accepted by the PET scanner available at the SMG center.
- Ultimately, a framework is proposed for modeling the uncertainty in the quantification processes, along with an estimation of the uncertainty achievable in clinical conditions (Section 4).
- Siemens Biograph mCT Flow: TOF PET/CT scanner (Siemens Medical Solutions, USA) available at IRCCS—Istituto Scientifico Romagnolo per lo Studio dei Tumori (IRST) “Dino Amadori” (Meldola, Italy);
- Siemens Biograph mCT: TOF PET/CT scanner (Siemens Medical Solutions USA) available at Fondazione Policlinino Universitario Agostino Gemelli IRCCS (Rome, Italy)
- GE Discovery DST BGO scanner (General Electric, Milwaukee, WI, USA) available at Ospedale Santa Maria Goretti (Latina, Italy)
2. Materials and Methods
2.1. Absolute Scanner Calibration
- is the radionuclide activity used in the calibration procedure,
- is the volume of the phantom used in the calibration procedure,
- is the acquisition start time,
- is the reference calibration time,
- is the radionuclide physical half-life, and
- is the acquisition duration.
2.2. Preparation of a Traceable Phantom for 90Y-PET Studies
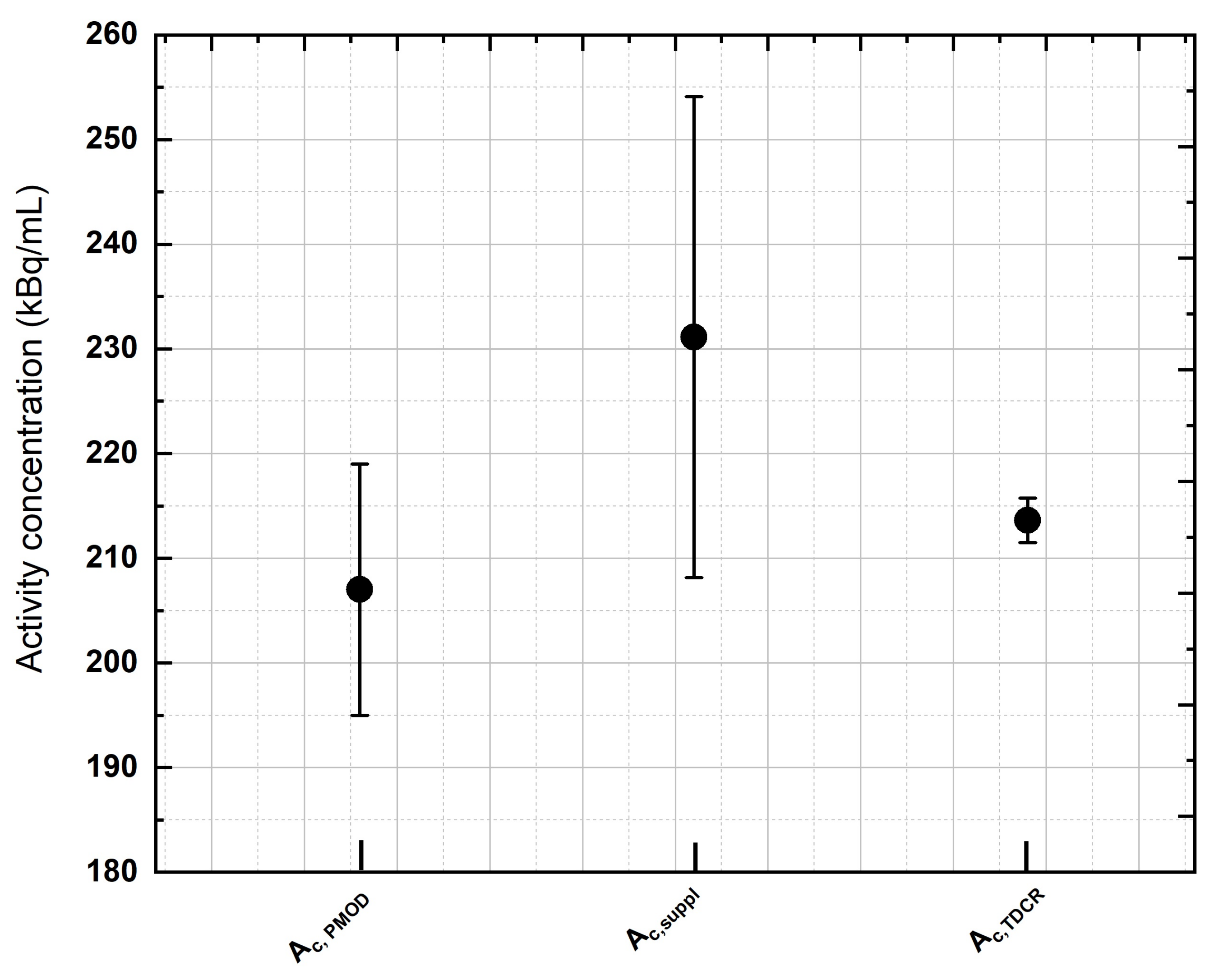
2.3. 90Y Activity Concentration Measurements
2.4. Quantitative Imaging on 90Y Clinical Acquisitions
2.4.1. Half-Life Correction
2.4.2. Branching Ratio Correction
2.4.3. 90Y Quantification
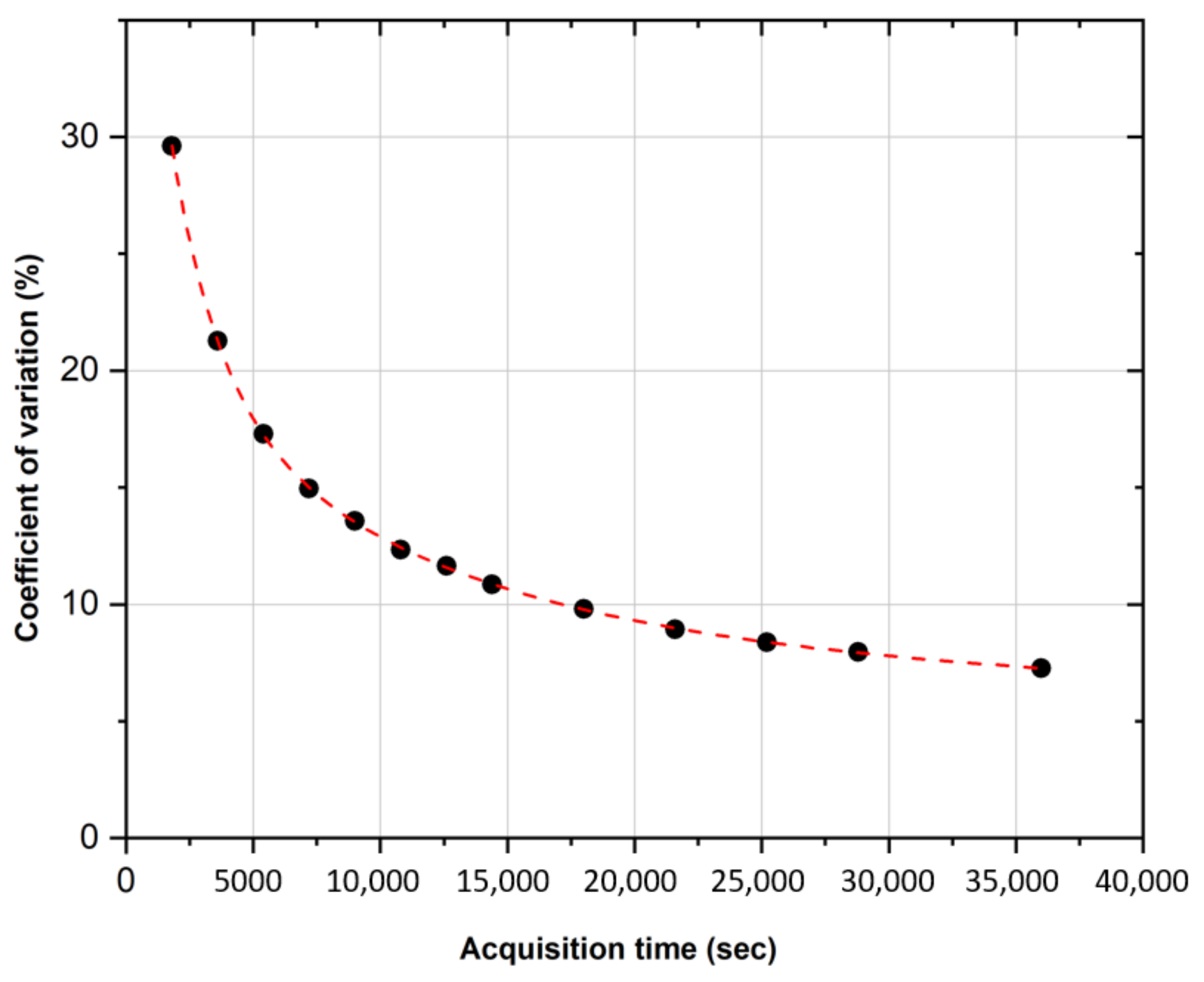
2.5. Evaluation of Uncertainty
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| MRT | Molecular Radiation Therapy |
| MetroMRT | Metrology for Molecular Radiation Therapy |
| MRTDosimetry | Molecular Radiation Therapy Dosimetry |
| TARE | Transarterial Radioembolization |
| GUM | Guide to the Expression of Uncertainty in Measurements |
| EANM | European Association of Nuclear Medicine |
| TDCR | Triple-to-Double-Coincidence Ratio |
| DTPA | Diethylenetriaminepentaacetic Acid |
| PET | Positron Emission Tomography |
| TOF | Time Of Flight |
References
- Sgouros, G.; Bodei, L.; McDevitt, M.R.; Nedrow, J.R. Radiopharmaceutical therapy in cancer: Clinical advances and challenges. Nat. Rev. Drug Discov. 2020, 19, 589–608. [Google Scholar] [CrossRef]
- Gear, J.I.; Cox, M.G.; Gustafsson, J.; Gleisner, K.S.; Murray, I.; Glatting, G.; Konijnenberg, M. EANM practical guidance on uncertainty analysis for molecular ra-diotherapy absorbed dose calculations. Eur. J. Nucl. Med. Mol. Imaging 2018, 45, 2456–2474. [Google Scholar] [CrossRef]
- D’Arienzo, M.; Cox, M. Uncertainty analysis in the calibration of an emission tomography system for quantitative imaging. Comput. Math. Methods Med. 2017, 2017, 9830386. [Google Scholar] [CrossRef]
- D’Arienzo, M.; Capogni, M.; Smyth, V.; Cox, M.; Johansson, L.; Solc, J.; Bobin, C.; Rabus, H.; Joulaeizadeh, L. Metrological Issues in Molecular Radiotherapy. EPJ Web Conf. 2014, 77, 22. [Google Scholar] [CrossRef]
- Tran-Gia, J.; Salas-Ramirez, M.; Lassmann, M. What you see is not what you get: On the accuracy of voxel-based dosimetry in molecular radiotherapy. J. Nucl. Med. 2020, 61, 1178–1186. [Google Scholar] [CrossRef]
- MetroMRT—Metrology for Molecular Radiation Therapy. Available online: http://projects.npl.co.uk/metromrt/ (accessed on 11 July 2023).
- MRTDosimetry. Available online: https://osf.io/69nge/ (accessed on 11 July 2023).
- Pasciak, A.S.; Bourgeois, A.C.; McKinney, J.M.; Chang, T.T.; Osborne, D.R.; Acuff, S.N.; Bradley, Y.C. Radioembolization and the dynamic role of 90Y-PET/CT. Front. Oncol. 2014, 4, 38. [Google Scholar] [CrossRef]
- Ungania, S.; D’Arienzo, M.; Mezzenga, E.; Pizzi, G.; Vallati, G.; Ianiro, A.; Rea, S.; Sciuto, R.; Soriani, A.; Strigari, L. A Workflow for Dosimetry of 90Y Radio-embolization Based on Quantitative 99mTc-MAA SPECT/CT Imaging and a 3D-Printed Phantom. Appl. Sci. 2022, 12, 10541. [Google Scholar] [CrossRef]
- Willowson, K.P.; Tapner, M.; QUEST Investigator Team; Bailey, D.L. A multicenter comparison of quantitative 90 Y-PET/CT for dosimetric purposes after radioembolization with resin microspheres: The QUEST phantom study. Eur. J. Nucl. Med. Mol. Imaging 2015, 42, 1202–1222. [Google Scholar] [CrossRef]
- Gates, V.L.; Esmail, A.A.; Marshall, K.; Spies, S.; Salem, R. Internal pair production of 90Y permits hepatic localization of microspheres using routine PET: Proof of concept. J. Nucl. Med. 2011, 52, 72–76. [Google Scholar] [CrossRef]
- D’Arienzo, M. Emission of β+ Particles Via Internal Pair Production in the 0+ – 0+ Transition of 90Zr: Historical Background and Current Applications in Nuclear Medicine Imaging. Atoms 2013, 1, 2–12. [Google Scholar] [CrossRef]
- Spreafico, C.; Maccauro, M.; Mazzaferro, V.; Chiesa, C. The dosimetric importance of the number of 90 Y microspheres in liver transarterial radioembolization (TARE). Eur. J. Nucl. Med. Mol. Imaging 2014, 41, 634–638. [Google Scholar] [CrossRef]
- Hardy-Abeloos, C.; Lazarev, S.; Ru, M.; Kim, E.; Fischman, A.; Moshier, E.; Rosenzweig, K.; Buckstein, M. Safety and efficacy of liver stereotactic body radiation therapy for hepatocellular carcinoma after segmental transarterial radioembolization. Int. J. Radiat. Oncol. Biol. Phys. 2019, 105, 968–976. [Google Scholar] [CrossRef]
- Tomozawa, Y.; Jahangiri, Y.; Pathak, P.; Kolbeck, K.J.; Schenning, R.C.; Kaufman, J.A.; Farsad, K. Long-term toxicity after transarterial radioembolization with yttrium-90 using resin microspheres for neuroendocrine tumor liver metastases. J. Vasc. Interv. Radiol. 2018, 29, 858–865. [Google Scholar] [CrossRef]
- Milano, A.; Gil, A.V.; Fabrizi, E.; Cremonesi, M.; Veronese, I.; Gallo, S.; Lanconelli, N.; Faccini, R.; Pacilio, M. In Silico Validation of MCID Platform for Monte Carlo-Based Voxel Dosimetry Applied to 90Y-Radioembolization of Liver Malignancies. Appl. Sci. 2021, 11, 1939. [Google Scholar] [CrossRef]
- Pistone, D.; Italiano, A.; Auditore, L.; Mandaglio, G.; Campenní, A.; Baldari, S.; Amato, E. Relevance of artefacts in99mTc-MAA SPECT scans on pre-therapy pa-tient-specific90Y TARE internal dosimetry: A GATE Monte Carlo study. Phys. Med. Biol. 2022, 67, 115002. [Google Scholar] [CrossRef]
- JCGM Joint Committee for Guides in Metrology. Evaluation of Measurement Data—Guide to the Expression of Uncertainty in Measurement; JCM 100:2008. Available online: https://www.bipm.org/documents/20126/2071204/JCGM_100_2008_E.pdf (accessed on 11 July 2023).
- Van der Veen, A.M.; Cox, M.G.; Possolo, A. GUM guidance on developing and using measurement models. Accredit. Qual. Assur. 2022, 27, 295–297. [Google Scholar] [CrossRef]
- Cherry, S.; Sorenson, J.; Phelps, M. Physics in Nuclear Medicine; Elsevier/Saunders: Philadelphia, PA, USA, 2012. [Google Scholar]
- National Electrical Manufacturers Association. Performance Measurements of Gamma Cameras; NEMA NU 1-2007; National Electrical Manufacturers Association: Rosslyn, VA, USA, 2007. [Google Scholar]
- Capogni, M.; De Felice, P.A. Prototype of a portable TDCR system at ENEA. Appl. Radiat. Isot. 2014, 93, 45–51. [Google Scholar] [CrossRef]
- Goedicke, A.; Berker, Y.; Verburg, F.; Behrendt, F.; Winz, O.; Mottaghy, F. Study-Parameter Impact in Quantitative 90-Yttrium PET Imaging for Radioembolization Treatment Monitoring and Dosimetry. IEEE Trans. Med. Imaging 2013, 32, 485–492. [Google Scholar] [CrossRef]
- Gadd, R.; Baker, M.; Nijran, K.S.; Owens, S.; Thomas, W.; Woods, M.J.; Zananiri, F. Measurement Good Practice Guide No. 93: Protocol for Establishing and Maintaining the Calibration of Medical Radionuclide Calibrators and Their Quality Control; National Physical Laboratory: Teddington, UK, 2006. [Google Scholar]
- Dryák, P.; Šolc, J. Measurement of the branching ratio related to the internal pair production of Y-90. Appl. Radiat. Isot. 2020, 156, 108942. [Google Scholar] [CrossRef]
- Decay Data Evaluation Project (DDEP) Database. Available online: http://www.lnhb.fr/ddep_wg/ (accessed on 22 April 2023).
- Fenwick, A.J.; Wevrett, J.L.; Ferreira, K.M.; Denis-Bacelar, A.M.; Robinson, A.P. Quantitative imaging, dosimetry and metrology; Where do National Metrology Institutes fit in? Appl. Radiat. Isot. 2018, 134, 74–78. [Google Scholar] [CrossRef]
- Carlier, T.; Willowson, K.P.; Fourkal, E.; Bailey, D.L.; Doss, M.; Conti, M. (90)Y -PET imaging: Exploring limitations and accuracy under conditions of low counts and high random fraction. Med. Phys. 2015, 42, 4295–4309. [Google Scholar] [CrossRef]
- Strydhorst, J.; Carlier, T.; Dieudonné, A.; Conti, M.; Buvat, I. A gate evaluation of the sources of error in quantitative 90Y-PET. Med. Phys. 2016, 43, 5320. [Google Scholar] [CrossRef]
- Capotosti, A.; Moretti, R.; Milano, A.; Nardini, M.; Cusumano, D.; Annunziata, S.; Capogni, M.; D’Arienzo, M.; Placidi, L.; Indovina, L. Up-to-Date Optimization of the 90Y-PET/CT Reconstruction Protocol for Volumetric Quantification in Trans-Arterial Ra-dioEmbolization (TARE) Procedures in the Era of Theranostics. Appl. Sci. 2022, 12, 8418. [Google Scholar] [CrossRef]
- Tapp, K.N.; Lea, W.B.; Johnson, M.S.; Tann, M.; Fletcher, J.W.; Hutchins, G.D. The impact of image reconstruction bias on PET/CT 90Y dosimetry after radioembolization. J. Nucl. Med. Off. Publ. Soc. Nucl. Med. 2014, 55, 1452–1458. [Google Scholar] [CrossRef]
- Fourkal, E.; Veltchev, I.; Lin, M.; Koren, S.; Meyer, J.; Doss, M.; Yu, J.Q. 3D inpatient dose reconstruction from the PET-CT imaging of 90Y microspheres for metastatic cancer to the liver: Feasibility study. Med. Phys. 2013, 40, 081702. [Google Scholar] [CrossRef][Green Version]
- Sunderland, J.; Christian, P.; Kiss, T. (2015) PET/CT scanner validation for clinical trials-reasons for failure, recipes for success: The Clinical Trials Network (CTN) experience. J. Nucl. Med. 2015, 56, 1737. [Google Scholar]
- Park, M.A.; Mahmood, A.; Zimmerman, R.E.; Limpa-Amara, N.; Makrigiorgos, G.M.; Moore, S.C. Adsorption of metallic radionuclides on plastic phantom walls. Med. Phys. 2008, 35, 1606–1610. [Google Scholar] [CrossRef]
- Fenwick, A.; Baker, M.; Ferreira, K.; Keightley, J. Comparison of Y-90 Measurements in UK Hospitals, NPL Report IR 20. 2011. Available online: https://eprintspublications.npl.co.uk/5213/1/IR20.pdf (accessed on 11 July 2023).
- Ferreira, K.; Fenwick, A.; Arinc, A.; Johansson, L. Standardisation of 90Y and determination of calibration factors for 90Y microspheres (resin) for the NPL secondary ionisation chamber and a Capintec CRC-25R. Appl. Radiat. Isot. 2015, 109, 226–230. [Google Scholar]
- Kossert, K.; Bokeloh, K.; Ehlers, M.; Nähle, O.; Scheibe, O.; Schwarz, U.; Thieme, K. Comparison of 90Y activity measurements in nuclear medicine in Germany. Appl. Radiat. Isot. 2015, 109, 247–249. [Google Scholar] [CrossRef]
- Woods, M.; Munster, A.; Sephton, J.; Lucas, S.; Walsh, C. Calibration of the NPL secondary standard radionuclide calibrator for 32P, 89Sr and 90Y. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 1996, 369, 698–702. [Google Scholar] [CrossRef]
- AAPM Task Group 181. The Selection, Use, Calibration, and Quality Assurance of Radionuclide Calibrators Used in Nuclear Medicine (2012) Report of AAPM Task Group 181. Available online: https://www.aapm.org/pubs/reports/rpt_181.pdf (accessed on 11 July 2023).
- Zimmerman, B.; Ratel, G. Report of the CIPM Key Comparison CCRI(II)-K2 Y-90. Metrologia 42, 06001. 2005. Available online: https://iopscience.iop.org/article/10.1088/0026-1394/42/1A/06001/meta (accessed on 11 July 2023).
- Dezarn, W.; Kennedy, A. SU-FF-T-380: Significant differences exist across institutions in 90Y activities compared to reference standard. Med. Phys. 2007, 34, 2489. [Google Scholar] [CrossRef]
- Selwyn, R.G.; Nicles, R.J.; Thomadsen, B.R.; DeWerd, L.A.; Micka, J.A. A new internal pair production branching ratio of 90Y: The development of a non-destructive assay for 90Y and 90Sr. Appl. Radiat. Isot. 2007, 65, 318–327. [Google Scholar] [CrossRef] [PubMed]
- Pibida, L.; Zimmerman, B.E.; King, L.; Fitzgerald, R.; Bergeron, D.E.; Napoli, E.; Cessna, J.T. Determination of the internal pair production branching ratio of 90Y. Appl. Radiat. Isot. Incl. Data Instrum. Methods Use Agric. Ind. Med. 2020, 156, 108943. [Google Scholar] [CrossRef]

| Site | Scanner Model | 90Y-Supported | PET Calibration Source |
|---|---|---|---|
| Santa Maria Goretti Hospital (SMG), Latina | GE Discovery DST (General Electric, Milwaukee, WI, USA) | No | Cylindrical phantom, 18F solution (2% uncertainty, k = 1) |
| Gemelli Hospital (GH), Rome | TOF Siemens Biograph mCT (Siemens Medical Solutions USA) | Yes | Cylindrical phantom, 18F solution (1.7% uncertainty, k = 1) |
| IRST Tumor Center (IRST), Meldola | TOF Siemens Biograph mCT Flow (Siemens Medical Solutions USA) | Yes | Cylindrical phantom, 18F solution (1.7% uncertainty, k = 1) |
| Site | True 90Y Activity | Reconstruction Algorithm | Applied Corrections | CT Scan Parameters |
|---|---|---|---|---|
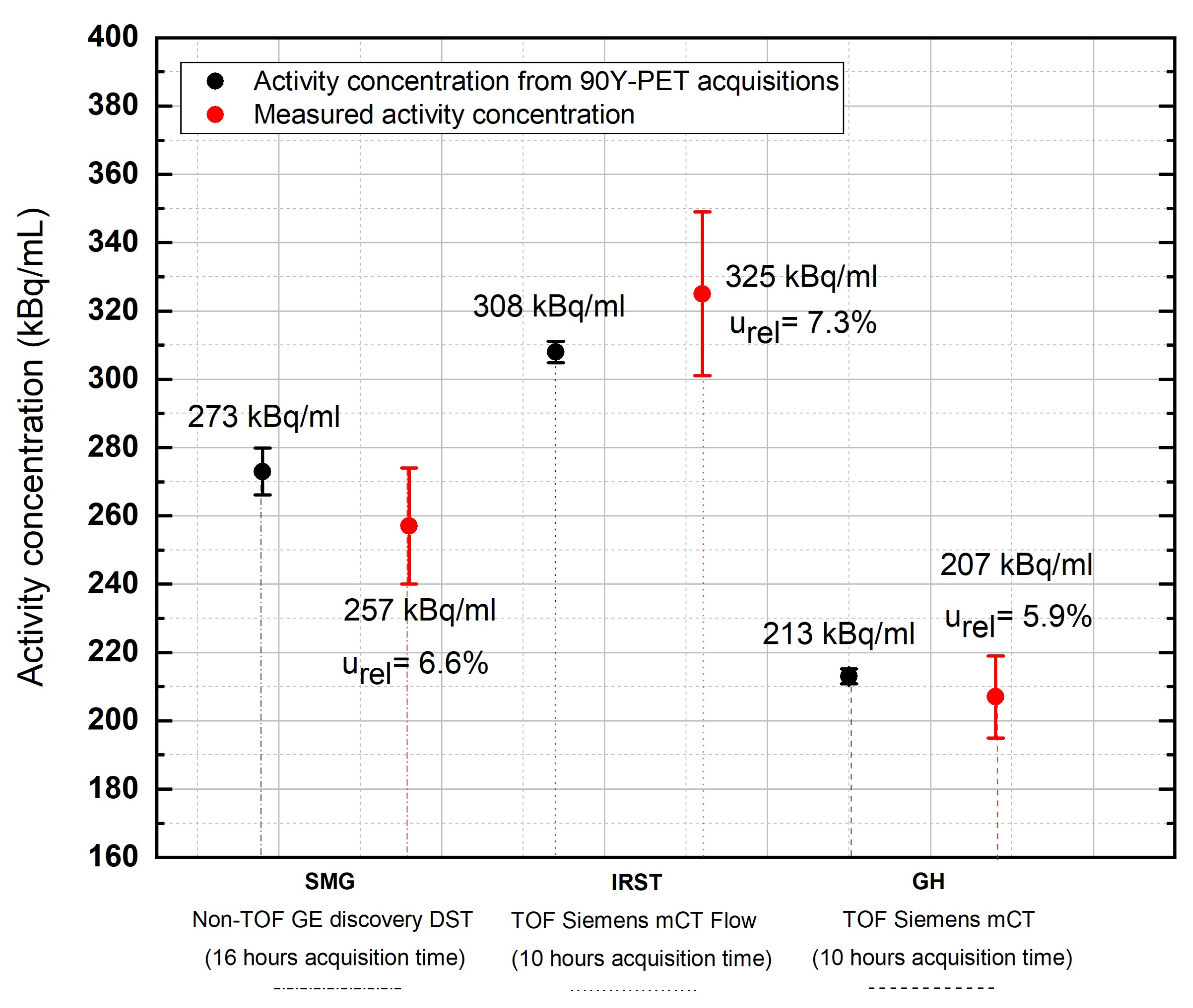
| SMG—Latina (GE Discovery DST), 16 h scan | 273 kBq/mL | 3D OSEM (15 subsets, 2 iterations) | Uniformity, attenuation scatter, decay, dead-time, and randomness | 120 kV, 60 mAs |
| GH—Rome (TOF Siemens Biograph mCT), 10 h scan | 213 kBq/mL | 3D TOF-OSEM (21 subsets, 1 iteration) | Uniformity, attenuation scatter, decay, dead-time, and randomness | 120 kV, 50 mAs |
| IRST—Meldola (TOF Siemens Biograph mCT Flow), 10 h scan | 308 kBq/mL | 3D TOF-OSEM (21 subsets, 1 iteration) | Uniformity, attenuation scatter, decay, dead-time, and randomness | 120 kV, 80 mAs |
| GE Discovery DST (SMG) | Siemens Biograph mCT Flow (IRST) | Siemens Biograph mCT (GH) | |
|---|---|---|---|
| True phantom | (273 ± 7) kBq/mL | (308 ± 3) kBq/mL | (213 ± 2) kBq/mL |
| Recovered | (257 ± 17) kBq/mL | (325 ± 24) kBq/mL | (207 ± 12) kBq/mL |
| Deviation | % | +5.5% | % |
| Uncertainty Component | GE Discovery DST (SMG) | Siemens Biograph mCT Flow (IRST) | Siemens Biograph mCT (GH) |
|---|---|---|---|
| 0.5% | 0.5% | 0.5% | |
| 6.2% | 7.0% | 5.5% | |
| 0.5% | 0.5% | 0.5% | |
| 2.0% | 1.7% | 1.7% | |
| 0.1% | 0.1% | 0.1% | |
| 0.1% | 0.1% | 0.1% | |
| 0.1% | 0.1% | 0.1% | |
| 0.1% | 0.1% | 0.1% | |
| 1.2% | 1.2% | 1.2% | |
| 0.2% | 0.2% | 0.2% | |
| Acquisition time | 16 h | 10 h | 10 h |
| 6.6% | 7.3% | 5.9% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
D’Arienzo, M.; Mezzenga, E.; Capotosti, A.; Bagni, O.; Filippi, L.; Capogni, M.; Indovina, L.; Sarnelli, A. The Importance of Uncertainty Analysis and Traceable Measurements in Routine Quantitative 90Y-PET Molecular Radiotherapy: A Multicenter Experience. Pharmaceuticals 2023, 16, 1142. https://doi.org/10.3390/ph16081142
D’Arienzo M, Mezzenga E, Capotosti A, Bagni O, Filippi L, Capogni M, Indovina L, Sarnelli A. The Importance of Uncertainty Analysis and Traceable Measurements in Routine Quantitative 90Y-PET Molecular Radiotherapy: A Multicenter Experience. Pharmaceuticals. 2023; 16(8):1142. https://doi.org/10.3390/ph16081142
Chicago/Turabian StyleD’Arienzo, Marco, Emilio Mezzenga, Amedeo Capotosti, Oreste Bagni, Luca Filippi, Marco Capogni, Luca Indovina, and Anna Sarnelli. 2023. "The Importance of Uncertainty Analysis and Traceable Measurements in Routine Quantitative 90Y-PET Molecular Radiotherapy: A Multicenter Experience" Pharmaceuticals 16, no. 8: 1142. https://doi.org/10.3390/ph16081142
APA StyleD’Arienzo, M., Mezzenga, E., Capotosti, A., Bagni, O., Filippi, L., Capogni, M., Indovina, L., & Sarnelli, A. (2023). The Importance of Uncertainty Analysis and Traceable Measurements in Routine Quantitative 90Y-PET Molecular Radiotherapy: A Multicenter Experience. Pharmaceuticals, 16(8), 1142. https://doi.org/10.3390/ph16081142







