An Experimental Study of the Pull-In Voltage in RF MEMS Switches Fabricated by Au Electroplating and Standard Wet Release: Considering the Bridge Geometry
Abstract
1. Introduction
2. Summarizing in Brief the Theoretical Background
3. Experimental
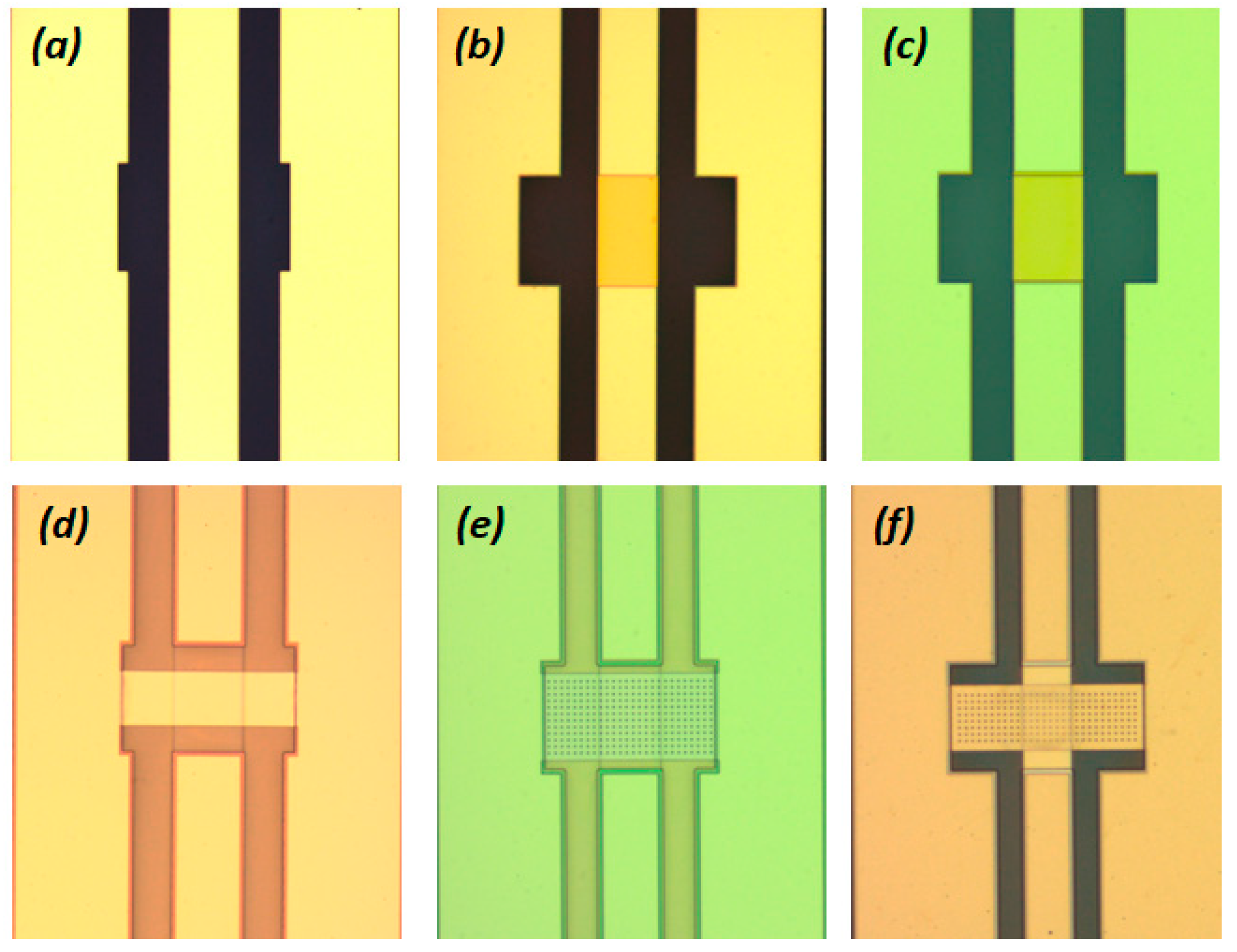
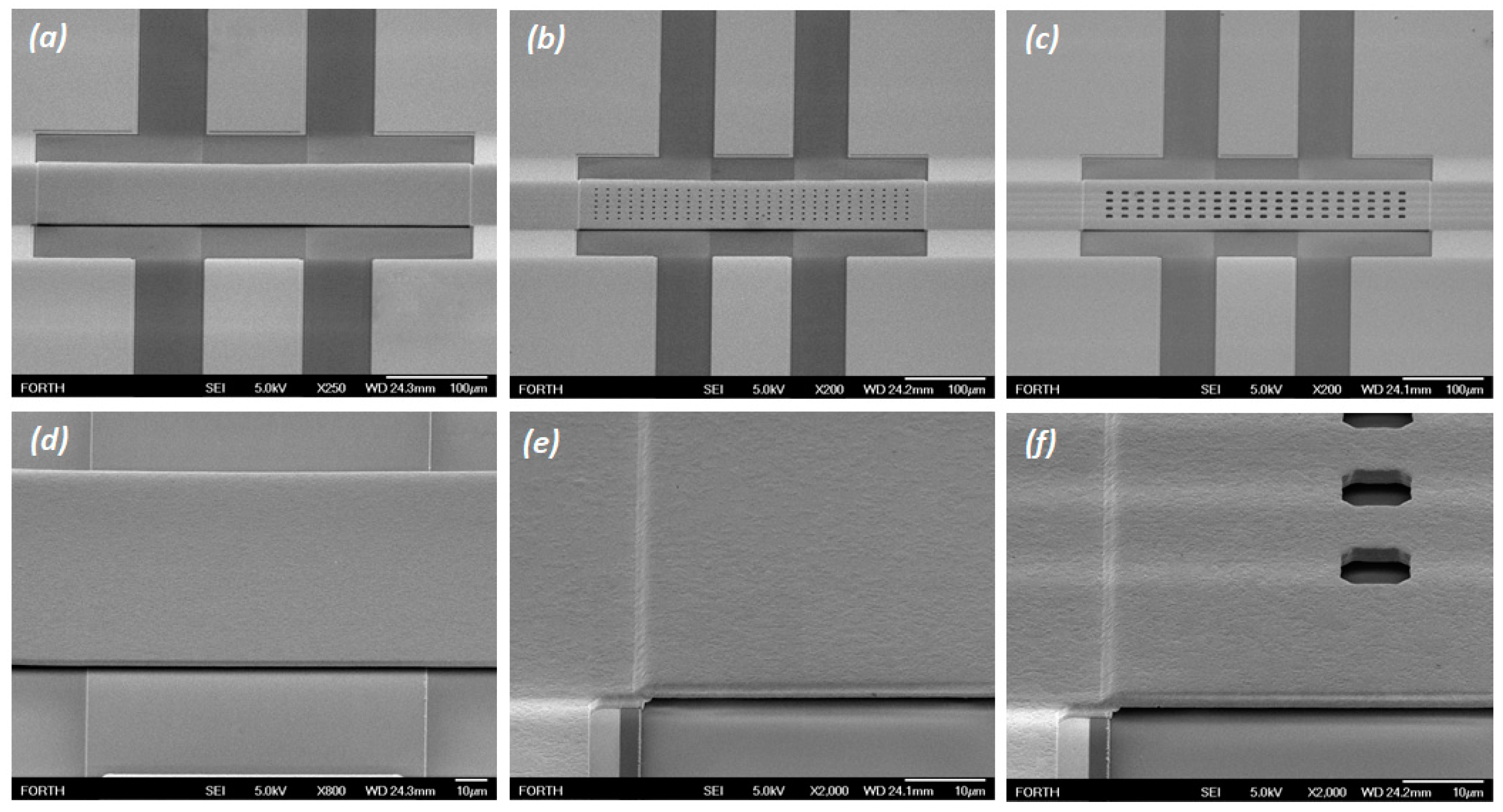
3.1. RF MEMS Fabrication
3.2. RF MEMS Preliminary Characterization
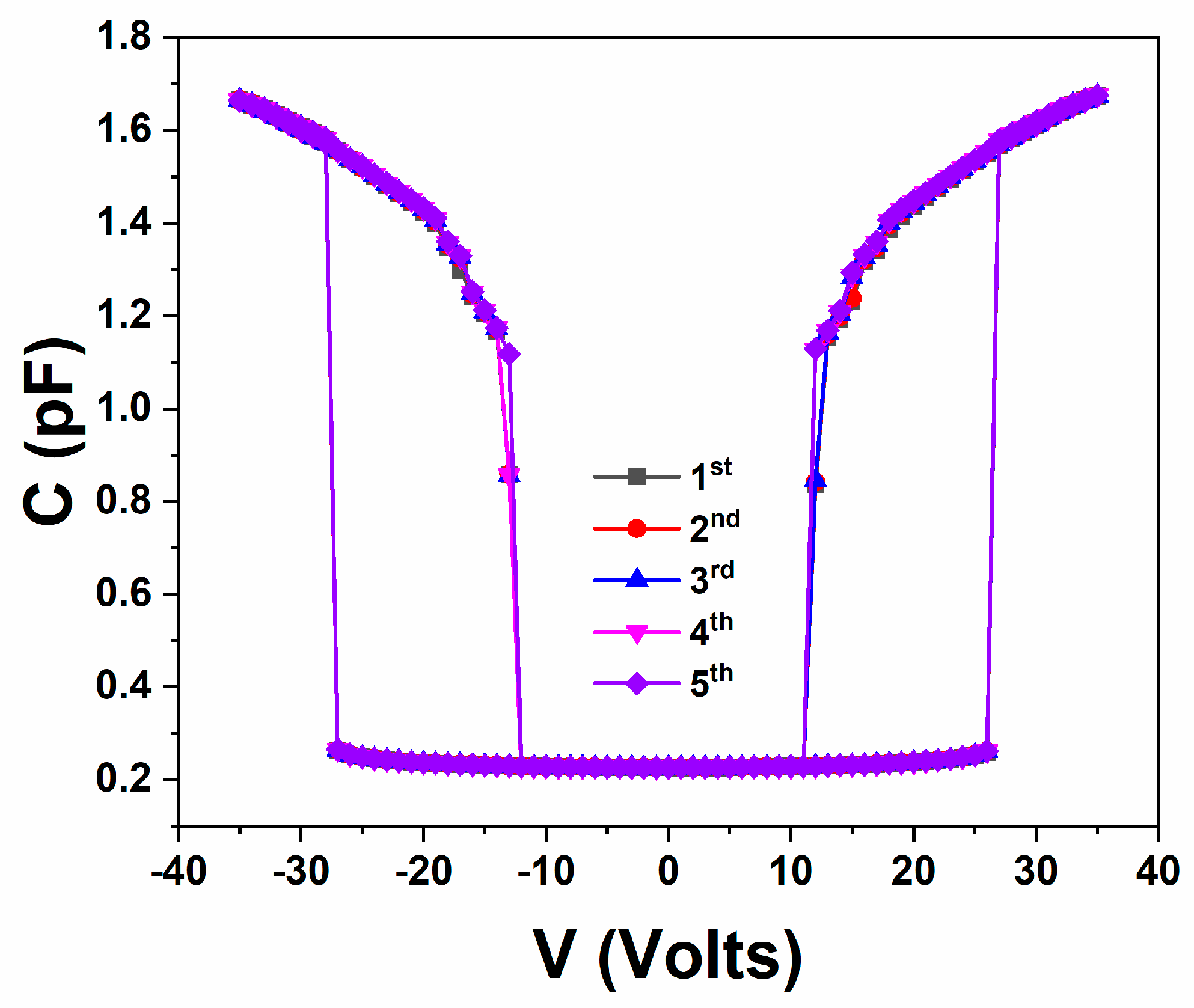
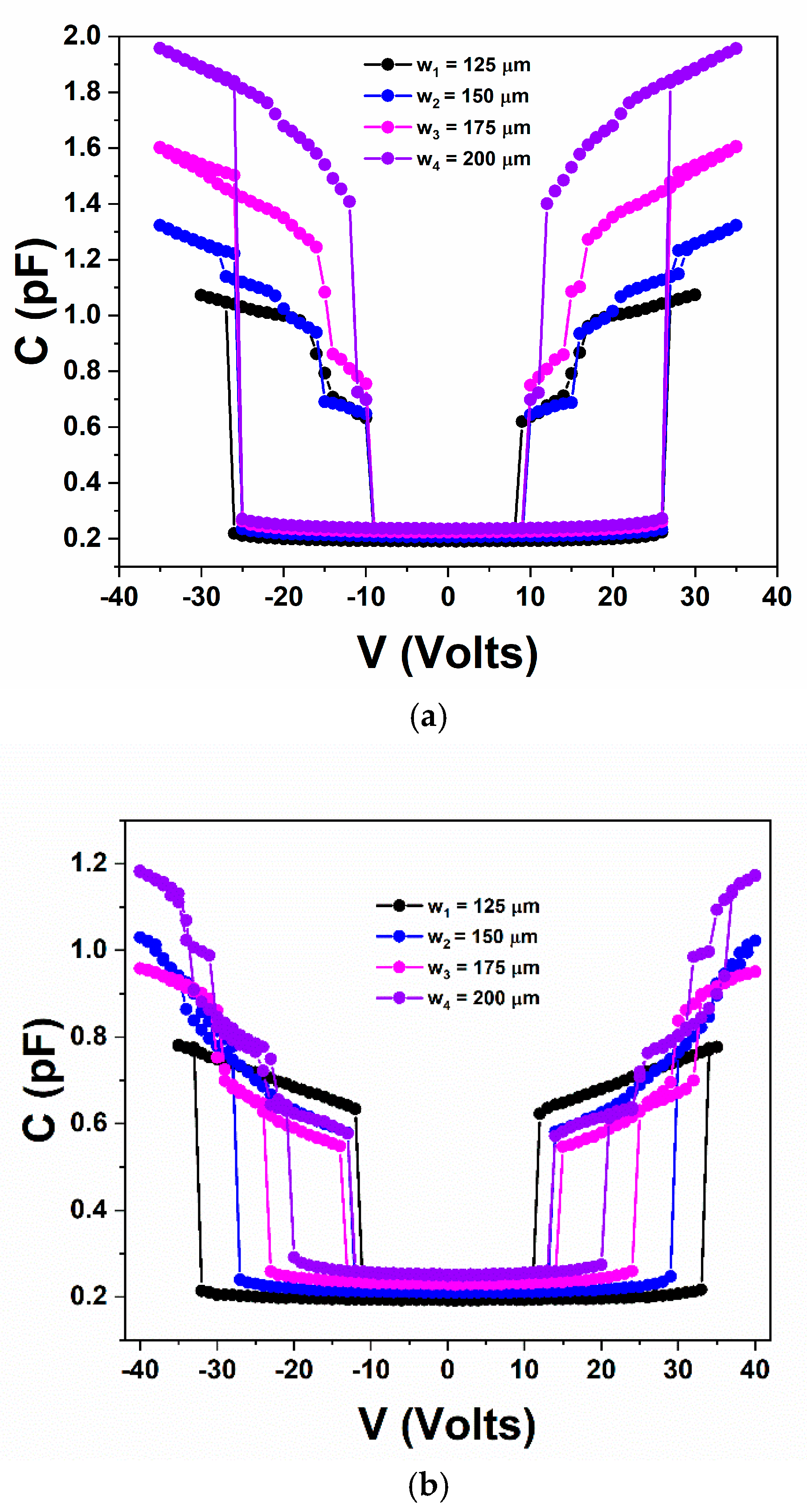
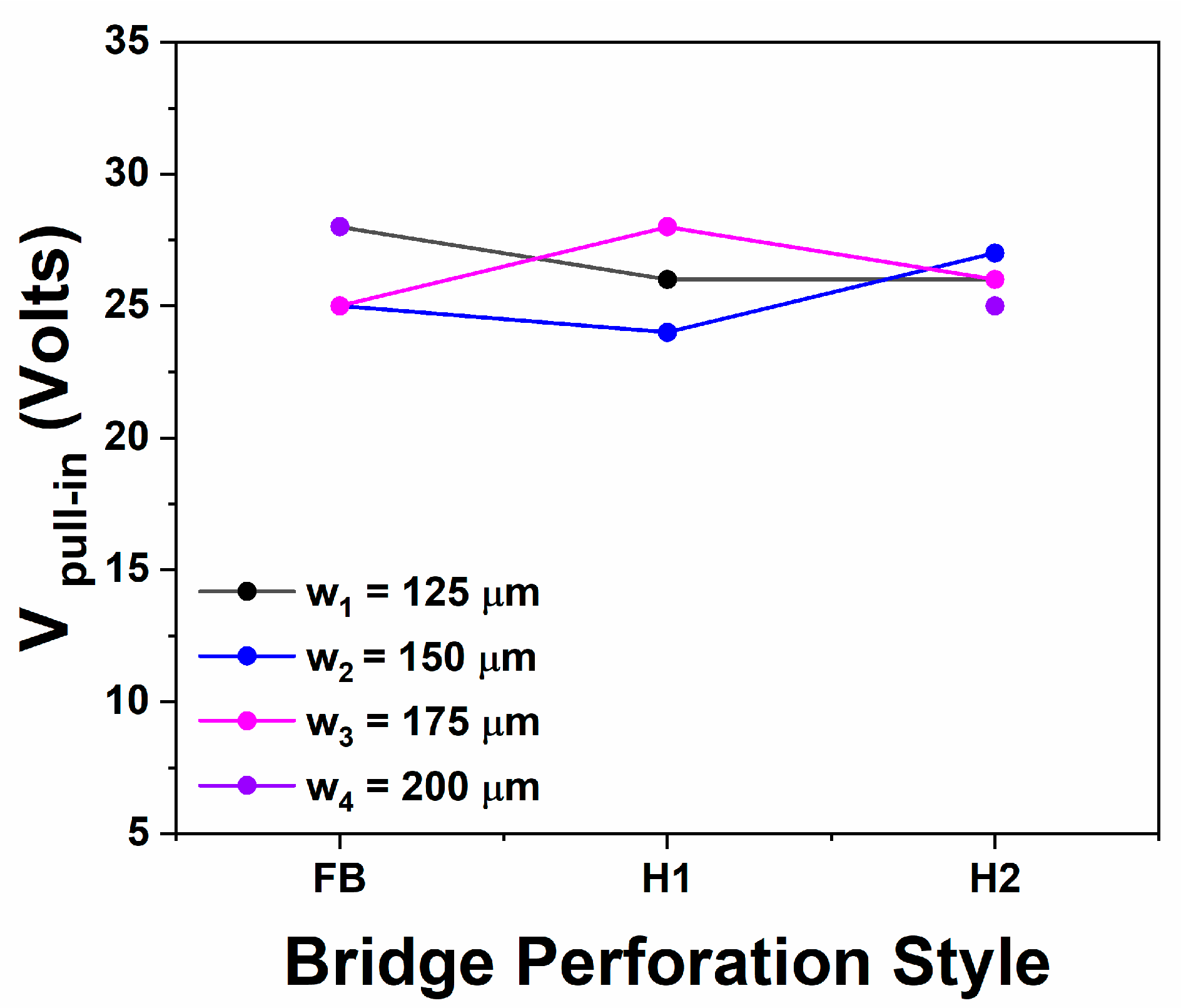
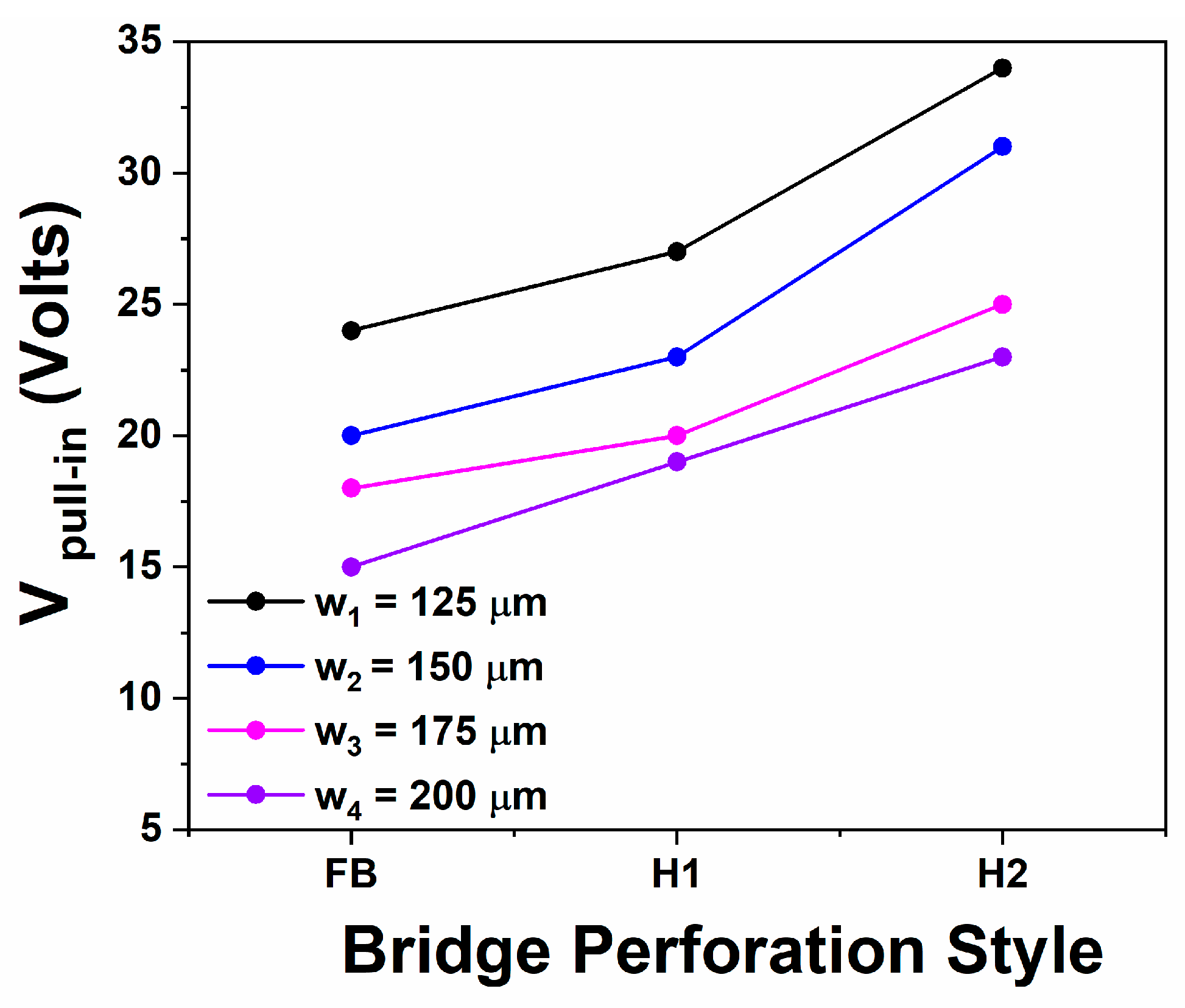
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ma, L.Y.; Soin, N.; Daut, M.H.M.; Hatta, S.F.W.M. Comprehensive Study on RF-MEMS Switches Used for 5G Scenario. IEEE Access 2019, 7, 107506–107522. [Google Scholar] [CrossRef]
- Iannacci, J.; Poor, H.V. Review and Perspectives of Micro/Nano Technologies as Key-Enablers of 6G. IEEE Access 2022, 10, 55428–55458. [Google Scholar] [CrossRef]
- Nan, X.; Jia, Q.; Lv, F.; Wang, X.; Wu, G.; Zhao, Y.; Qin, B.; Hao, J.; Cao, X.; Mei, S.; et al. A review of research on RF MEMS for metaverse interactions. J. Micromech. Microeng. 2024, 34, 083003. [Google Scholar] [CrossRef]
- Shao, B.; Lu, C.; Xiang, Y.; Li, F.; Song, M. Comprehensive Review of RF MEMS Switches in Satellite Communications. Sensors 2024, 24, 3135. [Google Scholar] [CrossRef]
- Van Caekenberghe, K. RF MEMS on the radar. IEEE Microw. Mag. 2009, 10, 99–116. [Google Scholar] [CrossRef]
- Rebeiz, G.M. RF MEMS: Theory, Design and Technology; John Willey & Sons: Hoboken, NJ, USA, 2003. [Google Scholar]
- Zhang, L.X.; Zhao, Y.P. Electromechanical model of RF MEMS switches. Microsyst. Technol. 2003, 9, 420–426. [Google Scholar] [CrossRef]
- Marcelli, R.; Lucibello, A.; De Angelis, G.; Proietti, E.; Comastri, D. Mechanical modelling of capacitive RF MEMS shunt switches. Microsyst. Technol. 2010, 16, 1057–1064. [Google Scholar] [CrossRef]
- Mulloni, V.; Solazzi, F.; Ficorella, F.; Collini, A.; Margesin, B. Influence of temperature on the actuation voltage of RF-MEMS switches. Microelectron. Reliab. 2013, 53, 706–711. [Google Scholar] [CrossRef]
- Chu, C.H.; Shih, W.P.; Chung, S.Y.; Tsai, H.C.; Shing, T.K.; Chang, P.Z. A low actuation voltage electrostatic actuator for RF MEMS switch applications. J. Micromech. Microeng. 2007, 17, 1649–1656. [Google Scholar] [CrossRef]
- Khodadady, K.; Ganji, B.A. Design and modeling of a novel RF MEMS series switch with low actuation voltage. Microsyst. Technol. 2016, 22, 2921–2929. [Google Scholar] [CrossRef]
- Kumar, P.A.; Rao, K.S.; Balaji, B.; Aditya, M.; Maity, N.P.; Maity, R.; Maity, S.; Sinawi, A.E.; Guha, K.; Sravani, K.G. Low Pull-in-Voltage RF-MEMS Shunt Switch for 5G Millimeter Wave Applications. Trans. Electr. Electron. Mater. 2021, 22, 821–832. [Google Scholar] [CrossRef]
- Deng, K.; Yang, F.; Wang, Y.; Lai, C.; Han, K. Design and Fabrication of a Ka Band RF MEMS Switch with High Capacitance Ratio and Low Actuation Voltage. Micromachines 2022, 13, 37. [Google Scholar] [CrossRef]
- Van Spengen, W.M.; Puers, R.; Mertens, R.; De Wolf, I. A comprehensive model to predict the charging and reliability of capacitive RF MEMS switches. J. Micromech. Microeng. 2004, 14, 514–521. [Google Scholar] [CrossRef]
- Van Spengen, W.M. Capacitive RF MEMS switch dielectric charging and reliability: A critical review with recommendations. J. Micromech. Microeng. 2012, 22, 074001. [Google Scholar] [CrossRef]
- Koutsoureli, M.; Birbiliotis, D.; Michalas, L.; Papaioannou, G. Dielectric charging in MEMS capacitive switches a persisting reliability issue, available models and assessment methods. In Proceedings of the 16th Mediterranean Microwave Symposium (MMS), Abu Dhabi, United Arab Emirates, 14–16 November 2016. [Google Scholar]
- Yuan, X.; Peng, Z.; Hwang, J.C.M.; Forehand, D.; Goldsmith, C.L. Acceleration of Dielectric Charging in RF MEMS Capacitive Switches. IEEE Trans. Dev. Mater. Reliabil. 2006, 6, 556–563. [Google Scholar] [CrossRef]
- Matmat, M.; Coccetti, F.; Marty, A.; Plana, R.; Escriba, C.; Fourniols, J.Y.; Esteve, D. Capacitive RF MEMS analytical predictive reliability and lifetime characterization. Microelectr. Reliab. 2009, 49, 1304–1308. [Google Scholar] [CrossRef]
- Koutsoureli, M.; Michalas, L.; Papaioannou, G. Charge collection mechanism in MEMS capacitive switches. In Proceedings of the IEEE International Reliability Physics Symposium (IRPS), Anaheim, CA, USA, 15–19 April 2012; pp. ME.2.1–ME.2.5. [Google Scholar]
- Michalas, L.; Koutsoureli, M.; Saada, S.; Mer–Calfati, C.; Leuliet, A.; Martins, P.; Bansropun, S.; Papaioannou, G.; Bergonzo, P.; Ziaei, A. Electrical assessment of diamond MIM capacitors and modeling of MEMS capacitive switch discharging. J. Micromech. Microeng. 2014, 24, 115017. [Google Scholar] [CrossRef]
- Pillans, B.; Kleber, J.; Goldsmith, C.; Eberly, M. RF power handling of capacitive RF MEMS devices. In Proceedings of the IEEE MTT-S International Microwave Symposium Digest, Seattle, WA, USA, 2–7 June 2002; Volume 1, pp. 329–332. [Google Scholar]
- Reid, J.R.; Starman, L.A.; Webster, R.T. RF actuation of capacitive MEMS switches. In Proceedings of the IEEE MTT-S International Microwave Symposium Digest, Philadelphia, PA, USA, 8–13 June 2003; Volume 3, pp. 1919–1922. [Google Scholar]
- Garg, A.; Venkattraman, A.; Kovacs, A.; Alexeenko, A.; Peroulis, D. Direct measurement of field emission current in E-static MEMS structures. In Proceedings of the 2011 IEEE 24th International Conference on Micro Electro Mechanical Systems, Cancun, Mexico, 23–27 January 2011; pp. 412–415. [Google Scholar]
- Venkattraman, A.; Garg, A.; Peroulis, D.; Alexeenko, A. Direct measurements and numerical simulations of gas charging in microelectromechanical system capacitive switches. Appl. Phys. Lett. 2012, 100, 083503. [Google Scholar] [CrossRef]
- Michalas, L.; Garg, A.; Venkattraman, A.; Koutsoureli, M.; Alexeenko, A.; Peroulis, D.; Papaioannou, G. A study of field emission process in electrostatically actuated MEMS switches. Microelectron. Reliab. 2012, 52, 2267–2271. [Google Scholar] [CrossRef]
- Michalas, L.; Koutsoureli, M.; Papaioannou, G. Probing contactless injection dielectric charging in RF MEMS capacitive switches. Electron. Lett. 2014, 50, 766–768. [Google Scholar] [CrossRef]
- Blondy, P.; Peroulis, D. Handling RF Power: The Latest Advances in RF-MEMS Tunable Filters. IEEE Microw. Mag. 2013, 14, 24–38. [Google Scholar] [CrossRef]
- Mulloni, V.; Giacomozzi, F.; Margesin, B. Controlling stress and stress gradient during the release process in gold suspended micro-structures. Sens. Actuators A 2010, 162, 93–99. [Google Scholar] [CrossRef]
- Sharma, A.; Bansal, D.; Kumar, A.; Kumar, D.; Rangra, K. Residual stress control during the growth and release process in gold suspended microstructures. In Proceedings of the Micromachining and Microfabrication Process Technology, XIX, San Francisco, CA, USA, 1–6 February 2014; Volume 8973. [Google Scholar]
- Mulloni, V.; Colpo, S.; Faes, A.; Margesin, B. A simple analytical method for residual stress measurement on suspended MEM structures using surface profilometry. J. Micromech. Microeng. 2013, 23, 025025. [Google Scholar] [CrossRef]
- Somà, A.; Saleem, M.M. Modeling and experimental verification of thermally induced residual stress in RF-MEMS. J. Micromech. Microeng. 2015, 25, 055007. [Google Scholar] [CrossRef]
- Dutta, S.; Imran, M.; Pal, R.; Jain, K.K.; Chatterjee, R. Effect of residual stress on RF MEMS switch. Microsyst. Technol. 2011, 17, 1739–1745. [Google Scholar] [CrossRef]
- Bansal, D.; Bajpai, A.; Kumar, P.; Kaur, M.; Kumar, A. Effect of Stress on Pull-in Voltage of RF MEMS SPDT Switch. IEEE Trans. Electr. Dev. 2020, 67, 2147–2152. [Google Scholar] [CrossRef]
- Zhang, Y.; Sun, J.; Liu, H.; Liu, Z. Modeling and Measurement of Thermal–Mechanical-Stress-Creep Effect for RF MEMS Switch Up to 200 C. Micromachines 2022, 13, 166. [Google Scholar] [CrossRef]
- Dutta, S.; Pandey, A. Overview of residual stress in MEMS structures: Its origin, measurement, and control. J. Mater. Sci. Mater. Electron. 2021, 32, 6705–6741. [Google Scholar] [CrossRef]
- Michalas, L.; Konstantinidis, K.; Farinelli, P.; Mandic, D.P.; Konstantinidis, G. RF MEMS Switch Design Methodology by Electromagnetic Simulations and Machine Learning. In Proceedings of the 52nd European Microwave Conference (EuMC), Milan, Italy, 27–29 September 2022; pp. 369–372. [Google Scholar]
- Birmpiliotis, D.; Czarnecki, P.; Koutsoureli, M.; Papaioannou, G.; De Wolf, I. Assessment of dielectric charging in capacitive MEMS switches fabricated on Si substrate with thin oxide film. Microelectron. Eng. 2016, 159, 209–214. [Google Scholar] [CrossRef]
- Rottenberg, X.; De Wolf, I.; Nauwelaers, B.K.J.C.; De Raedt, W.; Tilmans, H.A.C. Analytical Model of the DC Actuation of Electrostatic MEMS Devices with Distributed Dielectric Charging and Nonplanar Electrodes. J. Microelectromechan. Syst. 2007, 16, 1243–1253. [Google Scholar] [CrossRef]
- Badia, M.F.B.; Buitrago, E.; Ionescu, A.M. RF MEMS Shunt Capacitive Switches Using AlN Compared to Si3N4 Dielectric. J. Microelectromechan. Syst. 2012, 21, 1229–1240. [Google Scholar] [CrossRef]
- Palego, C.; Deng, J.; Peng, Z.; Halder, S.; Hwang, J.C.M.; Forehand, D.I.; Scarbrough, D.; Goldsmith, C.L.; Johnston, I.; Sampath, S.K.; et al. Robustness of RF MEMS Capacitive Switches with Molybdenum Membranes. IEEE Trans. Microw. Theor. Techniq. 2009, 57, 3262–3269. [Google Scholar] [CrossRef]
- Philippine, M.A.; Sigmund, O.; Rebeiz, G.M.; Kenny, T.W. Topology Optimization of Stressed Capacitive RF MEMS Switches. J. Microelectromech. Syst. 2013, 22, 206–2015. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Michalas, L.; Stavrinidis, G.; Tsagaraki, K.; Stavrinidis, A.; Konstantinidis, G. An Experimental Study of the Pull-In Voltage in RF MEMS Switches Fabricated by Au Electroplating and Standard Wet Release: Considering the Bridge Geometry. Sensors 2025, 25, 1877. https://doi.org/10.3390/s25061877
Michalas L, Stavrinidis G, Tsagaraki K, Stavrinidis A, Konstantinidis G. An Experimental Study of the Pull-In Voltage in RF MEMS Switches Fabricated by Au Electroplating and Standard Wet Release: Considering the Bridge Geometry. Sensors. 2025; 25(6):1877. https://doi.org/10.3390/s25061877
Chicago/Turabian StyleMichalas, Loukas, George Stavrinidis, Katerina Tsagaraki, Antonis Stavrinidis, and George Konstantinidis. 2025. "An Experimental Study of the Pull-In Voltage in RF MEMS Switches Fabricated by Au Electroplating and Standard Wet Release: Considering the Bridge Geometry" Sensors 25, no. 6: 1877. https://doi.org/10.3390/s25061877
APA StyleMichalas, L., Stavrinidis, G., Tsagaraki, K., Stavrinidis, A., & Konstantinidis, G. (2025). An Experimental Study of the Pull-In Voltage in RF MEMS Switches Fabricated by Au Electroplating and Standard Wet Release: Considering the Bridge Geometry. Sensors, 25(6), 1877. https://doi.org/10.3390/s25061877





