Evaluating a 3D Ultrasound Imaging Resolution of Single Transmitter/Receiver with Coding Mask by Extracting Phase Information
Abstract
1. Introduction
- to develop ultrasound imaging based on single element with coding mask by numerically solving the image model equation,
- to apply the proposed method by integrating the frequency subband compound and SCM, and
- to evaluate the image performance by comparing it with other methods by varying the single scatterer position in the region of interest.
2. Methods
2.1. Image Model
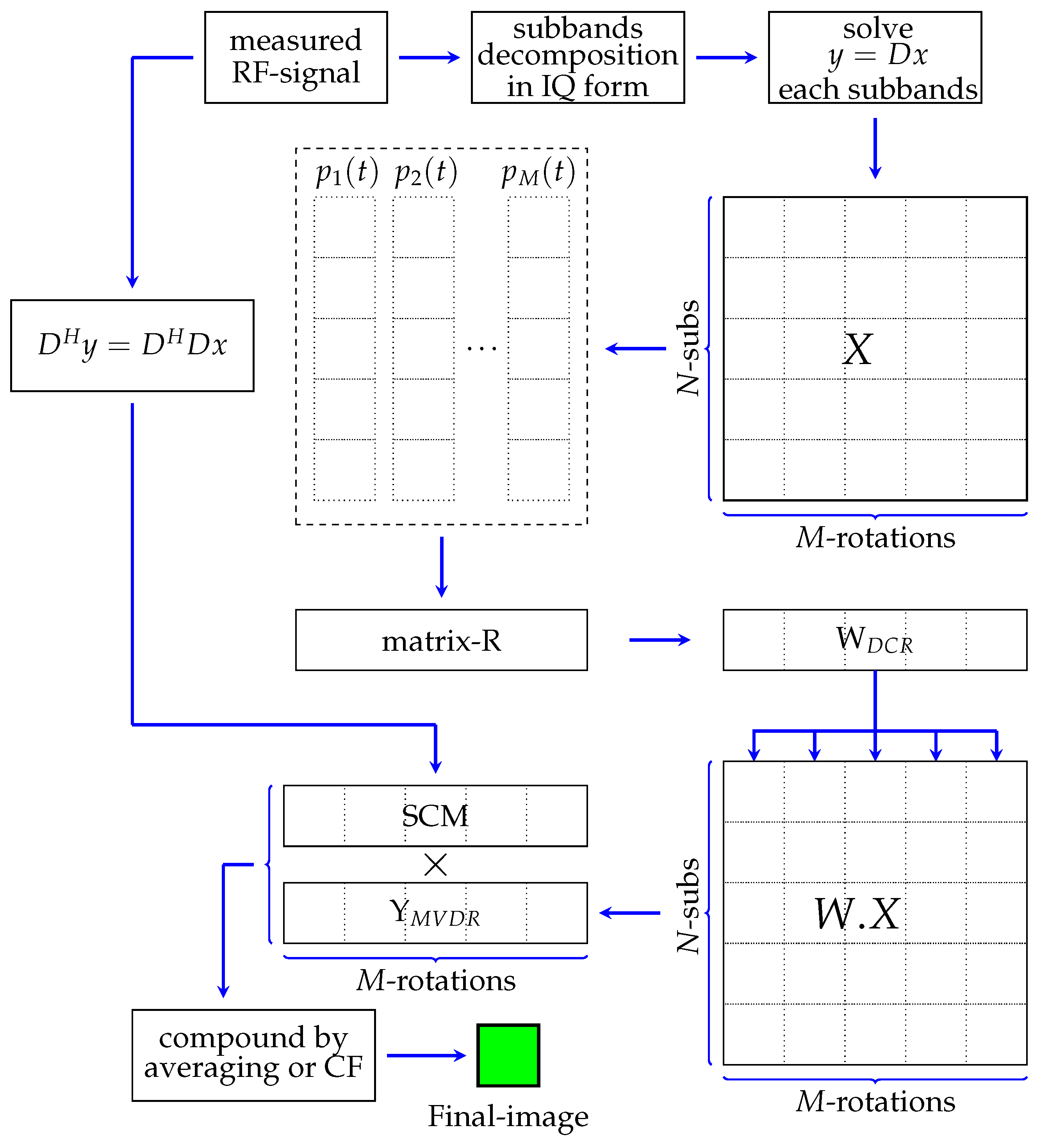
2.2. Proposed Method: Super-Resolution Weighted Frequency Subbands Compound
2.3. Previous Methods for Image Construction Were Based on a Single Transducer
- Method-B: This method uses weighted frequency subband compounds to obtain images for each rotation. The original wide band of the measured signal was decomposed into several narrow subbands with their different center of frequency. The subband decomposition was applied to vector-y and matrix-D for all rotation angles. The images were constructed by numerically solving a linear equation of the image model with different subbands and angles of rotation. The weight of each subband was calculated and summed up for each angle of rotation using the minimum variance distortion-free response (MVDR) method [29].
- Method-C: Based on the image model, , the SCM profile was extracted from the measured data for each angle of rotation. The final image at different rotation angles was obtained by multiplying the SCM profile with the image-x computed by numerically solving the LE [30].
- Method-D: The image model was modified by applying the D-compression to the original image model resulting in . The SCM profile was determined based on the modified image model. On the other hand, the solution-x of this equation was computed by analytically solving . The final image of each rotation was achieved by properly multiplying the image-x and the SCM profile.
3. Simulation and Experiment Results
3.1. Simulation Model
3.2. Simulation Result
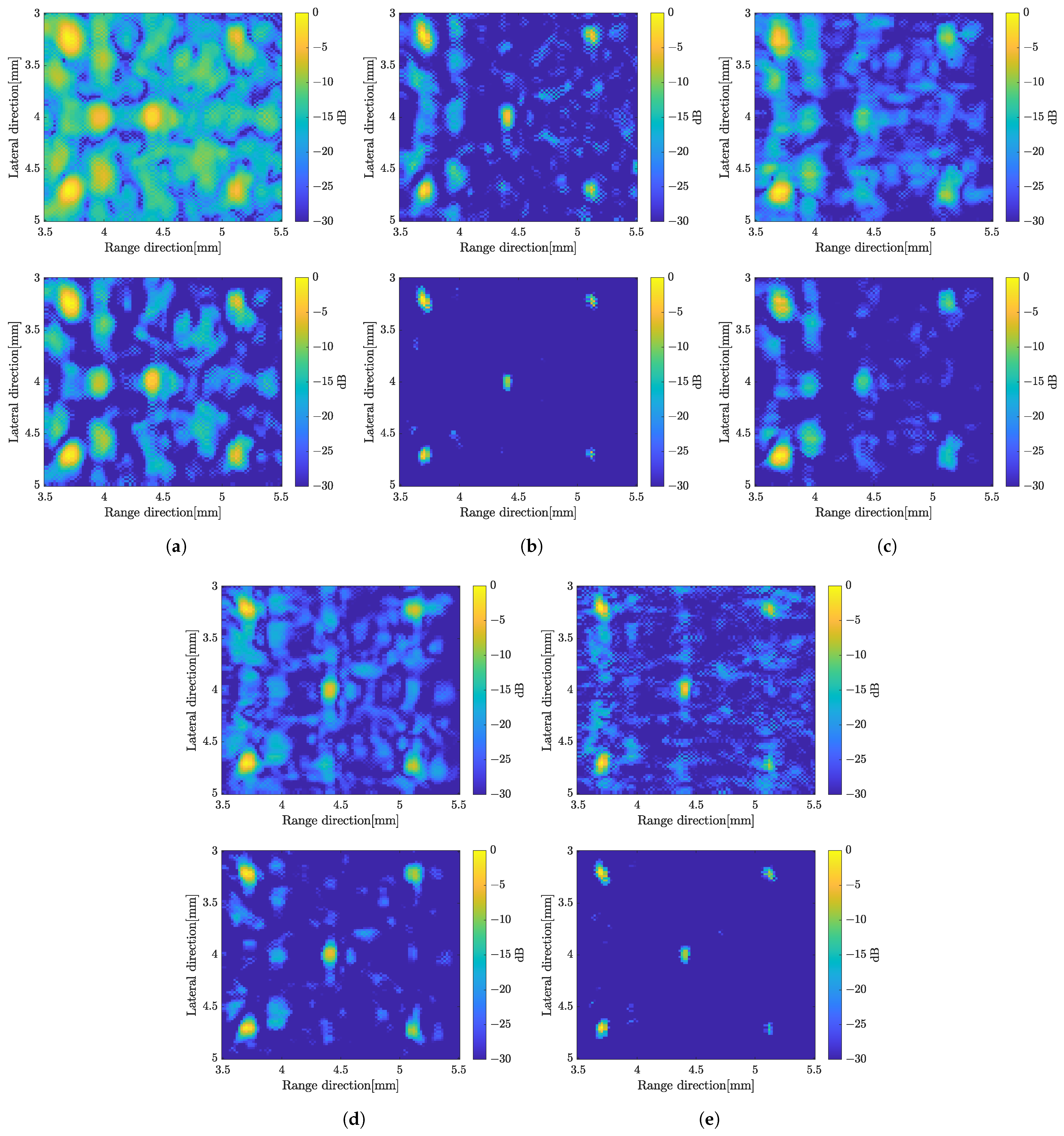
3.2.1. Basic Method: Numerically Solving a Linear Equation of Image Model
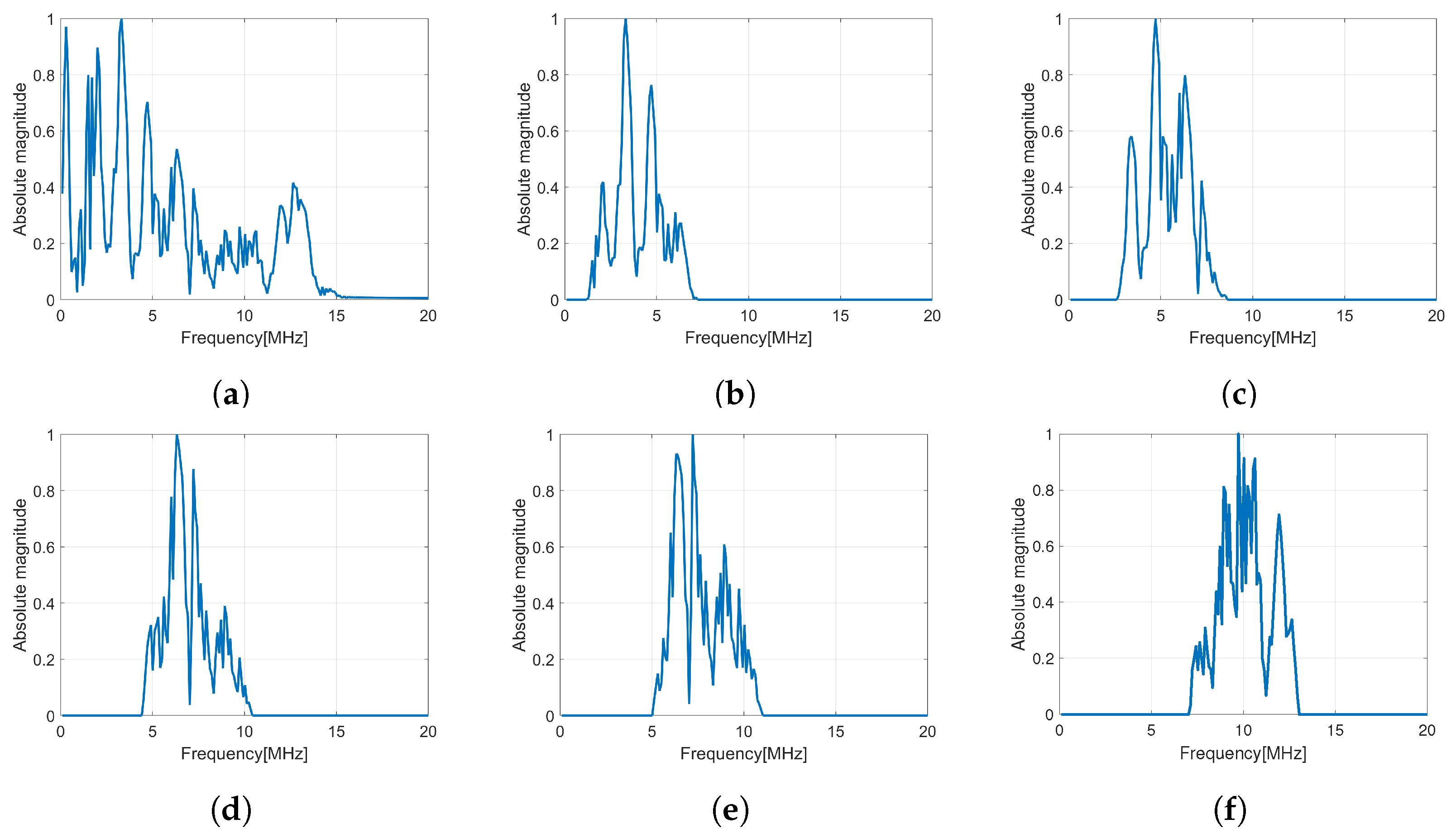
3.2.2. Frequency Subbands Compound
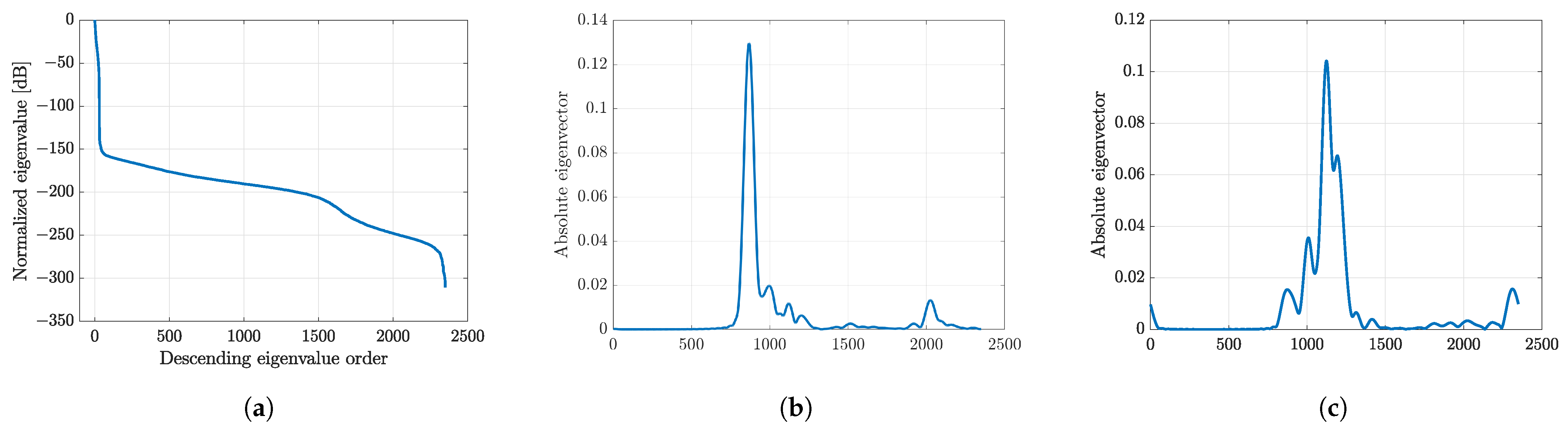
3.2.3. Super-Resolution Method (SCM)
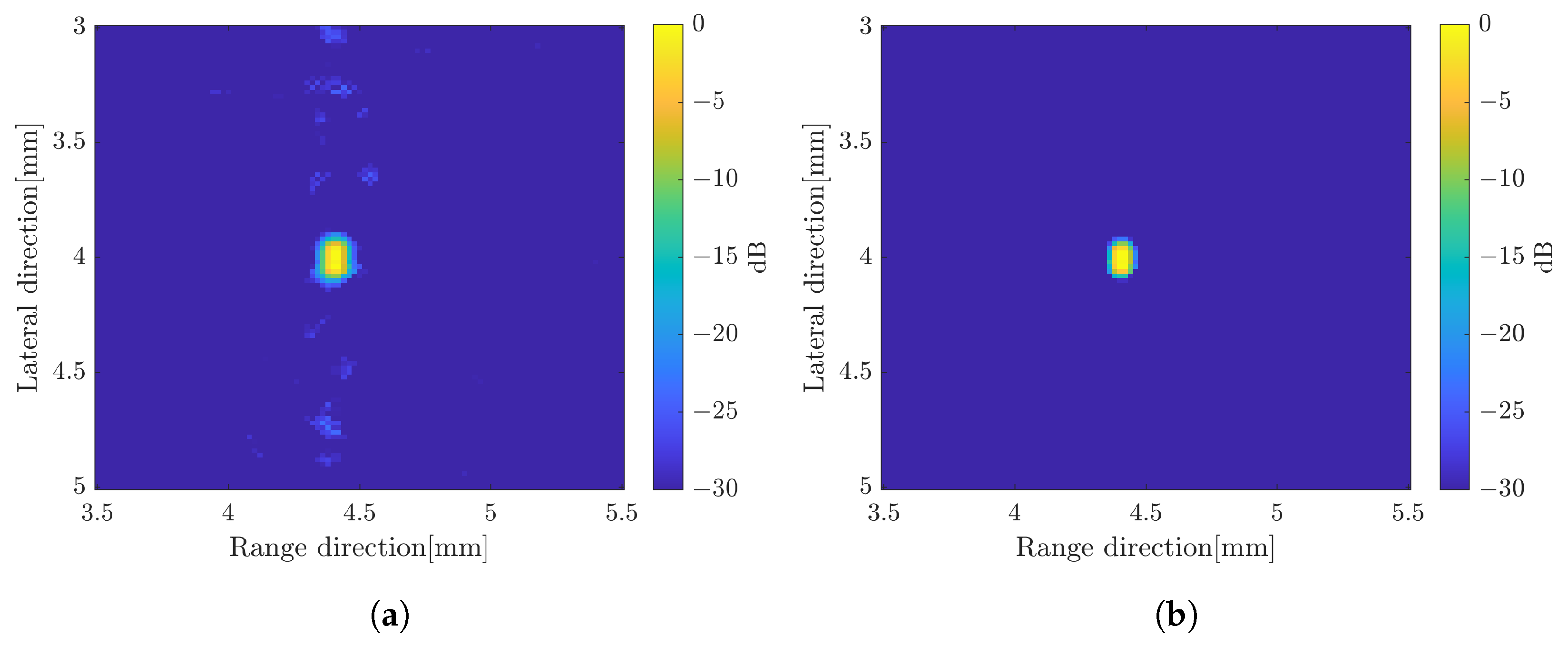
3.2.4. Proposed Method
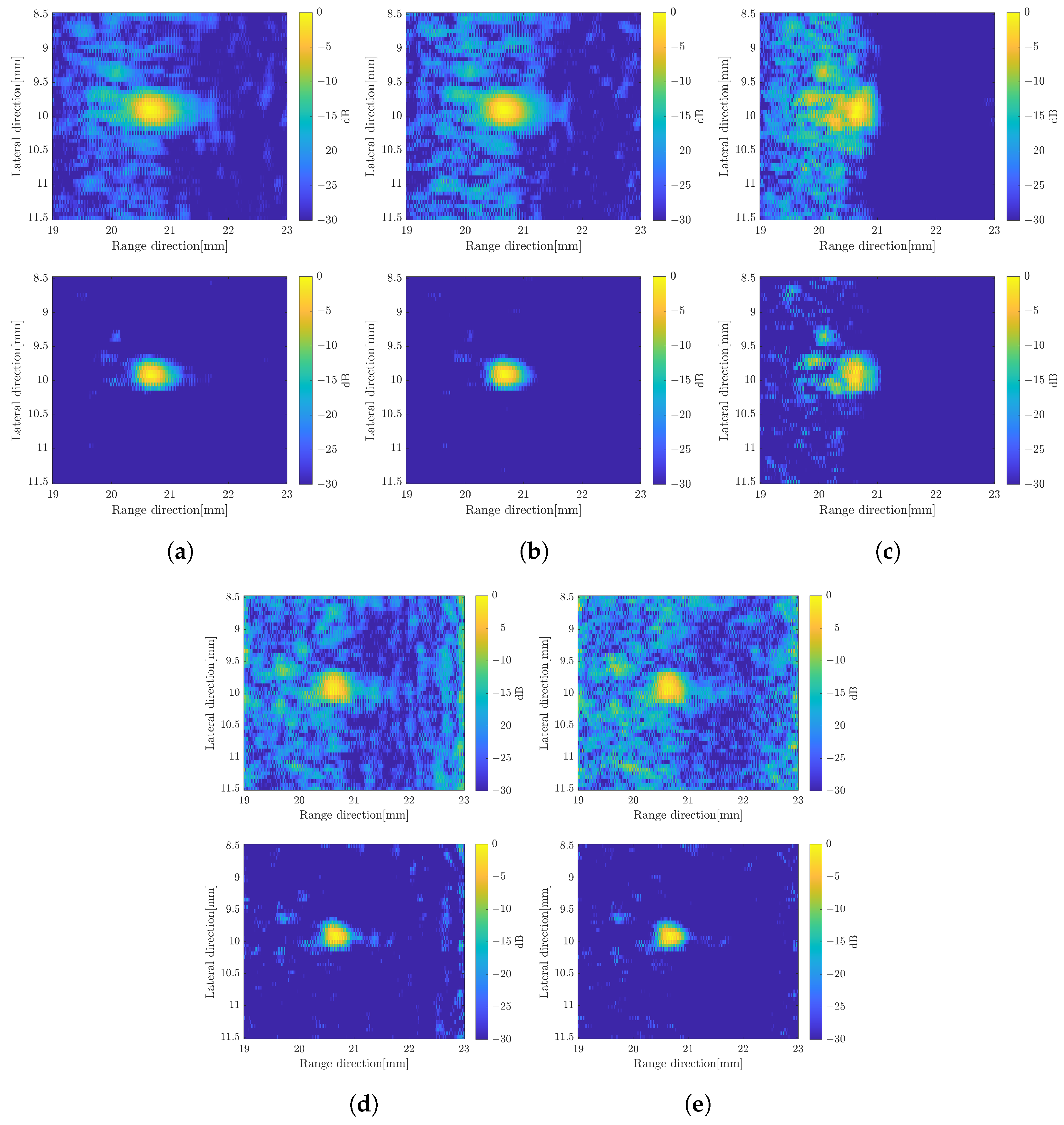
3.2.5. Evaluate the Methods with Multiple Scatterers
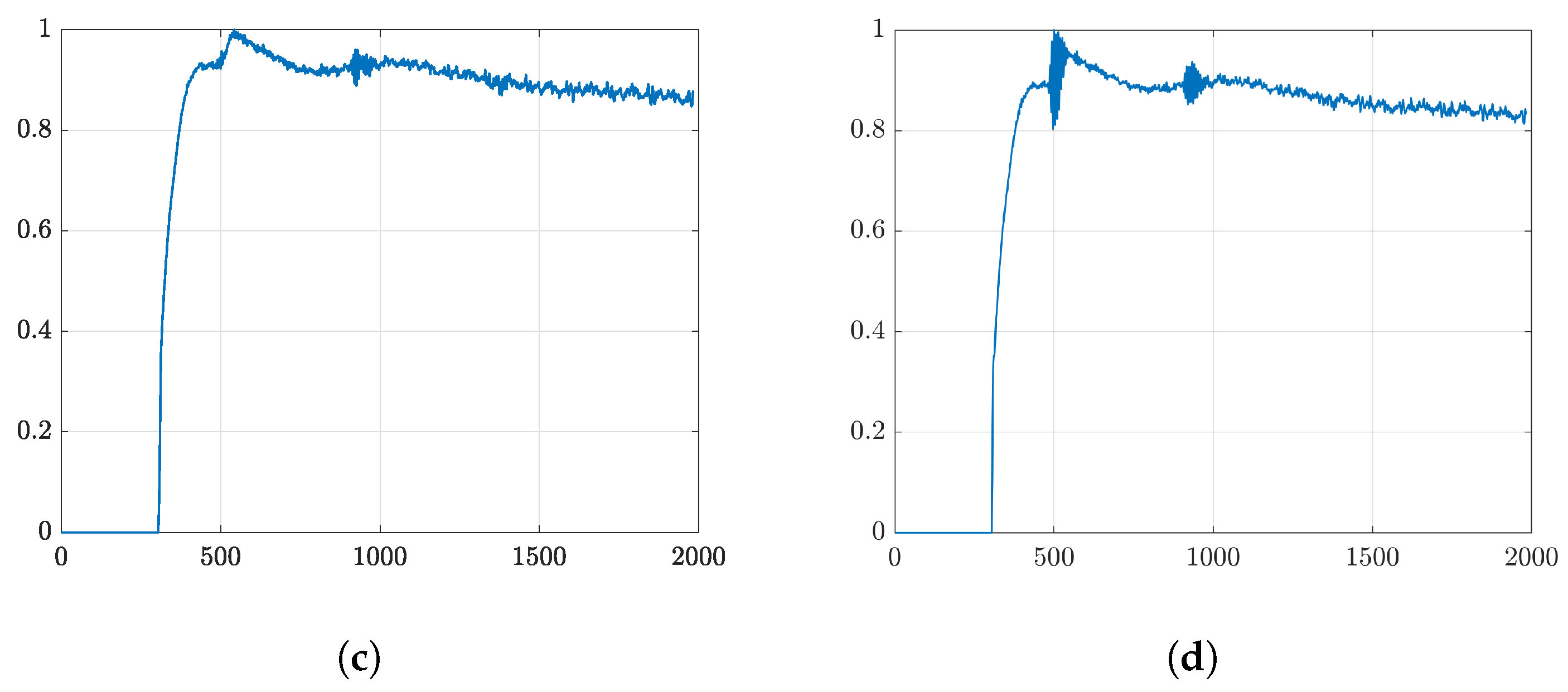
3.3. Experimental Condition
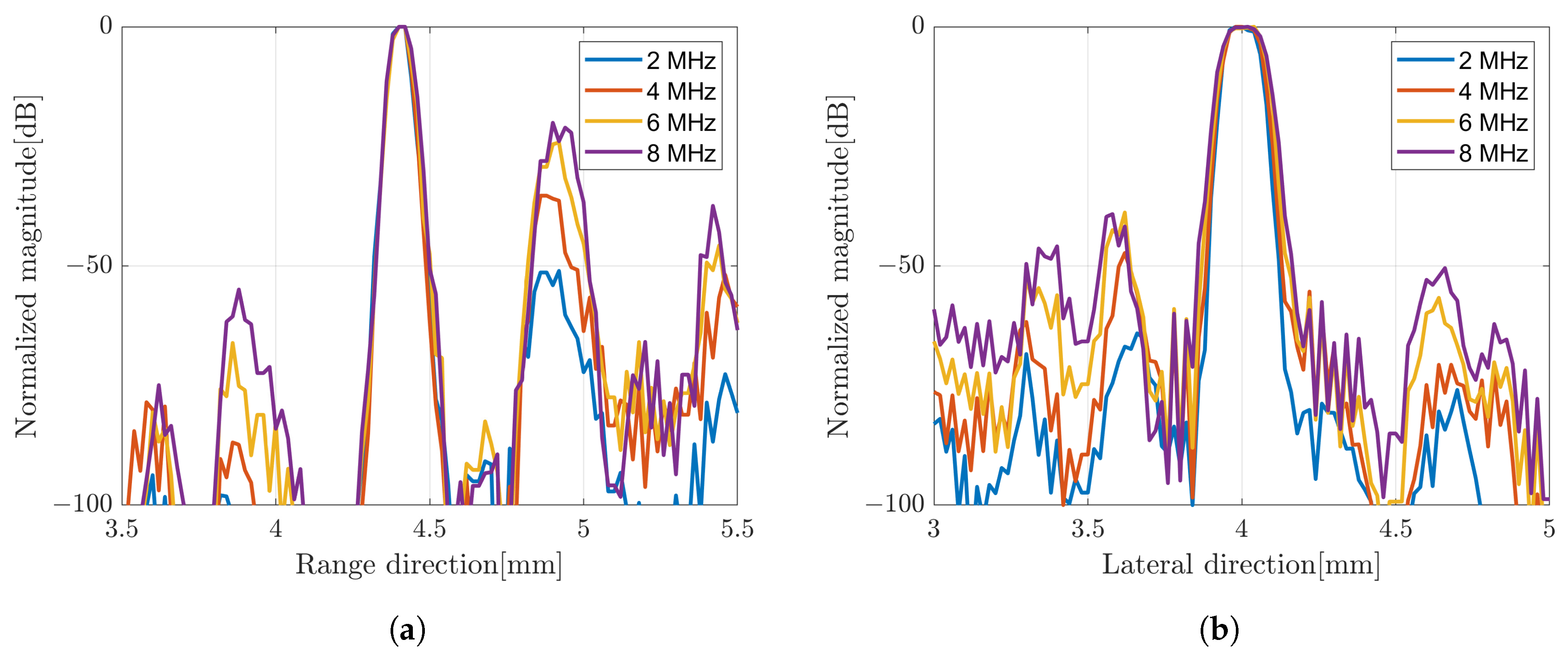
3.4. Experimental Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. Frequency Subband Compound

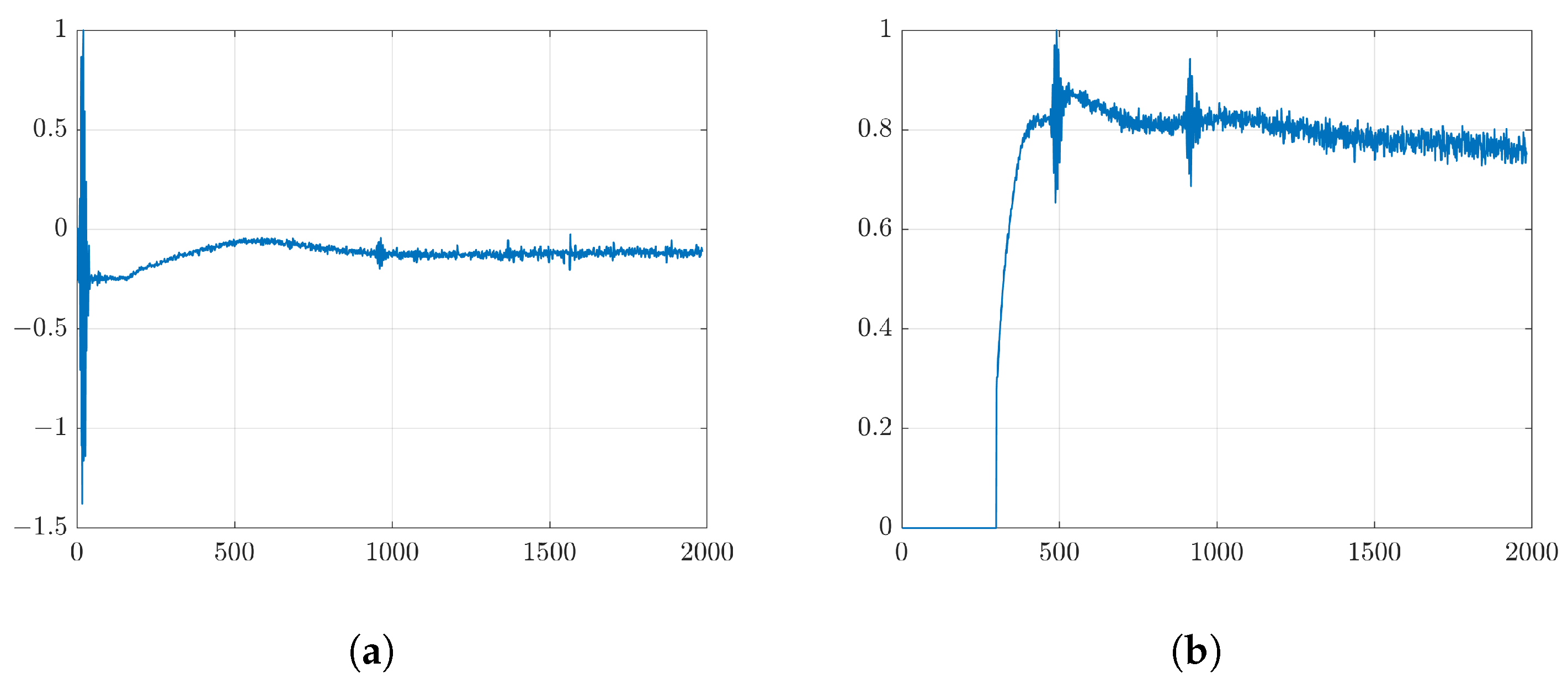
Appendix B. Super-Resolution Method (SCM)
Appendix C. Coherence Factor Beamformer
References
- Synnevag, J.F.; Austeng, A.; Holm, S. Adaptive beamforming applied to medical ultrasound imaging. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2007, 54, 1606–1613. [Google Scholar] [CrossRef]
- Hasegawa, H. Improvement of range spatial resolution of medical ultrasound imaging by element-domain signal processing. Jpn. J. Appl. Phys. 2017, 56, 07JF02. [Google Scholar] [CrossRef]
- Danilouchkine, M.G.; van Neer, P.L.M.J.; Verweij, M.D.; Matte, G.M.; Vletter, W.B.; van der Steen, A.F.W.; de Jong, N. Single pulse frequency compounding protocol for superharmonic imaging. Phys. Med. Biol. 2013, 58, 4791–4805. [Google Scholar] [CrossRef][Green Version]
- Youn, J.; Luijten, B.; Bo Stuart, M.; Eldar, Y.C.; van Sloun, R.J.G.; Arendt Jensen, J. Deep learning models for fast ultrasound localization microscopy. In Proceedings of the IEEE International Ultrasonics Symposium, Las Vegas, NV, USA, 7–11 September 2020; pp. 1–4. [Google Scholar]
- Matrone, G.; Savoia, A.S.; Caliano, G.; Magenes, G. The delay multiply and sum beamforming algorithm in ultrasound B-mode medical imaging. IEEE Trans. Med. Imaging 2015, 34, 940–949. [Google Scholar] [CrossRef] [PubMed]
- Sannou, F.; Nagaoka, R.; Hasegawa, H. Estimation of speed of sound using coherence factor and signal-to-noise ratio for improvement of performance of ultrasonic beamformer. Jpn. J. Appl. Phys. 2020, 59, SKKE14. [Google Scholar] [CrossRef]
- Camacho, J.; Parrilla, M.; Fritsch, C. Phase coherence imaging. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2009, 56, 958–974. [Google Scholar] [CrossRef]
- Misaridis, T.; Jensen, J.A. Use of modulated excitation signals in medical ultrasound. Part II: Design and performance for medical imaging applications. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2005, 52, 192–207. [Google Scholar] [CrossRef]
- Lan, Z.; Jin, L.; Feng, S.; Zheng, C.; Han, Z.; Peng, H. Joint generalized coherence factor and minimum variance beamformer for synthetic aperture ultrasound imaging. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2021, 68, 1167–1183. [Google Scholar] [CrossRef] [PubMed]
- Yan, J.; Wang, B.; Riemer, K.; Shearer, J.H.; Lerendegui, M.; Toulemonde, M.; Rowlands, C.J.; Weinberg, P.D.; Tang, M.-X. Fast 3D super-resolution ultrasound with adaptive weight-based beamforming. IEEE Trans. Biomed. Eng. 2023, 70, 2752–2761. [Google Scholar] [CrossRef]
- Tanter, M.; Fink, M. Ultrafast imaging in biomedical ultrasound. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2014, 61, 102–119. [Google Scholar] [CrossRef]
- Nguyen, N.Q.; Prager, R.W. A spatial coherence approach to minimum variance beamforming for plane-wave compounding. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2018, 65, 522–534. [Google Scholar] [CrossRef]
- Ni, P.; Lee, H.N. High-resolution ultrasound imaging using random interference. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2020, 67, 1785–1799. [Google Scholar] [CrossRef] [PubMed]
- Sternini, S.; Pau, A.; Di Scalea, F.L. Minimum-variance imaging in plates using guided-wave-mode beamforming. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2019, 66, 1906–1919. [Google Scholar] [CrossRef]
- Janjic, J.; Kruizinga, P.; van der Meulen, P.; Springeling, G.; Mastik, F.; Leus, G.; Bosch, J.G.; van der Steen, A.F.W.; van Soest, G. Structured ultrasound microscopy. Appl. Phys. Lett. 2018, 112, 251901. [Google Scholar] [CrossRef]
- Kruizinga, P.; van der Meulen, P.; Fedjajevs, A.; Mastik, F.; Springeling, G.; de Jong, N.; Bosch, J.G.; Leus, G. Compressive 3D ultrasound imaging using a single sensor. Sci. Adv. 2017, 3, e170142. [Google Scholar] [CrossRef] [PubMed]
- Sheng, J.; Cai, H.; Wang, Y.; Chen, X.; Xu, Y. Improved Exponential Phase Mask for Generating Defocus Invariance of Wavefront Coding Systems. Appl. Sci. 2022, 12, 5290. [Google Scholar] [CrossRef]
- Luijten, B.; Cohen, R.; de Bruijn, F.J.; Schmeitz, H.A.W.; Mischi, M.; Eldar, Y.C.; van Sloun, R.J.G. Adaptive ultrasound beamforming using deep learning. IEEE Trans. Med. Imaging 2020, 39, 3967–3978. [Google Scholar] [CrossRef]
- Oelze, M.L. Bandwidth and resolution enhancement through pulse compression. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2007, 54, 768–781. [Google Scholar] [CrossRef] [PubMed]
- Prieur, F.; Rindal, O.M.H.; Austeng, A. Signal coherence and image amplitude with the filtered delay multiply and sum beamformer. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2018, 65, 1133–1140. [Google Scholar] [CrossRef]
- Nilsen, C.C.; Holm, S. Wiener beamforming and the coherence factor in ultrasound imaging. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2010, 57, 1329–1346. [Google Scholar] [CrossRef]
- Shen, C.-C.; Xing, Y.-Q.; Jeng, G. Autocorrelation-based generalized coherence factor for low-complexity adaptive beamforming. Ultrasonics 2016, 72, 177–183. [Google Scholar] [CrossRef]
- Capon, J. High-resolution frequency-wavenumber spectrum analysis. Proc. IEEE 1969, 57, 1408–1418. [Google Scholar] [CrossRef]
- Vignom, F.; Burcher, M.R. Capon beamforming in medical ultrasound imaging with focused beams. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2008, 55, 619–628. [Google Scholar] [CrossRef]
- Asl, B.M.; Mahloojifar, A. A low-complexity adaptive beamformer for ultrasound imaging using structured covariance matrix. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2012, 59, 660–667. [Google Scholar] [CrossRef]
- Labyed, Y.; Huang, L. Ultrasound time-reversal MUSIC imaging with diffraction and attenuation compensation. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2012, 59, 2186–2200. [Google Scholar] [CrossRef] [PubMed]
- Labyed, Y.; Huang, L. Super-resolution ultrasound imaging using a phase-coherent MUSIC method with compensation for the phase response of transducer elements. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2013, 60, 1048–1060. [Google Scholar] [CrossRef] [PubMed]
- Fujiwara, M.; Okubo, K.; Tagawa, N. Novel technique for high resolution ultrasound super resolution FM-Chirp Correlation Method (SCM). In Proceedings of the IEEE International Ultrasonics Symposium, Rome, Italy, 20–23 September 2009; pp. 2390–2393. [Google Scholar]
- Syaryadhi, M.; Tagawa, N.; Yang, M. Weighted frequency subband compounding in ultrasonic imaging sensor consisting of a single transducer and a random coding mask. In Proceedings of the 2023 IEEE Applied Sensing Conference (APSCON), Bangalore, India, 23–25 January 2023; pp. 1–3. [Google Scholar]
- Syaryadhi, M.; Zheng, J.; Tagawa, N.; Yang, M. Super-resolution based on frequency dependence of echo phase rotation in 3D ultrasound imaging by spatially encoded transmit/receive. In Proceedings of the 2023 International Congress on Ultrasonics, Beijing, China, 18–21 September 2023. [Google Scholar]
- Zheng, J.; Tagawa, N.; Yoshizawa, M.; Irie, T. Plane wave beamforming with adaptively weighted frequency compound using bandpass filtering. Jpn. J. Appl. Phys. 2021, 60, SDDB08. [Google Scholar] [CrossRef]
- Li, P.C.; Li, M.L. Adaptive imaging using the generalized coherence factor. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2003, 50, 128–141. [Google Scholar]

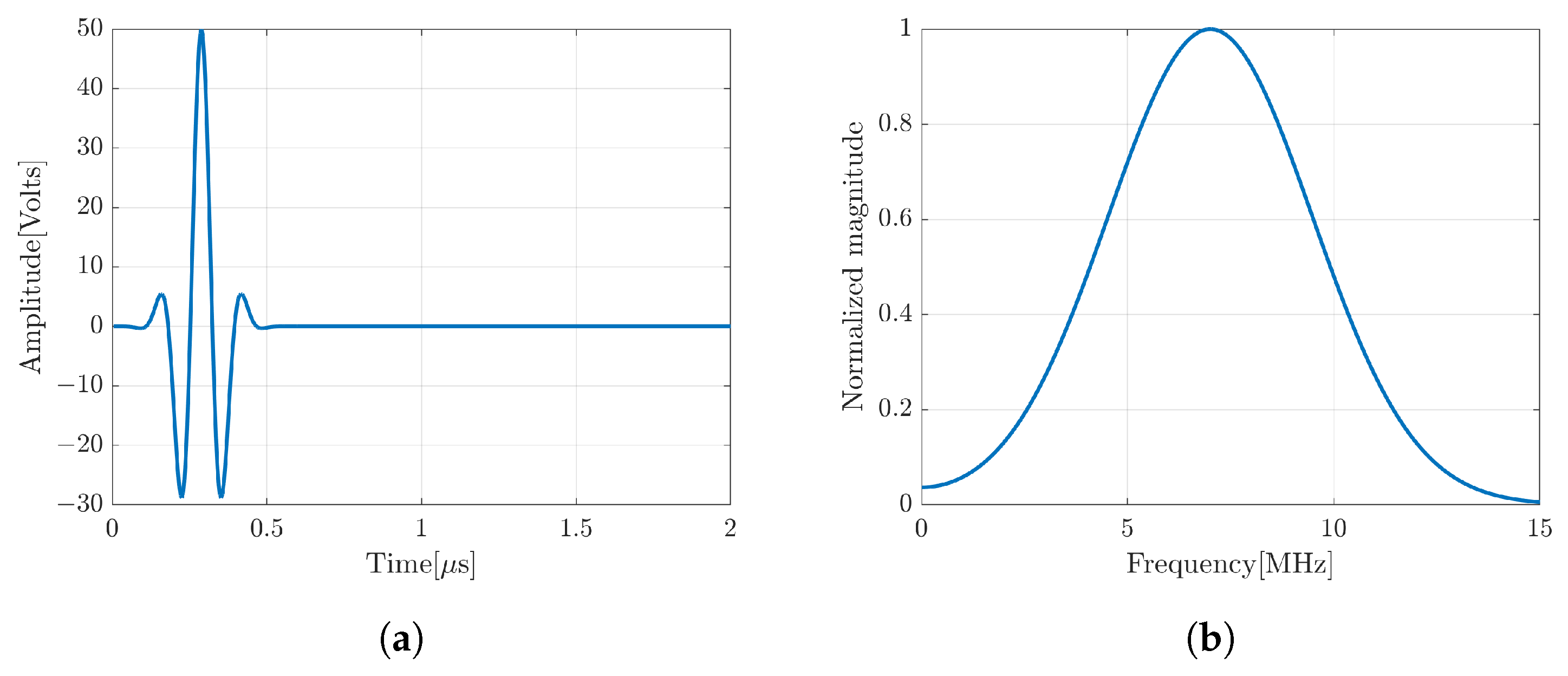












| Parameter | Value |
|---|---|
| Short transmission pulse voltage | 50 Volts |
| Device length | 5 mm |
| Center of frequency | 7 MHz |
| Backing thickness | 1.25 mm |
| PZT transducer: | |
| - thickness | 0.165 mm |
| - density | 7500 kg/m3 |
| - dielectric constant | 1700 |
| Coding mask: | |
| - material | Plastic |
| - density | 1060 kg/m3 |
| - bulk velocity | 2340 m/s |
| - number of patches | 30 |
| - randomized thickness | 0.083–0.335 mm/(0.25–1.00) |
| Scatterer radius | 0.1 mm |
| Distance from the scatterer to the surface of the transducer | 2.5 mm |
| Region of interest (ROI) size | 2 mm × 2 mm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Syaryadhi, M.; Nakazawa, E.; Tagawa, N.; Yang, M. Evaluating a 3D Ultrasound Imaging Resolution of Single Transmitter/Receiver with Coding Mask by Extracting Phase Information. Sensors 2024, 24, 1496. https://doi.org/10.3390/s24051496
Syaryadhi M, Nakazawa E, Tagawa N, Yang M. Evaluating a 3D Ultrasound Imaging Resolution of Single Transmitter/Receiver with Coding Mask by Extracting Phase Information. Sensors. 2024; 24(5):1496. https://doi.org/10.3390/s24051496
Chicago/Turabian StyleSyaryadhi, Mohammad, Eiko Nakazawa, Norio Tagawa, and Ming Yang. 2024. "Evaluating a 3D Ultrasound Imaging Resolution of Single Transmitter/Receiver with Coding Mask by Extracting Phase Information" Sensors 24, no. 5: 1496. https://doi.org/10.3390/s24051496
APA StyleSyaryadhi, M., Nakazawa, E., Tagawa, N., & Yang, M. (2024). Evaluating a 3D Ultrasound Imaging Resolution of Single Transmitter/Receiver with Coding Mask by Extracting Phase Information. Sensors, 24(5), 1496. https://doi.org/10.3390/s24051496






