A 4 × 4 Active Antenna Array with Adjustable Beam Steering
Abstract
1. Introduction
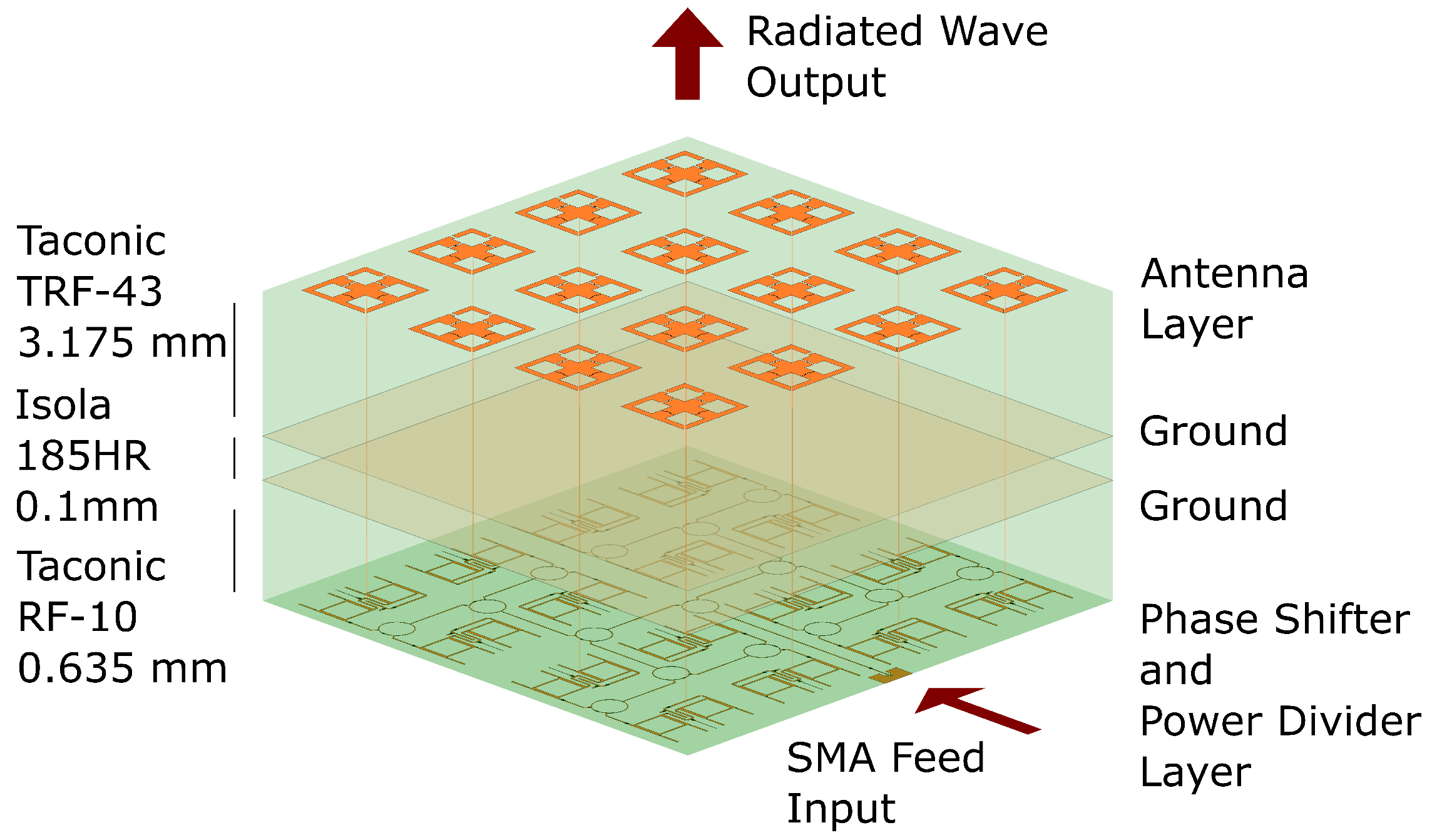
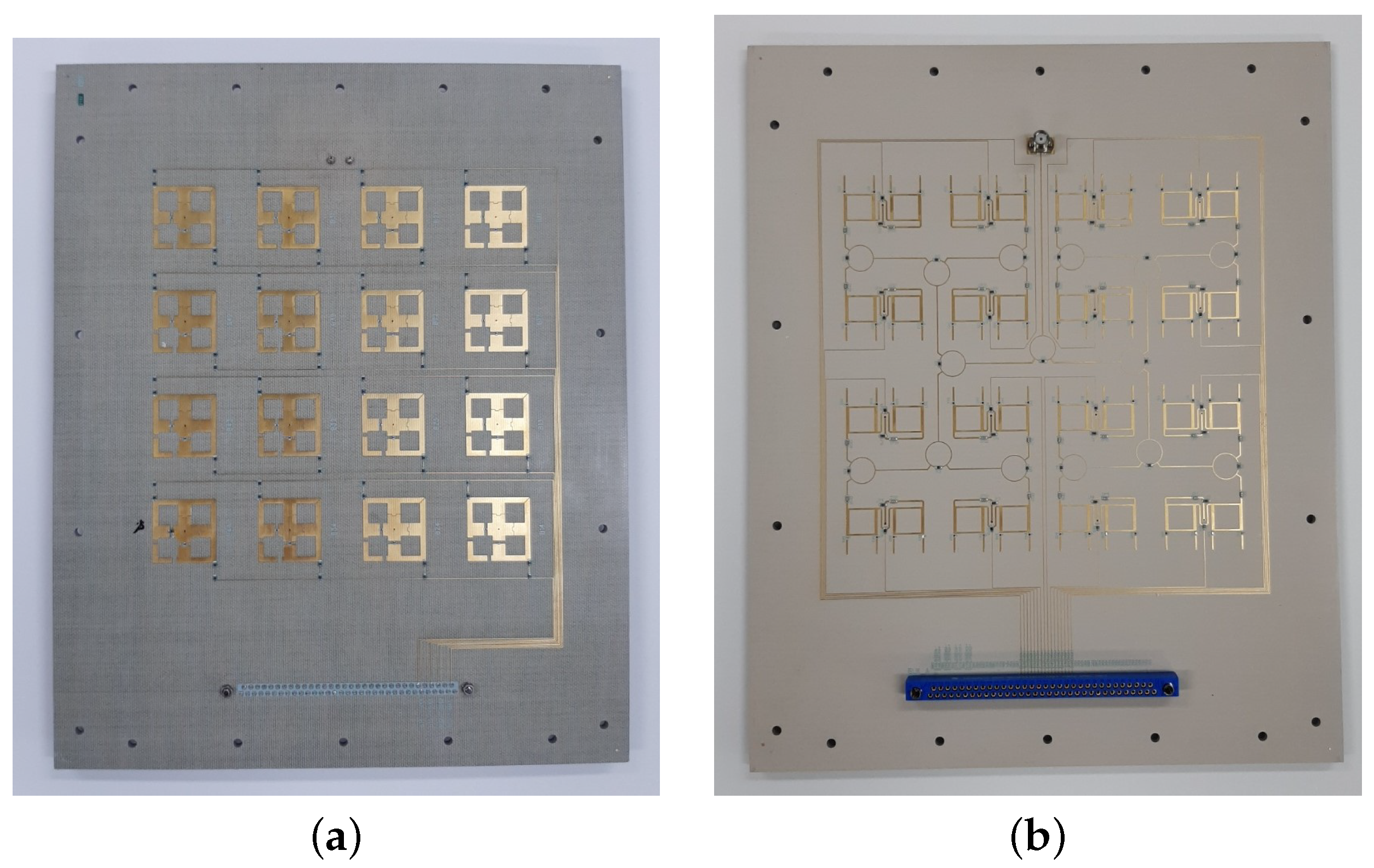
2. Complete Structure
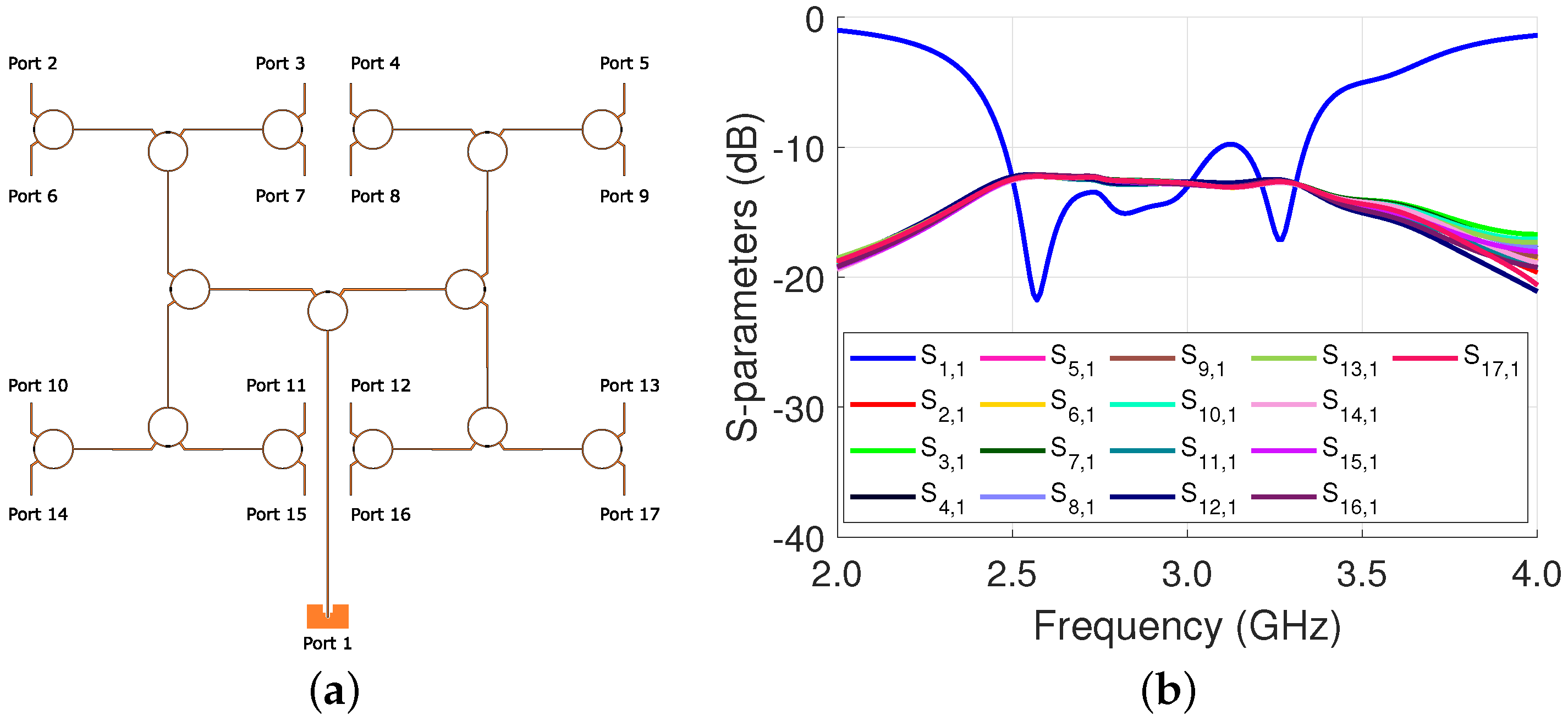
2.1. Power Divider
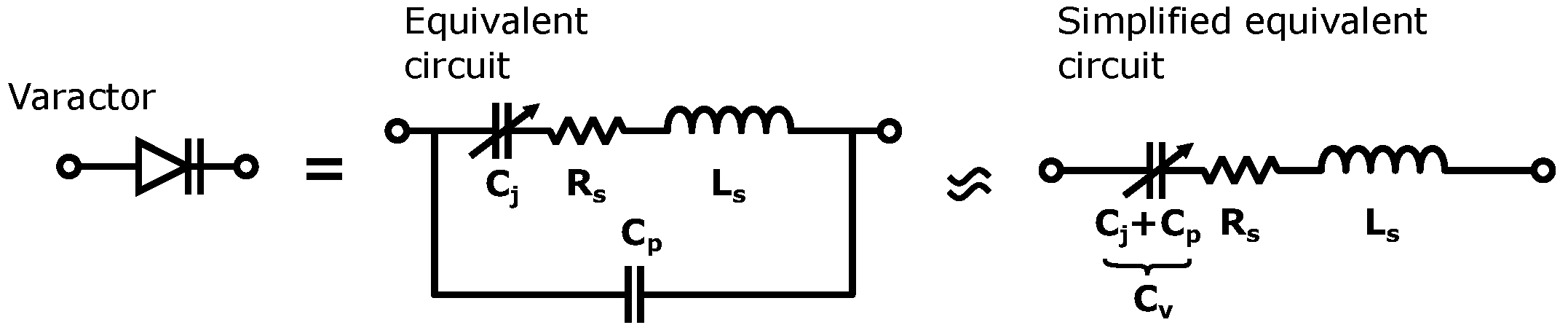
2.2. Phase Shifter
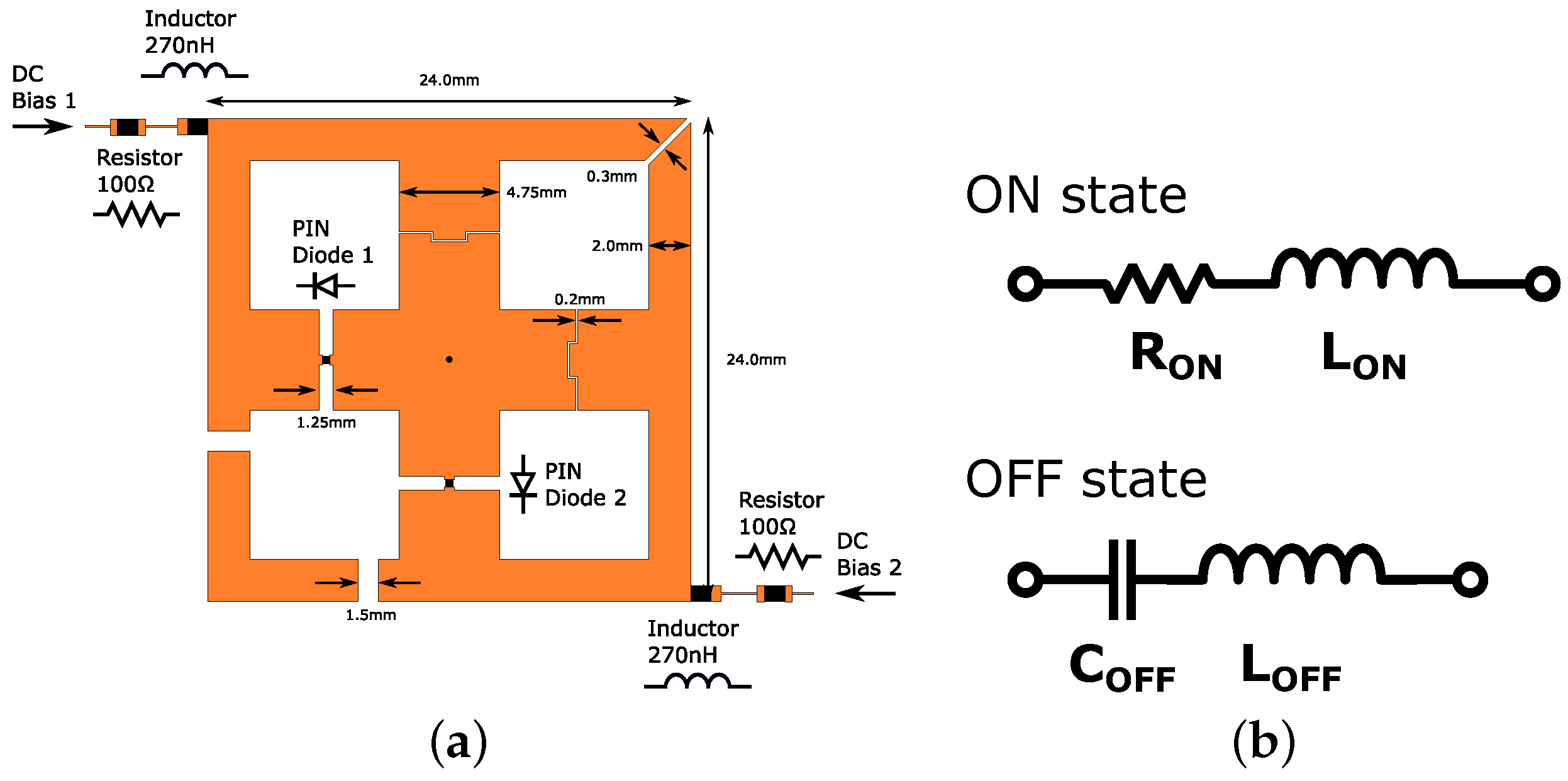
2.3. Antenna
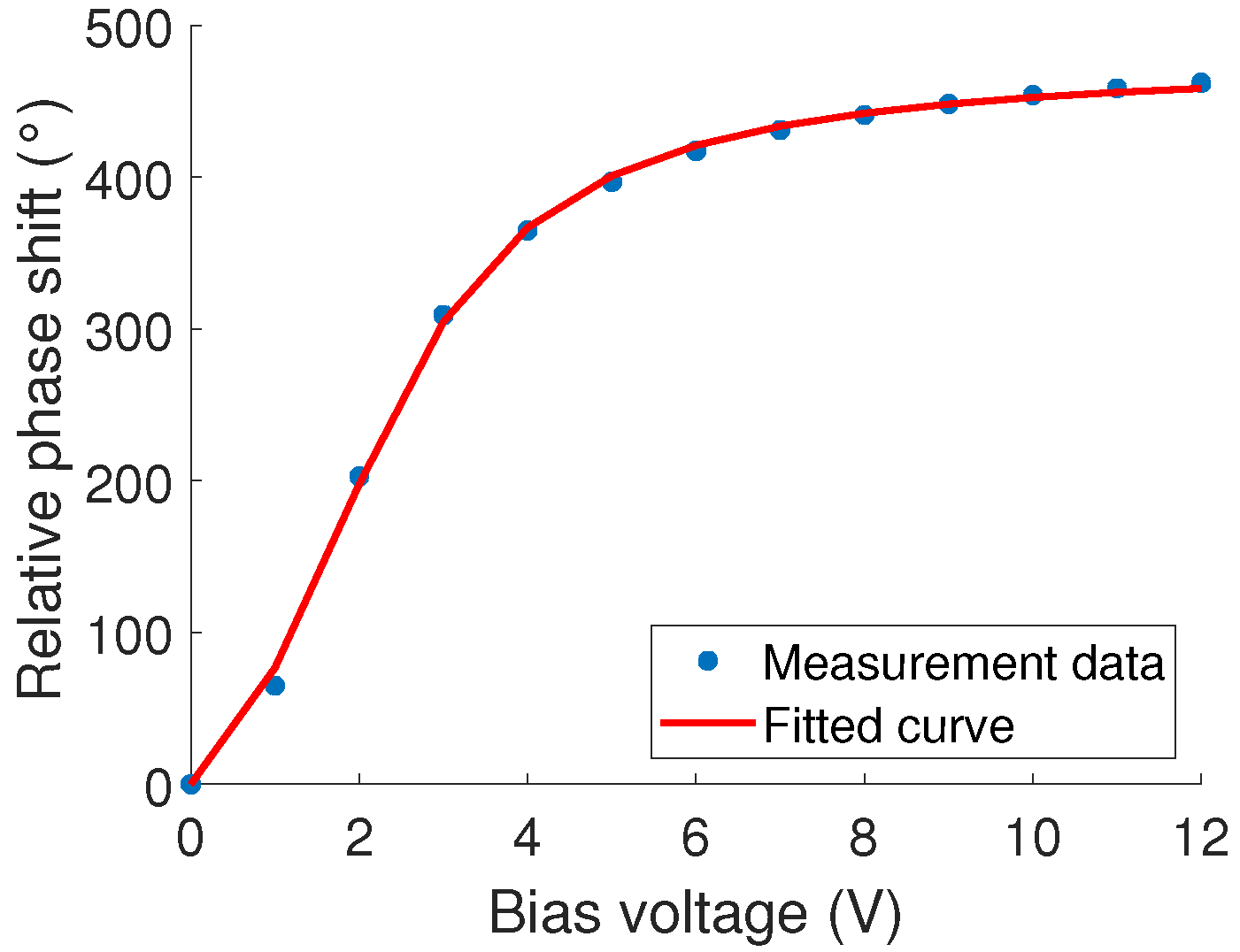
3. Phase Shift Characterization
3.1. Error Analysis
4. Far-Field Measurements
4.1. Beamforming Strategy
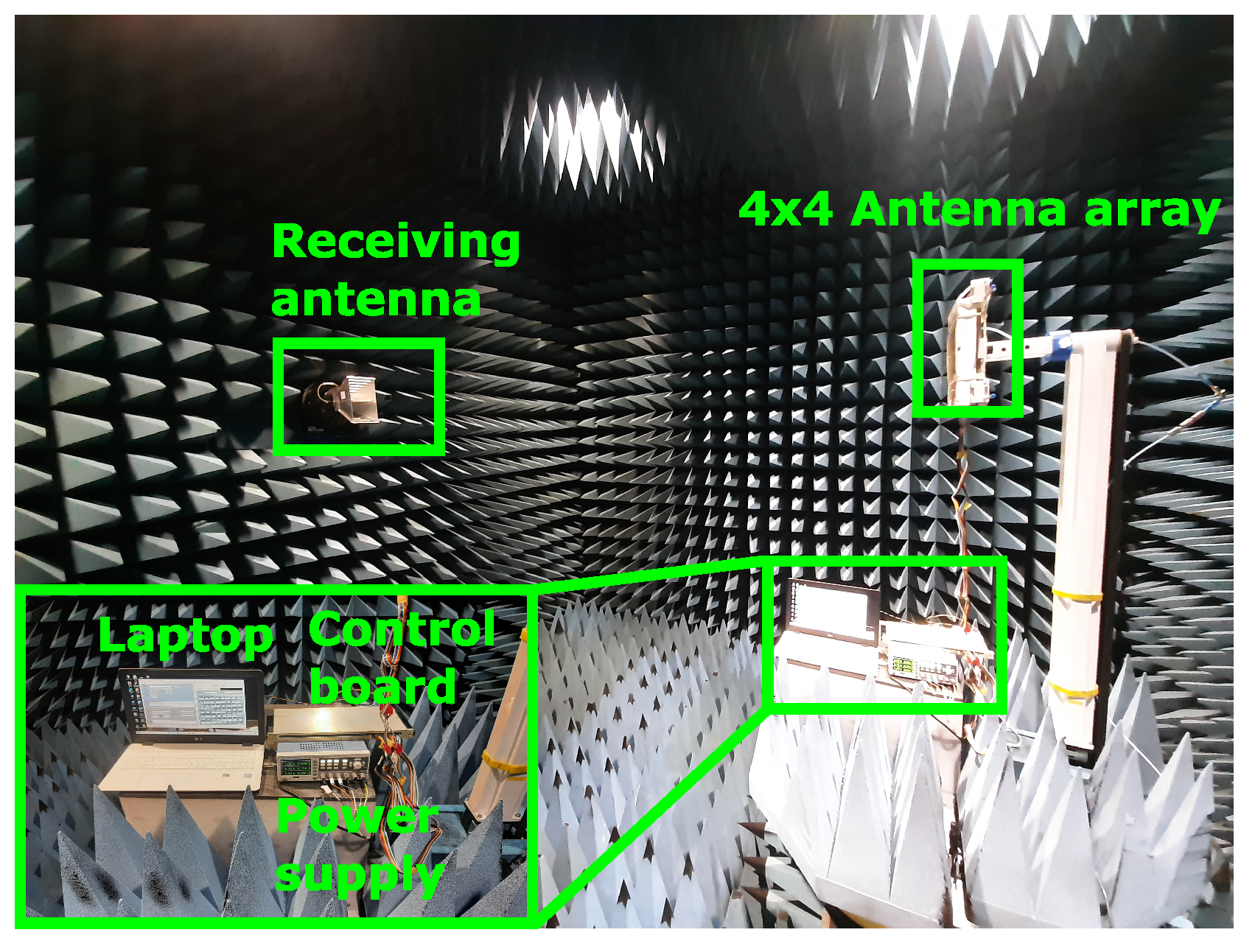
4.2. Measurement Setup
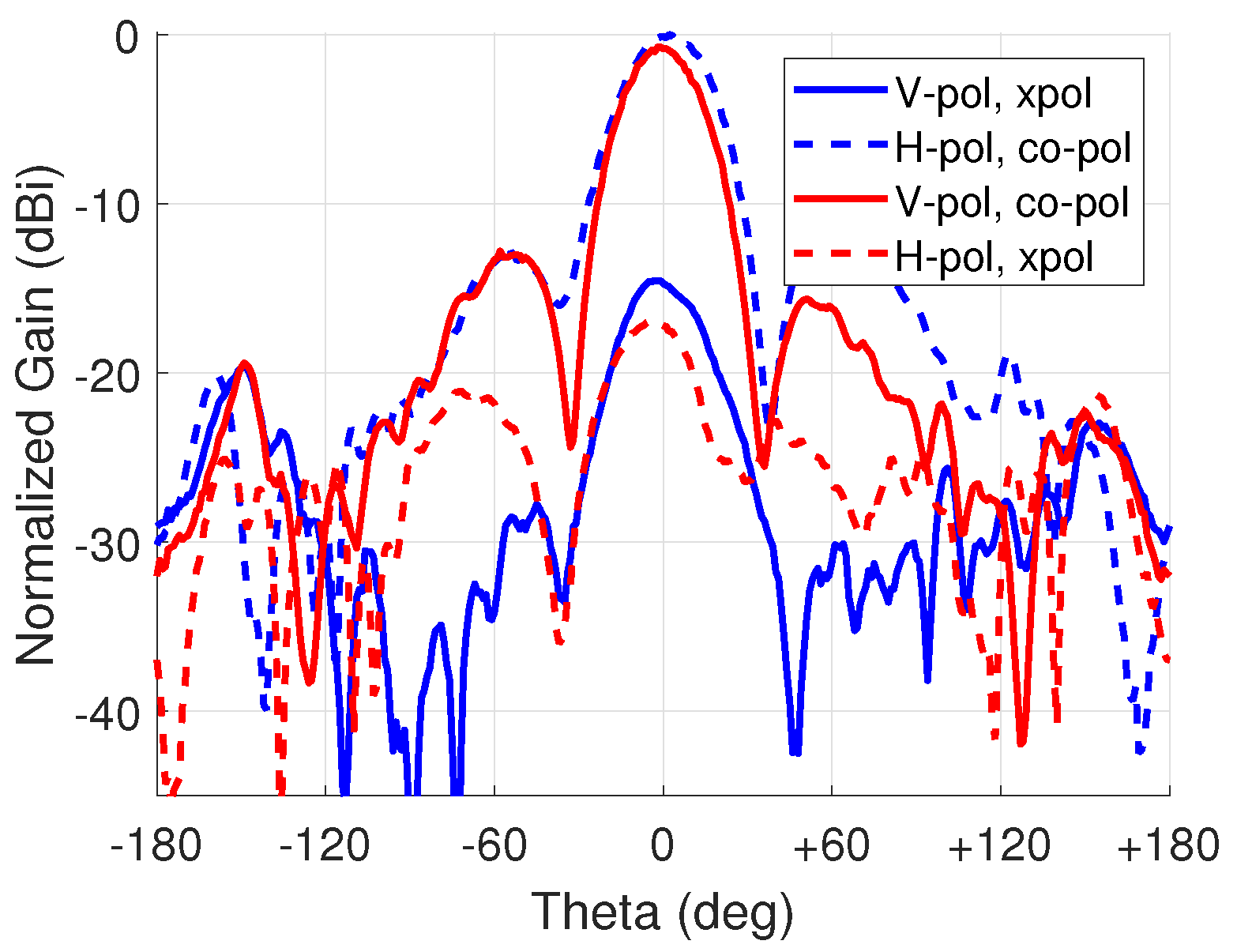
4.3. Measurement Results
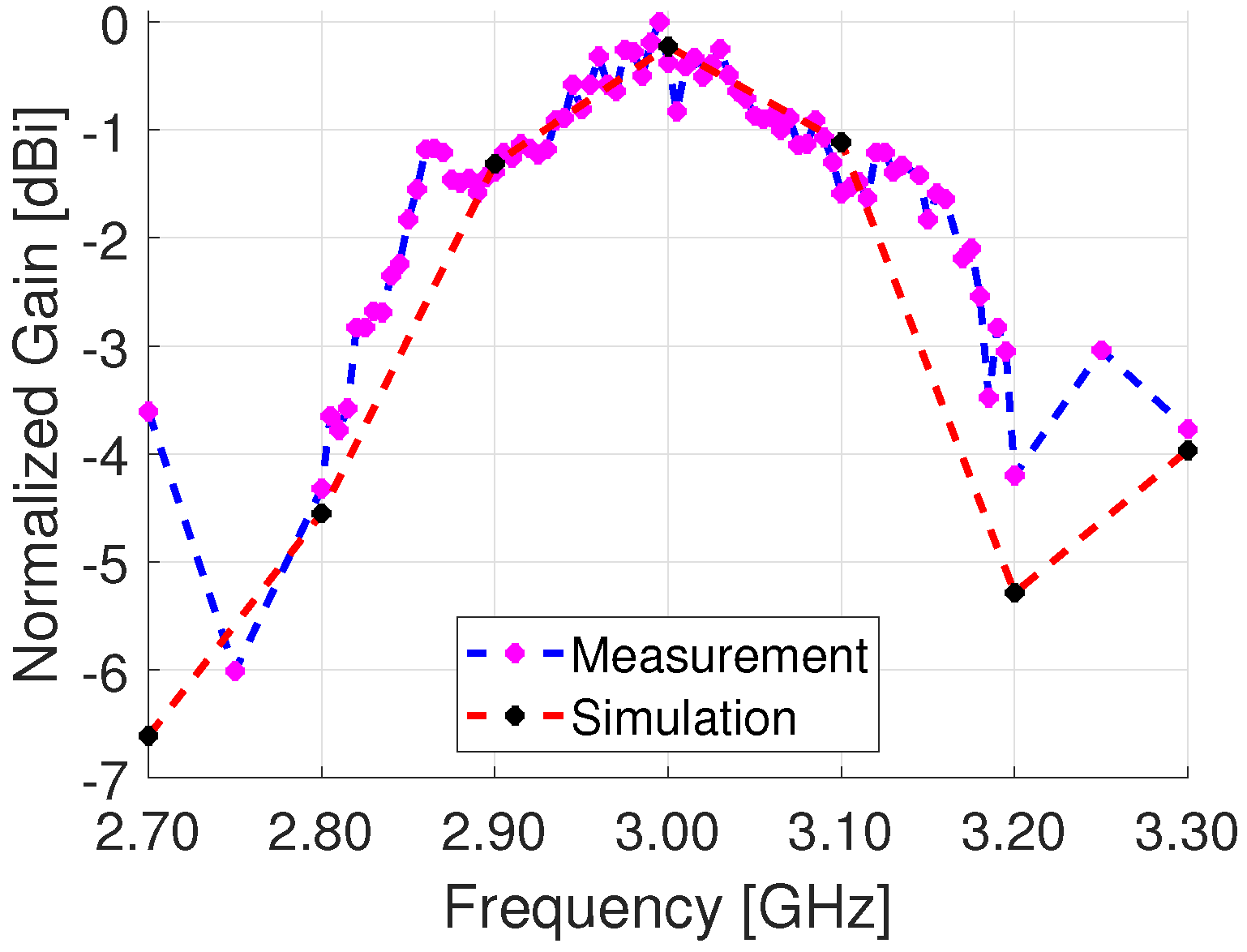
4.4. Comparison with Simulation
5. Discussion
5.1. Power Budget
5.2. Comparison with Other Works
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Balanis, C. Antenna Theory: Analysis and Design, 4th ed.; Wiley: Hoboken, NJ, USA, 2016; pp. 285–293. [Google Scholar]
- Meng, X.; Nekovee, M.; Wu, D. The Design and Analysis of Electronically Reconfigurable Liquid Crystal-Based Reflectarray Metasurface for 6G Beamforming, Beamsteering, and Beamsplitting. IEEE Access 2021, 9, 155564–155575. [Google Scholar] [CrossRef]
- Li, W.; Wang, Y.M.; Hei, Y.; Li, B.; Shi, X. A Compact Low-Profile Reconfigurable Metasurface Antenna with Polarization and Pattern Diversities. IEEE Antennas Wirel. Propag. Lett. 2021, 20, 1170–1174. [Google Scholar] [CrossRef]
- Zhu, H.L.; Liu, X.H.; Cheung, S.W.; Yuk, T.I. Frequency-Reconfigurable Antenna Using Metasurface. IEEE Trans. Antennas Propag. 2014, 62, 80–85. [Google Scholar] [CrossRef]
- Wang, M.; Liao, D.; Dai, J.Y.; Chan, C.H. Dual-Polarized Reconfigurable Metasurface for Multifunctional Control of Electromagnetic Waves. IEEE Trans. Antennas Propag. 2022, 70, 4539–4548. [Google Scholar] [CrossRef]
- Rao, J.; Zhang, Y.; Tang, S.; Li, Z.; Shen, S.; Chiu, C.Y.; Murch, R. A Novel Reconfigurable Intelligent Surface for Wide-Angle Passive Beamforming. IEEE Trans. Microw. Theory Tech. 2022, 70, 5427–5439. [Google Scholar] [CrossRef]
- Ibrahim, E.; Nilsson, R.; Van De Beek, J. Binary Polarization Shift Keying with Reconfigurable Intelligent Surfaces. IEEE Wirel. Commun. Lett. 2022, 11, 908–912. [Google Scholar] [CrossRef]
- Lin, H.; Yu, W.; Tang, R.; Jin, J.; Wang, Y.; Xiong, J.; Wu, Y.; Zhao, J. A dual-band reconfigurable intelligent metasurface with beam steering. J. Phys. Appl. Phys. 2022, 55, 245002. [Google Scholar] [CrossRef]
- Zou, X.; Yao, J.; Chung, K.L.; Lai, G.; Zeng, W.; Gu, W. A Comparative Study Between Reconfigurable Intelligent Surface and Reflectarray Antenna. In Proceedings of the 2022 IEEE 5th International Conference on Electronic Information and Communication Technology (ICEICT), Hefei, China, 21–23 August 2022; pp. 846–848. [Google Scholar] [CrossRef]
- Björnson, E.; Wymeersch, H.; Matthiesen, B.; Popovski, P.; Sanguinetti, L.; de Carvalho, E. Reconfigurable Intelligent Surfaces: A signal processing perspective with wireless applications. IEEE Signal Process. Mag. 2022, 39, 135–158. [Google Scholar] [CrossRef]
- Cheng, Q.; Zhang, L.; Dai, J.Y.; Tang, W.; Ke, J.C.; Liu, S.; Liang, J.C.; Jin, S.; Cui, T.J. Reconfigurable Intelligent Surfaces: Simplified-Architecture Transmitters—From Theory to Implementations. Proc. IEEE 2022, 110, 1266–1289. [Google Scholar] [CrossRef]
- Abb, M.; Wang, Y.; de Groot, C.H.; Muskens, O.L. Hotspot-mediated ultrafast nonlinear control of multifrequency plasmonic nanoantennas. Nat. Commun. 2014, 5, 4869. [Google Scholar] [CrossRef]
- Reis, J.R.; Vala, M.; Caldeirinha, R.F.S. Review Paper on Transmitarray Antennas. IEEE Access 2019, 7, 94171–94188. [Google Scholar] [CrossRef]
- Huang, C.; Pan, W.; Ma, X.; Zhao, B.; Cui, J.; Luo, X. Using Reconfigurable Transmitarray to Achieve Beam-Steering and Polarization Manipulation Applications. IEEE Trans. Antennas Propag. 2015, 63, 4801–4810. [Google Scholar] [CrossRef]
- Lau, J.Y.; Hum, S.V. A Wideband Reconfigurable Transmitarray Element. IEEE Trans. Antennas Propag. 2012, 60, 1303–1311. [Google Scholar] [CrossRef]
- Nicholls, J.G.; Hum, S.V. Full-Space Electronic Beam-Steering Transmitarray with Integrated Leaky-Wave Feed. IEEE Trans. Antennas Propag. 2016, 64, 3410–3422. [Google Scholar] [CrossRef]
- Reis, J.R.; Caldeirinha, R.F.S.; Hammoudeh, A.; Copner, N. Electronically Reconfigurable FSS-Inspired Transmitarray for 2-D Beamsteering. IEEE Trans. Antennas Propag. 2017, 65, 4880–4885. [Google Scholar] [CrossRef]
- Di Palma, L.; Clemente, A.; Dussopt, L.; Sauleau, R.; Potier, P.; Pouliguen, P. Circularly-Polarized Reconfigurable Transmitarray in Ka-Band with Beam Scanning and Polarization Switching Capabilities. IEEE Trans. Antennas Propag. 2017, 65, 529–540. [Google Scholar] [CrossRef]
- Luo, C.W.; Zhao, G.; Jiao, Y.C.; Chen, G.T.; Yan, Y.D. Wideband 1 bit Reconfigurable Transmitarray Antenna Based on Polarization Rotation Element. IEEE Antennas Wirel. Propag. Lett. 2021, 20, 798–802. [Google Scholar] [CrossRef]
- Wan, X.; Xiao, Q.; Zhang, Y.Z.; Li, Y.; Eisenbeis, J.; Wang, J.W.; Huang, Z.A.; Liu, H.X.; Zwick, T.; Cui, T.J. Reconfigurable Sum and Difference Beams Based on a Binary Programmable Metasurface. IEEE Antennas Wirel. Propag. Lett. 2021, 20, 381–385. [Google Scholar] [CrossRef]
- Wang, Y.; Xu, S.; Yang, F.; Li, M. A Novel 1 Bit Wide-Angle Beam Scanning Reconfigurable Transmitarray Antenna Using an Equivalent Magnetic Dipole Element. IEEE Trans. Antennas Propag. 2020, 68, 5691–5695. [Google Scholar] [CrossRef]
- Wang, Z.X.; Yang, H.; Shao, R.; Wu, J.W.; Liu, G.; Zhai, F.; Cheng, Q.; Cui, T.J. A Planar 4-Bit Reconfigurable Antenna Array Based on the Design Philosophy of Information Metasurfaces. Engineering 2022, 17, 64–74. [Google Scholar] [CrossRef]
- Huang, C.; Pan, W.; Luo, X. Low-Loss Circularly Polarized Transmitarray for Beam Steering Application. IEEE Trans. Antennas Propag. 2016, 64, 4471–4476. [Google Scholar] [CrossRef]
- Li, X.; Yang, H.; Shao, R.; Zhai, F.; Liu, G.; Wang, Z.X.; Gao, H.F.; Fan, G.; Wu, J.W.; Cheng, Q.; et al. Low-Cost and High-Performance 5-Bit Programmable Phased Array at Ku-Band. Prog. Electromagn. Res. 2022, 175, 29–43. [Google Scholar] [CrossRef]
- Tang, J.; Xu, S.; Yang, F.; Li, M. Design and Measurement of a Reconfigurable Transmitarray Antenna with Compact Varactor-Based Phase Shifters. IEEE Antennas Wirel. Propag. Lett. 2021, 20, 1998–2002. [Google Scholar] [CrossRef]
- Yang, H.; Yang, F.; Xu, S.; Li, M.; Cao, X.; Gao, J.; Zheng, Y. A Study of Phase Quantization Effects for Reconfigurable Reflectarray Antennas. IEEE Antennas Wirel. Propag. Lett. 2017, 16, 302–305. [Google Scholar] [CrossRef]
- Vilenskiy, A.; Makurin, M.; Poshisholina, E.; Lee, C. Design Technique for Varactor Analog Phase Shifters with Equalized Losses. Prog. Electromagn. Res. C 2018, 86, 1–16. [Google Scholar] [CrossRef]
- Burdin, F.; Iskandar, Z.; Podevin, F.; Ferrari, P. Design of Compact Reflection-Type Phase Shifters with High Figure-of-Merit. IEEE Trans. Microw. Theory Tech. 2015, 63, 1883–1893. [Google Scholar] [CrossRef]
- Cory, R. The Nuts and Bolts of Tuning Varactors; Technical Report; Skyworks Solutions, Inc.: Irvine, CA, USA, 2009; Available online: https://www.highfrequencyelectronics.com/Feb09/HFE0209_Cory.pdf (accessed on 11 August 2022).
- Macom. Solderable GaAs Constant Gamma Flip-Chip Varactor Diode. Available online: https://cdn.macom.com/datasheets/MAVR-000120-1411.pdf (accessed on 11 August 2022).
- Schühler, M.; Schmidt, C.; Weber, J.; Wansch, R.; Hein, M. Phase shifters based on PIN-diodes and varactors: Two concepts by comparison. In Information Technology and Electrical Engineering-Devices and Systems, Materials and Technologies for the Future; Faculty of Electrical and Information Technology, Technische Universität Ilmenau: Ilmenau, Germany, 2009; Volume 51, p. 2006. [Google Scholar]
- Kirillov, V.; Kozlov, D.; Bulja, S. Series vs. Parallel Reflection-Type Phase Shifters. IEEE Access 2020, 8, 189276–189286. [Google Scholar] [CrossRef]
- Macom. MA4AGP907 MA4AGFCP910. Available online: https://cdn.macom.com/datasheets/MA4AGP907__MA4AGFCP910.pdf (accessed on 11 August 2022).
- Usta, E.; Türker Tokan, N. Effects of Surface Finish Material on Millimeter-Wave Antenna Performance. IEEE Trans. Compon. Packag. Manuf. Technol. 2019, 9, 815–821. [Google Scholar] [CrossRef]
- Taconic. Advanced PCB Materials Product Selection Guide. Available online: http://www.taconic.co.kr/download/ProductSelectionGuide.pdf (accessed on 19 December 2022).









| Parameter | Value |
|---|---|
| 1.23 nH | |
| 1.23 pF | |
| 1.92 V | |
| m | 2.06 |
| 0.25 pF |
| Row 3 | Row 4 | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| Unit Cell | Calculated | Measured | Voltage | Error | Unit Cell | Calculated | Measured | Voltage | Error |
| B12 | 0.0 | 0.0 | 4.4 V | 0.0 | B11 | 0.0 | 0.0 | 4.4 V | 0.0 |
| B22 | 25.0 | 23.3 | 5.3 V | 1.7 | B21 | 25.0 | 25.3 | 5.3 V | −0.3 |
| B32 | 50.0 | 49.2 | 7.0 V | 0.8 | B31 | 50.0 | 50.3 | 7.0 V | −0.3 |
| B42 | 75.0 | 75.0 | 12.0 V | 0.0 | B42 | 75.0 | 79.7 | 12.0 V | −4.6 |
| Row 3 | Row 4 | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| Unit Cell | Calculated | Measured | Voltage | Error | Unit Cell | Calculated | Measured | Voltage | Error |
| B12 | 0.0 | 0.0 | 1.9 V | 0.0 | B11 | 0.0 | 0.0 | 1.9 V | 0.0 |
| B22 | 92.6 | 97.7 | 2.7 V | −5.2 | B21 | 92.6 | 94.5 | 2.7 V | −1.9 |
| B32 | 185.1 | 174.9 | 4.0 V | 10.2 | B31 | 185.1 | 168.8 | 4.0 V | 16.3 |
| B42 | 277.7 | 270.5 | 12.0 V | 7.2 | B42 | 277.7 | 269.0 | 12.0 V | 8.7 |
| Target Angle | Measured Angle | Angle Error | Control Angle | Peak Gain | SLL |
|---|---|---|---|---|---|
| 4.4 dBi | −11.4 dB | ||||
| 4.7 dBi | −12.0 dB | ||||
| 4.6 dBi | −11.4 dB | ||||
| 4.6 dBi | −10.6 dB | ||||
| 3.8 dBi | −8.5 dB | ||||
| 3.4 dBi | −7.4 dB | ||||
| 3.4 dBi | −7.2 dB | ||||
| 3.1 dBi | −7.6 dB | ||||
| 3.0 dBi | −7.4 dB | ||||
| 2.8 dBi | −7.8 dB |
| Parameter | Value |
|---|---|
| Aperture size | 160 mm × 160 mm |
| Ideal Directivity | 15.1 dBi |
| Measured gain | 4.4 dBi |
| Feed network loss | 0.8 dB |
| Phase shifter loss | 0.8 dB |
| PIN diode loss | 0.4 dB |
| Fabrication tolerance losses | 0.8 dB |
| ENIG and conductor loss | 5.8 dB |
| Surface roughness | 2.1 dB |
| Reference | Peak Gain | Steered Gain (Angle) | Scan Loss | Max Steering Error | Phase Profile | Characterization | Frequency | Array Size | Aperture Efficiency |
|---|---|---|---|---|---|---|---|---|---|
| [18] | 20.8 dBi | 18.3 dBi (-40) | 2.5 dB | N/A | Discrete | Single, waveguide | 29 GHz | 20 × 20 | 9.5% |
| [14] | 17.0 dBi | 14.9 dBi (40) | 2.1 dB | N/A | Continuous | Datasheet 1 | 5.4 GHz | 8 × 8 | 28.5% |
| [23] | 14.6 dBi | 12.9 dBi (45) | 1.9 dB | N/A | Continuous & Discrete | Datasheet 1 | 4.8 GHz | 6 × 6 | 27.6% |
| [15] | 15.0 dBi | 13.4 dBi (40) | 1.6 dB | N/A | Continuous | Individual, method unclear | 5.0 GHz | 6 × 6 | 28.2% |
| [24] | 13.8 dBi | N/A (60) | 2.8 dB | 0.9 | Discrete | Method unclear | 14.8 GHz | 24 × 2 | 6.3% |
| [19] | 16.8 dBi | 14.5 dB (40) | 2.3 dB | N/A | Discrete | Datasheet 1 | 5.0 GHz | 16 × 16 | 18.4% |
| [16] | 15.6 dBi | 13.4 dB (45) | 2.2 dB | 1.9 | Continuous | Individual, near field probe | 4.8 GHz | 6 × 6 | 34.0% |
| [17] | 19.9 dBi | N/A | N/A | 16 | Continuous | Single unit cell | 5.2 GHz | 5 × 5 | N/A |
| [25] | 23.7 dBi | 20.0 dBi (−45) | 3.7 dB | N/A | Continuous and discrete | Datasheet 1 | 5.6 GHz | 16 × 16 | 33.5% |
| [20] | 21.3 dBi | 15.4 dBi (60) | 5.9 dB | N/A | Discrete | Datasheet 1 | 28 GHz | 20 × 20 | 12.5% |
| [21] | 21.4 dBi | 19.9 dBi (40) | 1.5 dB | N/A | Discrete | Datasheet 1 | 13.5 GHz | 16 × 16 | 14.8% |
| [22] | 13.4 dBi | 10.7 dBi (45) | 2.7 dB | 0.8 | Discrete | Single, PIN & phase shifter measurement | 12 GHz | 16 × 2 | 24.5% |
| This work | 4.7 dBi | 3.0 dBi (40) | 1.7 dB | 2 | Continuous | Measurement, average phase shift | 3 GHz | 8.6% | |
| 2.8 dBi (45) | 1.9 dB |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Verho, S.; Nguyen, V.T.; Chung, J.-Y. A 4 × 4 Active Antenna Array with Adjustable Beam Steering. Sensors 2023, 23, 1324. https://doi.org/10.3390/s23031324
Verho S, Nguyen VT, Chung J-Y. A 4 × 4 Active Antenna Array with Adjustable Beam Steering. Sensors. 2023; 23(3):1324. https://doi.org/10.3390/s23031324
Chicago/Turabian StyleVerho, Sebastian, Van Thang Nguyen, and Jae-Young Chung. 2023. "A 4 × 4 Active Antenna Array with Adjustable Beam Steering" Sensors 23, no. 3: 1324. https://doi.org/10.3390/s23031324
APA StyleVerho, S., Nguyen, V. T., & Chung, J.-Y. (2023). A 4 × 4 Active Antenna Array with Adjustable Beam Steering. Sensors, 23(3), 1324. https://doi.org/10.3390/s23031324





