Generation of Mixed-OAM-Carrying Waves Using Huygens’ Metasurface for Mm-Wave Applications
Abstract
1. Introduction
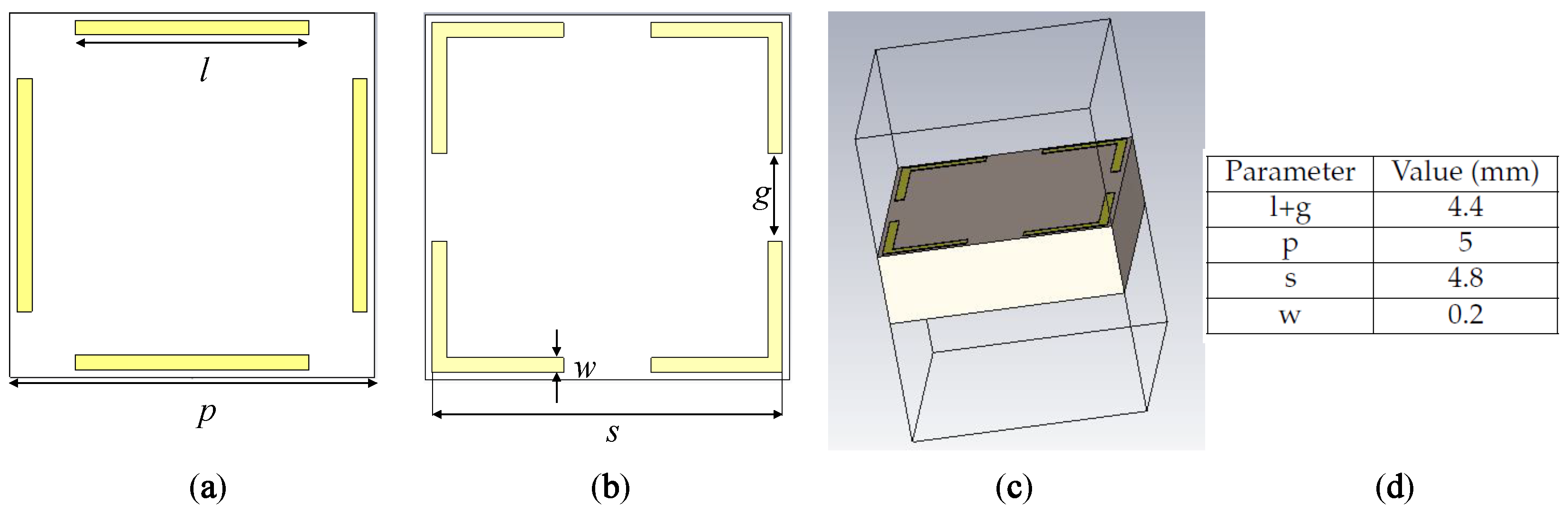
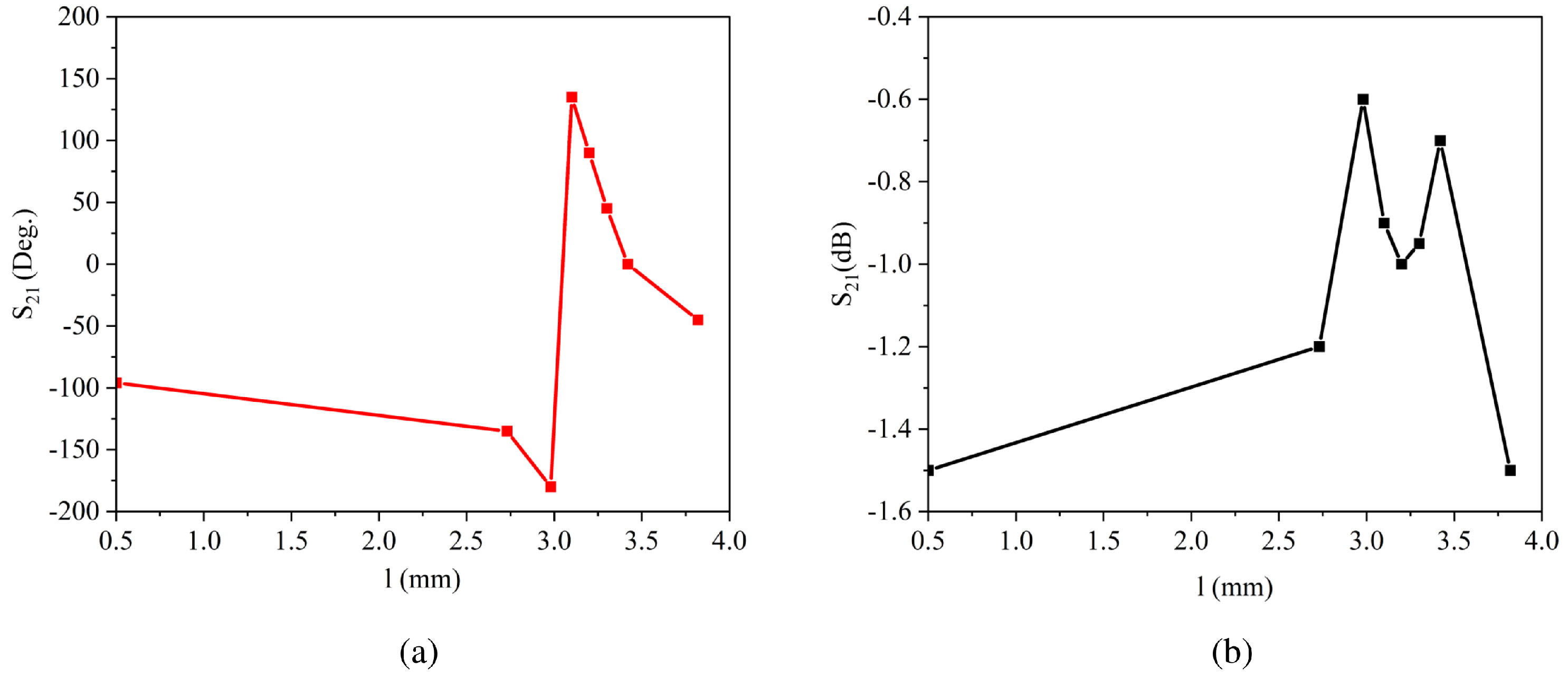
2. Unit Cell Characteristics
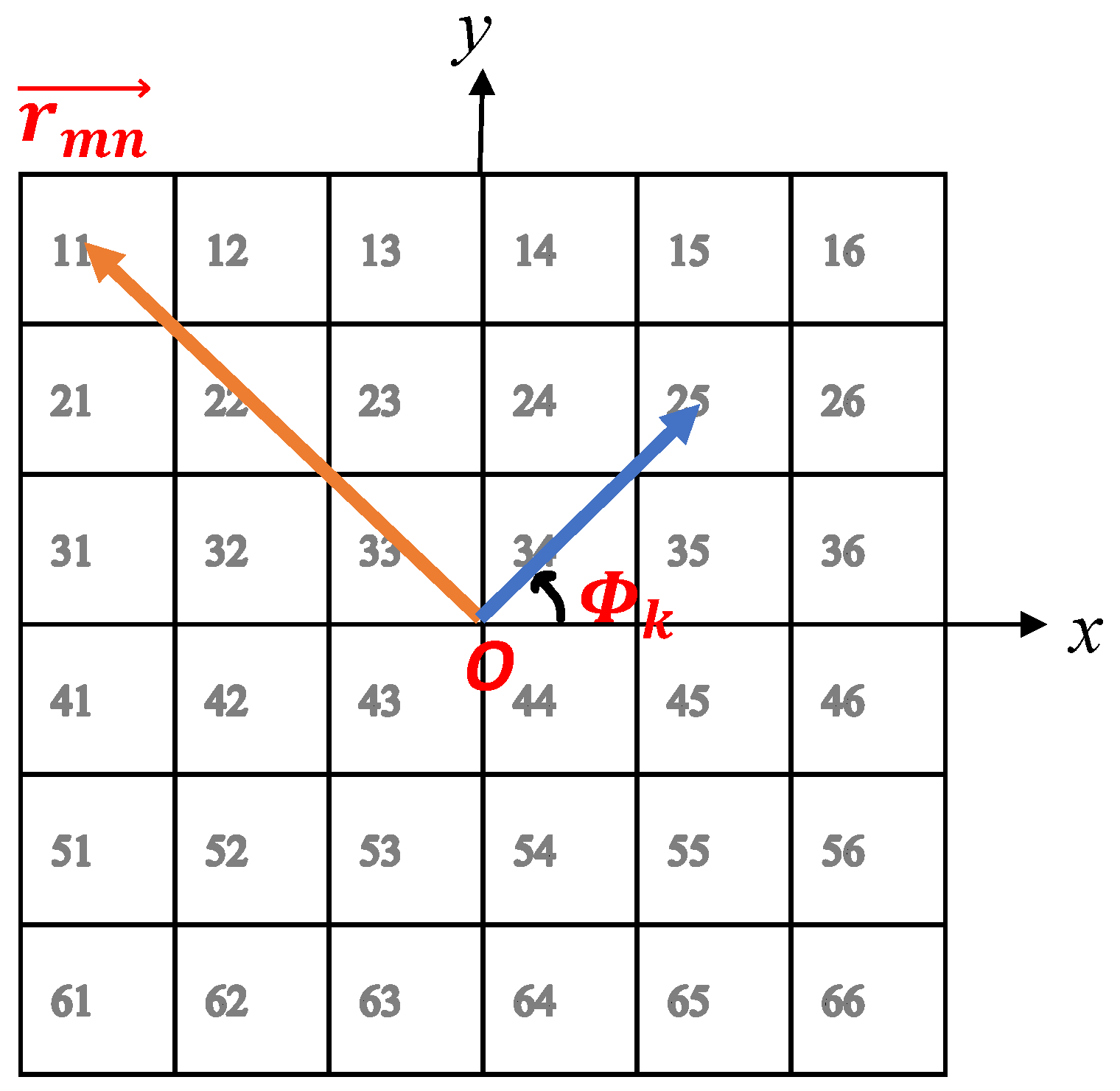
3. Mixed-OAM-Generating TA Design
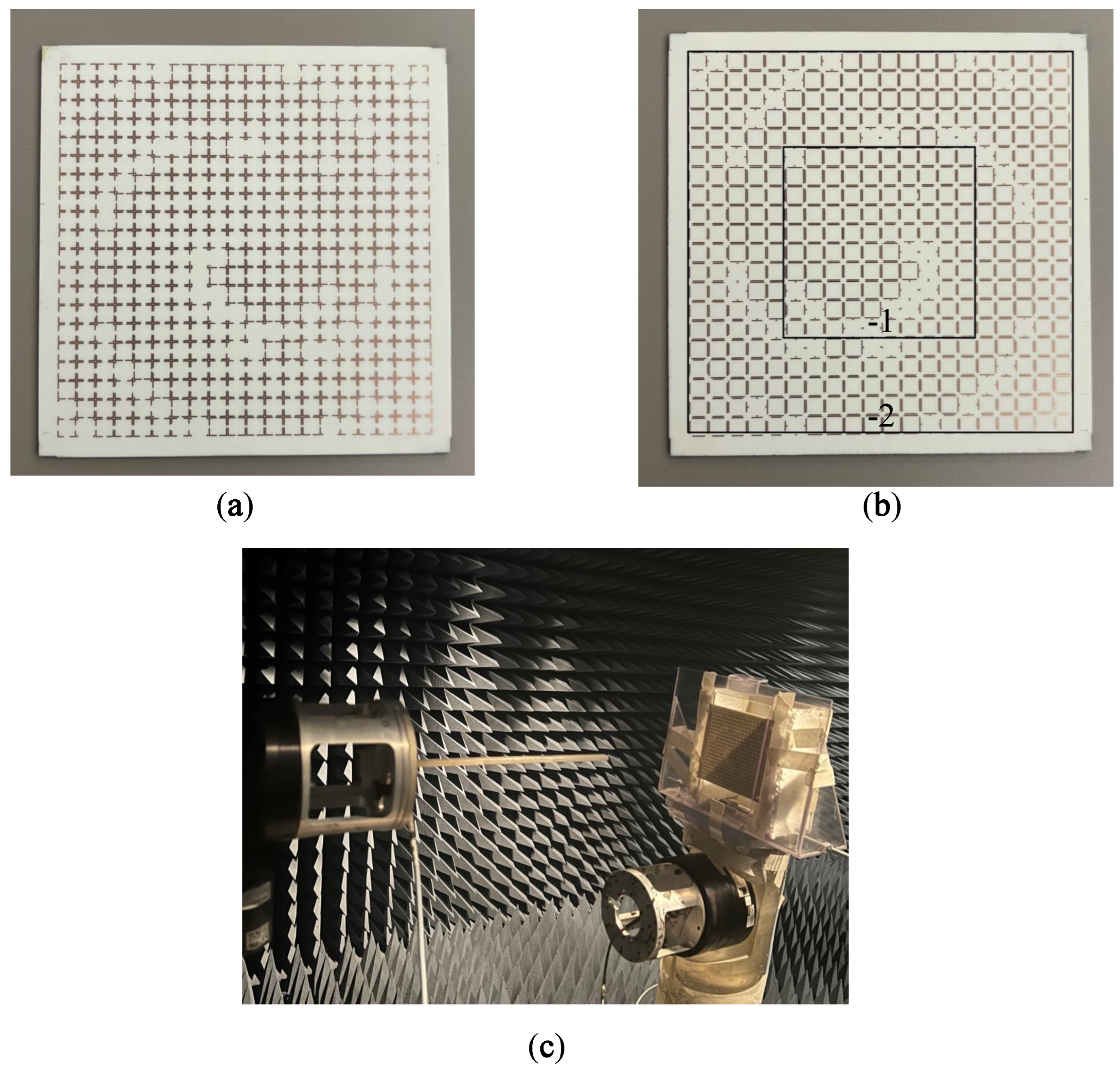
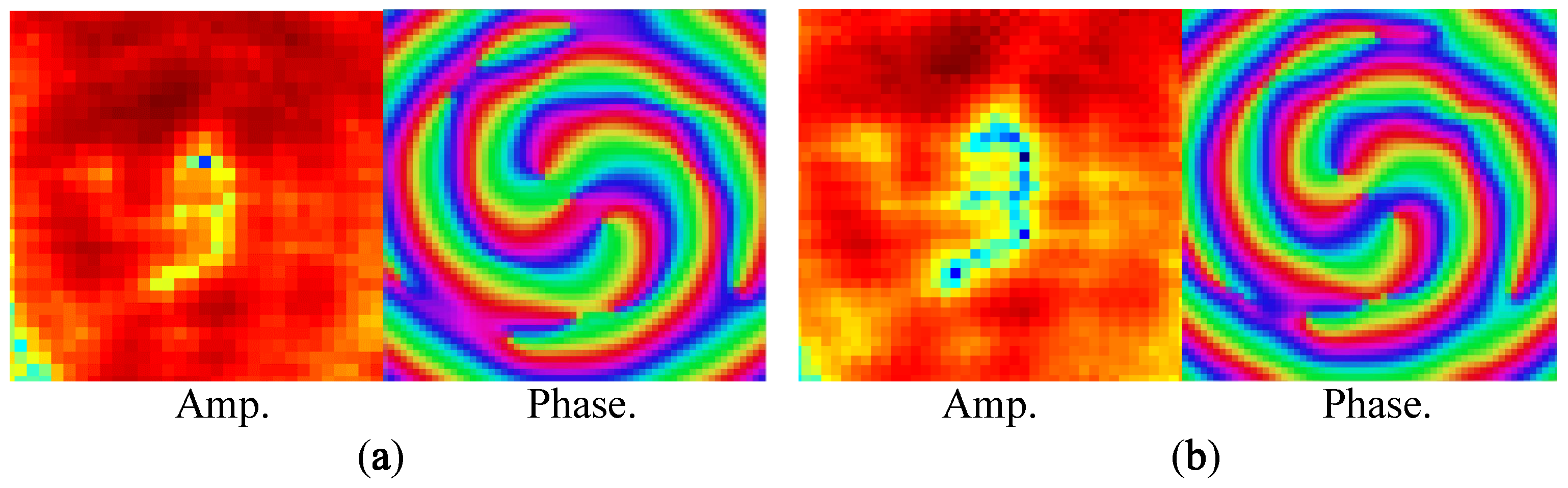
4. Experimental Verification
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Yan, Y.; Xie, G.; Lavery, M.P.J.; Huang, H.; Ahmed, N.; Bao, C.; Ren, Y.; Cao, Y.; Li, L.; Zhao, Z.; et al. High-capacity millimetre-wave communications with orbital angular momentum multiplexing. Nat. Commun. 2014, 5, 4876. [Google Scholar] [CrossRef] [PubMed]
- Hui, X.; Zheng, S.; Chen, Y.; Hu, Y.; Jin, X.; Chi, H.; Zhang, X. Multiplexed millimeter wave communication with dual orbital angular momentum (OAM) mode antennas. Sci. Rep. 2015, 5, 10148. [Google Scholar] [CrossRef] [PubMed]
- Liu, Q.; Chen, Z.N.; Liu, Y.; Li, F.; Chen, Y.; Mo, Z. Circular polarization and mode reconfigurable wideband orbital angular momentum patch array antenna. IEEE Trans. Antennas Propag. 2018, 66, 1796–1804. [Google Scholar] [CrossRef]
- Li, H.; Kang, L.; Dong, K. Generating tunable orbital angular momentum radio beams with dual-circular-polarization and dual-mode characteristics. IEEE Access 2020, 8, 211248–211254. [Google Scholar] [CrossRef]
- Liu, B.; Lin, G.; Cui, Y.; Li, R. An orbital angular momentum (OAM) mode reconfigurable antenna for channel capacity improvement and digital data encoding. Sci. Rep. 2017, 7, 9852. [Google Scholar] [CrossRef]
- Kang, L.; Li, H.; Zhou, J.; Zheng, S. An OAM-mode reconfigurable array antenna with polarization agility. IEEE Access 2020, 8, 40445–40452. [Google Scholar] [CrossRef]
- Li, W.; Zhang, L.; Yang, S.; Zhuo, K.; Ye, L.; Liu, Q.H. A reconfigurable second-order OAM patch antenna with simple structure. IEEE Antennas Wirel. Propag. Lett. 2020, 19, 1531–1535. [Google Scholar] [CrossRef]
- Huang, H.; Zhang, Z. A single fed wideband mode-reconfigurable OAM metasurface CP antenna array with simple feeding scheme. Int. J. RF Microw. Comput.-Aided Eng. 2021, 31, e22499. [Google Scholar] [CrossRef]
- Wu, J.; Zhang, Z.; Ren, X.; Huang, Z.; Wu, X. A broadband electronically mode-reconfigurable orbital angular momentum metasurface antenna. IEEE Antennas Wirel. Propag. Lett. 2019, 18, 1482–1486. [Google Scholar] [CrossRef]
- Kang, L.; Li, H.; Zhou, J.; Zheng, S.; Gao, S. A mode-reconfigurable orbital angular momentum antenna with simplified feeding scheme. IEEE Trans. Antennas Propag. 2019, 67, 4866–4871. [Google Scholar] [CrossRef]
- Wang, Z.; Pan, X.; Yang, F.; Xu, S.; Li, M. Real-time mode switching and beam scanning of high-gain OAM waves using a 1-bit reconfigurable reflectarray antenna. Electronics 2020, 9, 2181. [Google Scholar] [CrossRef]
- Liu, B.; Li, S.; He, Y.; Li, Y.; Wong, S.-W. Generation of an orbital-angular-momentum-mode-reconfigurable beam by a broadband 1-bit electronically reconfigurable transmitarray. Phys. Rev. Appl. 2021, 15, 044035. [Google Scholar] [CrossRef]
- Li, L.; Zhou, X. Mechanically reconfigurable single-arm spiral antenna array for generation of broadband circularly polarized orbital angular momentum vortex waves. Sci. Rep. 2018, 8, 5128. [Google Scholar] [CrossRef] [PubMed]
- Liao, Z.; Che, Y.; Liu, L.; Pan, B.C.; Cai, B.G.; Zhou, J.N.; Luo, G.Q.; Liu, Y. Reconfigurable Vector Vortex Beams Using Spoof Surface Plasmon Ring Resonators. IEEE Trans. Antennas Propag. 2022, 70, 6795–6803. [Google Scholar] [CrossRef]
- Ming, J.; Shi, Y. A mode reconfigurable orbital angular momentum water antenna. IEEE Access 2020, 8, 89152–89160. [Google Scholar] [CrossRef]
- Naseri, H.; PourMohammadi, P.; Melouki, N.; Iqbal, A.; Denidni, T.A. A Low-profile Antenna System for Generating Reconfigurable OAM-carrying Beams. IEEE Antennas Wirel. Propag. Lett. 2022, 22, 402–406. [Google Scholar] [CrossRef]
- Li, H.; Kang, L.; Wei, F.; Cai, Y.-M.; Yin, Y.-Z. A low-profile dual-polarized microstrip antenna array for dual-mode OAM applications. IEEE Antennas Wirel. Propag. Lett. 2017, 16, 3022–3025. [Google Scholar] [CrossRef]
- Qin, F.; Li, L.; Liu, Y.; Cheng, W.; Zhang, H. A four-mode OAM antenna array with equal divergence angle. IEEE Antennas Wirel. Propag. Lett. 2019, 18, 1941–1945. [Google Scholar] [CrossRef]
- Zhang, Y.-M.; Li, J.-L. Analyses and full-duplex applications of circularly polarized OAM arrays using sequentially rotated configuration. IEEE Trans. Antennas Propag. 2018, 66, 7010–7020. [Google Scholar] [CrossRef]
- Xu, X.; Mazzinghi, A.; Freni, A.; Hirokawa, J. Simultaneous generation of three OAM modes by using a RLSA fed by a waveguide circuit for 60 GHz-band radiative near-field region OAM multiplexing. IEEE Trans. Antennas Propag. 2020, 69, 1249–1259. [Google Scholar] [CrossRef]
- Yoo, J.-U.; Son, H.-W. Quad-mode radial uniform circular array antenna for OAM multiplexing. IET Microw. Antennas Propag. 2020, 14, 728–733. [Google Scholar] [CrossRef]
- Guo, Z.-G.; Yang, G.-M. Radial uniform circular antenna array for dual-mode OAM communication. IEEE Antennas Wirel. Propag. Lett. 2016, 16, 404–407. [Google Scholar] [CrossRef]
- Palacin, B.; Sharshavina, K.; Nguyen, K.; Capet, N. An 8 × 8 Butler matrix for generation of waves carrying Orbital Angular Momentum (OAM). In Proceedings of the 8th European Conference on Antennas and Propagation (EuCAP 2014), Hague, The Netherlands, 6–11 April 2014; pp. 2814–2818. [Google Scholar]
- Bai, X.-D.; Liang, X.-L.; Sun, Y.-T.; Hu, P.-C.; Yao, Y.; Wang, K.; Geng, J.-P.; Jin, R.-H. Experimental array for generating dual circularly-polarized dual-mode OAM radio beams. Sci. Rep. 2017, 7, 40099. [Google Scholar] [CrossRef] [PubMed]
- Liu, D.; Gui, L.; Zhang, Z.; Chen, H.; Song, G.; Jiang, T. Multiplexed OAM wave communication with two-OAM-mode antenna systems. IEEE Access 2018, 7, 4160–4166. [Google Scholar] [CrossRef]
- Mazzinghi, A.; Freni, A. Simultaneous generation of pseudo-Bessel vortex modes with a RLSA. IEEE Antennas Wirel. Propag. Lett. 2017, 16, 1747–1750. [Google Scholar] [CrossRef]
- Huang, Y.; Qi, Z.; Li, Q.; Zhu, H.; Li, X.; Li, X.; Jiang, X. A broadband multi-OAM-mode four-feed circular patch antenna with high mode purity. In Proceedings of the 2018 IEEE Asia-Pacific Conference on Antennas and Propagation (APCAP), Auckland, New Zealand, 5–8 August 2018; pp. 494–495. [Google Scholar]
- Singh, S.; Upadhayay, M.D.; Pal, S. OAM wave generation using square-shaped patch antenna as slot array equivalence. IEEE Antennas Wirel. Propag. Lett. 2020, 19, 680–684. [Google Scholar] [CrossRef]
- Guo, C.; Zhao, X.; Zhu, C.; Xu, P.; Zhang, Y. An OAM patch antenna design and its array for higher order OAM mode generation. IEEE Antennas Wirel. Propag. Lett. 2019, 18, 816–820. [Google Scholar] [CrossRef]
- Tang, H.; Yu, C.; Xiao, S.; Yi, Z.; Wu, Y.; Kang, K. Single-Fed OAM antenna based on half-mode substrate integrated waveguide. In Proceedings of the 2018 IEEE Asia-Pacific Conference on Antennas and Propagation (APCAP), Auckland, New Zealand, 5–8 August 2018; pp. 116–117. [Google Scholar]
- Zhang, W.; Zheng, S.; Hui, X.; Chen, Y.; Jin, X.; Chi, H.; Zhang, X. Four-OAM-mode antenna with traveling-wave ring-slot structure. IEEE Antennas Wirel. Propag. Lett. 2016, 16, 194–197. [Google Scholar] [CrossRef]
- Liang, J.; Zhang, S. Orbital angular momentum (OAM) generation by cylinder dielectric resonator antenna for future wireless communications. IEEE Access 2016, 4, 9570–9574. [Google Scholar] [CrossRef]
- Yang, Y.; Wang, Z.; Wang, S.; Zhou, Q.; Shen, F.; Jiang, H.; Wu, Z.; Zeng, B.; Guo, Z.; Gong, Y. Designing a water-immersed rectangular horn antenna for generating underwater OAM waves. Electronics 2019, 8, 1224. [Google Scholar] [CrossRef]
- Wang, L.; Chen, H.; Guo, K.; Shen, F.; Guo, Z. An inner-and outer-fed dual-arm archimedean spiral antenna for generating multiple orbital angular momentum modes. Electronics 2019, 8, 251. [Google Scholar] [CrossRef]
- Zheng, S.; Hui, X.; Jin, X.; Chi, H.; Zhang, X. Transmission characteristics of a twisted radio wave based on circular traveling-wave antenna. IEEE Trans. Antennas Propag. 2015, 63, 1530–1536. [Google Scholar] [CrossRef]
- Zhang, Q.; Chen, W.; Sun, H.; Fu, J.; Zhao, Y.; Feng, H.; Zhang, K. A Circular-Polarized Vortex Beams Generation with Orbital Angular Momentum Based on A Leaky-wave Antenna. IEEE Antennas Wirel. Propag. Lett. 2023, 3241248. [Google Scholar] [CrossRef]
- Esfandiyari, M.; Lalbakhsh, A.; Jarchi, S.; Ghaffari-Miab, M.; Mahtaj, H.N.; Simorangkir, R.B.V.B. Tunable terahertz filter/antenna-sensor using graphene-based metamaterials. Mater. Des. 2022, 220, 110855. [Google Scholar] [CrossRef]
- Lalbakhsh, A.; Afzal, M.U.; Hayat, T.; Esselle, K.P.; Mandal, K. All-metal wideband metasurface for near-field transformation of medium-to-high gain electromagnetic sources. Sci. Rep. 2021, 11, 9421. [Google Scholar] [CrossRef] [PubMed]
- Das, P.; Mandal, K.; Lalbakhsh, A. Beam-steering of microstrip antenna using single-layer FSS based phase-shifting surface. Int. J. RF Microw. Comput.-Aided Eng. 2022, 32, e23033. [Google Scholar] [CrossRef]
- Adibi, S.; Honarvar, M.A.; Lalbakhsh, A. Gain enhancement of wideband circularly polarized UWB antenna using FSS. Radio Sci. 2021, 56, 1–8. [Google Scholar] [CrossRef]
- Lalbakhsh, A.; Afzal, M.U.; Esselle, K.P.; Smith, S.L. All-metal wideband frequency-selective surface bandpass filter for TE and TM polarizations. IEEE Trans. Antennas Propag. 2022, 70, 2790–2800. [Google Scholar] [CrossRef]
- Wang, L.; Shi, H.; Yi, J.; Dong, L.; Liu, H.; Zhang, A.; Xu, Z. Suspended Metasurface for Broadband High-Efficiency Vortex Beam Generation. Materials 2022, 15, 707. [Google Scholar] [CrossRef]
- Qin, F.; Wan, L.; Li, L.; Zhang, H.; Wei, G.; Gao, S. A transmission metasurface for generating OAM beams. IEEE Antennas Wirel. Propag. Lett. 2018, 17, 1793–1796. [Google Scholar] [CrossRef]
- Xu, J.; Bi, K.; Zhang, R.; Hao, Y.; Lan, C.; McDonald-Maier, K.D.; Zhai, X.; Zhang, Z.; Huang, S. A small-divergence-angle orbital angular momentum metasurface antenna. Research 2019, 2019, 9686213. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Li, X.; Chen, L.; Pu, M.; Jin, J.; Hong, M.; Luo, X. Orbital angular momentum multiplexing and demultiplexing by a single metasurface. Adv. Opt. Mater. 2017, 5, 1600502. [Google Scholar] [CrossRef]
- Yang, X.; Zhou, Y.; Wang, G. A wideband transmission metasurface for generating Bessel beam carrying orbital angular momentum. Int. J. RF Microw. Comput.-Aided Eng. 2019, 29, e21941. [Google Scholar] [CrossRef]
- Huang, H.-F.; Huang, H.-M. Millimeter-wave wideband high efficiency circular Airy OAM multibeams with multiplexing OAM modes based on transmission metasurfaces. Prog. Electromagn. Res. 2022, 173, 151–159. [Google Scholar] [CrossRef]
- Wang, H.; Yu, S.; Kou, N.; Ding, Z.; Zhang, Z. Cylindrical holographic impedance metasurface for OAM vortex wave generation. Appl. Phys. Lett. 2022, 120, 143504. [Google Scholar] [CrossRef]
- Akram, M.R.; Bai, X.; Jin, R.; Vandenbosch, G.A.E.; Premaratne, M.; Zhu, W. Photon spin Hall effect-based ultra-thin transmissive metasurface for efficient generation of OAM waves. IEEE Trans. Antennas Propag. 2019, 67, 4650–4658. [Google Scholar] [CrossRef]
- Lv, H.-H.; Huang, Q.-L.; Yi, X.-J.; Hou, J.-Q.; Shi, X.-W. Low-profile transmitting metasurface using single dielectric substrate for OAM generation. IEEE Antennas Wirel. Propag. Lett. 2020, 19, 881–885. [Google Scholar] [CrossRef]
- Shi, H.; Wang, L.; Li, G.; Yi, J.; Liu, H.; Zhang, A.; Xu, Z. Guided-Wave Inspired Metasurfaces for Multifunctional Vortex Beam Generation and Manipulation. J. Light. Technol. 2022. [Google Scholar] [CrossRef]
- Cheng, L.; Hong, W.; Hao, Z.-C. Generation of electromagnetic waves with arbitrary orbital angular momentum modes. Sci. Rep. 2014, 4, 4814. [Google Scholar] [CrossRef]
- Shahmirzadi, A.V.; Badamchi, Z.; Badamchi, B.; Subbaraman, H. Generating Concentrically Embedded Spatially Divided OAM Carrying Vortex Beams Using Transmitarrays. IEEE Trans. Antennas Propag. 2021, 69, 8436–8448. [Google Scholar] [CrossRef]
- Shahmirzadi, A.V.; Kishk, A.A. OAM Carrying Vortex Beam Mode Interconversion Using Modular Cascaded Transmitarrays. IEEE Trans. Microw. Theory Tech. 2022, 70, 3591–3605. [Google Scholar] [CrossRef]
- Feng, Q.; Kong, X.; Shan, M.; Lin, Y.; Li, L.; Cui, T.J. Multi-orbital-angular-momentum-mode vortex wave multiplexing and demultiplexing with shared-aperture reflective metasurfaces. Phys. Rev. Appl. 2022, 17, 034017. [Google Scholar] [CrossRef]
- Chung, H.; Kim, D.; Choi, E.; Lee, J. E-Band Metasurface-Based Orbital Angular Momentum Multiplexing and Demultiplexing. Laser Photonics Rev. 2022, 16, 2100456. [Google Scholar] [CrossRef]
- Xue, C.; Sun, J.; Niu, L.; Lou, Q. Ultrathin Dual-Polarized Huygens’ Metasurface: Design and Application. Ann. Phys. 2020, 532, 2000151. [Google Scholar] [CrossRef]
- Yu, S.; Li, L.; Shi, G.; Zhu, C.; Shi, Y. Generating multiple orbital angular momentum vortex beams using a metasurface in radio frequency domain. Appl. Phys. Lett. 2016, 108, 241901. [Google Scholar] [CrossRef]
- Xue, H.; Han, J.; Zhang, S.; Tian, Y.; Hou, J.; Li, L. Co-Modulation of Spin Angular Momentum and High-Order Orbital Angular Momentum Based on Anisotropic Holographic Metasurfaces. IEEE Trans. Antennas Propag. 2023. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Naseri, H.; PourMohammadi, P.; Melouki, N.; Ahmed, F.; Iqbal, A.; Denidni, T.A. Generation of Mixed-OAM-Carrying Waves Using Huygens’ Metasurface for Mm-Wave Applications. Sensors 2023, 23, 2590. https://doi.org/10.3390/s23052590
Naseri H, PourMohammadi P, Melouki N, Ahmed F, Iqbal A, Denidni TA. Generation of Mixed-OAM-Carrying Waves Using Huygens’ Metasurface for Mm-Wave Applications. Sensors. 2023; 23(5):2590. https://doi.org/10.3390/s23052590
Chicago/Turabian StyleNaseri, Hassan, Peyman PourMohammadi, Nouredddine Melouki, Fahad Ahmed, Amjad Iqbal, and Tayeb A. Denidni. 2023. "Generation of Mixed-OAM-Carrying Waves Using Huygens’ Metasurface for Mm-Wave Applications" Sensors 23, no. 5: 2590. https://doi.org/10.3390/s23052590
APA StyleNaseri, H., PourMohammadi, P., Melouki, N., Ahmed, F., Iqbal, A., & Denidni, T. A. (2023). Generation of Mixed-OAM-Carrying Waves Using Huygens’ Metasurface for Mm-Wave Applications. Sensors, 23(5), 2590. https://doi.org/10.3390/s23052590





