Polyimide-On-Silicon 2D Piezoelectric Micromachined Ultrasound Transducer (PMUT) Array
Abstract
1. Introduction
2. Device Design
3. COMSOL Simulations
4. Device Fabrication
5. Characterization Results and Discussion
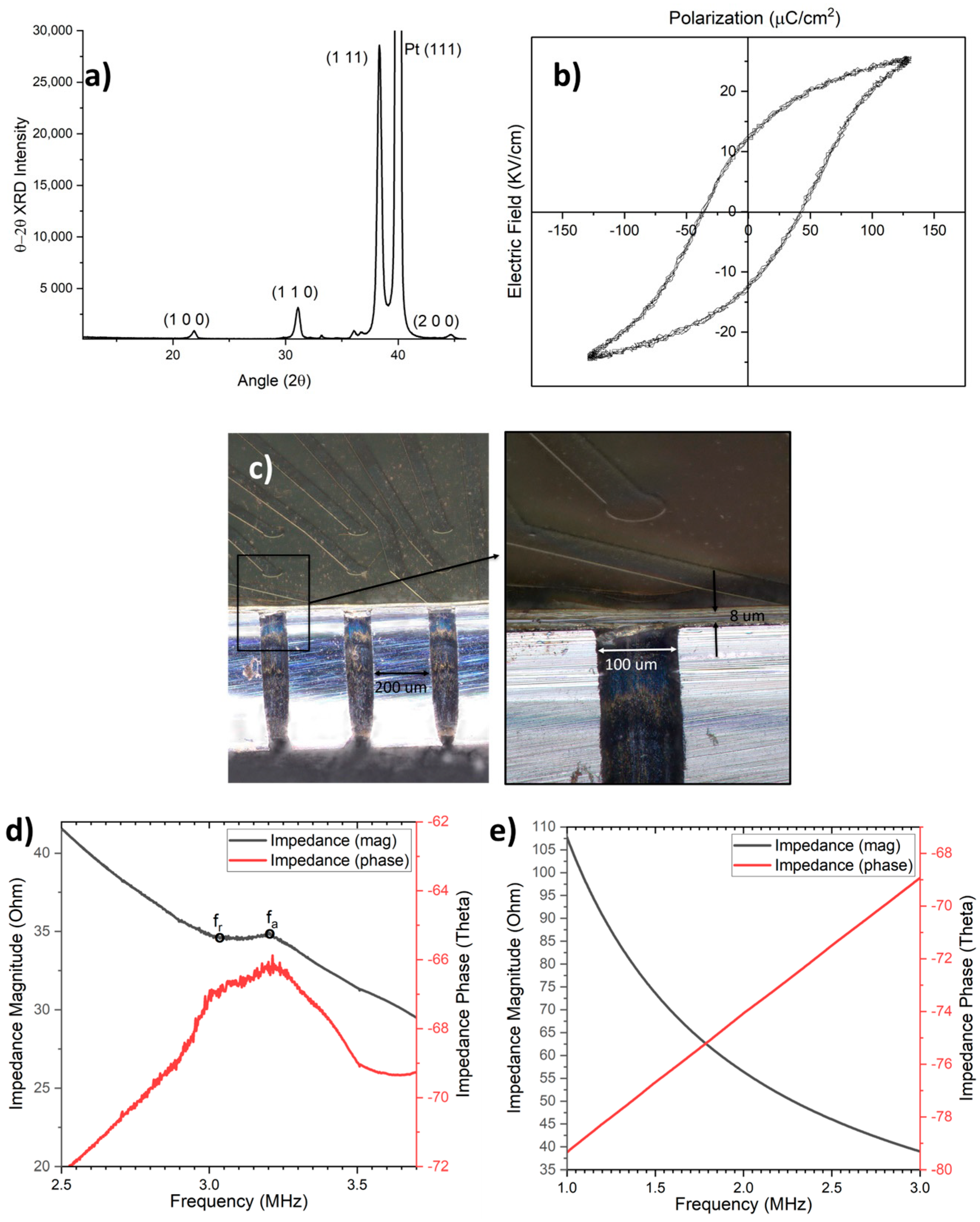
5.1. Material and Electrical Characterization
5.2. Vibration Measurements
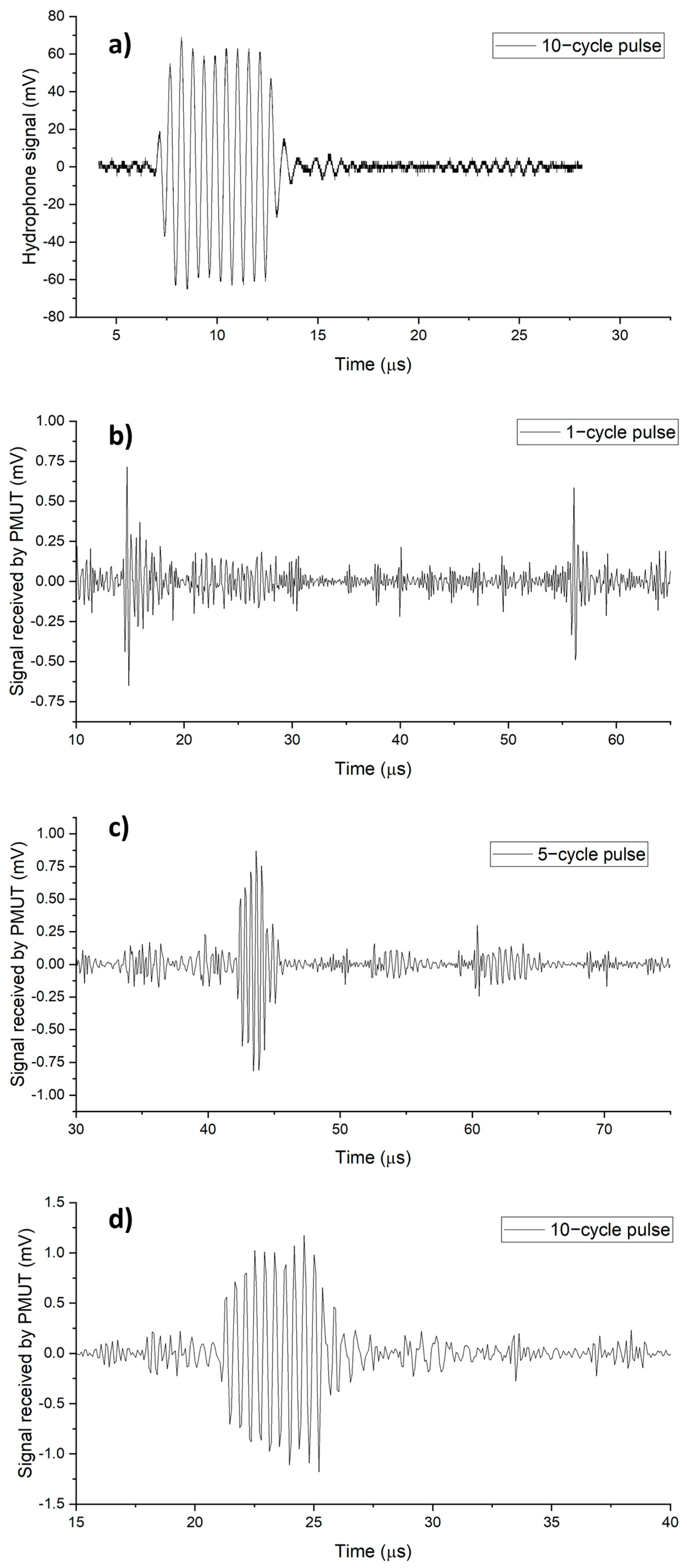
5.3. Underwater Acoustic Pressure Measurements
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- De Luca, R.; Forzoni, L.; Gelli, F.; Bamber, J. An educational overview of ultrasound probe types and their fields of application. Arch. Acoust. 2021, 46, 3–15. [Google Scholar]
- Chen, A.I.H.; Wong, L.L.P.; Yeow, J.T.W. Medical Imaging: Technology and Applications; CRC Press: Boca Raton, FL, USA, 2013; pp. 253–271. [Google Scholar]
- Shung, K.K.; Zippuro, M. Ultrasonic transducers and arrays. IEEE Eng. Med. Biol. Mag. 1996, 15, 20–30. [Google Scholar] [CrossRef]
- Eccardt, P.; Niederer, K. Micromachined ultrasound transducers with improved coupling factors from a CMOS compatible process. Ultrasonics 2000, 38, 774–780. [Google Scholar] [CrossRef]
- Stephens, D.N.; Truong, U.T.; Nikoozadeh, A.; Oralkan, Ö.; Seo, C.H.; Cannata, J.; Dentinger, A.; Thomenius, K.; De La Rama, A.; Nguyen, T.; et al. First in vivo use of a capacitive micromachined ultrasound transducer array–based imaging and ablation catheter. J. Ultrasound Med. 2012, 31, 247–256. [Google Scholar] [CrossRef]
- Omidvar, A.; Cretu, E.; Rohling, R.; Cresswell, M.; Hodgson, A.J. Flexible PolyCMUTs: Fabrication and Characterization of a Flexible Polymer-Based Capacitive Micromachined Ultrasonic Array for Conformal Ultrasonography. Adv. Mater. Technol. 2022, 8, 2201316. [Google Scholar] [CrossRef]
- Eom, C.B.; Trolier-McKinstry, S. Thin-film piezoelectric MEMS. MRS Bull. 2012, 37, 1007–1017. [Google Scholar] [CrossRef]
- Kim, B.H.; Lee, H.S.; Kim, S.W.; Kang, P.; Park, Y.S. Hydrodynamic responses of a piezoelectric driven MEMS inkjet print-head. Sens. Actuators A Phys. 2014, 210, 131–140. [Google Scholar] [CrossRef]
- Zhu, Y.; Liu, W.; Jia, K.; Liao, W.; Xie, H. A piezoelectric unimorph actuator based tip-tilt-piston micromirror with high fill factor and small tilt and lateral shift. Sens. Actuators A Phys. 2011, 167, 495–501. [Google Scholar] [CrossRef]
- Smith, G.L.; Rudy, R.Q.; Polcawich, R.G.; DeVoe, D.L. Integrated thin-film piezoelectric traveling wave ultrasonic motors. Sens. Actuators A Phys. 2012, 188, 305–311. [Google Scholar] [CrossRef]
- Rinaldi, M.; Zuniga, C.; Zuo, C.; Piazza, G. Super-high-frequency two-port AlN contour-mode resonators for RF applications. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2010, 57, 38–45. [Google Scholar] [CrossRef]
- Wang, H.; Yu, Y.; Chen, Z.; Yang, H.; Jiang, H.; Xie, H. Design and fabrication of a piezoelectric micromachined ultrasonic transducer array based on ceramic PZT. In Proceedings of the 2018 IEEE SENSORS, New Delhi, India, 28–31 October 2018. [Google Scholar]
- Sadeghpour, S.; Kraft, M.; Puers, R. Design and fabrication strategy for an efficient lead zirconate titanate based piezoelectric micromachined ultrasound transducer. J. Micromechan. Microeng. 2019, 29, 125002. [Google Scholar] [CrossRef]
- Sadeghpour, S.; Joshi, S.V.; Wang, C.; Kraft, M. Novel phased array piezoelectric micromachined ultrasound transducers (PMUTs) for medical imaging. IEEE Open J. Ultrason. Ferroelectr. Freq. Control 2022, 2, 194–202. [Google Scholar] [CrossRef]
- Joshi, S.V.; Sadeghpour, S.; Kraft, M. Fabrication of high frequency 2D flexible PMUT array. In Proceedings of the 2023 IEEE 36th International Conference on Micro Electro Mechanical Systems (MEMS), Munich, Germany, 15–19 January 2023. [Google Scholar]
- Jung, J.; Lee, W.; Kang, W.; Shin, E.; Ryu, J.; Choi, H. Review of piezoelectric micromachined ultrasonic transducers and their applications. J. Micromech. Microeng. 2017, 27, 113001. [Google Scholar] [CrossRef]
- Jeong, Y.; Genoe, J.; Gijsenbergh, P.; Segers, J.; Heremans, P.L.; Cheyns, D. Fully flexible PMUT based on polymer materials and stress compensation by adaptive frequency driving. J. Microelectromech. Syst. 2021, 30, 137–143. [Google Scholar] [CrossRef]
- Smith, G.L.; Pulskamp, J.S.; Sanchez, L.M.; Potrepka, D.M.; Proie, R.M.; Ivanov, T.G.; Rudy, R.Q.; Nothwang, W.D.; Bedair, S.S.; Meyer, C.D.; et al. Pzt-based piezoelectric mems technology. J. Am. Ceram. Soc. 2012, 95, 1777–1792. [Google Scholar] [CrossRef]
- Qiu, Y.; Gigliotti, J.V.; Wallace, M.; Griggio, F.; Demore, C.E.; Cochran, S.; Trolier-McKinstry, S. Piezoelectric micromachined ultrasound transducer (PMUT) arrays for integrated sensing, actuation and imaging. Sensors 2015, 15, 8020–8041. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z.; Zhu, W.; Zhu, H.; Miao, J.; Chao, C.; Zhao, C.; Tan, O.K. Fabrication and Characterization of Piezoelectric Micromachined Ultrasonic Transducers with Thick Composite PZT Films. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2005, 52, 2289–2297. [Google Scholar] [CrossRef] [PubMed]
- Griggio, F.; Demore, C.E.; Kim, H.; Gigliotti, J.; Qiu, Y.; Jackson, T.N.; Choi, K.; Tutwiler, R.L.; Cochran, S.; Trolier-McKinstry, S. Micromachined Diaphragm Transducers for Miniaturised Ultrasound Arrays. In Proceedings of the IEEE International Ultrasonics Symposium, Dresden, Germany, 7–10 October 2012. [Google Scholar]
- Perçin, G.; Atalar, A.; Levent Degertekin, F.; Khuri-Yakub, B.T. Micromachined Two-Dimensional Array Piezoelectrically Actuated Transducers. Appl. Phys. Lett. 1998, 72, 1397–1399. [Google Scholar] [CrossRef]
- Sekiguchi, T.; Yoshida, S.; Kanamori, Y.; Tanaka, S. Epitaxial Pb(Zr,Ti)O3-Based Piezoelectric Micromachined Ultrasonic Transducer Fabricated on Silicon-On-Nothing (SON) Structure. In Proceedings of the 2023 IEEE 36th International Conference on Micro Electro Mechanical Systems (MEMS), Munich, Germany, 15–19 January 2023; pp. 139–142. [Google Scholar] [CrossRef]
- Jiang, X.; Lu, Y.; Tang, H.Y.; Tsai, J.M.; Ng, E.J.; Daneman, M.J.; Boser, B.E.; Horsley, D.A. Monolithic ultrasound fingerprint sensor. Microsyst. Nanoeng. 2017, 3, 17059. [Google Scholar] [CrossRef]
- Piezoelectric Tonpilz Transducer. Available online: https://www.comsol.com/model/piezoelectric-tonpilz-transducer-11478 (accessed on 20 January 2023).
- Wang, H.; Godara, M.; Chen, Z.; Xie, H. A one-step residue-free wet etching process of ceramic PZT for piezoelectric transducers. Sens. Actuators A 2019, 290, 130–136. [Google Scholar] [CrossRef]
- Rodríguez-Aranda, M.C.; Calderón-Piñar, F.; Espinoza-Beltrán, F.J.; Flores-Ruiz, F.J.; León-Sarabia, E.; Mayén-Mondragón, R.; Yáñez-Limón, J.M. Ferroelectric hysteresis and improved fatigue of PZT (53/47) films fabricated by a simplified sol–gel acetic-acid route. J. Mater. Sci. Mater. Electron 2014, 25, 4806–4813. [Google Scholar] [CrossRef]
- Shin, E.; Yeo, H.G.; Yeon, A.; Jin, C.; Park, W.; Lee, S.C.; Choi, H. Development of a high-density piezoelectric micromachined ultrasonic transducer array based on patterned aluminum nitride thin film. Micromachines 2020, 11, 623. [Google Scholar] [CrossRef] [PubMed]
- Cai, J.; Wang, Y.; Jiang, D.; Zhang, S.; Gu, Y.A.; Lou, L.; Gao, F.; Wu, T. Beyond fundamental resonance mode: High-order multi-band ALN PMUT for in vivo photoacoustic imaging. Microsyst. Nanoeng. 2022, 8, 116. [Google Scholar] [CrossRef]
- Hadimioglu, B.; Khuri-Yakub, B.T. Polymer films as acoustic matching layers. In Proceedings of the IEEE Symposium on Ultrasonics, Honolulu, HI, USA, 4–7 December 1990; Volume 3, pp. 1337–1340. [Google Scholar]
- Liu, Z.; Yoshida, S.; Horsley, D.A.; Tanaka, S. Fabrication and Characterization of Row-Column Addressed PMUT Array with Monocrystalline PZT Thin Film Toward Creating Ultrasonic Imager. Sens. Actuators A Phys. 2022, 342, 113666. [Google Scholar] [CrossRef]
- Ledesma, E.; Zamora, I.; Uranga, A.; Torres, F.; Barniol, N. Enhancing AlN PMUTs Acoustic Responsivity within a MEMS-on-CMOS Process. Sensors 2021, 21, 8447. [Google Scholar] [CrossRef]
- Wang, Q.; Luo, G.; Kusano, Y.; Horsley, D.A. Low thermal budget surface micromachining process for piezoelectric micromachined ultrasonic transducer arrays with in-situ vacuum sealed cavities. In Proceedings of the Hilton Head Workshop 2018: A Solid-State Sensors, Actuators and Microsystems Workshop, Hilton Head Island, SC, USA, 3–7 June 2018; pp. 245–248. [Google Scholar]
- Tan, M.; Chen, C.; Chen, Z.; Janjic, J.; Daeichin, V.; Chang, Z.-Y.; Noothout, E.; Van Soest, G.; Verweij, M.D.; De Jong, N.; et al. A front-end ASIC with high-voltage transmit switching and receive digitization for 3-D forward-looking intravascular ultrasound imaging. IEEE J. Solid-State Circuits 2018, 53, 2284–2297. [Google Scholar] [CrossRef]
- Hu, H.; Huang, H.; Li, M.; Gao, X.; Yin, L.; Qi, R.; Wu, R.S.; Chen, X.; Ma, Y.; Shi, K.; et al. A wearable cardiac ultrasound imager. Nature 2023, 613, 667–675. [Google Scholar] [CrossRef]
- Wang, C.; Chen, X.; Wang, L.; Makihata, M.; Liu, H.C.; Zhou, T.; Zhao, X. Bioadhesive ultrasound for long-term continuous imaging of diverse organs. Science 2022, 377, 517–523. [Google Scholar] [CrossRef] [PubMed]
- Jiang, X.; Tang, H.Y.; Lu, Y.; Ng, E.J.; Tsai, J.M.; Boser, B.E.; Horsley, D.A. Ultrasonic fingerprint sensor with transmit beamforming based on a PMUT array bonded to CMOS circuitry. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2017, 64, 1401–1408. [Google Scholar] [CrossRef]
- Abbey, C.K.; Nguyen, N.Q.; Insana, M.F. Effects of frequency and bandwidth on diagnostic information transfer in ultrasonic B-Mode imaging. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2012, 59, 1115–1126. [Google Scholar] [CrossRef]
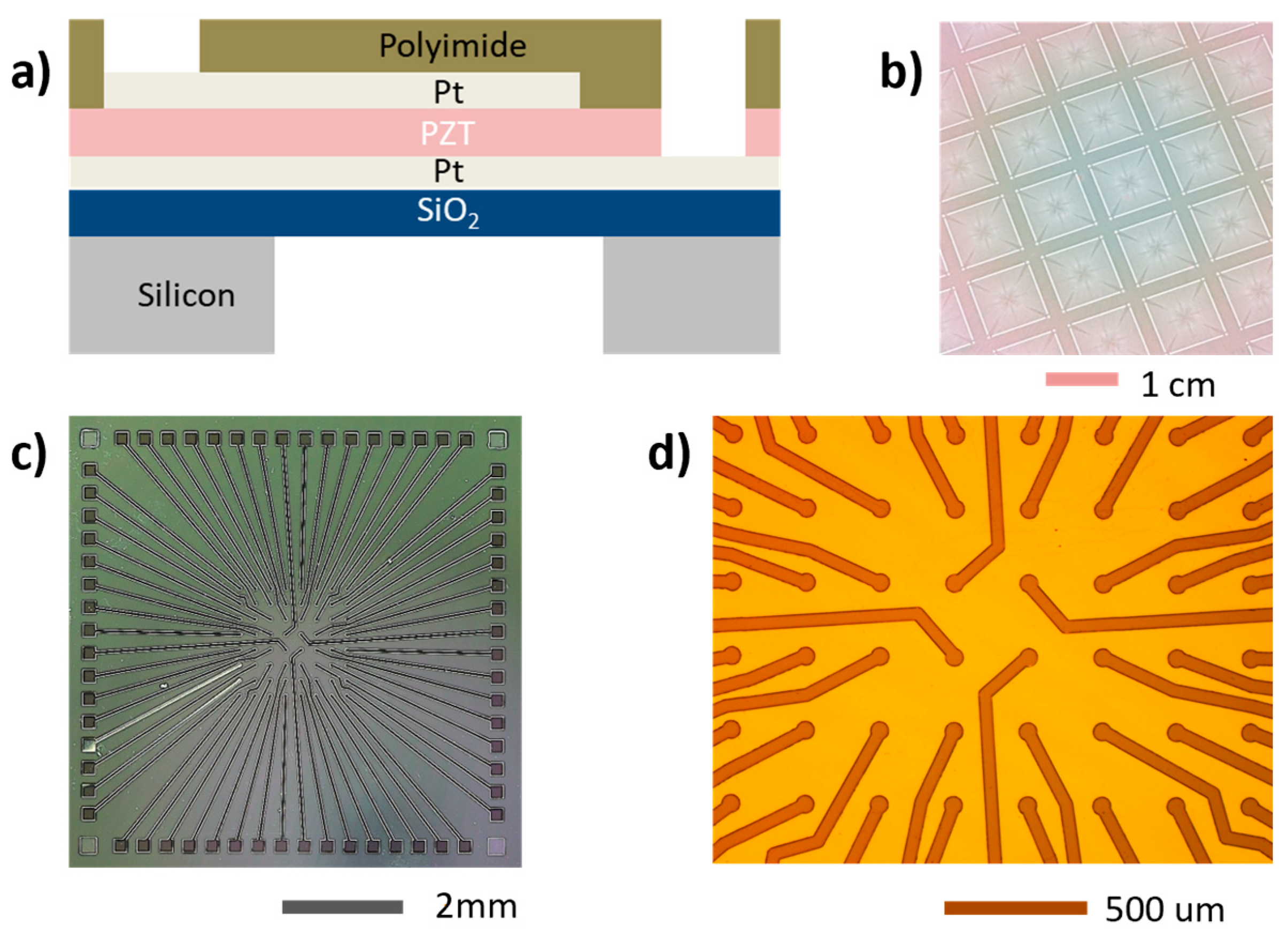

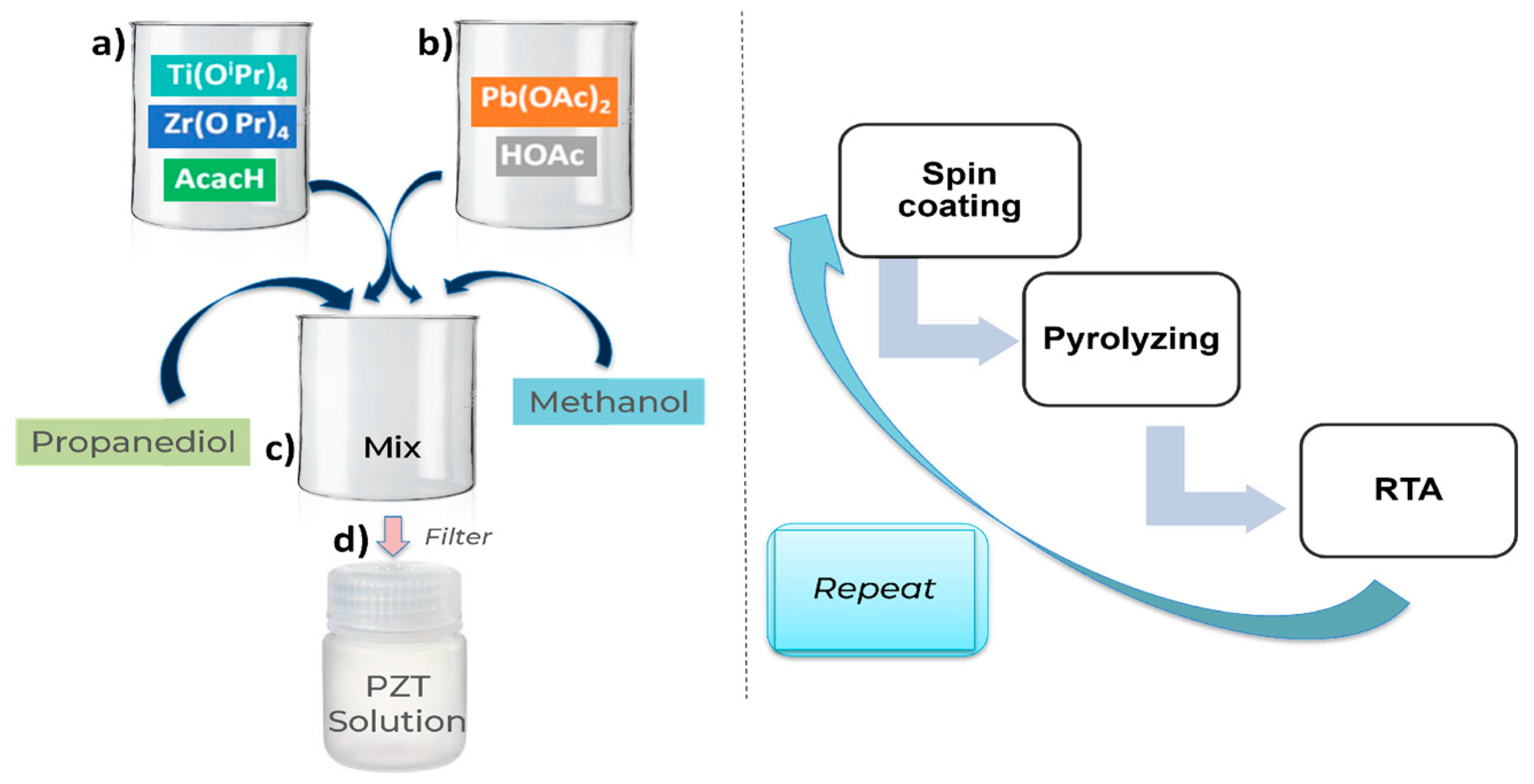
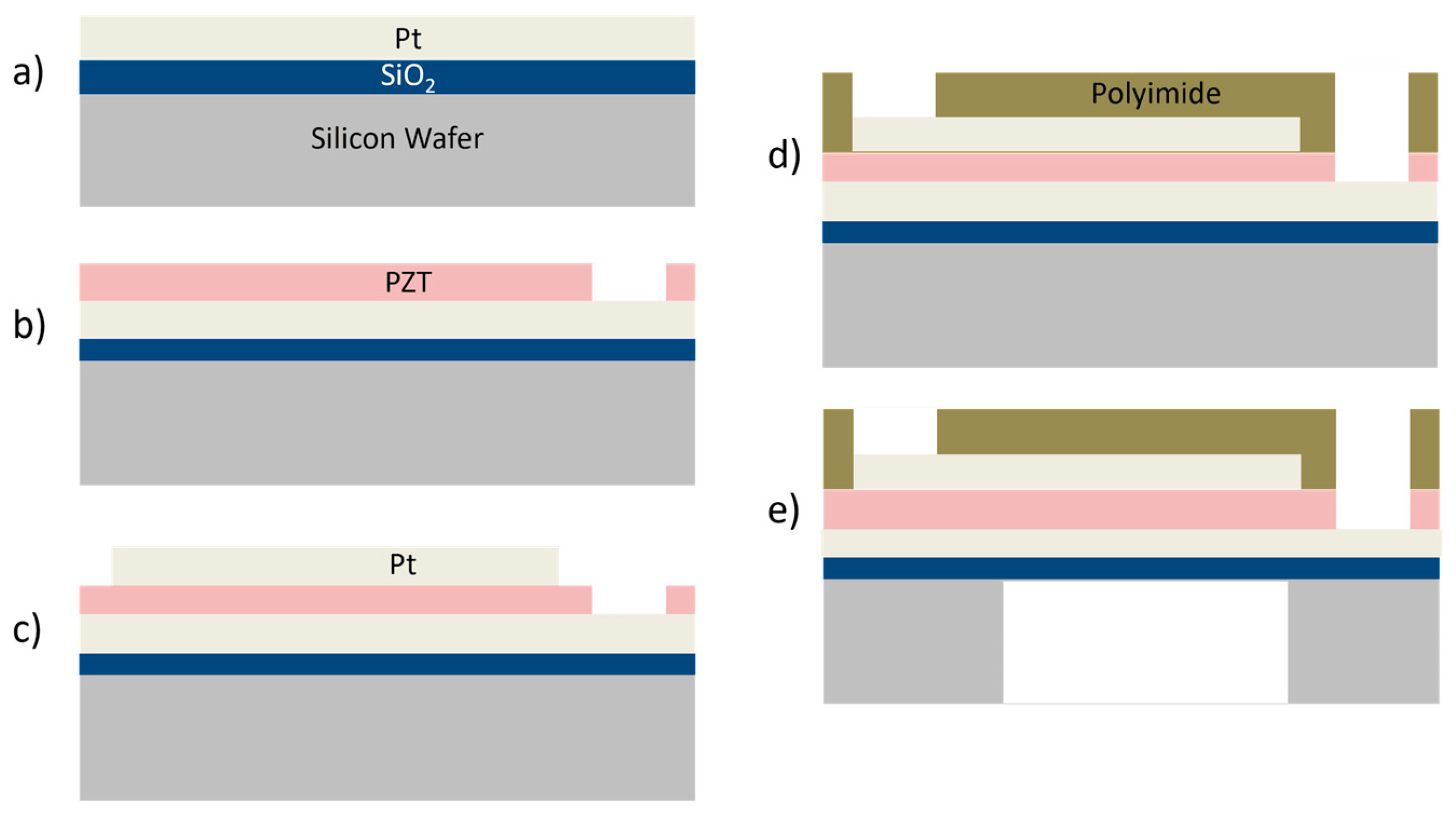


| Parameter | Relevant for | Chosen Range |
|---|---|---|
| Membrane diameter | Resonance frequency, acoustic pressure | 100 µm |
| Array size | Targeted application areas, for instance, affect imaging footprint in biomedical imaging | 8 × 8 (2 mm × 2 mm) |
| Array pitch | Lateral resolution | 300 µm |
| Resonance frequency | Chosen based on application, also relevant for axial resolution in imaging | 2–2.5 MHz |
| Material | Function | Thickness (µm) | Young’s Modulus (GPa) | Density (Kg/m3) | Poisson’s Ratio | Deposition Method |
|---|---|---|---|---|---|---|
| Polyimide | Membrane passive layer | 6 | 8.5 | 1420 | 0.34 | Spin coating |
| PZT | Piezo layer | 1 | 80 | 7500 | 0.3 | Sol–gel |
| Pt | Top, bottom electrodes | 0.2 | 168 | 21,400 | 0.39 | RF sputtering |
| Silicon oxide | Etch stop for DRIE | 0.5 | 70 | 2200 | 0.17 | Thermal wet oxidation |
| Silicon | Membrane sidewall | 525 | 140 | 2330 | 0.265 | -- |
| Reference | [32] | [33] | [34] | This Work |
|---|---|---|---|---|
| Technology | SOI, AlN | SOI, AlN | SOI, PZT | Conventional silicon wafer with polyimide membrane, PZT |
| Surface pressure (kPa/V) | 4.9 | 2.9 | 27 | 9.2 |
| Parameter | Theoretical Calculation | Simulation Result | Experimental Findings |
|---|---|---|---|
| In−air resonance frequency | 4.35 MHz | 3.2 MHz | |
| Underwater resonance frequency | 2.34 MHz | 1.8–2 MHz | 1.7 MHz (70% fractional bandwidth |
| Underwater membrane displacement | 1.45 nm/V | 1.3 nm/V (3 nm/V in−air) | |
| Underwater surface pressure | 9.2 kPa/V (Calculated based on the measured membrane displacement underwater) | 9.5 kPa/V | |
| Transmit pressure sensitivity at 5 mm | 32 Pa/V | 40 Pa/V (15 mV/MPa as a receiver) | |
| In-air effective coupling coefficient and interelement crosstalk | 14%, 1% respectively (Exp) | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Joshi, S.V.; Sadeghpour, S.; Kraft, M. Polyimide-On-Silicon 2D Piezoelectric Micromachined Ultrasound Transducer (PMUT) Array. Sensors 2023, 23, 4826. https://doi.org/10.3390/s23104826
Joshi SV, Sadeghpour S, Kraft M. Polyimide-On-Silicon 2D Piezoelectric Micromachined Ultrasound Transducer (PMUT) Array. Sensors. 2023; 23(10):4826. https://doi.org/10.3390/s23104826
Chicago/Turabian StyleJoshi, Sanjog Vilas, Sina Sadeghpour, and Michael Kraft. 2023. "Polyimide-On-Silicon 2D Piezoelectric Micromachined Ultrasound Transducer (PMUT) Array" Sensors 23, no. 10: 4826. https://doi.org/10.3390/s23104826
APA StyleJoshi, S. V., Sadeghpour, S., & Kraft, M. (2023). Polyimide-On-Silicon 2D Piezoelectric Micromachined Ultrasound Transducer (PMUT) Array. Sensors, 23(10), 4826. https://doi.org/10.3390/s23104826








