An Inductive Sensor for Two-Dimensional Displacement Measurement
Abstract
1. Introduction
2. Structure and Measurement Principles
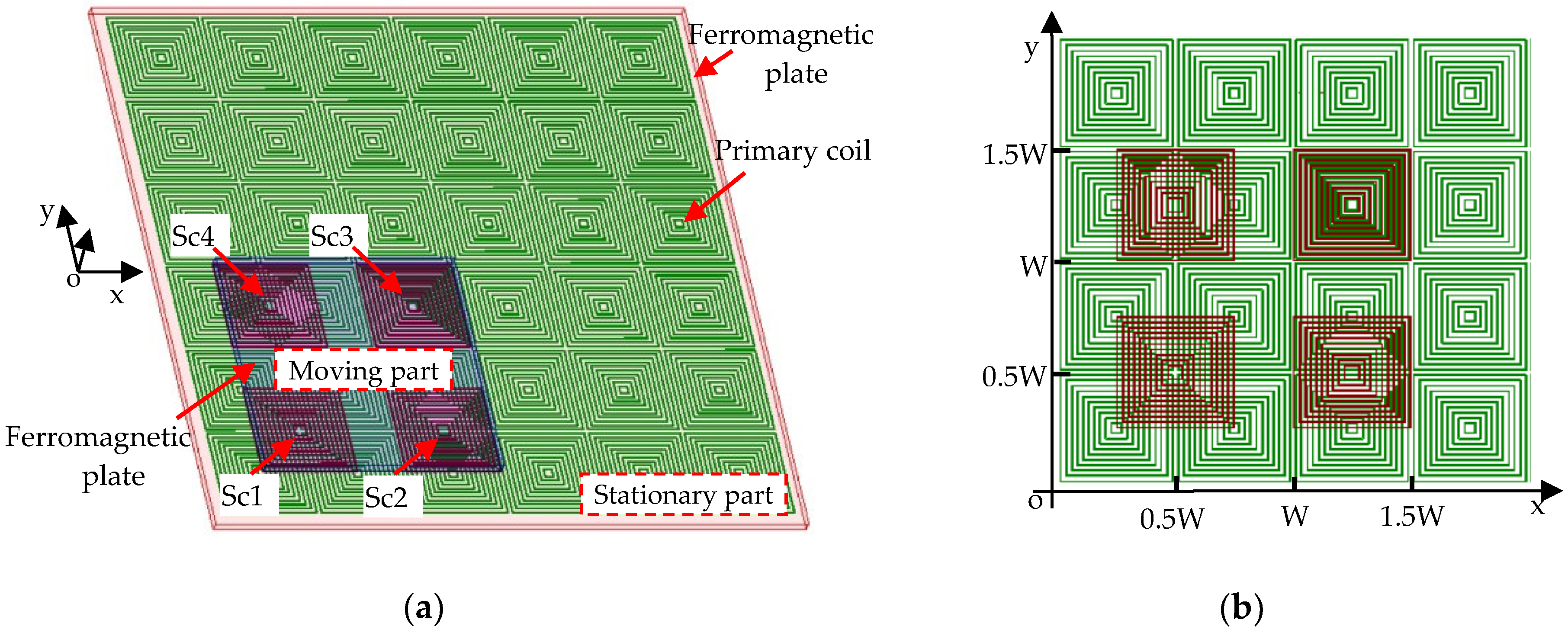
2.1. Structure of the Sensor
2.2. Electro-Magnetic Induction
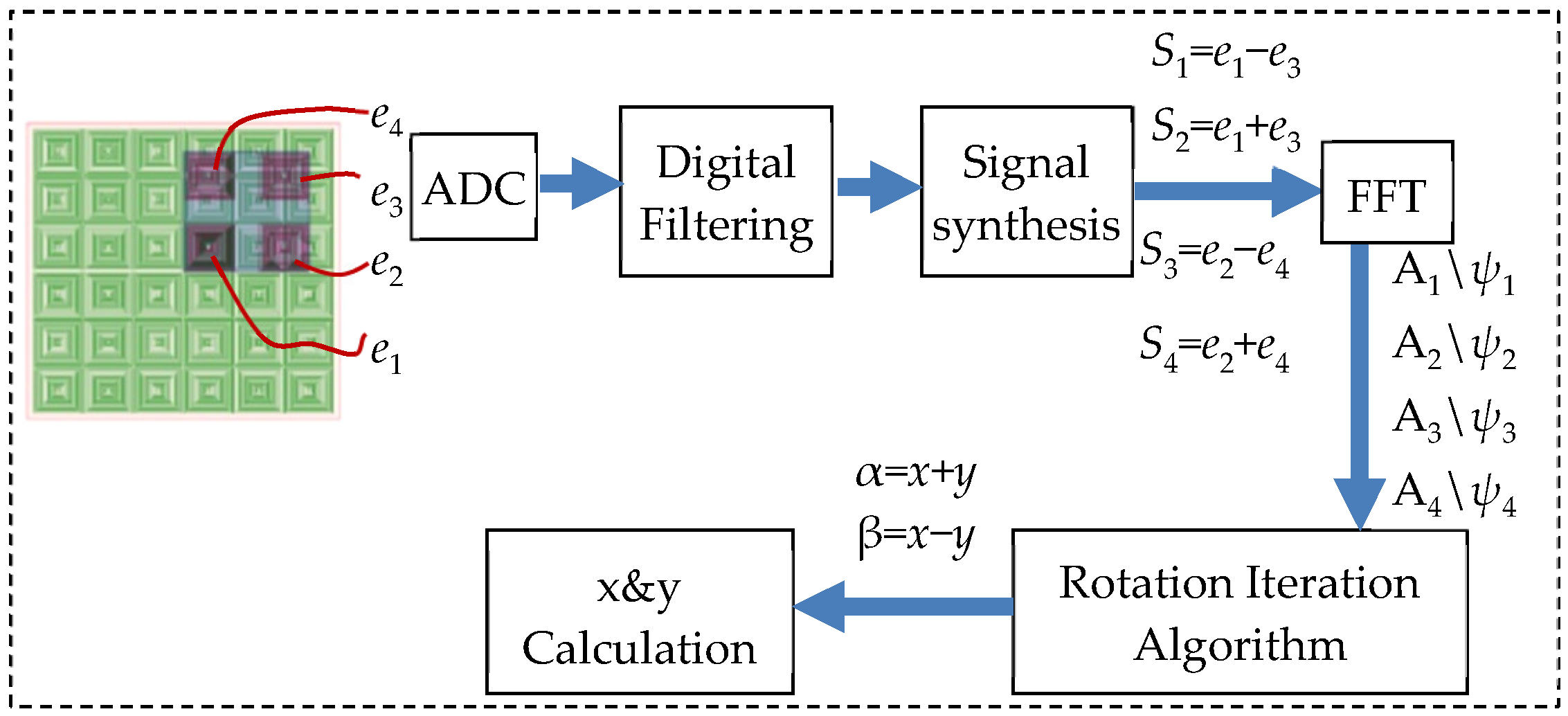
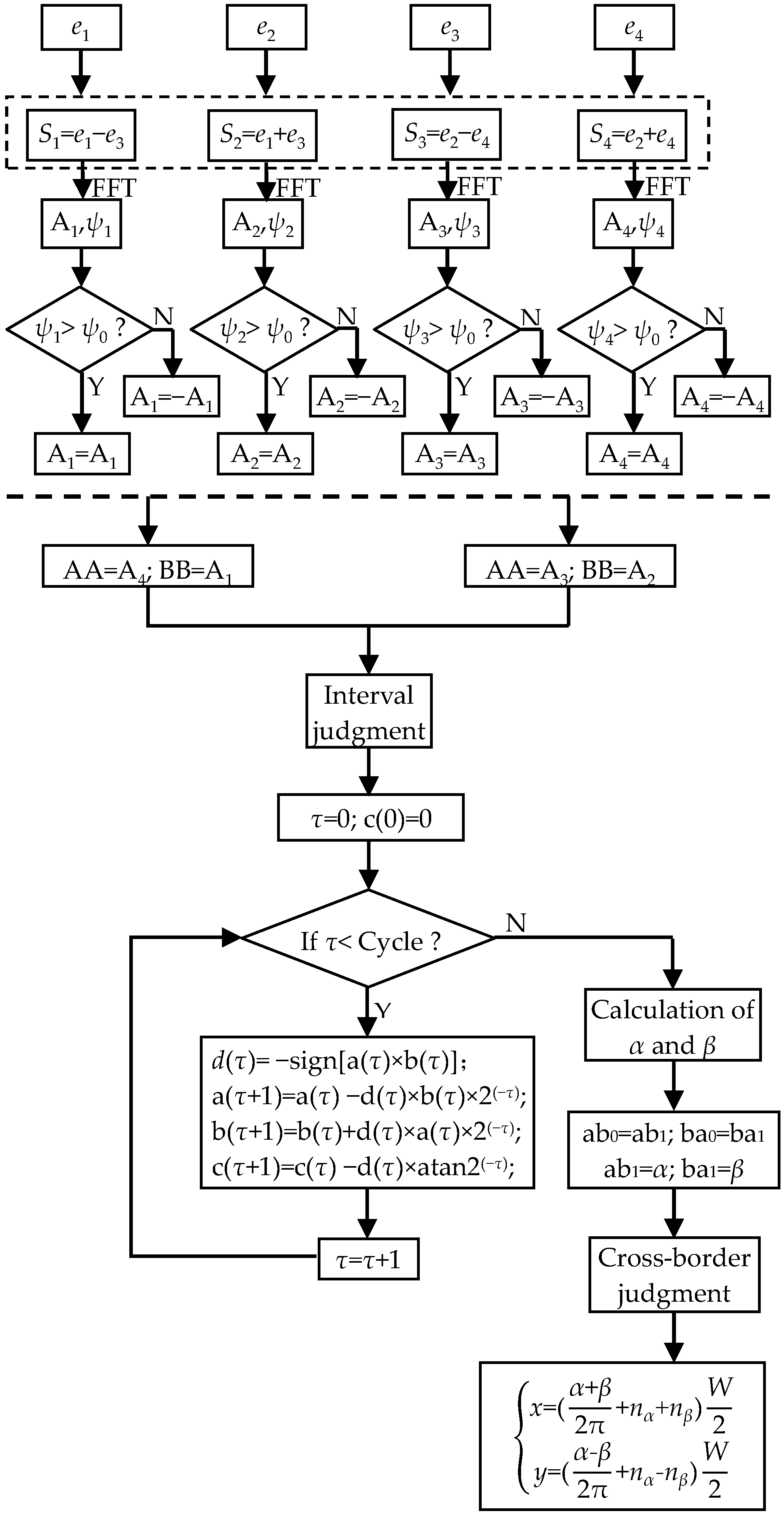
2.3. Resolving of Displacements
3. Simulation of Sensor Model
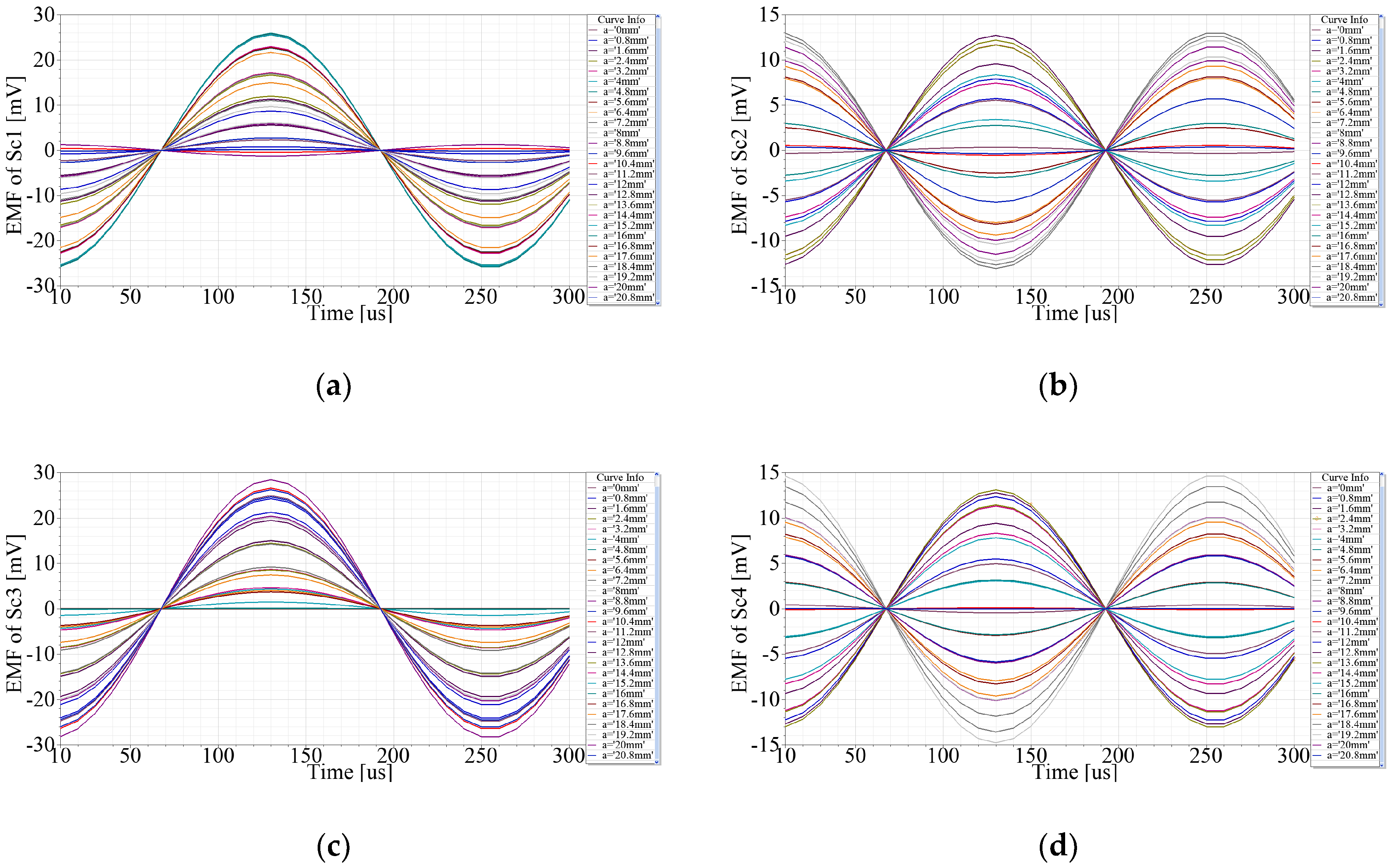
3.1. FEA Simulation
3.2. Numerical Simulation
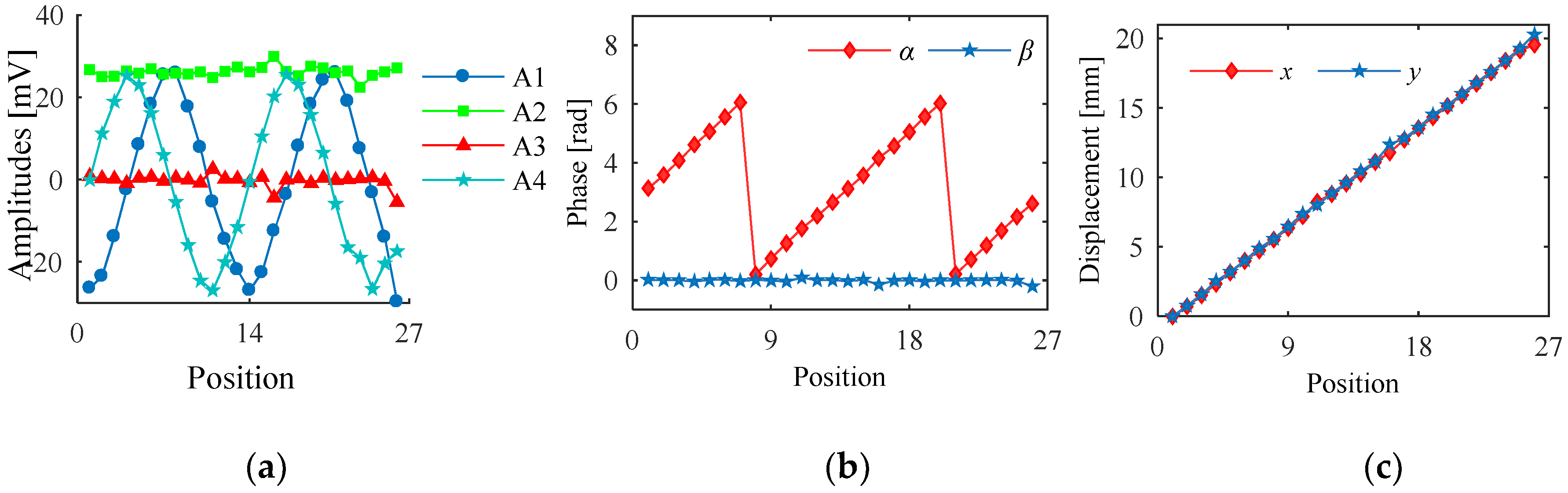
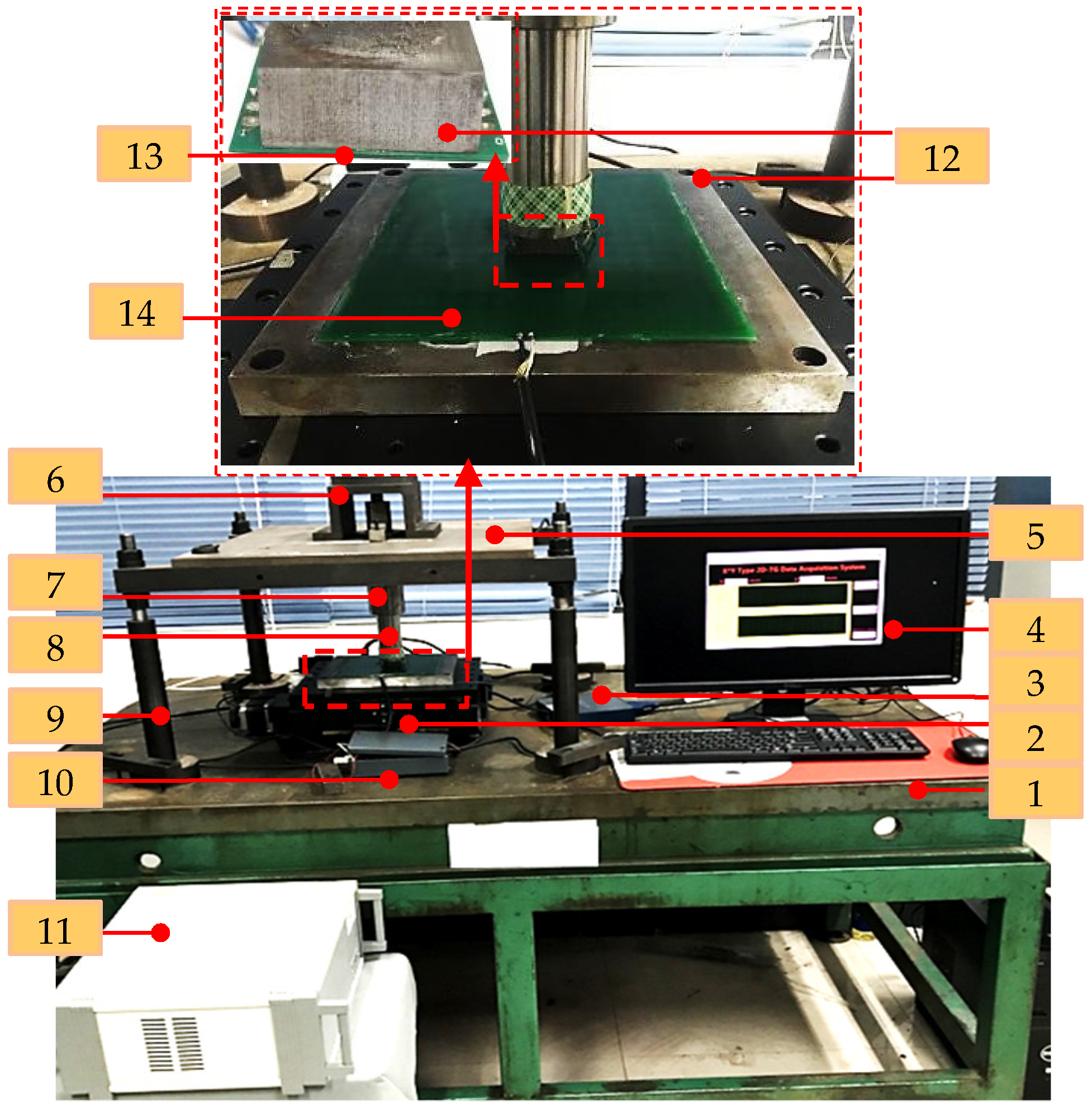
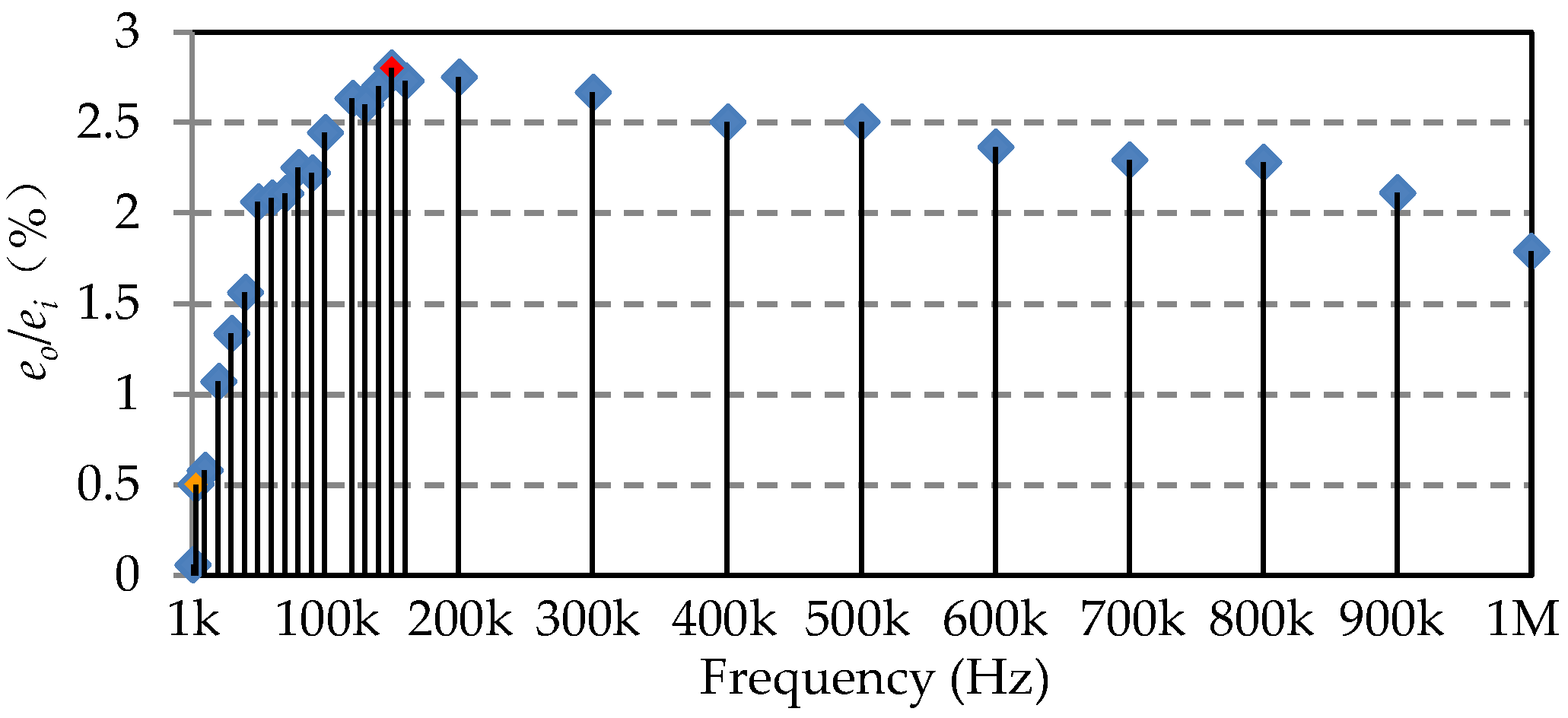
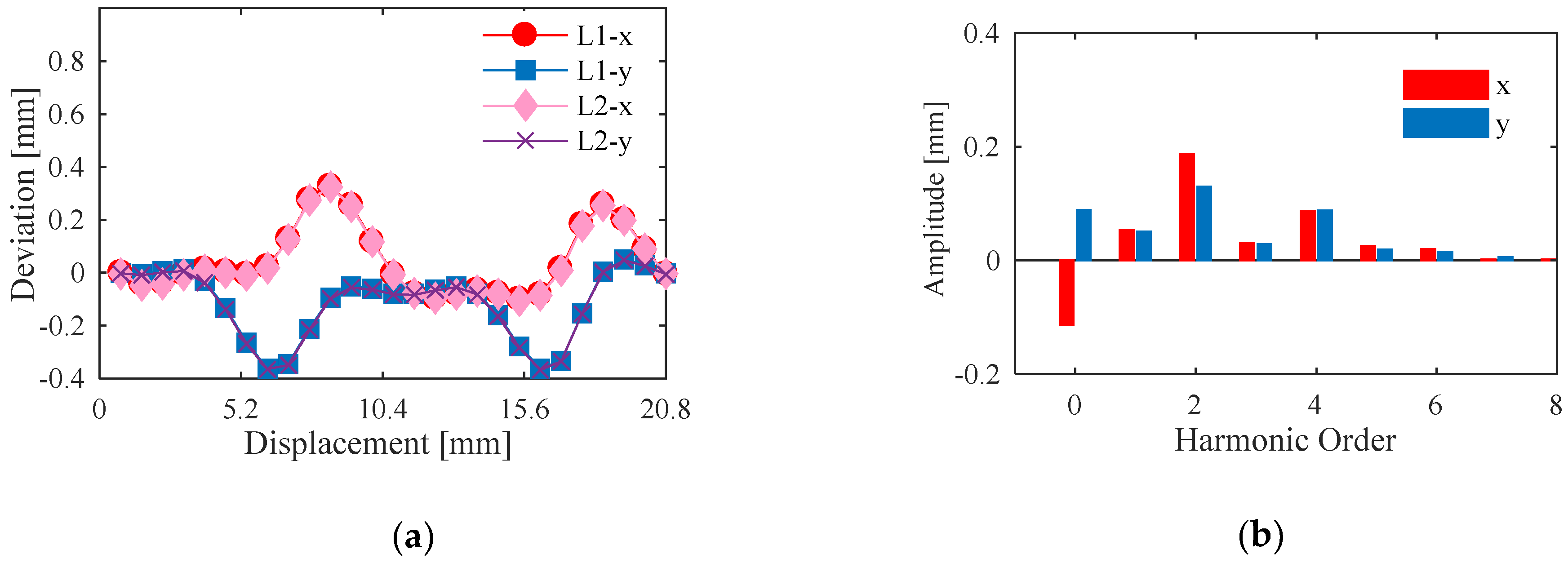
4. Experiment and Results
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Manske, E.; Jäger, G.; Hausotte, T.; Füßl, R. Recent developments and challenges of nanopositioning and nanomeasuring technology. Meas. Sci. Technol. 2012, 23, 074001. [Google Scholar] [CrossRef]
- Gao, W.; Kim, S.-W.; Bosse, H.; Haitjema, H.; Chen, Y.; Lu, X.; Knapp, W.; Weckenmann, A.; Estler, W.; Kunzmann, H. Measurement technologies for precision positioning. CIRP Ann. Manuf. Technol. 2015, 64, 773–796. [Google Scholar] [CrossRef]
- Liu, C.H.; Jywe, W.-Y.; Jeng, Y.-R.; Hsu, T.-H.; Li, Y.-T. Design and control of a long-traveling nano-positioning stage. Precis. Eng. 2010, 34, 497–506. [Google Scholar] [CrossRef]
- Yagüe, J.A.; Aguilar, J.; Valenzuela, M.; Lope, M.A. Traceability in the calibration of low cost opto-electronic sensors for measuring 2D displacement. AIP Conf. Proc. 2009, 1181, 244–253. [Google Scholar]
- Laser Interferometer. Available online: https://sios-de.com/products/length-measurement/laser-inter-ferometer/ (accessed on 26 December 2019).
- Tiemann, I.; Spaeth, C.; Wallner, G.; Metz, G.; Israel, W.; Yamaryo, Y.; Shimomura, T.; Kubo, T.; Wakasa, T.; Morosawa, T.; et al. An international length comparison using vacuum comparators and a photoelectric incremental encoder as transfer standard. Precis. Eng. 2008, 32, 1–6. [Google Scholar] [CrossRef]
- Fleming, A.J. A rewiew of nanometer resolution position sensors: Operation and performance. Sens. Actuators A Phys. 2013, 190, 106–126. [Google Scholar] [CrossRef]
- Fan, K.; Lee, B.-C.; Chung, Y.-C. A planar laser diffraction encoder in littrow configuration for 2D nanometric positioning. Int. J. Autom. Smart Technol. 2011, 1, 93–99. [Google Scholar] [CrossRef]
- Ishikawa, I.; Sawada, R.; Higurashi, E.; Sanada, S.; Chino, D. Integrated micro-displacement sensor that measures tilting angle and linear movement of an external mirror. Sens. Actuators A Phys. 2007, 138, 269–275. [Google Scholar] [CrossRef]
- Zhu, K.; Guo, B.; Lu, Y.; Zhang, S.; Tan, Y. Single-spot two-dimensional displacement measurement based on self-mixing interferometry. Optica 2017, 4, 729–735. [Google Scholar] [CrossRef]
- Khiat, A.; Lamarque, F.; Prelle, C.; Pouille, P.; Leester-Schädel, M.; Büttgenbach, S. Two-dimension fiber optic sensor for high-resolution and long-range linear measurements. Sens. Actuators A Phys. 2010, 158, 43–50. [Google Scholar] [CrossRef]
- Wei, P.; Lu, X.; Qiao, D.; Zou, L.; Huang, X.; Tan, J.; Lu, Z. Two-dimensional displacement measurement based on two parallel gratings. Rev. Sci. Instrum. 2018, 89, 065105. [Google Scholar] [CrossRef] [PubMed]
- Lin, J.; Guan, J.; Wen, F.; Tan, J. High-resolution and wide range displacement measurement based on planar grating. Opt. Commun. 2017, 404, 132–138. [Google Scholar] [CrossRef]
- Hu, P.; Chang, D.; Tan, J.; Yang, R.-T.; Yang, H.-X.; Fu, H.-J. Displacement measuring grating interferometer: A review. Front. Inf. Technol. Electron. Eng. 2019, 20, 631–654. [Google Scholar] [CrossRef]
- Kimura, A.; Gao, W.; Arai, Y.; Zeng, L. Design and construction of a two-degree-of-freedom linear encoder for nanometric measurement of stage position and straightness. Precis. Eng. 2010, 34, 145–155. [Google Scholar] [CrossRef]
- Yu, J.-P.; Wang, W.; Lu, K.-Q.; Mei, D.-Q.; Chen, Z.-C. A planar capacitive sensor for 2D long-range displacement measurement. J. Zhejiang Univ. Sci. C 2013, 14, 252–257. [Google Scholar] [CrossRef]
- Yu, J.-P.; Wang, W.; Li, X.; Zhou, Z.-Z. A novel quadrature signal estimation method for a planar capacitive incremental displacement sensor. Meas. Sci. Rev. 2016, 16, 127–133. [Google Scholar] [CrossRef]
- Yu, H.; Zhang, Y.; Shen, M.; Zhang, H.; Gao, Z.; Wang, D. Planar position sensor based on mono sensing electrode and hybrid-frequency excitation. Sensors 2016, 16, 691. [Google Scholar] [CrossRef]
- Zhao, B.; Wang, L.; Tan, J. Design and realization of a three degrees of freedom displacement measurement system composed of hall sensors based on magnetic field fitting by an elliptic function. Sensors 2015, 15, 22530–22546. [Google Scholar] [CrossRef]
- Liang, R.H.; Cheng, K.Y.; Su, C.H.; Weng, C.T.; Chen, B.Y.; Yang, D.N. GaussSense: Attachable stylus sensing using magnetic sensor grid. In Proceedings of the 25th Annual ACM Symposium on User Interface Software and Technology, Cambridge, MA, USA, 7–10 October 2012; pp. 319–326. [Google Scholar]
- Hojjat, Y.; Karafi, M.R.; Ghanbari, M.; Lotfi, M. Development of an inductive encoder for simultaneous measurement of two-dimensional displacement. Int. J. Adv. Manuf. Technol. 2010, 53, 681–688. [Google Scholar] [CrossRef]
- Djuric, S. Performance analysis of a planar displacement sensor with inductive spiral coils. IEEE Trans. Magn. 2014, 50, 1–4. [Google Scholar] [CrossRef]
- Wu, L.; Tang, Q.; Chen, X.; Xu, S.; Gou, L.; Wang, Y. A novel two-dimensional sensor with inductive spiral coils. IEEE Sens. J. 2019, 19, 4857–4865. [Google Scholar] [CrossRef]
- Damnjanovic, M.; Zivanov, L.; Nagy, L.; Djuric, S.; Biberdzic, B. A novel approach to extending the linearity range of displacement inductive sensor. IEEE Trans. Magn. 2008, 44, 4123–4126. [Google Scholar] [CrossRef]
- Djuric, S.M.; Djuric, N.M.; Damnjanovic, M.S. The optimal useful measurement range of an inductive displacement sensor. Inf. Midem 2015, 45, 132–141. [Google Scholar]
- Anandan, N.; George, B. Design and development of a planar linear variable differential transformer for displacement sensing. IEEE Sens. J. 2017, 17, 5298–5305. [Google Scholar] [CrossRef]
- Babu, A.; George, B. Design and development of a new non-contact inductive displacement sensor. IEEE Sens. J. 2017, 18, 976–984. [Google Scholar] [CrossRef]
- Jiao, D.; Ni, L.; Zhu, X.; Zhe, J.; Zhao, Z.; Lyu, Y.; Liu, Z. Measuring gaps using planar inductive sensors based on calculating mutual inductance. Sens. Actuators A Phys. 2019, 295, 59–69. [Google Scholar] [CrossRef]
- Cheng, Y.; Shu, Y. A new analytical calculation of the mutual inductance of the coaxial spiral rectangular coils. IEEE Trans. Magn. 2013, 50, 1–6. [Google Scholar] [CrossRef]
- Aggarwal, S.; Meher, P.K.; Khare, K. Concept, design, and implementation of reconfigurable CORDIC. IEEE Trans. Very Large Scale Integr. (VLSI) Syst. 2015, 24, 1588–1592. [Google Scholar] [CrossRef]
- Lu, M.; Huang, R.; Yin, W.; Zhao, Q.; Peyton, A. Measurement of permeability for ferrous metallic plates using a novel lift-off compensation technique on phase signature. IEEE Sens. J. 2019, 19, 7440–7446. [Google Scholar] [CrossRef]
- Lu, M.; Yin, L.; Peyton, A.J.; Yin, W. A novel compensation algorithm for thickness measurement immune to lift-off variations using eddy current method. IEEE Trans. Instrum. Meas. 2016, 65, 2773–2779. [Google Scholar]




| Parameter | Settings |
|---|---|
| Turns of each spiral coils of primary coil | 5 turns |
| Turns of Sc1,Sc2,Sc3,Sc4 | 10 turns |
| Resistance of Sc1,Sc2,Sc3,Sc4 | 1 GΩ |
| Amplitude and frequency of the current in primary coil | 0.1 A/4kHz |
| Start and stop time of simulation | 10 μs, 300 μs |
| Material of the ferromagnetic plate | Steel_1045 |
| Size of the ferromagnetic plate | 75 mm × 75 mm × 1 mm |
| Material of the coils | copper |
| Size of the primary coil | 64.6 mm × 64.6 mm |
| Size of the secondary coil | 26 mm × 26 mm |
| Number of spiral coils of primary coil | 6 × 6 |
| Conductor width\space\thickness | 0.1 mm |
| Center distance of two successive spiral coil of primary coil | 10.4 mm |
| Length\width of the outmost turn of spiral coil of primary coil | 5 mm |
| Length\width of the outmost turn of spiral coil in Sc1~Sc4 | 5.2 mm |
| Center distance between Sc1 and Sc2 | 15.6 mm |
| Center distance between Sc1 and Sc4 | 15.6 mm |
| Air-gap thickness between the spiral coils and the ferromagnetic plate | 0.1 mm |
| Air-gap thickness between the primary and secondary coil | 0.1 mm |
| Moving path of the secondary coil | y = x |
| Displacements and linear step along x- and y-axis | 20.8 mm, 0.8 mm |
| No. | Condition | Condition | α and β |
|---|---|---|---|
| Ⅰ | AA>BB&&BB>=0 &&|AA|>|BB | a(0)=|BB|; b(0)=|AA| | α, β=c(50) |
| Ⅱ | BB>=AA&&AA>=0 &&|BB|>|AA| | a(0)=|AA|;b(0)=|BB| | α, β=π/2−c(50) |
| Ⅲ | BB>=0&&AA<0 &&|BB|>=|AA| | a(0)=|AA|;b(0)=|BB| | α, β=π/2+c(50) |
| Ⅳ | BB>=0&&AA<0 &&|AA|>|BB| | a(0)=|BB|;b(0)=|AA| | α, β=π−c(50) |
| Ⅴ | BB<0&&AA<BB &&|AA|>=|BB| | a(0)=|BB|;b(0)=|AA| | α, β=π+c(50) |
| Ⅵ | AA<0&&AA>=BB &&|BB|>=|AA| | a(0)=|AA|;b(0)=|BB| | α, β=3π/2−c(50) |
| Ⅶ | AA>=0&&BB<0 &&|BB|>=|AA| | a(0)=|AA|; b(0)=|BB| | α, β=3π/2+c(50) |
| Ⅷ | AA>=0&&BB<0 &&|AA|>|BB| | a(0)=|BB|; b(0)=|AA| | α, β=2π−c(50) |
| No. | Condition | nα and nβ |
|---|---|---|
| Ⅰ | (ab1−ab0)<−(2π−π/20) && (ab1−ab0)> −2π && (ba1−ba0)< − (2π−π/20) && (ba1−ba0)> −2π | nα= nα+1; nβ= nβ+1 |
| Ⅱ | (ab1−ab0)<2π && (ab1−ab0)>(2π−π/20) && (ba1−ba0)<2π && (ba1−ba0)>(2π−π/20) | nα= nα−1;nβ= nβ−1 |
| Ⅲ | (ab1−ab0)<2π && (ab1−ab0)>(2π−π/20) && (ba1−ba0)< − (2π−π/20) && (ba1−ba0)>2π | nα= nα−1;nβ= nβ+1 |
| Ⅳ | (ab1−ab0)< − (2π−π/20) && (ab1−ab0)> −2π && (ba1−ba0)<2π && (ba1−ba0)>(2π−π/20) | nα= nα+1; nβ= nβ−1 |
| Ⅴ | (ab1−ab0)<2π && (ab1−ab0)>(2π−π/20) && |ba1−ba0|<π/20 | nα= nα−1; nβ= nβ |
| Ⅵ | |ab1−ab0|<π/20 && (ba1−ba0)< π/20 && (ba1−ba0)>(2π−π/20) | nα= nα; nβ= nβ−1 |
| Ⅶ | (ab1−ab0)< − (2π−π/20) && (ab1−ab0)> −2π && |ba1−ba0|<π/20 | nα= nα+1; nβ= nβ |
| Ⅷ | |ab1−ab0|<π/20 && (ba1−ba0)< − (2π−π/20) && (ba1−ba0)> −2π | nα= nα; nβ= nβ+1 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, L.; Xu, S.; Zhong, Z.; Mou, C.; Wang, X. An Inductive Sensor for Two-Dimensional Displacement Measurement. Sensors 2020, 20, 1819. https://doi.org/10.3390/s20071819
Wu L, Xu S, Zhong Z, Mou C, Wang X. An Inductive Sensor for Two-Dimensional Displacement Measurement. Sensors. 2020; 20(7):1819. https://doi.org/10.3390/s20071819
Chicago/Turabian StyleWu, Liang, Shi Xu, Ziqiang Zhong, Chuan Mou, and Xinda Wang. 2020. "An Inductive Sensor for Two-Dimensional Displacement Measurement" Sensors 20, no. 7: 1819. https://doi.org/10.3390/s20071819
APA StyleWu, L., Xu, S., Zhong, Z., Mou, C., & Wang, X. (2020). An Inductive Sensor for Two-Dimensional Displacement Measurement. Sensors, 20(7), 1819. https://doi.org/10.3390/s20071819





