A CMOS-MEMS BEOL 2-axis Lorentz-Force Magnetometer with Device-Level Offset Cancellation
Abstract
:1. Introduction
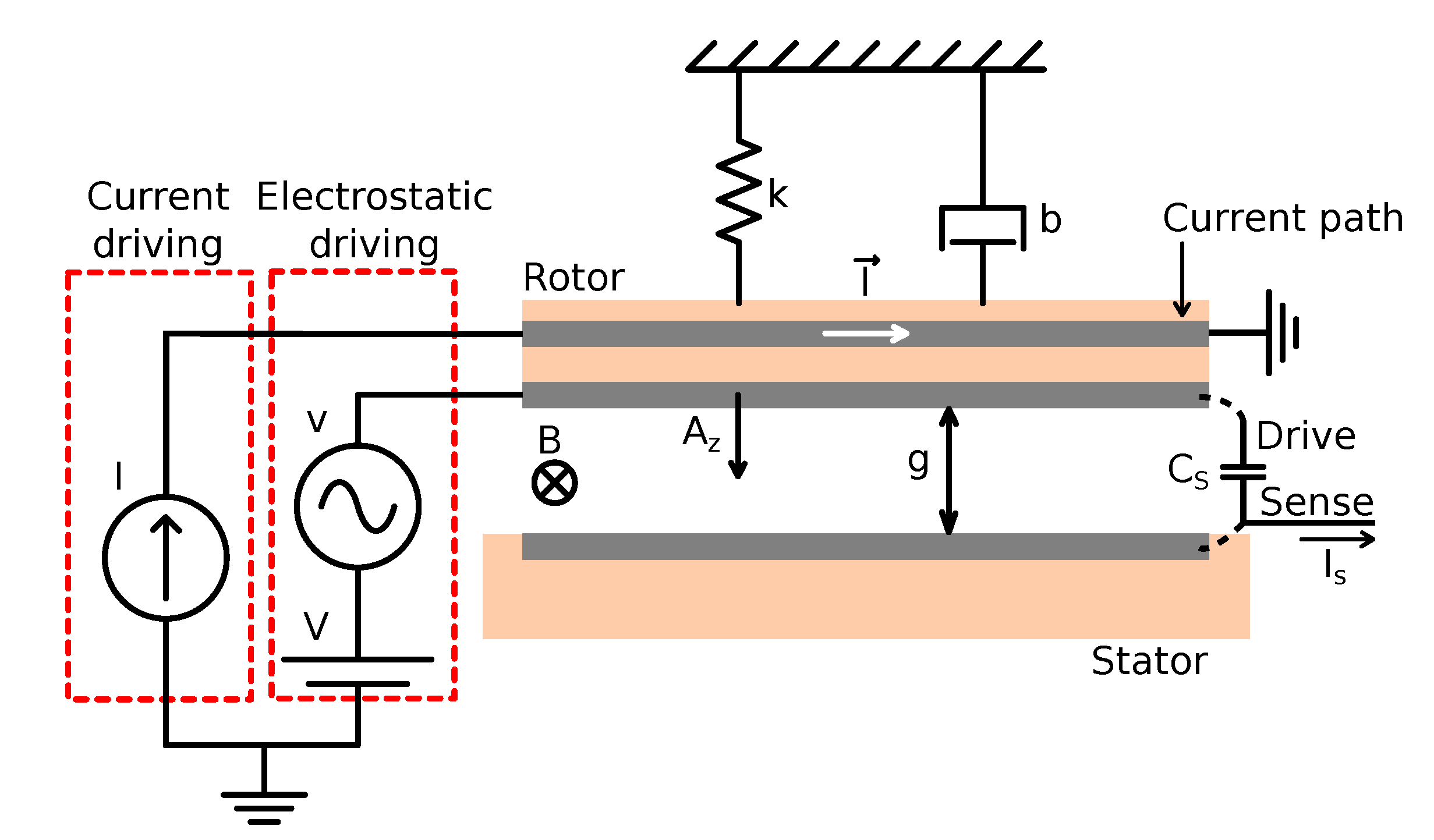
2. Device Working Principle
2.1. Device Characterization
3. Offset Problems in Lorentz-Force Magnetometers: State of the Art
3.1. Offset Arising from Voltage Driving
- Plate actuation is a consequence of the resulting electrostatic force between the MEMS stator and rotor when applying an ac voltage driving to the device. Such electrostatic force generates a plate displacement that causes a gap change and a capacitance variation, as illustrated in Equations (2) and (3) respectively. In most AM Lorentz-force magnetometers, the electrostatic and current drivings are usually applied with the same frequency and phase. While plate displacement due to current driving (Lorentz-force) is desirable, displacement due to the electrostatic driving is an unwanted offset component. Such offset source is problematic as it reduces the dynamic range and has been demonstrated to worsen the long term instability [5,9].
- Signal feedthrough is an offset that arises when interfacing the MEMS device with the readout electronics. If the sensor is placed in a capacitive half Wheatstone bridge readout circuit amplified with a fully-differential amplifier fed back with capacitors, the output signal as a function of the voltage driving iswhere is the amplifier feedback capacitance and is the capacitance difference between the sensor and the bridge capacitances. In differential sensors with ideal matching this feedthrough is completely compensated. The same happens with capacitive bridges ideally matched by using adjustable capacitors. However, MEMS capacitance drifts with temperature as a result of device springs coefficient change [15,16,17]. Moreover, MEMS capacitance may present variations due to fabrication and release non-idealities [18,19].
3.2. Offset Arising from Current Driving
- The fact that the resistance of the current carrying structure is not zero generates a voltage drop across the MEMS current driving path. This voltage drop between the MEMS current source and sink is translated into a distributed electrostatic force along the device that generates a plate displacement. Such issue is even worse in differential devices with current driven in series, as the resulting electrostatic force suffers an important mismatch. Some works [21,22,23] propose the adjustment of the voltage levels at these electrodes in order to compensate the mentioned electrostatic force imbalance. However, such solution requires manual adjustment, which is not feasible in mass production.
- There exists a parasitic capacitive coupling between the current carrying path and the sense node, which results in a current feedthrough directly to the device output. In Ref. [14], a capacitive network between the current carrying path and the amplifier input is used to partly compensate this offset source, similar to the solution proposed in Ref. [24]. In Ref. [5] this source of offset is removed, together with other offset sources by using a complex modulation and demodulation strategy. Unfortunately, the proposed complex circuit still presents some amount of offset due to imperfections of the implemented circuitry.
3.3. Total Offset
4. Proposed Device
5. Experimental Results
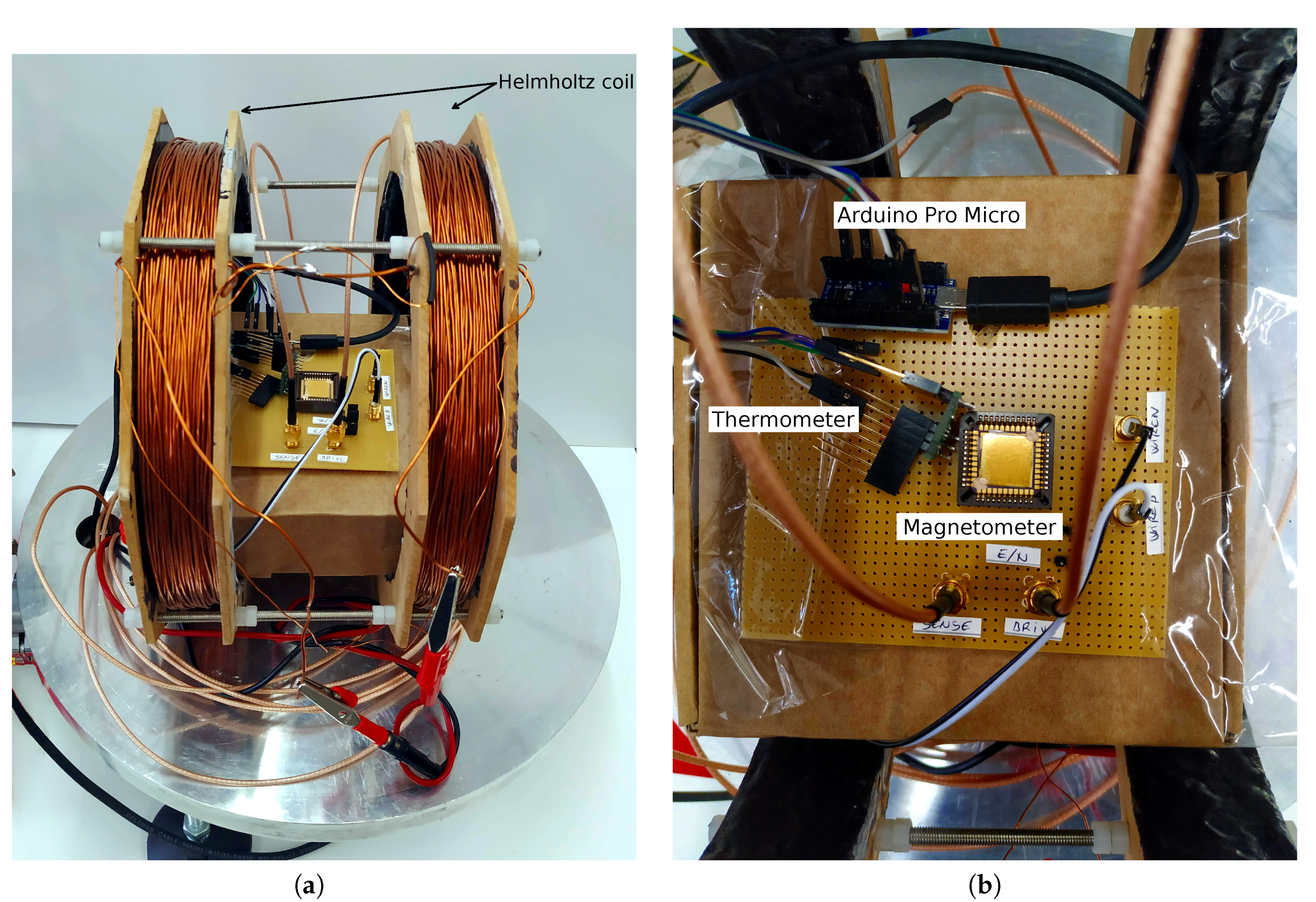
5.1. Measurement Setups
5.1.1. Wafer Level Batch Measurements
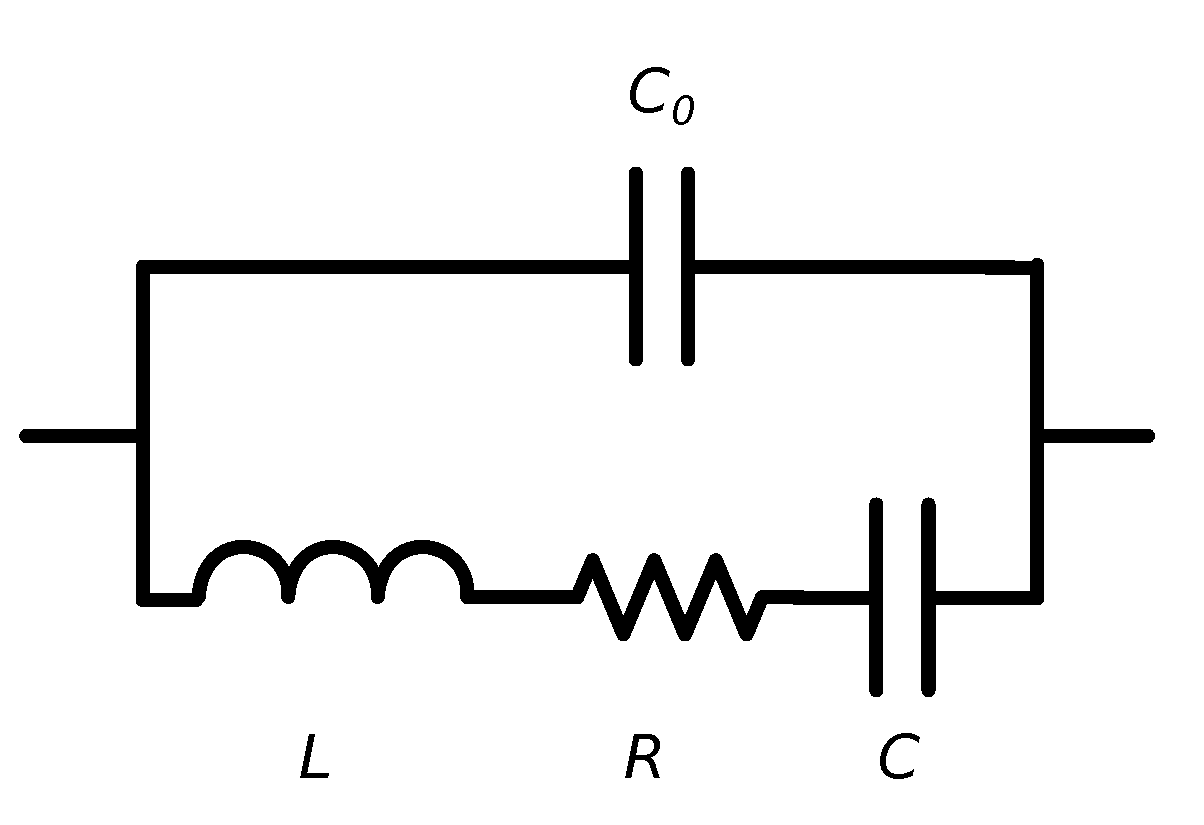
- Drive to Sense capacitance and capacitance variation when sweeping the biasing voltage (C-V).
- Resonance measurements at ambient pressure.
- Current driving path resistance.
- Current driving electrode to Sense node parasitic capacitive coupling.
5.1.2. Packaged Sample Vacuum Measurements
5.1.3. Thermal Characterization
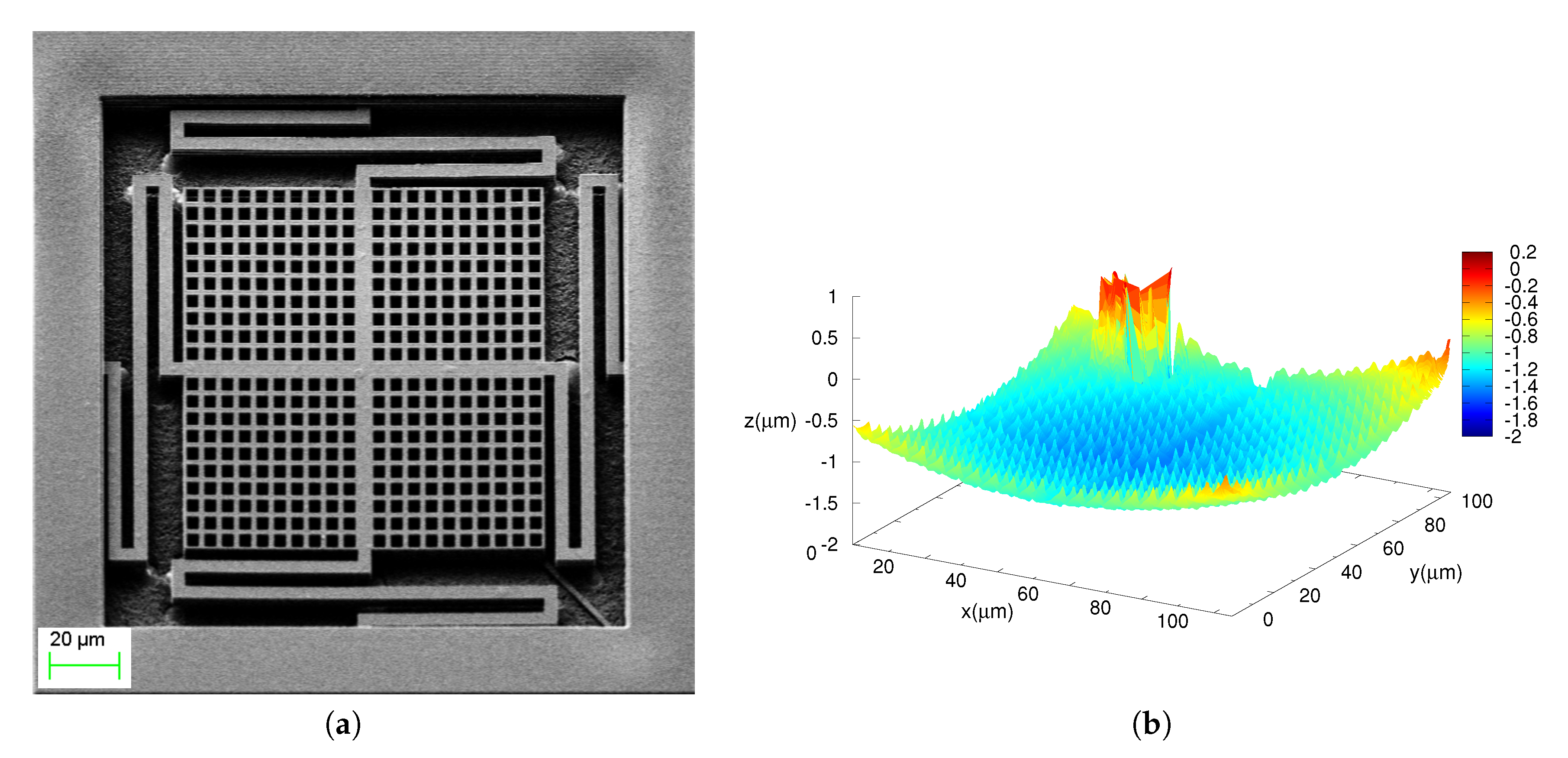
5.2. Optical Analysis
5.3. Capacitance and C-V Variation Measurements
5.4. Resonance Measurements at Ambient Pressure
5.5. Current Driving to Sense Node Capacitive Coupling
5.6. Device Sensitivity to Magnetic Field
5.7. Device Sensitivity to Temperature
5.8. Device Sensitivity to Pressure
6. Discussion
6.1. Figures Recalculation
6.2. BEOL Metal Layers Curvature
6.3. Temperature Variations
7. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Magnetic Sensor. Market and Technology Report-November 2017; Technical Report; Yole Développement: Villeurbanne, France, 2017. [Google Scholar]
- Lahrach, A. eCompass Comparative Report; Technical Report; System Plus Consulting: Nantes, France, 2015. [Google Scholar]
- Lenz, J.; Edelstein, S. Magnetic sensors and their applications. IEEE Sens J. 2006, 6, 631–649. [Google Scholar] [CrossRef]
- Kyynäräinen, J.; Saarilahti, J.; Kattelus, H.; Kärkkäinen, A.; Meinander, T.; Oja, A.; Pekko, P.; Seppä, H.; Suhonen, M.; Kuisma, H.; et al. A 3D micromechanical compass. Sens. Actuators A 2008, 142, 561–568. [Google Scholar] [CrossRef]
- Sonmezoglu, S.; Horsley, D.A. Reducing Offset and Bias Instability in Lorentz Force Magnetic Sensors Through Bias Chopping. J. Microelectromech. Syst. 2017, 26, 169–178. [Google Scholar] [CrossRef]
- Ghosh, S.; Lee, J.E. Eleventh Order Lamb Wave Mode Biconvex Piezoelectric Lorentz Force Magnetometer for Scaling Up Responsivity and Bandwidth. In Proceedings of the 20th International Conference on Solid-State Sensors, Actuators and Microsystems Eurosensors XXXIII (TRANSDUCERS EUROSENSORS XXXIII), Berlin, Germany, 23–27 June 2019; pp. 146–149. [Google Scholar]
- Michalik, P.; Sánchez-Chiva, J.M.; Fernández, D.; Madrenas, J. CMOS BEOL-embedded z-axis accelerometer. Electron. Lett 2015, 51, 865–867. [Google Scholar] [CrossRef] [Green Version]
- Banerji, S.; Fernández, D.; Madrenas, J. Characterization of CMOS-MEMS Resonant Pressure Sensors. IEEE Sens. J. 2017, 17, 6653–6661. [Google Scholar] [CrossRef] [Green Version]
- Li, M.; Horsley, D.A. Offset Suppression in a Micromachined Lorentz Force Magnetic Sensor by Current Chopping. J. Microelectromech. Syst. 2014, 23, 1477–1484. [Google Scholar]
- Li, M.; Sonmezoglu, S.; Horsley, D.A. Extended Bandwidth Lorentz Force Magnetometer Based on Quadrature Frequency Modulation. J. Microelectromech. Syst. 2015, 24, 333–342. [Google Scholar] [CrossRef]
- Sunier, R.; Vancura, T.; Li, Y.; Kirstein, K.; Baltes, H.; Brand, O. Resonant Magnetic Field Sensor With Frequency Output. J. Microelectromech. Syst. 2006, 15, 1098–1107. [Google Scholar] [CrossRef]
- Bahreyni, B.; Shafai, C. A Resonant Micromachined Magnetic Field Sensor. IEEE Sens. J. 2007, 7, 1326–1334. [Google Scholar] [CrossRef]
- Li, M.; Ng, E.J.; Hong, V.A.; Ahn, C.H.; Yang, Y.; Kenny, T.W.; Horsley, D.A. Lorentz force magnetometer using a micromechanical oscillator. Appl. Phys. Lett. 2013, 103, 173504. [Google Scholar] [CrossRef] [Green Version]
- Sánchez-Chiva, J.M.; Valle, J.; Fernández, D.; Madrenas, J. A Mixed-Signal Control System for Lorentz-Force Resonant MEMS Magnetometers. IEEE Sens. J. 2019, 19, 7479–7488. [Google Scholar] [CrossRef] [Green Version]
- Hsu, W.T.; Nguyen, C.T. Stiffness-compensated temperature-insensitive micromechanical resonators. In Proceedings of the fifteenth IEEE International Conference on Micro Electro Mechanical Systems, Las Vegas, NV, USA, 24 January 2002; pp. 731–734. [Google Scholar]
- Chen, W.C.; Fang, W.; Li, S.S. A generalized CMOS-MEMS platform for micromechanical resonators monolithically integrated with circuits. J. Microelectromech. Syst. 2011, 21, 065012. [Google Scholar] [CrossRef]
- Jan, M.T.; Ahmad, F.; Hamid, N.H.B.; Khir, M.H.B.M.; Shoaib, M.; Ashraf, K. Temperature dependent Young’s modulus and quality factor of CMOS-MEMS resonator: Modelling and experimental approach. Microelectron. Reliab. 2016, 57, 64–70. [Google Scholar] [CrossRef]
- Valle, J.; Fernández, D.; Madrenas, J. Experimental Analysis of Vapor HF Etch Rate and Its Wafer Level Uniformity on a CMOS-MEMS Process. J. Microelectromech. Syst. 2016, 25, 401–412. [Google Scholar] [CrossRef]
- Valle, J.; Fernández, D.; Madrenas, J.; Barrachina, L. Curvature of BEOL Cantilevers in CMOS-MEMS Processes. J. Microelectromech. Syst. 2017, 26, 895–909. [Google Scholar] [CrossRef] [Green Version]
- Minotti, P.; Brenna, S.; Laghi, G.; Bonfanti, A.G.; Langfelder, G.; Lacaita, A.L. A Sub-400-nT/, 775-μW, Multi-Loop MEMS Magnetometer With Integrated Readout Electronics. J. Microelectromech. Syst. 2015, 24, 1938–1950. [Google Scholar] [CrossRef]
- Laghi, G.; Marra, C.R.; Minotti, P.; Tocchio, A.; Langfelder, G. A 3-D Micromechanical Multi-Loop Magnetometer Driven Off-Resonance by an On-Chip Resonator. J. Microelectromech. Syst. 2016, 25, 637–651. [Google Scholar] [CrossRef] [Green Version]
- Thompson, M.J.; Horsley, D.A. Parametrically Amplified Z-Axis Lorentz Force Magnetometer. J. Microelectromech. Syst. 2011, 20, 702–710. [Google Scholar] [CrossRef]
- Li, M.; Rouf, V.T.; Thompson, M.J.; Horsley, D.A. Three-Axis Lorentz-Force Magnetic Sensor for Electronic Compass Applications. J. Microelectromech. Syst. 2012, 21, 1002–1010. [Google Scholar] [CrossRef]
- Lee, J.Y.; Seshia, A. Parasitic feedthrough cancellation techniques for enhanced electrical characterization of electrostatic microresonators. Sens. Actuators A 2009, 156, 36–42. [Google Scholar] [CrossRef]
- Yen, T.; Tsai, M.; Chang, C.; Liu, Y.; Li, S.; Chen, R.; Chiou, J.; Fang, W. Improvement of CMOS-MEMS accelerometer using the symmetric layers stacking design. In Proceedings of the SENSORS, 2011 IEEE, Limerick, Ireland, 28–31 October 2011; pp. 145–148. [Google Scholar]
- Tsai, M.; Liu, Y.; Liang, K.; Fang, W. Monolithic CMOS-MEMS Pure Oxide Tri-Axis Accelerometers for Temperature Stabilization and Performance Enhancement. J. Microelectromech. Syst. 2015, 24, 1916–1927. [Google Scholar] [CrossRef]
- Michalik, P.; Fernández, D.; Wietstruck, D.; Kaynak, M.; Madrenas, J. Experiments on MEMS Integration in 0.25 μm CMOS Process. Sensors 2018, 18, 2111. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sánchez-Chiva, J.M.; Fernández, D.; Madrenas, J. A test setup for the characterization of Lorentz-force MEMS magnetometers. In Proceedings of the 27th IEEE International Conference on Electronics, Circuits and Systems (ICECS), Glasgow, Scotland, 23–25 November 2020. accepted as presentation. [Google Scholar]
- Kim, B.; Hopcroft, M.A.; Candler, R.N.; Jha, C.M.; Agarwal, M.; Melamud, R.; Chandorkar, S.A.; Yama, G.; Kenny, T.W. Temperature Dependence of Quality Factor in MEMS Resonators. J. Microelectromech. Syst. 2008, 17, 755–766. [Google Scholar] [CrossRef] [Green Version]
- Downey, R.H.; Karunasiri, G. Reduced Residual Stress Curvature and Branched Comb Fingers Increase Sensitivity of MEMS Acoustic Sensor. J. Microelectromech. Syst. 2014, 23, 417–423. [Google Scholar] [CrossRef]
- Cheng, C.L.; Tsai, M.H.; Fang, W. Determining the thermal expansion coefficient of thin films for a CMOS MEMS process using test cantilevers. J. Microelectromech. Syst. 2015, 25. [Google Scholar] [CrossRef]
- Buffa, C. MEMS Lorentz Force Magnetometers; Springer: Cham, Switzerland, 2017; p. 55. [Google Scholar]




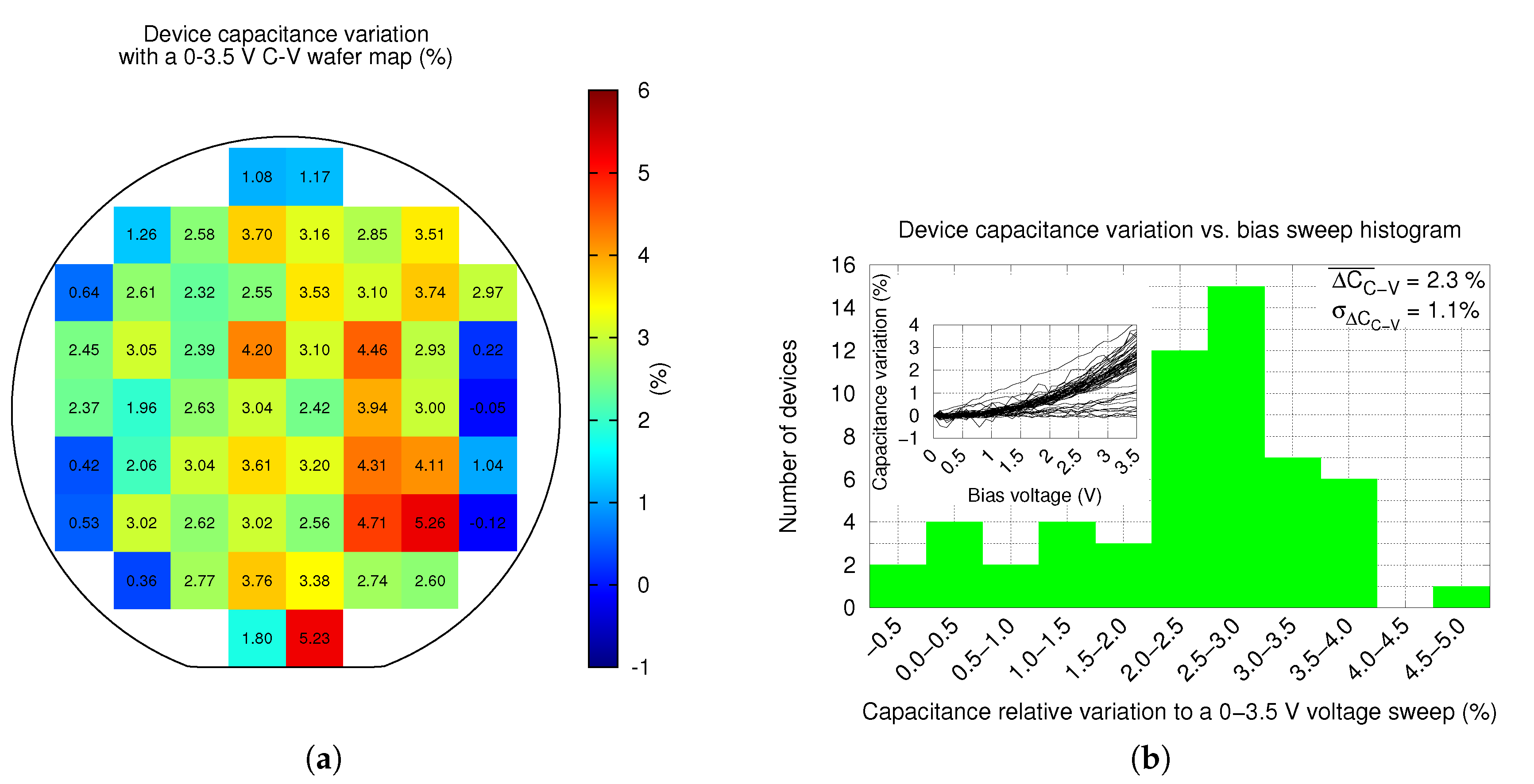
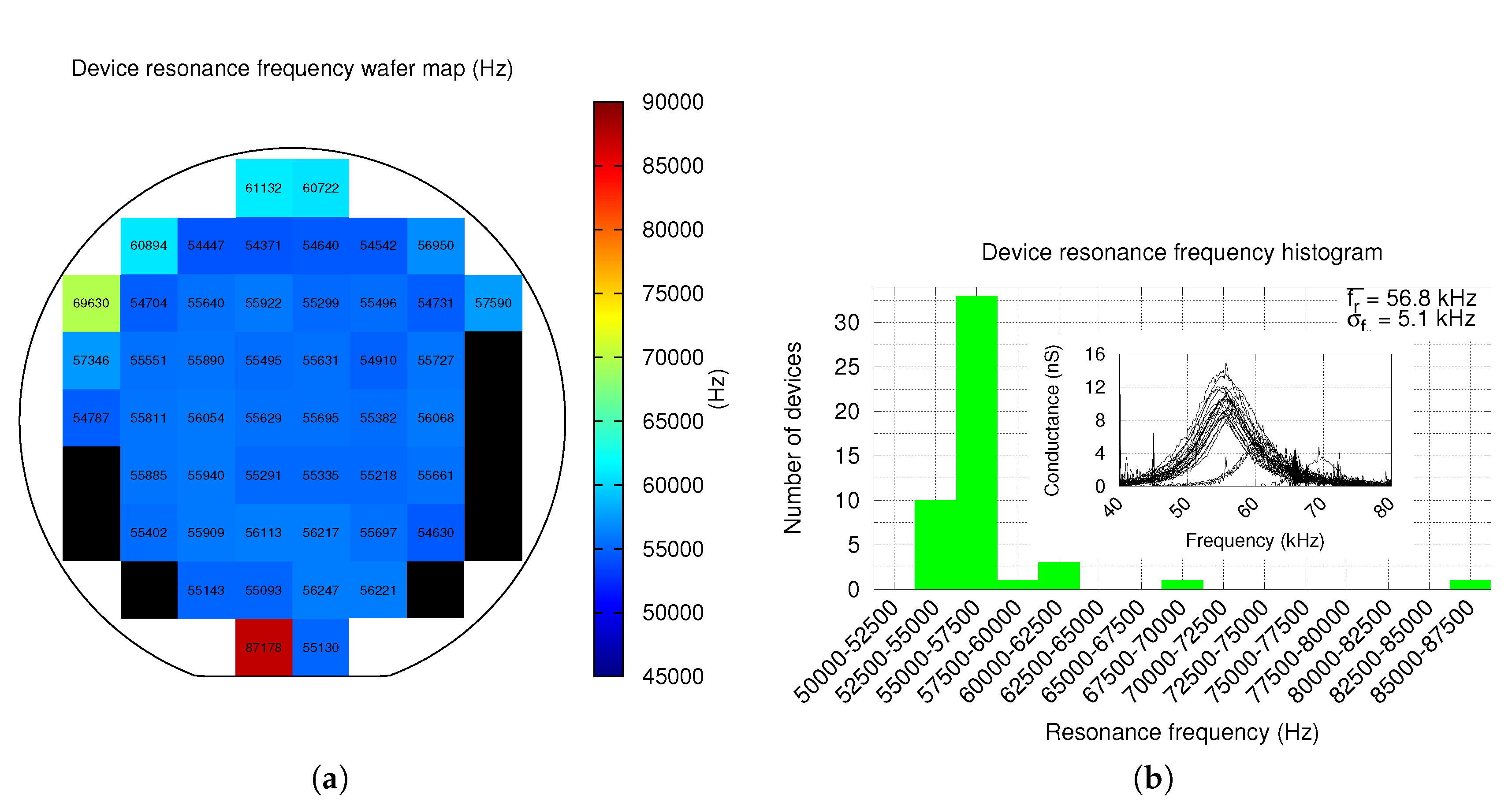
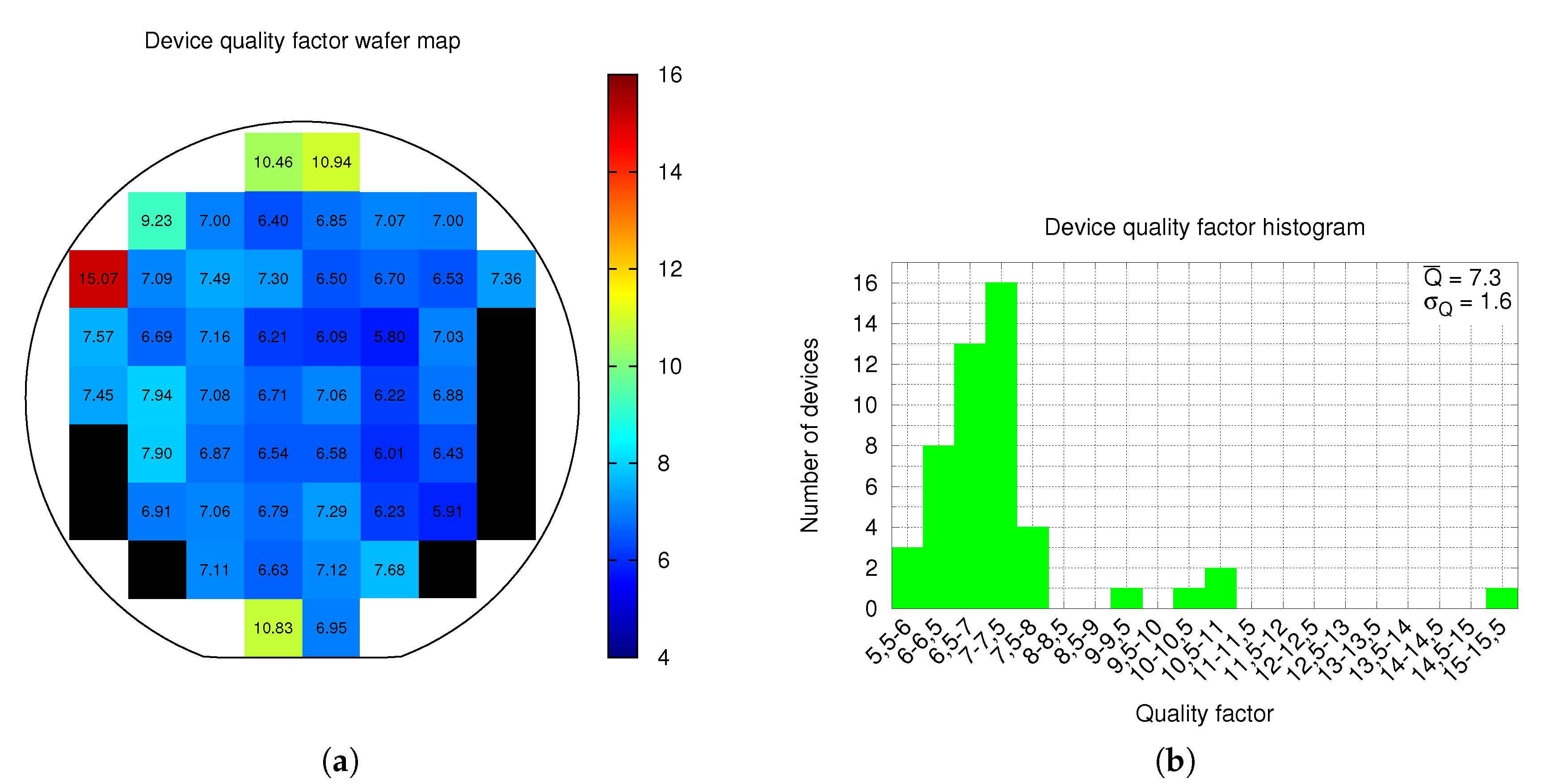


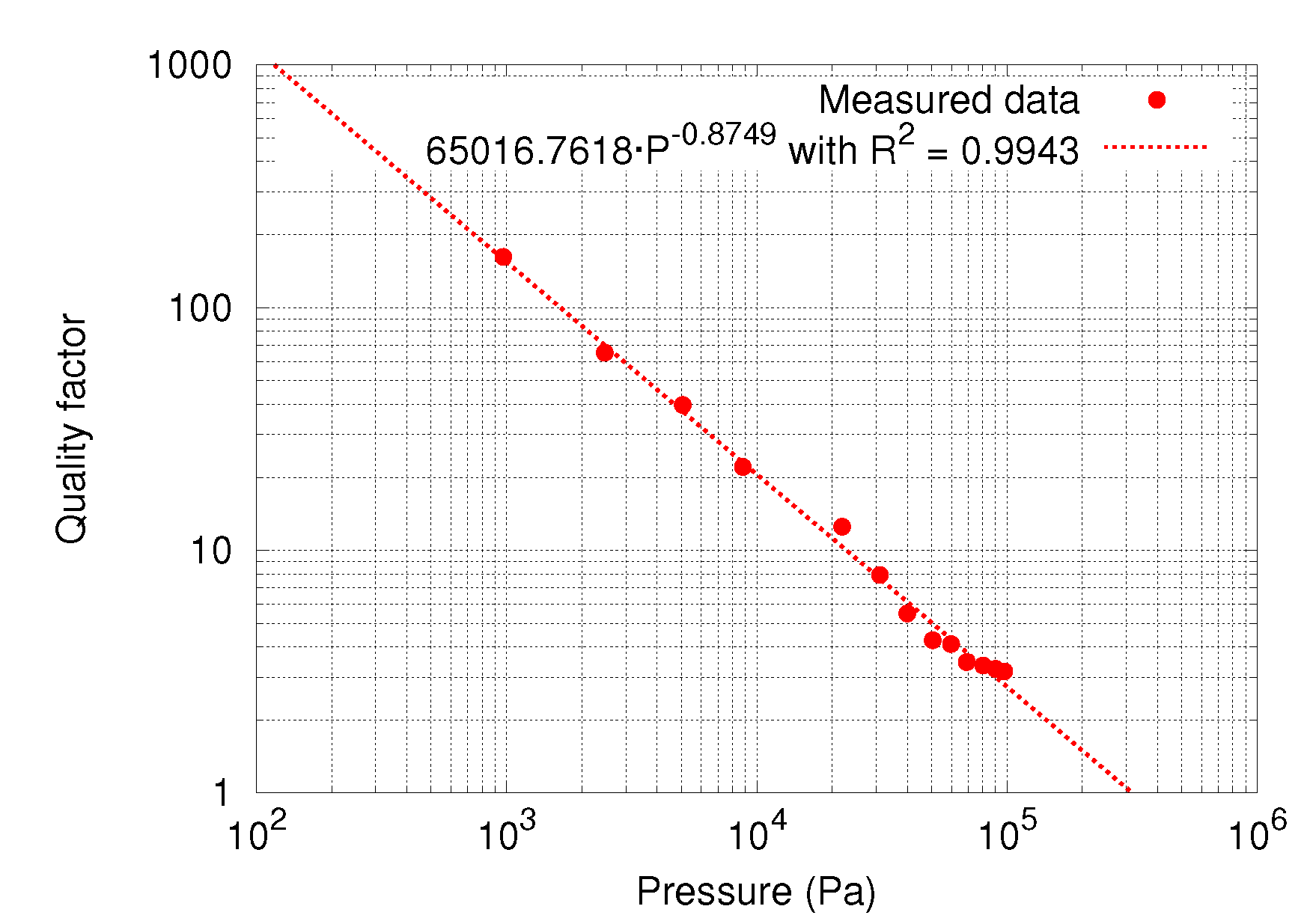

| (fF) | (fF) | (%) | (%) | |
|---|---|---|---|---|
| All Samples | 118.2 | 17.7 | 2.3 | 1.1 |
| Only Samples that Resonate | 112.4 | 10.1 | 2.7 | 0.8 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sánchez-Chiva, J.M.; Valle, J.; Fernández, D.; Madrenas, J. A CMOS-MEMS BEOL 2-axis Lorentz-Force Magnetometer with Device-Level Offset Cancellation. Sensors 2020, 20, 5899. https://doi.org/10.3390/s20205899
Sánchez-Chiva JM, Valle J, Fernández D, Madrenas J. A CMOS-MEMS BEOL 2-axis Lorentz-Force Magnetometer with Device-Level Offset Cancellation. Sensors. 2020; 20(20):5899. https://doi.org/10.3390/s20205899
Chicago/Turabian StyleSánchez-Chiva, Josep Maria, Juan Valle, Daniel Fernández, and Jordi Madrenas. 2020. "A CMOS-MEMS BEOL 2-axis Lorentz-Force Magnetometer with Device-Level Offset Cancellation" Sensors 20, no. 20: 5899. https://doi.org/10.3390/s20205899
APA StyleSánchez-Chiva, J. M., Valle, J., Fernández, D., & Madrenas, J. (2020). A CMOS-MEMS BEOL 2-axis Lorentz-Force Magnetometer with Device-Level Offset Cancellation. Sensors, 20(20), 5899. https://doi.org/10.3390/s20205899





