Static Electromechanical Characteristic of a Three-Layer Circular Piezoelectric Transducer
Abstract
1. Introduction
2. Electromechanical Characteristic of a Three-Layer Circular Piezoelectric Transducer
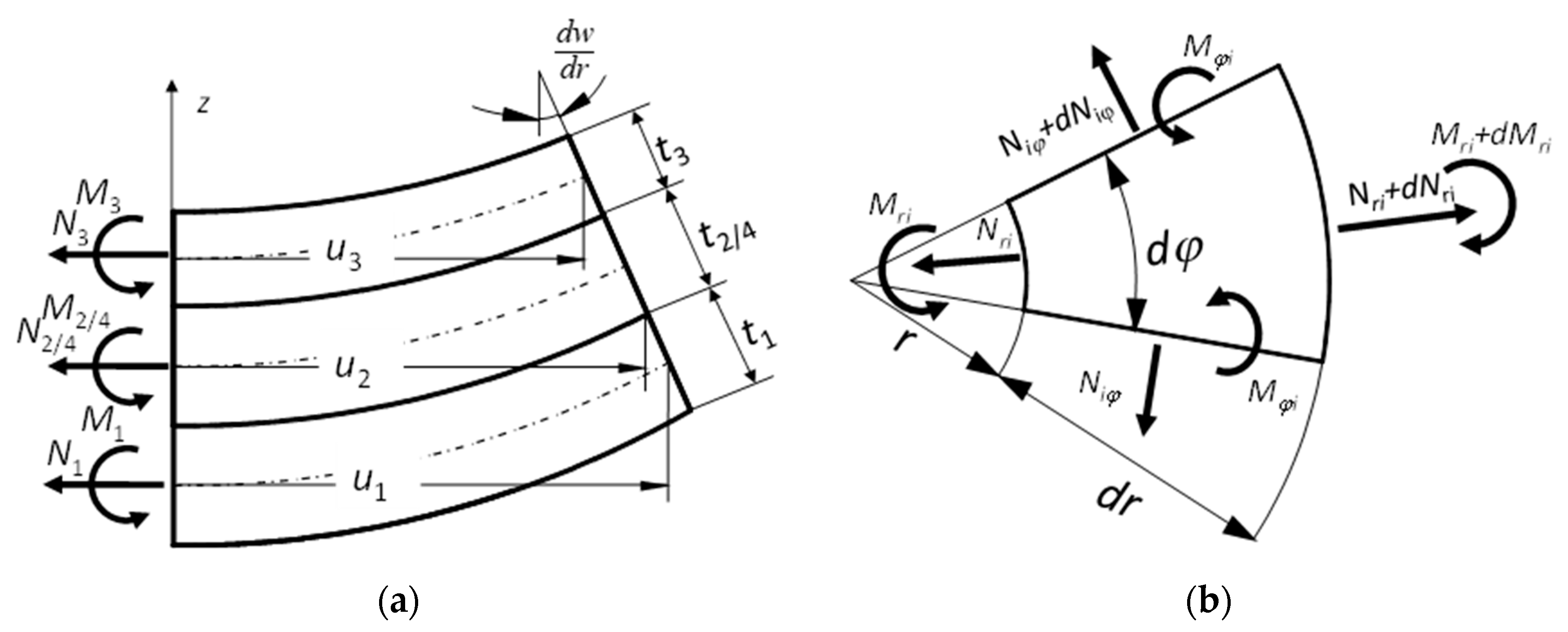
2.1. Basic Assumptions
- the total thickness of all layers is much smaller than their radius, therefore the Plate Theory [29] was used to determine transducer deflection;
- the thickness of the adhesive layers and electrodes is very small and has no effect on the transducer deflection;
- between individual transducer layers there are no slips and the cross-sections remain plane after deformation.
- in the piezoelectric disk, only transverse piezoelectric effect occurs.
2.2. Analytical Description of Transducer Deformation
- for the interval r < Ro:
- for the interval Ro < r < R
- for the interval r < Ro:
- for the interval Ro < r < R
- for the interval r < Ro:
- for the interval Ro < r < R
- for the interval r < Ro:
- for the interval Ro < r < R:
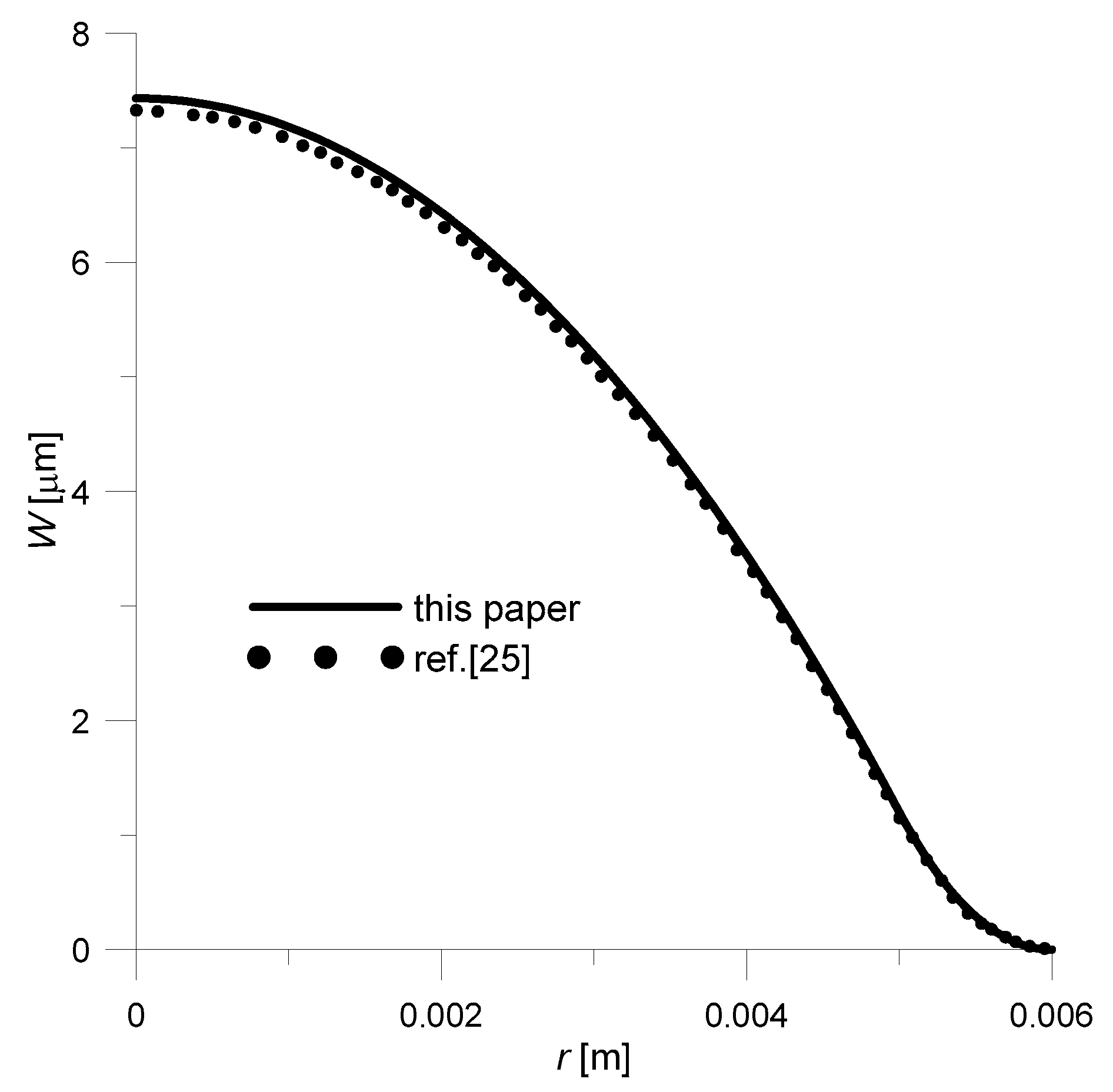
3. Verification of the Analytical Solution
- (a)
- geometrical dimensions: R = 2.54 × 10−2 m, Ro = 1.27 × 10−2 m, t1 = 5.08 × 10−4 m, t2 = 1.127 × 10−4 m
- (b)
- material data:
- bottom layer made of aluminium; E1 = 70 × 109 Pa, ν1 = 0.33;
- piezoelectric disk made of PZT-5H (Piezo Material Lead Zirconate Titanate); E2 = 1/s11 = 6.06 × 1010 Pa, ν2 = −s12/s11 = 0.289, d31 = −2.74 × 10−10 m/V;
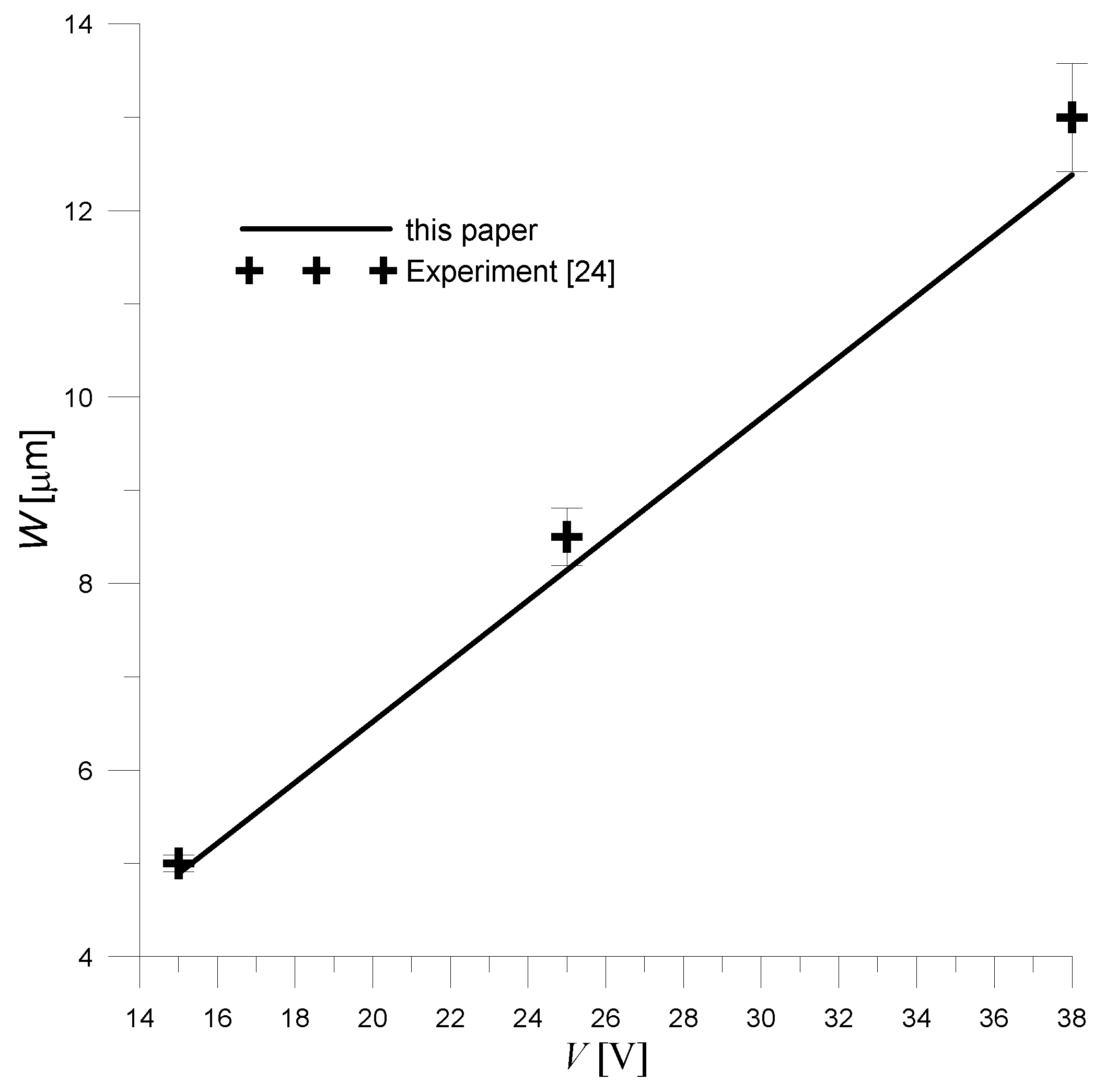
- (a)
- geometrical dimensions: R = 6 × 10−2 m, Ro = 5.5 × 10−2 m, t1 = 2.5 × 10−4 m, t2 = t4 = 2.5 × 10−4 m, t3 = 3 × 10−4 m.
- (b)
- material data:
- bottom layer made of copper; E1= 13 × 1010 Pa, ν1 = 0.34;
- upper layer made of PTFE (Polytetrafluoroethylene); E3 = 0.4 × 109 Pa, ν3 = 0.46;
- piezoelectric disk made of PZT-5H (Piezo Material Lead Zirconate Titanate); E2 = 1/s11 = 6.06 × 1010 Pa, ν2 = −s12/s11 = 0.289, d31 = −2.74 × 10−10 m/V;
- the middle ring made of foam; E4 = 35.8 × 106 Pa, ν4 = 0.383.
- (c)
- applied electrical load: V = −100 V.
4. Influence of Geometrical-Material Parameters on the Electromechanical Characteristics of a Three-Layer Transducer
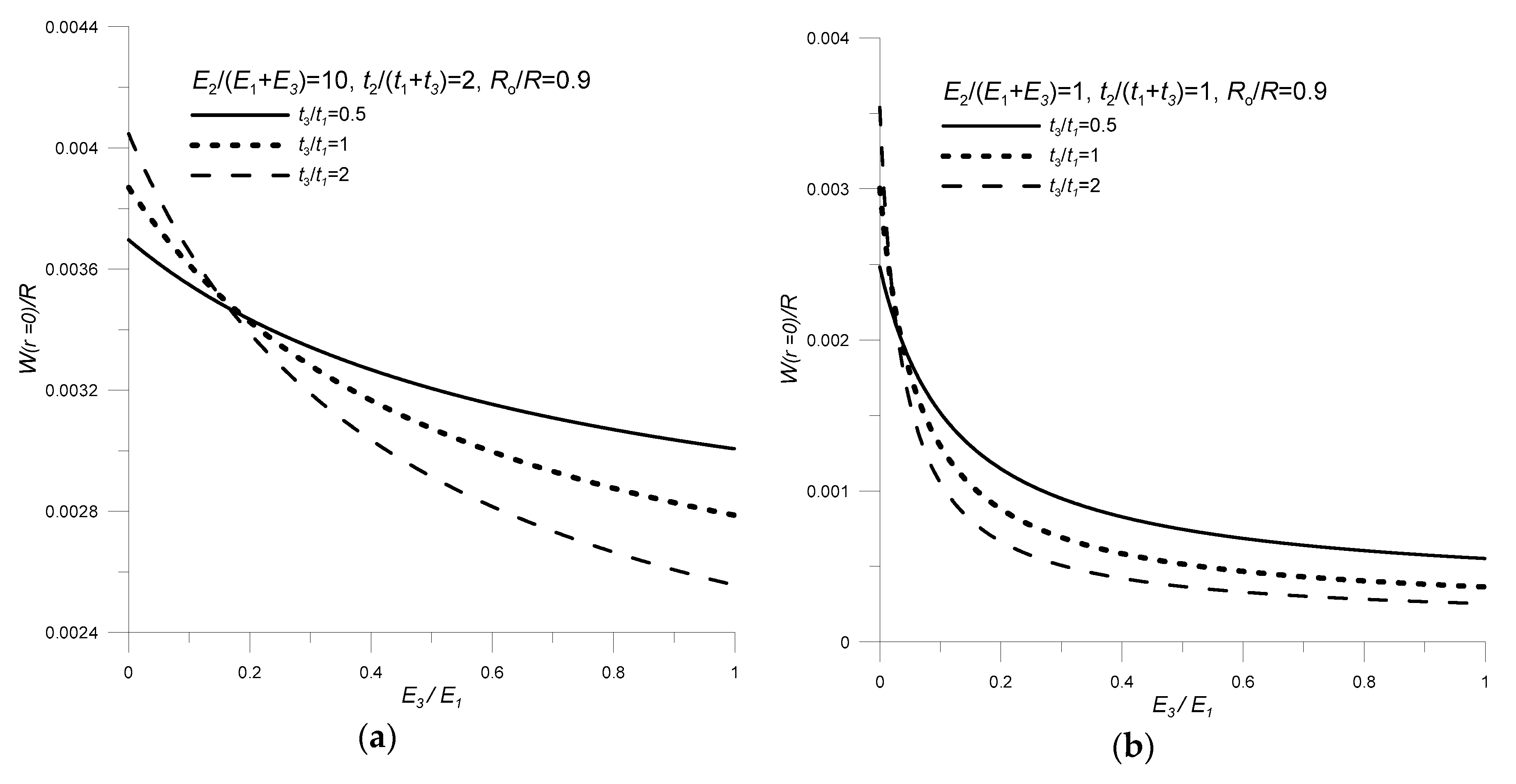
- the relative thickness of piezo and non-piezo elements: tg = t2/(t1 + t3);
- the elastic moduli ratio of piezo and non-piezoelectric components: Eg = E2/(E1 + E3);
- the relative radius of piezoelectric disk and non-piezoelectric layers: Rg = Ro/R;
- the relative thickness of the top and bottom layers: tnp = t3/t1;
- the ratio of elastic moduli of non-electrical layers: Enp = E3/E1.
- the transducer deflection increases as the rigidity of one of the non-electrical components decreases (Enp decrease);
- an increase in the relative thickness tnp, depending on the ratio of elastic moduli Enp, may cause an increase or decrease in the transducer deflection value.
5. Summary and Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Ştefănescu, D.M. Piezoelectric Force Transducers (PZFTs). In Handbook of Force Transducers: Principles and Components; Ştefănescu, D.M., Ed.; Springer: Berlin/Heidelberg, Germany, 2011; pp. 109–130. ISBN 978-3-642-18296-9. [Google Scholar]
- Zhao, Q.; Wang, L.; Zhao, K.; Yang, H. Development of a Novel Piezoelectric Sensing System for Pavement Dynamic Load Identification. Sensors 2019, 19, 4668. [Google Scholar] [CrossRef] [PubMed]
- Afzal, M.S.; Shim, H.; Roh, Y. Design of a Piezoelectric Multilayered Structure for Ultrasound Sensors Using the Equivalent Circuit Method. Sensors 2018, 18, 4491. [Google Scholar] [CrossRef] [PubMed]
- DeVoe, D.L. Piezoelectric thin film micromechanical beam resonators. Sens. Actuators A Phys. 2001, 88, 263–272. [Google Scholar] [CrossRef]
- Tzou, H. Piezoelectric Shells: Distributed Sensing and Control of Continua; Solid Mechanics and Its Applications; Springer: Dordrecht, The Netherlands, 1993; ISBN 978-94-010-4784-5. [Google Scholar]
- Curie, P.J.; Curie, J. Crystal Physics-Development by Pressure 0/Polar Electricity in Hemihedral Crystals with Inclined Faces. CR Acad. Sci. 1880, 91, 294. (In French) [Google Scholar]
- Berlincourt, D.A.; Curran, D.R.; Jaffe, H. Piezoelectric and Piezomagnetic Materials and Their Function in Transducers. In Physical Acoustics; Mason, W.P., Ed.; Academic Press Inc.: London, UK, 1964; pp. 169–270. ISBN 978-1-4832-2857-0. [Google Scholar]
- Nguyen, V.-T.; Kumar, P.; Leong, J.Y.C. Finite Element Modellingand Simulations of Piezoelectric Actuators Responses with Uncertainty Quantification. Computation 2018, 6, 60. [Google Scholar] [CrossRef]
- Rahmoune, M.; Osmont, D. Classic finite elements for simulation of piezoelectric smart structures. Mechanics 2010, 86, 50–57. [Google Scholar]
- Dobrucki, A.B.; Pruchnicki, P. Theory of piezoelectric axisymmetric bimorph. Sens. Actuators A Phys. 1997, 58, 203–212. [Google Scholar] [CrossRef]
- Borawski, A. Common Methods in Analysing the Tribological Properties of Brake Pads and Discs—Review. Acta Mech. Autom. 2019, 13, 189–199. [Google Scholar] [CrossRef]
- Chen, N.; Yan, P.; Ouyang, J. A generalized approach on bending and stress analysis of beams with piezoelectric material bonded. Sens. Actuators A Phys. 2019, 290, 54–61. [Google Scholar] [CrossRef]
- Wang, Q.M.; Cross, L.E. Constitutive equations of symmetrical triple layer piezoelectric benders. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 1999, 46, 1343–1351. [Google Scholar] [CrossRef]
- Ballas, R.G.; Schlaak, H.F.; Schmid, A.J. The constituent equations of piezoelectric multilayer bending actuators in closed analytical form and experimental results. Sens. Actuators A Phys. 2006, 130–131, 91–98. [Google Scholar] [CrossRef]
- Xiang, H.J.; Shi, Z.F. Static analysis for multi-layered piezoelectric cantilevers. Int. J. Solids Struct. 2008, 45, 113–128. [Google Scholar] [CrossRef]
- Park, J.-K.; Moon, W.-K. Constitutive relations for piezoelectric benders under various boundary conditions. Sens. Actuators A Phys. 2005, 117, 159–167. [Google Scholar] [CrossRef]
- Raeisifard, H.; Bahrami, M.N.; Yousefi-Koma, A.; Fard, H.R. Static characterization and pull-in voltage of a micro-switch under both electrostatic and piezoelectric excitations. Eur. J. Mech. Solids 2014, 44, 116–124. [Google Scholar] [CrossRef]
- Mieczkowski, G. Electromechanical characteristics of piezoelectric converters with freely defined boundary conditions and geometry. Mechanics 2016, 22, 265–272. [Google Scholar] [CrossRef][Green Version]
- Mieczkowski, G. The constituent equations of piezoelectric cantilevered three-layer actuators with various external loads and geometry. J. Theor. Appl. Mech. 2017, 55, 69–86. [Google Scholar] [CrossRef]
- Mieczkowski, G. Optimization and Prediction of Durability and Utility Features of Three-Layer Piezoelectric Transducers. Mechanics 2018, 24, 335–342. [Google Scholar] [CrossRef]
- Li, S.; Chen, S. Analytical analysis of a circular PZT actuator for valveless micropumps. Sens. Actuators A Phys. 2003, 104, 151–161. [Google Scholar] [CrossRef]
- Adelman, N.T.; Stavsky, Y. Flexural–extensional behavior of composite piezoelectric circular plates. J. Acoust. Soc. Am. 1980, 67, 819–822. [Google Scholar] [CrossRef]
- Takesaburo, Y.; Yuji, N. Determination of Optimum Dimensions for Unimorph Type Piezo-Electric Loudspeaker. Trans. Inst. Electron. Inf. Commun. Eng. A 1993, 76, 1261–1269. [Google Scholar]
- Mo, C.; Wright, R.; Slaughter, W.S.; Clark, W.W. Behaviour of a unimorph circular piezoelectric actuator. Smart Mater. Struct. 2006, 15, 1094–1102. [Google Scholar] [CrossRef]
- Guan, E.; Ge, Y.; Liu, J.; Yan, W.; Zhao, Y. Piezoelectric Micro-Pump Suction Cup Design and Research on the Optimal Static Driving Characteristics. In Proceedings of the Intelligent Robotics and Applications; Huang, Y., Wu, H., Liu, H., Yin, Z., Eds.; Springer International Publishing: Cham, Switzerland, 2017; pp. 26–38. [Google Scholar]
- Yihua, H.; Wenjin, H. Research on the displacement function and equivalent circuit of circular flexural vibration mode piezoelectric ceramic composite transducers. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2013, 60, 218–234. [Google Scholar] [CrossRef] [PubMed]
- Li, X.; Du, H.; Xu, L.; Hu, Y.; Xu, L. Optimization of a circular thin-film piezoelectric actuator lying on a clamped multilayered elastic plate. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2009, 56, 1469–1475. [Google Scholar] [PubMed]
- Dong, S.; Du, X.-H.; Bouchilloux, P.; Uchino, K. Piezoelectric Ring-Morph Actuators for Valve Application. J. Electroceram. 2002, 8, 155–161. [Google Scholar] [CrossRef]
- Timoshenko, S.; Woinowsky-Krieger, S. Theory of Plates and Shells, 2nd ed.; McGraw-Hill College: New York, NY, USA, 1959; ISBN 978-0-07-064779-4. [Google Scholar]
- Bishop, R. The Mechatronics Handbook. Available online: https://www.twirpx.com/file/382469/ (accessed on 14 November 2019).
- Borawski, A. Modification of a fourth generation LPG installation improving the power supply to a spark ignition engine. Eksploatacja i Niezawodność/Maint. Reliab. 2015, 17, 1–6. [Google Scholar] [CrossRef]
- Łukaszewicz, A. Nonlinear Numerical Model of Friction Heating during Rotary Friction Welding. J. Frict. Wear 2018, 39, 476–482. [Google Scholar] [CrossRef]
- Szpica, D. Research on the influence of LPG/CNG injector outlet nozzle diameter on uneven fuel dosage. Transport 2018, 33, 186–196. [Google Scholar] [CrossRef]
- Trochimczuk, R.; Łukaszewicz, A.; Mikołajczyk, T.; Aggogeri, F.; Borboni, A. Finite element method stiffness analysis of a novel telemanipulator for minimally invasive surgery. Simulation 2019, 95, 1015–1025. [Google Scholar] [CrossRef]
- Pulawski, G.; Szpica, D. The modelling of the compression ignition engine powered with diesel fuel with LPG admixture. Mechanics 2015, 21, 493–499. [Google Scholar] [CrossRef]
- Structural Mechanics Module, COMSOL Documentation. Available online: https://doc.comsol.com/5.5/docserver/#!TOC:/com.comsol.help.sme/toc.xml:SOURCE:resource_tocfile_-1058655296.html (accessed on 30 November 2019).
- MEMS Module, COMSOL Documentation. Available online: https://doc.comsol.com/5.5/docserver/#!TOC:/com.comsol.help.mems/toc.xml:SOURCE:resource_tocfile_573990530.html (accessed on 30 November 2019).
- Piezoelectric Shear-Actuated Beam. Available online: https://www.comsol.com/model/piezoelectric-shear-actuated-beam-24 (accessed on 30 November 2019).
- Cao, L.; Mantell, S.; Polla, D. Design and simulation of an implantable medical drug delivery system using microelectromechanical systems technology. Sens. Actuators A Phys. 2001, 94, 117–125. [Google Scholar] [CrossRef]
- Szpica, D.; Borawski, A.; Mieczkowski, G. New Concept of Low-Pressure Gas-Phase Injector. In Proceedings of the 23rd International Scientific Conference “Mechanika 2018”; Fedaravičius, A., Griškevičius, P., Kibirkštis, E., Leišis, V., Mikalauskas, R., Pilkaitė, T., Rimašauskas, M., Eds.; Kaunas University of Technology: Kaunas, Lithuania, 2018; pp. 173–177. [Google Scholar]
- Koch-Dücker, H.-J.; Papert, U. Antilock braking system (ABS). In Brakes, Brake Control and Driver Assistance Systems: Function, Regulation and Components; Bosch Professional Automotive Information; Reif, K., Ed.; Springer Fachmedien: Wiesbaden, Germany, 2014; pp. 74–93. ISBN 978-3-658-03978-3. [Google Scholar]



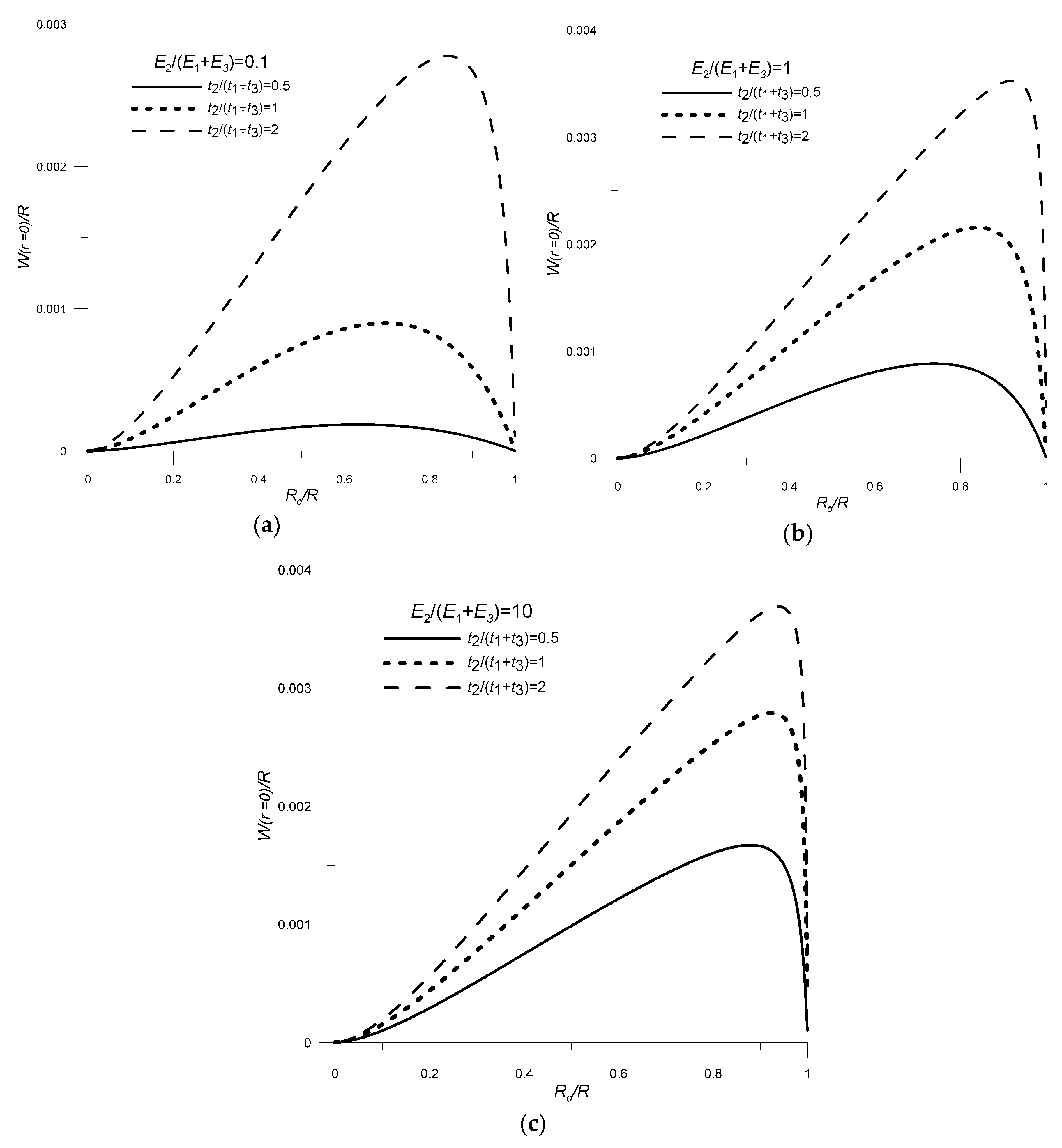
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mieczkowski, G.; Borawski, A.; Szpica, D. Static Electromechanical Characteristic of a Three-Layer Circular Piezoelectric Transducer. Sensors 2020, 20, 222. https://doi.org/10.3390/s20010222
Mieczkowski G, Borawski A, Szpica D. Static Electromechanical Characteristic of a Three-Layer Circular Piezoelectric Transducer. Sensors. 2020; 20(1):222. https://doi.org/10.3390/s20010222
Chicago/Turabian StyleMieczkowski, Grzegorz, Andrzej Borawski, and Dariusz Szpica. 2020. "Static Electromechanical Characteristic of a Three-Layer Circular Piezoelectric Transducer" Sensors 20, no. 1: 222. https://doi.org/10.3390/s20010222
APA StyleMieczkowski, G., Borawski, A., & Szpica, D. (2020). Static Electromechanical Characteristic of a Three-Layer Circular Piezoelectric Transducer. Sensors, 20(1), 222. https://doi.org/10.3390/s20010222






