An Approach to Measure Tilt Motion, Straightness and Position of Precision Linear Stage with a 3D Sinusoidal-Groove Linear Reflective Grating and Triangular Wave-Based Subdivision Method
Abstract
1. Introduction
2. Overall Scheme and Measurement Principle
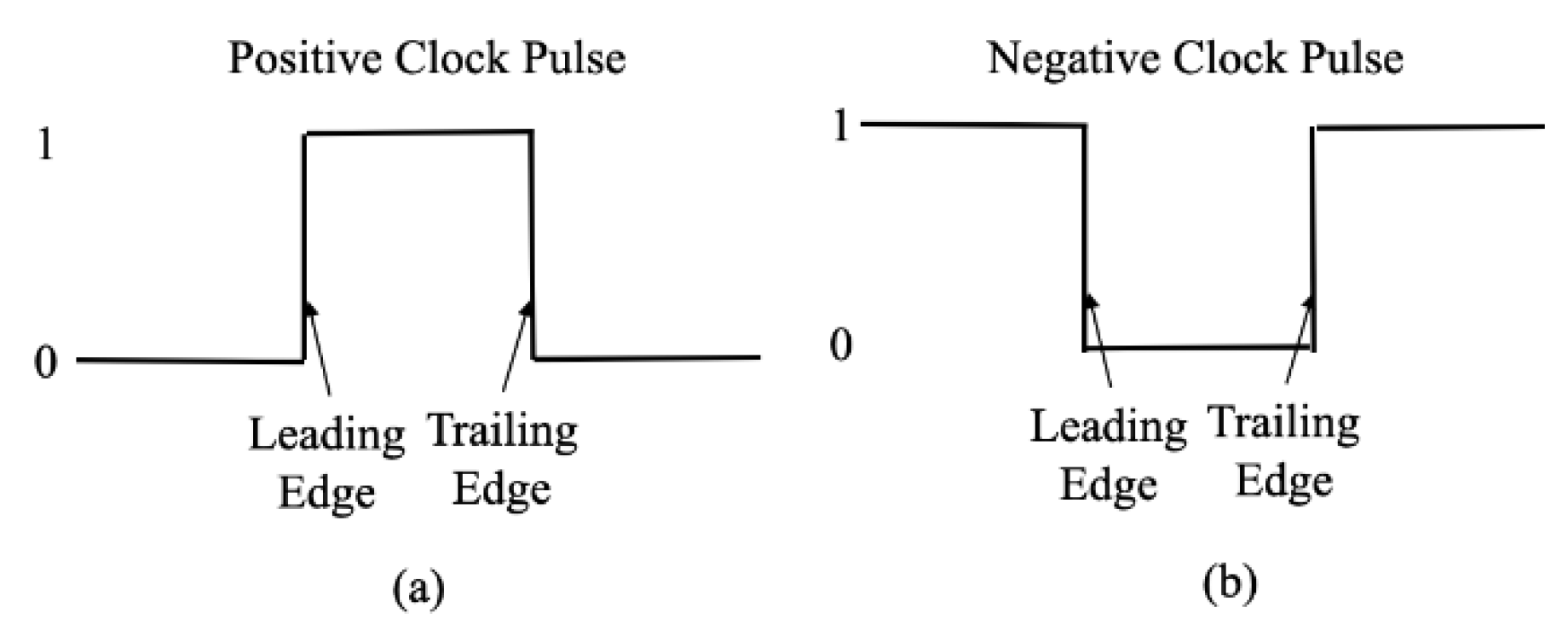
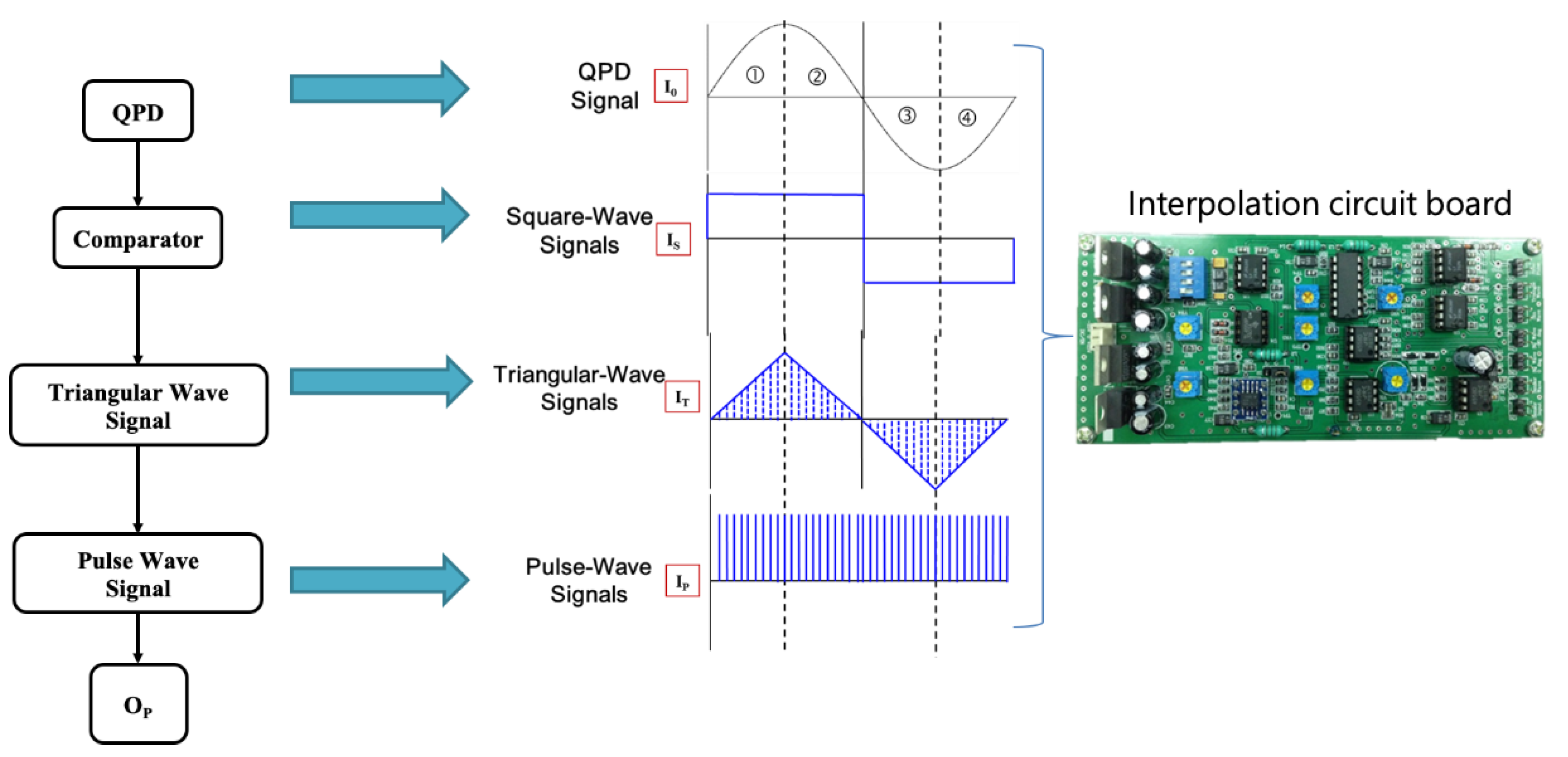
3. Analyzing the Sequence Signal by Using the Triangular Wave-Based Pulse Triggering Method
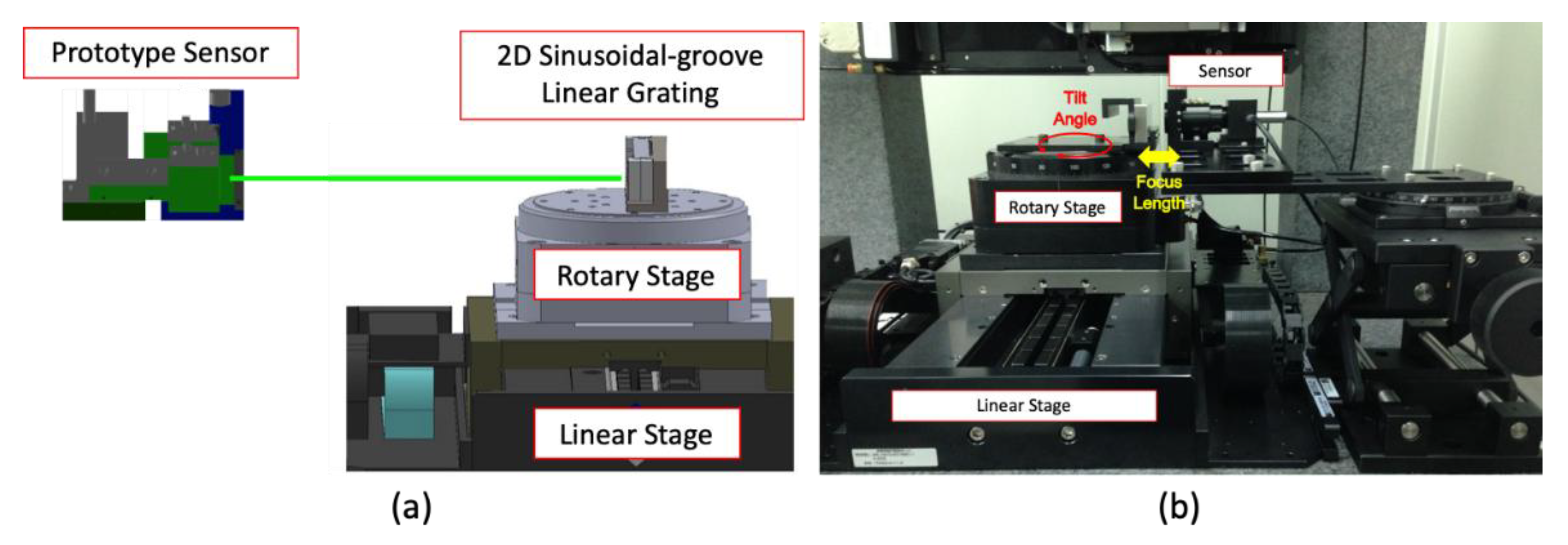
4. Experimental Setup
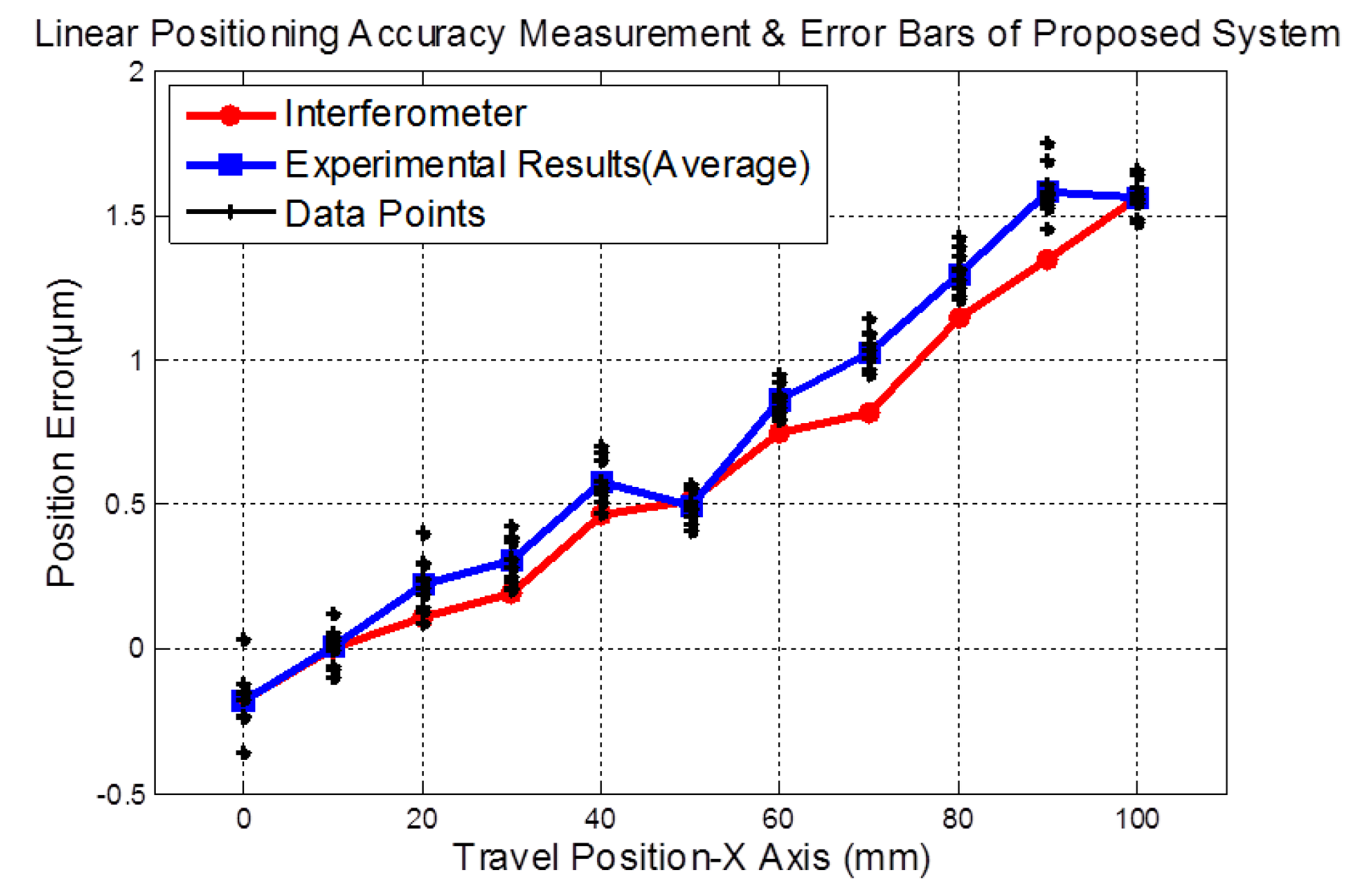
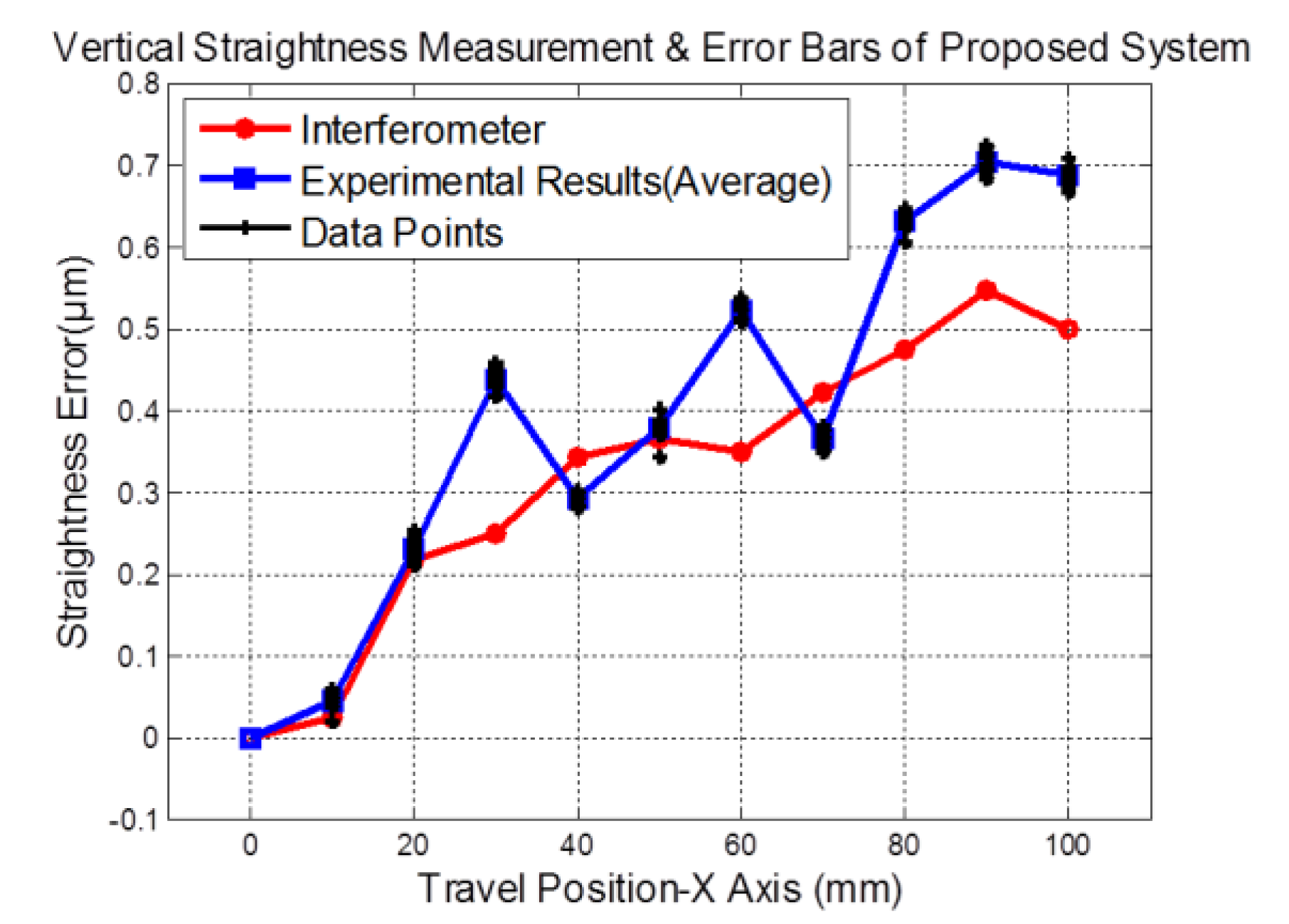
5. Experimental Results
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- ASME. Methods for Performance Evaluation of Computer Numerically Controlled Machining Centers B5.54; ASME: New York, NY, USA, 2005. [Google Scholar]
- ISO 230-1:2012. Test Code for Machine Tools—Part 1: Geometric Accuracy of Machines Operating under No-Load or Finishing Conditions; ISO: London, UK, 2012. [Google Scholar]
- Kim, O.; Lee, S.; Han, D. Positioning performance and straightness error compensation of the magnetic levitation stage supported by the linear magnetic bearing. IEEE Trans. Ind. Electron. 2003, 50, 374–378. [Google Scholar]
- Kang, M.S.; Kim, D.H.; Yoon, J.S.; Park, B.S.; Lee, J.K. Straightness Error Compensation Servo-system for Single-axis Linear Motor Stage. World Acad. Sci. Eng. Technol. 2009, 50, 158–162. [Google Scholar]
- Gao, W.; Arai, Y.; Shibuya, A.; Kiyono, S.; Park, C.H. Measurement of multi-degree-of-freedom error motions of a precision linear air-bearing stage. Precis. Eng. 2006, 30, 96–103. [Google Scholar] [CrossRef]
- King, R.J.; Raine, K.W. Polarimetry Applied to Alignment and Angle Measurement. Opt. Eng. 1981, 201, 39–43. [Google Scholar] [CrossRef]
- Yin, C.; Chen, W.Z. Two-dimensional automatic straightness measurement system based on optical activity. Opt. Eng. 1991, 304, 480–482. [Google Scholar]
- Armstrong, T.R.; Fitzgerald, M.P. Autocollimator based on the laser head of a compact disc player. Meas. Sci. Technol. 1992, 3, 1072–1076. [Google Scholar]
- Yang, P.; Takamura, T.; Takahashi, S.; Takamasu, K.; Sato, O.; Osawa, S.; Takatsuji, T. Development of high-precision micro-coordinate measuring machine: Multi-probe measurement system for measuring yaw and straightness motion error of XY linear stage. Precis. Eng. 2011, 35, 424–430. [Google Scholar]
- Yang, P.; Takamura, T.; Takahashi, S.; Takamasu, K.; Sato, O.; Osawa, S.; Takatsuji, T. Multi-probe scanning system comprising three laser interferometers and one autocollimator for measuring flat bar mirror profile with nanometer accuracy. Precis. Eng. 2011, 35, 686–692. [Google Scholar]
- Fan, K.C.; Chen, M.J. A 6-degree-of-freedom measurement system for the accuracy of X-Y stages. Precis. Eng. 2000, 24, 15–23. [Google Scholar] [CrossRef]
- Chen, B.; Zhang, E.; Yang, L.; Li, C.; Tang, W.; Feng, Q. A laser interferometer for measuring straightness and its position based on heterodyne interferometry. Rev. Sci. Instrum. 2009, 80, 115113. [Google Scholar] [CrossRef] [PubMed]
- Rahneberg, I.; Büchner, H.J.; Jäger, J. Optical system for the simultaneous measurement of two-dimensional straightness errors and the roll angle. Opt. Express 2015, 23, 9052–9073. [Google Scholar]
- Jywe, W.Y.; Liu, C.H.; Shien, W.H.; Shyu, L.H.; Fang, T.H.; Sheu, Y.H.; Hsu, T.H.; Hsieh, C.C. Developed of a multi-degree of freedoms measuring system and an error compensation technique for machine tools. J. Phys. Conf. Ser. 2006, 48, 761–765. [Google Scholar] [CrossRef]
- Kimura, A.; Gao, W.; Zeng, L.J. Position and out-of-straightness measurement of a precision linear air-bearing stage by using a two-degree-of-freedom linear encoder. Meas. Sci. Technol. 2010, 21, 054005. [Google Scholar] [CrossRef]
- Liu, C.H.; Cheng, C.H. Development of a grating based multi-degree-of-freedom laser linear encoder using diffracted light. Sens. Actuators A Phys. 2012, 181, 87–93. [Google Scholar] [CrossRef]
- Wang, C.; Zhong, F.; Eills, J.D. Two-dimensional straightness measurement based on optical knife-edge sensing. Rev. Sci. Instrum. 2017, 88, 095109. [Google Scholar] [CrossRef] [PubMed]
- Lee, C.B.; Lee, S.K.; Tarbutton, J.A. Novel design and sensitivity analysis of displacement measurement system utilizing knife edge diffraction for nanopositioning stages. Rev. Sci. Instrum. 2014, 85, 095113. [Google Scholar] [CrossRef] [PubMed]
- Zhu, L.J.; Li, L.; Liu, J.H.; Zhang, Z.H. A method for measuring the guideway straightness error based on polarized interference principle. Int. J. Mach. Tools Manuf. 2009, 49, 285–290. [Google Scholar] [CrossRef]
- Huang, P.; Li, Y.; Wei, H.Y. Straightness measurement system based on phase sensitive detection technique. In Proceedings of the 2013 International Conference on Optical Instruments and Technology: Optoelectronic Measurement Technology and Systems, Beijing, China, 19 December 2013. [Google Scholar]
- Jenkins, F.A.; White, H.E. Fundamentals of Optics; McGraw-Hill: New York, NY, USA, 1976. [Google Scholar]



© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tsai, H.-A.; Lo, Y.-L. An Approach to Measure Tilt Motion, Straightness and Position of Precision Linear Stage with a 3D Sinusoidal-Groove Linear Reflective Grating and Triangular Wave-Based Subdivision Method. Sensors 2019, 19, 2816. https://doi.org/10.3390/s19122816
Tsai H-A, Lo Y-L. An Approach to Measure Tilt Motion, Straightness and Position of Precision Linear Stage with a 3D Sinusoidal-Groove Linear Reflective Grating and Triangular Wave-Based Subdivision Method. Sensors. 2019; 19(12):2816. https://doi.org/10.3390/s19122816
Chicago/Turabian StyleTsai, Hsiu-An, and Yu-Lung Lo. 2019. "An Approach to Measure Tilt Motion, Straightness and Position of Precision Linear Stage with a 3D Sinusoidal-Groove Linear Reflective Grating and Triangular Wave-Based Subdivision Method" Sensors 19, no. 12: 2816. https://doi.org/10.3390/s19122816
APA StyleTsai, H.-A., & Lo, Y.-L. (2019). An Approach to Measure Tilt Motion, Straightness and Position of Precision Linear Stage with a 3D Sinusoidal-Groove Linear Reflective Grating and Triangular Wave-Based Subdivision Method. Sensors, 19(12), 2816. https://doi.org/10.3390/s19122816




