Comparison of Metal-Backed Free-Space and Open-Ended Coaxial Probe Techniques for the Dielectric Characterization of Aeronautical Composites †
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials under Test
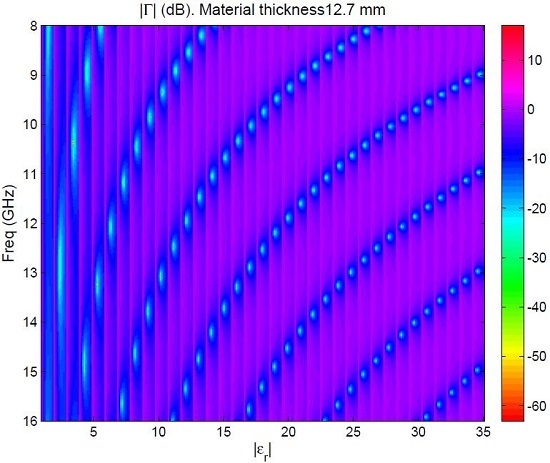
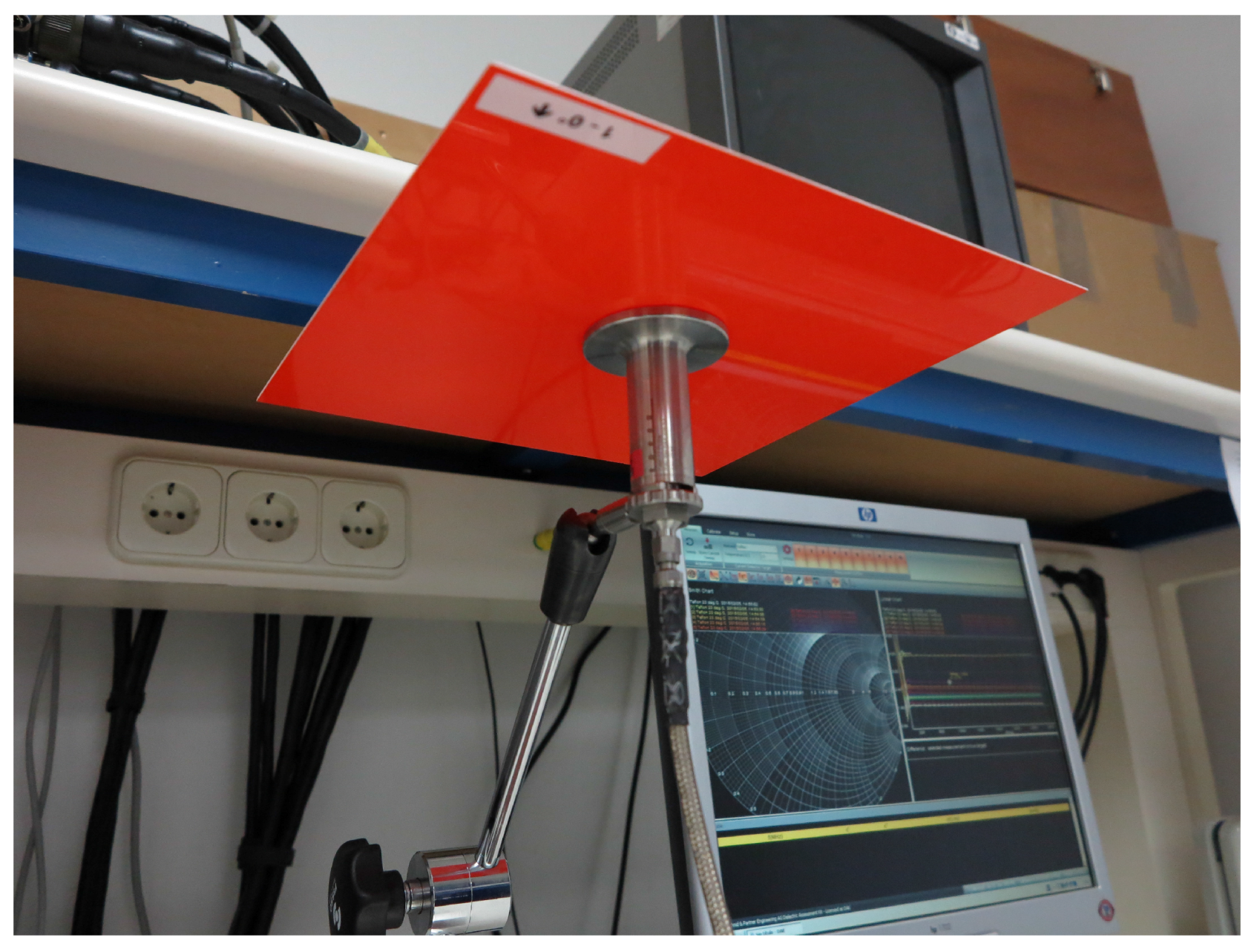
2.2. Metal-Backed Free-Space Methodology
- Initialize a population array of M particles with random positions and velocities in the N-dimensional space.
- For each particle, evaluate the fitness function and update its local and global best solution in the N-dimensional space ( and respectively, being ).
- For each dimension (), update the velocity of each particle according to the relative locations of and following Equation (4)where subindex k denotes an iteration, is the velocity of the particle associated to dimension n and iteration k, the particle location in dimension n for iteration k, and are scaling factors, such that determines the influence of the local best location on the velocity, and determines how much a particle is influenced by the rest; and are random numbers between 0.0 and 1.0, and w is the inertial weight () that is introduced to determine to what extent each particle remains along its original course.
- Update particle location on the N-dimensional space according to Equation (5), where :
- Repeat the process from step 2 until the termination criteria is met.
2.3. Open-Ended Coaxial Probe
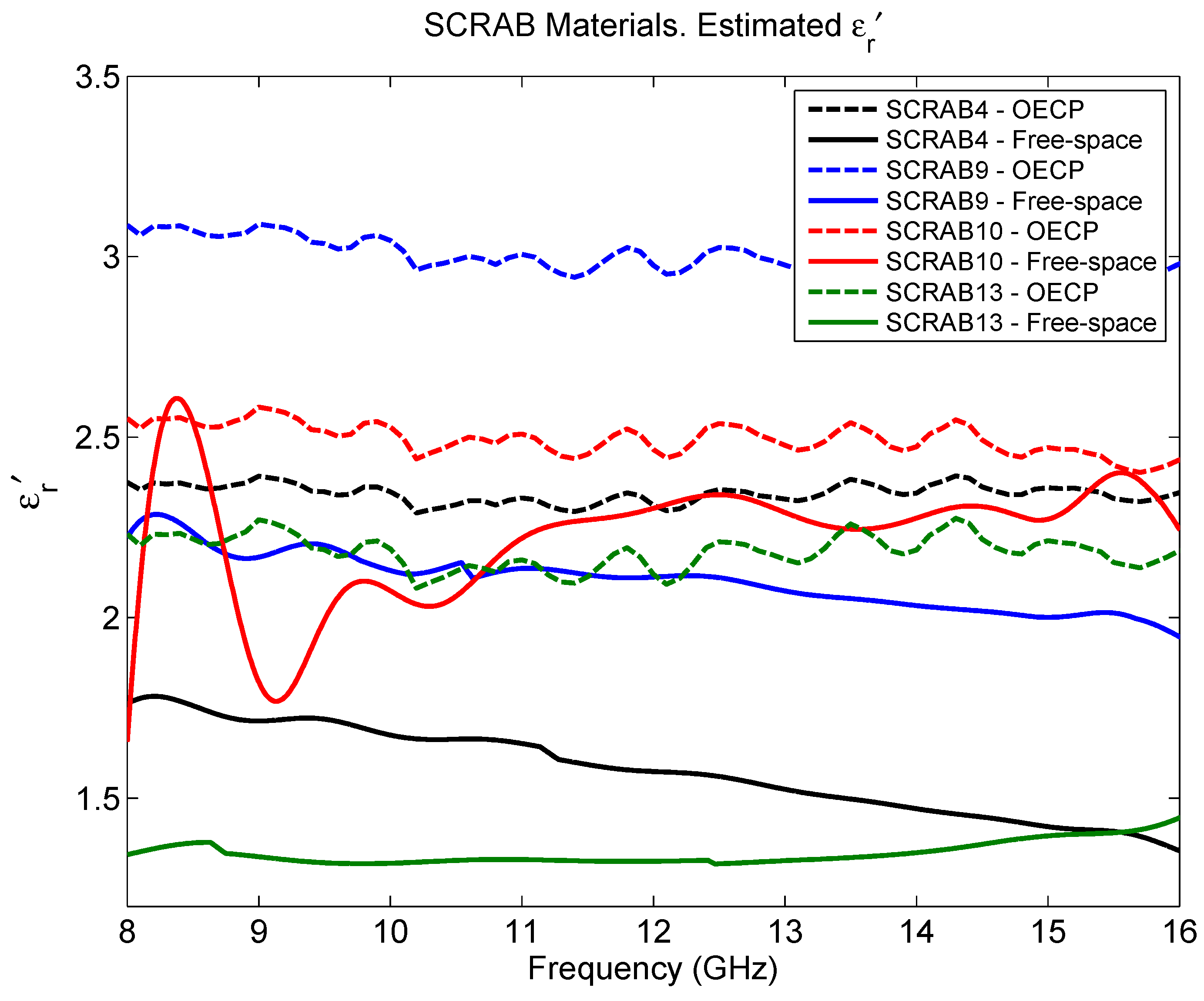
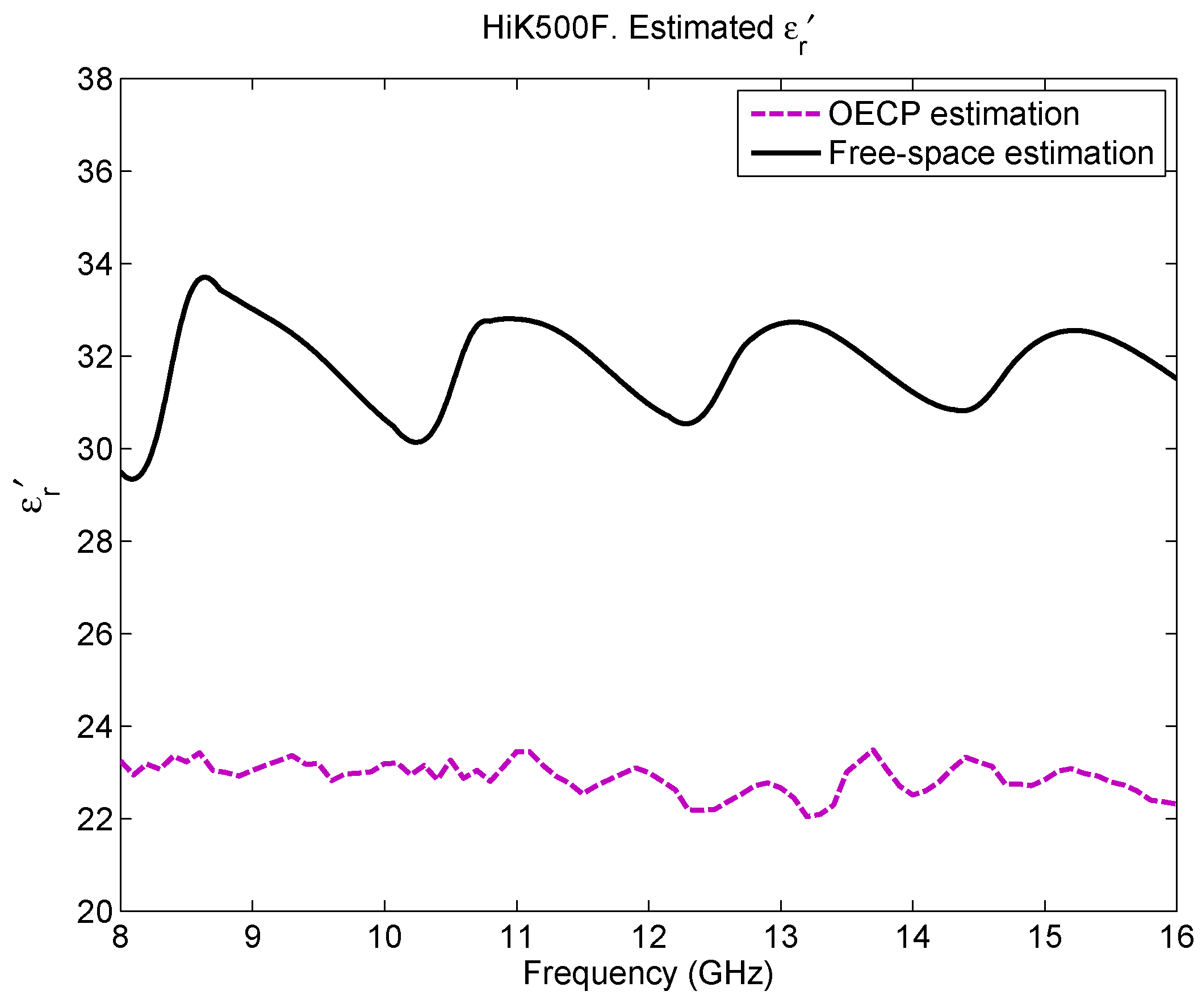
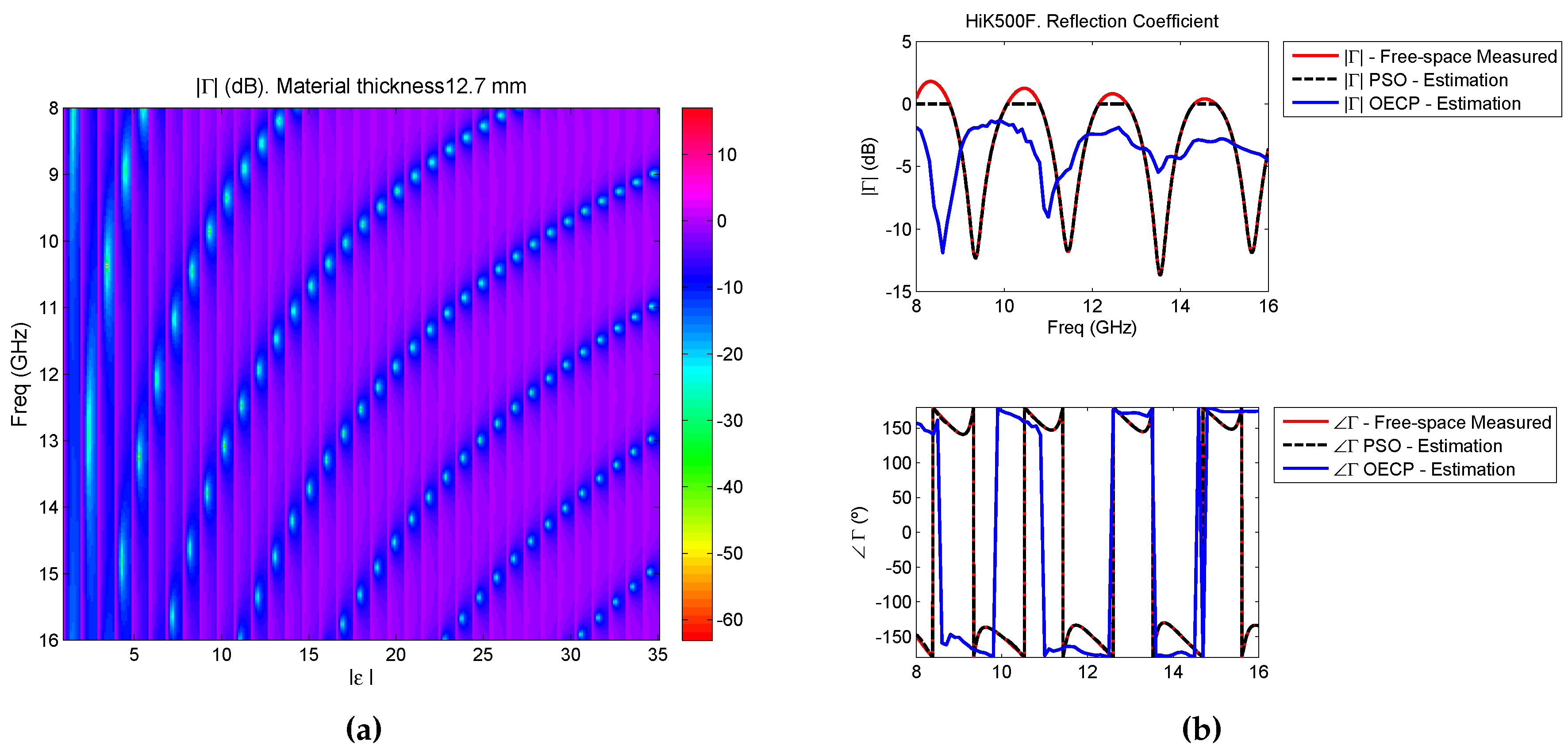
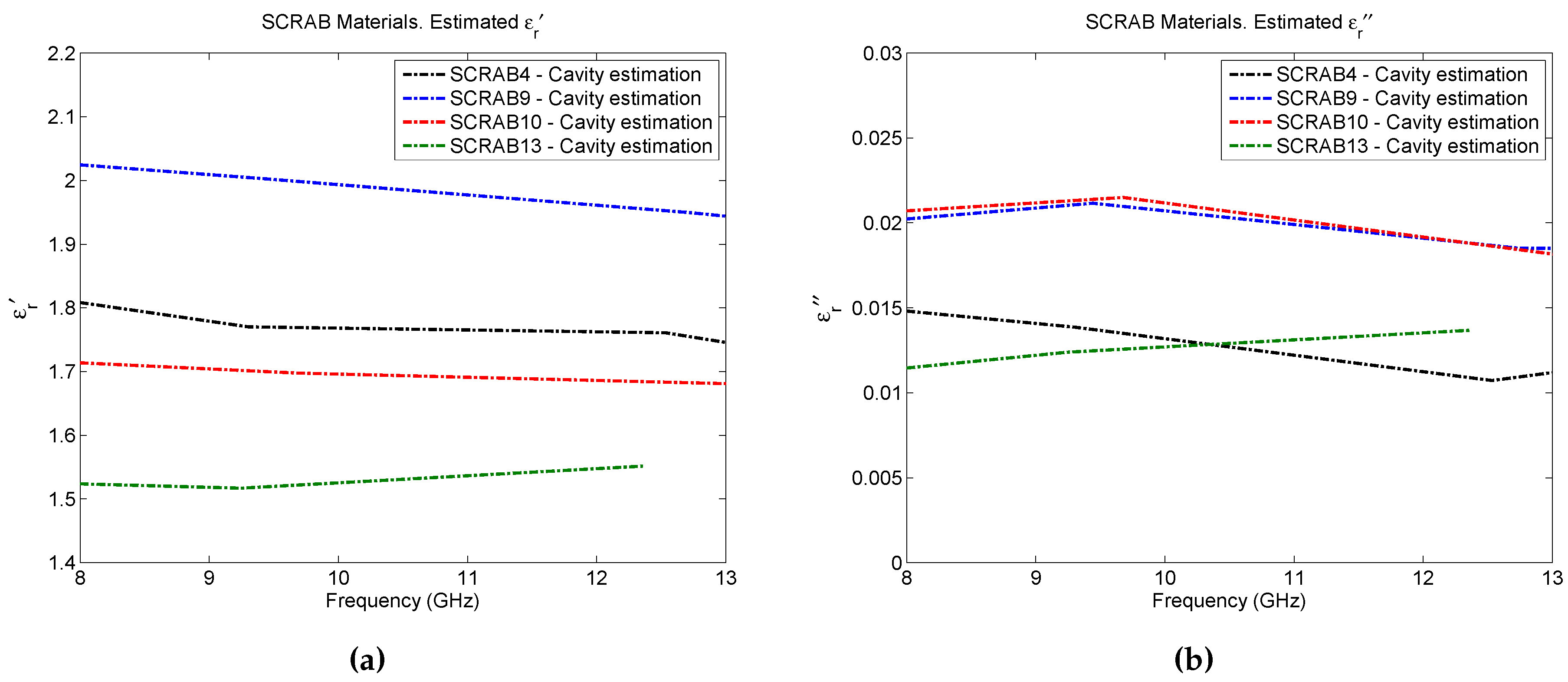
3. Results and Discussion
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Chen, L.F.; Ong, C.K.; Neo, C.P.; Varadan, V.V.; Varadan, V.K. Microwave Electronics: Measurement and Materials Characterization; John Wiley & Sons, Ltd.: West Sussex, England, 2004. [Google Scholar]
- Tereshchenko, O.; Buesink, F.; Leferink, F. Measurement of complex permittivity of composite materials using waveguide method. In Proceedings of the 10th International Symposium on Electromagnetic Compatibility (EMC Europe 2011), York, UK, 26–30 September 2011; pp. 52–56.
- Büyüköztürk, O.; Yu, T.Y.; Ortega, J.A. A methodology for determining complex permittivity of construction materials based on transmission-only coherent, wide-bandwidth free-space measurements. Cem. Concr. Compos. 2006, 28, 349–359. [Google Scholar]
- Baker, A.; Dutton, S.; Kelly, D. Composite Materials for Aircraft Structures, 2nd ed.; American Institute of Aeronautics and Astronautics (AIAA): Virginia, VA, USA, 2004. [Google Scholar]
- Administration, F.A. Aviation Maintenance Technician Handbook—Airframe Volume 1; United States Department of Transportation, Federal Aviation Administration, Airman Testing Standards Branch: Oklahoma City, OK, USA, 2012. [Google Scholar]
- Micheli, D.; Vricella, A.; Pastore, R.; Marchetti, M. Synthesis and electromagnetic characterization of frequency selective radar absorbing materials using carbon nanopowders. Carbon 2014, 77, 756–774. [Google Scholar] [CrossRef]
- EUROCAE. ED-107A-Guide to Certification of Aircraft in a High-Intensity Radiated Field (HIRF) Environment; European Organization for Civil Aviation Equipment (EUROCAE): Malakoff, France, 2010. [Google Scholar]
- Keysigth Technologies. Application Note 5989-2589. Basics of Measuring the Dielectric Properties of Materials; Keysight Technologies: Santa Rosa, CA, USA, 2014. [Google Scholar]
- Jilani, M.T.; Rehman, M.Z.; Khan, A.M.; Khan, M.T.; Ali, S.M. A Brief Review of Measuring Techniques for Characterization of Dielectric Materials. Int. J. Inf. Technol. Electr. Eng. 2012, 1, 1–5. [Google Scholar]
- Schultz, J.W. Focused Beam Methods: Measuring Microwave Materials in Free Space; CreateSpace Independent Publishing Platform: Atlanta, GA, USA, 2012. [Google Scholar]
- Baker-Jarvis, J.; Janezic, M.D.; Degroot, D.C. High-frequency dielectric measurements. IEEE Instrum. Meas. Mag. 2010, 13, 24–31. [Google Scholar] [CrossRef]
- Sistemas de Control Remoto S.L. Available online: http://scrtargets.es (accessed on 27 March 2016).
- Von Hippel, A. Dielectric Materials and Applications; Artech House: Norwood, MA, USA, 1995. [Google Scholar]
- Ghodgaonkar, D.; Varadan, V.; Varadan, V.K. A free-space method for measurement of dielectric constants and loss tangents at microwave frequencies. IEEE Trans. Instrum. Meas. 1989, 38, 789–793. [Google Scholar] [CrossRef]
- Escot, D.; Poyatos, D.; Aguilar, J.; Montiel, I.; González, I.; de Adana, F. Indoor 3D Full Polarimetric Bistatic Spherical Facility for Electromagnetic Tests. IEEE Antennas Propag. Mag. 2010, 52, 112–118. [Google Scholar] [CrossRef]
- Awang, Z.; Zaki, F.A.M.; Baba, N.H.; Zoolfakar, A.S.; Bakar, R.A. A free-space method for complex permittivity measurement of bulk and thin film dielectrics at microwave frequencies. Prog. Electromagn. Res. B 2013, 51, 307–328. [Google Scholar] [CrossRef]
- Aris, M.A.B.; Kadir, E.A.; Ghodgaonkar, D.K.; Khadri, N. Comparison of Reflection and Transmission Method and Metal Back Method Measurement of Dielectric properties of transformer oil using free space microwave measurement system in 8–12 GHz frequency range. In Proceedings of the APMC 2009 Asia Pacific Microwave Conference, Singapore, 7–10 December 2009; pp. 1609–1612.
- Dunsmore, J. Gating effects in time domain transforms. In Proceedings of the 72nd ARFTG Microwave Measurement Symposium, Portland, OR, USA, 9–12 December 2008; pp. 1–8.
- Kennedy, J.; Eberhart, R. Particle swarm optimization. In Proceedings of the IEEE International Conference on Neural Networks, Perth, Austrilia, 27 November–1 December 1995; Volume 4, pp. 1942–1948.
- Eberhart; Shi, Y. Particle swarm optimization: Developments, applications and resources. In Proceedings of the 2001 Congress on Evolutionary Computation, Seoul, Korea, 27–30 May 2001; Volume 1, pp. 81–86.
- SPEAG, Schmid & Partner Engineering AG. Available online: http://www.speag.com/products/dak/dielectric-measurements/ (accessed on 1 November 2015).
- Emerson & Cuming Microwave Products. Available online: http://www.eccosorb.com/products-eccostock-hik500f.htm (accessed on 31 March 2016).
- Material Measurement Solutions, Damaskos, Inc. PO Box 469, Concordville, PA. Available online: http://www.damaskosinc.com/cavitiy.htm (accessed on 28 April 2016).
- Knott, P.; Perkuhn, R. Non-destructive permittivity measurement of thin dielectric sheets. Quality conformance testing for the Tracking and Imaging Radar TIRA. In Proceedings of the 2015 German Microwave Conference, Nuremberg, Germany, 16–18 Mar 2015; pp. 25–28.




| Material Name | Height (mm) | Length (mm) | Thickness (mm) |
|---|---|---|---|
| SCRAB 4 | 200 | 200 | 6.3 |
| SCRAB 9 | 200 | 200 | 4 |
| SCRAB 10 | 200 | 200 | 2.3 |
| SCRAB 13 | 200 | 200 | 9 |
| Teflon | 200 | 200 | 10 |
| HiK500F | 200 | 200 | 12.7 |
| Material | Thickness (mm) | OECP- | OECP- | Free-Space- | Free-Space- |
|---|---|---|---|---|---|
| SCRAB 4 | 6.3 | 2.34 | 3.4 | 1.58 | 3.6 |
| SCRAB 9 | 4 | 3.01 | 3.9 | 2.10 | 9.4 |
| SCRAB 10 | 2.3 | 2.49 | 10.6 | 2.23 | 2.0 |
| SCRAB 13 | 9 | 2.18 | 6.1 | 1.35 | 3.0 |
| Material | Thickness (mm) | OECP- | OECP- | Free-Space- | Free-Space- |
|---|---|---|---|---|---|
| Eccostock HiK500F | 12.7 | 22.89 | 2.0 | 31.83 | 5.0 |
| Material | OECP- | OECP- | Free-Space- | Free-Space- | Cavity- | Cavity- |
|---|---|---|---|---|---|---|
| SCRAB 4 | 2.34 | 4.7 | 1.66 | 5.7 | 1.77 | 1.3 |
| SCRAB 9 | 3.01 | 5.7 | 2.15 | 9.6 | 1.99 | 2.0 |
| SCRAB 10 | 2.51 | 10.6 | 2.18 | 7.1 | 1.70 | 2.0 |
| SCRAB 13 | 2.18 | 7.6 | 1.33 | 2.5 | 1.53 | 1.3 |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
López-Rodríguez, P.; Escot-Bocanegra, D.; Poyatos-Martínez, D.; Weinmann, F. Comparison of Metal-Backed Free-Space and Open-Ended Coaxial Probe Techniques for the Dielectric Characterization of Aeronautical Composites. Sensors 2016, 16, 967. https://doi.org/10.3390/s16070967
López-Rodríguez P, Escot-Bocanegra D, Poyatos-Martínez D, Weinmann F. Comparison of Metal-Backed Free-Space and Open-Ended Coaxial Probe Techniques for the Dielectric Characterization of Aeronautical Composites. Sensors. 2016; 16(7):967. https://doi.org/10.3390/s16070967
Chicago/Turabian StyleLópez-Rodríguez, Patricia, David Escot-Bocanegra, David Poyatos-Martínez, and Frank Weinmann. 2016. "Comparison of Metal-Backed Free-Space and Open-Ended Coaxial Probe Techniques for the Dielectric Characterization of Aeronautical Composites" Sensors 16, no. 7: 967. https://doi.org/10.3390/s16070967
APA StyleLópez-Rodríguez, P., Escot-Bocanegra, D., Poyatos-Martínez, D., & Weinmann, F. (2016). Comparison of Metal-Backed Free-Space and Open-Ended Coaxial Probe Techniques for the Dielectric Characterization of Aeronautical Composites. Sensors, 16(7), 967. https://doi.org/10.3390/s16070967