Portable Wind Energy Harvesters for Low-Power Applications: A Survey
Abstract
:1. Introduction
2. Agile Wind Energy Harvesting Techniques
- (1)
- Collect the mechanical stresses from ambient sources, which are applied on the mechanical active part of the harvester;
- (2)
- The kinetic energy offered by wind as vibration sources is converted to electrical energy;
- (3)
- Process the generated power to make it as DC voltage and store it in a super-capacitor cell.
2.1. Piezoelectric-Based Generators
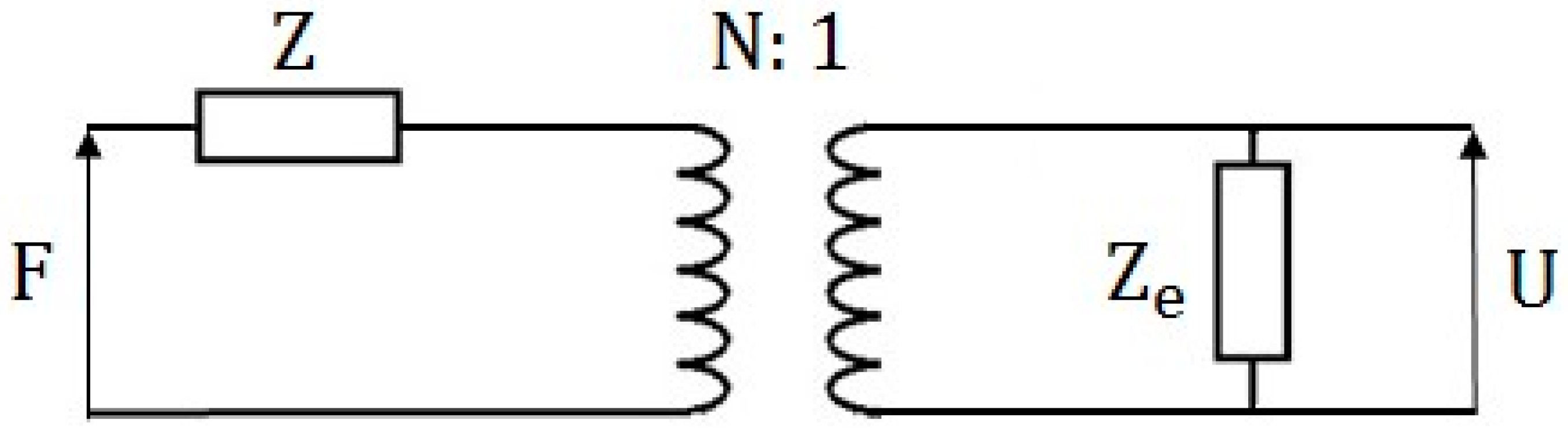
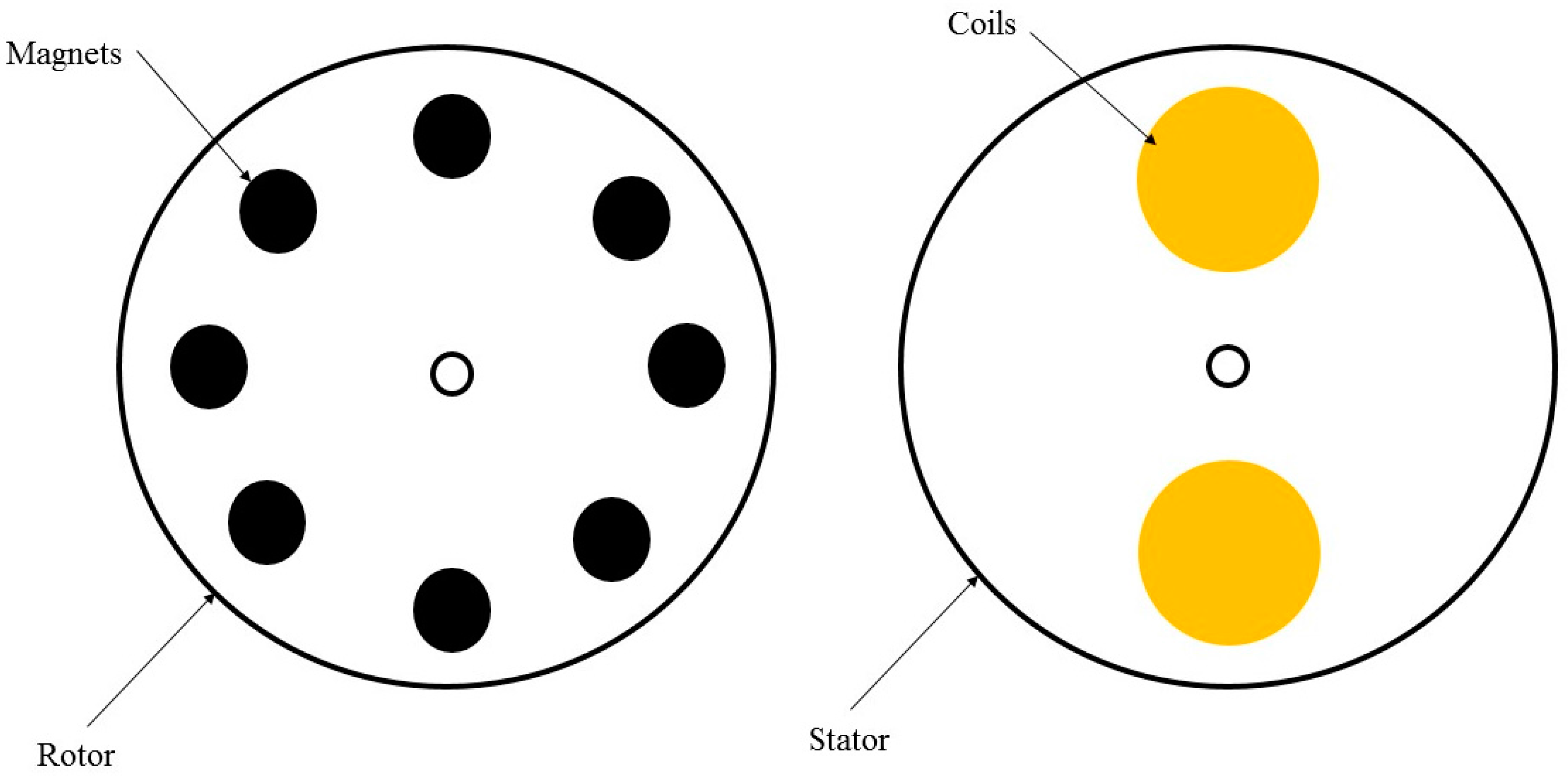
2.2. Electromagnetic-Based Generators
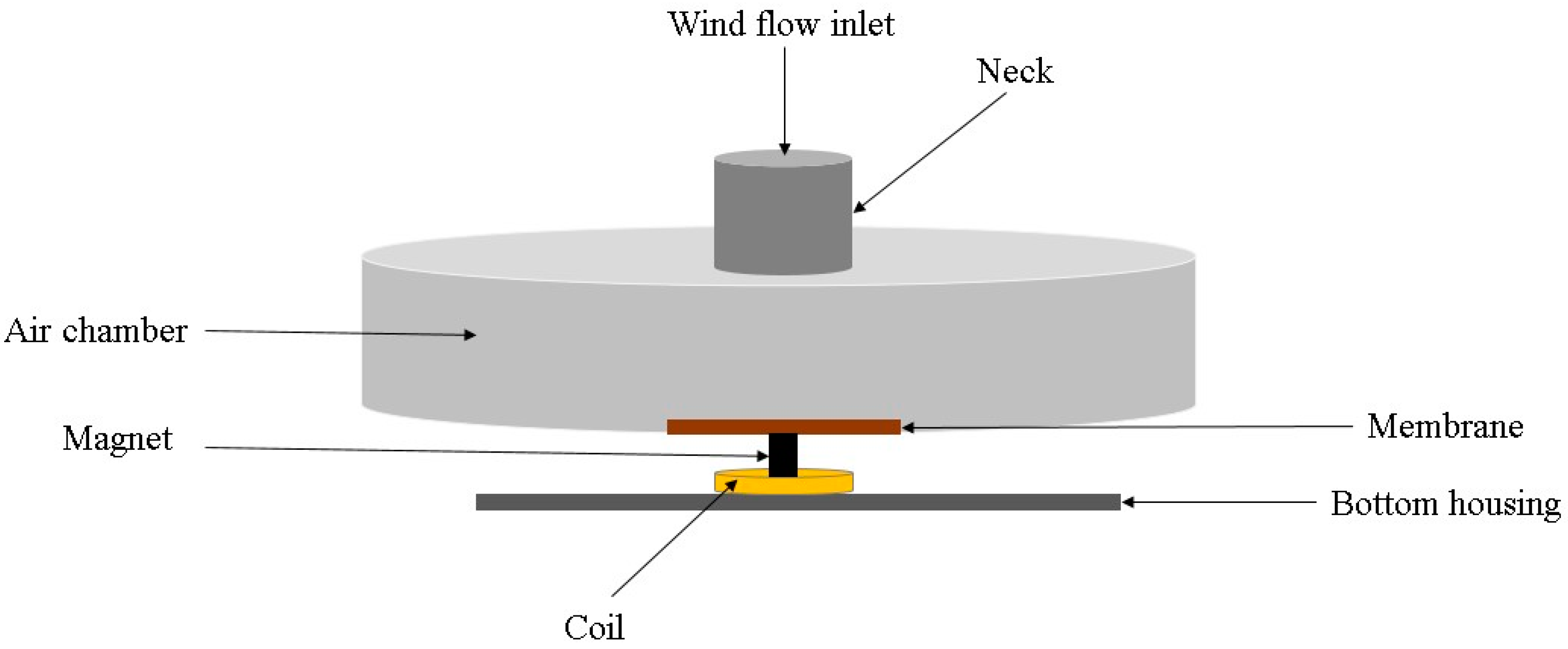
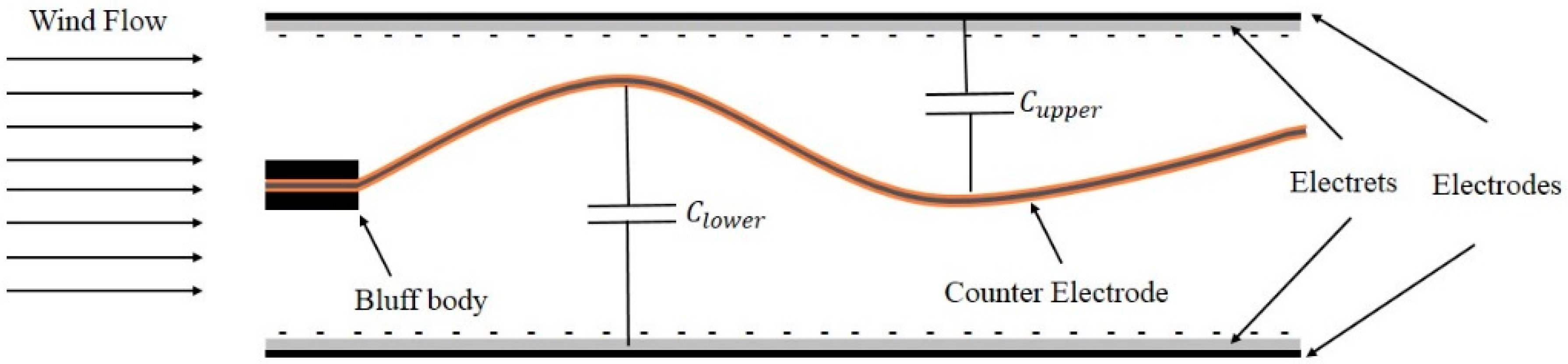
2.3. Electrostatic-Based Generators
3. Portable Wind Energy Harvesting Mechanical Mechanisms
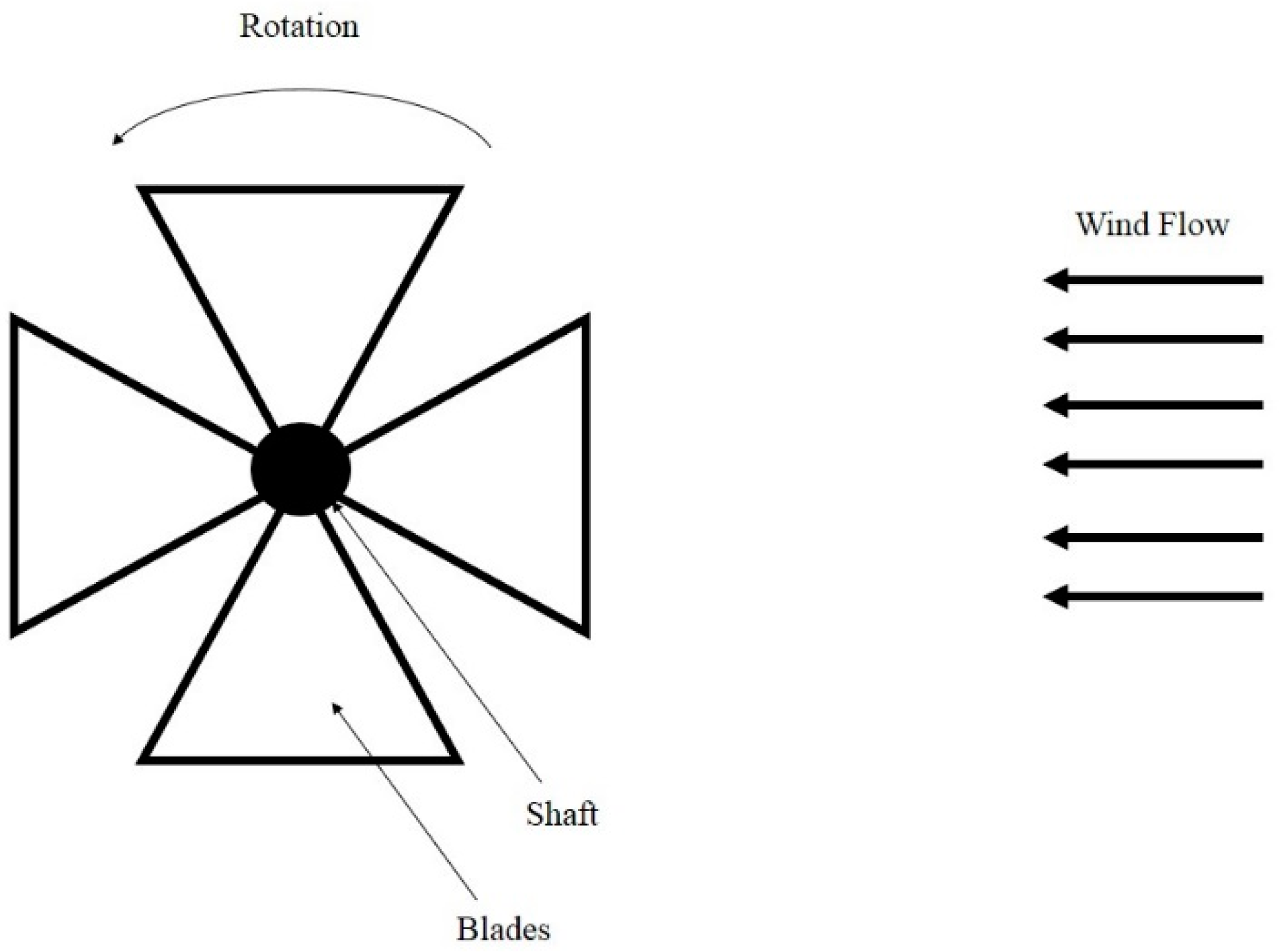
3.1. Rotational Harvesters
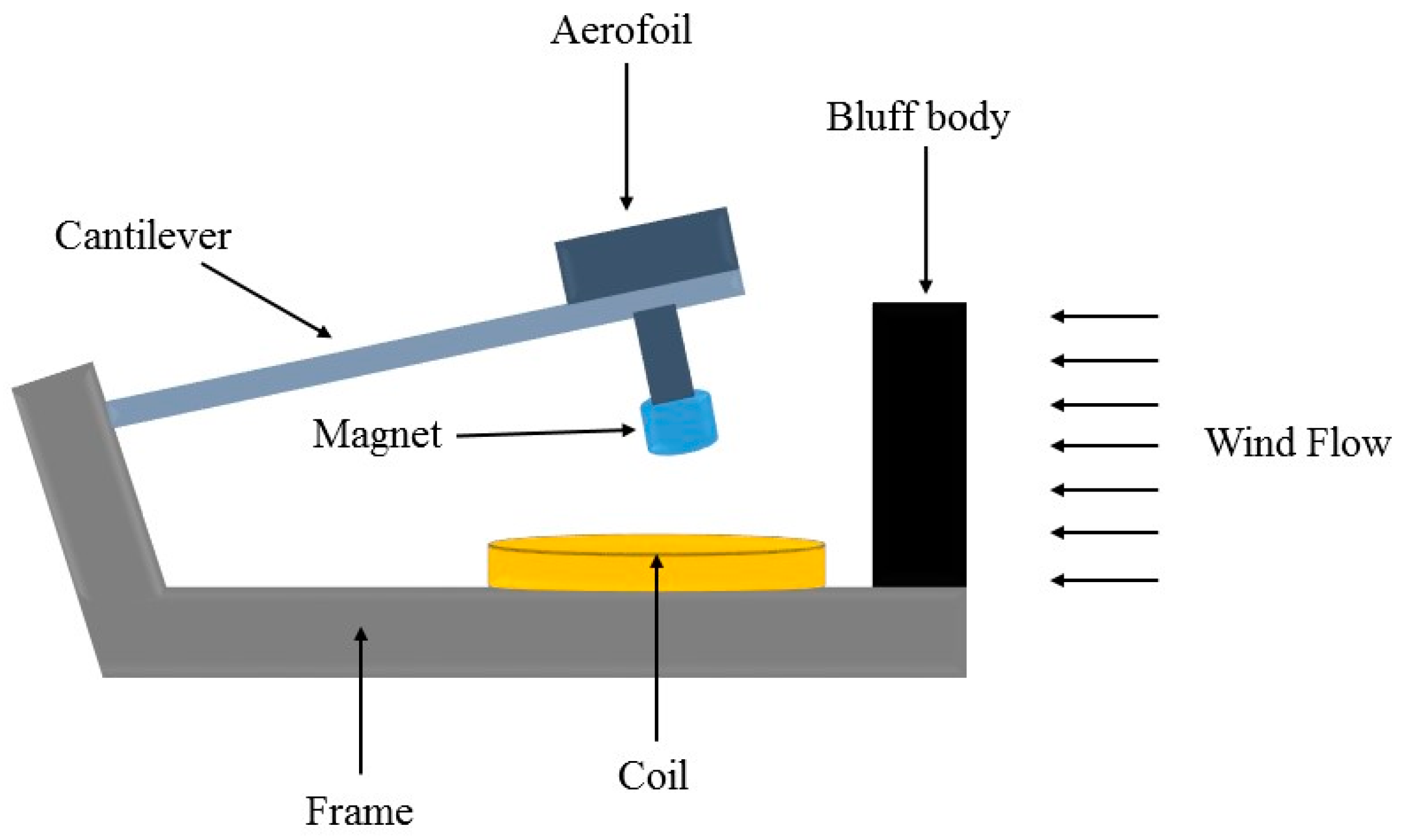
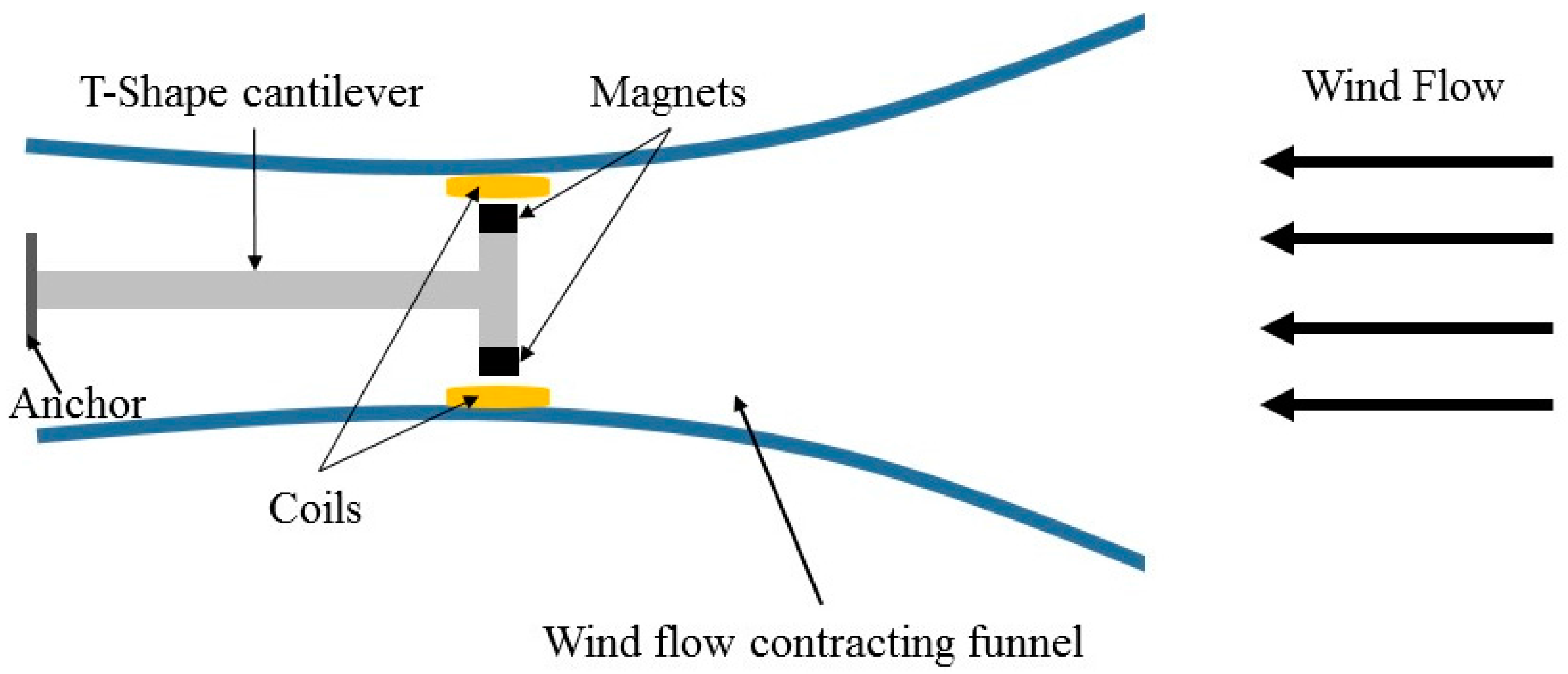
3.2. Aeroelastic Harvesters
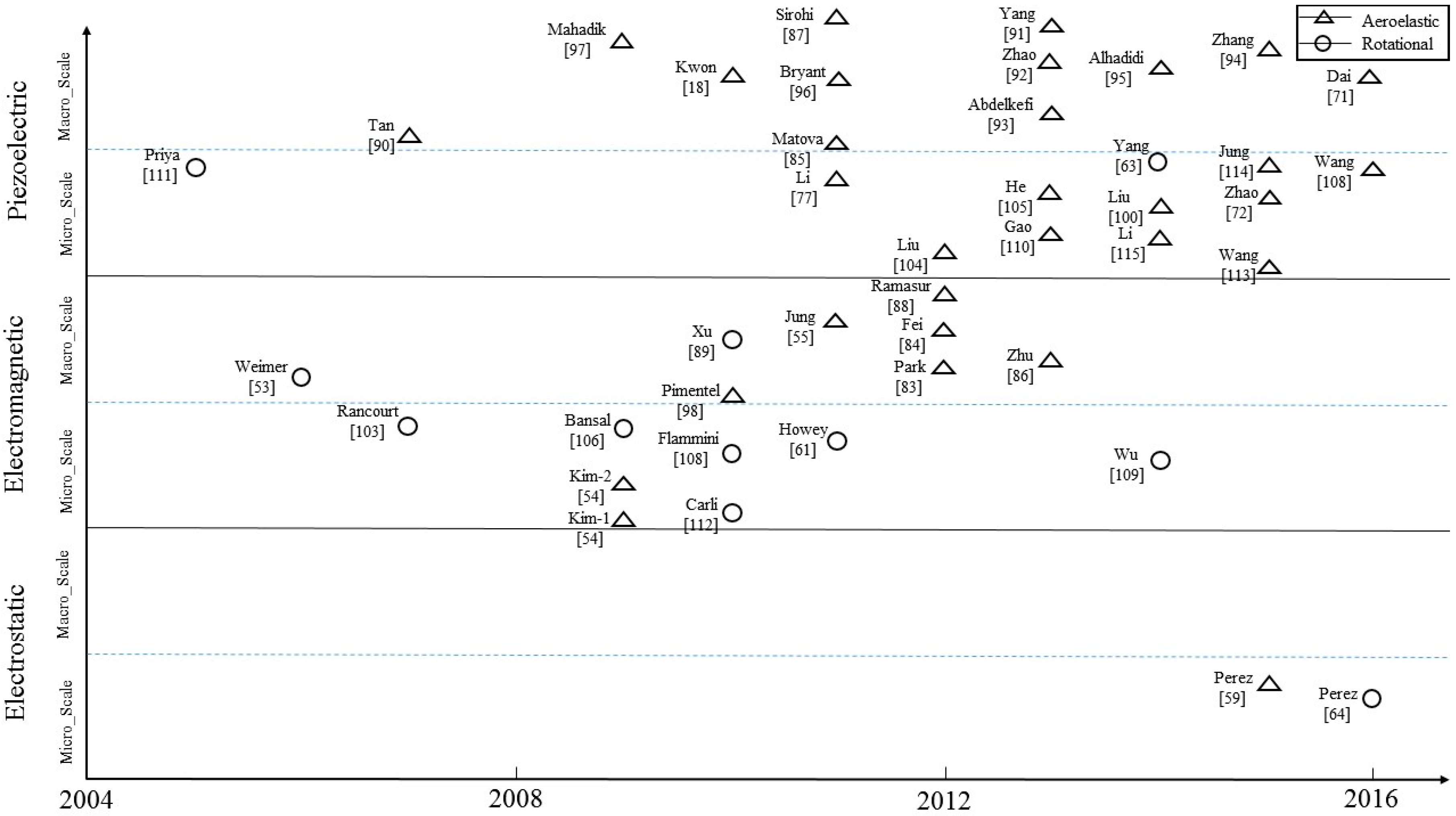
4. Dimension of Portable Wind Energy Harvesters
4.1. Macro-Scale Harvesters
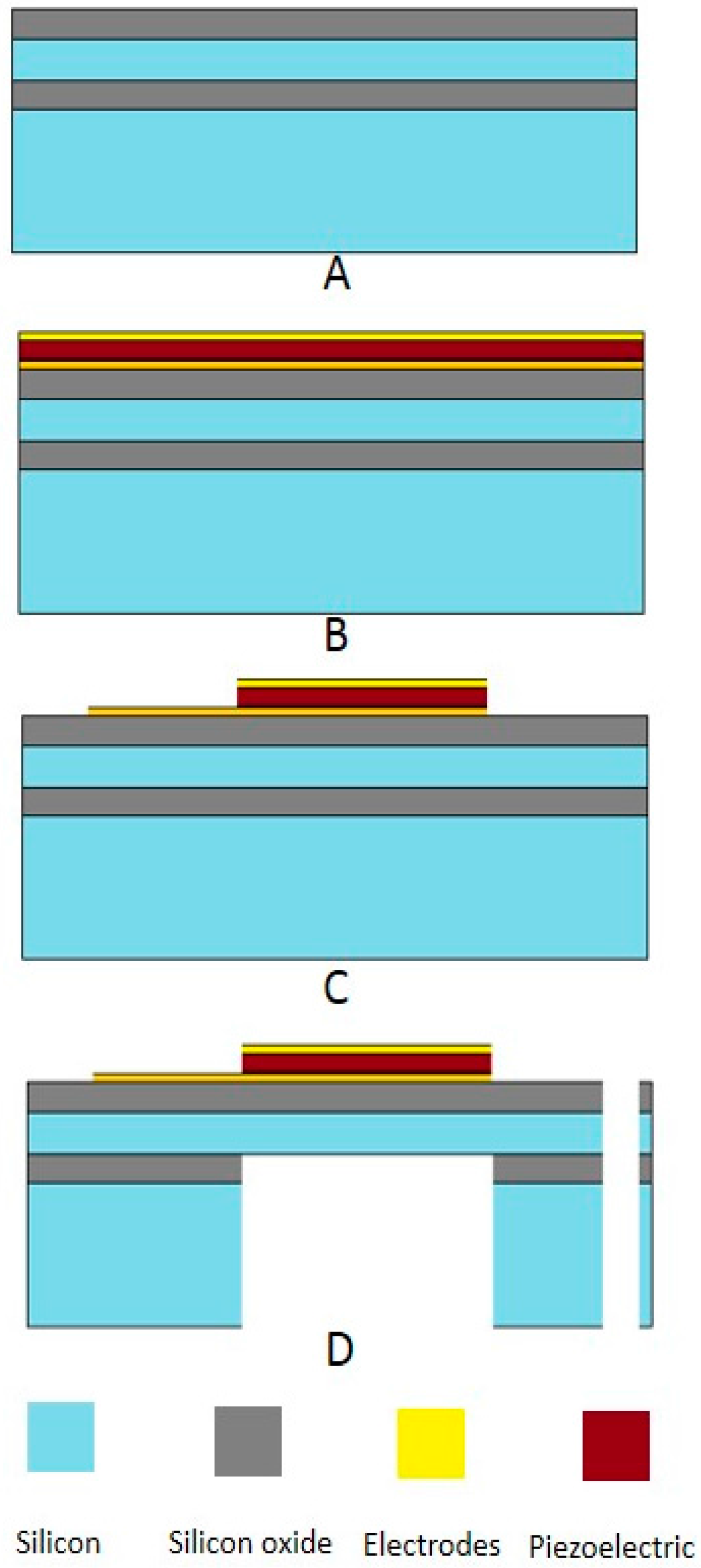
4.2. Micro-Scale Harvesters
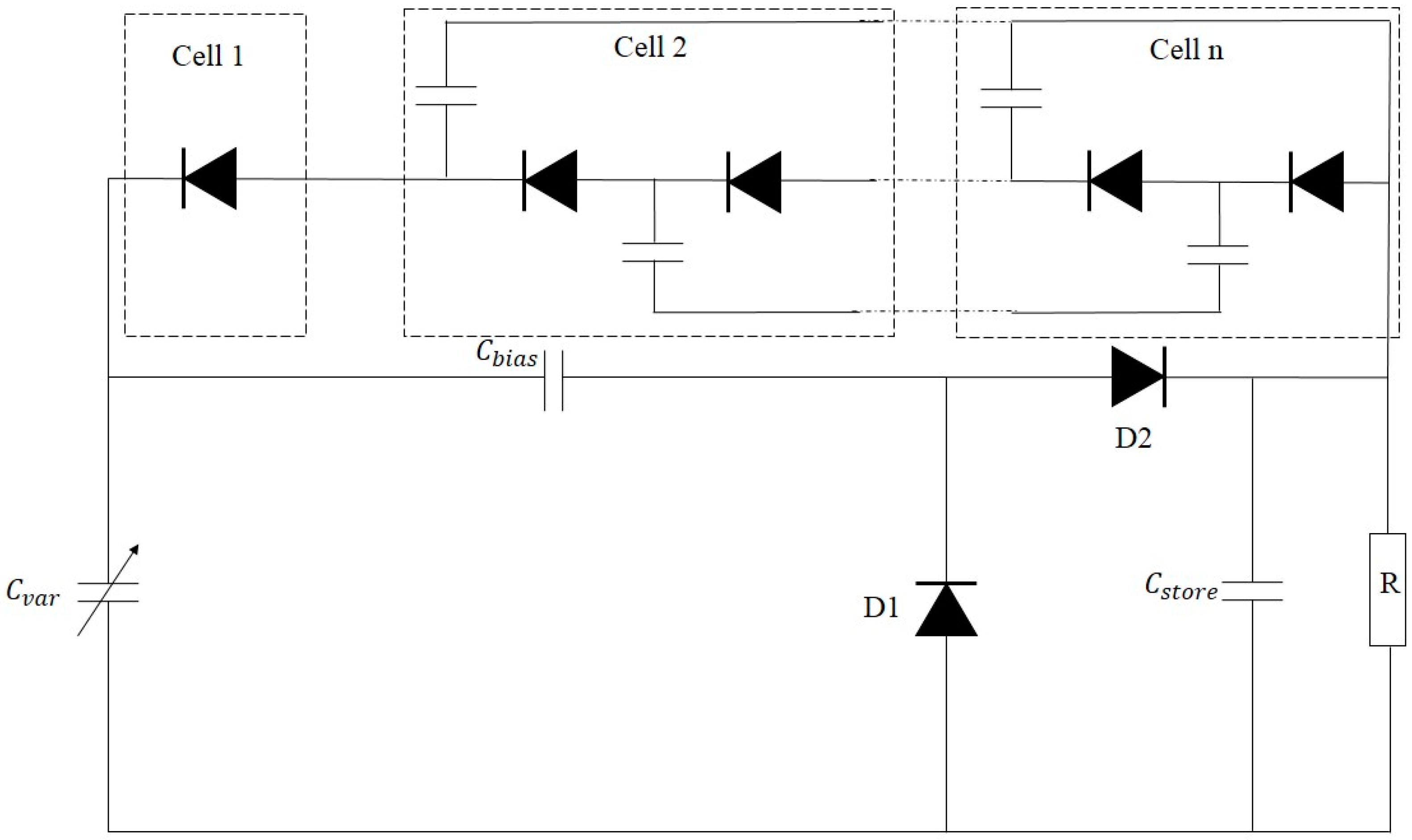
5. Power Management Systems
6. Conclusions and Discussions
Acknowledgments
Conflicts of Interest
References
- Rasouli, M.; Phee, L.S.J. Energy sources and their development for application in medical devices. Expert Rev. Med. Devices 2010, 7, 693–709. [Google Scholar] [CrossRef] [PubMed]
- Nabavi, S.; Yaralioglu, G. High Quality Factor Resonant Mass Sensors Based on CMUT for Immersion in Liquid. In Proceedings of the 14th international workshop on Micromachined Ultrasonic Transducers (MUT), Deresden, Germany, 19–20 May 2015.
- Mitcheson, P.D.; Yeatman, E.M.; Rao, G.K.; Holmes, A.S.; Green, T.C. Energy harvesting from human and machine motion for wireless electronic devices. IEEE Proc. 2008, 96, 1457–1486. [Google Scholar] [CrossRef]
- Anton, S.R.; Inman, D.J. Vibration energy harvesting for unmanned aerial vehicles. In Proceedings of the 15th International Symposium on Smart Structures and Materials & Nondestructive Evaluation and Health Monitoring, San Diego, CA, USA, 9 March 2008.
- Beeby, S.P.; Tudor, M.J.; White, N.M. Energy harvesting vibration sources for microsystems applications. Meas. Sci. Technol. 2006, 17, R175. [Google Scholar] [CrossRef]
- Enz, C.C.; El-Hoiydi, A.; Decotignie, J.-D.; Peiris, V. WiseNET: An ultralow-power wireless sensor network solution. Computer (Long. Beach. Calif.) 2004, 37, 62–70. [Google Scholar] [CrossRef]
- Kovacs, G.T.A.; Maluf, N.I.; Petersen, K.E. Bulk micromachining of silicon. IEEE Proc. 1998, 86, 1536–1551. [Google Scholar] [CrossRef]
- Bustillo, J.M.; Howe, R.T.; Muller, R.S. Surface micromachining for microelectromechanical systems. IEEE Proc. 1998, 86, 1552–1574. [Google Scholar] [CrossRef]
- Becker, E.; Ehrfeld, W.; Hagmann, P.; Maner, A.; Münchmeyer, D. Fabrication of microstructures with high aspect ratios and great structural heights by synchrotron radiation lithography, galvanoforming, and plastic moulding (LIGA process). Microelectron. Eng. 1986, 4, 35–56. [Google Scholar] [CrossRef]
- Zhang, L.; Jangkrajarng, N.; Bhattacharya, S.; Shi, C.J.R. Parasitic-aware optimization and retargeting of analog layouts: A symbolic-template approach. IEEE Trans. Comput. Aided Des. Integr. Circuits Syst. 2008, 27, 791–802. [Google Scholar] [CrossRef]
- Dong, X.; Zhang, L. Lithography-Aware Analog Layout Retargeting. IEEE Trans. Comput. Aided Des. Integr. Circuits Syst. 2016, 35, 232–245. [Google Scholar] [CrossRef]
- Ho, C.-M.; Tai, Y.-C. Micro-electro-mechanical-systems (MEMS) and fluid flows. Annu. Rev. Fluid Mech. 1998, 30, 579–612. [Google Scholar] [CrossRef]
- Miles, R.W.; Hynes, K.M.; Forbes, I. Photovoltaic solar cells: An overview of state-of-the-art cell development and environmental issues. Prog. Cryst. Growth Charact. Mater. 2005, 51, 1–42. [Google Scholar] [CrossRef]
- Simon, J.; Caroff, T. Device for Generating Current and/or Voltage Based on a Thermoelectric Module Placed in a Flowing Fluid. U.S. Patent US9099942 B2, 4 August 2015. [Google Scholar]
- Chen, J.; Yan, Z.; Wu, L. The influence of Thomson effect on the maximum power output and maximum efficiency of a thermoelectric generator. J. Appl. Phys. 1996, 79, 8823–8828. [Google Scholar] [CrossRef]
- Reilly, E.K.; Miller, L.M.; Fain, R.; Wright, P. A study of ambient vibrations for piezoelectric energy conversion. In Proceedings of the PowerMEMS 2009, Washington DC, USA, 1–4 December 2009; pp. 312–315.
- Ackermann, T.; Söder, L. An overview of wind energy-status 2002. Renew. Sustain. Energy Rev. 2002, 6, 67–127. [Google Scholar] [CrossRef]
- Kwon, S.-D. A T-shaped piezoelectric cantilever for fluid energy harvesting. Appl. Phys. Lett. 2010, 97, 164102. [Google Scholar] [CrossRef]
- Xiao, Q.; Zhu, Q. A review on flow energy harvesters based on flapping foils. J. Fluids Struct. 2014, 46, 174–191. [Google Scholar] [CrossRef]
- Xie, X.D.; Wang, Q.; Wu, N. Energy harvesting from transverse ocean waves by a piezoelectric plate. Int. J. Eng. Sci. 2014, 81, 41–48. [Google Scholar] [CrossRef]
- Wu, N.; Wang, Q.; Xie, X. Ocean wave energy harvesting with a piezoelectric coupled buoy structure. Appl. Ocean Res. 2015, 50, 110–118. [Google Scholar] [CrossRef]
- Baranov, A.; Spirjakin, D.; Akbari, S.; Somov, A. Optimization of power consumption for gas sensor nodes: A survey. Sens. Actuators A Phys. 2015, 233, 279–289. [Google Scholar] [CrossRef]
- Al Ahmad, M. Piezoelectric water drop energy harvesting. J. Electron. Mater. 2014, 43, 452–458. [Google Scholar] [CrossRef]
- Roundy, S.; Wright, P.K. A piezoelectric vibration based generator for wireless electronics. Smart Mater. Struct. 2004, 13, 1131. [Google Scholar] [CrossRef]
- Jha, A.R. Wind Turbine Technology; CRC Press: Boca Raton, FL, USA, 2010. [Google Scholar]
- Holmes, A. Energy Harvesting from Fluid Flows. In Micro Energy Harvesting; Briand, D., Yeatman, E., Roundy, S., Troccaz, J., Eds.; Wiley: Weinheim, Germany, 2015. [Google Scholar]
- Carroll, C.B. Energy Harvesting EEL. U.S. Patent US6424079 B1, 23 July 2002. [Google Scholar]
- Betz, A.; From, D.N. Windmills in the Light of Modern Research. Naturwissenschaften 1927, 15, 905–914. [Google Scholar] [CrossRef]
- Xu, R.; Kim, S.G. Figures of merits of piezoelectric materials in energy harvesters. In Proceedings of the PowerMEMS 2012, Atlanta, GA, USA, 2–5 December 2012.
- Aktakka, E.E.; Peterson, R.L.; Najafi, K. Thinned-PZT on SOI process and design optimization for piezoelectric inertial energy harvesting. In Proceedings of the 18th International Conference on Solid-State Sensors, Actuators and Microsystems (TRANSDUCERS), Anchorage, AK, USA, 21–25 June 2011; pp. 1649–1652.
- Yang, B.; Lee, C.; Xiang, W.; Xie, J.; He, J.H.; Kotlanka, R.K.; Low, S.P.; Feng, H. Electromagnetic energy harvesting from vibrations of multiple frequencies. J. Micromech. Microeng. 2009, 19, 35001. [Google Scholar] [CrossRef]
- Meninger, S.; Mur-Miranda, J.O.; Amirtharajah, R.; Chandrakasan, A.P.; Lang, J.H. Vibration-to-electric energy conversion. IEEE Trans. Very Large Scale Integr. Syst. 2001, 9, 64–76. [Google Scholar] [CrossRef]
- Shomalnasab, G.; Zhang, L. New Analytic Model of Coupling and Substrate Capacitance in Nanometer Technologies. IEEE Trans. Very Large Scale Integr. Syst. 2015, 23, 1268–1280. [Google Scholar] [CrossRef]
- Zhu, Y.; Moheimani, S.O.R.; Yuce, M.R. A 2-DOF MEMS ultrasonic energy harvester. IEEE Sens. J. 2011, 11, 155–161. [Google Scholar]
- Erturk, A.; Inman, D.J. Piezoelectric Energy Harvesting; John Wiley & Sons: West Sussex, UK, 2011. [Google Scholar]
- Spreemann, D.; Manoli, Y. Electromagnetic Vibration Energy Harvesting Devices: Architectures, Design, Modeling and Optimization; Springer Science & Business Media: New York, NY, USA, 2012; Volume 35. [Google Scholar]
- Arroyo, E.; Badel, A.; Formosa, F.; Wu, Y.; Qiu, J. Comparison of electromagnetic and piezoelectric vibration energy harvesters: Model and experiments. Sens. Actuators A Phys. 2012, 183, 148–156. [Google Scholar] [CrossRef]
- Basset, P.; Blokhina, E.; Galayko, D. Electrostatic Kinetic Energy Harvesting; John Wiley & Sons: Hoboken, NJ, USA, 2016. [Google Scholar]
- Elliott, A.D.T.; Miller, L.M.; Halvorsen, E.; Wright, P.K.; Mitcheson, P.D. Which is better, electrostatic or piezoelectric energy harvesting systems? J. Phys. Conf. Ser. 2015, 660, 012128. [Google Scholar] [CrossRef]
- Sulchek, T.; Grow, R.J.; Yaralioglu, G.; Minne, S.C.; Quate, C.F.; Manalis, S.R.; Kiraz, A.; Aydine, A.; Atalar, A. Parallel atomic force microscopy with optical interferometric detection. Appl. Phys. Lett. 2001, 78, 1787–1789. [Google Scholar] [CrossRef]
- Ajitsaria, J.; Choe, S.-Y.; Shen, D.; Kim, D.J. Modeling and analysis of a bimorph piezoelectric cantilever beam for voltage generation. Smart Mater. Struct. 2007, 16, 447. [Google Scholar] [CrossRef]
- Yaralioglu, G. Ultrasonic heating and temperature measurement in microfluidic channels. Sens. Actuators A Phys. 2011, 170, 1–7. [Google Scholar] [CrossRef]
- Levinshtein, M.E.; Rumyantsev, S.L.; Shur, M.S. Properties of Advanced Semiconductor Materials: GaN, AIN, InN, BN, SiC, SiGe; John Wiley & Sons: New York, NY, USA, 2001. [Google Scholar]
- Lee, B.S.; Lin, S.C.; Wu, W.J.; Wang, X.Y.; Chang, P.Z.; Lee, C.K. Piezoelectric MEMS generators fabricated with an aerosol deposition PZT thin film. J. Micromech. Microeng. 2009, 19, 65014. [Google Scholar] [CrossRef]
- Bardaweel, H.; Al Hattamleh, O.; Richards, R.; Bahr, D.; Richards, C. A comparison of piezoelectric materials for MEMS power generation. In Proceedings of the 6th International Workshop on Micro and Nanotechnology for Power Generation and Energy Conversion Applications, Berkeley, CA, USA, 29 November–1 December 2006.
- Renaud, M.; Fiorini, P.; van Schaijk, R.; Van Hoof, C. Harvesting energy from the motion of human limbs: The design and analysis of an impact-based piezoelectric generator. Smart Mater. Struct. 2009, 18, 35001. [Google Scholar] [CrossRef]
- Poulin, G.; Sarraute, E.; Costa, F. Generation of electrical energy for portable devices: Comparative study of an electromagnetic and a piezoelectric system. Sens. Actuators A Phys. 2004, 116, 461–471. [Google Scholar] [CrossRef]
- Schubel, P.J.; Crossley, R.J. Wind turbine blade design. Energies 2012, 5, 3425–3449. [Google Scholar] [CrossRef]
- Xu, R.; Lei, A.; Dahl-Petersen, C.; Hansen, K.; Guizzetti, M.; Birkelund, K.; Thomsen, E.V.; Hansen, O. Screen printed PZT/PZT thick film bimorph MEMS cantilever device for vibration energy harvesting. Sens. Actuators A Phys. 2012, 188, 383–388. [Google Scholar] [CrossRef]
- Wen, Z.; Deng, L.; Zhao, X.; Shang, Z.; Yuan, C.; She, Y. Improving voltage output with PZT beam array for MEMS-based vibration energy harvester: Theory and experiment. Microsyst. Technol. 2015, 21, 331–339. [Google Scholar] [CrossRef]
- Norhisam, M.; Norrimah, A.; Wagiran, R.; Sidek, R.M.; Mariun, N.; Wakiwaka, H. Consideration of theoretical equation for output voltage of linear displacement sensor using meander coil and pattern guide. Sens. Actuators A Phys. 2008, 147, 470–473. [Google Scholar] [CrossRef]
- Cheng, S.; Wang, N.; Arnold, D.P. Modeling of magnetic vibrational energy harvesters using equivalent circuit representations. J. Micromech. Microeng. 2007, 17, 2328. [Google Scholar] [CrossRef]
- Weimer, M.A.; Zane, R. Remote area wind energy harvesting for low-power autonomous sensors. In Proceedings of the 37th IEEE Power Electronics Specialists Conference (PESC ‘06), Jeju, Korea, 18–22 June 2006; pp. 1–5.
- Kim, S.-H.; Ji, C.-H.; Galle, P.; Herrault, F.; Wu, X.; Lee, J.-H.; Choi, C.-A.; Allen, M.G. An electromagnetic energy scavenger from direct airflow. J. Micromech. Microeng. 2009, 19, 94010. [Google Scholar] [CrossRef]
- Jung, H.-J.; Kim, I.-H.; Jang, S.-J. An energy harvesting system using the wind-induced vibration of a stay cable for powering a wireless sensor node. Smart Mater. Struct. 2011, 20, 75001. [Google Scholar] [CrossRef]
- Wang, F.; Hansen, O. Electrostatic energy harvesting device with out-of-the-plane gap closing scheme. Sens. Actuators A Phys. 2014, 211, 131–137. [Google Scholar] [CrossRef]
- Boisseau, S.; Despesse, G.; Seddik, B.A. Electrostatic conversion for vibration energy harvesting. In Small-Scale Energy Harvesting; Lallart, M., Ed.; INTECH: Lexington, KY, USA, 2012. [Google Scholar]
- Boisseau, S.; Despesse, G.; Ricart, T.; Defay, E.; Sylvestre, A. Cantilever-based electret energy harvesters. Smart Mater. Struct. 2011, 20, 105013. [Google Scholar] [CrossRef]
- Perez, M.; Boisseau, S.; Gasnier, P.; Willemin, J.; Reboud, J.L. An electret-based aeroelastic flutter energy harvester. Smart Mater. Struct. 2015, 24, 35004. [Google Scholar] [CrossRef]
- Jonkman, J.M.; Butterfield, S.; Musial, W.; Scott, G. Definition of a 5-MW Reference wind Turbine for Offshore System Development; National Renewable Energy Laboratory: Golden, CO, USA, 2009. [Google Scholar]
- Howey, D.A.; Bansal, A.; Holmes, A.S. Design and performance of a centimetre-scale shrouded wind turbine for energy harvesting. Smart Mater. Struct. 2011, 20, 85021. [Google Scholar] [CrossRef]
- Priya, S.; Chen, C.-T.; Fye, D.; Zahnd, J. Piezoelectric windmill: A novel solution to remote sensing. Jpn. J. Appl. Phys. 2005, 44, L104. [Google Scholar] [CrossRef]
- Yang, Y.; Shen, Q.; Jin, J.; Wang, Y.; Qian, W.; Yuan, D. Rotational piezoelectric wind energy harvesting using impact-induced resonance. Appl. Phys. Lett. 2014, 105, 53901. [Google Scholar] [CrossRef]
- Perez, M.; Boisseau, S.; Gasnier, P.; Willemin, J.; Geisler, M.; Reboud, J.L. A CM scale electret-based electrostatic wind turbine for low-speed energy harvesting applications. Smart Mater. Struct. 2016, 25, 45015. [Google Scholar] [CrossRef]
- Bibo, A. Investigation of Concurrent Energy Harvesting from Ambient Vibrations and Wind. Ph.D. Thesis, Clemson University, Clemson, SC, USA, August 2014. [Google Scholar]
- Deivasigamani, A.; McCarthy, J.M.; John, S.; Watkins, S.; Trivailo, P.; Coman, F. Piezoelectric energy harvesting from wind using coupled bending-torsional vibrations. Mod. Appl. Sci. 2014, 8, 106. [Google Scholar] [CrossRef]
- McCarthy, J.M.; Watkins, S.; Deivasigamani, A.; John, S.J. Fluttering energy harvesters in the wind: A review. J. Sound Vib. 2016, 361, 355–377. [Google Scholar] [CrossRef]
- Abdelkefi, A. Aeroelastic energy harvesting: A review. Int. J. Eng. Sci. 2016, 100, 112–135. [Google Scholar] [CrossRef]
- Zhu, D.; Beeby, S.; Tudor, J.; White, N.; Harris, N. A novel miniature wind generator for wireless sensing applications. In Proceedings of the IEEE Sensors, Kona, HI, USA, 1–4 November 2010; pp. 1415–1418.
- Zhang, M.; Wang, J. Experimental Study on Piezoelectric Energy Harvesting from Vortex-Induced Vibrations and Wake-Induced Vibrations. J. Sens. 2016, 2016, 2673292. [Google Scholar] [CrossRef]
- Dai, H.L.; Abdelkefi, A.; Yang, Y.; Wang, L. Orientation of bluff body for designing efficient energy harvesters from vortex-induced vibrations. Appl. Phys. Lett. 2016, 108, 53902. [Google Scholar] [CrossRef]
- Argentina, M.; Mahadevan, L. Fluid-flow-induced flutter of a flag. Proc. Natl. Acad. Sci. USA 2005, 102, 1829–1834. [Google Scholar] [CrossRef] [PubMed]
- Bibo, A.; Daqaq, M.F. Investigation of concurrent energy harvesting from ambient vibrations and wind using a single piezoelectric generator. Appl. Phys. Lett. 2013, 102, 243904. [Google Scholar] [CrossRef]
- Strganac, T.W.; Ko, J.; Thompson, D.E. Identification and control of limit cycle oscillations in aeroelastic systems. J. Guid. Control Dyn. 2000, 23, 1127–1133. [Google Scholar] [CrossRef]
- Abdelkefi, A.; Hajj, M.R.; Nayfeh, A.H. Piezoelectric energy harvesting from transverse galloping of bluff bodies. Smart Mater. Struct. 2012, 22, 15014. [Google Scholar] [CrossRef]
- Sirohi, J.; Mahadik, R. Harvesting wind energy using a galloping piezoelectric beam. J. Vib. Acoust. 2012, 134, 11009. [Google Scholar] [CrossRef]
- Li, S.; Yuan, J.; Lipson, H. Ambient wind energy harvesting using cross-flow fluttering. J. Appl. Phys. 2011, 109, 26104. [Google Scholar] [CrossRef]
- Li, S.; Lipson, H. Vertical-stalk flapping-leaf generator for wind energy harvesting. In Proceedings of the ASME Conference on Smart Materials, Adaptive Structures and Intelligent Systems, Oxnard, CA, USA, 21–23 September 2009; pp. 611–619.
- Violette, R.; De Langre, E.; Szydlowski, J. Computation of vortex-induced vibrations of long structures using a wake oscillator model: Comparison with DNS and experiments. Comput. Struct. 2007, 85, 1134–1141. [Google Scholar] [CrossRef]
- Dai, H.L.; Abdelkefi, A.; Wang, L. Vortex-induced vibrations mitigation through a nonlinear energy sink. Commun. Nonlinear Sci. Numer. Simul. 2016, 42, 22–36. [Google Scholar] [CrossRef]
- Abdelmoula, H.; Abdelkefi, A. The potential of electrical impedance on the performance of galloping systems for energy harvesting and control applications. J. Sound Vib. 2016, 370, 191–208. [Google Scholar] [CrossRef]
- Zhao, J.; Yang, J.; Lin, Z.; Zhao, N.; Liu, J.; Wen, Y.; Li, P. An arc-shaped piezoelectric generator for multi-directional wind energy harvesting. Sens. Actuators A Phys. 2015, 236, 173–179. [Google Scholar] [CrossRef]
- Park, J.-K.; Kim, K.-M.; Kwon, S.-D.; Law, K.H. An aero-elastic flutter based electromagnetic energy harvester with wind speed augmenting funnel. In Proceedings of the International Conference on Advances in Wind and Structures, Seoul, Korea, 26–29 August 2012.
- Fei, F.; Mai, J.D.; Li, W.J. A wind-flutter energy converter for powering wireless sensors. Sens. Actuators A Phys. 2012, 173, 163–171. [Google Scholar] [CrossRef]
- Matova, S.P.; Elfrink, R.; Vullers, R.J.M.; Van Schaijk, R. Harvesting energy from airflow with a michromachined piezoelectric harvester inside a Helmholtz resonator. J. Micromech. Microeng. 2011, 21, 104001. [Google Scholar] [CrossRef]
- Zhu, D.; Beeby, S.P.; Tudor, M.J.; White, N.M.; Harris, N.R. Novel miniature airflow energy harvester for wireless sensing applications in buildings. IEEE Sens. J. 2013, 13, 691–700. [Google Scholar] [CrossRef]
- Sirohi, J.; Mahadik, R. Piezoelectric wind energy harvester for low-power sensors. J. Intell. Mater. Syst. Struct. 2011. [Google Scholar] [CrossRef]
- Ramasur, D.; Hancke, G.P. A wind energy harvester for low power wireless sensor networks. In Proceesings of the 2012 IEEE International Instrumentation and Measurement Technology Conference (I2MTC), Graz, Austria, 13–16 May 2012; pp. 2623–2627.
- Xu, F.J.; Yuan, F.-G.; Hu, J.Z.; Qiu, Y.P. Design of a miniature wind turbine for powering wireless sensors. In Proceedings of the SPIE Sensors and Smart Structures Technologies for Civil, Mechanical, and Aerospace Systems, San Diego, CA, USA, 6–9 March 2010.
- Tan, Y.K.; Panda, S.K. A novel piezoelectric based wind energy harvester for low-power autonomous wind speed sensor. In Proceedings of the 33rd Annual Conference of the IEEE Industrial Electronics Society (IECON 2007), Taipei, Taiwan, 5–8 November 2007; pp. 2175–2180.
- Yang, Y.; Zhao, L.; Tang, L. Comparative study of tip cross-sections for efficient galloping energy harvesting. Appl. Phys. Lett. 2013, 102, 6–10. [Google Scholar] [CrossRef]
- Zhao, L.; Tang, L.; Yang, Y. Comparison of modeling methods and parametric study for a piezoelectric wind energy harvester. Smart Mater. Struct. 2013, 22, 125003. [Google Scholar] [CrossRef]
- Abdelkefi, A.; Scanlon, J.M.; McDowell, E.; Hajj, M.R. Performance enhancement of piezoelectric energy harvesters from wake galloping. Appl. Phys. Lett. 2013, 103, 33903. [Google Scholar] [CrossRef]
- Zhang, C.; He, X.-F.; Li, S.-Y.; Cheng, Y.-Q.; Rao, Y. A wind energy powered wireless temperature sensor node. Sensors 2015, 15, 5020–5031. [Google Scholar] [CrossRef] [PubMed]
- Alhadidi, A.H.; Bibo, A.; Daqaq, M.F. Flow Energy Harvesters With a Nonlinear Restoring Force. In Proceedings of the ASME Conference on Smart Materials, Adaptive Structures and Intelligent Systems, Newport, RI, USA, 8–10 September 2014.
- Bryant, M.; Garcia, E. Modeling and testing of a novel aeroelastic flutter energy harvester. J. Vib. Acoust. 2011, 133, 11010. [Google Scholar] [CrossRef]
- Mahadik, R.R.; Sirohi, J. Harvesting Wind Energy Using a Galloping Piezoelectric Beam. In Proceedings of the ASME Conference on Smart Materials, Adaptive Structures and Intelligent Systems, Oxnard, CA, USA, 21–23 September 2009; pp. 443–450.
- Pimentel, D.; Musilek, P.; Knight, A.; Heckenbergerova, J. Characterization of a wind flutter generator. In Proceedings of the 2010 9th International Conference on Environment and Electrical Engineering (EEEIC), Prague, Czech Republic, 16–19 May 2010; pp. 81–84.
- Zhang, L.; Masek, V.; Sanatdoost, N.N. Structural optimization of Z-axis tuning-fork MEMS gyroscopes for enhancing reliability and resolution. Microsyst. Technol. 2015, 21, 1187–1201. [Google Scholar] [CrossRef]
- Liu, H.; Zhang, S.; Kobayashi, T.; Chen, T.; Lee, C. Flow sensing and energy harvesting characteristics of a wind-driven piezoelectric Pb (Zr0.52, Ti0.48) O3 microcantilever. IET Micro Nano Lett. 2014, 9, 286–289. [Google Scholar] [CrossRef]
- Sari, I.; Balkan, T.; Kulah, H. An electromagnetic micro power generator for wideband environmental vibrations. Sens. Actuators A Phys. 2008, 145, 405–413. [Google Scholar] [CrossRef]
- Park, J.C.; Bang, D.H.; Park, J.Y. Micro-fabricated electromagnetic power generator to scavenge low ambient vibration. IEEE Trans. Magn. 2010, 46, 1937–1942. [Google Scholar] [CrossRef]
- Rancourt, D.; Tabesh, A.; Fréchette, L.G. Evaluation of centimeter-scale micro windmills: Aerodynamics and electromagnetic power generation. In Proceedings of the PowerMEMS, Freiburg, Germany, 28–29 November 2007; Volume 20079.
- Jung, H.J.; Song, Y.; Hong, S.K.; Yang, C.H.; Hwang, S.J.; Jeong, S.Y.; Sung, T.H. Design and optimization of piezoelectric impact-based micro wind energy harvester for wireless sensor network. Sens. Actuators A Phys. 2015, 222, 314–321. [Google Scholar] [CrossRef]
- Liu, H.; Zhang, S.; Kathiresan, R.; Kobayashi, T.; Lee, C. Development of piezoelectric microcantilever flow sensor with wind-driven energy harvesting capability. Appl. Phys. Lett. 2012, 100, 2010–2013. [Google Scholar] [CrossRef]
- He, X.; Shang, Z.; Cheng, Y.; Zhu, Y. A micromachined low-frequency piezoelectric harvester for vibration and wind energy scavenging. J. Micromech. Microeng. 2013, 23, 125009. [Google Scholar] [CrossRef]
- Bansal, A.; Howey, D.A.; Holmes, A.S. CM-scale air turbine and generator for energy harvesting from low-speed flows. In Proceedings of the 2009 International Solid-State Sensors, Actuators and Microsystems Conference (TRANSDUCERS), Denver, CO, USA, 21–25 June 2009; pp. 529–532.
- Wang, K.X.; Bu, L.; Chen, J.M.; Song, L. Frequency-tunable airflow energy harvester using variable aperture helmholtz resonator and piezoelectric cantilever. In Proceedings of the 18th International Conference on Solid-State Sensors, Actuators and Microsystems (TRANSDUCERS), Anchorage, AK, USA, 21–25 June 2015; pp. 1945–1948.
- Flammini, A.; Marioli, D.; Sardini, E.; Serpelloni, M. An autonomous sensor with energy harvesting capability for airflow speed measurements. In Proceedings of the 2010 IEEE Instrumentation and Measurement Technology Conference (I2MTC), Austin, TX, USA, 3–6 May 2010; pp. 892–897.
- Wu, X.; Lee, D.-W. An electromagnetic energy harvesting device based on high efficiency windmill structure for wireless forest fire monitoring application. Sens. Actuators A Phys. 2014, 219, 73–79. [Google Scholar] [CrossRef]
- Gao, X.; Shih, W.-H.; Shih, W.Y. Flow energy harvesting using piezoelectric cantilevers with cylindrical extension. IEEE Trans. Ind. Electron. 2013, 60, 1116–1118. [Google Scholar] [CrossRef]
- Priya, S. Modeling of electric energy harvesting using piezoelectric windmill. Appl. Phys. Lett. 2005, 87, 184101. [Google Scholar] [CrossRef]
- Carli, D.; Brunelli, D.; Bertozzi, D.; Benini, L. A high-efficiency wind-flow energy harvester using micro turbine. In Proceedings of the Power Electronics Electrical Drives Automation and Motion (SPEEDAM), Pisa, Italy, 14–16 June 2010; pp. 778–783.
- Wang, Y.; Wang, L.; Cheng, T.; Song, Z.; Qin, F. Sealed piezoelectric energy harvester driven by hyperbaric air load. Appl. Phys. Lett. 2016, 108, 33902. [Google Scholar] [CrossRef]
- Li, D.J.; Hong, S.; Gu, S.; Choi, Y.; Nakhmanson, S.; Heinonen, O.; Karpeev, D.; No, K. Polymer piezoelectric energy harvesters for low wind speed. Appl. Phys. Lett. 2014, 104, 12902. [Google Scholar]
- Zhao, X.; Shang, Z.; Luo, G.; Deng, L. A vibration energy harvester using AlN piezoelectric cantilever array. Microelectron. Eng. 2015, 142, 47–51. [Google Scholar] [CrossRef]
- Pan, F.; Samaddar, T. Charge Pump Circuit Design; McGraw Hill Professional: New York, NY, USA, 2006. [Google Scholar]
- Torah, R.N.; Tudor, M.J.; Patel, K.; Garcia, I.N.; Beeby, S.P. Autonomous low power microsystem powered by vibration energy harvesting. In Proceedings of the 2007 IEEE Sensors, Atlanta, GA, USA, 28–31 October 2007; pp. 264–267.
- Zargarani, A.; Mahmoodi, N. Investigating Piezoelectric Energy Harvesting Circuits for Piezoelectric Flags. In Proceedings of the ASME Conference on Smart Materials, Adaptive Structures and Intelligent Systems, Colorado Springs, CO, USA, 21–23 September 2015.
- Tan, Y.K.; Panda, S.K. Optimized wind energy harvesting system using resistance emulator and active rectifier for wireless sensor nodes. IEEE Trans. Power Electron. 2011, 26, 38–50. [Google Scholar]
- Niazi, K.; Goudarzi, M. Pyroelectric effect in PZT and PMN-0.25 PT ceramics for hybrid energy harvesting in different interface circuits. Ger. J. Renew. Sustain. Energy Res. 2015, 1, 23–29. [Google Scholar]
- Shi, G.; Xia, Y.; Ye, Y.; Qian, L.; Li, Q. An efficient self-powered synchronous electric charge extraction interface circuit for piezoelectric energy harvesting systems. J. Intell. Mater. Syst. Struct. 2016. [Google Scholar] [CrossRef]
- Zhao, L.; Yang, Y. Analytical solutions for galloping-based piezoelectric energy harvesters with various interfacing circuits. Smart Mater. Struct. 2015, 24, 75023. [Google Scholar] [CrossRef]
- Wei, J.; Risquez, S.; Mathias, H.; Lefeuvre, E.; Costa, F. Simple and efficient interface circuit for vibration electrostatic energy harvesters. In Proceedings of the 2015 IEEE Sensors, Busan, Korea, 1–4 November 2015; pp. 1–4.
- Linear Technology, LTC3588–1 Piezoelectric Energy Harvesting Power Supply. Available online: http://www.linear.com/product/LTC3588-1 (accessed on 25 March 2016).
- Infinit Power Solutions, THINERGY Micro-Energy Cells. Available online: http://www.cytech.com/products-ips (accessed on 25 March 2016).






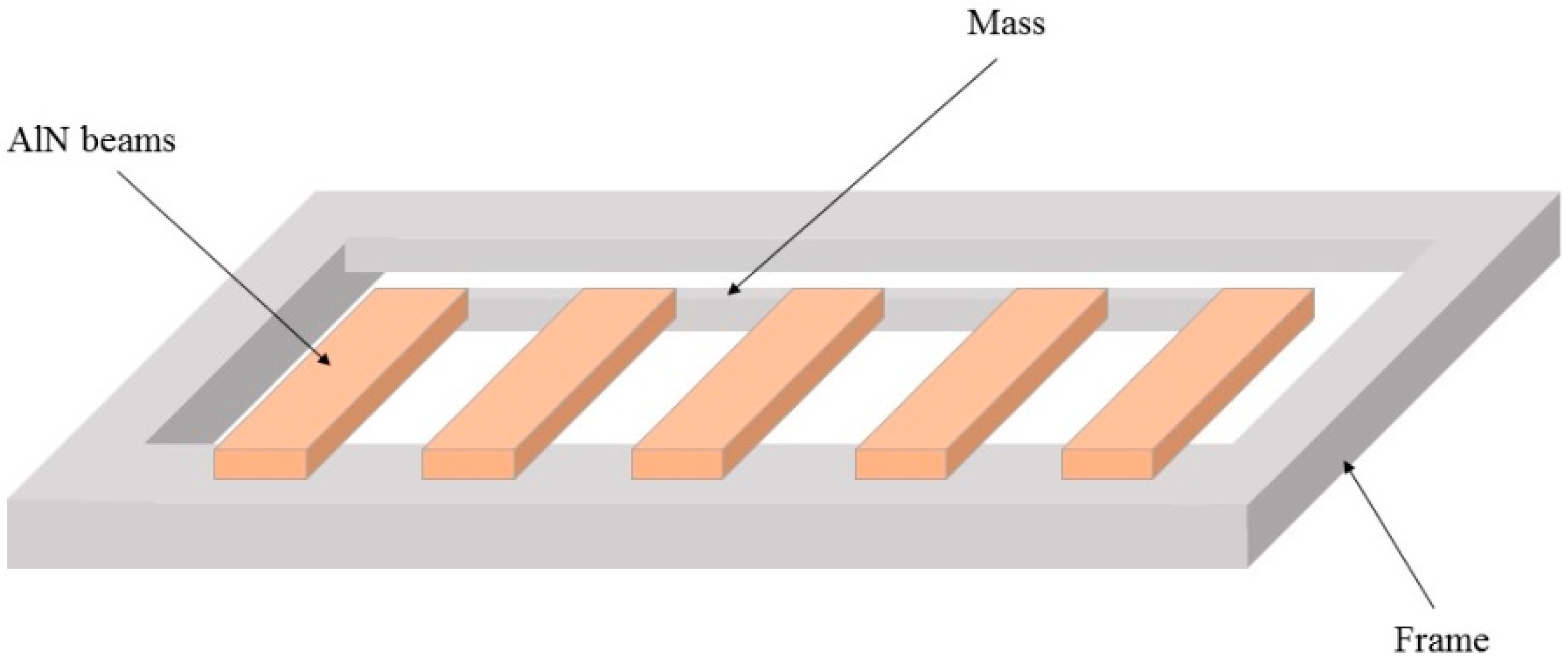
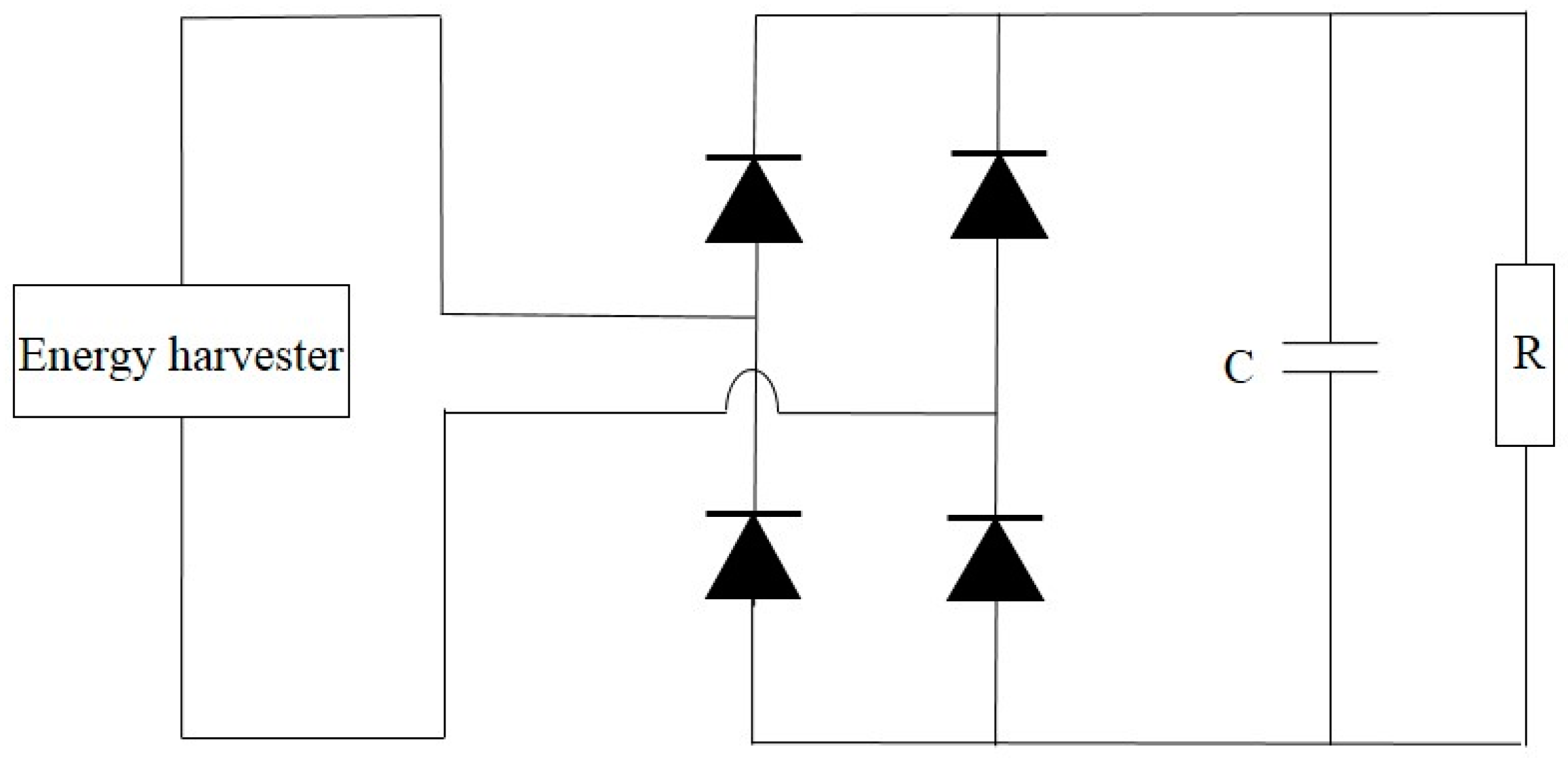
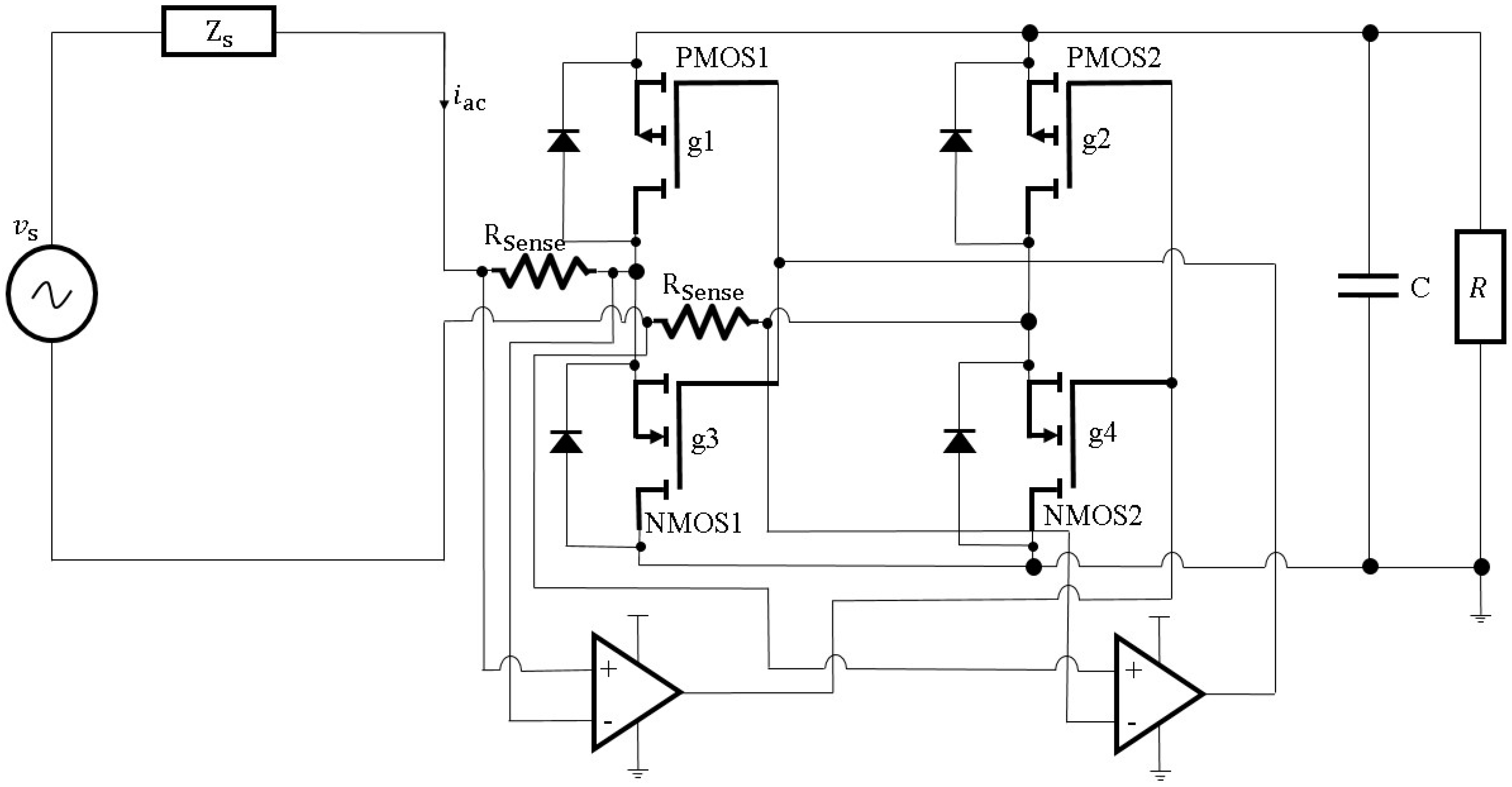

| Ambient Source | Power Density |
|---|---|
| Solar in outdoor | 100 mW/cm2 |
| Wind at 4.47 m/s speed | 10.4 mW/cm3 |
| Thermal at | 60 μW/cm2 |
| Water drop with size of 0.35 mL at 3.43 m/s speed | 30.67 μW/cm2 |
| ZnO | AlN | PZT |
|---|---|---|
| 0.34 | 0.47 | 1 |
| Ref | Size (mm) | Voltage | Peak Power | Normalized Power Density (μW × s/(mm3 × m)) | Power Coefficient (%) | Wind Speed (m/s) | Generation Technique | Mechanical Mechanism |
|---|---|---|---|---|---|---|---|---|
| [53] | φ = 76.2 | 0.15 V | 651 μW | - | - | 17.5 | EM | Rotational |
| [89] | φ = 76 | - | 6 mW | - | 8.13 | 3 | EM | Rotational |
| [90] | 76.7 × 12.7 × 2.2 | 8.8 V | 155 μW | 0.01 | 0.09 | 6.7 | PZ | Aeroelastic |
| [55] | φ = 80, t = 10 | 1.60 V | 27.14 mW | 0.1 | 5.7 | 5.4 | EM | Aeroelastic |
| [85] | φ = 80, t = 170 | 80 mV | 2 μW | 1.7 × 10−7 | 2.4 × 10−5 | 14 | PZ | Aeroelastic |
| [71] | 90 × 10 × 0.6 | 12 V | 145 μW | 0.08 | 0.63 | 3.5 | PZ | Aeroelastic |
| [18] | 100 × 60 × 30 | - | 4 mW | 0.006 | 1.73 | 4 | PZ | Aeroelastic |
| [86] | 141 × 100 × 55 | 3.8 V | 573 μW | 1.8 × 10−4 | 0.11 | 4 | EM | Aeroelastic |
| [91] | 150 × 30 × 0.6 | - | 8.4 mW | 0.4 | 0.61 | 8 | PZ | Aeroelastic |
| [92] | 150 × 30 × 1.1 | - | 6 mW | 0.15 | 0.43 | 8 | PZ | Aeroelastic |
| [93] | 152.4 × 18 × 0.305 | - | 9.5 μW | 0.007 | 0.12 | 1.69 | PZ | Aeroelastic |
| [87] | 161 ×250 × 0.635 | 30 V | 53 mW | 0.4 | 1.6 | 5.18 | PZ | Aeroelastic |
| [94] | 200 × 15 × 0.8 | - | 4.5 mW | 0.191 | 0.26 | 9.8 | PZ | Aeroelastic |
| [83] | 203.2 × 50.8 × 50.8 | 2.1 V | - | - | - | 7 | EM | Aeroelastic |
| [95] | 209 × 24 × 1 | 32 V | 4 mW | 0.073 | 0.1 | 11 | PZ | Aeroelastic |
| [96] | 254 × 254 × 0.381 | - | 2.2 mW | 0.011 | 0.011 | 7.9 | PZ | Aeroelastic |
| [97] | 325 × 36.2 × 0.267 | 30 V | 1.14 mW | 0.077 | 0.15 | 4.69 | PZ | Aeroelastic |
| [88] | 490 × 20 ×0.2 | 6 V | 70 mW | 5.1 | 3.46 | 7 | EM | Aeroelastic |
| [98] | 620 ×290 × 750 | 6 V | 171 mW | 6.3 × 10−5 | 0.02 | 20 | EM | Aeroelastic |
| [84] | 1000 × 25 × 0.2 | 3.3 V | 7 mW | 0.47 | 1.72 | 3 | EM | Aeroelastic |
| Ref | Size (mm) | Voltage | Peak Power | Normalized Power Density (μW × s/(mm3 × m)) | Power Coefficient (%) | Wind Speed (m/s) | Generation Technique | Mechanical Mechanism |
|---|---|---|---|---|---|---|---|---|
| [100] | 2 × 1.65 × 0.005 | 24 mV | 34 nW | 0.39 | 0.012 | 5.2 | PZ | Aeroelastic |
| [105] | 3 × 0.3 × 0.008 | 18.1 mV | 3.3 nW | 0.03 | 1.6 × 10−4 | 15.6 | PZ | Aeroelastic |
| [106] | 3 × 8 × 0.035 | 965 mV | 2.27 μW | 0.17 | 0.004 | 16.3 | PZ | Aeroelastic |
| [54] | 12 × 12 × 6 | 81 mV | - | - | - | - | EM | Aeroelastic |
| [54] | φ = 19, t = 5 | 4 mV | - | - | - | 5 | EM | Aeroelastic |
| [107] | φ = 20 | - | 4.3 mW | - | 2.3 | 10 | EM | Rotational |
| [108] | 23 × 4 × 0.130 | 1.6 V | 0.64 μW | 0.004 | 3.4 × 10−4 | 15 | PZ | Aeroelastic |
| [61] | φ = 32 | - | 2.5 mW | - | 1.51 | 7 | EM | Rotational |
| [64] | φ = 40, t = 10 | - | 1.8 mW | 0.01 | 0.24 | 10 | ES | Rotational |
| [109] | φ = 40 | 0.6 V | 16 mW | - | 2.11 | 9 | EM | Rotational |
| [103] | φ = 42 | - | 130 mW | - | 9.4 | 11.83 | EM | Rotational |
| [63] | 47 × 20 × 0.5 | 13 V | 613 μW | - | - | 200 r/min | PZ | Rotational |
| [110] | 75 × 60 × 30 | 5.2 V | 60 mW | 0.02 | 0.39 | 18 | EM | Rotational |
| [59] | 50 × 15 ×0.030 | 200 V | 2.1 mW | 3.1 | 0.29 | 30 | ES | Aeroelastic |
| [111] | 58 × 10 × 0.202 | 4.3 V | 30 μW | 0.05 | 0.06 | 5 | PZ | Aeroelastic |
| [112] | φ = 53 | 5 V | 7.5 mW | - | 6.32 | 4.47 | PZ | Rotational |
| [82] | 60 × 40 × 0.06 | 34 V | 1.73 mW | 0.71 | 0.03 | 17 | PZ | Aeroelastic |
| [113] | φ = 63, t=41 | 4.68 V | 10 mW | 0.02 | 5.2 | 4.67 | EM | Rotational |
| [114] | φ = 68, t = 30 | 70.90 V | 9.30 mW | - | - | - | PZ | Aeroelastic |
| [104] | 69 × 37 × 0.24 | 3.3 V | 1 mW | 0.81 | 8.1 | 2 | PZ | Aeroelastic |
| [77] | 72 × 16 × 0.41 | 3.7 V | 615 μW | 0.2 | 0.2 | 7 | PZ | Aeroelastic |
| [115] | 75 × 20 × 0.004 | 1.2 V | 0.98 μW | 0.041 | 0.002 | 3.9 | PZ | Aeroelastic |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nabavi, S.; Zhang, L. Portable Wind Energy Harvesters for Low-Power Applications: A Survey. Sensors 2016, 16, 1101. https://doi.org/10.3390/s16071101
Nabavi S, Zhang L. Portable Wind Energy Harvesters for Low-Power Applications: A Survey. Sensors. 2016; 16(7):1101. https://doi.org/10.3390/s16071101
Chicago/Turabian StyleNabavi, Seyedfakhreddin, and Lihong Zhang. 2016. "Portable Wind Energy Harvesters for Low-Power Applications: A Survey" Sensors 16, no. 7: 1101. https://doi.org/10.3390/s16071101
APA StyleNabavi, S., & Zhang, L. (2016). Portable Wind Energy Harvesters for Low-Power Applications: A Survey. Sensors, 16(7), 1101. https://doi.org/10.3390/s16071101






