Self-Oscillation-Based Frequency Tracking for the Drive and Detection of Resonance Magnetometers
Abstract
:1. Introduction
2. Resonance Magnetometer Based on Lorentz-Force
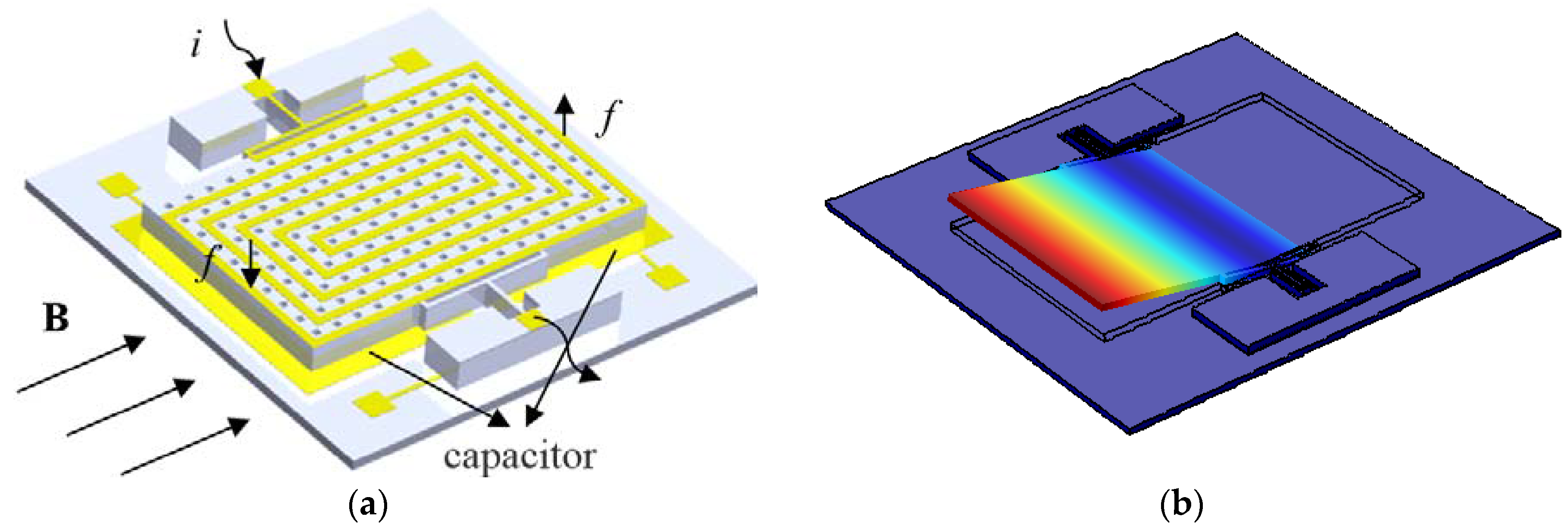
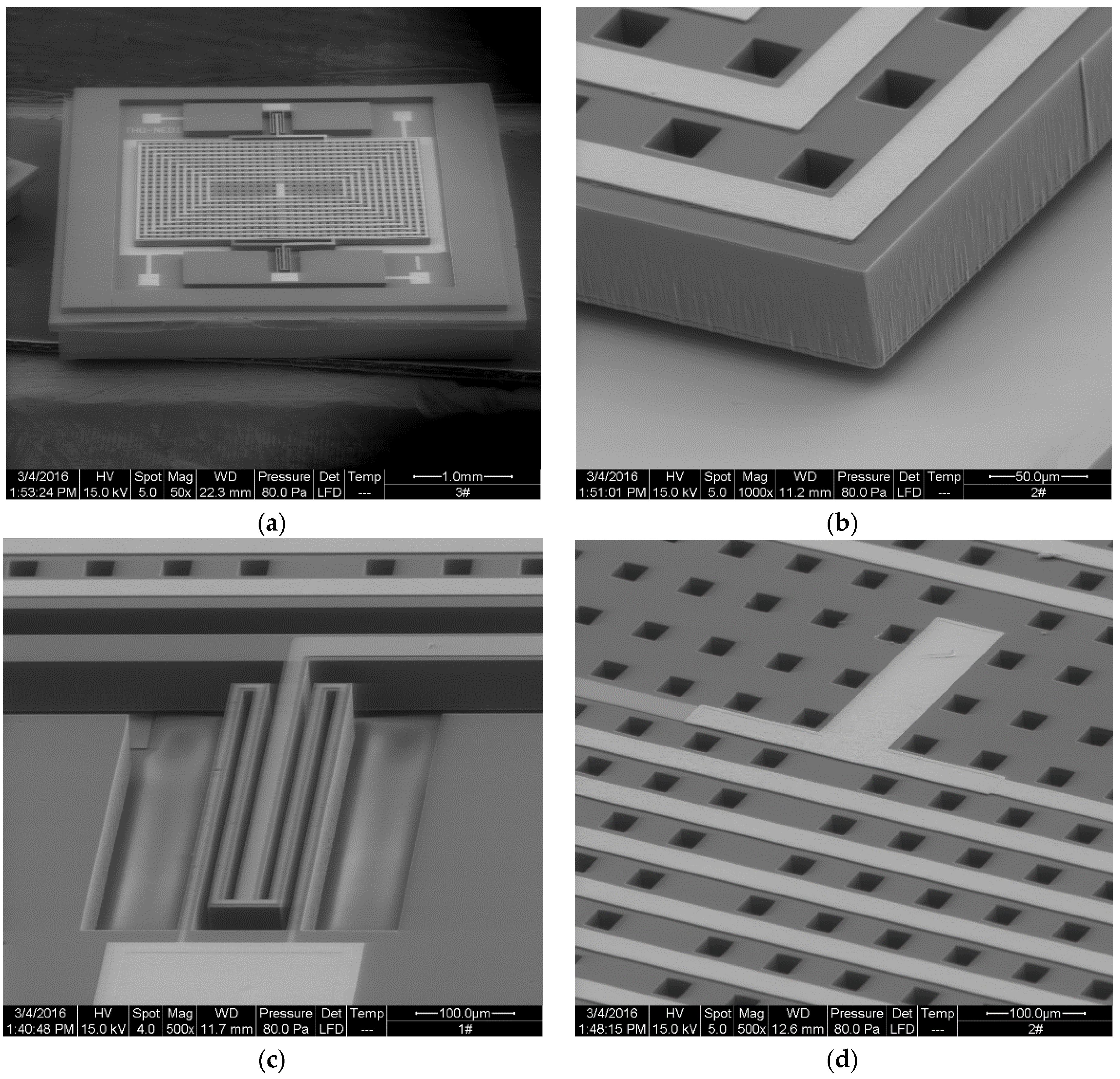
2.1. Working Principle and Fabrication
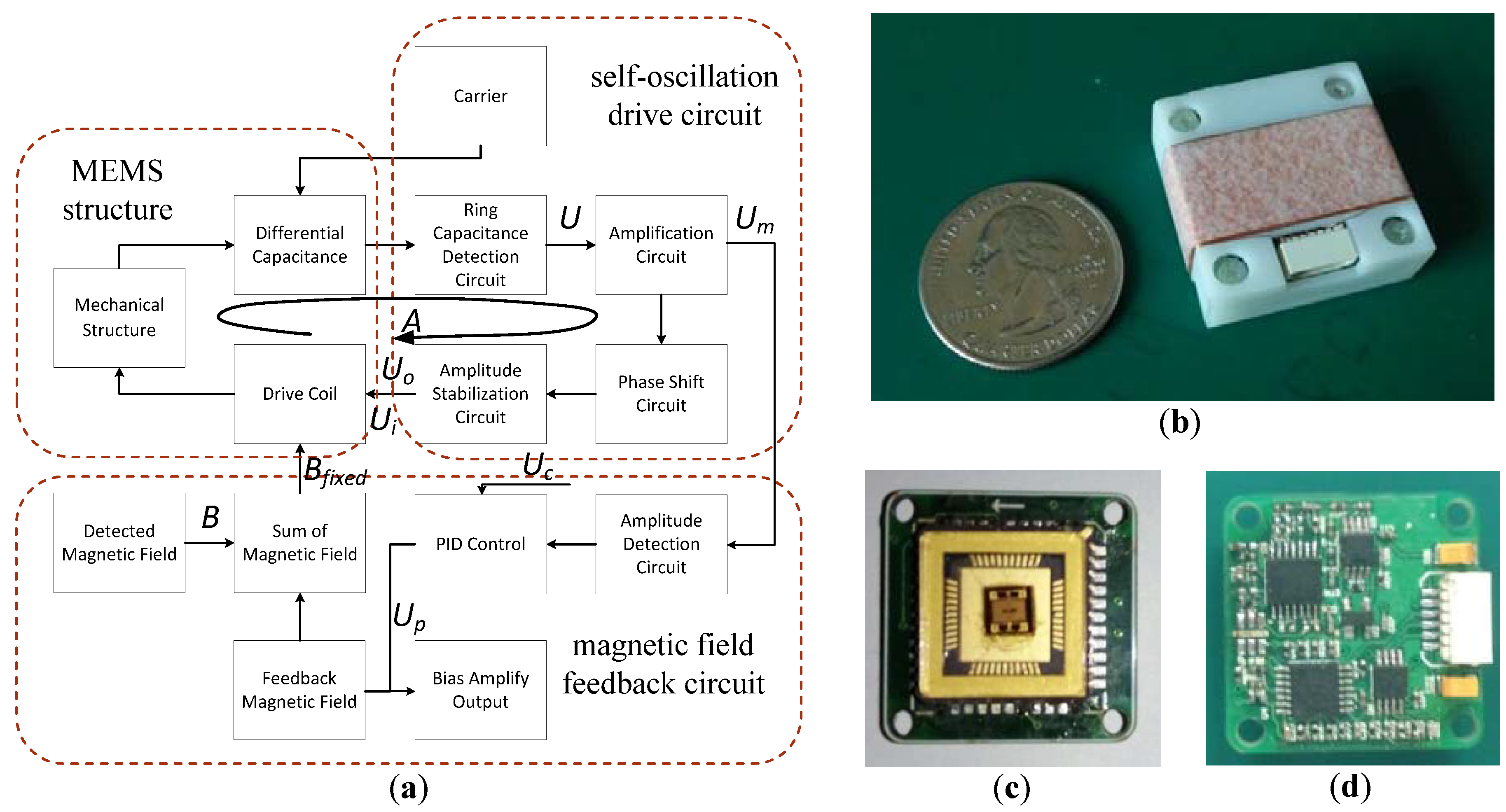
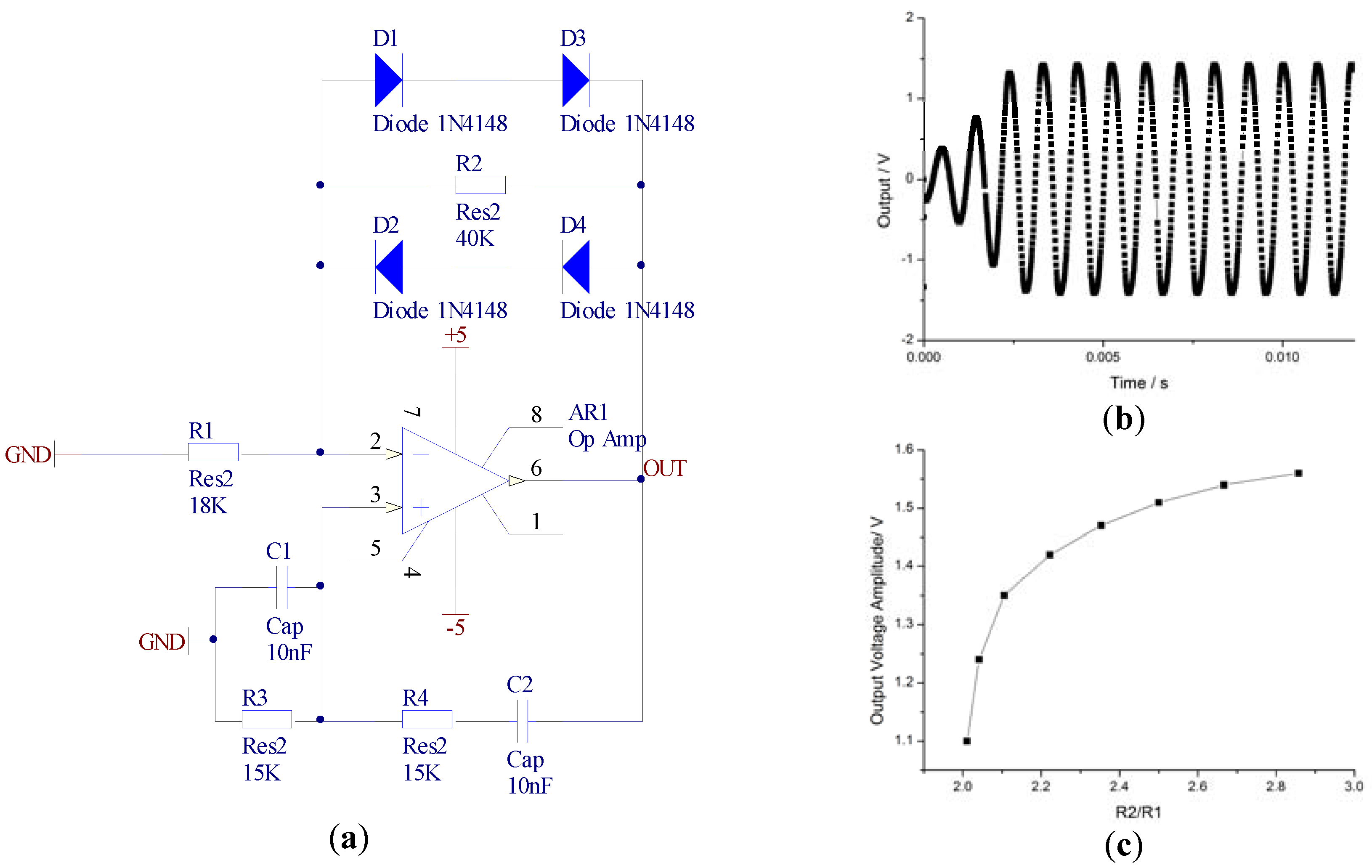
2.2. Frequency Tracking Principle Based on Self-Oscillation
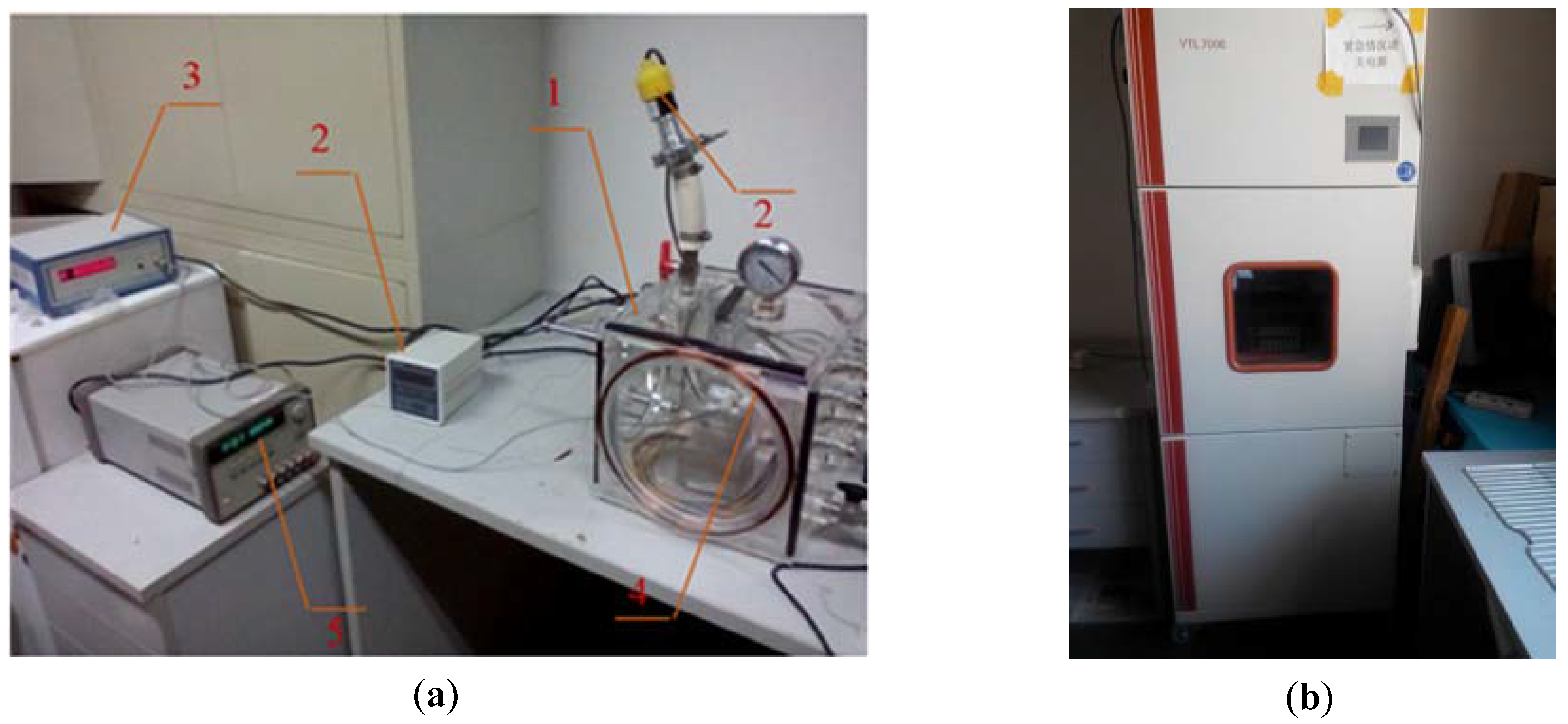
3. Testing the Magnetometer
4. Experimental Results
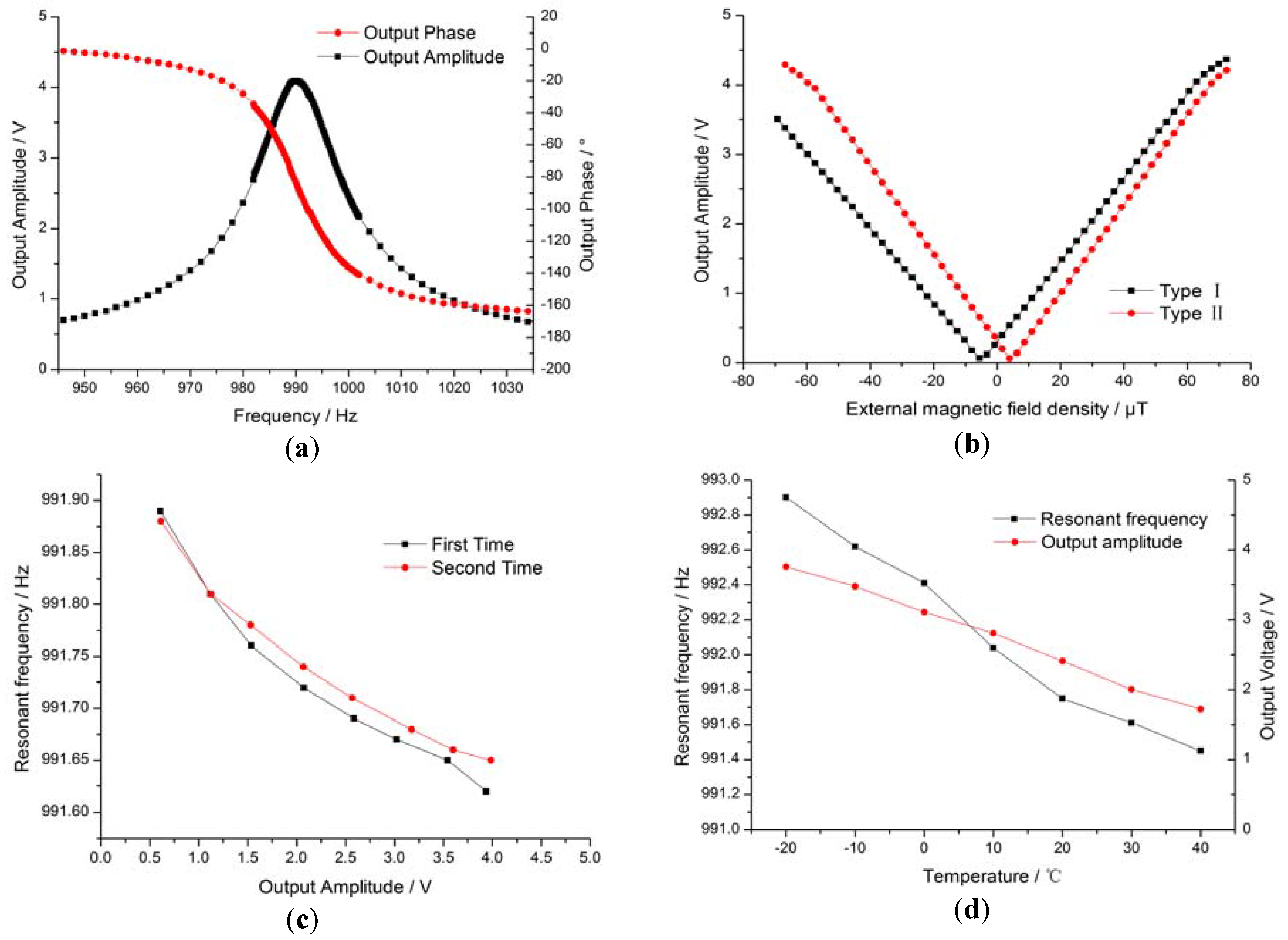
4.1. Features of the Open-Loop Prototype
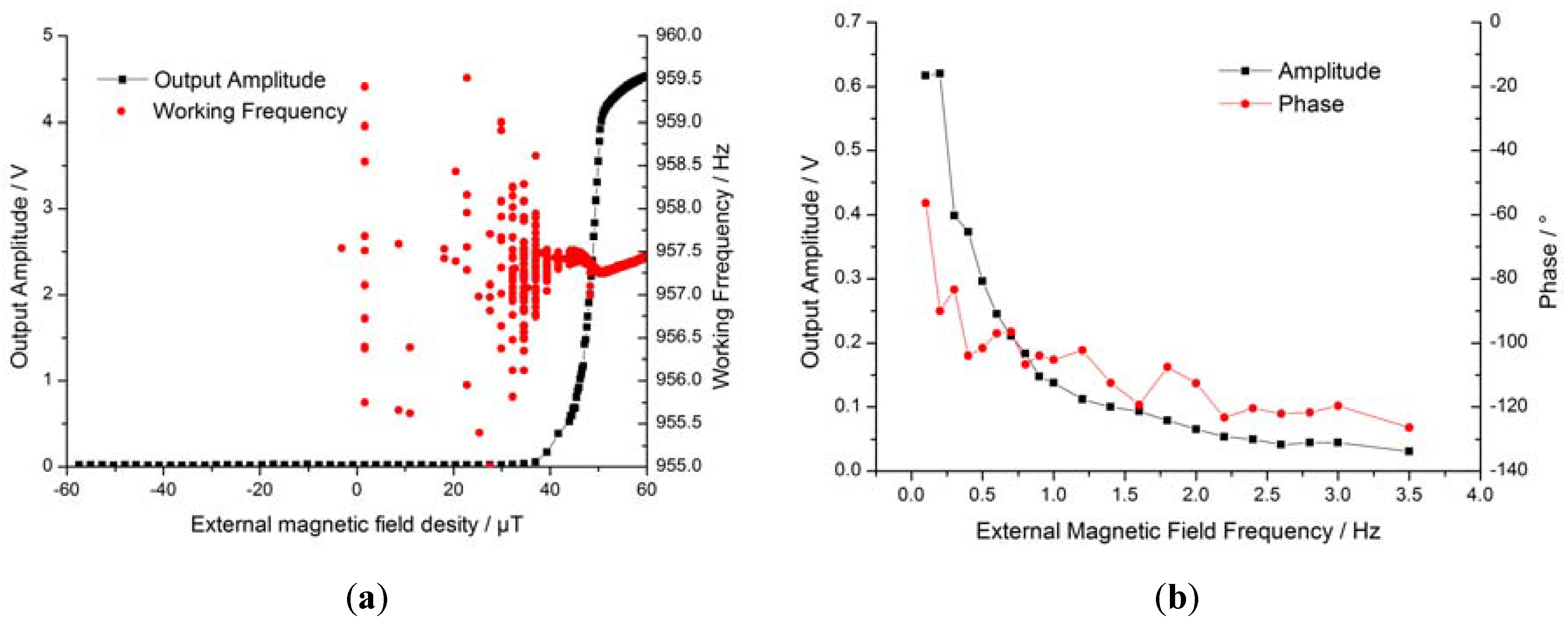
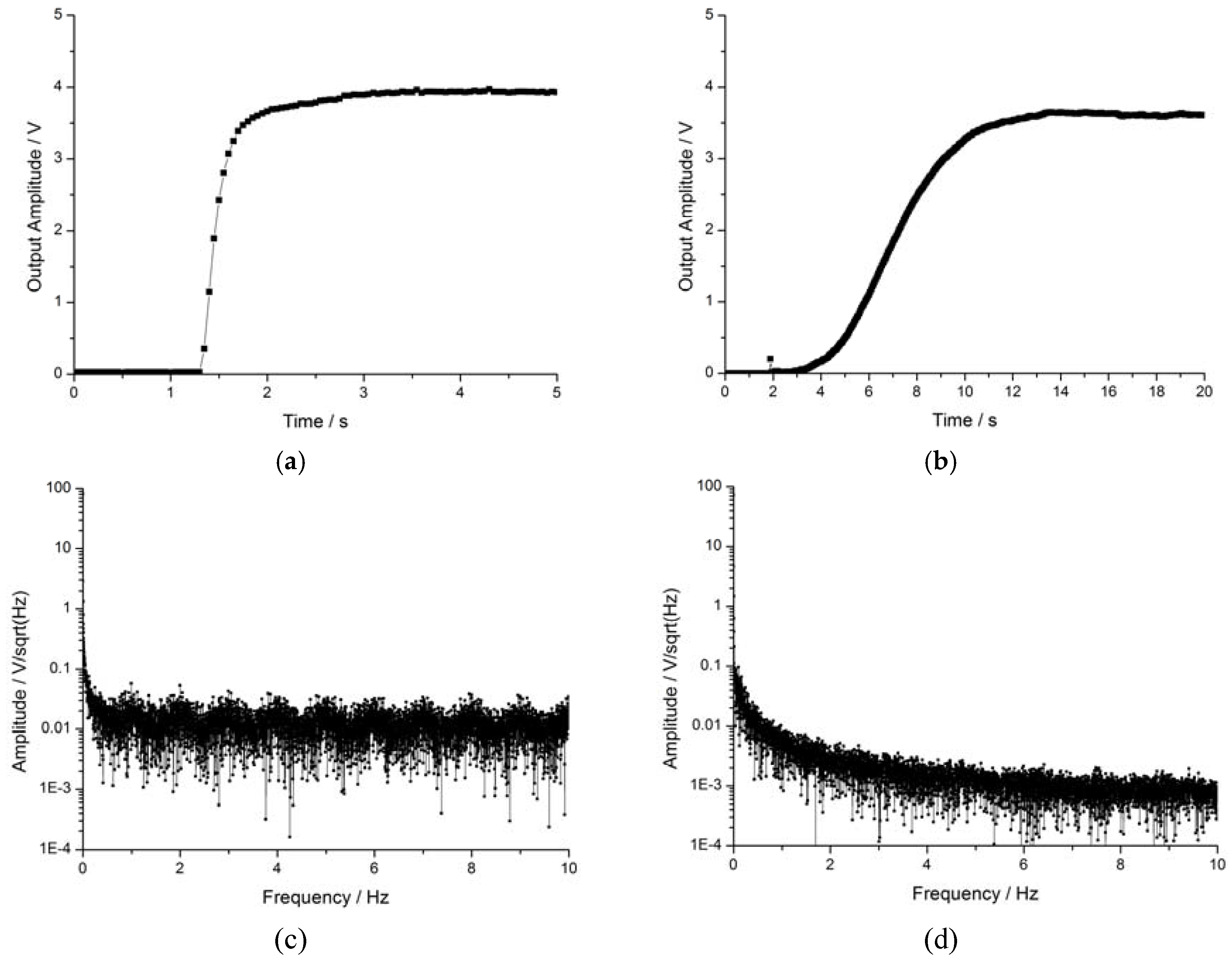
4.2. Features of Self-Oscillation Closed-Loop
4.3. Features of the Closed-Loopcoil Feedback Prototype
5. Conclusions
Author Contributions
Conflicts of Interest
References
- Donzier, E.; Lefort, O.; Spirkovitch, S.; Baillieu, F. Integrated magnetic field sensor. Sens. Actuators A Phys. 1991, 26, 357–361. [Google Scholar] [CrossRef]
- Eyre, B.; Pister, K.S.J.; Kaiser, W. Resonant mechanical magnetic sensor in standard cmos. IEEE Electron Device Lett. 1998, 19, 496–498. [Google Scholar] [CrossRef]
- Kádár, Z.; Bossche, A.; Mollinger, J. Integrated resonant magnetic field sensor. Sens. Actuators A Phys. 1997, 41, 66–69. [Google Scholar] [CrossRef]
- Keplinger, F.; Kvasnica, S.; Hauser, H.; Grossinger, R. Optical readouts of cantilever bending designed for high magnetic field application. IEEE Trans. Magn. 2003, 39, 3304–3306. [Google Scholar] [CrossRef]
- Sunier, R.; Vancura, T.; Li, Y.; Kirstein, K.U. Resonant magnetic field sensor with frequency output. J. Microelectromech. Syst. 2006, 15, 1098–1107. [Google Scholar] [CrossRef]
- Kyynäräinen, J.; Saarilahti, J.; Kattelus, H.; Kärkkäinen, A.; Meinander, T.; Oja, A.; Pekko, P.; Seppä, H.; Suhonen, M.; Kuisma, H. A 3D micromechanical compass. Sens. Actuators A Phys. 2008, 142, 561–568. [Google Scholar] [CrossRef]
- Li, M.; Rouf, V.T.; Thompson, M.J.; Horsley, D.A. Three-axis lorentz-force magnetic sensor for electronic compass applications. J. Microelectromech. Syst. 2012, 21, 1002–1010. [Google Scholar] [CrossRef]
- Minotti, P.; Brenna, S.; Laghi, G.; Bonfanti, A.G.; Langfelder, G.; Lacaita, A.L. A sub-400-nt/, 775-, multi-loop MEMS magnetometer with integrated readout electronics. J. Microelectromech. Syst. 2015, 24, 1938–1950. [Google Scholar] [CrossRef]
- Li, M.; Sonmezoglu, S.; Horsley, D.A. Extended bandwidth lorentz force magnetometer based on quadrature frequency modulation. J. Microelectromech. Syst. 2015, 24, 333–342. [Google Scholar] [CrossRef]
- Sonmezoglu, S.; Li, M.; Horsley, D. Force-rebalanced lorentz force magnetometer based on a micromachined oscillator. Appl. Phys. Lett. 2015, 106, 093504. [Google Scholar] [CrossRef]
- Lara-Castro, M.; Herrera-May, A.; Juarez-Aguirre, R.; López-Huerta, F.; Ceron-Alvarez, C.; Cortes-Mestizo, I.; Morales-Gonzalez, E.; Vazquez-Leal, H.; Dominguez-Nicolas, S. Portable signal conditioning system of a MEMS magnetic field sensor for industrial applications. Microsyst. Technol. 2016, 1–9. [Google Scholar] [CrossRef]
- Bagherinia, M.; Bruggi, M.; Corigliano, A.; Mariani, S.; Horsley, D.A.; Li, M.; Lasalandra, E. An efficient earth magnetic field MEMS sensor: Modeling, experimental results, and optimization. J. Microelectromech. Syst. 2015, 24, 887–895. [Google Scholar] [CrossRef]
- Herrera-May, A.; Lara-Castro, M.; López-Huerta, F.; Gkotsis, P.; Raskin, J.-P.; Figueras, E. A MEMS-based magnetic field sensor with simple resonant structure and linear electrical response. Microelectron. Eng. 2015, 142, 12–21. [Google Scholar] [CrossRef]
- Laghi, G.; Dellea, S.; Longoni, A.; Minotti, P.; Tocchio, A.; Zerbini, S.; Langfelder, G. Torsional MEMS magnetometer operated off-resonance for in-plane magnetic field detection. Sens. Actuators A Phys. 2015, 229, 218–226. [Google Scholar] [CrossRef]
- Juárez-Aguirre, R.; Domínguez-Nicolás, S.M.; Manjarrez, E.; Tapia, J.A.; Figueras, E.; Vázquez-Leal, H.; Aguilera-Cortés, L.A.; Herrera-May, A.L. Digital signal processing by virtual instrumentation of a MEMS magnetic field sensor for biomedical applications. Sensors 2013, 13, 15068–15084. [Google Scholar] [CrossRef] [PubMed]
- Domínguez-Nicolás, S.M.; Juarez-Aguirre, R.; García-Ramírez, P.J.; Herrera-May, A.L. Signal conditioning system with a 4–20 mA output for a resonant magnetic field sensor based on MEMS technology. IEEE Sens. J. 2012, 12, 935–942. [Google Scholar] [CrossRef]
- Herrera-May, A.L.; García-Ramírez, P.J.; Aguilera-Cortés, L.A.; Martínez-Castillo, J.; Sauceda-Carvajal, A.; García-González, L.; Figueras-Costa, E. A resonant magnetic field microsensor with high quality factor at atmospheric pressure. J. Micromech. Microeng. 2009, 19, 365–372. [Google Scholar] [CrossRef]
- Buffa, C.; Langfelder, G.; Longoni, A.; Frangi, A. Compact MEMS magnetometers for inertial measurement units. In Proceedings of the 2012 IEEE Sensors, Taipei, Taiwan, 28–31 October 2012; pp. 1–4.
- Wu, G.; Xu, D.; Xiong, B.; Feng, D. Resonant magnetic field sensor with capacitive driving and electromagnetic induction sensing. IEEE Electron Device Lett. 2013, 34, 459–461. [Google Scholar] [CrossRef]
- Ren, D.; Wu, L.; Yan, M.; Cui, M.; You, Z.; Hu, M. Design and analyses of a MEMS based resonant magnetometer. Sensors 2009, 9, 6951–6966. [Google Scholar] [CrossRef] [PubMed]
- Ng, L.; Li, M.R. Self-oscillation loop design and measurement for an MEMS resonant accelerometer. Int. J. Adapt. Control Signal Process. 2013, 27, 859–872. [Google Scholar]
- Hyun, C.; Lee, J.G.; Kang, T. Precise oscillation loop for a resonant type MEMS inertial sensors. In Proceedings of the 2006 SICE-ICASE International Joint Conference, Busan, Korea, 18–21 October 2006; pp. 1953–1958.
- Mukhopadhyay, A.D.; Antonio, D.; Jung, I.W.; López, D. Self-sustained oscillation of MEMS torsional micromirrors. ProcSpie 2012, 8252, 560–569. [Google Scholar]
- Wang, Z.; Li, Z.; Lu, W. A new self-oscillation loop for MEMS vibratory gyroscopes. In Proceedings of the 7th International Conference on ASIC, Guilin, China, 22–25 October 2007; pp. 1046–1049.
- Wang, Z.F.; Lu, W.G.; Li, F.; Li, Z.H. Theoretical analysis and numerical simulation of closed-loop self-oscillation system for MEMS vibratory gyroscopes. Chin. J. Sens. Actuators 2008, 8, 010. [Google Scholar]
- Hilton, P. Robust stability analysis of a phase-locked loop voltage-controlled oscillator circuit. In Proceedings of the American Control Conference, San Diego, CA, USA, 2–4 June 1999; pp. 425–429.
- Shahriary, I.; McNab, K.M. Phase-Locked Loop Frequency Tracking Device Including a Direct Digital Synthesizer. U.S. Patent 5,184,092 A, 2 Feburary 1993. [Google Scholar]
- Sun, X.; Horowitz, R.; Komvopoulos, K. Stability and resolution analysis of a phase-locked loop natural frequency tracking system for MEMS fatigue testing. J. Dyn. Syst. Meas. Control 2002, 124, 599–605. [Google Scholar] [CrossRef]
- Wu, H.M.; Yang, H.G.; Yin, T.; Zhang, H. Stability analysis of MEMS gyroscope drive loop based on CPPLL. In Proceedings of the Asia Pacific Conference on Postgraduate Research in Microelectronics and Electronics (PrimeAsia), Macau, China, 6–7 October 2011; pp. 45–48.
- Kádár, Z.; Bossche, A.; Sarro, P.; Mollinger, J. Magnetic-field measurements using an integrated resonant magnetic-field sensor. Sens. Actuators A Phys. 1998, 70, 225–232. [Google Scholar] [CrossRef]
- Emmerich, H.; Schofthaler, M. Magnetic field measurements with a novel surface micromachined magnetic-field sensor. IEEE Trans. Electron Devices 2000, 47, 972–977. [Google Scholar] [CrossRef]
- Emmerich, H.; Schofthaler, M.; Knauss, U. A novel micromachined magnetic-field sensor. In Proceedings of the Twelfth IEEE International Conference on Micro Electro Mechanical Systems, Orlando, FL, USA, 21–21 January 1999; pp. 94–99.
- Thompson, M.J.; Horsley, D.A. Parametrically amplified-axis lorentz force magnetometer. J. Microelectromech. Syst. 2011, 20, 702–710. [Google Scholar] [CrossRef]
- Alandry, B.; Latorre, L.; Mailly, F.; Nouet, P. A fully integrated inertial measurement unit: Application to attitude and heading determination. IEEE Sens. J. 2011, 11, 2852–2860. [Google Scholar] [CrossRef]
- Li, M.; Ng, E.J.; Hong, V.A.; Ahn, C.H.; Yang, Y.; Kenny, T.W.; Horsley, D.A. Single-structure 3-axis lorentz force magnetometer with sub-30 nt/√HZ resolution. In Proceedings of the 2014 IEEE 27th International Conference on Micro Electro Mechanical Systems (MEMS), San Francisco, CA, USA, 26–30 January 2014; pp. 80–83.
- Langfelder, G.; Laghi, G.; Minotti, P.; Tocchio, A.; Longoni, A. Off-resonance low-pressure operation of lorentz force MEMS magnetometers. IEEE Trans. Ind. Electron. 2014, 61, 7124–7130. [Google Scholar] [CrossRef]
- Bahreyni, B.; Shafai, C. A resonant micromachined magnetic field sensor. IEEE Sens. J. 2007, 7, 1326–1334. [Google Scholar] [CrossRef]
- Wu, J. Theory of Elasticity; Tongji University Press: Shanghai, China, 1993. [Google Scholar]
- Chen, H.; Zhang, R.; Zhou, B.; Chen, Z. Research on thermal characteristic and compensation algorithm for MEMS-gyroscope. J. Transducer Technol. 2004, 23, 24–26. [Google Scholar]


| Author | Dimensions/μm | Bandwidth/Hz | Resolution/nT·mA/√Hz | Current/mA |
|---|---|---|---|---|
| Kadar [30] | 2800 × 1400 | N.A. | 217 | 10 |
| Emmerich [31] | 1300 × 500 | 1–10 | 186 | 1 |
| Kyynäräinen | 2000 × 400 | 2 | 7 | 0.1 |
| Thompson [32,33] | 2000 × 1000 | 1 | 87 | 2.7 |
| Alandry [34] | 370 × 330 | 10 | 1161 | 4.5 |
| Li [35] | 2000 × 2000 | 1.9 | 95 | 8.2 |
| Langfelder [36] | 868 × 89 | 160 | 520 | 0.05 |
| Lara-Castro | 700 × 600 | N.A. | 700 | 20 |
| This study | 3000 × 2000 | 0.3 | 130 | 0.4 |
| Component | Parameter | Component | Parameter |
|---|---|---|---|
| Pendulum | 3000 μm × 2000 μm × 60 μm | Coil layers | 2 |
| Hole | 30 μm × 30 μm | Coil turns | 10 |
| Hole number | 864 | Coil width | 30 μm |
| Beam length | 1350 μm 1 | Coil thickness | 2 μm |
| Beam thickness | 60 μm | Capacitor plate | 2300 μm × 1490 μm × 0.3 μm |
| Beam width | 30 μm(Type I) 1 28 μm (Type II) 1 | Distance between upper and lower plates | 10 μm |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tian, Z.; Ren, D.; You, Z. Self-Oscillation-Based Frequency Tracking for the Drive and Detection of Resonance Magnetometers. Sensors 2016, 16, 744. https://doi.org/10.3390/s16050744
Tian Z, Ren D, You Z. Self-Oscillation-Based Frequency Tracking for the Drive and Detection of Resonance Magnetometers. Sensors. 2016; 16(5):744. https://doi.org/10.3390/s16050744
Chicago/Turabian StyleTian, Zheng, Dahai Ren, and Zheng You. 2016. "Self-Oscillation-Based Frequency Tracking for the Drive and Detection of Resonance Magnetometers" Sensors 16, no. 5: 744. https://doi.org/10.3390/s16050744
APA StyleTian, Z., Ren, D., & You, Z. (2016). Self-Oscillation-Based Frequency Tracking for the Drive and Detection of Resonance Magnetometers. Sensors, 16(5), 744. https://doi.org/10.3390/s16050744







