Regulatory Processes in Populations of Forest Insects (A Case Study of Insect Species Damaging the Pine Pinus sylvestris L. in Forests of SIBERIA)
Abstract
1. Introduction
2. Materials and Methods
3. Results
- The studied time series was tested for stationarity and, if necessary, transformed to the stationary time series.
- The order of the AR equation, characterizing the number of significant feedbacks, was estimated.
- Based on the results of steps 1 and 2, absolute values and signs of feedback coefficients were calculated, using the data of long-term surveys.
- Reliability of the calculations was assessed.
- -
- “Repair” of the time series of population dynamics and replacement of the zero values of population densities with values that are smaller by a factor of two than the minimal values of the measured insect population densities for the entire measurement period;
- -
- Conversion to logarithmic scale in order to reduce data scattering (the replacement of the zero density values in the step above forms the prerequisite);
- -
- Filtering the high-frequency component of the time series in order to reduce the errors in insect surveys. The Hann window is used [35]:
- -
- The partial autocorrelation function, PACF, is calculated [36] to determine the order k of autoregressive Equation (1). The order of autoregressive equation is characterized by the maximal PACF;
- -
- Model (2) is considered as a regression equation with {y(i)} values known from survey data, and conventional methods are used to determine the unknown coefficients a0, …., ak;
- -
- The accuracy of the calculations using the model equation of insect population dynamics is estimated from the value of coefficient of determination, R2, characterizing the portion of variance of logarithmic insect abundance calculated using the AR model; the significance of coefficients of the AR model is estimated by Student’s t-test and Fisher’s test [26];
- -
- Assessment of the synchrony of the time series of transformed data and the model time series is based on the value of the cross-correlation function (CCF) [30]. For synchronous time series, the maximal value of CCF (k = 0) is close to 1;
- -
- -
- The stability of the time series of population dynamics for a given habitat is estimated from the stability margin η calculated using coefficients a1,…, ak of model (2) for this habitat. The greater the stability margin η, the higher the stability of the dynamics series. The calculation procedure is given in Supplement S3.
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Berryman, A.A. What causes population cycles of forest Lepidoptera? Trends Ecol. Evol. 1996, 11, 28–32. [Google Scholar] [CrossRef] [PubMed]
- Turchin, P. Rarity of density dependence or population regulation with lags? Nature 1990, 344, 660–663. [Google Scholar] [CrossRef]
- Berryman, A.A. The Theory and Classification of Outbreaks. In Insect Outbreaks; Barbosa, P., Schultz, J.C., Eds.; Academic Press: San Diego, CA, USA, 1987; pp. 3–30. [Google Scholar]
- Wallner, W.E. Factors affecting insect population dynamics: Differences between outbreak and non-outbreak species. Annu. Rev. Entomol. 1987, 32, 317–340. [Google Scholar] [CrossRef]
- Liebhold, A.M.; McManus, M.L. Does larval dispersal cause the expansion of gypsy moth outbreaks. North J. Appl. For. 1991, 8, 95–98. [Google Scholar] [CrossRef]
- Royama, T. Analytical Population Dynamics; Chapman & Hall: London, UK, 1992; 387p. [Google Scholar]
- Turner, M.G.; O’Neill, R.V.; Gardner, R.H.; Milne, B.T. Effects of changing spatial scale on the analysis of landscape pattern. Landsc. Ecol. 1989, 3, 153–162. [Google Scholar] [CrossRef]
- Wiens, J.A.; Stenseth, N.C.; Van Horne, B.; Ims, R.A. Ecological mechanisms and landscape ecology. Oikos 1993, 66, 369–380. [Google Scholar] [CrossRef]
- Johnson, D.M.; Bjørnstad, O.N.; Liebhold, A.M. Landscape geometry and travelling waves in the larch budmoth. Ecol. Lett. 2004, 7, 967–974. [Google Scholar] [CrossRef]
- Peltonen, M.; Liebhold, A.M.; Bjørnstad, O.M.; Williams, D.W. Spatial synchrony in forest insect outbreaks: Roles of regional stochasticity and dispersal. Ecology 2002, 83, 3120–3129. [Google Scholar] [CrossRef]
- Raimondo, S.; Lebhold, A.M.; Strazanac, J.S.; Butler, L. Population synchrony within and among Lepidoptera species in relation to weather, phylogeny, and larval phenology. Ecol. Entomol. 2004, 29, 96–105. [Google Scholar] [CrossRef]
- Liebhold, A.; Koenig, W.; Bjornstad, O.N. Spatial Synchrony in population dynamics. Annu. Rev. Ecol. Evol. Syst. 2004, 35, 467–490. [Google Scholar] [CrossRef]
- Sherriff, R.L.; Berg, E.E.; Miller, A.E. Climate variability and spruce beetle (Dendroctonusrufipennis) outbreaks in south-central and south-west Alaska. Ecology 2011, 92, 1459–1470. [Google Scholar] [CrossRef] [PubMed]
- Chapman, T.B.; Veblen, T.T.; Schoennagel, T. Spatiotemporal patterns of mountain pine beetle activity in the southern Rocky Mountains. Ecology 2012, 93, 2175–2185. [Google Scholar] [CrossRef] [PubMed]
- Bentz, B.J.; Vandygriff, J.; Jensen, C.; Coleman, T.; Maloney, P.; Smith, S.; Grady, A.; Schen-Langenheim, G. Mountain pine beetle voltinism and life history characteristics across latitudinal and elevational gradients in the western United States. For. Sci. 2014, 60, 434–449. [Google Scholar] [CrossRef]
- Hart, S.J.; Veblen, T.T.; Eisenhart, K.S.; Jarvis, D.; Kulakowski, D. Drought induces spruce beetle (Dendroctonusrufipennis) outbreaks across northwestern Colorado. Ecology 2014, 95, 930–939. [Google Scholar] [CrossRef]
- Bentz, B.J.; Regniere, J.; Fettig, C.J.; Hansen, E.M.; Hayes, J.L.; Hicke, J.A.; Kelsey, R.G.; Negro’n, J.F.; Seybold, S.J. Climate change and bark beetles of the Western United States and Canada: Direct and indirect effects. BioScience 2010, 60, 602–613. [Google Scholar] [CrossRef]
- Senf, C.; Campbell, E.M.; Pflugmacher, D.; Wulder, M.A.; Hostert, P. A multi-scale analysis of western spruce budworm outbreak dynamics. Landsc. Ecol. 2017, 32, 501–514. [Google Scholar] [CrossRef]
- Seidl, R.; Müller, J.; Hothorn, T.; Bässler, C.; Heurich, M.; Kautz, M. Small beetle, large-scale drivers: How regional and landscape factors affect outbreaks of the european spruce bark beetle. J. Appl. Ecol. 2016, 53, 530–540. [Google Scholar] [CrossRef]
- Raffa, K.F.; Aukema, B.H.; Bentz, B.J.; Carroll, A.L.; Hicke, J.A.; Turner, M.G.; Romme, W.H. Cross-scale drivers of natural disturbances prone to anthropogenic amplification: The dynamics of bark beetle eruptions. Bioscience 2008, 58, 501–517. [Google Scholar] [CrossRef]
- Moran, P.A.P. The statistical analysis of the Canadian lynx cycle. II. Synchronization and meteorology. Aust. J. Zool. 1953, 1, 291–298. [Google Scholar] [CrossRef]
- Baars, M.A.; Van Dijk, T.S. Population dynamics of two carabid beetles at a Dutch heatland. J. Anim. Ecol. 1984, 53, 375–388. [Google Scholar] [CrossRef]
- Bjørnstad, O.N.; Ims, R.A.; Lambin, X. Spatial population dynamics: Analyzing patterns and processes of population synchrony. Trends Ecol. Evol. 1999, 11, 427–431. [Google Scholar] [CrossRef] [PubMed]
- Bjornstad, O.N.; Bascompte, J. Synchrony and second order spatial correlation in host–parasitoid system. J. Anim. Ecol. 2001, 70, 924–933. [Google Scholar] [CrossRef]
- Maron, J.L.; Harrison, S. Spatial patterns formation in an insect host-parasitoid system. Science 1997, 278, 1619–1621. [Google Scholar] [CrossRef] [PubMed]
- Pollard, J.H. A Handbook of Numerical and Statistical Techniques; Cambridge University Press: Cambridge, UK, 1979; 368p. [Google Scholar]
- Volney, W.J.A.; Fleming, R.A. Climate change and impacts of boreal forest insects. Agric. Ecosyst. Environ. 2000, 82, 283–294. [Google Scholar] [CrossRef]
- Williams, D.W.; Liebhold, A. Influence of weather on synchrony of gypsy moth (Lepidoptera: Lymantridae) outbreaks in New England. Environ. Entomol. 1995, 24, 987–995. [Google Scholar] [CrossRef]
- Anderson, T.W. The Statistical Analysis of Time Series; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 1971; 704p. [Google Scholar]
- Jenkins, G.M.; Watts, D.G. Spectral Analysis and Its Applications; Holden-Day: San Francisco, CA, USA, 1969; 525p. [Google Scholar]
- Kendall, M.G.; Stewart, A. The Advanced Theory of Statistics: Design and Analysis, and Time Series; Charles Griffin: Glasgow, UK, 1973; 780p. [Google Scholar]
- Marple, S.L., Jr. Digital Spectral Analysis: With Applications; Prentice-Hall: Englewood Cliffs, NJ, USA, 1987; 492p. [Google Scholar]
- Palnikova, E.N.; Sviderskaya, I.V.; Soukhovolsky, V.G. Pine Looper in Siberian Forests; Nauka: Novosibirsk, Russia, 2002; 252p. (In Russian) [Google Scholar]
- Isaev, A.S.; Soukhovolsky, V.G.; Tarasova, O.V.; Palnikova, E.N.; Kovalev, A.V. Forest Insect Population Dynamics, Outbreaks and Global Warming Effects; Wiley: Hoboken, NJ, USA, 2017; 298p. [Google Scholar]
- Hamming, R.W. Digital Filters; Courier Corporation: Chelmsford, MA, USA, 1989; 284p. [Google Scholar]
- Box, G.E.P.; Jenkins, G.M. Time Series Analysis: Forecasting and Control; Holden-Day: San Francisco, CA, USA, 1970; 784p. [Google Scholar]
- Dorf, R.C.; Bishop, R.H. Modern Control Systems; Prentice Hall: Englewood Cliffs, NJ, USA, 2008; 1018p. [Google Scholar]
- Gaiduk, A.R.; Belyaev, V.E.; Pyavchenko, T.A. Automatic Control Theory: Examples and Problems; Lan: St. Petersburg, Russia, 2001; 464p. (In Russian) [Google Scholar]
- Kim, D.P. Theory of Automatic Control; Fizmatlit: Moscow, Russian, 2007; Volume 1, 312p. (In Russian) [Google Scholar]


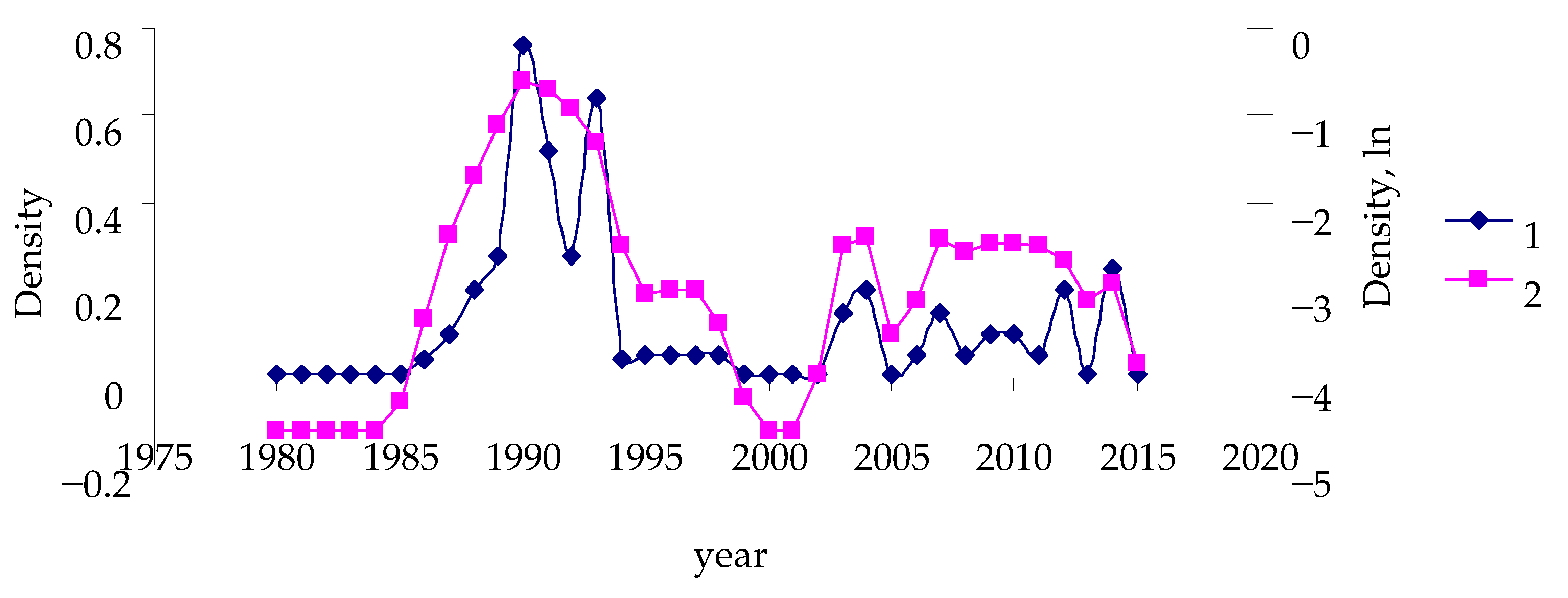
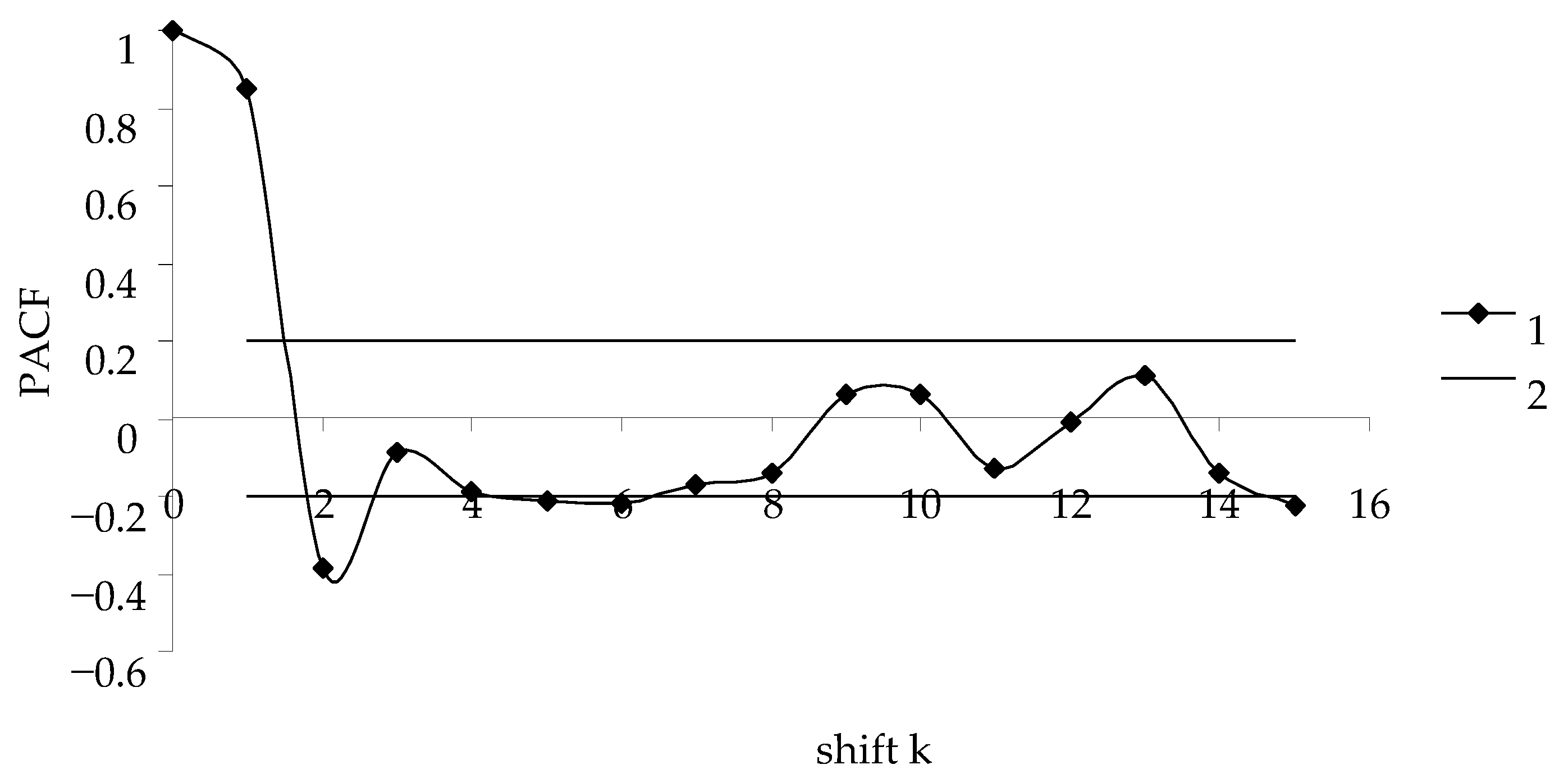
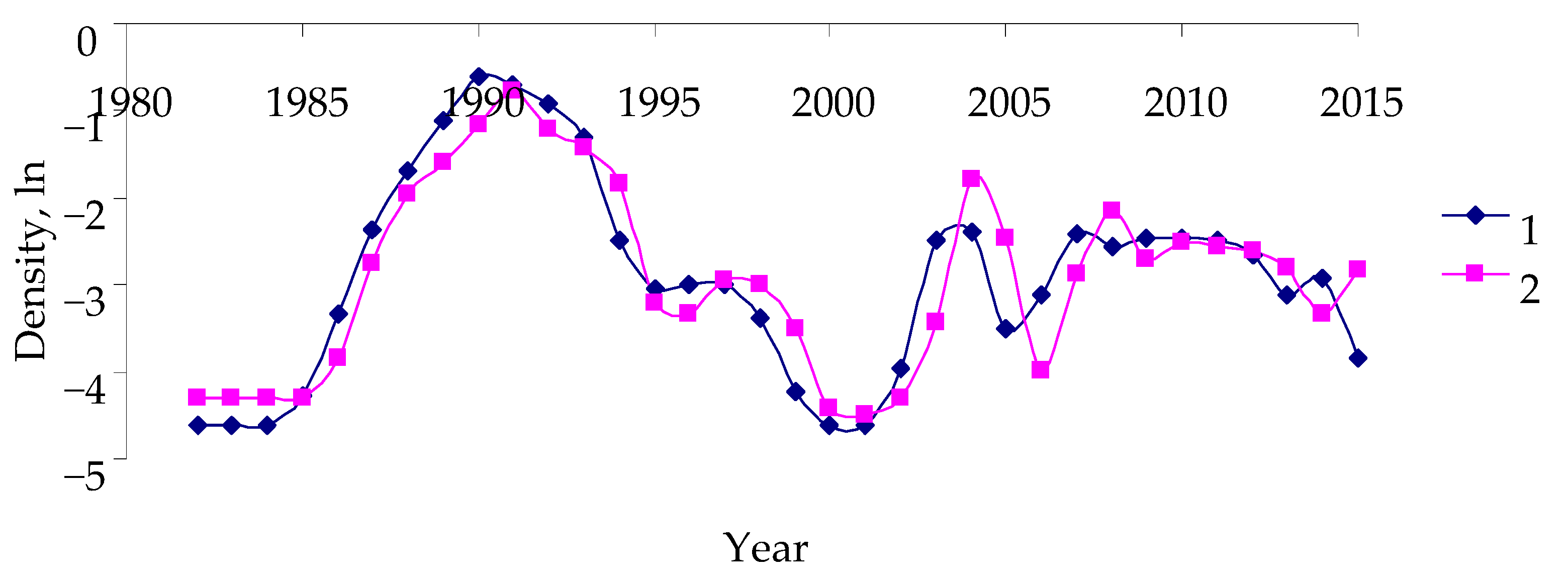
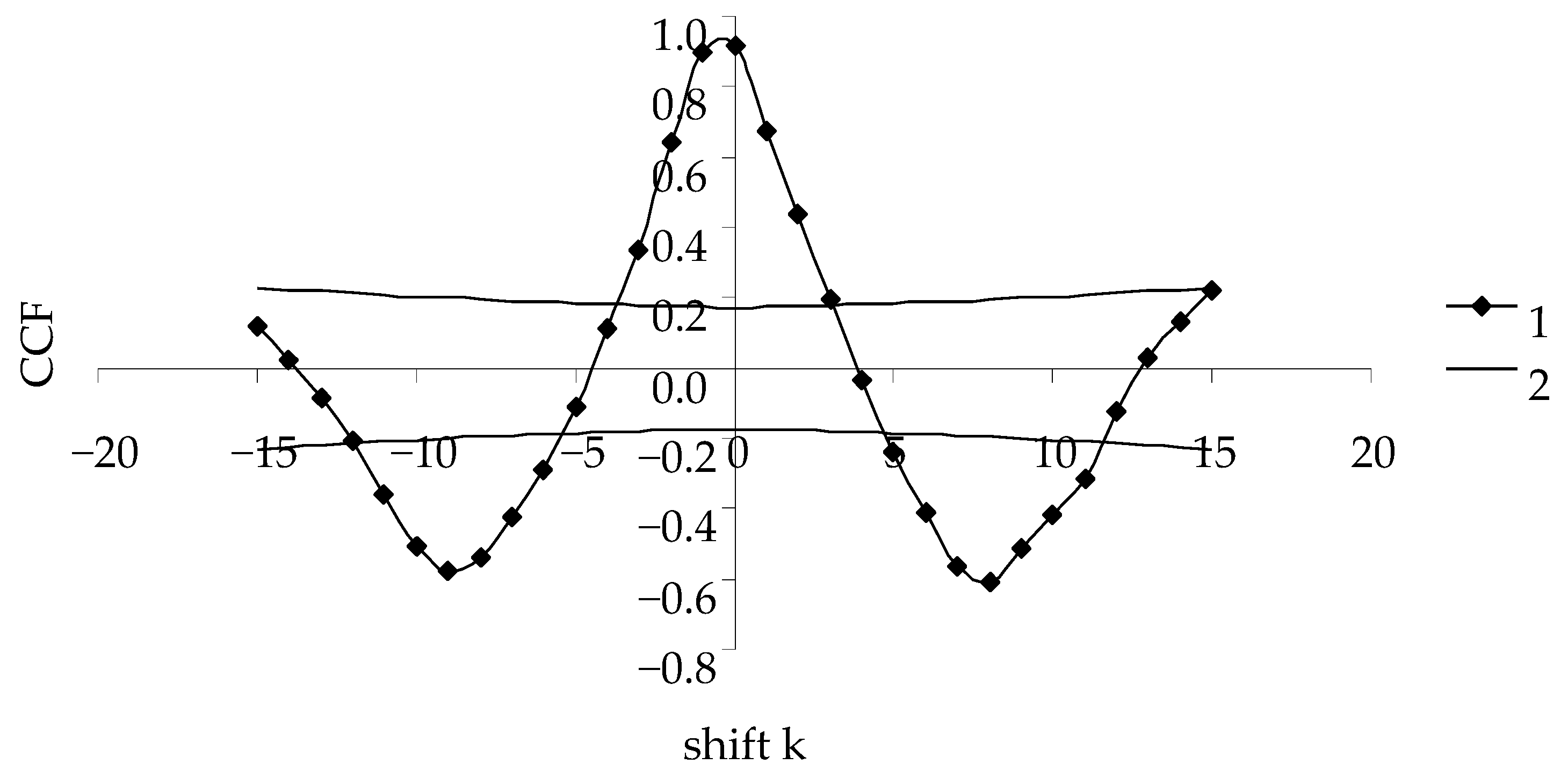

| Habitat | Habitat | ||||
|---|---|---|---|---|---|
| Plakor | Top | Dune | Terrace | Lake | |
| Plakor | 0.00 | 1.6 | 1.2 | 1.8 | 2.9 |
| Top | 0.00 | 2.3 | 2.1 | 3.8 | |
| Dune | 0.00 | 1.0 | 3.9 | ||
| Terrace | 0.00 | 4.7 | |||
| Habitat | Parameters | Species | ||||
|---|---|---|---|---|---|---|
| B. piniarius | S. liturata | G. virens | M. pallipes | D. pini | ||
| Top | Average density <N> | 0.72 | 0.09 | 0.14 | 0.10 | 0.16 |
| standart deviations | 1.96 | 0.14 | 0.22 | 0.15 | 0.28 | |
| maximal density Nmax | 10.88 | 0.55 | 1.00 | 0.60 | 1.30 | |
| Plakor | Average density <N> | 0.68 | 0.05 | 0.13 | 0.06 | 0.15 |
| standart deviations | 1.28 | 0.07 | 0.23 | 0.11 | 0.27 | |
| maximal density Nmax | 5.60 | 0.24 | 1.10 | 0.45 | 1.20 | |
| Lake | Average density <N> | 0.18 | 0.19 | 0.30 | 0.15 | 0.09 |
| standart deviations | 0.31 | 0.38 | 0.59 | 0.41 | 0.13 | |
| maximal density Nmax | 1.56 | 2.05 | 2.68 | 2.25 | 0.65 | |
| Terrace | Average density <N> | 0.11 | 0.17 | 0.14 | 0.04 | 0.38 |
| standart deviations | 0.18 | 0.30 | 0.31 | 0.06 | 0.77 | |
| maximal density Nmax | 0.76 | 1.56 | 1.36 | 0.24 | 3.08 | |
| Dune | Average density <N> | 1.46 | 0.16 | 0.20 | 0.05 | 0.33 |
| standart deviations | 3.39 | 0.25 | 0.25 | 0.08 | 0.64 | |
| maximal density Nmax | 13.51 | 1.32 | 0.92 | 0.40 | 2.96 | |
| Variables | Coefficient | Std.Err. | t(29) | p-Value |
|---|---|---|---|---|
| a0 | −0.565 | 0.235 | −2.406 | 0.022 |
| y(i-2) | −0.530 | 0.155 | −3.427 | 0.002 |
| y(i-1) | 1.339 | 0.159 | 8.410 | 0.000 |
| adjR2 | 0.820 | 0.000 | ||
| F | 74.400 | |||
| η | 0.08 |
| Species | Average Stability Margin η for a Species | Habitat | Average Stability Stability Margin η for a Habitat |
|---|---|---|---|
| B. piniaius | 0.13 | Top | 0.38 |
| S. liturata | 0.12 | Plakor | 0.21 |
| G. virens | 0.40 | Lake | 0.20 |
| M. pallipes | 0.46 | Terrace | 0.28 |
| D. pini | 0.12 | Dune | 0.16 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Soukhovolsky, V.; Ovchinnikova, T.; Tarasova, O.; Ivanova, Y.; Kovalev, A. Regulatory Processes in Populations of Forest Insects (A Case Study of Insect Species Damaging the Pine Pinus sylvestris L. in Forests of SIBERIA). Diversity 2022, 14, 1038. https://doi.org/10.3390/d14121038
Soukhovolsky V, Ovchinnikova T, Tarasova O, Ivanova Y, Kovalev A. Regulatory Processes in Populations of Forest Insects (A Case Study of Insect Species Damaging the Pine Pinus sylvestris L. in Forests of SIBERIA). Diversity. 2022; 14(12):1038. https://doi.org/10.3390/d14121038
Chicago/Turabian StyleSoukhovolsky, Vladislav, Tamara Ovchinnikova, Olga Tarasova, Yulia Ivanova, and Anton Kovalev. 2022. "Regulatory Processes in Populations of Forest Insects (A Case Study of Insect Species Damaging the Pine Pinus sylvestris L. in Forests of SIBERIA)" Diversity 14, no. 12: 1038. https://doi.org/10.3390/d14121038
APA StyleSoukhovolsky, V., Ovchinnikova, T., Tarasova, O., Ivanova, Y., & Kovalev, A. (2022). Regulatory Processes in Populations of Forest Insects (A Case Study of Insect Species Damaging the Pine Pinus sylvestris L. in Forests of SIBERIA). Diversity, 14(12), 1038. https://doi.org/10.3390/d14121038






