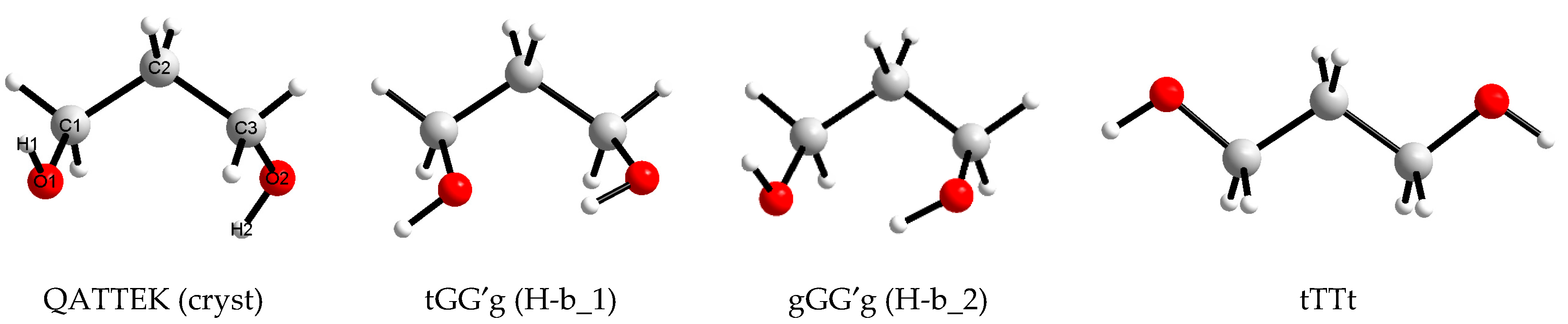
Figure 1.
Views and designations of 1,3-propanediol conformations used in this work to create initial boxes for molecular dynamics modeling.
Figure 1.
Views and designations of 1,3-propanediol conformations used in this work to create initial boxes for molecular dynamics modeling.
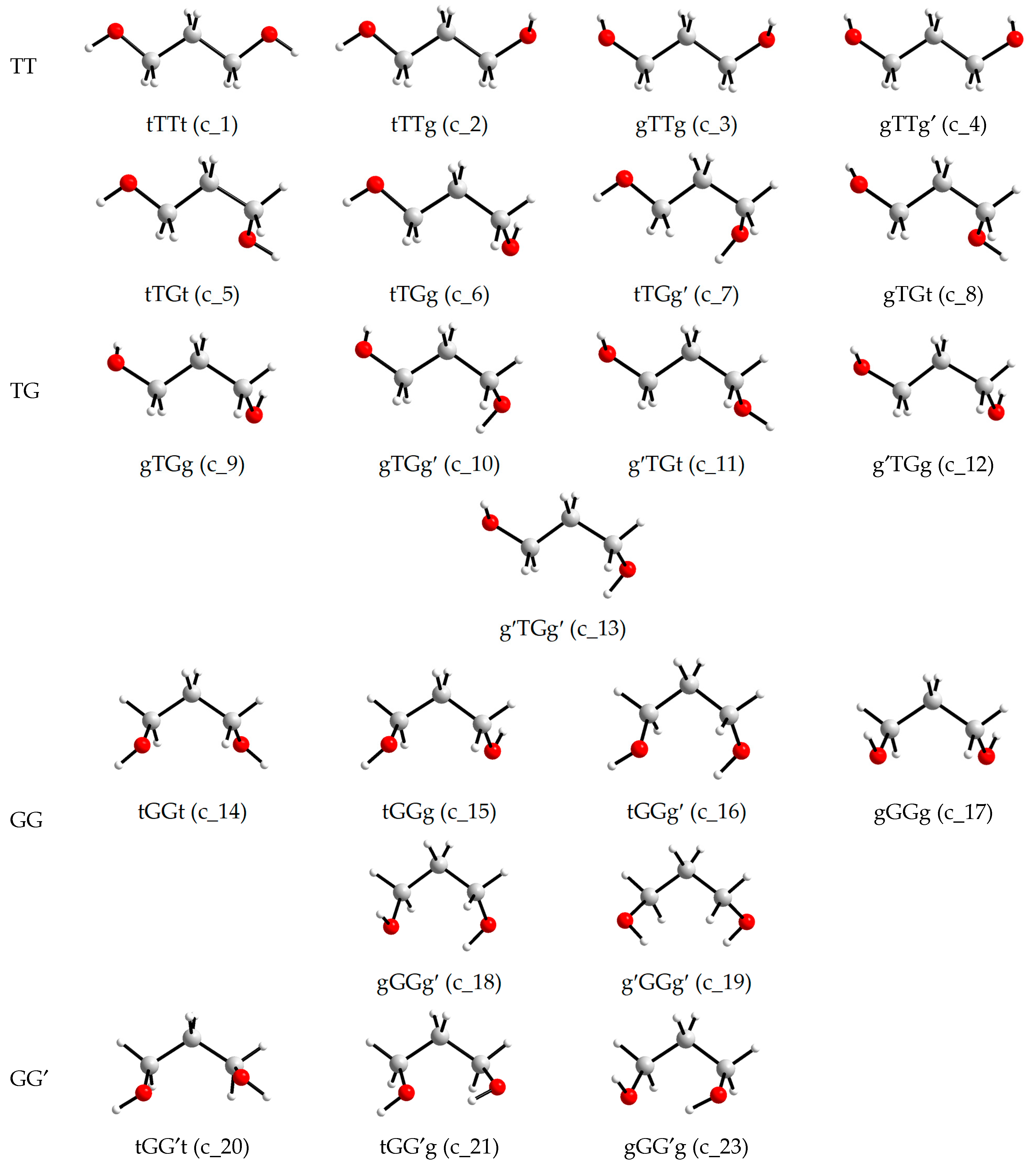
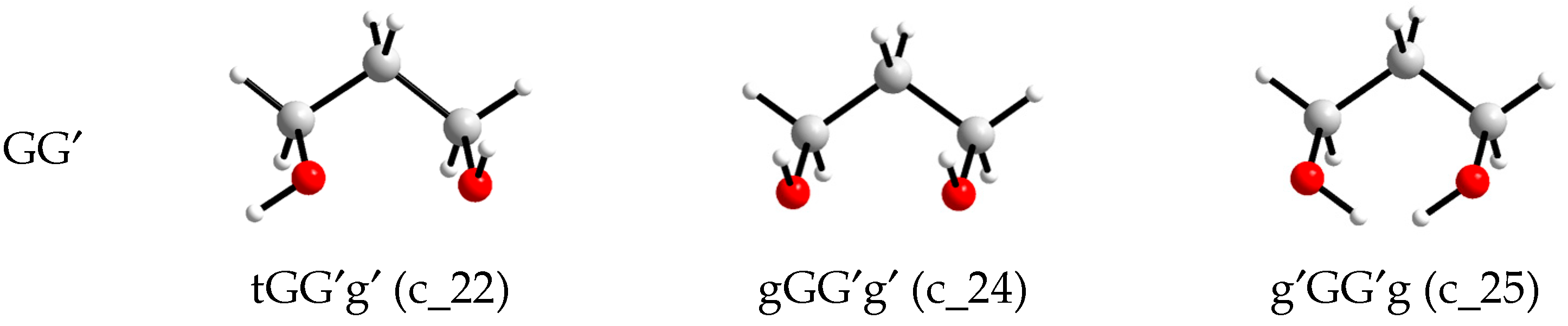
Figure 2.
Conformers of an individual 1,3-propanediol molecule (i.e., conformations corresponding to energy minima) according to the MP2/aug-cc-pVDZ or MP2/aug-cc-pVTZ optimizations.
Figure 2.
Conformers of an individual 1,3-propanediol molecule (i.e., conformations corresponding to energy minima) according to the MP2/aug-cc-pVDZ or MP2/aug-cc-pVTZ optimizations.
Figure 3.
Conformations of an individual 1,3-propanediol molecule that transform into conformers with other designations upon the MP2/aug-cc-pVDZ or MP2/aug-cc-pVTZ optimizations.
Figure 3.
Conformations of an individual 1,3-propanediol molecule that transform into conformers with other designations upon the MP2/aug-cc-pVDZ or MP2/aug-cc-pVTZ optimizations.
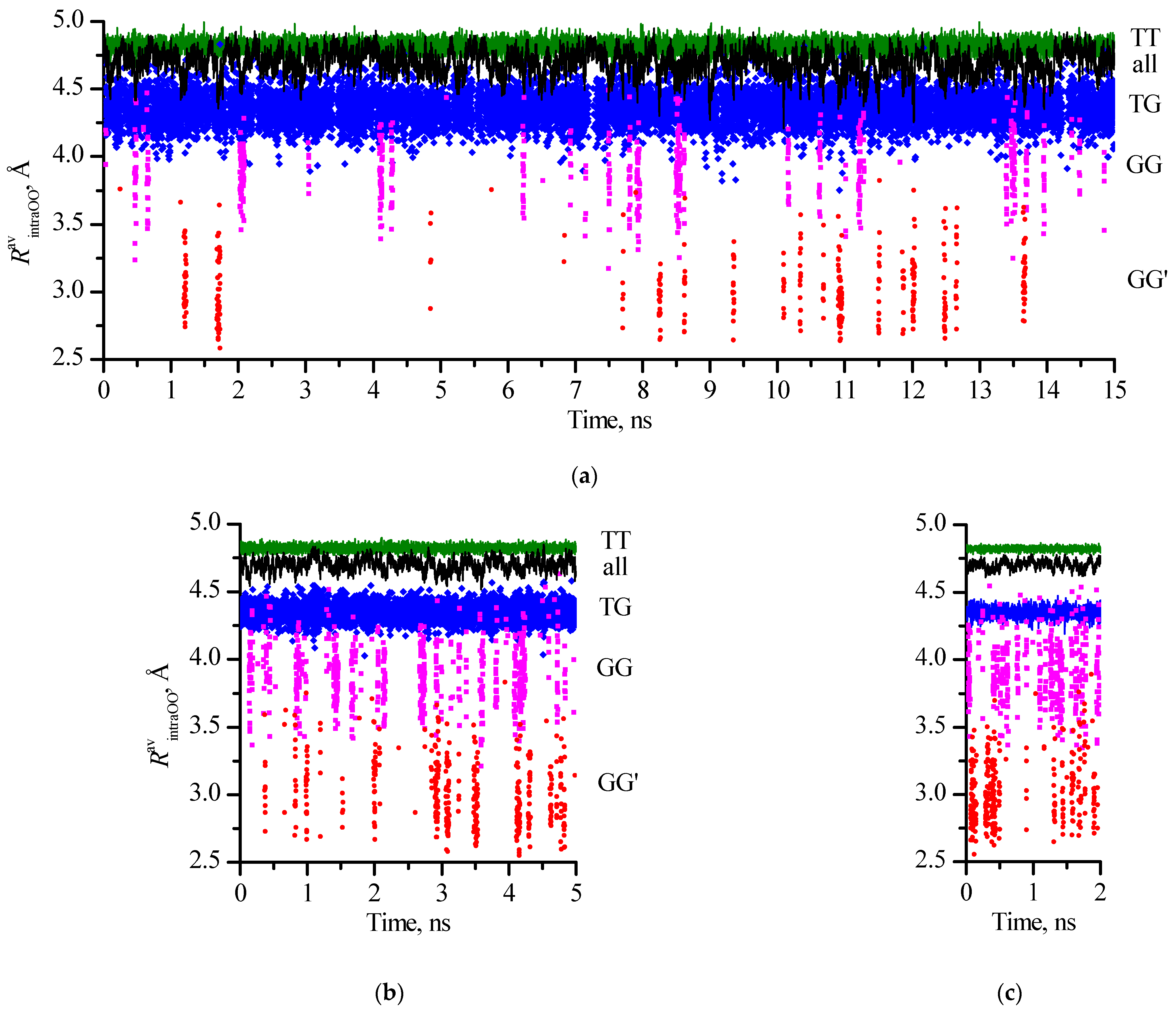
Figure 4.
Distributions of O…O intramolecular distances in 1,3-propanediol molecules along molecular dynamics trajectories (averaged at each time point over the number of molecules in the particular families or the whole systems) for different size systems: 800_tTTt (a), 2700_cryst (b), and 6400_cryst (c).
Figure 4.
Distributions of O…O intramolecular distances in 1,3-propanediol molecules along molecular dynamics trajectories (averaged at each time point over the number of molecules in the particular families or the whole systems) for different size systems: 800_tTTt (a), 2700_cryst (b), and 6400_cryst (c).
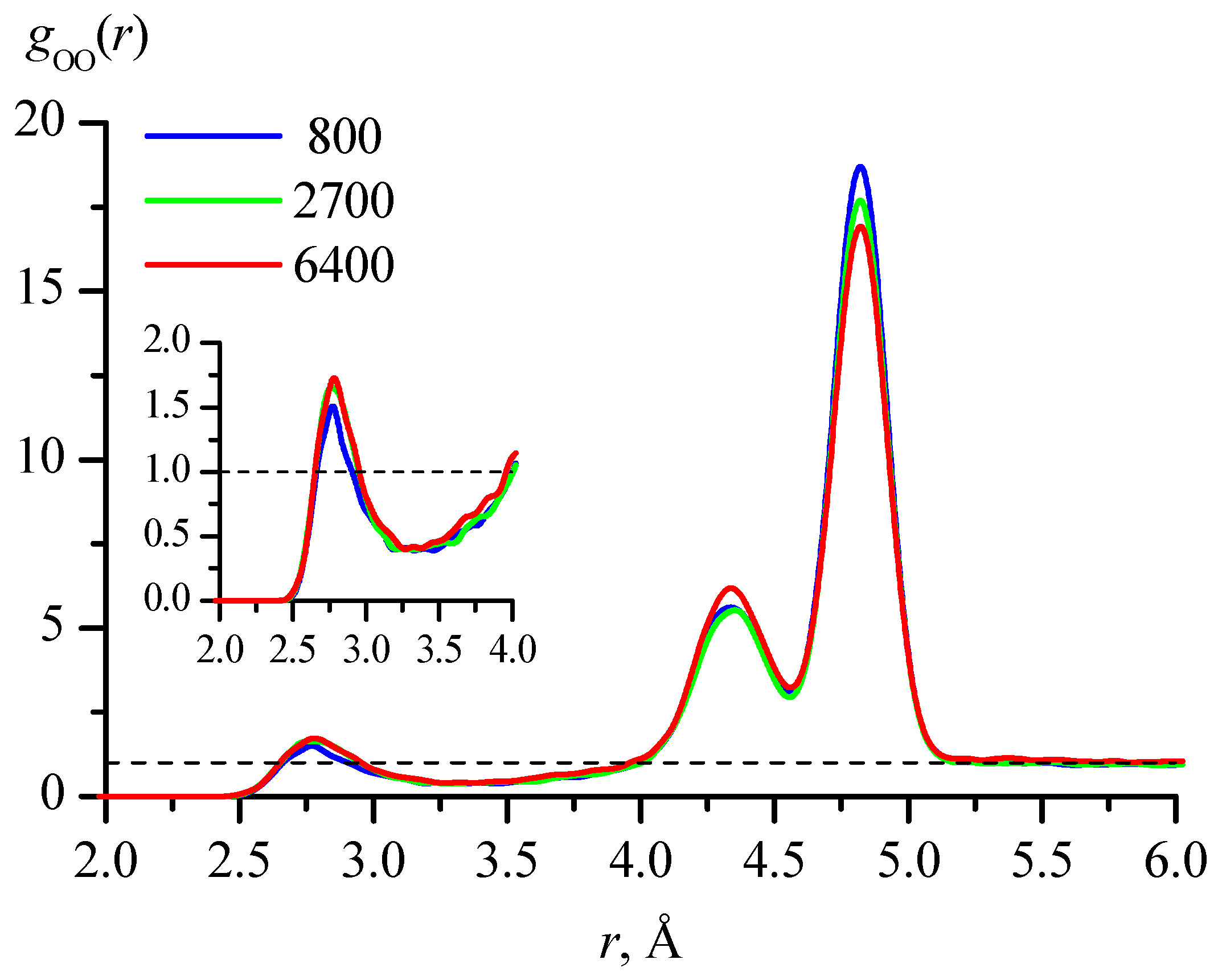
Figure 5.
Radial distribution functions gOO(r), where O are oxygen atoms in hydroxyl groups, calculated for the 800_tTTt, 2700_cryst, and 6400_cryst systems with averaging of values obtained with 1 ps time step for trajectories of 10, 4, and 1 ns, respectively. The inset shows a larger view of the region corresponding to the first peak.
Figure 5.
Radial distribution functions gOO(r), where O are oxygen atoms in hydroxyl groups, calculated for the 800_tTTt, 2700_cryst, and 6400_cryst systems with averaging of values obtained with 1 ps time step for trajectories of 10, 4, and 1 ns, respectively. The inset shows a larger view of the region corresponding to the first peak.
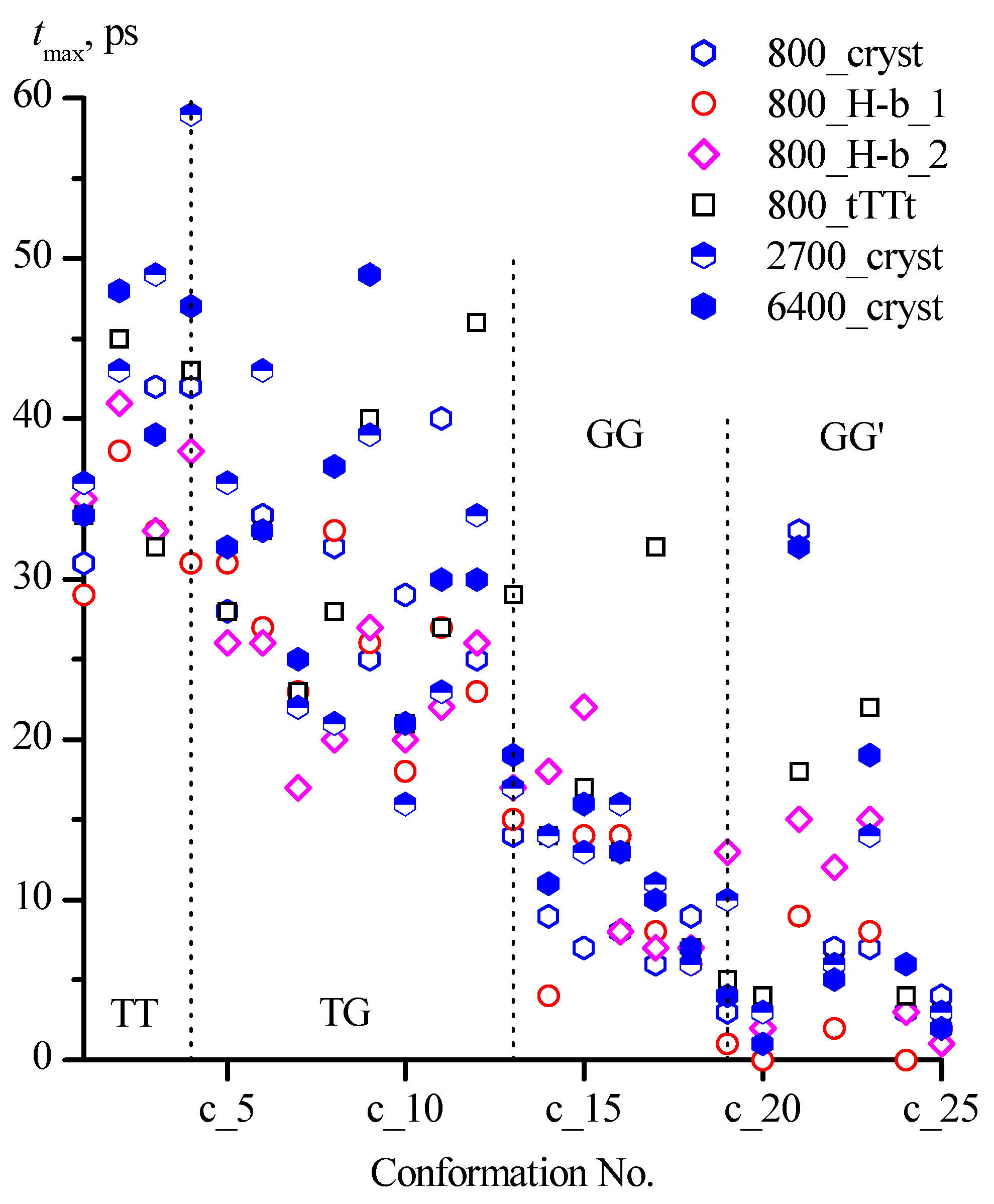
Figure 6.
Maximum lifetimes of 1,3-propanediol conformations with different designations in systems under consideration.
Figure 6.
Maximum lifetimes of 1,3-propanediol conformations with different designations in systems under consideration.
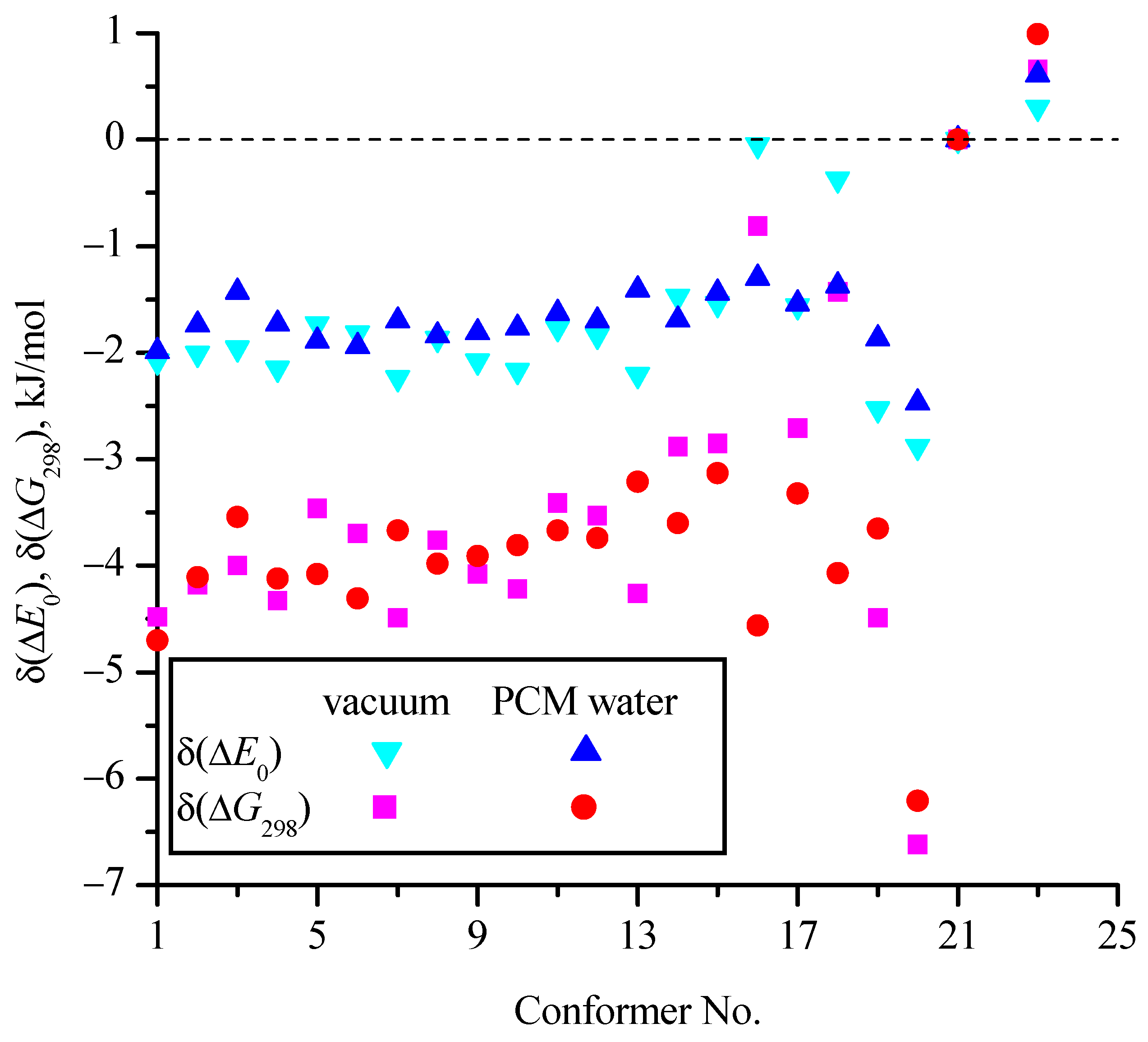
Figure 7.
Change in relative (compared to c_21) energies of conformers with the zero-point corrections (δ(ΔE0) = ΔE0 − ΔEel) and thermal corrections to Gibbs energy (δ(ΔG298) = ΔG298 − ΔEel) for calculations performed at the MP2/aug-cc-pVDZ level in vacuum and using the PCM model for an implicit aqueous environment.
Figure 7.
Change in relative (compared to c_21) energies of conformers with the zero-point corrections (δ(ΔE0) = ΔE0 − ΔEel) and thermal corrections to Gibbs energy (δ(ΔG298) = ΔG298 − ΔEel) for calculations performed at the MP2/aug-cc-pVDZ level in vacuum and using the PCM model for an implicit aqueous environment.
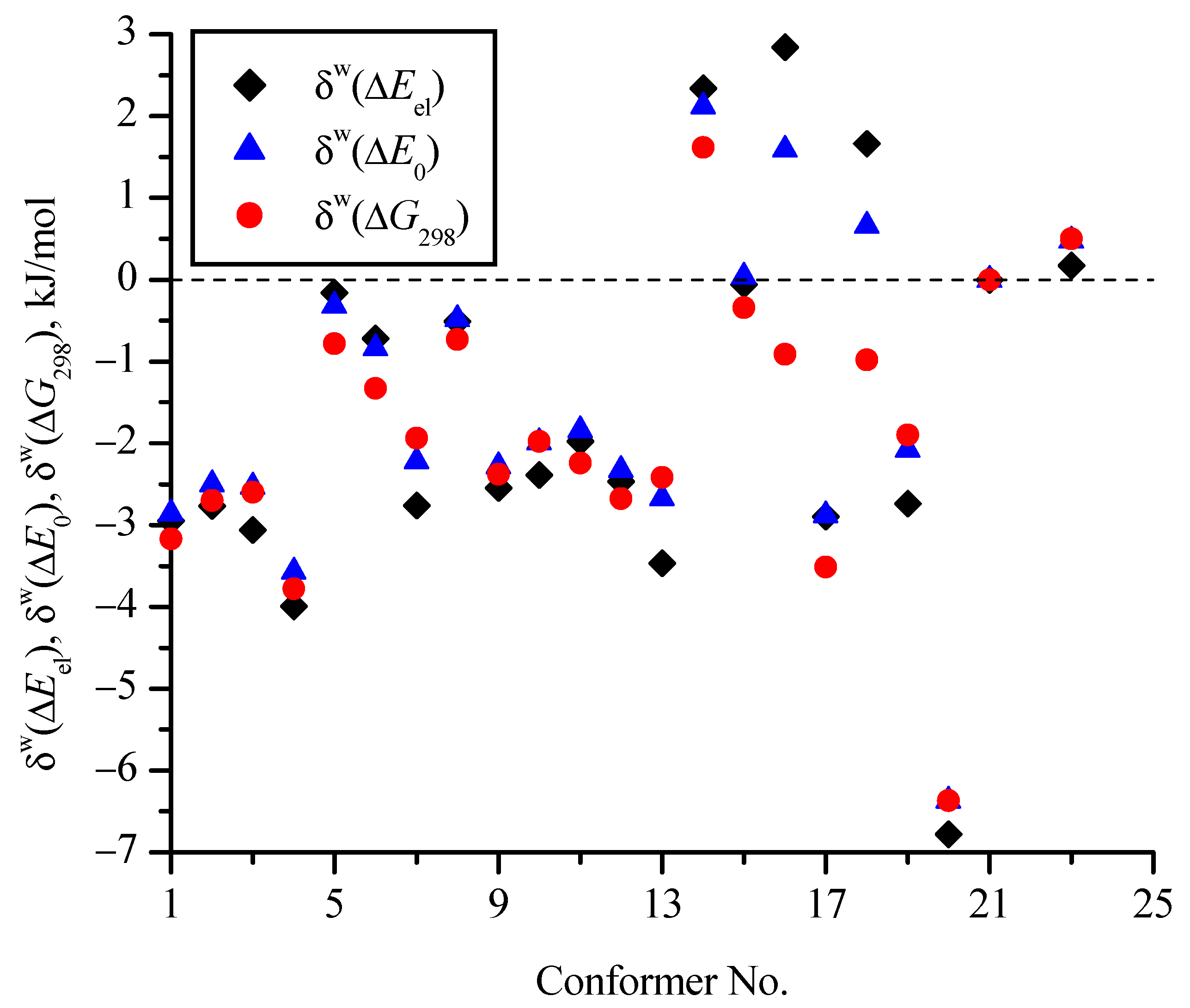
Figure 8.
Change in relative (compared to c_21) energies of conformers when using the PCM model for an implicit aqueous environment compared to calculations in vacuum (δw(ΔEel) = ΔEelw − ΔEelv and similarly for δw(ΔE0) and δw(ΔG298)) (all calculations at the MP2/aug-cc-pVDZ level).
Figure 8.
Change in relative (compared to c_21) energies of conformers when using the PCM model for an implicit aqueous environment compared to calculations in vacuum (δw(ΔEel) = ΔEelw − ΔEelv and similarly for δw(ΔE0) and δw(ΔG298)) (all calculations at the MP2/aug-cc-pVDZ level).
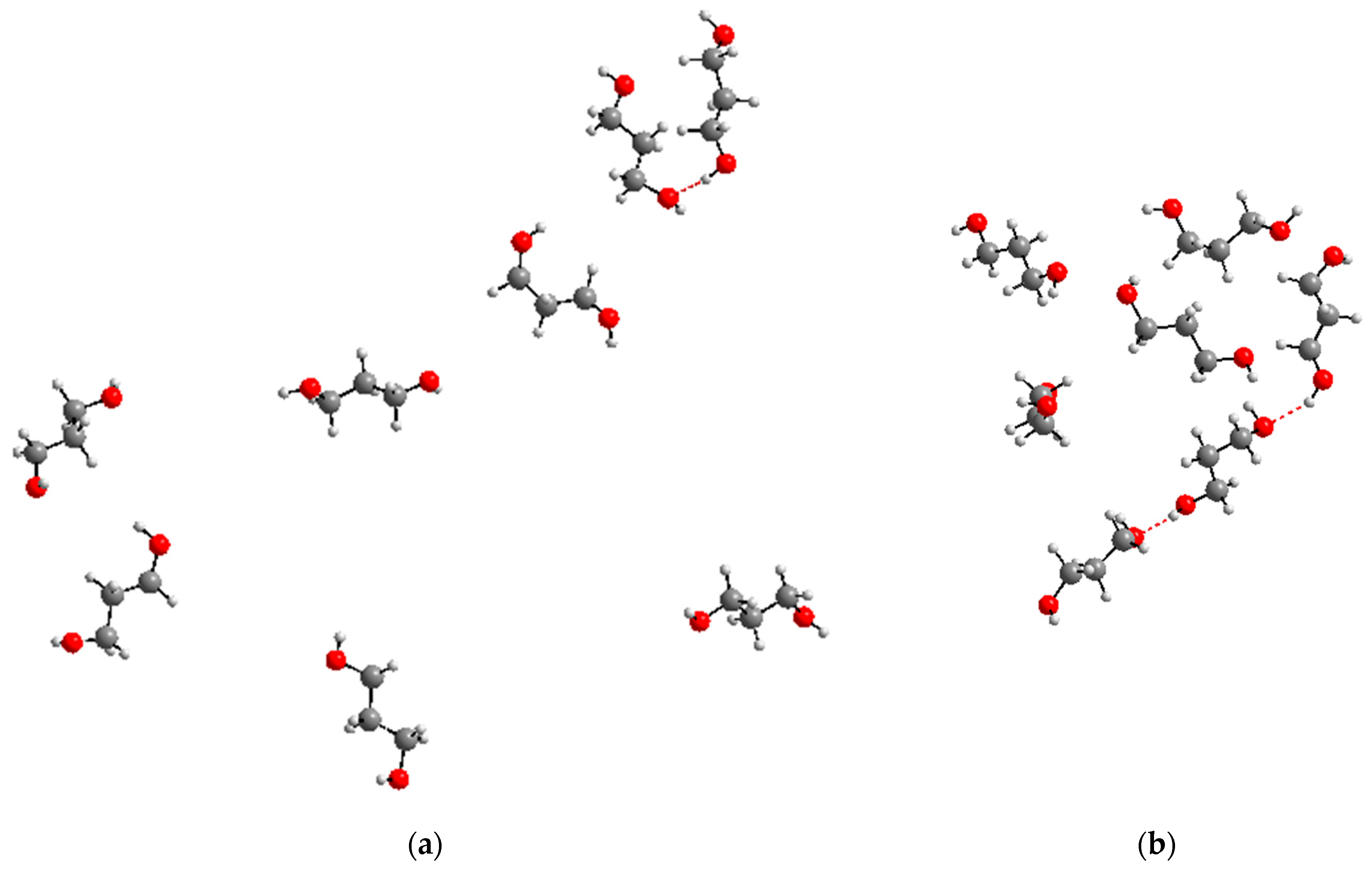
Figure 9.
Examples of the formation of intermolecular hydrogen bonds between 1,3-propanediol molecules (indicated by the red dashed line) in the systems under consideration: snapshot for the 800_tTTt system (a), part of the snapshot for the 2700_cryst system (b) (water molecules not shown for clarity).
Figure 9.
Examples of the formation of intermolecular hydrogen bonds between 1,3-propanediol molecules (indicated by the red dashed line) in the systems under consideration: snapshot for the 800_tTTt system (a), part of the snapshot for the 2700_cryst system (b) (water molecules not shown for clarity).
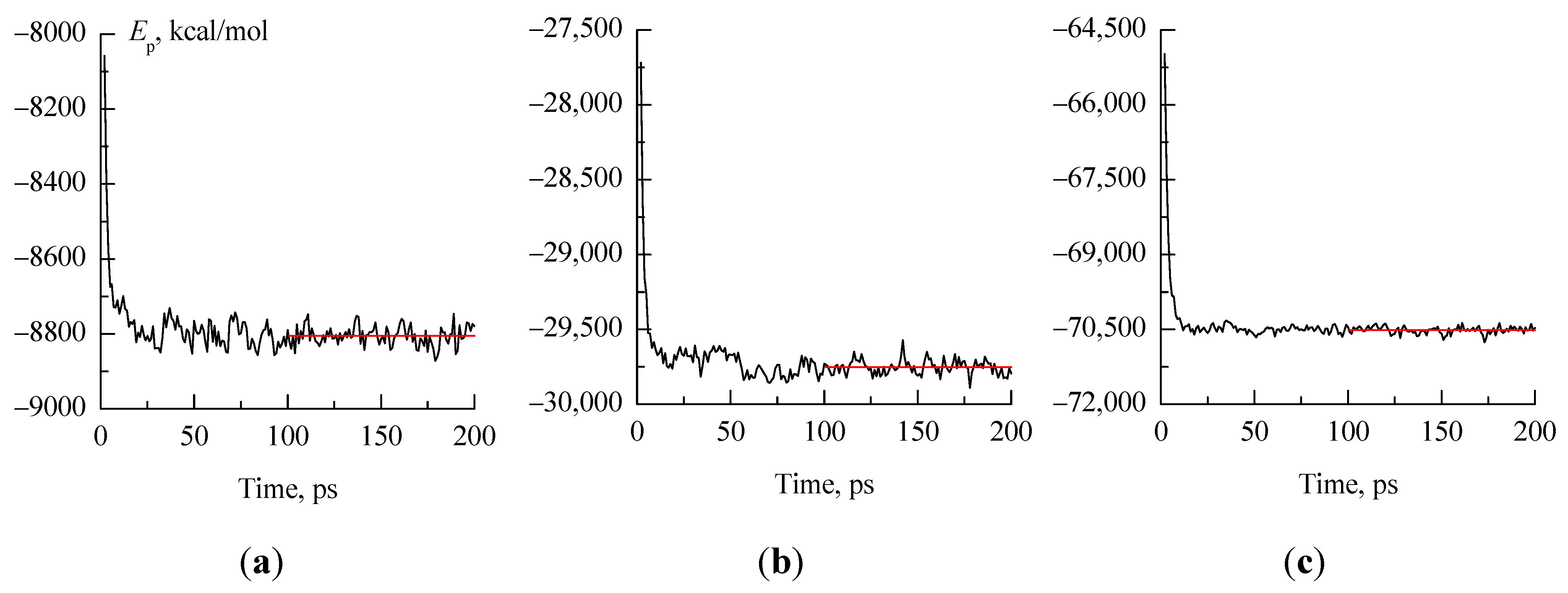
Figure 10.
Potential energy values (Ep, kcal/mol) for systems 800_H-b_1 (a), 2700_cryst (b), 6400_cryst (c) in the first 200 ps after the start of molecular dynamics simulations. The red lines demonstrate the stability of the mean values of Ep after 100 ps.
Figure 10.
Potential energy values (Ep, kcal/mol) for systems 800_H-b_1 (a), 2700_cryst (b), 6400_cryst (c) in the first 200 ps after the start of molecular dynamics simulations. The red lines demonstrate the stability of the mean values of Ep after 100 ps.
Table 1.
Relative energies (ΔEel, ΔE0 and ΔG298 in kJ/mol) for 1,3-propanediol conformers optimized from designated conformations in vacuum and in implicit water using PCM model.
Table 1.
Relative energies (ΔEel, ΔE0 and ΔG298 in kJ/mol) for 1,3-propanediol conformers optimized from designated conformations in vacuum and in implicit water using PCM model.
| Conformation * | In Vacuum | In Water (PCM Model) |
|---|
| MP2/aug-cc-pVDZ | MP2/aug-cc-pVTZ | MP2/aug-cc-pVDZ |
|---|
| ΔEel | ΔE0 | ΔG298 | ΔEel | ΔE0 | ΔG298 | ΔEel | ΔE0 | ΔG298 |
|---|
| tTTt (c_1) | 13.25 | 11.17 | 8.77 | 13.39 | 11.07 | 8.68 | 10.30 | 8.31 | 5.60 |
| tTTg (c_2) | 13.25 | 11.24 | 9.07 | 13.27 | 11.12 | 8.92 | 10.48 | 8.74 | 6.37 |
| gTTg (c_3) | 13.70 | 11.74 | 9.70 | 13.60 | 11.61 | 9.57 | 10.64 | 9.21 | 7.10 |
| gTTg′ (c_4) | 14.81 | 12.66 | 10.48 | 14.72 | 12.52 | 10.31 | 10.82 | 9.09 | 6.70 |
| tTGt (c_5) | 8.69 | 6.96 | 5.23 | 9.06 | 7.10 | 5.24 | 8.53 | 6.64 | 4.45 |
| tTGg (c_6) | 9.66 | 7.84 | 5.96 | 9.89 | 7.91 | 6.00 | 8.94 | 7.00 | 4.63 |
| tTGg′ (c_7) | 13.36 | 11.12 | 8.87 | 13.43 | 11.18 | 8.99 | 10.60 | 8.90 | 6.93 |
| gTGt (c_8) | 9.43 | 7.56 | 5.67 | 9.66 | 7.69 | 5.72 | 8.92 | 7.08 | 4.94 |
| gTGg (c_9) | 11.98 | 9.90 | 7.90 | 12.12 | 9.96 | 7.89 | 9.43 | 7.62 | 5.52 |
| gTGg′ (c_10) | 13.46 | 11.29 | 9.24 | 13.42 | 11.32 | 9.31 | 11.07 | 9.30 | 7.26 |
| g′TGt (c_11) | 10.39 | 8.62 | 6.98 | 10.65 | 8.74 | 7.02 | 8.41 | 6.78 | 4.74 |
| g′TGg (c_12) | 11.29 | 9.45 | 7.76 | 11.41 | 9.52 | 7.82 | 8.82 | 7.12 | 5.08 |
| g′TGg′ (c_13) | 14.26 | 12.05 | 10.00 | 14.16 | 12.04 | 10.04 | 10.79 | 9.38 | 7.58 |
| tGGt (c_14) | 4.64 | 3.17 | 1.76 | 5.13 | 3.44 | 1.81 | 6.98 | 5.29 | 3.38 |
| tGGg (c_15) | 7.06 | 5.52 | 4.21 | 7.47 | 5.74 | 4.25 | 7.00 | 5.56 | 3.87 |
| tGGg′ (c_16) | 4.85 | 4.80 | 4.04 | 5.29 | 5.06 | 4.01 | 7.69 | 6.39 | 3.13 |
| gGGg (c_17) | 10.07 | 8.51 | 7.36 | 10.44 | 8.75 | 7.49 | 7.17 | 5.63 | 3.85 |
| gGGg′ (c_18) | 6.28 | 5.91 | 4.85 | 6.71 | 6.17 | 4.79 | 7.94 | 6.57 | 3.87 |
| g′GGg′ (c_19) | 13.77 | 11.24 | 9.28 | 13.67 | 11.45 | 9.66 | 11.03 | 9.16 | 7.38 |
| tGG′t (c_20) | 23.54 | 20.66 | 16.92 | 23.83 | 20.83 | 17.01 | 16.76 | 14.29 | 10.55 |
| tGG′g (c_21) | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| tGG′g′ (c_22) | transforms to c_21 | transforms to c_21 | transforms to c_21 |
| gGG′g (c_23) | 0.82 | 1.12 | 1.48 | 0.99 | 1.28 | 1.62 | 0.99 | 1.60 | 1.98 |
| gGG′g′ (c_24) | transforms to c_23 | transforms to c_23 | transforms to c_23 |
| g′GG′g (c_25) | transforms to c_21 | transforms to c_21 | transforms to c_21 |
Table 2.
Some geometric parameters for the 1,3-propanediol molecule in the crystal (QATTEK) and the gGGg′ conformer optimized by MP2/aug-cc-pVDZ and MP2/aug-cc-pVTZ methods.
Table 2.
Some geometric parameters for the 1,3-propanediol molecule in the crystal (QATTEK) and the gGGg′ conformer optimized by MP2/aug-cc-pVDZ and MP2/aug-cc-pVTZ methods.
| Parameters | QATTEK | MP2/aug-cc-pVDZ | MP2/aug-cc-pVTZ |
|---|
| H1O1C1C2, ° | 67.6 | 65.2 | 65.3 |
| O1C1C2C3, ° | 61.5 | 46.2 | 47.1 |
| C1C2C3O2, ° | 69.4 | 49.1 | 50.3 |
| C2C3O2H2, ° | −82.5 | −76.0 | −76.4 |
| O1…O2, Å | 3.72 | 3.14 | 3.16 |
| O1…H2–O2, ° | 105.6 | 118.9 | 117.9 |
Table 3.
Main parameters of considered systems: short notation, the total number of molecules in boxes with periodic boundary conditions (N), number of 1,3-propanediol molecules (N13PD), the initial conformation of 1,3-propanediol, simulation time after equilibration (t), number of 1,3-propanediol conformations under consideration (Nconf).
Table 3.
Main parameters of considered systems: short notation, the total number of molecules in boxes with periodic boundary conditions (N), number of 1,3-propanediol molecules (N13PD), the initial conformation of 1,3-propanediol, simulation time after equilibration (t), number of 1,3-propanediol conformations under consideration (Nconf).
| System Notation | N | N13PD | Initial Conformation of 13PD | t, ns | Nconf |
|---|
| 800_cryst | 800 | 8 | QATTEK (crystal variant of gGGg′) | 5 | 40,000 |
| 800_H-b_1 | 800 | 8 | tGG′g (opt_MP2/aug-cc-pVDZ) | 5 | 40,000 |
| 800_H-b_2 | 800 | 8 | gGG′g (opt_MP2/aug-cc-pVDZ) | 5 | 40,000 |
| 800_tTTt | 800 | 8 | tTTt (opt_MP2/aug-cc-pVDZ) | 15 | 120,000 |
| 2700_cryst | 2700 | 27 | QATTEK (crystal variant of gGGg′) | 5 | 135,000 |
| 6400_cryst | 6400 | 64 | QATTEK (crystal variant of gGGg′) | 2 | 128,000 |
Table 4.
Calculated and experimental density values for 1 mol. % 1,3-propanediol aqueous solution at 298.15 K and atmospheric pressure.
Table 4.
Calculated and experimental density values for 1 mol. % 1,3-propanediol aqueous solution at 298.15 K and atmospheric pressure.
| System | Trajectory Length, ns | Step, ps | Density, g/cm3 |
|---|
| 800_cryst | 5 | 1 | 0.9978 ± 1 × 10−4 |
| 800_H-b_1 | 5 | 1 | 0.9979 ± 1 × 10−4 |
| 800_H-b_2 | 5 | 1 | 0.9978 ± 9 × 10−5 |
| 800_tTTt | 15 | 1/0.1 | 0.9979 ± 5 × 10−5/2 × 10−5 |
| 2700_cryst | 5 | 1/0.1 | 0.9977 ± 5 × 10−5/2 × 10−5 |
| 6400_cryst | 2 | 1/0.1 | 0.9977 ± 5 × 10−5/2 × 10−5 |
| Experiment (this work) | | | 0.9994 ± 3 × 10−5 |
| Experiment [27] (x13PD = 1.03 mol. %) | | | 0.9999 ± 3 × 10−6 |
Table 5.
Contents (φ, % of the numbers of all conformations during simulations) of 1,3-propanediol conformations with different designations and their average lifetimes (tav, ps *) in systems under consideration.
Table 5.
Contents (φ, % of the numbers of all conformations during simulations) of 1,3-propanediol conformations with different designations and their average lifetimes (tav, ps *) in systems under consideration.
| Conformation | Family | 800_cryst | 800_H-b_1 | 800_H-b_2 | 800_tTTt | 2700_cryst | 6400_cryst |
|---|
| φ | tav | φ | tav | φ | tav | φ | tav | φ | tav | φ | tav |
|---|
| tTTt (c_1) | TT | 15.5 | 3.6 | 16.1 | 3.6 | 15.1 | 3.6 | 15.2 ** | 3.5 | 15.6 | 3.5 | 15.5 | 3.6 |
| tTTg (c_2) | 36.8 | 3.5 | 38.7 | 3.5 | 37.2 | 3.6 | 37.9 | 3.6 | 38.0 | 3.5 | 37.8 | 3.5 |
| gTTg (c_3) | 11.1 | 3.6 | 11.9 | 3.5 | 11.1 | 3.6 | 11.1 | 3.4 | 11.3 | 3.4 | 11.6 | 3.6 |
| gTTg′ (c_4) | 10.5 | 3.5 | 11.1 | 3.4 | 10.5 | 3.6 | 10.5 | 3.4 | 10.7 | 3.4 | 11.0 | 3.5 |
| tTGt (c_5) | TG | 5.2 | 3.2 | 4.7 | 3.5 | 5.3 | 3.3 | 5.0 | 3.4 | 5.1 | 3.6 | 5.4 | 3.6 |
| tTGg (c_6) | 3.6 | 4.3 | 4.0 | 4.3 | 4.0 | 3.9 | 4.0 | 4.0 | 3.8 | 4.2 | 3.6 | 3.9 |
| tTGg′ (c_7) | 2.2 | 2.7 | 1.7 | 2.8 | 1.9 | 2.8 | 1.8 | 2.8 | 1.8 | 2.8 | 2.0 | 2.7 |
| gTGt (c_8) | 3.7 | 3.5 | 2.7 | 3.5 | 3.0 | 3.3 | 3.3 | 3.5 | 3.2 | 3.5 | 3.2 | 3.4 |
| gTGg (c_9) | 2.0 | 4.0 | 1.8 | 3.8 | 2.4 | 4.7 | 2.2 | 3.8 | 2.4 | 4.3 | 2.2 | 4.0 |
| gTGg′ (c_10) | 1.3 | 2.7 | 0.9 | 2.5 | 1.1 | 2.8 | 1.1 | 2.5 | 1.0 | 2.6 | 1.2 | 2.6 |
| g′TGt (c_11) | 3.5 | 3.5 | 2.6 | 3.3 | 3.3 | 3.5 | 3.2 | 3.3 | 2.9 | 3.3 | 2.7 | 3.1 |
| g′TGg (c_12) | 2.4 | 4.0 | 2.3 | 4.0 | 2.7 | 3.9 | 2.6 | 4.0 | 2.1 | 3.6 | 2.1 | 3.7 |
| g′TGg′ (c_13) | 1.0 | 2.3 | 0.8 | 2.3 | 1.0 | 2.5 | 1.2 | 2.7 | 1.0 | 2.8 | 1.0 | 2.6 |
| tGGt (c_14) | GG | 0.1 | 2.9 | 0.05 | 1.7 | 0.1 | 2.9 | 0.1 | 3.4 | 0.1 | 3.1 | 0.1 | 2.8 |
| tGGg (c_15) | 0.1 | 2.6 | 0.2 | 3.0 | 0.4 | 4.6 | 0.1 | 3.5 | 0.1 | 3.9 | 0.1 | 3.1 |
| tGGg′ (c_16) | 0.1 | 2.4 | 0.1 | 3.2 | 0.1 | 2.6 | 0.1 | 3.0 | 0.1 | 3.0 | 0.1 | 2.8 |
| gGGg (c_17) | 0.04 | 4.5 | 0.04 | 7.0 | 0.1 | 2.5 | 0.1 | 4.7 | 0.02 | 2.9 | 0.03 | 2.7 |
| gGGg′ (c_18) | 0.05 | 2.5 | 0.1 | 2.6 | 0.1 | 3.3 | 0.1 | 2.3 | 0.1 | 2.3 | 0.05 | 2.9 |
| g′GGg′ (c_19) | 0.02 | 1.8 | 0.002 | 1 | 0.05 | 5.0 | 0.01 | 1.9 | 0.02 | 2.1 | 0.005 | 2.3 |
| tGG′t (c_20) | GG′ | 0.01 | 2.0 | 0 | 0 | 0.005 | 2 | 0.01 | 3.0 | 0.01 | 1.4 | 0.004 | 1.0 |
| tGG′g (c_21) | 0.3 | 4.0 | 0.05 | 4.0 | 0.1 | 4.3 | 0.2 | 4.8 | 0.2 | 6.2 | 0.1 | 5.8 |
| tGG′g′ (c_22) | 0.1 | 2.3 | 0.01 | 1.5 | 0.03 | 6.5 | 0.03 | 2.0 | 0.02 | 1.4 | 0.01 | 1.5 |
| gGG′g (c_23) | 0.1 | 3.3 | 0.04 | 2.3 | 0.1 | 3.1 | 0.1 | 4.6 | 0.1 | 4.1 | 0.1 | 3.7 |
| gGG′g′ (c_24) | 0.02 | 1.5 | 0 | 0 | 0.01 | 1.7 | 0.01 | 1.8 | 0.01 | 2.0 | 0.01 | 1.5 |
| g′GG′g (c_25) | 0.03 | 1.5 | 0.01 | 1.5 | 0.005 | 1.0 | 0.01 | 1.4 | 0.01 | 1.4 | 0.01 | 1.1 |
Table 6.
Maximum lifetimes (tmax, ps) of 1,3-propanediol conformations with different designations in systems under consideration (data for conformations that were the starting points in particular systems are in italics).
Table 6.
Maximum lifetimes (tmax, ps) of 1,3-propanediol conformations with different designations in systems under consideration (data for conformations that were the starting points in particular systems are in italics).
| Conformation | Family | 800_cryst | 800_H-b_1 | 800_H-b_2 | 800_tTTt | 2700_cryst | 6400_cryst |
|---|
| tTTt (c_1) | TT | 31 | 29 | 35 | 34 | 36 | 34 |
| tTTg (c_2) | 48 | 38 | 41 | 45 | 43 | 48 |
| gTTg (c_3) | 42 | 33 | 33 | 32 | 49 | 39 |
| gTTg′ (c_4) | 42 | 31 | 38 | 43 | 59 | 47 |
| tTGt (c_5) | TG | 28 | 31 | 26 | 28 | 36 | 32 |
| tTGg (c_6) | 34 | 27 | 26 | 33 | 43 | 33 |
| tTGg′ (c_7) | 22 | 23 | 17 | 23 | 22 | 25 |
| gTGt (c_8) | 32 | 33 | 20 | 28 | 21 | 37 |
| gTGg (c_9) | 25 | 26 | 27 | 40 | 39 | 49 |
| gTGg′ (c_10) | 29 | 18 | 20 | 21 | 16 | 21 |
| g′TGt (c_11) | 40 | 27 | 22 | 27 | 23 | 30 |
| g′TGg (c_12) | 25 | 23 | 26 | 46 | 34 | 30 |
| g′TGg′ (c_13) | 14 | 15 | 17 | 29 | 17 | 19 |
| tGGt (c_14) | GG | 9 | 4 | 18 | 14 | 14 | 11 |
| tGGg (c_15) | 7 | 14 | 22 | 17 | 13 | 16 |
| tGGg′ (c_16) | 8 | 14 | 8 | 13 | 16 | 13 |
| gGGg (c_17) | 6 | 8 | 7 | 32 | 11 | 10 |
| gGGg′ (c_18) | 9 | 6 | 7 | 7 | 6 | 7 |
| g′GGg′ (c_19) | 3 | 1 | 13 | 5 | 10 | 4 |
| tGG′t (c_20) | GG′ | 3 | 0 | 2 | 4 | 3 | 1 |
| tGG′g (c_21) | 33 | 9 | 15 | 18 | 32 | 32 |
| tGG′g′ (c_22) | 7 | 2 | 12 | 6 | 6 | 5 |
| gGG′g (c_23) | 7 | 8 | 15 | 22 | 14 | 19 |
| gGG′g′ (c_24) | 3 | 0 | 3 | 4 | 6 | 6 |
| g′GG′g (c_25) | 4 | 2 | 1 | 3 | 3 | 2 |
Table 7.
Average lifetimes (ps) for the conformation families of 1,3-propanediol.
Table 7.
Average lifetimes (ps) for the conformation families of 1,3-propanediol.
| Family | 800_cryst | 800_H-b_1 | 800_H-b_2 | 800_tTTt | 2700_cryst | 6400_cryst |
|---|
| TT | 103 | 124 | 116 | 110 | 108 | 110 |
| TG | 32 | 32 | 36 | 33 | 31 | 32 |
| GG | 13 | 11 | 22 | 16 | 13 | 12 |
| GG′ | 12 | 7 | 16 | 14 | 12 | 11 |