Next Article in Journal
Tuning Solvation Dynamics of Electrolytes at Their Eutectic Point Through Halide Identity
Previous Article in Journal
Catalytic Methanation over Natural Clay-Supported Nickel Catalysts
Previous Article in Special Issue
Intramolecular Versus Intermolecular Diels–Alder Reactions: Insights from Molecular Electron Density Theory
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Molecules
Volume 30
Issue 10
10.3390/molecules30102112
molecules-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Quantum Chemical Studies on the Prototropic and Acid/Base Equilibria for 2-Aminopyrrole in Vacuo—Role of CH Tautomers in the Design of Strong Brønsted Imino N-Bases

by
Ewa Daniela Raczyńska
1,*,
Pierre-Charles Maria
2 and
Jean-François Gal
2
1
Department of Chemistry, Warsaw University of Life Sciences (SGGW), ul. Nowoursynowska 159c, 02-776 Warsaw, Poland
2
Institut de Chimie de Nice, University Côte d’Azur, UMR 7272 CNRS, Parc Valrose, 06108 Nice, France
*
Author to whom correspondence should be addressed.
Molecules 2025, 30(10), 2112; https://doi.org/10.3390/molecules30102112
Submission received: 16 April 2025 / Revised: 6 May 2025 / Accepted: 7 May 2025 / Published: 9 May 2025
(This article belongs to the Special Issue Quantum Chemical Calculations of Molecular Reaction Processes)
Browse Figures
Versions Notes

Abstract

:
In the quest of the pivotal origin of the very strong gas-phase proton basicity for some iminopyrrole derivatives, proposed in the literature on the basis of quantum chemical calculations, the full tautomeric and acid/base equilibria were investigated in vacuo for 2-aminopyrrole exhibiting enamino–imino tautomerism. Thermochemistry of these processes investigated at the Density Functional Theory (DFT) level indicates a lower stability for the imino than for the enamino tautomers. However, the imino N atom in the imino forms displays an exceptionally high basicity, particularly in the minor and rare tautomers containing at least one tautomeric proton at the pyrrole C atom. This explains why derivatives of CH tautomers (being free of prototropy) display exceptionally high gas-phase proton basicity. As predicted by the Maksić group using quantum chemical methods, these derivatives can be considered as good organic imino N-superbase candidates. Unfortunately, some other structures of iminopyrrole derivatives (proposed by the same group) possess labile protons, and, thus, exhibit prototropy, resulting in the transformation into the more stable but less basic aminopyrrole derivatives under synthesis conditions or acid/base equilibria measurements.

1. Introduction

Organic compounds containing the five-membered ring made up of four C-sp2 and one N atom, called the pyrrole system, have drawn particular attention of chemists, biochemists, microbiologists, and pharmacologists because of their presence in many biologically active structures (see for example Refs. [1,2,3,4,5,6,7,8]). Pyrrole itself is not found in living organisms, but its cyclic subunit can be found in the skeleton of numerous molecules found in living organisms. For example, it is present in bicyclic 1H-indole (2,3-benzopyrrole) and its derivatives such as the amino acid L-tryptophan, the biogenic amine tryptamine, the neurotransmitter serotonin, and the hormone melatonin [9,10,11,12]. The pyrrole scaffold appears also in the structures of porphyrins of heme, chlorophylls, chlorins, porphyrinogens, vitamin B12, bile pigments (bilirubin and biliverdin), and also alkaloids isolated from various marine organisms, insects, fungi, or bacteria [3,13,14]. The pyrrole ring is a building part of numerous natural and synthetic products that has found diverse pharmacological applications [1,2,3,4,5,6,7,8]. Natural and synthetic pyrrole systems display anti-bacterial, anti-fungal, anti-protozoal, insecticidal, anti-viral, anti-malarial, anti-tubercular, anti-inflammatory, anti-depressant, anti-convulsant, anti-ulcer, anti-diabetic, anti-hypertensive, anti-psychotic, anti-cancer, anti-HIV activity, etc. [1,2,3,4,5,6,7,8]. Some of them have been applied as lipid-lowering, cytotoxic, anti-Parkinson, and anti-Alzheimer agents, enzyme inhibitors (e.g., HMG-CoA reductase, histone deacetylase, DPP IV, and GSNOR), and receptor antagonists (e.g., mGluR1, GnRH, dopaminergic, progesterone, and androgen) [1,4].
Because of these biological activities and pharmacological applications of the systems containing the pyrrole ring, detailed examinations of intra- and intermolecular proton-transfer processes are required to well describe structure–activity relationships. For example, the acid/base properties of the parent compound have already been investigated by various experimental techniques in different environments. Unsubstituted pyrrole exhibits exceptionally weak Brønsted acidity and basicity in solution [9,15,16,1718], as well as in the gas phase [19,20,21,22]. However, adding various “pushing” (electron-donating) and “pulling” (electron-accepting) substituent groups on the pyrrole ring has led to some interesting derivatives, which can be classified as strong organic N-bases in the gas phase [23,24].
With the aim to unravel the origins of very strong gas-phase proton basicity of the push–pull pyrrole derivatives, we chose here the simplest molecule, containing one pushing amino group at the 2-position, 2-aminopyrrole (AP in Figure 1 and hereafter). This compound and some of its derivatives (substituted at N1, C3, C4, and/or C5) belong to the family of tautomeric heterocycles, like other azoles [25,26,27,28,29]. Some tautomers of neutral and protonated AP have already been investigated [26,27,28,29], but there is no complete examination on the thermochemistry of all possible intra- and intermolecular proton-transfers in AP, nor on its acidity/basicity parameters. Nevertheless, we can find numerous articles in the literature on the synthesis and experimental structure-confirmations of AP and its derivatives published in the last thirty years (see for example Refs. [27,28,29,30,31,32,33,34,35]). Although 2-aminopyrrole itself is not sufficiently stable, it can be preserved for a long time in the form of its protonated salts, e.g., trifluoroacetate, perchlorate, or tetraphenylborate, and can be easily isolated just before an experimental investigation from one of its salts by adding one or more equivalents of an organic base, e.g., triethylamine [31]. For example, substituted 2-aminopyrroles have been successfully used for the syntheses of the marine alkaloid rigidins A, B, C, and D [36,37]. Their general formulae are shown in Figure 1. Since they may display prototropy [25,38,39], all labile (tautomeric) protons are indicated in this figure.
Considering the scarcity of the literature data on the proton-transfer processes for 2-aminopyrrole, the complete tautomeric and acid/base equilibria in vacuo have been studied during this work, employing quantum chemical calculations. Contrary to the experimental techniques, which detect mainly major and some minor isomers (with percentage contents >1%), only theoretical methods give the opportunity to study all possible isomeric forms and all possible proton-transfer equilibria. In this way, thermochemistry of the complete proton-transfer processes could be examined for 2-aminopyrrole. Additionally, the detailed analysis of proton transfers for AP could shed some light on the origins of superbasicity in iminopyrrole derivatives, reported by the Maksić group [23,24], and signaled by us in Ref. [40].

2. Results and Discussion

2.1. Possible Prototropic Tautomers for Neutral, Deprotonated, and Protonated AP

Neutral 2-aminopyrrole (AP) contains two labile (tautomeric) protons, one proton attached to the endo N1 atom, and the other proton linked to the exo N6 atom (Figure 1). Each of them can move between the n-π conjugated sites (N1, C2, C3, C4, C5, and/or N6; see Scheme S1 in Supplementary Materials). Hence, we can distinguish five amino tautomers (AP16, AP26, AP36, AP46, and AP56), analogous to those for tautomeric unsubstituted pyrrole, and additionally three imino tautomers (IP13, IP15, and IP34), in which the exo amino group is engaged in the tautomeric conversions (Figure 2). Note that the figures in the bold labels of isomers correspond to the positions of the tautomeric protons. Two extreme isomers for each imino form, one containing a synperiplanar (a) and the other one having an antiperiplanar imino H atom (b) vis-à-vis N1, arise from the configurational (geometric) isomerism about the C2=N6 double bond (see Scheme S2 in Supplementary Materials). Consequently, eleven isomers can be written for neutral 2-aminopyrrole. The geometric isomers (a and b) can be called “rotamers” of the exo C2=N6H group.
In all tautomeric rearrangements, π-electrons move in concert with the proton transfers. According to the Pauling definition of prototropy [38], all prototropic tautomers of 2-aminopyrrole are written without separation of the positive and negative charges, as displayed by the AP resonance structures (see Scheme S1 in Supplementary Materials). In the amino tautomer AP16, the labile protons are at the N1 and N6 atoms, and we call this the NHNH form. The amino tautomers AP26, AP36, AP46, and AP56, and also the imino isomers IP13a, IP13b, IP15a, and IP15b, possess the labile protons at the N (N6 or N1) and C atoms (C2, C3, C4, or C5). They can be named CHNH forms. In the last imino isomers IP34a and IP34b, the labile protons are at the C3 and C4 atoms, and we call them the CHCH forms. Note that our analysis for the first time refers to the complete tautomeric mixture of eleven prototropic isomers of 2-aminopyrrole. Dewar, De Rosa, and their co-workers [26,27] analyzed only one amino form (AP16) and two imino tautomers (IP13 and IP15) in their studies on tautomerism for neutral 2-aminopyrrole. Other possible tautomers were ignored. It is not clear why only three tautomers were studied, and we complete here the previously reported results.
The mono-deprotonated AP isomers are formed by loss of one labile proton in the amino and imino isomers of the tautomeric mixture of the neutral derivative. This process leads to the tautomeric mixture AP− containing the mono-anionic isomers, which now contains only one tautomeric proton. Mono-deprotonation of the amino tautomers AP16, AP26, AP36, AP46, and AP56 at the N1, C2, C3, C4, and C5 atoms, respectively, gives one common mono-anionic isomer AP6− possessing the labile proton at N6 (Figure 3). In this reaction, the AP amino isomers can alternatively lose one labile proton at the amino N6 atom {in syn- (a) or anti-periplanar (b) position vis-à-vis N1}, leading to the corresponding isomers of the mono-deprotonated form: AP1a−, AP1b−, AP2a−, AP2b−, AP3a−, AP3b−, AP4a−, AP4b−, AP5a−, and AP5b−. The isomers AP1a− and AP1b− can also be formed after mono-deprotonation of the imino isomers IP13a, IP13b, IP15a, and IP15b at the C3 and C5 atom, respectively. In turn, mono-deprotonation of IP13a, IP13b, IP15a, and IP15b at N1 gives AP3a−, AP3b−, AP5a−, and AP5b−, respectively, while for IP34a and IP34b at C3 it leads to AP4a− and AP4b−, and for IP34a and IP34b at C4 it leads to AP3a− and AP3b−. To our knowledge, the eleven isomers of mono-anionic 2-aminopyrrole (AP−) have not yet been reported in the literature, and this is the first complete analysis of its tautomeric mixture.
When the tautomeric mixture of neutral 2-aminopyrrole (AP) gains one proton in the mono-protonation reaction, the mono-cationic tautomeric mixture is formed (AP+). The six mono-cationic isomers AP116+, AP126+, AP136+, AP146+, AP156+, and AP166+ can be derived by mono-protonation of the neutral amino tautomer AP16 at N1, C2, C3, C4, C5, and N6, respectively (Figure 4). Alternatively, the four tautomers AP126+, AP136+, AP146+, and AP156+ can be formed when one proton is gained by N1 in the tautomers AP26, AP36, AP46, and AP56. The stability of AP266+, AP366+, AP466+, and AP566+, mono-protonated at N6, can also be verified. The mono-protonation of the two isomers a and b of the imino tautomers IP13, IP15, and IP34 at N6 leads to the common mono-cations AP136+, AP156+, and AP346+, respectively. The two isomers a and b of the imino tautomer IP34 can be also mono-protonated at N1, leading to the isomeric pair AP134a+ and AP134b+. Consequently, each of the thirteen possible isomers of the mono-cationic form AP+ contains three labile (tautomeric) protons and displays prototropy. In the literature, we found two theoretical reports on prototropic tautomerism in mono-protonated 2-aminopyrrole. De Rosa and co-workers [27,28] discussed only three mono-cationic tautomers, AP136+, AP156+, and AP166+, referring to the mono-protonation of the amino isomer AP16 at C3, C5, or N6, respectively. Regarding this tautomerism in vacuo, the authors applied quantum chemical calculations at diverse levels of theory {HF, MP2, MP4SDQ, QCISD, and QCISD(T)} using various basis sets, but their analyses gave an incomplete picture of the tautomeric mixture of AP+.

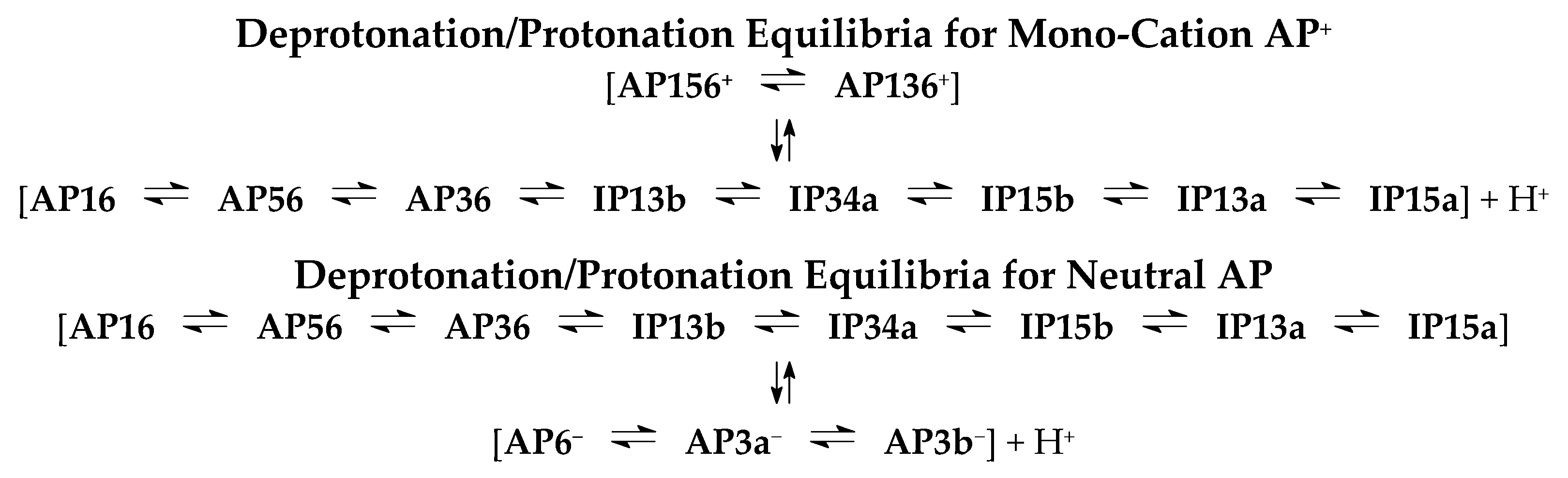
2.2. Thermochemistry of Prototropic Conversions for AP, AP−, and AP+

Quantum chemical calculations were carried out for possible tautomers/rotamers of neutral and ionic forms of 2-aminopyrrole using the DFT (Density Functional Theory) method [41] with the B3LYP (Becke three-parameter hybrid exchange functional and non-local correlation functional of Lee, Yang, and Par) functional [42,43] and the 6-311+G(d,p) basis set with polarization and diffuse functions [44]. This level of theory has been proposed by Koppel, Leito, Maksić and their co-workers as adequate for investigating the thermochemistry of proton-transfer processes in the gas phase, as well as for estimations of the gas-phase proton acidity/basicity parameters [24,45,46]. All isomers given in Figure 2, Figure 3 and Figure 4 were optimized without symmetry constraint and their energy minima (with positive frequencies) calculated at the DFT(B3LYP)/6-311+G(d,p) level. Their atom coordinates, electronic energies, and thermochemical data are included in Tables S1 and S2, respectively (Supplementary Materials). The mole-fractions of all isomers (xi) in the tautomeric mixtures of neutral and ionic 2-aminopyrrole were estimated using the relative Gibbs energies (ΔGs) calculated at 298.15 K as the difference between a given isomer and the most stable one. For calculations, Equation (1) was employed.
xi = exp{−ΔG(APi, APi−, or APi+)/RT}/{Σ1nexp[−ΔG(APi, APi−, or APi+)/RT]}
As was expected, the amino tautomer AP16 (Figure 2) is the most stable form {displays the lowest Gibbs energy (G)} for neutral 2-aminopyrrole. The other amino and imino tautomers possess higher G values. Their relative Gibbs energies (ΔG) are between 20 and 80 kJ mol−1 (Table 1). The participation of some of them in the tautomeric mixture of AP can be significant, and thus they can be considered in the acid/base equilibria. The Mezey et al. rule [47,48], used in quantum chemical stability-estimations, helps us to select important isomers. This rule was derived about forty-five years ago for prototropic tautomers of the nucleobases guanine and adenine, and for their protonated forms. Authors used the methodology and ab initio levels of quantum chemical calculations suitable at that time. Although quantum chemical methods and computers have progressed since that time, we can still use this rule for tautomeric systems, because tautomeric equilibria are very sensitive to environment, and tautomeric preferences can change, for example, when going from the gas phase through various solutions to the solid state, as well as from neutral to ionized forms [25,39,49]. According to the Mezey rule, the lowest limit of significant relative Gibbs energies between the favored and other isomers in the isomeric mixture cannot be higher than 40 kJ mol−1. Employing this rule, we can neglect only three isomers in the isomeric mixture: two amino forms (AP46 and AP26) and one imino isomer (IP34b). On the basis of our DFT-calculated ΔG values (<40 kJ mol−1), the stability order of the remaining eight isomers is as follows: AP16 > AP56 > AP36 > IP13b > IP34a > IP15b > IP13a > IP15a.
Note that some imino isomers (IP13b, IP34a, and IP15b) exhibit ΔGs close to those of the amino ones (AP56 and AP36). This means that various types of prototropic conversions between the conjugated atoms of the pyrrole ring, as well as between the conjugated exo N6 atom and endo C atoms, could be detected in the tautomeric mixture of neutral AP. Moreover, regarding the ΔG values of the configurational isomers a and b for the imino tautomers IP13, IP34, and IP15, we can draw an additional observation. The favorable interactions between the functional groups, i.e., between N1H and the n-electron pair of N6 in IP13b and IP15b, and between N6H and the n-electron pair of N1 in IP34a, decrease the ΔG values by 4.4, 3.2, and 14.1 kJ mol−1, respectively, in comparison to those of their rotamers with the unfavorable interactions, i.e., repulsion between N1H and N6H in IP13a and IP15a, and repulsion between the n-electrons pairs of N1 and N6 in IP34b. This observation indicates without any doubt that the effects of geometric isomerism on the stability of the imino tautomers cannot be ignored. However, Dewar and co-workers [26] estimating the heat of atomization for the AP tautomers using the semi-empirical SCF MO method with π-approximation considered only three isomers (AP16, IP13 and IP15) without any information, which isomers (a or b) for the imino tautomers have been selected. On the other hand, De Rosa and co-workers [27] took the geometrical isomerism for the imino isomers into account and applied higher levels of theory {HF, MP2, QCSID, and QCSID(T)} but chose the same three tautomers for investigations of tautomerism in AP. The other tautomer/rotamers have not been considered in their article. Our work completes the limited current knowledge on the topic. It can be mentioned here that when rotation about single/double bonds is structurally limited in isolated molecules or in crystal adducts, additional stabilizing hydrogen–hydrogen interactions may be considered [50,51].
The loss of the labile proton at the N1, C2, C3, C4, or C5 atom in the amino tautomers of AP gives the mono-anionic tautomer AP6− (Figure 3) of the lowest Gibbs energy. The ΔG values for the other ten tautomers/rotamers of AP− are close to or higher than 40 kJ mol−1 (Table 2). According to the Mezey limit [47,48], only two additional isomers, AP3a− and AP3b−, can significantly contribute to the isomeric mixture. Since their percentage contents are low (0.0202 and 0.00002%, respectively), they may not be detected in experiments carried out in vacuo or in non-polar solvents. The other eight isomers can be treated as exceptionally rare forms. They possess ΔGs higher than 40 kJ mol−1. They may be considered in mechanisms of particular reactions carried out in the presence of extremely strong bases. To our knowledge, there are no literature data on prototropy in mono-deprotonated 2-aminopyrrole. According to our DFT calculations, the tautomer AP6− is preferred as a mono-deprotonated form. If we look at the major tautomer AP16 of neutral 2-aminopyrrole, the exo N1 atom can be regarded as the favored site of deprotonation. This indicates that 2-aminopyrrole belongs to the family of organic NH-acids.
DFT-calculations carried out for all thirteen isomers of mono-protonated form of 2-aminopyrrole (Figure 4) clearly show that the tautomer AP156+ has the lowest Gibbs energy (Table 3), and only one additional tautomer, AP126+, contributes significantly to the composition of the tautomeric mixture of AP+ (ΔG < 40 kJ mol−1). However, its percentage content (0.006%) seems to be too low for experimental detection, and only the major tautomer AP156+ can be experimentally observed in vacuo or in non-polar solvents. Indeed, the presence of the CH2 group in the mono-cation of 2-aminopyrrole has been confirmed in the 1H and 13C NMR spectra recorded for AP+ acetate salt in glacial acetic acid [27], and also for AP+ tetraphenylborate salt in (CD3)2CO [31], though solvent effects may have a significant impact on the fraction of rare isomers. The other eleven tautomers/rotamers of AP+ possess ΔGs higher than 40 kJ mol−1 in vacuo, but they may be considered in mechanisms of particular reactions carried out in the presence of extremely strong acids.
Using various quantum chemical methods {HF, MP2, MP4SDQ, QCISD, and QCISD(T)} and different basis sets, Fradera et al. [28] investigated three tautomers of mono-protonated 2-aminopyrrole (AP166+, AP156+, and AP136+). Our DFT results for these three tautomers are in good agreement with those computed at the highest levels of theory {MP4SDQ, QCISD, and QCISD(T)}. At each level of theory, the tautomer AP156+ is favored for the mono-protonated form. Since the tautomer AP16 is the major form for neutral 2-aminopyrrole, the C5 atom can be considered as the favored site of mono-protonation. This means that 2-aminopyrrole, although possessing two N atoms, belongs to the family of organic C-bases.

2.3. HOMED Indices Estimated for Neutral and Ionic Tautomers/Rotamers of 2-Aminopyrrole

The stability order of tautomeric forms and electron delocalization are somehow related [38,39]. This can be measured quantitatively by the structural descriptor HOMED (Harmonic Oscillator Model of Electron Delocalization) [52,53], which is a recent modification of the HOMA (Harmonic Oscillator Model of Aromaticity) index, proposed fifty years ago by Kruszewski and Krygowski [54,55,56]. The HOMED descriptor can be applied to any delocalized compound, σ-π, n-π, and π-π conjugated systems, as well as aromatic molecules [49,52,53,57], whereas the HOMA index is mainly a quantitative measure of aromaticity [54,55,56]. For HOMED estimation, Equation (2) can be used here. It is analogous to that proposed by Krygowski in 1993 for the reformulated HOMA index [56]. For HOMED, only the parameterization is different [52,53] from that of HOMA [56].
HOMED = 1 − {α(CC)Σ[Ro(CC) − Ri(CC)]2 + α(CN)Σ[Ro(CN) − Ri(CN)]2}/n
In Equation (2), α(CC) and α(CN) are the normalization constants for the CC and CN bonds, as described in Refs. [52,53]; Ro(CC) and Ro(CN) are the optimum bond-lengths for the reference molecules (benzene and 1,3,5-triazine) computed at the same DFT-level of theory as that applied for the neutral and ionic AP-isomers; Ri(CC) and Ri(CN) are the calculated bond-lengths in the investigated structures; and n is the number of bonds taken into account in the HOMED estimation. The HOMED parameters used in this work are given in Table S3 (Supplementary Materials).
According to the parameterization procedure, the HOMED index is close to unity for aromatic compounds [52,53], like the original [54,55] and reformulated HOMA descriptor [56]. The two geometry-based parameters (HOMED and HOMA) have a common anchor point in the scale for aromatic hydrocarbons: benzene [52,53,54,55,56]. For N-containing aromatic systems, the two indices are close to unity when Ri(CC) and Ri(CN) are close to the defined Ro(CC) and Ro(CN) for the corresponding aromatic reference molecules. In such cases, investigated molecules present a resonance stability analogous to that in benzene and its hetero-analogs. Note that in N-containing tautomeric systems, aromatic isomers frequently possess the lowest Gibbs energies, and they predominate in the tautomeric mixtures [25,39,52,53].
On the other hand, the HOMED index is equal to zero for the hypothetical reference molecules cyclohexa-1,3,5-triene (Kekulé structure of benzene) and its triaza derivative (Kekulé structure of 1,3,5-triazine), possessing the localized CC and CN single and double bonds, lengths of which are equal to those for ethane, ethene, methylamine, and methylimine, respectively, like in the original HOMA index [54,55]. The resonance stabilization for this kind of system (hypothetical Kekulé structure) can be neglected. In the case of tautomeric systems, isomers with HOMED close to zero usually possess considerably higher Gibbs energies than the aromatic structures. The same is true for structures with negative HOMED values. This takes place in particular cases, when the CC and/or CN single bonds are longer than those in ethane and/or methylamine, and/or the CC and/or CN double bonds are shorter than those in ethene and/or methylimine. The negative HOMED values indicate that electrons in the investigated structures are less delocalized than in the hypothetical reference molecules.
Using the above-mentioned anchor points in the HOMED parameterization procedure (analogous aromatic structures, benzene and 1,3,5-triazine, for HOMED = 1, and their analogous hypothetical Kekulé structures for HOMED = 0), the HOMED index could appropriately measure any type of electron delocalization in σ-π, n-π, and π-π conjugated molecules. Meanwhile, the anchor points for defining zero in the reformulated HOMA procedure are based on differently conjugated reference molecules, i.e., moderately π-π conjugated 1,3-butadiene for CC bonds, and very weakly delocalized methylamine and methylimine for CN bonds [56]. These different measures of the CC and CN bonds have led to some discrepancies for some conjugated systems with HOMED < 0.9, particularly for rare CH tautomers of tautomeric hetero-compounds [49,53]. For this reason, we applied here the HOMED descriptor to quantitatively describe bond length alternation in AP isomers. For more details on parameterization of the HOMED index, see Refs. [52,53].
Table 4 summarizes the HOMED values calculated for all DFT structures of neutral (AP), mono-deprotonated (AP−), and mono-protonated (AP+) prototropic isomers of 2-aminopyrrole. HOMED5 corresponds to the pyrrole ring containing five bonds between heavy atoms, and HOMED6 refers to the entire molecule with six bonds, including the C2N6 bond. Among possible prototropic isomers of neutral 2-aminopyrrole, only one NHNH tautomer (AP16) of the lowest Gibbs energy displays an aromatic character for the ring (HOMED5 0.924). The aromaticity of the pyrrole ring in 2-aminopyrrole has also been confirmed by the magnetic criterion NICS (Nucleus-Independent Chemical Shifts). Elguero and co-workers [58], investigating series of aminoazoles, found a strongly negative NICS value (−14.7) for the ring in 2-aminopyrrrole, like for other aromatic azoles. AP16 contains six labile electrons (two n-electrons of the endo N1 atom and four π-electrons of the endo C2–C5 atoms) well delocalized in the ring. The other NHCH isomers possess one C-sp3 and four labile electrons in the ring. Consequently, they lose the ring aromaticity, their π-electrons being considerably less delocalized than in AP16. Their HOMED5 indices are not higher than 0.5. Two configurational isomers of the imino tautomer (IP34a and IP34b), containing the labile protons at two C-sp3 (C3 and C4) and one pair of labile electrons in the ring, have the lowest HOMED5 values (close to zero). When the exo N6 atom is included in the structural-descriptor estimations, the variations of the HOMED6 values are analogous to those of HOMED5. The highest HOMED6 value is for the tautomer AP16 (0.825), and the lowest ones are for IP34a and IP34b (close to zero). For other isomers, the HOMED6 values are not higher than 0.5.
Perusal of the HOMED indices calculated for all eleven mono-anionic isomers of 2-aminopyrrole indicates that the favored NH isomer AP6− has the most delocalized labile electrons. Its HOMED5 value is close to unity (0.958) and corresponds to an aromatic system. The mono-deprotonation at N1 increases electron delocalization in the ring in comparison to that in the neutral tautomer A16 (0.924). Together with electron delocalization, the negative charge is also well delocalized in the ring. On the other hand, the exo NH2 group in AP6− participates to a lesser extent in electron and charge delocalization despite its electron-donor effect. The C2N6 bond length (1.4438 Å) in AP6− is closer to the single CN bond in methylamine computed at the same DFT level (1.4658 Å) than that in AP16 (1.4169 Å). Consequently, the value of HOMED6 for AP6− is considerably lower (0.777) than that for AP16 (0.825). The other NH isomers, AP1a− and AP1b−, seem to be moderately delocalized. Their HOMED5 and HOMED6 values are close to 0.8. The exo group participates in electron and charge delocalization. Note that the HOMED values are higher for AP1b− than AP1a−, indicating important effects of intramolecular interactions on electron delocalization. The favorable interaction between N6H and the n-electron pair of N1 in AP1b− increases electron delocalization in the system and the HOMED values in comparison to AP1a−, in which the unfavorable interaction (repulsion) between N1H and N6H takes place. These effects of intramolecular interactions between the functional groups influence also the HOMED values in the neutral prototropic isomers, but they are weaker than those in mono-anionic isomers. The HOMED indices for all CH isomers are lower than 0.4 because of the C-sp3 presence in the five-membered ring. The isomer AP3a− shows the highest HOMED values (close to 0.4), for which both electron and charge can be delocalized, and, additionally, the n-electrons of N1 can favorably interact with N6H. The negative HOMED values are calculated for isomers AP2a− and AP2b−, for which the negative charge cannot be delocalized with labile electrons.
Since 2-aminopyrrole seems to be favorably mono-protonated at the endo C5 atom, the tautomer AP156+ of the lowest Gibbs energy is moderately delocalized. Its HOMED5 (0.468) and HOMED6 (0.606) values are lower than those (0.922 and 0.669, respectively) of AP166+, possessing higher Gibbs energy, but still showing some aromaticity of the ring after mono-protonation at N6. In AP156+, the exo N6 atom with its n-electron pair can participate in delocalization of the labile electrons and charge (like in less stable AP136+ and AP146+), while in AP166+ there is no such possibility. Consequently, the C2N6 bond length in AP156+ (1.3247 Å) is close to the CN bond length in aromatic 1,3,5-triazine (1.3342 Å), while that in AP166+ (1.4641 Å) is close to the CN single bond in methylamine (1.4658 Å). These trends explain the differences between the HOMED5 and HOMED6 values for the two tautomers: HOMED5 < HOMED6 for AP156+ and HOMED5 > HOMED6 for AP166+. The lack of participation of N6 in electron delocalization takes also place for other tautomers of high Gibbs energies protonated at this atom, such as AP266+, AP366+, AP466+, and AP566+, for which HOMED5 is between 0.3 and 0.5, while HOMED6 is lower than 0.1. The mono-protonation of AP at N1 is unlikely because it completely destroys electron delocalization and aromaticity of the ring. The two HOMED indices are close to zero for AP116+. The same is true for AP126+, AP134a+, and AP134b+.

2.4. Microscopic Gas-Phase Proton Acidity/Basicity Parameters for Tautomers/Rotamers of AP

On the basis of the thermochemical data calculated at the DFT level for individual tautomers/rotamers of neutral, mono-deprotonated, and mono-protonated 2-aminopyrrole (Table S2 in Supplementary Material), we can estimate the so-called microscopic (partial) acidity/basicity parameters for particular acid/base sites. For example, for the favored neutral tautomer AP16 (Figure 2), we can calculate the acidity parameters for the acid NH groups (N1H and N6H) containing the tautomeric (labile) protons and the basicity parameters for each heavy atom (N1, C2, C3, C4, C5, and N6).
Generally, in the gas phase, the acid/base strength can be measured in two scales [20,21,22]. In one of them, deprotonation enthalpy (DPE), frequently applied for neutral acids (AH), and proton affinity (PA), usually employed for neutral bases (B), correspond to the enthalpy change of the deprotonation/protonation Equilibrium (3). In the other one, gas-phase acidity (GA) and gas-phase basicity (GB) refer to the Gibbs energy change of the same Equilibrium (3). The commonly used terms and abbreviations for gas-phase proton acidity/basicity parameters (DPE or PA, and GA or GB) have historical grounds and are adopted here.
AH (or BH+) ⇄ A− (or B) + H+
The microscopic acidity (DPEmicro and GAmicro) parameters for acid groups and the microscopic basicity (PAmicro and GBmicro) parameters for the base sites can be calculated using Equations (4) and (5), respectively. In these equations, Hi and Gi refer to the enthalpy and Gibbs energy, respectively, calculated at 298.15 K for the corresponding tautomers playing the base or acid role; H(H+) = 6.2 kJ mol−1, and G(H+) = −26.3 kJ mol−1 at 298.15 K [59,60]. The Equations (4) and (5) are analogous to those for compounds being free of prototropy [20,21,22]. Note that a stronger base has higher PA and GB values, and a stronger acid has lower DPE and GA values.
DPEmicro(AH or BH+) = Hi(A− or B) + H(H+) − Hi(AH or BH+) = PAmicro(A− or B)
GAmicro(AH or BH+) = Gi(A− or B) + G(H+) − Gi(AH or BH+) = GBmicro(A− or B)
The DFT-calculated microscopic acidity/basicity parameters for all eleven prototropic isomers of neutral 2-aminopyrrole are included in Figure 5. First perusal of their values shows that change of positions of labile protons affect both the acidity of NH and CH groups, as well as the basicity of heavy atoms. For individual isomers, the DPE/GA and PA/GB values indicate also which base site displays the stronger basicity and can be considered as the favored site of protonation and which acid site exhibits the stronger acidity and can be treated as the preferred site of deprotonation.
In the case of AP16, the PA/GB order for heavy atoms is as follows: C5 > C3 > C4 > C2 > N6 > N1. The C5 atom seems to be the favored site of protonation and the N1 atom the last one. However, the N1H group is more acidic than N6H and seems to be preferentially deprotonated. Quite a different situation takes place for the amino CH tautomers AP26, AP36, AP46, and AP56. The DPE/GA values for N6H are higher than those for C2H, C3H, C4H, and C5H, respectively, and thus the corresponding CH group (possessing the labile proton) is more easily deprotonated. Among N atoms, the imino N1 atom displays higher basicity parameters and is the most favorably protonated for the amino tautomers. For both isomers (a and b) of the imino tautomers IP13, IP15, and IP34, the situation is different, and N6 can be preferentially protonated. Concerning mono-deprotonation, N1H appears to be favorably deprotonated in IP13a, IP13b, and IP15a, while it is C5H in IP15b and C4H in IP34a and IP34b. Interestingly, the imino N6 atom in IP15a and IP15b exhibits very high PA values (>1000 kJ mol−1), indicating that derivatives of IP15a and IP15b, being free of prototropy, can be good candidates for superbases (see Section 2.6).

2.5. Thermochemistry of Acid/Base Equilibria in Vacuo for AP

When the equilibrium method is applied for gas-phase proton acidity/basicity measurements, a mixture of isomeric molecules of tautomeric compound can be present in the FT-ICR (Fourier Transform Ion Cyclotron Resonance) mass spectrometer apparatus. In this technique, positive and negative ions are studied separately. After ionization, in positive or negative mode, the ionic forms (mono-cation AP+ or mono-anion AP−) can be produced. Interacting with the corresponding reference molecules (base B or acid AH) introduced into the FT-ICR cell, the acid/base equilibria can be established and the acidity/basicity parameters measured. We can write the following general tautomeric and acid/base equilibria for 2-aminopyrrole, considering the Mezey limit of isomer significance [47,48]. In Scheme 1, the tautomeric mixtures of mono-cationic, neutral, and mono-anionic forms contain two, eight, and three isomers, respectively (see their relative Gibbs energies and percentage contents in Table 1, Table 2 and Table 3, respectively). All of them can be considered for quantum chemical estimation of the macroscopic acidity/basicity parameters.
In the case of the mono-deprotonation of the neutral tautomeric mixture (AP) to the mono-anionic tautomeric mixture (AP−), Equation (6) can be used for estimation of the macroscopic gas-phase acidity parameter, GAmacro. In the case of the mono-deprotonation reaction of the mono-cationic tautomeric mixture (AP+) to the neutral tautomeric mixture (AP), Equation (7) can be employed for estimation of the macroscopic gas-phase basicity parameter, GBmacro. As could be expected, the GAmacro and GBmacro values (1468.8 and 941.4 kJ mol−1, respectively) calculated for the corresponding reactions given in Scheme 1 are equal to the GAmicro and GBmicro of N1H and C5 in AP16, respectively (Figure 5). The reason is that the percentage contents of AP6−, AP16, and AP56+ are very high (>99.9%) in comparison to those of other isomers, for which their contribution in the tautomeric mixtures are exceptionally low. For the same reason, the DPEmacro and PAmacro values are estimated in an analogous way as for GAmacro and GBmacro, but in the enthalpy scale (1497.8 and 977.9 kJ mol−1, respectively) are equal to the DPEmicro and PAmicro of N1H and C5 in AP16, respectively (Figure 5). From a statistical point of view, this indicates that at the DFT level, only the favored isomers can be considered for gaseous acid/base equilibria of 2-aminopyrrole.
GAmacro(AP) = Σ1nxiG(APi−) + G(H+) − Σ1nxiG(APi)
GBmacro (AP) = Σ1nxiG(APi) + G(H+) − Σ1nxiG(APi+)
To our knowledge, there are no experimental nor theoretical gas-phase proton acidity/basicity data for 2-aminopyrrole reported in the literature. However, we can compare the DPE, GA, PA, and GB calculated for AP16 with the literature experimental data for unsubstituted pyrrole [19,20,21,22]. AP seems to possess almost the same acid strength of the N1H group, but it seems to be a considerably stronger C-base (by ca. 100 kJ mol−1) than its parent system. This observation indicates that the strong electron-donor effect of the exo NH2 group could be responsible for the enhancement of basicity of C5 in AP.

2.6. Importance of CH Tautomers in the Design of Superbasic Imines

As signaled above, some tautomers of 2-aminopyrrole possessing one labile proton at the C atom display exceptionally high PAs of the imino N atom (close to or higher than 1000 kJ mol−1). This takes place for the imino N1 atom in the amino tautomers AP36 and AP56, and also for the imino N6 atom in the imino isomers IP13a, IP13b, IP15a, and IP15b. Since these isomers are rare forms, their acidity/basicity parameters can be difficult or even impossible to measure. Nevertheless, certain alkyl derivatives, being free of prototropy, may be synthesized and their gas-phase basicity parameters measured.
Among the molecules studied by the Maksić group [23], we can select derivatives, for which superbasicity takes its origin from the imino CH tautomer IP15 of 2-aminopyrrole. Three iminopyrrole systems (IP1–IP3, given in Figure 6) are free of tautomeric conversions, and their basicity parameters (PAs > 1000 kJ mol−1) show that they can be classified as superbases. However, the effect of geometrical isomerism about the C=N double bond on basicity parameter has not been discussed, and the PA values, estimated using the Hartree–Fock model scaled for organic N-bases, contain some uncertainty. Difference between PAs of IP1 and IP2 are attributed to the polarizability effect of the −CH3 group present at the imino N atom (site of protonation), whereas that between the PAs of IP1 and IP3 may be a consequence of both the polarizability and field/inductive effects of the –(CH2)3N(CH3)2 substituent linked to the site of protonation as well as the chelation effect of the proton by two basic sites, the imino N atom in the iminopyrrole part and the amino N atom in a “scorpio” conformation of the substituent. Note that a diradical character discussed recently for N-heterocyclic quinodimethane [61] has not been documented for IP1–IP3 in Ref. [23]. No information on the wave-function stabilities for these systems has been given in that work, nor in the review article [24]. The closed-shell (singlet) structures for the base and mono-protonated forms have been studied, and the PA values for the imino N-atom predicted. It would be interesting to study the possible open-shell (diradical) structures for various push–pull iminopyrroles (being free of prototropy), and to characterize their diradical character by different descriptors based on the structure, reactivity, orbital overlap, etc. Such an investigation and characterization is out of the scope of the present work.
Unfortunately, the other iminopyrrole systems with the IP15 fragment, proposed by the Masić group in Ref. [23] as candidates for new superbases, possess labile (tautomeric) protons, and thus, they can tautomerize to more stable amino forms. For example, 2-imino-5-diaminomethylidene-3-pyrroline (IDAMP, shown in Figure 7) contains three tautomeric protons at amino N atoms and nine conjugated tautomeric sites (all heavy atoms). Like in 2-aminopyrrole, heavy atoms of exo groups can participate in the tautomeric conversions together with heavy atoms of the pyrrole ring. For example, the exo groups, imino (=N6H) at the 2-position and diaminomethylidene {=C7(NH2)2} at the 5-position, can convert during tautomerization to more favorable tautomeric groups, amino (−NH2) and carboximidamido {amidine, −C(=NH)NH2} substituents, leading to the expected 5-aminopyrrole-2-amidine (APA, IUPAC name: 5-amino-1H-pyrrole-2-carboximidamide), possessing the aromatic pyrrole ring. This exciting prototropy phenomenon and its effect on the favored site of protonation and gas-phase basicity parameters will be examined in the future.

3. Computational Details

For all quantum chemical calculations, the Gaussian 03 programs were used [62]. Geometries of all neutral and ionic isomers of investigated pyrrole derivatives were optimized without symmetry constraint in their ground states in vacuo at the DFT(B3LYP)/6-311+G(d,p) level [41,42,43,44], as previously described in Refs. [40,49,52,53,57,63]. Their atom coordinates and DFT-calculated electronic energies are summarized in Table S1 (Supplementary Materials). Vibrational frequencies and thermochemical parameters such as enthalpy (H), entropy (S), and Gibbs energy (G) were calculated at 298.15 K using the same method as that employed for geometry optimization. The DFT-calculated thermochemical data, such as H, G, and S, are listed in Table S2 (Supplementary Materials). The parameters of Equation (2) applied in the HOMED estimations and taken from Refs. [52,53] are included in Table S3 (Supplementary Materials).

4. Conclusions

Application of quantum chemical methods to the tautomeric 2-aminopyrrole (AP) system opened the possibility to study the intramolecular proton-transfer equilibria (prototropy). Simultaneously, the intermolecular (acid/base) proton-transfer reactions could be examined. In this way, the thermochemistry of the corresponding proton-transfer processes could be determined in vacuo. We analyzed the properties of all possible tautomers for neutral as well as for ionic (mono-deprotonated and mono-protonated) forms, and estimated their amounts in the tautomeric mixtures. We also determined acidity/basicity parameters at the micro- and macro-scale. Using AP as a simple example of a tautomeric derivative, we could explain the origin of the strong basicity of some iminopyrroles proposed in the literature [23,24] being free of prototropy. Factors that dictate the strong basicity of N-bases have been recently discussed [64]. Here, we highlighted the role of CH tautomers in the design of strong Brønsted imino N-bases. We additionally reported that the iminopyrrole systems containing tautomeric protons most probably tautomerize to more favorable tautomeric aminopyrrole derivatives, like AP, displaying considerably lower basicity parameters than iminopyrroles. The nature of the site of protonation and of substituent effects explain this striking difference.

Supplementary Materials

The following information can be downloaded at https://www.mdpi.com/article/10.3390/molecules30102112/s1. Table S1. DFT-calculated atom coordinates and electronic energies for neutral and ionic isomers of 2-aminopyrrole. Table S2. DFT-calculated enthalpies, Gibbs energies, and entropies for neutral and ionic isomers of 2-aminopyrrole. Table S3. Parameters used for HOMED estimation. Scheme S1. Resonance structures for 2-aminopyrrole showing acid and base sites for intramolecular proton-transfers (prototropy). Scheme S2. Resonance structures for the imino tautomers explaining isomerism of exo imino group.

Author Contributions

E.D.R.: article concept, calculations, results analysis, supervision, writing, and editing; P.-C.M.: supervision and writing; J.-F.G.: supervision and writing. All authors have read and agreed to the published version of the manuscript.

Funding

This research received no external funding.

Institutional Review Board Statement

Not applicable.

Informed Consent Statement

Not applicable.

Data Availability Statement

The original contributions presented in this study are included in the article/Supplementary Material. Further inquiries can be directed to the corresponding author.

Acknowledgments

P.-C.M. and J.-F.G. thank the Université Côte d’Azur and the Institut de Chimie de Nice (CNRS UMR 7272) for their continuous support. E.D.R. thanks the Warsaw University of Life Sciences (SGGW) for support.

Conflicts of Interest

The authors declare no conflicts of interest.

References

  1. Bhardwaj, V.; Gumber, D.; Abbot, V.; Dhiman, S.; Sharma, P. Pyrrole: A Resourceful Small Molecule in Key Medical Hetero-Aromatics. RSC Adv. 2015, 5, 15233. [Google Scholar] [CrossRef]
  2. Gholap, S.S. Pyrrole: An Emerging Scaffold for Construction of Valuable Therapeutic Agents. Eur. J. Med. Chem. 2016, 110, 13–31. [Google Scholar] [CrossRef] [PubMed]
  3. Domagala, A.; Jarosz, T.; Lapkowski, M. Living on Pyrrolic Foundations—Advances in Natural and Artificial Bioactive Pyrrole Derivatives. Eur. J. Med. Chem. 2015, 100, 176–187. [Google Scholar] [CrossRef] [PubMed]
  4. Ahmad, S.; Alam, O.; Naim, M.J.; Shaquiquzzaman, M.; Alam, M.M.; Iqbal, M. Pyrrole: An Insight into Recent Pharmacological Advances with Structure Activity Relationship. Eur. J. Med. Chem. 2018, 157, 527–561. [Google Scholar] [CrossRef]
  5. Mateev, E.; Georgieva, M.; Zlatkov, A. Pyrrrole as an Important Scaffold of Anticancer Drugs: Recent Advances. J. Pharm. Pharm. Sci. 2022, 25, 24–40. [Google Scholar] [CrossRef]
  6. Jeelan Basha, N.; Basavarajaiah, S.M.; Shyamsunder, K. Therapeutic Potential of Pyrrole and Pyrrolidine Analogs: An Update. Mol. Divers 2022, 26, 2915–2937. [Google Scholar] [CrossRef]
  7. Ganesh, B.H.; Raj, A.G.; Aruchamy, B.; Nanjan, P.; Drago, C.; Ramani, P. Pyrrole: A Decisive Scaffold for the Development of Therapeutic Agents and Structure-Activity Relationship. ChemMedChem 2023, 19, e202300447. [Google Scholar] [CrossRef]
  8. Long, L.; Zhang, H.; Zhou, Z.H.; Duan, L.; Fan, D.; Wang, R.; Xu, S.; Qiao, D.; Zhu, W. Pyrrole-Containing Hybrids as Potential Anticancer Agents: An insight into Current Developments and Structure-Activity Relationships. Eur. J. Med. Chem. 2024, 273, 116470. [Google Scholar] [CrossRef]
  9. Joule, J.A.; Mills, K. Heterocyclic Chemistry, 5th ed; Wiley: Chichester, UK, 2010. [Google Scholar]
  10. Nelson, D.L.; Cox, M.M. Principles of Biochemistry, 7th ed; W.H. Freeman and Company: New York, NY, USA, 2017. [Google Scholar]
  11. Palego, L.; Betti, L.; Rossi, A.; Giannaccini, G. Tryptophan Biochemistry: Structural, Nutritional, Metabolic, and Medicinal Aspects in Humans. J. Amino Acids 2016, 2016, 8952520. [Google Scholar] [CrossRef]
  12. Walsh, C.T.; Garneau-Tsodikova, S.; Howard-Jones, A.R. Biological Formation of Pyrroles: Nature’s Logic and Enzymatic Machinery. Nat. Prod. Rep. 2006, 23, 517–531. [Google Scholar] [CrossRef]
  13. Nikolaou, V.; Nikoloudakis, E.; Ladomenou, K.; Charalambidis, G.; Coutsolelos, A.G. Porphyrins—Valuable Pigments of Life. Front. Chem. Biol. 2024, 2, 1346465. [Google Scholar] [CrossRef]
  14. Seipp, K.; Geske, L.; Opatz, T. Marine Pyrrole Alkaloids. Mar. Drugs 2021, 19, 514. [Google Scholar] [CrossRef]
  15. Cirrincione, G.; Almerico, A.M.; Aiello, E.; Dattolo, G. Pyrroles, Part Two, The Synthesis, Reactivity and Physical Properties of Substituted Pyrroles; Jones, R.A., Ed.; John Wiley & Sons Inc.: New York, NY, USA, 1992; Chap. 3. [Google Scholar]
  16. Navqui, M.; Fernando, Q. The Basic Strength of Pyrrole. J. Org. Chem. 1960, 25, 551–554. [Google Scholar]
  17. Chiang, Y.; Whipple, E.B. The Protonation of Pyrroles. J. Org. Chem. 1963, 85, 2763–2767. [Google Scholar]
  18. Balon, M.; Carmona, M.C.; MuHoz, M.A.; Hidalgo, J. The Acid-Base Properties of Pyrrole and its Benzologs Indole and Carbazole. A Reexamination from the Excess Acidity Method. Terrahedron 1989, 45, 7501–7504. [Google Scholar]
  19. Cumming, J.B.; Kebarle, P. Summary of Gas Phase Measurements Involving Acids AH. Entropy Changes in Proton Transfer Reactions Involving Negative Ions. Bond Dissociation Energies (D(A−H) and Electron Affinities EA(A). Can. J. Chem. 1978, 56, 1–9. [Google Scholar]
  20. Bartmess, J.E.; Scott, J.A.; McIver, R.T., Jr. The Gas Phase Acidity Scale from Methanol to Phenol. J. Am. Chem. Soc. 1979, 101, 6047–6056. [Google Scholar]
  21. Hunter, E.P.L.; Lias, S.G. Evaluated Gas Phase Basicities and Proton Affinities of Molecules: An Update. J. Phys. Chem. Ref. Data 1998, 27, 413–656. [Google Scholar]
  22. Linstrom, P.J.; Mallard, W.G. (Eds.) NIST Chemistry WebBook, NIST Standard Reference Database No. 69; National Institute of Standards and Technology: Gaithersburg, MD, USA, 2014. Available online: http://webbook.nist.gov/chemistry (accessed on 11 February 2025).
  23. Maksić, Z.B.; Glasovac, Z.; Despotović, I. Predicted High Proton Affinity of Poly-2,5-dihydropyrrolimines—The Aromatic Domino Effects. J. Phys. Org. Chem. 2002, 15, 499–508. [Google Scholar]
  24. Maksić, Z.B.; Kovačević, B.; Vianello, R. Advances in Determining the Absolute Proton Affinities of Neutral Organic Molecules in the Gas Phase and Their Interpretation: A Theoretical Account. Chem. Rev. 2012, 112, 5240–5270. [Google Scholar] [CrossRef]
  25. Katritzky, A.R.; Lagowski, J.M. Prototropic Tautomerism of Heteroaromatic Compounds: III. Five-Membered Rings and One Hetero Atom. Adv. Heterocycl. Chem. 1963, 2, 1–26. [Google Scholar]
  26. Bodor, N.; Dewar, M.J.S.; Harget, A.J. Ground States of Conjugated Molecules. XIX. Tautomerism of Heteroaromatic Hydroxy and Amino Derivatives and Nucleotide Bases. J. Am. Chem. Soc. 1970, 92, 2929–2936. [Google Scholar] [CrossRef]
  27. De Rosa, M.; Issac, R.P.; Marquez, M.; Orozco, M.; Luque, F.J.; Timken, M.D. 2-Aminopyrrole and Simple 1-Substituted 2-Aminopyrroles: Preparation and Ab Initio Study on the Effect of Solvent on the Amino-Imino Tautomeric Equilibrium. J. Chem. Soc. Perkin Trans 2 1999, 1999, 1433–1437. [Google Scholar] [CrossRef]
  28. Fradera, X.; De Rosa, M.; Orozo, M.; Luque, F. Tautomeric Cojugate Acids of 2-Aminopyrroles: Effect of Substituents, Solvation and Cosolute. Theor. Chem. Acc. 2004, 111, 223–230. [Google Scholar] [CrossRef]
  29. De Rosa, M.; Arnold, D.; O’Hare, B. The First Example of Tautomerism in 2-Aminopyrroles: Effect of Structure and Solvent. Tetrahedron Lett. 2009, 50, 12–14. [Google Scholar] [CrossRef]
  30. De Rosa, M.; Issac, R.P.; Houghton, G. First Synthesis of 2-Aminopyrrole and Simple 1-Substituted-2-Aminopyrroles. Observation of Fast Proton Exchange at C-5. Tetrahedron Lett. 1995, 36, 9261–9264. [Google Scholar] [CrossRef]
  31. De Rosa, M.; Sellitto, I.; Issac, R.P.; Ralph, J.; Timken, M.D. Preparation and Characterization of Tetraphenylborate Salts of 2-Aminopyrrole and 1-Alkyl-2-aminopyrroles. J. Chem. Res. (S) 1999, 1999, 262–263. [Google Scholar]
  32. Demir, A.S.; Emrullahoglu, M. An Effective New Synthesis of 2-Aminopyrrole-4-carboxylates. Tetrahedron 2005, 61, 10482–10489. [Google Scholar] [CrossRef]
  33. Wang, K.; Dömling, A. Design of a Versatile Multicomponent Reaction Leading to 2-Amino-5-ketoaryl Pyrroles. Chem. Biol. Drug Des. 2010, 75, 277–283. [Google Scholar] [CrossRef]
  34. Diana-Rivero, R.; Halsvik, B.; García Tellado, F.; Tejedor, D. Short and Modulator Synthesis of Substituted 2-Aminopyrroles. Org. Lett. 2021, 23, 4078–4082. [Google Scholar] [CrossRef]
  35. Aksu, K.; Őzgeriş, B.; Tűmer, F. Synthesis and Characterization of New N-substituted 2-Aminopyrrole Derivatives. Org. Commun. 2019, 12, 38–42. [Google Scholar] [CrossRef]
  36. Frolova, L.V.; Evdokimov, N.M.; Hayden, K.; Malik, I.; Rogelj, S.; Kornienko, A.; Magedov, I.V. One-Pot Multicomponent Synthesis of Diversely Substituted 2-Aminopyrroles. A Short General Synthesis of Rigidins A, B, C, and D. Org. Lett. 2011, 13, 1118–1121. [Google Scholar] [CrossRef] [PubMed]
  37. Cao, B.; Ding, H.; Yang, R.; Wang, X.; Xiao, Q. Total Synthesis of a Marine Alkaloid, Rigidin E. Mar. Drugs 2012, 10, 1412–1421. [Google Scholar] [CrossRef]
  38. Pauling, L. The Nature of the Chemical Bond, 3rd ed.; Cornell University Press: New York, NY, USA, 1960. [Google Scholar]
  39. Raczyńska, E.D.; Kosińska, W.; Ośmiałowski, B.; Gawinecki, R. Tautomeric Equilibria in Relation to pi-Delocalization. Chem. Rev. 2005, 105, 3561–3612. [Google Scholar] [CrossRef]
  40. Raczyńska, E.D.; Gal, J.-F.; Maria, P.-C. Strong Bases and beyond: The Prominent Contribution of Neutral Push-Pull Organic Molecules towards Superbases in the Gas-Phase. Int. J. Mol. Sci. 2024, 25, 5591. [Google Scholar] [CrossRef]
  41. Parr, R.G.; Yang, W. Density Functional Theory of Atoms and Molecular Orbital Theory; Oxford University Press: New York, NY, USA, 1989. [Google Scholar]
  42. Becke, A.D. Density-Functional Thermochemistry. III. The Role of Exact Exchange. J. Chem. Phys. 1993, 98, 5648–5652. [Google Scholar] [CrossRef]
  43. Lee, C.; Yang, W.; Parr, R.G. Development of the Colle-Salvetti Correlation-Energy Formula into a Functional of the Electron Density. Phys. Rev. B 1988, 37, 785–789. [Google Scholar] [CrossRef]
  44. Hehre, W.J.; Radom, L.; Schleyer, P.v.R.; Pople, J.A. Ab Initio Molecular Theory; Wiley: New York, NY, USA, 1986. [Google Scholar]
  45. Kolomeitsev, A.A.; Koppel, I.A.; Rodima, T.; Barten, J.; Lork, E.; Röschenthaler, G.-V.; Kaljurand, I.; Kütt, A.; Koppel, I.; Mäemets, V.; et al. Guanidinophosphazenes: Design, Synthesis, and Basicity in THF and in the Gas Phase. J. Am. Chem. Soc. 2005, 127, 17656–17666. [Google Scholar] [CrossRef]
  46. Leito, I.; Koppel, I.A.; Koppel, I.; Kaupmees, K.; Tshepelevitsh, S.; Saame, J. Basicity Limits of Neutral Organic Superbases. Angew. Chem. Int. Ed. 2015, 54, 9262–9265. [Google Scholar] [CrossRef]
  47. Mezey, P.G.; Ladik, J.J. A Non-Empirical Molecular Orbital Study on the Relative Stabilities of Adenine and Guanine Tautomers. Theor. Chim. Acta 1979, 52, 129–145. [Google Scholar] [CrossRef]
  48. Mezey, P.G.; Ladik, J.J.; Barry, M. Non-Empirical SCF MO Studies on the Protonation of Biopolymer Constituents II. Protonation of Adenine, Guanine and their Tautomeric Forms. Theor. Chim. Acta 1980, 54, 251–258. [Google Scholar] [CrossRef]
  49. Raczyńska, E.D. On Prototropy and Bond Length Alternation in Neutral and Ionized Pyrimidine Bases and Their Model Azines in Vacuo. Molecules 2023, 28, 7282. [Google Scholar] [CrossRef] [PubMed]
  50. Freindorf, M.; McCutcheon, M.; Beiranvand, N.; Kraka, E. Dihydrogen Bonding—Seen through the Eyes of Vibrational Spectroscopy. Molecules 2023, 28, 263. [Google Scholar] [CrossRef] [PubMed]
  51. Matta, C.F.; Hernández-Trujillo, J.; Tang, T.-H.; Bader, R.F.W. Hydrogen-Hydrogen Bonding: A Stabilizing Interaction in Molecules and Crystals. Chem. Eur. J. 2003, 9, 1940–1951. [Google Scholar] [CrossRef]
  52. Raczyńska, E.D.; Hallmann, M.; Kolczyńska, K.; Stępniewski, T.M. On the Harmonic Oscillator Model of Electron Delocalization (HOMED) Index and Its Application to Heteroatomic π-Electron Systems. Symmetry 2010, 2, 1485–1509. [Google Scholar] [CrossRef]
  53. Raczyńska, E.D. Application of the Extended HOMED (Harmonic Oscillator Model of Aromaticity) Index to Simple and Tautomeric Five-Membered Heteroaromatic Cycles with C, N, O, P, and S Atoms. Symmetry 2019, 11, 146. [Google Scholar] [CrossRef]
  54. Kruszewski, J.; Krygowski, T.M. Definition of Aromaticity Basing on the Harmonic Oscillator Model. Tetrahedron Lett. 1972, 13, 3839–3842. [Google Scholar] [CrossRef]
  55. Krygowski, T.M.; Kruszewski, J. Aromaticity of Thiophene, Pyrrole and Furan in Terms of Aromaticity Indices and Hammett σ Constants. Bull. Acad. Pol. Sci. Chim. 1974, 22, 871–876. [Google Scholar]
  56. Krygowski, T.M. Crystallographic Studies of Inter- and Intramolecular Interactions Reflected in Aromatic Character of π-Electron Systems. J. Chem. Inform. Comput. Sci. 1993, 33, 70–78. [Google Scholar] [CrossRef]
  57. Raczyńska, E.D. On Some Origins of Tautomeric Preferences in Neutral Creatinine in Vacuo: Search for Analogies and Differences in Cyclic Azoles and Azines. Symmetry 2024, 16, 98. [Google Scholar] [CrossRef]
  58. Infantes, L.; Foces-Foces, C.; Cabildo, P.; Claramunt, R.M.; Mó, O.; Yáňez, M.; Elguero, J. The Structure of Aminoazoles and Its Relationship with Aromaticity: Crystal and Molecular Structure of Two Polymorfic Forms of 4-Aminopyrazole. Heterocycles 1998, 49, 157–168. [Google Scholar]
  59. Bartmess, J.E. Thermodynamics of the Electron and the Proton. J. Phys. Chem. 1994, 98, 6420–6424, Erratum in J. Phys. Chem. 1995, 99, 6755. [Google Scholar] [CrossRef]
  60. Fifen, J.J.; Dhaouadi, Z.; Nsangou, M. Revision of the Thermodynamics of the Proton in the Gas Phase. J. Phys. Chem. A 2014, 118, 11090–11097. [Google Scholar] [CrossRef]
  61. Ariai, J.; Ziegler, M.; Würtele, C.; Gellrich, U. An N-Heterocyclic Quinodimethane: A Strong Organic Lewis Base Exhibiting Diradical Reactivity. Angew. Chem. Int. Ed. 2024, 63, e202316720. [Google Scholar] [CrossRef]
  62. Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Montgomery, J.A., Jr.; Vreven, T.; Kudin, K.N.; Burant, J.C.; et al. Gaussian-03, Revision E.01; Gaussian, Inc.: Wallingford, CT, USA, 2004. [Google Scholar]
  63. Raczyńska, E.D.; Gal, J.-F.; Maria, P.-C. Potential Push-Pull Carbon Superbases Based on Methyl Substitution of Rare Tautomers of Imines. Molecules 2025, 30, 474. [Google Scholar] [CrossRef]
  64. Kulsha, A.V.; Ivashkevich, O.A.; Lyakhov, D.A.; Michels, D. Strong Bases Design: Key Techniques and Stability Issues. Int. J. Mol. Sci. 2024, 25, 8716. [Google Scholar] [CrossRef]
Molecules 30 02112 g001
Figure 1. Structures of 2-aminopyrrole (AP, investigated in this work) and marine alkaloids (rigidin A, B, C, and D, containing AP part). Labile (tautomeric) protons are indicated in bold red. The numbering of heavy atoms is noted in the AP formula.
Figure 1. Structures of 2-aminopyrrole (AP, investigated in this work) and marine alkaloids (rigidin A, B, C, and D, containing AP part). Labile (tautomeric) protons are indicated in bold red. The numbering of heavy atoms is noted in the AP formula.
Molecules 30 02112 g001
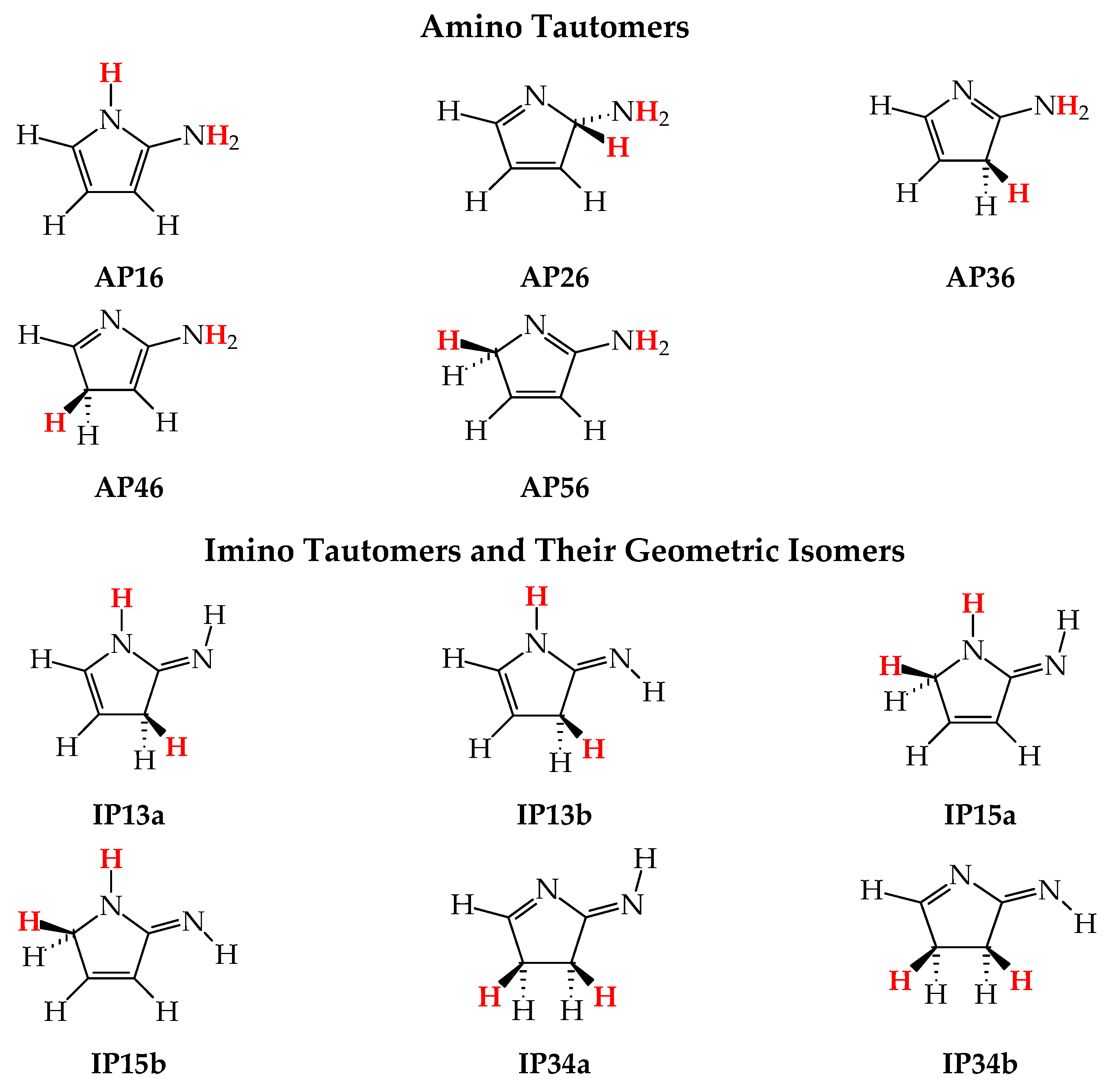
Molecules 30 02112 g002
Figure 2. The eleven possible prototropic isomers for neutral 2-aminopyrrole. Labile (tautomeric) protons are indicated in bold red.
Figure 2. The eleven possible prototropic isomers for neutral 2-aminopyrrole. Labile (tautomeric) protons are indicated in bold red.
Molecules 30 02112 g002
Molecules 30 02112 g003
Figure 3. The eleven prototropic isomers possible for mono-deprotonated 2-aminopyrrole. The labile (tautomeric) proton is indicated in bold red.
Figure 3. The eleven prototropic isomers possible for mono-deprotonated 2-aminopyrrole. The labile (tautomeric) proton is indicated in bold red.
Molecules 30 02112 g003
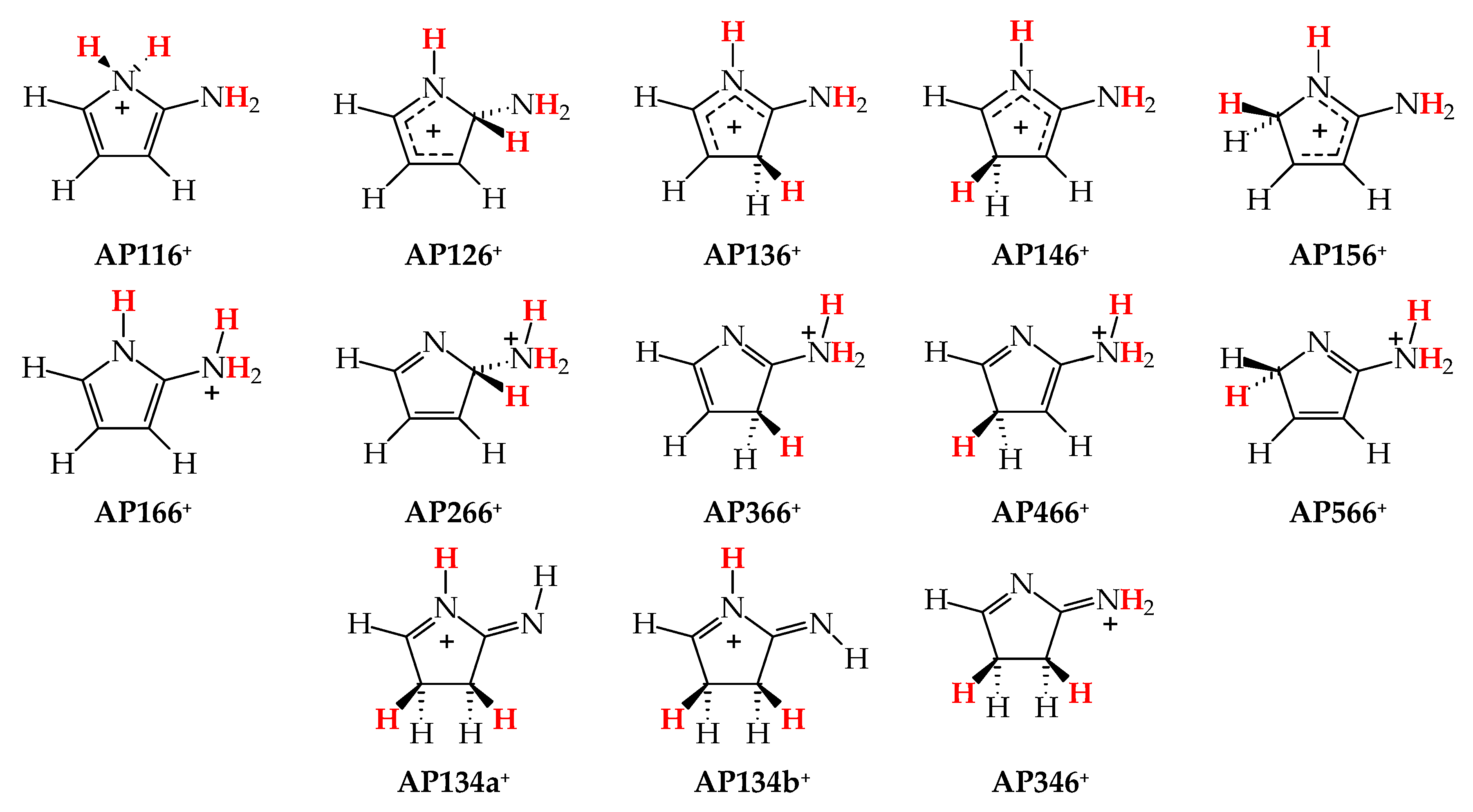
Molecules 30 02112 g004
Figure 4. The thirteen prototropic isomers considered in this work for mono-protonated 2-aminopyrrole. Labile (tautomeric) protons are indicated in bold red.
Figure 4. The thirteen prototropic isomers considered in this work for mono-protonated 2-aminopyrrole. Labile (tautomeric) protons are indicated in bold red.
Molecules 30 02112 g004
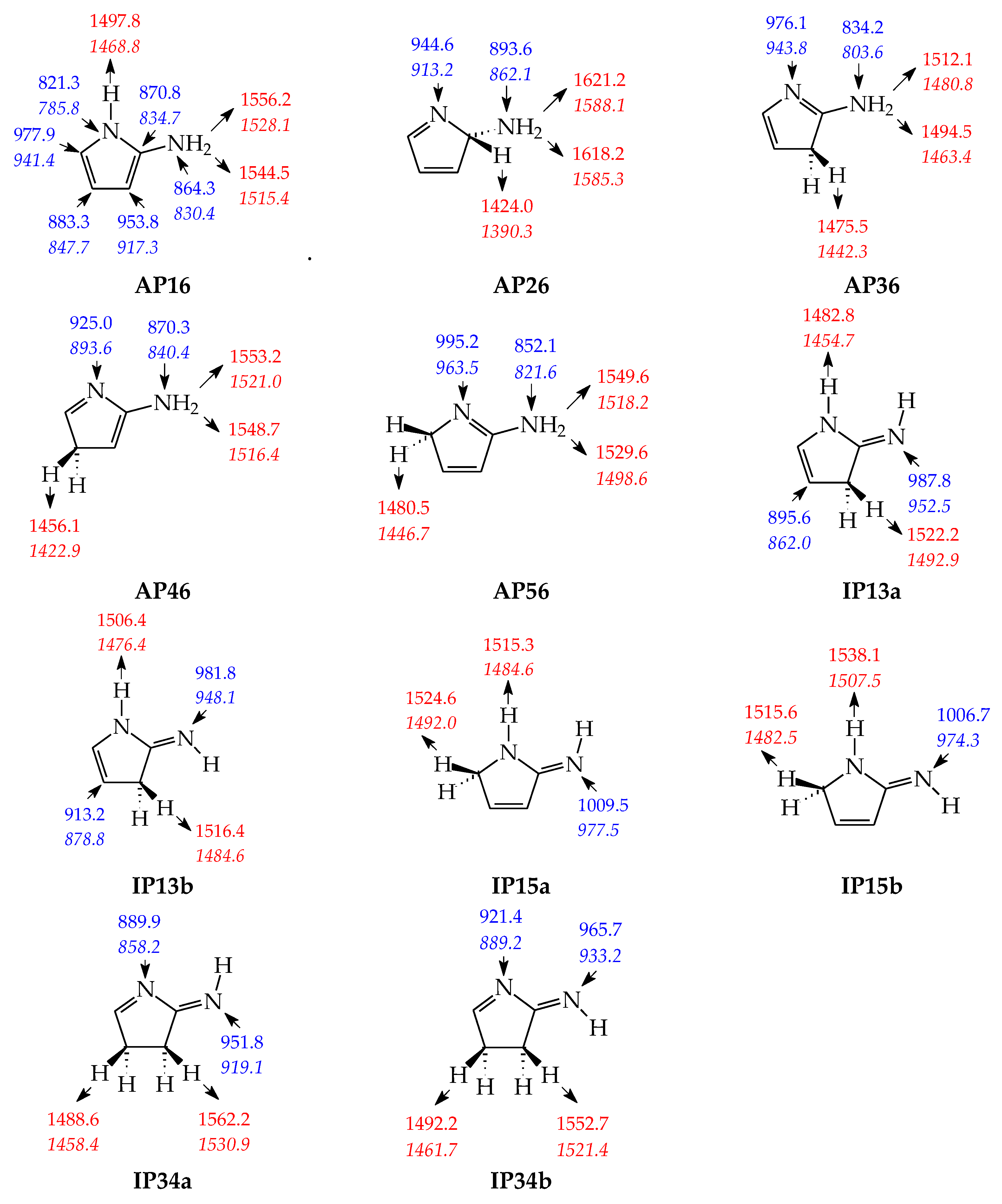
Molecules 30 02112 g005
Figure 5. Microscopic gas-phase proton acidity/basicity parameters calculated at the DFT level for particular acid and base sites in isomers of neutral 2-aminopyrrole. DPE/GA for acid sites and PA/GB for base sites are indicated in red and blue (normal/italic style), respectively.
Figure 5. Microscopic gas-phase proton acidity/basicity parameters calculated at the DFT level for particular acid and base sites in isomers of neutral 2-aminopyrrole. DPE/GA for acid sites and PA/GB for base sites are indicated in red and blue (normal/italic style), respectively.
Molecules 30 02112 g005
Molecules 30 02112 sch001
Scheme 1. Tautomeric and acid/base equilibria possible for 2-aminopyrrole during gas-phase proton acidity/basicity measurements according to the equilibrium method. Participation of reference molecules is not shown. Note that in FT-ICR (as well as in other mass spectrometry techniques), the equilibria involving positive and negative ions are studied separately.
Scheme 1. Tautomeric and acid/base equilibria possible for 2-aminopyrrole during gas-phase proton acidity/basicity measurements according to the equilibrium method. Participation of reference molecules is not shown. Note that in FT-ICR (as well as in other mass spectrometry techniques), the equilibria involving positive and negative ions are studied separately.
Molecules 30 02112 sch001
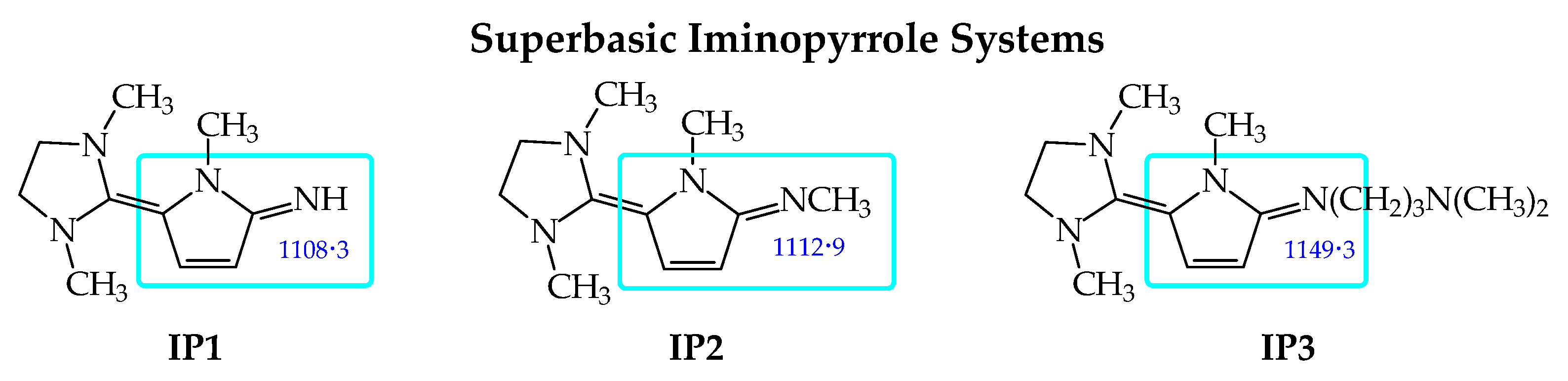
Molecules 30 02112 g006
Figure 6. Push–pull superbases in which the iminopyrrole part of the CH tautomer (IP15) of 2-aminopyrrole is framed in turquoise. Their Pas (in kJ mol−1), calculated for the imino N atom as the protonation site using the Hartree–Fock model and scaled for organic N-bases by Maksić and co-workers [23], are indicated in blue.
Figure 6. Push–pull superbases in which the iminopyrrole part of the CH tautomer (IP15) of 2-aminopyrrole is framed in turquoise. Their Pas (in kJ mol−1), calculated for the imino N atom as the protonation site using the Hartree–Fock model and scaled for organic N-bases by Maksić and co-workers [23], are indicated in blue.
Molecules 30 02112 g006
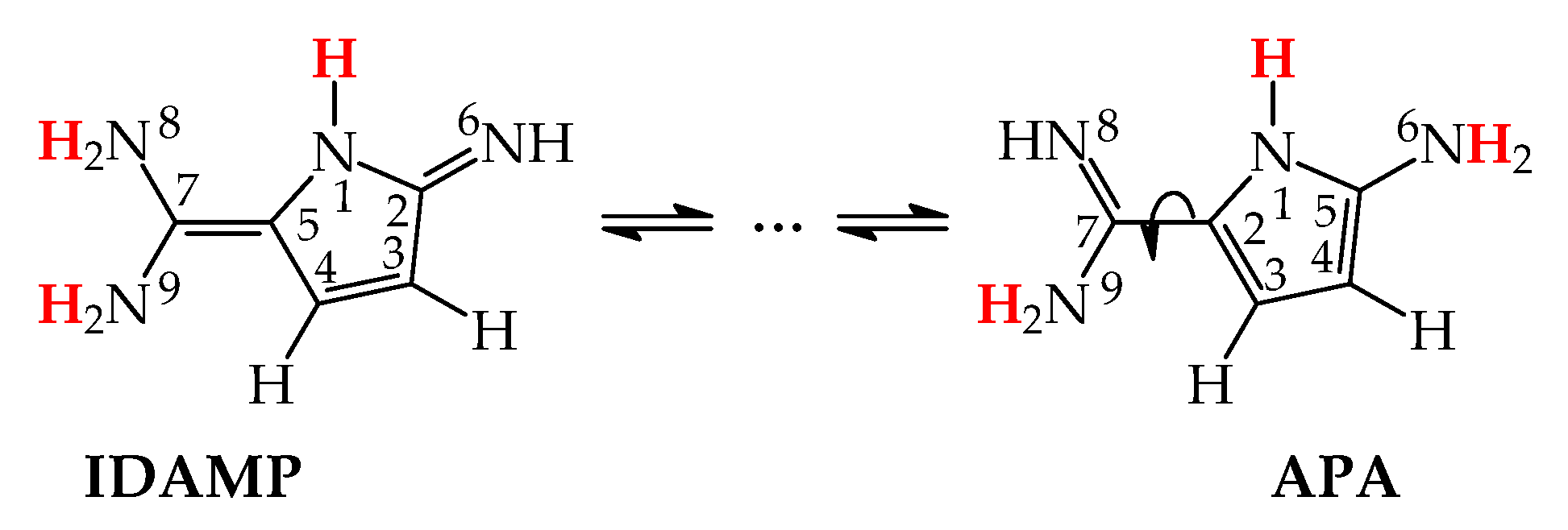
Molecules 30 02112 g007
Figure 7. Structure of 2-imino-5-diaminomethylidene-3-pyrroline (IDAMP, proposed as a strong imino N-base by Maksić in Ref. [23]) and 5-aminopyrrole-2-amidine (APA, expected favored form of IDAMP). Labile (tautomeric) protons are indicated in bold red. The numbering of heavy atoms is displayed in IDAMP and APA formulae.
Figure 7. Structure of 2-imino-5-diaminomethylidene-3-pyrroline (IDAMP, proposed as a strong imino N-base by Maksić in Ref. [23]) and 5-aminopyrrole-2-amidine (APA, expected favored form of IDAMP). Labile (tautomeric) protons are indicated in bold red. The numbering of heavy atoms is displayed in IDAMP and APA formulae.
Molecules 30 02112 g007
Table 1. Relative enthalpies, Gibbs energies, entropy terms (ΔH, ΔG, and TΔS in kJ mol−1 at 298.15 K), and mole fractions (xi in %) calculated at the DFT level for isomers of neutral 2-aminopyrrole (structures given in Figure 2).
Table 1. Relative enthalpies, Gibbs energies, entropy terms (ΔH, ΔG, and TΔS in kJ mol−1 at 298.15 K), and mole fractions (xi in %) calculated at the DFT level for isomers of neutral 2-aminopyrrole (structures given in Figure 2).
Neutral IsomerΔH−TΔSΔGxi
AP160.00.00.099.9838
AP5617.44.722.10.0135
AP3622.44.126.40.0023
IP13b28.12.730.80.0004
IP34a28.33.131.40.0003
IP15b28.84.032.80.0002
IP13a34.01.135.2<0.0001
IP15a31.74.436.0<0.0001
IP34b42.23.345.5<0.0001
AP4641.74.245.9<0.0001
AP2673.84.578.4<0.0001
Table 2. Relative enthalpies, Gibbs energies, entropy terms (ΔH, ΔG, and TΔS in kJ mol−1 at 298.15 K), and mole fractions (xi in %) estimated at the DFT level for mono-deprotonated AP− isomers (structures displayed in Figure 3).
Table 2. Relative enthalpies, Gibbs energies, entropy terms (ΔH, ΔG, and TΔS in kJ mol−1 at 298.15 K), and mole fractions (xi in %) estimated at the DFT level for mono-deprotonated AP− isomers (structures displayed in Figure 3).
Anionic IsomerΔH−TΔSΔGxi
AP6−0.00.00.099.9798
AP3a−19.02.121.10.0202
AP3b−36.61.838.4<0.0001
AP1b−46.60.046.6<0.0001
AP5a−49.12.851.9<0.0001
AP1a−58.40.959.3<0.0001
AP5b−69.12.471.6<0.0001
AP4a−92.60.993.5<0.0001
AP4b−97.11.198.2<0.0001
AP2a−194.30.7195.0<0.0001
AP2b−197.20.6197.8<0.0001
Table 3. Relative enthalpies, Gibbs energies, entropy terms (ΔH, ΔG, and TΔS in kJ mol−1 at 298.15 K), and mole fractions (xi in %) estimated at the DFT level for mono-protonated AP+ isomers (structures presented in Figure 4).
Table 3. Relative enthalpies, Gibbs energies, entropy terms (ΔH, ΔG, and TΔS in kJ mol−1 at 298.15 K), and mole fractions (xi in %) estimated at the DFT level for mono-protonated AP+ isomers (structures presented in Figure 4).
Cationic IsomerΔH−TΔSΔGxi
AP156+0.00.00.099.9940
AP136+24.10.024.10.0060
AP346+54.3−0.653.8<0.0001
AP146+94.6−0.893.7<0.0001
AP134b+98.7−0.997.8<0.0001
AP126+107.1−0.4106.7<0.0001
AP166+113.5−2.5111.0<0.0001
AP134a+116.3−1.6114.6<0.0001
AP566+143.1−1.1142.0<0.0001
AP466+149.3−2.3147.0<0.0001
AP116+156.6−1.0155.6<0.0001
AP266+158.2−0.4157.7<0.0001
AP366+165.9−1.6164.3<0.0001
Table 4. HOMED indices calculated for DFT structures of all possible prototropic isomers of neutral and ionic 2-aminopyrrole. HOMED5 and HOMED6 refer to the five-membered ring and to the entire molecule, respectively.
Table 4. HOMED indices calculated for DFT structures of all possible prototropic isomers of neutral and ionic 2-aminopyrrole. HOMED5 and HOMED6 refer to the five-membered ring and to the entire molecule, respectively.
IsomerHOMED5HOMED6IsomerHOMED5HOMED6
AP160.9240.825AP4b−0.2750.321
AP260.2310.077AP5a−0.3290.371
AP360.3880.411AP5b−0.3050.349
AP460.4340.437AP6−0.9580.777
AP560.3810.393AP116+−0.0500.005
IP13a0.3140.304AP126+0.0870.074
IP13b0.3060.298AP136+0.3820.419
IP15a0.3340.333AP146+0.4870.502
IP15b0.3600.358AP156+0.4680.606
IP34a−0.029−0.036AP166+0.9220.669
IP34b−0.056−0.057AP266+0.363−0.130
AP1a−0.7550.772AP366+0.3850.082
AP1b−0.8160.828AP466+0.4920.072
AP2a−−0.182−0.164AP566+0.4030.072
AP2b−−0.271−0.283AP134a+−0.127−0.179
AP3a−0.3620.390AP134b+−0.063−0.108
AP3b−0.3050.340AP346+0.2800.307
AP4a−0.2200.269
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

Share and Cite

MDPI and ACS Style

Raczyńska, E.D.; Maria, P.-C.; Gal, J.-F. Quantum Chemical Studies on the Prototropic and Acid/Base Equilibria for 2-Aminopyrrole in Vacuo—Role of CH Tautomers in the Design of Strong Brønsted Imino N-Bases. Molecules 2025, 30, 2112. https://doi.org/10.3390/molecules30102112

AMA Style

Raczyńska ED, Maria P-C, Gal J-F. Quantum Chemical Studies on the Prototropic and Acid/Base Equilibria for 2-Aminopyrrole in Vacuo—Role of CH Tautomers in the Design of Strong Brønsted Imino N-Bases. Molecules. 2025; 30(10):2112. https://doi.org/10.3390/molecules30102112

Chicago/Turabian Style

Raczyńska, Ewa Daniela, Pierre-Charles Maria, and Jean-François Gal. 2025. "Quantum Chemical Studies on the Prototropic and Acid/Base Equilibria for 2-Aminopyrrole in Vacuo—Role of CH Tautomers in the Design of Strong Brønsted Imino N-Bases" Molecules 30, no. 10: 2112. https://doi.org/10.3390/molecules30102112

APA Style

Raczyńska, E. D., Maria, P.-C., & Gal, J.-F. (2025). Quantum Chemical Studies on the Prototropic and Acid/Base Equilibria for 2-Aminopyrrole in Vacuo—Role of CH Tautomers in the Design of Strong Brønsted Imino N-Bases. Molecules, 30(10), 2112. https://doi.org/10.3390/molecules30102112

Article Metrics

Back to TopTop